1 Introduction

Gear system is widely applied in various machineries as power transmission equipment, whose dynamic performance directly affects the stability of system. However, the nonlinear factors consisting of the static transmission error, meshing stiffness, tooth face friction, gear backlash and so forth, are prone to deteriorating vibration response of gear pair, which may cause the system to become unpredictable and uncontrollable. Thus, it is of importance to determine accurately the dynamic characteristics of gear set for avoiding serious vibration and ensuring the running stability of machinery [1, 2].

To improve the dynamic performance of gear transmission system, a sea of studies have been carried out in recent decades. Kahraman and Singh [3,4,5] simplified the gear system to the mathematical models with single degree of freedom (single DOF) or multi-DOF and then analyzed the dynamic behaviors of system with the effect of clearance and meshing stiffness. Raghothama and Narayanan [6] investigated the periodic and chaotic motions in gear–rotor–bearing system by adopting the incremental harmonic balance method (IHBM) and numerical simulation method. Theodossiades and Natsiavas [7] used the analytical methodology to research the dynamics of a gear pair system with backlash and time-dependent mesh stiffness and then verified the obtained results by numerical calculation. The influences of meshing damping, amplitude of meshing stiffness and static torque on dynamic characteristic of two gear pairs were described by Al-Shyyab and Kahraman [8, 9]. Then, Shen et al. [10] derived a system model of a spur gear pair with single-DOF, in which the influences of damping ratio and the excitation amplitude on system response were analyzed by using IHBM. Likewise, to determine the effect of backlash on dynamic characteristics in two-stage gear system, Walha et al. [11] developed a torsional dynamic model and used Newton–Raphson algorithm to obtain the dynamic behaviors of system. Considering the nonlinear suspension, Chang-Jian [12] studied the dynamic response of a gear–bearing system, which demonstrated the motion forms of the system by using nonlinear dynamic analysis method. In Refs. [13, 14], Melnikov analytical method was applied by Farshidianfar and Saghafi to predict the key values of system parameter for the occurrence of homoclinic bifurcation and onset of chaos. With the aim to obtain the torsional dynamic behaviors of wind turbine gearbox, Zhao and Ji [15] built a nonlinear dynamic model under the internal and external excitations and thus the influences of excitation parameters on the dynamic responses of system were investigated through a numerical integration method. Wang et al. [16] proposed a three-DOF torsional dynamic model of the locomotive traction system to carry out the dynamic analysis. The dynamic behaviors of system were identified and described by time-domain response diagram, phase plane diagram, Poincaré map and bifurcation diagram. In addition, Gou et al. [17] modified a dynamic model of a gear system to analyze the influence of flash temperature on the dynamic responses of system, in which the temperature stiffness was constructed and considered into the mathematical model. The analysis result confirmed that the developed method of the flash temperature is useful. For the purpose of investigating the relationship between backlash and bearing clearance, Liu et al. [18] considered several kinds of forces such as the backside contact force, bearing forces and shift of the bearing position in the model, where the detailed research was carried out in quasi-static and dynamic analyses.

Considering the effect of friction on the dynamic features of the gear system, Howard et al. [19] established a dynamic model with 16-DOF and simulated the dynamic behaviors under different working conditions. Vaishya and Singh [20] presented a dynamic model of a gear pair with meshing stiffness, damping and tooth face friction. Then, they [21] analyzed further the effect of the strong nonlinearity caused by sliding friction on the system and discussed the dynamic behaviors to the change of key parameters. Several sliding friction formulations applied in the spur gear system are examined and compared in the influence on the dynamic response in Ref [22]. Subsequently, Wang et al. [23] investigated and compared the nonlinear phenomena in a gear pair with the friction and non-friction and obtained the crucial differences in the system behavior. Considering meshing stiffness, backlash and friction, Chen et al. [24] provided a dynamic model for a gear pair system with multi-DOF and predicted the motion states. Based on the model above, Moradi [25] studied the nonlinear oscillations with the backlash nonlinearity, and confirmed the dynamic responses of the system consisting of primary, super-harmonic and sub-harmonic resonances by using the multiple scale method. Xiang et al. [26] built a six-DOF mathematical model of a spur gear pair with surface friction and used the support stiffness and rotational speed as control parameters to analyze the nonlinear dynamic features. Based on a quasi-static wear model and a translational–rotational-coupled nonlinear dynamic model, Liu et al. [27] developed a wear prediction method to describe the relationship between surface wear and dynamic responses of system. The numerical results revealed that the interaction relation between the above two is strong. A six-DOF model of gear pair under friction–vibration interactions was built by Jiang and Liu [28], in which the dynamic responses of system with sliding friction were obtained. Wang et al. [29] presented a dynamic model for locomotive gear transmission system with tooth surface and performed the investigation for the influence of tooth surface friction on the parametric vibration stability. Zhou et al. [30] proposed a gear–rotor–bearing model with 16-DOF, which took into consideration friction force, gravity, eccentricity and so on, and studied the influences of mean load and friction coefficient on the dynamic behaviors using numerical integration method. The corresponding results indicated that the friction force could increase the vibration amplitude of system.

From above, a multitude of works have investigated the nonlinear dynamics with and without frictional force, respectively. Nevertheless, the dynamic model with the frictional force in some references should be improved. It is due to the deficiency that only the frictional force under single-tooth meshing area is considered. Additionally, the meshing force is assumed to be uniform distribution between the tooth pairs when the frictional force under double-tooth meshing area is considered in the gear system. Overcoming the two shortcomings above, the influence of frictional force on the dynamic response of gear system can be more factually reflected based on the proposed dynamic model. Rotational speed, damping ratio and meshing backlash as control parameters are used to research the dynamic characteristics of the gear system in detail. Meantime, the nonlinear dynamic behaviors of the system are discussed utilizing time-domain waveform diagram, frequency plot, phase portrait, Poincaré map and dynamic load diagram.

The structure of the study is organized into four sections. The dynamic model and equation of motion for a spur gear pair system are presented in Sect. 2, where the nonlinearity of friction factor and time-varying meshing stiffness are described as well. In Sect. 3, the equation of motion for the system is solved by numerical method and then bifurcation and chaos features with different bifurcation parameters are investigated. Finally, some brief conclusions are drawn from the research in Sect. 4.

2 Description of dynamic model for a spur gear pair system

2.1 Time-varying meshing stiffness

In order to ensure the steady gear transmission, the meshing coincidence degree of gears ε is not generally an integer, which is under the range of 1–2. Therefore, the alternation meshing between single tooth and double teeth occurs, leading to the change of meshing stiffness periodically. When calculating the meshing stiffness, only the abrupt changes caused by the single-tooth meshing and double-tooth meshing alternately are taken into account in many researches. However, the change of the stiffness of the tooth itself with the movement of the meshing point is neglected. The way to deal with meshing stiffness may result in large error and cannot reflect the actual situation of gear meshing. Based on the previous research [26], the extending period method is thus introduced to calculate the time-varying meshing stiffness so as to analyze conveniently and accurately the meshing situation and the dynamic meshing force between the meshing teeth.

Figure 1 presents the meshing schematic diagram of the spur gear pair. In Fig. 1a, rai and rbi are the radius of addendum circle and base circle of the gear system, respectively. ωi denotes the rotational speed of the gear i (i = 1, 2), respectively. N2 and N1 are the starting point and ending point of theoretical meshing line, respectively. It is assumed that the gear pair starts meshing in point B2 and moves along the meshing line N1N2 to the point B1 out of contact. From Fig. 1b, it can be seen in the moving distance N1N2 of the meshing points that B2K2 and K1B1 are double-tooth meshing areas and K1K2 is the single-tooth meshing area, respectively.

Fig. 1
figure 1

Schematic illustration of meshing between the gear pairs. a Sketch of meshing at the end surface of gears. b Physical interpretation of meshing coincidence degree

Full size image

The situation of gear meshing is expanded in time axis, as shown in Fig. 2. Here, Tm = 2π/z1ω1 = 2π/z2ω2 is the meshing period. T1 is equal to Tm, where T1 represents the time that the meshing point moves a basic pitch Pn along the meshing line. According to the definition of mesh coincidence degree, ε = B1B2/Pn. Hence, T2 = ε · Tm, in which T2 is the time that the meshing point moves from point B2 to point B1. In addition, t1 refers to the time that the meshing point is under the double-tooth meshing area. Likewise, t2 shows the time that the meshing point is in the single-tooth meshing area. In time domain, the meshing gear pair can be divided into odd meshing gear pair and even meshing gear pair. It can be found from the Fig. 2 that the time interval between the two odd meshing gear pairs or two even meshing gear pairs is 2T1. For instance, the meshing point moves from point B2 to point C, and in the time process, tooth pair 1 is out of meshing and tooth pair 3 starts to be in meshing. However, it clearly shows that the tooth pair 1 does not involve in the meshing when it moves from B1 to C, which means that the meshing stiffness is zero. Therefore, the time-varying meshing stiffness of odd meshing gear with the period 2T1 can be expressed as follows:

Fig. 2
figure 2

Sketch of mesh stiffness extending on meshing time

Full size image
$$k_{1} (t) = \left\{ {\begin{array}{*{20}l} {k(t)} \hfill & {\;\;\;{\kern 1pt} 0 < t < T_{2} } \hfill \\ 0 \hfill & {\;\;\;{\kern 1pt} T_{2} < t < 2T_{1} } \hfill \\ \end{array} } \right.$$
(1)

Similarly, the period of even meshing gear pair is also 2T1, in which the meshing stiffness of even meshing gear pair can be written as \(k_{2} (t) = k_{1} (t + 2T_{ 1} )\). Therefore, when the gear backlash is 2bc, the elastic restoring force Fki, i.e., the dynamic load of the gear transmission [31], is defined as follows:

$$F_{ki} = k_{i} (t) \cdot f(x)\quad (i = 1,2)$$
(2)

where f(x) refers to the nonlinear backlash function which could adopt the following expression:

$$f(x) = \left\{ {\begin{array}{*{20}l} {x - b_{c} ,} \hfill & {\quad x > b_{c} } \hfill \\ {0,} \hfill & {\quad \left| x \right| \le b_{c} } \hfill \\ {x + b_{c} ,} \hfill & {\quad x < - \,b_{c} } \hfill \\ \end{array} } \right.$$
(3)

Here, bc is half of the tooth backlash and x is the total relative elastic deformation.

According to the nonlinear backlash function f(x) in Fig. 3, there are three impact states consisting of no impact (state I), single-sided impact (state II) and double-sided impact (state III) in a gear system [2, 3]. From Fig. 3, when x is in the range of xmin > bc or xmax < − bc, there is no tooth separation where the gear system shows no impact in state I. It means that the dynamic load Fki is always greater than zero. Then, when x is at xmax > bc and xmin > − bc or xmax < bc and xmin < − bc, the gear system has single-sided impact in state II, in which the dynamic load Fki would have zero value but wll not have negative values. Additionally, the double-sided impact (state III) will occur in the gear system when x is in the range of xmin < − bc and xmax > bc. The corresponding Fki will have negative values.

Fig. 3
figure 3

Backlash nonlinear function

Full size image

The damping force can be defined as

$$F_{ci} = c_{mi} \cdot \dot{x}$$
(4)

where cmi denotes the meshing damping for the gear pair i (i = 1, 2).

Hence, the dynamics meshing force can be written as:

$$F_{i} = F_{ki} + F_{ci} \quad \left( {i = 1,2} \right)$$
(5)

2.2 Nonlinearity of friction factor

Based on the actual situation of gear meshing, the relative sliding velocity direction of the tooth surface for the meshing gear pair is opposite around the pitch circle. Meantime, the meshing force of the tooth surface changes with the movement of meshing point. Hence, the direction of the frictional force at the pitch could be changed abruptly by the above reasons. The point that the tooth pair 1 in Fig. 2 starts to enter meshing is selected as a starting point. Thus, the direction coefficient of sliding friction force of tooth surface can be expressed with period 2T1 as follows:

$$\lambda_{i} = \left\{ {\begin{array}{*{20}l} 1 \hfill & {\;\;\;{\kern 1pt} 0 < t < t_{2} } \hfill & {} \hfill \\ { - {\kern 1pt} 1} \hfill & {\;\;\;{\kern 1pt} t_{2} < t < T_{2} } \hfill & {\;\;\;{\kern 1pt} (i = 1,2)} \hfill \\ 0 \hfill & {\;\;\;{\kern 1pt} T_{2} < t < 2T_{1} } \hfill & {} \hfill \\ \end{array} } \right.$$
(6)

As a function of the Coulomb’s law of friction, the magnitude of the frictional force is proportional to the positive pressure. The friction force can be expressed by

$$F_{fi} = \lambda_{i} \mu F_{i} \quad (i = 1,2)$$
(7)

where μ represents the coefficient of friction and Fi is the dynamics meshing load.

In terms of gear geometry in Fig. 1a, the frictional force arms are expressed as follows [32]:

$$\begin{aligned} l_{11} (t) & = (r_{{\rm b}1} + r_{{\rm b}2} )\tan \alpha - \sqrt {r_{{{\text{a}}2}}^{2} - r_{{{\text{b}}2}}^{2} } + r_{{{\text{b}}1}} \omega_{1} t \\ l_{12} (t) & = (r_{{\rm b}1} + r_{{\rm b}2} )\tan \alpha - \sqrt {r_{{{\text{a}}2}}^{2} - r_{{{\text{b}}2}}^{2} } + r_{{{\text{b}}1}} \omega_{1} t + P_{n} = l_{11} (t + T_{1} ) \\ l_{21} (t) & = \sqrt {r_{{{\text{a}}2}}^{2} - r_{{{\text{b}}2}}^{2} } - r_{{{\text{b}}1}} \omega_{1} t \\ l_{22} (t) & = \sqrt {r_{{{\text{a}}2}}^{2} - r_{{{\text{b}}2}}^{2} } - r_{{{\text{b}}1}} \omega_{1} t + P_{n} = l_{21} (t + T_{1} ) \, \\ \end{aligned}$$
(8)

where l1i and l2i are the frictional force arms of the tooth pair i (i = 1, 2), respectively.

Then, the friction torque of gear i caused by friction force between ith tooth pair can be given by:

$$T_{f1i} = F_{fi} \cdot l_{1i} \quad T_{f2i} = F_{fi} \cdot l_{2i} \quad (i = 1,2)$$
(9)

From the previous analysis, the meshing force, friction force and friction torque between the gear pair are written as:

$$F = \sum\limits_{i = 1}^{N} {F_{i} } \quad F_{f} = \sum\limits_{i = 1}^{N} {F_{fi} } \quad T_{f1} = \sum\limits_{i = 1}^{N} {T_{f1i} } \quad T_{f2} = \sum\limits_{i = 1}^{N} {T_{f2i} } \quad (i = 1,2)$$
(10)

where N is the maximum number of pairs of meshing gears and can be obtained as

$$N = {\text{ceil}}(\varepsilon )$$
(11)

Here, ceil(ε) is the smallest integer that is greater than or equal to ε.

2.3 Equations of the gear pair system

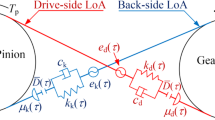
The generalized lumped dynamic model with torsional-DOF for a spur gear pair system is provided, ignoring the transverse and axial elastic deformation of the drive shaft and the elastic deformation of the support system, as shown in Fig. 4. For the sake of convenient calculation in the model, it is assumed that the driving and driven gears are standard involute gears. The meshing stiffness and damping parameter are same on the unit tooth width. And the sliding friction factors are equal and constant in the system, neglecting the rolling friction. Here, θ1 and θ2 represent the torsional vibration displacement of the driving and driven gears, respectively. I1 and I2 show the moment of inertia for the two gears, respectively. m1 and m2 mean the equivalent mass of the two gears, respectively. rb1 and rb2 represent the radius of base circles of the gear system, respectively. Tin and Tou are the torques acting on the driving and driven gears, respectively. Ff refers to the sliding friction between the gears. In addition, k(t), cm, e(t) and 2bc are time-varying meshing stiffness, meshing damping, the static transmission error and gear backlash in the gear pair system, respectively.

Fig. 4
figure 4

Dynamic model of a spur gear pair system with sliding friction

Full size image

According to Newton’s laws of motion, the dynamic differential equations of the gear pair system in Fig. 4 can be expressed as follows:

$$\begin{aligned} & I_{1} \ddot{\theta }_{1} + r_{{{\text{b}}1}} F + T_{f1} = T_{\text{in}} \\ & I_{2} \ddot{\theta }_{2} - r_{{{\text{b}}2}} F - T_{f2} = - \,T_{\text{ou}} \\ \end{aligned}$$
(12)

Due to the presence of gear backlash, the gear pair contains a rigid body displacement [33]. To eliminate the rigid body displacement that makes the equation solvable, the relative coordinate x is thus described in the gear system, which can be represented by

$$x = r_{{{\text{b}}1}} \dot{\theta }_{1} - r_{{{\text{b}}2}} \dot{\theta }_{2} - e(t)$$
(13)

where e(t) = em + er · sin(ωt + φ) refers to the static transmission error, in which em is the mean value, er represents the volatility value, and φ stands for the initial phase of error.

Substituting the relative coordinate x as a new degree of freedom into Eq. (12), Eq. (12) can then be rewritten as the following expressions:

$$\ddot{x} + \frac{1}{{m_{\text{e}} }}\left[ {\eta_{1} (t) + \eta_{2} (t)} \right]c_{{m}} \dot{x} + \frac{1}{{m_{\text{e}} }}\left[ {\eta_{1} (t)k_{1} (t) + \eta_{2} (t)k_{2} (t)} \right]f(x) = \frac{F}{{m_{\text{e}} }} - \ddot{e}(t)$$
(14)

where me = 1/(1/me1 + 1/me2) shows the equivalent mass of the gear pair. Here, me1 and me2 are the equivalent mass of the driving and driven gears, respectively, which can be obtained by me1 = I1/r 2b1 and me2 = I2/r 2b2 . And, F = T1/rb1 = T2/rb2 is the average force caused by external torque. In addition, η1(t) and η2(t) are the period function about frictional force arms, which are written as follows:

$$\begin{aligned} & \eta_{1} (t) = 1 + m_{\text{e}} \mu \lambda_{1} \left( {\frac{{L_{11} }}{{m_{1} r_{{{\text{b}}1}} }} + \frac{{L_{12} }}{{m_{2} r_{{{\text{b}}2}} }}} \right) \\ & \eta_{2} (t) = 1 + m_{\text{e}} \mu \lambda_{2} \left( {\frac{{L_{21} }}{{m_{1} r_{{{\text{b}}1}} }} + \frac{{L_{22} }}{{m_{2} r_{{{\text{b}}2}} }}} \right) \\ \end{aligned}$$
(15)

In order to improve the accuracy of calculation, Eq. (14) should be transformed with dimensionless form.

Then, the dimensionless parameters τ = ωnt and nominal dimension bn are introduced, where the system response parameters could be expressed with \(x = Xb_{n}\), \(\dot{x} = \dot{X}b_{n} \omega_{n}\), \(\ddot{x} = \ddot{X}b_{n} \omega_{n}^{2}\) and Ω = ω/ωn. Additionally, the dimensionless nonlinear backlash function f(X) can be described as follows:

$$f(X) = \left\{ {\begin{array}{*{20}l} {X - b,} \hfill & {\;\;\;{\kern 1pt} X > b} \hfill \\ {0,} \hfill & {\;\;\;{\kern 1pt} \left| X \right| \le b} \hfill \\ {X + b,} \hfill & {\;\;\;{\kern 1pt} X < - \,b} \hfill \\ \end{array} } \right.$$
(16)

where b = bc/bn.

Hence, the dimensionless equation of system can be represented by:

$$\ddot{X} + 2\zeta \left[ {\eta_{1} (\tau ) + \eta_{2} (\tau )} \right]\dot{X} + \frac{1}{{k_{m} }}\left[ {\eta_{1} (\tau )k_{1} (\tau ) + \eta_{2} (\tau )k_{2} (\tau )} \right]f(X) = F_{m} + F_{a} \varOmega^{2} \cos (\varOmega \tau + \varphi )$$
(17)

where \(\zeta = \frac{{c_{m} }}{{2m_{\text{e}} \omega_{n} }}\), \(F_{m} = \frac{F}{{b_{n} m_{\text{e}} \omega_{n}^{2} }}\), \(F_{a} = \frac{{e_{r} }}{{b_{n} }}\).

In addition, the dimensionless dynamic load can be given by

$$F_{k} = \frac{1}{{k_{m} }}\left[ {\eta_{1} (\tau )k_{1} (\tau ) + \eta_{2} (\tau )k_{2} (\tau )} \right]f(X)$$
(18)

3 Numerical simulation and discussion

Owing to the existence of the tooth surface friction, time-varying stiffness, backlash and the static transmission error, the gear system is a complex system with nonlinearity and time variation. To understand comprehensively the dynamic features of system, rotational speed Ω, damping ratio ζ and backlash b are selected as bifurcation parameter to investigate the influences on the system response, in which the corresponding dynamic equations are solved using Runge–Kutta numerical method with the initial conditions X(0) = 0 and \(\dot{X}(0) = 0\). The dynamic responses of the gear system with sliding friction can be systematically analyzed from bifurcation diagrams and 3-D frequency spectrum. The main physical parameters of system are listed in Table 1. Besides, when the external load varies, the force ratio Fm/Fa that reflects the carrying capacity of gear transmission system also changes. Apparently, in order to study the effect of Fm and Fa on dynamic behaviors, it is essential to analyze the bifurcation and chaos characteristics under lightly and heavily loaded conditions, respectively [3].

Table 1 Parameters of the gear pair system
Full size table

3.1 Model validation

Tooth surface friction force has an evident effect on the dynamic behaviors of the gear system. Thus, a number of literatures have considered the sliding friction factor in the dynamic models, where some models with friction should be perfected owing to the fact that the obtained friction force cannot factually reflect the effect on the vibration of the gear pair system. Therefore, time-domain waveform of the relative coordinate along the line of meshing is illustrated in Fig. 5 to verify the accuracy of the dynamic model for a spur gear pair system with sliding friction in this paper. The contrast between the proposed model and the model in the literatures is first carried out without friction to prove the correctness of the model of this study and, second, performed under friction condition to analyze the difference between models.

Fig. 5
figure 5

Time-domain waveform. a Without friction; b with friction coefficient μ = 0.07

Full size image

When the coefficient of friction μ is zero and the other parameters of the model in Fig. 4 are the same as those in Ref. [5], Fig. 5a explicitly shows that the displacement X is equal to the displacement P in Ref. [5], which means that the built model without friction is equivalent to the model in Ref. [5]. Then, considering the friction factor that the friction coefficient μ is 0.07, the displacement X is larger than the displacement P in Fig. 5b. It clearly indicates that tooth face friction could aggravate the vibration of gear pair, which is in agreement with the research conclusion in Ref. [30]. Besides, the friction force of tooth surface is briefly expressed as the product of the contact force between the gear teeth and friction coefficient in Ref. [29]. Thus, keeping the system parameters same with the above analysis, the displacement Y can be solved as seen in Fig. 5b, in which the method of calculating the friction force in Ref. [29] is adopted. It could be observed that the displacement Y is less than displacement P, which seems to reveal that the friction could suppress the system response. The result is caused by the unreasonable assumptions that the dynamic change of friction force is omitted. Overall, based on the models in the literature, the proposed model with friction force is reasonably improved that can factually reflect the influence of tooth surface friction on gear vibration.

3.2 Effect of rotational speed Ω on bifurcation and chaos characteristics

Rotational speed Ω is one of the main physical parameters for the gear transmission system. Thus, Ω is chosen as a control parameter to investigate the influence on the bifurcation and chaos feature of system. Meanwhile, the other system parameters are listed as follows: friction coefficient μ = 0.07, damping ratio ζ = 0.07, gear backlash b = 1, force ratio Fm/Fa = 0.5 or 2; namely, the gear system is under lightly or heavily loaded condition. Figures 6 and 7 show the bifurcation diagrams and three-dimensional (3-D) spectrum programs with the varying rotational speed Ω under different loaded conditions. From these figures, when Ω increases, the system presents complex motion forms, including period motion, i.e., period-one and multi-period motions, chaotic motion and so on. Specifically, comparing Fig. 6a with Fig. 6b, it can be clearly found that the system undergoes the wider region of chaotic motion under the condition of light load than that under the condition of heavy load, where the chaos is nearly replaced by period motion under the condition of heavy load. The phenomenon can be inspected from the corresponding 3-D spectrum programs in Fig. 7. Chaotic motion always means that the system is under the unstable and uncontrollable state. It could be concluded that the stable motion occupies the dominant position under the heavily loaded condition, in which there is similar conclusion in Ref. [5].

Fig. 6
figure 6

Bifurcation diagrams with the varying of Ω under lightly and heavily loaded conditions, respectively

Full size image
Fig. 7
figure 7

3-D frequency spectrum with the varying of Ω under lightly and heavily loaded conditions, respectively

Full size image

In order to clearly reveal the motion states under lightly loaded condition, time-domain waveform, frequency plot, phase diagram, Poincaré map and dynamic load curve are used to demonstrate the dynamic features of system from Figs. 8, 9, 10, 11, 12, 13, 14, 15, 16 and 17. In the range of Ω < 0.78, the gear pair keeps in the period-one state. Under Ω = 0.3, the time-domain waveform is a single periodic sinusoid, frequency plot possesses an obvious amplitude peak, the phase diagram is an approximate ellipse, and there is only a point in the Poincaré map, as shown in Fig. 8a–c. These reveal that the system is in period-one state. The dynamic load in Fig. 8d is always greater than zero, which demonstrates the meshing gear pairs are in no impact state (state I). In other words, there cannot appear separation between the meshing teeth, which also represents the system is under the stable state. Although jump discontinuity phenomena appears when Ω = 0.61, the motion form of the gear system does not change in Fig. 6a. As Ω increases, the system response changes from period-one to period-two motion. Meantime, the meshing state of gear pair transforms from no impact to single-sided impact state. Choosing Ω = 0.83, frequency plot in Fig. 9 comes out demultiplication frequency component, namely 0.5Ω and 1.5Ω. And the meshing gear pair is in state between no impact state and single-sided impact state alternately in Fig. 8d due to the discontinuous presence of dynamic load Fk = 0. Besides, single-sided impact state represents a repeated state that the meshing gear contact, separate, re-contact only on the positive tooth surface, which reveals the instability of the system. By increasing the rotational speed Ω further, the gear pair keeps under the single-sided impact state and the dynamic load Fk increases gradually at 0.83 < Ω < 1.04. Furthermore, the system response switches between period-two and period-four motions twice, which can be observed in Figs. 9, 10, 11 and 12. Then, Ω keeps increasing even further, the system starts into chaotic motion. For instance, at Ω = 1.05, there appear continuous frequency components in frequency spectrum diagram, the phase diagram is disorder, and a great deal of discrete points evolved into two fractal structures exist in the Poincaré map in Fig. 13, which shows the characteristics of chaos. Before entering the next chaotic window, gear pair experiences a narrow region of period-five motion. As shown in Fig. 14, under Ω = 1.2, the time-domain waveform is a periodic motion and the Poincaré map has five discrete points, revealing that the system is under period-five motion state. Then, the system goes into the chaos once more. Likewise, when Ω = 1.5, Fig. 15 displays the chaotic behavior of system. Even though system is in chaotic motion under the above two values, the fractal part from Fig. 15c is different with that in Fig. 13c. Increasing the rotational speed Ω sequentially, the gear system turns into period-two state from the chaos motion, and finally converges to the period-one motion, as seen in Figs. 16 and 17. Overall, it could be clearly observed from Figs. 8, 9, 10, 11, 12, 13, 14, 15, 16 and 17 that the dynamic load Fk of the system under chaotic motion is generally larger than that under period motion, which could deteriorate the dynamic responses of system. In addition, when rotational speed Ω changes, the meshing state of gear pairs only has no impact and single-sided impact, except the double-sided impact. It is thus imperative to choose the suitable parameter to control the motion state of the system and then reduce the vibration and avoid the chaos.

Fig. 8
figure 8

Dynamic response curves at Ω = 0.3. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 9
figure 9

Dynamic response curves at Ω = 0.83. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 10
figure 10

Dynamic response curves at Ω = 0.941. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 11
figure 11

Dynamic response curves at Ω = 0.958. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 12
figure 12

Dynamic response curves at Ω = 1.0. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 13
figure 13

Dynamic response curves at Ω = 1.05. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 14
figure 14

Dynamic response curves at Ω = 1.2. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 15
figure 15

Dynamic response curves at Ω = 1.5. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 16
figure 16

Dynamic response curves at Ω = 1.82. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image
Fig. 17
figure 17

Dynamic response curves at Ω = 2.1. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load curve

Full size image

3.3 Effect of damping ratio ζ on bifurcation and chaos characteristics

To study the influence of damping ratio ζ on the dynamic behaviors, the damping ratio ζ is selected as the varying parameter in the corresponding bifurcation diagram and 3-D frequency spectrum. The other parameters of the system used in the next numerical simulation are as follows: μ = 0.07, Ω = 1, b = 1 and Fm/Fa = 0.5 or 2. Thus, Figs. 18 and 19 illustrate the bifurcation diagrams and 3-D frequency spectrums of the gear system under lightly and heavily loaded conditions, in which damping ratio ζ is under the range of 0 < ζ < 0.2.

Fig. 18
figure 18

Bifurcation diagrams with the varying of ζ under lightly and heavily loaded conditions, respectively

Full size image
Fig. 19
figure 19

3-D frequency spectrum with the varying of ζ under lightly and heavily loaded conditions, respectively

Full size image

As seen in Fig. 18a, under lightly loaded condition, the gear system shows diverse motion states including period-one, multi-period and chaotic motions. Therefore, it is indispensable to determine motion state of system in detail by using time-domain waveform, frequency plot, phase diagram, Poincaré map and dynamic load. When ζ > 0.17, the time-domain waveform is periodic sinusoid, frequency plot has an amplitude peak, the phase diagram is an approximate ellipse, and the Poincaré map occurs a single point. The result indicates that the gear system is in period-one motion. The corresponding dynamic load is zero but has no negative value, where there exists single-sided impact when meshing. As damping ratio ζ is decreased, the system bifurcates into period two in the interval of 0.07 < ζ < 0.17. For instance, when ζ = 0.15, the time-domain waveform is period-two harmonic response, frequency plot shows harmonics frequencies including 0.5Ω and 1.5Ω, the phase diagram is a non-circular closed curve and the Poincaré map has two discrete points, which can be observed from Fig. 21a–c. When ζ is 0.115 around, there appears jump discontinuity phenomena, where the vibration displacement changes abruptly whereas the system is also in the state of period two. Further decreasing damping ratio ζ, the gear system goes into period-four motion, even turns into period-eight motion. When ζ is equal to 0.068, the Poincaré map is four discrete points, revealing that system motion is period four, as shown in Fig. 22c. Similarly, under ζ = 0.061, the Poincaré map in Fig. 23c is eight discrete points and the complex frequencies about Ω/4, Ω/2, 3Ω/4, Ω, 5Ω/4, 3Ω/2 appear in frequency diagram. These mean that the system is in period-eight motion. Finally, the gear system displays under the chaotic motion in the range of ζ < 0.058. As ζ = 0.05, the time-domain waveform is non-periodic curve, there are continuous and different frequency components in frequency plot, many disordered curves occur in the phase diagram, and the Poincaré map has lots of discrete points, as shown in Fig. 24a–c. When damping ratio ζ decreases, the dynamic loads have zero value among Figs. 20d, 21d, 22d, 23d and 24d, indicating that the system is always in the single-sided impact state (state II), while the amplitude of dynamic load increases gradually. In other words, as the damping ratio ζ is chosen to the smaller value, the dynamic load largens. Apparently, there exists the similar change trend of vibration amplitude as can be seen in frequency plot. However, as damping ratio ζ decreases further, the dynamic load has a negative value, which exhibits that the state of meshing gear pairs turns from single-sided impact into double-sided impact in Fig. 25 and could aggravate the dynamic characteristic of system.

Fig. 20
figure 20

Dynamic response curve at ζ = 0.19. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 21
figure 21

Dynamic response curve at ζ = 0.15. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 22
figure 22

Dynamic response curve at ζ = 0.068. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 23
figure 23

Dynamic response curve at ζ = 0.061. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 24
figure 24

Dynamic response curve at ζ = 0.05. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 25
figure 25

Dynamic loads at ζ = 0.01, 0.03

Full size image

From Fig. 18b, the period motions are the main motion responses of system in range of 0 < ζ <0.2 under the heavily loaded condition. It should be pointed out that the system is in the chaotic motion only at very low values of damping ratio ζ, where there appear continuous and complex frequencies observed from the corresponding 3-D frequency spectrum. Hence, it clearly shows that the dynamic behaviors of system are more complex under the condition of light load than under the condition of heavy load. Partly, it is due to the fact that the static meshing force will increase as well with the increase of external load and the contact states consisting of double-sided and single-sided impacts may disappear gradually and then make gear pair meshing continuously.

Through above analysis, as damping ratio ζ decreases from 0.2 to 0, the gear pair starts with period-one state, and then goes through multi-period motion, i.e. period-two, period-four and period-eight, and finally bifurcates into chaotic motion. Keeping rotational speed, gear backlash and other parameters constant, it could enhance stability and reliability of the gear system as ζ increases, owing to the energy consumed by the power transmission.

3.4 Effect of gear backlash b on bifurcation and chaos characteristics

Considering manufacture and assembly errors, gear lubrication and so forth, there exists backlash all the time in gear system. The strong nonlinearity caused by gear backlash makes the meshing contact state of gear pair change frequently. Under single-sided impact, double-sided impact or alternate states between the above two impact forms, the system may be in violent vibration. Thus, it is vital to analyze the effect of gear backlash b on the dynamic behaviors of system.

In this subsection, the gear backlash b is selected as a control parameter in the bifurcation diagram and 3-D frequency spectrum. Meantime, the other parameters in the gear system are set as follows: ζ = 0.07, Ω = 1, μ = 0.07 and Fm/Fa = 0.5 or 2. Then, the bifurcation diagrams and the corresponding 3-D frequency spectrums with the change of b under lightly and heavily loaded conditions, respectively, are illustrated in Figs. 26 and 27. Under lightly loaded condition, as gear backlash b increases, the gear system shows rich bifurcation features which contains period-doubling bifurcation, reverse bifurcation and so forth, indicating the system goes through complex motion states. However, under heavily loaded condition, with the increasing of b, the gear is always at period-one state, as shown in Fig. 26b. It means that gear backlash b does not affect the motion property and only changes the displacement amplitude of system.

Fig. 26
figure 26

Bifurcation diagrams with the varying of b with respect to X under lightly and heavily loaded conditions, respectively

Full size image
Fig. 27
figure 27

3-D frequency spectrum with the varying of b under lightly and heavily loaded conditions, respectively

Full size image

Owing to the complexity of bifurcation character, it is inevitable to show clearly the evolution process about motion states of gear system under lightly loaded condition. Figure 28 exhibits the particular bifurcation diagram with the range of 0.1 ≤ b ≤ 0.7. Time-domain waveform, phase diagram, frequency plot, Poincaré map and dynamic load diagram are used to interpret the dynamic behaviors of gear set. When b < 0.2, the time-domain waveform is a single periodic sinusoid, frequency plot exists an amplitude of excitation frequency, the phase diagram is sealed curve, and there is only a point in corresponding Poincaré map, as shown in Fig. 29a–c. The results represent the system undergoes period-one motion. The dynamic load alternately has a positive value and a negative value in Fig. 29d, indicating the meshing gear pair is under alternation states among no impact, single-sided impact and double-sided impact. However, in period motion, the system is subjected to severe tooth meshing states including tooth separation, tooth impact and large dynamic load, leading to the worse dynamic characteristic of system. Then, the system enters into the periodic doubling state, namely period-two and period-four forms. As gear backlash b keeps increasing, the system gets into chaotic motion in the scope of 0.22 < b < 0.27. When b = 0.25, the time-domain waveform shows the non-periodic motion, the phase diagram becomes disorder, and the Poincaré map is evolved into the fractal structure, as shown in Fig. 30. These results mean the system is in the chaotic motion. However, the corresponding dynamic load is equal or lesser than zero in most of time of gear meshing process, which shows the meshing contact state of gear pair is under the state between single-sided impact and double-sided impact frequently alternately. After the width of chaotic motion region, the system bifurcates to period-three motion, as shown in Fig. 31. Comparing Fig. 31d with Fig. 30d, the time that dynamic load is greater than zero increases in Fig. 31d, which demonstrates no impact state appears frequently. Subsequently, the system enters into chaos motion again in the interval of 0.31 < b < 0.35, where Fig. 32 illustrates the chaos state at b = 0.32. As b increases further, the system gets into period-four motion. When b = 0.36, the time-domain waveform shows the four periodic curve, and there are four discrete points in Poincaré map, as seen in Fig. 33a–c. The dynamic load still shows that the gear pair often switches meshing contact forms among the three impact states. And then, the gear system goes into period-two state by the route of reverse bifurcation. Keeping gear backlash b increasing further, the system enters into the chaotic motion once more. When b = 0.53, the time-domain waveform represents the non-periodic curve, the phase diagram is disorder and the Poincaré map is evolved into two fractal structures, as shown in Fig. 34a–c. Likewise, Fig. 34d suggests the meshing gear pair goes into severe contact states. Before getting into the next window of chaotic state, there exists a certain width of period-eight motion. When b = 0.55, there are eight discrete points in Poincaré map in Fig. 35c, indicating the system is under the period-eight motion. Then, the gear system goes into the chaos motion, as shown in Fig. 36. Increasing the gear backlash b even further, the system gets back to the period motion. At b = 0.605, the time-domain waveform, phase diagram and Poincaré map all show that the system enters period-four motion in Fig. 37. Then, the window of chaos motion appears again, as shown in Fig. 38. After the region of chaos, the gear pair bifurcates to period-four motion and then goes through period-two form, finally turns into period-four motion. When b = 1, the corresponding dynamic load has the negative value no longer, as illustrated in Fig. 39. It means that the meshing contact of gear pair only has no impact state and single-sided impact state.

Fig. 28
figure 28

Under lightly loaded condition, bifurcation diagram with the range of 0.1 ≤ b ≤ 0.7

Full size image
Fig. 29
figure 29

Dynamic response curve at b = 0.1. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 30
figure 30

Dynamic response curve at b = 0.25. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 31
figure 31

Dynamic response curve at b = 0.28. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 32
figure 32

Dynamic response curve at b = 0.32. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 33
figure 33

Dynamic response curve at b = 0.36. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 34
figure 34

Dynamic response curve at b = 0.53. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 35
figure 35

Dynamic response curve at b = 0.55. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 36
figure 36

Dynamic response curve at b = 0.586. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 37
figure 37

Dynamic response curve at b = 0.605. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 38
figure 38

Dynamic response curve at b = 0.618. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image
Fig. 39
figure 39

Dynamic response curve at b = 1. a Time-domain waveform, b frequency plot, c phase diagram and Poincaré map, d dynamic load

Full size image

Based on the analysis above, it can be concluded that the varying gear backlash b gives rise to complex bifurcation features that the system goes through a diverse range of motion forms. In the chaotic state, the motions of system become unpredictable and uncontrollable. In addition, the dynamic load is high sensitivity to the change of gear backlash, which results in different impact states. Therefore, it is crucial to choose the suitable value of gear backlash for suppressing vibration of gear system, controlling impact state and avoiding chaos.

4 Conclusion

This paper builds a nonlinear dynamic model of a spur gear pair, which includes several nonlinear factors such as tooth face friction, static transmission error, backlash and time-varying stiffness. According to the extending period method, the time-varying meshing stiffness of gear pair is calculated. Meantime, the tooth face friction is derived based on the meshing principle. Then, the dynamic differential equation of the system is solved by applying Runge–Kutta numerical integration method. Rotational speed, damping ratio and backlash are selected as the control parameters to analyze the effect on the bifurcation and chaos characteristics under different loaded conditions. The dynamic responses of system are determined and discussed in detail from time-domain waveform diagram, frequency plot, phase portrait, Poincaré map and dynamic load diagram.

The analysis results show that as rotational speed increases under the condition of light load, the gear system initiates with periodic state, then goes through chaos, and lastly turns into the period motion again, showing rich motion forms. The corresponding meshing contact states are changed from no impact to single-sided impact state. However, under the condition of heavy load, the system only is in period motion including period-one and period double forms. Likewise, decreasing the damping ratio, the gear system turns into the chaotic motion via the channel of period double motion. The corresponding dynamic load is gradually increased with the decreasing of the damping ratio, where the meshing contact state of system is transferred from single-sided impact to double-sided impact. By contrast, when the gear system is under the condition of heavy load, the width of chaos region becomes narrow extremely, which is almost superseded by periodic motion. Besides, when gear backlash changes, there are several windows of the chaotic motion in bifurcation diagrams under lightly loaded condition. When gear backlash is smaller value, the dynamic load has a negative value, which means the meshing gear pair is in the state of double-sided impact. Whereas the system is always in period-one state with gear backlash increasing under heavily loaded condition. Overall, the nonlinear dynamic responses of gear system with friction are investigated in detail; especially, the proper values of system parameters should be chosen to reduce vibration amplitude, avoid the chaos and enhance the stability of system.

Though the bifurcation and chaos features for a spur gear pair system with sliding friction have been analyzed, there are some crucial topics that should be investigated in the future. Therefore, the stability analysis and the effect of friction on vibration displacement, chaos and bifurcation of gear system will be studied in the next stage.