1 Introduction

Because of the highly correlated with human life, traffic flow and its related problem, which can been seen as physical phenomena [1,2,3,4,5], have been widely investigated by using different methodologies [6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58,59,60,61,62]. A series of experiments had been done for investigating the mechanism of traffic flow and identifying the influencing factors [14,15,16,17]. Many interesting non-equilibrium phenomena such as phase transition, density waves, stop-and-go flows, local clusters and ghost jams had been observed. In order to make a better understanding and give a well explanation for traffic flow, there is a demand for realistic and quantitative models that can duplicate the phenomena observed in a real situation and explain the evolution mechanism of phase transition. To achieve this goal, various traffic flow models including the continuum models [6,7,8,9,10,11,12,13], car-following models [20,21,22,23,24,25,26,27,28,29,30], and lattice hydrodynamic models [31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58,59,60,61,62] were proposed by many scholars with different backgrounds.

Lattice hydrodynamic model, which was deduced based on the car-following models and the continuum models, was firstly proposed by Nagatani in Ref. [33]. By using the linear stability theory and nonlinear analysis method, Nagatani found the neutral stability line and the modified Korteweg-de Vries (MKdV) equation successfully, and derived the solutions of kink density wave in the MKdV. Based on the model proposed by Nagatani, many researchers had proposed many extended versions with the consideration of different factors like anticipation effect [42, 48, 49], driver’s characteristics [40, 50, 53, 54], forward and backward looking effect [44, 57], traffic interruption probability [41, 51], and (multiply) density difference effect [47, 55, 56]. Passing behavior, as one of the inevitable but dangerous driving behaviors, had been investigated by some scholars. In Ref. [37], Nagatani proposed an extended lattice model considering the effect of passing and found that passing has an important effect on traffic flow. Gupta and Redhu [38] proposed an new lattice model considering driver’s anticipation behavior with passing effect and found that traffic jam can be suppressed efficiently by considering the anticipation effect. The result revealed that the negative effect of passing upon traffic flow can be alleviated by taking anticipation effect into account. Furthermore, Gupta et al. [39] proposed a lattice model to investigate the effect of multi-phase optimal velocity function upon traffic flow with passing and concluded that the proposed model can significantly enhance the stability of traffic flow for any value of passing constant even in the case of multi-phase optimal velocity model. In Ref. [40], the effect of driver’s characteristics on traffic flow with passing had been investigated. However, to our knowledge, the effect of road condition upon traffic flow with passing has not been discussed until now.

In general, traffic is a combination of people, vehicles, and roads. As the implementation of traffic infrastructure, road is an important influence factor on traffic flow. Considering road situation, Tang et al. [24] proposed a car-following model to investigate the effect of road conditions on traffic flow. Zhu and Zhang [60] proposed a lattice model considering the effect of gradient. In Ref. [62], Zhou and Shi investigated the effects of radian and angle going into curved road on traffic flow by using an extended lattice hydrodynamic model; the results showed that these two factors had an important influence on the stability of traffic flow. From the results, we can see traffic flow on curved road is more complicated than the one on straight highway. And it deserves further study by considering other factors. Passing, as we know, is a significant factor which greatly affect the stability and safety of traffic flow on curved road. Considering traffic safety, passing is prohibited when vehicles running on curved road especially sharp turn. But with the development of road construction, there exists multi-lane traffic system in modern traffic. In addition, aggressive drivers tend to overtake when passing conditions are satisfied even on curved road. Hence, we propose an extended lattice model to study the effect of passing on traffic flow on curved road.

The paper is organized as follows. In Sect. 2, the model is formulated by considering passing behavior for traffic flow on curved road. The stability analysis is obtained by using linear stability analysis in Sect. 3. We can see the stability condition varies with the parameter of passing \(\gamma \). In Sect. 4, the Burgers, Korteweg-de Vries (KdV), and MKdV equations are derived in three types of traffic flow regions by using nonlinear analysis. The simulations are given in Sect. 5. Section 6 is the summary.

2 Model

In Ref. [62], Zhou and Shi proposed a lattice hydrodynamic model to investigate traffic flow on curved road. The model is

$$\begin{aligned} \partial _{t}\rho (j,t)+\frac{\rho _{0}}{\sin \theta _j}\partial _{j}\rho (j,t)v(j,t)=0, \end{aligned}$$
(1)

with the following evolution equation of traffic flux \(\rho v\) at site j

$$\begin{aligned} \rho (j,t+\tau )v(j,t+\tau )=\frac{\rho _{0}}{\sin \theta _j}V(\rho (j+1,t)), \end{aligned}$$
(2)

where \(\theta _j\) represents the radian at site j of the curved road. \(\rho _{0}\) is the total average density. \(\rho (j,t), v(j,t)\) denote the density and velocity at site j at time t, respectively. \(V(\rho (j,t))\) is the optimal velocity function. Here we select \(V(\rho (j,t))\) as follows [62]

$$\begin{aligned} V(\rho (j,t))= & {} k\frac{\sqrt{\mu g R}}{2}\left\{ \tanh \left[ \frac{2}{\rho _{0}}-\frac{\rho (j,t)}{\rho _{0}^{2}}- \frac{1}{\rho _{c}}\right] \right. \nonumber \\&\left. +\tanh \left( \frac{1}{\rho _{c}}\right) \phantom {\left[ \frac{2}{\rho _{0}}-\frac{\rho (j,t)}{\rho _{0}^{2}}- \frac{1}{\rho _{c}}\right] }\right\} , \end{aligned}$$
(3)

where \(\rho _{c}\) is the critical density and it is equal to the inverse of the safety distance [50]. R is the radius of curved road, g is gravity acceleration. k is the control parameter of \(v_{{\text {max}}}\). Because when running on curved road, drivers always slow down with the consideration of safety, the velocity is far less than the maximal velocity.

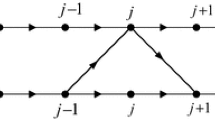
In terms of Nagatani’s idea [37], passing occurs when the traffic current on site j is larger than the one on site \(j+1\). It is assumed that the traffic quantity of the passing on site j is proportional to the difference between the optimal traffic currents on sites j and \(j+1\). Hence, we modify the evolution Eq. (2) by taking passing effect into account, that is

$$\begin{aligned} \begin{aligned}&\rho (j,t+\tau )v(j,t+\tau )\\&\quad =\frac{\rho _{0}}{\sin \theta _j}V(\rho (j+1,t))+\frac{\gamma \rho _0}{\sin \theta _j}\\&\quad \quad \times [V(\rho (j+1,t))-V(\rho (j+2,t))]. \end{aligned} \end{aligned}$$
(4)

Here \(\gamma \) is the parameter of passing.

By inserting Eq. (4) into Eq. (1), and when site j is sufficiently close to site \(j-1\), then \(\theta _j\approx \theta _{j-1}\). The total density equation is obtained as

$$\begin{aligned} \begin{aligned}&\rho (j,t+2\tau )-\rho (j,t+\tau )\\&\quad +\frac{\tau \rho _0^2}{\sin ^2\theta _j}[V(\rho (j+1,t))-V(\rho (j,t))]\\&\quad -\frac{\gamma \tau \rho _0^2}{\sin ^2\theta _j}[V(\rho (j+2,t))-2V(\rho (j+1,t))\\&\quad +V(\rho (j,t))]=0. \end{aligned} \end{aligned}$$
(5)

3 Linear stability analysis

We apply the linear stability theory to analyze the traffic flow model described by Eq. (5). Supposing the vehicles running on curved road with the uniform density \(\rho _{0}\) and optimal velocity \(V(\rho _{0})\), then we get the uniform steady state solution \(\rho (j,t)\) for Eq. (5)

$$\begin{aligned} \rho (j,t)=\rho _{0}, v(j,t)=V(\rho _{0}). \end{aligned}$$
(6)

Assuming y(j, t) be a small deviation from the uniform steady solution, that is

$$\begin{aligned} \rho (j,t)=\rho _{0}+y(j,t). \end{aligned}$$
(7)

Inserting it and Eq. (6) into Eq. (5), then the linearized equation for y(j, t) is obtained from Eq. (5)

$$\begin{aligned} \begin{aligned}&y(j,t+2\tau )-y(j,t+\tau )\\&\quad +\frac{\tau \rho _0^2}{\sin ^2\theta _j}V'(\rho _{0})[y(j+1,t)-y(j,t)]\\&\quad -\frac{\gamma \tau \rho _0^2}{\sin ^2\theta _j}V'(\rho _{0})[y(j+2,t)\\&\quad -2y(j+1,t)+y(j,t)]=0, \end{aligned} \end{aligned}$$
(8)

where \(V'(\rho _{0})\) is the derivative of optimal velocity function \(V(\rho )\) at point \(\rho =\rho _{0}\). Expand \(y(j,t)\propto \exp [ikj+zt]\) resulting in the following equation of z

$$\begin{aligned} \begin{aligned}&{\text {e}}^{2z\tau }-{\text {e}}^{z\tau }+\frac{\tau \rho _0^2}{\sin ^2 \theta _j}V'(\rho _{0})({\text {e}}^{ik}-1)\\&\quad +\frac{\gamma \tau \rho _0^2}{\sin ^2\theta _j}V'(\rho _{0})(2{\text {e}}^{ik}-{\text {e}}^{2ik}- 1)=0, \end{aligned} \end{aligned}$$
(9)

where \(z = z_{1}(ik)+z_{2}(ik)^{2} +\cdots \), and inserts it into Eq. (9), the first- and second-order terms of ik are obtained

$$\begin{aligned} z_{1}= & {} - \frac{\rho _0^2}{\sin ^2\theta _j}V'(\rho _{0}), \end{aligned}$$
(10)
$$\begin{aligned} z_{2}= & {} -\frac{3\tau \rho _{0}^4V'^{2}(\rho _{0})}{2\sin ^4\theta _j}-\frac{(1-2\gamma )\rho _{0}^2V'(\rho _{0})}{2\sin ^2\theta _j}. \end{aligned}$$
(11)

If \(z_{2}>0\), the uniform steady state becomes stable, while the uniform steady state becomes unstable if \(z_{2}<0\). Then the stable condition for traffic flow is

$$\begin{aligned} \tau < \frac{-(1-2\gamma )\sin ^2\theta _j}{3\rho _{0}^2V'(\rho _{0})}. \end{aligned}$$
(12)

Moreover, for small disturbances of long wave length, the neutral stability condition is given by

$$\begin{aligned} \tau _{s}=\frac{-(1-2\gamma )\sin ^2\theta _j}{3\rho _{0}^2V'(\rho _{0})}. \end{aligned}$$
(13)

When \(\theta _j=\pi /2\), the results are agreed with the ones in Ref. [37]. When \(\gamma =0\), the results are agreed with the ones in Ref. [62].

The neutral stability lines (solid lines) in the space \((\rho ,a)\) (\(a=1/\tau \)) for different values of \(\gamma \) with \(\rho _{0}=\rho _{c}=0.2, \theta _j=\pi /4, \mu =0.3, R=20, k=0.14\) are shown in Fig. 1. The region, which is within the neutral stability line, is the unstable region. The apex of each curve represents the critical point \((\rho _c,a_c)\). From Fig. 1, it is shown that the unstable region enlarges with increasing \(\gamma \), which means traffic flow becomes unstable with the increasing of \(\gamma \).

Fig. 1
figure 1

Phase diagram in the \((\rho ,a)\) space with \(\rho _0=\rho _c=0.2, \mu =0.3, R=20, k=0.14\) for different \(\gamma \)

Full size image

4 Nonlinear analysis

In this section, we study the nonlinear behavior of traffic flow described by Eq. (5). Note that the Eq. (5) is a nonlinear partial differential equation, and it is difficult to get the exact and analytical solution for Eq. (5). To overcome this difficulty, we apply the reductive perturbation method introduced in Ref. [38] to solve Eq. (5). We introduce slow scales for space variable j and time variable t and define slow variables X and T for \( 0 <\varepsilon \ll 1\) [57]

$$\begin{aligned} X =\varepsilon (j+bt),\qquad T =\varepsilon ^{s}t \end{aligned}$$
(14)

where b is a constant to be determined. Assuming

$$\begin{aligned} \rho (j,t) = \rho _{0} +\varepsilon ^{l} R(X,T). \end{aligned}$$
(15)

By substituting Eqs. (14)–(15) into Eq. (5) and expanding to the \(s+l+1\) order of \(\varepsilon \), we obtain the following nonlinear partial differential equation

$$\begin{aligned}&\varepsilon ^{l+1}\Big [b+\frac{\rho _{0}^2V'(\rho _{0})}{\sin ^2\theta _j}\Big ]\partial _{X}R\nonumber \\&\quad +\,\varepsilon ^{l+2}\Big [\frac{3b^{2}\tau }{2}+\frac{(1-2\gamma )\rho _{0}^2V'(\rho _{0})}{2\sin ^2\theta _j}\Big ]\partial _{X}^{2}R\nonumber \\&\quad +\,\varepsilon ^{l+3}\Big [\frac{7b^{3}\tau ^{2}}{6}+\frac{(1-6\gamma )\rho _{0}^2V'(\rho _{0})}{6\sin ^2\theta _j}\Big ]\partial _{X}^{3}R\nonumber \\&\quad +\,\varepsilon ^{l+4}\Big [\frac{5b^{4}\tau ^{3}}{8}+\frac{(1-14\gamma )\rho _{0}^2V'(\rho _{0})}{24\sin ^2\theta _j}\Big ]\partial _{X}^{4}R\\&\quad +\,\varepsilon ^{2l+1}\frac{\rho _{0}^2V''(\rho _{0})}{2\sin ^2\theta _j}\partial _{X}R^{2}+\varepsilon ^{2l+2}\frac{(1-2\gamma )\rho _{0}^2V''(\rho _{0})}{4\sin ^2\theta _j}\partial _{X}^{2}R^{2}\nonumber \\&\quad +\,\varepsilon ^{3l+1}\frac{\rho _{0}^2V'''(\rho _{0})}{6\sin ^2\theta _j}\partial _{X}R^{3}\nonumber \\&\quad +\,\varepsilon ^{3l+2}\frac{(1-2\gamma )\rho _{0}^2V'''(\rho _{0})}{12\sin ^2\theta _j}\partial _{X}^{2}R^{3}\nonumber \\&\quad +\,\varepsilon ^{s+l}\partial _{T}R +\varepsilon ^{s+l+1}3b\tau \partial _{X}\partial _{T}R=0.\nonumber \end{aligned}$$
(16)

Assuming \(s= 3,l = 1\), the following nonlinear partial differential equation is obtained from Eq.(16),

$$\begin{aligned} \begin{aligned}&\varepsilon ^{2}\Big [b+\frac{\rho _{c}^2V'(\rho _{c})}{\sin ^2\theta _j}\Big ]\partial _{X}R\\&\quad +\varepsilon ^{3}\Big [\frac{3b^{2}\tau }{2}+\frac{(1-2\gamma )\rho _{c}^2V'(\rho _{c})}{2\sin ^2\theta _j}\Big ]\partial _{X}^{2}R\\&\quad +\varepsilon ^{4}\Big \{\Big [\frac{(1-6\gamma )\rho _{c}^2V'(\rho _{c})}{6\sin ^2\theta _j}+\frac{7b^{3}\tau ^{2}}{6} \Big ]\partial _{X}^{3}R\\&\quad +\frac{\rho _{c}^2V'''(\rho _{c})}{6\sin ^2\theta _j}\partial _{X}R^{3}+\partial _{T}R\Big \}\\&\quad +\varepsilon ^{5}\Big \{\Big [\frac{5b^{4}\tau ^{3}}{8}+\frac{(1-14\gamma )\rho _{c}^2V'(\rho _{c})}{24\sin ^2\theta _j}\Big ]\partial _{X}^{4}R\\&\quad +\frac{(1-2\gamma )\rho _{c}^2V'''(\rho _{c})}{12\sin ^2\theta _j}\partial _{X}^{2}R^{3}+3b\tau \partial _{X}\partial _{T}R\Big \}=0. \end{aligned} \end{aligned}$$
(17)

Supposing

$$\begin{aligned} \frac{\tau }{\tau _{c}}=1+\varepsilon ^{2} \end{aligned}$$
(18)

for \(\tau \) near the critical point \((h_{c},1/\tau _{c})\), where \(\tau _{c}=\frac{-(1-2\gamma )\sin ^2\theta _j}{3\rho _{c}^2V'(\rho _{c})}\). Let \(b = -\frac{\rho _{c}^2V'(\rho _{c})}{\sin ^2\theta _j}\), the second- and third-order terms of \(\varepsilon \) can be eliminated from Eq. (17). Then Eq. (17) can be rewritten as

$$\begin{aligned} \begin{aligned}&\varepsilon ^{4}\left[ \partial _{T}R-g_{1}\partial ^{3}_{X}R+g_{2}\partial _{X}R^{3}\right] \\&\quad +\varepsilon ^{5}\left[ g_{3}\partial ^{2}_{X}R+g_{4}\partial ^{2}_{X}R^{3}+g_{5}\partial ^{4}_{X}R\right] =0 \end{aligned} \end{aligned}$$
(19)

where

$$\begin{aligned} g_{1}= & {} -\frac{(1-13\gamma -14\gamma ^2)\rho _{c}^2V'(\rho _{c})}{27\sin ^2\theta _j},\\ g_{2}= & {} \frac{\rho _{c}^2V'''(\rho _{c})}{6\sin ^2\theta _j},\\ g_{3}= & {} -\frac{(1-2\gamma )\rho _{c}^2V'(\rho _{c})}{2\sin ^2\theta _j},\\ g_{4}= & {} -\frac{(1-2\gamma )\rho _{c}^2V'''(\rho _{c})}{12\sin ^2\theta _j},\\ g_{5}= & {} -\frac{(1-6\gamma +39\gamma ^2+46\gamma ^3)\rho _{c}^2V'(\rho _{c})}{54\sin ^2\theta _j}. \end{aligned}$$

In order to derive the standard MKdV equation with higher-order correction, we make the following transformation in Eq. (19)

$$\begin{aligned} \begin{aligned} T_{m}&= -\frac{(1-13\gamma -14\gamma ^2)\rho _{c}^2V'(\rho _{c})}{27\sin ^2\theta _j}T, \\ R_{m}&= 3\sqrt{\frac{V'''(\rho _c)}{(28\gamma ^2+26\gamma -2)V'(\rho _c)}} R, \end{aligned} \end{aligned}$$
(20)

with an assumption that

$$\begin{aligned} 1-13\gamma -14\gamma ^2>0. \end{aligned}$$
(21)

Then, we obtain the standard MKdV equation with higher-order correction term

$$\begin{aligned} \begin{aligned}&\partial _{T_{m}}R_{m}-\partial ^{3}_{X}R_{m}+\partial _{X}R_{m}^{3}\\&\quad +\frac{\varepsilon }{g_{1}}\left[ \phantom {\frac{g_{1}g_{4}}{g_{2}}}g_{3}\partial ^{2}_{X}R_{m}\right. \\&\left. \quad +\frac{g_{1}g_{4}}{g_{2}}\partial ^{2}_{X}R_{m}^{3}+g_{5}\partial ^{4}_{X}R_{m}\right] =0. \end{aligned} \end{aligned}$$
(22)

If we ignore the \(O(\varepsilon )\) term in Eq. (22), it is just the MKdV equation with the kink-antikink solution

$$\begin{aligned} R_{m0}(X,T_{m}) = \sqrt{B}\tanh \sqrt{\frac{B}{2}}(X -BT_{m}). \end{aligned}$$
(23)

In order to obtain the value of propagation velocity B for the kink-antikink solution, the solvability condition [58,59,60]

$$\begin{aligned} (R_{m0},M[R_{m0}])\equiv \int ^{\infty } _{-\infty }{\text {d}}X_{k}R_{m0}M[R_{m0}]= 0 \end{aligned}$$
(24)

must be satisfied, here \(M[R_{m0}]\) is the \(O(\varepsilon )\) term in Eq. (22).

$$\begin{aligned} B=\frac{5g_{2}g_{3}}{2g_{2}g_{5} -3g_{1}g_{4}}. \end{aligned}$$
(25)

Inserting Eq. (20) into Eq. (23), we get the solution of the MKdV equation

$$\begin{aligned} R(X,T) = \sqrt{\frac{g_{1}B}{g_{2}}}\tanh \sqrt{\frac{B}{2}}(X -Bg_{1}T). \end{aligned}$$
(26)

Then, we gain the kink-antikink solution of the density

$$\begin{aligned} \begin{aligned} \rho (j,t)&=\rho _{c} + \sqrt{\frac{g_{1}B}{g_{2}}\left( \frac{\tau }{\tau _{c}}-1\right) }{\text {tanh}} \left[ \sqrt{\frac{B}{2}\left( \frac{\tau }{\tau _{c}}-1\right) }\right. \\&\quad \left. \left( j-\frac{\rho _{c}^2V'(\rho _{c})}{\sin ^2\theta _j}t-Bg_{1}\left( \frac{\tau }{\tau _{c}} -1\right) t\right) \right] . \end{aligned} \end{aligned}$$
(27)

And the amplitude C of the kink-antikink solution Eq. (27) is given by

$$\begin{aligned} C =\sqrt{\frac{g_{1}B}{g_{2}}\left( \frac{\tau }{\tau _{c}}-1\right) }. \end{aligned}$$

From the above process, we can find that the kink solution exists only if condition (21) is hold. Hence, the existence condition for kink solution is \(\gamma <\frac{1}{14}\). Otherwise, we can not deduce the MKdV Eq. (22). That means the MkdV equation only exists when \(\frac{-6\sin ^2\theta _j}{21\rho _{c}^2V'(\rho _ {c})}<a<a_c\).

The kink solution represents the coexisting phase, which consists of the freely moving phase with low density and the congested phase with high density. The coexisting curve can be described by \(\rho = \rho _{c} \pm C \). Therefore, we get the coexisting curve in the \((\rho ,a)\) plane for \(\gamma <\frac{1}{14}\).

In Fig. 1, when \(\gamma =0\), the dash line is the coexisting curve. The coexisting curve and the neutral stability line are similar to the conventional gas–liquid phase transition. Three regions in traffic flow are distinguished: the unstable region which is within the neutral stability line, the metastable region which is between the neutral stability line and the coexisting curve and the stable region which is out of the coexisting curve. When \(\gamma =0.1,0.2,0.3\), the condition (22) is not satisfied. Hence, coexisting curves do not exist and are not shown in Fig. 1.

In Fig. 2, the solid lines show the plots of \(a_c\) against \(\gamma \) for different \(\theta _j\) with \(\rho _0=\rho _c=0.2, \mu =0.3, k=0.14, R=20\). From Fig. 2, the critical sensitivity \(a_c\) increases with the increase in \(\gamma \), which means traffic flow becomes unstable with the increasing of \(\gamma \). Moreover, the curves \(a_c\) represent the phase boundaries between no jam and kink jam for \(\gamma <1/14\) and no jam with chaotic jam for \(\gamma \ge 1/14\). For \(\gamma \ge 1/14\), the unstable region is further divided into two subregions: kink jam and chaotic jam. The boundary between kink and chaotic jam is the line \(a=\frac{7k\sqrt{\mu gR}}{4\sin ^2\theta _j}\) (dash lines).

Fig. 2
figure 2

Phase diagram in the \((\gamma ,a)\) space with \(\rho _0=\rho _c=0.2, R=20, \mu =0.3, k=0.14\) for different \(\theta _j\)

Full size image

Next, we study the nonlinear behavior in the stable and metastable regions, respectively. Firstly, we discuss the triangular shock waves of traffic flow in the stable region. The nonlinear partial differential equation is obtained from Eq. (16) for \(s= 2,l = 1\).

$$\begin{aligned} \begin{aligned}&\varepsilon ^{2}\Big [b+\frac{\rho _{0}^2V'(\rho _{0})}{\sin ^2\theta _j}\Big ]\partial _{X}R\\&\quad +\varepsilon ^{3}\left\{ \phantom {\left[ \frac{3b^{2}\tau }{2}+\frac{(1-2\gamma )\rho _{0}^2V'(\rho _{0})}{2\sin ^2\theta _j} \right] }\partial _{T}R+\frac{\rho _{0}^2V''(\rho _{0})}{2\sin ^2\theta _j}\partial _{X}R^{2}\right. \\&\quad \left. +\left[ \frac{3b^{2}\tau }{2}+\frac{(1-2\gamma )\rho _{0}^2V'(\rho _{0})}{2\sin ^2\theta _j} \right] \partial _{X}^{2}R\right\} =0. \end{aligned} \end{aligned}$$
(28)

Taking \(b = -\frac{\rho _{0}^2V'(\rho _{0})}{\sin ^2\theta _j}\), the second-order term of \(\varepsilon \) is eliminated in Eq. (28). We obtain the following partial differential equation

$$\begin{aligned} \begin{aligned}&\partial _{T}R+\frac{\rho _{0}^2V''(\rho _{0})}{\sin ^2\theta _j}R\partial _{X}R\\&\quad =\frac{-(1-2\gamma )\sin ^2\theta _j-3\rho _{0}^2V'(\rho _{0})\tau }{2\sin ^4\theta _j}\rho _{0}^2V'(\rho _{0})\partial _{X}^{2}R. \end{aligned} \end{aligned}$$
(29)

In accordance with criterion Eq. (13), the coefficient of the second derivative of Eq. (29) is positive in the stable region. Therefore, in the stable region, Eq. (29) is just the Burgers equation. If R(X, 0) is of compact support, then the solution R(X, T) of Eq. (29) is

$$\begin{aligned} \begin{aligned} R(X,T)&= \frac{\sin ^2\theta _j}{|\rho _{0}^2V''(\rho _{0})|T}\left[ X-\frac{\eta _{n+1}+\eta _{n}}{2}\right] \\&\quad -\frac{\sin ^2\theta _j(\eta _{n+1}-\eta _{n})}{2|\rho _{0}^2V''(\rho _{0})|T}\\&\quad \tanh \bigg [\frac{-(1-2\gamma )\sin ^2\theta _j-3\rho _{0}^2V'(\rho _{0})\tau }{2\sin ^2\theta _j}\\&\quad \times V_{0}'(\rho _{0})\frac{(\eta _{n+1}-\eta _{n})(X-\xi _{n})}{4|V''(\rho _{0})|T}\bigg ]. \end{aligned} \end{aligned}$$
(30)

where \(\xi _{n}\) are the coordinates of the shock fronts and \(\eta _{n}\) are the coordinates of the intersections of the slopes with the x-axis \((n = 1,2,...,N)\). As \(O(\frac{1}{T})\), R(X, T) decays to 0 when \(T\rightarrow +\infty \). That means any shock wave expressed by Eq. (30) in stable traffic flow region will evolve to a uniform flow when time is sufficient large.

Secondly, we discuss the soliton waves of traffic flow in the metastable region for \(\gamma <1/14\). The nonlinear partial differential equation is obtained from Eq. (16) for \(s = 3,l = 2\).

$$\begin{aligned} \begin{aligned}&\varepsilon ^{3}\Big [b+\frac{\rho _{0}^2V'(\rho _{0})}{\sin ^2\theta _j}\Big ]\partial _{X}R\\&\quad +\varepsilon ^{4}\Big [\frac{3b^{2}\tau }{2}+\frac{(1-2\gamma )\rho _{0}^2V'(\rho _{0})}{2\sin ^2\theta _j}\Big ]\partial _{X}^{2}R\\&\quad +\varepsilon ^{5}\Big \{\partial _{T}R+\frac{\rho _{0}^2V''(\rho _{0})}{2\sin ^2\theta _j}\partial _{X}R^{2}\\&\quad +\Big [\frac{7b^{3}\tau ^{2}}{6}+\frac{(1-6\gamma )\rho _{0}^2V'(\rho _{0})}{6\sin ^2\theta _j}\Big ]\partial _{X}^{3}R\Big \}\\&\quad +\varepsilon ^{6}\Big \{\frac{(1-2\gamma )\rho _{0}^2V''(\rho _{0})}{4\sin ^2\theta _j}\partial _{X}^{2}R^{2} +3b\tau \partial _{X}\partial _{T}R\\&\quad +\Big [\frac{5b^{4}\tau ^{3}}{8}+\frac{(1-14\gamma )\rho _{0}^2V'(\rho _{0})}{24\sin ^2\theta _j}\Big ]\partial _{X}^{4}R\Big \}=0. \end{aligned} \end{aligned}$$
(31)

Near the neutral stability line in the unstable region, let

$$\begin{aligned} \frac{\tau }{\tau _{s}}=1-\varepsilon ^{2}. \end{aligned}$$
(32)

By taking \(b=-\frac{\rho _{0}^2V'(\rho _{0})}{\sin ^2\theta _j}\), the third- and fourth-order terms of \(\varepsilon \) are eliminated from Eq. (31), and Eq. (31) can be rewritten as

$$\begin{aligned} \begin{aligned}&\varepsilon ^{5}\left[ \partial _{T}R-f_{1}\partial ^{3}_{X}R-f_{2}R\partial _{X}R\right] \\&\quad +\varepsilon ^{6}\left[ -f_{3}\partial ^{2}_{X}R+f_{4}\partial ^{2}_{X}R^{2}+f_{5}\partial ^{4}_{X}R\right] =0 \end{aligned} \end{aligned}$$
(33)

where

$$\begin{aligned} f_{1}= & {} -\frac{(1-13\gamma -14\gamma ^2)\rho _{0}^2V'(\rho _{0})}{27\sin ^2\theta _j},\\ f_{2}= & {} -\frac{\rho _{0}^2V''(\rho _{0})}{2\sin ^2\theta _j},\\ f_{3}= & {} -\frac{(1-2\gamma )\rho _{0}^2V'(\rho _{0})}{2\sin ^2\theta _j},\\ f_{4}= & {} -\frac{(1-2\gamma )\rho _{0}^2V''(\rho _{0})}{4\sin ^2\theta _j},\\ f_{5}= & {} -\frac{(1-6\gamma +39\gamma ^2+46\gamma ^3)\rho _{0}^2V'(\rho _{0})}{54\sin ^2\theta _j}. \end{aligned}$$

In order to derive the standard KdV equation with higher-order correction, we make the following transformation in Eq. (33)

$$\begin{aligned} T= & {} \sqrt{f_{1}}T_{k}, \nonumber \\ X= & {} - \sqrt{f_{1}}X_{k},\nonumber \\ R= & {} \frac{1}{f_{2}} R_{k}. \end{aligned}$$
(34)

By using Eq. (34), we obtain the standard KdV equation with higher-order correction term

$$\begin{aligned} \begin{aligned}&\partial _{T_{k}}R_{k}+\partial ^{3}_{X_{k}}R_{k}+R_{k}\partial _{X_{k}}R_{k}\\&\quad +\frac{\varepsilon }{\sqrt{f_{1}}}\left[ \phantom {\frac{f_{5}}{f_{1}}\partial ^{4}_{X_{k}}}-f_{3}\partial ^{2}_{X_{k}}R_{k}\right. \\&\quad \left. + \frac{f_{4}}{f_{2}}\partial ^{2}_{X_{k}}R_{k}^{2}+\frac{f_{5}}{f_{1}}\partial ^{4}_{X_{k}}R_{k}\right] =0. \end{aligned} \end{aligned}$$
(35)

Next, we assume that \(R_{k}(X_{k},T_{k}) = R_{0}(X_{k},T_{k})+\varepsilon R_{1}(X_{k},T_{k})\) to consider the \(O(\varepsilon )\) correction in Eq. (35). If we ignore the \(O(\varepsilon )\) term in Eq. (35), it is just the KdV equation with soliton solutions

$$\begin{aligned} R_{0}(X_{k},T_{k}) = A {\text {sech}}^{2} \left[ \sqrt{\frac{A}{12}}\left( X_{k}-\frac{A}{3}T_{k}\right) \right] . \end{aligned}$$
(36)

Similar to the process of deriving the value of propagation velocity B for MKdV equation, we obtain the amplitude A for the soliton solution as follows

$$\begin{aligned} A = \frac{21f_{1}f_{2}f_{3}}{24f_{1}f_{4} -5f_{2}f_{5}}. \end{aligned}$$
(37)

Substituting the values of \(f_{1}-f_{5}\) into Eq. (37), we get the value of A. Substituting each variable by the original one, we obtain soliton solutions of the density

$$\begin{aligned} \begin{aligned} \rho (j,t)&= \rho _{0} +\frac{A}{f_{2}}\left( \frac{\tau }{\tau _{s}}-1\right) \\&\quad {\text {sech}}^{2}\left[ \sqrt{\frac{A}{12f_{1}} \left( \frac{\tau }{\tau _{s}}-1\right) }\right. \\&\quad \left. \left( j-\frac{\rho _{0}^2V'(\rho _{0})}{\sin ^2\theta _j}t +\frac{A}{3}\left( \frac{\tau }{\tau _{s}}-1\right) t\right) \right] . \end{aligned} \end{aligned}$$
(38)

Now, we have derived the soliton density wave described by the KdV equation near the neutral stability line.

Fig. 3
figure 3

Traffic patterns from time \(t = 99,000\) to \(t = 100,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 4
figure 4

Density profiles \(\rho (j,t)\) at time \(t = 90,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 5
figure 5

Phase space plot at site \(j=1\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 6
figure 6

Phase space plot at site \(j=20\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 7
figure 7

Phase space plot at site \(j=60\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 8
figure 8

Phase space plot at site \(j=100\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 9
figure 9

Hysteresis loop of flux at site \(j=60\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 10
figure 10

Hysteresis loop of velocity at site \(j=60\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=2.8, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma \) = a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image

5 Simulation

In order to check the theoretical results, we carry out numerical simulations in this Section. The initial conditions of the numerical simulation are as follows: There are \(N =100\) lattices in the system, and the periodical boundary condition is applied. The initial perturbations are adopted as follows: \(\rho (j,0) = \rho _0=\rho _c= 0.2\). The local densities \(\rho (N/2, 1)\) and \(\rho (N/2-1, 1)\) at sites N / 2 and \(N/2-1\) at time \(t = 1\) are set as 0.15 and 0.25, \(k=0.14, R=20, \mu =0.3\). The radian of the curved road is from \(\theta _0=\pi /4\) to \(\theta _N= \pi /3\), where \(\theta _0\) and \(\theta _N\) represent the angle going into and leaving out curved road, respectively.

Figure 3 shows the traffic patterns after a sufficiently long time \(t = 100,000\) for different \(\gamma \) with \(a=2.8\). In Fig. 3, the patterns (a)–(d) exhibit the time evolution of the density \(\rho (j,t)\) for \(\gamma = 0, 0.06, 0.2, 0.4\), respectively. The initial disturbance leads to the kink density waves as shown in patterns (b)–(d). The small amplitude disturbance grows into congested flow since the stability condition is not satisfied. When the stability condition is satisfied, the small amplitude disturbance will dissipate, and traffic flow becomes uniform which is shown in pattern (a). The results show that with the increasing of \(\gamma \), traffic flow will become unstable and traffic congestion occur. Figure 4 shows the density profile obtained at \(t=90,000\) corresponding to Fig. 3. And it makes us see the evolution of the density with the small disturbances more clearly.

Figure 5 represents the phase space plot of density difference \(\rho (j,t)-\rho (j,t-1)\) against \(\rho (t)\) for \(t = 60,000s-70,000s\) at site \(j=1\) corresponding to Fig. 3. For pattern (a) in Fig. 5, the limit cycle leads to a single point which represents the uniform flow in the stable region. The patterns (b)–(d) exhibit the characteristic of periodicity in the form of limit cycle, and the nodes on the right sides as well as on the left sides are corresponding to the traffic states within and out of the kink traffic jam. When \(a = 2.8\), the jamming transition occurs among freely moving phase, the coexisting phase with kink density wave and the uniformly congested phase with an increase in the value of \(\gamma \).

Figures 6, 7 and 8 represent the phase space plot of density difference \(\rho (j,t)-\rho (j,t-1)\) against \(\rho (t)\) at site \(j=20, 60, 100\) for \(t = 60,000s-70,000s\) corresponding to Fig. 3, respectively. From Figs. 5c, 6c, 7c and 8c, we can see that compared with other segments of curved road, traffic flow with passing easily becomes unstable and complicated at the entrance and exit of curved road.

Figures 9 and 10 show the hysteresis loop of the flux and velocity for different \(\gamma \) at site \(j=60\) for \(t = 60,000s-70,000s\) corresponding to Fig. 3, respectively. From Fig. 9, it shows that with the decreasing of the value of \(\gamma \), the size of loops will shrink. When \(\gamma =0\) in Fig. 9a, the stability condition is held, traffic flow is stable, the hysteresis loop will not be generated, and in phase space, there will be only a point on the optimal current curve instead. From Fig. 10, it shows that with the increasing of the value of \(\gamma \), the size of loops will expand. When \(\gamma =0\) in Fig. 10a, the stability condition is held, the traffic flow is stable, the hysteresis loop will not be generated, and in phase space, there will be only a point on the optimal velocity curve instead.

Fig. 11
figure 11

Traffic patterns from time \(t = 99,000\) to \(t = 100,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 12
figure 12

Density profiles \(\rho (j,t)\) at time \(t = 90,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 13
figure 13

Phase space plot at site \(j=1\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 14
figure 14

Phase space plot at site \(j=20\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 15
figure 15

Phase space plot at site \(j=60\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 16
figure 16

Phase space plot at site \(j=100\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 17
figure 17

Hysteresis loop of flux at site \(j=60\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image
Fig. 18
figure 18

Hysteresis loop of velocity at site \(j=60\) from time \(t = 60,000\) to \(t = 70,000\) with \(a=3.5, \rho _0=\rho _c=0.2, \mu =0.3, k=0.14, \theta _0=\pi /4, \theta _N=\pi /3\) for \(\gamma =\) a 0, b 0.06, c 0.2, d 0.4, respectively

Full size image

Figure 11 shows the traffic patterns after a sufficiently long time \(t = 100,000\) for different \(\gamma \) with \(a=3.5\). In Fig. 11, the patterns (a)–(d) exhibit the time evolution of the density \(\rho (j,t)\) for \(\gamma = 0, 0.06, 0.2, 0.4\), respectively. When the stability condition is satisfied, the small amplitude disturbance will dissipate and traffic flow becomes uniform which is shown in patterns (a) and (b). For patterns (c) and (d), the stability condition is not satisfied; hence, the traffic flow is unstable due to the initial disturbance. Furthermore, we can see the traffic patterns in Fig. 11c, d are different from the ones in Fig. 3b–d. In Fig. 11c, d, the density waves band with one another, break up and propagates in the backward direction. The main reason is that \(a\ge \frac{7k\sqrt{\mu gR}}{4\sin ^2\theta _j}\), which means the traffic is in chaotic jam phase; hence, traffic flow becomes chaotic. Figure 12 shows the density profile obtained at \(t=90,000\) corresponding to Fig. 11.

Figure 13 represents the phase space plot of density difference \(\rho (j,t)-\rho (j,t-1)\) against \(\rho (t)\) for \(t = 60,000s-70,000s\) at site \(j=1\) corresponding to Fig. 11. For patterns (a) and (b), the uniform flow in the stable region is represented by a single point. Patterns (c) and (d) exhibit the behavior characteristics of chaos; the patterns exhibit dispersed plots around a closed loop due to the irregular traffic waves. This is the characteristics of chaos.

Figures 14, 15 and 16 represent the phase space plot of density difference \(\rho (j,t)-\rho (j,t-1)\) against \(\rho (t)\) at site \(j=20, 60, 100\) for \(t = 60,000s-70,000s\) corresponding to Fig. 11, respectively. From Figs. 13d, 14d, 15d and 16d, we can see that compared with other segments of curved road, traffic flow with passing easily becomes unstable at the entrance and exit of curved road, especially the entrance of curved road.

Figures 17 and 18 show the hysteresis loop of the flux and velocity for different \(\gamma \) at site \(j=60\) for \(t = 60,000s-70,000s\) corresponding to Fig. 11, respectively. From Fig. 17, it shows that with the decreasing of the value of \(\gamma \), the size of loops will shrink. When \(\gamma =0, 0.06\) in Fig. 17a, b, the stability condition is held, traffic flow is stable, the hysteresis loop will not be generated, and in phase space, there will be only a point on the optimal current curve instead. When \(\gamma =0.2, 0.4\), the hysteresis loops exhibit the behavior characteristics of chaos. From Fig. 18, it shows that with the increasing of the value of \(\gamma \), the size of loops will be expansion. When \(\gamma =0, 0.06\) in Fig. 18a, b, the stability condition is held, the traffic flow is stable, and in phase space, there will be only a point on the optimal velocity curve instead. When \(\gamma =0.2, 0.4\), the hysteresis loops exhibit dispersed plots around a closed loop due to the irregular traffic behavior. This is the characteristics of chaos.

Moreover, From Figs. 3c and 11c (Figs. 3d, 11d), we can see that for constant \(\gamma \) which is larger than the critical value, the instable region is divided into kink region and chaotic region. And the congested flow occurs from chaotic jam to kink jam with decreasing sensitivity a.

6 Summary

In order to investigate the effect of passing on traffic flow on curved road, we propose an extended lattice hydrodynamic model for traffic flow on curved road by taking passing into account. We obtain the stability condition of the proposed model by the use of linear stability theory. The stability condition shows that passing behavior play an important role in influencing the stability of traffic flow. The nonlinear wave equations including Burgers, KdV and MKdV are obtained to describe traffic flow behavior in different regions, respectively. The analytical and simulation results show that reducing passing behavior may enhance the stability of traffic flow. Jamming transition occurs between uniform flow and kink jam when \(\gamma \) is less than the critical value. When \(\gamma \) is larger than the critical value, jamming transition occurs from uniform flow to irregular wave through chaotic phase with decreasing sensitivity a. In addition, compared with other segments of curved road, traffic flow with passing easily becomes unstable and complicated at the entrance and exit of curved road, especially at the entrance of curved road. Such findings mean that at the entrance and exit of curved road, passing behavior should be prohibited. As to other segments of curved road, when passing conditions are satisfied, passing behavior could be taken if necessary.