1 Introduction

The theory of solitons plays a vital role in various areas of Physics and Engineering. It has very wide applications in nonlinear optics, fluid dynamics, nuclear physics, biophysics, plasma physics and many more [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25]. A soliton is usually associated with solution of a nonlinear evolution equation which represents a wave of permanent form. Because of that the study of nonlinear evolution equations has become a very important area in the fields of physics, mathematics and engineering. There are many newly developed techniques to carry out integration of these equations. These methods include exponential function method, G’/G method, sine-cosine method, tanh-coth method, Adomian decomposition method, Wadati trace method and many more.

The Klein–Gordon equation (KGE) is a very important equation in the study of nonlinear evolution equations which has wide applications in the field of Quantum Physics [1,2,3,4,5,6,7,8,9,10,11,12,13,14]. This paper focuses five various generalized forms of this equation. It is obtained topological and non-topological soliton solutions as well as Jacobi elliptic function solutions of the generalized KGEs in this study.

2 Governing equation

The gKGE that will be considered in this study is given by [1]

$$\begin{aligned} \left( q^{m}\right) _{tt}-k^{2}\left( q^{m}\right) _{xx}+F(q)=0 \end{aligned}$$
(1)

where k is a real number, m is a positive integer and \(m\ge 1.\) In the case of \(m=1,\) the gKGE reduces the regular KGE [10, 12]. In (1), q(x, t) represents the quantized field describing the particle, and F(q) is a continuous nonlinear function and it can be written as

$$\begin{aligned} F(q)=-\frac{\partial U}{\partial q} \end{aligned}$$
(2)

where \(U\left( q\right) \) is a potential function. The function F(q) will be addressed as five various forms in this paper as follows:

$$\begin{aligned} F(q)= & {} aq^{m}-bq^{2m} \end{aligned}$$
(3)
$$\begin{aligned} F(q)= & {} aq^{m}-bq^{3m} \end{aligned}$$
(4)
$$\begin{aligned} F(q)= & {} aq^{m}-bq^{n} \end{aligned}$$
(5)
$$\begin{aligned} F(q)= & {} aq^{m}-bq^{n}+cq^{2n-m} \end{aligned}$$
(6)
$$\begin{aligned} F(q)= & {} aq^{m}-bq^{m-n}+cq^{n+m} \end{aligned}$$
(7)

Here, a, b and c are real-valued constants for above-mentioned five forms.

3 Form-I

In this case considering Eqs. (1) and (3), the generalized form of the quadratic nonlinear KGE is given by

$$\begin{aligned} \left( q^{m}\right) _{tt}-k^{2}\left( q^{m}\right) _{xx}+aq^{m}-bq^{2m}=0. \end{aligned}$$
(8)

We first introduce the following hypothesis

$$\begin{aligned} q\left( x,t\right) =Asn^{p}\left( \tau ,\ell \right) , \end{aligned}$$
(9)

where

$$\begin{aligned} \tau =B\left( x-vt\right) . \end{aligned}$$
(10)

Here, \(\ell \) is the modulus of Jacobi elliptic function and it is defined as \(0<\ell <1\). A represents the amplitude, B represents the inverse width of the soliton, v is the soliton velocity and the unknown index p will be determined. It is obtained from the Eq. (9)

$$\begin{aligned} \left( q^{m}\right) _{tt}= & {} A^{m}B^{2}\left( mp-1\right) mpv^{2} sn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&-\,A^{m}B^{2}mpv^{2}\left( mp+\ell -\ell ^{2}+mp\ell ^{2}\right) \nonumber \\&\times \, sn^{mp}\left( \tau ,\ell \right) \nonumber \\&+\,A^{m}B^{2}mpv^{2}\ell \left( 1+mp\ell \right) sn^{mp+2}\left( \tau ,\ell \right) ,\nonumber \\ \end{aligned}$$
(11)
$$\begin{aligned} \left( q^{m}\right) _{xx}= & {} A^{m}B^{2}\left( mp-1\right) mpsn^{mp-2} \left( \tau ,\ell \right) \nonumber \\&-\,A^{m}B^{2}mp\left( mp+\ell -\ell ^{2}+mp\ell ^{2}\right) \nonumber \\&\times \, sn^{mp}\left( \tau ,\ell \right) \nonumber \\&+A^{m}B^{2}mp\ell \left( 1+mp\ell \right) sn^{mp+2}\left( \tau \ ,\ell \right) . \end{aligned}$$
(12)

Substituting (11) and (12) into (8) yields

$$\begin{aligned}&A^{m}B^{2}\left( mp-1\right) mp\left( v^{2}-k^{2}\right) sn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&\quad -\,A^{m}B^{2}mp\left( mp+\ell -\ell ^{2}+mp\ell ^{2}\right) \nonumber \\&\quad \times \,\left( v^{2}-k^{2}\right) sn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad +\,A^{m} B^{2}mpv^{2}\ell \left( 1+mp\ell \right) \left( v^{2}-k^{2}\right) sn^{mp+2}\left( \tau ,\ell \right) \nonumber \\&\quad +\,aA^{m}sn^{mp}\left( \tau ,\ell \right) -\,bA^{2m}sn^{2mp}\left( \tau ,\ell \right) =0. \end{aligned}$$
(13)

Now, from (13) matching the exponents \(mp+2\) and 2mp, one needs to have

$$\begin{aligned} mp+2=2mp, \end{aligned}$$
(14)

which leads to

$$\begin{aligned} p=\frac{2}{m}. \end{aligned}$$
(15)

Equating coefficients of them and setting coefficients of \(sn^{mp+j}\left( \tau ,\ell \right) ,\) for \(j=-2,0\), to zero in (13), as these are linearly independent functions, yields

$$\begin{aligned} A=\left[ \frac{a\ell \left( 1+2\ell \right) }{b\left( 2+\ell +\ell ^{2}\right) }\right] ^{\frac{1}{m}} \end{aligned}$$
(16)

and

$$\begin{aligned} B=\sqrt{\frac{a}{2\left( 2+\ell +\ell ^{2}\right) \left( v^{2}-k^{2}\right) }} \end{aligned}$$
(17)

From (17), it is important to note that

$$\begin{aligned} a\left( v^{2}-k^{2}\right) >0 \end{aligned}$$

and if m is even, \(ab>0\) in (16). Thus, Jacobi elliptic function solution of (8) is given by

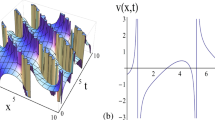
$$\begin{aligned} q(x,t)= & {} \left[ \frac{a\ell \left( 1+2\ell \right) }{b\left( 2+\ell +\ell ^{2}\right) }sn^{2}\right. \nonumber \\&\left. \left\{ \sqrt{\frac{a}{2\left( 2+\ell +\ell ^{2}\right) \left( v^{2}-k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{m}}\nonumber \\ \end{aligned}$$
(18)

If the modulus \(\ell \rightarrow 1\) in Eq. (18), we get following topological soliton solution

$$\begin{aligned} q(x,t)=\left[ \frac{3a}{4b}\tanh ^{2}\left\{ \sqrt{\frac{a}{8\left( v^{2}-k^{2}\right) }}\left( x-vt\right) \right\} \right] ^{\frac{1}{m} }\nonumber \\ \end{aligned}$$
(19)

Now, to get other solutions of (8), we use the assumption as

$$\begin{aligned} q\left( x,t\right) =Acn^{p}\left( \tau ,\ell \right) , \end{aligned}$$
(20)

Thus, one obtains

$$\begin{aligned} \left( q^{m}\right) _{tt}= & {} -A^{m}B^{2}\left( mp-1\right) mp\nonumber \\&\quad \times \left( \ell ^{2}-1\right) v^{2}cn^{mp-2}\left( \tau ,\ell \right) -A^{m}B^{2}mpv^{2}\nonumber \\&\quad \times \left( mp+\ell \left( \ell -1\right) -2mp\ell ^{2}\right) cn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad -A^{m}B^{2}mpv^{2}\ell \left( 1+mp\ell \right) cn^{mp+2}\left( \tau ,\ell \right) , \nonumber \\ \end{aligned}$$
(21)
$$\begin{aligned} \left( q^{m}\right) _{xx}= & {} -A^{m}B^{2}\left( mp-1\right) \left( \ell ^{2}-1\right) cn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&\quad -A^{m}B^{2}mp\left( mp+\ell \left( \ell -1\right) -2mp\ell ^{2}\right) \nonumber \\&\quad \times cn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad -A^{m}B^{2}mp\ell \left( 1+mp\ell \right) cn^{mp+2}\left( \tau ,\ell \right) . \end{aligned}$$
(22)

So, Eq. (8) reduces

$$\begin{aligned}&-A^{m}B^{2}\left( mp-1\right) mp\left( \ell ^{2}-1\right) \nonumber \\&\quad \times \left( v^{2}-k^{2}\right) cn^{mp-2}\left( \tau ,\ell \right) -A^{m}B^{2}\nonumber \\&\quad \times mp\left( mp+\ell \left( \ell -1\right) -2mp\ell ^{2}\right) \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times cn^{mp}\left( \tau ,\ell \right) -A^{m}B^{2}mpv^{2} \ell \nonumber \\&\quad \times \left( 1+mp\ell \right) \nonumber \\&\quad \times \left( v^{2}-k^{2}\right) cn^{mp+2}\left( \tau ,\ell \right) +aA^{m} cn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad -\, bA^{2m}cn^{2mp}\left( \tau ,\ell \right) =0. \end{aligned}$$
(23)

Matching the exponents \(mp+2\) and 2mp gives the same value of which is in (15). Equating coefficients of them and setting coefficients of \(cn^{mp+j}\left( \tau ,\ell \right) \) to zero for \(j=-2,0\), one gets

$$\begin{aligned} A=\left[ \frac{a\ell \left( 1+2\ell \right) }{b\left( -2+\ell +3\ell ^{2}\right) }\right] ^{\frac{1}{m}} \end{aligned}$$
(24)

and

$$\begin{aligned} B=\sqrt{\frac{a}{2\left( 2-\ell -3\ell ^{2}\right) \left( v^{2}-k^{2}\right) }} \end{aligned}$$
(25)

It is important to note that

$$\begin{aligned} a\left( 2-\ell -3\ell ^{2}\right) \left( v^{2}-k^{2}\right) >0 \end{aligned}$$

in (25) and also

$$\begin{aligned} ab\left( -2+\ell +3\ell ^{2}\right) >0 \end{aligned}$$

if m is even in (24). So, another Jacobi elliptic function solution of (8) is given by

$$\begin{aligned} q(x,t)= & {} \left[ \frac{a\ell \left( 1+2\ell \right) }{b\left( -2+\ell +3\ell ^{2}\right) }\right. \nonumber \\&\left. \times cn^{2}\left\{ \sqrt{\frac{a}{2\left( 2-\ell -3\ell ^{2}\right) \left( v^{2}-k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{m}}\nonumber \\ \end{aligned}$$
(26)

From (26), if the modulus \(\ell \rightarrow 1,\) we get following 1-soliton solution of (8).

$$\begin{aligned} q(x,t)=\left[ \frac{3a}{2b}\sec h^{2}\left\{ \frac{1}{2}\sqrt{\frac{a}{\left( k^{2}-v^{2}\right) }}\left( x-vt\right) \right\} \right] ^{\frac{1}{m}}\nonumber \\ \end{aligned}$$
(27)

where \(a\left( v^{2}-k^{2}\right) >0\) and if m is even \(ab>0.\)

Remark 1

1-soliton solution (27) is identical to solution in [1].

4 Form-II

Taking into consideration Eqs. (1) and (4) together gives

$$\begin{aligned} \left( q^{m}\right) _{tt}-k^{2}\left( q^{m}\right) _{xx}+aq^{m} -bq^{3m}=0. \end{aligned}$$
(28)

This equation is the generalized form of the phi-four model. In order to get solutions of this equation, we use the same starting assumption as given by (9). Substituting (11) and (12) into (28) yields

$$\begin{aligned}&A^{m}B^{2}\left( mp-1\right) mp\left( v^{2}-k^{2}\right) sn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&\quad -A^{m}B^{2}mp\nonumber \\&\quad \times \left( mp+\ell -\ell ^{2}+mp\ell ^{2}\right) \left( v^{2}-k^{2}\right) sn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad + A^{m}B^{2}mpv^{2}\ell \left( 1+mp\ell \right) \nonumber \\&\quad \times \left( v^{2}-k^{2}\right) sn^{mp+2}\left( \tau ,\ell \right) +aA^{m} sn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad -\, bA^{3m}sn^{3mp}\left( \tau ,\ell \right) =0. \end{aligned}$$
(29)

For the exponent of sn in the third and last terms to match up, one needs to have

$$\begin{aligned} mp+2=3mp, \end{aligned}$$
(30)

which yields

$$\begin{aligned} p=\frac{1}{m}. \end{aligned}$$
(31)

Equating coefficients of this third and last terms and setting coefficients of \(sn^{mp+j}\left( \tau ,\ell \right) \), for \(j=-2,0\), to zero in (29) yields

$$\begin{aligned} A=\left[ \frac{a\ell }{b}\right] ^{\frac{1}{2m}} \end{aligned}$$
(32)

and

$$\begin{aligned} B=\sqrt{\frac{a}{\left( 1+\ell \right) \left( v^{2}-k^{2}\right) }} \end{aligned}$$
(33)

Equations (32) and (33) naturally introduce the constraints \(ab>0\) and \(a\left( v^{2}-k^{2}\right) >0\), respectively. Thus, Jacobi elliptic function solution of (28) is given by

$$\begin{aligned}&q(x,t)\nonumber \\&\quad =\left[ \sqrt{\frac{a\ell }{b}}sn^{2}\left\{ \sqrt{\frac{a}{\left( 1+\ell \right) \left( v^{2}-k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{m}} \nonumber \\ \end{aligned}$$
(34)

If the modulus \(\ell \rightarrow 1\) in Eq. (34), we get following topological soliton solution

$$\begin{aligned} q(x,t)=\left[ \sqrt{\frac{a}{b}}\tanh \left\{ \sqrt{\frac{a}{v^{2}-k^{2}} }\left( x-vt\right) \right\} \right] ^{\frac{1}{m}} \end{aligned}$$
(35)

Now, to get other solutions of (28), we use the assumption as given by (20). \( Inserting this assumption into \) (28), we obtain

$$\begin{aligned}&-A^{m}B^{2}\left( mp-1\right) mp\left( \ell ^{2}-1\right) \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times cn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&\quad -A^{m}B^{2}mp\left( mp+\ell \left( \ell -1\right) -2mp\ell ^{2}\right) \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times cn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad -A^{m}B^{2}mpv^{2}\ell \left( 1+mp\ell \right) \left( v^{2}-k^{2}\right) cn^{mp+2}\left( \tau ,\ell \right) \nonumber \\&\quad +\,aA^{m}cn^{mp}\left( \tau ,\ell \right) -\,bA^{3m}cn^{3mp}\left( \tau ,\ell \right) =0. \end{aligned}$$
(36)

Equating the exponents \(mp+2\) and 3mp gives the same value of which is in (31). The functions \(cn^{mp+j}\left( \tau ,\ell \right) ,\) \(j=-2,0\), in (36) are linearly independent. So, their respective coefficients must vanish. Thus, one gets

$$\begin{aligned} A=\left[ \frac{a\ell \left( 1+\ell \right) }{b\left( -1+\ell +\ell ^{2}\right) }\right] ^{\frac{1}{2m}} \end{aligned}$$
(37)

and

$$\begin{aligned} B=\sqrt{\frac{a}{\left( 1-\ell -\ell ^{2}\right) \left( v^{2}-k^{2}\right) }} \end{aligned}$$
(38)

Equations (37) and (38) gives the constraints

$$\begin{aligned} ab\left( -2+\ell +3\ell ^{2}\right) >0 \end{aligned}$$

and

$$\begin{aligned} a\left( 1-\ell -\ell ^{2}\right) \left( v^{2}-k^{2}\right) >0 \end{aligned}$$

respectively. So, another Jacobi elliptic function solution of (28) is given by

$$\begin{aligned} q(x,t)= & {} \left[ \sqrt{\frac{a\ell \left( 1+\ell \right) }{b\left( -1+\ell +\ell ^{2}\right) }}cn^{2}\right. \nonumber \\&\times \left. \left\{ \sqrt{\frac{a}{\left( 1-\ell -\ell ^{2}\right) \left( v^{2}-k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{m}}\nonumber \\ \end{aligned}$$
(39)

From (39), if the modulus \(\ell \rightarrow 1,\) we get following 1-soliton solution of (28).

$$\begin{aligned} q(x,t)=\left[ \sqrt{\frac{2a}{b}}\sec h\left\{ \sqrt{\frac{a}{k^{2}-v^{2}} }\left( x-vt\right) \right\} \right] ^{\frac{1}{m}} \end{aligned}$$
(40)

with the conditions \(a\left( v^{2}-k^{2}\right) >0\) and \(ab>0.\)

Remark 2

1-soliton solution (40) is identical to solution in [1].

5 Form-III

In this case, considering Eqs. (1) and (5) gives

$$\begin{aligned} \left( q^{m}\right) _{tt}-k^{2}\left( q^{m}\right) _{xx}+aq^{m} -bq^{n}=0. \end{aligned}$$
(41)

This is known as generalized form of the nonlinear KGE. The special case \(m=1\) and \(n=3\) is called the \(\phi ^{6}\) model that appears in solid state physics, condensed matter physics and quantum field theory [11]. We use the same starting assumption as given by (9) to find the solutions of this equation. Substituting (11) and (12) into (41) yields

$$\begin{aligned}&A^{m}B^{2}\left( mp-1\right) mp\left( v^{2}-k^{2}\right) sn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&\quad -\,A^{m}B^{2}mp\left( mp+\ell -\ell ^{2}+mp\ell ^{2}\right) \left( v^{2} -k^{2}\right) \nonumber \\&\quad \times \, sn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad +\,A^{m}B^{2}mpv^{2}\ell \left( 1+mp\ell \right) \left( v^{2}-k^{2}\right) sn^{mp+2}\left( \tau ,\ell \right) \nonumber \\&\quad +\,aA^{m}sn^{mp}\left( \tau ,\ell \right) -\,bA^{n}sn^{np}\left( \tau ,\ell \right) =0. \end{aligned}$$
(42)

Now, from (42), for the exponents of sn in the third and last terms to match up, we take

$$\begin{aligned} mp+2=np, \end{aligned}$$
(43)

which leads to

$$\begin{aligned} p=\frac{2}{n-m}. \end{aligned}$$
(44)

Equating coefficients of them and setting coefficients of \(sn^{mp+j}\left( \tau ,\ell \right) ~\left( j=-2,0\right) \) to zero in (42) yields

$$\begin{aligned} A=\left[ \frac{a\ell \left( n-m+2\ell m\right) }{b\left[ 2m+\left( n-m\right) \left( \ell -\ell ^{2}\right) +2\ell m\right] }\right] ^{\frac{1}{n-m}} \end{aligned}$$
(45)

and

$$\begin{aligned} B=\sqrt{\frac{a\left( n-m\right) ^{2}}{2m\left[ 2m+\left( n-m\right) \left( \ell -\ell ^{2}\right) +2\ell m\right] \left( v^{2}-k^{2}\right) } }\nonumber \\ \end{aligned}$$
(46)

From (46), it is important to note that

$$\begin{aligned} a\left[ 2m+\left( n-m\right) \left( \ell -\ell ^{2}\right) +2\ell m\right] \left( v^{2}-k^{2}\right) >0, \end{aligned}$$

and also from (45)

$$\begin{aligned}&ab\left( n-m+2\ell m\right) \left[ 2m+\left( n-m\right) \left( \ell -\ell ^{2}\right) +2\ell m\right] \nonumber \\&\quad >0, \end{aligned}$$

if \(n-m\) is even. Thus, Jacobi elliptic function solution of (41) is given by

$$\begin{aligned} q(x,t)&=\left[ \frac{a\ell \left( n-m+2\ell m\right) }{b\left[ 2m+\left( n-m\right) \left( \ell -\ell ^{2}\right) +2\ell m\right] }\right. \nonumber \\&\quad \times \left. sn^{2}\left\{ \sqrt{\frac{a\left( n-m\right) ^{2} }{2m\left[ 2m+\left( n-m\right) \left( \ell -\ell ^{2}\right) +2\ell m\right] \left( v^{2}-k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{n-m}} \end{aligned}$$
(47)

If the modulus \(\ell \rightarrow 1\) in Eq. (47), we get following topological soliton solution

$$\begin{aligned}&q(x,t)\nonumber \\&\quad =\left[ \frac{a\left( n+m\right) }{4bm}\tanh ^{2}\left\{ \frac{n-m}{2m}\sqrt{\frac{a}{\left( v^{2}-k^{2}\right) }}\left( x-vt\right) \right\} \right] ^{\frac{1}{n-m}}\nonumber \\ \end{aligned}$$
(48)

providing \(a\left( v^{2}-k^{2}\right) >0\).

Now, to get other solutions of (41), we use the assumption as given by (20). Puttingthenecessary derivativesinto (41), we have

$$\begin{aligned}&-A^{m}B^{2}\left( mp-1\right) mp\left( \ell ^{2}-1\right) \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times \, cn^{mp-2}\left( \tau ,\ell \right) \nonumber \\&\quad -\,A^{m}B^{2}mp\left( mp+\ell \left( \ell -1\right) -2mp\ell ^{2}\right) \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times \, cn^{mp}\left( \tau ,\ell \right) \nonumber \\&\quad -\,A^{m}B^{2}mpv^{2}\ell \left( 1+mp\ell \right) \left( v^{2}-k^{2}\right) cn^{mp+2}\left( \tau ,\ell \right) \nonumber \\&\quad +\,aA^{m}cn^{mp}\left( \tau ,\ell \right) -\,bA^{n}cn^{np}\left( \tau ,\ell \right) =0. \end{aligned}$$
(49)

Doing similar operations as above, one gets

$$\begin{aligned} A=\left[ \frac{a\ell \left( m-n-2\ell m\right) }{b\left[ 2m\left( 1-2\ell ^{2}\right) +\ell \left( \ell -1\right) \left( n-m\right) \right] }\right] ^{\frac{1}{n-m}}\nonumber \\ \end{aligned}$$
(50)

and

$$\begin{aligned} B=\sqrt{\frac{a\left( n-m\right) ^{2}}{\left[ 2m\left( 1-2\ell ^{2}\right) +\ell \left( \ell -1\right) \left( n-m\right) \right] \left( v^{2} -k^{2}\right) }}\nonumber \\ \end{aligned}$$
(51)

It is important to note that

$$\begin{aligned} a\left[ 2m\left( 1-2\ell ^{2}\right) +\ell \left( \ell -1\right) \left( n-m\right) \right] \left( v^{2}-k^{2}\right) >0 \end{aligned}$$

in (51) and also

$$\begin{aligned}&ab\left( m-n-2\ell m\right) \\&\quad \left[ 2m\left( 1-2\ell ^{2}\right) +\ell \left( \ell -1\right) \left( n-m\right) \right] >0 \end{aligned}$$

if \(n-m\) is even, in (50). So, we have following solution

$$\begin{aligned} q(x,t)&=\left[ \frac{a\ell \left( m-n-2\ell m\right) }{b\left[ 2m\left( 1-2\ell ^{2}\right) +\ell \left( \ell -1\right) \left( n-m\right) \right] }\right. \nonumber \\&\quad \times \left. cn^{2}\left\{ \sqrt{\frac{a\left( n-m\right) ^{2} }{\left[ 2m\left( 1-2\ell ^{2}\right) +\ell \left( \ell -1\right) \left( n-m\right) \right] \left( v^{2}-k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{n-m}} \end{aligned}$$
(52)

From (52), if the modulus \(\ell \rightarrow 1,\) we get following 1-soliton solution of (41).

$$\begin{aligned} q(x,t)= & {} \left[ \frac{a\left( m+n\right) }{2bm}\sec h^{2}\right. \nonumber \\&\left. \left\{ \frac{n-m}{2m}\sqrt{\frac{a}{k^{2}-v^{2}}}\left( x-vt\right) \right\} \right] ^{\frac{1}{n-m}} \end{aligned}$$
(53)

where \(a\left( v^{2}-k^{2}\right) >0\) and if \(n-m\) is even, \(ab>0.\)

Remark 2

1-soliton solution (53) is identical to solution in [1].

6 Form-IV

In this part, considering Eqs. (1) and (6) gives

$$\begin{aligned} \left( q^{m}\right) _{tt}-k^{2}\left( q^{m}\right) _{xx}+aq^{m} -bq^{n}+cq^{2n-m}=0.\nonumber \\ \end{aligned}$$
(54)

Equation (54) known as the generalized form of the second type nonlinear KGE [10, 12]. In order to get solutions of this equation, we take the starting assumption as

$$\begin{aligned} q(x,t)=A\left[ D+sn\left( \tau ,\ell \right) \right] ^{p} \end{aligned}$$
(55)

where D is a constant. From (55) yields

$$\begin{aligned} \left( q^{m}\right) _{tt}&=-A^{m}B^{2}mpv^{2}\left( 1+p\right) \left( 1+\ell ^{2}D^{2}\right) \nonumber \\&\quad \times \left( 1+D^{2}\right) \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp-2}\nonumber \\&\quad +A^{m}B^{2}mpv^{2}\left[ -D\left( 1+2p+\ell +2p\ell ^{2}\right) \right. \nonumber \\&\quad \left. -\ell D^{3}\left( 1+3\ell +4p\ell \right) \right] \nonumber \\&\quad \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp-1}+A^{m}B^{2} mpv^{2}\nonumber \\&\quad \times \left[ p-\ell +\ell D^{2}\left( 1-2\ell -3p\ell \right) \right] \nonumber \\&\quad \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp}-A^{m}B^{2} mpv^{2}\nonumber \\&\quad \times \left[ 3\ell D-\ell ^{2}D\left( 1+4p\right) \right] \nonumber \\&\quad \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+1}+A^{m}B^{2} mpv^{2}\ell \nonumber \\&\quad \times \left( 1-p\ell \right) \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+2} \end{aligned}$$
(56)
$$\begin{aligned} \left( q^{m}\right) _{xx}&=-A^{m}B^{2}mp\left( 1+p\right) \left( 1+\ell ^{2}D^{2}\right) \left( 1+D^{2}\right) \nonumber \\&\quad \times \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp-2}\nonumber \\&\quad +A^{m}B^{2}mp\left[ -D\left( 1+2p+\ell +2p\ell ^{2}\right) \right. \nonumber \\&\quad -\left. \ell D^{3}\left( 1+3\ell +4p\ell \right) \right] \nonumber \\&\quad \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp-1}+A^{m}B^{2}mp\nonumber \\&\quad \times \left[ p-\ell +\ell D^{2}\left( 1-2\ell -3p\ell \right) \right] \nonumber \\&\quad \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp}-A^{m}B^{2}mp\nonumber \\&\quad \times \left[ 3\ell D-\ell ^{2}D\left( 1+4p\right) \right] \nonumber \\&\quad \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+1}+A^{m}B^{2} mp\ell \left( 1-p\ell \right) \nonumber \\&\quad \times \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+2} \end{aligned}$$
(57)

Substituting (56) and (57) into (54) yields

$$\begin{aligned}&-A^{m}B^{2}mp\left( 1+p\right) \left( 1+\ell ^{2}D^{2}\right) \left( 1+D^{2}\right) \nonumber \\&\quad \times \,\left( v^{2}-k^{2}\right) \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp-2}\nonumber \\&\quad +\,A^{m}B^{2}mp\left[ -D\left( 1+2p+\ell +2p\ell ^{2}\right) \right. \nonumber \\&\quad \left. -\,\ell D^{3}\left( 1+3\ell +4p\ell \right) \right] \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times \,\left[ D+sn\left( \tau ,\ell \right) \right] ^{mp-1}\nonumber \\&\quad +\,A^{m}B^{2}mp\left[ p-\ell +\ell D^{2}\left( 1-2\ell -3p\ell \right) \right] \nonumber \\&\quad \times \,\left( v^{2}-k^{2}\right) \left[ D+sn\left( \tau ,\ell \right) \right] ^{mp} \nonumber \\&\quad -\,A^{m}B^{2}mp\left[ 3\ell D-\ell ^{2}D\left( 1+4p\right) \right] \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times \,\left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+1} \nonumber \\&\quad +\,A^{m}B^{2}mp\ell \left( 1-p\ell \right) \left( v^{2}-k^{2}\right) \nonumber \\&\quad \times \,\left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+2}+aA^{m}\left[ D+sn\left( \tau ,\ell \right) \right] ^{mp}\nonumber \\&\quad -\,bA^{n}\left[ D+sn\left( \tau ,\ell \right) \right] ^{np}+cA^{2n-m}\nonumber \\&\quad \times \left[ D+sn\left( \tau ,\ell \right) \right] ^{\left( 2n-m\right) p}=0. \end{aligned}$$
(58)

Now, from (58), matching the exponents \(mp+1\) and np gives

$$\begin{aligned} p=\frac{1}{n-m}. \end{aligned}$$
(59)

which is also obtained matching the exponents \(mp+2\) and \(\left( 2n-m\right) p.\) Equating coefficients of them and setting the coefficients of \(\left[ D+sn\left( \tau ,\ell \right) \right] ^{mp+j}\) to zero, for \(j=-2,-1,0\), in (58) yields

$$\begin{aligned} A= & {} \left[ \frac{a\ell D\left[ 3\left( n-m\right) -\left( n-m-4\right) \ell \right] }{b\left[ 1-\ell \left( n-m\right) +\ell D^{2}\left( n-m\right) \left( 1-2l\right) -3\ell \right] }\right] ^{\frac{1}{n-m}}, \nonumber \\ \end{aligned}$$
(60)
$$\begin{aligned} B= & {} \sqrt{\frac{a\left( n-m\right) ^{2}}{m\left[ 1-\ell \left( n-m\right) +\ell D^{2}\left( n-m\right) \left( 1-2l\right) -3\ell \right] \left( k^{2}-v^{2}\right) }} \nonumber \\ \end{aligned}$$
(61)

and

$$\begin{aligned} D=\sqrt{\frac{b^{2}\left( n-m+\ell \right) \left[ \ell \left( n-m\right) -1\right] }{b^{2}\ell \left( n-m+\ell \right) \left[ \left( n-m\right) \left( 1-2\ell \right) -3\ell \right] -ca\ell \left[ 3\left( n-m\right) -\left( n-m-4\right) \ell \right] ^{2}}} \end{aligned}$$
(62)

where radicands are positive. Thus, finally, the Jacobi elliptic function solution of (54) is given by

$$\begin{aligned} q(x,t)=A\left[ D+sn\left\{ B\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{n-m}} \end{aligned}$$
(63)

where the amplitude A,  the inverse width B and the constant D are, respectively, given by (60) ,(61) and (62) . From (63), if the modulus \(\ell \rightarrow 1,\) we get following topological soliton solution of (54).

$$\begin{aligned} q(x,t)=A^{*}\left[ D^{*}+\tanh B^{*}\left( x-vt\right) \right] ^{\frac{1}{n-m}} \end{aligned}$$
(64)

where

$$\begin{aligned} A^{*}= & {} \frac{2aD\left( n-m+2\right) }{b\left[ 1+m-n+D^{2}\left( m-n-3\right) \right] \left( k^{2}-v^{2}\right) } \nonumber \\\end{aligned}$$
(65)
$$\begin{aligned} B^{*}= & {} \sqrt{\frac{a\left( n-m\right) ^{2}}{m\left[ 1+m-n+D^{2}\left( m-n-3\right) \right] \left( k^{2}-v^{2}\right) }} \nonumber \\ \end{aligned}$$
(66)

and

$$\begin{aligned} D^{*}=\sqrt{\frac{b^{2}\left( n-m+1\right) \left( n-m-1\right) }{b^{2}\left( n-m+1\right) \left( m-n-3\right) -4ca\left( n-m+2\right) ^{2}}} \nonumber \\ \end{aligned}$$
(67)

Now, to get other solutions of (54), we use the assumption as

$$\begin{aligned} q\left( x,t\right) =A\left[ D+cn\left( \tau ,\ell \right) \right] ^{p}, \end{aligned}$$
(68)

Thus, one obtains

$$\begin{aligned} \left( q^{m}\right) _{tt} =&A^{m}B^{2}mpv^{2}\left[ \ell ^{2} -1+D^{2}\left( 2\ell ^{2}-1\right) \right. \nonumber \\&\left. \times \left( 1-p\right) +\ell ^{2}D^{4}\left( 1-p\right) +p\left( 1-\ell ^{2}\right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp-2}-A^{m}B^{2} mpv^{2}D\nonumber \\&\times \left[ 1+\ell +\ell ^{2}\right. +2\left( p-1\right) \left( 2\ell ^{2}-1\right) \nonumber \\&\left. + \ell D^{2}\left( 1+\ell \left( 5-4p\right) \right) \right] \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp-1}\nonumber \\&+A^{m}B^{2}mpv^{2}\times \left[ \ell D^{2}\left( -1+5\ell -6p\ell \right) \right. \nonumber \\&+\,\ell \left( 1-\ell \right) +\left. p\left( 2\ell ^{2}-1\right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp}\nonumber \\&+A^{m}B^{2}mpv^{2}\ell D\left[ 3+\ell \left( -1+4p\right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp+1}\nonumber \\&-A^{m}B^{2}mpv^{2}\ell \left( 1+p\ell \right) \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp+2} \end{aligned}$$
(69)
$$\begin{aligned} \left( q^{m}\right) _{xx} =\,&A^{m}B^{2}mp\left[ \ell ^{2}-1+D^{2}\left( 2\ell ^{2}-1\right) \left( 1-p\right) \right. \nonumber \\&\left. +\,\ell ^{2}D^{4}\left( 1-p\right) +p\left( 1-\ell ^{2}\right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp-2}-A^{m} B^{2}mpD\nonumber \\&\times \left[ 1+\ell +\ell ^{2}\right. +2\left( p-1\right) \left( 2\ell ^{2}-1\right) \nonumber \\&\left. +\, \ell D^{2}\left( 1+\ell \left( 5-4p\right) \right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp-1}+A^{m}B^{2}mp\nonumber \\&\times \left[ \ell D^{2}\left( -1+5\ell -6p\ell \right) +\ell \left( 1-\ell \right) \right. \nonumber \\&\left. +\,p\left( 2\ell ^{2}-1\right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp}\nonumber \\&+A^{m}B^{2}mp\ell D\left[ 3+\ell \left( -1+4p\right) \right] \nonumber \\&\times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp+1}\nonumber \\&-A^{m}B^{2}mp\ell \left( 1+p\ell \right) \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp+2} \end{aligned}$$
(70)

So, Eq. (54) reduces

$$\begin{aligned}&A^{m}B^{2}mp\left[ \ell ^{2}-1+D^{2}\left( 2\ell ^{2}-1\right) \left( 1-p\right) \right. \nonumber \\&\quad \left. +\,\ell ^{2}D^{4}\left( 1-p\right) +p\left( 1-\ell ^{2}\right) \right] \nonumber \\&\quad \times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp-2}-A^{m}B^{2}mpD\left[ 1+\ell +\ell ^{2}\right. \nonumber \\&\quad +\,2\left( p-1\right) \left( 2\ell ^{2}-1\right) \left. +\ell D^{2}\left( 1+\ell \left( 5-4p\right) \right) \right] \nonumber \\&\quad \times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp-1}\nonumber \\&\quad +\,A^{m}B^{2}mp\left[ \ell D^{2}\left( -1+5\ell -6p\ell \right) \right. \nonumber \\&\quad \left. +\ell \left( 1-\ell \right) +p\left( 2\ell ^{2}-1\right) \right] \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp}\nonumber \\&\quad +\,A^{m}B^{2}mp\ell D\left[ 3+\ell \left( -1+4p\right) \right] \nonumber \\&\quad \times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp+1}-A^{m}B^{2}mp\ell \left( 1+p\ell \right) \nonumber \\&\quad \times \left[ D+cn\left( \tau ,\ell \right) \right] ^{mp+2}aA^{m}\left[ D+cn\left( \tau ,\ell \right) \right] ^{mp}\nonumber \\&\quad -\,bA^{n}\left[ D+cn\left( \tau ,\ell \right) \right] ^{np}\nonumber \\&\quad +\,cA^{2n-m}\left[ D+cn\left( \tau ,\ell \right) \right] ^{\left( 2n-m\right) p}=0. \end{aligned}$$
(71)

Equating the exponents \(mp+1\) and np gives the same value of which is in (59). This value is also obtained equating the exponents \(mp+2\) and \(\left( 2n-m\right) p.\) Equating coefficients of them and setting the coefficients of \(\left[ D+cn\left( \mu \xi ,m\right) \right] ^{mp+j}\) \(\left( j=-2,-1,0\right) \) to zero gives

$$\begin{aligned} A= & {} \left[ \frac{a\ell D\left[ \ell \left( n-m-4\right) -3\left( n-m\right) \right] }{b\left[ \ell D^{2}\left\{ \left( n-m\right) \left( 5\ell -1\right) -6\ell \right\} +\ell \left( 1-\ell \right) \left( n-m\right) +2\ell ^{2}-1\right] }\right] ^{\frac{1}{n-m}}, \end{aligned}$$
(72)
$$\begin{aligned} B= & {} \sqrt{\frac{a\left( n-m\right) ^{2}}{m\left[ \ell D^{2}\left\{ \left( n-m\right) \left( 5\ell -1\right) -6\ell \right\} +\ell \left( 1-\ell \right) \left( n-m\right) +2\ell ^{2}-1\right] \left( k^{2} -v^{2}\right) }} \end{aligned}$$
(73)

and

$$\begin{aligned} D=\sqrt{\frac{b^{2}\ell \left( m-n-\ell \right) \left[ \ell \left( 1-\ell \right) \left( n-m\right) -2\ell ^{2}-1\right] }{b^{2}\ell \left( m-n-\ell \right) \left[ \left( n-m\right) \left( 5\ell -1\right) -6\ell \right] -ca\left[ \ell \left( n-m-4\right) -3\left( n-m\right) \right] ^{2}}} \end{aligned}$$
(74)

where radicands are positive. Thus, finally, we obtain

$$\begin{aligned} q(x,t)=A\left[ D+cn\left\{ B\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{n-m}} \end{aligned}$$
(75)

where the amplitude A,  the inverse width B and the constant D are, respectively, given by (72) ,(73) and (74). From (75), when the modulus \(\ell \rightarrow 1,\) we get following 1-soliton solution of (54).

$$\begin{aligned} q(x,t)=A^{*}\left[ D^{*}+\sec hB^{*}\left( x-vt\right) \right] ^{\frac{1}{n-m}} \end{aligned}$$
(76)

Here,

$$\begin{aligned} A^{*}= & {} \left[ \frac{2aD\left( m-n-2\right) }{b\left[ 2D^{2}\left( 2\left( n-m\right) -3\right) +1\right] \left( k^{2}-v^{2}\right) }\right] ^{\frac{1}{n-m}} \nonumber \\ \end{aligned}$$
(77)
$$\begin{aligned} B^{*}= & {} \sqrt{\frac{a\left( n-m\right) ^{2}}{m\left[ 2D^{2}\left( 2\left( n-m\right) -3\right) +1\right] \left( k^{2}-v^{2}\right) }} \nonumber \\ \end{aligned}$$
(78)

and

$$\begin{aligned} D^{*}=\sqrt{\frac{b^{2}\left( m-n-1\right) }{2b^{2}\left( m-n-1\right) \left( 2\left( n-m\right) -3\right) -ca\left( 2m-2n-4\right) ^{2}}} \end{aligned}$$

where radicants are positive.

7 Form V

The generalized form of the quadratic nonlinear KGE is given by

$$\begin{aligned} \left( q^{m}\right) _{tt}-k^{2}\left( q^{m}\right) _{xx}+aq^{m} -bq^{m-n}+cq^{n+m}=0.\nonumber \\ \end{aligned}$$
(79)

It is necessary to have \(n>0\) and \(n\ne 2,4\) for solitons to exist. In order to solve (79), we introduce the same assumption as in (9). Putting necessary derivatives into (79) and then setting the exponents and the coefficients \(sn^{mp-2}\left( \tau ,\ell \right) \) and \(sn^{\left( m-n\right) p}\left( \tau ,\ell \right) \) and also \(sn^{mp+2} \left( \tau ,\ell \right) \) and \(sn^{\left( m+n\right) p}\left( \tau ,\ell \right) \) equal to one another yields

$$\begin{aligned} p= & {} \frac{2}{n} \end{aligned}$$
(80)
$$\begin{aligned} A= & {} \left[ \frac{b\left[ 2m\left( 1+\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] }{a\left( 2m-n\right) }\right] ^{\frac{1}{n} } \end{aligned}$$
(81)

and

$$\begin{aligned} B=\sqrt{\frac{an^{2}}{2m\left[ 2m\left( 1+\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] \left( v^{2}-k^{2}\right) }}\nonumber \\ \end{aligned}$$
(82)

Furthermore, the constraint relation between the nonlinear coefficients a, b, c and the exponents m and n given by

$$\begin{aligned}&a^{2}\ell \left( 2m-n\right) \left( n+2m\ell \right) \nonumber \\&\quad =n^{2}cb\left[ 2m\left( 1+\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] ^{2} \end{aligned}$$
(83)

From (82), it is important to note that

$$\begin{aligned} a\left[ 2m\left( 1+\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] \left( v^{2}-k^{2}\right) >0 \end{aligned}$$

and from (81) the radicant is positive, if n is even. So Jacobi elliptic function solution of (79) is given by

$$\begin{aligned} q(x,t)&=\left[ \frac{b\left[ 2m\left( 1+\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] }{a\left( 2m-n\right) }\right. \nonumber \\&\quad \left. \times sn^{2}\left\{ \sqrt{\frac{an^{2}}{2m\left[ 2m\left( 1+\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] \left( v^{2} -k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{n} } \end{aligned}$$
(84)

If the modulus \(\ell \rightarrow 1\) in Eq. (84), we get following topological soliton solution

$$\begin{aligned} q(x,t)= & {} \left[ \frac{4mb}{a\left( 2m-n\right) }\tanh ^{2}\right. \nonumber \\&\left. \times \left\{ \sqrt{\frac{an^{2}}{8m^{2}\left( v^{2}-k^{2}\right) }}\left( x-vt\right) \right\} \right] ^{\frac{1}{n}} \end{aligned}$$
(85)

where \(a\left( v^{2}-k^{2}\right) >0\) and if n is even \(ab\left( 2m-n\right) >0.\)

Now, to get other solutions of (79), we use the hypothesis given by (20). Doing similar operations, one gets the same value of p which is in (80) and also yields

$$\begin{aligned} A=\left[ \frac{b\left[ 2m\left( 1-2\ell ^{2}\right) +n\ell \left( \ell -1\right) \right] }{a\left( 2m-n\right) \left( \ell ^{2}-1\right) }\right] ^{\frac{1}{n}} \end{aligned}$$
(86)

and

$$\begin{aligned} B=\sqrt{\frac{an^{2}}{2m\left[ 2m\left( 1-2\ell ^{2}\right) +n\ell \left( \ell -1\right) \right] \left( v^{2}-k^{2}\right) }}\nonumber \\ \end{aligned}$$
(87)

In addition, it is obtained the following constraint relation

$$\begin{aligned}&a^{2}\left( 2m-n\right) \left( n+2m\ell \right) \left( \ell ^{2}-1\right) \nonumber \\&\quad =cb\left[ 2m\left( 1-\ell ^{2}\right) +n\ell \left( 1-\ell \right) \right] ^{2} \end{aligned}$$
(88)

From (87), it is important to note that

$$\begin{aligned} a\left[ 2m\left( 1-2\ell ^{2}\right) +n\ell \left( \ell -1\right) \right] \left( v^{2}-k^{2}\right) >0 \end{aligned}$$

and from (86) the radicant is positive, if n is even. So, we have

$$\begin{aligned} q(x,t)&=\left[ \frac{b\left[ 2m\left( 1-2\ell ^{2}\right) +n\ell \left( \ell -1\right) \right] }{a\left( 2m-n\right) \left( \ell ^{2}-1\right) }\right. \nonumber \\&\quad \left. \times cn^{2}\left\{ \sqrt{\frac{an^{2}}{2m\left[ 2m\left( 1-2\ell ^{2}\right) +n\ell \left( \ell -1\right) \right] \left( v^{2} -k^{2}\right) }}\left( x-vt\right) ,\ell \right\} \right] ^{\frac{1}{n} } \end{aligned}$$
(89)

If the modulus \(\ell \rightarrow 0\) in Eq. (89), we get 1-soliton solution as

$$\begin{aligned} q(x,t)= & {} \left[ \frac{2mb}{a\left( n-2m\right) }\cos ^{2}\right. \nonumber \\&\left. \times \left\{ \sqrt{\frac{an^{2}}{4m^{2}\left( v^{2}-k^{2}\right) }}\left( x-vt\right) \right\} \right] ^{\frac{1}{n}} \end{aligned}$$
(90)

where \(a\left( v^{2}-k^{2}\right) >0\) and if n is even \(ab\left( n-2m\right) >0\).

8 Conclusion

This paper considers the gKGE with five different forms of nonlinearity. Jacobi elliptic functions are used to get soliton solutions of each of these cases. The necessary constraint conditions are presented for the existence of solitons. We use sn and cn Jacobi elliptic functions to obtain soliton solutions of this equations. It needs to be noted that by using the rest of Jacobi elliptic functions, and it is possible to obtain other travelling wave solutions of the gKGE. Furthermore, other nonlinear evolution equations in the literature can be solved by using this technique.