Semiclassical Magnetization Dynamics and Electron Paramagnetic Resonance in Presence of Magnetic Fluctuations in Strongly Correlated Systems

Abstract

Semiclassical magnetization dynamics in presence of magnetic fluctuations (including the quantum ones) is derived for a strongly correlated electronic system in the region of the linear magnetic response. Landau–Lifshitz (LL) and Gilbert (G) type equations are obtained with the effective parameters depending on the type of magnetic fluctuations and their magnitude and applied to evaluation of electron paramagnetic resonance (EPR) problem in Faraday geometry. It is shown that in the studied systems LL and G equations may not be equivalent except the case of weak relaxation, where consistent Landau–Lifshitz–Gilbert (CLLG) equation may be considered. Whereas G equation is affected by quantum fluctuations solely, the LL and CLLG equations may be renormalized by magnetic fluctuations of any nature. In contrast to G equation, the LL and CLLG magnetization dynamics may be characterized by the anisotropic relaxation term caused by anisotropic magnetic fluctuations. A consequence of anisotropic relaxation is the unusual polarization effect consisting in strong dependence of the EPR line magnitude on orientation of vector h of the oscillating magnetic field with respect to the crystal structure, so that EPR may be suppressed for some directions of h. In the case of dominating quantum fluctuations, the LL and CLLG equations may lead to a universal relation between fluctuation induced contributions to the EPR line width ΔW and g-factor Δg in the form $\Delta W/\Delta g = a_{0} k_{B} T/\mu_{B}$, where a₀ is a numerical coefficient of the order of unity and independent of the quantum fluctuation magnitude. The applicability of the proposed semiclassical magnetization dynamics models to the EPR in spin nematic phases and detection by EPR method of a new group of magnetic phenomena – spin fluctuation transitions is discussed.

1 Introduction

In recent years, considerable progress was achieved in application of electron paramagnetic resonance (EPR) for studying various systems with strong electronic correlations. Accurate theoretical consideration of dynamical magnetic susceptibility in magnetic field was provided in several important cases, namely for heavy fermion and Kondo systems [1,2,3,4,5,6], including those with the hidden order [3,4,5,6], and an antiferromagnetic (AF) S = 1/2 one dimensional (1D) quantum spin chains [7, 8]. The analysis of the experimental situation shows that the EPR may be successfully treated in the framework of the semiclassical equation for the magnetization motion, including reasonably good description of the EPR line shape in metallic strongly correlated electron systems (SCES) [9], although they are essentially quantum materials. This, at least in part, may be explained by the fact that Landau–Lifshitz equation of motion, in spite of its semiclassical nature, it may be used for accounting the quantum two-level system of non-interacting spins in magnetic field H described by the Hamiltonian ${\hat{\text{H}}} = - \frac{\hbar }{2}({\upsigma },{\upomega }_{H} ) \cdot (1 - i\lambda )$, where σ is Pauli vector operator, ħω_H corresponds to the levels spacing in Zeeman splitting and λ denotes relaxation parameter responsible for the levels broadening [10]. Interesting, that even in the most model case like quasi 1D AF S = 1/2 quantum spin chains experiment shows appearance of the EPR modes with unusual polarization characteristics, which are not foreseen in theoretical treatment [7, 8] but may be quantitatively explained by application of semiclassical spin dynamics with the anisotropic g-tensor and relaxation time [12].

Apparently, consistent and rigorous consideration of the EPR in SCES will remain rare due to complexity of the systems with concentrated and strongly interacting magnetic system. Thus, the semiclassical approach may have an advantage, because it merely describes the magnetization rotation around a magnetic field and does not require exact accounting of the spins and itinerant electrons (if any) interactions, which form the magnetic state and control temperature and field dependence of the magnetization M(H,T) in SCES. However, the realistic use of semiclassical spin dynamics in the considered case requires accounting of the magnetic fluctuations, characteristic for various SCES and affecting the EPR physical picture [1, 2].

There are numerous works, where stochastic terms are added to semiclassical equation of magnetization motion. In most cases, fluctuations are supposed to originate from random magnetic field [13,14,15,16]. For spintronic applications, the equation of motion may contain a torque related to a spin current with a stochastic component [14]. A separate problem is accounting of quantum fluctuations, which may be considered by adding random torques of special form [17]. It should be noted that according to [17] quantum fluctuation effects may be observed in the diapason $T < T^{*} \sim \hbar \omega_{H} /k_{B}$, which may imply high-frequency EPR experiments. Indeed, for $\omega_{H} /2\pi \sim 100$ GHz characteristic temperature is T ^* ~ 4.5 K and therefore liquid helium temperatures are sufficient to examine influence of quantum fluctuations.

A typical way of accounting semiclassical spin dynamics with random variables consists in transition from an equation describing magnetization dynamics to some Fokker–Planck type equation for the probability of the direction of magnetization [13,14,15,16,17]. This idea is apparent in theoretical sense but may not be directly applicable to calculation of EPR in SCES for several reasons. At first, this approach assumes that the magnetization of the described object is saturated. This is not the case in almost any experiment, where EPR is studied, as long as in particular SCES the oscillating magnetization may not be saturated for particular magnetic field and temperature [9]. Secondly, saturated magnetization rules out possible fluctuations of the magnetization, which will certainly results in depleting of the physical picture of EPR in SCES. Thirdly, it is not clear how derived Fokker–Planck equation should be used to compute EPR, and actually this calculation was never carried out within this ansatz [13,14,15,16,17].

An alternative way of accounting magnetic fluctuations in the semiclassical spin dynamics was suggested in [18]. This approach is based on the fact that semiclassical equation of the magnetization motion, taken “as is”, may be successfully used for quantitative description of the EPR line shape and finding full set of spectroscopic parameters including g-factor (hyromagnetic ratio), line width (relaxation parameter) and oscillating magnetization at the resonance field for the temperature at which experiment is conducted [9]. Therefore, if EPR in some SCES is detected, it means that applied oscillating magnetic field causes precession of the magnetization around the steady magnetic field. However, in SCES, semiclassical spin dynamics may be disturbed by magnetic fluctuations superimposed on regular motion. In this situation, it is assumed that the equation for the regular motion may be obtained by averaging of the equation of the magnetization motion with respect to magnetic fluctuations. In this case, the modified equation will contain effective parameters following from the averaging procedure, which will depend on magnetic fluctuation characteristics [18]. Physically, this means that fluctuations are treated as a fast process with respect to the timescale of regular spin precession 2π/ω_H. As it is shown in [18] this approach may be applied for accounting of quantum fluctuations. The application of Heisenberg uncertainty principle suggests that in this case it is sufficient to take into account quantum fluctuations of the magnetization along the external magnetic field and fluctuations of spin precession frequency [18].

In the present work, we are aimed at analysis of the general case, when magnetization and magnetic field appearing in the equation of motion may fluctuate in any direction in space and may be of either of quantum or non-quantum nature. The paper is organized as follows. It turns out that the specification of exact form of the relaxation term is of crucial importance for the studied problem. For that reason, we are starting with the problem of equivalence of the Landau–Lifshitz and Gilbert equations. Our analysis shows that these equations in fact appear essentially different, but in the case of weak relaxation it is possible to suggest a new consistent equation possessing Landau–Lifshitz form. This consideration is followed by short description of possible way to implement quantum fluctuations in semiclassical magnetization dynamics. After that, the results of averaging of various equations of motion together with the EPR computations are provided. We show that accounting of magnetic fluctuations may lead to EPR problem with an anisotropic relaxation. Physical consequences of the proposed model including unusual polarization effect and universal relations linking g-factor and the line width are discussed. In the concluding part, we consider several experimental results relevant to suggested approach for EPR description in strongly correlated electron systems.

2 Landau–Lifshitz and Gilbert Equations

There are two semiclassical equations describing magnetization vector M dynamics in external magnetic field H, namely Landau–Lifshitz (LL) and Gilbert (G) equations. In the LL equation

$$\frac{{d{\mathbf{M}}}}{dt} = \gamma_{LL} [{\mathbf{M}},{\mathbf{H}}] + {\mathbf{R}}_{{{\text{LL}}}}$$

(1)

the relaxation torque R_LL is given by the double vector product

$${\mathbf{R}}_{{{\text{LL}}}} = - \lambda \cdot [{\mathbf{M}},[{\mathbf{M}},{\mathbf{H}}]].$$

(2)

Here γ_LL and λ denote hyromagnetic ratio and relaxation parameter respectively. The G equation was purposed as an alternative to LL equation and has the form [18]

$$\frac{{d{\mathbf{M}}}}{dt} = \gamma_{G} \left[ {{\mathbf{M}},{\mathbf{H}} - \eta \frac{{d{\mathbf{M}}}}{dt}} \right] = \gamma_{G} [{\mathbf{M}},{\mathbf{H}}] - \gamma_{G} \eta \cdot \left[ {{\mathbf{M}},\frac{{d{\mathbf{M}}}}{dt}} \right],$$

(3)

where γ_G is the hyromagnetic ratio, and the relaxation torque

$${\mathbf{R}}_{{\text{G}}} = - \gamma_{G} \eta \cdot [{\mathbf{M}},\frac{{d{\mathbf{M}}}}{dt}]$$

(4)

is written in an analogy with the mechanical torque $[{\mathbf{r}},{\mathbf{F}}_{f} ] = - \frac{1}{\tau }[{\mathbf{r}},\frac{{d{\mathbf{r}}}}{dt}]$ for the viscous friction force ${\mathbf{F}}_{f} = - \frac{{\mathbf{v}}}{\tau } = - \frac{1}{\tau }\frac{{d{\mathbf{r}}}}{dt}$ depending on the relaxation time τ. However, on the contrary to mechanics, the relaxation parameter η in Gilbert Eqs. (3)–(4) has dimension of time rather than inverse time. Both Landau–Lifshitz and Gilbert equations keep the length of the magnetization vector $M = \left| {\mathbf{M}} \right|$.

Equations (1)–(2) and (3)–(4) are claimed to be equivalent [10, 19] and often referred to as Landau-Lifshits–Gilbert equation. Nevertheless, there is a fundamental difference between LL and G equation, as it was realized long ago [20]. The comparison of the relaxation torques R_LL and R_G is shown in Fig. 1, panels a–b. In spherical coordinates, R_LL depends on the polar angle θ between vectors M and H (Fig. 1a) whereas R_G depends on both polar θ and azimuthal φ angles (Fig. 1b). As long as directions of R_LL and R_G may not coincide (Fig. 1b), magnetization dynamics described by LL and G equations should be different in general case.

Fig. 1

a Landau–Lifshitz type of relaxation; b Gilbert type of relaxation; c Faraday geometry for excitation of EPR; d EPR in Faraday geometry in presence of magnetic fluctuations

The thesis of equivalence of LL and G equations is based on simple formal transformation. When the derivative dM/dt from Eq. (1) is substituted into Eq. (3), the Gilbert equation may be rewritten as

$$\frac{{d{\mathbf{M}}}}{dt} = \gamma_{G} (1 - \lambda \eta M^{2} )[{\mathbf{M}},{\mathbf{H}}] - \gamma_{G} \gamma_{LL} \eta \cdot [{\mathbf{M}},[{\mathbf{M}},{\mathbf{H}}]].$$

(5)

Thus, depending on which equation is considered primary, the LL holds if

$$\gamma_{LL} = \frac{{\gamma_{G} }}{{1 + (\eta \gamma_{G} M)^{2} }},$$

(6)

$$\lambda = \frac{{\eta \gamma_{G}^{2} }}{{1 + (\eta \gamma_{G} M)^{2} }},$$

(7)

and, respectively, G equation is valid for the values

$$\gamma_{G} = \gamma_{LL} + \frac{{\lambda^{2} M^{2} }}{\gamma },$$

(8)

$$\eta = \frac{\lambda }{{\gamma_{LL}^{2} + \lambda^{2} M^{2} }}.$$

(9)

When the magnetization is saturated, M = const, Eqs. (6)–(9) merely represents the links between constants in LL and G equations, and from the formal mathematical point of view it is possible to consider both descriptions of spin dynamics as equivalent [10, 19]. However, the parameters in Eqs. (1)–(2) and (3)–(4) have physical meaning and formal manipulation with them poses certain questions. Indeed, in the LL and G equation, the parameters γ_LL and γ_G describe rotation of the magnetization and the parameters λ and η are responsible for relaxation. Rotation and relaxation are different physical processes and, consequently, it is difficult to imagine a situation where the aforementioned parameters may be mixed as suggested by Eqs. (6)–(9). In addition, for a quantum mechanical system, which spin dynamics we are aimed to describing on semiclassical level, the spacing between energy levels is $\hbar \omega_{H}$, where $\omega_{H} = \gamma H$ depends on the hyromagnetic ratio γ. Therefore, the hyromagnetic ratio is a fundamental constant for the considered problem. Thus, it is logical to have the same parameters γ_LL and γ_G in LL and G equations, so that $\gamma_{LL} = \gamma_{G} = \gamma$. However, the equality $\gamma_{LL} = \gamma_{G}$ may be valid only in the case when relaxation process is missing [Eqs. (6)–(9)]. In any case, the effective character of either γ_G or γ_LL should follow from some strong arguments.

We see that even for saturated magnetization M = const the requirement of LL and G equations formal equivalence appears as a supposition, which may look controversial in physical sense. The situation of linear response $M(H,T) = \chi_{0} (T) \cdot H$ is considered in the present work. Thus, if LL and G equations are assumed as equivalent, the relations between the hyromagnetic ratios γ_LL and γ_G as well as between relaxation parameters λ and η become dependent on temperature and magnetic field [Eqs. (6)–(9)]. This is hardly possible for any general reasons and may be kept as an ad hoc assumption. Therefore, the standard formal transformation of the LL and G equations, which is typically used for establishing their equivalence [10, 19], is at least insufficient for making this kind of conclusion in the studied case.

However, the above consideration does not rule out the possibility of equivalence but rather shows that the approach [10, 19] is unsatisfactory. There is another way to investigate possible equivalence of LL and G equations, when they are considered as semiclassical images of the same quantum mechanical system. Consequently, it is necessary to calculate some physical property (in our case EPR) using both types of semiclassical magnetization dynamics and compare results.

In calculation of the EPR problem, we assume Faraday geometry (Fig. 1c). The magnetization vector M(t) and external magnetic field H(t) are given by

$${\mathbf{M}}(t) = {\mathbf{M}}_{0} + {\mathbf{m}}(t),$$

(10)

$${\mathbf{H}}(t) = {\mathbf{H}}_{0} + {\mathbf{h}}(t).$$

(11)

Equilibrium static magnetization M₀ and steady magnetic field H₀ are aligned along the z-axis. Forced magnetic oscillations occur in the x–y plane, where vectors ${\mathbf{m}}(t) = {\mathbf{i}} \cdot m_{x} (t) + {\mathbf{j}} \cdot m_{y} (t)$ and ${\mathbf{h}}(t) = {\mathbf{i}} \cdot h_{x} (t) + {\mathbf{j}} \cdot h_{y} (t)$ are located. Hereafter we assume that excitation magnetic field h(t) is linearly polarized that, for example, corresponds to the case of EPR in cylindrical cavity operating at TE₀₁₁ mode [9]. Static magnetization M₀ and steady magnetic field H₀ are linked via static magnetic susceptibility

$${\mathbf{M}}_{0} = \chi_{0} {\mathbf{H}}_{0} ,$$

(12)

and, as usual [21], the relations $m(t) < < M_{0}$ and $h(t) < < H_{0}$ hold, so that the problem is treated in the linear in m approximation. For harmonical excitation at frequency ω the excitation field and magnetic response may be taken in the form: ${\mathbf{h}}(t)\sim \exp ( - i\omega t)$, ${\mathbf{m}}(t)\sim \exp ( - i\omega t)$. Then the LL equation may be written as

$$\left( {\begin{array}{*{20}c} { - i\omega + \nu } & { - \omega_{H} } \\ {\omega_{H} } & { - i\omega + \nu } \\ \end{array} } \right) \cdot \left( {\begin{array}{*{20}c} {m_{x} } \\ {m_{y} } \\ \end{array} } \right) = \chi_{0} \omega_{H} \left( {\begin{array}{*{20}c} { - h_{y} } \\ {h_{x} } \\ \end{array} } \right),$$

(13)

where $\omega_{H} = \gamma_{LL} H_{0}$. The relaxation frequency ν in Eq. (13) depends on magnetic field

$$\nu = \chi_{0} \omega_{H}^{2} /\nu_{0} .$$

(14)

Here the characteristic frequency $\nu_{0} = \gamma^{2} /\lambda$ is introduced. When the system Eq. (13) is solved, it is possible finding the radiation power absorbed in EPR: $P = {\text{Im}} \{ {\mathbf{m}} \cdot {\mathbf{h}}^{*} \}$ [21]. The result may be presented as a function of the dimensionless variable $x = \omega /\omega_{H}$ and relaxation parameter $\alpha_{LL} = \chi_{0} \omega /\nu_{0}$

$$P_{LL} (x) = \chi_{0} h_{0}^{2} \frac{{2\alpha_{LL} }}{{\left( {1 - x^{2} + \frac{{\alpha_{LL}^{2} }}{{x^{2} }}} \right)^{2} + 4\alpha_{LL}^{2} }}.$$

(15)

The analogue of the system Eq. (13), which follows from the G equation, is essentially different

$$\left( {\begin{array}{*{20}c} { - i\omega } & { - \omega_{H} (1 - i\alpha_{G} )} \\ {\omega_{H} (1 - i\alpha_{G} )} & { - i\omega } \\ \end{array} } \right) \cdot \left( {\begin{array}{*{20}c} {m_{x} } \\ {m_{y} } \\ \end{array} } \right) = \chi_{0} \omega_{H} \left( {\begin{array}{*{20}c} { - h_{y} } \\ {h_{x} } \\ \end{array} } \right),$$

(16)

as long as relaxation described by $\alpha_{G} = \chi_{0} \omega \eta$ is added not to the diagonal elements, but to the off-diagonal elements. In Eq. (16) the frequency ω_H equals $\omega_{H} = \gamma_{G} H$. Thus, in Landau–Lifshitz equation, the relaxation is accounted by introducing complex frequency of radiation, whereas the complex magnetization rotation frequency is characteristic to Gilbert variant of the magnetization dynamics. The solution of the system (16) gives

$$P_{G} (x) = \chi_{0} h_{0}^{2} \frac{{\alpha_{G} + \alpha_{G}^{3} + \alpha_{G} x^{2} }}{{(x^{2} - 1 + \alpha_{G}^{2} )^{2} + 4\alpha_{G}^{2} }},$$

(17)

where $x = \omega /\omega_{H}$. In Eqs. (15) and (17) h₀ is the magnitude of the oscillating magnetic field: $h_{0}^{2} = h_{x}^{2} + h_{y}^{2} .$

In the limit of weak relaxation $\alpha_{LL} ,\alpha_{G} < < 1$, the Eqs. (15) and (17) may be written in the same Lorentz form

$$P_{L} (x) = \frac{1}{2\alpha } \cdot \frac{{\chi_{0} h_{0}^{2} }}{{\left( {\frac{1 - x}{\alpha }} \right)^{2} + 1}},$$

(18)

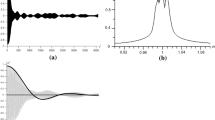
with α = α_LL or α = α_G. Therefore, if $\alpha_{LL} = \alpha_{G}$ and $\gamma_{LL} = \gamma_{G} = \gamma$ Landau–Lifshitz and Gilbert equations may be considered as giving the same description of EPR. The direct matching of Eqs. (15), (17), (18) is presented in Fig. 1 for $\alpha = \alpha_{LL} = \alpha_{G} = 0.1$. It is visible that Landau–Lifshitz and Gilbert variants of semiclassical magnetization dynamics provide almost the same EPR line shape of nearly Lorentz type, with the weak difference at the wings of the $P(x)$ function.

This coincidence breaks when the relaxation parameter α is increased. For α = 0.5 the EPR line shape is essentially different in the LL and G cases and there is no sense to use Lorentz approximation at all (see inset in Fig. 2). The discrepancy between two types of semiclassical magnetization dynamics may be easily seen from comparison of the EPR absorption maximum position x_max dependence on the relaxation parameter α. Equation (15) suggests that in the LL case $x_{\max LL} = \sqrt {\frac{1}{2} + \sqrt {\frac{1}{4} + \alpha_{LL}^{2} } }$ and for weak relaxation $x_{\max LL} \approx 1 + \alpha_{LL}^{2} /2.$ At the same time, the EPR line obtained for Gilbert type semiclassical spin dynamics has maximum at $x_{\max G} = \sqrt {2\sqrt {1 + \alpha_{G}^{2} } - (1 + \alpha_{G}^{2} )}$. The expansion of the latter expression at low $\alpha_{G}$ starts from $\alpha_{G}^{4}$ rather than $\alpha_{G}^{2}$ and gives $x_{\max G} \approx 1 - \alpha_{G}^{4} /8$. It is apparent that in both LL and G cases the enhancement of relaxation parameter causes the increase of the EPR line width $W\sim \alpha_{LL} ,\alpha_{G}$, but the corresponding shift of the resonance position is different: the $x_{\max LL}$ increases with $\alpha_{LL}$, whereas the $x_{\max G}$ decreases with $\alpha_{G}$. Moreover, the position of the resonance maximum for the Gilbert-type magnetization dynamics reaches zero $x_{\max G} (\alpha_{G} ) = 0$ at $\alpha_{G} = \sqrt 3$ and therefore resonance EPR absorption may be completely destroyed by damping in contrast to LL case.

Fig. 2

Comparison of the solutions for EPR provided by Landau–Lifshitz (LL) and Gilbert (G) equations for two damping parameters α = 0.1 (main panel) and α = 0.5 (inset). The approximation of the EPR line by Lorentz function (L) is also shown

The above consideration is illustrated by Figs. 3 and 4, where the evolution of the EPR line shape in the interval $\alpha_{LL} ,\alpha_{G} \le 1.5$ for the Landau–Lifshitz and Gilbert equations is shown in Figs. 3, 4 respectively. The plot of the $P(x,\alpha )$ function normalized on maximal absorption value $P_{\max } (\alpha )$ suggests that in the LL case, the enhancement of relaxation causes the resonance shift and broadening, while the resonant form of $P(x)$ is kept (Fig. 3). However, in the G case, the main effect is the EPR line broadening rather than shift of the resonance position. Additionally, the effect of the α_G increase is more pronounced and for α_G the $P(x)$ function given by Eq. (17) transforms into a broad non-resonant feature (Fig. 4). Therefore, the evolution with the relaxation parameter of the EPR line shape modeled by LL or G equations is indeed qualitatively different and, consequently, it is not possible to treat these descriptions of the semiclassical magnetization dynamics as equivalent, except the limit of weak relaxation. The EPR lines are broad in strongly correlated materials [9], i.e. the relation $\alpha_{LL} ,\alpha_{G} < < 1$ corresponding to a narrow resonance may not be applicable. Thus, it is possible to expect that for the EPR in SCES, Landau-Lishitz and Gilbert equations become different models, serving semicalassical images of different quantum systems providing an alternative description of the EPR line shape and resonance shift. As long as g-factor is proportional to the ratio $\omega /H$, the Landau–Lifshitz type systems corresponds to the simultaneous increase of the g-factor and EPR line width W, whereas in the Gilbert type systems the enhancement of W is accompanied by decrease of g-factor. Therefore, the most adequate model may be obtained by comparison with experiment. Below we will show that the discrepancy between LL and G equation is even deeper with respect to accounting of effect of magnetic fluctuations.

Fig. 3

Evolution of the EPR line shape by relaxation parameter for Landau–Lifshitz equation of magnetization motion (3D plot and contour map). The EPR line is normalized by the maximal absorption amplitude

Fig. 4

Evolution of the EPR line shape by relaxation parameter for Gilbert equation of magnetization motion (3D plot and contour map). The EPR line is normalized by the maximal absorption amplitude

The above consideration allows suggesting an additional way for description of semiclassical magnetization dynamics in the case $\alpha < < 1$. In the absence of relaxation, Eqs. (1)–(2) and (3)–(4) are equivalent if the equalities $\gamma_{LL} = \gamma_{G} = \gamma$ hold. The adding of the relaxation may be done in an iteration manner by substituting dM/dt in the right part of Eq. (3) by $\gamma [{\mathbf{M}},{\mathbf{H}}]$, which lead to LL form

$$\frac{{d{\mathbf{M}}}}{dt} = \gamma [{\mathbf{M}},{\mathbf{H}}] - \gamma^{2} \eta \cdot [{\mathbf{M}},[{\mathbf{M}},{\mathbf{H}}]]$$

(19)

with the relaxation parameter $\lambda = \gamma^{2} \eta$ and $\gamma_{LL} = \gamma$. Formally Eq. (19) corresponds to $\alpha_{LL} = \alpha_{G} = \chi_{0} \omega \eta$ and is valid if $\alpha_{LL}^{2} /2 < < 1$. Practically, the consistency between LL and G approaches may be kept for $\alpha_{LL} ,\alpha_{G} \le 0.1$ (Fig. 2), and in this limit the “viscous friction type” relaxation introduced by Gilbert is kept.

Below we will consider Eq. (19) as a specific type of Landau–Lifshitz one with the special λ value without direct linking to the case $\alpha < < 1$ and precise association with the Gilbert type of relaxation. It will be shown in the next section that the choice of the relaxation parameter dependence on the hyromagnetic ratio γ is important when quantum fluctuations are included in a semiclassical description. Following its origin, the Eq. (19) is referred below as consistent Landau–Lifshitz–Gilbert equation (CLLG) and will be analyzed together with the Landau–Lifshitz and Gilbert equations.

3 The Accounting of Quantum Fluctuations

Following the idea proposed in [18] we suppose that Heisenberg uncertainty relations can be used to describe quantum fluctuations. Since magnetization dynamics of a semiclassical system is considered, it is necessary to consider Heisenberg uncertainty principle in a semiclassical limit [22,23,24]. In this case, the Heisenberg inequalities must correspond to the minimum value of the product of uncertainties and therefore degenerate into equalities [22,23,24].

In the considered semi-classical case, the uncertainty relation for the physical quantity $\phi$ has the form [23]

$$\Delta E \cdot \Delta \varphi = \hbar \frac{\partial \varphi }{{\partial t}},$$

(20)

where ΔE is the uncertainty of the energy of the system E. Taking φ as the angle of rotation around the magnetic field direction, we get

$$\Delta E \cdot \Delta \varphi = \hbar \omega_{H} .$$

(21)

Uncertainty of the angle Δφ is connected with the uncertainty of related mechanical moment L [23]

$$\Delta L \cdot \Delta \varphi = \frac{\hbar }{2},$$

(22)

where the system is assumed to rotate around the z-axis and ΔL stands for the uncertainty of the z-component of the mechanical moment. Multiplication of both sides of expression (22) by $e/2mc$ links uncertainties of magnetic moment M_z and angle φ,

$$\Delta M_{z} \cdot \Delta \varphi = \frac{{\mu_{B} }}{2}.$$

(23)

Here e and m are charge and mass of electron, c is light velocity. After excluding Δφ from Eqs. (21) and (23) and using expression ΔE = ħΔω_H it is possible to obtain a relation linking Δω_H and ΔM_z

$$\frac{{\Delta \omega_{H} }}{{\omega_{H} }} = \frac{{2\Delta M_{z} }}{{\mu_{B} }}.$$

(24)

In above consideration, the magnetic moment and its fluctuations are considered per magnetic ion. Thus, in the case of quantum fluctuations, the uncertainties of the rotation frequency and the magnetic moment turn out to be proportional to each other and quantum fluctuations of the magnetization ΔM_z and the frequency Δω_H will be interconnected, as a result of which the average $< \Delta \omega_{H} \Delta M_{z} >$ will be different from zero [18]. The quantum nature of the Eqs. (20)–(24) suggests that the uncertainty Δω_H will be valid even in the case H = const. As long as $\omega_{H} = \gamma H$, frequency fluctuations will be taken into account by replacing γ → γ + Δγ in a semiclassical equation of motion, where Δγ corresponds to fluctuations of the gyromagnetic ratio, which have a quantum nature. It follows from the above analysis that in the considered EPR geometry, the correlator < ΔγΔM >  =  < ΔγΔM_z > k may represent quantum fluctuation effects in the averaged equation of motion [18]. Apparently, the correlator < ΔγΔM_z > is proportional to the mean square of quantum fluctuations of magnetization < ΔM_z² > , so that $< \Delta \gamma \Delta M_{z} > = 2\gamma < \Delta M_{z}^{2} > /\mu_{B}$ [18].

4 Averaged Equations for the Magnetization Motion

4.1 The Model

Now fluctuations of the magnetization ΔM and magnetic field ΔH should be introduced and therefore the Eqs. (10)–(11) are replaced by

$${\mathbf{M}} = \text{M}_{0} {\mathbf{k}} + \Delta {\mathbf{M}} + {\mathbf{m}}(t),$$

(25)

$${\mathbf{H}} = H_{0} {\mathbf{k}} + \Delta {\mathbf{H}} + {\mathbf{h}}(t).$$

(26)

In calculation of CW EPR we will keep in mind Faraday geometry as above. It is supposed that fluctuations represent fast motion with respect to magnetization rotation. For that reason, it is possible to perform averaging first and then apply time differentiation operation to m(t) representing relatively slow magnetization motion. This physical picture is shown in Fig. 1d schematically.

Following [18], the averaging procedure is performed under assumption < ΔM >  = 0, < ΔH >  = 0 and postulated independence of the spatial directions. Therefore, the correlators $< \Delta P_{i} \Delta Q_{j} >$ take the form: $< \Delta P_{i} \Delta Q_{j} > \sim \delta_{ij}$, where $\Delta P_{i} = \Delta M_{i} ,\Delta H_{i} ,d\Delta M_{i} /dt$, $\Delta Q_{j} = \Delta M_{j} ,\Delta H_{j} ,d\Delta M_{j} /dt$ and $\delta_{ij}$ is Kronecker symbol. To simplify the problem, the correlators $< \Delta P_{i}^{p} \Delta Q_{j}^{q} >$ for p, q, > 1 and $< \Delta P_{i}^{p} \Delta Q_{j}^{q} \Delta L_{k}^{l} >$ for p,q,l ≥ 1 are taken zero, which means that further calculation is limited by square corrections. Hereafter, we assume that any non-zero correlator is an independent on time parameter of the model. In order to present the results in more compact form, the linear relations between M₀, ΔM and H₀, ΔH are supposed

$$M_{0} = \chi_{0} H_{0} ,$$

(27)

$$\Delta {\mathbf{M}} = \chi_{f} \Delta {\mathbf{H}}{.}$$

(28)

Here χ₀ is static susceptibility as above and χ_f is some effective parameter which is discussed later.

In the general case, the fluctuation of magnetization ΔM may include quantum fluctuation. Quantum effects are taken into account by adding fluctuations of hyromagnetic ratio Δγ as described in Sect. 3. Thus, the average and following correlators < Δγ > ,$< \Delta \gamma \Delta M_{x} >$,$< \Delta \gamma \Delta M_{y} >$ are equal zero, whereas the correlator $< \Delta \gamma \Delta M_{z} > \ne 0$ is finite if $\Delta M_{z}$ originates from quantum fluctuations [18]. In the present work, we additionally assume $< \Delta \gamma \Delta H_{z} > \ne 0$ if $\chi_{s} \ne 0$ and keep square corrections only.

As in usual accounting of magnetic resonance [21], the case close to equilibrium $\left| {{\mathbf{M}} - M_{0} {\mathbf{k}}} \right| < < M_{0}$ and the case of weak excitation h(t) <  < H₀ are considered. Therefore, further calculations are done in linear in m approximation and the relaxation term is considered as independent of the oscillating magnetic field h. The averaging of the Eq. (1) gives a solution for averaged oscillating magnetization m(t), and the absorbed power related to periodic motion in EPR experiment P may be estimated as $P\sim \frac{{\omega_{H} }}{2\pi }\int\limits_{0}^{{2\pi /\omega_{H} }} {{\mathbf{m}}(t){\mathbf{h}}(t)dt}$, where ω_H is the magnetization rotation frequency for averaged magnetization dynamics. For the harmonic excitation ${\mathbf{h}}(t)\sim \exp ( - i\omega t)$, it is possible to use the method of complex amplitudes as above and find P from the scalar product $P_{{}} \sim {\text{Im}} \{ ({\mathbf{m}},{\mathbf{h}}^{*} )\}$. It is worth noting that in the case χ_f ≠ 0 the baseline will contain fluctuation induced contribution of the order $< \Delta {\mathbf{M}}^{2} > /\chi_{f}$.

4.2 Averaged Magnetization Dynamics Without Relaxation

In the case λ, η = 0 all semiclassical equations of the magnetization motion are identical and the averaging of the left and right parts of the Eqs. (1), (3) and (19) gives accordingly:

$$< \frac{{d{\mathbf{M}}}}{dt} > = \frac{{d{\mathbf{m}}}}{dt} = {\mathbf{i}}\frac{{dm_{x} }}{dt} + {\mathbf{j}}\frac{{dm_{y} }}{dt},$$

(29)

$$\begin{gathered} \hfill < (\gamma + \Delta \gamma )[{\mathbf{M}},{\mathbf{H}}] > = \left[ {\gamma H_{0} \left( {1 + \frac{{ < \Delta \gamma \Delta H_{z} >_{QF} }}{{\gamma H_{0} }}} \right)m_{y} - \gamma M_{0} \left( {1 + \frac{{ < \Delta \gamma \Delta M_{z} >_{QF} }}{{\gamma M_{0} }}} \right)h_{y} } \right] \cdot {\mathbf{i}} + \\ \hfill \left[ { - \gamma H_{0} \left( {1 + \frac{{ < \Delta \gamma \Delta H_{z} >_{QF} }}{{\gamma H_{0} }}} \right)m_{x} + \gamma M_{0} \left( {1 + \frac{{ < \Delta \gamma \Delta M_{z} >_{QF} }}{{\gamma M_{0} }}} \right)h_{x} } \right] \cdot {\mathbf{j }}{.} \\ \end{gathered}$$

(30)

Here and after the index QF underlines that corresponding correlators are non zero for quantum fluctuations only. The modified equation of motion follows from the Eqs. (29)–(30)

$$\frac{{dm_{x} }}{dt} = \omega_{H} \left( {1 + b} \right)m_{y} - \gamma M_{0} \left( {1 + a} \right)h_{y} ,$$

(31)

$$\frac{{dm_{y} }}{dt} = - \omega_{H} \left( {1 + b} \right)m_{x} + \gamma M_{0} \left( {1 + a} \right)h_{x} ,$$

(32)

with the renormalized by quantum effects rotation frequency and excitation torque. The parameters a and b are given by

$$a = \frac{{ < \Delta \gamma \Delta M_{z} >_{QF} }}{{\gamma M_{0} }} = 2\frac{{ < \Delta M_{z}^{2} >_{QF} }}{{\mu_{B} M_{0} }} = 2\frac{{M_{0} }}{{\mu_{B} }}\frac{{ < \Delta M_{z}^{2} >_{QF} }}{{M_{0}^{2} }},$$

(33)

$$b = \frac{{\chi_{0} }}{{\chi_{f} }}a.$$

(34)

In expression (33) $\Delta M_{z}$ represents contribution from quantum fluctuations solely. As before, the magnetization M₀ is measured in units of Bohr magneton per magnetic ion.

4.3 Averaged Gilbert Equation

The result of the relaxation term averaging in Gilbert equation [formula (4)] can be easily foreseen. Indeed, vector product (4) mixes different projections of ΔM and dΔM/dt on the coordinate axes. For that reason the only possible effect may originate from replacement γ → γ + Δγ, i.e. from quantum fluctuations. The correlator $< \Delta \gamma \cdot d\Delta M_{z} /dt >$, which may be written as $< \Delta \gamma \cdot d\Delta \gamma /dt > \mu_{B} /2\gamma$ with the help of Eq. (24), requires some discussion. The above supposition that time differentiation corresponds to a “slow variable” and non-zero correlators are not functions of time leads to an estimate $< \Delta \gamma \cdot d\Delta M_{z} /dt > \approx (\mu_{B} /4\gamma )d < \Delta \gamma^{2} > /dt = 0$. Finally, Gilbert equation for Faraday geometry in linear approximation reduces to the averaged system

$$\frac{{dm_{x} }}{dt} = \omega_{H} \left( {1 + b} \right)m_{y} - \gamma M_{0} \left( {1 + a} \right)h_{y} + \gamma \eta M_{0} (1 + a)\frac{{dm_{y} }}{dt},$$

(35)

$$\frac{{dm_{y} }}{dt} = - \omega_{H} \left( {1 + b} \right)m_{y} + \gamma M_{0} \left( {1 + a} \right)h_{x} - \gamma \eta M_{0} (1 + a)\frac{{dm_{x} }}{dt}.$$

(36)

Application of the complex amplitudes method for harmonic excitation ${\mathbf{h}}(t)\sim \exp ( - i\omega t)$ gives the solution of the Eqs. (35)–(36) for EPR absorbed power P_G (x) in a form similar to that provided by Eq. (17) with the variable x renormalized by quantum fluctuations $x = \omega /\omega_{H} (1 + b)$, and replacements $\chi_{0} \to \tilde{\chi }_{0}$ and $\alpha_{G} \to \eta \omega \tilde{\chi }_{0}$, where $\tilde{\chi }_{0} = \chi_{0} (1 + a)/(1 + b)$.

4.4 Averaged Landau–Lifshitz and Consistent Landau–Lifshitz-Gilbert Equation

For the averaging of the double vector product in the expression for the LL relaxation term, it is useful to consider transverse fluctuations $\Delta {\mathbf{M}}_{ \bot }$, $\Delta {\mathbf{H}}_{ \bot }$ in x–y plane and the longitudinal ones along z-axis $\Delta {\mathbf{M}}_{{|{|}}} = \Delta M_{z} {\mathbf{k}}$ and $\Delta {\mathbf{H}}_{{|{|}}} = \Delta H_{z} {\mathbf{k}}$. The result may be presented in the form

$${\mathbf{R}}_{LL} ({\mathbf{m}}) = {\mathbf{R}}_{ \bot } ({\mathbf{m}}_{ \bot } ) + {\mathbf{R}}_{||} ({\mathbf{m}}_{||} ) + {\mathbf{R}}_{0} ,$$

(37)

where ${\mathbf{m}}_{ \bot } = m_{x} {\mathbf{i}} + m_{y} {\mathbf{j}}$ and ${\mathbf{m}}_{||} = m_{z} {\mathbf{k}}$. The terms in the right part of Eq. (37) are given by

$${\mathbf{R}}_{ \bot } = - \lambda H_{0} M_{0} \left( {1 + \frac{{ < \Delta {\mathbf{H}} \cdot \Delta {\mathbf{M}} > }}{{H_{0} M_{0} }}} \right){\mathbf{m}}_{ \bot } + \lambda < \Delta {\mathbf{H}}_{ \bot } (\Delta {\mathbf{M}}_{ \bot } ,{\mathbf{m}}_{ \bot } ) >$$

(38)

$${\mathbf{R}}_{||} = - \lambda < \Delta {\mathbf{H}}_{ \bot } \cdot \Delta {\mathbf{M}}_{ \bot } > {\mathbf{m}}_{||}$$

(39)

$${\mathbf{R}}_{0} = - \lambda M_{0} < \Delta {\mathbf{M}}_{ \bot }^{2} > \left( {\frac{1}{{\chi_{f} }} - \frac{1}{{\chi_{0} }}} \right) \cdot {\mathbf{k}}{.}$$

(40)

The consistency of the proposed model requires R₀ = 0, which is possible if either $\chi_{f} = \chi_{0}$, or $< \Delta {\mathbf{M}}_{ \bot }^{2} > = 0$. Therefore, the LL relaxation term strongly depends on the spatial characteristics of magnetic fluctuations. When fluctuations occur solely along the external magnetic field direction (z-axis), i.e. the only projection ΔM_z is non-zero, the relations R₀ = 0 and R_||= 0 hold simultaneously and the fluctuation contribution to R_⊥ depends on the ΔM_z mean square

$${\mathbf{R}}_{ \bot } = - \lambda H_{0} M_{0} \left( {1 + \frac{{\chi_{0} }}{{\chi_{f} }}\frac{{ < \Delta M_{z}^{2} > }}{{M_{0}^{2} }}} \right){\mathbf{m}}_{ \bot } .$$

(41)

The Eq. (41) is valid for any χ _f, which means that in this case the considered model may have an additional parameter controlling the effect of magnetic fluctuations, which may be enhanced or suppressed depending on the relation between χ₀ and χ_f.

In the transverse case $\Delta {\mathbf{M}}_{ \bot } ,\Delta {\mathbf{H}}_{ \bot } \ne 0$ and the requirement R₀ = 0 may be fulfilled the case $\chi_{f} \equiv \chi_{0}$ only. Than, the Eq. (38) is reduced to

$${\mathbf{R}}_{ \bot } = - \lambda H_{0} M_{0} \left[ {\left( {1 + \frac{{ < \Delta M_{z}^{2} > + < \Delta M_{y}^{2} > }}{{M_{0}^{2} }}} \right)m_{x} \cdot {\mathbf{i}} + \left( {1 + \frac{{ < \Delta M_{z}^{2} > + < \Delta M_{x}^{2} > }}{{M_{0}^{2} }}} \right)m_{y} \cdot {\mathbf{j}}} \right].$$

(42)

It is visible that anisotropic fluctuations in x–y plane with $< \Delta M_{x}^{2} > \ne < \Delta M_{y}^{2} >$ may induce magnetization dynamics with anisotropic relaxation. This results in new effects in EPR described in the next section.

Averaging of the relaxation term in CLLG Eq. (19) results in appearance of the additional terms in Eqs. (41, 42) which may originate from quantum fluctuations due to replacement γ → γ + Δγ and accounting the correlator $< \Delta \gamma \Delta M_{z} >_{QF}$ and average $< \Delta \gamma^{2} >$. The modified Eq. (42) acquires the form

$${\mathbf{R}}_{ \bot } = - \tilde{\lambda }H_{0} M_{0} [\left( {1 + c_{x} } \right)m_{x} \cdot {\mathbf{i}} + \left( {1 + c_{y} } \right)m_{y} \cdot {\mathbf{j}}]$$

(43)

with $\tilde{\lambda } = \eta \gamma^{2}$ and

$$c_{x,y} = \frac{{ < \Delta \gamma^{2} > }}{{\gamma^{2} }} + \frac{{\chi_{0} }}{{\chi_{f} }}\left( {2\frac{{ < \Delta \gamma \Delta M_{z} >_{QF} }}{{\gamma M_{0} }} + \frac{{ < \Delta M_{z}^{2} > }}{{M_{0}^{2} }}} \right)$$

(44)

for the longitudinal case when the fluctuations in x–y plane are missing. The transverse fluctuations give accordingly

$$c_{x,y} = \frac{{ < \Delta \gamma^{2} > }}{{\gamma^{2} }} + 2\frac{{ < \Delta \gamma \Delta M_{z} >_{QF} }}{{\gamma M_{0} }} + \frac{{ < \Delta M_{z}^{2} > + < \Delta M_{y,x}^{2} > }}{{M_{0}^{2} }}.$$

(45)

Note that the averages $< \Delta M_{z}^{2} >$, $< \Delta M_{x}^{2} >$ and $< \Delta M_{y}^{2} >$ in the above formulae include all possible magnetic fluctuations including quantum ones. At the same time, the correlator $< \Delta \gamma \Delta M_{z} >_{QF}$ depends on the “quantum part” of ΔM_z only.

The averaging of the relaxation term allows finding averaged LL and CLLG equations for the semiclassical magnetization dynamics, which may be applied for the case of EPR in Faraday geometry

$$\frac{{dm_{x} }}{dt} = \omega_{H} \left( {1 + b} \right)m_{y} - \gamma M_{0} \left( {1 + a} \right)h_{y} - \nu_{0} (1 + c_{x} )m_{x} ,$$

(46)

$$\frac{{dm_{y} }}{dt} = - \omega_{H} \left( {1 + b} \right)m_{y} + \gamma M_{0} \left( {1 + a} \right)h_{x} - \nu_{0} (1 + c_{y} )m_{y} .$$

(47)

Here $\nu_{0} = \lambda \chi_{0} \omega_{H}^{2} /\gamma^{2}$ for LL equation and $\nu_{0} = \eta \chi_{0} \omega_{H}^{2}$ for CLLG equation.

5 Electron Paramagnetic Resonance with the Anisotropic Relaxation Term

The computation of the absorbed power in CW EPR with the help of the system Eqs. (46)–(47) for a harmonic excitation at frequency ω and linearly polarized vector h gives

$$P(x) = P_{0} \frac{{\alpha (1 + z)\{ [\alpha (1 - z)/2]\sin 2\phi + 1\} }}{{(1 - x^{2} + \alpha^{2} z^{2} /x^{2} )^{2} + \alpha^{2} (1 + z)^{2} }},$$

(48)

where

$$P_{0} = \chi_{0} \frac{1 + a}{{1 + b}}h_{0}^{2} ,$$

(49)

$$x = \omega /\omega_{H} (1 + b),$$

(50)

$$z = \frac{{1 + c_{y} }}{{1 + c_{x} }},$$

(51)

$$\alpha = \alpha_{0} \frac{{1 + c_{x} }}{{(1 + b)^{2} }},$$

(52)

and α₀ equals $\lambda \chi_{0} \omega /\gamma^{2}$ or $\eta \chi_{0} \omega$ for LL and CLLG equations respectively. In Eq. (48) $\phi$ is the angle between x-axis and vector h, so that $h_{x} = h_{0} \cos \phi$ and $h_{y} = h_{0} \sin \phi$. In EPR oscillation mode, the corresponding amplitudes for the projections of the vector m are

$$m_{x} = m_{0} {\text{Re}} \left\{ {\frac{\sin \phi - (z\alpha /x - i \cdot x)\cos \phi }{{(\alpha /x - i \cdot x)(z\alpha /x - i \cdot x) + 1}}} \right\},$$

(53)

$$m_{y} = m_{0} {\text{Re}} \left\{ {\frac{\sin \phi + (\alpha /x - i \cdot x)\cos \phi }{{(\alpha /x - i \cdot x)(z\alpha /x - i \cdot x) + 1}}} \right\}.$$

(54)

Here $m_{0} = \chi_{0} h_{0} (1 + a)/(1 + b)$.

We see that for the anisotropic relaxation term and LL or CLLG magnetization dynamics the EPR line may depend on the angle $\phi$ if $z \ne 1$, i.e. the averages $< \Delta M_{x}^{2} >$ and $< \Delta M_{y}^{2} >$ are different. In the isotropic case, when transverse fluctuations are identical ($< \Delta M_{x}^{2} > \equiv < \Delta M_{y}^{2} >$), or in the longitudinal case, the parameter z equals 1 and dependence on the orientation of vector h in x–y plane is lifted in Eq. (48). Thus, for $z = 1$ the P(x) has the same functional form as P_LL(x) given by Eq. (15).

The dependence on $\phi$ constitutes a new polarization effect in EPR, which may become pronounced for a broad EPR lines characteristic for SCES (Figs. 5, 6). It is visible, that for z = 0.5 and relaxation parameter α = 0.3 only weak modulation of the EPR line magnitude and width induced by the change of location of vector h occurs (Fig. 5). However, when α is increased up to α = 3 and EPR line becomes broad, it is possible expecting strong polarization effect with 180° symmetry for the same anisotropy parameter z = 0.5. According to Fig. 6 the EPR line may be almost suppressed for certain angle $\phi$.

Fig. 5

Polarization effect in EPR following from Landau–Lifshitz equation and consistent Landau–Lifshitz-Gilbert equation with anisotropic relaxation (3D plot and contour map). The case of relatively narrow EPR line (α = 0.3) and moderate anisotropy (z = 0.5)

Fig. 6

Polarization effect in EPR following from Landau–Lifshitz equation and consistent Landau–Lifshitz-Gilbert equation with anisotropic relaxation (3D plot and contour map). The case of broad EPR line (α = 3) and moderate anisotropy (z = 0.5)

Sometimes it is stated that magnetic resonance probes eigen modes of magnetic oscillations, which are often described as a circular rotation of the magnetization around an external field [21]. This is correct for the modes without damping, but in SCES damping can never be neglected. Additionally, the EPR problem is a problem of forced oscillations rather than eigen modes problem. Therefore, it may be important to take into account that real magnetic oscillation mode may be far from round precession. The solution given by Eqs. (53)–(54) is visualized in Figs. 7, 8 for the two cross-sections of the P(x) plot in Fig. 6, illustrating x dependence corresponding to the fixed angle $\phi$ at which EPR line is the biggest (Fig. 7) and showing angle dependence for the x = const corresponding to the position of the P(x) maximum (Fig. 8). The trajectory of the vector M in x–y plane has a complicated quasi-elliptical shape with noticeable elongation and, for certain values of parameters, become almost linear.

Fig. 7 

Evolution of the magnetic oscillations mode by the parameter $x = \omega /\gamma H_{0} (1 + b)$ for the angle $\phi = 45^{o}$ between vector h and x-axis corresponding to the maximal amplitude of EPR in Fig. 6. The values of relaxation α and anisotropy z parameters are the same as in Fig. 6

Fig. 8 

Evolution of the magnetic oscillations mode by angle $\phi$ between vector h and x-axis. The parameter $x = \omega /\gamma H_{0} (1 + b) = 1.5$ corresponds to the section of EPR absorption in Fig. 6 passing trough maxima. The values of relaxation α and anisotropy z parameters are the same as in Fig. 6

6 Fluctuation Induced Universal Relations Between EPR Parameters

In the framework of LL or CLLG magnetization dynamics, special links between the EPR line parameters may appear. For example, let us consider a situation when quantum fluctuations dominate and relaxation is weak. Then the resonance condition is $\omega \approx \gamma H(1 + b)$ and, as long as g-factor proportional to the ratio $\omega /H$, the fluctuation induced correction to this parameter will be $\Delta g \approx g_{0} \cdot b$. In the case of an isotropic relaxation term, the correction to EPR line width may be estimated as $\Delta W = W_{0} c_{x,y}$. Here, g₀ and W₀ denotes the g-factor value and EPR line width unperturbed by magnetic fluctuations. If the CLLG magnetization dynamics is assumed, it is possible to estimate $< \Delta \gamma^{2} > /\gamma^{2} \approx (\Delta g/g_{0} )^{2}$. Assuming small corrections to the g-factor, it is possible to neglect this term in Eqs. (44)–(45). In this situation, the Eqs. (33)–(34), (41)–(42) and (44)–(45) suggest that either for longitudinal or transverse fluctuations the ratio ΔW/Δg is independent of χ_f and χ₀, and does not include possible temperature dependence caused by these parameters. Combining the aforementioned equations and Eq. (27) it is possible to come to the formula linking magnetic fluctuation induced corrections

$$\frac{\Delta W}{{\Delta g}} \approx \frac{{W_{0} }}{{g_{0} }}\left( {n + \frac{{\mu_{B} }}{{k\chi_{0} H_{0} }}} \right),$$

(55)

where the coefficient n equals 2 for CLLG equation and equals 1 for LL equation. The coefficient k is the same for CLLG and LL equations and acquires the values k = 2 for the longitudinal fluctuations and k = 1 for transverse fluctuations. The simplest estimate can be done assuming Curie–Weiss magnetic susceptibility $\chi_{0} = (g_{0} J\mu_{B} )^{2} /k_{B} T$ for a magnetic ion with a quantum number J and level broadening of the order ~ k_BT corresponding to $\Delta W\sim 2k_{B} T/\mu_{B}$ in the units of magnetic field. After that we get

$$\frac{\Delta W}{{\Delta g}}\sim \frac{2}{{g_{0} }}\left( {n + \frac{{k_{B} T}}{{k(g_{0} J)^{2} \mu_{B} H_{0} }}} \right)\frac{{k_{B} T}}{{\mu_{B} }}.$$

(56)

The second term in brackets may be neglected if temperature is low enough and in this diapason a universal relation should hold

$$\frac{\Delta W}{{\Delta g}}\sim a_{0} \cdot \frac{{k_{B} T}}{{\mu_{B} }}, \, a_{0} = \frac{2n}{{g_{0} }},$$

(57)

where dependence of the material type appears via unperturbed g-factor g₀ only. It is worth noting that the relation (57) does not depend on the fluctuations magnitude and is characterized by numerical coefficient a₀ of the order of unity.

Landau–Lifshitz magnetization dynamics may lead to another type of universal relation between the EPR line width and g-factor. In the intermediate range of α, the dependence $x_{\max LL} = \sqrt {\frac{1}{2} + \sqrt {\frac{1}{4} + \alpha_{LL}^{2} } }$ may be close to linear one, $x_{\max LL} \sim \alpha_{LL}$, whereas $W\sim \alpha_{LL}$ as usual. This case may correspond to the case

$$\frac{W}{\Delta g} \approx const,$$

(58)

when line width and g-factor shift are proportional to each other and it is reasonable to expect that the proportionality coefficient is almost independent of temperature.

7 Conclusions: The Averaged Magnetization Dynamics and Experiment

The result of the present work may be formulated as obtaining simple analytical relations for relaxation parameters containing contribution of the magnetization fluctuation magnitude in the cases of Gilbert, Landau–Lifshitz and consistent Landau–Lifshitz–Gilbert equations for semiclassical magnetization dynamics in the region of linear magnetic response. In spite of the common belief, for noticeable relaxation leading to broad EPR lines the solutions provided by Landau–Lifshitz and Gilbert equations are essentially deviate and therefore various phenomenological equations for magnetization dynamics may reflect different physical situations in EPR in strongly correlated materials. We may expect that whereas Gilbert equation is fully controlled by quantum fluctuations only, Landau–Lifshitz and consistent Landau–Lifshitz–Gilbert equations depends on magnetic fluctuations of any nature. Therefore, the right choice of semiclassical spin dynamics description in a strongly correlated system with the magnetic fluctuations may be of crucial importance.

This means that the final judgment depends on experiment, as usual. For that reason, the available to date EPR experiments probably relevant to considered models should be considered. We wish to mark, that the Gilbert equation is intuitively expected in the paradigm of the damped resonance, because in this model the EPR line broadening accompanied by negative g-factor shift, i.e. by an increase of the resonance magnetic field measured at ω = const. From that point of view, a variety of various systems (not necessarily the strongly correlated ones) should display such behavior [25]. In this sense, the opposite behavior provided by Landau–Lifshitz and consistent Landau–Lifshitz-Gilbert equations may look controversial. However, there is a variety of strongly correlated systems which seems following predictions for these type of models.

Firstly, the variant of the averaged LL model with the relaxation parameter λ proportional to the hyromagnetic ratio γ and dominating quantum fluctuations suggested in [18] was successfully applied for description of high frequency (60 GHz) EPR in spiral magnet MnSi [26]. However, as we see, the assumed proportionality λ ~ γ is a formal result of making LL and G equations equivalent   [10], which hardly has proper justification. In view of more rigorous treatment in the present work, the case studied in [26] corresponds to either G or CLLG equation with quantum fluctuations of magnetization and missing fluctuations of magnetic field, i.e. with the parameter b = 0 [Eqs. (30)–(34)].

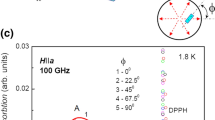
When magnetization dynamics of Landau–Lifshitz type is chosen, several interesting opportunities emerge. For example, an anisotropic relaxation may appear as a consequence of the broken equivalence between the fluctuations $< \Delta M_{x}^{2} >$ and $< \Delta M_{y}^{2} >$. The model predicts new polarization effect, when excitation of EPR may depend on the direction of oscillating magnetic field as described in the Sect. 5. This unusual behavior is reported for a quasi one dimensional quantum spin chain system CuGeO₃ doped with Co impurity long ago [27, 28] and has been quantitatively explained by formal introducing of the anisotropic relaxation frequency and g-tensor in the recent work [12]. The present study suggests a possible physical reason for appearance of an anisotropic relaxation.

It should be added that the considered polarization effect may serve as a marker of spin nematic state in the systems with the hidden quadrupole order [29]. In this case, the paramagnetic phase is isotropic with respect to magnetic fluctuations: $< \Delta M_{x}^{2} > = < \Delta M_{x}^{2} >$, whereas the phase with the quadrupole order is characterized by different magnetic fluctuation magnitude along x and y axes so that $< \Delta M_{x}^{2} > \ne < \Delta M_{x}^{2} >$ [29]. Probably the best study of spin nematic effect is carried for strongly correlated metal CeB₆, where it was predicted in theory [29] and observed experimentally [30, 31]. The phase with hidden order in this material is characterized for T < 3 K by an anisotropic line width depending on the orientation of steady magnetic field with respect to the crystal axes [29] which may be explained by the complex interplay between ferromagnetic and antiferromagnetic correlations affecting magnetization fluctuation amplitude [5, 6, 9]. However, the possibility of the polarization effect in EPR spectra was not investigated in CeB₆.

For the CuGeO₃:Co the EPR mode with pronounced polarization effect develops below T ~ 40 K and, like in CeB₆, depends on the steady magnetic field direction [26, 27]. At the same time, the theoretical supposition that the unusual properties of the magnetic resonance in CuGeO₃:Co may be related to spin nematic effect was never done even at qualitative level. Thus, in our opinion, the situation with the polarization effect in spin nematic phases requires clarification and further experiments in this direction may be rewarding. Nevertheless, it is possible to point out that in CuGeO₃:Co the EPR lines are strongly broadened [26, 27] and in CeB₆ the EPR lines are relatively narrow [30] at low temperatures. According to our analysis in Sect. 5, the narrow EPR line is less sensitive to orientation of the oscillating magnetic field (Figs. 5, 6) and therefore the proposed model at least does not contradict to experimental data available to date.

The universal link between some contributions to the line width and to the g-factor were first obtained as a consequence of Oshikawa–Affleck theory [7, 8] in the Refs. [32, 33]. The ratio of the corrections ΔW to Δg caused by presence of staggered field in one dimensional antiferromagnetic S = 1/2 shin chain has the form (57) with the proportionality coefficient equal to 1.99 [32, 33]. Interesting that for CLLG magnetization dynamics and g₀≈2 the coefficient in Eq. (57) is almost the same as in Oshikawa-Affleck theory: $2n/g_{0} \approx 2$. However, physical reasons causing the universal relations in [7, 8] and in the present study are essentially different. In Oshikawa-Affleck theory the EPR line width appears in exact solution for particular spin chain system as a result of symmetry breaking caused by anisotropic terms in spin Hamiltonian [7, 8] and consequently has a regular nature. In our model, the universal relation (57) is completely quantum magnetic fluctuation driven and is not confined by one dimensional systems and thus being more general. At the same time, both “sources” of universal relations may lead to close proportionality coefficients, thus making interpretation of experimental data ambiguous.

It is possible to add that relation W/Δg = const [Eq. (58)], which may be expected in LL model, was observed in the paramagnetic phase of GdB₆ [34]. Therefore, further look for universal relations between EPR parameters may become interesting topic for future experimental and theoretical investigations.

As a concluding remark, it is possible to say that the relation between relaxation parameter giving EPR line width and magnitude of magnetic fluctuations is characteristic for any type of magnetization dynamics considered. This justifies the applicability of EPR for studying of a new group of magnetic phenomena – spin fluctuation transitions [9, 35, 36]. A spin-fluctuation transition is a change in the characteristics of magnetic fluctuations (in many cases having an abrupt character) under the influence of control parameters (for example, temperature or material composition) that is not directly related to the formation of phases with long-range magnetic order [9, 35]. At present, spin fluctuation transitions are observed in helical magnets MnSi and Mn_1-xFe_xSi, magnetic semiconductors Hg_1–xMn_xTe, doped compensated semiconductors Ge:As(Ga), and strongly correlated metal with hidden order CeB₆ [35, 36]. The results of the present work show that EPR is the powerful and direct tool for investigation of these interesting magnetic phenomena as long as parameters of magnetic resonance contains direct information about magnetic fluctuations.