Set-monotonicity implies Kelly-strategyproofness

Abstract

This paper studies the strategic manipulation of set-valued social choice functions according to Kelly’s preference extension, which prescribes that one set of alternatives is preferred to another if and only if all elements of the former are preferred to all elements of the latter. It is shown that set-monotonicity—a new variant of Maskin-monotonicity—implies Kelly-strategyproofness in comprehensive subdomains of the linear domain. Interestingly, there are a handful of appealing Condorcet extensions—such as the top cycle, the minimal covering set, and the bipartisan set—that satisfy set-monotonicity even in the unrestricted linear domain, thereby answering questions raised independently by Barberà (J Econ Theory 15(2):266–278(1977a)) and Kelly (Econometrica 45(2):439–446 (1977)).

1 Introduction

One of the central results in microeconomic theory states that every non-trivial social choice function (SCF)—a function mapping individual preferences to a collective choice—is susceptible to strategic manipulation (Gibbard 1973; Satterthwaite 1975). However, the classic theorem by Gibbard and Satterthwaite only applies to resolute,i.e., single-valued, SCFs. The notion of a resolute SCF is rather restricted and artificial.¹ For example, consider a situation with two agents and two alternatives such that each agent prefers a different alternative. The problem is not that a resolute SCF has to pick a single alternative (which is a well-motivated practical requirement), but that it has to pick a single alternative based on the individual preferences alone (see also, Kelly 1977). As a consequence, resoluteness is at variance with elementary notions of fairness such as neutrality and anonymity.

In order to remedy this shortcoming, Gibbard (1977) went on to characterize the class of strategyproof social decision schemes (SDSs), i.e., aggregation functions that yield probability distributions over the set of alternatives rather than single alternatives (see also Gibbard 1978; Barberà 1979). This class consists of rather degenerate SDSs and Gibbard’s characterization is therefore often interpreted as another impossibility result. However, Gibbard’s theorem rests on unusually strong assumptions with respect to the agents’ preferences. In contrast to the traditional setup in social choice theory, which typically only involves ordinal preferences, his result relies on the expected utility axioms of Neumann and Morgenstern (1947), and hence on the existence of linear utility functions, in order to compare lotteries over alternatives.

The gap between Gibbard and Satterthwaite’s theorem for resolute SCFs and Gibbard’s theorem for SDSs has been filled by a number of impossibility results with varying underlying notions of how to compare sets of alternatives with each other (e.g., Gärdenfors 1976; Barberà 1977a, b; Kelly 1977; Feldman 1979a; MacIntyre and Pattanaik 1981; Bandyopadhyay 1982, 1983b; Duggan and Schwartz 2000; Barberà et al. 2001; Ching and Zhou 2002; Sato 2008; Umezawa 2009), many of which are surveyed by Taylor (2005) and Barberà (2010). In this paper, we will be concerned with the one of the weakest (and therefore least controversial) preference extensions from alternatives to sets due to Kelly (1977). According to this definition, a set of alternatives is weakly preferred to another set of alternatives if all elements of the former are weakly preferred to all elements of the latter. A nice aspect of Kelly’s preference extension is that its underlying behavioral assumptions are quite minimalistic (which strengthens impossibility results), yet reasonable enough to motivate meaningful positive results. Kelly’s extension models that the agents are complete unaware of the tie-breaking mechanism that is used to eventually pick a single alternative. The question pursued in this paper is whether this uncertainty can be exploited to achieve strategyproofness.

Barberà (1977a) and Kelly (1977) have shown independently that all non-trivial SCFs that are rationalizable via a quasi-transitive relation are manipulable according to Kelly’s extension.² However, it is it well-known that (quasi-transitive) rationalizability by itself is unduly restrictive (see, e.g., Mas-Colell and Sonnenschein 1972). As a consequence, Kelly (1977) concludes his paper by contemplating that “one plausible interpretation of such a theorem is that, rather than demonstrating the impossibility of reasonable strategy-proof SCFs, it is part of a critique of the regularity [rationalizability] conditions” and Barberà (1977a) states that “whether a nonrationalizable collective choice rule exists which is not manipulable and always leads to nonempty choices for nonempty finite issues is an open question.” Also referring to nonrationalizable choice functions, Kelly (1977) writes: “it is an open question how far nondictatorship can be strengthened in this sort of direction and still avoid impossibility results.” The condition of rationalizability has been significantly weakened in subsequent impossibility results (MacIntyre and Pattanaik 1981; Bandyopadhyay 1982, 1983b). At the same time, it has been noted that more positive results can be obtained for antisymmetric (i.e., linear) individual preferences. In particular, it was shown that the omninomination rule (Gärdenfors 1976), the Pareto rule (Feldman 1979a, b), the Condorcet rule (Gärdenfors 1976; Nehring 2000), and the top cycle (MacIntyre and Pattanaik 1981; Bandyopadhyay 1983a; Sanver and Zwicker 2012) are strategyproof when preference are linear (see Remark 1 for more details about these SCFs). However, all these rules are very indecisive and the latter two may even return Pareto-dominated alternatives.

In this paper, we propose a new variant of Maskin-monotonicity for set-valued SCFs called set-monotonicity and show that all set-monotonic SCFs are strategyproof in sufficiently rich subdomains of the linear domain. This covers all of the positive results mentioned above and proves that some—much more discriminating—SCFs are strategyproof. Set-monotonicity requires the invariance of choice sets under the weakening of unchosen alternatives and is satisfied by the omninomination rule, the Pareto rule, the Condorcet rule, and a handful of appealing Condorcet extensions such as top cycle, the minimal covering set, and the bipartisan set. Since set-monotonicity coincides with Maskin-monotonicity in the context of resolute SCFs, this characterization can be seen as a set-valued generalization of the Muller and Satterthwaite (1977) theorem.

Nehring (2000) has proved a similar extension of the Muller-Sattherthwaite theorem by showing that Maskin-monotonicity implies strategyproofness of set-valued SCFs in a sense marginally weaker than that of Kelly (see also Remark 6).³ However, while Maskin-monotonicity is prohibitive in the general domain, set-monotonicity is not. The conditions themselves are independent, but we show that Maskin-monotonicity implies set-monotonicity when assuming independence of unchosen alternatives.

We conclude the paper with a number of remarks concerning group-strategyproofness, stronger preference extensions, weak preferences, weaker domain conditions, and strategic abstention.

2 Preliminaries

Let $N=\{1,\ldots ,n\}$ be a finite set of agents, $A$ a finite and nonempty set of alternatives, and $\fancyscript{L}$ the set of all linear (i.e., complete, transitive, and antisymmetric) preference relations over $A$. For $R_i\in \fancyscript{L}$, $x \mathrel {R_i} y$ denotes that agent $i$ values alternative $x$ at least as much as alternative $y$. We write $P_i$ for the strict part of $R_i$, i.e.,  $x \mathrel {P_i} y$ if $x \mathrel {R_i} y$ but not $y \mathrel {R_i} x$. The (strict) lower contour set of alternative $x$ with respect to $P_i$ is denoted by $L(x,R_i)=\{y\in A :x\mathrel {P_i} y\}$. For convenience, we will represent preference relations as comma-separated lists. For example, $a \mathrel {P_i} b \mathrel {P_i} c$ will be written as $R_i :a, b, c$. Two distinct alternatives $x$ and $y$ are adjacent in $R_i$ if there is no $z$ with $x\mathrel {P_i} z \mathrel {P_i} y$.

A (Cartesian) domain of preference profiles $\fancyscript{D}$ is defined as $\fancyscript{D}=\prod _{i\in N} \fancyscript{D}_i \subseteq \fancyscript{L}^N$. The maximal domain $\fancyscript{L}^N$ will be referred to as the general domain. We say that $R^{\prime \prime }$ lies in the comprehensive closure of $R$ and $R^\prime$ if for all $i\in N$, $R_i\cap R^\prime _i\subseteq R^{\prime \prime }_i$. A domain $\fancyscript{D}$ is comprehensive if for all $R,R^\prime \in \fancyscript{D}$ and $R^{\prime \prime }\in \fancyscript{L}^N$ such that $R^{\prime \prime }$ lies in the comprehensive closure of $R$ and $R^\prime$, $R^{\prime \prime }\in \fancyscript{D}$ (Nehring 2000). For example, the domain of all linear extensions of a fixed partial order is comprehensive. For a given preference profile $R\in \fancyscript{D}$, $R_{-i}=(R_1,\ldots ,R_{i-1},R_{i+1},\ldots ,R_n)$ denotes the vector of all preference relations except that of agent $i$. An alternative $x\in A$ is called a Condorcet winner if $|\{i\in N:x \mathrel {P_i} y\}|> n/2$ for all $y\in A{\setminus } \{x\}$.

Our central object of study are SCFs. A social choice function (SCF) is a function $f$ that maps a preference profile $R\in \fancyscript{D}$ to a nonempty subset of alternatives $f(R)$. $f$ is resolute if $|f(R)|=1$ for all $R\in \fancyscript{D}$. A Condorcet extension is an SCF that uniquely selects a Condorcet winner whenever one exists.

2.1 Monotonicity

We will consider three variants of monotonicity: a weak standard notion and two strengthenings, one of which was proposed by Maskin (1999) and one of which is new to this paper. For a given preference profile $R$, an agent $i$, and two adjacent alternatives $x,y$ such that $y\mathrel {P_i} x$, $R^{i:(x,y)}$ denotes the preference profile in which agent $i$ swapped alternatives $x$ and $y$ and that is otherwise identical to $R$.

Definition 1

Let $R,R^\prime \in \fancyscript{D}$, $i\in N$,  and  $x,y\in A$ such that $R^\prime = R^{i:(x,y)}$. Then, SCF $f$ satisfies monotonicity, Maskin-monotonicity, or set-monotonicity, if

$$\begin{aligned} x\in f(R)&\text { implies } x\in f(R^\prime ), \end{aligned}$$

(monotonicity)

$$\begin{aligned} z\in f(R) \,\text { and }\, y\in A{\setminus }\{z\}&\text { implies } z\in f(R^\prime ),\text { or} \end{aligned}$$

(Maskin-monotonicity)

$$\begin{aligned} Z=f(R) \,\text { and }\, y\in A{\setminus } Z&\text { implies } Z=f(R^\prime ), \text {respectively}. \end{aligned}$$

(set-monotonicity)

The intuitive meaning of these definitions is as follows. An SCF satisfies monotonicity if a chosen alternative remains in the choice set when it is strengthened with respect to another alternative; it satisfies Maskin-monotonicity if a chosen alternative remains in the choice set when weakening another alternative; and it satisfies set-monotonicity if the choice set is invariant under the weakening of unchosen alternatives.⁴ $^{,}$ ⁵

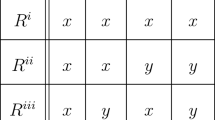
Clearly, Maskin-monotonicity implies monotonicity and, as will be shown in Proposition 2, set-monotonicity also implies monotonicity. Despite the similar appearance, set-monotonicity is logically independent of Maskin-monotonicity. Set-monotonicity has a stronger antecedent and a stronger consequence. For example, consider a comprehensive single-agent domain consisting of the relations $R_1$ and $R^\prime _1$ given by

$$\begin{aligned}&R_1:a, b, c, \quad \text {and}\\&R^\prime _1:b, a, c. \end{aligned}$$

If we define SCF $f$ by letting $f((R_1))=\{c\}$ and $f((R^\prime _1))=\{b,c\}$, $f$ satisfies Maskin-monotonicity, but violates set-monotonicity. If, on the other hand, we define $f$ by letting $f((R_1))=\{a,b,c\}$ and $f((R^\prime _1))=\{b,a\}$, $f$ satisfies set-monotonicity, but violates Maskin-monotonicity.

Set-monotonicity coincides with Maskin-monotonicity in the context of resolute SCFs. Under the condition of independence of unchosen alternatives which is satisfied by various set-valued SCFs, set-monotonicity is weaker than Maskin-monotonicity. Moreover, set-monotonicity implies independence of unchosen alternatives. Independence of unchosen alternatives was introduced by Laslier (1997) in the context of tournament solutions (as “independence of the losers”) and requires that the choice set is invariant under modifications of the preference profile with respect to unchosen alternatives.

Definition 2

An SCF $f$ satisfies independence of unchosen alternatives (IUA) if for all $R,R^\prime \in \fancyscript{D}$ such that $R_i|_{\{x,y\}}=R^\prime _i|_{\{x,y\}}$ for all $x\in f(R)$, $y\in A$, and $i\in N$, $f(R)=f(R^\prime )$.

Proposition 1

Maskin-monotonicity and IUA imply set-monotonicity.

Proof

Let $f$ be an SCF, $R\in \fancyscript{D}$, $i\in N$, $x\in A$, $y\in A{\setminus } f(R)$, and $R^\prime =R^{i:(x,y)}$. Maskin-monotonicity implies that $f(R)\subseteq f(R')$. Now, assume for contradiction that there is some $x^\prime \in f(R^\prime ){\setminus } f(R)$. Since $x^\prime \not \in f(R)$, it follows from Maskin-monotonicity that there is some $y^\prime \in A$ that is strengthened with respect to $x^\prime$ when moving from $R^\prime$ to $R$. Hence, $R^\prime = R^{i:(x^\prime ,y^\prime )}$, $x^\prime =x$, and $y^\prime =y$. Since $x=x^\prime \not \in f(R)$ and $y\not \in f(R)$ by assumption, IUA implies that $f(R)=f(R^\prime )$, a contradiction.$\square$

Proposition 2

Set-monotonicity implies monotonicity and IUA.

Proof

We first show that set-monotonicity implies monotonicity. Let $f$ be a set-monotonic SCF, $R\in \fancyscript{D}$, $i\in N$, $x\in f(R)$, $y\in A$, and $R^\prime \in R^{i:(x,y)}$. Clearly, in case $y\not \in f(R)$, set-monotonicity implies that $f(R^\prime )=f(R)$ and thus $x\in f(R^\prime )$. If, on the other hand, $y\in f(R)$, assume for contradiction that $x\not \in f(R^\prime )$. When moving from $R^\prime$ to $R$, $y$ is strengthened with respect to outside alternative $x$, and set-monotonicity again implies that $f(R)=f(R^\prime )$, a contradiction. The fact that set-monotonicity implies IUA is straightforward from the definitions.$\square$

2.2 Strategyproofness

An SCF is manipulable if an agent can misrepresent his preferences in order to obtain a more preferred outcome. Whether one choice set is preferred to another depends on how the preferences over individual alternatives are to be extended to sets of alternatives. In the absence of information about the tie-breaking mechanism that eventually picks a single alternative from any choice set, preferences over choice sets are obtained by the conservative extension $\widehat{R}_i$ (Kelly 1977), where for any pair of nonempty sets $X,Y\subseteq A$ and preference relation $R_i$,

Clearly, in all but the simplest cases, $\widehat{R}_i$ is incomplete, i.e., many pairs of choice sets are incomparable. The strict part of $\widehat{R}_i$ is denoted by $\widehat{P}_i$, i.e., $X \mathrel {\widehat{P}_i} Y$ if and only if $X \mathrel {\widehat{R}_i} Y$ and $x \mathrel {P_i} y$ for at least one pair of $x\in X$ and $y\in Y$. For linear preferences, $X \mathrel {\widehat{P}_i} Y$ if and only if $x \mathrel {P_i} y$ for all $x\in X$ and $y\in Y$ with $x\ne y$. Hence, $|X\cap Y|\le 1$.

Definition 3

An SCF is Kelly-strategyproof if there exist no $R, R^\prime \in \fancyscript{D}$ and $i\in N$ with $R_{-i}=R^\prime _{-i}$ such that $f(R^\prime ) \mathrel {\widehat{P}_i} f(R)$.

Kelly-strategyproofness is a very weak notion of strategyproofness. Nevertheless, most well-known SCFs such as plurality, Borda’s rule, Copeland’s rule, Slater’s rule, or plurality with runoff fail to be Kelly-strategyproof in the general domain (see, e.g., Taylor 2005, Theorem 2.2.2).

Muller and Satterthwaite (1977) have shown that, in the general domain, a resolute SCF is strategyproof if and only if it satisfies Maskin-monotonicity. Unfortunately, as famously shown by Gibbard (1973) and Satterthwaite (1975), only dictatorial or imposing resolute SCFs satisfy Maskin-monotonicity in the general domain. However, Maskin-monotonicity still implies strategyproofness of resolute SCFs in many restricted domains of interest (see, e.g., Klaus and Bochet 2013), including the class of comprehensive domains considered in this paper (Nehring 2000).

3 Result

Theorem 1

Every set-monotonic SCF on a comprehensive domain is Kelly-strategyproof.

Proof

Let $f$ be a set-monotonic SCF and $\fancyscript{D}$ a comprehensive domain. We first show that set-monotonicity is equivalent to a version of set-monotonicity that is not restricted to pairwise swaps of adjacent alternatives. Rather, we require that choice sets may only change if an alternative is removed from a lower contour set of a chosen alternative. For $R,R^\prime \in \fancyscript{D}$ and $i\in N$ with $R_{-i}=R^\prime _{-i}$, we say that $R^\prime$ is an $f$-improvement over $R$ if for all $x\in f(R)$, $L(x,P_i)\subseteq L(x,P^\prime _i)$. We claim that if $R^\prime$ is an $f$-improvement over $R$, then $f(R^\prime )=f(R)$. The statement can be shown by induction on $d(R,R^\prime )=|R_i{\setminus } R^\prime _i|$. The induction basis is trivially satisfied because $R=R^\prime$ if $d(R,R^\prime )=0$. Assume the statement is true for all $R$ and $R^\prime$ with $d(R,R^\prime )< k$ and consider $R$ and $R'$ such that $d(R,R^\prime )=k$. Since $R\ne R^\prime$, there have to be two alternatives $x,y\in A$ such that $x\mathrel {P_i} y$ and $y\mathrel {P^\prime _i} x$. Due to the transitivity of $R_i$ and $R^\prime _i$, we may furthermore assume that $x$ and $y$ are adjacent in $R_i$. (However, $x$ and $y$ need not be adjacent in $R^\prime _i$.) $x\not \in f(R)$ because otherwise $R^\prime$ is not an $f$-improvement over $R$. Set-monotonicity implies that $f(R^{i:(y,x)})=f(R)$ and comprehensiveness that $R^{i:(y,x)}\in \fancyscript{D}$. It follows from $d(R^{i:(y,x)},R^\prime )<k$ and the induction hypothesis that $f(R^{i:(y,x)})=f(R^\prime )$. Hence, $f(R)=f(R^\prime )$,

Now, for the proof of the statement of the theorem, assume for contradiction that $f$ is not Kelly-strategyproof. Then, there have to be $R,R^\prime \in \fancyscript{D}$, and $i\in N$ with $R_{-i}=R^\prime _{-i}$ such that $f(R^\prime )\mathrel {\widehat{P}_{i}} f(R)$. The latter obviously entails that $f(R)\ne f(R^\prime )$. The proof idea is to find some $R^*$ in the comprehensive closure of $R$ and $R^\prime$ (and hence in $\fancyscript{D}$) such that $R^*$ is an $f$-improvement over both $R$ and $R^\prime$, which implies that $f(R)=f(R^\prime )$, a contradiction.

Let $m\in f(R^\prime )$ such that $L(m,P_i)\cap f(R^\prime )=\emptyset$. In other words, $m$ is the alternative in $f(R^\prime )$ that is ranked lowest in $R_i$. Note that, if $f(R)\cap f(R^\prime )\ne \emptyset$, then $f(R)\cap f(R^\prime ) = \{m\}$. Next, we partition $A$ into the strict lower contour set and the upper contour set of $m$ with respect to $R_i$, i.e., $L = L(m,P_i)$ and $U=A{\setminus } L$ and define a new preference profile by letting

$$\begin{aligned} R^*_i = R_i|_L \cup R^\prime _i|_U \cup \{(x,y):x\in U, y\in L\}, \end{aligned}$$

i.e., the upper part of $R_i^*$ is ranked as in $R_i^\prime$ and the lower part as in $R_i$, and $R^*=(R_{-i},R_i^*)=(R^\prime _{-i},R_i^*)$.

$R^*$ lies in the comprehensive closure of $R$ and $R^\prime$ (and hence in $\fancyscript{D}$) because for all $x,y\in A$ with $x\mathrel {R_i^*} y$, we have $x\mathrel {R_i} y$ or $x\mathrel {R^\prime _i} y$. For $x,y\in U$, this follows from $R^*_i|_U = R'_i|_U$; for $x,y\in L$, from $R^*_i|_L = R_i|_L$; and for $x\in U, y\in L$ from $(x,y)\in R_i$.

$R^*$ is an $f$-improvement over $R$ because $f(R)\subseteq L\cup \{m\}$ and $L(x,P^*_i)=L(x,P_i)$ for all $x\in L$ and $L(m,P^*_i)\supseteq L(m,P_i)=L$. Set-monotonicity then implies that $f(R^*)=f(R)$. $R^*$ is an $f$-improvement over $R'$ because $f(R^\prime )\subseteq U$ and $L(x,P^*_i)=L(x,P^\prime _i)\cup L$ for all $x\in U$. Hence, set-monotonicity implies that $f(R^*)=f(R^\prime )\ne f(R)$, a contradiction.$\square$

We conclude the paper with nine remarks.

Remark 1

(Set-monotonic SCFs) There are a number of rather attractive SCFs that satisfy set-monotonicity in the general domain $\fancyscript{L}^N$. In particular, every monotonic SCF that satisfies the strong superset property (SSP) (i.e., choice sets are invariant under the removal of unchosen alternatives) also satisfies set-monotonicity.⁶ Prominent Condorcet extensions that satisfy both SSP and monotonicity include the Condorcet rule (which selects a Condorcet winner whenever one exists and returns all alternatives otherwise), the top cycle (also known as weak closure maximality, GETCHA, or the Smith set) (Good 1971; Smith 1973; Bordes 1976; Sen 1977; Schwartz 1986), the minimal covering set (Dutta 1990), the bipartisan set (Laffond et al. 1993) and variations of these (see Laslier 1997; Dutta and Laslier 1999; Laslier 2000; Brandt 2011).⁷ $^{,}$ ⁸ Two other SCFs that satisfy set-monotonicity are the Pareto rule and the omninomination rule (which returns all alternatives that are top-ranked by at least one agent).

Remark 2

(Coarsenings of Kelly-strategyproof SCFs) For two SCFs $f,f^\prime$, we say that $f$ is a coarsening of $f^\prime$ if $f^\prime (R)\subseteq f(R)$ for all $R\in \fancyscript{D}$. Kelly’s preference extension has the useful property that $X \mathrel {\widehat{P}_i} Y$ implies $X^\prime \mathrel {\widehat{P}_i} Y^\prime$ for all non-singleton subsets $X^\prime \subseteq X$ and $Y^\prime \subseteq Y$. Hence, every coarsening $f$ of a Kelly-strategyproof SCF $f^\prime$ is Kelly-strategyproof if $f(R)=f^\prime (R)$ whenever $|f^\prime (R)|=1$. As a consequence, the (McKelvey) uncovered set (see Duggan 2013), a coarsening of the minimal covering set that returns singletons if and only if there is a Condorcet winner, is Kelly-strategyproof, even though it violates set-monotonicity.⁹ In light of these comments, it seems interesting to try to identify inclusion-minimal Kelly-strategyproof SCFs.

Remark 3

(Group-strategyproofness) The proof of Theorem 1 can be straightforwardly extended to show that no group of agents can misstate their preferences in order to obtain a more preferred outcome.

Remark 4

(Fishburn-strategyproofness) It has been shown in other work that Theorem 1 does not carry over to slightly more complete set extensions due to Fishburn and Gärdenfors (Brandt and Brill 2011). In fact, Pareto-optimality and Fishburn-strategyproofness are already incompatible within the class of majoritarian SCFs (Brandt and Geist 2014).

Remark 5

(Necessary conditions) It seems like there are no natural necessary conditions for Kelly-strategyproofness as long as preferences are linear. For example, any SCF $f$ such that $|f(R)|>(|A|/2)+1$ for all $R\in \fancyscript{D}$ satisfies Kelly-strategyproofness simply because no pair of resulting choice sets is comparable. This observation allows one to easily construct Kelly-strategyproof SCFs that violate set-monotonicity, Maskin-monotonicity, or any other reasonable form of monotonicity.

Remark 6

(Weak preferences) When individual preference relations do not have to be antisymmetric, a number of results mentioned in the introduction rule out the possibility of reasonable Kelly-strategyproof SCFs. A new result of this kind, which strengthens some existing theorems, is given as Theorem 2 in the Appendix.

The proof of Theorem 1 can be adapted for weak preferences to show that set-monotonicity implies Nehring’s generalized strategyproofness, a weakening of Kelly-strategyproofness (Nehring 2000).¹⁰ Generalized strategyproofness is defined by letting agent $i$ prefer set $X$ to set $Y$ if and only if $x \mathrel {P_i} y$ for all $x\in X$ and $y\in Y$.

Remark 7

(Matching markets) Theorem 1 can be straightforwardly extended to domains in which the indifference relation is fixed for each agent. This is, for example, the case in matching markets where each agent is typically assumed to be indifferent between all matchings in which his assignment is identical.

Remark 8

(Connected domains) Theorem 1 does not hold for a weakening of comprehensiveness, which Nehring (2000) refers to as connectedness. A domain $\fancyscript{D}$ is connected if for all $i\in N$, $R_i,R_i^\prime \in \fancyscript{D}_i$, the following holds: if there is some $R_i^{\prime \prime } \in \fancyscript{L}{\setminus }\{R_i,R_i^\prime \}$ with $R\cap R^\prime \subseteq R^{\prime \prime }$, then there is some $R_i^{\prime \prime }\in \fancyscript{D}_i{\setminus }\{R_i,R_i^\prime \}$ with $R\cap R^\prime \subseteq R^{\prime \prime }$. The following single-agent SCF $f$, defined on a connected—but not comprehensive—domain of size three, has been adapted from Nehring (2000) and satisfies set-monotonicity while it violates Kelly-strategyproofness:

$$\begin{aligned}&R_1 :a, b, c, d, \quad f((R_1))=\{c\},\\&R^\prime _1 :a, b, d, c, \quad f((R^\prime _1))=\{a,b,c,d\},\\&R^{\prime \prime }_1 :b, a, d, c, \quad f((R^{\prime \prime }_1))=\{b\}. \end{aligned}$$

Remark 9

(Strategic abstention) Fishburn and Brams (1983) introduced a particularly natural variant of strategic manipulation where agents obtain a more preferred outcome by abstaining the election. Moulin (1988) has shown that every resolute Condorcet extension can be manipulated in this way and thus suffers from the so-called no-show paradox. However, Moulin’s proof strongly relies on resoluteness. If preferences over sets are given by Kelly’s extension, set-valued Condorcet extension that satisfy Kelly-strategyproofness also cannot be manipulated by abstention under very mild conditions. This is, for instance, the case for all SCFs whose outcome only depends on pairwise majority margins (which covers all of the Condorcet extensions mentioned in Remark 1) because any manipulation by abstention can be turned into a manipulation by strategic misrepresentation.¹¹

Appendix

For the domain of transitive and complete (but not necessarily antisymmetric) preference relations $\fancyscript{R}^N$, we show that all Condorcet extensions are Kelly-manipulable. This strengthens Theorem 3 by Gärdenfors (1976) and Theorem 8.1.2 by Taylor (2005), who showed the same statement for a weaker notion of manipulability and a weaker notion of Condorcet winners, respectively. When assuming that pairwise choices are made according to majority rule, this also strengthens Theorems 1 and 2 by MacIntyre and Pattanaik (1981). However, our construction requires that the number of agents is linear in the number of alternatives.

Theorem 2

No Condorcet extension is Kelly-strategyproof in domain $\fancyscript{R}^N$ when there are more than two alternatives.

Proof

Let $A=\{a_1,\dots ,a_m\}$ with $m\ge 3$ and consider the following preference profile $R$ with $3m$ agents. In the representation below, sets denote indifference classes of the agents.

For every alternative $a_i$, there are two agents who prefer every alternative to $a_i$ and are otherwise indifferent. Moreover, for every alternative $a_i$ there is one agent who prefers every alternative except $a_{i+1}$ to $a_i$, ranks $a_{i+1}$ below $a_i$, and is otherwise indifferent.

Since $f(R)$ yields a nonempty choice set, there has to be some $a_i\in f(R)$. Due to the symmetry of the preference profile, we may assume without loss of generality that $a_2\in f(R)$. Now, let

$$\begin{aligned} R_3^\prime ,R_4^\prime :a_1, \{a_3,\ldots ,a_m\},a_2 \end{aligned}$$

and define $R^\prime =(R_{-3},R^\prime _3)$ and $R^{\prime \prime }=(R^\prime _{-4},R^\prime _4)$. That is, $R^\prime$ is identical to $R$, except that agent 3 lifted $a_1$ on top and $R^{\prime \prime }$ is identical to $R^\prime$, except that agent 4 lifted $a_1$ on top. Observe that $f(R^{\prime \prime })=\{a_1\}$ because $a_1$ is the Condorcet winner in $R^{\prime \prime }$.

In case that $a_2\not \in f(R^\prime )$, agent 3 can manipulate as follows. Suppose $R$ is the true preference profile. Then, the least favorable alternative of agent 3 is chosen (possibly among other alternatives). He can misstate his preferences as in $R^\prime$ such that $a_2$ is not chosen. Since he is indifferent between all other alternatives, $f(R^\prime ) \mathrel {\widehat{P}_3} f(R)$.

If $a_2\in f(R^\prime )$, agent 4 can manipulate similarly. Suppose $R'$ is the true preference profile. Again, the least favorable alternative of agent 4 is chosen. By misstating his preferences as in $R^{\prime \prime }$, he can assure that one of his preferred alternatives, namely $a_1$, is selected exclusively because it is the Condorcet winner in $R^{\prime \prime }$. Hence, $f(R^{\prime \prime }) \mathrel {\widehat{P}^\prime _4} f(R^\prime )$.$\square$