1 Introduction

Nowadays, functionally graded materials (FGMs) are widely used and applied in various areas of engineering, industry, and technology for macro-scale structures, micro-scale structures, and nano-scale structures (Reddy [1], Neves et al. [2], Swaminathan et al. [3], Lee et al. [4], Yan and Liang et al. [5, 6], Kumar et al. [7], Sahmani et al. [8], and Vinh et al. [9,10,11]). Therefore, understanding the mechanical and thermal behaviors of these structures became one of the most challenges of researchers. For example, Natarajan et al. [12] studied the vibration and static bending of functionally graded (FG) sandwich plates using an accurate theory. Neves et al. [13] analyzed the static bending and free vibration of FG plates via a quasi-3D theory with sinusoidal shear deformation shape function. Zenkour [14] presented a comprehensive analysis of FG sandwich plates using higher-order shear deformation theory (HSDT). In another work [15], he developed a simple four-unknown refined theory for bending analysis of FG plates. Thai et al. [16, 17] studied the bending and free vibration behaviors of FG plates using first-order shear deformation theory (FSDT) and the HSDT. Demirhan et al. [18] developed a Levy solution based on a four-unknown plate theory for the bending analysis of FG sandwich plates. Vinh et al. [19] developed a single variable shear deformation theory for free vibration analysis of rectangular FG plates. Pandey et al. [20] studied the mechanical behaviors of FG sandwich plates using higher-order layerwise theory. The porosity usually appears due to the fabrication of FGMs; they strongly affect the thermal and mechanical responses of these structures. Rezaei et al. [21] investigated the free vibration of FG plates with porosity using a simple four-unknown plate theory and an analytical approach. Akbas [22] analyzed the free vibration and static bending of the FG plates with porosity. Riadh et al. [23] analyzed the free vibration response of FG porous plates using an HSDT and normal deformation theory. Zeng et al. [24] studied the nonlinear vibration of piezoelectric sandwich nanoplates with FG porous core. Vinh et al. [25] studied the mechanical behaviors of FG sandwich plates using the HSDT and finite element method. Pradhan et al. [26] studied the free vibration of FG cylindrical shells with various boundary conditions (BCs). Woo et al. [27] studied the nonlinear behavior of FG plates and shallow shells. Khare et al. [28] studied the free vibration of composite and sandwich laminates using higher-order facet shell elements. Fadaee et al. [29] developed a Levy type solution for free vibration analysis of the FG spherical shell panel. Amabili [30] and Alijani et al. [31] analyzed nonlinear vibration of doubly curved shallow shells. Jouneghani et al. [32] studied the free vibration of the FG doubly curved shells using the FSDT. Santos et al. [33] developed a semi-analytical finite element model for the analysis of FG cylindrical shells. Viola et al. [34] developed a generalized unconstrained third-order shear deformation theory (TSDT) for the static bending analysis of FG conical shells and panels. Wattanasakulpong et al. [35] analyzed the free vibration of the FG doubly curved shells with stiffeners embedded in the thermal environment. Tornabene et al. [36] studied the effects of agglomeration on the free vibration of FG carbon-nanotube-reinforced laminated composite doubly curved shells. Punera et al. [37, 38] studied the free vibration of FG open cylindrical shells and laminated cylindrical shells using several HSDTs. Aliyari et al. [39] applied the differential quadrature method (DQM) to analyze the static bending and free vibration of sandwich cylindrical shells with FG core and viscoelastic interface. The bending and free vibration analysis of isotropic and sandwich FG doubly curved shallow shells had been investigated by Chen et al. [40], Wang et al. [41], Arefi et al. [42], and Szekrenyes et al. [43] using several HSDTs. The dynamic stability of bi-directional FG porous cylindrical shells embedded in an elastic foundation had been studied by Allahkarami et al. [44] using the TSDT. Liu et al. [45] investigated the free vibration of FG sandwich shallow shells in thermal environments using a differential quadrature hierarchical finite element method.

The thermal and mechanical behaviors of micro-scale structures and nano-scale structures were also investigated by many researchers using many higher-order continuum theories. Eringen et al. [46, 47] developed a nonlocal elasticity theory for the analysis of nanostructures. In the nonlocal elasticity theory, the stress at any point depends on all neighbor points of solid. The modified couple stress theory was applied to analyze microstructures by Zare et al. [48], Jouneghani et al. [48], Faleh et al. [49], and Razavi et al. [50]. The strain gradient theory and nonlocal strain gradient theory (Karami et al. [51,52,53,54], Sahmani et al. [55], and Shariati et al. [56]), and doublet mechanics (Eltaher et al. [57]) were also developed to analyze nanostructures. Among them, the nonlocal elasticity theory has been employed to analyze the nanobeams, nanoplates, and nanoshells by many researchers. Reddy [58] applied nonlocal elasticity theory to analyze the bending, free vibration, and buckling of nanobeams with various beam theories including the Euler–Bernoulli, Timoshenko, Reddy, and Levison beam theories. Natarajan et al. [59] studied size-dependent free vibration behavior of FG nanoplates. Aghababaei et al. [60] developed nonlocal TSDT and applied it to analyze the bending and free vibration of nanoplates. Aksencer et al. [61] applied the Levy type solution in combination with nonlocal elasticity theory to study the vibration and buckling of nanoplates. Ansari et al. [62] presented the vibration characteristics of multi-layered graphene sheets. Nazemnezhad et al. [63] analyzed nonlocal nonlinear free vibration of FG nanobeams. Belarbi et al. [64] developed a nonlocal finite element model for the static bending and buckling analysis of FG nanobeams using novel shear deformation theory. Ghandourah et al. [65] investigated the dynamic response of FG nonlocal nanobeam with different porosity models. Thai et al. [66] developed a nonlocal sinusoidal plate theory for micro/nanoscale plate analysis. Hoa et al. [67] analyzed the bending and free vibration of FG nanoplates using a novel single variable shear deformation theory. Daneshmehr et al. [68] analyzed size-dependent free vibration of nanoplates via HSDT and nonlocal elasticity theory. Anjomshoa et al. [69] analyzed vibration of circular and elliptical nanoplates resting on an elastic foundation via the nonlocal Mindlin plate theory and Galerkin method. Mechab et al. [70] examined the free vibration of FG porous nanoplates resting on Winkler-Pasternak elastic foundations via a two-variable refined plate theory. Sobhy [71] developed a nonlocal quasi-3D theory for the vibration and buckling analysis of FG nanoplates. Arefi et al. [72, 73] applied nonlocal elasticity theory in combination with several HSDTs and finite element methods to analyze the free vibration and buckling behaviors of FG nanobeams, piezoelectric doubly curved shallow nanoshells.

Numerous works have been done on the investigation of the thermal and mechanical behaviors of the micro/nanostructures using the derivative form of the nonlocal elasticity theory. However, the derivative form of the nonlocal elasticity theory is established based on an assumption that the nonlocal parameter is constant throughout the body (Eringen [47]), therefore, it is capable only for analysis of homogeneous isotropic nanostructures. It should be noticed that the FGMs are inhomogeneous materials, therefore, the material components vary throughout the body. Hence, the material properties including nonlocal parameters of FG structures should be the material-dependent properties (Vinh et al. [74,75,76,77], Salehipour et al. [78], Batra [79]). This is also the main goal of this study to modify the nonlocal elasticity theory, so the spatial variation of the nonlocal parameters through the thickness of the FG structures is considered. Then, a combination of the FSDT and nonlocal elasticity theory with spatial variation nonlocal parameters is established to analyze free vibration of the FG doubly curved shallow nanoshells with porosity. A comprehensive analysis of the effects of some parameters on the free vibration of the FG doubly curved shallow nanoshells is carried out in detail.

2 Functionally graded porous doubly curved shallow nanoshells

2.1 Functionally graded porous materials

In this study, a doubly curved shallow nanoshell is made of FG that is a combination of metal and ceramic phases. The volume fractions of metal and ceramic vary through the thickness of the shells. The effective material properties of the FG perfect shells, such as Young’s modulus, mass density and Poisson’s ratio, are calculated as the following formula:

$$\begin{gathered} E(z) = E_{{\text{c}}} V_{{\text{c}}} (z) + E_{{\text{m}}} V_{{\text{m}}} (z), \hfill \\ \rho (z) = \rho_{{\text{c}}} V_{{\text{c}}} (z) + \rho_{{\text{m}}} V_{{\text{m}}} (z), \hfill \\ \nu (z) = \nu_{{\text{c}}} V_{{\text{c}}} (z) + \nu_{{\text{m}}} V_{{\text{m}}} (z), \hfill \\ \end{gathered}$$
(1)

where \(V_{{\text{c}}}\) and \(V_{{\text{m}}}\) are, respectively, the volume fraction of ceramic and metal components, which are calculated as follows:

$$\begin{gathered} V_{{\text{c}}} (z) = \left( {\frac{z}{h} + \frac{1}{2}} \right)^{k} , \hfill \\ V_{{\text{m}}} (z) = 1 - V_{{\text{c}}} (z), \hfill \\ \end{gathered}$$
(2)

where \(h\) is the thickness of the shell, and \(k\) is the power-law index of the volume fraction.

In the present study, two kinds of porosity distribution, including even, and uneven porosities, are considered.

2.1.1 Imperfect FG nanoshell with even porosity distribution

In the case of even porosity distribution, the effective material properties are calculated as:

$$\begin{gathered} E(z) = E_{{\text{c}}} V_{{\text{c}}} (z) + E_{{\text{m}}} V_{{\text{m}}} (z) - \frac{\xi }{2}\left( {E_{{\text{c}}} + E_{{\text{m}}} } \right), \hfill \\ \rho (z) = \rho_{{\text{c}}} V_{{\text{c}}} (z) + \rho_{{\text{m}}} V_{{\text{m}}} (z) - \frac{\xi }{2}\left( {\rho_{{\text{c}}} + \rho_{{\text{m}}} } \right), \hfill \\ \nu (z) = \nu_{{\text{c}}} V_{{\text{c}}} (z) + \nu_{{\text{m}}} V_{{\text{m}}} (z) - \frac{\xi }{2}\left( {\nu_{{\text{c}}} + \nu_{{\text{m}}} } \right), \hfill \\ \end{gathered}$$
(3)

where \(\xi\) is the porosity coefficient.

2.1.2 Imperfect FG nanoshell with uneven porosity distribution

For uneven porosity distribution, the effective material properties are calculated as follows:

$$\begin{gathered} E(z) = E_{{\text{c}}} V_{{\text{c}}} (z) + E_{{\text{m}}} V_{{\text{m}}} (z) - \frac{\xi }{2}\left( {E_{{\text{c}}} + E_{{\text{m}}} } \right)\left( {1 - \frac{2\left| z \right|}{h}} \right), \hfill \\ \rho (z) = \rho_{{\text{c}}} V_{{\text{c}}} (z) + \rho_{{\text{m}}} V_{{\text{m}}} (z) - \frac{\xi }{2}\left( {\rho_{{\text{c}}} + \rho_{{\text{m}}} } \right)\left( {1 - \frac{2\left| z \right|}{h}} \right), \hfill \\ \nu (z) = \nu_{{\text{c}}} V_{{\text{c}}} (z) + \nu_{{\text{m}}} V_{{\text{m}}} (z) - \frac{\xi }{2}\left( {\nu_{{\text{c}}} + \nu_{{\text{m}}} } \right)\left( {1 - \frac{2\left| z \right|}{h}} \right), \hfill \\ \end{gathered}$$
(4)

where \(\xi\) is the porosity coefficient.

2.2 Doubly curved shallow nanoshell model

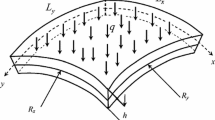
In this study, an FG doubly curved shallow nanoshell that is defined in a curvilinear orthogonal coordinate system \(Oxyz\) is considered. In which, \(Ox\) and \(Oy\) refer to the principal lines of curvature on the middle surface (\(z = 0\)), \(Oz\) is perpendicular to the middle surface itself. The principal radius of the shells with respect to \(x\)-axes is \(R_{1}\) and the principal radius with respect to \(y\)-axes is \(R_{2}\). The geometry of the FG doubly curved shallow nanoshells is exhibited in Fig. 1.

Fig. 1
figure 1

The geometry of the FG doubly curved shallow nanoshells with porosity

Full size image

By changing the components of curvature of the shells, some special types of shell structure can be achieved as follows:

For \(R_{1} = R_{2} \to \infty\): the shell becomes a flat plate (FL plate);

For \(R_{1} = R_{2}\): the shell becomes a spherical shell (SP shell);

For \(R_{1} = - R_{2}\): the shell becomes hyperbolic parabolic shell (HP shell);

For \(R_{2} \to \infty\): the shell becomes a cylindrical shell (CY shell).

These four types of shell structures are examined in this study and they are illustrated in Fig. 2.

Fig. 2
figure 2

Four types of the FG doubly curved shallow nanoshells

Full size image

3 Theoretical formulation

3.1 Kinematics of the present model

3.1.1 Displacement field

Based on the first-order shear deformation theory (FSDT), the displacement field for the FG porous doubly curved shallow nanoshells can be expressed as follows:

$$\begin{gathered} u(x,y,z,t) = u_{0} (x,y,t) + z\varphi_{x} (x,y,t) \hfill \\ v(x,y,z,t) = v_{0} (x,y,t) + z\varphi_{y} (x,y,t) \hfill \\ w(x,y,z,t) = w_{0} (x,y,t). \hfill \\ \end{gathered}$$
(5)

In which \(u_{0} ,\;v_{0} ,\) and \(w_{0}\) denote, respectively, in-plane and transverse displacement components at the mid-plane of the FG porous doubly curved shallow nanoshells; \(\varphi_{x}\) and \(\varphi_{y}\) are, respectively, the rotations of a transverse normal about the y-axis and x-axis.

3.1.2 Strain–displacement relations

In the case of shallow shells, the strain–displacement relations of the FG porous doubly curved shallow nanoshells should consider the effects of the curvature components. In this study, two Lamé parameters \(A_{1}\) and \(A_{2}\) are assumed as \(A_{1} = A_{2} = 1\), that related to the correspondence between curvilinear abscissae and principal coordinates. Therefore, the strain fields of the shells are expressed as follows (Jouneghani et al. [32]):

$$\begin{gathered} \varepsilon_{x} = \frac{1}{{r_{1} }}e_{x}^{0} + \frac{z}{{r_{1} }}\kappa_{x} , \hfill \\ \varepsilon_{y} = \frac{1}{{r_{2} }}e_{y}^{0} + \frac{z}{{r_{1} }}\kappa_{y} , \hfill \\ \gamma_{xy} = \frac{1}{{r_{2} }}e_{xy}^{0} + \frac{1}{{r_{1} }}e_{yx}^{0} + \frac{z}{{r_{2} }}\kappa_{xy}^{0} + \frac{z}{{r_{1} }}\kappa_{yx}^{0} , \hfill \\ \gamma_{xz} = \gamma_{xz}^{0} , \hfill \\ \gamma_{yz} = \gamma_{yz}^{0} , \hfill \\ \end{gathered}$$
(6)

where,

$$\begin{gathered} e_{x}^{0} = \frac{\partial u}{{\partial x}} + \frac{w}{{R_{1} }},\;e_{y}^{0} = \frac{\partial v}{{\partial y}} + \frac{w}{{R_{2} }},\;e_{xy}^{0} = \frac{\partial u}{{\partial y}},\;e_{yx}^{0} = \frac{\partial v}{{\partial x}}, \hfill \\ \kappa_{x} = \frac{{\partial \varphi_{x} }}{\partial x},\;\kappa_{y} = \frac{{\partial \varphi_{y} }}{\partial y},\;\kappa_{xy} = \frac{{\partial \varphi_{x} }}{\partial y},\;\kappa_{yx} = \frac{{\partial \varphi_{y} }}{\partial x}, \hfill \\ \gamma_{xz}^{0} = \varphi_{x} + \frac{\partial w}{{\partial x}} - \frac{u}{{R_{1} }},\;\gamma_{yz}^{0} = \varphi_{y} + \frac{\partial w}{{\partial y}} - \frac{v}{{R_{2} }}. \hfill \\ \end{gathered}$$
(7)

In which \(r_{1} = 1 + \frac{z}{{R_{1} }},\;r_{2} = 1 + \frac{z}{{R_{2} }}\).

3.2 The nonlocal constitutive relations

To take into account the small-scale effects on micro-/nanostructure behaviors, Eringen [46, 47] developed a nonlocal elasticity theory in both integral and differential forms. The differential form of the nonlocal elasticity theory is achieved from the integral form based on an assumption of a constant nonlocal parameter. The expression of the nonlocal elasticity theory in a derivative form is as follows:

$$\left( {1 - \mu \nabla^{2} } \right)\sigma_{ij} = t_{ij} ,$$
(8)

where \(\sigma_{ij} ,\;t_{ij}\) are respectively the nonlocal and local stress tensors, \(\nabla^{2} = {{\partial^{2} } \mathord{\left/ {\vphantom {{\partial^{2} } {\partial x^{2} }}} \right. \kern-\nulldelimiterspace} {\partial x^{2} }} + {{\partial^{2} } \mathord{\left/ {\vphantom {{\partial^{2} } {\partial y^{2} }}} \right. \kern-\nulldelimiterspace} {\partial y^{2} }}\) is the second Laplace operator, \(\mu = \left( {e_{0} a} \right)^{2} \;({\text{nm}}^{2} )\) is the nonlocal parameter. In which \(e_{0}\) is a material constant which is determined via experimental or atomistic dynamic, \(a\) is an internal characteristic length. The classical nonlocal elasticity theory in the differential form is only compatible for the analysis of isotropic homogeneous nanostructures. In the cases of FG structures, the material properties, including nonlocal parameter, vary through the thickness of the structures as well. Thus, the variation of the nonlocal parameter must be considered. By making a further assumption of the variation of the nonlocal parameter through the thickness direction of the FG doubly curved shallow nanoshells, the nonlocal constitutive relations of the shells can be expressed as follows:

$$\left\{ \begin{gathered} t_{x} \hfill \\ t_{y} \hfill \\ t_{xy} \hfill \\ \end{gathered} \right\} = \left( {1 - \mu (z)\nabla^{2} } \right)\left\{ \begin{gathered} \sigma_{x} \hfill \\ \sigma_{y} \hfill \\ \tau_{xy} \hfill \\ \end{gathered} \right\} = \left[ {\begin{array}{*{20}c} {C_{11} } & {C_{12} } & 0 \\ {C_{21} } & {C_{22} } & 0 \\ 0 & 0 & {C_{66} } \\ \end{array} } \right]\left\{ \begin{gathered} \varepsilon_{x} \hfill \\ \varepsilon_{y} \hfill \\ \gamma_{xy} \hfill \\ \end{gathered} \right\},$$
(9)
$$\left\{ \begin{gathered} t_{yz} \hfill \\ t_{xz} \hfill \\ \end{gathered} \right\} = \left( {1 - \mu (z)\nabla^{2} } \right)\left\{ \begin{gathered} \tau_{yz} \hfill \\ \tau_{xz} \hfill \\ \end{gathered} \right\} = k_{{\text{s}}} \left[ {\begin{array}{*{20}c} {C_{44} } & 0 \\ 0 & {C_{55} } \\ \end{array} } \right]\left\{ \begin{gathered} \gamma_{yz} \hfill \\ \gamma_{xz} \hfill \\ \end{gathered} \right\},$$
(10)

where \(k_{{\text{s}}} = 5/6\) is the shear correction factor, and

$$C_{11} = C_{22} = \frac{E(z)}{{1 - \nu (z)^{2} }},\;C_{12} = C_{21} = \frac{\nu (z)E(z)}{{1 - \nu (z)^{2} }},\;C_{44} = C_{55} = C_{66} = \frac{E(z)}{{2\left( {1 + \nu (z)} \right)}}.$$
(11)

The nonlocal parameter is assumed to vary through the thickness of the shells as other material properties, therefore, the effective nonlocal parameter of the FG nanoshells is calculated as follows:

$$\mu (z) = \mu_{{\text{c}}} V_{{\text{c}}} (z) + \mu_{{\text{m}}} V_{{\text{m}}} (z).$$
(12)

This is the main novelty of the present work in comparison to other available works on the analysis of functionally graded nanostructures. The modified nonlocal elasticity theory relegated to classical nonlocal elasticity theory when \(\mu (z) = \mu_{{\text{m}}} = \mu_{{\text{c}}} = \mu = {\text{const}}\).

3.3 Equations of motion

In this work, Hamilton’s principle is employed to obtain the governing equation of motion for the free vibration analyses of FG porous doubly curved shallow nanoshell, which is given as:

$$0 = \int_{0}^{T} {\left( {\delta U - \delta K} \right){\text{d}}t} ,$$
(13)

where \(\delta U\) is the variation of the strain energy and \(\delta K\) is the variation of the kinetic energy of the FG doubly curved shallow nanoshells. The expression of the first variation of the strain energy is given by:

$$\delta U = \int\limits_{A}^{{}} {\int\limits_{ - h/2}^{h/2} {\left( {\sigma_{x} \delta \varepsilon_{x} + \sigma_{y} \delta \varepsilon_{y} + \tau_{xy} \delta \gamma_{xy} + \tau_{xz} \delta \gamma_{xz} + \tau_{yz} \delta \gamma_{yz} } \right)r_{1} r_{2} {\text{d}}z{\text{d}}x{\text{d}}y} } .$$
(14)

The first variation of the kinetic energy of the shells is computed as the following formula:

$$\delta K = \int\limits_{A} {\int\limits_{ - h/2}^{h/2} {\left( {\dot{u}\delta \dot{u} + \dot{v}\delta \dot{v} + \dot{w}\delta \dot{w}} \right)\rho (z){\text{d}}z{\text{d}}A} } .$$
(15)

By introducing the Eq. (6) into Eq. (14), and substituting Eq. (5) into Eq. (15) with considering the nonlocal relations of Eqs. (9) and (10), the governing equations of motion of the FG doubly curved shallow nanoshells are derived from Eq. (13) as follows

$$\begin{gathered} \delta u:\;\frac{{\partial N_{x} }}{\partial x} + \frac{{\partial N_{xy} }}{\partial y} + \frac{{Q_{x} }}{{R_{1} }} = I_{0} \ddot{u} + I_{1} \ddot{\varphi }_{x} - \nabla^{2} \left( {J_{0} \ddot{u} + J_{1} \ddot{\varphi }_{x} } \right), \hfill \\ \delta v:\;\frac{{\partial N_{y} }}{\partial y} + \frac{{\partial N_{yx} }}{\partial x} + \frac{{Q_{y} }}{{R_{2} }} = I_{0} \ddot{v} + I_{1} \ddot{\varphi }_{y} - \nabla^{2} \left( {J_{0} \ddot{v} + J_{1} \ddot{\varphi }_{y} } \right), \hfill \\ \delta w:\frac{{\partial Q_{x} }}{\partial x} + \frac{{\partial Q_{y} }}{\partial y} - \frac{{N_{x} }}{{R_{1} }} - \frac{{N_{y} }}{{R_{2} }} = I_{0} \ddot{w} - \nabla^{2} \left( {J_{0} \ddot{w}} \right), \hfill \\ \delta \varphi_{x} :\frac{{\partial M_{x} }}{\partial x} + \frac{{\partial M_{xy} }}{\partial y} - Q_{x} = I_{1} \ddot{u} + I_{2} \ddot{\varphi }_{x} - \nabla^{2} \left( {J_{1} \ddot{u} + J_{2} \ddot{\varphi }_{x} } \right), \hfill \\ \delta \varphi_{y} :\frac{{\partial M_{y} }}{\partial y} + \frac{{\partial M_{yx} }}{\partial x} - Q_{y} = I_{1} \ddot{v} + I_{2} \ddot{\varphi }_{y} - \nabla^{2} \left( {J_{1} \ddot{v} + J_{2} \ddot{\varphi }_{y} } \right), \hfill \\ \end{gathered}$$
(16)

where \(N_{i} ,\;M_{i}\) and \(Q_{i}\) are the local stress resultants which are calculated by

$$\left\{ \begin{gathered} N_{x} \hfill \\ N_{y} \hfill \\ N_{xy} \hfill \\ N_{yx} \hfill \\ \end{gathered} \right\} = \left[ {\begin{array}{*{20}c} {A_{11} } & {A_{12} } & 0 & 0 \\ {A_{21} } & {A_{22} } & 0 & 0 \\ 0 & 0 & {A_{66}^{11} } & {A_{66}^{12} } \\ 0 & 0 & {A_{66}^{21} } & {A_{66}^{22} } \\ \end{array} } \right]\left\{ \begin{gathered} e_{x}^{0} \hfill \\ e_{y}^{0} \hfill \\ e_{xy}^{0} \hfill \\ e_{yx}^{0} \hfill \\ \end{gathered} \right\} + \left[ {\begin{array}{*{20}c} {B_{11} } & {B_{12} } & 0 & 0 \\ {B_{21} } & {B_{22} } & 0 & 0 \\ 0 & 0 & {B_{66}^{11} } & {B_{66}^{12} } \\ 0 & 0 & {B_{66}^{21} } & {B_{66}^{22} } \\ \end{array} } \right]\left\{ \begin{gathered} \kappa_{x}^{{}} \hfill \\ \kappa_{y}^{{}} \hfill \\ \kappa_{xy}^{{}} \hfill \\ \kappa_{yx}^{{}} \hfill \\ \end{gathered} \right\},$$
(17)
$$\left\{ \begin{gathered} M_{x} \hfill \\ M_{y} \hfill \\ M_{xy} \hfill \\ M_{yx} \hfill \\ \end{gathered} \right\} = \left[ {\begin{array}{*{20}c} {B_{11} } & {B_{12} } & 0 & 0 \\ {B_{21} } & {B_{22} } & 0 & 0 \\ 0 & 0 & {B_{66}^{11} } & {B_{66}^{12} } \\ 0 & 0 & {B_{66}^{21} } & {B_{66}^{22} } \\ \end{array} } \right]\left\{ \begin{gathered} e_{x}^{0} \hfill \\ e_{y}^{0} \hfill \\ e_{xy}^{0} \hfill \\ e_{yx}^{0} \hfill \\ \end{gathered} \right\} + \left[ {\begin{array}{*{20}c} {D_{11} } & {D_{12} } & 0 & 0 \\ {D_{21} } & {D_{22} } & 0 & 0 \\ 0 & 0 & {D_{66}^{11} } & {D_{66}^{12} } \\ 0 & 0 & {D_{66}^{21} } & {D_{66}^{22} } \\ \end{array} } \right]\left\{ \begin{gathered} \kappa_{x}^{{}} \hfill \\ \kappa_{y}^{{}} \hfill \\ \kappa_{xy}^{{}} \hfill \\ \kappa_{yx}^{{}} \hfill \\ \end{gathered} \right\},$$
(18)
$$\left\{ {\begin{array}{*{20}c} {Q_{x} } \\ {Q_{y} } \\ \end{array} } \right\} = \left[ {\begin{array}{*{20}c} {S_{55} } & 0 \\ 0 & {S_{44} } \\ \end{array} } \right]\left\{ \begin{gathered} \gamma_{xz}^{0} \hfill \\ \gamma_{yz}^{0} \hfill \\ \end{gathered} \right\},$$
(19)

where

$$\begin{gathered} \left( {A_{11} ,\;A_{12} ,\;A_{21} ,A_{22} } \right) = \int\limits_{ - h/2}^{h/2} {\left( {\frac{{r_{2} }}{{r_{1} }}C_{11} ,\;C_{12} ,\;C_{21} ,\;\frac{{r_{1} }}{{r_{2} }}C_{22} } \right){\text{d}}z} , \hfill \\ \left( {A_{66}^{11} ,\;A_{66}^{12} ,\;A_{66}^{21} ,\;A_{66}^{22} } \right) = \int\limits_{ - h/2}^{h/2} {\left( {\frac{{r_{1} }}{{r_{2} }}C_{66} ,\;C_{66} ,\;\;C_{66} ,\;\frac{{r_{2} }}{{r_{1} }}C_{66} } \right){\text{d}}z} , \hfill \\ \left( {B_{11} ,\;B_{12} ,\;B_{21} ,B_{22} } \right) = \int\limits_{ - h/2}^{h/2} {\left( {\frac{{r_{2} }}{{r_{1} }}C_{11} ,\;C_{12} ,\;C_{21} ,\;\frac{{r_{1} }}{{r_{2} }}C_{22} } \right)z{\text{d}}z} , \hfill \\ \left( {B_{66}^{11} ,\;B_{66}^{12} ,\;B_{66}^{21} ,\;B_{66}^{22} } \right) = \int\limits_{ - h/2}^{h/2} {\left( {\;\frac{{r_{1} }}{{r_{2} }}C_{66} ,\;C_{66} ,\;\;C_{66} ,\;\frac{{r_{2} }}{{r_{1} }}C_{66} } \right)z{\text{d}}z} , \hfill \\ \left( {D_{11} ,\;D_{12} ,\;D_{21} ,D_{22} } \right) = \int\limits_{ - h/2}^{h/2} {\left( {\frac{{r_{2} }}{{r_{1} }}C_{11} ,\;C_{12} ,\;C_{21} ,\;\frac{{r_{1} }}{{r_{2} }}C_{22} ,} \right)z^{2} {\text{d}}z} , \hfill \\ \left( {D_{66}^{11} ,\;D_{66}^{12} ,\;D_{66}^{21} ,\;D_{66}^{22} } \right) = \int\limits_{ - h/2}^{h/2} {\left( {\;\frac{{r_{1} }}{{r_{2} }}C_{66} ,\;C_{66} ,\;\;C_{66} ,\;\frac{{r_{2} }}{{r_{1} }}C_{66} } \right)z^{2} {\text{d}}z} , \hfill \\ \left( {S_{44} ,\;S_{55} } \right) = \int\limits_{ - h/2}^{h/2} {k_{s} \left( {r_{1} C_{44} ,r_{2} C_{55} } \right){\text{d}}z} . \hfill \\ \end{gathered}$$
(20)

The coefficients \(I_{0} ,I_{1} ,I_{2}\) and \(J_{0} ,J_{1} ,J_{2}\) are estimated as the following formulas

$$\begin{gathered} \left( {I_{0} ,I_{1} ,I_{2} } \right) = \int\limits_{ - h/2}^{h/2} {\rho (z)\left( {1,\;z,\;\;z^{2} } \right)r_{1} r_{2} {\text{d}}z} , \hfill \\ \left( {J_{0} ,J_{1} ,J_{2} } \right) = \int\limits_{ - h/2}^{h/2} {\mu (z)\rho (z)\left( {1,\;z,\;z^{2} } \right)r_{1} r_{2} {\text{d}}z} . \hfill \\ \end{gathered}$$
(21)

It is obvious that when the nonlocal parameters are constant \(\mu (z) = \mu_{{\text{m}}} = \mu_{{\text{c}}} = \mu\), one gets:

$$\left( {J_{0} ,J_{1} ,J_{2} } \right) = \mu \left( {I_{0} ,I_{1} ,I_{2} } \right).$$
(22)

It is clear that when the nonlocal parameters are constant, the governing equations of motion Eq. (16) become the conventional governing equations of motion of the FG doubly curved shallow nanoshells with a constant nonlocal parameter.

4 Analytical solution

In this study, a simply supported FG doubly curved shallow nanoshells with porosity is considered. Equations of motion are analytically solved by using the Navier solution procedure. The unknown displacement functions of the FG doubly curved shallow nanoshells are given as the following:

$$\begin{gathered} u(x,y,t) = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {U_{mn} \;e^{i\omega t} \cos \alpha x\sin \beta y} } \hfill \\ v(x,y,t) = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {V_{mn} \;e^{i\omega t} \sin \alpha x\cos \beta y} } \hfill \\ w(x,y,t) = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {W_{mn} \;e^{i\omega t} \sin \alpha x\sin \beta y} } \hfill \\ \varphi_{x} (x,y,t) = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {X_{mn} \;e^{i\omega t} \cos \alpha x\sin \beta y} } \hfill \\ \varphi_{y} (x,y,t) = \sum\limits_{m = 1}^{\infty } {\sum\limits_{n = 1}^{\infty } {Y_{mn} \;e^{i\omega t} \sin \alpha x\cos \beta y} } \hfill \\ \end{gathered}$$
(23)

where \(\alpha = m\pi /a\) and \(\beta = n\pi /b\), \(\left\{ {\text{q}} \right\} = \left\{ {U_{mn} ,V_{mn} ,W_{mn} ,X_{mn} ,\;Y_{mn} } \right\}^{T}\) are the vector of unknown coefficients, \(\omega\) is the frequency of the FG doubly curved shallow nanoshells.

Substituting Eq. (23) into Eqs. (5) and (16), the governing equations of motion for free vibration analysis of the FG porous doubly curved shallow nanoshells can be stated in the form of a generalized eigenvalue problem.

$$\left( {\left[ K \right] - \omega^{2} \left[ M \right]} \right)\left\{ q \right\} = {\mathbf{0}},$$
(24)

where \([K]\) is the stiffness matrix and \([M]\) is the mass matrix.

The elements of the stiffness matrix \([{\text{K}} ]\) and the mass matrix \([{\text{M}} ]\) are computed as the following:

$$\begin{gathered} k_{11} = A_{11} \alpha^{2} + \beta^{2} A_{66}^{11} + \frac{{S_{55} }}{{R_{1}^{2} }};k_{12} = \beta \alpha A_{12} + \beta \alpha A_{66}^{12} ;k_{13} = - \frac{{\alpha A_{11} }}{{R_{1} }} - \frac{{\alpha A_{12} }}{{R_{2} }} - \frac{{\alpha S_{55} }}{{R_{1} }}; \hfill \\ k_{14} = B_{11} \alpha^{2} + \beta^{2} B_{66}^{11} - \frac{{S_{55} }}{{R_{1} }};k_{15} = \beta \alpha B_{12} + \beta \alpha B_{66}^{12} ;k_{21} = \alpha \beta A_{21} + \alpha \beta A_{66}^{21} ; \hfill \\ k_{22} = A_{22} \beta^{2} + A_{66}^{22} \alpha^{2} + \frac{{S_{44} }}{{R_{2}^{2} }};k_{23} = - \frac{{A_{21} \beta }}{{R_{1} }} - \frac{{\beta A_{22} }}{{R_{2} }} - \frac{{\beta S_{44} }}{{R_{2} }};k_{24} = \alpha \beta B_{21} + \alpha \beta B_{66}^{21} ; \hfill \\ k_{25} = B_{22} \beta^{2} + B_{66}^{22} \alpha^{2} - \frac{{S_{44} }}{{R_{2} }};k_{31} = - \frac{{\alpha A_{11} }}{{R_{1} }} - \frac{{A_{21} \alpha }}{{R_{2} }} - \frac{{\alpha S_{55} }}{{R_{1} }};k_{32} = - \frac{{\beta A_{12} }}{{R_{1} }} - \frac{{\beta A_{22} }}{{R_{2} }} - \frac{{\beta S_{44} }}{{R_{2} }}; \hfill \\ k_{33} = S_{44} \beta^{2} + S_{55} \alpha^{2} + \frac{{A_{11} }}{{R_{1}^{2} }} + \frac{{A_{12} }}{{R_{1} R_{2} }} + \frac{{A_{21} }}{{R_{1} R_{2} }} + \frac{{A_{22} }}{{R_{2}^{2} }};k_{34} = S_{55} \alpha - \frac{{B_{11} \alpha }}{{R_{1} }} - \frac{{B_{21} \alpha }}{{R_{2} }}; \hfill \\ k_{35} = S_{44} \beta - \frac{{B_{12} \beta }}{{R_{1} }} - \frac{{B_{22} \beta }}{{R_{2} }};k_{41} = B_{11} \alpha^{2} + \beta^{2} B_{66}^{11} - \frac{{S_{55} }}{{R_{1} }};k_{42} = B_{12} \alpha \beta + B_{66}^{12} \alpha \beta ; \hfill \\ k_{43} = S_{55} \alpha - \frac{{B_{11} \alpha }}{{R_{1} }} - \frac{{B_{12} \alpha }}{{R_{2} }};k_{44} = D_{11} \alpha^{2} + D_{66}^{11} \beta^{2} + S_{55} ;k_{45} = \alpha \beta D_{12} + \alpha \beta D_{66}^{12} ; \hfill \\ k_{51} = \alpha \beta B_{21} + \alpha \beta B_{66}^{21} ;k_{52} = B_{22} \beta^{2} + B_{66}^{22} \alpha^{2} - \frac{{S_{44} }}{{R_{2} }};k_{53} = S_{44} \beta - \frac{{B_{21} \beta }}{{R_{1} }} - \frac{{B_{22} \beta }}{{R_{2} }}; \hfill \\ k_{54} = \alpha \beta D_{21} + \alpha \beta D_{66}^{21} ;k_{55} = D_{22} \beta^{2} + D_{66}^{22} \alpha^{2} + S_{44} ; \hfill \\ \end{gathered}$$
(25)
$$\begin{aligned} & m_{11} = \left( {\alpha^{2} + \beta^{2} } \right)J_{0} + I_{0} ;\,\, m_{12} = 0;\,\,m_{13} = 0;\,\,m_{14} = \left( {\alpha^{2} + \beta^{2} } \right)J_{1} + I_{1} ;\,\,m_{15} = 0; \nonumber\\ &m_{21} = 0;\,\,m_{22} = \left( {\alpha^{2} + \beta^{2} } \right)J_{0} + I_{0} ;\,\,m_{23} = 0;\,\,m_{24} = 0;\,\,m_{25} = \left( {\alpha^{2} + \beta^{2} } \right)J_{1} + I_{1} ; \nonumber\\ &m_{31} = 0;\,\,m_{32} = 0;\,\,m_{33} = \left( {\alpha^{2} + \beta^{2} } \right)J_{0} + I_{0} ;\,\,m_{34} = 0;\,\,m_{35} = 0; \nonumber\\ & m_{41} = \left( {\alpha^{2} + \beta^{2} } \right)J_{1} + I_{1} ;\,\,m_{42} = 0;\,\,m_{43} = 0;\,\,m_{44} = \left( {\alpha^{2} + \beta^{2} } \right)J_{2} + I_{2} ;\,\,m_{45} = 0; \nonumber\\ & m_{51} = 0;\,\,m_{52} = \left( {\alpha^{2} + \beta^{2} } \right)J_{1} + I_{1} ;\,\,m_{53} = 0;\,\,m_{54} = 0;\,\,m_{55} = \left( {\alpha^{2} + \beta^{2} } \right)J_{2} + I_{2} ; \end{aligned}$$
(26)

By solving Eq. (24), the frequencies and responding eigenvectors of the FG doubly curved shallow nanoshells are achieved.

5 Numerical results and discussion

The following individual material is considered and their material properties are given in Table 1.

Table 1 Material properties used in the numerical analysis
Full size table

5.1 Verification study

At the first, to assess the accuracy and robustness of the proposed formulation, the free vibration analysis of simply supported isotropic homogeneous spherical shells is considered. The geometric and mechanical properties of the shells are: \(a = b = 1.0118\;{\text{m}}\), \(h = 0.0191\;{\text{m}}\), \(R_{1} = R_{2} = R = 1.91\;{\text{m}}\), \(E = 1\;{\text{Pa}}\), \(\rho = 1\;{\text{kg/m}}^{{3}}\), and \(\nu = 0.3\). The frequencies of the shells using the present formulation and those of Khare et al. [28] and Fadaee et al. [29] are presented in Table 2. It can be seen, from Table 2, that the present results are very close to the published solutions.

Table 2 Comparison of the frequencies of the isotropic homogeneous SP shells
Full size table

After verifying the performance and correctness of the proposed formulation, the free vibration of simply supported FG doubly curved porous shells is carried out. The FG shells are graded from metal to ceramic (\({\text{(SUS304)}}_{(1)} {\text{/Al}}_{{2}} {\text{O}}_{{3}}\)). The geometric properties of the FG shells are: \(a = b = 1\;{\text{m}}\), \(h = 0.01\;{\text{m}}\), \(R_{1} = R_{2} = 1\;{\text{m}}\) for spherical shells, \(R_{1} = - R_{2} = 1\;{\text{m}}\) for hyperbolic paraboloidal shells, and \(R_{1} = 1,\;R_{2} = \infty\) for cylindrical shells. The distribution of porosity is even through the thickness of the shells, and the effective material properties of the FG are estimated by Eq. (3). The non-dimensional frequencies of the FG shells are calculated by \(\hat{\omega } = \omega \left( {{{a^{2} } \mathord{\left/ {\vphantom {{a^{2} } h}} \right. \kern-\nulldelimiterspace} h}} \right)\sqrt {{{\rho_{{\text{c}}} } \mathord{\left/ {\vphantom {{\rho_{{\text{c}}} } {E_{{\text{c}}} }}} \right. \kern-\nulldelimiterspace} {E_{{\text{c}}} }}}\). Table 3 gives the comparison between the present results and the solutions of Jouneghani et al. [32]. It is obvious that the results of the present formulation agree well with those of the published data.

Table 3 Comparison study of frequencies of FG doubly curved shells
Full size table

To validate the present size-dependent model, the results of the proposed model are compared with those of Karami et al. [51], and Natarajan et al. [59]. The FG nanoplates are made of \({\text{Si}}_{{3}} {\text{N}}_{{4}} {\text{/(SUS304)}}_{(2)}\) with the dimensions of \(a = b = 10\;{\text{nm}}\). The effective material properties are calculated using the Mori–Tanaka homogenization scheme (Natarajan et al. [59]). The non-dimensional fundamental frequencies are calculated by \(\tilde{\omega } = \omega h\sqrt {{{\rho_{{\text{c}}} } \mathord{\left/ {\vphantom {{\rho_{{\text{c}}} } {G_{{\text{c}}} }}} \right. \kern-\nulldelimiterspace} {G_{{\text{c}}} }}}\), \(G_{c} = {{E_{c} } \mathord{\left/ {\vphantom {{E_{c} } {\left( {2\left( {1 + \nu_{c} } \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + \nu_{c} } \right)} \right)}}\). It should be noticed that when the radius of curvature goes towards infinity, the doubly curved shallow nanoshell becomes a nanoplate. The frequencies of the nanoplates are given in Table 4, the comparison shows an adequate level of agreement.

Table 4 The comparison of the non-dimensional fundamental frequencies of the FG nanoplates
Full size table

5.2 Parameter study

In this section, an \({\text{(SUS304)}}_{(1)} {\text{/Al}}_{{2}} {\text{O}}_{{3}}\) doubly curved shallow nanoshell with \(S = a.b = 100\;({\text{nm}}^{2} )\) is examined. The effective Young’s modulus, mass density through the thickness of the perfect FG nanoshells are presented in Fig. 3. The effective Young’s modulus and mass density of the FG porous nanoshell with even and uneven porosity distributions are presented, respectively, in Figs. 4 and 5. Figure 6 demonstrates the variation of the effective nonlocal parameters through the thickness of the FG nanoshells.

Fig. 3
figure 3

The variation of the effective Young’s modulus and mass density through the thickness of the FG perfect nanoshells

Full size image
Fig. 4
figure 4

The variation of the effective Young’s modulus and mass density through the thickness of the FG nanoshells with even porosity distribution (\(\xi = 0.3\))

Full size image
Fig. 5
figure 5

The variation of the effective Young’s modulus and mass density through the thickness of the FG nanoshells with uneven porosity distribution (\(\xi = 0.3\))

Full size image
Fig. 6
figure 6

The variation of the effective nonlocal parameter through the thickness of the FG nanoshells

Full size image

For convenience, the following non-dimensional frequencies are used in the parametric study.

$$\Phi = \omega \frac{{S_{0} }}{{h_{0} }}\sqrt {\frac{{\rho_{{\text{c}}} }}{{E_{{\text{c}}} }}} ,\;h_{0} = 1\;{\text{nm,}}\;S_{0} = 100\;{\text{nm}}^{2} .$$
(27)

The non-dimensional fundamental frequencies of perfect and imperfect FG FL nanoplates, SP nanoshells, HP nanoshells, and CY nanoshells are presented in Tables 5, 6, 7. In which, the dimensions of the shells are \(a = b = 10\;{\text{nm}}\), \(h = 0.5\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = 20\;{\text{nm}}\) for SP nanoshells, \(R_{1} = - R_{2} = 20\;{\text{nm}}\) for HP nanoshells, \(R_{1} = 20\;{\text{nm,}}\;R_{2} = \infty\) for CY nanoshells. According to these tables, it is obvious that the fundamental frequencies of the SP shells are the highest, while those of the HP shells are the smallest. The frequencies of the FG doubly curved shallow nanoshells for \(k = 1\) are higher than those of FG doubly curved shallow nanoshells with \(k = 2\). The reason is that the volume fraction of the ceramic components of the FG doubly curved shallow nanoshells with \(k = 1\) is higher than that of the FG doubly curved shallow nanoshells with \(k = 2\), therefore, the stiffness of the FG shells with \(k = 1\) is greater than the FG shells with \(k = 2\). Besides, the consideration of the nonlocal parameters leads to the reduction of the stiffness of the nanoshells, and resulting in a reduction of the frequencies of the FG doubly curved shallow nanoshells. In addition, the frequencies of the FG porous doubly curved shallow nanoshells with even distribution are smaller than those of perfect ones, whereas the frequencies of the FG porous doubly curved shallow nanoshells with uneven distribution are higher than those of the perfect ones.

Table 5 The non-dimensional fundamental frequency of the FG perfect doubly curved shallow nanoshells
Full size table
Table 6 The non-dimensional fundamental frequency of the FG doubly curved shallow nanoshells with even porosity distribution (\(\xi = 0.3\))
Full size table
Table 7 The non-dimensional fundamental frequency of the FG doubly curved shallow nanoshells with uneven porosity distribution (\(\xi = 0.3\))
Full size table

Table 8 demonstrates the effects of the porosity distribution and nonlocal parameters on the three modes of \((1,1)\), \((2,2)\), and \((3,3)\) of the FG doubly curved shallow nanoshells for two cases of even and uneven porosity distributions. Table 8 shows that the nonlocal parameters affect on both lower and higher frequencies of the porous nanoshells. Moreover, the fundamental frequencies of the FG doubly curved shallow nanoshells depend significantly on the type of shells, but the higher frequencies of different types of FG doubly curved shallow nanoshells are similar.

Table 8 The non-dimensional frequencies of the FG doubly curved shallow nanoshells with different porosity coefficients and nonlocal parameters
Full size table

5.2.1 Effects of nonlocal parameters

In this subsection, the influence of the nonlocal parameters on the fundamental frequencies of the FG porous doubly curved nanoshells is investigated. The geometric properties of the nanoshells are: \(a = b = 10\;{\text{nm}}\), \(h = 0.5\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = 20\;{\text{nm}}\) for SP nanoshells, \(R_{1} = - R_{2} = 20\;{\text{nm}}\) for HP nanoshells, and \(R_{1} = 20\;{\text{nm,}}\;R_{2} = \infty\) for CY nanoshells. The porosity coefficient is taken as \(\xi = 0.3\). Figure 7 illustrates the variation of the fundamental frequencies of the FG nanoshells versus the variation of the metal nonlocal parameter \(\mu_{{\text{m}}}\). In general, the increase of the metal nonlocal parameter leads to a decrease of the fundamental frequencies of the FG porous doubly curved shallow nanoshells except the full-ceramic shells. The effect of the metal nonlocal parameter on metal-rich shells are more significant than the ceramic-rich ones. Figure 8 demonstrates the effect of the ceramic nonlocal parameter \(\mu_{{\text{c}}}\) in the fundamental frequencies of the FG porous doubly curved shallow nanoshells. For the both cases of porosity distributions i.e., even and uneven, the increase of the ceramic nonlocal parameter leads to a reduction of the fundamental frequencies of the FG porous doubly curved shallow nanoshells. It should be noticed that the effect of the ceramic nonlocal parameter \(\mu_{c}\) on the frequencies of the FG nanoshells with a small power-law index are more significant than the FG porous doubly curved shallow nanoshells with a higher power-law index.

Fig. 7
figure 7

The variation of the non-dimensional frequencies of FG porous doubly curved shallow nanoshells versus the variation of the nonlocal parameter \(\mu_{{\text{m}}}\)

Full size image
Fig. 8
figure 8

The variation of the nondimensional frequencies of FG porous doubly curved shallow nanoshells versus the variation of the nonlocal parameter \(\mu_{{\text{c}}}\)

Full size image

5.2.2 Effects of porosity

Next, the effects of the porosity coefficient on the free vibration behavior of the FG porous doubly curved shallow nanoshells are studied. The dimensions of the nanoshells are \(a = b = 10\;{\text{nm}}\), \(h = 0.5\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = 20\;{\text{nm}}\) for SP nanoshells, \(R_{1} = - R_{2} = 20\;{\text{nm}}\) for HP nanoshells, \(R_{1} = 20\;{\text{nm,}}\;R_{2} = \infty\) for CY nanoshells. The computed results are obtained for the power-law index k equal to 2 (\(k = 2\)). The variation of the non-dimensional frequencies of the FG doubly curved shells with the functions of the porosity coefficient are shown in Fig. 9. It is noticeable, for the four types of nanoshells, that the fundamental frequencies of the FG porous doubly curved shallow nanoshells with an even porosity distribution decrease as the porosity coefficient increases. In the case of uneven porosity distribution, the increase in the porosity coefficient \(\xi\) leads to an increase of FL nanoplates, HP nanoshells, and CY nanoshells, but it leads to a decrease of the fundamental frequencies of the SP nanoshells. Hence, the effect of the porosity coefficient on the frequencies of the FG porous doubly curved shallow nanoshells not only depend on the porosity coefficient, the distributions of the porosity, but also on the type of the shells. The finding results are a new result of this study, which will serve as a benchmark for future analysis of FG porous doubly curved shallow nanoshells.

Fig. 9
figure 9

The effect of the porosity coefficient on the frequencies of the FG porous doubly curved shallow nanoshells

Full size image

5.2.3 Effect of the power-law index

Continuously, the influence of the power-law index \(k\) on the fee vibration of the FG porous doubly curved shallow nanoshells is considered. The dimensions of the shells are \(a = b = 10\;{\text{nm}}\), \(h = 0.5\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = 20\;{\text{nm}}\) for SP nanoshells, \(R_{1} = - R_{2} = 20\;{\text{nm}}\) for HP nanoshells, and \(R_{1} = 20\;{\text{nm,}}\;R_{2} = \infty\) for CY nanoshells. The porosity coefficient is taken as \(\xi = 0.3\). The power-law index is varied from 0 to 10. The non-dimensional frequencies of the FG doubly curved shells are illustrated in Fig. 10. This figure shows that the increase of the power-law index leads to a decrease of the fundamental frequencies of the FG porous doubly curved shallow nanoshells. When the power-law index increases from 0 to 2, the frequencies of the FG porous doubly curved shallow nanoshell decrease rapidly, and when the power-law index increases from 2 to 10, the frequencies of the FG porous doubly curved shallow nanoshells decrease slowly. Again, the frequencies of the HP shells are the highest, while those of HP shells are the smallest.

Fig. 10
figure 10

The influence of the power-law index on the frequencies of FG doubly curved shallow nanoshells

Full size image

5.2.4 Effects of side-to-thickness ratio

Constantly, the effect of the side-to-thickness ratio \(a{/}h\) on the non-dimensional frequencies of the FG porous doubly curved shallow nanoshells are investigated. In this case, the dimensions of the shells are \(a = b = 10\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = 20\;{\text{nm}}\) for SP nanoshells, \(R_{1} = - R_{2} = 20\;{\text{nm}}\) for HP nanoshells, \(R_{1} = 20\;{\text{nm,}}\;R_{2} = \infty\) for CY nanoshells. The obtained results are calculated for the nonlocal parameters equal to 1 and 4 (\(\mu_{{\text{m}}} = 1\), \(\mu_{{\text{c}}} = 4\)), the power-law index equal to 2 (\(k = 2\)), the porosity coefficient equal to 0.3 (\(\xi = 0.3\)). Different values of the side-to-thickness ratio are considered (\(a{/}h = 10,\;15,\;20,\;30,\;40,\;50,\;100\)). The variation of the non-dimensional fundamental frequencies of the FG porous doubly curved shallow nanoshells is presented in Table 9. According to this table, it is clear that when the side-to-thickness ratio increases, the frequencies of the FG porous doubly curved shallow nanoshell decrease rapidly because the increase of the side-to-thickness ratio leads to a reduction in thickness of the shells, and then the stiffness of the shells decreases.

Table 9 The effect of side-to-thickness ratio on the fundamental frequencies of the FG porous doubly curved shallow nanoshells
Full size table

5.2.5 Effects of radius-to-side ratio

Figure 11 demonstrates the variation of the non-dimensional frequencies of the FG porous doubly curved shallow nanoshells with various values of radius-to-side ratio \(R{/}a\). The geometric properties of the shells are \(a = b = 10\;{\text{nm}}\), \(h = 0.5\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = R\) for SP nanoshells, \(R_{1} = - R_{2} = R\) for HP nanoshells, \(R_{1} = R{,}\;R_{2} = \infty\) for CY nanoshells. The power-law index is assumed as \(k = 2\), and the porosity coefficient is taken as \(\xi = 0.3\). The variation of the non-dimensional fundamental frequencies of the FG porous doubly curved shallow nanoshells is presented in Fig. 11 as the radius-to-side ratio varies from 2 to 10. When the radius-to-side ratio increases, the frequencies of the FL nanoplates, CY nanoshells decrease, while those of HP shells increase. The frequencies of the SP nanoshells, HP nanoshells, and CY nanoshells reach the frequencies of the FL nanoplates when the radius-to-side ratio is high enough. The reason is that when the radius-to-side ratio is high enough, the SP nanoshells, HP nanoshells, and CY nanoshells become FL nanoplates.

Fig. 11
figure 11

The effects of radius-to-side ratio on the frequencies of the FG porous doubly curved shallow nanoshells

Full size image

5.2.6 Effects of aspect ratio

Lastly, the effect of the aspect ratio (a/b) on the frequencies of the FG porous doubly curved shallow nanoshells are investigated. In this case, \(S = ab = 100\;{\text{nm}}^{2} = {\text{const}}\), \(h = 0.5\;{\text{nm}}\), \(R_{1} = R_{2} = \infty\) for FL nanoplates, \(R_{1} = R_{2} = 20\;{\text{nm}}\) for SP nanoshells, \(R_{1} = - R_{2} = 20\;{\text{nm}}\) for HP nanoshells, \(R_{1} = 20\;{\text{nm,}}\;R_{2} = \infty\) for CY nanoshells. For this study, the power-law index is taken as \(k = 2\), the porosity coefficient is \(\xi = 0.3\), and the aspect ratio \(a{/}b\) varies from 0.5 to 2. The variation of the non-dimensional fundamental frequencies of the FG nanoshells is illustrated in Fig. 12. For the three cases of FL nanoplates, SP nanoshells, and HP nanoshells, the frequencies decrease as the aspect ratio increases from 0.5 to 1. When the aspect ratio increases from 1 to 2, the minimum frequency occurs at \(a/b = 1\). In the case of CY nanoshells, the frequencies decrease when the aspect ratio increases from 0.5 to 1.5, and those increase when the aspect ratio increases from 1.5 to 2, the minimum frequency occurs at \(a/b = 1.5\).

Fig. 12
figure 12

The variation of the non-dimensional frequencies of FG porous doubly curved shallow nanoshells versus the aspect ratio (a/b)

Full size image

6 Conclusions

In this work, the free vibration behavior of FG porous doubly curved shallow nanoshells with variable nonlocal parameters has been investigated using FSDT and nonlocal elasticity theory. Four types of FG porous doubly curved shallow nanoshells with various porosity distributions have been considered. The nonlocal elasticity theory has been modified to take into account for the variation of the nonlocal parameters through the thickness of the FG porous doubly curved shallow nanoshells. The governing equations of motion are established via Hamilton’s principle and they are solved via Navier’s solution. The accuracy and correctness of the proposed algorithm are demonstrated through several comparison studies. A comprehensive parametric study has been carried out to illustrate the couple-effect of porosity and nonlocal parameter variation. Based on the numerical results, the following remarkable conclusions can be achieved.

  • The consideration of the nonlocal parameters leads to a reduction in the frequencies of the FG porous doubly curved shallow nanoshells.

  • The effect of the nonlocal parameters depends not only on their values but also on the power-law index of the FG porous doubly curved shallow nanoshells.

  • The effect of porosity on the free vibration behavior of the FG porous doubly curved shallow nanoshells depends on the value of the porosity coefficient, the porosity distributions, and the curvature of the shells.

The numerical results of the work show that the variation of the nonlocal parameters has significant influences on the free vibration behavior of the FG porous doubly curved shallow nanoshells. The present algorithm can be extended to analyze the behaviors of other nanostructures with a variation of the nonlocal parameters. The numerical results of this study can serve as benchmark solutions for future work.