1 Introduction

In modern nonlinear science, one of the essential models is the nonlinear Schrödinger equation. The nonlinear Schrödinger equation is closely related to a lot of nonlinear problems in theoretical physics such as nonlinear quantum field theory, nonlinear optics, Bose–Einstein Condensate, condensed matter, plasma physics etc. One of the finest solutions of the nonlinear Schrödinger equation is called solitons (Hasegawa and Kodama 1995; Agrawal 1995; Serkin and Hasegawa 2000a, 2000b, 2002; Serkin et al. 2001). In an optical fiber, optical solitons were generated by the equilibrium between dispersion or group velocity dispersion (GVD) and nonlinear effects (Lamb 1980). Earlier the theoretical investigation of optical soliton was studied by Hasegawa and Tappert (1973a, 1973b) and it was experimentally justified by Mollenauer et al. (1980). The anomalous dispersion results the bright soliton whereas normal dispersion gives the dark soliton solution for the nonlinear Schrödinger equation (Shabat and Zakharov 1972; Zakharov and Shabat 1973). Optical solitons are a viable option for the next generation of ultra-fast optical communication systems.

In real problems, the optical fibers are inhomogeneous due to the variation in the distance between lattice points and some manufacturing problems. Due to some potential applications, the study on propagation of optical solitons in inhomogeneous fibers are essential and it can be governed by variable coefficient nonlinear Schrödinger equations (Liu and Tian 2012). One natural, simple way to account for inhomogeneities in the fiber is to incorporate variable coefficients. The parity-time symmetric potential and the effects of harmonic on 3D nonlinear Schrödinger equation with variable coefficients have been studied by Dai et al. (2014). The rogue wave is constructed to the (3 + 1) dimensional nonlinear Schrödinger equation with variable coefficients and the effects of external potentials are investigated (Dai and Zhu 2014). In an optical fiber, inhomogeneous arises due to the various factors as follows: (i) the distance between two atoms no longer constant through the fiber. (ii) variation in the diameter and etc. These factors are mainly influence the dispersion, nonlinearity, loss and gain parameters in the inhomogeneous optical fibers. This means that the parameters will be varying with the propagation distance. In the case of femtosecond soliton propagation, standard nonlinear Schrödinger equation is inadequate. Hence, higher order effects are also taken into account and it can be inhomogeneous higher order nonlinear Schrödinger equation (Hao et al. 2004; Zhang et al. 2005; Tian and Zhou 2005; Meng et al. 2008). The investigation has been made to study the femtosecond solitons in inhomogeneous optical fiber medium, which is described by the higher order nonlinear Schrödinger equation with variable coefficients (Liu et al. 2010). Recently the application of inhomogeneous nonlinear optical fibers with dispersion and nonlinearity for a number of purposes, i.e., dispersion management and control of solitons (Xu et al. 2003), modulation instability stimulation (Malomed 1993), compression of pulse (Tajima 1987), and amplification of soliton in long range communication (Peng et al. 1998), has been investigated computationally. Guo et al. investigated the conservation laws for the generalized version of nonlinear Schrödinger equation with Maxwell Bloch (Guo et al. 2012). Recently one of the solitonic structure i.e., dromion structure is observed in attosecond sixth order inhomogeneous nonlinear Schrödinger equation (Prathap et al. 2018). Many effects are currently investigating the propagation dynamics of optical solitons in inhomogeneous optical fiber media. The problem of nonlinear wave propagation in inhomogeneous media has received a lot of attention in recent years, and it has a lot of applications.

In general, if the system receives some kind of an external time-dependent or space-dependent force then that system can be called as nonautonomous system. Due to the special properties of nonautonomous solitons, it can be used in various applications such as optical soliton telecommunication, soliton lasers, ultra soliton switches etc. The width, amplitudes and pulse center can be changed by managing the system parameters such as dispersion, nonlinearity and gain (Serkin et al. 2010). Subramanian et al. have been discussed the properties of optical solitons by choosing the variable coefficients for the inhomogeneous nonlinear Schrödinger equation Maxwell-Bloch system (Subramanian et al. 2017). The generalized nonautonomous nonlinear Schrödinger equations with variable coefficients and external potential has been extensively studied by Belyaeva et al. (2011). The time and space dependent coefficients of generalized nonautonomous cubic-quintic nonlinear Schrödinger equation with external potentials are investigated by He and Li (2011). The cubic quintic nonlinear Schrödinger equation with linear-lattice potential and spatiotemporal modulation of nonlinearities was studied by You et al. (2014). Mani Rajan et al. (2013) studied the generalized nonlinear Schrödinger Maxwell-Bloch equation with some forms of external potentials. Under various circumstances, the Ref, (Serkin et al. 2007) has been made to study the propagation of nonautonomous solitons with external potentials. The propagation of nonautonomous solitons with cubic quintic effect has been investigated in Porsezian et al. (2007). With the consideration of constant nonlinearity, nonlinear Schrödinger equation with combined effects of inhomogeneous dispersion and external harmonic potential have been reported (Raghuraman et al. 2021). There are only fewer studies about the fascinating properties of the space and time dependent coefficients for the nonautonomous systems.

This paper is organized in the following way: In Sect. 1, we have discussed the introduction of the nonautonomous optical solitons and properties of nonautonomous systems. The Sect. 2 is devoted to the Lax pair for the nonautonomous Hirota equation with variable coefficients. In Sect. 3, three and four soliton solutions are generated by means of Darboux transformation for the generalized nonautonomous nonlinear system based on the constructed Lax pair. In Sect. 4, obtained four soliton solutions are graphically illustrated with explanations. Finally, the paper is concluded with Sect. 5.

2 Nonautonomous Hirota equation with Lax pair

We consider the generalized nonautonomous Hirota equation, which describes the dynamics of the ultra-short optical pulse propagating in the nonlinear inhomogeneous optical fiber medium as,

$$i\frac{\partial q}{{\partial z}} - i\alpha (z)\frac{\partial q}{{\partial t}} - i\beta (z)\left( {t\frac{\partial q}{{\partial t}} + q} \right) + \gamma (z)\left( {\frac{{\partial^{2} q}}{{\partial t^{2} }} + 2\left| q \right|^{2} q} \right) - i\delta (z)\left( {\frac{{\partial^{3} q}}{{\partial t^{3} }} + 6\left| q \right|^{2} q_{t} } \right) = 0$$
(1)

where \(q(z,t)\) is a complex envelope of the field, \(\alpha (z)\) and \(\beta (z)\) are the coefficients of nonautonomous terms while \(\gamma (z)\) and \(\delta (z)\) are related to the coefficients of autonomous terms. Autonomous and nonautonomous variables are defined in terms of arbitrary functions, and suffixes denote partial differentiation. We can construct a new Lax pair of Eq. (1) By Ablowitz-Kaup-Newell-Segur Scheme (AKNS) (Ablowitz et al. 1973). The linear eigenvalue problem for the Eq. (1) is written as

$$\Phi_{t} = U\Phi \;{\text{and}}\;\Phi_{z} = V\Phi$$
(2)

where, \(\Phi = (\Phi_{1} ,\Phi_{2} )^{T}\) and \(T\) is referred as transpose of the matrix.

\(U\) and \(V\) are represented as,

$$U = i\zeta \sigma_{3} + iQ,$$
(3)
$$V = i\alpha (\sigma_{3} \zeta + Q) + i\beta t(\sigma_{3} \zeta + Q) + \gamma V_{1} + \delta V_{2} ,$$
(4)

where \(\zeta = \xi e^{{\int {\beta dz} }}\) and \(\xi\) is a complex number.

Let us define

$$V_{1} = 2i\sigma_{3} \zeta^{2} + 2iQ\zeta - i\sigma_{3} Q^{2} + \sigma_{3} Q_{t} ,$$
(5)
$$V_{2} = - 4i\sigma_{3} \zeta^{3} - 4iQ\zeta^{2} + 2(i\sigma_{3} Q^{2} - \sigma_{3} Q_{t} )\zeta + iQ_{tt} + 2iQ^{3} + Q_{t} Q - QQ_{t} ,$$
(6)
$$\sigma_{3} = \left( {\begin{array}{*{20}c} 1 & 0 \\ 0 & { - 1} \\ \end{array} } \right)\;{\text{and}}\;Q = \left( {\begin{array}{*{20}c} 0 & {q^{*} } \\ { - q} & 0 \\ \end{array} } \right)$$
(7)

In the Lax pair (2), ϛ is non-isospectral parameter. The superscript * stands for a complex conjugate. It is very easy to verify the compatibility condition and the compatibility condition \(U_{Z} - V_{t} + UV - VU = 0\) gives rise to the Eq. (1).

3 Darboux transformation

In the context of soliton theory, Darboux transformation method is one of the effective tools to obtain the exact soliton solution for a nonlinear equation. By employing Darboux transformation method, one can construct n-number of soliton solutions based on the Lax pair (Mani Rajan and Bhuvaneshwari 2018; Matveev and Salle 1991). The Lax pair based Darboux transformation approach was recently developed and applied to the construction of soliton solutions for higher order nonlinear Schrödinger equation (Xu et al. 2002). To attain the four soliton solutions, 2 × 2 linear eigenvalue problem associated with Eq. (1) is considered and mathematical calculations are executed as given below.

Firstly, the Darboux transformation is introduced as

$$T[1] = \xi - \xi_{1}^{*} + (\xi_{1}^{*} - \xi_{1} )\frac{{\Phi_{1} \Phi_{1}^{\dag } }}{{\Phi_{1}^{\dag } \Phi_{1} }}\;{\text{and}}\;\Phi_{1} = \left( {\begin{array}{*{20}c} {\phi {}_{1}} \\ {\psi_{1} } \\ \end{array} } \right)$$
(8)

where the \(\Phi_{1}\) is a special solution for Lax pair at \(\xi = \xi_{1}\).

Similarly, the transformation for the linear eigenvalue problem is written as

$$\Phi_{t} [1] = U_{1} \Phi [1]\;{\text{and}}\;U_{1} = i\zeta_{1} \sigma_{3} + iQ_{1}$$
(9)

where

$$Q_{1} = \left( {\begin{array}{*{20}c} 0 & {q[1]*} \\ {q[1]} & 0 \\ \end{array} } \right)\;{\text{and}}\;\zeta_{1} = \xi_{1} e^{{\int {\beta dz} }}$$
(10)

The Darboux transformation for Eq. (1) in the form of

$$Q_{1} = Q + \sigma_{3} S - S\sigma_{3}$$
(11)

with \(S = H\Lambda H^{ - 1}\).

where \(H\) is a nonsingular matrix and it is defined as

$$H = \left( {\begin{array}{*{20}c} {\phi_{1} } & { - \psi_{1}^{*} } \\ { - \psi_{1} } & { - \phi_{1}^{*} } \\ \end{array} } \right)\;{\text{and}}\;\Lambda = \left( {\begin{array}{*{20}c} {\zeta_{1} } & 0 \\ 0 & {\zeta_{1}^{*} } \\ \end{array} } \right).$$
(12)

One can get the Darboux transformation for the one soliton solution from Eq. (11) as follows

$$q[1] = q[0] - 2(\xi_{1} - \xi_{1}^{*} )e^{{\int {\beta dz} }} \frac{{\psi_{1} \phi {}_{1}^{*} }}{{\left| {\phi_{1} } \right|^{2} + \left| {\psi_{1} } \right|^{2} }}\;{\text{and}}\;q[1]^{*} = q[0]^{*} - 2(\xi_{1} - \xi_{1}^{*} )e^{{\int {\beta dz} }} \frac{{\psi_{1}^{*} \phi_{1} }}{{\left| {\phi_{1} } \right|^{2} + \left| {\psi_{1} } \right|^{2} }}$$
(13)

where \(\xi_{1} = a_{1} + ib_{1} ,\) here \(a_{1}\) and \(b_{1}\) arbitrary coefficients.

The Darboux transformation for two soliton solution is given by

$$q[2] = q[1] - 2(\xi_{2} - \xi_{2}^{*} )e^{{\int {\beta dz} }} \frac{{\psi_{2} \phi {}_{2}^{*} }}{{\left| {\phi_{2} } \right|^{2} + \left| {\psi_{2} } \right|^{2} }}$$

where \(\xi_{2} = a_{2} + ib_{2}\) and we have

$$q[2] = q[0] - 2e^{{\int {\beta dz} }} \left[ {(\xi_{1} - \xi_{1}^{*} )\frac{{\psi_{1} \phi {}_{1}^{*} }}{{\left| {\phi_{1} } \right|^{2} + \left| {\psi_{1} } \right|^{2} }} + (\xi_{2} - \xi_{2}^{*} )\frac{{\psi_{2} \phi {}_{2}^{*} }}{{\left| {\phi_{2} } \right|^{2} + \left| {\psi_{2} } \right|^{2} }}} \right]$$
(14)

The N- soliton solution by Darboux transformation for the given system can be written as

$$q[N] = q[0] - \sum\limits_{N = 1}^{n} {\left[ {2e^{{\int {\beta dz} }} (\xi_{N} - \xi_{N}^{*} )\frac{{\psi_{N} \phi {}_{N}^{*} }}{{\left| {\phi_{N} } \right|^{2} + \left| {\psi_{N} } \right|^{2} }}} \right]}$$
(15)

where

$$\phi_{N} = (\lambda_{N} - \lambda_{N - 1}^{*} )\phi_{N - 1} (\lambda_{N} ) - \frac{{B_{N - 1} }}{{A_{N - 1} }}(\lambda_{N - 1} - \lambda_{N - 1}^{*} )\phi_{1} (\lambda_{N} )$$
(16)
$$\psi_{N} = (\lambda_{N + 1} - \lambda_{N}^{*} )\psi_{1} (\lambda_{N + 1} ) - \frac{{B_{N - 1} }}{{A_{N - 1} }}(\lambda_{N} - \lambda_{N}^{*} )\psi_{1} (\lambda_{N + 1} )$$
(17)
$$A_{N - 1} = \left| {\phi_{N - 1} (\lambda_{N - 1} )} \right|^{2} + \left| {\psi_{N - 1} (\lambda_{N - 1} )} \right|^{2}$$
$$B_{N - 1} = \phi_{N - 1} (\lambda_{N - 1} )\phi_{N - 1}^{*} (\lambda_{N} ) + \psi_{N - 1} (\lambda_{N - 1} )\psi_{N - 1}^{*} (\lambda_{N} )$$

Equation (15) is the general form soliton solutions. Using this solutions, one can generate any number of soliton solutions for the considered theoretical model. In the present work, as an example, three and four soliton solutions are provided. For \(\delta = 0\) in the expression (1), many researchers have been examined nonautonomous solitons under second-order dispersion and Kerr nonlinearity using the Lax-pair and Darboux transformations (Yang et al. 2004, 2011; Kruglov et al. 2005).

3.1 Three soliton solution

By setting \(N = 3\) we can write the three-soliton solution in the following form

$$q[3] = - 2i\exp (\int {\beta dz)} \left( \frac{J}{K} \right)$$
(18)

where

$$\begin{aligned} J = & \frac{1}{4}{\text{iexp(}} - 2\left( {\theta_{1} + \theta_{2} } \right) - {\text{i}}\varphi_{2} )Sech(2\theta_{1} )^{2} Sech(2\theta_{2} )(8{\text{Cos}} (2\left( {\varphi_{1} - \varphi_{2} } \right))b_{1}^{2} + 8{\text{Cosh}} (2\left( {\theta_{1} + \theta_{2} } \right))b_{1}^{2} \\ & + 2{\text{exp(}}2\smallint \beta (z){\text{d}}z)\left( {1 + {\text{Cosh}} (4\theta_{1} )} \right)\left( {\left( {a_{1} - a_{2} } \right)^{2} + \left( {b_{1} + b_{2} } \right)^{2} } \right) - 8{\text{exp(}}\smallint \beta (z){\text{d}}z){\text{Cosh}} (2\theta_{1} )b_{1} \\ & ({\text{Cosh}} (\theta_{1} + \theta_{2} - {\text{i}}\varphi_{1} + {\text{i}}\varphi_{2} )\left( {{\text{i}}a_{1} - {\text{i}}a_{2} + b_{1} + b_{2} } \right) + {\text{Cosh}} (\theta_{1} + \theta_{2} + {\text{i}}\left( {\varphi_{1} - \varphi_{2} } \right))\left( { - {\text{i}}a_{1} + {\text{i}}a_{2} + b_{1} + b_{2} } \right))) \\ & ({\text{exp(}}2{\text{i}}\varphi_{3} )\left( {1 + {\text{exp(}}4\theta_{3} )} \right)(2{\text{exp(}}2\left( {\theta_{1} + {\text{i}}\left( {\varphi_{1} + \varphi_{2} } \right)} \right))\left( {1 + {\text{exp(}}4\theta_{2} )} \right)b_{1} + {\text{exp(}}2\theta_{2} )\left( {1 + {\text{exp(}}4\theta_{1} )} \right)b_{2} ) \\ & + {\text{exp(}}2\theta_{3} + 2{\text{i}}\varphi_{2} )\left( {1 + {\text{exp(}}4\theta_{1} )} \right)\left( {1 + {\text{exp(}}4\theta_{2} )} \right)b_{3} ) \\ \end{aligned}$$
$$\begin{aligned} {\text{K}}\,{ = }\, & {\text{exp(i}}\left( {\varphi_{2} + 2\varphi_{3} } \right))\left( {1 + {\text{exp(}}4\theta_{3} )} \right)(2{\text{exp(}}2\smallint \beta (z){\text{d}}z){\text{Cosh}} (2\theta_{1} )\left( {\left( {a_{1} - a_{2} } \right)^{2} + \left( {b_{1} + b_{2} } \right)^{2} } \right) \\ & + \,4b_{1} (\left( {{\text{Cos}} (2\left( {\varphi_{1} - \varphi_{2} } \right)) + {\text{Cosh}} (2\left( {\theta_{1} + \theta_{2} } \right))} \right)Sech(2\theta_{1} )b_{1} - {\text{exp(}}\smallint \beta (z){\text{d}}z)({\text{Cosh}} (\theta_{1} + \theta_{2} - {\text{i}}\varphi_{1} + {\text{i}}\varphi_{2} ) \\ & \left( {{\text{i}}a_{1} - {\text{i}}a_{2} + b_{1} + b_{2} } \right) + {\text{Cosh}} (\theta_{1} + \theta_{2} + {\text{i}}\left( {\varphi_{1} - \varphi_{2} } \right))\left( { - {\text{i}}a_{1} + {\text{i}}a_{2} + b_{1} + b_{2} } \right)))) \\ \end{aligned}$$

and

$$\begin{aligned} \theta_{1} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{1} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{1} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{1} b_{1} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{1}^{2} b_{1} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{1}^{3} \\ \end{aligned}$$
$$\begin{aligned} \theta_{2} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{2} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{2} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{2} b_{2} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{2}^{2} b_{2} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{2}^{3} \\ \end{aligned}$$
$$\begin{aligned} \theta_{3} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{3} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{3} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{3} b_{3} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{3}^{2} b_{3} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{3}^{3} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{1} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{1} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{1} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{1}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{1}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{1}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{1} b_{1}^{2} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{2} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{2} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{2} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{2}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{2}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{2}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{2} b_{2}^{2} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{3} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{3} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{3} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{3}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{3}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{3}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{3} b_{3}^{2} \\ \end{aligned}$$

3.2 Four soliton solutions

For four soliton solution, one can choose \(N = 4\) in Eq. (15), then the solution can be written as,

$$\begin{gathered} {\text{q}}[{\text{4}}]{\text{ = }}\left( {2ie^{{4\int \beta (z){\kern 1pt} dz - 2i\varphi _{4} }} \left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} - ib_{3} } \right) + \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2i\varphi _{3} }} + e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{4} } \right)}} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} - ib_{4} } \right)} \right)} \right. \hfill \\ \left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{3} } \right)}} + e^{{2i\varphi _{4} }} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }} + e^{{\int \beta (z)dz}} \left( {a_{4} + ib_{4} } \right)} \right)\left( { - a_{3} + a_{4} - ib_{3} + } \right. \hfill \\ \left( {2e^{{2\theta _{3} - 2\theta _{4} + i\left( {\varphi _{2} - \varphi _{3} - 2\varphi _{4} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)\left( {e^{{2\left( {\theta _{3} + \theta _{4} } \right)}} + e^{{2i\left( {\varphi _{3} + \varphi _{4} } \right)}} } \right)b_{3} \left( { - i(2e^{{\theta _{3} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{3} + \theta _{4} + i\varphi _{3} + i\varphi _{4} }} + 2e^{{3\theta _{3} + 2\theta _{4} + 2i\varphi _{4} }} } \right.} \right. \hfill \\ - e^{{\int \beta (z){\kern 1pt} dz + \theta _{4} + i\left( {\varphi _{3} + \varphi _{4} } \right)}} )b_{3} + \left. {\left. {e^{{\int \beta (z){\kern 1pt} dz + \theta _{4} + i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {1 + e^{{4\theta _{3} }} } \right)\left( {a_{3} - a_{4} + ib_{4} } \right)} \right)} \right)/\left( {\left( {1 + e^{{4\theta _{3} }} } \right)\left( {2e^{{\theta _{2} }} \left( {e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{2} } \right)}} + e^{{2i\varphi _{3} }} } \right)b_{1} + e^{{\theta _{3} }} } \right.} \right. \hfill \\ \left( {2e^{{3\theta _{2} + 5\theta _{3} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + 4\theta _{3} + i\varphi _{2} + i\varphi _{3} }} + 2e^{{\theta _{2} + 3\theta _{3} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + i\left( {\varphi _{2} + \varphi _{3} } \right)}} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} } \right)b_{2} - \hfill \\ \left. {\left. {\left. {ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)\left( {1 + e^{{4\theta _{3} }} } \right)\left( {a_{2} - a_{3} - ib_{3} } \right)} \right)} \right) - ib_{4} } \right)\left( { - a_{3} + a_{4} + ib_{3} - } \right.(2ie^{{4\theta _{3} + 2i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)^{2} b_{3} \hfill \\ \left( {e^{{ - \theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{2} + ib_{2} } \right) - \frac{{2ie^{{\theta _{2} - \theta _{3} - i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{2} } \right)}} + e^{{2i\varphi _{3} }} } \right)b_{2} }}{{1 + e^{{4\theta _{2} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{3} + ib_{3} } \right)} \right)} \right. \hfill \\ \left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{3} } \right)}} + e^{{2i\varphi _{4} }} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} + ib_{4} } \right)} \right) + e^{{\theta _{3} + \theta _{4} + i\left( {\varphi _{3} + \varphi _{4} } \right)}} \hfill \\ \left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{2} + ib_{2} } \right) + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{3} + ib_{3} } \right) - \frac{{2ie^{{\theta _{2} - \theta _{3} - i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{2} } \right)}} + e^{{2i\varphi _{3} }} } \right)b_{3} }}{{1 + e^{{4\theta _{2} }} }}} \right) \hfill \\ \left. {\left. {\left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{3} } \right)}} + e^{{2i\varphi _{4} }} } \right)b_{3} }}{{1 + e^{{2\left( {\theta _{3} + \theta _{4} } \right)}} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} + ib_{4} } \right)} \right)} \right)} \right)/ \hfill \\ \left( {\left( {1 + e^{{4\theta _{3} }} } \right)(\left( {2e^{{3\theta _{2} + 2\theta _{3} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + \theta _{3} + i\varphi _{2} + i\varphi _{3} }} + 2e^{{\theta _{2} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} } \right)b_{2} - } \right.ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \hfill \\ \left( {1 + e^{{4\theta _{2} }} } \right)\left( {a_{2} - a_{3} - ib_{3} } \right))(\left( {2e^{{\theta _{2} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + \theta _{3} + i\varphi _{2} + i\varphi _{3} }} + 2e^{{3\theta _{2} + 2\theta _{3} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} } \right)b_{2} + \hfill \\ ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)\left( {a_{2} - a_{3} + ib_{3} } \right))) + ib_{4} )b_{4} \left( { - a_{3} + a_{4} + \frac{{i\left( {2B_{{31}} b_{2} + A_{3} b_{3} - A_{3} b_{4} } \right)}}{{A_{3} }}} \right) \hfill \\ \left( { - a_{3} + a_{4} - \frac{{i\left( {A_{3} b_{3} - A_{3} b_{4} + 2b_{2} B_{3} } \right)}}{{A_{3} }}} \right))/\left( {e^{{4\int \beta (z){\kern 1pt} dz - 2\theta _{4} }} (e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} - ib_{3} } \right) + \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2i\varphi _{3} }} + e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{4} } \right)}} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }}} \right. \hfill \\ + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} - ib_{4} } \right))\left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{3} } \right)}} + e^{{2i\varphi _{4} }} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} + ib_{4} } \right)} \right)\left( { - a_{3} + a_{4} } \right. \hfill \\ \end{gathered}$$
$$\begin{gathered} - ib_{3} + \left( {2ie^{{4\theta _{3} + 2i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)^{2} b_{3} \left( {e^{{ - \theta _{3} - \theta _{4} + i\left( {\varphi _{3} + \varphi _{4} } \right)}} (e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{2} - ib_{2} } \right) + \frac{{2ie^{{\theta _{2} - \theta _{3} - i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {e^{{2i\varphi _{2} }} + e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{3} } \right)}} } \right)b_{2} }}{{1 + e^{{4\theta _{2} }} }}} \right.} \right. \hfill \\ + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{3} - ib_{3} } \right))\left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} - ib_{3} } \right) + \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2i\varphi _{3} }} + e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{4} } \right)}} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} - ib_{4} } \right)} \right) + \hfill \\ e^{{\theta _{3} + \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{2} - ib_{2} } \right) + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{3} - ib_{3} } \right) + \frac{{2ie^{{\theta _{2} - \theta _{3} - i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {e^{{2i\varphi _{2} }} + e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{3} } \right)}} } \right)b_{3} }}{{1 + e^{{4\theta _{2} }} }}} \right) \hfill \\ \left. {\left. {\left( {e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} - ib_{3} } \right) + \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2i\varphi _{3} }} + e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{4} } \right)}} } \right)b_{3} }}{{1 + e^{{2\left( {\theta _{3} + \theta _{4} } \right)}} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} - ib_{4} } \right)} \right)} \right)} \right)/ \hfill \\ \left( {\left( {1 + e^{{4\theta _{3} }} } \right)(\left( {2e^{{3\theta _{2} + 2\theta _{3} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + \theta _{3} + i\varphi _{2} + i\varphi _{3} }} + 2e^{{\theta _{2} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} } \right)b_{2} - ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)} \right. \hfill \\ \left( {a_{2} - a_{3} - ib_{3} } \right))\left( {2e^{{\theta _{2} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + \theta _{3} + i\varphi _{2} + i\varphi _{3} }} + 2e^{{3\theta _{2} + 2\theta _{3} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} } \right)b_{2} + ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \hfill \\ \left( {1 + e^{{4\theta _{2} }} } \right)\left( {a_{2} - a_{3} + ib_{3} } \right))) - ib_{4} )\left( { - a_{3} + a_{4} + ib_{3} - } \right.\left( {2ie^{{4\theta _{3} + 2i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)^{2} b_{3} \left( {e^{{ - \theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} (} \right.} \right.e^{{\int \beta (z){\kern 1pt} dz}} \hfill \\ \left( { - a_{2} + ib_{2} } \right) - \frac{{2ie^{{\theta _{2} - \theta _{3} - i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{2} } \right)}} + e^{{2i\varphi _{3} }} } \right)b_{2} }}{{1 + e^{{4\theta _{2} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{3} + ib_{3} } \right))(e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \hfill \\ \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{3} } \right)}} + e^{{2i\varphi _{4} }} } \right)b_{3} }}{{1 + e^{{4\theta _{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} + ib_{4} } \right)) + e^{{\theta _{3} + \theta _{4} + i\left( {\varphi _{3} + \varphi _{4} } \right)}} (e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{2} + ib_{2} } \right) + \hfill \\ e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{3} + ib_{3} } \right) - \frac{{2ie^{{\theta _{2} - \theta _{3} - i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {e^{{2\left( {\theta _{2} + \theta _{3} + i\varphi _{2} } \right)}} + e^{{2i\varphi _{3} }} } \right)b_{3} }}{{1 + e^{{4\theta _{2} }} }})(e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \hfill \\ \frac{{2ie^{{\theta _{3} - \theta _{4} - i\left( {\varphi _{3} + \varphi _{4} } \right)}} \left( {e^{{2\left( {\theta _{3} + \theta _{4} + i\varphi _{3} } \right)}} + e^{{2i\varphi _{4} }} } \right)b_{3} }}{{1 + e^{{2\left( {\theta _{3} + \theta _{4} } \right)}} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} + ib_{4} } \right))))/\left( {\left( {1 + e^{{4\theta _{3} }} } \right)(2e^{{3\theta _{2} + 2\theta _{3} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + \theta _{3} + i\varphi _{2} + i\varphi _{3} }} + } \right. \hfill \\ 2e^{{\theta _{2} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} )b_{2} - ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)\left( {a_{2} - a_{3} - ib_{3} } \right))(2e^{{\theta _{2} + 2i\varphi _{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta _{2} + \theta _{3} + i\varphi _{2} + i\varphi _{3} }} + \hfill \\ 2e^{{3\theta _{2} + 2\theta _{3} + 2i\varphi _{3} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} )b_{2} + ie^{{\int \beta (z){\kern 1pt} dz + \theta _{3} + i\left( {\varphi _{2} + \varphi _{3} } \right)}} \left( {1 + e^{{4\theta _{2} }} } \right)\left( {a_{2} - a_{3} + ib_{3} } \right)) + ib_{4} )( - a_{3} + a_{4} + \hfill \\ \frac{{i\left( {2{\text{B}}_{{31}} b_{2} + A_{3} b_{3} - A_{3} b_{4} } \right)}}{{A_{3} }})\left( { - a_{3} + a_{4} - \frac{{i\left( {A_{3} b_{3} - A_{3} b_{4} + 2b_{2} B_{4} } \right)}}{{A_{3} }}} \right) + e^{{4\int \beta (z){\kern 1pt} dz + 2\theta _{4} }} (e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} - ib_{3} } \right) \hfill \\ \end{gathered}$$
$$\begin{gathered} \frac{{2ie^{{\theta_{3} - \theta_{4} - i\left( {\varphi_{3} + \varphi_{4} } \right)}} \left( {e^{{2i\varphi_{3} }} + e^{{2\left( {\theta_{3} + \theta_{4} + i\varphi_{4} } \right)}} } \right)b_{3} }}{{1 + e^{{4\theta_{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} - ib_{4} } \right))(e^{{\int \beta (z){\kern 1pt} dz}} \left( { - a_{3} + ib_{3} } \right) - \hfill \\ \frac{{2ie^{{\theta_{3} - \theta_{4} - i\left( {\varphi_{3} + \varphi_{4} } \right)}} \left( {e^{{2\left( {\theta_{3} + \theta_{4} + i\varphi_{3} } \right)}} + e^{{2i\varphi_{4} }} } \right)b_{3} }}{{1 + e^{{4\theta_{3} }} }} + e^{{\int \beta (z){\kern 1pt} dz}} \left( {a_{4} + ib_{4} } \right))(\left( { - a_{3} + a_{4} - ib_{3} + } \right.2e^{{2\theta_{3} - 2\theta_{4} + i\left( {\varphi_{2} - \varphi_{3} - 2\varphi_{4} } \right)}} \left( {1 + e^{{4\theta_{2} }} } \right) \hfill \\ \left( {e^{{2\left( {\theta_{3} + \theta_{4} } \right)}} + e^{{2i\left( {\varphi_{3} + \varphi_{4} } \right)}} } \right)b_{3} \left( { - i\left( {2e^{{\theta_{3} + 2i\varphi_{3} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{3} + \theta_{4} + i\varphi_{3} + i\varphi_{4} }} + 2e^{{3\theta_{3} + 2\theta_{4} + 2i\varphi_{4} }} - e^{{\int \beta (z){\kern 1pt} dz + \theta_{4} + i\left( {\varphi_{3} + \varphi_{4} } \right)}} } \right)b_{3} + } \right. \hfill \\ \left. {\left. {e^{{\int \beta (z){\kern 1pt} dz + \theta_{4} + i\left( {\varphi_{3} + \varphi_{4} } \right)}} \left( {1 + e^{{4\theta_{3} }} } \right)\left( {a_{3} - a_{4} + ib_{4} } \right)} \right)} \right)/(\left( {1 + e^{{4\theta_{3} }} } \right)(2e^{{\theta_{2} }} \left( {e^{{2\left( {\theta_{2} + \theta_{3} + i\varphi_{2} } \right)}} + e^{{2i\varphi_{3} }} } \right)b_{1} + e^{{\theta_{3} }} (2e^{{3\theta_{2} + 5\theta_{3} + 2i\varphi_{2} }} - \hfill \\ e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{2} + 4\theta_{3} + i\varphi_{2} + i\varphi_{3} }} + 2e^{{\theta_{2} + 3\theta_{3} + 2i\varphi_{3} }} - e^{{\int \beta (z){\kern 1pt} dz + i\left( {\varphi_{2} + \varphi_{3} } \right)}} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{2} + i\left( {\varphi_{2} + \varphi_{3} } \right)}} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{3} + i\left( {\varphi_{2} + \varphi_{3} } \right)}} )b_{2} - \hfill \\ \left. {\left. {\left. {ie^{{\int \beta (z){\kern 1pt} dz + \theta_{3} + i\left( {\varphi_{2} + \varphi_{3} } \right)}} \left( {1 + e^{{4\theta_{2} }} } \right)\left( {1 + e^{{4\theta_{3} }} } \right)\left( {a_{2} - a_{3} - ib_{3} } \right)} \right)} \right) - ib_{4} } \right)\left( { - a_{3} + a_{4} + ib_{3} + } \right. \hfill \\ (2e^{{2\theta_{3} - 2\theta_{4} + i\left( {\varphi_{2} - \varphi_{3} - 2\varphi_{4} } \right)}} \left( {1 + e^{{4\theta_{2} }} } \right)\left( {1 + e^{{2\left( {\theta_{3} + \theta_{4} + i\left( {\varphi_{3} + \varphi_{4} } \right)} \right)}} } \right)b_{3} i(2e^{{3\theta_{3} + 2\theta_{4} + 2i\varphi_{3} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{3} + \theta_{4} + i\varphi_{3} + i\varphi_{4} }} + 2e^{{\theta_{3} + 2i\varphi_{4} }} - \hfill \\ e^{{\int \beta (z){\kern 1pt} dz + \theta_{4} + i\left( {\varphi_{3} + \varphi_{4} } \right)}} )b_{3} + e^{{\int \beta (z){\kern 1pt} dz + \theta_{4} + i\left( {\varphi_{3} + \varphi_{4} } \right)}} \left( {1 + e^{{4\theta_{3} }} } \right)\left( {a_{3} - a_{4} - ib_{4} } \right)))/\left( {\left( {1 + e^{{4\theta_{3} }} } \right)\left( {2e^{{\theta_{2} }} \left( {e^{{2i\varphi_{2} }} + e^{{2\left( {\theta_{2} + \theta_{3} + i\varphi_{3} } \right)}} } \right)b_{1} + e^{{\theta_{3} }} } \right.} \right. \hfill \\ \left( {2e^{{\theta_{2} + 3\theta_{3} + 2i\varphi_{2} }} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{2} + 4\theta_{3} + i\varphi_{2} + i\varphi_{3} }} + 2e^{{3\theta_{2} + 5\theta_{3} + 2i\varphi_{3} }} - e^{{\int \beta (z){\kern 1pt} dz + i\left( {\varphi_{2} + \varphi_{3} } \right)}} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{2} + i\left( {\varphi_{2} + \varphi_{3} } \right)}} - e^{{\int \beta (z){\kern 1pt} dz + 4\theta_{3} + i\left( {\varphi_{2} + \varphi_{3} } \right)}} } \right)b_{2} + \hfill \\ \left. {\left. {\left. {ie^{{\int \beta (z){\kern 1pt} dz + \theta_{3} + i\left( {\varphi_{2} + \varphi_{3} } \right)}} \left( {1 + e^{{4\theta_{2} }} } \right)\left( {1 + e^{{4\theta_{3} }} } \right)\left( {a_{2} - a_{3} + ib_{3} } \right)} \right)} \right) + ib_{4} } \right)\left( { - a_{3} + a_{4} + \frac{{i\left( {2{\text{B}}_{31} b_{2} + A_{3} b_{3} - A_{3} b_{4} } \right)}}{{A_{3} }}} \right) \hfill \\ \left( { - a_{3} + a_{4} - \frac{{i\left( {A_{3} b_{3} - A_{3} b_{4} + 2b_{2} B_{3} } \right)}}{{A_{3} }}} \right)) \hfill \\ \end{gathered}$$
(19)

where

$$\begin{aligned} \theta_{1} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{1} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{1} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{1} b_{1} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{1}^{2} b_{1} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{1}^{3} \\ \end{aligned}$$
$$\begin{aligned} \theta_{2} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{2} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{2} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{2} b_{2} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{2}^{2} b_{2} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{2}^{3} \\ \end{aligned}$$
$$\begin{aligned} \theta_{3} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{3} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{3} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{3} b_{3} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{3}^{2} b_{3} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{3}^{3} \\ \end{aligned}$$
$$\begin{aligned} \theta_{4} = & - {\text{exp(}}\smallint \beta (z){\text{d}}z)tb_{4} - \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)b_{4} - 4\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{4} b_{4} \\ & + 12\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{4}^{2} b_{4} - 4\left( {\smallint {\text{exp(}}3\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)b_{4}^{3} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{1} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{1} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{1} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{1}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{1}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{1}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{1} b_{1}^{2} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{2} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{2} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{2} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{2}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{2}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{2}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{2} b_{2}^{2} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{3} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{3} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{3} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{3}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{3}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{3}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{3} b_{3}^{2} \\ \end{aligned}$$
$$\begin{aligned} \varphi_{4} \,{ = }\, & {\text{exp(}}\smallint \beta (z){\text{d}}z)ta_{4} + \left( {\smallint {\text{exp(}}\smallint \beta (z){\text{d}}z)\alpha (z){\text{d}}z} \right)a_{4} + 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)a_{4}^{2} \\ & - \,4\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{4}^{3} - 2\left( {\smallint {\text{exp(2}}\smallint \beta (z){\text{d}}z)\gamma (z){\text{d}}z} \right)b_{4}^{2} + 12\left( {\smallint {\text{exp(3}}\smallint \beta (z){\text{d}}z)\delta (z){\text{d}}z} \right)a_{4} b_{4}^{2} \\ \end{aligned}$$

and \(A_{3} {, }B_{3} {, }B_{31}\) is trigonometric functions.

4 Result and discussion

From the derived exact four soliton solution, we can fully understand the dynamics of four solitons in optical fiber system. To generate the applications and investigate the physical properties of the obtained four soliton solution of Eq. (1) needs a particular choice of the arbitrary functions and also the arbitrary coefficients are used to control the dynamics of the solitons.

4.1 Exponential profile

By considering the coefficients of nonautonomous terms are set to zero, the system (1) ultimately indicates the autonomous nonlinear Schrödinger equation system. Then the autonomous and nonautonomous terms have the following form (Mani Rajan et al. 2020; Karthikeyaraj et al. 2019; Prathap et al. 2019),

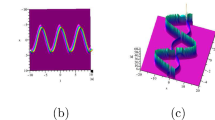
$$\beta (z) = 0,\,\alpha (z) = 0,\,\gamma (z) = z,\,\delta (z) = {\text{Exp(}} - z{)}{\text{.}}$$
(20)

By using the aforementioned function to the Eq. (19), the four-soliton solution will be interacting at \(z = 0\), and then the solitons regain their original shape after interaction. The four soliton's amplitude and width are invariant during the propagation in a nonlinear fiber. The exponential profile for the autonomous solitons are represented in Fig. 1(a) and (b) represents the contour plot. We hope the above results are useful for high-capacity long distance optical fiber communications.

Fig. 1
figure 1

The evolution of the autonomous four soliton solution a when \({\text{a}}_{1} = - 1.96,{\text{b}}_{1} = - 2.91,{\text{a}}_{2} = 0.89,{\text{b}}_{2} = 1.86,{\text{a}}_{3} = - 1.13,{\text{b}}_{3} = 1.5,{\text{a}}_{4} = - 0.63,{\text{b}}_{4} = - 0.9.\) b Corresponding contour plot

Full size image

With the consideration of nonautonomous terms, the dynamics of four solitons are different from the autonomous case. For the nonautonomous soliton, the arbitrary parameters having the following form,

$$\beta (z) = z,\,\alpha (z) = {\text{Exp(}} - z),\,\gamma (z) = z,\,\delta (z) = {\text{Exp(}} - z)$$
(21)

The propagation property of nonautonomous solitons is portrayed in Fig. 2(a) by fixing the above parameters in Eq. (19). There is a slight difference in between autonomous and nonautonomous plots. Nonautonomous solitons are comparable to autonomous solitons in that they both have the same amplitude. After and before the interaction, the solitons are compressed, becoming narrower and narrower as they propagate. We can easily verify the compression behavior from the contour plots Fig. 2(b). The compression profile can be used to achieve the ultrashort pulse.

Fig. 2
figure 2

The evolution of the nonautonomous four soliton solution a when \({\text{a}}_{1} = 1.5,{\text{b}}_{1} = 1.9,{\text{a}}_{2} = 1.9,{\text{b}}_{2} = - 2.1,{\text{a}}_{3} = 0.9,{\text{b}}_{3} = - 1.5,{\text{a}}_{4} = 0.7,{\text{b}}_{4} = 1.9.\) b Corresponding contour plot

Full size image

4.2 Soliton fission

For the choice of Hyperbolic profile (22) in the obtained four soliton solutions, soliton splitting behavior is observed. Few novel works have been carried out by several researchers using hyperbolic profile (Dai et al. 2012; Arun Prakash et al. 2016),

$$\beta (z) = 0,\,\alpha (z) = 0,\,\gamma (z) = z,\,\delta (z) = \frac{L}{{\left( {C - 1} \right)z + L}}.$$
(22)

where \(C\) is an arbitrary constant and \(L\) is a length of the medium. For the lower value of \(C\), the four solitons are closely packed together. Initially four solitons emerge from \(t = 0\), the distance between the four solitons will be increases as they propagate in the medium. During the propagation, the amplitude and width of the solitons are invariant during the travel in the medium and the four solitons are do not interact with each other which is shown in the Fig. 3(a) and (b). This kind of analysis will be beneficial to achieve the high-capacity transmission networks in soliton-based communication system. Similarly for higher value of \(C\), the separation between solitons is increasing rapidly. Hence, C is a key parameter to control the soliton’s width in the optical communication system.

Fig. 3
figure 3

The evolution of the autonomous four soliton solution a when \({\text{a}}_{1} = 0.2,{\text{b}}_{1} = 0.9,{\text{a}}_{2} = - 0.6,{\text{b}}_{2} = - 1.1,{\text{a}}_{3} = - 0.2,{\text{b}}_{3} = - 0.8,{\text{a}}_{4} = - 0.5,{\text{b}}_{4} = 1.1,\) \({\text{C = 24, L = 15}}{.}\) b Corresponding contour plot

Full size image

Since the present work is mainly focused on the study of nonautonomous solitons, the nonautonomous coefficients are included and having the following form,

$$\beta \left( z \right) = 1,\,\,\alpha (z) = \frac{L}{(C - 1)z + L}\,\,\gamma (z) = 1\,\,\delta (z) = \frac{L}{(C - 1)z + L}$$
(23)

Initially the nonautonomous solitons are closely packed together, when \(z > 0\) then the distance between solitons is gradually increases as they propagate. When comparing with the autonomous case, the spacing between solitons grows significantly and effectively compressed as clearly depicted in Fig. 4(a) and b.

Fig. 4
figure 4

The evolution of the nonautonomous four soliton solution a when \({\text{a}}_{1} = 1.2,{\text{b}}_{1} = 1.4,{\text{a}}_{2} = 1.1,{\text{b}}_{2} = - 1.6,{\text{a}}_{3} = 0.,{\text{b}}_{3} = 1.0,{\text{a}}_{4} = 0.6,{\text{b}}_{4} = - 1.3,\)\({\text{C = 24, L = 15}}{.}\) b Corresponding contour plot

Full size image

4.3 Phase shifted Soliton

Consider the optical pulse compression problem in a dispersion decreasing optical cable. For this sake we consider the arbitrary parameter as following (Porsezian et al. 2007),

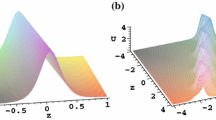
$$\beta (z) = 0,\,\alpha (z) = 0,\,\gamma (z) = z,\,\delta (z) = \frac{1}{z}.$$
(24)

For the choice of above parameter, the resultant soliton amplitude is not changing during the propagation. The solitons are emerging from \(t = 6\), initially the four solitons are merged together, after that the solitons are keeping their propagation in a linear path. The Fig. 5(a) provides the soliton management regime under the above parameters. From the density plot Fig. 5(b), we may infer that the soliton width is constant throughout the inhomogeneous fiber medium which indicates the dispersion less soliton propagation under the management of inhomogeneous functions.

Fig. 5
figure 5

The evolution of the autonomous four soliton solution a when \({\text{a}}_{1} = - 0.9,{\text{b}}_{1} = 1.9,{\text{a}}_{2} = - 0.2,{\text{b}}_{2} = 0.7,{\text{a}}_{3} = - 0.1,{\text{b}}_{3} = - 1.1,{\text{a}}_{4} = 0.1,{\text{b}}_{4} = 1.2.\) b Corresponding contour plot

Full size image

In order to demonstrate the influence of nonautonomous terms on the soliton dynamics, the variable coefficients are considered as following,

$$\beta (z) = 1,\,\alpha (z) = 1,\,\gamma (z) = z,\,\delta (z) = \frac{1}{z}.$$
(25)

For the choice of functions as given in the expression (25), amplitude and width of the solitons are varying significantly along the optical fiber medium. In an optical fiber communication system, this effect can be applied to control the pulse in an effective manner. It should be mention that all four solitons are getting parabolic trajectory as clearly depicted in Fig. 6(a) and the contour plot is represented in Fig. 6(b). This kind of soliton shaping in a dispersion fiber is reported recently (Liu et al. 2016). This result will helpful to control the soliton direction in an inhomogeneous optical fiber medium.

Fig. 6
figure 6

The evolution of the nonautonomous four soliton solution a when \({\text{a}}_{1} = 1.7,{\text{b}}_{1} = - 1.2,{\text{a}}_{2} = 2.8,{\text{b}}_{2} = 0.9,{\text{a}}_{3} = - 5.7,{\text{b}}_{3} = 2.2,{\text{a}}_{4} = - 3.9,{\text{b}}_{4} = 1.7.\) b Corresponding contour plot

Full size image

4.4 S-shaped soliton

Soliton control through variable coefficients is a new technology. By properly selecting the profile for variable coefficients, one can get desirable shape for the soliton. As an example, we attained S-Shaped autonomous solitons as follows (Xia et al. 2016; Karthikeyaraj et al. 2018),

$$\beta (z) = 0,\,\alpha (z) = 0,\,\gamma (z) = s{\text{ech(z + 5),}}\,\delta (z) = c{\text{osh(z + 5)}}.$$
(26)

The choice of above parameters which provides the S-shaped soliton results in soliton attraction with compressed structure. Recently, for the higher order nonlinear Schrödinger equation with variable coefficients, similar structure has been observed (Feng et al. 2015; Yang et al. 2017). The S-shaped autonomous solitons are represented in Fig. 7(a) and with respective contour plot is depicted in Fig. 7(b).

Fig. 7
figure 7

The evolution of the autonomous four soliton solution a when \({\text{a}}_{1} = - 0.6,{\text{b}}_{1} = 1.05,{\text{a}}_{2} = - 0.2,{\text{b}}_{2} = - 0.9,{\text{a}}_{3} = - 0.1,{\text{b}}_{3} = - 0.5,{\text{a}}_{4} = - 0.3,{\text{b}}_{4} = 0.6.\) b Corresponding contour plot

Full size image

In the case of nonautonomous S-Shaped soliton, the following parameters was chosen,

$$\beta \left( z \right) = 0.1,\,\,\alpha (z) = {\text{Cos(z + 5),}}\,\,\gamma (z) = {\text{Sech(z + 5),}}\,\,\delta (z) = {\text{Cosh(z + 5)}}.$$
(27)

When nonautonomous terms taken into account, width of the solitons is mainly affected as shown in Fig. 8. Especially, on both sides of the tails in the S-shape, solitons getting compression. The wave width is gradually decreases, as they propagate in the medium. We can easily verify the compression behavior of S-shaped soliton from the contour plot. The nonautonomous S-Shaped soliton is represented in the Fig. 8(a) with corresponding contour plot is represented in Fig. 8(b). Using various analytical methods, transmission of optical solitons has been investigated through obtained soliton solutions for many types of nonlinear equations (Guo et al. 2020, Song et al. 2020; Shen et al. 2021, 2022; Shan et al. 2022).

Fig. 8
figure 8

The evolution of the nonautonomous four soliton solution a when \({\text{a}}_{1} = - 0.6,{\text{b}}_{1} = - 0.65,{\text{a}}_{2} = - 0.3,{\text{b}}_{2} = - 0.6,{\text{a}}_{3} = - 0.4,{\text{b}}_{3} = - 0.8,{\text{a}}_{4} = - 0.7,{\text{b}}_{4} = - 0.9.\) b Corresponding contour plot

Full size image

5 Conclusion

In this paper, we generate three and four soliton solutions for generalized nonautonomous Hirota equation that can be used to describe the soliton propagation in an inhomogeneous nonlinear optical fiber. By employing Darboux transformation method based on the Lax pair, three and four soliton solutions are attained. The properties of obtained four soliton solutions are investigated through some graphical illustrations. In order to understand the soliton solutions related to physical problems, various dynamical behaviors of four solitons are examined through properly selecting the variable coefficients. Through the tailoring of arbitrary functions, different soliton properties have been explained in detail. Obtained results are connected to some optical applications such as soliton management, optical switching, route controlling devices etc.