On the quintic time-dependent coefficient derivative nonlinear Schrödinger equation in hydrodynamics or fiber optics

Abstract

Under investigation in this paper is a quintic time-dependent coefficient derivative nonlinear Schrödinger equation for certain hydrodynamic wave packets or a medium with the negative refractive index. A gauge transformation is found to obtain the equivalent form of the equation. With respect to the wave envelope for the free water surface displacement or envelope of the electric field, Painlevé integrable condition, different from that in the existing literature, is derived, with which the bilinear forms and N-soliton solutions are constructed. Asymptotic analysis illustrates that the interactions between the bright and bound solitons as well as between the bright solitons and Kuznetsov–Ma breathers are elastic with certain conditions, while some other interactions are inelastic under other conditions. Propagation paths and velocities for the solitons are both affected by the dispersion coefficient function when the relations among the coefficients are linear, or affected by the dispersion coefficient, self-steepening coefficient and cubic nonlinearity functions when the relations among the coefficients are nonlinear. Under different conditions, bell-shaped solitons can evolve into the bound solitons or Kuznetsov–Ma breathers, respectively. Interactions between the bright and parabolic (or hyperbolic) solitons are related to the dispersion coefficient, self-steepening coefficient and cubic nonlinearity functions. Compression effect on the propagation paths of the solitons, caused by the dispersion coefficient, is observed.

1 Introduction

In recent years, there have been a variety of researches on the nonlinear evolution equations (NLEEs) [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27]. Studies on the interactions of the localized waves have been extended to the higher-order and higher-dimensional NLEEs [8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27] and multi-component coupled NLEEs [28,29,30,31,32,33,34,35,36,37].

Derivative nonlinear Schrödinger (DNLS) equations have been investigated due to the applications in plasmas, fluids and fiber optics [38,39,40,41,42,43,44,45]. Quintic DNLS equations have been applied in the media with negative refractive indices, inhomogeneous plasmas and hydrodynamic wave packets [46,47,48,49,50,51]. A quintic time-dependent coefficient DNLS equation,

$$\begin{aligned} i{u_t} + \lambda \left( t \right) {u_{xx}} + i\alpha \left( t \right) {\left| u \right| ^2}{u_x} + \mu \left( t \right) {\left| u \right| ^2}u + \nu \left( t \right) {\left| u \right| ^4}u = 0, \end{aligned}$$

(1)

has been used to describe certain hydrodynamic wave packets [48] or a medium with the negative refractive index [51], where $i=\sqrt{-1}$, u(x, t) is the wave envelope for the free water surface displacement or envelope of the electric field [50], $\mu (t)$ and $\nu (t)$ represent the cubic and quintic nonlinearities, respectively, $\lambda (t)$ denotes the dispersion coefficient, $\alpha (t)$ is the self-steepening coefficient, t and x denote not only the slow time and spatial coordinate traveling with the group velocity in hydrodynamics, but also the propagation distance and retarded time in the context of optical fiber physics [48, 51]. A class of the chirped soliton-like solutions including the bright and kink solitons for Eq. (1) has been derived via the trial equation method [51]. Special cases of Eq. (1) have been seen as follows:

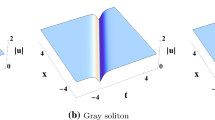
• When $[{\lambda ( t ),~\alpha ( t ),~\mu ( t ),~\nu ( t )}] = [ {1,~-1,~0,~\frac{1}{2}} ]$, Eq. (1) has been reduced to the Gerdjikov–Ivanov equation in the Madelung fluid [47]. Constraints on the soliton types have been derived, including the bright soliton, dark soliton, up-shifted bright, upper-shifted bright, gray soliton and black soliton types [47].

• When $\lambda (t)$, $\alpha (t)$, $\mu (t)$ and $\nu (t)$ are the constant coefficients, Eq. (1) has been reduced to the quintic DNLS equation in hydrodynamics or fiber optics [48, 50], to describe how a water wave packet deforms and eventually is destroyed as it propagates shoreward from the deep to shallow water via the Newton–Raphson method [50]. “Gray” soliton on a continuous-wave background, i.e., the “dark” localized mode with a nonzero minimum in the intensity, has been derived via two integrals of motion [48].

• When $[{\lambda (t),~\alpha (t),~\mu (t),~\nu (t)}] = [{1,~1,~0,~0}]$, Eq. (1) has been reduced to the Chen–Lee–Liu equation for the nonlinear optical pulses in a quadratic nonlinear crystal involving the self-steepening without any concomitant self-phase-modulation [43], with the soliton, breather, multi-rogue wave and rational solutions constructed [45].

Painlevé analysis has been used to derive the Painlevé integrable condition and transformation for the bilinear forms [52,53,54,55]. Asymptotic analysis has been used to investigate the solitons before and after the interactions, with which the relevant physical properties of the solitons have been derived [56, 57].

However, to our knowledge, under the constraint different from that in Ref. [48], the effects of the time-dependent coefficients $\alpha (t)$, $\varLambda (t)$, $\mu (t)$ and $\nu (t)$ on the interactions among the solitons for Eq. (1) have not been investigated. In Sect. 2, we will give the gauge transformation for an equivalent form of Eq. (1). In Sect. 3, Painlevé integrable condition for Eq. (1), different from that in Ref. [48], will be derived. In Sect. 4, we will obtain the bilinear forms and N-soliton solutions for Eq. (1). In Sect. 5, asymptotic analysis on the interactions among the solitons will be conducted. In Sect. 6, influence of $\alpha (t)$, $\varLambda (t)$, $\mu (t)$ and $\nu (t)$ on the interactions will be discussed. In Sect. 7, we will give the conclusions.

2 Equivalent form of Eq. (1)

Motivated by Ref. [58], introducing the gauge transformation

$$\begin{aligned} \tilde{u} = u{\mathrm{e}}^{-\frac{1}{2}i\kappa \int {\left| u \right| ^2\mathrm{d}x} }, \end{aligned}$$

(2)

we hereby find that the equation

$$\begin{aligned}&i{{\tilde{u}}_t} + \lambda \left( t \right) {{\tilde{u}}_{xx}} + i\alpha \left( t \right) {({\left| {\tilde{u}} \right| ^2}\tilde{u})_x} + \mu \left( t \right) {\left| {\tilde{u}} \right| ^2}\tilde{u}\nonumber \\&\quad + \nu \left( t \right) {\left| {\tilde{u}} \right| ^4}\tilde{u} = 0 \end{aligned}$$

(3)

can be transformed to Eq. (1), where $\kappa =\frac{\lambda (t)}{\alpha (t)}$ is a nonzero real constant. Meanwhile, $\left| {\tilde{u}} \right| = \left| u \right|$ indicates that Transformation (2) amounts to an amplitude-dependent phase shift. We have found that Eq. (3) is the equivalent form of Eq. (1).¹

3 Painlevé analysis for Eq. (1)

Motivated by Refs. [53, 54], Painlevé integrability for Eq. (1) can be analyzed via the coupled system,

$$\begin{aligned}&i{u_t} + \lambda \left( t \right) {u_{xx}} + \mu \left( t \right) {u^2}v + i\alpha \left( t \right) uv{u_x}\nonumber \\&\quad + \nu \left( t \right) {u^3}{v^2} = 0, \end{aligned}$$

(4a)

$$\begin{aligned}&i{v_t} - \lambda \left( t \right) {v_{xx}} - \mu \left( t \right) {v^2}u + i\alpha \left( t \right) vu{v_x}\nonumber \\&\quad - \nu \left( t \right) {v^3}{u^2} = 0, \end{aligned}$$

(4b)

where $v=u^*$ and $*$ denotes the complex conjugate.

Motivated by Ref. [55], the solutions for Eq. (4) can be expanded in terms of the Laurent series, as follows:

$$\begin{aligned} u = {\phi ^{ - a\gamma }}\sum \limits _{j = 0}^{+\infty } {{q_j}^a{\phi ^j}},~v = {\phi ^{ - b\beta }}\sum \limits _{j = 0}^{+\infty } {{r_j}^b{\phi ^j}}, \end{aligned}$$

(5)

where $\phi$, $q_j$ and $r_j$ are the analytic functions with respect ro x and t, j is a nonnegative integer, a and b are both the real constants, while $\gamma$ and $\beta$ are both the positive integers.

The leading orders of the solutions for Eq. (4) are assumed as

$$\begin{aligned} u\sim {q_0^a}\,{\phi ^{ -a \gamma }},~~v\sim {r_0^b}\,{\phi ^{ -b \beta }}, \end{aligned}$$

(6)

where ${r_0}$ and ${q_0}$ are nonzero in the neighborhood of a non-characteristic movable singularity manifold. Substituting Expressions (6) into Eq. (4) and balancing the highest-order nonlinear and linear terms, we obtain

$$\begin{aligned}&a\,\gamma + b\,\beta = 1, \end{aligned}$$

(7a)

$$\begin{aligned}&{q_0}^a\,{r_0}^b = 2i( 1 - 2a\gamma )\,\frac{{\lambda \left( t \right) }}{{\alpha \left( t \right) }}\,{\phi _x}, \end{aligned}$$

(7b)

and derive the variable-coefficient constraint as

$$\begin{aligned} \alpha {\left( t \right) ^2} + \frac{{4{{\left( {2a\gamma - 1 } \right) }^2}}}{{3a\gamma \left( { a\gamma - 1 } \right) }}\lambda \left( t \right) \nu \left( t \right) = 0. \end{aligned}$$

(8)

Then, to find the resonances, substituting

$$\begin{aligned} u\sim {q_0}^a{\phi ^{ - a\gamma }} + {q_j}^a{\phi ^{ - a\gamma + j}},~ v\sim {r_0}^b{\phi ^{ - b\beta }} + {r_j}^b{\phi ^{ - b\beta + j}} \end{aligned}$$

(9)

into Eq. (4), we make the sum of the terms with the lowest power of $\phi$ in Eqs. (4a) and (4b) to vanish, respectively. Due to the arbitrariness of the corresponding ${q_j}$ and ${r_j}$ for the resonance point j, we can obtain

$$\begin{aligned} \left( { j-3 } \right) \left( { j - 2 } \right) j\left( {j + 1} \right) \frac{{{16{\left( { 2a\gamma - 1 } \right) }^4}}}{{9{a^2}{\gamma ^2}{{( a\gamma - 1 )}^2}}}{\nu (t)}^2{\lambda ( t)}^2 =0. \end{aligned}$$

(10)

Due to Eq. (10), the resonances occur at $j= -1,~0,~2$ and 3, while $j=-1$ corresponds to the arbitrariness of $\phi$.

To find the compatibility conditions for Eq. (4), We truncate Expression (5) at $j=3$ as

$$\begin{aligned} u = {\phi ^{ - a\gamma }}\sum \limits _{j = 0}^3 {{q_j}^a{\phi ^j}},~v = {\phi ^{ - b\beta }}\sum \limits _{j = 0}^3 {{r_j}^b{\phi ^j}}, \end{aligned}$$

(11)

and substitute Expressions (11) and Constraint (8) into Eq. (4). We make the coefficients of ${\phi ^{ - a\alpha - 2 - j}}$ in Eq. (4a) and ${\phi ^{ a\alpha - 3 - j}}$ in Eq. (4b) at $j=0,~2$ and 3 to vanish, so that we find that the compatibility condition at $j=0,~2$ and 3 is satisfied identically with $a=\frac{1}{2}(1\pm \sqrt{3})$, $b=\frac{1}{2}(1\mp \sqrt{3})$ and $\gamma =\beta =1$. The compatibility condition is derived from Constraint (8), as

$$\begin{aligned} {\alpha (t)}^2 + 8\lambda \left( t \right) \nu \left( t \right) = 0, \end{aligned}$$

(12)

which is different from that in Ref. [48]. Therefore, under variable-coefficient constraint (12), Eq. (1) is Painlevé integrable.

4 Bilinear forms and soliton solutions for Eq. (1)

Due to Constraint (12), introducing the transformation

$$\begin{aligned} u = \frac{g}{f}, \end{aligned}$$

(13)

substituting Eqs. (13) into (1), we derive the bilinear forms for Eq. (1) as

$$\begin{aligned}&\left[ {i{D_t} + \lambda \left( t \right) D_x^2} \right] \left( {g \cdot f} \right) = 0, \end{aligned}$$

(14a)

$$\begin{aligned}&\lambda \left( t \right) {D_x}\left( {f \cdot {f^ * }} \right) - \frac{1}{2}i\alpha \left( t \right) {\left| g \right| ^2} = 0, \end{aligned}$$

(14b)

$$\begin{aligned}&\lambda \left( t \right) D_x^2\left( {f \cdot {f^ * }} \right) - \frac{1}{2}i\alpha \left( t \right) {D_x}\left( {g \cdot {g^ * }} \right) - \mu \left( t \right) {\left| g \right| ^2} = 0, \end{aligned}$$

(14c)

where g and f are the complex differential functions and the bilinear operators $D_{t}$ and $D_{x}$ are defined by [1, 2, 61]

$$\begin{aligned} \begin{aligned} D_x^p D_t^q \left( {\varPhi \cdot \varPsi } \right)&= {{{\left( {\frac{\partial }{{\partial x}} - \frac{\partial }{{\partial x'}}}\right) }^p}{{\left( {\frac{\partial }{{\partial t}} - \frac{\partial }{{\partial t'}}} \right) }^q}} \\&\quad \times {\varPhi \left( {x,t} \right) \varPsi \left( {x',t'} \right) }|_{t' = t,x' = x}, \end{aligned} \end{aligned}$$

(15)

where $\varPhi (x,t)$ is a differentiable function with respect to x and t, $\varPsi (x^{\prime },t^{\prime })$ is a differentiable function with respect to the formal variables $x^{\prime }$ and $t^{\prime }$, while p and q are both the positive integers.

Note that the N-soliton solutions for Eq. (1) are marked as $u_N$, where N is a positive integer. Based on Eqs. (13) and (14), expanding f and g, the N-soliton solutions for Eq. (1) can be expressed as

$$\begin{aligned} u_{N}=\frac{g}{f}, \end{aligned}$$

(16)

with

$$\begin{aligned} f = 1 + \sum \limits _{n = 1}^N {{\varepsilon ^{2n}}{f_{2n}}},~~~~g = \sum \limits _{n = 1}^N {{\varepsilon ^{2n - 1}}{g_{2n - 1}}}, \end{aligned}$$

(17)

where n is a positive integer and $\varepsilon$ is a formal expansion parameter.

4.1 One-soliton solutions for Eq. (1)

Truncating Eq. (17) at $N=1$ and setting $\varepsilon =1$, we solve Eq. (14) and obtain the analytic one-soliton solutions for Eq. (1) as

$$\begin{aligned} \begin{aligned} u_{1}&=\frac{ g_{1} }{ 1+ f_{2} }\\&=\frac{{{m_1}}}{{2\sqrt{{m_{12}}} }}\text {sec}h\left[ {\text {Re}(\theta _1) + \ln {\sqrt{{m_{12}}} } } \right] {{\mathrm{e}}^{i\text {Im}(\theta _1)}}, \end{aligned} \end{aligned}$$

(18)

with

$$\begin{aligned} {\theta _1}= & {} {k_1}x +ik^{2}_{1}\int \lambda (t)\hbox {d}t,~~{g_1} = {m_1}{\hbox {e}^{{\theta _1}}},\\ {f_2}= & {} {m_{12}}{{\mathrm{e}}^{{\theta _1} + \theta _1^*}},~~{k_1} = i\frac{{{\mathrm{}}{m_1}{m_{12}}m_1^ * \alpha \left( t \right) }}{{8{{\left[ \text {Im}(m_{12}) \right] }^2}\lambda \left( t \right) }} + i\frac{{\mu \left( t \right) }}{{\alpha \left( t \right) }}, \end{aligned}$$

where $m_1$ and $m_{12}$ are the complex constants, $\text {Im}(\bullet )$ and $\text {Re}(\bullet )$ denote the imaginary and real parts of $\bullet$, respectively. Particularly, $\text {Im}(m_{12})\ne 0$.

4.2 Two-soliton solutions for Eq. (1)

Truncating Eq. (17) at $N=2$ and setting $\varepsilon =1$, similar to the process in Sect. 4.1, we can derive the analytic two-soliton solutions for Eq. (1) as

$$\begin{aligned} u_{2}=\frac{ g_{1} + g_{3}}{1+ f_{2} + f_{4}}, \end{aligned}$$

(19)

with

$$\begin{aligned}&{\theta _j} = {k_j}x + ik^{2}_{j}\int \lambda (t)\hbox {d}t + \delta _{j}, ~{g_1} ={\hbox {e}^{{\theta _1}}} + {\hbox {e}^{{\theta _2}}},\\&{g_3} = {m_{123}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _1^*}} + {m_{124}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _2^*}},\\&{f_2} = {m_{13}}{{\mathrm{e}}^{{\theta _1} + \theta _1^*}} + {m_{24}}{{\mathrm{e}}^{{\theta _2} + \theta _2^*}} + {m_{23}}{{\mathrm{e}}^{{\theta _2} + \theta _1^*}}\\&\qquad \quad +\,{m_{14}}{{\mathrm{e}}^{{\theta _1} + \theta _2^*}},\\&{m_{{s_1},{s_2}+2}} = \frac{{\left[ {i{k_{s_1}}\alpha \left( t \right) + \mu \left( t \right) } \right] }}{{2{{\left( {{k_{s_1}} + k_{s_2}^ * } \right) }^2}\lambda \left( t \right) }},\\&{f_4} = {m_{1234}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _1^* + \theta _2^*}},\\&{m_{{s_1},{s_2},{s_3}+2}} = \frac{{{{\left( {{k_{s_1}} - {k_{s_2}}} \right) }^2}\left[ { ik_{s_3}^ * \alpha \left( t \right) + \mu \left( t \right) } \right] }}{{2{{\left( {{k_{s_1}} + k_{s_3}^ * } \right) }^2}{{\left( {{k_{s_2}} + k_{s_3}^ * } \right) }^2}\lambda \left( t \right) }},\\&{m_{1234}} = \frac{{{{\left( {{k_1} - {k_2}} \right) }^2}{{\left( {k_1^ * - k_2^ * } \right) }^2}\left[ {i{k_2}\alpha \left( t \right) +\mu \left( t \right) } \right] }}{{4{{\left( {{k_1} + k_1^ * } \right) }^2}{{\left( {{k_2} + k_1^ * } \right) }^2}{{\left( {{k_1} + k_2^ * } \right) }^2}{{\left( {{k_2} + k_2^ * } \right) }^2}}}\\&~~~~~~~~\qquad \times \left[ {i{k_1}\alpha \left( t \right) +\mu \left( t \right) } \right] {\lambda {\left( t \right) }}^{-2}, \end{aligned}$$

where $\delta _{j}$’s $(j=1,2)$ are the real constants, $s_1 < s_2$ and $s_1,s_2,s_3=1,~2$.

4.3 Three-soliton solutions for Eq. (1)

Truncating Eq. (17) at $N=3$ and setting $\varepsilon =1$, similar to the process in Sect. 4.2, we can derive the analytic three-soliton solutions for Eq. (1) as

$$\begin{aligned} u_{3}= \frac{{{g_1} + {g_3} + {g_5}}}{{1 + {f_2} + {f_4} + {f_6}}}, \end{aligned}$$

(20)

with

$$\begin{aligned}&{g_1} = {\hbox {e}^{{\theta _1}}} + {\hbox {e}^{{\theta _2}}} + {\hbox {e}^{{\theta _3}}},\\&{f_6} = m_{123456}~{{\mathrm{e}}^{{\theta _1} + {\theta _2} + {\theta _3} + \theta _1^* + \theta _2^* + \theta _3^*}},\\&{g_5} = {m _{12345}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + {\theta _3} + \theta _1^* + \theta _2^*}} \\&~~~~~~~~+ {m _{12346}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + {\theta _3} + \theta _1^* + \theta _3^*}}\\&~~~~~~~~+ {m _{12356}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + {\theta _3} + \theta _2^* + \theta _3^*}},\\&{g_3} = {m _{124}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _1^*}} + {m _{125}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _2^*}}\\&~~~~~~~~ + {m _{126}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _3^*}}+ {m _{134}}{{\mathrm{e}}^{{\theta _1} + {\theta _3} + \theta _1^*}} \\&~~~~~~~~ + {m _{135}}{{\mathrm{e}}^{{\theta _1} + {\theta _3} + \theta _2^*}}+ {m _{234}}{{\mathrm{e}}^{{\theta _2} + {\theta _3} + \theta _1^*}}\\&~~~~~~~~+ {m _{235}}{{\mathrm{e}}^{{\theta _3} + {\theta _2} + \theta _2^*}} + {m _{136}}{{\mathrm{e}}^{{\theta _1} + {\theta _3} + \theta _3^*}}\\&~~~~~~~~+ {m _{236}}{{\mathrm{e}}^{{\theta _2} + {\theta _3} + \theta _3^*}},\\&{f_2} = {m _{14}}{{\mathrm{e}}^{{\theta _1} + \theta _1^*}} + {m _{25}}{{\mathrm{e}}^{{\theta _2} + \theta _2^*}} + {m _{15}}{{\mathrm{e}}^{{\theta _1} + \theta _2^*}} \\&~~~~~~~~+ {m _{24}}{{\mathrm{e}}^{{\theta _2} + \theta _1^*}}+ {m _{36}}{{\mathrm{e}}^{{\theta _3} + \theta _3^*}} + {m _{34}}{{\mathrm{e}}^{{\theta _3} + \theta _1^*}}\\&~~~~~~~~ + {m _{35}}{{\mathrm{e}}^{{\theta _3} + \theta _2^*}}+ {m _{26}}{{\mathrm{e}}^{{\theta _2} + \theta _3^*}} + {m _{16}}{{\mathrm{e}}^{{\theta _1} + \theta _3^*}},\\&{f_4} = {m _{1245}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _1^* + \theta _2^*}} + {m _{1346}}{{\mathrm{e}}^{{\theta _1} + {\theta _3} + \theta _1^* + \theta _3^*}} \\&~~~~~~~~+ {m _{2356}}{{\mathrm{e}}^{{\theta _3} + {\theta _2} + \theta _3^* + \theta _2^*}} + {m _{1246}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _1^* + \theta _3^*}}\\&~~~~~~~~ + {m _{1256}}{{\mathrm{e}}^{{\theta _1} + {\theta _2} + \theta _2^* + \theta _3^*}} + {m _{1345}}{{\mathrm{e}}^{{\theta _1} + {\theta _3} + \theta _1^* + \theta _2^*}}\\&~~~~~~~~ + {m _{1356}}{{\mathrm{e}}^{{\theta _1} + {\theta _3} + \theta _2^* + \theta _3^*}} + {m _{2345}}{{\mathrm{e}}^{{\theta _3} + {\theta _2} + \theta _1^* + \theta _2^*}} \\&~~~~~~~~+ {m _{2346}}{{\mathrm{e}}^{{\theta _3} + {\theta _2} + \theta _1^* + \theta _3^*}},\\&{m _{s_{1}, s_{2}+3}} = \frac{{i{\mathrm{}}{k_{s_{1}}}\alpha \left( t \right) + \mu \left( t \right) }}{{2\lambda \left( t \right) {{\left( {{k_{s_{1}}} + k_{s_{2}}^ * } \right) }^2}}},\\&{m _{s_{1},s_{2},s_{3}+3}} = \frac{\left[ { i{\mathrm{}}k_{s_{3}}^ * \alpha \left( t \right) + \mu \left( t \right) } \right] {{{\left( {{k_{s_{1}}} - {k_{s_{2}}}} \right) }^2}}}{{2\lambda \left( t \right) {{\left( {{k_{s_{1}}} + k_{s_{3}}^ * } \right) }^2}{{\left( {{k_{s_{2}}} + k_{s_{3}}^ * } \right) }^2}}},\\&{m _{{s_{1}}, {s_{2}},{s_{3}}+3,{s_{4}}+3}} = \frac{{{{\left( {{k_{s_{1}}} - {k_{s_{2}}}} \right) }^2}{{\left( {k_{s_{3}}^ * - k_{s_{4}}^ * } \right) }^2}}}{{4\lambda {{\left( t \right) }^2}{{\left( {{k_{s_{1}}} + k_{s_{3}}^ * } \right) }^2}{{\left( {{k_{s_{1}}} + k_{s_{3}}^ * } \right) }^2}}}\\&~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\times \frac{\prod \nolimits _{j = {s_{1}},{s_{2}}} {\left[ i{{k_j}\alpha \left( t \right) +\mu \left( t \right) } \right] }}{{{\left( {{k_{s_{2}}} + k_{s_{4}}^ * } \right) }^2}{{\left( {{k_{s_{2}}} + k_{s_{4}}^ * } \right) }^2}},\\&{m _{1,2, 3,{s_{1}}+3,{s_{2}}+3}} = \frac{{{{\left( {{k_1} - {k_2}} \right) }^2}{{\left( {{k_1} - {k_3}} \right) }^2}{{\left( {{k_3} - {k_2}} \right) }^2}}}{{4\lambda {{\left( t \right) }^2}{{{({k_j} + k_{s_{2}}^ * )}^2}{{({k_j} + k_{s_{1}}^ * )}^2}}}}\\&~~~~~~~~\times {{\left( {k_{s_{1}}^ * - k_{s_{2}}^ * } \right) }^2}\prod \limits _{j = {s_{1}},{s_{2}}} {\left[ i{{k_j}\alpha \left( t \right) +\mu \left( t \right) } \right] },\\&m_{123456} = \frac{{ {{\left| {{k_3} - {k_2}} \right| }^4}{{\left| {{k_1} - {k_2}} \right| }^4}{{\left| {{k_3} - {k_1}} \right| }^4}}}{{8\lambda {{\left( t \right) }^3}\prod \nolimits _{j = 1}^3 {{{({k_j} + k_1^ * )}^2}} {{({k_j} + k_2^ * )}^2}{{({k_j} + k_3^ * )}^2}}}\\&~~~~~~~~~~~~~~~~~\times \prod \limits _{j = 1}^3 {\left[ i{{k_j}\alpha \left( t \right) + \mu \left( t \right) } \right] }, \end{aligned}$$

where ${\theta _j} = {k_j}x + ik^{2}_{j}\int \lambda (t)\hbox {d}t + \delta _{j}$, $\delta _{j}$’s $(j=1,2,3)$ are the real constants, $s_1<s_2$, $s_3<s_4$ and $s_1,s_2,s_3,s_4 = 1,2,3$.

4.4 N-soliton solutions for Eq. (1)

Substituting Eqs. (17) into (14), we solve Eq. (14). The analytic N-soliton solutions for Eq. (1),

$$\begin{aligned} u_{N} = \frac{g}{f}, \end{aligned}$$

(21)

are obtained under Constraint (12), while g and f in Eq. (21) are transformed to

$$\begin{aligned} {g}&= \sum \limits _{n = 1}^N {{\varepsilon ^{2n - 1}}\left[ {\sum \limits _{{N_\rho },{N_\eta ^{\prime }-N}}^g {{\varGamma _{N_{1},\ldots ,N_{n},N^{\prime }_{1},\ldots ,N^{\prime }_{n-1}}}}}\right. }\nonumber \\&\quad \times {\left. {{{\exp {\left( {\sum \limits _{\rho = 1}^n {{\theta _{{N_\rho }}}} + \sum \limits _{\eta = 1}^{n - 1} {\theta _{{N^{\prime }_\eta }-N}^ * } } \right) }}} } \right] }, \end{aligned}$$

(22a)

$$\begin{aligned} {f}&= 1 + \sum \limits _{n = 1}^N {{\varepsilon ^{2n}}\left[ {\sum \limits _{{N_\rho },{N_\eta ^{\prime }-N}}^f {{\varGamma _{N_{1},\ldots ,N_{n},N^{\prime }_{1},\ldots ,N^{\prime }_{n}}}}}\right. }\nonumber \\&\quad \times {\left. {{{\exp {\left( {\sum \limits _{\rho = 1}^n {{\theta _{{N_\rho }}}} + \sum \limits _{j = 1}^n {\theta _{{N^{\prime }_\eta }-N}^ * } } \right) }}} } \right] }, \end{aligned}$$

(22b)

with

$$\begin{aligned}&\theta _{n}=k_{n}x + \omega _{n}(t)+\delta _n ,~~~\omega _{n}(t)=ik^{2}_{n}\int \lambda (t)\hbox {d}t,\\&\varGamma _{N_{1},\ldots ,N_{n},N^{\prime }_{1},\ldots ,N^{\prime }_{n-1}} = \frac{\prod \nolimits _{\rho =1}^{n-1}{\left[ i k^{*}_{N^{\prime }_{\rho ^{\prime }}}\alpha (t)+\mu (t)\right] }}{\left[ 2\lambda (t)\right] ^{n-1} \prod \nolimits _{\begin{array}{c} 1\le \rho \le n,\\ 1\le \rho ^{\prime } \le n-1 \end{array}} {\left( k_{N_\rho }+k^{*}_{N^{\prime }_{\rho ^{\prime }}}\right) ^2}}\\&\quad \times \prod _{\begin{array}{c} 1 \le \rho ,\eta \le n,\\ 1\le \rho ^{\prime },\eta ^{\prime }\le n-1 \end{array}}^{\rho \ne \eta ,\rho ^{\prime }\ne \eta ^{\prime }} {\left[ \left( k_{N_\rho }-k_{N_\eta }\right) ^2 \left( k^{*}_{N^{\prime }_{\rho ^{\prime }}-N}-k^{*}_{N^{\prime }_{\eta ^{\prime }}-N}\right) ^2\right] },\\&\varGamma _{N_{1},\ldots ,N_{n},N^{\prime }_{1},\ldots ,N^{\prime }_{n}}~~ = \frac{\prod \nolimits _{\rho =1}^{n}{\left[ i k_{N_\rho }\alpha (t)+\mu (t)\right] }}{\left[ 2\lambda (t)\right] ^{n} \prod \nolimits _{1\le \rho ,\rho ^{\prime } \le n} {\left( k_{N_\rho }+k^{*}_{N^{\prime }_{\rho ^{\prime }}}\right) ^2}}\\&\quad \times \prod _{1 \le \rho ,\eta ,\rho ^{\prime },\eta ^{\prime } \le n}^{\rho \ne \eta , \rho ^{\prime }\ne \eta ^{\prime }} {\left[ \left( k_{N_\rho }-k_{N_\eta }\right) ^2 \left( k^{*}_{N^{\prime }_{\rho ^{\prime }}-N}-k^{*}_{N^{\prime }_{\eta ^{\prime }}-N}\right) ^2\right] }, \end{aligned}$$

where $\theta _{{N_\rho }}, \theta _{{N^{\prime }_\eta }-N} \in \left\{ {{\theta _n}} \right\} _{n = 1}^N$, ${N_1}< {N_n}< {N^{\prime }_1}< {N^{\prime }_n}$, $\varGamma _{N_{1}}=1$, ${k_n}$’s are the complex constants, ${\theta _{{N_\rho }}}$’s (${\theta _{{N_\eta }}}$’s) are different with each other and $\sum \nolimits _{{N_\rho },{{N^{\prime }_\eta }-N}}^g$ ($\sum \nolimits _{{N_\rho },{{N^{\prime }_\eta }-N}}^f$) indicates the sum of all the possibilities of ${\sum \nolimits _{\rho = 1}^n {{\theta _{{N_\rho }}}}}$$+$${\sum \nolimits _{\eta = 1}^{n - 1} {\theta _{{N^{\prime }_\eta }-N}^ * } }$ ($\sum \nolimits _{\rho = 1}^n {{\theta _{{N_\rho }}}} \,{+}\, \sum \nolimits _{\eta = 1}^{n} {\theta _{{N^{\prime }_\eta }-N}^ * }$) for n. g and f contain $\sum \nolimits _{n = 1}^N {C_N^nC_N^{n - 1}}$ and $\sum \nolimits _{n = 1}^N {C_N^nC_N^{n}} + 1$ terms, respectively. When $\varepsilon =1$, we can obtain the N-soliton solutions for Eq. (1) via Eq. (22).

5 Asymptotic analysis

Without loss of generality, we conduct the asymptotic analysis on the two-soliton solutions and three-soliton solutions, i.e., $u_{2}$ and $u_{3}$, for illustrating the solitonic interactions.

Table 1 Properties of the solitonic interaction for the two-soliton solutions

For the two-soliton solutions, when $m_{1234}\ne 0$, we have the following:

(1) Before the interaction ($t\rightarrow -\infty$):

$$\begin{aligned} S_{21}^ -&= \frac{{{m_1}}}{{2\sqrt{{m_{13}}} }}\text {sec}h\left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _1}) + \ln \sqrt{{m_{13}}} } \right] {{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _1})}},\nonumber \\&\quad \left[ {{\mathop {\mathrm{Re}}\nolimits } ({\theta _2}) \rightarrow - \infty ,~{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _1}) \rightarrow 0 } \right] , \end{aligned}$$

(23a)

$$\begin{aligned} S_{22}^ -&= \frac{{{m_2}}}{{2\sqrt{{m_{24}}} }}\text {sec}h\left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _2}) + \ln \sqrt{{m_{24}}} } \right] {{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _2})}},\nonumber \\&\quad \left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _1}) \rightarrow - \infty ,~{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _2}) \rightarrow 0 } \right] , \end{aligned}$$

(23b)

(2) After the interaction ($t\rightarrow +\infty$):

$$\begin{aligned} S_{21}^ +&= \frac{{{m_{124}}}}{{2\sqrt{{m_{24}}}\sqrt{{m_{1234}}} }}\text {sec}h\left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _1}) + \ln {\frac{\sqrt{m_{1234}}}{\sqrt{m_{24}}}} } \right] {{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _1})}},\nonumber \\&\quad \left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _2}) \rightarrow + \infty ,~{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _1}) \rightarrow 0 } \right] , \end{aligned}$$

(24a)

$$\begin{aligned} S_{22}^ +&= \frac{{{m_{123}}}}{{2\sqrt{{m_{13}}}\sqrt{{m_{1234}}} }}\text {sec}h\left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _2}) + \ln {\frac{\sqrt{{m_{1234}}}}{\sqrt{{m_{13}}}}} } \right] {{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _2})}},\nonumber \\&\quad \left[ {{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _1}) \rightarrow + \infty ,~{\mathop {{\mathrm{Re}}}\nolimits } ({\theta _2}) \rightarrow 0 } \right] , \end{aligned}$$

(24b)

where ${S_{2,{\zeta }}^ -}$’s (or ${S_{2,{\zeta }}^ +}$’s) denote the asymptotic expressions for the two solitons $S_{2,{\zeta }}$’s $({\zeta }=1,2)$ before (or after) the interaction for $u_2$, respectively. Based on Eqs. (23) and (24), the relevant properties of each soliton during the interaction for $u_{2}$, including the widths $W_{2,{\zeta }}$, amplitudes $A_{2,{\zeta }}$, velocities $V_{2,{\zeta }}$, initial phases $P^{\mp }_{2,{\zeta }}$, phase shifts $\varDelta _{2,{\zeta }}$ and propagation paths $\varPhi ^{\mp }_{2,{\zeta }}$, are listed in Table 1, where the first subscript corresponds to the two-soliton solutions, while the second subscript corresponds to the ${\zeta }$th soliton within the two-soliton solutions.

For the three-soliton solutions, when $m_{123456}\ne 0$, we have the following:

(3) Before the interaction ($t\rightarrow -\infty$):

$$\begin{aligned} S_{31}^ -&= \frac{1}{{2\sqrt{{m_{14}}} }} \text {sec}h\left[ {{\mathop {\text {Re}}\nolimits } ({\theta _1}) + \ln \sqrt{{m_{14}}} } \right] {{\mathrm{e}}^{i{\mathop {\text {Im}}\nolimits } ({\theta _1})}},\nonumber \\&\quad \left[ {{\mathop {\text {Re}}\nolimits } ({\theta _3}),{\mathop {\text {Re}}\nolimits } ({\theta _2}) \rightarrow - \infty ,{\mathop {\text {Re}}\nolimits } ({\theta _1}) \sim 0 } \right] , \end{aligned}$$

(25a)

$$\begin{aligned} S_{32}^ -&= \frac{1}{{2\sqrt{{m_{25}}} }}\text {sec}h\left[ {{\mathop {\text {Re}}\nolimits } ({\theta _2}) + \ln \sqrt{{m_{25}}} } \right] {{\mathrm{e}}^{i{\mathop {\text {Im}}\nolimits } ({\theta _2})}},\nonumber \\&\quad \left[ {{\mathop {\text {Re}}\nolimits } ({\theta _3}),{\mathop {\text {Re}}\nolimits } ({\theta _1}) \rightarrow - \infty ,{\mathop {\text {Re}}\nolimits } ({\theta _2}) \sim 0 } \right] , \end{aligned}$$

(25b)

$$\begin{aligned} S_{33}^ -&= \frac{1}{{2\sqrt{{m_{36}}} }}\text {sec}h\left[ {{\mathop {\mathrm{Re}}\nolimits } ({\theta _3}) + \ln \sqrt{{m_{36}}} } \right] {{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _3})}},\nonumber \\&\quad \left[ {{\mathop {\mathrm{Re}}\nolimits } ({\theta _2}),{\mathop {\mathrm{Re}}\nolimits } ({\theta _1}) \rightarrow - \infty ,{\mathop {\mathrm{Re}}\nolimits } ({\theta _3}) \sim 0 } \right] , \end{aligned}$$

(25c)

Table 2 Properties of the solitonic interaction for the three-soliton solutions

(4) After the interaction ($t\rightarrow +\infty$):

$$\begin{aligned} S_{31}^ +&= \frac{{{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _1})}}m_{12356}}{{2\sqrt{{m_{2356}}}\sqrt{{m_{123456}}} }} \nonumber \\&\quad \times \text {sec}h\left[ {\mathop {\mathrm{Re}}\nolimits } ({\theta _1}) + \ln {\frac{\sqrt{m_{123456}}}{\sqrt{m_{2356}}}}\right] ,\nonumber \\&\quad \left[ {{\mathop {\mathrm{Re}}\nolimits } ({\theta _3}),{\mathop {\mathrm{Re}}\nolimits } ({\theta _2}) \rightarrow + \infty ,{\mathop {\mathrm{Re}}\nolimits } ({\theta _1}) \sim 0 }\right] ,\end{aligned}$$

(26a)

$$\begin{aligned} S_{32}^ +&= \frac{{{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _2})}}{{m_{12346}}}}{{2\sqrt{{m_{1346}}}\sqrt{{m_{123456}}} }}\nonumber \\&\quad \times \text {sec}h\left[ {\mathop {\mathrm{Re}}\nolimits } ({\theta _2}) + \ln {\frac{\sqrt{m_{123456}}}{\sqrt{m_{1346}}} }\right] ,\nonumber \\&\quad \left[ {{\mathop {\mathrm{Re}}\nolimits } ({\theta _3}),{\mathop {\mathrm{Re}}\nolimits } ({\theta _1}) \rightarrow + \infty ,{\mathop {\mathrm{Re}}\nolimits } ({\theta _2}) \sim 0 } \right] ,\end{aligned}$$

(26b)

$$\begin{aligned} S_{33}^ +&= \frac{{{\mathrm{e}}^{i{\mathop {\mathrm{Im}}\nolimits } ({\theta _3})}}{{m_{12345}}}}{{2\sqrt{m_{1245}}\sqrt{m_{123456}} }}\nonumber \\&\quad \times \text {sec}h\left[ {\mathop {\mathrm{Re}}\nolimits } ({\theta _3}) + \ln {\frac{\sqrt{m_{123456}}}{\sqrt{m_{1245}}} }\right] ,\nonumber \\&\quad \left[ {{\mathop {\mathrm{Re}}\nolimits } ({\theta _2}),{\mathop {\mathrm{Re}}\nolimits } ({\theta _1}) \rightarrow + \infty ,{\mathop {\mathrm{Re}}\nolimits } ({\theta _3}) \sim 0 } \right] , \end{aligned}$$

(26c)

where ${S_{3,{\xi }}^ -}$’s (or ${S_{3,{\xi }}^ +}$’s) denote the asymptotic expressions for the three solitons $S_{3,{\xi }}$’s (${\xi }=1,2,3$) before (or after) the interaction for $u_3$, respectively. Based on Eqs. (25) and (26), the relevant properties for each soliton during the interaction for $u_{3}$, including the widths $W_{3,{\xi }}$, amplitudes $A_{3,{\xi }}$, velocities $V_{3,{\xi }}$, initial phases $P^{\mp }_{3,{\xi }}$, phase shifts $\varDelta _{3,{\xi }}$ and propagation paths $\varPhi ^{\mp }_{3,{\xi }}$ are listed in Table 2, where the first subscript corresponds to the three-soliton solutions, while the second subscript corresponds to the ${\xi }$th soliton within the three-soliton solutions.

Based on Tables 1 and 2, the widths $W_{N,\varrho }$, amplitudes $A_{N,\varrho }$ and velocities $V_{N,\varrho }$ ($N=2,3$ and ${\varrho }={\zeta },{\xi }$) keep unchanged after the interaction, and then the interaction between (or among) the two (or three) solitons may be elastic or inelastic with the phase shifts $\varDelta _{N,\varrho }$’s when $m_{1234}\ne 0$ (or $m_{123456}\ne 0$). $A_{N,\varrho }$, $P^{\mp }_{N,\varrho }$ and $V_{N,\varrho }$ are related to $\alpha (t)$, $\lambda (t)$ and $\mu (t)$ while the $W_{N,\varrho }$ and $\varDelta _{N,\varrho }$ are related to the wave numbers $k_\varrho$’s but not $\alpha (t)$, $\lambda (t)$ and $\mu (t)$.

When $\varLambda _{\varrho }=0$, $\alpha (t)=\rho _1 \lambda (t)=\rho _2 \mu (t)$, where $\rho _1$ and $\rho _2$ are the constants. The $V_{N,\varrho }$ and $\varPhi ^{\mp }_{N,\varrho }$ ($N=2,3$ and ${\varrho }={\zeta },{\xi }$) for each soliton can be reduced as

$$\begin{aligned}&V_{N,\varrho }=2\lambda (t)\,\text {Im}(k_\varrho ), \end{aligned}$$

(27)

and

$$\begin{aligned}&\varPhi ^{-}_{N,\varrho }:\text {Re}(k_\varrho )\left[ x-2\text {Im}(k_\varrho ) \int \lambda (t)\hbox {d}t\right] \nonumber \\&\quad +\frac{1}{2}\ln \frac{(1+ik_\varrho \rho _2)\rho _1}{8{\text {Re}(k_\varrho )}^2\rho _2}=\text {const.}, \end{aligned}$$

(28a)

$$\begin{aligned}&\varPhi ^{+}_{N,\varrho }:\text {Re}(k_\varrho )\left[ x-2\text {Im}(k_\varrho ) \int \lambda (t)\hbox {d}t\right] \nonumber \\&\quad +\frac{1}{2}\ln \frac{(1+ik_\varrho \rho _2)\rho _1}{8{\text {Re}(k_\varrho )}^2\rho _2} \nonumber \\&\quad +2\ln \prod \limits _{N^{\prime }}^{1,\cdot , \widehat{\varrho },\cdot ,N}{\frac{|k_\varrho -k_{N^{\prime }}|}{|k_\varrho +k^{*}_{N^{\prime }}|}} =\text {const.}, \end{aligned}$$

(28b)

where $\widehat{\varrho }$ indicates the $\varrho$ is omitted. Particularly, the $A_{N,\varrho }$ and $P^{\mp }_{N,\varrho }$ ($N=2,3$) are only related to $k_\varrho$’s, while the velocities are related to $\lambda (t)$ and $k_\varrho$’s under $\varLambda _\varrho =0$. According to Eq. (28), $\varPhi ^{\mp }_{N,\varrho }$ for each soliton are related to $\lambda (t)$ and $k_\varrho$’s.

Table 3 Properties of the one-soliton solutions

Fig. 1

Interactions between the two solitons for (19) under Constraint (12); a$k_{1} = 1-i$, $k_{2} = 1-2i$ and $\alpha (t) = \lambda (t) = \mu (t) = t$; b the same as a except that $k_{2} = 1$; c the same as a except that $\alpha (t) = \lambda (t) = \mu (t) =-t$; d the same as a except that $\alpha (t) = \lambda (t) = \mu (t) = t^2$

Fig. 2

Interactions among the three solitons for (20) under Constraint (12) with $k_{3} = 1 - 2i$, $\alpha (t) = \lambda (t) = \mu (t) = t^2$; a$k_{1} = 1-3i$ and $k_{2} = 1-i$; b$k_{1} = 1$ and $k_{2} = 1-i$; c$k_{1} = 1$ and $k_{2} = 1.3$; d the same as a except that $\alpha (t) = \lambda (t) = \mu (t) = t$

When $\varLambda _{\varrho }\ne 0$, the relations among $\alpha (t)$, $\lambda (t)$ and $\mu (t)$ are nonlinear. The $V_{N,\varrho }$ and $\varPhi ^{\mp }_{N,\varrho }$ ($N=2,3$ and ${\varrho }={\zeta },{\xi }$) for each soliton are related to $\alpha (t)$, $\beta (t)$, $\mu (t)$ and $k_\varrho$ and derived from Tables 1 and 2, as

$$\begin{aligned} \begin{aligned} V_{N,\varrho }&=2\lambda (t)\,\text {Im}(k_\varrho )-\frac{k_\varrho \left[ \lambda (t)\alpha (t)^{\prime }-\alpha (t)\lambda (t)^{\prime }\right] }{2\lambda (t)\text {Re}(k_\varrho )\left[ k_\varrho \alpha (t)-i\mu (t)\right] }\\&\quad +\frac{i\left[ \lambda (t)\mu (t)^{\prime }-\mu (t)\lambda (t)^{\prime } \right] }{2\lambda (t)\text {Re}(k_\varrho )\left[ k_\varrho \alpha (t)-i\mu (t)\right] } \end{aligned} \end{aligned}$$

(29)

and

$$\begin{aligned}&\varPhi ^{-}_{N,\varrho }:\text {Re}(k_\varrho )\left[ x-2\text {Im}(k_\varrho ) \int \lambda (t)\hbox {d}t\right] \nonumber \\&\quad +\frac{1}{2}\ln \frac{[\mu (t)+ik_\varrho \alpha (t)]}{8{\text {Re}(k_\varrho )}^2\lambda (t)}=\text {const.}, \end{aligned}$$

(30a)

$$\begin{aligned}&\varPhi ^{+}_{N,\varrho }:\text {Re}(k_\varrho )\left[ x-2\text {Im}(k_\varrho ) \int \lambda (t)\hbox {d}t\right] \nonumber \\&\quad +\frac{1}{2}\ln \frac{[\mu (t)+ik_\varrho \alpha (t)]}{8{\text {Re}(k_\varrho )}^2\lambda (t)} \nonumber \\&\quad +2\ln \prod \limits _{N^{\prime }}^{1,\cdot , \widehat{\varrho },\cdot ,N}{\frac{|k_\varrho -k_{N^{\prime }}|}{|k_\varrho +k^{*}_{N^{\prime }}|}}=\text {const.}, \end{aligned}$$

(30b)

which are more complex than those in Eqs. (27) and (28) under $\varLambda _{\varrho }=0$, i.e., $\alpha (t)=\rho _1 \lambda (t)=\rho _2 \mu (t)$.

6 Discussion

Due to Constraint (12), $\alpha (t)\lambda (t)\nu (t)\ne 0$. According to Solutions (18), we can obtain the width, amplitude, initial phase, velocity and propagation path for the one-soliton solutions, listed in Table 3.

Because the formation of the interaction requires two or more solitons, we will analyze the interactions via Solutions (19) and (20) for Eq. (1). For simplicity, if $\delta _j$’s $(j=1,2,3)$ are not mentioned in the figure captions for Solutions (19) and (20), then $\delta _j=0$. We can see the solitons in Figs. 1–7. We will analyze the interactions under the conditions $\varLambda _\varrho =0$ and $\varLambda _\varrho \ne 0$, respectively. When $\varLambda _\varrho =0$, i.e., $\alpha (t)=\rho _1 \lambda (t)=\rho _2 \mu (t)$, the solitonic interactions are shown in Figs. 1–4.

When $\rho _1=\rho _2=1$ and $\text {Im}(k_{\zeta })$’s are fixed, according to Eq. (27), parabolic solitons change the propagation directions as $\lambda (t)$ changes from t to $-t$ (or $t^2$ to $-t^2$), as shown in Fig. 1a, c. According to Eq. (28), the function types of $\lambda (t)$ and the values of $\text {Im}(k_2)$ can both affect the propagation paths, e.g., $\lambda (t) = t$ in Fig. 1a versus $\lambda (t) = t^2$ in Fig. 1d, while $\text {Im}(k_2)=-2$ in Fig. 1a versus $\text {Im}(k_2)=0$ in Fig. 1b. Particularly, $V_{2,{\zeta }}$ indicates that the corresponding soliton propagates along the t direction, as shown in Fig. 1b.

When $\rho _1=\rho _2=1$, the interactions between/among the bright and parabolic (or hyperbolic) solitons for the two (or three)-soliton solutions are displayed in Fig. 1 (or 2). Figure 2 shows the similar propagation phenomena to Fig. 1, except that when two of $V_{3,{\xi }}$’s $({\xi }=1,2,3)$ are equal to zero, the corresponding two bright solitons interact with the hyperbolic soliton, as shown in Fig. 2c, and then the two bright solitons interact with each other and result in the bound solitons.

Fig. 3

Interactions between the bright soliton and breathers for (19) under Constraint (12) with $k_{2} = -2$; a$k_{1} = -1$ and $\alpha (t) = \lambda (t) = \mu (t) = 1$ (elastic); b$k_{1} = 1$ and $\alpha (t) = \lambda (t) = \mu (t) = 1$ (elastic); c$k_{1} = 1$ and $\alpha (t) = \lambda (t) = \mu (t) = t$ (inelastic)

Fig. 4

Interactions among the bright soliton and Kuznetsov–Ma breathers for (20) under Constraint (12) with $k_{1} = 1$, $k_{2} = 0.3$, $k_{3} = 0.4$, $\delta _{1} = 5$, $\delta _{2} = 1.6$ and $\delta _{3} = -3$; a$\alpha (t)=\lambda (t) = \mu (t) = 1$; b$\alpha (t)=\mu (t) = 1$ and $\lambda (t) =\frac{2}{3}$; c$\alpha (t)= 1$, $\lambda (t) = \frac{2}{3}$ and $\mu (t) =\frac{1}{20}$; d$\alpha (t)= 4$, $\lambda (t) = \frac{2}{3}$ and $\mu (t) =\frac{1}{20}$

Fig. 5

Inelastic interactions between the solitons for (19) under Constraint (12) with $\alpha (t) = \mu (t) = t$ and $\lambda (t)=t^3$; a$k_{1} = 1-i$ and $k_{2} = 2-i$; b$k_{1} = 1$ and $k_{2}=2-i$; c$k_{1}=1$ and $k_{2}=2$

Fig. 6

Inelastic interactions among the solitons for (20) under Constraint (12) with $\alpha (t) = \mu (t) = t$ and $\lambda (t)=t^3$; a$k_{1} = 1-i$, $k_{2} = 2-i$ and $k_{3} = 3-i$; b$k_{1} = 1$, $k_{2} = 2-i$ and $k_{3} = 3-i$; c$k_{1} = 1$, $k_{2} = 2$ and $k_{3} = 3$

Fig. 7

Inelastic interactions for (19) under Constraint (12) with $\alpha (t)=\mu (t)=\text {sin}(t)$, $k_{1} = 1+i$ and $k_{2} = 2-i$; a$\lambda (t)=\text {sin}(t)$; b$\lambda (t)=\text {sin}(2t)$; c$\lambda (t)=\frac{1}{2}\text {sin}(2t)$

For the two-soliton solutions, with $\rho _1=\rho _2=1$, $\lambda (t)=1$ and $k_{2} = -2$, the propagation varies from the interaction between the bright and Kuznetsov–Ma breathers to the bound solitons, which corresponds to the change of $k_{1}$ from $-1$ to 1, i.e., Fig. 3a to 3b. Compared with the two solitons in Fig. 3b, when $\lambda (t)=t$, the two solitons interact with each other and result in the bound solitons, as displayed in Fig. 3c.

For the three-soliton solutions, Fig. 4 shows that the interaction between the bright and Kuznetsov–Ma breathers evolves to the interaction between the bright and bound solitons. When $\rho _1=\rho _2=1$, $\delta _{1} = 5$, $\delta _{2} = 1.6$, $\delta _{3} = -3$, $k_{1}=1$, $k_{2}=0.3$ and $k_{3}=0.4$, the bright soliton propagates with the Kuznetsov–Ma breathers in parallel with $\alpha (t)=\lambda (t)=\mu (t)=1$, as shown in Fig. 4a. Compared with Fig. 4a, Fig. 4b displays that the propagation period changes longer with $\lambda (t)=\frac{2}{3}$. Compared with Fig. 4b, Fig. 4c reveals that the Kuznetsov–Ma breathers evolve to the bound solitons with $\mu (t)=\frac{1}{20}$. Compared with Fig. 4c, Fig. 4d indicates that the amplitudes of the three solitons become lower with $\alpha (t)=4$.

When $\varLambda _\varrho \ne 0$, i.e., the relations among $\alpha (t)$, $\lambda (t)$ and $\mu (t)$ are nonlinear; the solitonic interactions are shown in Figs. 5–7.

With $\alpha (t) = \mu (t) = t$ and $\lambda (t)=t^3$, when $\text {Im}(k_\varrho )=0$, $V_{N,\varrho }=-\frac{1}{\text {Re}(k_\varrho )}\frac{1}{t}$ ($N=2,3$ and ${\varrho }={\zeta },{\xi }$) and then the corresponding soliton propagation is not parallel to the t coordinate, as shown in Fig. 5b and 6b (or Figs. 5c, 6c), while $A_{N,\varrho }=\frac{\text {Re}(k_\varrho )}{[1+{\text {Re}(k_\varrho )}^2] ^{\frac{1}{4}}}|t|$ and then the amplitude is proportional to |t|, as shown in Figs. 5 and 6. Amplitudes and velocities of the three solitons change with t during the interaction, implying that the interactions are inelastic.

With the other parameters fixed, $\varPhi _{2,{\zeta }}$’s (${\zeta }=1,2$) are affected by the integral of $\lambda (t)$ with respect to t and the propagation directions for the two solitons are mutually opposite, as shown in Fig. 7. Propagation path of the two-soliton solutions in Fig. 7c is similar to that in Fig. 7a except that it is compressed by 50% in the x and t directions, respectively, which is caused by $\lambda (t)=\frac{1}{2}\text {sin}(2t)$.

7 Conclusions

In this paper, attention has been focused on a quintic time-dependent coefficient DNLS equation, i.e., Eq. (1), for certain hydrodynamic wave packets or a medium with the negative refractive index. We have found Gauge transformation (2) to obtain the equivalent form of Eq (1), i.e., Eq. (3). With respect to u, the wave envelope for the free water surface displacement or envelope of the electric field, we have obtained variable-coefficient constraint (12), different from that in Ref. [48], via the Painlevé analysis, derived N-soliton solutions (21) via bilinear forms (14) and analyzed the solitonic interactions for two-soliton solutions (19) and three-soliton solutions (20) via the asymptotic analysis. Properties for the one-, two- and three-soliton solutions are listed in Tables 1, 2 and 3, respectively.

Based on the asymptotic analysis, classifying the interactions under different conditions, we have revealed two cases of the interactions between (or among) the two (or three) solitons:

• Case 1: Relations among the self-steepening coefficient $\alpha (t)$, dispersion coefficient $\lambda (t)$ and cubic nonlinearity $\mu (t)$ are linear. According to Eqs. (27) and (28), velocities $V_{N,\varrho }$’s ($N=2,3$, $\varrho =1,2,3$) and propagation paths $\varPhi ^{\mp }_{N,\varrho }$’s of the solitons have been both demonstrated to be correlated with $\lambda (t)$. When the propagation paths are $x=\text {const.}$, the corresponding solitons have been observed to propagate along the t direction, as shown in Figs. 1 and 2. With the phase shifts $\varDelta _{N,\varrho }$’s changing, we have found that the two solitons in parallel change to the bound solitons, as shown in Fig. 3. It has been observed that the Kuznetsov–Ma breathers change to the bound solitons with $\mu (t)=\frac{1}{20}$ while the propagation period increases as $\lambda (t)$ decreases, as shown in Fig. 4. Interactions are elastic when $\alpha (t)$, $\lambda (t)$ and $\mu (t)$ are constants, as shown in Fig. 4.

• Case 2: Relations among $\alpha (t)$, $\lambda (t)$ and $\mu (t)$ are nonlinear. According to Eqs. (29) and (30), amplitude $A_{N,\varrho }$, velocity $V_{N,\varrho }$ and propagation path $\varPhi ^{\mp }_{N,\varrho }$ of the soliton have been demonstrated to be correlated with $\alpha (t)$, $\lambda (t)$ and $\mu (t)$. Amplitudes $A_{N,\varrho }$’s, velocities $V_{N,\varrho }$’s and propagation paths $\varPhi ^{\mp }_{N,\varrho }$’s of the two or three solitons change with t during the interactions, implying that the interactions are inelastic, as shown in Figs. 5 and 6. We have found that there is a compression effect on the propagation paths of the two solitons, which is caused by $\lambda (t)$, as shown in Fig. 7.