Abstract
The finite-time Mittag-Leffler stability for fractional-order quaternion-valued memristive neural networks (FQMNNs) with impulsive effect is studied here. A new mathematical expression of the quaternion-value memductance (memristance) is proposed according to the feature of the quaternion-valued memristive and a new class of FQMNNs is designed. In quaternion field, by using the framework of Filippov solutions as well as differential inclusion theoretical analysis, suitable Lyapunov-functional and some fractional inequality techniques, the existence of unique equilibrium point and Mittag-Leffler stability in finite time analysis for considered impulsive FQMNNs have been established with the order \(0<\beta <1\). Then, for the fractional order \(\beta \) satisfying \(1<\beta <2\) and by ignoring the impulsive effects, a new sufficient criterion are given to ensure the finite time stability of considered new FQMNNs system by the employment of Laplace transform, Mittag-Leffler function and generalized Gronwall inequality. Furthermore, the asymptotic stability of such system with order \(1<\beta <2\) have been investigated. Ultimately, the accuracy and validity of obtained finite time stability criteria are supported by two numerical examples.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.Avoid common mistakes on your manuscript.
1 Introduction
In 1695, the foundation of non-integer order calculus, which is a generalization integer order differential and integrals was first of all discussed through Guillaume de Leibnitz and Gottfried Wilhelm Leibnitz, and its development were inch by inch for long period. Until recently, it has been a great research topic due to the fact many fractional order models play a crucial role in many real world objects. Comparing to an integer order dynamical model, fractional order dynamical model is more accuracy, non-local and has weakly singular kernels but integer order dynamical behavior fails in this aspect. From the application perspective, an electronic implementation of an artificial neural network model, many researchers have combined the fractional order calculus into neural networks to look at the fractional order neural network model (FONNs). Currently, fractional order calculus has been very promising areas of research and thus successfully applied in both theoretical and applicable manners [1,2,3,4]. It is well known that stability is the primary condition of the several systems [5,6,7,8]. At present, the stability analysis of neural networks and differential equations models become a hot topic and some excellent has been reported, see [9,10,11,12,13,14,15,16]. In [14], the authors investigated the stability criteria of Riemann–Liouville sense fractional order impulsive fuzzy neural networks with delay, and proposed the global asymptotic stability analysis by using fractional Barbalat’s lemma and Lyapunov stability theory. In [17], the author researched stability analysis of fractional order delayed neural networks and several conditions to ensure the existence, uniqueness and finite time stability were established based Gronwall’s inequality, method of iteration and contraction mapping principle. In [18], the authors demonstrated the finite time stability analysis of fractional order neural networks by means of estimates of Mittag-Leffler functions, generalized Gronwall’s inequality and Laplace transform.
The idea of memristor has been analyzed thinking about that 1971, at the same time as Leon Chua has proposed for the first time in a properly-organized and mathematically described manner [19]. Despite the fact that, the concept of memristor-like gadgets has been counseled in advance in 1960 by way of Bernard [20], Leon Chua changed into the primary one not simplest to offer a possible foundation for memristor existence, however also to estimate and mathematically describe its meant conduct and residences. Almost after 40 years for memristor to change from a definitely theoretic idea into workable usage. In 2008 a gathering of researchers from Hewlett-Packard Labs lead through Stan Williams has finally formulated by means of practically working memristor [21]. In [22], Kim et al., was successfully initiated by Memristor bridge synapse architecture and resolve the difficulty of the problem of nonvolatile synaptic weight garage and put in force a recently proposed hardware learning techniques. After this memristor has found various applications in numerous interdisciplinary field [23,24,25]. In general, fractional order memristor based neural networks model (FMBNNs) is an improved fractional order neural networks model by traditional resistor replaced by memristors. Many authors have investigated the several dynamical behaviors of fractional-order memristor based neural networks. In [26], the authors applied a Holder inequality to analyze the finite stability of fractional order delayed memristive complex-valued neural networks with order, both \(0<\beta <0.5\) and \(0.5\le \beta <1\), respectively. In [27], Rakkiyappan et al. has deliberated the finite time stability of fractional order complex valued neural networks with fractional order \(1<\beta <2\) by using generalized Gronwall inequality and Holder inequality.
Quaternion algebra is a standout amongst the most renowned type and its universal extension case of real-valued and complex-valued numbers, which became first of all originated by means of Hamilton in 1843 [28]. Recently, Quaternion-valued neural networks (QVNNs) has attracted considerable attention owing to its widespread applications in various disciplines in attitude control, satellite tracking, image processing, computer graphics, three dimensional modelling, four dimensional modelling and extensively investigated by many researchers, see [29,30,31,32,33,34,35] for instance. Comparing to real-valued MNNs and complex-valued MNNs, Quaternion-valued memristive neural networks (QVMNNs) is more storage capacity and complicated properties and it consists quaternion memristive connection weights, system state, and neuron activation functions. In color image compression, real-valued MNNs and complex-valued MNNs may be likewise used to transmit the colour signals yet with moderately poor impact. Truth be told, by means of three primary colours red, green and blue can be changed over into three signals about the three essential colors with certain proportion which may be transmitted by means of three channels i, j and k of the QVMNNs, and afterward changed over into the colour images too. However, real-valued MNNs and complex-valued MNNs can’t understand this ideal impact. As a result of the non-commutativity, conventional techniques used to investigate the stability of real-valued MNNs and complex-valued MNNs cannot be directly applied to the similar problems of QVMNNs. In this manner, the investigation on the fractional order QVMNNs dynamical behaviors in both theory and applications has turned out to be urgent and mandatory. Consequently, the dynamics of integer order QVNNs have been taken into consideration by means of many research scholars and a large number of great outcomes has been gained in the existing literature [36,37,38,39]. But there is little attention about the dynamics of FQNNs have been found in the existing literatures. For example, the authors in [40] presented the global Mittag-Leffler stability and global Mittag-Leffer synchronization analysis of FQNNs with linear threshold neurons by using matrix eigenvalue, M-matrix theory and Lyapunov method. In [41], the robust asymptotical stability and robust asymptotical synchronization of memristor based fractional order QVMNNs with time delays and parameter uncertainties by using nonsmooth analysis, fractional order comparison principle and Lyapunov direct method.
On the other hand, many physical processes are distinguished by abrupt changes at certain moments of time in the real-world problems. These abrupt changes were mentioned as impulsive phenomena. These impulses can influence the dynamical performance of the system trajectory from original direction in a moment [42,43,44]. Hence, the dynamical behaviours of FONNs might be described more accurately by considering the impulse. Moreover, the impulsive fractional-order neural networks showed more advanced in describing the hereditary and memory properties for various materials and processes comparing to the impulsive integer order neural networks. Along these lines, the investigation on the dynamic behavior of FONNs with impulsive effects becomes more essential ones and some excellent results have been devoted in more as of late [45,46,47]. For example in [45], the authors gave some existence, uniqueness and Mittag-Leffler stability criteria for impulsive FONNs in terms of linear matrix inequality based on topological degree properties and positive definite quadratic Lyapunov function. In [46], by using contraction mapping principle, the linear growth condition of activation function and positive definite quadratic Lyapunov function, the author have investigated about the global Mittag-Leffler stability of FONNs with one side Lipschitz condition and impulsive effects. In [47], by means of contraction mapping principle, fractional order comparison principle and fractional order absolute valued Lyapunov functional with one norm, the global asymptotical stability of a class of impulsive FONNs in complex field was demonstrated. Limin et al. [47] investigated the asymptotic stability of impulsive delayed fractional order complex valued neural networks with order \(0<\beta <1\) by fractional order comparison principle and Lyapunov functional. However, there are few articles focused on the stability analysis of memristor based neural networks with impulsive effects. To the best of author’s knowledge, nevertheless, Mittag-Leffler finite time stability analysis of fractional order impulsive QVMNNs dynamical behaviours has not been investigated yet.
Sparked by the above reason and discussion, we try to investigate the finite-time Mittag-Leffler stability of fractional-order quaternion-valued memristive neural networks with order \(0<\beta <1\) and \(1<\beta <2\), the problem remains open, and is no article in existing literature. Consequently, we can try to remedy this hard and essential problem. The main challenge and contribution of this work are highlighted in the following aspects:
- 1.
A new mathematical expression of the quaternion-value memductance (memristance) is proposed according to the feature of the quaternion-valued memristive and a new class of FQMNNs is designed.
- 2.
The new brand of novel sufficient criterion proposed first to ensure the existence and finite time Mittag-Leffler stability of impulsive FQMNNs with order \(0<\beta <1\) by means of Banach contraction mapping principle and fractional order Lyapunov functional.
- 3.
When \(\beta \) satisfying \(1<\beta <2\) and the model at the absence of impulsive effects, the finite time stability criteria are introduced by using Laplace transform, Mittag-Leffler function and generalized Gronwall inequality.
- 4.
As some special cases of proposed results, we also investigate the asymptotic stability of FQMNNs with fractional order \(1<\beta <2\).
- 5.
Most of the FNNs have not now taken into consideration quaternion memristive connection weights, system state, and neuron activation, especially FNNs model, however, our results make it up.
The rest of the proposed work is furnished as follows: In Sect. 2, the basic concepts of quaternion algebra, some necessary definitions about fractional order calculus are listed. Further, some necessary assumptions and finite-time Mittag-Leffler stability definitions together with a few beneficial lemmas needed in this paper are given. The main results with order \(0<\beta <1\) and \(1<\beta <2\) are established in Sect. 3. Two numerical examples and their computer simulations are provided to illustrate the effectiveness of the acquired results in Sect. 4. At last, Sect. 5 ends with conclusions.
2 Preliminaries
In this section, we will recall some basic knowledge of quaternion algebraic concepts and fractional order calculus. In addition, some lemmas and problem statement are presented, which serve for the following sections.
2.1 Quaternion Algebra
As a type of super complex number, quaternion consists a real part and three imaginary parts, and a real quaternion or quaternion y can be expressed as:
where \(h,q,w,z\in \mathbb {R}\), and the imaginary roots i, j, k satisfy the Hamilton multiplication rules:
From the above Hamilton rules, the quaternion multiplication is non commutative. The quaternion set is denoted by:
\(\mathbb {Q}^{m}\) signify the set of all m dimensional quaternion space. The operation of addition and subtraction in quaternion field are similar as those in complex numbers or vectors, by
where \(y=h+iq+jw+kz\) and \(z=\tilde{h}+i\tilde{q} +j\tilde{w}+k\tilde{z}\). According to Hamilton multiplication rules (1), the product of yz is described as:
The absolute values of y is described by:
For a quaternion valued function x(t) is denoted by \(y(t)=h(t)+iq(t)+jw(t)+kz(t)\), where \(h(t),\;q(t),\;w(t),\;z(t)\) are all real-valued function. Furthermore, the norm of y of vector quaternion \(y=\big (y_1,\ldots ,y_m\big )^T\in \mathbb {Q}^m\) is given by
2.2 Basic Tools of Fractional Calculus
Definition 2.1
[48] The Riemann–Liouville fractional integral of y(t) is defined as:
where \(\beta \in \mathbb {R}^{+}\).
Definition 2.2
[48] The Caputo-type fractional integral of y(t) is defined as:
where \(\beta \in \mathbb {R}^{+},\;m\in \mathbb {Z}^{+}\).
Proposition 1
[48] The linearity of Caputo-type fractional derivation is defined by
Definition 2.3
[48] The Mittag-Leffler function with two parameter is defined as
where \(\beta ,\;\sigma \in \mathbb {R}^{+},\;z\in \mathbb {C}\).
Definition 2.4
[48] For \(m-1<\beta <m\), the Laplace transform of the Mittag-Leffler function with two parameter:
where s and t are both variables in Laplace domain and time domain, respectively.
Lemma 2.5
[48] When \(\beta \in (0,2),\;\sigma >0\) and \(\omega \in (\frac{\beta \pi }{2},\min \{\beta \pi ,\pi \})\), then there exist two known positive scalars \(\Lambda _1>0,\;\Lambda _2>0\), such that
where \(|arg(z)|\le \omega \), \(|z|\ge 0\).
Lemma 2.6
[49] Let g(t) and u(t) are locally integrable and non negative function on the interval [0, b) and \(d(t)\le F\) defined on [0, b), where \(F>0\) is a constant. If \(\beta >0\) and the following relationships hold:
then we have
if u(t) is non decreasing on [0, b), then g(t) satisfies
Lemma 2.7
[50] If y(t) is the continuously derivable function, the following relationship true almost everywhere:
Lemma 2.8
[51] When \(\beta \in [0,2),\;\sigma >0\) and matrix A is diagonal stable, then there exist greatest eigenvalues of A, namely \(\varsigma \), such that
If \(\sigma =1,2,\beta \) and \(0\le \beta \), then
Lemma 2.9
[52] Let H(t) be a continuous derivable function on \([0,+\infty )\) satisfying
for constants \(\varepsilon _{1},\;\varepsilon _{2}\ge 0\), then
2.3 Problem Statement
In this paper, we consider a class of fractional-order quaternion-valued memristive neural networks (FQMNNs) with impulsive effects described by:
where \(p,s\in \{1,2,\ldots ,m\},\;t\ge 0\), \(D^{\beta }\) is the Caputo fractional derivative of order \(\beta \;(0<\beta <1)\), \(y_{p}(t)\in \mathbb {Q}\) signifies the state vector of the pth neuron at time t, \(a_{i}\) signifies the self feedback connection weights of pth neurons, \(L_{p}(t)\in \mathbb {Q}\) is time-varying external inputs, \(f_{s}\big (y_{s}(t)\big )\) stands for nonlinear quaternion-valued activation function of the sth neurons at time t, \(u_{ps}\big ( y_{s}(t)\big )\) is quaternion-valued memristive connection strengths, that can be discontinuous. The impulsive moment \(t_\tau ,\;\tau =1,2,\ldots \) satisfy \(0<t_1<t_2,\ldots \), \(\lim _{t\rightarrow \infty }t_\tau =+\infty \), \(y_{p}(t^{+}_{\tau })=\lim _{t\rightarrow t^{+}_{\tau }}y_{p}(t)\) and \(y_{p}(t^{-}_{\tau }) = \lim _{t\rightarrow t^{-}_{\tau }}y_{p}(t)\) are the right and left limits of \(y_{p}(t_{\tau })\), respectively. Without loss of generality we assume that, \(y_{p}(t_{\tau })=y_{p}(t^{-}_{\tau })\), which implies the solution of FQMNNs (2) is left continuous at time \(t_\tau \), the initial states of FQMNNs (2) is describes as \(y_{p}(0)=y_{p0}\).
Let
and \(\partial \Upsilon \) stands for the boundary of domain (3). The memristive connection weight is defined by:
for \(p,s\in \{1,2,\ldots ,m\}\), where \(F^{R}_{s},\;F^{I}_{s}, \;F^{J}_{s},\;F^{K}_{s}\) are known positive constants, \(\hat{u}_{ps},\check{u}_{ps}\in \mathbb {Q}\).
Since, the memristive connection strength of FQMNNs (2) is the sense of discontinuity form. As a result, the traditional solution for fractional order differential equations does not suitable to FQMNNs (2). In this case, we need to study the concept of Filippov solutions of considering the fractional order discontinuous right-hand side system.
By means of set valued mapping analysis and differential inclusion theory [53], FQMNNs (2) can be written as follows:
Let \(y_{s}=h_s+iq_s+jw_s+kz_s\in \mathbb {Q}\) and \(f_{s}(y_s)\) can be expressed by splitting into its a real part and three imaginary parts as follows:
Moreover, let \(u_{ps}(y_s)=u^{R}_{ps}(y_s)+iu^{I}_{ps}(y_s) +ju^{J}_{ps}(y_s) +ku^{K}_{ps}(y_s)\), \(\hat{u}_{ps}(y_s) =\hat{u}^{R}_{ps}(y_s)+i\hat{u}^{I}_{ps}(y_s)+j\hat{u}^{J}_{ps}(y_s) +k\hat{u}^{K}_{ps}(y_s)\), \(\check{u}_{ps}(y_s)=\check{u}^{R}_{ps} (y_s) +i\check{u}^{I}_{ps}(y_s)+j\check{u}^{J}_{ps}(y_s) +k\check{u}^{K}_{ps}(y_s)\). Then, we have
for \(p,s=1,2\ldots ,n\). Let \(y_{p}=h_p+iq_p+jw_p+kz_p\in \mathbb {Q}\), FQMNNs (2) can be expressed as follows:
By using differential inclusion of (8)–(11), one has
Equivalently, there exist \(\lambda ^{R}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{R}_{ps}\}\big ( \cdot \big )\), \(\lambda ^{I}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{I}_{ps}\}\big ( \cdot \big )\), \(\lambda ^{J}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{J}_{ps}\}\big ( \cdot \big )\) and \(\lambda ^{K}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{K}_{ps}\}\big ( \cdot \big )\) such that
In order to prove our stability results, for FQMNNs (2), we need the following assumptions and Lemma.
Assumption
\([\mathcal {A}_{1}]\) For any \(y_{s}\in \partial \Upsilon \), \(f_s(y_s)=0\).
Assumption
\([\mathcal {A}_{2}]\) For any \(y_1=h_1+iq_1+jw_1+kz_1,\;y_2 =h_2+iq_2+jw_2+kz_2\in \mathbb {Q}\) and \(s\in \{1,2,\ldots ,m\}\), there exist positive scalars \(\Phi ^{R1}_{s},\;\Phi ^{R2}_{s}, \;\Phi ^{R3}_{s},\;\Phi ^{R4}_{s}\), \(\Phi ^{I1}_{s},\;\Phi ^{I2}_{s},\;\Phi ^{I3}_{s},\;\Phi ^{I4}_{s}\), \(\Phi ^{J1}_{s},\;\Phi ^{J2}_{s},\;\Phi ^{J3}_{s},\;\Phi ^{J4}_{s}\), \(\Phi ^{K1}_{s},\;\Phi ^{K2}_{s},\;\Phi ^{K3}_{s},\;\Phi ^{K4}_{s}\) such that
Remark 2.10
Let \(f^{R}_s(y_s)=\tilde{f}^{R}_{s}\big ( h_{s},q_{s},w_{s},z_{s}\big )\), \(f^{I}_s(y_s)=\tilde{f}^{I}_{s}\big ( h_{s},q_{s},w_{s},z_{s}\big )\), \(f^{J}_s(y_s)=\tilde{f}^{J}_{s}\big ( h_{s},q_{s},w_{s},z_{s}\big )\) and \(f^{K}_s(y_s)=\tilde{f}^{K}_{s}\big ( h_{s},q_{s},w_{s},z_{s}\big )\). Assumption \([\mathcal {A}_{2}]\) holds if and only if \(\tilde{f}^{R}_{s}\big ( \pm F^{R}_{s},q_{s},w_{s},z_{s}\big ) =\tilde{f}^{I}_{s}\big (h_{s}, \pm F^{I}_{s},w_{s},z_{s}\big ) =\tilde{f}^{J}_{s}\big (h_{s}, q_{s},\pm F^{J}_{s},z_{s}\big ) =\tilde{f}^{K}_{s}\big (h_{s}, q_{s},w_{s},\pm F^{K}_{s}\big )\equiv 0\), for any \(h_{s},q_{s},w_{s},z_{s}\in \mathbb {R}\).
Remark 2.11
The first order partial derivatives of \(\tilde{f}^{R}_{s}\big (\cdot ,\cdot ,\cdot ,\cdot \big )\), \(\tilde{f}^{I}_{s}\big (\cdot ,\cdot ,\cdot ,\cdot \big )\), \(\tilde{f}^{J}_{s}\big (\cdot ,\cdot ,\cdot ,\cdot \big )\), \(\tilde{f}^{K}_{s}\big (\cdot ,\cdot ,\cdot ,\cdot \big )\) with respect to h, q, w, z exists and are continuous and bounded, that is, there exist positive constants \(\Phi ^{R1}_{s},\;\Phi ^{R2}_{s},\;\Phi ^{R3}_{s},\;\Phi ^{R4}_{s}\), \(\Phi ^{I1}_{s},\;\Phi ^{I2}_{s},\;\Phi ^{I3}_{s},\;\Phi ^{I4}_{s}\), \(\Phi ^{J1}_{s},\;\Phi ^{J2}_{s},\;\Phi ^{J3}_{s},\;\Phi ^{J4}_{s}\), \(\Phi ^{K1}_{s},\;\Phi ^{K2}_{s},\;\Phi ^{K3}_{s},\;\Phi ^{K4}_{s}\) such that
Therefore, Assumption \([\mathcal {A}_{2}]\) satisfied by means of mean value theorem for multi-variable functions.
Lemma 2.12
Under Assumptions \([\mathcal {A}_{1}]\) and \([\mathcal {A}_{2}]\), for any \(y_1=h_1+iq_1+jw_1+kz_1,\;y_2=h_2+iq_2+jw_2+kz_2\in \mathbb {Q}\), there is \(\tilde{f}^{R}_{s}\big ( \pm F^{R}_{s},q_{s},w_{s},z_{s}\big )=0\), then
where \(p,s\in \{1,2,\ldots ,m\}\), \(u^{R}_{ps}=\max \{|\hat{u}^{R}_{ps}|,|\check{u}^{R}_{ps}|\}\), \(u^{I}_{ps}=\max \{|\hat{u}^{I}_{ps}|,|\check{u}^{I}_{ps}|\}\), \(u^{J}_{ps}=\max \{|\hat{u}^{J}_{ps}|,|\check{u}^{J}_{ps}|\}\), and \(u^{K}_{ps}=\max \{|\hat{u}^{K}_{ps}|,|\check{u}^{K}_{ps}|\}\).
Proof
From (16) is equivalent to prove
The proof of (16) will be splitted into the following two cases.
Case 1. \(y_1,y_2 \overline{\in }\;\; \Upsilon ^{T}_{p}\). Then \(\lambda ^{R}_{ps}\big ( y_{1}\big )=\lambda ^{R}_{ps}\big ( y_{2}\big )\), hence
Case 2. \(\,y_1\in \Upsilon ^{T}_{p}\) and \(y_2\; \overline{\in }\; \Upsilon ^{T}_{p}\)\(\Big (or \Big )\;y_2\in \Upsilon ^{T}_{p}\) and \(y_1 \overline{\in }\; \Upsilon ^{T}_{p}\). The Proof of the case 2 can be dived into two subcases, which is followed by
Subcase (i) \(\,F^{R}_{s}\le h_{2}\). Since \(\tilde{f}^{R}_{s}\big ( F^{R}_{s},q_1,w_1,z_1 \big ) =\tilde{f}^{R}_{s}\big ( F^{R}_{s},q_2,w_2,z_2 \big )=0\), one has
Subcase (ii) \(-F^{R}_{s}\le h_{2}\). Since \(\tilde{f}^{R}_{s}\big ( -F^{R}_{s},q_1,w_1,z_1 \big ) =\tilde{f}^{R}_{s}\big ( -F^{R}_{s},q_2,w_2,z_2 \big )=0\), one has
From the above two cases, inequality (16) holds, thus the proof of (20) is finished. The rest of the proof of (17)–(19) are similar to the proof of (16). Hence, it is omitted here. \(\square \)
Corollary 2.13
Under Assumptions \([\mathcal {A}_{1}]\) and \([\mathcal {A}_{2}]\), for any \(y_1=h_1+iq_1+jw_1+kz_1,\;y_2=h_2+iq_2+jw_2+kz_2\in \mathbb {Q}\), there is \(\tilde{f}^{R}_{s}\big ( \pm F^{R}_{s},q_{s}, w_{s},z_{s}\big ) = \tilde{f}^{I}_{s}\big (h_{s}, \pm F^{I}_{s},w_{s},z_{s}\big ) =\tilde{f}^{J}_{s}\big (h_{s}, q_{s},\pm F^{J}_{s},z_{s}\big )= \tilde{f}^{K}_{s}\big (h_{s}, q_{s},w_{s},\pm F^{K}_{s}\big )= 0\), then
where \(p,s\in \{1,2,\ldots ,m\}\), \(u^{R}_{ps}\), \(u^{I}_{ps}\), \(u^{J}_{ps}\), and \(u^{K}_{ps}\) are already defined in Lemma 2.12.
Considering \(y'_{p}(t)=\big ( y'_{1}(t),\ldots ,y'_{m}(t) \big )^{T}\) and \(y''_{p}(t)=\big ( y''_{1}(t),\ldots ,y''_{m}(t)\big )^{T}\) are any two solutions of FQMNNs (2) with different initial condition \(y'_{p}(0)=y'_{0p}\) and \(y''_{p}(0)=y''_{0p}\) for \(p\in \{1,2,\ldots ,m\}\).
Let \(y'_{p}(t)=h'_p(t)+iq'_p(t)+jw'_p(t)+kz'_p(t)\) and \(y''_{p}(t)=h''_p(t)+iq''_p(t)+jw''_p(t)+kz''_p(t)\), then
almost everywhere \(t\ge t_{0}\) and
where \(\lambda ^{R}_{ps}\big ( \cdot \big ),\;\tilde{\lambda }^{R}_{ps} \big ( \cdot \big )\in \overline{co}\{u^{R}_{ps}\}\big ( \cdot \big )\), \(\lambda ^{I}_{ps}\big ( \cdot \big ),\;\tilde{\lambda }^{I}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{I}_{ps}\}\big ( \cdot \big )\), \(\lambda ^{J}_{ps}\big ( \cdot \big ),\;\tilde{\lambda }^{J}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{J}_{ps}\}\big ( \cdot \big )\) and \(\lambda ^{K}_{ps}\big ( \cdot \big ),\;\tilde{\lambda }^{K}_{ps}\big ( \cdot \big )\in \overline{co}\{u^{K}_{ps}\}\big ( \cdot \big )\).
Definition 2.14
[18] Let \(y'(t)=\big (y'_{1}(t),\ldots ,y'_{m}(t)\big )^{T}\) and \(y''(t)=\big (y''_{1}(t),\ldots ,y''_{m}(t)\big )^{T}\) are any solutions of FQMNNs (2) is said to be Mittag-Leffler stable in finite time with respecting to \(\{\rho ,\varrho ,T\}\), if there exist constants \(\rho>\varrho >0\) such that
when
where \(t_{0}\) is starting time.
Definition 2.15
Let \(y'(t)=\big (y'_{1}(t),\ldots ,y'_{m}(t)\big )^T\) and \(y''(t)=\big (y''_{1}(t),\ldots ,y''_{m}(t)\big )^T\) are any solutions of FQMNNs (2) is said to be asymptotically stable, if
Let \(x_{p}(t)=y'_{p}(t)-y''_{p}(t)\), where \(x_{p}(t)=x^{R}_{p}(t)+ix^{I}_{p}(t)+jx^{J}_{p}(t)+kx^{K}_{p}(t)\), that is \(x^{R}_{p}(t)=h'_{p}(t)-h''_{p}(t)\), \(x^{I}_{p}(t)=q'_{p}(t)-q''_{p}(t)\), \(x^{J}_{p}(t)=w'_{p}(t)-w''_{p}(t)\) and \(x^{K}_{p}(t)=z'_{p}(t)-z''_{p}(t)\), then the error system is
where
Remark 2.16
In FQMNNs (2), if \(y_{p}(t)=h_{p}(t)+iq_{p}(t)\), and all the coefficients of (2) are assumed to complex coefficients, at that point FQMNNs (2) will turn to impulsive finite-time stability of fractional order memristive neural networks in complex field; If all the coefficients of (2) are assumed to real coefficients, at that point FQMNNs (2) will turn to impulsive finite-time stability of fractional order memristive neural networks in real field.
3 Main Results
In this section, we will present the finite time stability results for FQMNNs (2) with fractional order \(0<\beta <1\) and \(1<\beta <2\).
3.1 Fractional-Order \(0<\beta <1\)
In this subsection, we studies some novel sufficient conditions to guarantee the finite time stability of the solutions of impulsive FQMNNs (2) by using Lyapunov function, Mittag-Leffler function and fractional-order differential inequalities.
Theorem 3.1
Under Assumptions \([\mathcal {A}_1]-[\mathcal {A}_{2}]\), FQMNNs (2) is finite-time Mittag-Leffler stable if the following relationship holds:
- (i)
There exists constants \(\theta _{p\tau }, \;\varepsilon _{p\tau },\;\eta _{p\tau },\;\sigma _{p\tau }\), the functions \(S^{R}_{p\tau }(\cdot ),\;S^{I}_{p\tau }(\cdot ), \;S^{J}_{p\tau }(\cdot )\) and \(S^{K}_{p\tau }(\cdot )\) satisfies
$$\begin{aligned} S^{R}_{p_\tau }(h'_{p}(t)-h''_{p}(t))= & {} -\,\theta _{p\tau }(h'_{p}(t) -h''_{p}(t)),\;0<\theta _{p\tau }<2,\\ S^{I}_{p_\tau }(q'_{p}(t)-q''_{p}(t))= & {} -\,\varepsilon _{p\tau }(q'_{p}(t) -q''_{p}(t)),\;0<\varepsilon _{p\tau }<2,\\ S^{J}_{p_\tau }(w'_{p}(t)-w''_{p}(t))= & {} -\,\eta _{p\tau }(w'_{p}(t) -w''_{p}(t)),\;0<\eta _{p\tau }<2,\\ S^{K}_{p_\tau }(z'_{p}(t)-z''_{p}(t))= & {} -\,\sigma _{p\tau }(z'_{p}(t) -z''_{p}(t)),\;0<\sigma _{p\tau }<2. \end{aligned}$$ - (ii)
If there are m positive constants \(\xi _{p},\;\zeta _{p}\;\gamma _{p},\;\alpha _{p},\;p=1,2,\ldots ,m\) such that
$$\begin{aligned} F_{1p}= & {} -\xi _{p}a_{p}+\sum _{s=1}^{m}u^{R}_{sp} \big [\xi _{s}\Phi _{p}^{R1} +\zeta _{s}\Phi _{p}^{I1}+\gamma _{s}\Phi _{p}^{J1} +\alpha _{s}\Phi _{s}^{K1}\big ]\\&+\,\sum _{s=1}^{m}u^{I}_{sp}\big [\xi _{s}\Phi _{p}^{I1} +\zeta _{s}\Phi _{p}^{R1} +\gamma _{s}\Phi _{p}^{K1}+\alpha _{p}\Phi _{s}^{J1}\big ]\\&+\,\sum _{s=1}^{m}u^{J}_{sp} \big [\xi _{s}\Phi _{p}^{J1}+\zeta _{s}\Phi _{p}^{K1}+\gamma _{s}\Phi _{p}^{R1} +\alpha _{s}\Phi _{s}^{I1}\big ]\\&+\,\sum _{s=1}^{m}u^{K}_{sp} \big [\xi _{s}\Phi _{p}^{K1} +\zeta _{s}\Phi _{p}^{J1}+\gamma _{s}\Phi _{p}^{I1} +\alpha _{p}\Phi _{s}^{R1}\big ]<0\\ F_{2p}= & {} -\zeta _{p}a_{p}+\sum _{s=1}^{m}u^{R}_{sp} \big [\xi _{s}\Phi _{p}^{R2} +\zeta _{s}\Phi _{p}^{I2}+\gamma _{s}\Phi _{p}^{J2} +\alpha _{s}\Phi _{s}^{K2}\big ]\\&+\,\sum _{s=1}^{m}u^{I}_{sp}\big [\xi _{s}\Phi _{p}^{I2} +\zeta _{s}\Phi _{p}^{R2}+\gamma _{s}\Phi _{p}^{K2} +\alpha _{p}\Phi _{s}^{J2}\big ]\\&+\,\sum _{s=1}^{m}u^{J}_{sp}\big [\xi _{s}\Phi _{p}^{J2} +\zeta _{s}\Phi _{p}^{K2}+\gamma _{s}\Phi _{p}^{R2} +\alpha _{s}\Phi _{s}^{I2}\big ]\\&+\,\sum _{s=1}^{m}u^{K}_{sp}\big [\xi _{s}\Phi _{p}^{K2} +\zeta _{s}\Phi _{p}^{J2}+\gamma _{s}\Phi _{p}^{I2} +\alpha _{p}\Phi _{s}^{R2}\big ]<0\\ F_{3p}= & {} -\gamma _{p}a_{p}+\sum _{s=1}^{m}u^{R}_{sp} \big [\xi _{s}\Phi _{p}^{R3} +\zeta _{s}\Phi _{p}^{I3}+\gamma _{s}\Phi _{p}^{J3} +\alpha _{s}\Phi _{s}^{K3}\big ]\\&+\,\sum _{s=1}^{m}u^{I}_{sp}\big [\xi _{s}\Phi _{p}^{I3} +\zeta _{s}\Phi _{p}^{R3}+\gamma _{s}\Phi _{p}^{K3} +\alpha _{p}\Phi _{s}^{J3}\big ]\\&+\,\sum _{s=1}^{m}u^{J}_{sp}\big [\xi _{s}\Phi _{p}^{J3} +\zeta _{s}\Phi _{p}^{K3}+\gamma _{s}\Phi _{p}^{R3} +\alpha _{s}\Phi _{s}^{I3}\big ]\\&+\,\sum _{s=1}^{m}u^{K}_{sp}\big [\xi _{s}\Phi _{p}^{K3} +\zeta _{s}\Phi _{p}^{J3}+\gamma _{s}\Phi _{p}^{I3} +\alpha _{p}\Phi _{s}^{R3}\big ]<0\\ F_{4p}= & {} -\,\alpha _{p}a_{p}+\sum _{s=1}^{m}u^{R}_{sp} \big [\xi _{s}\Phi _{p}^{R4} +\zeta _{s}\Phi _{p}^{I4}+\gamma _{s}\Phi _{p}^{J4} +\alpha _{s}\Phi _{s}^{K4}\big ]\\&+\,\sum _{s=1}^{m}u^{I}_{sp}\big [\xi _{s}\Phi _{p}^{I4} +\zeta _{s}\Phi _{p}^{R4} +\gamma _{s}\Phi _{p}^{K4} +\alpha _{p}\Phi _{s}^{J4}\big ]\\&+\,\sum _{s=1}^{m}u^{J}_{sp}\big [\xi _{s}\Phi _{p}^{J4} +\zeta _{s}\Phi _{p}^{K4}+\gamma _{s}\Phi _{p}^{R4} +\alpha _{s}\Phi _{s}^{I4}\big ]\\&+\,\sum _{s=1}^{m}u^{K}_{sp}\big [\xi _{s}\Phi _{p}^{K4} +\zeta _{s}\Phi _{p}^{J4} +\gamma _{s}\Phi _{p}^{I4} +\alpha _{p}\Phi _{s}^{R4}\big ]<0. \end{aligned}$$ - (iii)
Furthermore,
$$\begin{aligned} E_{\beta ,1}\big (-\varpi t^{\beta } \big )<\frac{\rho }{\varrho } \end{aligned}$$where \(-\varpi =\max _{1\le p\le m}\big \{F_{1p},F_{2p}, F_{3p},F_{4p}\big \}>0\).
Proof
Consider the following Lyapunov functional
where
Firstly, we consider the case of \(t=t_\tau ,\;\tau =1,2,\ldots \), from condition (i) of Theorem 3.1, one has
Secondly, we consider the case of \(t\ne t_\tau ,\;\tau =1,2,\ldots \). Taking the fractional-order time derivative of V(t) along the trajectories of (30)–(33) and, based on Lemma 2.7, one can get
By virtue of Assumption \([\mathcal {A}_{2}]\) and Lemma 2.12, one gets
Then, we have
Then by virtue of Lemma 2.9, it is easy to get
That is,
then
By using the definition of \(\Vert (\cdot )\Vert _{1}\) and from (39), it can get
where \(\chi =\max _{1\le p\le 4m}\big \{\delta _{p}\big \}\). According to condition (iii) in Theorem 3.1, it is easily to get,
By utilizing Definition 2.14 and inequality (40), the FQMNNs (2) is finite-time Mittag-Leffler stable with respect to \(\{\varrho ,\rho ,T\}\) if there exists \(\Vert y'(0)-y''(0)\Vert \le \varrho \) then it implies \(\Vert y'(t)-y''(t)\Vert \le \rho \). The proof is accomplished. \(\square \)
Now we prove that the existence, uniqueness and global Mittag-Leffler stability of equilibrium point for FQMNNs (2).
Theorem 3.2
Under Assumptions \([\mathcal {A}_1]-[\mathcal {A}_{2}]\) and let \(L_{p}(t)\equiv L_{p}\in \mathbb {Q}\), FQMNNs (2) admits a unique equilibrium point which is finite time Mittag-Leffler stable if the following relationship holds:
- (i)
If there exist a m positive constants \(\delta _{p},\;p=1,2,\ldots ,m\) such that the following conditions hold:
$$\begin{aligned}&\delta _{p}a_{p}-\sum ^{m}_{s=1}\delta _{s}\Big [ u^{R}_{sp} \big ( \Phi ^{R1}_{p} +\Phi ^{R2}_{p}+\Phi ^{R3}_{p}+\Phi ^{R4}_{p}\big ) + u^{I}_{sp} \big ( \Phi ^{I1}_{p}+\Phi ^{I2}_{p}+\Phi ^{I3}_{p}+\Phi ^{I4}_{p}\big )\nonumber \\&\quad +\, u^{J}_{sp} \big ( \Phi ^{J1}_{p}+\Phi ^{J2}_{p}+\Phi ^{J3}_{p} +\Phi ^{J4}_{p}\big ) + u^{K}_{sp} \big ( \Phi ^{K1}_{p}+\Phi ^{K2}_{p} +\Phi ^{K3}_{p}+\Phi ^{K4}_{p}\big ) \Big ]>0, \end{aligned}$$(41)$$\begin{aligned}&\delta _{p}a_{p}-\sum ^{m}_{s=1}\delta _{s}\Big [ u^{R}_{sp} \big ( \Phi ^{I1}_{p}+\Phi ^{I2}_{p}+\Phi ^{I3}_{p}+\Phi ^{I4}_{p}\big )+ u^{I}_{sp} \big ( \Phi ^{R1}_{p}+\Phi ^{R2}_{p}+\Phi ^{R3}_{p}+\Phi ^{R4}_{p}\big )\nonumber \\&\quad +\, u^{J}_{sp} \big (\Phi ^{K1}_{p}+\Phi ^{K2}_{p}+\Phi ^{K3}_{p} +\Phi ^{K4}_{p} \big ) + u^{K}_{sp} \big ( \Phi ^{J1}_{p} +\Phi ^{J2}_{p}+\Phi ^{J3}_{p}+\Phi ^{J4}_{p}\big ) \Big ]>0, \end{aligned}$$(42)$$\begin{aligned}&\delta _{p}a_{p}-\sum ^{m}_{s=1}\delta _{s}\Big [ u^{R}_{sp} \big ( \Phi ^{J1}_{p}+\Phi ^{J2}_{p}+\Phi ^{J3}_{p}+\Phi ^{J4}_{p}\big )+ u^{I}_{sp} \big ( \Phi ^{K1}_{p}+\Phi ^{K2}_{p}+\Phi ^{K3}_{p}+\Phi ^{K4}_{p}\big )\nonumber \\&\quad +\, u^{J}_{sp} \big (\Phi ^{R1}_{p}+\Phi ^{R2}_{p}+\Phi ^{R3}_{p}+\Phi ^{R4}_{p} \big ) + u^{K}_{sp} \big ( \Phi ^{I1}_{p}+\Phi ^{I2}_{p}+\Phi ^{I3}_{p} +\Phi ^{I4}_{p}\big ) \Big ]>0, \end{aligned}$$(43)$$\begin{aligned}&\delta _{p}a_{p}-\sum ^{m}_{s=1}\delta _{s}\Big [ u^{R}_{sp} \big ( \Phi ^{K1}_{p}+\Phi ^{K2}_{p}+\Phi ^{K3}_{p}+\Phi ^{K4}_{p}\big )+ u^{I}_{sp} \big (\Phi ^{J1}_{p}+\Phi ^{J2}_{p}+\Phi ^{J3}_{p}+\Phi ^{J4}_{p}\big )\nonumber \\&\quad +\, u^{J}_{sp} \big (\Phi ^{I1}_{p}+\Phi ^{I2}_{p}+\Phi ^{I3}_{p}+\Phi ^{I4}_{p} \big ) + u^{K}_{sp} \big ( \Phi ^{R1}_{p}+\Phi ^{R2}_{p}+\Phi ^{R3}_{p} +\Phi ^{R4}_{p}\big ) \Big ]>0. \end{aligned}$$(44)
Proof
Given any \(\lambda _{ps}(\cdot )\in \overline{co}\{u_{ps}\}(\cdot )\), construct a contraction mapping \(\Lambda :\mathbb {Q}^{m}\rightarrow \mathbb {Q}^{m}\), \(\Lambda (y)=\big ( \Lambda _1(y),\ldots ,\Lambda _m(y) \big )^T\) and
Then
For any two quaternion vectors \(v_{1}=\big (v_{11},v_{12},\ldots ,v_{1m} \big )^{T}\), \(v_{2}=\big (v_{21},v_{22},\ldots ,v_{2m} \big )^{T}\in \mathbb {Q}^{m}\). Let \(v_{1s}=h_{1s}+iq_{1s}+jw_{1s}+kz_{1s}\), \(v_{2s}=h_{2s}+iq_{2s}+jw_{2s}+kz_{2s}\in \mathbb {Q}\). By virtue of Lemma 2.12, one has
By using Definition of \(\Vert \cdot \Vert _1\), and from (50)–(53), we have
Let
For any \(y=\big ( y_1,\ldots ,y_m \big )^T\in \mathbb {Q}^{m}\), let \(\Vert y\Vert _{1}=\sum ^{4m}_{p=1}|y_{p}|=\sum ^{m}_{p=1}\big [|y^{R}_{p}| +|y^{I}_{p}|+|y^{J}_{p}|+|y^{K}_{p}|\big ]\). From (47), one has
where \(\mu =\max \big \{ \max _{1\le p\le m}\{\mu ^{R}_{p}\},\max _{1\le p\le m}\{\mu ^{I}_{p}\}, \max _{1\le p\le m}\{\mu ^{J}_{p}\},\max _{1\le p\le m}\{\mu ^{K}_{p}\} \big \}\). From (34)–(37), it follows that \(\mu \in (0,1)\). According to (55), the mapping \(\Lambda :\mathbb {Q}^m\rightarrow \mathbb {Q}^m\) is a contraction mapping on \(\mathbb {Q}^{m}\). Therefore there exist a unique fixed point \(\Lambda (v^{\star })=v^{\star }\), i.e.,
Let \(y_{p}^{\star }=\frac{v^{\star }_p}{a_p\delta _{p}}\), it can obtain
that is
for \(p=1,2,\ldots ,m\), which implies that \(y^{\star }\) is an unique solution of FQMNNs (2). In addition, condition (41)–(44) imply conditions (ii) of Theorem 3.1 holds. By virtue of Theorem 3.1, if there exist a constant \(\varpi \) such that
Therefore, unique solution of FQMNNs (2) is finite-time Mittag-Leffler stable with respect to \(\{\varrho ,\rho ,T\}\) if there exists \(\Vert y(0)-y^{\star }\Vert \le \varrho \) then it implies \(\Vert y(t)-y^{\star }\Vert \le \rho \), and the proof of Theorem is completed. \(\square \)
Remark 3.3
When \(\beta =1\), model (2) degenerates into integer order finite time stability of QVMNNs.
Remark 3.4
When the state vector \(y_{p}(t)\), the memristive connection weight \(u_{ps}(y_s(t))\), the nonlinear activation \(f_{s}(y_s(t))\) and external input \(L_{p}(t)\) are all in complex domain (or real domain), model (2) can be reduced into finite time stability of complex (or real) valued memristive neural networks. So proposed in this model is more advanced.
Remark 3.5
The impulsive FQMNNs (2) is the corresponding closed-loop system to the control system
where \(\Delta _{p}(t)=\sum ^{+\infty }_{\tau =1} \delta (t-t_\tau ),\;p\in \{1,2,\ldots ,m\}\) is the control inputs, \(\delta (t)\) is the Dirac impulsive function. The impulsive controller has an effect on abrupt change of the states of (2) at \(t=t_\tau \) due to which the states of units change from the position \(y_{p}(t^{-}_\tau )\) into the position \(y_{p}(t^{+}_\tau )\), the function \(S_{p\tau }\) characterize the magnitudes of the impulse effects on the units \(y_{p}\) at \(t=t_\tau \). i.e., \(\Delta _{p}(t)\) is an impulsive controller of the FQMNNs
Therefore, Theorem 3.1 represents the general method of impulsive control law (3.3) for FQMNNs (57). The constants \(\theta _{p\tau }\), \(\varepsilon _{p\tau }\), \(\eta _{p\tau }\) and \(\sigma _{p\tau }\) in condition (i) in Theorem 3.1 characterize the stabilizing impulses. Therefore, our obtained results in Theorem 3.1 can be used to design impulsive control law under the controlled FQMNNs (2) are finite time stabilized onto FQMNNs (57).
3.2 Fractional-order \(1<\beta <2\)
When the impulses are not taken into consideration, FQMNNs (2) degenerates into the following expression:
or equivalently,
where \(A=diag\{a_1,\ldots ,a_m\}\), \(y(t)=\big ( y_{1}(t),\ldots ,y_{m}(t) \big )^T\), \(U(y(t))=\big (u_{ps}(y_{p}(t))\big )_{n\times n}\), \(f(y(t))=\big ( y_1(t),\ldots ,y_{m}(t) \big )^{T}\), \(L(t)=(L_1(t),\ldots ,L_{m}(t))^{T}\).
For the sake of convenience, we define \(U^{R} =\big (u^{R}_{ps} \big )_{m\times m}\), \(U^{I}=\big ( u^{I}_{ps} \big )_{m\times m}\), \(U^{J}=\big ( u^{J}_{ps} \big )_{m\times m}\), \(U^{K}=\big ( u^{K}_{ps} \big )_{m\times m}\), \(\Phi ^{R1}=\max _{1\le s\le m}\{\Phi _{s}^{R1}\}\), \(\Phi ^{R2}=\max _{1\le s\le m}\{\Phi _{s}^{R2}\}\), \(\Phi ^{R3}=\max _{1\le s\le m}\{\Phi _{s}^{R3}\}\), \(\Phi ^{R4}=\max _{1\le s\le m}\{\Phi _{s}^{R4}\}\), \(\Phi ^{I1}=\max _{1\le s\le m} \{\Phi _{s}^{I1}\}\), \(\Phi ^{I2}=\max _{1\le s\le m}\{\Phi _{s}^{I2}\}\), \(\Phi ^{I3}=\max _{1\le s\le m}\{\Phi _{s}^{I3}\}\), \(\Phi ^{I4}=\max _{1\le s\le m}\{\Phi _{s}^{I4}\}\), \(\Phi ^{J1}=\max _{1\le s\le m}\{\Phi _{s}^{J1}\}\), \(\Phi ^{J2}=\max _{1\le s\le m}\{\Phi _{s}^{J2}\}\), \(\Phi ^{J3}=\max _{1\le s\le m}\{\Phi _{s}^{J3}\}\), \(\Phi ^{J4}=\max _{1\le s\le m}\{\Phi _{s}^{J4}\}\), \(\Phi ^{K1}=\max _{1\le s\le m}\{\Phi _{s}^{K1}\}\), \(\Phi ^{K2}=\max _{1\le s\le m}\{\Phi _{s}^{K2}\}\), \(\Phi ^{K3}=\max _{1\le s\le m}\{\Phi _{s}^{K3}\}\) and \(\Phi ^{K4}=\max _{1\le s\le m}\{\Phi _{s}^{K4}\}\).
Then, we state the finite time stability results of FQMNNs (59) as the following theorem.
Theorem 3.6
Under Assumptions \([\mathcal {A}_1]-[\mathcal {A}_{2}]\), FQMNNs (59) is finite-time stable if the following relationship holds:
where \(\theta =\min _{1\le p\le m}\{a_{p}\}\), \(\Upsilon (t)=\max \{g_{1}(t),g_{2}(t),g_{3}(t),g_{4}(t)\}\),
Proof
Let \(y'(t)=h'(t)+iq'(t)+jw'(t)+kz'(t)\) and \(y''(t)=h''(t)+iq''(t)+jw''(t)+kz''(t)\) are any two solutions of FQMNNs (59) with initial values \(y'(0)=y'_0,\;y''(0)=y''_0\) and \(x(t)=y''(t)-y'(t)\), \(\eta (0)=y'(0)-y''(0)\), it can be obtained that
where \(\Psi ^{RR}\big ( \cdot \big )\), \(\Psi ^{II}\big ( \cdot \big )\), \(\Psi ^{JJ}\big ( \cdot \big )\Psi ^{KK}\big ( \cdot \big )\), \(\Psi ^{RI}\big ( \cdot \big )\), \(\Psi ^{IR}\big ( \cdot \big )\), \(\Psi ^{JK}\big ( \cdot \big )\), \(\Psi ^{KJ}\big ( \cdot \big )\), \(\Psi ^{RJ}\big ( \cdot \big )\), \(\Psi ^{IK}\big ( \cdot \big )\), \(\Psi ^{JR}\big ( \cdot \big )\), \(\Psi ^{KI}\big ( \cdot \big )\), \(\Psi ^{RK}\big ( \cdot \big )\), \(\Psi ^{IJ}\big ( \cdot \big )\), \(\Psi ^{JI}\big ( \cdot \big )\) and \(\Psi ^{KR}\big ( \cdot \big )\) are already defined in Sect. 2.
By virtue of Lemma 2.12 and, from \([\mathcal {A}_1]-[\mathcal {A}_{2}]\), it can be followed that
Use Laplace transform and inverse Laplace transform of both sides of (61)–(64), one has
By application of Lemma 2.8, and from (65), we have
Similarly,
\(\Vert x^{R}(t)\Vert +\Vert x^{I}(t)\Vert +\Vert x^{J}(t)\Vert +\Vert x^{K}(t)\Vert \)
We denote \(g_{\tau }(t)=\Vert U^{R}\Vert \Big (\Phi ^{R\tau } +\Phi ^{I\tau }+\Phi ^{J\tau }+\Phi ^{K\tau } \Big ) +\Vert U^{I}\Vert \Big (\Phi ^{I\tau }+\Phi ^{R\tau }+\Phi ^{K\tau }+\Phi ^{J\tau }\Big ) +\Vert U^{J}\Vert \Big (\Phi ^{J\tau }+\Phi ^{K\tau }+\Phi ^{R\tau }+\Phi ^{I\tau } \Big ) +\Vert U^{K}\Vert \Big (\Phi ^{K\tau }+\Phi ^{J\tau }+\Phi ^{R\tau } +\Phi ^{I\tau }\Big ),\;\tau =1,2,3,4,\; \varepsilon (t) =\Big [\big \Vert \eta ^{R}_{0}(0)\big \Vert +\big \Vert \eta ^{I}_{0}(0)\big \Vert +\big \Vert \eta ^{J}_{0}(0)\big \Vert +\big \Vert \eta ^{K}_{0}(0)\big \Vert \Big ] +t\big [\big \Vert \eta ^{R}_{1}(0)\big \Vert +\big \Vert \eta ^{I}_{1}(0)\big \Vert +\big \Vert \eta ^{J}_{1}(0)\big \Vert +\big \Vert \eta ^{K}_{1}(0)\big \Vert \Big ] =\Vert \eta _{0}(0)\Vert +t\Vert \eta _{1}(0)\Vert \), from inequality (73), we have
In light of famous generalized Gronwall Lemma 2.6, it follows that
Let \(\Vert \eta (0)\Vert <\varrho \), then we have
Hence, if the condition of Theorem 3.5 hold, we gain \(\Vert x(t)\Vert<\rho ,\;0\le t<T\), where \(\Upsilon (t)=\max _{1\le \tau \le 4}\{g_{\tau }(t)\}\). It means FQMNNs (59) is finite-time stable via Definition 2.14. Proof completed. \(\square \)
Corollary 3.7
Under Assumptions \([\mathcal {A}_1]-[\mathcal {A}_{2}]\), FQMNNs (59) is asymptotically stable if the following relationship holds:
where \(\Upsilon (t)\) and \(\theta \) both respectively, are defined in Theorem 3.5.
Proof
According to the proof Theorem 3.5, from (74), we can get
By means of Lemma 2.5, there exists two positive constants \(\Lambda _1,\Lambda _2>0\) such that
That is
Hence, if the condition of Corollary 3.6 hold, from (75), \(x(t)\rightarrow 0\), as \(t\rightarrow +\infty \), which implies FQMNNs (59) is asymptotically stable based on Definition 2.15. Proof completed. \(\square \)
Remark 3.8
If the memristive connection weights of FQMNNs (2) is invariable,i.e., \(u_{ps}\big ( y_{s}(t)\big )\) is constant, then FQMNNs (2) degenerates into finite-time stability of fractional order quaternion-valued neural networks.
4 Numerical Examples
To verify the advantage of the above theoretical results, two numerical computer simulations are performed in the following few lines.
Example 4.1
Consider the following two dimensional FQMNNs:
for \(p=1,2,\;t\ge 0\), where \(\beta =0.91\), \(\theta _{1\tau }=\theta _{2\tau }=1.7\), \(\varepsilon _{1\tau }=\varepsilon _{2\tau }=1.65\), \(\eta _{1\tau }=\eta _{2\tau }=1.5\), \(\sigma _{1\tau }=\sigma _{2\tau }=1.65\), \(a_{1}=a_{2}=6\), \(L_{1}(t)=L_{2}(t)=0\), the activation function \(f_{s} \big (y_{s}\big )=\tanh ( y_{s}),\;s=1,2\), and the memristive connection weights as follows:
here
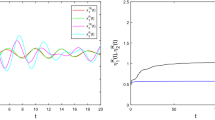
It is obvious that the assumption \([\mathcal {A}_{1}]-[\mathcal {A}_{2}]\) holds with \(\Phi _{R\iota }=0.1\), \(\Phi _{I\iota }=0.15\), \(\Phi _{J\iota }=0.2\), \(\Phi _{K\iota }=0.25\) for \(\iota =1,2,3,4\). By Theorem 3.1, we select \(\xi _{p}=1,\;\zeta _{p}=1.5,\;\gamma _{p}=2,\;\alpha _{p} =1.5\) and by using of above values, and from conditions of Theorem 3.1, we have \(F_{11}=-1.605,\;F_{12}=-1.405\), \(F_{21}=-3.105,\;F_{22}=-2.905\), \(F_{31}=-4.605,\;F_{32}=-7.605\), \(F_{41}=-1.605,\;F_{42}=-7.405\). Obviously, the conditions of Theorem 3.1 holds, and t is replaced by T. Let from \(\rho =9,\;\varrho =0.9\), \(E_{\beta ,1}\big (-\varpi t^{\beta } \big )<\frac{\rho }{\varrho }\). Therefore, FQMNNs (76) is stable in finite time, that is \(T=0.7339\). From the Numerical simulations, the initial values of (76) are selected as: \(y(0) =\big (1.4-1.5i+1.5j-1.6k,-1.2+0.45i-1.2j+k \big )^{T}\). In Figs. 1, 2, 3 and 4 presents the state trajectories of a real part h(t) and three imaginary parts \(q(t),\;w(t),\;z(t)\) of system (76), which confirms the validity of Theorem 3.1.
Example 4.2
Consider the following two dimensional FQMNNs:
for \(p=1,2,\;t\ge 0\), where \(\beta =1.75\), \(a_{1}=a_{2}=8\), \(L_{1}(t)=L_{2}(t)=0\), the activation function \(f_{s} \big (y_{s}\big )=\tanh ( y_{s}),\;s=1,2\), and the memristive connection weights as follows:
here
It is obvious that the assumption \([\mathcal {A}_{1}]-[\mathcal {A}_{2}]\) holds with \(\Phi _{R1}=0.1\), \(\Phi _{R2}=0.05\), \(\Phi _{R3}=0.2\), \(\Phi _{R4}=0.15\), \(\Phi _{I\iota }=0.2\), \(\Phi _{J1}=0.3\), \(\Phi _{J2}=0.4\), \(\Phi _{J3}=0.1=\Phi _{J4}=0.25\), \(\Phi _{K\iota }=0.35\) for \(\iota =1,2,3,4\). The initial values of FQMNNs (76) are chosen as: \(y(0)=\big ( -1.5+0.7i+1.5j+2.4k,1.3-0.8i+2.4j-2.5k \big )^{T}\). Now, \(\Vert U^{R}\Vert =\Vert U^{I}\Vert =0.75\), \(\Vert U^{J}\Vert =0.85\), \(\Vert U^{K}\Vert =1.45\), \(\theta =6\), \(\Gamma (1.75)=0.919\), then \(\Upsilon (t)\Gamma (1.75)=5.3302\). Let \(\varrho =0.5\) and \(\rho =5\), from
Therefore, FQMNNs (77) is stable in finite time, that is \(T=1.984\). Furthermore,
then FQMNNs (77) is globally asymptotically stable based on Corollary 3.6. In Figs. 5, 6, 7 and 8 depicts the state curves of a real part h(t) and three imaginary parts \(q(t),\;w(t),\;z(t)\) of system (77), which also assure the effectiveness of Theorem 3.5.
5 Conclusion
Hereof, the finite time stability of fractional order quaternion-valued memristor-based neural networks with order \(0<\beta <1\) and \(1<\beta <2\) has been studied. First of all, A new mathematical expression of the quaternion-value memductance (memristance) is proposed according to the feature of the quaternion-valued memristive and a new class of FQMNNs is designed. Secondly, via differential inclusion theory, Filippov’s solution, contraction mapping principle, Lyapunov function, and concept of quaternion algebra, the existence and finite time Mittag-Leffler stability criteria of FQMNNs with impulses have obtained when fractional order satisfying \(0<\beta <1\). Moreover, when impulsive effects are not taken into consideration, the finite-time stability and asymptotic stability conditions of FQMNNs with order \(1<\beta <2\) was established with the aid of Mittag-Leffler function and the generalized Gronwall-inequality. Finally, we provide two numerical simulations to illustrate the correctness of the proposed main consequences.
References
Song C, Fei S, Cao J, Huang C (2019) Robust synchronization of fractional-order uncertain chaotic systems based on output feedback sliding mode control. Mathematics 7(7):599. https://doi.org/10.3390/math7070599
Huang C, Zhao X, Wang X, Wang Z, Xiao M, Cao J (2019) Disparate delays-induced bifurcations in a fractional-order neural network. J Franklin Inst 356(5):2825–2846
Huang C, Cao J (2018) Impact of leakage delay on bifurcation in high-order fractional BAM neural networks. Neural Netw 98:223–235
Rajchakit G, Pratap A, Raja R, Cao J, Alzabut J, Huang C (2019) Hybrid control scheme for projective lag synchronization of Riemann–Liouville sense fractional order memristive BAM neural networks with mixed delays. Mathematics 7(8):759. https://doi.org/10.3390/math7080759
Chen B, Chen J (2016) Global \(O(t^{-\alpha })\) stability and global asymptotical periodicity for a non-autonomous fractional-order neural networks with time-varying delays. Neural Netw 73:47–57
Huang C, Long X, Huang L, Fu S (2019) Stability of almost periodic Nicholson’s blowflies model involving patch structure and mortality terms. Can Math Bull. https://doi.org/10.4153/S0008439519000511
Long X, Gong S (2019) New results on stability of Nicholson’s blowflies equation with multiple pairs of time-varying delays. Appl Math Lett. https://doi.org/10.1016/j.aml.2019.106027
Liu Y, Tong L, Lou J, Lu J, Cao J (2017) Sampled-data control for the synchronization of Boolean control networks. IEEE Trans Cybern. https://doi.org/10.1109/TCYB.2017.2779781
Huang C, Qiao Y, Huang L, Agarwal RP (2018) Dynamical behaviors of a food-chain model with stage structure and time delays. Adv Differ Equ 2018:186. https://doi.org/10.1186/s13662-018-1589-8
Huang C, Zhang H, Huang L (2019) Almost periodicity analysis for a delayed Nicholson’s blowflies model with nonlinear density-dependent mortality term. Commun Pure Appl Anal 18(6):3337–3349
Huang C, Zhang H, Cao J, Hu H (2019) Stability and Hopf bifurcation of a delayed prey-predator model with disease in the predator. Int J Bifurc Chaos 29(7):1950091 23 Pages
Huang C, Yang Z, Yi T, Zou X (2014) On the basins of attraction for a class of delay differential equations with non-monotone bistable nonlinearities. J Differ Equ 256(7):2101–2114
Rakkiyappan R, Sivaranjani R, Velmurugan G, Cao J (2016) Analysis of global \(O(t^{-\alpha })\) stability and global asymptotical periodicity for a class of fractional-order complex-valued neural networks with time varying delays. Neural Netw 77:51–69
Chen J, Li C, Yang X (2018) Asymptotic stability of delayed fractional-order fuzzy neural networks with impulse effects. J Franklin Inst 355(15):7595–7608
Rakkiyappan R, Velmurugan G, Cao J (2015) Stability analysis of fractional-order complex-valued neural networks with time delays. Chaos Solitons Fractals 78:297–316
Liu P, Nie X, Liang J, Cao J (2018) Multiple Mittag-Leffler stability of fractional-order competitive neural networks with Gaussian activation functions. Neural Netw 108:452–465
Yang X, Song Q, Liu Y, Zhao Z (2015) Finite-time stability analysis of fractional-order neural networks with delay. Neurocomputing 152:19–26
Wu R, Hei X, Chen L (2013) Finite-Time stability of fractional-order neural networks with delay. Commun Theor Phys 60:189–193
Chua L (1971) Memristor: the missing circuit element. IEEE Trans Circuits Theory 18:507–519
Bernard W (1960) An adaptive adaline neuron using chemical memistors, Stanford Electronics Laboratories Technical Report 1553-2
Strukov D, Snider G, Stewart D, Williams R (2008) The missing memristor found. Nature 453:80–83
Kim H, Sah M, Yang C, Roska T, Chua L (2012) Memristor bridge synapses. Proc IEEE 100(6):2061–2070. https://doi.org/10.1109/JPROC.2011.2166749 [6074916]
Miller K, Nalwa K, Bergerud A, Neihart N, Chaudhary S (2010) Memristive behavior in thin anodic titania. IEEE Electron Device Lett 31(7):737–739
Sun J, Shen Y, Yin Q, Xu C (2012) Compound synchronization of four memristor chaotic oscillator systems and secure communication. Chaos 22(4):1–10
Corinto F, Ascoli A, Gilli M (2011) Nonlinear dynamics of memristor oscillators. IEEE Trans Circuits Syst I 58(6):1323–1336
Wang L, Song Q, Liu R, Zhao Z, Alsaadi F (2017) Finite-time stability analysis of fractional-order complex-valued memristor-based neural networks with both leakage and time-varying delays. Neurocomputing 245:86–101
Rakkiyappan R, Velmurugan G, Cao J (2014) Finite-time stability analysis of fractional-order complex-valued memristor-based neural networks with time delays. Nonlinear Dyn 78:2823–2836
Sudbery A (1979) Quaternionic analysis, Math. Proc. Camb. Phil. Soc
Hu J, Zeng C, Tan J (2017) Boundedness and periodicity for linear threshold discrete-time quaternion-valued neural network with time-delays. Neurocomputing 267:417–425
Tu Z, Cao J, Ahmed A, Hayat T (2017) Global dissipativity analysis for delayed quaternion-valued neural networks. Neural Netw 89:97–104
Tu Z, Zhao Y, Ding N, Feng N, Wei Z (2019) Stability analysis of quaternion-valued neural networks with both discrete and distributed delays. Appl Math Comput 343:342–353
Tan M, Liu Y, Xu D (2019) Multistability analysis of delayed quaternion-valued neural networks with nonmonotonic piecewise nonlinear activation functions. Appl Math Comput 341:229–255
You X, Song Q, Liang J, Liu Y, Alsaadi F (2018) Global \(\mu \)-stability of quaternion-valued neural networks with mixed time-varying delays. Neurocomputing 290:12–25
Samidurai R, Sriraman R, Zhu S (2019) Leakage delay-dependent stability analysis for complex-valued neural networks with discrete and distributed time-varying delays. Neurocomputing 338:262–273
Samidurai R, Sriraman R, Cao J, Tu Z (2018) Effects of leakage delay on global asymptotic stability of complex-valued neural networks with interval time-varying delays via new complex-valued Jensens inequality. Int J Adapt Control Signal Process 32:1294–1312
Tan M, Liu Y, Xu D (2019) Multistability analysis of delayed quaternion valued neural networks with nonmonotonic piecewise nonlinear activation functions. Appl Math Comput 341:229–255
Zhou Y, Li C, Chen L, Huang T (2018) Global exponential stability of memristive Cohen–Grossberg neural networks with mixed delays and impulse time window. Neurocomputing 275:2384–2391
Ning L, Cao J (2018) Global dissipativity analysis of quaternion-valued memristor-based neural networks with proportional delay. Neurocomputing 321:103–113
Liu Y, Zheng Y, Lu J, Cao J, Rutkowski L (2019) Constrained quaternion-variable convex optimization: a quaternion-valued recurrent neural network approach. IEEE Trans Neural Netw Learn Syst. https://doi.org/10.1109/TNNLS.2019.2916597
Yang X, Li C, Song Q, Chen J, Huang J (2018) Global Mittag-Leffler stability and synchronization analysis of fractional-order quaternion-valued neural networks with linear threshold neurons. Neural Netw 105:88–103
Xiao J, Zhong S (2019) Synchronization and stability of delayed fractional-order memristive quaternion-valued neural networks with parameter uncertainties. Neurocomputing 363:321–338
Li X, Ding Y (2017) Razumikhin-type theorems for time-delay systems with persistent impulses. Syst Control Lett 107:22–27
Yang X, Li X, Xi Q, Duan P (2018) Review of stability and stabilization for impulsive delayed systems. Math Biosci Eng 15(6):1495–1515
Li X, Ho DWC, Cao J (2019) Finite-time stability and settling-time estimation of nonlinear impulsive systems. Automatica 99:361–368
Wu H, Zhang X, Xue S, Wang L, Wang Y (2016) LMI conditions to global Mittag-Leffler stability of fractional-order neural networks with impulses. Neurocomputing 193:148–154
Zhang X, Niu P, Ma Y, Wei Y, Li Guoqiang (2017) Global Mittag-Leffler stability analysis of fractional-order impulsive neural networks with one-side Lipschitz condition. Neural Netw 94:67–75
Wang L, Song Q, Liu Y, Zhao Z, Alsaadi F (2017) Global asymptotic stability of impulsive fractional-order complex-valued neural networks with time delay. Neurocomputing 243:49–59
Podlubny I (1999) Fractional differential equations. Academic Press, San Diego
Ye H, Gao J, Ding Y (2007) A generalized Gronwall inequality and its application to a fractional differential equation. J Math Anal Appl 328(2):1075–1081
Zhang S, Yu Y, Wang H (2015) Mittag-Leffler stability of fractional-order Hopfield neural networks. Nonlinear Anal Hybrid Syst 16:104–121
De la Sen M (2011) About robust stability of Caputo linear fractional dynamic systems with time delays through fixed point theory, Fixed Point Theory and Applications (1), Article ID: 867932, 1-19
Chen B, Chen J (2015) Global asymptotical \(\omega \)-periodicity of a fractional-order non-autonomous neural networks. Neural Netw 68:78–88
Filippov AF (1988) Differential equations with discontinuous right-hand sides. Kluwer, Dordrecht
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Pratap, A., Raja, R., Alzabut, J. et al. Finite-Time Mittag-Leffler Stability of Fractional-Order Quaternion-Valued Memristive Neural Networks with Impulses. Neural Process Lett 51, 1485–1526 (2020). https://doi.org/10.1007/s11063-019-10154-1
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11063-019-10154-1