1 Introduction

Cosmologies with anisotropic models are being investigated in recent times. The spatially homogeneous and anisotropic Bianchi type models are more interesting because of their ability to explain the cosmic evolution in early phase of universe. More ever, Bianchi type models have simple mathematical forms. Quadrupole anisotropy is an intrinsic property of the background radiation. The observed quadrupole amplitude has a lower value compared to that predicted from the best fit ΛCDM model. As supported from experimental data from Wilkinson Microwave Anisotropy Probe (WMAP), the low value of the quadrupole anisotropy seems to be inescapable [1]. Anisotropic models can also account for certain large scale anomalies of the isotropic standard cosmologies such as detection of large- scale velocity flows significantly larger than those predicted in standard cosmology, a statistically significant alignment and planarity of the CMB quadrupole and octupole modes and the observed large scale alignment in quasar polarization vectors [2]. However, it should be noted here that there still persists uncertainty on these large angle anisotropies and they remain as open problems.

Newtonian gravitational constant G appears as a coupling constant between the matter field and geometry of the universe in Einstein’s general theory of relativity and it is usually considered as a constant. However, recent experimental as well as theoretical calculations offer that it should vary with time. Dirac first raised the interesting possibility that in a changing universe the constant may also change [3, 4] with time. After Dirac’s proposal, there have been many attempts to modify Einstein’s general relativity with the incorporation of time varying G. Empirically, local constraints on the rate of variation of G can be derived from Lunar Laser Ranging. From the ranging data to the Viking landers on Mars, the simple time variation in the effective Newtonian gravitational constant \(\frac{\dot{G}}{G}\) could be limited to (0.2±0.4)×10−11 yr−1 [5]. However, the quoted errors in this estimation are much large, which may have stemmed from the lack of knowledge of the masses of asteroids. There are also measurements which offer a variation of such fundamental constants. The experimental range of variation of \(\frac{\dot{G}}{G}\) seems to be \(10^{-12}\mbox{ yr}^{-1} < \frac{\dot{G}}{G} < 10^{-10}\mbox{ yr}^{-1}\) [6].

Classical cosmological models with cosmological constant gained much importance in recent times because of its implications in explaining the present accelerated phase of expansion of universe. A non-zero positive cosmological constant Λ∼2h 2×10−56 cm−2 is required to account for the observations [7–10]. The old and unsettled cosmological constant problem [11–14], centers around the large difference in the theoretical prediction of Λ from quantum theories associating the vacuum state energy density and its observational values. The observed small but non-zero positive cosmological constant requires a fine tuning problem in physics. In order to get an adhoc solution to the problem, an evolving cosmological constant has been considered by many authors [15–18]. In view of this, cosmological models with variable Newtonian gravitational constant G and cosmological constant Λ have generated a lot of interest in recent times. The time variation of G is intimately related to the time variation of the cosmological constant Λ [19, 20]. If one considers a time varying cosmological constant term, then it is necessary that one should consider a time varying gravitational constant term in the usual Einstein field equation. This approach is interesting in the sense that it provides a link in the variation of the Newtonian gravitational constant with the cosmological constant while leaving the form of Einstein field equations formally unchanged. Many authors, Arbab [21–23], Singh et al. [24], Tiwari [25], Mukhopadhyay et al. [26], Ram and Verma [27], Singh [28], Yadav [29], Sharif and Khanum [30], Pradhan et al. [31–34], Singh et al. [35] have discussed G varying cosmologies.

In the present work, we have investigated LRS Bianchi type-I bulk viscous anisotropic cosmological model in the framework of Einstein’s relativity with variable gravitational and cosmological constants. In order to get viable models, we have assumed that the shear scalar is directly proportional to the scalar expansion and the contribution of the bulk viscosity to the total pressure is proportional to the rest energy density. Such a barotropic bulk viscous pressure is required to explain the accelerated expansion of the universe [36, 37]. The motivation behind the work is to investigate the dynamics of the anisotropic model pertaining to the time variation of Newtonian gravitational constant keeping in view the limits of the Supernova constraints.

The organization of the paper is as follows. In Sect. 2, the basic equations for the anisotropic LRSBI model have been derived assuming the shear scalar to be directly proportional to the scalar expansion. The dynamics of the universe are discussed for the imperfect bulk viscous cosmic fluid with time varying gravitational constant and a rolling down dark energy component in Sect. 3. The statefinder parameters which provide a better idea about the geometrical evolution of universe are derived in Sect. 4. In Sect. 5, the supernova constraints concerning the luminosity distance along with look back time have been discussed. At the end, the results of the present work are summarized in Sect. 6.

2 The Basic Equations for Anisotropic Universe

The classical accelerated phase of expansion of the universe is modeled through a cosmological constant in Einstein’s field equation

$$ R_{ij} - \frac{1}{2} g_{ij} R = - 8 \pi G ( t ) T_{ij} + \varLambda( t ) g_{ij}, $$
(1)

where the symbols have their usual meaning. The universe is believed to have a perfect fluid distribution with dissipative phenomena coming from bulk viscosity. The presence of bulk viscosity in the cosmic fluid has already been recognized in connection with the observed accelerated expansion [36, 37]. In the field equation (1), the unit is chosen in such a manner that the speed of light in vacuum is unity.

In commoving coordinates, the energy-momentum tensor is given by

$$ T_{ij} =(\rho+ \overline{p} )u^{i} u_{j} + \overline{p} g_{ij}, $$
(2)

where u i are the four velocity vectors defined as \(u^{i} = \delta_{4}^{i}\) and they satisfy the relation u i u j =−1. ρ is the proper rest energy density and \(\overline{p}\) is the total effective pressure which includes the proper pressure p and contribution of bulk viscosity to pressure such that \(\overline{p} =p-\xi(t) u_{;l}^{l}\), ξ(t) being the time dependent bulk viscous coefficient.

Anisotropic LRSBI universe is modeled through the metric

$$ d s^{2} =-d t^{2} + A^{2} \bigl(d x^{2} +d y^{2} \bigr)+ B^{2} \, d z^{2}, $$
(3)

where the metric potentials A and B are considered as the functions of cosmic time only. The plane symmetric metric is considered in such a way that the axis of symmetry lies along z-direction. The expansion scalar \(\theta= u_{;l}^{l}\) for this metric can be expressed as

$$ \theta=2 \frac{\dot{A}}{A} + \frac{\dot{B}}{B}. $$
(4)

The overhead dots on the metric potentials represent the ordinary time derivatives. Defining the directional Hubble parameters as \(H_{1} = \frac{\dot{A}}{A}\) and \(H_{2} = \frac{\dot{B}}{B}\), the mean Hubble parameter can be written as \(H= \frac{1}{3} ( 2H_{1} + H_{2} )\) and \(\theta= u_{;l}^{l} =3H\). The shear scalar σ for the metric (3) is defined as

$$ \sigma^{2} = \frac{1}{2} \biggl[ \sum _{i} H_{i}^{2} - \frac{1}{3} \theta^{2} \biggr] = \frac{1}{3} ( H_{1} - H_{2} )^{2}. $$
(5)

The shear scalar may be taken to be proportional to the expansion scalar which envisages a linear relationship between the Hubble parameters H 1 and H 2,

$$ H_{2} =k H_{1} $$
(6)

which leads to a relation between the metric potentials A and B as

$$ B= A^{k}, $$
(7)

where the constant k is assumed to be positive and it takes care of the anisotropic nature of the model. If k=1, the model is isotropic and anisotropic otherwise. The mean Hubble parameter can now be expressed as \(H= \frac{1}{3} ( k+2 ) H_{1}\). Consequently, the shear scalar can be expressed in terms of the Hubble parameter H as

$$ \sigma^{2} =3 \biggl( \frac{k-1}{k+2} \biggr)^{2} H^{2}. $$
(8)

The field equation (1), for the metric (3) now assumes the explicit forms

$$ \dot{H} +3 H^{2} =0, $$
(9)

and

$$ 9(2k+1) H^{2} = (k+2)^{2} [ 8\pi G\rho+ \varLambda ]. $$
(10)

Equation (9) yields,

$$ H= \frac{1}{3t+ k_{1}}, $$
(11)

where k 1 is an integration constant. Consequently, the radius scale factor of the universe can be

$$ a= a_{1} \biggl( \frac{3}{k_{1}} t+1 \biggr)^{\frac{1}{3}}. $$
(12)

a 1 is a positive constant. The metric potentials can be expressed as \(A= A_{0} ( 3t+ k_{1} )^{\frac{1}{k+2}}\) and \(B= B_{0} ( 3t+ k_{1} )^{\frac{k}{k+2}}\) where A 0 and B 0 are positive integration constants. The deceleration parameter \(q=- \frac{\ddot{a}}{a H^{2}} =-1- \frac{\dot{H}}{H^{2}} =2\) comes out to be a positive constant implying that the universe is decelerating in the present time. At the present time, t=t 0, the Hubble parameter H=H 0 and the radius scale factor a=a 0. In terms of the respective values at the present time, the Hubble parameter and the radius scale factor can be expressed as

$$ H ( t ) = \frac{H_{0}}{3 H_{0} ( t- t_{0} ) +1} $$
(13)

and

$$ a ( t ) = a_{0} \bigl[ 3 H_{0} ( t- t_{0} ) +1 \bigr]^{1/3}. $$
(14)

The vanishing covariant divergence of the Einstein tensor \(R_{ij} - \frac{1}{2} g_{ij} R\) yields [38–40],

$$ \dot{\rho} +3H ( \rho+ \overline{p} ) + \biggl( \frac{\dot{G}}{G} \rho+ \frac{\dot{\varLambda}}{8\pi G} \biggr) =0. $$
(15)

Assuming that the total matter content of the universe is conserved, the energy density of the matter obeys the usual conservation law \(T_{;j}^{ij} =0\). Therefore Eq. (15) splits into two independent equations,

$$ \dot{\rho} +3H ( \rho+ \overline{p} ) =0 $$
(16)

and

$$ \frac{\dot{G}}{G} =- \frac{\dot{\varLambda}}{8\pi G\rho}. $$
(17)

It is evident from Eq. (17) that, the time variation of Newtonian Gravitational constant is linked with the time dependence of cosmological constant. A rolling down cosmological constant in classical cosmological models is considered to account for the recent observations envisaging an accelerated expansion of the universe which necessitates the use of a time varying Newtonian Gravitational constant in the field equations.

3 Bulk Viscous Model

A barotropic bulk viscous pressure defined through ξθ=ζρ with ζ≥0 is required to explain the observed accelerated expansion of the universe [36, 37]. For a barotropic cosmic fluid, the proper pressure is related to the energy density as p=ω 0 ρ, 0≤ω 0≤1. The combined effect of the proper pressure and the bulk viscous barotropic pressure leads to a total negative effective pressure \(\overline{p} =\omega\rho\), where the new equation of state parameter ω is related to the old one ω 0 as ω 0−ζ. The antigravity effect of the total negative effective pressure provides the necessary acceleration. The observational limits on the equation of state parameter ω from SN Ia data are −1.67<ω<−0.62 [41] and that from a combination of SN Ia data with CMB anisotropy and galaxy clustering statistics are −1.3<ω<−0.79 [42]. Inflation at an early stage scales the parameter ω, which combined with the above data and dark energy constraint ω>−1 suggests a physical condition [43].

For a barotropic bulk viscous cosmic fluid, (16) reduces to

$$ \frac{\dot{\rho}}{\rho} =-3 ( \omega+1 ) H $$
(18)

which on integration yields,

$$ \rho= \frac{\rho_{1}}{(3t+ k_{1} )^{(\omega+1)}}, $$
(19)

where ρ 1 is an integration constant.

The proper pressure becomes

$$ p= \frac{\omega_{0} \rho_{1}}{(3t+ k_{1} )^{(\omega+1)}}. $$
(20)

In the present epoch, t=t 0, the rest energy density of the universe becomes ρ=ρ c , and (19) reduces to

$$ \rho= \rho_{c} \biggl( \frac{H}{H_{0}} \biggr)^{(\omega+1)}. $$
(21)

The time variation of the rest energy density and the proper pressure depends on the value of the equation of state parameter ω. If ω<−1, then the rest energy density and proper pressure increase with the growth of time and the other way around if ω>−1. Since the rest energy density of the universe should decrease with the growth of cosmic time, the reasonable limit for the equation of state parameter should be ω>−1. The scalar expansion, the shear scalar and the coefficient of bulk viscosity can be expressed as

$$ \theta= \frac{3}{3t+ k_{1}}, $$
(22)
$$ \sigma^{2} =3 \biggl( \frac{k-1}{k+2} \biggr)^{2} \frac{1}{(3t+ k_{1} )^{2}} $$
(23)

and

$$ \xi= \biggl( \frac{\zeta\rho_{0}}{3} \biggr) \frac{1}{(3t+ k_{1} )^{\omega}}. $$
(24)

In terms of the Hubble parameter in the present time these quantities can be expresses as

$$ \theta= \frac{\theta_{0}}{ [ 3 H_{0} ( t- t_{0} ) +1 ]}, $$
(25)
$$ \sigma^{2} = \frac{\sigma_{0}^{2}}{ [ 3 H_{0} ( t- t_{0} ) +1 ]^{2}}, $$
(26)

and

$$ \xi= \frac{\xi_{0}}{ [ 3 H_{0} ( t- t_{0} ) +1 ]^{\omega}}. $$
(27)

In the above equations (25)–(27), θ 0, σ 0 and ξ 0 represent the values at the present time. In the beginning of universe, i.e. at t→0, the scalar expansion and the shear scalar assume large constant values, where as with the growth of cosmic time they decrease to null values as t→∞. Since \(\lim_{t\rightarrow\infty} \frac{\sigma}{\theta} \neq0\), the anisotropy of the model is maintained throughout. The coefficient of viscosity ξ evolves with time and its evolution depends on ω. For ω>0, it decreases with time whereas it increases for ω<0. For ω>0 and t<t 0, ξ>ξ 0 and for the time afterwards the present epoch ξ<ξ 0. The time dependence of the bulk viscosity becomes just the opposite if ω<0.

The differentiation of (10) with respect to time along with (11) and (17), results into

$$ G= \frac{18(2k+1)}{8\pi\rho_{0} (\omega+1) (k+2)^{2}} \biggl( \frac{3 H_{0} ( t- t_{0} ) +1}{H_{0}} \biggr)^{(\omega-1)} $$
(28)

and consequently,

$$ \varLambda=9 \frac{2k+1}{(k+2)^{2}} \biggl( \frac{\omega-1}{\omega+1} \biggr) \biggl( \frac{H_{0}}{3 H_{0} ( t- t_{0} ) +1} \biggr)^{2}. $$
(29)

If the Newtonian gravitational constant and the cosmological constant in the present time are represented by G 0 and Λ 0 respectively then (28) and (29) reduce to

$$ G= G_{0} \bigl[ 3 H_{0} ( t- t_{0} ) +1 \bigr]^{(\omega-1)} $$
(30)

and

$$ \varLambda= \frac{\varLambda_{0}}{ [ 3 H_{0} ( t- t_{0} ) +1 ]^{2}}. $$
(31)

The cosmological constant can also be expressed in terms of the Newtonian gravitational constant as

$$ \varLambda= \varLambda_{0} \biggl( \frac{G}{G_{0}} \biggr)^{- \frac{2}{\omega-1}}. $$
(32)

The Newtonian gravitational constant G varies with time. G increases with the increase in time if ω>1 and G decreases with the increase in time if ω<1. If ω=1, G becomes a pure constant and equals to its present value. The cosmological constant Λ evolves from a large value at the beginning of the universe to a very small value at a large cosmic time. It is interesting to note that the time variation of the cosmological constant does not depend on the equation of state parameter whereas the time variation of the G depends on it. Present day observation requires a small but positive cosmological constant in classical cosmological models to account for the observed accelerated expansion of the universe. It is evident from (29) that, \(\varLambda_{0} = 9 \frac{2k+1}{(k+2)^{2}} ( \frac{\omega-1}{\omega+1} ) ( H_{0} )^{2}\) can be positive only if ω>1 or ω<−1. However, a decaying rest energy density favours ω>−1. The consideration of positive Λ 0 along with the fact that the rest energy density should decrease with time, leads to an increasing G during the cosmic evolution. It is interesting to note from the outcome of this model that, at the beginning of the universe, G has a small value which depends on the value of the effective equation of state parameter ω and the anisotropic parameter k. The sign of G is positive for ω>0 and is negative for ω<−1. Based upon the physical constraints, it can be inferred that G comes out to be positive for the present model. As is evident from the field equation (1), the vanishing G implies that, at the beginning of the universe, the dominant role for energy is played by the cosmological constant. Equation (32) shows that the increment or decrement in G is decided by the variation in the cosmological constant and both the variation is governed by the value of the effective equation of state parameter. In the absence of bulk viscosity ω=ω 0, 0≤ω 0≤1 and hence the Newtonian gravitational constant G decrease with time. As is evident from (29), the cosmological constant becomes negative or zero in the absence of bulk viscosity. Since the observational facts favour a positive cosmological constant at the present epoch, inclusion of bulk viscosity becomes a necessity in the present model.

The relationship between the growth of the scale factor and the variation of the gravitational constant can be determined from the relation

$$ G= G_{0} \biggl( \frac{a}{a_{0}} \biggr)^{3(\omega-1)}. $$
(33)

The Newtonian gravitational constant depends on the growth of the radius scale factor. With the growth of the radius scale factor, whether G will increase or decrease that is decided by the equation of state parameter. For an increasing G, ω>1 and for a decreasing G, ω<1. From (28), it can be inferred that \(\frac{\dot{G}}{ G} = \frac{3 ( \omega-1 ) H_{0}}{ [ 3 H_{0} ( t- t_{0} ) +1 ]}\) which comes out to be a positive quantity. The relationship between the cosmological constant and the mean Hubble parameter becomes Λ∝H 2 which is equivalent to \(\varLambda \propto \rho^{ ( \frac{2}{\omega+1} )}\). For a Machian cosmological solution, the quantity Gρ should satisfy the condition Gρ∝H 2. The LRSBI model satisfies this condition for Machian solution.

4 State Finder Parameters for Varying G

The state finder parameters pair {r,s} has great geometrical significance. The statefinder pair is a geometrical diagnosis in the sense that it depends upon the expansion factor and hence upon the metric describing space-time. In the expansion history of the universe if the deceleration parameter q is negative, there is cosmic acceleration and cosmic deceleration for a positive value of q. In most of the situation it is very difficult to extract information on the dynamical evolution of the equation of state parameter from q. In order to get information about the dynamical properties of the equation of state parameter, higher order derivatives of the scale factor are required. These statefinder parameters involving the second and third time derivatives of radius scale factor of the universe are used in the holographic dark energy models to differentiate between different models. These parameters are defined as

$$ r= \frac{\dddot{a}}{a H^{3}}, $$
(34)

and

$$ s= \frac{r- \varOmega}{3(q- \frac{\varOmega}{2} )}, $$
(35)

where,

$$\varOmega= \varOmega_{m} + \varOmega_{\varLambda} = \frac{3(2k+1)}{(k+2)^{2}}. $$

The state finder parameter r can also be expressed in terms of the deceleration parameter q as \(r=2 q^{2} +q- \frac{\dot{q}}{H}\) and is also called cosmic jerk. Since for the present model q=2, i.e. \(\dot{q} =0\), the r−q relation is described through r=2q 2+q. For the present model, the state finder parameters can be expressed as [44]

$$ r=10 $$
(36)

and

$$ s= \frac{2 0(k+2)^{2} -6(2k+1)}{12 (k+2)^{2} -9(2k+1)} $$
(37)

so that \(s= \frac{1}{10} ( \frac{2 0(k+2)^{2} -6(2k+1)}{12 (k+2)^{2} -9(2k+1)} ) r\).

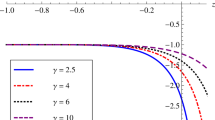
The trajectories in the s–r plane for different cosmological models have different behaviours. For a given value of the anisotropic parameter, the plot of s∼r behaves like a straight line. If the values of the pair {r,s} in the present time can be extracted from precise observational data in model independent manner, various cosmological models can then be well tested.

5 Supernovae Constraints: Luminosity Distance

The luminosity distance is also considered as a better tool to determine the equation of state. For isotropic models the luminosity distance is defined as

$$ d_{L} =d(1+z), $$
(38)

where the redshift satisfies \(1+z= \frac{a_{0}}{a}\), a 0 is the value of the radius scale factor in the present epoch and d is the proper distance. The proper distance between a source and observer is calculated from the fact that, if a photon emitted by a source with coordinate d at time t and received by an observer located at the origin at a time t 0, then

$$ d= \int_{a}^{a_{0}} \frac{d a}{a \dot{a}} = a_{0} \int_{t}^{t_{0}} \frac{dt}{a}. $$
(39)

In case of isotropic models, two galaxies with same redshift appear to be at the same luminosity distance from the observer. But in case of anisotropic metric as in (3), we can obtain different distances for a given redshift. In other words the brightness depends on the distance and the angle of observation [45].

For an anisotropic metric with symmetry axis lying along z-axis the luminosity distance d L of a source at redshift, seen along a direction \(\hat{p} ( |\hat{p}| = a_{0} )\) is given by [2, 46, 47]

$$ d_{L} = ( z, \hat{p} ) =(1+z) \int_{t}^{t_{0}} \frac{dt}{ ( \sum_{i} A_{i}^{2} \hat{p_{i}}^{2} )^{1/2}}, $$
(40)

where A 1=A 2=A and A 3=B and

$$ 1+z= \biggl( \sum_{i} \frac{\hat{p}_{i}^{2}}{A_{i}^{2}} \biggr)^{1/2}. $$
(41)

It may be noted here that, for small observational angles these expressions reduce to the usual isotropic relations. In the present work, we restrict ourselves to the Supernovae present within small observational angles.

For the present model with the radius scale factor given in Eq. (14) the proper distance becomes

$$ d= \frac{1}{2 H_{0}} \biggl[ 1- \biggl( \frac{H_{0}}{H} \biggr)^{\frac{2}{3}} \biggr] $$
(42)

and in terms of the redshift

$$ d= \frac{1}{2 H_{0}} \bigl[ 1- ( 1+z )^{-2} \bigr]. $$
(43)

For early universe z→∞, the proper distance \(d\rightarrow \frac{1}{2 H_{0}}\) and at late times z→−1, the proper distance approaches a large value behaving like \(d\sim\frac{ ( 1+z )^{-2}}{2 H_{0}}\).

The Luminosity distance can now be expressed as

$$ d_{L} = \frac{1}{2 H_{0}} \bigl[ (1+z)- ( 1+z )^{-1} \bigr]. $$
(44)

For early universe with inflationary epoch, z→∞, the Luminosity distance d L →∞ and at late times z→−1, it behaves like \(d_{L} \sim- \frac{ ( 1+z )^{-1}}{2 H_{0}}\).

If a photon is emitted by a source at the instant t and received at time t 0, then the photon travel time or the look back time t−t 0 is defined as

$$ t- t_{0} =- \int_{0}^{z} \frac{dz}{H ( z ) (1+z)}. $$
(45)

From Eqs. (13) and (14), we get

$$ t- t_{0} = \frac{1}{3 H_{0}} \biggl[ \frac{H_{0}}{H} -1 \biggr], $$
(46)

which can be expressed in terms of the redshift as

$$ t- t_{0} = \frac{1}{3 H_{0}} \bigl[ ( 1+z )^{-3} -1 \bigr]. $$
(47)

At the early inflationary epoch, the look back time becomes \(- \frac{1}{3 H_{0}}\) and at late time of evolution \(t- t_{0} \sim\frac{ ( 1+z )^{-3}}{3 H_{0}}\).

Recent observations of Supernova Ia provide information regarding the second and third derivatives of the luminosity distance, d 2 and d 3 respectively, with respect to the redshift. Particularly the third derivative d 3 is more important as it contains information about the curvature of the universe and the state finder parameter r. For a flat universe, these parameters are expressed as

$$ d_{2} = \frac{1}{2} (1- q_{0} ), $$
(48)
$$ d_{3} = \frac{1}{6} \bigl(3 q_{0}^{2} + q_{0} - r_{0} -1\bigr), $$
(49)

where q 0 and r 0 represent the respective values at the present epoch. Since q=2 and r=10, d 2 and d 3 assume the values −0.5 and 2.25 respectively.

6 Summary and Conclusion

We have investigated bulk viscous anisotropic LRSBI cosmological model in the framework of General Relativity with variable gravitational and cosmological constants. The time varying nature of the cosmological constant is very much linked to the time variation of the gravitational constant. In order to get viable models, we have assumed that the shear scalar is proportional to the scalar expansion and the contribution of bulk viscosity to pressure is proportional to the rest energy density. The time varying nature of Λ and G depends on the bulk viscosity and the anisotropy of the universe. The extracted time variation of the G satisfies the Machian cosmological solution. In the absence of bulk viscosity, the gravitational constant decreases with time and increases otherwise. In the bulk viscous model, the cosmological constant decays from a very large value at the beginning of the universe to a small positive value. However in the absence of bulk viscosity, the cosmological constant assumes negative value which necessitates the inclusion of bulk viscosity in the present model. The variation of G depends on the variation of the cosmological constant and the radius scale factor. The equation of state parameter and hence the bulk viscosity affects the time variation of the properties of the universe. We have calculated the state finder parameter or jerk parameter for the model discussed to get idea about the dynamics of the universe. Our calculations of the Supernova constraints concerning the luminosity distance provide reasonable results.