1 Introduction

Two events predicted by the conventional uncoupled thermoelasticity model do not correspond to the physical facts. First, there are no elastic components in this theory's heat conduction equation; second, the heat equation is of the parabolic type, implying that heat waves would propagate at limitless velocities. To solve the first weakness, Biot [1] proposed the idea of coupled thermoelasticity. This theory's governing equations are associated, which solves the classical theory's first contradiction. The heat equation for the coupled model is likewise parabolic; therefore, both theories suffer from the second flaw. Several researchers have proposed new formulations of thermoelasticity to replace the coupled Biot theory [1]. Since the heat equations associated with these models are hyperbolic, they immediately avoid the challenge of infinite propagation velocities found in both uncoupled and coupled thermoelastic models.

Using the Maxwell–Cattaneo law of heat conduction, Lord and Shulman [2] developed the theory of generalized thermoelasticity with one relaxation time for isotropic materials (labeled as the LS theory). Because this theory's heat equation is of the wave type, it assures that heat and elastic waves propagate at limited speeds. The rest of the governing equations for this theory, such as the equations of motion and constitutive relations, are identical to those for the coupled and uncoupled models. By proposing a novel thermoelastic inequality, Green and Lindsay [3] established the theory of thermoelasticity with two relaxation durations (named the GL model). Not only does this theory change the heat equation, but it also changes the equations of the associated theory. When the medium under examination has a center of symmetry, the classical Fourier equation of heat conduction is not disturbed.

Green and Naghdi [4,5,6] made sufficient changes to the constitutive equations to allow consideration of a noticeably larger class of heat flow problems known as GN-I, GN-II, and GN-III. The independent constitutive variables in GN models contain a phrase termed “thermal displacement gradient”. When the different theories are linearized, GN-I's heat transport equation is identical to the classical heat equation, but GN-II and GN-III both allow for the transmission of finite-speed thermal signals [6]. The presence of a thermal damping component in the GN-III theory allows heat energy to be dissipated. Additional contributions to this theory can be detected in [7,8,9,10,11,12].

The Moore-Gibson-Thompson (MGT) equation has grown more important in recent years, as evidenced by numerous academic articles devoted to its study and interpretation. The theory was founded on a third-order differential equation, which is crucial to many fluid dynamics [13]. Quintanilla [14, 15] is constructing a novel heat conduction model under the MGT equation. Abouelregal et al. [16,17,18] prepared the proposed modified heat equation after adding the relaxation parameter in the GN-III model and using the energy equation. The number of articles on this theory has been greatly increased since the advent of the MGT equation [19,20,21,22].

Some materials, such as semiconductors, offer a variety of physical characteristics that are useful during research. According to the principle of thermoelasticity, semiconductor materials may only be classed as elastic materials. The relevance of semiconductors in current technology was recently highlighted when they were utilized to produce electrical energy from sunlight while also being subjected to laser pulses [23]. Semiconductor materials are utilized as nanomaterials in various fields of mechanical and electrical engineering, and they have a variety of uses in modern industry, including transistors, screens, and solar cells. The photothermal theory has recently been applied to semiconductor media to produce sustainable energy technologies. Several mathematical-physical models were studied to characterize the overlap between the photothermal equations and the thermoelasticity equations. Gordon et al. [24] first introduced electronic deformations to photothermal spectroscopy. While using a laser source, photoacoustic spectroscopy is utilized in the context of sensitive analytical procedures to measure the velocity of sound of some semiconductor materials [25]. Many applications in current engineering industries employ wave propagation during electro-deformations of semiconductor medium in photothermal processing techniques [26,27,28,29,30,31,32,33,34].

The sample is regularly elastically and electro-deformed by the photo-excited carriers. The mechanism for electronic deformation is founded on the fact that photogenerated plasma in the semiconductor produces crystal lattice deformation, i.e., deformation of the conductive potential and valence strips in the semiconductor. This can result in local stress in the sample via photo-stimulated carriers. This strain in turn can create plasma waves in the semiconductor by regular elastic local similar to thermal wave production [35].

The damage caused by laser use of optical materials is a distinct area. For more than 50 years, several publications have attempted to address the topic from a theoretical and experimental standpoint. Because pulsed laser technologies are widely used in material processing and non-destructive detection and characterization, the excitation of thermoelastic waves by a pulsed laser in solids is of significant interest. When a solid absorbs a laser pulse, it creates a localized temperature rise, which induces thermal expansion and forms a thermoelastic wave in the material. Two influences become significant in ultra-short pulsed laser heating [36]. A high-powered focused laser beam may heat materials to thousands of degrees Kelvin. The temperature of continuous-wave laser heating may be monitored remotely with great precision by fitting the spectrum irradiance to a blackbody curve. Heating using a pulsed laser offers several benefits, but the temperature increases and falls in nanoseconds, necessitating quick electronics and time-consuming methods to estimate the temperature [37].

Metal softening and/or hardness, annealing of crystalline or polycrystalline materials, diffusion of dopants in semiconductors, synthesis of compounds and thin films, polymerization of polymers, and other applications of laser heating are all possible. Thermally triggered processes, bulk diffusion, and phase transitions have all been aided by laser annealing. Laser heating has the benefit of being both spatially and temporally localized. Additionally, by establishing thermal gradients, sample temperatures considerably above the sample chamber's capacity can be obtained, such as heated samples in diamond anvil cells [38, 39].

Laser heating has a number of benefits over traditional techniques, including accuracy, local therapy, and low cost. Absorption occurs when a high-intensity laser interacts with a solid surface. As a result, the substrate material gains internal energy, and the irradiated zone releases heat. Since the process is often rapid, temperature gradients in the irradiated zone remain considerable. As a result, there is a lot of thermal strain and thermally generated tension in this area. Furthermore, from the standpoint of application, the end result is critical in the laser treatment procedure. High stress levels in the irradiated zone may cause the surface to fail due to stress-induced cracking. As a result, caution must be exercised during the laser therapy procedure [40].

The mechanical, electric, and thermal properties of semiconductor materials vary with changes in temperature. When a temperature gradient due to the absorption of light occurs in semiconductor elastic materials, it causes an electric potential difference between endpoints in the semiconductor. Several authors have analyzed the uncoupled and coupled systems of plasma, thermodynamic, and elastic equations as well as the different effects of thermal as well as electronic deformation in semiconductors using classical models. According to a literature review, there is no work examining the photothermal interactions of semiconductor cylinders subjected to ultrashort pulsed laser heating and photogenerated plasmas, as well as the properties of temperature-dependent materials. The primary motivation for conducting a comprehensive examination of such issues in this study is the importance of the issue and its multiple applications. This theoretical study is also, like spectroscopic methods, one of the important experimental tools that are used to investigate the micro and macro properties of semiconductors.

When a semiconductor material sample is lit, pairs of superconductors are formed, which propagate through the material according to density gradients. Each pair carries about the same amount of energy as the material's band gap. As the surplus electron joins with the hole, the energy is deposited, causing local heating of the lattice. As a result, the temperature distribution in the sample will be calculated, which is based on the optical absorption, mass recombination, and surface features in and on the sample. The photothermal process is the development of a temperature field in a material using diffusion carriers and optically induced recombination. This study addresses the case of low temperature rises in an infinite solid cylinder and gives a formulation of the theory guiding the photothermal effect. This type of research, which is an extension of the optical diffraction approach, has significant benefits in that it directly determines both the thermal and electronic transport parameters within the semiconductor block as well as at the surface or interface. Another important benefit is the ability to physically calculate and evaluate these transport properties using a mathematical model.

The purpose of this paper is to investigate disturbances in infinite, isotropic, homogeneous thermoelastic semiconductor materials using the Moore-Gibson-Thompson (MGT) heat conduction equation. The propagation of waves in semiconductor materials has many applications in various fields of science and technology, namely atomic physics, industrial engineering, thermal power plants, submarine structures, pressure vessels, aerospace, chemical pipes, and metallurgy. The effect of thermo-electronic deformation in semiconductors has been investigated, including partially coupled plasma, thermal mechanisms, and elastic waves. We used the normal mode method analysis to solve a system of partial differential equations in this phenomenon under proper boundary conditions. Physical field quantities are introduced analytically and graphed, along with some analytical comparisons. The results obtained were compared with the results of the work of other researchers.

2 Basic equations

The generalized coupled hyperbolic plasma and generalized thermal and elastic equations, for thermoelastic semiconductors with isotropic and homogeneous electronic, thermal, and elastic properties are given by:

Equations of motion:

$$\sigma_{ij,j} + F_{i} = \varrho \ddot{u}_{i}$$
(1)

The constitutive equations:

$$\sigma_{ij} = C_{ijkl} e_{kl} - \left( {\beta_{ij} \theta + d_{nij} N} \right)$$
(2)

The strain–displacement relations:

$$e_{ij} = \frac{1}{2}\left( {u_{i,j} + u_{j,i} } \right)$$
(3)

In Eqs. (1)–(3), \(\sigma_{ij}\) are the stress components, \(\varrho\) is the density of the material, \(u_{i}\) are the displacement components, \(F_{i}\) are the body force components, and \(i,j,k = 1,2,3\), \(e_{ij}\) is the strain tensor, \(e_{kk} = e\) is the cubical dilatation, \(d_{nij} = d_{ni} \delta_{ij}\) are the difference in deformation potential of the conduction and valence bands and \(C_{ijkl}\) are the elastic constants for material, \(\beta_{ij} = \beta_{i} \delta_{ij}\) are the stress-temperature coefficients. Also, \(\theta = T - T_{0}\) denotes the thermodynamical temperature, \(T_{0}\) is the reference temperature, and \(N\) is the carrier density.

The coupled plasma-thermal-elastic wave equation can be written as [27, 41]

$$\left( {D_{Eij} N_{,j} } \right)_{,i} = \varrho \frac{\partial N}{{\partial t}} + \frac{1}{\tau }N + \kappa \theta + G$$
(4)

where \(D_{Eij}\) are the diffusion coefficients, \(\kappa\) is the thermal activation coupling parameter, \(\tau\) is the lifetime of photogenerated electron–hole pairs and \(G\) is the carrier photogeneration ‘‘source’’ term. In the case of harmonic modulation lasers, Vasilev and Sandomirskii [42] first found that the thermal activation coupling parameter \(\kappa\) is insignificant at low temperatures.

Cattaneo-Vernotte in [43,44,45] introduced a wider Fourier law by adding the thermal relaxation \(\tau_{0}\) to the vector of the heat flow \(\vec{q}\) as

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\vec{q} = - K_{ij} \vec{\nabla }\theta$$
(5)

In Eq. (1), \(K_{ij}\) refers to the thermal conductivity tensor.

The improved Fourier law based on the GN-III model can be represented as [6]

$$\vec{q} = - K_{ij} \,\vec{\nabla }\theta - K_{ij}^{*} \vec{\nabla }\vartheta$$
(6)

where the function \(\vartheta\) denotes thermal displacement which satisfies \(\dot{\vartheta } = \theta\) and the parameters \(K_{ij}^{*}\) refer to the thermal conductivity rates. The equation for the energy balance can be written as [10, 46]

$$\varrho C_{E} \frac{\partial \theta }{{\partial t}} + T_{0} \frac{\partial }{\partial t}\left( {\beta_{ij} e_{ij} } \right) = - \vec{\nabla } \cdot \vec{q} + Q$$
(7)

where \(C_{E}\) is the specific heat at constant volume, \(Q\) is the heat source.

The combination of the improved Fourier law proposed in (6) with the energy Eq. (7) has the same weakness as Fourier's normal theory, predicting that thermal waves are spreading immediately. Quintanilla [14, 15] and Abouelregal et al. [16,17,18] prepared the proposed modified heat equation after adding the relaxation parameter in the GN-III model. The modified Fourier's law would then take the following form [14, 15]

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\vec{q} = - K_{ij} \,\vec{\nabla }\theta - K_{ij}^{*} \vec{\nabla }\vartheta$$
(8)

Consider that the semiconductor elastic medium is subjected to light beams from the outside and that the excited free electrons create a carrier-free charge density with semiconductor gap energy \(E_{g}\). Because of the absorbed optical energy, there is a change in electronic deformation and elastic vibrations. Thermal-elastic-plasma waves will affect the overall form of the heat conductivity equation in this situation. The modified Fourier law for semiconductor materials with plasma effects in a generalized version can be written as follows:

$$\left( {1 + \tau_{0} \frac{\partial }{\partial t}} \right)\vec{q} = - K_{ij} \,\vec{\nabla }\theta - K_{ij}^{*} \vec{\nabla }\vartheta - \int {\frac{{E_{g} }}{\tau }\frac{\partial N}{{\partial t}}d\vec{x}}$$
(9)

The photo-excitation effect is represented by the final term in Eq. (9). When the above equation is differentiated with respect to \(\vec{x}\), the result is

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\left( {\vec{\nabla } \cdot \vec{q}} \right) = - \vec{\nabla } \cdot \left( {K_{ij} \,\vec{\nabla }\theta } \right) - \vec{\nabla } \cdot \left( {K_{ij}^{*} \vec{\nabla }\vartheta } \right) - \frac{{E_{g} }}{\tau }\frac{\partial N}{{\partial t}}$$
(10)

By substituting Eq. (10) into Eq. (7), the modified heat conduction equation with thermal memory that explains the interaction between the thermal-plasma-elastic waves may be derived as

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\left[ {\varrho C_{E} \frac{{\partial^{2} \theta }}{{\partial t^{2} }} + T_{0} \frac{{\partial^{2} }}{{\partial t^{2} }}\left( {\beta_{ij} u_{i,j} } \right) - \varrho \frac{\partial Q}{{\partial t}}} \right] = \left( {K_{ij} \dot{\theta }_{,j} } \right)_{,i} + \left( {K_{ij}^{*} \theta_{,j} } \right)_{,i} + \frac{{E_{g} }}{\tau }\frac{{\partial^{2} N}}{{\partial t^{2} }}$$
(11)

We assume that the adjacent free space is permeated by an initial magnetic field \(\vec{H}\). This generates an induced electro-field \(\vec{E}\) and induced magnetic field \(\overrightarrow { h}\) that fulfills the magnetic equations of Maxwell and is sufficient for slowly moving media:

$$\vec{J} = \nabla \times \vec{h},{ }\nabla \times \vec{E} = - \mu_{0} \frac{{\partial \vec{h}}}{\partial t},{ }\vec{E} = - \mu_{0} \left( {\frac{{\partial \vec{h}}}{\partial t} \times \vec{H}} \right),{ }\nabla \cdot \vec{h} = 0$$
(12)
$$\tau_{ij} = \mu_{0} \left[ {H_{i} h_{j} + H_{j} h_{i} - H_{k} h_{k} \delta_{ij} } \right]$$
(13)

where \(\mu_{0}\) is the magnetic permeability, \(\vec{J}\) is the current density and \(\tau_{ij}\) is the Maxwell stress tensor.

3 Formulation of the problem

In this section, wave propagation on a cylindrical cavity semiconductor material during the photo-thermo-elastic process is investigated using the constructed combined plasma, thermal, and elastic wave model. It was thought to use an infinite material, which is an elastic semiconductor with a cylindrical cavity, and thermoelastic, isotropic, and uniform properties. Decreasing the heat flux of the laser pulse resulted in photogeneration of carrier density, which is confined to the inner surface of the cavity.

The cylindrical coordinates \(\left( {\rho ,\phi ,z} \right)\) with the \(z\)-axis aligned along the cylinder axis are considered. Due to symmetry, the semiconductor medium with a cylindrical cavity is assumed to cover the area \(\rho_{0} \le \rho < \infty\), and its state may be described in terms of the time \(t\) and distance \(\rho\). Initially, the temperature in the cylinder is steady and uniform \(T_{0}\). Furthermore, for the regularity constraint, all physical field variables are assumed to be finite within the medium.

The only non-vanishing displacement components and displacement–strain relations are

$$u_{\rho } = u\left( {\rho ,t} \right),{ }e_{\rho \rho } = \frac{u}{\rho },{ }e_{\phi \phi } = \frac{\partial u}{{\partial \rho }}$$
(14)

The stress–strain-temperature-carrier relations (2) will be the form

$$\begin{aligned} \sigma_{\rho \rho } & = 2\mu \frac{\partial u}{{\partial \rho }} + \lambda e - \left( {3\lambda + 2\mu } \right)\left( {\alpha_{t} \theta + \delta_{n} N} \right), \\ \sigma_{\phi \phi } & = 2\mu \frac{u}{\rho } + \lambda e - \left( {3\lambda + 2\mu } \right)\left( {\alpha_{t} \theta + \delta_{n} N} \right), \\ \sigma_{zz} & = \lambda e - \left( {3\lambda + 2\mu } \right)\left( {\alpha_{t} \theta + {\text{d}}_{n} N} \right) \\ \end{aligned}$$
(15)

where \(\alpha_{t}\) is the linear thermal expansion coefficient, \(\delta_{n}\) is the electronic deformation coefficient, \(\lambda\), \(\mu\) are the Lame’s constants, and \(e = \frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }\). When the Lorentz force \(F_{\rho }\) is taken into account, the dynamic motion equation becomes

$$\frac{{\partial \sigma_{\rho \rho } }}{\partial \rho } + \frac{1}{\rho }\left( {\sigma_{\rho \rho } - \sigma_{\phi \phi } } \right) + F_{\rho } = \varrho \frac{{\partial^{2} u}}{{\partial t^{2} }}$$
(16)

Assume the cylinder is immersed in a magnetic field of constant strength \(\overrightarrow {{{ }H}}_{0} = \left( {0,\;0,\;H_{0} } \right)\). According to Eq. (12), we obtain

$${ }\vec{E} = \left( {0,\;\mu_{0} H_{0} \frac{\partial u}{{\partial t}},\;0} \right),\;\vec{J} = \left( {0,\frac{\partial }{\partial \rho }\left( {\frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }} \right),\;0} \right),\;\vec{h} = \left( {0,\;0,\;\frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }} \right)$$
(17)

The magnetic field \(\vec{H}_{0}\) induces the radial component of the Lorentz force \(F_{\rho }\), which is given by

$$F_{\rho } = \mu_{0} \left( {\vec{J} \times \vec{H}_{0} } \right)_{\rho }$$
(18)

Thus, we have \(F_{\rho }\) and Maxwell's stress \(\tau_{\rho \rho }\) from Eqs. (13) and (17) as

$$F_{r} = \mu_{0} H_{0}^{2} \frac{\partial }{\partial \rho }\left( {\frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }} \right),\quad \tau_{\rho \rho } = \mu_{0} H_{0}^{2} \frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }$$
(19)

Inserting Eqs. (15) and (19) into Eq. (16), then we have

$$\left( {\lambda + 2\mu + \mu_{0} H_{0}^{2} } \right)\frac{\partial }{\partial \rho }\left( {\frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }} \right) - \gamma \frac{\partial \theta }{{\partial \rho }} - d_{n} \frac{\partial N}{{\partial \rho }} = \varrho \frac{{\partial^{2} u}}{{\partial t^{2} }}$$
(20)

where \(\left\{ {\gamma ,{ }d_{n} } \right\} = \left( {3\lambda + 2\mu } \right)\left\{ {\alpha_{t} ,\delta_{n} } \right\}\).

When we apply the operator \(\frac{1}{\rho }\frac{{\partial \left( {\rho u} \right)}}{\partial \rho }\) to both sides of Eq. (20), we obtain

$$\left( {\lambda + 2\mu + \mu_{0} H_{0}^{2} } \right)\nabla^{2} e - \gamma \nabla^{2} \theta - d_{n} \nabla^{2} N = \varrho \frac{{\partial^{2} e}}{{\partial t^{2} }}$$
(21)

In the cylindrical coordinate system, the Laplacian operator is given by \(\nabla^{2} = \frac{{\partial^{2} }}{{\partial \rho^{2} }} + \frac{1}{\rho }\frac{\partial }{\partial \rho }\). Without any heat sources (\(Q = 0\)), the generalized modified MGTE heat conduction Eq. (11) will be as follows:

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\left[ {\varrho C_{E} \frac{{\partial^{2} \theta }}{{\partial t^{2} }} + \gamma T_{0} \frac{{\partial^{2} e}}{{\partial t^{2} }}} \right] = K\nabla^{2} \dot{\theta } + K^{*} \nabla^{2} \theta + \frac{{E_{g} }}{\tau }\frac{{\partial^{2} N}}{{\partial t^{2} }}$$
(22)

The governing equations can easily be transformed into dimensionless forms. As a result, the dimensionless variables listed below are presented:

$$\begin{aligned} \left\{ {\rho ^{\prime}, u^{\prime}} \right\} & = v_{0} \eta \left\{ {\rho ,u} \right\}, \left\{ {t^{\prime},\tau_{0}^{^{\prime}} ,\tau ^{\prime}} \right\} = v_{0}^{2} \eta \left\{ {t,\tau_{0} ,\tau } \right\},\left\{ {\theta ^{\prime},N^{\prime}} \right\} = \frac{1}{{\rho v_{0}^{2} }}\left\{ {\gamma \theta ,d_{n} n} \right\}, \\ \left\{ {\sigma_{ij}^{^{\prime}} ,\tau_{\rho \rho }^{^{\prime}} } \right\} & = \frac{1}{{\rho v_{0}^{2} }}\left\{ {\sigma_{ij} ,\tau_{\rho \rho } } \right\}, \eta = \frac{{\rho C_{E} }}{K}, v_{0}^{2} = v_{1}^{2} + v_{a}^{2} \\ \end{aligned}$$
(23)

In Eq. (23), the parameter \(v_{1} = \sqrt {\frac{\lambda + 2\mu }{\varrho }}\) represents the dilatational wave speed, and the factor \(v_{a} = \sqrt {\frac{{\mu_{0} H_{0}^{2} }}{\varrho }}\) symbolizes the medium Alfven wave speed. The governing equations can be rewritten in the following forms if the primes are ignored:

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\left[ {\frac{{\partial^{2} \theta }}{{\partial t^{2} }} + \varepsilon_{1} \frac{{\partial^{2} e}}{{\partial t^{2} }}} \right] = \left( {\frac{\partial }{\partial t} + \omega^{*} } \right)\nabla^{2} \theta + \varepsilon_{2} \frac{{\partial^{2} N}}{{\partial t^{2} }}$$
(24)
$$\nabla^{2} e - \nabla^{2} \theta - \nabla^{2} N = \frac{{\partial^{2} e}}{{\partial t^{2} }}$$
(25)
$$\nabla^{2} N = g_{1} \frac{\partial N}{{\partial t}} + g_{2} N + g_{3} \theta$$
(26)
$$\begin{aligned} \sigma_{\rho \rho } & = 2\beta^{2} \frac{\partial u}{{\partial \rho }} + \left( {1 - 2\beta^{2} } \right)e - \theta - N, \\ \sigma_{\phi \phi } & = 2\beta^{2} \frac{u}{\rho } + \left( {1 - 2\beta^{2} } \right)e - \theta - N, \\ \sigma_{zz} & = \left( {1 - 2\beta^{2} } \right)e - \theta - N \\ \end{aligned}$$
(27)

where

$$\begin{aligned} \beta^{2} & = \frac{\mu }{\lambda + 2\mu },\;\varepsilon_{1} = \frac{{\gamma^{2} T_{0} }}{{\rho^{2} C_{e} c_{0}^{2} }},\;\omega^{*} = \frac{{K^{*} }}{{c_{0}^{2} K}},\;\varepsilon_{2} = \frac{{\gamma E_{g} v_{0}^{2} \eta }}{{\tau d_{{\text{n}}} \varrho C_{E} }}{ }, \\ \eta_{0} & = \left( {c_{0} \eta } \right)^{2} ,\;g_{1} = \frac{\rho }{{D_{E} \eta_{0} }},\;g_{2} = \frac{1}{{D_{E} \eta_{0} \tau_{1} }},\;g_{3} = \frac{{\kappa d_{n} }}{{\gamma D_{E} \eta_{0} }} \\ \end{aligned}$$
(28)

The initial conditions of the problem are taken as

$$\begin{aligned} u\left( {\rho ,0} \right) & = 0 = \frac{{\partial u\left( {\rho ,0} \right)}}{\partial \rho }, N\left( {\rho ,0} \right) = 0 = \frac{{\partial N\left( {\rho ,0} \right)}}{\partial \rho }, \\ \theta \left( {\rho ,0} \right) & = 0 = \frac{{\partial \theta \left( {\rho ,0} \right)}}{\partial \rho } \\ \end{aligned}$$
(29)

We suppose that the cylinder's interior surface is traction-free. Then the mechanical boundary conditions in this case can be expressed as

$$\sigma_{\rho \rho } \left( {\rho ,t} \right) = 0 \quad {\text{at}} \;\rho = \rho_{0}$$
(30)

Also, we assume that a variable heat flux in the form of exponentially laser pulsed heat is applied to the boundary surface \(\rho = \rho_{0}\). As a result, the following boundary condition may be applied [47]:

$$q_{\rho } = q_{0} \frac{{t^{2} }}{{16t_{p}^{2} }}{ }e^{{ - t/t_{p} }} , \quad {\text{at}}\quad \rho = \rho_{0}$$
(31)

where \(q_{0}\) is a constant and \(t_{p}\) is the pulsing heat flux duration time.

Using the modified Fourier’s Law (8), after using dimensionless variables (23), there will be

$$\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\dot{q}_{\rho } = - \,\left( {\frac{\partial }{\partial t} + \omega^{*} } \right) \frac{\partial \theta }{{\partial \rho }}$$
(32)

Equations (31) and (32) are decoupled to provide the following boundary condition:

$$\frac{{q_{0} }}{{16t_{p}^{2} }}\left( {1 + \tau_{0} { }\frac{\partial }{\partial t}} \right)\frac{\partial }{\partial t}\left( {t^{2} { }e^{{ - t/t_{p} }} } \right) = - \,\left( {\frac{\partial }{\partial t} + \omega^{*} } \right) \frac{\partial \theta }{{\partial \rho }}\,\, {\text{at}}\,\, \rho = \rho_{0}$$
(33)

The carriers can reach the sample surface during the diffusion phase, with a finite probability of recombination. As a result, the carrier density boundary condition may be written as follows:

$$D_{E} \frac{\partial N}{{\partial \rho }} = s_{v} N\quad {\text{at}}\quad \rho = \rho_{0}$$
(34)

where \(s_{v}\) is the surface recombination velocity.

4 Solution in the domain of the Laplace transform

The Laplace transform is utilized for solving linear differential equations with constant coefficients. In control system engineering, the Laplace transformation is very important. Laplace transforms of various functions must be performed to examine the control system. In order to analyze the dynamic control system, both the characteristics of the Laplace transform and the inverse Laplace transformation are employed. The Laplace transform of a function \(g\left( t \right)\), which is denoted by \({\mathcal{L}}\left[ {g\left( t \right){ }} \right]\) or by \(\overline{g}\left( s \right)\), is defined by the following equation:

$${\mathcal{L}}\left[ {g\left( t \right){ }} \right] = \overline{g}\left( s \right) = \int\limits_{0}^{\infty } {g\left( t \right)e^{ - st} {\text{d}}t} , s > 0$$
(35)

We get the following results by using the Laplace transform approach to Eqs. (24)–(27):

$$\left( {\nabla^{2} - \psi } \right)\overline{\theta } = \psi \varepsilon_{1} \overline{e} - \varepsilon_{2} s^{2} \overline{N}$$
(36)
$$\left( {\nabla^{2} - s^{2} } \right)\overline{e} = \nabla^{2} \overline{\theta } + \nabla^{2} \overline{N}$$
(37)
$$\left( {\nabla^{2} - g_{4} } \right)\overline{N} = g_{3} \overline{\theta }$$
(38)
$$\left[ {\begin{array}{*{20}c} {\overline{\sigma }_{\rho \rho } } \\ {\overline{\sigma }_{\phi \phi } } \\ {\overline{\sigma }_{zz} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {2\beta^{2} } & 0 & {\left( {1 - 2\beta^{2} } \right)} & { - 1} & { - 1} \\ 0 & {2\beta^{2} } & {\left( {1 - 2\beta^{2} } \right)} & { - 1} & { - 1} \\ 0 & 0 & {\left( {1 - 2\beta^{2} } \right)} & { - 1} & { - 1} \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\frac{{\partial \overline{u}}}{\partial \rho }} \\ {\frac{{\overline{u}}}{\rho }} \\ {\overline{\theta }} \\ {\overline{N}} \\ \end{array} } \right]$$
(39)

where \(\psi = s^{2} \left( {1 + \tau_{0} { }s} \right)/\left( {{\text{s}} + \omega^{*} } \right)\).

When Eqs. (36)–(38) are decoupled, then we get

$$\left( {\nabla^{6} - \alpha_{2} \nabla^{4} + \alpha_{1} \nabla^{2} - \alpha_{0} } \right)\left\{ {\overline{\theta },\overline{N},\overline{e}} \right\} = 0,$$
(40)

where \(\alpha_{2}\), \(\alpha_{1}\) and \(\alpha_{0}\) are specified by

$$\begin{aligned} \alpha_{2} & = s^{2} + g_{7} + \frac{{g_{6} }}{{g_{3} }},\alpha_{1} = s^{2} g_{7} + g_{8} + \frac{{g_{6} g_{5} }}{{g_{3} }},\alpha_{0} = s^{2} g_{8} , \\ g_{4} & = sg_{1} + g_{2} ,{ }g_{5} = g_{4} - g_{3} ,{ }g_{6} = { }g_{3} \psi \varepsilon_{1} , \\ g_{7} & = g_{4} + \psi , g_{8} = g_{4} \psi + { }s^{2} g_{3} \varepsilon_{2} \\ \end{aligned}$$
(41)

Presenting \(\lambda_{i} ,\) \(\left( {i = 1,\;2,\;3} \right)\) into Eq. (40), we obtain

$$\left( {\nabla^{2} - \lambda_{1}^{2} } \right)\left( {\nabla^{2} - \lambda_{2}^{2} } \right)\left( {\nabla^{2} - \lambda_{3}^{2} } \right)\left\{ {\overline{\theta },\;\overline{N},\;\overline{e}} \right\} = 0$$
(42)

where \(\lambda_{1}^{2}\), \(\lambda_{2}^{2}\) and \(\lambda_{3}^{2}\) are the roots of the equation

$$\lambda^{6} - \alpha_{2} \lambda^{3} + \alpha_{1} \lambda^{2} - \alpha_{0} = 0$$
(43)

which are given by

$$\begin{aligned} \lambda_{1}^{2} & = \frac{1}{3}\left[ {2\beta_{0} \sin \left( {\gamma_{0} } \right) + \alpha_{2} } \right], \\ \lambda_{2}^{2} & = - \frac{1}{3}\beta_{0} \left[ {\sin \left( {\gamma_{0} } \right) + \sqrt 3 \cos \left( {\gamma_{0} } \right)} \right] + \frac{1}{3}\alpha_{2} , \\ \lambda_{2}^{2} & = \frac{1}{3}\beta_{0} \left[ {\sqrt 3 \cos \left( {\gamma_{0} } \right) - \sin \left( {\gamma_{0} } \right)} \right] + \frac{1}{3}\alpha_{2} \\ \end{aligned}$$
(44)

with

$$\beta_{0} = \sqrt {\alpha_{2}^{2} - 3\alpha_{1} } ,{ }\gamma_{0} = \frac{1}{3}\sin^{ - 1} \left( { - \frac{{2\alpha_{2}^{3} - 9\alpha_{2} \alpha_{1} + 27\alpha_{0} }}{{2\beta_{0}^{3} }}} \right)$$
(45)

The general solution to Eq. (42) can be written as

$$\left\{ {\overline{e}, \;\overline{\theta },\;\overline{N}} \right\} = \mathop \sum \limits_{i = 1}^{3} \left\{ {1,\;L_{i} ,\;H_{i} } \right\}A_{i} K_{0} \left( {\lambda_{i} \rho } \right)$$
(46)

where \(K_{{\text{n}}} \left( {{ }.{ }} \right)\) indicates the second type of modified Bessel functions of order \(n\). The function \(K_{{\text{n}}} \left( {{ }.{ }} \right)\) is exponentially decaying, unlike conventional Bessel functions, which are oscillatory. The three parameters \(A_{i} ,\) \(\left( {i = 1,\;2,\;3} \right)\) are dependent on \(s\). In addition, \(L_{i}\) and \(M_{i}\) are two distinct factors that are correlated to \(A_{i}\). We get the following relations by inserting Eq. (46) into Eqs. (36)–(38)

$$H_{i} = \frac{{g_{3} \left( {\lambda_{i}^{2} - s^{2} } \right)}}{{\lambda_{i}^{4} - g_{5} \lambda_{i}^{2} }},\quad L_{n} = \frac{{\left( {\lambda_{i}^{2} - s^{2} } \right)\left( {\lambda_{i}^{2} - g_{4} } \right)}}{{\lambda_{i}^{4} - g_{5} \lambda_{i}^{2} }}, \quad i = 1,\;2,\;3$$
(47)

The cubical dilatation e in the Laplace transform domain is expressed as

$$\overline{e} = \frac{1}{\rho }\frac{\partial }{\partial \rho }\left( {\rho \overline{u}} \right) = \mathop \sum \limits_{i = 1}^{3} A_{i} K_{0} \left( {\lambda_{i} \overline{\rho }} \right)$$
(48)

When both sides of the above equation are integrated with regard to \(\rho\), we obtain

$$\overline{u} = - \mathop \sum \limits_{i = 1}^{3} \frac{1}{{m_{i} }}A_{i} K_{1} \left( {\lambda_{i} \rho } \right)$$
(49)

Equation (49) is differentiated in terms of \(\rho\) to provide

$$\frac{{{\text{d}}\overline{u}}}{{{\text{d}}\rho }} = \mathop \sum \limits_{i = 1}^{3} A_{i} \left( {K_{0} \left( {\lambda_{i} \rho } \right) + \frac{1}{{\rho { }\lambda_{i} }}K_{1} \left( {\lambda_{i} \rho } \right)} \right)$$
(50)

Consequently, the closed form solutions for thermal stresses are as follows:

$$\begin{aligned} \overline{\sigma }_{\rho \rho } & = \mathop \sum \limits_{i = 1}^{3} A_{i} \left( {\Omega_{i} K_{0} \left( {\lambda_{i} \rho } \right) + \frac{{2\beta^{2} }}{{\rho \lambda_{i} }}K_{1} \left( {\lambda_{i} \rho } \right)} \right), \\ \overline{\sigma }_{\phi \phi } & = \mathop \sum \limits_{i = 1}^{3} A_{i} \left( {\Phi_{i} K_{0} \left( {\lambda_{i} \rho } \right) + \frac{{2\beta^{2} }}{{\rho \lambda_{i} }}K_{1} \left( {\lambda_{i} \rho } \right)} \right), \\ \overline{\sigma }_{zz} & = \mathop \sum \limits_{i = 1}^{3} A_{i} \Phi_{i} K_{0} \left( {\lambda_{i} \rho } \right) \\ \end{aligned}$$
(51)

where

$$\Omega_{i} = 1 - L_{i} - H_{i,} \quad \Phi_{i} = 1 - 2\beta^{2} - L_{i} - H_{i}$$
(52)

The non-dimensional Maxwell's stress \(\overline{\tau }_{\rho \rho }\) is given by

$$\overline{\tau }_{\rho \rho } = \frac{{v_{a}^{2} }}{{v_{0}^{2} }}e = \frac{{v_{a}^{2} }}{{v_{0}^{2} }}\mathop \sum \limits_{i = 1}^{3} A_{i} K_{0} \left( {\lambda_{i} \rho } \right)$$
(53)

The boundary conditions (30), (33) and (34) have the following forms after performing the Laplace transform:

$$\begin{array}{*{20}c} {\left. {\frac{{\partial \overline{\theta }}}{\partial \rho }} \right|_{{\rho = \rho_{0} }} = - \frac{{sq_{0} \left( {1 + s{\text{t}}0} \right){\text{tp}}^{3} }}{{8t_{p}^{2} \left( {s + \omega^{*} } \right)\left( {1 + s{\text{tp}}} \right)^{3} }} = - \overline{G}\left( s \right),} \\ {\overline{\sigma }_{\rho \rho } \left( {\rho_{0} ,{\text{s}}} \right) = 0,} \\ {D_{E} \left. {\frac{{\partial \overline{N}}}{\partial \rho }} \right|_{{\rho = \rho_{0} }} = s_{f} \overline{N}\left( {\rho_{0} ,s} \right)} \\ \end{array}$$
(54)

Equations (46) and (51) are substituted into Eq. (54), giving

$$\begin{array}{*{20}l} {\mathop \sum \limits_{i = 1}^{3} \lambda_{i} A_{i} K_{1} \left( {\rho_{0} { }\lambda_{i} } \right) = \overline{G}\left( s \right),} \hfill \\ {\mathop \sum \limits_{i = 1}^{3} \frac{1}{{m_{i} }}A_{i} K_{1} \left( {\rho_{0} { }\lambda_{i} } \right) = 0,} \hfill \\ {\mathop \sum \limits_{i = 1}^{3} A_{i} H_{i} \left( {\left( {D_{E} - s_{v} } \right)K_{0} \left( {\lambda_{i} \rho_{0} } \right) + \frac{{D_{E} }}{{\rho_{0} { }\lambda_{i} }}K_{1} \left( {\lambda_{i} \rho_{0} } \right)} \right) = 0} \hfill \\ \end{array}$$
(55)

We derive the values of the parameters \(A_{i} ,\) \(\left( {i = 1,\;2,\;3} \right)\) by solving the system (55).

The inversion of Laplace transforms was obtained in this study using an accurate and efficient numerical approach based on Fourier series expansion [48]. Any function in the Laplace domain can be inverted to the time domain using this method as follows:

$$\Gamma \left( {\rho ,t} \right) = \frac{{e^{ct} }}{t}\left( {\frac{1}{2}\overline{\Gamma }\left( {\rho ,c} \right) + {\text{Re}} \mathop \sum \limits_{j = 1}^{{N_{f} }} \overline{\Gamma }\left( {\rho ,c + \frac{ij\pi }{t}} \right)\left( { - 1} \right)^{j} } \right)$$
(56)

where \(N_{f}\) denotes the number of terms, \({\text{Re}}\) denotes the real part, and \(i\) denotes the imaginary number unit. Numerous numerical tests have demonstrated that the value of the parameter \(c\) fulfills the relation \(c\tau \cong 4.7\), allowing for quicker convergence [49].

5 Numerical results

To demonstrate the theoretical results obtained in the previous sections, we will now present some numerical findings. Using Mathematica software, the influence of the modified photothermal heat equation (MGTPT), which is defined by the Moore-Gibson-Thompson (MGT) equation, on the studied physical fields can now be seen as graphical representations and tables. In the theoretical analysis, isotropic silicon (Si) is used as the semiconductor solid material in the device. The physical parameters utilized at \(T_{0} = 300{\text{ K}}\) are as follows [26]:

$$\begin{array}{*{20}l} {\lambda = 3.64 \times 10^{10} \,{\text{kg m}}^{ - 1} {\text{s}}^{ - 2} ,\;\mu = 5.46 \times 10^{10} \,{\text{kg m}}^{ - 1} {\text{s}}^{ - 2} ,\;\rho = 2330 \,{\text{kg m}}^{ - 3} ,} \hfill \\ {K = 1.51 \,{\text{W}} {\text{m}}^{ - 1} {\text{K}}^{ - 1} ,\;C_{E} = 6.95 \times 10^{2} \, {\text{J}} {\text{kg}} {\text{K}}^{ - 1} ,\;d_{n} = - 9 \times 10^{ - 31} \,{\text{m}}^{3} , } \hfill \\ {E_{g} = 1.11 \,{\text{eV}},\;D_{E} = 2.5 \times 10^{ - 3} \,{\text{m}}^{2} {\text{s}}^{ - 1} ,\;s_{f} = 2\, {\text{m}} {\text{s}}^{ - 1} ,\;\tau = 5 \times 10^{ - 5} \, {\text{s}}} \hfill \\ \end{array}$$

In the context of the coupled theory of thermal-plasma-elastic waves under the MGTE model, the numerical approach described in (56) has been used to distribute the distribution of non-dimensional temperature \(\theta\), the radial displacement \(u\), the radial and hoop stresses \(\sigma_{\rho \rho }\) and \(\sigma_{\phi \phi }\), Maxwell's stress \(\tau_{\rho \rho }\) and the absolute carrier density \(N\) along the radial direction of the cylinder. Figures 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 and 11 graphically depict the numerical findings for time \(t = 0.12{\text{s}}\) and \(\rho_{0} = 1\).

Fig. 1
figure 1

The temperature variation \(\theta\) for different models of photo-thermoelasticity

Full size image
Fig. 2
figure 2

The displacement variation \(u\) for different models of photo-thermoelasticity

Full size image
Fig. 3
figure 3

The carrier density \(N\) variation for different models of photo-thermoelasticity

Full size image
Fig. 4
figure 4

The radial stress variation \(\sigma_{\rho \rho }\) for different models of photo-thermoelasticity

Full size image
Fig. 5
figure 5

The hoop stress variation \(\sigma_{\phi \phi }\) for different models of photo-thermoelasticity

Full size image
Fig. 6
figure 6

The Maxwell's stress \(\tau_{\rho \rho }\) for different models of photo-thermoelasticity

Full size image
Fig. 7
figure 7

The 3D plot of the temperature \(\theta\) versus the distance \(\rho\) and instant time \(t\)

Full size image
Fig. 8
figure 8

The 3D plot of the displacement \(u\) versus the radial distance \(\rho\) and instant time \(t\)

Full size image
Fig. 9
figure 9

The 3D plot of the carrier density \(N\) versus the distance \(\rho\) and instant time \(t\)

Full size image
Fig. 10
figure 10

The 3D plot of the radial stress \(\sigma_{\rho \rho }\) versus the radial distance \(\rho\) and instant time \(t\)

Full size image
Fig. 11
figure 11

The 3D plot of the Maxwell stress \(\tau_{\rho \rho }\) versus the radial distance \(\rho\) and instant time \(t\)

Full size image

5.1 Verification of the results

To validate the numerical results and the validity of the proposed MGTPT photo-thermoelastic model, a numerical comparison with the results of Abbas and Aly [50], Lotfy et al. [51] and Khamis et al. [52] and Abouelregal [53] was done. The governing equations in [51,52,53,53] were applied using the Lord and Shulman [2], Green–Naghdi [4], and Green and Lindsay [3] photo-thermoelastic models, whereas the current model introduced and applied the Moore-Gibson-Thompson photo-thermoelastic model (MGTPT) [43]. By comparing the results, it was discovered that the thermal parameters \(\tau_{0}\) and \(K^{*}\) of the photothermal model (MGTPT) have a significant influence on the studied fields, despite the agreement in behavior and convergence in numerical values. In addition, the presence of the thermal parameters \(\tau_{0}\) in the proposed model reduces and relaxes the mechanical and thermal wave behavior.

In three additional cases, numerical calculations will be performed for all studied field variables.

5.2 Comparison of several thermoelasticity models

The suggested Moore-Gibson-Thompson photothermal (MGTPT) model is a generalization of several photo-thermoelasticity models used in this study. It was provided not only to generalize but also to solve some of the physical consequences found in some earlier models. In the first and second sections, many inconsistencies are addressed.

Many earlier models of photo-thermoelasticity can be derived as special instances by reference to the modified Moore–Gibson–Thompson heat conduction equation (MGTPT). When \(\tau_{0} = K^{*} = 0\), the coupled photo-thermoelasticity theory (CPTE) is obtained, and when \(K^{*} = 0\), the generalized Lord and Shulman photo-thermoelasticity model (PLS) is obtained. Furthermore, the photothermal Green and Naghdi model (PGN-III) can be obtained if the relaxation parameter \(\tau_{0} = 0\) is omitted, and the photothermal Green and Naghdi model (PGN-II) may be obtained if the term that contains the parameter \(K\) is absent. If \(\tau_{0} ,K^{*} > 0\), this indicates that the photothermal model (MGTPT) is used.

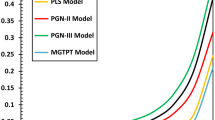
The present subsection will examine the comparison of the newly given model with other previous photothermal models in order to verify them. To illustrate comparison and research, as well as for practical purposes, tables and figures will be used to illustrate discussions and comparisons. Tables 1, 2, 3, 4, 5 and 6 as well as Figs. 1, 2, 3, 4, 5 and 6 show the changes in investigated studied fields against radial distance \(\rho\) for the CPTE, PLS, PGN-II, PGN-III, and MGTPT models. In this case, the non-dimensional carrier lifetime parameter \(\tau\) and the pulsing heat flux duration time \(t_{p}\) are fixed (\(\tau = 0.01,\;t_{p} = 0.15\)).

Table 1 The variation of the temperature \(\theta\) against the radial distance \(\rho\)
Full size table
Table 2 The variation of the displacement \(u\) against the radial distance \(\rho\)
Full size table
Table 3 The variation of the carrier density \(N\) against the radial distance \(\rho\)
Full size table
Table 4 The variation of the radial stress \(\sigma_{\rho \rho }\) against the radial distance \(\rho\)
Full size table
Table 5 The variation of the hoop stress \(\sigma_{\phi \phi }\) against the radial distance \(\rho\)
Full size table
Table 6 The Maxwell's stress \(\tau_{\rho \rho }\) against the radial distance \(\rho\)
Full size table

The change of the absolute value of non-dimensional temperature \(\theta\) with regard to radial distance \(\rho\) is shown in Fig. 1. It has been discovered that as \(\rho\) increases, the temperature \(\theta\) values increase until they reach a maximum around \(\rho = 1.2\), after which they decrease monotonically with increasing distance \(\rho\). The magnitudes of carrier density \(N\) as a function of distance \(\rho\) are depicted in Fig. 2. The graphic shows that the carrier density \(N\) value is highest near the surface and subsequently declines in a monotonous manner as the distance from the cavity increases. Figure 3 shows how the radial displacement \(u\) varies with distance \(\rho\). The displacement grows rapidly with increasing r until it reaches the peak point, after which it decreases monotonically from the boundary surface away from the application of the laser pulse heat flux.

Figures 4 and 5 illustrate the radial and hoop stresses \(\sigma_{\rho \rho }\) and \(\sigma_{\phi \phi }\), in photo-thermoelastic media for various photothermal models with regard to radius \(\rho\) for different models of photothermal. The radial pressure \(\sigma_{\rho \rho }\) on the cavity surface (\(\rho = 1\)) is zero in all representations, which corresponds to the case of the mechanical boundary of the problem where the cavity surface is free of traction. From the figures, it can be observed that thermal stresses are usually compressive. In Fig. 6, the change in Maxwell stress \(\tau_{\rho \rho }\) in relation to distance \(\rho\) was displayed. Figure 2 shows that the Maxwell stress \(\tau_{\rho \rho }\) variance takes its highest surface value and declines rapidly until reaching its lowest point, then grows extremely monotonously until it disappears.

In addition to the previous observations from Tables 1, 2, 3, 4, 5 and 6, we can mention some noteworthy facts.

  • The existence of variations in the fields is obviously developing over time in the photothermal MGTPT model and has an important influence across all domain profiles examined.

  • The thermal parameters \({\tau }_{0}\) and \({K}^{*}\) has a significant impact on all of the domains investigated, as seen in the graphs.

  • The phenomena of restricted thermal transmission velocity of the photo-thermoelasticity MGTPT theory is well understood in all Figures and Tables.

  • Different domain distributions have a limited prevalence. This is in contrast to cases where coupling and decoupling theorems in photo-thermoelasticity have an infinite propagation rate, which results in nonzero values for all functions anywhere in the medium.

  • The coupled photothermal model (CPTE), as well as modified and generalized photothermal models (PLS, PGNII, PGNIII, and MGTPT), give values that are different in magnitude but similar in behavior at the surface of the semiconducting cylinder, where the boundary conditions appear.

  • For all physical domains, all curves converge, where \(\rho\) tends to zero to meet the regularity requirement.

  • The temperature of GN-III is likewise found to be higher than that of MGTE, with comparable results for the PLS and MGTPT models.

  • The results of the frequently used GN-III thermal elasticity model show that it varies considerably from the GN-II thermal elasticity models in terms of reduced energy dissipation.

  • In the LS and MGTE models, the inclusion of the relaxation parameter might result in a slower temperature decline.

  • The solution instantly fills the entire medium in the case of the CPTE coupled and the PGNIII model. The solution is thus not the same zero for every tiny value of time (although it may be very small). The solution achieved with the MGTPT photo-thermoelasticity model equations, however, shows the comportment of final wave propagation velocities.

  • The PGN-III results also show convergence from the conventional elasticity model (CPTE), which, unlike other generalized thermoelasticity models, does not vanish quickly inside the body due to heat. This is fully consistent with Quintanilla's claims [14] and Abouelregal et al. observations [17,18,19].

  • In one section of the cylinder, all kinds of pressure are compressed, while in another area, there is tension. Tensile highlights that the medium next to the cylinder surface is positioned throughout time, and this conforms to the information given in [54]. Furthermore, the higher value and amplitude of fields measured at the cavity surface are obvious with the rise in radial breadth. The explanations for this occurrence are included in [55].

  • For small time values, the solution is located in a limited area of space enclosing the spherical cavity, and beyond this region is identically null. The area becomes longer and fills up the middle of the medium. The boundary of the wavefront is located. All models provide almost identical findings for long time values. This is because the second sound effects are not long enough.

5.3 The effect of the instant time

For constant values of carrier lifetime \(\tau\) and laser pulse duration \(t_{p}\) parameters, Figs. 7, 8, 9, 10 and 11 depict changes in the behavior of photothermal field variables as functions of distance \(\rho\) and time \(t\) in 3D plots. In this case, the discussion was carried out in the context of the proposed model of photo-thermoelasticity (MGTPT), which includes relaxation time. Figures 7, 8, and 9 show that as time \(t\) passes, the carrier density \(N\), temperature \(\theta\), and the amount of displacement \(u\) distributions increase along the radial direction. Figures 10 and 11 demonstrate the change of radial stress \(\sigma_{\rho \rho }\) and Maxwell stress \(\tau_{\rho \rho }\) against the radial distance \(\rho\) over the instant time \(t\). It was discovered that when the values of time along the \(\rho\)-direction grow, the distributions decrease.

All of these diagrams demonstrate the phenomena of limited propagation speeds. The photothermal impacts of the surrounding medium are confined to an area near the cavity for the smallest values of time taken into account. For the maximum value of time, this area grows to fill the whole medium. The propagation of wavefronts from the cavity of an unbounded medium is shown in this region. The classical coupled photothermal theory (CPTE), on the other hand, does not have this problem since thermal impacts are instantly felt throughout the medium.

Due to laser pulse heat flux, the medium attached to the cylindrical cavity surface is expanded, and the others are compressively deformed. Deformation is a process that is dynamic. Over time, the growth zone is progressively moving inside and getting bigger and bigger. The displacement \(u\) is, therefore, increasing and growing. At a given moment, the nonzero radial area is limited owing to the thermal-and plasma-based wave effect. It implies that photothermal transfers with a limited velocity over time into the depth of the material. The more instantly the thermal area and the displacement are evaluated, the more appropriate.

The medium at the cavity surface is subject to a tensile stress that increases with time. The presence of stress at the cavity surface could be attributed to the effects of cross-effects caused by the connection of the temperature, photothermal, and strain fields. Due to these intersectional effects, the laser excitation leads to the generation of a further photothermal field of thermal stress.

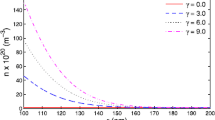
5.4 The effect of carrier lifetime and laser pulse duration parameters

Exploration of lasers was useful to understand the properties of a material's inner structure, and as a result, various modern applications in physical sciences, engineering, and medicine have emerged. The thermal effect of a non-Gaussian laser on a thermoelastic material that is employed as a heat source is highly relevant under the influence of extended thermoelastic theories.

The use of photothermal excitation of shorter elastic pulses (high-frequency elastic waves) in various fields of practical physics is now of great interest. When a laser beam (laser light source) was incident on an intracavity sample, the laser pulses caused the temperature to increase. The free carrier's chargeintensity appears after all excited electrons have been photo-excited. The fact that photothermal-produced ultrasonic waves contain information about the producing medium and surrounding media has enabled the creation of one-dimensional theoretical models.

Theoretical and practical ways of obtaining information on carrier intrinsic concentrations and a long carrier lifetime \(\tau\) are critical criteria for modeling semiconductor devices in order to comprehend and improve device physics and performance. The minority carrier movement and effective carrier lifetime parameter \(\tau\) of the absorber material for solar cells affect the open-circuit voltage and photo-generated current density \(N\) in particular.

The phenomena of microwave heating using pulsed lasers is investigated in this section. The solid cylinder is constructed of silicon and heated by a pulsed non-Gaussian laser beam with a duration of \(t_{p}\), causing the vibration to be dampened by thermoelasticity. Energy dissipation occurs when temperature and stress combine, turning mechanical energy into permanent heat energy. Table 7 shows how the duration of a laser pulse \(t_{p}\) and the lifespan parameter \(\tau\), influence the dimensionless thermo-physical investigated fields at \(\rho = 1.1\). The study in this subsection will be carried out in light of Moore-Gibson-Thomson's photo-thermoelastic modified theory (MGTPT).

Table 7 Effects of carrier lifetime and laser pulse duration parameters on the dimensionless physical fields
Full size table

The investigated fields, comprising temperature, displacement carrier density, and thermal stress components, are dependent not only on distance \(\rho\) and time \(t\) but also on the duration of the laser pulse \(t_{p}\) and the photo-generated carrier lifespan parameter \(\tau\), as shown in Table 7. Moreover, thermal and mechanical waves are more sensitive to the change of laser duration \(t_{p}\) than to the change of lifetime \(\tau\).

The values of the temperature \(\theta\), carrier density \(N\), and radial displacement \(u\) have all been demonstrated to decrease as the time of the laser pulse \(t_{p}\) grows, but the values of thermal stress \(\sigma_{\rho \rho }\) and Maxwell's stress \(\tau_{\rho \rho }\) have both increased. From the Table, it can be seen that by increasing the photo-generated carrier lifetime parameter \(\tau\), we find that there is an increase in the temperature, carrier density \(N\), the magnitudes of the thermal stress \(\sigma_{\rho \rho }\) and Maxwell's stress \(\tau_{\rho \rho }\). However, the radial displacement \(u\) distribution decreases with the increase in value of the lifetime parameter \(\tau\).

In the investigation of the macroscopic output of some materials pertaining to photothermal materials, which dominate in the determination of material characteristics, the carrier lifetime parameter \(\tau\) will play a significant role. All of these findings demonstrate the concept of limited heat dispersion rates. For designers of novel materials and other disciplines of materials science and physical engineering, the results obtained in this example might be useful in the presence of plasma waves and elasticity. Because of flaws and a lack of information about material characteristics, measuring carrier lifetime in many thin-film photovoltaic materials may be challenging.

Rapidly varying contraction and expansion cause temperature fluctuations in materials susceptible to heat transmission by conduction [56]. Because pulsed laser technologies are widely utilized in material processing, nondestructive testing, and characterization, this mechanism has attracted a lot of attention [57].

6 Conclusion

This study offers a new photothermoelastic model of heat conduction based on the Moore-Gibson-Thompson equation. The improved photothermal model includes the Type III Green-Naghdi model, as well as the Lord and Shulman heat transfer equation. This expanded model is used to explore the interaction of heat, plasma, and elastic waves in semiconducting materials. Only a few reviews of the literature on our model have been published due to the complexity of the governing equations in extended photothermal theory. As special instances, many thermoelastic and photothermal models may be developed from a given model.

According to the discussions, the following important conclusions can be summarized:

  • Thermal parameters have a major influence on the distributions of photothermal field values. In the fields studied, the effects of laser pulse duration and lifetime characteristics are also important.

  • The temperature passes through the medium as a finite velocity wave in the new stretched thermal image-flexible MGTPT model rather than as an infinite velocity wave in the classical models. Thus, the generalized MGTPT model can be considered better in understanding the photo-thermoelastic process than the PGN-III model. Coupled photo-thermoelastic and generalized models provide close results for high time values. On the contrary, when we examine the short value of time, the situation is quite different.

  • Due to the laser pulsed heat flow, the medium attached to the surface of the cylindrical cavity is expanded, and the cylinder is compactly deformed.

  • When comparing the solutions, it can be concluded that the lifespan coefficient is an important phenomenon and has a great influence on the distribution of different physical domains.

The proposed work in this study is a theoretical basis for describing the photoacoustic effect in semiconductors that takes into account the thermal effects, diffusion, and recombination of carriers. The ideas presented in this study can also be very useful to scientists working in areas such as physics, material design, thermal efficiency, and geophysics. Finally, the method used in the present work can be used to address a variety of thermoelastic and thermodynamic problems.