Keywords

1 Introduction

As the jib crane is used for material handling purpose in most of the manufacturing industries. Jib crane having a hanging arm which acts as a cantilever beam thus it is subjected to axial compressive load and moment; also, it is subjected to the vibrations caused by various heavy machinery it is entrapped by cracks which further turn into failure. The cracks may develop from the long-term service, impact applied cyclic loads, aerodynamic loads, shrinkage, etc. Therefore, it is very essential to analysis the behaviour of buckling, stability of column, vibration analysis, crack failure [1,2,3,4,5]. A jib crane comprises of a derrick bolstered on a level bar that is cantilever from a vertical section, the flat bar turns about the vertical hub framed by the segment to give an even range to the crane [4]. The beam also serves as the track for the hoist trolley to provide radial travel along the length of the beam (Fig. 1). Standard capacities of a jib crane range up to about 5000 kg and can rotate 180–360°. The mechanical properties of structural steel are mentioned in Table 1.

Fig. 1
figure 1

Jib crane model

Full size image
Table 1 Mechanical properties
Full size table

2 Modelling Software

CATIA is used to create model of column [6,7,8]. Solid model of column is shown in Fig. 2.

Fig. 2
figure 2

Solid model of column

Full size image

2.1 Analysis Software

The ANSYS 12.0 is used for structural analysis of column [9,10,11]. Material data selected for pre-process is structural steel. Input parameters for ANSYS are given in Table 2.

Table 2 Input parameters for ANSYS 12
Full size table

2.2 Beam Element

SOLID45 is utilized for the 3D displaying of strong structures [12, 13]. The component is characterized by eight hubs having three degrees of opportunity at every hub: interpretations in the nodal x, y and z headings as shown in Fig. 3. The element has plasticity, creep, swelling, stress stiffening and large deflection. The degrees of freedoms for solid45 beam element are UX, UY and UZ.

Fig. 3
figure 3

Geometry of solid45 element

Full size image

2.3 Mesh Model and Boundary Conditions

Mapped mesh model generated using tetrahedron 4 nodes as shown in Fig. 4. Total number of nodes are 8138, and total number of elements are 4278.

Fig. 4
figure 4

Mesh model of jib crane column

Full size image

Boundary conditions taken as shown in Fig. 5 with ‘A’—Zero displacement provided in ‘X’ Direction ‘B’—Zero displacement provided in ‘Y’ Direction ‘C’—Zero displacement provided in ‘Z’ Direction ‘D’—Force applied of 1764 N. Static structural analysis of column without crack is shown in Fig. 6.

Fig. 5
figure 5

Boundary conditions

Full size image
Fig. 6
figure 6

Static structural analysis of column without crack

Full size image

3 Static Structural Analysis of Column with Single Crack

Consider crack in column of jib crane as depth 13 mm, width 6.5 mm, thickness 2 mm, position 212 mm (from top) as shown in Fig. 7. The analysis results for column with single crack are shown in Figs. 8, 9 and 10.

Fig. 7
figure 7

Column with single crack (Line diagram)

Full size image
Fig. 8
figure 8

Column with single crack (Structural analysis)

Full size image
Fig. 9
figure 9

Maximum stress zone

Full size image
Fig. 10
figure 10

Buckling analysis of column with one crack

Full size image

Load multiplier for single cracked column is given in Table 3. Load multiplier factor is computed 658.68, and thus, the critical load for buckling is calculated as:

Table 3 Load multiplier data for single crack
Full size table

Critical load = Load multiplier × Force applied.

Critical load = 684.37 × 1764.

Critical load for a single crack column = 1207.22 k.

3.1 Static Structural Analysis of Column with Multiple Crack

Consider multiple cracks in column (Fig. 11) with their locations as depth 13 mm, width 6.5 mm, thickness 2 mm, position 1st crack 212 mm (from top end), position 2nd crack 227 mm (from top end), position 3rd crack 257 mm (from top end). Analysis results for column with multiple crack are shown in Figs. 12 and 13.

Fig. 11
figure 11

Model of jib crane column with multiple cracks

Full size image
Fig. 12
figure 12

Static structural analysis of column with multiple cracks

Full size image
Fig. 13
figure 13

Buckling analysis of column with multiple cracks

Full size image
Table 4 Load multiplier data for multiple crack
Full size table

4 Buckling Load Calculation for Multiple Cracks

Load multiplier factor is calculated as 676.04 as shown in Table 4, and thus, the critical load can be calculated as:

Critical load = Load multiplier × Force applied.

Critical load = 676.04 × 1764.

Critical load for a multi-cracked column = 1192 kN.

4.1 Transfer Matrix Method

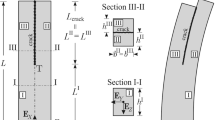
In the numerical model, the split areas are commonly spoken to as massless rotational springs. Additionally, local adaptability coefficients of these springs are elements of the profundities of splits and the distance across of the cross-segment of the segment (Fig. 14).

Fig. 14
figure 14

Segments in transfer matrix method

Full size image

The differential equation for buckling of the first segment can be written as:

$$\frac{{{\text{d}}^{{4}} y_{{1}} }}{{{\text{d}}x^{{4}} }} + \, \frac{{k^{{2}} {\text{d}}^{{2}} y_{{1}} }}{{{\text{d}}x^{{2}} }} = \, 0$$

Solution of above differential equation is: y1(x) = A1 + A2 x + A3sin(kx) + A4cos(kx).

Where k2 = P/EI, and P is the axial compressive force.

The generalized solution for above equation is given by:

$$T_{{1}} = \, \left[ {B\left( {x_{{{\text{c1}}}} } \right)} \right]\left[ {B\left( 0 \right)} \right]^{{ - {1}}}$$

where [T1] is called ‘the transfer matrix’.

and

$$\left[ {B\left( x \right)} \right]{ } = { }\left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} 1 & x & {{\text{sin}}\left( {kx} \right)} & {{\text{cos}}\left( {kx} \right)} \\ 0 & 1 & {k{\text{cos}}\left( {kx} \right)} & { - k{\text{sin}}\left( {kx} \right)} \\ 0 & 0 & {p{\text{sin}}\left( {kx} \right)} & {p{\text{cos}}\left( {kx} \right)} \\ 0 & { - p} & 0 & 0 \\ \end{array} } \\ \end{array} } \right]$$

After applying boundary condition (one end fixed and other end is free) in above equation will reduce to:

$$\left[ {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {T11} & {T12} \\ {T21} & {T22} \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {y1\left( 0 \right)} \\ {\theta 1\left( 0 \right)} \\ \end{array} } \right]$$

5 Results and Discussion

5.1 Buckling Load for Single Column Using Transfer Matrix Method

The generalized equation for single crack column:

$${\text{cos}}\left( {kL} \right) - {\text{EICi}}k{\text{ sin}}\left( {\beta kL} \right){\text{ cos}}\left[ {\left( {{1} - \beta } \right)kL} \right] \, = \, 0$$
$$k{2 } = P/{\text{EI}}$$

where β = xCi/L, and Ci is flexibility of cracked column.

For jib crane column.

L = 360 mm.

D = 50 mm.

β = 0.58.

Ci = 0.26.

k = 0.00170.

Thus after substitution of values of L, β, Ci and k in above equations, critical buckling load for single cracked jib crane column (Pcr) is calculated as 1740 kN.

5.2 Buckling Load for Multiple Column Using Transfer Matrix Method

The generalized equation for multiple cracks is:

$$\begin{aligned} &{\text{cos}}\left( {{\text{kL}}} \right) - {\text{EICi}}k\Sigma {\text{ sin}}\left( {\beta ikL} \right){\text{cos}}\left[ {\left( {{1} - \beta i} \right)kL} \right] \, \hfill \\&\quad + \, \left( {{\text{EI}}} \right){\text{2k2}}\left\{ {{\text{C1C2sin}}\left( {\beta {1}kL} \right){\text{sin}}\left[ {\left( {\beta {2} - \beta {1}} \right)kL} \right]{\text{cos}}\left[ {\left( {{1} - \beta {2}} \right)kL} \right]} \right\} \, \hfill \\&\quad + {\text{ C2C3sin}}\left( {\beta {2}kL} \right){\text{sin}}\left[ {\left( {\beta {3} - \beta {2}} \right)kL} \right]{\text{cos}}\left[ {\left( {{1} - \beta {3}} \right)kL} \right] = 0 \hfill \\ \end{aligned}$$

For jib crane column with multiple cracks.

L = 360 mm, i = 1 … 3.

D = 50 mm.

β1 = 0.58,

β2 = 0.63,

β3 = 0.71,

Ci = 0. 26,

k = 0.0001497.

k2 = P/EI.

Thus after substitution of values of L, β1, β2, β3, C and k in above equation, critical buckling load for single cracked jib crane column (Pcr) is calculated as 1345.81 kN.

Thus, the buckling load values obtained by ANSYS and transfer method are compared and provided in Table 5.

Table 5 Comparison of buckling load for single and multiple crack
Full size table

6 Conclusion

It considered that a changing position of cracks causes more reduction in critical buckling load than in case of multiple cracks. It shows the variation of the critical load to the buckling load depending upon the dimension crack depth a/D for the dimension crack location of x/L. If the location of the crack changes nearer to fixed end where the buckling moment is maximum results in a greater reduction in the critical buckling load and is therefore more critical in the stability behaviour of the column.

From transfer matrix method, the critical buckling load of multiple cracked column is 22% lower in comparison with single cracked column. From ANSYS12, the critical buckling load of multiple cracked column is 13% lower in comparison with single cracked column.