Integrating Arithmetic and Algebra in a Collaborative Learning and Computational Environment Using ACODESA

Abstract

In the transition from arithmetic to algebra and in light of the disjunction between the natural and symbolic approach to algebra and the choice of a natural way of learning, this paper discusses the development of a cognitive control structure in pupils when they are faced with a mathematical task. Researchers sought to develop, in novice pupils in both Quebec (12–13 years old) and Mexico (14–15 years old), an arithmetic-algebraic thinking structure that would promote mathematics competencies in a method based on collaborative learning, scientific debate and self-reflection (ACODESA, acronym which comes from the French abbreviation of Apprentissage collaboratif, Débat scientifique, Autoréflexion), and immersed in an activity theory approach. This paper promotes the equal use of both paper and pencil and technology in order to solve a mathematical task in a sociocultural and technological environment.

Introduction

Over the course of the last century , the mathematics curriculum took arithmetic as a proper subject for study at primary school level education , and algebra as a proper subject for secondary school level . This dissociation influenced research in mathematics education , which, in turn, reverberated through the academic programs implemented. Examining the work of psychologists before the 1940s, Brownell (1942) noted that psychologists used “puzzles ” in the study of intelligence to analyse processes related to the “insight ” involved when individuals solved such problems . Brownell proposed a radical change focusing on the study of the resolution of the arithmetic verbal problems used in textbooks .

Brownell’s work (Ibid.) attracted the attention of psychologists and educators interested in studying the skills involved in solving arithmetic word problems as a means of understanding the phenomena linked to learning mathematics .

The experience of solving puzzle type problems (where, for example , from 9 matches one is required to build four equilateral triangles , and then, from 6 matches , one is asked to build the same number of equilateral triangles ) gave rise to the analysis of solving problems that had single or multiple solutions . From a psychological point of view, these factors led, in the case of a problem with one solution, to an analysis of convergent thinking linked to direct efforts towards achieving a goal . In the case of problems with multiple solutions , this led to an analysis of both divergent thinking (Guilford 1967) and creativity (Bear 1993). In fact , Guilford’s model (Ibid.) stressed the importance of developing divergent before convergent thinking . Gradually, Brownell’s approach led to an extensive research strand focusing on the phenomena related to the resolution of arithmetic problems in primary school and the development of arithmetic thinking .

What Is Arithmetic Thinking?

Brownell’s characterisation (Ibid.) of exercises , problems and puzzles encouraged psychologists and mathematics teachers to focus their research on the study of arithmetic problem solving . Polya (1945) expanded problem solving to other levels of education , thus promoting the emergence of a new paradigm . Some mathematics educators followed this trend and contributed their own new theoretical approaches (RME through the influence of Freudenthal; Mason et al. 1982; Schoenfeld 1985; Santos-Trigo 2010). Returning to primary school level , for example , Vergnaud’s work (1990) on solving arithmetic problems led to the identification of both arithmetic in problem solving and conceptualisation in primary school, and led to the theoretical approach related to “conceptual fields”.

Similar approaches led to some research products in order to characterise arithmetic thinking . For example , Verschaffel and De Corte (1996), taking into account the research conducted in the 1990s, propose arithmetic thinking related to: (a) number concepts and number sense ; (b) the meaning of arithmetic operations ; (c) control of basic arithmetic facts; (d) mental and written arithmetic; and, (e) word problems using digital literacy and arithmetic skills .

While progress was made in the study of learning problems linked to the resolution of arithmetic problems , research continued toward an understanding of the problems related to learning algebra (Booth 1988). The notion of variable began to be studied (Sutherland 1993), thus promoting investigation into the learning of covariation between variables (Carlson 2002) and the identification of the role of the variable as an unknown , as a large number , and as a variation between variables from a functional point of view (Trigueros and Ursini 2008).

Given the organisation of the curriculum , which designated arithmetic for primary school and algebra for secondary school, researchers began to talk about the problems related to the transition from one level to the other. At the same time , the emergence of the notion of epistemological obstacle in the French school (Brousseau 1976/1983) possibly reinforced this idea of a “break ” between arithmetic and algebra . Vergnaud (1988) points out that the transition from arithmetic to algebra is linked to an epistemological obstacle . Other approaches, related to the notion of the unknown in solving linear equations , led to the notion of a “cut” between arithmetic thinking and algebraic thinking (Filloy and Rojano 1989) or even the cognitive obstacle (Herscovics and Linchevski 1994). These studies announced the need to characterise algebraic thinking.

What Is Algebraic Thinking?

As mentioned in the previous section , the research paradigm linked to the “thinking break ” between arithmetic and algebra was essential for the characterisation of algebraic thinking. Under this paradigm , Kieran (2007) characterises algebraic thinking using a model called GTG_m: Generational algebraic activities involve the forming of expressions and equations ; Transformational activities such as factoring, expanding , and substituting; and, Global /meta-level mathematical activities such as problem solving and modelling. An analysis of this model reveals that, in the past, much of the secondary school level research focused on the teaching of algebra in section G and T of Kieran’s model . It is likely that the G_m section is linked to the Freudenthal School’s research results regarding realistic mathematics , at the heart of which approach is mathematical modelling .

Visual Aspects in Curricular Change in Mathematics

In the early 1990s, an important curricular change in the field of mathematics began. The visual aspects were highlighted in curriculum changes, promoting displays of the mathematical aspects. A clear example can be seen in the US Standards (NCTM 2000). In this context , geometric aspects in problem solving began to be included in algebra . It was explicitly important to approach a concept through the use of different representations of that concept. From a curricular standpoint as well as from a general standpoint related to research in mathematics education , mathematical visualisation has attracted the attention of researchers. These changes began from a curricular perspective , with a new approach to teaching algebra and the promotion of a geometric-algebraic approach to algebra . Progressing along this research line , for example , Zimmermann and Cunningham (1991) begin the preface of their book with the question : What is visualisation in mathematics? This study explicitly referred to an important role in the production of external representations:

Mathematical visualization is the process of forming images (mentally, or with pencil and paper , or with the aid of technology ) and using such images effectively for mathematical discovery and understanding. (p. 3)

Technology influenced enormously in these changes. Graphical representations that caused major programming problems were resolved, thus giving rise to the production of computer software and enabling an approach to mathematics from the multiple representations user standpoint .

Early Algebra and the Emergence of a New Paradigm

While the previous section discussed the “rupture ” approach to characterise arithmetic thinking and algebraic thinking, little by little other research programs arose, which were initially tied to the idea of the “generalization of arithmetic” (see Mason 1996; and Lee 1996). Along these lines, Radford (1996) comments how these authors stressed an approach to the learning problem regarding “algebra as a generalised arithmetic”, and goes on to discuss the role of the unknown and the equation :

The above discussion suggests that the algebraic concepts of unknowns and equations appear to be intrinsically bound to the problem -solving approach, and that the concepts of variable and formula appear to be intrinsically bound to the pattern generalization approach. Thus generalization and problem solving approaches appear to be mutual complementary fields in teaching algebra . How can we connect these approaches in the classroom ? I think this is an open question (p. 111).

From this perspective , a new paradigm was born. Kaput (1995, 2000) proposes a research program under the following guidelines , with the first two at the heart of the learning of algebra and the other three completing this learning:

• 1. (Kernel) Algebra as a generalization and formalization of patterns and constraints , with, especially, but not exclusively, Algebra as Generalized Arithmetic Reasoning and Algebra as Generalized Quantitative Reasoning

• 2. Kernel) Algebra as syntactically guided manipulations of formalism

• 3. (Topic-strand ) Algebra as the study of structures and systems, abstracted from computations and relations

• 4. (Topic-strand ) Algebra as the study of functions, relationships and joint variation

• 5. (Language aspect) Algebra as a cluster of (a) modelling and (b) Phenomena-controlling languages. (2000, p. 3)

Kaput called this research program Algebrafying the K-12 Curriculum (2000), while Carpenter et al. (2003, 2005) initiated the Early algebra research project in 1996. This new paradigm formed part of research programs in the twenty-first century . Thus, the Early Algebra movement , in which Carpenter and Kaput played an important early role, is well situated in the USA. The book Early Algebraization , edited by Cai et Knuth (2011), shows the progress of research in that area in other countries. In this book, one can appreciate a division between the “enthusiastic” and “cautious” researchers with regard to the Early Algebra movement.

Among the enthusiasts are Blanton and Kaput (2011), as are Britt and Irwin (2011, p. 139), who even criticised Filloy and Rojano’s approach by highlighting Carraher et al. (2006) with regard to their Early Algebra proposal . Similarly, Schliemann et al. (2012) show how algebraic notation can be introduced in elementary school in order to develop mathematical content , stating: “The 5th grade lessons focused on algebraic notation for representing word problems , leading to linear equations with a single variable or with variables on both sides of the equal sign .” (p. 115).

Among the cautious, are Cooper and Warren (2011), who argue that:

The results have shown the negative effect of closure on generalisation in symbolic representations, the predominance of single variance generalisation over covariant generalisation in tabular representations, and the reduced ability to readily identify commonalities and relationships in enactive and iconic representations. (p. 187)

In this regard , Radford (2011, p. 304) states that: “... the idea of introducing algebra in the early years remains clouded by the lack of clear distinction between what is arithmetic and what is algebraic”. On this, the debate remains open, for example , Lins and Kaput (2004) characterising the movement as:

…algebrafied elementary mathematics would empower students, particularly by fostering a greater degree of generality in their thinking and an increased ability to communicate that generality. (p. 58)

As spokespersons for the Early Algebra working group at ICMI 12^th (Lins and Kaput 2004), they openly criticised past generations, whose results were exclusively related to “sad histories”, and specified that, in contrast, the Early Algebra movement presents research results linked to “happy stories” regarding the experience of learning algebra content .

The Third Excluded Strikes Back!

In light of the research results described above, this study approached the problems of learning algebra by introducing new variables that could not be left out of the discussion. As new theoretical approaches about learning algebra are born, so are different general learning paradigms. Research in the last century was strongly cognitivist, with Harel et al. (2006), surprised to learn that, in the PME studies on the period of 1995–2005, most of the investigations related to Advanced Mathematical Thinking were much more cognitive and less socio-constructivist or sociocultural . While communication in the mathematics classroom emerges as an essential element, the literature begins to show that researchers are inclined towards a socio-constructivist or sociocultural approach.

Our Theoretical Approach to an Introduction to Learning Algebra

The research objectives for this study are founded on a cultural approach, which takes into account the theory of activity and which views communication in the classroom as essential. The work of Engeström (1999) is taken as a culturally unifying approach, as advocated by Vygostky (1962), incorporating Leontiev’s (1978) activity theory , in which communication is an essential element in the building of knowledge as described by Voloshinov (1973). Our approach to Radford’s processes of signification , is immersed in a mathematics classroom teaching method named ACODESA (see Hitt 2007; Hitt and González-Martín 2015; Hitt et al. 2017), that also take into account a self-reflection component.

An analysis of the literature on the followers of the Early Algebra movement shows that some research is aimed at building a “Fast Track” from arithmetic to algebra . This study posits that a functional approach to algebra should be followed , such as that developed in both Passaro (2009) and Hitt and González-Martín (2015). This study concurs with some followers of Early Algebra, in that the use of patterns is able to generate generalisation processes in pupils , and, thus, proposes, in the context of the use of patterns, the following:

• Generalisation . Construction of the subsequent term in a series when the previous terms are provided. Construction of an intermediate term when the previous and subsequent terms are provided. Construction of a term when the term in the series is a “large number ” and when the first terms of the series have been provided. Construction processes for “any term from the series .”

This study considers generalisation in the context of a pattern, where, rather than as a way of moving quickly from arithmetic to algebra , it is an element used to integrate into the pupils ’ mathematical structure. This will enable the pupils to develop the skills of prediction , argumentation and validation (Saboya et al. 2015), and will assist them in their transition from arithmetic to algebra and vice versa. Indeed, a research program is proposed here that would develop arithmetic-algebraic thinking within a sociocultural context of knowledge construction.

As described above, this chapter, seeks to make a modest contribution , in that it represents the beginning of a research program. Our research is focused on specific content related to the construction of polygonal numbers in a sociocultural environment within Engeström’s post-Vygotskian model (1999), and takes into account the results of those post-Vygotskian authors considered as comprising the fifth generation , such as Nardi (1997):

The object of activity theory is to understand the unity of consciousness and activity. Activity theory incorporates strong notions of intentionality, history, mediation, collaboration and development in constructing consciousness. (p. 4)

As the use of technology in the learning of mathematics is a variable yet to be mentioned here, Mariotti’s (2012) work on the role of artefacts as mediators in a learning process is integral to the inclusion of technology in a sociocultural environment.

The use of patterns , and especially the construct of generalisation , is related to mathematical visualisation . Visualisation , as mentioned by Duval (2002), is different from perception. In our case, then, the following applies:

• Visualisation . Considering perception as something created by the individual – a “transparent” mental image depicting the situation with which s/he are faced – visualisation requires the transformation of representations associated with the task at hand, and the ability to articulate other representations that emerge in pupils ’ resolution processes , as associated with the task.

This study is not only interested in institutional representations (which can be associated with a register of representations, as described by Duval 1995). It is also concerned with the non-institutional semiotic representations that can be produced in a visualisation process (diSessa et al. 1991; Hitt 2013; Hitt and González-Martín 2015; Mariotti 2012) and which emerge in a semiotic process of signification (Radford 2003) when pupils follow a process of resolving a mathematical activity immersed in a technological setting .

• Institutional representation. Representation found in textbooks , computer screens or those used by the mathematics teacher.

• Non-institutional representation. Representation produced by pupils , as linked to actions undertaken in a process of resolving a non-routine activity different of the institutional representation.

Since the method proposed here is related to polygonal numbers and the use of technology , ideas related to the construction of polygonal numbers that date back to the time of the Greeks were considered here. Furthermore, including technology as one of the variables led to the inclusion of Healy and Sutherland (1990) and Hitt (1994), who, in Excel environments and Excel and LOGO environments, respectively, conducted investigations into the construction of polygonal numbers by secondary school and pre-service teachers respectively.

Healy and Sutherland (Ibid.) mention that the result obtained by those secondary level pupils (in the Excel environment) that expresses a relationship linked to the calculation of a triangular number “n”, “trig. Δn = na before + position”, is a non-institutional representation linked to a process of iteration . Hitt (ibid.) criticises these results, indicating that activities in an exclusively Excel environment provoke “an anchor” which does not allow them to switch to a classical algebraic context . Hitt (Ibid.) aimed to combine working with paper and pencil with the use of an applet constructed using the LOGO program. In light of these results and considering new theoretical and curricular contributions , both approaches are of contemporary importance , in that they generate diversified thinking for the production of non-institutional representations and iteration processes . Secondly, they enable the careful design of activities that promote the use of paper and pencil , while also fostering the evolution of the non-institutional representations that emerge at the initial stage into institutional representations within meaningful processes (a broader discussion on task design is provided in chapter “Task Design in a Paper and Pencil and Technological Environment to Promote Inclusive Learning: An Example with Polygonal Numbers” of this volume ).

Methodology

Our research was developed within two populations , with one group from Quebec comprising 13 first grade secondary school pupils (aged 12–13 years old), and the other from Mexico, which consisted of 14 third year secondary school pupils (aged 14–15 year-old). Pupils agreed voluntarily to take part in the experiment, which aimed to gain insight into the problem , as occurring in the two populations individually, rather than comparing results.

• The Quebec experiment used Excel and an applet called POLY (see below), which had been designed exclusively for this activity (Cortés and Hitt 2012). Two researchers, known here as R₁ and R₂, developed the teaching experiment in a sociocultural setting . Two cameras and several voice recorders were used in this experiment.

• The Mexican experiment used a calculator (TI -Nspire) instead of Excel and the POLY applet . The activities were developed by one teacher, known here as P₁, and another researcher, known here as R₃. One camera was used in this experiment.

This study adheres to a teaching method known as ACODESA is divided into 5 steps (fully explained in chapter “Task Design in a Paper and Pencil and Technological Environment to Promote Inclusive Learning: An Example with Polygonal Numbers”):

• Individual work: production of official and non-official representations related to the task.

• Teamwork on the same task. Process of prediction , argumentation and validation .

• Debate (could become scientific debate ). Process of argumentation and validation .

• Self-reflection (individual work in a process of reconstruction )

• Process of institutionalisation.

Engeström’s (Ibid.) model was used to organise the ACODESA steps, taking into account a sociocultural learning setting . In previous research undertaken by these authors (see Hitt 2007; Hitt 2011; and, Hitt and González-Martín 2015), the self-reflection step was implemented immediately after plenary discussion . Due to problems of knowledge retention (Hitt and González-Martín idem; Karsenty 2003; Thompson 2002), for this experiment we decided that for the self-reflection step, it would be interesting to implemented 45 days after the plenary debate.

The two first activities were implemented as an introductory activity in order to remind pupils of some of the Excel commands and provide a historical approach to polygonal numbers . The ACODESA method was implemented after the two previous activities.

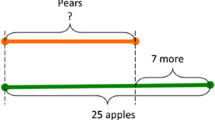
• 1. Resolution of two arithmetic word problems in a paper and pencil setting and a plenary discussion about how, according to the population, to solve them with either Excel or a calculator . This was implemented to remind pupils how to use Excel or a calculator.

• 2. Introduction to polygonal numbers from a historical point of view.

• 3. Invitation to the populations to solve the activity in line with the ACODESA characteristics shown in Fig. 1.

  Fig. 1

  

  ACODESA , as immersed in activity theory in line with Engeström’s model

The tasks were used in both countries with only a few changes.

Fig. 2

First five questions in a paper and pencil environment

The second part of the activity was designed to work with Excel or CAS and to be verified with the POLY applet .

Analysis of the Quebec Results

The first introductory part of the session comprised the individual resolution of the two word arithmetic problems using paper and pencil , and a plenary discussion about how to solve the same problems using Excel . Researcher R₁ conducted the plenary discussion, immediately after which Researcher R₂ conducted a short historical introduction to polygonal numbers and then initiated the first part of the ACODESA activity related to polygonal numbers. In this first step, individual work was required, as was work in a pencil and paper environment.

Once the pupils had undertaken the first individual explorations, R₂ organised the teamwork , with Team G₁ comprising three girls , Team G₂ comprising 3 girls, Team G₃ comprising 3 boys and a girl, and Team G₄ comprising a boy and two girls. Only one computer was permitted for each team.

Teamwork and the First Results

After pupils exchanged ideas , R₂ requested a plenary discussion , asking each team to present its findings on how to calculate the 11th Triangular number (T₁₁). Three teams (G₁, G₂ and G₄) presented their findings, while members of Team G₃ mentioned that their strategy was similar to the first team (see Fig. 3).

Fig. 3

Second part of the activity

An initial and surprising outcome was the emergence of three different strategies. Their initial production (see Fig. 3) indicates that the pupils have undertaken a process of visualisation . They realised that it is possible to move along the diagonal , adding balls progressively, while one can add to the number of balls along the diagonal in an arithmetic progression . Pupils presented the first three iconic figures with their respective values and a process of generalisation in order to calculate T₁₁ (see Fig. 4).

Fig. 4

The representation used by Team G₁

It seems that these pupils have undertaken a visualisation process in order to construct a general numerical progression . The action of adding balls along on the diagonal is transformed by adding the number of balls to the arithmetic progression, thus abandoning the iconic representation used to calculate the 3rd triangular number .

Team G₂ presented the results of their calculation of T₁₁ with a single figure, indicating that, in the first column, one should place 11 balls, and then reduce the number of balls in the next column by one (10) in order to reach, at the end, only one ball, imagining the 1st column with 11 balls, the next with 10, and so on (see Fig. 5). An initial process of visualisation and generalisation is then made with only one drawing, thus inducing a numerical process which is inverted, as compared to that presented by the first team in Fig. 5. Team G₂’s visualisation process is more compact than Team G₁, in that the team members made a direct iconic representation of T₁₁, expressing their process arithmetically. This example is generic in that they were able to represent any triangular number under this visual representation.

Fig. 5

The representation used by Team G₂

Team G₃ mentioned that they had a similar approach to Team G₁. A boy from Team G₄ (named G₄-1 hereafter) then approached the blackboard . From his first representation onwards, this pupil substituted the iconic ball-based representation for a more practical one, explaining that whenever one passed from one triangular number to the next, one had to add the appropriate number (see Fig. 6, below and left ).

Fig. 6

The representations used by Team G₄ to calculate T₁₁

While giving his explanation, he suddenly changed the strategy without discussing this with his team members. He changed the representation he was using to calculate T₁₁ for one which enabled him to construct both an iterative process to calculate T₁₁ and a generic algorithm for any Triangular number (see Fig. 6). It seems that, through a process of signification (Radford 2003), the pupil was constructing a sign that enabled him to arrive at an iterative process for the calculation of triangular numbers.

An analysis of the pupils ’ written productions reveals that there were pupils in each team who undertook iconic calculations solely counting ball by ball. One female member of Team G₃ said nothing in response to the “leader” of the group indicating that they had done something similar to Team G₁, when in fact she had actually done something similar to Team G₂.

Process of Generalisation

R₂ then requested that teamwork continue, approaching team G₄ and mentioning that, when undertaking a calculation , they should show their working. A female member of Team G4 (known as G₄-2) interjected by saying that she did not understand how to calculate T₈₃, thus initiating a dialogue between G₄ and R₂, with G₄-1 and G₄-2 mainly involved in the discussion.

R₂ :

You must calculate it ... and show what you did.

(...)

G₄-2:

I do not understand.

G₄-2:

I do not understand.

R₂ :

Well, here we do not tell you the number of....

G₄-1:

Is this number related to the diagonal ?

G₄-2:

The number on the side ?

(...)

G₄-1:

I take 83 on the side or on the diagonal and then you can count 1, 2, and 3 up to 83.

R₂ :

It’s interesting, you have two different strategies.

In this excerpt, trying to assimilate that which was presented to the whole group by her teammate G₄-1, G₄-2 ceases to refer to the iterative process, instead only associating the number of balls, either vertically , at the base , or on the diagonal , and, thus, jumping from one triangular number to the next.

Once the pupils had worked in teams, R₂ again requested a process of recapitulation in a large group discussion, asking pupils how they would perform the calculation of the triangular number T₈₃ (Fig. 7).

Fig. 7

Dialogue between R₂ and the group in a plenary session

This extract is extremely important to the research conducted in this study. Pupils proposed a calculation of T₈₃ that is identical to that proposed by Team G₁ for the triangular number T₁₁ – namely T₈₃ = 1 + 2 + 3 +  ⋯  + 83. R₂ tried to verbalise the calculation in terms of a generalisation for any triangular number . Pupils had no difficulty with this kind of process of generalisation. The symbolic process was executed naturally, with the assignation of a variable seeming not to disturb pupils at all. Even when the researcher proposed the use of a heart (♥) as a variable, this did not appear to disrupt the pupils in any way.

While, right up until this point , it is possible to say that pupils have been following various processes of generalisation , the question remains as to who, precisely, undertook this process. Throughout this process, pupils generally seemed to show that there was consensus . How stable was the pupils ’ knowledge as it emerged from a process of communication in the mathematics class? Can these pupils retain these results in the future?

Once this part of the activity had been completed, R₂ asked the pupils to return to work in teams, suddenly announcing “I can calculate any triangular number with three operations . Can you?” This was a question that resonated with some pupils , as described below.

Generalisation and Emergence of the Concept of the Variable

At this stage , pupils could use Excel in order to continue the activity. The idea of using a single computer introduced an unanticipated variable. The owner of the computer determined the user. For example , in Team G_3, the owner of the computer (a boy) was the only user. This reminded researchers of Hoyles’ (1988) recommendation that attention should be paid to the constitution of a team when mixing boys and girls in a computational setting .

Once teamwork had commenced, pupil G₄-1 called R₁ over , saying that his group had formed a strategy to calculate any triangular number . He mentioned that their strategy involved taking any triangular number , for example 101, adding 1, dividing by two and multiplying the result by 101. R₁ told them to use another number , such as100. Then, G₄-1, stating that he would add one to 100 and divide the result by two, suddenly stopped, turned to his companion (G₄-2) and asked whether it made sense to get a decimal number when dividing by two. R₁ suggested that they discuss their strategy again and use the Poly applet to verify their results. R₁ remarked that the team had used Excel to calculate the triangular numbers up to T₈₄ in a column and that they had the operation (indicated in Fig. 8) in their workbook.

Fig. 8

Technology and paper and pencil – the construction of a strategy

This is another key episode in this research . Pupils already had an algorithm to calculate T₈₃, which was to add 1 + 2 + 3 +  ⋯  + 83. It was G₄-1who put the iterative algorithm on the board (see Fig. 6), using it with Excel to calculate up to T₈₄. The hypothesis explored in this study is that, using T₈₃ and T₈₄, the pupil built his new algorithm.

Fig. 9

Construction of a general strategy to calculate any triangular number

Both the design of the activity and the pupils ’ processes show the possibility of reconciling work with pencil and paper and technology . In addition , and very importantly, the team destabilization generated by R₁’s question about using an even triangular number (T₁₀₀) caused the team to reflect on that which is expressed by the use of the letter “x” in the arithmetic operations (see Fig. 9). It seems that pupil G₄-1 had written 100 + 1 divided by two (“no matter what you get”), in this case x, the result (x) must be multiplied by 100. Finally, the pupils used the POLY applet to ensure that the conjecture obtained might work with other triangular numbers.

The POLY applet is able to show a series of polygonal numbers or to give a specific polygonal number (as in Fig. 10). Due to screen limitation problems , if a polygonal number is too big, the applet can only provide the numeric result and thus excludes the figure.

Fig. 10

Examples using POLY (series of triangular numbers and the fifth pentagonal number , including partition into triangles if required)

Discovery of an Algebraic Expression for Calculating any Polygonal Number

The session was ending and R₂ called for a plenary discussion . G₄-1 asked to present what his team had found. G₄-1 exemplified their strategy with T₄₆ and explained their algorithm . After R₂ explicitly asked him to explain how they had discovered their strategy, he repeated the algorithm, but did not mention how the discovery was made. It seemed that he did not understand R₂’s question .

When the bell rang and R₂ had finished the session , a girl’s voice , almost drowned out by the noise made by the pupils , indicated that she would have liked to know how the three operations could be used to calculate any triangular number . R₂ mentioned that there was no time for that explanation and that she would show it to her later. However, as G₄-1 mentioned that he knew this, R₁ and R₂ asked him to write it on the blackboard , even though the entire class was on their feet and ready to leave the classroom , whereupon G₄-1 wrote the following algebraic expression (see Fig. 11).

Fig. 11

Symbolising in a communication process

The researchers reacted very positively to this development at the end of this stage of ACODESA , deciding at that time to interview G₄-1 to obtain more information about the process of constructing the algebraic expression . The interview provided a few elements of which researchers were already aware. While G₄-1 insisted that it was through the POLY applet that he had come to discover the formula , both pupil productions (Fig. 9) and R₁’s discussion with Team G₄ seem to indicate that the discovery took place while working with either Excel and pencil and paper , with Poly allowing them to check their conjecture .

Self-Reflection Phase Without Technology. What Happened 45 Days Later?

Aware of the problem with student retention of the mathematics that they learn and also aware, thus, that “consensus is ephemeral ”, the authors decided to make the self-reflection phase as different as possible to that which had had been undertaken in other experiments. Also, given that a talented pupil had been discovered in the sample, it was decided that an additional challenge would be added to the self-reflection activity (which, while generally the same , excludes technology ) exclusively for him. So, in addition to the reconstruction process related to triangular numbers, he was asked to work with pentagonal numbers, something which was not dealt with in the classroom experiment.

It seems that G₄-1 did not pay attention to the examples given about triangular numbers, as he wrote that he already knew the formula to calculate any triangular number . He applied a wrong formula and did not check his results against the examples provided. However, and to the researchers’ surprise , in a paper and pencil task (the use of technology is not allowed in this phase) that followed a similar process of finding relationships among the first four examples provided for pentagonal numbers, he constructed both the fifth pentagonal number and a general expression that allowed him to calculate any pentagonal number .

Fig. 12

G₄-1′s production in the self-reflection phase

The results obtained are presented below. The data was collected on an individual basis before the teamwork and self-reflection phase 45 days later, with only eight pupils sampled from the other phases, plus others that could not be taken into account . Pupils 1–13 (the last being G₄-1) were identified in order to observe progress and setbacks.

Table 1 shows two setbacks and four advances , not counting pupil G₄-1, who used an incorrect algebraic expression to calculate the triangular numbers. The female pupil (subject 11) followed her teammates from Team G₃ passively .

Table 1 Results from the ACODESA self-reflection phase after 45 days

While her strategies were different, she did not discuss them with her teammates. The owner of the computer in team G₃ became the leader, which corresponds to Sela and Zaslavsky’s research (2007) with four people working together. This male pupil, when using the computer, showed his colleagues various things not related to the task, thus creating a situation unrelated to the requested task. Teams G₁ and G₂ were more homogeneous and presented more balanced participation, with both teams composed of girls . The computer owner from team G₄ was strongly committed to the task and adapted very quickly to the rhythm of his colleagues , with one of the setbacks for the team posed by G₄-2 (G₄-3 was not present during the self-reflection phase). More careful study is required to analyse the role of technology in sociocultural learning. In fact , this, bearing in mind Hoyles (1988) on Girls and computers and the results reported by Sela and Zaslavsky (2007), leads to the realisation of the importance of creating teams consisting of a maximum of two or three subjects , and of trying to balance the use of technology in each team.

Experiment Conducted in Mexico

The experiment conducted in Mexico proceeded as follows. Once the initial problems were resolved in order to introduce pupils to the TI -Nspire calculator , the teacher asked the pupils to work on the first five questions individually (see Fig. 2). Four teams with three pupils and one team with two pupils were formed, and in which each pupil had a calculator (TI-Nspire).

Individual Work

Two types of strategies emerged from the individual work – one linked to the drawings as shown in the examples, and the other to the formation of a table of values. Again, spontaneous representations linked to functional representations appeared in the communication process (Fig. 13).

Fig. 13

Spontaneous representations used by one pupil

Teamwork

One team made up of Diana, Karla and Omar underwent a reconciliation of strategies, with Diana and Karla making the calculation by counting balls from the drawings, while Omar used a table of values. Through this strategy integration process, Diana and Karla left their strategy and decided to use the table of values.

The pupils ’ individual productions and the film of the plenary session are the only evidence of the individual work carried out by the subjects . A useful research technique was that pupils were asked to write with red ink when working in teams (see Fig. 14).

Fig. 14

Karla’s individual work and teamwork

There is no evidence of how the formula was obtained . The formula appeared in Karla’s productions and was written in red ink during the teamwork phase. This shows that, in her team, she adopted the table of values and proposed an algebraic expression . At this secondary level pupils had already learned about the notation of variables, and can clearly be seen to use the variables x and y. The formula enables pupils to estimate the calculation of polygonal numbers . Our hypothesis is that having used y = x²/2 (related to base * height/ 2) and having realised that it did not work with the examples given in the table, these pupils decided to approximate the results, thus giving y = 0.52 * x ². They did not present their proposal in the large group discussion.

A surprising result is that many Mexican pupils participating in this research immediately associated the triangular arrangement of the triangular numbers, base * height/ 2, with the calculation of the area of a triangle, thinking that this was the algebraic expression required.

This was the first thing that emerged during the large group presentation, having been captured from the beginning of the pupil discussion.

Plenary Discussion

Monica, facing the blackboard , gave as example the T₈ (see Fig. 15), describing the following as necessary in order to calculate it.

Fig. 15

Monica presenting the calculation of T₈

R3 intervened, saying that, at this point , it was necessary to review her theory. She was then interrupted by the pupil Rob.

Rob:

... But... this, the triangular number 8 would be 36 and not 32 – then it cannot be.

R₃ :

Please come to the blackboard Rob.

Rob:

This is number 6 [pointing to the figure just produced by María]. We apply the formula that says the base multiplied by height would be 6 by 6 giving 36 divided by 2, giving us 18, while the triangular number would be 21. This, therefore, is not the formula [points to the base * height/ 2 formula].

P₁ :

What is the difference between. .. if you apply the formula that tells you, apply the formula. Write it there.

María:

[Writes the formula ].

P₁ :

In this case, what is the basis?

Rob:

[Writing] 6 * 6/2 is 18 and there is the triangular number .

P₁ :

What is the triangular number ?

Pupils:

21 [answering chorus]

Rob:

The difference would be 3.

P₁ :

The difference would be...?

Rob:

3.

Rob:

calculates the Triangular 8, and, typing the formula , obtains 32.

P₁ :

And what is it for the triangular number 8?

Rob:

It’s 36.

P₁ :

The 8?

Rob starts counting the balls for the figure that was already on the blackboard , and says that it is 36.

Pupils:

The difference is 4.

Rob realizes that the formula does not work and that he has shown a counter-example (similar to the comment made by the unidentified girl). However, so far he is not able to build the exact formula for triangular numbers.

EUREKA! Rectification of the Formula in a Scientific Debate

In the midst of the discussion, a surprised voice is heard, saying, “and from 8 it is four, then it would be half.”

Gaby:

Half of 8 is 4, and 4 is what is missing from 32 to 36 in the formula , then we have to put the base multiplied by height divided by two more.... [PAUSE] plus the half of [PAUSE] plus the half of the triangular number , half [PAUSE] half of the base .

While speaking, Gaby paused several times while she completed the transformation of her numerical idea into a geometric -algebraic idea . It is clear that the control element was provided by the arithmetic relationship and the transformation from that into a geometric relationship. However, something else occurred in the process of communication when Gaby was verbalising what she was thinking: there was a process of deduction. At this very moment, the pupils were rejecting their initial conjecture in favour of a new one, using the arguments to refute the conjecture.

P1:

Write it!

Pupil:

I think that we, all together, are arriving at something, not alone!

The sociocultural construction of knowledge has occurred at this stage of scientific debate , in accordance with ACODESA . The pupil openly expresses the co-construction generated through the debate.

Gaby goes to the blackboard to write the idea that she had just thought of.

Gaby:

How do I represent half the base ?

Interestingly, at this point , Gaby has difficulties in transforming the geometric argument “half the base ” into algebraic terms :

R₃ :

… One half, or that in half.

Gaby writes $\frac{b\times h}{2}+\frac{1}{2}$.

Many pupils worked together to undertake the final writing activity. In this process, Gaby was guided by her colleagues because she did not fully understand the process of algebrafying “half the base ”. Finally, she wrote: $\frac{b\times h}{2}+\frac{b}{2}$

P₁ :

And how do you represent the triangular number in that formula you are writing there? How do you represent the triangular number ?

Pupils:

.... The base or the height...

Gaby writes the formula $\frac{b\times h}{2}+\frac{b}{2}$.

P₁ :

Precisely , what she said, the height is the same as the...

Pupils:

The base .

P1:

Replace it and do not write the height.

Pupil:

It would be base times base .

Gaby misspelled the formula and was corrected by her peers, after which she wrote: $\frac{b\times b}{2}+\frac{b}{2}$.

P₁ :

Ok then, base times base is what?

Pupils:

Base squared.

Gaby writes finally the formula $\frac{b^2}{2}+\frac{b}{2}$.

P₁ :

Do you think that is the formula ? Verify it with the triangular number 15

Gaby:

Do I have to count the balls in a drawing?

P₁ :

No, no, no you have already got the formula !

Self-Reflection Phase Without Technology. What Happened 30 Days Later?

This phase, referred to here as self-reflection without technology , comprised a questionnaire (with slight modifications) similar to that completed by the participants 30 days previously. Only 10 of the 14 pupils participated, with the main idea at this stage being a reconstruction of what had been undertaken in the classroom .

The questionnaire for the self-reflection phase had three questions:

1st Question:

Calculate the 27th triangular number .

2nd Question:

Write the formula for calculating any triangular number .

3rd Question:

Using your formula , calculate the 313th triangular number .

The results are as follows: from the ten pupils , two continued the process of “drawing balls and counting”, while four of the ten rebuilt a similar expression related to the area of a triangle – b × h/2. A pupil was able to reconstruct the formula , but mistook the result of calculating T₂₇ by finding a triangular number to provide 27 as a result, with the closest being T₇ = 28. It is possible that he made a mistake when counting the balls. He followed the same strategy when calculating T₃₁₃. Among those who were able to reconstruct the right formula were Alejandra, Omar and Rob.

Conclusions

These results reveal the importance of building arithmetic -algebraic thinking in order to support algebraic thinking. The experiment conducted in Quebec with 1st year secondary pupils (11–12 years old) revealed the following:

• It was performed in a sociocultural environment, with a gradual construction of the concept of the variable, and patterns built from the visual work.

• The strategy consisted of visualisation processes that related drawings, arithmetic addition series , iterations and formulas. Pupils used natural language with letters representing variables.

• The validation process was supported by the use of technology .

• The availability of a device on the table , shows that its use is delicate (Hoyles 1988). In the case of one team, it was the owner of the computer who exclusively used it. In the team with a boy and two girls , the boy mostly used his computer, the girls used it when he was at the blackboard .

• Even though there was more progress than setbacks in the self-reflection phase, the results show that concluding that consensus had occurred should be undertaken with caution.

The experiment conducted in Mexico with 9th grade secondary pupils (14–15 year old) revealed the following:

• It was performed around visualising a process related to the area of a triangle, with use of variables to represent the variation (x, y, b, h).

• The validation process rested more on visual configurations.

• The technology was not widely used by the pupils . Plenary discussion and co-construction attracted the pupils ’ attention .

• Again, it showed that “ consensus is ephemeral ”, with only four out of ten able to rebuild the formula and one of them mistaking the number of the triangular with the result .

A surprising fact is that the institutional representation n (n + 1)/2 did not emerge in neither of the two populations . This demonstrates the importance of pupils ’ spontaneous representations in the construction of mathematical concepts (Hitt 2013; Hitt and González-Martín 2015). This reveals that evolution of spontaneous representations is important in a signification process. During the institutional stage under the ACODESA model , the teacher must collect different ideas and productions and relate them to the institutional representations.

Our research is taking into account the importance made in the 40s when psychologists payed attention to the importance to moving from analysis of pupils ’ performances when solving puzzles to the analysis of pupils ’ problem solving activity (see Brownell 1947). Our approach, in this technological era goes from an arithmetic context to an algebraic one, in a natural way using technology as a tool in a process of generalization and, in a sociocultural context of learning. In this way, pupils construct arithmetic relations (product of this is what is shown in Fig. 9) that permit them to control their process of generalization to an algebraic context in a milieu of creativity and autonomy (see Fig. 12). The results are showing the importance to promote the production of spontaneous representations and conversions among them even if they are not the institutional representations. This contrast directly with Kirshner’s approach (2000) concerning his ideas of exercises , probes and puzzles ; and, about his restricted approach to learning algebra focusing on the algebraic register about visually salient rules (Kirshner 2004). Our research takes as central a task-design where the but is related to enchained tasks, to be solved by the pupils in a sociocultural milieu, and the teacher role is to promote students’ reflexion and productions of spontaneous representations, not only the algebraic one.