Abstract
During the forming of flat sheet metal into a more complex shape, a number of plastic instabilities may occur subsequently. A ‘plastic instability’ occurs when the zone of plastic deformation is suddenly confined to a smaller zone. The first plastic instability which usually occurs in forming processes is the onset of diffuse necking, in which plastic deformation is confined to a smaller zone, but with typical dimensions that are still in the order of magnitude of the part’s dimensions.
The original version of the chapter was revised: The erratum to this chapter is available at 10.1007/978-3-319-44070-5_8
Access provided by Autonomous University of Puebla. Download chapter PDF
Similar content being viewed by others
Keywords
These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.
5.1 Failure in Sheet Metal Forming Operations
During the forming of flat sheet metal into a more complex shape, a number of plastic instabilities may occur subsequently. A ‘plastic instability’ occurs when the zone of plastic deformation is suddenly confined to a smaller zone. The first plastic instability which usually occurs in forming processes is the onset of diffuse necking, in which plastic deformation is confined to a smaller zone, but with typical dimensions that are still in the order of magnitude of the part’s dimensions. In common industrial practice, the presence of a diffuse neck in a formed part is considered to be acceptable (Dieter 1988). The ‘formability’ or ‘forming limit’ is thus determined by the onset of another type of plastic instability. For most materials and forming processes, this plastic instability is localized necking and so the terms ‘formability’ and ‘forming limit’ have been associated with localized necking in the literature.
In the next paragraphs, the different sequences of plastic instabilities which were found in the literature are described in more detail, giving an overview of the possible stages in the failure process. In each case, the final step for metal sheets, which are all ductile materials, is the onset of ductile failure, i.e. the coalescence of voids (resulting from the processes of void initiation and growth).
5.1.1 Diffuse Necking—Localized Necking—Ductile Fracture
As often observed in uniaxial tensile tests of sheet metal, diffuse necking is followed by localized necking. While the size of a diffuse neck is of the order of magnitude of the sample width, the width of the localized neck is only of the order of the sheet thickness (its length being of the order of magnitude of the sample width). After the onset of localized necking, strain is concentrated within the neck while the surrounding material returns to the elastic state. Consequently, the thickness within the neck drops drastically compared to the elastic surrounding. Localized necking is therefore also known as thinning instability. In the developed localized neck, a plane strain state exists with zero extension along the neck length (Marciniak and Kuczynski 1967).
After the onset of localized necking, the failure process can continue with ductile fracture through void coalescence within the neck, resulting in a cup-and-cone type of fracture in the terminology of fractography.
5.1.2 Diffuse Necking—Localized Necking—Shear Instability—Ductile Fracture
As shown in Bird et al. (1987) and Timothy (1989), the appearance of a macroscopic shear localization (over multiple grains) within the developed neck is possible, which is illustrated in Fig. 5.1. In Bird et al. (1987) and Carlson and Bird (1987), it is observed that shear localization initiates at the free surface within the neck, and that multiple shear bands can be found within a single localized neck.
Through-thickness section of ferrite-austenite steel deformed by plane strain punch stretching. Failure develops along two intersecting through-thickness, sample-scale shear bands (Carlson and Bird 1987)
5.1.3 Diffuse Necking—Shear Instability—Ductile Fracture
Various authors have reported sheet metal failure without localized necking. Examples that were found in the literature all deal with aluminium alloy sheets.
In Duncan and Bird (1978), the metallographic cross-section of one aluminium alloy shows a well-developed neck after tensile testing, while another alloy shows no necking but instead failure has occurred along a plane at oriented at about 45° to the sheet normal. A very similar observation is presented in Chien et al. (2004), but on two other aluminium alloys. Failure along the plane at 45° to the sheet normal is assumed to be the result of shear localization along this direction.
Also in Hu et al. (2008) these two types of failure are also seen, but in this case for the same alloy either after direct chill casting (DC) or strip casting (CC). The distribution of second phase particles is different under these casting conditions: for DC, particle distribution is more homogeneous and necking is pronounced in a tensile test, while for CC, more stringers of particles are present and a shear-type of failure is seen. Lademo et al. (2008) present two different failure types for an extruded and subsequently cold rolled AlZnMg alloy. In the fully annealed condition, uniaxial tensile test specimens showed shear bands within a developed neck, while after partial annealing parallel and intersecting shear bands over the sheet thickness and oblique to the sheet normal direction were observed. The authors attribute this difference to the strong anisotropy of the sheet in the partially annealed condition, resulting from the retained β-fibre deformation texture, while fully annealed, the sheet has a texture close to random.
Sang and Nishikawa (1983) present the fracture profile of a number of aluminium alloys under plane strain stretching at various temperatures. The observed fracture evolves from a shear-type fracture with no or small necking at low temperatures to a highly pronounced neck with cup-and-cone fracture at higher temperatures. The fracture morphology at room temperature depends on the alloy.
In pure bending of sheet metal, a similar failure mechanism is found, although shear bands do not extent throughout the whole sheet thickness. No references in the literature were found that report the appearance of localized necking in sheet under bending. Steninger and Melander (1982) subjected various steel grades to pure bending tests until failure. It is reported that after a certain homogeneous deformation of the outer fibres, shear bands appear near the outer surface, in which cracks are subsequently formed by void coalescence.
5.2 Forming Limit Diagram: Introduction
The formability is the capability of sheet metal to undergo plastic deformation to a given shape without defects. The defects have to be considered separately for the fundamental sheet metal forming procedures of deep-drawing and stretching. The difference between these types of stamping procedures is based on the mechanics of the forming process (see more details in Banabic et al. (2010a)).
The maximum values of the principal strains \( {\varepsilon}_{1} \) and \( {\varepsilon}_{2} \) can be determined by measuring the strains at failure (necking, fracture, wrinkling etc.) on sheet components covered with grids of circles. Gensamer (1946) was the first researcher who performed a thorough analysis of the strain localization phenomena in the case of sheet metals evolving along different load paths. He published a formability diagram that could be considered as the precursor of the FLCs. The research in this field was pioneered by Keeler (1961), Keeler and Backofen (1963) based on the observations of Gensamer (1946) that instead of using global indices the local deformations have to be considered (in the Fig. 5.2 is presented the Gensamer diagram reflected in mirror).
During forming the initial circles of the grid become ellipses. Keeler plotted the major strains against the minor strains obtained from such ellipses at fracture of parts after biaxial stretching (\( {\varepsilon}_{1} > 0;{\varepsilon}_{2} > 0) \) (see Fig. 5.3 and Keeler (1978)).
Forming Limit Diagram defined by Keeler (1978)
For numerous materials the critical area between the domains has been detected both by means of laboratory tests and by forming of industrial components. These measurements were conducted for various materials. The excellent correlation of the results was a proof that the forming limits in sheet metal forming can be evaluated very well by determining the Forming Limit Curve (FLC).
Later, Goodwin (1968) plotted the curve for the tension/compression domain (\( {\varepsilon}_{1} > 0;{\varepsilon}_{2} < 0) \) by using different mechanical tests. In this case, transverse compression allows for obtaining high values of tensile strains like in rolling or drawing.
The diagrams of Keeler (right side) and Goodwin (left side) are currently called the Forming Limit Diagram (FLD), see Fig. 5.4 and Keeler (1978). Connecting all of the points corresponding to limit strains leads to a Forming Limit Curve (FLC). The FLC splits the ‘fail’ (i.e. above the FLC) and ‘save’ (i.e. below the FLC) regions.
Forming Limit Diagram defined by Keeler (1978)
The Forming Limit Curve FLC is plotted on a Forming Limit Diagram (FLD). The intersection of the limit curve with the vertical axis (which represents the plane strain deformation (ε2 = 0)) is an important point of the FLD and is noted FLD0. The position of this point depends mainly on the strain hardening coefficient and also on thickness.
Today, depending on the kind of limit strains that is measured different types of FLD’s are determined: for necking and for fracture, see Fig. 5.5.
From subsequent experimental and theoretical research, even two more types of FLDs have emerged: the wrinkling limit diagram by Havranek (1977) (see Fig. 5.6) and the Stress Forming Limit Diagram (SFLD) by Arrieux and Boivin (1987) (see Fig. 5.7). The latter is not sensitive to the strain path.
Stress Forming Limit Diagram defined by Arrieux and Boivin (1987)
In order to extend the application of stress limit curves to a 3D stress state (presence of through-thickness components of compressive stress), Simha et al. (2007a) has introduced a new concept, namely Extended Stress-Based Limit Curve (XSFLC). The XSFLC represents the equivalent stress and mean stress at the onset of necking during in-plane loading. Figure 5.8 shows the three formulations of the Forming Limit Curve concept, namely: strain-based FLC (εFLC), stress-based FLC (σFLC) and Extended Stress-Based FLC (XSFLC), respectively. The equivalent stress and the mean stress are obtained through the expressions
Schematic of the Strain-Based Forming Limit Curve (εFLC), the Strain-Based Forming Limit Curve (σFLC) and the extended Strain-Based Forming Limit Curve (XSFLC) (Simha et al. 2007b)
where σ eq is the equivalent stress, and σ mean the mean stress, which is assumed to be positive in tension.
Figure 5.8 also presents the loading paths for the three cases: uniaxial stress, plane strain and biaxial stress. A thorough analysis of the conditions for the use of the XSFLC as a Formability Limit Curve under three-dimensional loading is presented in Simha et al. (2007b).
Forming Limit Curves are valid for one particular material alloy, temper and gauge combination. However material properties vary from batch to batch due to variation in the production process. Therefor a single Forming Limit Curve cannot be an exact description of the forming limit. Janssens et al. (2001) have proposed a more general concept, namely the Forming Limit Band (FLB) as a region covering the entire dispersion of the Forming Limit Curves (Fig. 5.9).
Forming Limit Band (FLB) for two steel grades (Janssens et al. 2001)
5.3 Experimental Formability Tests
The FLC should cover the entire deformation domain specific to the sheet metal forming processes. In general, the strain combinations span between those induced by uniaxial and equibiaxial surface loads. The subsequent discussion will insist on the experimental methods commonly used for investigating the deformation domain of the FLCs. First an overview is given of some experimental techniques designed for the determination of the whole or a partial forming limit diagram (FLD), i.e. Nakazima tests, Marciniak, tests stretch-bending tests, hydraulic bulging tests and tests performed in a tensile test machine (see more details in Banabic et al. (2010a). After the experimental formability techniques, experimental results on the influence of different factors (sheet curvature, thickness, temperature and strain rate on formability are discussed.
5.3.1 An Overview of Experimental Formability Tests
The most used procedures for the experimental determination of the FLCs are those based on the punch stretching principle. Keeler (1961) was the first researcher who adopted such a method. He used circular specimens and spherical punches with different radii in order to modify the load path. In general, the punch stretching test developed by Keeler is able to investigate only the right end of the tension-tension FLC branch. Hecker (1972) extended Keeler’s methodology to the whole tension-tension domain by improving the lubrication of the contact surface between punch and specimen. A notable development of this experimental procedure is due to Nakazima and Kikuma (1967). He used a hemispherical punch having a constant radius in combination with rectangular specimens with different widths (Fig. 5.10).
In this way, Nakazima was able to explore both the tension-compression and the tension-tension domains of the FLC. The Nakazima forming limit test is the most widely-spread method for experimental determination of the FLD. It uses a hemispherical punch with large diameter (in the order of 100 mm) to deform a clamped specimen until failure. Due to the punch curvature, a strain gradient exists in the sheet thickness direction, and also in the plane of the sheet. By using circular specimens with lateral notches, Hasek (1978) removed the main disadvantage of Nakazima test, namely the wrinkling of the wide specimens. Under biaxial stretching, the largest straining is not necessarily found at the punch apex (Keeler and Brazier 1975). The strain mode is determined by choosing the specimen width and/or lubrication conditions (Charpentier 1975). It was early recognised that the experimental determination of a FLC through the Nakazima test was prone to various test conditions such as punch geometry, lubrication conditions and limit strain measurement method. Consequently, several laboratory procedures were proposed for comparison purposes of different materials, such as the CRM-method (Bragard 1989). In this method, the limit strains are determined by a parabolic fit of the non-homogeneous strain field after the onset of necking. The use of Digital Image Correlation (DIC) for use of in-process strain measurement on a surface of the sheet, results in a more automated and thus less user-dependent measurement of the necking strains, as discussed in Geiger and Merklein (2003). Through DIC measurements, a relative small non-linearity of the strain path in the Nakazima test is found in Leppin et al. (2008): due to the hemispherical punch, a small initial equibiaxial strain is found on the convex sheet surface, independent of the sheet geometry. As a result, FLC0 determined from the Nakazima test is slightly shifted to the right in the FLD.
In the Marciniak forming limit test, first described in Marciniak et al. (1973), a punch with flat bottom deforms the sheet until failure in the flat part of the sheet occurs. Failure at the punch edge is avoided through use of an auxiliary sheet with a hole with appropriate dimensions in between the punch and test sheet. The flat region of the test sheet deforms homogeneously, except in the site where strain localization takes place. In the original paper, the test and auxiliary sheets are fully clamped around the punch, and the strain mode is determined by the punch geometry (having a circular, elliptical or rectangular bottom face). Grosnostajski and Dolny (1980) improved Marciniak’s test by changing the geometry of the specimen and carrier blank.
A standardized procedure for determination of the FLC based on Nakazima and Marciniak tests, using a 100 mm diameter cylindrical or hemispherical punch respectively, is found in ISO norm 12004 (2008). Various deformation modes are achieved by different sheet sample geometries. Additional information on the this standard can be found in Hotz and Timm (2008). In Vegter et al. (2008), the use of a rubber disc in the tribological system in the Nakazima test is analyzed through FE simulations. Although not described in the ISO norm 12004, it is quite common to use such a disc in order to achieve the highest strains and thus the neck at the apex of the Nakazima punch, a condition which is required in this norm.
In stretch-bending tests, a rectangular blank is clamped at two opposite edges and deformed under a cylindrical punch which has its axis along the direction of the clamped sheet edges. The punch diameter can vary from the order of the sheet thickness much larger values. The distribution and evolution of the strain field in stretch-bending can be quite complex. Uko et al. (1977) presents experimental results for HSLA steel under stretch-bending in which the inside surface thickness strain changes from compressive to tensile during testing. The observed deformation mode is near-plane strain (small negative minor strains).
The variability of sheet formability and sheet formability testing for a standardized stretch-bending test, named OSUFT, is explored in Karthik et al. (2002). Sensitivity of numerous parameters (the hold-down force, sheet thickness, sample width, deformation speed, lubrication conditions and seasoning of the tooling) to the punch stroke at failure was investigated. The variability of this test between different test laboratories was shown to be much less compared to Nakazima tests using plane-strain samples, making it more useful for material comparison purposes.
In Kitting et al. (2008), micrographic cross-sections are shown of failed sheet deformed through stretch-bending with varying punch radii. It can be seen that the double-sided neck observed at large radii changes into a single-sided neck at the convex sheet side for smaller radii. Also, punch penetration in the sheet can be seen in case the punch radius is of the order of the sheet thickness.
The positive-positive region (right branch) of the FLC can be reproduced in a hydraulic bulging device equipped with dies having circular or elliptic apertures. Different load paths belonging to the tension-tension domain result by varying the eccentricity of the elliptic aperture (Ranta-Eskola 1979). In hydraulic bulging, a fully clamped sheet is deformed through a die with circular or elliptical aperture through fluid pressure, usually oil. The deformation mechanism in hydraulic bulge testing with circular die aperture has been experimentally studied by Ranta-Eskola (1979). It is shown that at the bulge apex, sheet thinning is maximal, so it is the preferential site for plastic instability and failure. It is also pointed out that the sheet assumes a spherical shape at the apex, although the strain state can differ from equibiaxial loading due to in-plane anisotropy.
Forming limit tests in tensile test machines have been proposed for deformation modes of the left-hand side of the FLD. The uniaxial tension of flat specimens having circular notches (proposed by Brozzo and de Lucca (1971)) allows the exploration of the tension-compression range (left branch of the FLC). By using relatively wide specimens, it is also possible to reach the plane strain point. In Sang and Nishikawa (1983) and later in Timothy (1989), a plane strain state in a tensile test machine is obtained through the use of a clamping device with knife edges to prevent deformation in the width direction. Later, a methodology to obtain the full left-hand side of the FLD from tensile test specimens with different geometries has been proposed by Holmberg et al. (2004). As a conclusion, the uniaxial tension is suitable only for investigating the positive-negative domain of the FLC.
Figure 5.11 compares the results provided by different experimental methods developed in the seventh and eighth decades of the previous century. One may notice that none of those procedures are able to reproduce the whole deformation domain of the FLC. Aiming to overcome this drawback, as well as the discrepancies of the limit strains provided by different methodologies, a specialized IDDRG workgroup elaborated a standard proposal for the FLC determination recommending the use of the Nakazima or Marciniak tests. The proposal issued by IDDRG was subsequently adopted at international level in the form of the ISO 12004 standard ISO (2008). A description of the experimental procedures analyzed by the IDDRG workgroup and their comparison by means of a “robin test” performed in different laboratories participating in the standardization activity is given in Hotz and Timm (2008). A presentation of the determination of the FLCs is described in Geiger and Merklein (2003).
Banabic et al. (2013) proposed a new procedure for the experimental determination of the FLCs. The methodology is based on the hydraulic bulging of a double specimen (Fig. 5.12).
The upper blank has a pair of holes pierced in symmetric positions with respect to the centre, while the lower one acts both as a carrier and a deformable punch. By modifying the dimensions and position of the holes, it is possible to investigate the entire deformation range of the FLC. Figure 5.13 provides a synthetic presentation of the numerical results obtained in the case of the AA6016-T4 aluminium alloy. The results provided by the hydraulic bulging experiments performed with the same geometries of the specimens are also plotted on the diagram. One may notice a very good agreement between the numerical simulation and the experimental data, as well as the fact that the characteristic strain paths are closed to linearity in all cases.
The most important advantages of the method are the capability of investigating the whole strain range specific to the sheet metal forming processes, simplicity of the equipment, and reduction of the parasitic effects induced by the friction, as well as the occurrence of the necking in the polar region. The comparison between the FLCs determined using the new procedure and the Nakazima test shows minor differences. Figure 5.14 compares the FLCs obtained using the methodology proposed by the authors and the Nakazima test (according to the specifications of the international standard ISO 12004-2). In both cases, the limit strains have been measured using the ARAMIS system (Banabic et al. 2013).
5.3.2 Experimental Formability Observations Concerning the Influence of Sheet Curvature
A comparative study between the Nakazima and Marciniak tests for aluminium killed steel, Brass and cold rolled aluminium (Ghosh and Hecker 1975) showed a clear trend of higher formability determined from the Nakazima test. It is however preliminary to conclude from this study that sheet curvature during forming is the only reason for this since it was chosen to reduce the sheet thickness in the Nakazima test instead of using an auxiliary sheet.
In Levy (2002), an empirical law is presented for a number of steel grades to assess the increase in formability in sheet material after it has been subjected to a form of bending, being multiple bending during drawbead flow or bending occurring at corner radii of press tooling. As a rule of thumb, it is concluded that for material which has been subjected to this kind of stretch-bending, the FLD can be shifted upwards by an amount equal to 60 % of the thinning strain that was achieved in the drawbead or under small tool corners.
The beneficial effect of simultaneous bending and unbending during plane strain stretching was shown in Emmens and van den Boogaard (2008), in which a tensile test specimen was additionally subjected to small bending strains under three moving rollers.
5.3.3 Experimental Formability Observations Concerning the Influence of Sheet Thickness
The influence of the sheet thickness on the limit strains has been studied by Haberfield and Boyles (1973), Romano et al. (1976), Hiam and Lee (1978), Kleemola and Kumpulainen (1980) etc.
The plane strain intercept of the FLC (denoted FLC0), was already in the 1970s found to be dependent on the sheet thickness for a number of hot and cold rolled steels by Keeler and Brazier (1975), resulting in higher limit strains for thicker sheets.
Possible influencing factors which result in a general higher forming limit of thicker sheets are discussed in Marciniak (1977). The factors that depend on sheet thickness include through-thickness gradients of strain, stress and triaxiality, friction forces, tool contact pressure, and sheet metal homogeneity.
In Karthik et al. (2002), a standardized stretch-bend test (OSUFT) was used to show that thicker sheets failed at higher punch strokes, even though less draw-in under the drawbeads occurred for thicker sheets.
The influence of sheet thickness on the FLD is characterized by the following relationships Tisza and Kovács (2012):
-
the FLD for necking depends on sheet thickness (t 0) (see Fig. 5.15);
-
as the thickness rises, the curve rises on the plot (\( {\varepsilon}_{1} ;{\varepsilon}_{2} \));
-
The influence is high for pure expansion and vanishes for pure compression;
-
The influence of the thickness on the FLD0 increases linearly;
-
Along a linear strain path the rise of the FLD is proportional to the increase of thickness but this influence vanishes above a critical value.
The engineer can decide if an unsuccessful forming process may be improved by increasing the sheet thickness. This is especially important if the stress acting during the forming process is tensile in both principal directions.
5.3.4 Experimental Formability Observations Concerning the Combined Influence of Sheet Curvature and Thickness
Ghosh and Hecker (1975) showed that the choice of the experimental method used for the FLC determination (in-plane versus out-of-plane) influences the position of the limit curves. The influence of the punch curvature on the stretching limits has been studied first by Charpentier (1975). In Charpentier (1975) and Demeri (1986), it is shown that the limit strain is increased by increasing the sheet thickness, or by decreasing the punch radius in the Nakazima test. It is also observed that the strain distribution is less homogeneous for smaller punch radii. For the same non-dimensional bending curvature t/R (the ratio of sheet thickness to punch radius), it appears that the limit strain increase of a thicker sheet and a smaller punch radius is higher compared to a thinner sheet stretched under a smaller punch radius (Charpentier 1975) (see Fig. 5.16) (the experimental data was taken from Charpentier (1975)). Shi and Gerdeen (1991) performed a theoretical analysis of this influence using the Marciniak-Kuckzinsky model.
Influence of punch curvature on the FLC (Charpentier 1975)
Based on an experimental campaign of punch-stretching of steel alloys with various punch radii and sheet thicknesses, Tharrett and Stoughton (2003a) proposed that the strain on the concave side be used for comparison with FLC0 (concave-side rule), rather than the mid-plane strain which is a more conservative criterion. However, for two FCC materials (70/30 Brass and AA6010), this method resulted in an overestimate of the forming limit (Tharrett and Stoughton 2003b).
5.3.5 Experimental Formability Observations Concerning the Influence of Temperature
The influence of the temperature on the limit strains was studied first by Lange (1975) and later by Ayres and Wenner (1978), Kumpulainen et al. (1983), van den Boogaard (2002), Li and Ghosh (2004), Abedrabbo et al. (2006), etc. According to these researchers, the temperature has a different influence on the formability of different metallic alloys. For example, the formability of the AA 5754 alloy has a significant increase when the temperature rises even with small amounts (from 250 to 350 °C) (Fig. 5.17 and Li and Ghosh (2004)), while temperature variations in the same range have a very little influence on the formability of the AA 6111-T4 alloy (Fig. 5.18 and Li and Ghosh (2004)). The increase of the formability by raising the temperature of the material is frequently used in the case of the sheet metals having a poor formability at room temperature (some aluminium or magnesium alloys, high-strength steels, etc.).
Influence of the temperature on the FLC for the 5754 aluminium alloy (Li and Ghosh 2004)
Influence of the temperature on the FLC for the 6111-T4 aluminium alloy (Li and Ghosh 2004)
5.3.6 Experimental Formability Observations Concerning the Influence of Strain Rate
Drewes and Martini (1976) followed later by Ayres and Wenner (1978) and Percy (1980) have analyzed the influence of the strain rate on the limit strain. In general, the increase of the strain rate causes a downward displacement of the FLC, that is a diminishment of the formability. Such an example is shown in Fig. 5.19 and Percy (1980) and corresponds to the SPCEN-SD steel. Similar results were also obtained by Ayres and Wenner (1978). On the other hand, more recently, Balanethiram and Daehn (1994) have reported a significant increase of the formability when the strain rate is also increased for an OFHC copper. Gerdooei and Dariani (2009) have explained this effect based on the Johnson-Cook law. The different behaviour of the metallic materials from this point of view is a consequence of the different values of the strain-rate sensitivity index, as well as of the different mechanical response when the strain rate is modified.
Influence of the strain-rate on the FLC for SPCEN-SD steel (Percy 1980)
5.4 Forming Limit Models
Various theoretical models have been developing for the calculation of forming limit curves (Fig. 5.20). The first ones were proposed by Swift (1952) and Hill (1952) assuming homogeneous sheet metals (the so-called models of diffuse necking and localized necking), respectively). The Swift model has been developed later by Hora, so-called Modified Maximum Force Criterion-MMFC, (Hora and Tang 1994). Marciniak (1965) proposed a model taking into account that sheet metals are non-homogeneous from both the geometrical and the structural point of view. Storen and Rice (1975) developed a model based on the bifurcation theory. Dudzinski and Molinari (1991) used the method of linear perturbations for analyzing the strain localization and computing the limit strains.
Since the theoretical models are rather complex and need a profound knowledge of continuum mechanics and mathematics while their results are not always in agreement with experiments, some semi-empirical models have been developed in recent years.
In the next sections the most commonly used models are presented briefly with the focus on those based on the necking phenomenon (Swift and Hill), the Marciniak-Kuczynski and MMFC models.
5.4.1 Diffuse Necking Models
5.4.1.1 Swift’s Model
Considère (1885) approached for the first time the problem of plastic instability in uniaxial tension. In the case of ductile materials, two domains may be distinguished in the region of plastic straining. In the first domain the hardening influence on the traction force is stronger than the influence of the cross-section reduction. This is the so-called ‘domain of stable plastic straining’, being characterized by the fact that an increase of the traction force is needed in order to obtain an additional deformation of the specimen. In the second domain material hardening cannot compensate the decrease of the traction force due to the reduction of the specimen’s cross-section. This is the so-called ‘domain of unstable plastic straining’, being characterized by a decrease of the traction force, although the stress continues to increase.
The beginning of necking corresponds to the maximum of the traction force. From the mathematical point of view, this condition can be written in the form
By simple mathematical manipulations the following condition of plastic instability is obtain:
Assuming a Ludwik-Hollomon strain-hardening law,
condition (5.4) becomes
Hence, according to Considère’s criterion, a material obeying the Ludwick-Hollomon hardening law starts to neck when the strain is equal to the hardening coefficient.
Swift (1952) used the Considère criterion to determine the limit strains in biaxial tension. He analysed a sheet element loaded along two perpendicular directions and applied the Considère criterion for each direction. Assuming a strain hardening described by Eq. (5.5), he obtained the following expressions of the limit strains:
where f is the yield function.
By using different yield functions, it is possible to evaluate the limit strains as functions of the loading ratio α and the mathematical parameters of the material (hardening coefficient n, anisotropy coefficient r, strain-rate sensitivity m, etc.). As an example, if the Hill 1948 yield criterion is used, the limit strains are as follows:
The expressions of the limit strains associated to some other yield criteria (such as Hill 1979 and Hill 1993) are presented in Banabic and Dannenmann (2001). By computing the values of \( \varepsilon_{1}^{ * } \) and \( \varepsilon_{2}^{ * } \) for different loading ratios α and recording them in a rectangular coordinate system ε 1 , ε 2 the necking limit curve is obtain.
5.4.1.2 Modified Maximum Force Criterion (MMFC)
The ‘Modified Maximum Force Criterion’ (MMFC) for diffuse necking proposed by Hora and Tang (1994) is based on Considère’s maximum force criterion. The idea behind the MMFC-Model is to factor in an additional increase in hardening, which is triggered by the deviation from the initial, homogeneous stress condition—e.g. uniaxial tension—to the stress condition of local necking and with this to the point of plane strain (Fig. 5.21).
The mathematical expression of the criterion is:
Herein, \( {\upbeta} \) represents the strain rate ratio given by
The MMFC model can be written in a form independent of the yield criterion, i.e. it can accommodate any yield criterion. According to Hora and Tang (1994) the following relations are defined:
The stress ratio \( \upalpha \) takes the values \( 0 \le\upalpha \le 1, \) i.e. it ranges from uniaxial tension \( \left( {\upalpha = 0} \right) \) to equibiaxial tension \( \left( {\upalpha = 1} \right). \) \( \bar{\upsigma } \) is the equivalent stress defined by the yield criterion which is utilized in the necking analysis, see below. \( \bar{\upvarepsilon} \) is the equivalent plastic strain.
The function \( f\left(\upalpha \right) \) is obtained from:
Assuming the instantaneous yield stress is represented by the Swift hardening law, Hora’s necking criterion then reads (Hora and Tang 1994)
with \( {\upbeta }^{{\prime }} = d\upbeta /\text{d}\upalpha ,\quad \text{f}^{{\prime }} = \text{df}/\text{d}\upalpha ,\quad Y^{{\prime }} = dY/d\bar{\upvarepsilon}. \)
The primary unknown \( \bar{\upvarepsilon} \) can be easily calculated as the solution of the necking criterion given by Eq. (5.16) (which is, in general, a non-linear equation) using Newton’s method. Once the equivalent plastic strain at the onset of necking for a chosen linear strain path is calculated from Eq. (5.16), the major and minor in-plane strains corresponding to the onset of necking are found from
\( \bar{\upvarepsilon}^{ * } \) is the root of the necking criterion Eq. (5.16).
In order to take into account the influence of the thickness on the limit strains, an enhanced MMFC (eMMFC) has been recently proposed by Hora and his co-workers (Hora et al. 2003). A term is added to the original formulation (5.11). The eMMFC is expressed as
where, t is the thickness, r is the sheet curvature radius and \( e\left( {t,E = const} \right) = E_{0} \left( {\frac{t}{{t_{0} }}} \right)^{p} \) represents the influence of the thickness. The parameters E 0 , p and t 0 are determined using experimental data (Hora and Tong 2006).
Recently, Hora et al. (2013) investigated the influence of the yield loci and strain-hardening laws on the Forming Limit Curves using the MMFC model. Different explicit expressions of the MMFC model have been proposed based on some simplifications. A new formulation of the MMFC model Manopulo et al. (2015) has been proposed to accommodate this model with the Homogeneous Anisotropic Hardening (HAH) model proposed by Barlat et al. (2011). Using the new approach, the role of the distortional hardening on strain localization has been analyzed.
Banabic and Soare (2009) make more precise statements about the nature of the numerical instability of the MMFC model, asses the predictive capabilities of the criterion, and introduce a fitting parameter for its plane strain calibration. In order to improve the prediction of limit strains using the MMFC model, Paraianu et al. (2009), (2010) chose to introduce two fitting coefficients in the original model.
The advantage of the MMFC criteria can be found in their independence of the inhomogeneity assumption. These criteria could be used to calculate FLC for non-linear strain paths. A drawback of the MMFC models is the fact that they can be affected by a singularity that emerges if the yield locus contains straight line segments, as in the case of Barlat et al. (2003) or BBC 2005 (Banabic et al. 2005a) yield criteria. Banabic et al. (2015) removed this limitation of the MMFC criterion by modifying the initial formulation. As an example, the singularity noticed by Aretz (2004) in the case of the AA2090-T3 aluminium alloy is no more present when using the new formulation proposed in Banabic et al (2015) (see Fig. 5.22).
5.4.2 Localized Necking Model (Hill’s Model)
In the case of uniaxial tension, the localized necking develops along a direction which is inclined with respect to the loading direction. Hill (1952) assumed that the necking direction is coincident with the direction of zero-elongation and thus the straining in the necking region is due only to the sheet thinning.
The method used for obtaining the limit strains in this case is presented in Banabic and Dörr (1995). The expressions of these strains are as follows:
It can be seen that
This is the equation of a line parallel with the second bisectrix of the rectangular coordinate system ε 1 , ε 2 and intersecting the vertical axis at the point (0, n).
According to Eq. (5.21), the FLC computed on the basis of the Hill’s model does not depend on the yield criterion, but only on the value of the hardening coefficient.
5.4.3 Assessing the Formability of Metallic Sheets by Means of Localized and Diffuse Necking Models
5.4.3.1 Constitutive Equations
In what follows, sheet metals are assimilated to orthotropic membranes exhibiting a rigid-plastic behaviour. Their formability is analyzed in the context of active loading processes subjected to the constraints
where \( {\sigma}_{ij} = {\sigma}_{ji} \) and \( \dot{\varepsilon}_{ij} = \dot{\varepsilon}_{ji} \) respectively denote stress and strain-rate components expressed in the orthotropy frame defined by the rolling direction RD (axis 1), transverse direction TD (axis 2), and normal direction ND (axis 3). It is not difficult to observe that Eqs. (5.22) enforce a particular plane-stress state characterized by the absence of shearing effects. Under such circumstances, \( {\sigma}_{ii} \) and \( \dot{\varepsilon}_{ii} \left( {i = 1,2,3} \right) \) automatically become principal values of the corresponding stress and strain-rate tensors. In order to emphasize this significance, the following notations are adopted:
The rigid-plastic behaviour of sheet metals is described by the yield criterion
the flow rule
and the incompressibility condition
Equations (5.24) and (5.25) operate with the equivalent stress \( \bar{\sigma} \) (defined as a strictly convex and first-degree homogeneous function \( \bar{\sigma} = \bar{\sigma}\left( {{\sigma}_{1} ,{\sigma}_{2} } \right)) \), the equivalent strain \( \bar{\varepsilon}, \) and the yield parameter (controlled by a strictly increasing hardening law \( y = y\left( {\bar{\varepsilon}} \right)) \). For any load state having the property \( {\sigma}_{1} > 0, \) the quantities \( \bar{\sigma} \) and \( \partial \bar{\sigma}/\partial {\sigma}_{i} \left( {i = 1,2} \right) \) can be written in the form
where
Equations (5.27) and (5.28) are easily deducible from the following mathematical properties of the first-degree homogeneous function \( \bar{\sigma}{\kern 1pt} \):
With the aim of simplifying the future manipulations of the constitutive relationships, one denotes by \( g_{3} \) the opposite of the sum \( g_{1} + g_{2} {\kern 1pt} \):
As soon as Eqs. (5.27) and (5.31) are taken into account, Eq. (5.24) becomes
while Eqs. (5.25) and (5.26) get the unified formulation
The models described in the next section make use of the strain-path concept. This term designates a sequence of load states defined by a relationship between \( \dot{\varepsilon}_{1} \) and \( \dot{\varepsilon}_{2} . \) Only strain paths that induce a continuous thinning of the metallic sheet are relevant to the following analysis. Such a characteristic is enforced by the restriction \( \dot{\varepsilon}_{3} < 0 \) or, equivalently, \( \dot{\varepsilon}_{1} + \dot{\varepsilon}_{2} > 0 \) (see Eq. (5.26)). The analysis is further limited to the case when \( \dot{\varepsilon}_{1} \) is the major principal value of the strain-rate tensor, i.e. \( \dot{\varepsilon}_{1} > 0 \) and \( - \dot{\varepsilon}_{1} < \dot{\varepsilon}_{2} \le \dot{\varepsilon}_{1} . \) Any strain path having these properties can be represented in the form
where the bounds of the \( \beta \)—range correspond to the pure shear deformation
and balanced biaxial elongation
Under conditions (5.34)–(5.36), \( {\sigma}_{1} \) is a strictly positive quantity. Equations (5.33)–(5.36) can be thus combined to express \( {\beta} \) as a function of \( {\alpha} \) i.e.
where the bounds of the \( {\alpha} \)—range result by solving the equations
and
If \( \bar{\sigma} = \bar{\sigma}\left( {{\sigma}_{1} ,{\sigma}_{2} } \right) \) is strictly convex, Eqs. (5.38) and (5.39) have unique solutions. Assuming the same strict convexity constraint, one may prove that Eq. (5.37) also defines a one-to-one mapping \( {\alpha} \leftrightarrow {\beta} , \) with \( {\alpha}_{ \inf } < {\alpha} \le {\alpha}_{ \sup } \) and \( {\beta}_{ \inf } < {\beta} \le {\beta}_{ \sup } . \)
The plane-strain state \( \left( {\dot{\varepsilon}_{1} > 0\;{\text{and}}\;\dot{\varepsilon}_{ 2} { = 0}} \right) \) is of special interest for the models discussed below. In this case, conditions (5.34) enforce
the associated value of the principal stress ratio being uniquely determined by Eq. (5.37) rewritten as follows:
5.4.3.2 Localized and Diffuse Necking Models
From a theoretical perspective, localized necking is associated with the loss of carrying capability in a zero-extension plane. According to Hill (1952), the angle made by this plane with TD is (see Fig. 5.23a, as well as Eqs. (5.34)–(5.36) and (5.40))
One may notice that the square root in Eq. (5.42) has no significance for strictly positive values of the argument \( \dot{\varepsilon}_{2} /\dot{\varepsilon}_{1}. \) In such cases corresponding to biaxial elongation regimes \( (\dot{\varepsilon}_{1} > 0 \) and \( 0 < \dot{\varepsilon}_{2} \le \dot{\varepsilon}_{1} \)—see Eqs. (5.34)–(5.36) and (5.40)), the localized necking mechanism is inhibited because zero-extension planes do not exist.
For linear strain paths individualized by constant ratios \( \dot{\varepsilon}_{2} /\dot{\varepsilon}_{1} \) in the range \( - 1 < \dot{\varepsilon}_{2} /\dot{\varepsilon}_{1} \le 0, \) Hill’s model predicts that metallic sheets lose their carrying capability when
With the help of Eqs. (5.32)–(5.41) and (5.43) becomes
where
is the hardening modulus. Equation (5.44) can be used to determine the equivalent strain in the stage of localized necking, for a given value of the parameter \( {\alpha}. \) Let \( \bar{\varepsilon}_{\text{Hill}} \left( {\alpha} \right) \) denote the solution of Eq. (5.44). Due to the fact that rupture immediately follows the loss of carrying capability in the zero-extension plane (Hill 1952), \( \bar{\varepsilon}_{\text{Hill}} \left( {\alpha} \right) \) defines a limit value of the equivalent strain.
One assumes that diffuse necking begins as soon as the major cross-sectional force is maximized, i.e. when (Dorn and Thomsen 1947; Mattiasson et al. 2006)
In the particular case of a linear strain path, Eqs. (5.32)–(5.39) and (5.45) bring (5.46) to the form
For a given value of the parameter \( {\alpha} , \) Eq. (5.47) can be used to determine the equivalent strain accumulated by the metallic sheet up to the onset of diffuse necking. Let \( {}^{0}\bar{\varepsilon}_{\text{EMFC}} \left( {\alpha} \right) \) denoteFootnote 1 the solution of Eq. (5.47).
In its evolutionary phase, diffuse necking is described as a transition towards the plane-strain state at the level of a straight band perpendicular to RD (see Figs. 5.23b and 5.2). Three hypotheses are formulated with reference to this process (Mattiasson et al. 2006):
-
The linear character of the strain path is preserved in the non-necking regions.
-
The minor principal strain-rate remains uniformly distributed in the metallic sheet, i.e.
$$ \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\dot{\varepsilon }}_{2} = \dot{\varepsilon }_{2} . $$(5.48) -
The major cross-sectional force is kept at a maximum value inside the necking band, i.e. (see Eq. (5.46) for comparison).
$$ \frac{{\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\dot{\sigma }}_{ 1} }}{{\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\sigma }_{ 1} }} - \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\dot{\varepsilon }}_{ 1} = 0. $$(5.49)
Equations (5.48) and (5.49) use underlined symbols for the parameters of the necking band vs. plain symbols for the parameters of the non-necking domains. The subsequent relationships also adhere to this typographic convention.
With the help of Eqs. (5.28), (5.32)–(5.39), (5.45), Eqs. (5.48) and (5.49) can be rewritten in the explicit forms
and
respectively. The necking progress is controlled by Eqs. (5.50) and (5.51), together with the initial conditions
The discussion below focuses on describing the manner in which Eqs. (5.50)–(5.52) are used to determine the limit level of the equivalent strain \( \bar{\varepsilon}_{\text{EMFC}} \left( {\alpha} \right) \) that corresponds to a given value of the parameter \( {\alpha} . \)
If \( {\alpha} = {\alpha}_{{{\text{FLC}}_{0} }} , \) Eq. (5.50) and condition (5.41) enforce \( {\mathop{\alpha}\limits_{\sim}} = {\alpha}_{{{\text{FLC}}_{0} }} . \) Under such circumstances, Eq. (5.51) degenerates to Eq. (5.47), both of them being also coincident with Eq. (5.44) particularized for \( {\alpha} = {\alpha}_{{{\text{FLC}}_{0} }} . \) The onset of diffuse necking is thus immediately followed by rupture when the metallic sheet evolves along a plane-strain path, i.e.
On the other hand, if \( {\alpha} \ne {\alpha}_{{{\text{FLC}}_{0} }} , \) Eq. (5.50) and condition (5.41) also enforce \( \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha } \ne \alpha_{{{\text{FLC}}_{0} }} . \) In this case, the evolution of the necking band towards the plane-strain state is possible (see Fig. 5.24). Due to the fact that \( \dot{\bar{\varepsilon }}/\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\dot{\bar{\varepsilon }}} \to 0 \) for \( \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha } \to \alpha_{{{\text{FLC}}_{0} }} \) (see Eq (5.50) and condition (5.41)), a bottom threshold of the ratio \( \dot{\bar{\varepsilon }}/\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\dot{\bar{\varepsilon }}} \) must be fixed in order to avoid numerical difficulties when solving Eqs. (5.50) and (5.51):
If Eq. (5.50) is taken into account, condition (5.54) becomes
or, equivalently (see also Eqs. (5.52) and Fig. 5.24),
with \( {\alpha}_{{{\text{FLC}}_{ \mp \eta } }} \) determined as follows:
In the case \( {\alpha} \ne {\alpha}_{{{\text{FLC}}_{0} }} , \) the limit value \( \bar{\varepsilon}_{\text{EMFC}} \left( {\alpha} \right) \) results by integrating Eqs. (5.50) and (5.51) over a time interval that corresponds to the evolution of the parameter \( \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha } \) between the bounds \( \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha }_{\inf} \) and \( \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha }_{\sup } . \) This task is accomplished in a sequence of \( i_{ \hbox{max} } \) steps,
Equation (5.58) uses \(\left\lfloor {\kern 1pt}\,\blacksquare \; \right\rfloor\) as a symbol of the floor function. Each step of the computational procedure starts by incrementing \( \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha } \) (see also conditions (5.52) and (5.56)):
Equation (5.59) and many of the subsequent relationships involve quantities with upper-left index qualifiers. Their significance is explained below:
\( {}^{i - 1}{\kern 1pt}\,\blacksquare \; \to \) | State parameters associated to the reference configuration of the metallic sheet (known quantities either evaluated in the previous computational step or initialized by means of Eqs. (5.52)) |
\( {}^{i}{\kern 1pt} \blacksquare\; \to \) | State parameters associated to the current configuration of the metallic sheet (except for \( {}^{i}\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\alpha } , \) all these quantities are unknowns that must be determined) |
For solution purposes, Eqs. (5.50) and (5.51) are also rewritten in the incremental forms (see also Eqs. (5.52))
and
respectively. One may notice that Eq. (5.61) is able to determine \( {}^{i}\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\bar{\varepsilon }} . \) As soon as Eq. (5.61) is solved for the unknown \( {}^{i}\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\thicksim}$}}{\bar{\varepsilon }} , \) Eq. (5.60) allows evaluating \( {}^{i}\bar{\varepsilon } \):
The incremental procedure presented above must be performed \( i_{ \hbox{max} } \) times. The solution \( {}^{{i_{\hbox{max} } }}\bar{\varepsilon} \) obtained in the last step characterizes the formability of the metallic sheet from the point of view of the diffuse necking model:
Both \( \bar{\varepsilon}_{\text{Hill}} \left( {\alpha} \right) \) and \( \bar{\varepsilon}_{\text{EMFC}} \left( {\alpha} \right) \) should be used to define a limit value of the equivalent strain for a given value of the stress ratio \( {\alpha} \):
Under the assumption \( {\alpha} = {\text{const}} \)., the formability is equally characterized by two of the principal logarithmic strains (see Eqs. (5.33))
A common practice is to use \( {\varepsilon}_{1} = {\varepsilon}_{1} \left( {\alpha} \right) \) and \( {\varepsilon}_{2} = {\varepsilon}_{2} \left( {\alpha} \right) \) for this purpose. If \( {\varepsilon}_{2} = {\varepsilon}_{2} \left( {\alpha} \right) \) is a one-to-one mapping,Footnote 2 \( {\varepsilon}_{1} = {\varepsilon}_{1} \left( {\alpha} \right) \) and \( {\varepsilon}_{2} = {\varepsilon}_{2} \left( {\alpha} \right) \) can be replaced by a single function \( {\varepsilon}_{1} = {\varepsilon}_{1} \left( {{\varepsilon}_{2} } \right) \) that defines the Forming Limit Curve.
5.4.4 Marciniak-Kuckzynski (M-K) Model
5.4.4.1 Overview
Shortly after the introduction of the Forming Limit Diagram concept, on the basis of the experimental investigations concerning the strain localization of some specimens subjected to hydraulic bulging or punch stretching, Marciniak (1965) and Marciniak and Kuczynski (1967) developed a limit curve prediction model. This model is based on the hypothesis of the existence of imperfections in sheet metals. According to Marciniak’s hypothesis, sheet metals have, from manufacturing, geometrical imperfections (thickness variations) and/or structural imperfections (inclusions, gaps). In the forming process these imperfections progressively evolve and the plastic forming of the sheet metal is almost completely localized in them, leading to the necking of the sheet metal. The realism of this hypothesis has been experimentally analyzed by Azrin and Backofen (1970). This model has been intensely used and developed by researchers due to the advantages it offers: it has an intuitive physical background; it correctly predicts the influence of different process or material parameters on the limit strains; the predictions are precise enough; the model can be easily coupled with Finite Element simulation software for sheet metal forming processes. The main drawbacks of this model are: the prediction results are very sensitive to the constitutive equations used, as well as to the values of the non-homogeneity parameter; in the case of advanced material models, the equation system of the model is quite difficult to solve and lacks robustness.
A few years later, Marciniak (1968) made a thorough analysis of the strain localization phenomenon from the right side of the FLD and extended his initial model to cover this area. The models have periodically been brought in discussion by specialists in dedicated symposia (see Koistinen and Wang (1978), Hecker et al. (1978), Wagoner et al. (1989), Hora (2006), Hora and Volk (2014)) or in special sections in conferences (NUMISHEET, NUMIFORM, IDDRG, ESAFORM, etc.). Further developments of the Marciniak models are synthetically described in the review papers (Banabic et al. 2010b; Banabic 2010).
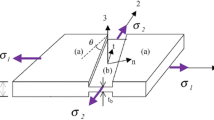
The analysis of the necking process has been performed assuming a geometrical non-homogeneity in the form of a thickness variation. This variation is usually due to some defects in the technological procedure used to obtain the sheet metal. The thickness variation is generally gentle. However, the theoretical model assumes a sudden variation in order to simplify the calculations (Fig. 5.25).
The theoretical model proposed by Marciniak and Kuczynski (1967) assumes that the specimen has two regions: region ‘a’ having a uniform thickness \( s_{0}^{a} \), and region ‘b’ having the thickness \( s_{0}^{b} \) (Fig. 5.25). The initial geometrical non-homogeneity of the specimen is described by the so-called ‘coefficient of geometrical non-homogeneity’, η, expressed as the ratio of the thickness in the two regions:
The strain and stress states in the two regions are analysed with respect to the principal strain \( \varepsilon_{1}^{b} \) in region ‘b’ and the principal strain \( \varepsilon_{1}^{a} \) in region ‘a’. When the ratio \( {{\varepsilon_{1}^{b} } \mathord{\left/ {\vphantom {{\varepsilon_{1}^{b} } {\varepsilon_{1}^{a} }}} \right. \kern-0pt} {\varepsilon_{1}^{a} }} \) becomes too high (infinite in theory, above 10 in practice), one may consider that the deformation of the specimen is localized in region ‘b’ (Fig. 5.26).
The shape and position of the curve \( \varepsilon_{1}^{a} \text{(}\varepsilon_{1}^{b} \text{)} \) depend on the value of the coefficient η. If η = 1 (geometrically homogeneous sheet), the curve becomes coincident with the first bisectrix. Thus this theory cannot model the strain localization for geometrically homogeneous sheets.
The value of the principal strain ε1a in region ‘a’ corresponding to non-significant straining of this region as compared to region ‘b’ (the straining being localized in region ‘b’) represents the limit strain ε1a* (Fig. 5.26). This strain together with the second principal strain ε2a* in region ‘a’ define a point of the Forming Limit Curve. By varying the strain ratios ρ = dε2/dε1, different points on the FLC are obtained. By scrolling the range 0 < ρ < 1, the FLC for biaxial tension (ε1 > 0, ε2 > 0) is obtained. In this range the orientation of the geometrical non-homogeneity with respect to the principal directions is assumed to be the same during the entire forming process.
The Marciniak model (1965) was further developed by Marciniak and Kuczynski (1967) and Marciniak et al. (1973), usually being briefly denominated the M-K model.
The M-K model was extended to the negative range of the FLD’s (ε 2 < 0) by Hutchinson and Neale (H-N model) (Hutchinson and Neale 1978a, 1978b, 1978c). The geometric H-N model is presented in Fig. 5.27. According with the original paper of Hutchinson and Neale (1978b), the inclination of the non- homogeneity varies with the main strains by a law having the form:
and the non-uniformity coefficient varies by a law having the form:
where, η 1 and η 0 are the current and initial non-uniformity coefficients, respectively.
5.4.4.2 Implicit Formulation of the M-K and H-N Models
Both M-K and H-N models assume that the strain localization is caused by a thickness imperfection represented as a groove in Fig. 5.27. According to this hypothesis, two regions of the sheet metal should be distinguished: A—non-defective zone; B—groove. At different stages of the straining process (identified by the time parameter t), the ratio
is used to describe the amplitude of the imperfection (\( {}^{t}s^{(A)} \) and \( {}^{t}s^{(B)} \) denote the current thickness of regions A and B, respectively—see Fig. 5.27).
Throughout this section, the sheet metal is considered to behave as an orthotropic membrane under the plane-stress conditions
The constraints written above are valid both for region A and region B. Equation (5.70) involves the components of the stress and strain-rate tensors expressed in the plastic orthotropy frame (1 and 2 are the indices associated to the rolling and transverse directions, respectively—see Fig. 5.27, while 3 is the index corresponding to the normal direction—not shown in Fig. 5.27).
One also assumes that the sheet metal is subjected to loads which do not produce tangential stresses and strains in the plastic orthotropy frame:
This constraint will be applied not only to the non-defective zone (as in the classical formulation of the H-N model), but also to the groove. Under such circumstances, the diagonal components of the stress and strain-rate tensors automatically become eigenvalues. In order to emphasize their significance, the following notations will be used:
The mechanical response of the sheet metal will be described by a rigid-plastic model. The main ingredient of the constitutive model is the yield criterion:
Equation (5.72) involves the following quantities:
\( {}^{t}\bar{\sigma} = {}^{t}\bar{\sigma}\left( {{}^{t}{\sigma}_{1} ,{}^{t}{\sigma}_{2} } \right) \ge 0 \)—equivalent stress (homogeneous function of the first degree)
\( {}^{t}\bar{\varepsilon} \ge 0 \)—equivalent (plastic) strain
\( {}^{t}Y = {}^{t}Y\left( {{}^{t}\bar{\varepsilon}} \right) > 0 \)—yield parameter controlled by a strictly increasing hardening law.
The non-zero components of the strain-rate tensor (considered fully plastic) are defined by the flow rule
and the incompressibility constraint
In order to preserve the simplicity of the formulation, one assumes that region A evolves along linear strain paths defined as follows:
Each strain path investigated when calculating a Forming Limit Curve will be identified by a constant value of the parameter \( \rho^{(A)} . \) Equation (5.75) automatically implies that \( {}^{t}\dot{\varepsilon}_{2}^{(A)} \) has the status of a minor principal strain-rate.
As shown in Fig. 5.27, the orientation of the groove is described by the angular parameter \( \varphi . \) One adopts the hypothesis \( 0^{ \circ } \le \varphi < 45^{ \circ } , \) thus considering that the necking band is closer to the direction of the minor principal strain-rate \( {}^{t}\dot{\varepsilon}_{2}^{(A)} . \) In order to find a formula for the calculation of the angular parameter \( \varphi , \) a local frame associated to the groove is defined. Its planar axes are individualized by the indices 1’ and 2’, being oriented as shown in Fig. 5.27. Let
be the strain-rate along the necking band. If \( - 1 < \rho^{(A)} \le 0, \) Eq. (5.76) could be used to find a zero-extension direction. Indeed, by enforcing
one obtains
i.e.
Equation (5.79) defines the orientation of the necking band for the left branch of the Forming Limit Curve. In fact, this formula is similar to that found by Hill for the same type of strain paths (Hill 1952).
If \( 0 < \rho^{(A)} \le 1, \) Eq. (5.76) does not allow the existence of zero-extension directions in the plane of the sheet metal. In such cases, as in the classical M-K model, one assumes that the necking band is oriented along the direction of the minor principal strain-rate \( {}^{t}\dot{\varepsilon}_{2}^{(A)} \):
Equations (5.79) and (5.80) can be unified in the general formula
It is easily noticeable that, for linear strain paths \( \left( {\rho^{(A)} = {\text{const.}}} \right), \) Eq. (5.81) implies the constancy of the angular parameter \( \varphi . \)
For any load state having the property \( {}^{t}{\sigma}_{1} > 0, \) the equivalent stress could be expressed as follows:
Equation (5.82) results from the fact that \( {}^{t}\bar{\sigma} \) is a first-degree homogeneous function. The partial derivatives \( \partial {}^{t}\bar{\sigma}/\partial {}^{t}{\sigma}_{\alpha} \left( {{\alpha} = 1,2} \right) \) are also homogeneous functions but of zero-degree. As a consequence, they are expressible under the form
The functions F and \( G_{\alpha} \left( {{\alpha} = 1,2} \right) \) are related only to the particular formulation of the equivalent stress adopted in the model. Equations (5.82) and (5.83) lead to the following expressions of the yield criterion and flow rule (see also Eqs. (5.72) and (5.73)):
The linear strain paths defined as in Eq. (5.75) fulfil the condition \( {}^{t}{\sigma}_{1}^{(A)} > 0. \) Under these circumstances, Eq. (5.85) can be applied to region A:
Equations (5.86) and (5.75) allow to obtain a relationship between \( \rho^{(A)} \) and \( {}^{t}\zeta^{(A)} \):
It is again noticeable that, for linear strain paths \( \left( {\rho^{(A)} = {\text{const.}}} \right), \) Eq. (5.87) implies the constancy of the principal stress ratio, i.e.
At the level of region A, Eqs. (5.84) and (5.85) can thus be written in the particular forms
Because the stress state in region B also fulfils the condition \( {}^{t}{\sigma}_{1}^{(B)} > 0, \) the corresponding ratio
can be defined. \( {}^{t}\zeta^{(B)} \) generally varies even if the strains in the non-defective zone evolve along a linear path. Due to this fact, Eqs. (5.84) and (5.85) should be written as follows when making reference to region B:
As in the classical formulation of the H-N model, two sets of constraints will be enforced at the interface between the regions A and B (see Fig. 5.29):
-
Continuity of the strain-rate along the necking band
$$ {}^{t}\dot{\varepsilon }_{2'2'}^{(A)} = {}^{t}\dot{\varepsilon }_{2'2'}^{(B)} $$(5.94) -
Equilibrium of the normal and tangential loads acting on the interface from both sides
$$ {}^{t}\sigma_{1'1'}^{(A)} \cdot {}^{t}s^{(A)} = {}^{t}\sigma_{1'1'}^{(B)} \cdot {}^{t}s^{(B)} , $$(5.95)$$ {}^{t}\sigma_{1'2'}^{(A)} \cdot {}^{t}s^{(A)} = {}^{t}\sigma_{1'2'}^{(B)} \cdot {}^{t}s^{(B)} . $$(5.96)
By making use of the thickness-defect parameter \( {}^{t}f \) (see Eq. (5.69)), one rewrites Eqs. (5.95) and (5.96) in the equivalent forms
The rotated tensor components involved in Eqs. (5.97) and (5.98) can be also expressed in terms of the principal stresses, thus obtaining
Because \( 0^{ \circ } \le \varphi < 45^{ \circ } , \) the above relationships may be rewritten as follows:
Finally, with the help of the principal stress ratios associated to regions A and B (see Eqs. (5.88), (5.91)), (5.101) and (5.102) become
In general, Eq. (5.103) cannot reduce to the trivial case 0 = 0. Under such circumstances, it is possible to divide Eqs. (5.104) by (5.103). After some simple manipulations, one obtains the following relationship between the principal stress ratios associated to regions A and B:
For the strain paths characterized by the condition \( - 1 < \rho^{(A)} < 0, \) Eq. (5.81) defines an angular parameter \( 0^{ \circ } < \varphi < 45^{ \circ } . \) In this case, Eq. (5.105) enforces \( {}^{t}\zeta^{(B)} = \zeta^{(A)} = {\text{const.}} \) The principal stress ratios associated to regions A and B are thus rigorously coincident and constant when \( - 1 < \rho^{(A)} < 0. \)
The plane-strain path \( \rho^{(A)} = 0 \) needs a separate discussion, as in this case Eq. (5.81) defines an angular parameter \( \varphi = 0^{ \circ } \) and Eq. (5.105) degenerates to the trivial form 0 = 0. When \( \varphi = 0^{ \circ } , \) the local frame associated to the groove is superimposed to the plastic orthotropy frame (1 = 1’ and 2 = 2’). The constraints given by Eqs. (5.77) and (5.94) now reduce to \( {}^{t}\dot{\varepsilon}_{2}^{(A)} = {}^{t}\dot{\varepsilon}_{2}^{(B)} = 0, \) meaning that region B evolves along the same plane-strain path and enforcing again the constancy of the principal stress ratio: \( {}^{t}\zeta^{(B)} = \zeta^{(A)} = {\text{const}}. \) One may thus conclude
For all the strain paths characterized by the condition \( 0 < \rho^{(A)} \le 1, \) Eq. (5.81) defines an angular parameter \( \varphi = 0^{ \circ } . \) In this case, Eq. (5.105) also degenerates to the trivial form 0 = 0, but Eq. (5.94) will not enforce the constancy of the stress ratio in region B as it takes the more general form \( {}^{t}\dot{\varepsilon}_{2}^{(A)} = {}^{t}\dot{\varepsilon}_{2}^{(B)} \) .
One may notice that, whatever is the value of the parameter \( \rho^{(A)} \) in the range \( - 1 < \rho^{(A)} \le 1, \) the equilibrium constraint given by Eq. (5.103) reduces to
due to Eqs. (5.106) and (5.81). For all the strain paths characterized by the condition \( - 1 < \rho^{(A)} \le 0, \) the above relationship becomes even simpler when combined with Eqs. (5.89), (5.92) and (5.106):
Equation (5.108) makes redundant the second equilibrium constraint expressed by Eq. (5.104). In fact, Eq. (5.108) has been deduced using Eq. (5.106) which is a corollary of Eq. (5.104).
In the case \( 0 < \rho^{(A)} \le 1, \) Eqs. (5.89) and (5.92) can be exploited to reformulate Eq. (5.107) as follows:
Again, Eq. (5.109) should not be accompanied by Eq. (5.104) because the second equilibrium constraint now degenerates to the trivial form 0 = 0.
The strain-compatibility enforced by Eq. (5.94) also deserves a discussion. In the case \( - 1 < \rho^{(A)} \le 0, \) this constraint becomes trivial (0 = 0) and redundant due to Eqs. (5.81) and (5.106) already included in the model. For the remaining strain paths \( 0 < \rho^{(A)} \le 1, \) Eq. (5.94) reduces to the simpler formulation (see also Eqs. (5.80), (5.90) and (5.93))
Equation (5.110) is non-trivial and accompanies Eq. (5.109) in the model used to calculate the right branch of the Forming Limit Curve.
The discussion below will focus on the presentation of the computational strategy used to solve the strain localization model. The evolution of the sheet metal up to the necking is analyzed for individual strain paths. Each of these paths is defined by a constant value of the parameter \( \rho^{(A)} \) in the range \( - 1 < \rho^{(A)} \le 1. \) The straining process is analyzed in an incremental manner. Let \( \left[ {T,T +\Delta T} \right] \) be the discrete time interval corresponding to one of the steps performed in the analysis. All the parameters associated to the T moment are known quantities both for the non-defective area and the groove. The corresponding configuration of the sheet metal is thus taken as a reference state. In particular, the parameters associated to the moment \( T = 0 \) are defined by the conditions \( {}^{0}\bar{\varepsilon}^{(A)} = {}^{0}\bar{\varepsilon}^{(B)} = 0, \) and \( {}^{0}{\varepsilon}_{\alpha}^{(A)} = {}^{0}{\varepsilon}_{\alpha}^{(B)} = 0 \) \( \left( {{\alpha} = 1,2} \right). \) The initial value of the thickness ratio \( 0 < {}^{0}f < 1 \) is also prescribed. As concerns the parameters corresponding to the \( T + \Delta T \) moment, they are unknown quantities and should be evaluated.
The computation is conducted by applying small increments of the equivalent strain to region A. In order to obtain sufficiently accurate results, these increments should remain small. During the numerical tests performed by the authors, \( \Delta \bar{\varepsilon}^{(A)} = 10^{ - 3} \div 10^{ - 4} \) has proved to be a good selection range.
Due to the fact that \( \rho^{(A)} \) uniquely defines the ratio of the principal stresses in region A, the parameter \( \zeta^{(A)} \) should be evaluated only once, namely at the beginning of each strain path. This task is accomplished by solving the equation (see Eqs. (5.87) and (5.88))
with respect to the unknown \( \zeta^{(A)} . \) In general, numerical procedures must be used to evaluate \( \zeta^{(A)} . \) During the tests performed by the authors, the bisection method has worked very well, especially when combined with a bracketing strategy.
As soon as \( \zeta^{(A)} \) is known, the increments of the principal strains in region A can be evaluated from Eq. (5.90) rewritten as
One may also notice that, for a given strain path, \( \Delta {\varepsilon}_{\alpha}^{(A)} \) \( \left( {{\alpha} = 1,2} \right) \) are constant quantities and should be computed only once.
At this stage, the parameters associated to the non-defective area of the sheet metal can be updated using the formulae
The solution procedure is now prepared to evaluate the groove parameters corresponding to the \( T + \Delta T \) moment. If \( - 1 < \rho^{(A)} \le 0 \) (left branch of the forming limit curve), the principal stress ratios are the same in regions A and B (see Eq. (5.106)). In this case, only the increment of the equivalent strain \( \Delta \bar{\varepsilon}^{(B)} \) should be found as a solution of Eq. (5.108) written for the \( T + \Delta T \) moment:
where the current thickness ratio \( {}^{T + \Delta T}f \) is expressible from Eqs. (5.69) and (5.74)
with \( \Delta {\varepsilon}_{\alpha}^{(B)} \) \( \left( {{\alpha} = 1,2} \right) \) resulting from Eqs. (5.93) and (5.106):
Equation (5.114) can be solved only in a numerical manner. Again, during the tests performed by the authors, the bisection method has proved excellent performances in combination with a bracketing strategy. After \( \Delta \bar{\varepsilon}^{(B)} \) is determined, the increments of the principal strains in region B can be easily evaluated from Eq. (5.116).
In the case \( 0 < \rho^{(A)} \le 1 \) (right branch of the Forming Limit Curve), the principal stress ratio associated to region B is no longer constant. As a consequence, two unknown quantities should be determined. They are the current principal stress ratio \( {}^{T + \Delta T}\zeta^{(B)} \) and the increment of the equivalent strain \( \Delta \bar{\varepsilon}^{(B)} . \) Fortunately, the strain-rate along the necking band does not vanish if \( 0 < \rho^{(A)} \le 1. \) Under such circumstances, Eq. (5.110) can be put in an incremental form and used to express \( \Delta \bar{\varepsilon}^{(B)} \) as a dependency on \( {}^{T + \Delta T}\zeta^{(B)} \) (see also Eq. (5.112)):
\( \Delta \bar{\varepsilon}^{(B)} \) given by Eq. (5.117) should be replaced in Eq. (5.109) written for the \( T + \Delta T \) moment, thus obtaining
The current thickness ratio \( {}^{T + \Delta T}f \) is still defined by Eq. (5.115), but the principal strain increments \( \Delta {\varepsilon}_{\alpha}^{(B)} \) \( \left( {{\alpha} = 1,2} \right) \) result now from a more complicated flow rule (see Eqs. (5.93) and (5.117)):
In conclusion, Eqs. (5.115) and (5.119) will bring Eq. (5.118) to a formulation involving only \( {}^{T + \Delta T}\zeta^{(B)} \) as unknown. Again, the numerical solution can be found using the bisection method combined with a bracketing strategy. After \( {}^{T + \Delta T}\zeta^{(B)} \) is determined, Eqs. (5.117) and (5.119) allow the evaluation of the increments \( \Delta \bar{\varepsilon}^{(B)} \) and \( \Delta {\varepsilon}_{\alpha}^{(B)} \) \( \left( {{\alpha} = 1,2} \right), \) respectively.
At this stage, the parameters associated to the defective area of the sheet metal can be updated using the formulae
The procedure described above is simple and efficient. Both for the left and right branches of the Forming Limit Curve, the problem consists in solving a unique non-linear equation. At the level of region A, it is always possible to find a solution by numerical techniques. Region B needs a more careful treatment from this point of view. Generally, strains accumulate faster in the groove. As previously shown, the model tries to enforce the equilibrium of the tractions along the interface with the non-defective area of the sheet metal. At higher strain levels, the bearing capability of the groove can be limited by the hardening law. In such cases, it is not possible to find the solution at the level of region B. The bearing limitation can be trapped by testing the value of the equivalent strain increment \( \Delta \bar{\varepsilon}^{(B)} \) during the bracketing procedure. If the search for an initial guess fails even for very large increments \( \Delta \bar{\varepsilon}^{(B)} \) one may deduce that region B has already attained its bearing limit. From a mechanical point of view, this situation corresponds to the occurrence of the necking phenomenon in the groove. As a consequence, the current values of the principal strains in region A should be considered as defining the limit state of the sheet metal.
The occurrence of the necking must be also checked after finding a numerical solution for the groove. Normally, the ratio \( \Delta \bar{\varepsilon}^{(B)} /\Delta \bar{\varepsilon}^{(A)} \) should be tested. If this quantity becomes very large (\( \Delta \bar{\varepsilon}^{(B)} /\Delta \bar{\varepsilon}^{(A)} \) > 100, for example), one may conclude that the necking has been initiated. The inspection of the strain path should be stopped as the current values of the principal strains in region A define the limit state. If the ratio \( \Delta \bar{\varepsilon}^{(B)} /\Delta \bar{\varepsilon}^{(A)} \) is not great enough, the computation will continue after applying a new increment of the equivalent plastic strain \( \Delta \bar{\varepsilon}^{(A)} \) to region A.
Different formulations of the equivalent stress (von Mises, Hill 1948; Barlat 1989, and Banabic et al. 2005a) and hardening laws (Hollomon, Swift, Voce, Ghosh, Hockett-Sherby, and AUTOFORM) have been implemented in the strain localization model presented above. In all cases, the numerical tests have shown a very good stability and robustness of the solution procedure. In order to validate the performances of the computational algorithm, its predictions have been compared with experimental data corresponding both to steel and aluminium alloys. As an example, Fig. 5.28 shows the comparison between the numerical results and the experimental data included in Benchmark 1 of the NUMISHEET 2008 conference (Volk et al. 2008) for the case of the AA5182-O aluminium alloy.
H-N prediction versus experiments (Volk et al. 2008) for AA5182-O aluminium alloy
5.4.4.3 Comparison of the FLC’s Predicted by Different Theoretical Models
During the last five decades, the theoretical model developed by Marciniak and Kuczynski Marciniak (1965) has been intensively used for calculating forming limit curves. More recently, several other approaches have been proposed. Among them, Hora’s MMFC model (Hora and Tang 1994) and its extension to the so-called EMFC model developed by Mattiasson and his co-workers Mattiasson et al. (2006) are also attractive due to their simplicity and good performances.
Figure 5.29 shows a comparison of the FLC’s predicted by the theoretical models mentioned above with experimental data obtained by the authors in the case of an AA6016-T4 sheet metal (1 mm thickness). All the calculations have been performed using the same plasticity model namely, the BBC2005 yield criterion and Swift’s hardening law. As one may notice in Fig. 5.29, the FLC’s predicted by the M-K and EMFC models are in very good agreement with the experimental data. The quality of the predictions given by the MMFC model is poorer. It seems that Hora’s assumption of a sudden evolution towards the plane-strain state in the necking region causes an understimation of the formability along the right branch of the FLC. From this point of view, the hypotheses on which the EMFC model is based are more realistic. It is also noticeable the characteristic peak on the left branch of the FLC predicted by the MMFC model. This strange behaviour is the consequence of a mathematical singularity that cannot be removed from Hora’s MMFC model (see Sect. 5.4.1.2 and Aretz (2004) for details).
5.4.4.4 Non-zero Thickness Stress
In sheet forming processes that imply a non-zero sheet curvature, e.g. in punch stretching and hydraulic bulging, the thickness stress varies from a minimum (i.e. compressive stress) at the concave side of the sheet to zero at the (stress-free) convex side. This stress component is much smaller than in-plane stresses (at least for not too high sheet curvatures) and are therefore usually neglected in sheet forming limit models. Under certain conditions however, the average thickness compressive stress can be very high and non-negligible, e.g. in double-sided hydroforming, during which pressure is applied to both sides of the sheet with a certain pressure difference, and in pinching regions in pressing operations (where there is double-side tool contact).
The effect of the normal pressure on the formability of sheet metals is well known and already used from long time ago in industry by Keeler (1970). During some forming operations (hydrostatic forming, incremental forming) the sheet is subjected to a significant normal pressure. Bridgman (1952) studied first time the influence of the hydrostatic pressure on the formability. Later this influence has been the subject of numerous experimental investigations, especially by Spitzig and Richmond (1984). The general conclusion was that the yield stress decrease and the formability increase with superimposed hydrostatic pressure. The increase in formability is usually explained by the closing of the micro-voids in the sheet and the slowing down of the nucleation of new ones due to the normal pressure exerted by the surrounding fluid Padwal et al. (1992). A systematic analysis of sheet failure under normal pressure without assuming ductile damage has been done in the last decades. The first theoretical analysis was performed by Ciumadin et al. (1990). Using a simple analytical model (similar with the Marciniak-Kuczynski model) they succeed to calculate the FLC for different values of the hydrostatic pressure. Gotoh et al. (1995) used the classical Swift’s and Hill’s criterion of instability and Stören and Rice’s condition together with the constitutive model developed by himself for localized necking. In Smith et al. (2003) and later in Matin and Smith (2005), forming limit equations are derived for a sheet deformation under a constant and non-zero ratio of sheet thickness stress over major in-plane stress. These models are based on the assumption of a strain-path independent FLSD, which is extended in the sense that the forming limit stress is also assumed to be independent of the sheet thickness stress. In Smith et al. (2003), the ratio of minor to major in-plane stresses are assumed to be constant during deformation, while in Matin and Smith (2005), it is the ratio of minor to major in-plane strains which is fixed.
Banabic and Soare (2008), Wu et al. (2009), Allwood and Shouler (2009) have analyzed the influence of the normal pressure on the Forming Limit Curve using an enhanced Marciniak model. The results presented in the last papers are closed one to another one. In the Fig. 5.32 is presented this influence based on the modified Marciniak model (Banabic and Soare 2008).
Figure 5.30 shows a significant increasing of the limit strains for any strain path (more significant in the equi-biaxial region) with the increasing of the superimposed hydrostatic pressure. Allwood and Shouler (2009) included in the MK model a six component stress tensor and proposed a new generalized forming limit diagram (GFLD) showing the influence on the limit strains both of the normal and through thickness stresses (see Fig. 5.31 and Allwood and Shouler (2009)).
The generalized Forming Limit Diagram for AA1050 aluminium alloy (Allwood and Shouler 2009)
The effect of the through-thickness normal stress on the forming limit diagram has been studied extensively in the last five years using different anisotropic yield criteria by Assempour et al. (2010), Liu and Meng (2012), Zhang et al. (2012), (2014a, b), Nurcheshmeh and Green (2014), Lang et al. (2015), Wang et al. (2015). A clarification of the influence of the hydrostatic pressure on the yield and flow of the metallic materials has been presented by Soare and Barlat (2014). A new approach to analyze the effect of hydrostatic pressure on the deformability of sheet metals was introduced by Xue (2010). He extended the one dimensional Considère condition for arbitrary three dimensional loadings (taking into account the hydrostatic pressure) and applied it to sheet metal forming. The bifurcation point for the localized deformation is considered from the sense of energy dissipation. Xue (2010) applied his models to calculate both the necking and the fractured limit strains, respectively (see Fig. 5.32 and Xue (2010) for the AA5182-0 aluminium alloy). With the proposed method the influence of the pressure on the limit strains has been also analysed.
The trend in formability that all these models show is that a more negative thickness stress (i.e. more compression) delays the onset of the localized necking instability, which is the same trend found in some experimental investigations mentioned above.
5.4.4.5 Non-zero Through-Thickness Shear Stress
Through-thickness shearing (TTS) in sheet metal is induced through sliding friction, especially against highly curved tooling for which normal contact pressure is high. Typical examples of these conditions may be found in incremental sheet forming and in the deep drawing over relatively sharp die corners and across draw beads. Research on the effect of TTS on formability has been originally initiated by the unusually high formability observed in incremental sheet forming (Allwood and Shouler 2009; Eyckens et al. 2009, 2011).
Before extending the MK framework to include TTS and giving some numeric examples on the effect of TTS on FLCs, we first look into a mathematical description of TTS. If the sheet undergoes in-plane deformation combined with through-thickness shearing, the strain mode can no longer be captured by a single variable. The plastic strain rate tensor \( {\mathbf{D}} \) can in this case be expressed as:
Here \( D_{11}^{{}} \) is the major in-plane strain rate. In (5.121) it is used as a scaling factor to define the in-plane strain mode \( \rho_{22} \), and also two independent ‘through-thickness shear modes’: \( \rho_{13} \) (in the direction 1, i.e. the major in-plane strain direction) and \( \rho_{23} \) (in the direction 2, i.e. the minor in-plane strain direction). From tensor analysis the relative through-thickness shearing along all other direction in the sheet plane may be derived, cf. (Eyckens et al. 2011). A typical result is visualized in the polar plot given by Fig. 5.33. The lengths of the lines that start from the origin of the coordinate system and that are bound by the grey-shaded area, reflect the magnitude of relative TTS along the respective directions. Along the 1- and 2-directions, these lengths correspond to \( \rho_{13} \) and \( \rho_{23} \) , respectively. Two enclosing, circle-shaped lobes can be seen: one has a positive sign of TTS associated with it, while the other has negative sign. Along the in-plane direction that separates these lobes, no TTS is acting. It may be shown that such a direction always exists, for any possible combination of \( \rho_{13} \) and \( \rho_{23} \) . At 90° with respect to this direction of ‘zero TTS’, the TTS has the maximal magnitude among all in-plane directions. The maximal magnitude of TTS is called the imposed TTS, and written as \( \rho_{TTS} \). It is imposed along the direction given by the angle \( {\alpha}_{TTS} \) with respect to the major in-plane straining direction. There is a one-to-one conversion between the sets of (\( \rho_{TTS} ,{\alpha}_{TTS} \) ) on one hand and (\( \rho_{13} ,\rho_{23} \) ) on the other hand.
Polar plot giving an example of the direction (\( {\alpha}_{TTS} \) ) and magnitude (\( \rho_{TTS} \) ) of the imposed through-thickness shear (TTS) onto a sheet, as well as the resulting TTS along any other in-plane direction (straight lines bounded by the grey area). Figure reproduced from Eyckens et al. (2011)
The generalization of the MK formability framework towards TTS, concerns both the force equilibrium equations and the geometric compatibility equations (Eyckens et al. 2009).
Extension of force equilibrium is straightforward: equilibrium equations of the in-plane normal and the in-plane shear components of force, are supplemented with one equilibrium equation of the out-of-plane shear component of force. The resulting set of 3 equilibrium equations is the scalar equivalent of the imposed equilibrium of the force vectors on either side of the boundary plane between matrix and groove: \( {\vec{f}}^{a} = {\vec{f}}^{b} \)
Geometric compatibility between matrix and groove is generally expressed in terms of the velocity gradients, which intrinsically considers not only the strain rate but also the rate of rigid body rotation. The compatibility conditions refer to components of the velocity gradient tensors within the matrix (\( {\mathbf{L}}^{\varvec{a}} \) ) and the groove (\( {\mathbf{L}}^{\varvec{b}} \) ), as expressed in a reference frame that is aligned with the (instantaneous) directions along the groove (t-direction) and normal to the groove within the sheet plane (n-direction). As demonstrated in Fig. 5.34a, 5 out of the 9 independent components of the velocity gradient need to be equal in both regions in order to ensure compatibility. Consequently, the remaining 4 components of the velocity gradient within the groove, are not constrained by their counterparts in the matrix material, as illustrated Fig. 5.34b.
a Geometric compatibility conditions and b unconstrained (i.e. ‘free’) components of the velocity gradient of the groove material. The initial configuration is depicted with wireframe. Figure reproduced from Eyckens et al. (2009)
Results from this extended MK model demonstrate that TTS may have a beneficial effect on formability, and that this depends not only on the magnitude but also on the direction of imposed TTS. Let us consider first the case of plane strain deformation with additional TTS along the direction of zero extension (minor in-plane strain direction), which is sketched in Fig. 5.35a. The (fixed) direction of the MK groove that determines the limit of formability, coincides in this case to the minor in-plane strain direction. The imposed through-thickness shearing induces non-zero out-of-plane shear stress \( {\sigma}_{t3} \) in both matrix and groove, while in the sheet plane, the plane strain deformation induces non-zero σ nn (= σ11) and σ tt (= σ22). It can be shown that for a von Mises material loaded under these deformation conditions, the stress mode u (in both matrix and groove) is contained in the hyperplane σ nn = 2σ tt, as shown in the 3-dimensional yield locus section. The matrix’ stress mode u a is fixed (monotonic loading). The groove stress mode u b however evolves during deformation, from close to u a towards the plane strain stress mode (indicated by a cross marked ‘PS’). This stress mode change is accompanied with a relative increase of σnn-component of stress, thereby delaying the onset of strain localization within the groove that is governed by equilibrium of the normal force component.
Plane strain loading superimposing TTS along a the direction of zero extension (coinciding with groove direction t) and b the major in-plane strain direction (coinciding with groove normal direction n). For both a and b, the relevant 3-dimensional section in stress space for a von Mises material is shown: major in-plane stress (σnn), minor in-plane stress (σtt) and the non-zero out-of-plane shear stress (σt3 for a and σn3 for b, respectively). For case a, the groove stress mode u b evolves towards the plane strain point ‘PS’ during deformation, while in case b it remains constant and equal to the matrix’ stress mode u a. Figure composed from Eyckens et al. (2009)
The results are however very different for plane strain with superimposed TTS along the major in-plane strain, cf. Fig. 5.35b. The critical direction of the MK groove with respect to formability, is also in this case along the direction of zero extension. TTS induces a non-zero σ n3 -component of stress. It can be derived from force equilibrium that the ratio σ n3 /σ nn is equal for both matrix and groove, which leads to the second hyperplane containing u a and u b that is drawn in Fig. 5.35b. As a result, the groove stress mode is required to be equal to the matrix stress mode, meaning that the stabilizing mechanism of groove stress mode evolution towards the plane strain point does not occur.
Figure 5.36 shows FLC predictions with increasing TTS along the minor in-plane strain direction (i.e. \( {\alpha}_{TTS} = 90^\circ \) ). Except for equibiaxial loading, imposing TTS along this direction generally improves formability. Figure 5.37 further illustrates the high sensitivity of the forming limit with respect to the direction of imposed TTS. TTS along the major in-plane strain direction (\( {\alpha}_{TTS} = 0^\circ \) ) does not significantly affect FLC (compare to FLC curve of \( \rho_{TTS} = 0 \) in the Fig. 5.38). The beneficial effect of TTS on the FLC is maximal if it is imposed along the minor in-plane strain direction (\( {\alpha}_{TTS} = 90^\circ \) ).
Calculated FLCs for materials with von Mises yield locus and with an anisotropic yield locus of AA3103 aluminium alloy, having various degrees of magnitude of imposed through-thickness shear \( \rho_{TTS} \) (different symbols). Direction of imposed TTS is given by: \( {\alpha}_{TTS} = 90^\circ \). Figure reproduced from Eyckens et al. (2011)
Calculated FLCs for materials with an anisotropic yield locus of AA3103 aluminium alloy, with varying imposed directions of TTS (\( {\alpha}_{TTS} \)). The magnitude of imposed TTS is \( \rho_{TTS} = 0.4. \) Figure reproduced from Eyckens et al. (2011)
Three theoretical FCC textures leading to significant differences in FLCs, from Yoshida and Kuroda (2012)
5.4.5 Crystal Plasticity Based FLC Prediction
Crystal plasticity modelling considers the main microstructural aspects of metallic alloys, namely the distribution of the individual metallic crystals or grains (with associated crystal orientation), possibly augmented with information of the morphologic appearance of microstructure, second phase particles (if present), or other microstructural aspects. Crystal plasticity modelling is in essence a multi-scale approach, linking the physical processes that accommodate plastic deformation on the scale of the crystal (e.g. plastic slip, deformation twinning) to the homogenized or macroscopic behaviour of the polycrystalline metallic aggregate. The microstructure determines the yield locus shape, which is intrinsically incorporated in crystal plasticity modelling. For single-phase materials with near-equiaxed grain morphology, the dominant microstructural feature linked to yield locus shape is the crystallographic texture. Deformation-induced evolution of texture will result in yield locus shape changes. Such effects can be readily studied by crystal plasticity.
In recent year, two different approaches have been mainly followed to couple crystal plasticity calculations to sheet formability prediction, namely the MK framework and FE modelling. They will be discussed next.
The development of ductile damage or voids in the microstructure, and their correlation to macroscopic forming limits, is another topic that has received significant attention in recent literature. An overview of such studies is also included.
5.4.5.1 Crystal Plasticity in MK Analysis
The pioneering studies in this field adopted a crystal-plasticity-derived yield locus in MK model framework (Barlat 1989; Bassani et al. 1979), thereby neglecting effects of deformation texture or other microstructural evolutions. In later years, the influence of deformation-induced texture evolution was explicitly investigated by direct coupling of the crystal plasticity as a material model. Due to limited computation power, the earlier investigations often made additional assumptions, e.g. that necking band is perpendicular to major strain direction (Zhou and Neale 1995), or that texture evolution should be identical in groove and surrounding matrix (Ratchev et al. 1994). In Tóth et al. (1996), the study of the formability of three measured aluminium textures shows the effect of texture evolution to equalize the forming limits of the different textures and lower them (in most strain paths of the right-hand side of the FLD). These earlier studies often adopt the Taylor-Bisschop-Hill polycrystal plasticity model.
Over the last decade, significant attention has been given to the study of ‘idealized textures’, in particular for aluminium (FCC structure). Such theoretical textures consist of randomly-generated orientations with certain spread around a single orientation (Wu et al. 2004a) or orientation fibre (Yoshida and Kuroda 2012). Formability is not only very sensitive to the chosen ideal orientation, but also to the texture spread around the central orientation (Wu et al. 2004a). This dependency can be linked to the sharpness of yield locus in the biaxial range (Wu et al. 2004a). The effect of the cube texture component (main recrystallization texture component in aluminium) is studied with a rate-dependent plasticity model in Wu et al. (2004a), where it was found that the spread around the ideal texture component determines the yield locus surface near equibiaxial stretching deformation to affect the formability under this deformation mode. However, the initial shape of yield locus does not completely determine formability in right-hand side of FLD. So-called distortional or textural hardening, i.e. yield locus shape change due to texture evolution, is as important a factor (Signorelli et al. 2009). In aluminium alloys for example, the cube fibre textures gives high forming limits due to the beneficial distortional hardening effect, in spite of an unfavourable initial yield locus shape with low r-value (Yoshida and Kuroda 2012).
In summary, in aluminium materials (FCC), rolling texture components generally have a negative effect on biaxial formability (Yoshida et al. 2007), whereas the cube texture (recrystallization texture component) can be beneficial (Wu et al. 2004a; Yoshida et al. 2007). Fewer studies have been done on BCC cubic materials such as ferritic steels; the study of Inal et al. (2005) suggests that sensitivity of texture evolution on formability is much less for BCC compared to FCC materials. For Mg sheet alloy (HCP crystal structure), besides dislocation-based plasticity also the mechanical twinning needs to be considered (Neil and Agnew 2009). Additional hardening due to twinning can promote the resistance to localized necking. Also in Wang et al. (2011), formability of Mg alloy is investigated, both with Taylor and VPSC models. Results show that texture evolution decreases forming limit under uniaxial and biaxial deformation paths, while it has little effect under near-plane strain deformation. Numerical investigation also reveals that formability can be significantly improved by altering the texture, in particular a rotation of the basal texture component.
Coupling of crystal plasticity with formability analysis that is highly no-linear requires sufficient attention to algorithmic implementation. For time integration scheme of MK model with crystal plasticity-based material modelling, it is referred to Knockaert et al. (2002); where focus is put on the consequences of texture evolution on the overall algorithm. Kim et al. (2013) compare Newton-Raphson and Nelder-Mead time integration schemes for crystal plasticity-based MK simulations.
In the current state-of-the art, crystal plasticity-based formability modelling does indeed give insightful guidelines with respect to optimized microstructure (Yoshida et al. 2009; Yoshida and Kuroda 2012; Wang et al. 2011). Figure 5.38 gives an example of increased theoretical formability through texture optimization. Even though this makes crystal plasticity an appealing approach to predict formability, often no clear improvement in FLC prediction is obtained from crystal plasticity with respect to recent phenomenological material modelling approaches (Chiba et al. (2013), cf. Fig. 5.39).
Comparison of advanced phenomenological and crystal plasticity material models in FLC prediction, from Chiba et al. (2013)
Advanced crystal plasticity models, which incorporate grain interaction and non-equiaxed grain morphology, can improve accuracy of FLC predictions compared to more basic Taylor crystal plasticity model, in which the local deformation inside each crystal is unaffected by its environment in the microstructure (Signorelli et al. 2009). For complex microstructures such as multi-phase materials, a microstructural representative volume element (RVE) may be defined through FE modelling to produce the constitutive behaviour in formability modelling framework. In Tadano et al. 2013, such RVE material modelling approach for single-phased material is used in MK analysis.
5.4.5.2 Formability Modelling Through Crystal Plasticity in FEM (CPFEM)
In Wu et al. (2004b), the FE mesh under consideration corresponds to a unit cell of the polycrystalline material. Each integration point corresponds to a grain and obeys single crystal plasticity theory. A discretized aluminium texture is used to assign grain orientations to specific integration points of the mesh, which is done arbitrarily. It is shown that a forming limit band rather than a single curve is obtained when several arbitrary assignments of grains to the FE mesh are considered. Texture evolution results in higher formability predictions on the right-hand side of the FLD for the material under study. It is emphasized that this approach does not require the ‘fitting’ parameter of the initial imperfection of the MK model, but on the other hand it is indicated that forming limits depend on the number of elements (i.e. grains) considered in the analysis. In Yoshida (2014), deformation-induced surface roughening and strain localization is investigated by crystal plasticity FE analysis, in particular regarding their dependency on grain size and number of grains over the sheet thickness. If the sheet has relatively few grains over the sheet thickness (less than 30), plane strain formability is found to be reduced due to significant surface roughening.
In Inal et al. (2002a, b) the Taylor theory of rate-dependent crystal plasticity is used in a plane-strain Crystal Plasticity Finite Element Model (CPFEM) to simulate the localization of deformation under plane strain tension. Strain localization occurs in two phases, firstly a through thickness neck is initiated, after which shear bands are formed within the neck. Texture evolution and strain rate sensitivity were identified as crucial parameters for shear band localization, since shear localization was shown to be completely suppressed when texture evolution during deformation was not considered or when strain rate sensitivity was taken to be high enough.
Hu et al. (2008) apply CPFEM (using a plane stress model) to simulate the onset of necking in uniaxial tension of an aluminium alloy containing second phase particles about an order of magnitude smaller than the average grain size. They found that necking band formation is determined mainly by the arrangement of the soft and hard grains in the model, with only a small influence of particle volume fraction and type of particle distribution (i.e. homogeneous or aligned in stringers). Also post-necking behaviour was studied with a plane strain model, showing the large influence of the type of particle distribution: a homogeneous distribution leads to a well-developed neck (i.e. a large fracture strain) with cup-and-cone ductile fracture, while low fracture strains are found in combination with a shear-type fracture in the presence of particle stringers. Experimentally it is also found that the presence of stringers (which is related to the method of sheet casting) produce a shear-type dominant failure with small necking.
5.4.6 Void Growth Based FLC Prediction
In Saanouni (2008), the isotropic damage model of Gurson is implemented into implicit and explicit FE analysis to predict the onset of necking in a hydraulic bulging process. The same material model is also used for prediction of the onset of ductile fracture in numerous bulk forming processes. Haddag et al. (2008) presents a FLC obtained from the implementation of the isotropic damage model of Lemaitre into implicit FE code. The damage parameters were obtained by inverse modelling from uniaxial, plane strain and equibiaxial tension tests and a simple shear test. The same authors apply a similar constitutive modelling approach to Rice’s bifurcation theory for FLC prediction in Haddag et al. (2009). Achani et al. (2007) use the isotropic damage model of Cockcroft and Latham to predict the onset of necking in a plane strain tension test after model parameter identification using a pure shear test. In Uthaisangsuk et al. (2008), the Nakazima test is simulated with FEM including the Gurson-Tvergaard-Needleman damage model. The damage parameters are estimated based on metallographic observations and other experimental techniques. The shape of the FLC obtained from the different FE simulations appears to be different than the experimental FLC, although the position of the numeric FLD corresponds reasonably well to the experiment.
5.4.6.1 Modelling of FLC using the GTN Model
One of the recently proposed approaches for FLC construction is the application of damage model. The determination of forming limits of metallic sheets using the GTN damage model has been performed according to different approaches. The most common ones include the approaches that use the numerical simulation of the traditional formability tests prdoposed by Nakazima an Kikuma (1967) and Marciniak and Kuczynski (1967) and the approaches that combine the GTN model with the Marciniak-Kuczynski model (Marciniak and Kuczynski 1967). This section introduces these approaches and their application in the construction of FLCs.
5.4.6.2 FLC Prediction by Numerical Simulation of Traditional Formability Tests
The Nakazima and Marciniak tests are standard traditional tests for the determination of FLCs. In these tests, metallic sheets with different shapes are stretched against a punch up to the onset of necking/fracture. By measuring the planar limit strains at the onset of necking, the Forming Limit Diagram (FLD) is plotted. Like the experimental tests, the forming limit strains of metallic sheets can be predicted by numerical simulation of Nakazima and Marciniak tests. On the other hand, the accuracy of the predicted limit strains will be affected by the necking criterion used in the simulations. One of the useful tools for the prediction of necking and determination of limit strains is the application of the GTN damage model. The GTN model defines the plastic behaviour of the sheet metal and its failure due to void evolution.
The effect of void growth on the forming limits was investigated by employing Gurson damage model (Gurson 1977) in the FE analysis, within the framework of membrane theory, of a punch stretching test (Chu 1980). The effect of other mechanisms of void evolution i.e. void nucleation and coalescence can be included in the investigations by using the GTN damage model (Abbasi et al. 2011, 2012a; Yoon et al. 2013). Abbasi et al. (2011), (2012a) predicted the FLC of an IF steel using an isotropic GTN damage model. A set of rectangular specimens with a length of 200 mm and different widths of 25, 50, 75, 100, 125, 150, 175 and 200 mm were simulated according to the Hecker test (Banabic et al. 2000). All specimens were formed until the final void volume fraction \( f_{F} \) for an element was fulfilled. The value of major and minor strains at the element with void volume fraction of \( f_{F} \) was selected as the failure strains. The constructed FLC based on the measured failure strains was in good agreement with experiments (see Fig. 5.40).
Comparison of FLDs obtained by experiments and GTN model Abbasi et al. (2012b)
As the metallic sheets are commonly produced by rolling, they exhibit high levels of anisotropy. Investigations using anisotropic GTN damage models show that the plastic anisotropy of the matrix in ductile sheet metal influences both the deformation behaviour and damage evolution of the material Chen and Dong (2009). Regarding this fact, Brunet et al. (1996) employed an anisotropic Gurson-Tvergaard model Gurson (1977), Tvergaard (1981) with Hill’48 quadratic yield criterion Hill (1948) in the analysis of necking in the simulation of a deep drawing process. The FLC of a mild steel sheet was determined by the numerical simulation of Marciniak tests. Kami et al. (2015) also used the anisotropic GTN model with Hill’48 quadratic yield criterion to determine the FLC of anisotropic sheet metals. The commercial ABAQUS/Explicit finite element code and the VUMAT implementation of the anisotropic GTN damage model were used to calculate the limit strains of an AA6016-T4 sheet. The hardening behaviour of the metallic sheet is described by Swift’s law:
The Hill’48 coefficients i.e. F, G, H, L, M, and N were calculated as 0.648, 0.644, 0.356, 1.5, 1.5 and 1.174, respectively. The GTN model parameters were calibrated using the force-displacement curve obtained from uniaxial tensile tests. Table 5.1 shows the values of the calibrated GTN model parameters:
The Forming Limit Curve has been constructed according to the specifications of the international standard ISO 12004-2 (2008) and by performing the Nakazima tests. Different strain paths have been tested by using different geometries of the specimens according to Fig. 5.41. The values of the width parameter w are 30, 55, 70, 90, 120, 145, and 185 mm, the last of them corresponding to a fully circular specimen. The Forming Limit Curve obtained by numerical simulations has been validated by comparison with experimental results.
To calculate the limit strains, the numerical simulations have been continued up to fracture. Three groups of nodes distributed along paths normal to the fracture section have been selected and the values of the major and the minor strains at these nodes have been measured on the frame corresponding to the stage just before the onset of fracture. One of the node paths used to measure the principal strains is shown in Fig. 5.42. Having the fracture section as shown in Fig. 5.42a, b facilitates the selection of node paths for strain measurement. The first node path passes through the middle of the fracture section (see Fig. 5.42), while the other two paths are located on each side of the first path with an approximate distance of 2 mm, being also parallel to each other. The values of the strains associated to these node paths were analyzed with the ARAMIS software and according to Bragard’s method (Bragard et al. 1972; D’Haeyer and Bragard 1975) to determine the corresponding point on the Forming Limit Curve.
The Forming Limit Curve obtained using the ARAMIS software is presented in Fig. 5.43. This figure indicates that the results obtained by numerical simulation using the GTN damage model are in good agreement with the experimental data. The comparison becomes even more favourable when confronted with the predictions of the MK and MMFC models see Fig. 5.43. One may notice from the diagram that the quality of the GTN predictions is far better, especially along the right branch of the Forming Limit Curve, where both MK and MMFC models overestimate the formability of the metallic sheet.
The finite element simulation with the GTN damage model as the material constitutive model was also used for the construction of Forming Limit Stress Diagram (FLSDs) (Uthaisangsuk et al. 2008; He et al. 2011). For this purpose, the Nakazima tests were simulated with sheet metal samples having the same length of 190 mm and varying widths from 55 up to 195 mm (Uthaisangsuk et al. (2008)). The FLSD obtained in this way was used for the prediction of crack initiation due to a two-step forming and hole expansion tests. The same investigation was performed by the application of FLD obtained by the experimental tests. It was observed that the FLSD obtained by the GTN model was able to predict the failure with reasonable accuracy. On the other hand, the experimental FLD failed to predict the crack initiation (Uthaisangsuk et al. (2008)). A similar work was reported by He et al. (2011) in which the FLSD of an AA5052-O1 sheet was determined using the GTN damage model. The cited authors performed numerical simulations of the Nakazima test on different samples with 10 mm to 180 mm widths (see Fig. 5.44).
The numerical simulation was allowed to continue until the onset of necking where the damaged elements with zero stress appear in the necking region. Three elements with close stress values (the stress difference between every two elements is within 10 %) were selected in the necking region and the mean value of the principal maximum and middle stresses at these elements were defined as the limit stresses. The stress values were measured at the last loading increment without the appearance of cracked elements. The same procedure has been used for the determination of limit strains. The numerical FLD obtained by using this approach showed good agreement with experimental results (He et al. 2011).
The GTN damage model was also used to predict FLDs for different types of sheets, dual phase and multi-phase steels (Uthaisangsuk et al. 2009, 2011; Ramazani et al. 2012), Tailor-welded blanks (TWBs) (Abbasi et al. 2012b, 2012c) and sandwich sheets (Parsa et al. 2013; Liu et al. 2013). Ramazani et al. (2012) determined the FLC of the DP600 steel by the numerical simulation of the Nakazima test. Furthermore, the ability of the GTN model to predict the damage in forming was assessed by FE simulation of a cross-die test. The results showed that the GTN damage model predicts the fracture with reasonable accuracy (Ramazani et al. 2012).
Almost the same procedure in Abbasi et al. (2012a) was used for the FLC prediction of IF-steel TWBs (Abbasi et al. 2012b, 2012c). The results showed that the predicted FLD by the GTN model does not properly agree with the FLD relevant to fracture initiation, but it is in fairly good conformance with the FLD that separates the safe region from other regions.
The GTN damage model was employed to construct the FLC of Al3105/polypropylene/Al3105 (Parsa et al. (2013)) and AA5052/polyethylene/AA5052 sandwich sheets (Liu et al. 2013). The FLC of these sandwich sheets was determined by numerical simulations of the Nakajima test where the GTN model was used as the constitutive model of the metallic face sheets. The effect of layer’s thickness on the formability of these sandwich sheets was investigated.
To find the limit strains, Liu et al. (2013) analyzed the evolution of the equivalent plastic strain in the neck region. As Fig. 5.45 illustrates, the evolution of the equivalent plastic strain in the localized neck point “a” and its vicinity point “b” were plotted as a function of the dome height. By comparing the equivalent plastic strain curves of these two points, one may notice that the strain values at these two points show similar tendencies during most of the deformation process, and this portion of the deformation process is called stability period. With the proceeding of deformation process, the equivalent plastic strain value at point “a” begins to show a rapid increase, which indicates that the instability period starts. When the equivalent plastic strain increment at point “a” exceeds by 7 times that at point “b”, the localized necking is assumed to occur and the final major and minor strains of element “a” calculated by linear interpolation are considered as the limit strains for the construction of forming limit diagrams.
Equivalent plastic strain distribution of localized neck element “a” and its neighbour element “b” Liu et al. (2013)
Besides the above mentioned studies, the GTN damage model was used for the investigation of formability under double-sided pressure forming Liu and Meng (2012) and also in the study of geometry and grain size effects on the formability of sheet metals in micro/meso scale plastic deformation (Xu et al. 2015). Liu et al. (2012) studied the effects of double-sided pressure on the formability of an AA5052-O aluminium alloy sheet metal under tension-compression deformation state. The left branch of the FLD was determined by numerical simulation of Nakazima tests under different double-sided pressure conditions. The GTN model was able to predict the limit strains in the left branch of the FLD with acceptable accuracy.
Xu et al. (2015) investigated the geometry and grain size effects on the formability of sheet metals in micro/meso scale plastic deformation using experiments and numerical simulation. For this purpose, the forming limit experiments were conducted based on the miniaturized (Holmberg et al. 2004) (for the left branch of FLC) and Marciniak (for the right branch of FLC) tests to estimate the formability of sheet metals under different loading conditions. The dimensions of the specimens used in these tests are shown in Fig. 5.46. The traditional Marciniak test was performed with the punch diameter of 10 mm, die diameter of 14 mm and the hole in the driving sheet centre of 3 mm diameter.
The dimensions of the Holmberg and Marciniak specimens used for the construction of FLC Xu et al. (2015)
For the numerical construction of FLC at micro/meso scale, an extended coupled damage model was first developed based on GTN and the Thomason (1985) models via considering the geometry and grain size effects on void evolution. The extended model was then employed in the numerical simulations of both Holmberg and Marciniak tests. In these simulations, when the void volume fraction just reaches to final void volume fraction \( f_{F} \) the major and minor strains are considered as the limit strains. The forming limits determined in this way are shown in Fig. 5.47 for a copper sheet with the thickness of 0.4 mm and different grain sizes of 23.7, 58.9 and 132.2 μm and (Xu et al. 2015). As one may notice, the extended GTN is qualitatively able to predict the changes in limit strains with the change of grain size (the numerically predicted limit strains decrease with the increase of grain size as in the case of experiments). Nevertheless, the extended GTN model underestimates the limit strains under biaxial stretch condition. The reason for this inaccuracy may be related to the identification procedure of the extended GTN model parameters. It is revealed that Thomason model tends to overestimate the ductility at low triaxiality (Benzerga 2002), hence the parameters identified based on the uniaxial tensile tests could be less accurate when applied to the biaxial stretch condition with higher triaxiality. In addition, the coalescence behaviour affected by shear loading is not considered either. This might also contribute to the difference between the experimental and predicted FLCs (Xu et al. 2015).
Comparison of limit strains in a copper sheet with the thickness of 0.4 mm and grain sizes of 23.7, 58.9 and 132.2 μm (Xu et al. 2015)
Uthaisangsuk et al. (2009), (2011) used a 3D representative volume element (RVE) to characterize multiphase microstructures of DP600 and TRIP600 steels and their distribution on the micro-level. Figure 5.48 illustrates an example of the RVE for a TRIP steel its microstructure consisting of ferrite, bainite, austenite and martensite (Uthaisangsuk et al. 2009). The GTN damage model was used in the finite element simulations of RVE for calculating the failure moment. The FLC’s of DP600 and TRIP600 steels were constructed based on the results of these simulations.
Representative volume elements (RVE) for a TRIP microstructure consisting of ferrite, bainite, austenite and martensite (Uthaisangsuk et al. 2009)
The RVE was defined as a cube and the experimentally measured constituent phase fractions were taken into account. To describe the random distribution of phases, a statistical algorithm was implemented. The RVE’s were used to investigate the critical areas of sheet samples in the Nakazima stretching-test. Therefore, boundary conditions for the RVEs were obtained from the local deformation fields in the macroscopic simulations (simulation of Nakazima tests with real dimensions of sheet samples). The influences of the local stress state on the crack initiation in the microstructure were considered by varying the sample dimensions. The GTN model was only employed for the softer ferritic matrix in the RVE model, where void initiation and void growth were observed in the experiments.
The comparison between the results of the RVE model and the experimental FLCs of DP600 and TRIP600 steels is presented in Fig. 5.49. As this figure shows, the limit strains predicted by the RVE model with GTN damage model underestimated the FLD curves in the biaxial stress condition whereas in the uniaxial tension and plane strain range the failure predictions exhibited similar strain levels as the experimental results. The reader is recommended to refer to Uthaisangsuk et al. (2009) for more discussions.
Comparison of numerical calculated strains at failure with experimental FLC’s for DP600 and TRIP600 steels (Uthaisangsuk et al. 2009)
Figure 5.50 shows the macroscopically simulated Nakazima samples and their corresponding RVE’s for the microstructure of TRIP600 at the moment of failure for the specimen having the dimensions 20 and 190 mm. The white regions are austenite and bainite, for which the GTN damage criterion was not applied. The failed elements reaching the critical damage values were observed close to the upper side of the RVE model for all sample geometries. This area belongs to the surface of the sheet samples, where cracking emerged in the experiments (Uthaisangsuk et al. 2009).
FE results from macroscopic and RVE simulations for the 20 and 190 mm sheet samples made from steel TRIP600 (Uthaisangsuk et al. 2009)
5.4.6.3 FLC Prediction by Combined M-K and GTN Models
The M-K model (Marciniak and Kuczynski 1967) assumes that the strain localization results from a thickness imperfection schematically represented as a groove in Fig. 5.27 and (Marciniak and Kuczynski 1967; Hutchinson and Neale 1978a). According to this hypothesis, two regions of the sheet metal should be distinguished: A—non-defective zone; B—groove. At different stages of the straining process, the parameter \( f = s^{(B)} /s^{(A)} ,0 < f < 1, \) is used to describe the amplitude of the imperfection (s (A) and s (B) denote the current thickness of regions A and B, respectively—see Fig. 5.27. The mechanical interconnection A–B is defined by a set of relationships enforcing the continuity of the strain-rate along the groove and the equilibrium of the normal and tangential loads acting on the interface from both sides (Banabic et al. 2010a). In the case of the MK model, necking is a consequence of the fact that the thickness strain tends to accumulate faster in region B.
To overcome some drawbacks of the MK model like the unrealistic assumption of a thickness defect, some researchers proposed to include void concentration in the defective zone. One approach developed according to this idea is the combination of MK and Gurson damage models. In this way, both ductile fracture caused by the evolution of voids and sheet metal instability contributes in the prediction of necking and limit strains.
Needleman and Triantafyllidis (1978) used the MK model in combination with Gurson damage model (Gurson 1977) to study the effect of void growth on the formability of biaxially stretched sheets. The imperfection was described by an increased initial volume concentration of voids in the incipient neck. The effect of the strain-hardening exponent and difference in initial void concentration between the material inside and outside the neck on the forming limit strains was studied Needleman and Triantafyllidis (1978). Because voids also evolve by nucleation, better predictions of plastic instability will be achieved when the nucleation of voids is taken into account in the Gurson model. The study on the effect of void nucleation on the forming limit of biaxially stretched sheets confirmed that the void nucleation has a significant effect on the limit strains Chu and Needleman (1980). The combined Gurson and MK models were also employed to study the effect of material properties on the forming limits of voided sheet metals (Melander 1983; Ragab et al. 2002).
Zadpoor et al. (2009) compared four different approaches of phenomenological ductile fracture modelling, the MK model, a modified Gurson damage model and a combined Gurson and MK approach to understand which of them can successfully predict the forming limits of high strength aluminium alloys like AA2024-T3. In the combined Gurson and MK approach, an initial imperfection in the sheet metal was assumed and a population of initial voids that are subject to further nucleation, growth and coalescence as deformation takes place was considered in both uniform and imperfection zones. The schematic drawing of this model is presented in Fig. 5.51. Once the void volume fraction of the imperfection zone reached the failure void volume fraction, the sheet was assumed to have failed and the strain values in the uniform zone were recorded as forming limits.
Schematic drawing of the combined Gurson and MK models (Zadpoor et al. 2009)
The results showed that the combination of the porous metal plasticity with the MK model improves the quality of the predictions of the porous metal plasticity model in the high end of the stress triaxiality values. Furthermore, it was found that the combined GTN and MK models and the modified Gurson model are more computationally expensive in comparison with the MK and the phenomenological ductile fracture models. Based on these results it was concluded that the phenomenological ductile fracture models can predict the forming limits with better accuracy and lower computational costs in comparison with the combined GTN and MK models (Zadpoor et al. 2009).
To include material anisotropy, an approximate macroscopic yield criterion based on the Gurson damage model (Gurson 1977) was developed by Liao et al. (1997) under plane stress conditions:
where, R is the anisotropy parameter, defined as the ratio of the transverse plastic strain rate to the through-thickness plastic strain rate under in-plane uniaxial loading conditions. Later, this model was used to investigate the failure of sheet metals under forming operations (Huang et al. 2000). The MK method was employed to predict plastic localization by assuming a slightly higher void volume fraction inside randomly oriented imperfection bands in a material element of interest. The effects of the anisotropy parameter R, the material/geometric inhomogeneities, and the potential surface curvature on the plastic localization were investigated Huang et al. (2000).
The finite element model of combined Gurson and MK model is presented in Fig. 5.52 and Simha et al. (2007a, b) used this model to construct a limit curve in terms of the invariants of mean stress and equivalent stress which is called extended stress-based formability curve (XSFLC) using the GTN damage model. The authors used a material model consisting of a homogeneous zone and a zone that contains voids (material inhomogeneity) to simulate the necking under plane strain and uniaxial stress load paths.
Schematic material model. Symmetry and displacement boundary conditions are shown. When \( u_{3} = 0, \) the model is loaded along the plane strain path (Simha et al. 2007b)
The GTN material model was used to simulate the necking through finite element computations. Then, a strain-based FLC is constructed for this model and this FLC is transformed into a XSFLC. By changing the ratio of displacement in \( x_{3} \) direction to displacement in \( x_{1} \) direction, different in-plane loading paths are applied to the model. The XSFLC was constructed for different values of the work hardening exponent and initial void volume fraction. The applicability of this model was satisfactory for the case of straight tube hydroforming with no end feed.
The applicability of the combined Gurson and MK models in the prediction of FLC’s of metal sheets under superimposed double-sided pressure was also investigated Liu et al. (2012). It was assumed that the imperfection zone has a certain initial volume fraction of voids. The schematic model is shown in Fig. 5.53. To find the limit strains, it was assumed that the failure takes place when the plastic strain increment in the inhomogeneous zone is 10 times larger than that in the homogeneous zone. The major and minor strains in the homogeneous zone were considered as the limit strains for the FLC construction (Liu et al. 2012). Figure 5.54 shows the predicted FLC’s at different values of the initial void volume fraction of the inhomogeneous zone \( f_{a0} \). As one may notice, the limit strains decrease with the increase of \( f_{a0} \).
Schematic of the MK model combined with Gurson model (Liu et al. 2012)
Comparison of FLC’s for different values of the initial void volume fraction in the inhomogeneous zone (Liu et al. 2012)
The interested reader is suggested to refer to Chien et al. (2004) and Son and Kim (2003) for more examples of the construction of FLC using the combined GTN and MK models.
In addition to the above mentioned works, the GTN model was employed according to other approaches for FLC prediction. A brief description of these approaches will be presented here. A numerical necking criterion based on the stress state in the neck was proposed by Brunet et al. (1998) and Brunet and Morestin (2001) for the determination of limit strains. The necking criterion was based on the load-instability and plane strain localization assumptions (Hora’s model (Hora and Tong 1994)) in which the failure of the material is defined by Gurson–Tvergaard damage model with Hill’48 and Barlat and Lian (1989) yield criteria. Similar studies using a non-local version of Gologanu–Leblond–Devaux model (Gologanu et al. 1993, 1994, 1997) were made to define the limit strains of anisotropic sheet metals (Brunet et al. 2004, 2005). Wang et al. (2013) used the anisotropic GTN damage model with Hill’s quadratic yield criterion for the prediction of fracture in warm stamping of an AZ31 magnesium alloy sheets and also for the construction of FLC’s at different temperatures. By the numerical simulation of the warm stamping process and recording the major and minor strains at the predicted place of fracture the FLC’s at different temperatures were constructed. The effect of temperature on the void growth, coalescence and fracture behaviour of the Mg alloy sheets were analyzed using the anisotropic GTN model (Wang et al. 2013). Jeong and Pan (1995) employed a modified yield criterion based on the Gurson model and the Coulomb’s yield criterion in the finite element modelling of a voided cube. Using these constitutive relations, Forming Limit Diagrams of rubber-modified plastics were constructed by measuring the critical localization strains under plane-stress biaxial loading. Grange et al. (2000) extended the GTN model to take into account the plastic anisotropy and viscoplasticity. They used this extended model for the FLC construction for a zirconium alloy containing various amounts of hydrogen.
5.4.6.4 Theoretical Model for Forming Limit Diagram Predictions Without Initial Inhomogeneity
5.4.6.4.1 Limit Analysis Interpretation of the MK Model
We will now show that the M-K model for the onset of necking admits a simple interpretation in terms of limit analysis or limit loads. Figure 5.55a shows the typical configuration of the homogeneous regions A along with the thickness defective region B. As it typical for the first quadrant of the FLD, we consider only the case where the region B is perpendicular to the direction of the major strain \( Ox_{1} \). To simplify the discussion we also suppose that the coordinate system of Fig. 5.55a is aligned with the rolling and transverse directions of the sheet, and therefore also with the orthotropy axes of the yield locus. In the region A we impose a constant strain rate ratio:
where d is the strain rate and all variables carry a superscript showing the region they belong to. The last equation is implied by the condition that the strain rates \( d_{11} ,d_{22} \) are principal strain rates. Equations (5.124) and conditions of plastic normality and plane stress uniquely define the stress tensor \( {\sigma}^{A} \) in region A. The equilibrium and compatibility conditions for the two regions are given by:
where t is the actual thickness. These conditions also uniquely define the stress and strain rate for the region B.
In the usual approach to the numerical solution of the MK model, these differential equations are integrated using an implicit Euler solver. During deformation, the strain rate in the region B rotates toward the plane strain condition where \( d_{22}^{B} = 0. \) Because of Eqs. (5.125), and the normality condition for region B, this implies that \( d_{11}^{B} \to \infty \) when \( d_{22}^{B} \to \infty . \) In turn, this implies an infinitely fast decrease of the thickness in region B which is the MK definition for incipient necking. In practice, the solver is stopped as soon as \( d_{11}^{B} /d_{22}^{B} \) becomes larger than a predefined value, usually 10.
We now propose an alternative explanation for the incipient necking predicted by the MK model. In Fig. 5.55b we have plotted the intersection of the yield loci for both regions A and B with the hyper-plane \( {\sigma}_{12} = {\sigma}_{13} = {\sigma}_{23} = {\sigma}_{33} = 0. \) In order to impose the equilibrium condition given by the first of Eqs. (5.125), we have scaled the yield loci with the respective actual thicknesses. Starting with the known scaled stress \( {\sigma}_{11}^{A} t^{A} \) in the region A, we seek the intersection of the line \( {\sigma}_{11}^{B} t^{B} = {\sigma}_{11}^{A} t^{A} \) with the scaled yield locus of the region B. There are three possibilities, labelled with (p, q, r) in Fig. 5.55b: two points of intersection, one tangent point, and no intersection.
For case (p), the two points have normals with different signs of \( d_{22}^{B} \) and therefore the correct choice is governed by the third of Eq. (5.125) and the sign of \( d_{22}^{A} \). The second case (r) is precisely the onset of necking in the MK model. As it easily inferred from Fig. 5.55b, this case has two equivalent interpretations:
where \( \Phi^{B} \) is the convex yield function for the region B. The second equation above shows that at the onset of necking the region B has reached its limit load for the \( x_{1} \) direction. The third case (r) reinforces this limit-load interpretation: when there is no intersection, the equilibrium condition requires that the stresses in region A are inside the convex yield locus. This means that region A is rigid, with no plastic deformation, and this implies:
so that region B is necessarily in the plane strain condition. We observe that this last case is incompatible with an imposed strain rate ratio in region A as required by Eq. (5.125); in this case the simplest solution is to switch to a constant stress ratio (shown with a dotted line in Fig. 5.55b).
In conclusion, we have shown that the onset of necking in the MK model is characterized by the attainment of a limit load for the defective region and a transition from a state of plastic deformation in both regions to one of plastic deformation inside the defective region and a rigid one inside the defect-free region.
5.4.6.4.2 Coalescence Models for Ductile Porous Materials
Initially the voids in a ductile porous material grow due the incompressibility of the surrounding material. In their pioneering work, Koplik and Needleman (1988) have numerically analyzed an elementary cell in a material with periodic voids, submitted to conditions of constant stress ratio (with axisymmetric loading and predominant axial stress); after some deformation the plastic flow becomes localized in the ligaments between the voids thus leading to an accelerated growth and subsequent coalescence of voids. An analytical model for the same elementary cell has been proposed by Gologanu et al. (2001), based on a sandwich model with three layers—a highly porous one surrounded by two sound layers. There are two possible regimes—one with rigid outer layers and the other with plastic sound layers. The evolution of intervoid distances may trigger the rigid/plastic regime and therefore the onset of coalescence. Recently, Leblond and Mottet (2008) have extended this analysis to the case of a combined axisymmetric and shear loading, treating within the same model the coalescence of voids and the formation of shear bands along voided sheets.
Independently, Thomason (1985) has provided an analytical solution for the critical normal stress acting on a periodic planar array of rectangular voids where only the ligaments between voids are under plastic flow, the upper and lower blocks being rigid. He then used this particular solution to determine the onset of coalescence by the following limit analysis recipe: use a non-localized plastic flow solution (given by some homogenized model for porous solids) as long as the normal stress given by this theory is below the critical stress; otherwise switch to the rigid blocks/plastic ligaments model.
Another successful model has been proposed by Perrin (1992). Similarly to the above models he follows the evolution of the distribution of voids and once a highly porous layer is formed, he applies to it the localized band bifurcation analysis of Rudnicki and Rice (1975).
Figure 5.56 shows a typical coalescence model. The essential ingredients are the anisotropic distribution of voids due to the plastic deformation (a), the consideration of the horizontal sound layers A and highly porous layer B (b) and finally the limit load interpretation for the onset of coalescence, completely analogous to the one in Fig. 5.55b pertaining to the onset of necking in the M-K model: when stresses in the sound region attain the maximal stress supported by the porous layer at q, there is a change in plastic regime toward a rigid behaviour of the sound regions (stresses in the sound region are inside the yield locus \( \Phi^{A} \) at r) while the porous layer remains in a strain-state compatible with this rigid behaviour \( d_{22}^{B} = d_{33}^{B} = 0. \)
Typical coalescence model based on limit analysis: distribution of voids after some deformation (a), elementary cell showing sound and highly porous layers (b) and limit load interpretation of the onset of coalescence with stress states in the sound regions before coalescence p, at the onset of coalescence q and during coalescence r (c)
5.4.6.5 Necking Model Based on Limit Analysis for Porous Sheets
Based on the observed analogy between the onset of sheet necking as predicted by the MK model (Marciniak and Kuczynski 1967) and the onset of coalescence of voids in a porous bulk solid, we develop now a new necking model without an initial imperfection based on limit analysis (LA).
Let us consider a porous sheet with a matrix obeying a rigid-plastic law with von Mises yield criterion. An initial isotropic distribution of voids (Fig. 5.57a) will evolve into an anisotropic one after a deformation of the sheet (Fig. 5.57b).
LA necking model: Spherical voids with an initial homogeneous distribution (a), in plane virtual localization band after a plastic deformation with vertical major strain (b), through-thickness virtual localization band after a biaxial deformation that rendered the voids oblate (c), method for determining the increased porosity in a virtual localization band (d)
We model the voided sheet using the ellipsoidal Gurson model from Gologanu et al. (1997) without taking into account the distribution of voids. At each moment we test for localization inside virtual bands with various normals n (Fig. 5.57c). For this test we do take into account the anisotropic distribution of voids that leads to an increased porosity inside the band. We first need to determine the mean void interspacings \( 2d_{xy} \) and \( 2d_{z} \) in the plane of the band and in the perpendicular direction z, parallel to n; then we need to estimate the thickness of the band \( 2d_{b} \) or equivalently the ratio \( c = d_{b} /d_{z} \) and finally we need a model for the limit load the band can still sustain.
Let us denote F the deformation gradient at the actual time. By assuming that the void interspacings are governed by the evolution of some elementary area and length, Leblond and Mottet (2008) were able to determine an expression for the ratio \( r = d_{xy} /d_{z} \). It is easy to generalize their result to the case of a distribution of voids that has already been submitted to some deformation gradient \( F_{0} \) prior to the analyzed deformation process, again starting from an isotropic distribution:
where n is the normal to the band (parallel to direction \( z) \).
The choice of the band thickness for coalescence models has been widely discussed in the literature. For example Thomason’s model is based on the choice \( 2d_{b} \) equal to the void height in the direction n, in order to best model plastic flow localization in the ligaments between voids. Based on experimental observations on sheet rupture showing that necking in general precedes void coalescence, we follow here the proposal of Perrin (1992) and Gologanu et al. (2001) and choose the thickness \( d_{b} \) such that the resulting elementary cell surrounding the void is the best possible approximation for an ellipsoid confocal with the void. This choice is compatible with using an ellipsoidal Gurson model for determining the limit load of the band; this model needs the porosity \( f^{p} \) and the shape factor \( S^{p} \) inside the band.
A supplementary difficulty appears if the band is not parallel to the void axes or if the void is not axisymmetric in the plane of the band. In this case, we determine an equivalent axisymmetric void by the following recipe: we project the initial void onto the plane xy to obtain an ellipse, we replace this ellipse with a circle of radius \( a_{xy} \) of same area and we determine the height \( a_{z} \) of the new void by imposing equal volumes for the original and new voids. In the sequel we will need only the following special case: the initial void is aligned with the sheet axes and has semiaxes \( a_{1} ,a_{2} ,a_{3} \) and the normal to the band is given by \( n = (n_{1} ,0,n_{3} ). \)
We note that for a initial void that is also axisymmetric (either prolate or oblate), the last equation defines uniquely the shape factor \( S^{p} \) of the projected void as a function of the initial shape factor S.
The confocality condition and the porosity are given now explicitly by:
Using Eqs. (5.128), (5.129) and (5.130) we obtain the final result for the porosity \( f^{p} \) inside the band:
For a spherical void, this expression reduces to \( c = r \) as proposed by Leblond and Mottet (2008).
We still need to provide an expression for the limit load of the virtual localization band. Let \( {\sigma}^{p} \) be the stress on the inclined band in Fig. 5.57d due to stress equilibrium and strain compatibility with the uniform sheet:
We note that at variance with coalescence models we do not impose \( d_{xx}^{p} = d_{xy}^{p} = 0 \) but rather \( {\sigma}_{xx}^{p} = {\sigma}_{xy}^{p} = 0 \) resulting from plane stress conditions. Let \( \Phi^{p} ({\sigma} ,f^{p} ,S^{p} ) = 0 \) be the yield surface of the porous band, where we have omitted the dependence on other state parameters. Then the limit load problem for the band can be written as:
There is no analytical solution of this equation; we solve it numerically using a formulation described elsewhere.
There is a supplementary condition for incipient necking that has generally been neglected in coalescence studies. The attainment of the limit load in the band is not sufficient, as the subsequent deformation of this band and increased hardening inside the band may instantaneously deactivate the limit load condition. For a vertical through thickness band this new condition is simply:
where the derivatives with respect to time \( \tau \) must be taken for a porous band that remains compatible with rigid blocks outside the band. The last equation is similar to the one used by Hill for the second quadrant and identical to Eq. (7) in Stören and Rice (1975) for the bifurcation along a band perpendicular to the major strain axis, without any consideration of a vertex on the yield surface. This shows that the new model contains as a special case the bifurcation theory of Stören and Rice, applied not to the uniform sheet but to a virtual band with increased porosity.
We do not present here the supplementary condition for an inclined band as we have found that it is always preceded by the limit load condition for strain paths close to the biaxial one, exactly where we expect that inclined bands may be first to localize.
The final model we use is that for a non-inclined band incipient necking is attained when both conditions are true:
while for an inclined band only the first condition is used.
5.4.6.5.1 Numerical Results
We first consider the simplest possible model for a porous sheet with a matrix having a rigid-plastic behaviour with von Mises yield surface, Swift hardening with \( K = 417\;\text{MPa},\,\,\varepsilon_{0} = 0.01 \) and a hardening exponent 0.245 and an initial porosity 0.01. We also suppose that voids are initially spherical and remain so during sheet deformation. Figure 5.58 compares the prediction of the new LA necking model with the MK model. The LA necking model shows two different regions: the first region, close to the plane-strain conditions, is one where the bifurcation condition Eq. (5.134) is attained before the limit load condition Eq. (5.133), while for the second region the reverse is true. For this spherical Gurson model the limit load in an inclined band was attained always after it was attained in the non-inclined band. The MK model results shown on the same Figure correspond to the case of an initial damage imperfection without thickness imperfection—the region B is porous but region A is sound, while the other curve corresponds to the dual case where the porosity is the same in both regions but there is an initial thickness imperfection with thickness ratio 0.99. We now consider the same porous material as before but we let the shape of the voids evolve toward oblate ellipsoids. Figure 5.59 compares again the results of the new LA necking model and MK models. In this case one observes that there appears a third region around the biaxial strain condition, where some inclined band attains the limit load before the non-inclined band.
5.4.7 Other Models
5.4.7.1 Bifurcation Models
Bifurcation models seek the conditions under which localized plastic plane-strain deformation is possible in a narrow band without loss of stress equilibrium. The first bifurcation model, that of Hill (1952), was limited to the left-hand side of the Forming Limit Curve since for deformation modes in the right-hand side, there exists no plain-strain direction for materials with smooth yield loci and normal plastic flow. By adopting the deformation theory of plasticity and allowing for vertex-formation on the yield locus, the extension to the right-hand side of the FLD was made by Stören and Rice (1975).
More recently, this bifurcation model has nevertheless been successfully used with the flow theory of plasticity, more commonly accepted in sheet metal forming. Flow theories in which non-normal plastic flow is allowed, are used in Hashiguchi and Protasov (2004), Ito et al. (2000), while Haddag et al. (2007) added the damage model of Chaboche into flow theory.
5.4.7.2 Perturbation Models
Dudzinski and Molinari (1991) proposed the use of the linearized theory of stability in order to determine the limit strains. This approach which considers the sheet to have homogeneous properties, is the linear perturbation technique for sheet necking analysis (Boudeau 1995; Boudeau and Gelin 1996). In this approach, a perturbation to the strain state at equilibrium is introduced, from which the limit strain is found as the conditions under which the perturbation increases in time. Recently, Jouve (2015) revisited the Dudzinski and Molinari theory and developed a 3D linear stability analysis to study the onset of the development of necking plastic instabilities.
5.4.8 Semi-empirical Models
Despite all the recent enhancements of the computational models (see Sect. 5.4.4), they are not able to give very accurate predictions of the limit strains in all the cases encountered in practical applications (different materials, thickness, forming rates, temperatures, strain paths, etc.). Due to this fact, the commercial finite-element codes still make use of experimental FLD’s or FLD’s calculated with semi-empirical models. Some of the widely-used semi-empirical models will be presented next.
Keeler and Brazier (1975) proposed an empirical relationship for calculating the limit strains corresponding to plane strain, ε 10:
where t is the sheet thickness (t ≤ 3 mm).
Assuming that the shape of the FLD remains the same and having determined the value of ε10, it is possible to obtain the FLD by translating the Keeler-Goodwin curve along the vertical coordinate axis.
Cayssials (1998), (1999) developed the Keeler-Brazier model by including both the coefficient of strain-rate sensitivity m and the ‘internal damage’ parameters. The limit strain is the solution of the equation
where a, b and c are material constants. As a first approximation, ε 10 can be expressed as follows:
Cayssials and Lemoine (2005) have extended the formulation (5.137) by including the anisotropy coefficient thus obtaining:
where r is the anisotropy coefficient.
More, by coupling the former model with the Stören and Rice model (Stören and Rice 1975) has been possible the extension of the new model also for drawing and stretching areas. So, the new model is able to predict the FLC for the complete domain, both for linear and non-linear strain paths, using only the mechanical parameters (yield stress, strain hardening coefficient, strain rate sensitivity index and anisotropy coefficient) and thickness of the material. The results obtained are in very good agreement with the experimental data for new grades of steel alloys (UHSS, DP, TRIP etc.) (Cayssials and Lemoine 2005).
Held et al. (2009) proposed a new semi-empirical approach for FLC prediction, which is valid for all sheet metal materials used in car body production. This approach uses a correlation of mechanical properties of uniaxial tensile test an experimentally determined limit strains.
Abspoel et al. (2013) proposed a new method to predict accurately the FLCs for a wide range of steel grades and thicknesses. The method is based on the correlations founded between the characteristic points of the FLC and the mechanical parameters. Four characteristic points were considered: uniaxial tension necking point, plane strain point, intermediate biaxial stretch point and equi-biaxial stretch point.
5.5 Commercial Programs for FLC Prediction
Based on the above mentioned models have been developed more commercial programs for the limit strains prediction.
5.5.1 FORM-CERT Program
Based on a Marciniak-Kuckzynski model, Jurco and Banabic (2005a, b); Banabic (2006) have developed so-called FORM-CERT commercial code. The BBC 2005 yield criterion is implemented in this model. This yield criterion can be reduced to simpler formulations (Hill 1948, Hill 1979, Barlat 1989, etc.). In this way, the yield criterion can be also used in the situations when only 2, 4, 5, 6, or 7 mechanical constants are available. The program consists in four modules: a graphical interface for input, a module for the identification and visualization of the yield surfaces, of the strain hardening laws and a module for calculating and visualizing the forming limit curves. The numerical results can be compared with experimental data, using the import/export facilities included in the program. FORM-CERT can be used as a standalone application for calculating FLC’s and comparing them with experimental data, or as an auxiliary tool for the finite-element simulation of sheet metal forming processes. In its current structure, the program offers useful functionalities both for research and industrial laboratories. A short description of this program will be presented in the next sections.
The program FORM-CERT developed in the CERTETA research centre consists in the following modules:
-
Identification module associated to the yield criterion (responsible for evaluating the coefficients of the yield criterion, as well as for the graphical output of the yield locus and planar distribution of the yield stress and r-coefficient).
-
Module for calculating and displaying the strain hardening law.
-
Module for calculating and displaying the forming limit diagram.
Figure 5.60 shows a structural diagram of the program. This diagram presents the modules mentioned above, as well as their interaction. We shall describe next the functionality of each module.
5.5.1.1 Calculation and Displaying the FLC
This is the main module of the program. The MK model (see Sect. 5.4.4) of the strain localization process has been implemented in the FORM-CERT program.
The graphical user interface provided by this module is divided in two regions (Fig. 5.61). The first one receives the input data: coefficients of the yield criterion and strain hardening law calculated by the modules mentioned bellow, a parameter specifying the thickness non-homogeneity factor and also the value of the strain increment used for computing the FLC.
At present, the FLD module works only for linear strain paths. The second region of the graphical user interface is used for plotting the FLC predicted. Several curves can be superimposed on the same diagram and also compared with experimental data (imported from ASCII files via the ‘Experimental data’ panel).
5.5.1.2 “Experimental Data” Module
This module is structured as a panel for acquiring input data (Fig. 5.62). The user has the possibility to type this data or to import it from ASCII files. The data can be plotted on diagrams or exported to other modules of the FORM-CERT program. All the diagrams generated by the modules mentioned above can be processed and also exported in different graphical formats (Bitmap, Windows Metafile, GIF, JPEG, Postscript, PDF, etc.). In addition, the results of the computations can be exported in a numerical format (via ASCII, XML, Excel, and HTML files).
5.5.2 Other Programs
Hora and his co-workers have developed MATFORM code (http://www.fominnotech.ethz.com) based on the MMFC model (Hora and Tang 1994; Hora et al. 2003; Hora 2006). This code is able to calculate and plot the limit strains and also the visualization of the strain hardening curve and yield loci using Hill (1948), Hill 1979, Hill 1990 and Barlat (1989) criteria. The program is useful for evaluation of most common experiments like tensile, bulge, Miauchi, torsion dilatometer and tube hydroforming tests. The program is very well documented and is able to export the constitutive models in FEM specific form for the application in the mostly spread FEM-codes like Autoform or PamStamp.
Using the CRACH algorithm (based on the MK model), Gese and Dell (2006); Dell et al. (2007), (2008) have developed two software: CrachLAB, a product for prediction of the initial FLC and CrachFEM a product for coupling with the FEM codes. Criteria for ductile and shear fracture have been included in CrachFEM to cover the whole variety of fracture modes for sheet materials. The material model used to calculate instability describes: the initial anisotropy (using Hill 1948 criterion), the combined isotropic-kinematic hardening and the strain rate sensitivity. CrachFEM is now included in the FEM codes PamStamp and PamCrash of ESI Group.
5.6 Conclusions
In the past, the FLC models provided an approximate description of the experimental results. Such models were used especially for obtaining qualitative information concerning the necking/tearing phenomena.
At present, the FLC models allow a sufficiently accurate prediction of the limit strains, but each model suffers from its own limitations. There is no model that can be applied to any sort of sheet metal, any type of crystallographic structure, any strain-path or any variation range of the process parameters (strain rate, temperature, pressure, etc.).
The future research will be focused on a more profound analysis of the phenomena accompanying the necking and fracture of the sheet metals. On the basis of the analysis, more realistic models will be developed in order to obtain better predictions of the limit strains. New models will be developed for prediction of the limit strains for special sheet metal forming processes: superplastic forming, forming at very high pressure, incremental forming etc. Commercial codes allowing the quick and accurate calculation of the FLC’s both for linear and complex strain-paths will be developed. The texture models will be also implemented in such commercial programs. The FLC computation will be included in the finite element codes used for the simulation of the sheet metal forming processes. The aim is to develop automatic decision tools (based on artificial intelligence methods) useful in the technological design departments. The stochastic modelling of the FLC’s will be developed in order to increase the robustness of the sheet metal forming simulation programs. More refined, accurate and objective experimental methods for the experimental determination of the limit strains (e.g. methods based on thermal or acoustic effects) will be also developed.
Notes
- 1.
EMFC is an acronym for “Extended Maximum Force Criterion” Mattiasson et al. (2006).
- 2.
This requirement is always met when \( \bar{\upsigma } = \bar{\upsigma }\left( {\upsigma_{1} ,\upsigma_{2} } \right) \) is a strictly convex function.
References
Abbasi M, Ketabchi M, Izadkhah H, Fatmehsaria DH, Aghbash AN (2011) Identification of GTN model parameters by application of response surface methodology. Procedia Eng 10:415–420
Abbasi M, Shafaat MA, Ketabchi M, Haghshenas DF, Abbasi M (2012a) Application of the GTN model to predict the forming limit diagram of IF-Steel. J Mech Sci Technol 26:345–352
Abbasi M, Bagheri B, Ketabchi M, Haghshenas DF (2012b) Application of response surface methodology to drive GTN model parameters and determine the FLD of tailor welded blank. Comp Mater Sci 53:368–376
Abbasi M, Ketabchi M, Ramazani A, Abbasi M, Prahl U (2012c) Investigation into the effects of weld zone and geometric discontinuity on the formability reduction of tailor welded blanks. Comp Mater Sci 59:158–164
Abedrabbo N, Pourboghrat F, Carsley J (2006) Forming of aluminium alloys at elevated temperatures—Part 2: numerical modelling and experimental verification. Int J Plast 22:342–373
Abspoel M, Scholting ME, Droog JMM (2013) A new method for predicting forming limit curves from mechanical properties. J Mat Proc Technol 213:759–769
Achani D, Eriksson M, Hopperstad OS, Lademo OG (2007) Modelling of local necking and fracture in aluminium alloys. In: César de Sá JMA, Santos DA (eds) Proceedings of NUMIFORM 2007, Porto, Portugal
Allwood JM, Shouler DR (2009) Generalised forming limit diagrams showing increased forming limits with non-planar stress states. Int J Plast 25:1207–1230
Aretz H (2004) Numerical restrictions of the modified maximum force criterion for prediction of forming limits in sheet metal forming. Model Simul Mater Sci Eng 12:677–692
Arrieux R, Boivin M (1987) Determination of the forming limit stress curve for anisotropic sheets. CIRP Ann 36:195–198
Assempour A, Nejadkhaki HK, Hashemi R (2010) Forming limit diagrams with the existence of through-thickness normal stress. Comp Mat Sci 48:504–508
Ayres RA, Wenner ML (1978) Strain and strain–rate hardening effect on punch stretching of 5182-O aluminium at elevated temperature. Sheet Metal Indus 55:1208–1216
Azrin M, Backofen WA (1970) The deformation and failure of a biaxially stretched sheet. Metall Trans 1:2857–2861
Balanethiram VS, Daehn GS (1994) Hyperplasticity-increased forming limits at high workpiece velocities. Scripta Metall 31:515–520
Banabic D (2006) Numerical prediction of FLC using the M-K-model combined with advanced material models. In: Hora P (ed) Numerical and experimental methods in prediction of forming limits in sheet forming and tube hydroforming processes, pp 37–42. ETH Zürich
Banabic D (2010) A review on recent developments of Marciniak-Kuczynski model. Comp Meth Mat Sci 10:225–237
Banabic D, Dannenmann E (2001) The influence of the yield locus shape on the limits strains. J Mat Proc Technol 109:9–12
Banabic D, Dörr RI (1995) Modelling of the sheet metal forming. Transilvania Press, Cluj Napoca (in Romanian)
Banabic D, Soare S (2008) On the effect of the normal pressure upon the forming limit strains. In: Hora P (ed) Proceedings of the 7th international conference and workshop on numerical simulation of 3D sheet metal forming processes. interlaken, pp 199–204
Banabic D, Soare S (2009) Assessment of the modified maximum force criterion for aluminium metallic sheets. Key Eng Mat 410–411:511–520
Banabic D, Bunge HJ, Pöhlandt K, Tekkaya AE (2000) Forming limits of sheet metal. In: Banabic D (ed) Formability of metallic materials. Springer, Berlin, pp 173–214
Banabic D, Aretz H, Comsa DS, Paraianu L (2005a) An improved analytical description of orthotropy in metallic sheets. Int J Plast 21:493–512
Banabic D, Aretz H, Paraianu L, Jurco P (2005b) Application of various FLD modelling approaches. Modell Simul Mater Sci Eng 13:759–769
Banabic D et al (2010a) Sheet metal forming processes. Springer, Heidelberg
Banabic D, Barlat F, Cazacu O, Kuwabara T (2010b) Advances in anisotropy and formability. Int J Mater Form 3:165–189
Banabic D, Lazarescu L, Paraianu L, Ciobanu I, Nicodim I, Comsa DS (2013) Development of a new procedure for the experimental determination of the forming limit curves. Ann CIRP 62:255–258
Banabic D, Lăzărescu L, Comşa DS (2015) Predictive performances of the Marciniak-Kuczynski model and Modified Maximum Force Criterion, Key Eng Mat 651–653:96–101
Barlat F (1989) Forming limit diagrams—predictions based on some microstructural aspects of materials. In: Wagoner RH, Chan KS, Keeler SP (eds) Forming limit diagrams: concepts, methods, and applications. The Minerals, Metals and Materials Society, Warrendale, pp 275–302
Barlat F, Lian J (1989) Plastic behaviour and stretchability of sheet metals. Part I: yield function for orthotropic sheets under plane stress conditions. Int J Plast 5:51–66
Barlat F, Brem JC, Yoon JW, Chung K, Dick RE, Choi SH, Pourboghrat F, Chu E, Lege DJ (2003) Plane stress yield function for aluminium alloy sheets. Part 1. Theory Int J Plast 19:1297–1319
Barlat F, Gracio JJ, Lee MG, Rauch EF, Vincze G (2011) An alternative to kinematic hardening in classical plasticity. Int J Plast 27:1309–1327
Bassani JL, Hutchinson JW, Neale KW (1979) On the prediction of necking in anisotropic sheets. In: Lippmann H (ed) Metal forming plasticity (IUTAM symposium). Springer, Germany, pp 1–13
Benzerga AA (2002) Micromechanics of coalescence in ductile fracture. J Mech Phys Solids 50:1331–1362
Bird JE, Newman KE, Narasimhan K, Carlson JM (1987) Heterogeneous initiation and growth of sample-scale shear bands during necking of Al-Mg sheet. Acta Metall 35:2971–2982
Boudeau N (1995) Prediction of instability in local elasto-plastic instabilities (in French). Ph.D. thesis, University of Franche-Compte, Besancon
Boudeau N, Gelin JC (1996) Post-processing of finite element results and prediction of the localized necking in sheet metal forming. J Mat Proc Technol 60:325–330
Bragard A (1989) The contribution of CRM to the FLD concept. In: Wagoner RH, Chan KS, Keeler SP (eds) Forming limit diagrams: concepts, methods, and applications. The minerals, metals and materials society, Warrendale, pp 9–19
Bragard A, Baret JC, Bonnarens H (1972) A simplified method to determine the FLD onset of localized necking. Rap Cent Rech Metall 33:53–63
Bridgman PW (1952) Studies in large plastic flow and fracture—with special emphasis on the effects of hydrostatic pressure. McGraw-Hill, New York
Brozzo P, de Lucca B (1971) On the interpretation of the formability limits of metals sheets and their evaluation by means of elementary tests. In: Proceedings of ICSTIS, Tokyo, pp 966–988
Brunet M, Morestin F (2001) Experimental and analytical necking studies of anisotropic sheet metals. J Mat Proc Technol 112:214–226
Brunet M, Morestin F, Mguil S (1996) The prediction of necking and failure in 3D. Sheet forming analysis using damage variable. J Phys IV 06:C6–473–C6-482
Brunet M, Mguil S, Morestin F (1998) Analytical and experimental studies of necking in sheet metal forming processes. J Mat Proc Technol 80–81:40–46
Brunet M, Morestin F, Walter H (2004) Damage identification for anisotropic sheet metals using a non-local damage model. Int J Damage Mech 13:35–57
Brunet M, Morestin F, Walter-Leberre H (2005) Failure analysis of anisotropic sheet-metals using a non-local plastic damage model. J Mat Proc Technol 170:457–470
Carlson JM, Bird JE (1987) Development of sample-scale shear bands during necking of ferrite-austenite sheet. Acta Metall 35:1675–1701
Cayssials F (1998) A new method for predicting FLC. In: IDDRG congress meeting working group III, Brussel, pp 1–6
Cayssials F (1999) The version of the ‘Cayssials’ FLC model. IDDRG Meeting Working Group III, Birmingham, pp 1–7
Cayssials F, Lemoine X (2005) Predictive model for FLC (arcelor model) upgraded to UHSS steels. In: Boudeau N (ed) Proceedings of the IDDRG conference, Besancon, pp 17.1–17.8
Charpentier PL (1975) Influence of punch curvature on the stretching limits of sheet steel. Metall Trans A 6A:1665–1669
Chen Z, Dong X (2009) The GTN damage model based on Hill’48 anisotropic yield criterion and its application in sheet metal forming. Comp Mat Sci 44:1013–1021
Chiba R, Takeuchi H, Kuroda M, Hakoyama T, Kuwabara T (2013) Theoretical and experimental study of forming limit strain of half-hard AA1100 aluminium alloy sheet. Comp Mat Sci 77:61–71
Chien WY, Pan J, Tang SC (2004) A combined necking and shear localization analysis for aluminium sheets under bi-axial stretching conditions. Int J Plast 20:1953–1981
Chu CC (1980) An analysis of localized necking in punch stretching. Int J Solids Struct 16:913–931
Chu CC, Needleman A (1980) Void nucleation effects in biaxially stretched sheets. J Eng Mat Technol 102:249–256
Ciumadin AS, Ersov BI, Ziaja D (1990) Effect of hydrostatic pressure on the forming limit deformation of sheet metals. Kuznecino-stampovocinoe proizvodsvo 9:241-24
Considère M (1885) L’emploi du fer et l’acier dans les constructions. Ann des Ponts et Chausses 9:574–775
D’Haeyer R, Bragard A (1975) Determination of the limiting strains at the onset of necking. Rap Cent Rech Metall 42:33–35
Dell H, Gese H, Oberhofer G (2007) CrachFEM—A comprehensive approach for the prediction of sheet metal failure. In: César de Sá JMA, Santos AD (eds) Materials processing and design: modeling, simulation and applications NUMIFORM 2007, Porto, pp 165–170
Dell H, Gese H, Oberhofer G (2008) Advanced yield loci and anisotropic hardening in the material model MF GENYLD+CRACHFEM. In: Hora P (ed) Proceedings of the 7th international conference and workshop on numerical simulation of 3D sheet metal forming processes, part 2. Interlaken, pp 49–54
Demeri MY (1986) Strain analysis of the hemispherical stretch-bend test. J App Metalworking 4:183–187
Dieter GE (1988) Mechanical metallurgy. McGraw-Hill, New York
Dorn JE, Thomsen EG (1947) The ductility of metals under general conditions of stress and strain. Trans Am Soc Met 39:741–772
Drewes EJ, Martini A (1976) Einfluss der Umformgeschwindigkeit auf die Grenzformaenderungen und die Formaenderungsverteilung von Feinblech. Archiv fuer Eissenhuettenwessen 47:167–172
Dudzinski D, Molinari A (1991) Perturbation analysis of thermoviscoplastic insta-bilities in biaxial loading. Int J Solids Struct 5:601–628
Duncan JL, Bird JE (1978) Die forming approximations for aluminium sheet. Sheet Metal Indus 51:1015–1025
Emmens WC, van den Boogaard AH (2008) Tensile tests with bending: a mechanism for incremental forming. In: Proceedings of the 11th ESAFORM conference on material forming (ESAFORM 2008), Lyon, France
Eyckens P, Van Bael A, Van Houtte P (2009) Marciniak-Kuczynski type modelling of the effect of through-thickness shear on the forming limits of sheet metal. Int J Plast 25:2249–2268
Eyckens P, Van Bael A, Van Houtte P (2011) An extended Marciniak-Kuczynski model for anisotropic sheet subjected to monotonic strain paths with through-thickness shear. Int J Plast 27:1577–1597
Fukui Y, Nakanishi K (1988) Effects of sheet thickness on the in-plane stretch forming limit in an aluminium sheet. JSME Int J 31:679–685
Geiger M, Merklein M (2003) Determination of forming limit diagrams—a new analysis method for characterization of materials formability. CIRP Ann Manuf Technol 52:213–216
Gensamer M (1946) Strength and ductility. Trans ASM 36:30–60
Gerdooei M, Mollaei Dariani B (2009) Strain rate-dependent Forming Limit Diagrams for sheet metals. J Eng Manuf 222:1651–1659
Gese H, Dell H (2006) Numerical prediction of FLC with the program CRACH. In: Hora P (ed) Numerical and experimental methods in prediction of forming limits in sheet forming and tube hydroforming processes, ETH Zürich, pp 43–49
Ghosh AK, Hecker SS (1975) Stretching limits in sheet metals: in-plane versus out-of-plane deformations. Metall Trans 5A:2161–2164
Gologanu M, Leblond JB, Devaux J (1993) Approximate models for ductile metals containing non-spherical voids—Case of axisymmetric prolate ellipsoidal cavities. J Mech Phys Solids 41:1723–1754
Gologanu M, Leblond JB, Devaux J (1994) Approximate models for ductile metals containing nonspherical voids—case of axisymmetric oblate ellipsoidal cavities. J Eng Mat Technol 116:290–297
Gologanu M, Leblond JB, Perrin G, Devaux J (1997) Recent extensions of Gurson’s model for porous ductile metals. In: Suquet P (ed) Continuum Micromechanics. Springer, Vienna, pp 61–130
Gologanu M, Leblond JB, Perrin G, Devaux J (2001) Theoretical models for void coalescence in porous ductile solids—I: Coalescence “in layers”. Int J Sol Struct 38:5581–5594
Goodwin GM (1968) Application of strain analysis to sheet metal forming problems in the press shop. Soc Auto Eng 680093:380–387
Gotoh M, Chung T, Iwata N (1995) Effect of out-of-plane stress on the forming limit strains of sheet metals. JSME Int J 38:123–132
Grange M, Besson J, Andrieu E (2000) An anisotropic Gurson type model to represent the ductile rupture of hydrided Zircaloy-4 sheets. Int J Fract 105:273–293
Grosnostajski J, Dolny A (1980) Determination of FLC by means of Marciniak punch. Mem Sci Rev Met 4:570–576
Gurson AL (1977) Continuum theory of ductile rupture by void nucleation and growth Part I-yield criteria and flow rules for porous ductile media. J Eng Mater Technol 99:2–15
Haberfield AB, Boyles MW (1973) Laboratory determined the FLC of sheet steel. Sheet Metal Indus 50:400–411
Haddag B, Balan T, Abed-Meraim F (2007) Investigation of advanced strain-path dependent material models for sheet metal forming simulations. Int J Plast 23:951–979
Haddag B, Abed-Meraim F, Balan T (2008) Strain localization and damage prediction during sheet metal forming. In: ESAFORM 2008, Lyon, France
Haddag B, Abed-Meraim F, Balan T (2009) Strain localization analysis using a large deformation anisotropic elastic–plastic model coupled with damage. Int J Plast 25:1970–1996
Hasek V (1978) Untersuchung und theoretische Beschreibung wichtiger Einflussgrossen auf das Grenzformaenderungschaubild. Blech, 25:213–220, 285–292, 493–499, 619–627
Hashiguchi K, Protasov A (2004) Localized necking analysis by the subloading surface model with tangential-strain rate and anisotropy. Int J Plast 20:1909–1930
Havranek B (1977) The effect of mechanical properties on wrinkling in conical shells. J Mech Working Technol 1:115–129
He M, Li F, Wang Z (2011) Forming Limit Stress Diagram prediction of aluminium alloy 5052 based on GTN model parameters determined by in situ tensile test. Chin J Aeronaut 24:378–386
Hecker SS (1972) A simple FLC technique and results on some aluminium alloy. In: Proceedings of 7th IDDRG congress, Amsterdam, pp 51–71
Hecker SS, Ghosh AK, Gegel HL (eds) (1978) Formability: analysis modeling and experimentation. Met Soc AIME, New York
Held C, Dadrich J, Denninger R, Schleich R, Weidenmann KA, Liewald M (2009) Semi-empirische Berechnungsmethode zur Bestimmung des Grenzforma¨ nder-ungsdiagramms auf Basis mechanischer Kennwerte. Mat-wiss. u. Werkstofftech. 40:831–835
Hiam J, Lee A (1978) Factors influencing the FLC of sheet steel. Sheet Metal Indus 50:400–411
Hill R (1948) A theory of the yielding and plastic flow of anisotropic metals. Roy Soc Lond Proc A 193:281–297
Hill R (1952) On discontinuous plastic states with special reference to localized necking in thin sheets. J Mech Phys Solid 1:19–30
Hill R (1979) Theoretical plasticity of textured aggregates. Math. Proc. Cambridge Philosophical Soc. 85:179–191
Hill R (1993) A user-friendly theory of orthotropic plasticity in sheet metals. Int. J. Mech. Sci. 15:19–25
Holmberg S, Enquist B, Thilderkvist P (2004) Evaluation of sheet metal formability by tensile tests. J Mater Process Technol 145:72–83
Hora P (2006) Proceedings of FLC 2006 workshop. Numerical and experimental methods in prediction of forming limits in sheet forming and tube hydroforming processes. ETH Zürich, Zürich
Hora P (2014) Proceedings of FTF 2014 workshop. Time-dependent methods for the evaluation of FLC, ETH Zürich, Zürich
Hora P, Tong L (1994) Prediction methods for ductile sheet metal failure using FE-simulation. In: da Rocha B (ed) Proceedings of IDDRG congress, Porto, pp 363-375
Hora P, Tong L (2006) Numerical prediction of FLC using the enhanced modified maximum force criterion (eMMFC). In: Hora P (ed) Numerical and experimental methods in prediction of forming limits in sheet forming and tube hydroforming processes. ETH Zürich, Zürich, pp 31–36
Hora P, Tong L, Reissner J (2003) Mathematical prediction of FLC using macroscopic instability criteria combined with micro structural crack propagation models. In: Khan A (ed) Proceedings of plasticity 2003 conference, pp 364–366
Hora P, Tong L, Berisha B (2013) Modified maximum force criterion, a model for the theoretical prediction of forming limit curves. Int J Mat Form 6:267–279
Hotz W, Timm J (2008) Experimental determination of forming limit curves (FLC). In: Hora P (ed) Numisheet 2008 (Proceedings of 7th international conference workshop on numerical simulation of 3D sheet metal forming processes), Interlaken, Switzerland, 1–5 September 2008, pp 271–278
Hu XH, Jain M, Wilkinson DS, Mishra RK (2008) Micro-structure-based finite element analysis of strain localization behaviour in AA5754 aluminium sheet. Acta Mat 56:3187–3201
Huang HM, Pan J, Tang SC (2000) Failure prediction in anisotropic sheet metals under forming operation with consideration of rotating principal stretch directions. Int J Plast 16:611–633
Hutchinson RW, Neale KW (1978a) Sheet necking I. In: Koistinen DP, Wang NM (eds) Mechanics of sheet metal forming. Plenum Press, New York, pp 111–126
Hutchinson RW, Neale KW (1978b) Sheet necking II. In: Koistinen DP, Wang NM (eds) Mechanics of sheet metal forming. Plenum Press, New York, pp 127–153
Hutchinson RW, Neale KW (1978c) Sheet necking III. In: Koistinen DP, Wang NM (eds) Mechanics of sheet metal forming. Plenum Press, New York, pp 269–285
Inal K, Wu PD, Neale KW (2002a) Finite element analysis of localization in FCC polycrystalline sheets under plane stress tension. Int J Solids Struct 39:3469–3486
Inal K, Wu PD, Neale KW (2002b) Instability and localized deformation in polycrystalline solids under plane-strain tension. Int J Solids Struct 39:983–1002
Inal K, Neale KW, Aboutajeddine A (2005) Forming limit comparisons for FCC and BCC sheets. Int J Plast 21:1255–1266
International Standard ISO 12004-2 (2008) Metallic materials-sheet and strip determination of forming limit curves. Part 2: determination of forming limit curves in the laboratory. International organization for standardization, Geneva
Ito K, Satoh K, Goya M, Yoshida T (2000) Prediction of limit strain in sheet metal forming processes by 3D analysis of localized necking. Int J Mech Sci 42:2233–2248
Janssens K, Lambert F, Vanrostenberghe S, Vermeulen M (2001) Statistical evaluation of the uncertainty of experimentally characterised forming limits of sheet steel. J Mater Process Technol 112:174–184
Jeong HY, Pan J (1995) A macroscopic constitutive law for porous solids with pressure-sensitive matrices and its implications to plastic flow localization. Int J Solids Struct 32:3669–3691
Jouve D (2015) Analytic study of the onset of plastic necking instabilities during biaxial tension tests on metallic plates. Eur J Mech A Solids 50:59–69
Jurco P, Banabic D (2005) A user-frienldy program for calculating forming limit diagrams. In: Banabic D (ed) Proceedings of 8th ESAFORM conference material forming, Cluj Napoca, pp 423–427
Jurco P, Banabic D (2005) A user-friendly program for analyzing the anisotropy and formability of sheet metals. In: Boudeau N (ed) Proceedings of IDDRG 2005 conference, Besancon, pp 26.1–26.8
Kami A, Mollaei Dariani B, Sadough Vanini A, Comsa DS, Banabic D (2015) Numerical determination of the forming limit curves of anisotropic sheet metals using GTN damage model. J Mater Process Technol 216:472–483
Karthik V, Comstock RJ, Hershberger DL, Wagoner RH (2002) Variability of sheet formability and formability testing. J Mater Process Technol 121:350–362
Keeler SP (1961) Plastic instability and fracture in sheet stretched over rigid punches. Ph.D. thesis, Massachusetts Institute of Technology, Boston
Keeler SP (1970) La formabilité est améliorée par pression hydrostatique. Machine Moderne, 65:43–45
Keeler SP (1978) Forming limit criteria—sheets. In: Burke JJ, Weiss V (eds) Advances in deformation processing. Plenum Press, New York, pp 127–158
Keeler SP, Backofen WA (1963) Plastic instability and fracture in sheets stretched over rigid punches. Trans ASM 56:25–48
Keeler SP, Brazier WG (1975) Relationship between laboratory material characterization and press-shop formability. In: Microalloying 75 (Proceedings of international symposium high-strength, low-alloy steels), Washington, DC, pp 517–530
Kim JH, Lee MG, Kim D, Barlat F (2013) Numerical procedures for predicting localization in sheet metals using crystal plasticity. Comp Mat Sci 72:107–115
Kitting D, Ofenheimer A, Jain M, Pauli H, Rabler G (2008) Experimental characterisation of failure of stretch-bend steel sheets. In: Hora P (ed) Numisheet 2008 (Proceedings of 7th international confernece workshop on numerical simulation of 3D sheet metal forming processes), 1–5 September 2008, Interlaken, pp 315–320
Kleemola HJ, Kumpulainen JO (1980) Factors influencing the FLD. Influence of sheet thickness. J Mater Process Technol 3:303–311
Knockaert R, Chastel Y, Massoni E (2002) Forming limits prediction using rate-independent polycrystalline plasticity. Int J Plast 18:231–247
Koistinen DP, Wang NM (eds) (1978) Mechanics of sheet metal forming. Plenum Press, New York
Koplik J, Needleman A (1988) Void growth and coalescence in porous plastic solids. Int J Solids Struct 24:835–853
Kumpulainen JO, Ranta-Eskola AJ, Rintamaa RHO (1983) Effects of temperature on deep-drawing of sheet metals. Trans ASME J Eng Mat Technol 105:119–127
Lademo OG, Pedersen KO, Berstad T, Furu T, Hopperstad OS (2008) An experimental and numerical study on the formability of textured AlZnMg alloys. Eur J Mech A Solids 27:116–140
Lang L et al (2015) Investigation on the effect of through thickness normal stress on forming limit at elevated temperature by using modified M-K model. Int J Mat Form 8:2011–2228
Lange E (1975) Die Bedeutung von Kennwerten und Verfahren zur Beurteilung des Umformverhaltens beim Tiefziehen von Feinblechen (I). Baender Blech Rohre 5:511–514
Leblond JB, Mottet G (2008) A theoretical approach of strain localization within thin planar bands in porous ductile materials. Comptes-Rendus Mécanique 336:176–189
Leppin C, Li J, Daniel D (2008) application of a method to correct the effect of non-proportional strain paths on nakazima test based forming limit curves. In: Hora P (ed) Numisheet 2008 (Proceedings of 7th international confernece workshop on numerical simulation of 3D sheet metal forming processes), 1–5 September 2008, Interlaken, pp 217–221
Levy BS (2002) The enhanced FLC effect—project team research report. The Auto/Steel Partnership, Southfield, MI. (http://www.a-sp.org)
Li D, Ghosh AK (2004) Biaxial warm forming behaviour of aluminium sheet alloys. J Mater Process Technol 145:281–293
Liao KC, Pan J, Tang SC (1997) Approximate yield criteria for anisotropic porous ductile sheet metals. Mech Mater 26:213–226
Liu JG, Meng QY (2012) Left-side of the Forming Limit Diagram (FLD) under superimposed double-sided pressure. Adv Mat Res 472:653–656
Liu J, Wang Z, Meng Q (2012) Numerical investigations on the influence of superimposed double-sided pressure on the formability of biaxially stretched AA6111-T4 sheet metal. J Mater Eng Perform 21:429–443
Liu J, Liu W, Xue W (2013) Forming Limit Diagram prediction of AA5052/polyethylene/AA5052 sandwich sheets. Mater Des 46:112–120
Manopulo N, Hora P, Peters P, Gorji M, Barlat F (2015) An extended Modified Maximum Force Criterion for the prediction of localized necking under non-proportional loading. Int J Plast 75:189–203
Marciniak Z (1965) Stability of plastic shells under tension with kinematic boundary condition. Arch Mech Stosorwanej 17:577–592
Marciniak Z (1968) Analysis of necking preceding fracture of sheet metal under tension. La Metallurgia Ital 8:701–709
Marciniak Z (1977) Sheet metal forming limits. In: Koistinen DP, Wang NM (eds) Mechanics of sheet metal forming: material behaviour and deformation analysis. Plenum Press, New York, pp 215–235
Marciniak Z, Kuczynski K (1967) Limit strains in the processes of stretch-forming sheet metal. Int J Mech Sci 9:609–620
Marciniak Z, Kuczynski K, Pokora T (1973) Influence of the plastic properties of a material on the forming limit diagram for sheet metal in tension. Int J Mech Sci 15:789–800
Matin PH, Smith LM (2005) Practical limitations to the influence of through-thickness normal stress on sheet metal formability. Int J Plast 21:671–690
Mattiasson K, Sigvant M, Larsson M (2006) Methods for forming limit prediction in ductile metal sheets. In: Santos AD, Barata da Rocha ADC (eds) Proceedings of IDDRG 2006 conference, Porto, pp 1–9
Melander A (1983) A new model of the forming limit diagram applied to experiments on four copper-base alloys. Mater Sci Eng 58:63–88
Nakazima K, Kikuma T (1967) Forming limits under biaxial stretching of sheet metals (in Japanese). Testu-to Hagane 53:455–458
Needleman A, Triantafyllidis N (1978) Void growth and local necking in biaxially stretched sheets. J Eng Mater Technol 100:164–169
Neil JC, Agnew SR (2009) Crystal plasticity-based forming limit prediction for non-cubic metals: application to Mg alloy AZ31B. Int J Plast 25:379–398
Nurcheshmeh M, Green DE (2014) The effect of normal stress on the formability of sheet metals under non-proportional loading. Int J Mech Sci 82:131–139
Padwal SB, Chaturvedi RC, Rao US (1992) Influence of superimposed hydrostatic tension on void growth in the neck of a metal sheet in biaxial stress fields (I, II). J Mater Proc Technol 32:91–107
Paraianu L, Dragos G, Bichis I, Comsa DS, Banabic D (2009) An improved version of the modified maximum force criterion (MMFC) used for predicting the localized necking in sheet metals. Proc Rom Acad 10:237–243
Paraianu L, Dragos G, Bichis I, Comsa DS, Banabic D (2010) A new formulation of the modified maximum force criterion (MMFC). Int J Mat Form 3:243–246
Parsa MH, Ettehad M, Matin PH (2013) Forming limit diagram determination of Al 3105 sheets and Al 3105/Polypropylene/Al 3105 sandwich sheets using numerical calculations and experimental investigations. J Eng Mater Technol 135:031003
Percy JH (1980) The effect of strain rate on the FLD for sheet metal. Ann CIRP 29:151–152
Perrin G (1992) Contribution a l’étude théorique et numérique de la rupture ductile des métaux, Ph.D. thesis, Ecole Polytechnique, Palaiseau, France
Ragab AR, Saleh C, Zaafarani NN (2002) Forming limit diagrams for kinematically hardened voided sheet metals. J Mater Process Technol 128:302–312
Ramazani A, Abbasi M, Prahl U, Bleck W (2012) Failure analysis of DP600 steel during the cross-die test. Comp Mater Sci 64:101–105
Ranta-Eskola AJ (1979) Use of the hydraulic bulge test in biaxial tensile testing. Int J Mech Sci 21:457–465
Ratchev P, Van Houtte P, Verlinden B, De Smet P, Neutjens P, Baartman R, Drent P (1994) Prediction of Forming Limit Diagrams of Al-Mg rolled sheets taking texture into account. Text Microstruct 22:219–231
Romano G, Rault D, Entringer M (1976) Utilization des courbes limites de formage et du mode de repartition des deformations comme critere de jugement de l’amptitude d’un acier extradoux a l’emboutissage. Mem Sci Rev Metal 73:372–383
Rudnicki JW, Rice JR (1975) Conditions for the localization of deformation in pressure-sensitive dilatant materials. J Mech Phys Solids 23:371–394
Saanouni K (2008) On the numerical prediction of the ductile fracture in metal forming. Eng Fract Mech 75:3545–3559
Sang H, Nishikawa Y (1983) A plane strain tensile apparatus. J Met 35:30–33
Shi MF, Gerdeen JC (1991) Effect of strain gradient and curvature on Forming Limit Diagrams for anisotropic sheets. J Mat Shaping Technol 9:253–268
Signorelli JW, Bertinetti MA, Turner PA (2009) Predictions of forming limit diagrams using a rate-dependent polycrystal self-consistent plasticity model. Int J Plast 25:1–25
Simha CHM, Gholipour J, Bardelcik A, Worswick MJ (2007a) Prediction of necking in tubular hydroforming using an extended stress-based FLC. ASME J Eng. Mater Technol 129:136–147
Simha CHM, Grantab R, Worswick MJ (2007b) Computational analysis of stress-based forming limit curves. Int J Solids Struct 44:8663–8684
Smith LM et al (2003) Influence of transverse normal stress on sheet metal formability. Int J Plast 19:1567–1583
Soare S, Barlat F (2014) About the influence of hydrostatic pressure on the yielding and flow of metallic polycrystals. J Mech Phys Solids 67:87–99
Son HS, Kim YS (2003) Prediction of forming limits for anisotropic sheets containing prolate ellipsoidal voids. Int J Mech Sci 45:1625–1643
Spitzig WA, Richmond O (1984) The effect of pressure on the flow stress of metals. Acta Metall 32:457–463
Steninger J, Melander A (1982) The relation between bendability, tensile properties and particle structure of low carbon steel. Scand J Metall 11:55–71
Storen S, Rice JR (1975) Localized necking in thin sheets. J Mech Phys Solids 23:421–441
Swift HW (1952) Plastic instability under plane stress. J Mech Phys Solids 1:1–18
Tadano Y, Yoshida K, Kuroda M (2013) Plastic flow localization analysis of heterogeneous materials using homogenization-based finite element method. Int J Mech Sci 72:63–74
Tharrett MR, Stoughton TB (2003) Stretch-bend forming limits of 1008 Ak steel. SAE SP (2003-01-1157), pp 123–129
Tharrett MR, Stoughton TB (2003b) Stretch-bend forming limits of 1008 Ak steel, 70/30 Brass, and 6010 Aluminium. In: Khan AS, Kazmi R, Zhou J (eds) Dislocations, plasticity and metal forming (The tenth international symposium plasticity current applications), Quebec, Canada, 7–11 July 2003. Neat Press, USA, pp 199–201
Thomason PF (1985) A three-dimensional model for ductile fracture by the growth and coalescence of microvoids. Acta Metall 33:1087–1095
Timothy SP (1989) A modified technique to measure formability in plane-strain tension. In: Wagoner RH, Chan KS, Keeler SP (eds) Forming limit diagrams: concepts, methods, and applications. The Minerals, Metals and Materials Society, Warrendale, pp 21–36
Tisza M, Kovács ZP (2012) New methods for predicting the formability of sheet metals. Prod Process Syst 5:45–54
Tóth LS, Hirsch J, Van Houtte P (1996) On the role of texture development in the forming limits of sheet metals. Int J Mech Sci 38:1117–1126
Tvergaard V (1981) Influence of voids on shear band instabilities under plane strain conditions. Int J Fract 17:389–407
Tvergaard V (1982) On localization in ductile materials containing spherical voids. Int J Fract 18:237–252
Tvergaard V, Needleman A (1984) Analysis of the cup-cone fracture in a round tensile bar. Acta Metall 32:157–169
Uko DK, Sowerby R, Duncan JL (1977) Strain distribution in the bending-under-tension test. CIM Bull 70:127–134
Uthaisangsuk V, Prahl U, Münstermann S, Bleck W (2008) Experimental and numerical failure criterion for formability prediction in sheet metal forming. Comp Mat Sci 43:43–50
Uthaisangsuk V, Prahl U, Bleck W (2009) Characterisation of formability behaviour of multiphase steels by micromechanical modelling. Int J Fract 157:55–69
Uthaisangsuk V, Prahl U, Bleck W (2011) Modelling of damage and failure in multiphase high strength DP and TRIP steels. Eng Fract Mech 78:469–486
van den Boogaard AH (2002) Thermally enhanced forming of aluminium sheet. Modeling and experiments. Ph.D. thesis, University of Twente
Vegter H, ten Horn CHLJ, Abspoel M (2008) Modeling of the forming limit curve by MK analysis and FE simulations. In: Hora P (ed) Numisheet 2008 (Proceedings of 7th international conference workshop on numerical simulation of 3D sheet metal forming processes), 1–5 September 2008, Interlaken, pp 187–192
Volk W, Illig R, Kupfer H, Wahlen A, Hora P, Kessler L, Hotz W (2008) Benchmark 1—virtual forming limit curves. In: Hora P (ed) Numisheet 2008 (Proceedings of 7th international conference workshop on numerical simulation of 3D sheet metal forming processes), 1–5 September 2008, Interlaken, pp 3–9
Wagoner RH, Chan KS, Keeler SP (eds) (1989) Forming limit diagrams: concepts, methods and applications. TMS, Warrendale
Wang H, Wu PD, Boyle KP, Neale KW (2011) On crystal plasticity formability analysis for magnesium alloy sheets. Int J Solids Struct 48:1000–1010
Wang R, Chen Z, Li Y, Dong C (2013) Failure analysis of AZ31 magnesium alloy sheets based on the extended GTN damage model. Int J Min Met Mater 20:1198–1207
Wang H, Wu PD, Lee SY, Wang J, Neale KW (2015) Numerical study of the effects of shear deformation and super-imposed hydrostatic pressure on the formability of AZ31B sheet at room temperature. Int J Mech Sci 92:70–79
Wu PD, MacEwen SR, Lloyd DJ, Neale KW (2004a) Effect of cube texture on sheet metal formability. Mat Sci Eng A 364:182–187
Wu PD, MacEwen SR, Lloyd DJ, Neale KW (2004b) A mesoscopic approach for predicting sheet metal formability. Modell Simul Mater Sci Eng 12:511
Wu PD, Embury JD, Lloyd DJ, Huang Y, Neale KW (2009) Effects of superimposed hydrostatic pressure on sheet metal formability. Int J Plast 25:1711–1725
Xu ZT, Peng LF, Fu MW, Lai XM (2015) Size effect affected formability of sheet metals in micro/meso scale plastic deformation: experiment and modeling. Int J Plast 68:34–54
Xue L (2010) Localization conditions and diffused necking for damage plastic solids. Eng Fract Mech 77:1275–1297
Yoon JS, Noh HG, Song WJ, Kang BS, Kim J (2013) Comparison of experimental and numerical failure criterion for formability prediction of automotive part. Adv Mater Res 658:354–360
Yoshida K (2014) Effects of grain-scale heterogeneity on surface roughness and sheet metal necking. Int J Mech Sci 83:48–56
Yoshida K, Kuroda M (2012) Numerical investigation on a key factor in superior stretchability of face-centered cubic polycrystalline sheets. Int J Mech Sci 58:47–56
Yoshida K, Ishizaka T, Kuroda M, Ikawa S (2007) The effects of texture on formability of aluminium alloy sheets. Acta Mater 55:4499–4506
Yoshida K, Tadano Y, Kuroda M (2009) Improvement in formability of aluminium alloy sheet by enhancing geometrical hardening. Comp Mat Scis 46:459–468
Zadpoor AA, Sinke J, Benedictus R (2009) Formability prediction of high strength aluminium sheets. Int J Plast 25:2269–2297
Zhang F, Chen J, Chen J, Lu J, Liu G, Yuan S (2012) Overview on constitutive modeling for hydroforming with the existence of through-thickness normal stress. J Mater Process Technol 212:2228–2237
Zhang F, Chen J, Chen J, Zhu X (2014a) Forming limit model evaluation for anisotropic sheet metals under through-thickness normal stress. Int J Mech Sci 89:40–46
Zhang F, Chen J, Chen J (2014b) Effect of through-thickness normal stress on forming limits under Yld 2003 yield criterion and M-K model. Int J Mech Sci 89:92–100
Zhou Y, Neale KW (1995) Predictions of forming limit diagrams using a rate-sensitive crystal plasticity. Int J Mech Sci 37:1–20
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2016 Springer International Publishing Switzerland
About this chapter
Cite this chapter
Banabic, D., Comsa, DS., Eyckens, P., Kami, A., Gologanu, M. (2016). Advanced Models for the Prediction of Forming Limit Curves. In: Banabic, D. (eds) Multiscale Modelling in Sheet Metal Forming. ESAFORM Bookseries on Material Forming. Springer, Cham. https://doi.org/10.1007/978-3-319-44070-5_5
Download citation
DOI: https://doi.org/10.1007/978-3-319-44070-5_5
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-44068-2
Online ISBN: 978-3-319-44070-5
eBook Packages: Chemistry and Materials ScienceChemistry and Material Science (R0)