1 Introduction

Wet agglomerates are a common type of granular materials either naturally formed by the action of capillary bridges between primary particles [1, 2], or as industrial products in the agglomeration processes in steel-making [3,4,5,6], pharmaceutical, and food industries [7, 8]. The physical and mechanical properties of such agglomerates depend on the discrete nature of material including primary particles and the binding liquid [9,10,11,12,13,14,15,16,17] as well as its collisional type [17,18,19]. Under the action of external forces due to contacting with surrounding particles [3, 16, 20,21,22,23], impacting with the drum walls [24], or colliding with the containing walls during storage [25], the mechanical strength of agglomerates changes and these agglomerates could also be deformed or disintegrated or rebounded depending on both of impact conditions and the interactions between primary particles inside agglomerates [18, 26,27,28,29,30,31,32]. These interactions become more complex when both cohesive and viscous effects of the binding liquid come into play in addition to the elastic and frictional contacts [17, 33]. Therefore, the prediction of the physical and mechanical behavior of cohesive granular materials such as wet particle agglomerates based on such complex interactions and impact loads plays an important role.

We study the case in which a single wet agglomerate impacts on a flat plane from its original height by considering a homogeneous distribution of the binding liquid inside the granule. Upon impact, the particles start moving as a result of occurring the relative displacements between particles and the external pressure such as particle gravity [34]. These collective movements generate the inertial stress \(\sigma _{\mathrm {i}} \sim \rho \langle d \rangle ^2 \dot{\gamma }^2\) , where \(\rho \) is the particle density, \(\langle d \rangle \) is the mean particle diameter, and \(\dot{\gamma }\) denotes the impact rate (or shear rate in granular flow) [35, 36]. Due to the presence of the capillary bonds between particles in contact and the domination of the cohesive stress \(\sigma _{\mathrm {c}} \sim \gamma _s/\langle d \rangle \) and viscous stress \(\sigma _{\mathrm {v}} \sim \eta \dot{\gamma }\) as compared to the particle gravity, the physical and mechanical properties of wet agglomerates are expected dependently on the actions of \(\sigma _{\mathrm {i}}\), \(\sigma _{\mathrm {c}}\), and \(\sigma _{\mathrm {v}}\) or their dimensionless parameters: the Capillary number \(\text{ Ca }\) and the Stokes number \(\text{ St }\), where \(\gamma _{\mathrm {s}}\) is the liquid–vapor surface tension, \(\eta \) is the liquid viscosity. The Capillary number \(\text{ Ca }\) is the ratio of the viscous stress \(\sigma _v\) to the cohesive stress \(\sigma _{\mathrm {c}}\) (\(\text{ Ca } = \sigma _{\mathrm {v}}/\sigma _{\mathrm {c}}\)). The Stokes number \(\text{ St }\) is the ratio of the \(\sigma _{\mathrm {i}}\) and \(\sigma _{\mathrm {v}}\) (\(\text{ St } = \sigma _{\mathrm {i}}/\sigma _{\mathrm {v}}\)).

Studying the physical and mechanical responses of a single agglomerate impacting a plane is represented via the evolution of the granule strength, breakage, deformation at its early-stage impact and final-stage deposition as well as during the impact. The wet agglomerate could still survive or disintegrate (or fully spread) at its final deposition stage depending on the nature properties of the binding liquid characterized by the cohesive and viscous stresses, the raw materials, and the impact condition [16, 37]. The mechanical strength of agglomerate increases proportionally to the cohesive stress and the connectivity of agglomerates that expressed as an analytical model [14]. Such agglomerate also deforms as a consequence of irreversibly breaking of capillary bridges between near-neighboring particles [14, 17, 38, 39]. All of these behaviors are expected to be controlled by the combinations of the cohesion and viscosity of the liquid bridges and the impact speed of wet agglomerate.

In fact, the combination of the cohesive and viscous effects of the binding liquid and the flow rate of wet granular materials have been introduced for different configurations in recent years. In pressure-controlled condition and free surface flows of cohesive granular materials, the cohesive stress strongly affects the rheology of such flows, and a combination of the inertial number I (defined as a square root of the ratio of \(\sigma _{\mathrm {i}}\) and the confining stress \(\sigma _{\mathrm {n}}\)) and the cohesion index \(\xi \), defined as a ratio of \(\sigma _{\mathrm {c}}\) and \(\sigma _{\mathrm {n}}\), can be used to scale the rheology of cohesive granular flows and the evolution of wet agglomerate characterized by its elongation, erosion, and breakage [17]. Similarly, when the lubrication effects come into play with the inertial effects of granular materials, a combination of I and the Stokes number \(\text{ St }\) can be used to scale the effective viscosities of the dense suspension [40] or the visco-inertial granular flows [41, 42]. Remarkably, Vo and coworkers [33] found the additive mechanism of cohesive force and viscous force in addition to the inertial force in each particle interaction. The rheological properties of complex granular flows characterized by the effective friction coefficient and packing fraction as well as the texture variables nicely collapse as a function of a modified inertial number which combines I, \(\xi \), and \(\text{ St }\). However, the model of wet particle agglomerates impacting a flat plane in this current work is a very different case as compared to the configuration of unsaturated granular flows, and whether the physical and mechanical responses of impact agglomerate can be described by a dimensionless parameter as above examples?

In this paper, the behavior of a single wet agglomerate impacting a rigid plane is studied by means of 3D discrete element method (DEM) simulations. The simulations were performed by using an approximate analytical expression of the capillary attraction forces and lubrication forces between near-neighboring particles having the gap no exceed a rupture distance in addition to the elastic and frictional forces at their contacts. We investigate the evolution of the mechanical strength at the early-stage plastic threshold and the deformation and connectivity at the final-stage deposition of the wet agglomerate by systematically varying a broad range of values of the liquid–vapor surface tension, the liquid viscosity, and the impact velocity. As we shall see, a nontrivial combination of the control parameters as a new dimensionless inertial number can scale the mechanical and physical properties of impact agglomerate.

In the following, we first give the physical assumptions and the numerical approach and generate the impact test in Sect. 2. Then, in Sect. 3, we analyze the mechanical strength, the deformation, the connectivity, and the final-stage deposition height of granule as a function of the impact speed. In Sect. 4, we introduce the power-law scaling behavior of impact agglomerate. Finally, we conclude in Sect. 5 with an impressive summary of noteworthy results and its possible extensions.

2 Numerical method and model preparation

Our numerical simulations are performed by means of the discrete element method (DEM) with an approximate analytical expression of the cohesive forces and the viscous forces as a function of separation distance between particles in addition to the elastic and frictional forces at their contacts. We first describe below the physical assumptions of the distribution and transport of the binding liquid underlying the impact model. Then, we introduce briefly the numerical algorithm and the model of the impact test of an agglomerate.

2.1 Physical assumptions

The DEM has been extensively used for the simulations of granular materials due to the advantage of providing access the particle interactions and easily varying broad range of values of the system parameters. The equations of motion of all particles are integrated according to the explicit step-wise scheme by taking into account the particle interactions [43,44,45]. In advanced DEM, it is possible to implement the liquid–solid interactions in addition to the solid–solid contacts [34, 46]. This approach requires the substantial particle computation of the liquid phase as well as the model for the transition of gas–liquid phase [41, 47, 48]. However, such model also requires much more computational cost and memory in order to discretize the liquid phase. For this reason, a balance between computational efficiency and physical realism is considered in advanced DEM simulations. In the “pendular” state of the binding liquid, it is found to represent the liquid phase by an approximate analytical expression of the capillary attraction forces and lubrication forces between particles having no exceed a debonding distance as well as considering a particle-scale model for the distribution and transport of the liquid [16, 17, 49].

The presence of the binding liquid inside agglomerates as the liquid clusters is naturally formed due to the condensation from a liquid vapor or mixing the liquid with the particles. For low amounts of the liquid in the pendular regime, the liquid is in the form of the binary bridges. As the liquid volume increases, the liquid cluster has more and more particles. In the thermodynamic equilibrium of the liquid, the liquid is homogeneously distributed and the cohesion forces of the binding liquid are controlled by the wetted surface of particles and the Laplace–Young pressure. In non-equilibrium state, the liquid is not uniformly distributed in granular packing. However, in our simulations, we do not consider the wetting and dispersion processes of the binding liquid, which represented in the nucleation step of agglomerates. This means the liquid is assumed to be initially homogeneous distributed inside wet agglomerates.

In practice, a part of an amount of the liquid is absorbed into the rough surface of primary particles, and this liquid volume is not involved in capillary bridges between particles. As a wet agglomerate falls down and impacts on a rigid plane at a high speed, the capillary bond between particles may be broken when the separation distance between particles exceeds their rupture distance, the liquid is then immediately shared proportional to the particle size due to the lubrication effects of the liquid, and this liquid volume is assumed to be mainly covered the particle asperities without re-forming [46, 50]. This simplification allows us to investigate the effects of liquid properties and impact velocity on the physical and mechanical responses of wet particle agglomerates. It is also worth introducing here that these above assumptions have been used for the simulations of the coalescence of granules during collision [9], the agglomeration of wet particles [16], the impact breakage of agglomerates [10, 11, 13, 51], and diametrical compression test of spherical agglomerates [12, 14]; these results were almost in agreement with experimental works.

2.2 Discrete element method

The discrete element method (DEM) has been used for simulations of the granular materials in many applications in different scientific fields including soil mechanics, soft matter physics, powder technology, and geological processes over the last forty years [43, 52,53,54,55]. In the DEM, each particle is modeled as a rigid grain and interacts with its near-neighboring particles via the normal and tangential forces [43, 55]. In order to calculate the interactions between particles, a time discretized form and a large repulsive stiffness of particles of this discrete approach are required [43]. The contact forces between particles are proportional to their normal and tangential relative displacements, and these particle movements are obtained by considering the step-wise integration based on the Newton second law. The equations of translational and rotational motion of a particle i with its radius \(R_i\) are obtained by integrating all forces including contact forces and liquid forces exert on particle i [43, 54].

$$\begin{aligned} m_{\mathrm {i}} \frac{\text{ d}^2 \mathbf {r}_{\mathrm {i}}}{\text{ d }t^2}= & {} \sum _{\mathrm {j}} [(f_{\mathrm {n}}^{ij} + f_{\mathrm {c}}^{ij} + f_{\mathrm {v}}^{ij})\mathbf {n}^{ij} + f_{\mathrm {t}}^{ij} \mathbf {t}^{ij}] + m_{\mathrm {i}} \mathbf {g} \nonumber , \\ \mathbf {I}_{\mathrm {i}} \frac{\text{ d }{\varvec{\omega }}_{\mathrm {i}}}{\text{ d }t}= & {} \sum _{\mathrm {j}} f_{\mathrm {t}}^{ij} \mathbf {c}^{ij} \times \mathbf {t}^{ij}, \end{aligned}$$
(1)

where i and j are two particles in contact and \(m_{\mathrm {i}}\) and \({\varvec{ r}}_{\mathrm {i}}\) are the mass and position of particle i, respectively. \(\mathbf {I}_{\mathrm {i}}\) and \({\varvec{\omega }} _{\mathrm {i}}\) denote the inertia matrix and angular velocity vector, respectively, and \({\varvec{g}}\) denotes the gravitational acceleration vector. \(\mathbf {n}^{ij}\) and \(\mathbf {t}^{ij}\) are the normal and tangential unit vectors, respectively. Meanwhile, \(\mathbf {n}^{ij}\) has the direction pointing from particle j to particle i, and the tangential unit vector has the direction opposite to the relative tangential displacement between these two particles. \(\mathbf {c}^{ij}\) is another unit vector pointing from the center of particle i to the contact point with particle j. In this current work, the equations of motion of all particles are assimilated by using a velocity Verlet time-stepping scheme [17, 43, 56].

In Eq. (1), the normal contact force \(f_n\) is calculated as a linear combination of the normal elastic force and normal damping force between two particles i and j [43, 57], and this means that the effects induced by the deformation at the contact are negligible as compared to the effects of cohesion and the contact viscosity.

$$\begin{aligned} \displaystyle {f_{\mathrm {n}} = f_{\mathrm {n}}^e + f_{\mathrm {n}}^d} \ . \end{aligned}$$
(2)

where \(f_{\mathrm {n}}^e=k_{\mathrm {n}} \delta _{\mathrm {n}}\) is the normal elastic force, expressed as a linear function of the normal elastic deflection \(\delta _{\mathrm {n}}\) at the contact point, \(k_n\) denotes the normal stiffness, and \(f_{\mathrm {n}}^d= \gamma _{\mathrm {n}} \dot{\delta }_{\mathrm {n}}\) denotes the damping force that is proportional to the relative normal velocity \(\dot{\delta }_{\mathrm {n}}\), where \(\gamma _{\mathrm {n}}\) is the normal viscous damping. These contact forces occur only when \(\delta _n < 0\), and this means that there is an overlap between particles in contact.

The tangential force \(f_{\mathrm {t}}\) is obtained by considering the Coulomb friction law, and this force is the sum of a tangential elastic force \(f_{\mathrm {t}}^e = k_{\mathrm {t}} \delta _{\mathrm {t}}\) and a tangential damping force \(f_{\mathrm {t}}^d = \gamma _{\mathrm {t}} \dot{\delta }_{\mathrm {t}} \), where \(k_{\mathrm {t}}\) and \(\gamma _{\mathrm {t}}\) denote the tangential stiffness and the tangential viscous damping parameter, respectively, and \(\dot{\delta }_{\mathrm {t}}\) denotes the tangential velocity, obtained as a derivation of the relative tangential displacement \(\delta _{\mathrm {t}}\). According to the Coulomb friction law, the tangential force is the minimum value between \((\mu f_{\mathrm {n}})\) and the sum of the tangential elastic force \(k_{\mathrm {t}}\delta _{\mathrm {t}}\) and tangential damping one \(\gamma _{\mathrm {t}} \dot{\delta }_{\mathrm {t}}\) [39, 43, 54]:

$$\begin{aligned} f_{\mathrm {t}} = -\!\min \left\{ (k_{\mathrm {t}}\delta _{\mathrm {t}} + \gamma _{\mathrm {t}} \dot{\delta }_{\mathrm {t}}), \mu f_{\mathrm {n}} \right\} . \end{aligned}$$
(3)
Fig. 1
figure 1

a A schematic drawing representation the capillary bond with the different forces acting between particle i and its neighboring particle j, b capillary attraction force \(f_{\mathrm {c}}\) plotted as a function of the normal elastic deflection distance \(\delta _{\mathrm {n}}\) up to the cutoff distance \(d_{\mathrm {c}}\) between particles i and j for different values of the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\)

Full size image

As mentioned above, the liquid is in the form of the capillary bonds between spherical particles [14, 58]. This binding liquid is assumed to be homogeneously distributed in the initial state of wet particle agglomerate. In our simulations, the capillary bridges are characterized by the liquid–vapor surface tension and the liquid viscosity. These nature properties of the liquid induce the capillary attraction forces and viscous forces [16, 33, 59]. These cohesive and viscous forces tend to increase the mechanical strength of the agglomerate. In this current work, we assumed that the binding liquid can be broken irreversibly due to the drainage and absorption on the particle rough surface. This means that the capillary bridges cannot be reformed during the test.

The capillary attraction force \(f_{\mathrm {c}}\) between two near-neighboring particles is proportional to the volume \(V_{\mathrm {b}}\) of the capillary bond, the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\), and the solid–liquid–gas contact angle \(\theta \). In our simulations, \(\theta \) is set to zero as a consequence of considering the full covering of the liquid binding on the particle surface. The capillary cohesion force \(f_{\mathrm {c}}\) is an approximate solution of the Laplace–Young equation [60,61,62], as given following and plotted in Fig. 1:

$$\begin{aligned} f_{\mathrm {c}} = \left\{ \begin{array}{@{}ll@{}} -\kappa \ R, &{} \text{ for }\ \delta _{\mathrm {n}} < 0, \\ -\kappa \ R\ e^{-\delta _{\mathrm {n}} /\lambda }, &{} \text{ for }\ 0 \le \delta _{\mathrm {n}} \le d_{\mathrm {c}}, \\ 0, &{} \text{ for }\ \delta _{\mathrm {n}} > d_{\mathrm {c}}, \end{array}\right. \end{aligned}$$
(4)

where \(R = \sqrt{R_{\mathrm {i}} R_{\mathrm {j}}}\) is the geometrical mean radius of two particles radii \(R_{\mathrm {i}}\) and \(R_{\mathrm {j}}\) and \(\kappa \) is the capillary force pre-factor, as given by:

$$\begin{aligned} \kappa = 2\pi \gamma _{\mathrm {s}} \cos \theta . \end{aligned}$$
(5)

This cohesion force was found to provide an excellent agreement with experimental data on the cohesion of wet granular materials [62]. The cutoff distance \(d_{\mathrm {c}}\) is given by [46]

$$\begin{aligned} \displaystyle {d_{\mathrm {c}} = \left( 1+\frac{\theta }{2}\right) V_{\mathrm {b}}^{1/3} } \ . \end{aligned}$$
(6)

\(\lambda \) (in Eq. 4) is the characteristic length, illustrated the exponential fall-off of the capillary attraction force, as given following:

$$\begin{aligned} \lambda = c\ h(r)\ \left( \frac{V_{\mathrm {b}}}{R'}\right) ^{1/2}, \end{aligned}$$
(7)

where \(R'= 2R_{\mathrm {i}} R_{\mathrm {j}}/(R_{\mathrm {i}}+R_{\mathrm {j}})\) denotes the harmonic mean radius between particles i and j, \(r\!=\!\max \{ R_{\mathrm {i}}/R_{\mathrm {j}} ; R_{\mathrm {j}}/R_{\mathrm {i}}\}\) is the size ratio between two primary particles i and j, \(h(r) = r^{-1/2}\), and \(c \simeq 0.9\) [57, 62]. Figure 1b displays the capillary cohesion force \(f_{\mathrm {c}}\), plotted as a function of the normal elastic deflection distance \(\delta _{\mathrm {n}}\) up to the cutoff distance \(d_{\mathrm {c}}\) between two near-neighboring particles for different values of the surface tension of the binding liquid.

The normal liquid viscous force \(f_{\mathrm {v}}\) in each capillary bond between two primary particles is proportional to the viscosity of the liquid \(\eta \), the particle size, and the relative normal velocity \(v_{\mathrm {n}}\), obtained by taking a derivation of the normal elastic deflection distance \(\delta _n\), but it is inversely proportional to the gap between two particles [16, 17, 33, 42], as given by:

$$\begin{aligned} f_{\mathrm {v}} = \frac{3}{2} \pi R^2 \eta \frac{{v_{\mathrm {n}}}}{\delta _{\mathrm {n}}}, \end{aligned}$$
(8)

where \(v_{\mathrm {n}}\) is a negative value when the normal elastic deflection distance is increasing. In the viscous points of view, the liquid viscous force tends to diverge when \(\delta _{\mathrm {n}}\) tends to zero. In order to prevent this divergence behavior of the lubrication forces, the characteristic length \(\delta _{\mathrm {0}}\) is defined, reflecting the roughness of the particle surface. For the positive separation distance \((\delta _{\mathrm {n}} > 0)\),

$$\begin{aligned} f_{\mathrm {v}} = \frac{3}{2} \pi R^2 \eta \frac{{v_{\mathrm {n}}}}{\delta _{\mathrm {n}}+\delta _{\mathrm {0}}}. \end{aligned}$$
(9)

The liquid viscous force is obtained as a largest value when occurring the overlap between near-neighboring particles (\(\delta _{\mathrm {n}} \leqslant 0\)),

$$\begin{aligned} f_{\mathrm {v}} = \frac{3}{2} \pi R^2 \eta \frac{{v_{\mathrm {n}}}}{\delta _{0}}. \end{aligned}$$
(10)

Similar to the cohesive force, the viscous force \(f_{\mathrm {v}}\) is expressed as a function of the normal elastic deflection distance \(\delta _{\mathrm {n}}\) up to the cutoff distance \(d_{c}\) between two particles for a given value of \(v_{\mathrm {n}}\) for different values of \(\eta \), as shown in Fig. 2. As already mentioned above, the relative normal velocity \(v_{\mathrm {n}}\) can be positive or negative corresponding to the contraction and extension of the binding liquid.

Fig. 2
figure 2

Lubrication force \(f_{\mathrm {v}}\) plotted as a function of the normal elastic deflection distance \(\delta _{\mathrm {n}}\) up to the cutoff distance \(d_{\mathrm {c}}\) between two primary particles for different values of the viscosity \(\eta \) of the binding liquid and a given value of the relative normal velocity \(v_{\mathrm {n}}\) (\(5 \times 10^{-3}\) m/s)

Full size image
Fig. 3
figure 3

a Snapshots of a wet agglomerate impacting a rigid plane and evolution of granule deformation from initiation to the final-stage deposition for a given value of the liquid–vapor surface tension (\(\gamma _{\text {s}} = 14.4\) N/m), the liquid viscosity (\(\eta =3000\) mPa s), and the initial velocity \(v_{\text {0}} = 4.0\) m/s. b Compressive and c tensile force chains of agglomerate at the early-stage impact, respectively

Full size image

2.3 Impact test

In order to construct a spherical agglomerate of wet primary particles, we first prepared a large sample of nearly 70.000 spherical particles by means of isotropic compaction in a cube. We introduced a weak size polydispersity by uniformly varying the particle diameter in a range \(d_{\mathrm {max}} = 2 d_{\mathrm {min}}\). The particle size distribution is assumed to be uniformed by particle volume fraction. The particle diameter is divided into three different size classes, and all the size classes have the same volume, i.e., a small number of large particle sizes and a large number of small particle sizes. This distribution leads to a dense granular packing due to the filling of small particles to the pore spaces between large particles. After all spherical particles reached an equilibrium state in the case of without considering the capillary attraction forces and viscous forces, a spherical probe was introduced in the center of the cube and its diameter was increased until reaching exactly 31.470 spherical particles inside the probe. Then, these spherical particles were extracted and the cohesive forces and viscous forces were activated between neighboring particles until reaching once more equilibrium state.

Table 1 Systematic parameters used in the simulations
Full size table

To investigate the mechanical strength, deformation, and connectivity of wet particle agglomerates at its early-stage, final deposition stage, and during the transition, we performed impact test, by leaving a wet agglomerate from a height that equals a half of its radius, measured from the lowest point of the agglomerate to a flat plane, as shown in Fig. 3. We ran totally 234 simulations by systematically varying the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) in a broad range [1.0, 14.4] N/m, the liquid viscosity \(\eta \) in a range [1.0, 3000.0] mPa s, and the initial velocity \(v_{\mathrm {0}}\) in a range [1.0, 5.0] m/s with the gravity set to \(g = 9.81\) m/s\(^2\). The impact velocity \(v_{\mathrm {i}}\) is at the impact time when the agglomerate starts colliding with the plane. The friction coefficient between primary particles and between particle and plane was set to 0.5. All the system parameters are given in Table 1. The data points presented in the following are average values for the agglomerate strength or extracted as a single value for the deposition and connectivity of such agglomerate.

Figure 4 displays the evolution of mean particle velocity v as a function of \(t\times g/v_{\mathrm {0}}\) for different values of the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) of capillary bridges with a given value of the liquid viscosity \(\eta \) and the initial impact velocity \(v_{\mathrm {0}}\). All primary wet particles are first assigned an initial velocity \(v_{\mathrm {0}}\) at their original positions. The granule falls down with a velocity that slightly increased due to the effects of particle gravity and then interacts with the plane at an impact velocity \(v_{\mathrm {i}}\). The agglomerate velocity then declines rapidly and undergoes the deposition stages due to the effects of liquid properties and the dissipation of particle energy via the solid and liquid interactions. This declination rate is proportional to the surface tension \(\gamma _{\mathrm {s}}\) of the liquid. The granule then reaches the stable state (the final-stage deposition) at the end of the impact test more or less fast depending on the cohesion of the liquid.

During an impact, the mechanical strength of wet particle agglomerate changes as a consequence of occurring the relative displacements and interactions between particles. The vertical stress \(\sigma _{\mathrm {zz}}\) of wet particle agglomerate can be obtained by considering the normal forces and the branch vectors joining the particle centers:

$$\begin{aligned} \sigma _{\mathrm {zz}} = \frac{1}{V_{\mathrm {a}}} \sum _{k=1}^{N_{\mathrm {b}}} f_{\mathrm {z}}^k \ell _{\mathrm {z}}^k=n_{\mathrm {b}} \langle f_{\mathrm {z}}^k \ell _{\mathrm {z}}^k \rangle _{\mathrm {k}}, \end{aligned}$$
(11)

where \(V_{\mathrm {a}}\) is the volume of agglomerate, \(N_{\mathrm {b}}\) denotes the number of capillary bonds in current step, \(f_{\mathrm {z}}^k\) and \(l_{\mathrm {z}}^k\) are the z-components of the force vector and branch vector of capillary bond k, respectively, and \(n_{\mathrm {b}} = N_{\mathrm {b}}/V_{\mathrm {a}}\) is the number density of the capillary bonds. The symbol \(\langle \cdots \rangle _{\mathrm {k}}\) denotes averaging over all contacts k in the volume.

Figure 5 shows the average vertical stress \(\sigma _{\mathrm {zz}}\) that is expressed as a function of \(t\times g/v_{\mathrm {0}}\) for different values of \(\gamma _{\mathrm {s}}\) and for a given value of the liquid viscosity and the impact velocity. The granule strength equals zero before occurring the impact with the plane corresponding to its initial stability. Then, the vertical stress soars immediately and reaches the early-stage plastic threshold. The time appearing the early-stage threshold is independent to the surface tension \(\gamma _{\mathrm {s}}\) of the liquid, whereas the value of the stress threshold is proportional to \(\gamma _{\mathrm {s}}\). This can be explained due to the immediate increase in the contact forces between primary particles after receiving the collisional force at the contact point between agglomerate and the flat plane, whereas there is no enough time for the particle movements. The threshold is a signature of the plastic behavior of wet particle agglomerate due to the rearrangement of primary particles and the tensile effects of the capillary bonds, which have the direction perpendicular to the impact direction, as easily visualized in the zoom in Fig. 3c. After that, the granule stress declines rapidly as a consequence of irreversibly broken of the capillary bonds. As we can see, the speed of this declination and the stabilized achievement is proportional to \(\gamma _{\mathrm {s}}\), which represented via the speed of reaching the final-stage deposition, as shown in Fig. 5.

Fig. 4
figure 4

Evolution of average velocity of primary particles for different values of the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) for a given value of the liquid viscosity \(\eta = 3000\) mPa s and the initial velocity \(v_{\mathrm {0}} = 4.0\) m/s

Full size image
Fig. 5
figure 5

Evolution of average vertical stress \(\sigma _{\mathrm {zz}}\) as a function of time for different values of the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) for a given value of the liquid viscosity \(\eta = 3000\) mPa s and the initial velocity \(v_{\mathrm {0}} = 4.0\) m/s

Full size image
Fig. 6
figure 6

The vertical size D of agglomerate normalized by its initial diameter \(D_{\mathrm {0}}\) as a function of \(t\times g/v_{\mathrm {0}}\) for different values of \(\gamma _{\mathrm {s}}\) for a given value of the liquid viscosity \(\eta = 3000\) mPa s and the initial velocity \(v_{\mathrm {0}} = 4.0\) m/s

Full size image
Fig. 7
figure 7

Loss coordination number \(Z_{\mathrm {lost}}\) of wet particle agglomerates as a function of \(t\times g/v_{\mathrm {0}}\) for different values of the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) for a given value of the liquid viscosity \(\eta = 3000\) mPa s and the initial velocity \(v_{\mathrm {0}} = 4.0\) m/s

Full size image

The average vertical stress of a wet particle agglomerate impacting a flat plane reflects the microstructure and the deformation of the granule, which depend on the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\), the liquid viscosity \(\eta \), and the impact velocity \(v_{\mathrm {i}}\). In our simulations, the granule deformation is characterized by the height D during the impact test, and the microstructure of wet agglomerate can be characterized by the number of loss capillary bridges \(Z_{\mathrm {lost}}\), defined as an average number of loss capillary bonds. Figure 6 displays \(D/D_{\mathrm {0}}\) as a function of \(t\times g/v_{\mathrm {0}}\) for broad range of values of \(\gamma _{\mathrm {s}}\) and for a given value of \(\eta = 3000\) mPa s and \(v_{\mathrm {0}} = 4.0\) m/s, where \(D_{\mathrm {0}}\) is the initial diameter of agglomerate. For each value of the liquid–vapor surface tension \(\gamma _s\), the granule has a final deposition height (or final deposition diameter) \(D_{\mathrm {stop}}\), and this increases proportionally to the surface tension of the liquid.

Figure 7 represents the evolution of the average number of loss capillary bonds \(Z_{\mathrm {lost}}\) as a function of the time for different values of \(\gamma _{\mathrm {s}}\). \(Z_{\mathrm {lost}}\) first increases rapidly when occurring the impact between agglomerate and plane. Then, this average number reaches a plateau in the deposition stages more less fast depending on the values of the liquid properties and the impact rate, and there is no more capillary bond broken in its final deposit stage. In the final-stage deposition, \(Z_{\mathrm {lost}}\) decreases proportionally to \(\gamma _{\mathrm {s}}\) of the binding liquid. This means that the granule is not abrupt rupture during the test, and it could be deformed or fully spread depending on the values of system parameters. Thus, the final deposition stage of the impact test is an important feature, which will be analyzed in more detail below.

3 Mechanical strength and deposition of agglomerate

We now consider two more parameters (the liquid viscosity and the impact velocity) which are varied in different ranges of their values. Due to the observation of the ductile behavior of wet particle agglomerates, the plastic threshold \(\sigma _{\mathrm {p}}\) is observed in all our numerical results by considering the average value of five data points closet to the peak value. Figure 8 displays the mean peak stress \(\sigma _{\mathrm {p}}\) normalized by the cohesive stress \(\sigma _{\mathrm {c}} = \gamma _{\mathrm {s}}/\langle d \rangle \) as a function of the impact velocity \(v_{\mathrm {i}}\) for all our 234 simulations. The impact velocity \(v_{\mathrm {i}}\) is obtained at the impact time when the lowest primary particles of the granules start touching the plane. In order to improve the visibility of the data points, we plotted them separately although they obtained at the same impact velocity \(v_{\mathrm {i}}\), as shown in Figs. 8, 9, and 10. As we can see, the granule strength increases proportionally to \(v_{\mathrm {i}}\) as well as the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) and the liquid viscosity \(\eta \). This can be explained by the fact that the strengthen of the liquid properties corresponding to the cohesive and viscous stresses of the binding liquid and the increase in the impact rate leads to the high early-stage forces inside agglomerates [63].

Fig. 8
figure 8

Peak strength \(\sigma _{\mathrm {p}}\) of agglomerates normalized by the cohesive stress \(\sigma _{\mathrm {c}} = \gamma _{\mathrm {s}}/\langle d \rangle \) as a function of the impact velocity \(v_{\mathrm {i}}\) for all of simulations. The error bars represent the standard deviation in each simulation during reaching plastic threshold. For each set of simulations, the symbols and their colors correspond to each value of \(\eta \) and \(v_{\mathrm {i}}\) and varying \(\gamma _{\mathrm {s}}\) in a broad range of values. (Color figure online)

Full size image
Fig. 9
figure 9

Deposit vertical diameter \(D_{\mathrm {stop}}\) normalized by initial diameter \(D_{\mathrm {0}}\) of wet agglomerates as a function of the impact velocity \(v_{\mathrm {i}}\) for all of simulations. For each set of simulations, the symbols and their colors represent for each value of \(\eta \) and \(v_{\mathrm {i}}\) and a broad range of values of \(\gamma _{\mathrm {s}}\). (Color figure online)

Full size image

The final-stage deposition behavior of impact agglomerates is also an interesting feature, which we analyze in more detail in order to get a better understanding of the effects of liquid properties and impact velocity on the impact dynamics of spherical agglomerates. We access the final deposition stage of granules by considering its height \(D_{\mathrm {stop}}\) and the loss coordination number \(Z_{\mathrm {lost}}\). Figure 9 shows the normalized granule height \(D_{\mathrm {stop}}/D_{\mathrm {0}}\) at its final deposit step as a function of \(v_{\mathrm {i}}\) for different values of \(\gamma _{\mathrm {s}}\) and the liquid viscosity \(\eta \). This ratio clearly increases proportional to \(\gamma _{\mathrm {s}}\) and \(\eta \), whereas it declines systematically as a function of the impact velocity \(v_{\mathrm {i}}\).

Figure 10 displays the loss coordination number \(Z_{\mathrm {lost}}\) at the final deposition stage as a function of the impact velocity \(v_{\mathrm {i}}\) for all of our simulations. The increase in the cohesion and the viscosity of the binding liquid leads to reduction in the number of loss capillary bonds, whereas the increase in the impact speed leads to an increase in the average number of loss capillary bridges. We also see that the increase in \(Z_{\mathrm {lost}}\) is much more significant with \(v_{\mathrm {i}}\) than the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\) and the liquid viscosity \(\eta \). However, there seems to have a unified tendency of the evolution of the plastic strength threshold, the deposition height, and the loss coordination number of wet agglomerates, which could be expected to scale by an appropriate parameter.

Fig. 10
figure 10

Average lost coordination number \(Z_{lost}\) of wet agglomerates as a function of the impact velocity \(v_{\mathrm {i}}\). The symbols and their colors represent for each value of \(\eta \) and \(v_{\mathrm {i}}\) and a broad range of values of the surface tension \(\gamma _{\mathrm {s}}\) of the binding liquid. (Color figure online)

Full size image

4 Power-law scaling behavior

The issue is whether all different data points of the agglomerate strength \(\sigma _{\mathrm {p}}/\sigma _{\mathrm {c}}\), the final-stage deposition height \(D_{\mathrm {stop}}/D_{\mathrm {0}}\), and loss coordination number \(Z_{\mathrm {lost}}\) at the final deposition stage of impact agglomerate can be expressed as a collapsed function of a general inertial number that combines the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\), the liquid viscosity \(\eta \), and the impact rate \(\dot{\gamma }= v_{\mathrm {i}}/D_{\mathrm {0}}\). Before considering this option, we first define two dimensionless numbers in our system: (1) The Capillary number \(\text{ Ca } = \sigma _{\mathrm {v}}/\sigma _{\mathrm {c}} = \eta \dot{\gamma }\langle d \rangle /\gamma _{\mathrm {s}}\) is a ratio of the viscous stress \(\sigma _{\mathrm {v}}\) and the cohesive stress \(\sigma _{\mathrm {c}}\) and (2) the Stokes number \(\text{ St } = \sigma _{\mathrm {i}}/\sigma _{\mathrm {v}} = \rho \langle d \rangle ^2 \dot{\gamma }/\eta \) is a ratio of the inertial stress \(\sigma _{\mathrm {i}}\) to the viscous stress \(\sigma _{\mathrm {v}}\).

In the steady-state confined shearing flows of unsaturated granular materials, Vo et al. [33] showed that the rheological properties and effective viscosities of such flows can be described as a general inertial number \(I_{\mathrm {m}}\) that combines the inertial number I, the cohesion index \(\xi \), and the Stokes number \(\text{ St }\) by considering the additive mechanism of stresses exert on particles. \(I_{\mathrm {m}}\) is expressed by the following function:

$$\begin{aligned} I_{\mathrm {m}} = I \left( \frac{1 + \beta /\text{St }}{1+\alpha \xi } \right) ^{1/2}, \end{aligned}$$
(12)

where I denotes the inertial number, defined as a ratio of the relaxation time and the impact time (\(I = \dot{\gamma }\langle d \rangle \sqrt{\rho /\sigma _{\mathrm {n}}}\)), in which \(\dot{\gamma }= v_{\mathrm {i}}/D_{\mathrm {0}}\) is the impact rate of an agglomerate on the flat plane, \(\langle d \rangle \) is the mean particle diameter, \(\rho \) denotes the particle density, and \(\sigma _{\mathrm {n}}\) is the confining pressure of the confined granular sample, the cohesion index \(\xi =\sigma _{\mathrm {c}}/\sigma _{\mathrm {n}}\), defined as a ratio of the cohesion stress \(\sigma _{\mathrm {c}}\) to the confining stress \(\sigma _{\mathrm {n}}\), and \(\alpha \) and \(\beta \) are the pre-factors that depend on material parameters.

However, in our current work, the gravity or the applied confining pressure does not prevail, the cohesive stress largely exceeds the confining stress, and the inertial stress is much more dominant as compared to the viscous stress exerts on particles. Thus, it is advantageous to compare the inertial stress \(\sigma _{\mathrm {i}}\) with the cohesive stress \(\sigma _{\mathrm {c}}\) rather than the confining stress \(\sigma _{\mathrm {n}}\). This means that the inertial number I in Eq. (12) can be easily replaced by the expression \(\sqrt{\text{ Ca } \times \text{ St } \times \xi }\). Hence, the generalized inertial number is re-expressed as a function of two dimensionless numbers: \(\text{ Ca }\) and \(\text{ St }\), which we called the Capillary–Stokes inertial number \(I_{\mathrm {CS}}\) in this impact test:

$$\begin{aligned} I_{\mathrm {CS}} = (\text{ Ca } \times \text{ St})^{1/2}. \end{aligned}$$
(13)
Fig. 11
figure 11

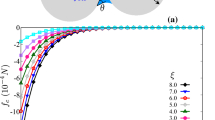
Normalized strength \(\sigma _{\mathrm {p}}/\sigma _{\mathrm {c}}\) of wet agglomerates as a function of Capillary–Stokes inertial number \(I_{\mathrm {CS}}\) defined by Eq. (13), expressed in log–log scale. The error bars represent the standard deviation of the data over the plastic threshold. The symbols and their colors correspond to the parameters that are varied in different ranges. The dark blue line is the functional form of Eq. (14). (Color figure online)

Full size image

Figure 11 shows the normalized strength \(\sigma _{\mathrm {p}}/\sigma _{\mathrm {c}}\) of wet agglomerates impacting a flat plane as a function of the Capillary–Stokes inertial number \(I_{\mathrm {CS}}\), which is independent to the viscosity of the binding liquid, allowing a remarkable collapse of all our simulation data. This observation can be expressed due to the domination of the inertial stress generated from the particle movements in the impact test as compared to the viscous stress of the liquid binding. \(I_{\mathrm {CS}}\) provides a unified description of the plastic stress threshold by capturing the effects of the particle interactions including cohesive and viscous stresses in addition to the elastic and frictional forces. All the data points are nicely fitted by the following power-law function form:

$$\begin{aligned} \frac{\sigma _{\mathrm {p}}}{\sigma _{\mathrm {c}}}(I_{\mathrm {CS}}) = a_{\mathrm {s}} I_{\mathrm {CS}}^2 + b_{\mathrm {s}} I_{\mathrm {CS}} + c_{\mathrm {s}}, \end{aligned}$$
(14)

with \(a_{\mathrm {s}} = 95.00\), \(b_{\mathrm {s}} = 8.10\), and \(c_{\mathrm {s}} = 0.17\). The fitting form reveals the power-law dependence of the mechanical strength of wet particle agglomerates on the Capillary–Stokes inertial number.

In order to get a better understanding of the evolution of the normalized peak stress \(\sigma _{\mathrm {p}}/\sigma _{\mathrm {c}}\) and the scaling behavior of this macroscopic strength of a single wet agglomerate impacting a flat surface, we analyze the micro-mechanical properties of wet granular materials. As previous studies, the macroscopic properties such as the shear strength and packing fraction of granular materials have its microscopic origins [15, 38, 64,65,66]. In the current work considering the rapid impact of agglomerates on the rigid plane, the microscopic properties are characterized by the mean normal compressive force \(\langle f_{\mathrm {n}}^+ \rangle \) and the mean normal tensile force \(\langle f_{\mathrm {n}}^- \rangle \) between primary particles at the peak stage. The compressive forces obtained along the impact direction, whereas the tensile forces have the direction perpendicular to the impact direction, as shown in Fig 3b and c. The normal tensile forces \(f_{\mathrm {n}}^-\) shown in this current work are its absolute values. Figure 12a and b displays the evolution of \(\langle f_{\mathrm {n}}^+ \rangle \) and \(\langle f_{\mathrm {n}}^- \rangle \) as a function of the impact velocity \(v_{\mathrm {i}}\) for all our simulations. It is interesting to see that both \(\langle f_{\mathrm {n}}^+ \rangle \) and \(\langle f_{\mathrm {n}}^- \rangle \) increase with increasing the liquid–vapor surface tension \(\gamma _{\mathrm {s}}\), the liquid viscosity \(\eta \), and the impact speed \(v_{\mathrm {i}}\). Remarkably, the mean normal compressive forces \(\langle f_{\mathrm {n}}^+ \rangle \) are almost double as compared to the mean normal tensile forces \(\langle f_{\mathrm {n}}^- \rangle \), and this means that the macroscopic strength of the agglomerate at its early-stage impact is mainly contributed by the compressive forces between primary particles.

Fig. 12
figure 12

Mean normal compressive force \(\langle f_{\mathrm {n}}^+ \rangle \) (a) and mean normal tensile force \(\langle f_{\mathrm {n}}^- \rangle \) (b) as a function of the impact velocity \(v_{\mathrm {i}}\) for all simulations. For each set of simulations, the symbols and their colors (as shown in Fig. 11) correspond to each value of \(\eta \) and \(v_{\mathrm {i}}\) and varying \(\gamma _{\mathrm {s}}\) in a broad range of values. (Color figure online)

Full size image

Indeed, Fig. 13a and b shows the normalized mean normal compressive force \(\langle f_{\mathrm {n}}^+ \rangle /f_{\mathrm {c}}\) and the normalized mean normal tensile force \(\langle f_{\mathrm {n}}^- \rangle /f_{\mathrm {c}}\) between primary particles that increase exponentially as a function of \(I_{\mathrm {CS}}\). Meanwhile, \(\langle f_{\mathrm {n}}^+ \rangle /f_{\mathrm {c}}\) nicely collapses on a master curve as a function of the Capillary–Stokes inertial number; \(\langle f_{\mathrm {n}}^- \rangle /f_{\mathrm {c}}\) does not behave well as a function of the same dimensionless inertial number \(I_{\mathrm {CS}}\). In particular, at low values of \(I_{\mathrm {CS}}\) corresponding to small values of the impact speed and large values of the liquid–vapor surface tension, the normalized mean tensile forces are nearly insensitive. \(\langle f_{\mathrm {n}}^- \rangle /f_{\mathrm {c}}\) then separates significantly with large values of \(I_{\mathrm {CS}}\). This separation behavior of the mean normal tensile forces may be due to the extension properties of the viscous interactions at high impact rate. Interestingly, the increasing tendencies of \(\langle f_{\mathrm {n}}^+ \rangle /f_{\mathrm {c}}\) and \(\langle f_{\mathrm {n}}^- \rangle /f_{\mathrm {c}}\) are nearly similar to that of the macroscopic strength of agglomerate at its early-stage impact. Thus, these observations strongly support for the increase and the scaling behavior of the normalized mechanical strength \(\sigma _{\mathrm {p}}/\sigma _{\mathrm {c}}\), as presented above.

Fig. 13
figure 13

Normalized mean normal compressive force \(\langle f_{\mathrm {n}}^+ \rangle \) (a) and normalized mean normal tensile force \(\langle f_{\mathrm {n}}^- \rangle \) (b) by the cohesion force \(f_{\mathrm {c}}\) as a function of \(I_{\mathrm {CS}}\) for all our simulations

Full size image

Although \(I_{\mathrm {CS}}\) provides a good description of the granule strength and the normal compressive forces at its early-stage impact, it is essential to check its robustness which reflects the final deposition stage of wet agglomerates. As mentioned above, the final deposition stage of agglomerate is characterized by its final-stage deposition height \(D_{\mathrm {stop}}\) that can be explained by the cumulative loss coordination number \(Z_{\mathrm {lost}}\). Figure 14 displays the normalized \(D_{\mathrm {stop}}/D_{\mathrm {0}}\) nearly collapses well on a master curve as a function of the same Capillary–Stokes inertial number \(I_{\mathrm {CS}}\). As we can see, the particle agglomerates are nearly insensitive with small values of \(I_{\mathrm {CS}}\) (\(\leqslant 3 \times 10^{-2}\)), and this value can be obtained by choosing appropriate values of the liquid–vapor surface tension and the liquid viscosity as well as controlling an impact velocity that is small enough. The impact agglomerates then rapidly deform as \(I_{\mathrm {CS}}\) exceeds approximately \(3\times 10^{-2}\) as a consequence of losing capillary bonds due to the increase in the impact energy. Remarkably, all the data points of \(D_{\mathrm {stop}}/D_{\mathrm {0}}\) are nice fitted by the inverse of a power-law function form:

$$\begin{aligned} \frac{D_{\mathrm {stop}}}{D_{\mathrm {0}}}(I_{\mathrm {CS}}) = \frac{1}{1 + d\times I_{\mathrm {CS}}^{n_{\mathrm {d}}}}, \end{aligned}$$
(15)

where \(d = 67.20\) and \(n_{\mathrm {d}} = 1.55\).

Fig. 14
figure 14

Normalized vertical diameter at the final deposit stage \(D_{\mathrm {stop}}\) by initial diameter \(D_{\mathrm {0}}\) of wet agglomerates as a function of Capillary–Stokes inertial number \(I_{\mathrm {CS}}\) defined by Eq. (13). The symbols and their colors are the same as those in Fig. 11. (Color figure online)

Full size image
Fig. 15
figure 15

Cumulative loss coordination number \(Z_{\mathrm {lost}}\) as a function of Capillary–Stokes inertial number \(I_{\mathrm {CS}}\)

Full size image

Thus, the mechanical strength and the final deposit deformation of agglomerates are nicely scaled as a function of the Capillary–Stokes inertial number. However, the texture variable does not follow this scaling. For example, the cumulative loss coordination number \(Z_{\mathrm {lost}}\) at the final deposition stage is not scaled by \(I_{\mathrm {CS}}\) as shown in Fig. 15. This could be explained by the fact that the coordination number or the cumulative loss coordination number affects the granule strength and the final-stage deposition height, but the important parameter is not only the number of contacts or the number of loss capillary contacts but also the orientations and the position of the contacts in the impact test.

5 Conclusions

In this paper, we investigate the dynamic properties and scaling behavior of a single wet particle agglomerate impacting a flat plane by means of 3D discrete element method simulations. The agglomerate is modeled by introducing an approximate analytical expression of the capillary attraction forces and lubrication forces in capillary contacts between particles having the separation distance no exceed the debonding distance in addition to the elastic and frictional forces at their contacts. In this work, we assumed that the liquid is homogeneously distributed in the initial state and irreversibly broken of the capillary bonds during the impact.

In our simulations, we quantified the impact properties of wet agglomerate characterized by the mechanical strength and the microscopic properties at the early-stage impact and the deformation and loss coordination number in the final deposition stage as well as during the impact. We varied systematically broad range of values of three different parameters: (i) the liquid–vapor surface tension \(\gamma _{\text {s}}\), (ii) the liquid viscosity \(\eta \), and (iii) the impact velocity \(v_{\text {i}}\) by varying the initial velocity \(v_{\text {0}}\).

As expected, the granule represents the ductile behavior and abrupt rupture due to losing capillary contacts and the tensile effects of the capillary bonds. The stress threshold occurs in the early-stage impact, and its values increase proportionally to the liquid–vapor surface tension, the liquid viscosity, and the impact velocity. The increase in the mechanical strength of agglomerates is due to the increase in the normal compressive forces and normal tensile forces between primary particles. The final-stage deposition height and loss coordination number also increase as a function of the impact speed, whereas they decrease proportionally to the cohesion stress and viscous stress of the liquid. Remarkably, by considering the stress additivity in each particle interaction such as cohesive and viscous stresses of the binding liquid in addition to the frictional and elastic forces, the Capillary–Stokes inertial number combining Capillary number and Stokes number nicely scales the granule stress threshold and the final-stage deposition height of agglomerates. By fitting the collapsed data, we determined two power-law function forms. These power-law scalings behaviors are the strong evidence for the role of the liquid properties and the impact speed (or the impact rate) in cohesive granular materials.

To define the scaling parameter (Capillary–Stokes inertial number), we first considered the complex flows of wet granular materials under imposing pressure-controlled condition. In this, the rheology and granular texture of such flows are controlled by the modified inertial number, which combines the inertial number, cohesion index, and the Stokes number by comparing different stresses with the confining stress [33]. However, if the cohesive stress is dominant as compared to the confining stress in our current work, it is essential to check the ratio of the inertial stress and viscous stress to the cohesive stress. As a result, the Capillary–Stokes inertial number was defined by only combining the Capillary number and Stokes number.

Our results presented in this numerical investigation show consistently the ability of our model to handle the dynamic impact of wet particle agglomerates on a flat plane. It can be extended to simulate the diametrical compression test, the impact of agglomerate on the drum walls or the collision between agglomerate and an intruder, in which the behavior of wet agglomerate can provide insight into the evolution of the mechanical strength and the physical properties such as their breakage, deformation, and microstructure. We thus propose these numerical expectations as well as considering the impact experiments to study the mechanical and physical responses of granule.