1 Introduction

Piezoelectric materials have been of great interest due to their exceptional electro-mechanical coupling characteristics. Functionally graded piezoelectric materials (FGPMs) have been developed to address the high stress concentration in the inner layer of piezoelectric materials and prevent corrosion between bonding layers. In recent years, there has been a growing interest in studying the behavior of FGPMs in micro/nanostructures, which are widely used in structural health monitoring, active vibration control, and electromechanical systems.

Several studies have investigated the behavior of FGPM porous nanoplates using analytical and numerical methods. Kiani et al. (2011) performed the thermo-electrical buckling of piezoelectric FG material Timoshenko beams, while Nguyen et al. (2019) reported on the vibration analysis of functionally graded piezoelectric material porous plates using the C0-type higher-order shear deformation theory (C0-HSDT). Joubaneh et al. (2015) analyzed the thermal buckling of a solid circular plate made of porous material with piezoelectric sensor-actuator patches on its boundary, and Su et al. (2018) reported an exact solution for the electro-mechanical vibration properties of FG piezoelectric plates with their overall boundary. Behjat et al. (2009) studied the static bending, free vibration, and dynamic behavior of FG piezoelectric panels using finite element analysis under various loading types, while Zhang et al. (2008) reported analytical solutions for investigating the behavior of FG piezoelectric cylindrical actuators under a harmonic electric field using elastic membrane and shell theory. The dynamic control of smart piezoelectric composite plates was presented in the publications (Phung-Van et al. 2017a, 2017b) while the static analysis of FGPM structures was investigated in the references (Brischetto and Carrera 2009;). Chen et al. (2002; 2002) analyzed the free vibration of FGPM rectangle-shaped plates and hollow spheres using the laminated approximation approach together with state space formulations.

Owing to the rapid development of nano technique, small-scale effects are very attractive to researchers. Because the nanostructures made of FGMs/piezoelectric materials are usually employed in structural health monitoring, active vibration control and electromechanical systems, the mechanical analysis of FGM/piezoelectric nanostructures (Phung-Van et al. 2020a,b) has been rapidly developed. The thermo-electrical buckling properties of FG piezoelectric Timoshenko nanobeams under in-plane thermal loading and applied electric voltage were investigated by Ebrahimi and Salari (2015) using a Navier type solution and elasticity theory of Eringen. Zenkour and Aljadani (2019b) analyzed the thermo-electrical buckling of the FG piezoelectric nanoscale plate based on the refined hyperbolic higher-order shear deformation theory (HSDT) and Eringen’s nonlocal theory. They also developed an analytical method for analysis of the thermal buckling of the actuated FG piezoelectric porous nanoplates (Zenkour and Aljadani 2019a). The nonlinear formulation of the FG piezoelectric material nanobeams was established by employing the Euler–Bernoulli model and the consistent size-dependent theory (Tadi 2016). Ebrahimi and Barati (2017) conducted an analytical study on the buckling behavior of higher-order shear deformable nanobeams made of FG piezoelectric materials implanted in an elastic foundation. Based on the novel modified couple stress theory and analytical solutions, Mehralian et al. (2016) proposed the size-dependent formulation of shear deformable FG piezoelectric cylindrical nanoshells. Li and Xiao (2021) performed the free vibration of a one-dimensional piezoelectric quasicrystal microbeam using the modified couple stress theory and differential quadrature technique.

The majority of previous research on FGPM structures has been based on analytical methods, with only a few studies focusing on micro/nanostructures. As we’ve known, to solving complex structures in engineering practices, numerical solutions are usually considered as the best candidate to use. This literature gap has prompted researchers to develop an effective numerical solution to analyze nanoplates integrated with piezoelectric materials. For calculating nanoplates, it always requires higher-order derivatives in governing equations. Recently, an effective numerical method, isogeometric analysis (IGA), which has shown several advanced properties, was firstly proposed by Hughes et al. (2005). Non-uniform rational B-spline (NURBS) basis functions are employed by IGA to accurately depict the geometry of structures and estimate the solution fields. One of its significant advantages is its ability to achieve any degree of smoothness by selecting the interpolation order, while lower continuity can be easily achieved through knot insertion. IGA has been effectively employed in various fields such as nanoplates (Phung-Van and Thai 2021; Phung-Van et al. 2021) and microplates (Thai 2020; Hung et al 2022), but its implementation to smart nanoplates is still restricted. Furthermore, the area of research concerning the size-dependent behavior of smart functionally graded piezoelectric nanoplates with porosity has not been thoroughly investigated. This paper aims to address this research gap by proposing an effective numerical approach for conducting such an analysis. The findings of this paper are expected to be of great interest to researchers due to the innovative approach and the potential implications of the results.

2 Functionally graded piezoelectric porous materials

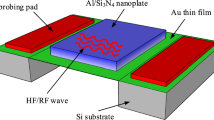
We now consider a functionally graded piezoelectric porous nanoplate (length a, width b, thickness h) under an electric field, as displayed in Fig. 1. In the FGPP, two piezoelectric materials consist of PZT-4 and PZT-5H to be mixed. Through the thickness direction, two porous distributions comprising of (i) even: positions of porosity are randomly distributed in the cross section (FGPP-I) and (ii) uneven: porosity is arranged around middle zone of the cross section (FGPP-II) are considered.

Fig. 1
figure 1

Geometry of FGPP nanoplate

Full size image

Based on the modified power law function scheme, the type of FGPP-I is expressed as

$$P\left( z \right) = P_{t} + \left( {P_{b} - P_{t} } \right)\left( {\frac{z}{h} + \frac{1}{2}} \right)^{n} - \frac{\alpha }{2}\left( {P_{b} + P_{t} } \right)$$
(1)

where P are the effective properties of the material, which include the elastic constants, piezoelectric constants, and dielectric constants; b and t represent the bottom and top surfaces of the plate, respectively; \(\alpha\) is the porosity parameter which defines by 0 < \(\alpha\) < 1 and n is the power index factor.

For the FGPP-II, the effective material property is defined as

$$P\left( z \right) = P_{t} + \left( {P_{b} - P_{t} } \right)\left( {\frac{z}{h} + \frac{1}{2}} \right)^{n} - \frac{\alpha }{2}\left( {P_{b} + P_{t} } \right)\left( {1 - \frac{2\left| z \right|}{h}} \right)$$
(2)

3 Mathematical formulations

3.1 Nonlocal Eringen’s theory

A nanoplate subjected to an external body force (fi) is considered in this research. The governing equations for a homogeneous anisotropic linearly piezoelectric material can be expressed as follows

$$\begin{gathered} \overline{\sigma }_{ij,j} + f_{i} = \rho \ddot{u}_{i} \, \,{\text{in}}\,\,V \hfill \\ \overline{D}_{i,i} = 0{\text{ in}}\,\,V \hfill \\ \end{gathered}$$
(3)

where \(\overline{\sigma }_{ij}\) is the nonlocal stress \(\ddot{u}_{i}\) is the acceleration field; \(\rho\) is the density mass; \(\overline{D}_{i}\) is the nonlocal electric potential and V is the volume.

Constitutive relations for the mechanical–electrical coupling of the FGPP nanoplates are given as

$$\begin{array}{*{20}c} {\sigma_{ij} = C_{{{\text{i}}jkl}} \varepsilon_{{_{kl} }} - e_{ijk} E_{k} } \\ {D_{i} = e_{ijk} \varepsilon_{{_{kl} }} + k_{ik} E_{k} } \\ \end{array}$$
(4)

where \(\sigma_{ij}\) and Di are the local stress and local electric displacement, respectively; \(\varepsilon_{kl}\) and \(E_{k}\) indicate the local strain and electric field components, respectively; \(C_{ijkl}\),\(e_{ijk}\) and \(k_{ik}\) are respectively the elastic, piezoelectric and dielectric constants.

According to the nonlocal law suggested by Eringen, the nonlocal stress \(\overline{\sigma }_{ij}\) and nonlocal electric displacement \(\overline{D}_{i}\) can be defined as

$$\begin{gathered} \overline{\sigma }_{ij} = \int_{V} {\varsigma \left( {\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|,\tau } \right)\sigma_{ij} {\text{d}}{\mathbf{x^{\prime}}}} \hfill \\ \overline{D}_{i} = \int_{V} {\varsigma \left( {\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|,\tau } \right)D_{i} {\text{d}}{\mathbf{x^{\prime}}}} \hfill \\ \end{gathered}$$
(5)

where \({\mathbf{x}}\) is a point in the body;\({\mathbf{x^{\prime}}}\) is a neighbor point of x; the kernel function \(\varsigma \left( {\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|,\tau } \right)\) indicates the nonlocal modulus; \(\tau\) indicates a material constant; \(\left| {{\mathbf{x^{\prime}}} - {\mathbf{x}}} \right|\) is the distance.

By applied the differential operator \(\left( {1 - \lambda \nabla^{2} } \right)\) for Eq. (5), it can be rewritten as (Lu et al. 2007)

$$\begin{gathered} \left( {1 - \lambda \nabla^{2} } \right)\overline{\sigma }_{ij} = \sigma_{ij} \,\,,\,\,\,\left( {1 - \lambda \nabla^{2} } \right)\overline{\sigma }_{ij,j} = \sigma_{ij,j} \hfill \\ \left( {1 - \lambda \nabla^{2} } \right)\overline{D}_{i} = D_{i} \,\,,\,\,\,\left( {1 - \lambda \nabla^{2} } \right)\overline{D}_{i,i} = D_{i,i} \,\, \hfill \\ \end{gathered}$$
(6)

where \(\lambda\) is the nonlocal parameter. Similarly, we can be also used the above differential operator for Eq. (3). It can be formulated as

$$\begin{gathered} \left( {1 - \lambda \nabla^{2} } \right)\overline{\sigma }_{ij,j} + \left( {1 - \lambda \nabla^{2} } \right)f_{i} = \left( {1 - \lambda \nabla^{2} } \right)\rho \ddot{u}_{i} \,\, \hfill \\ \left( {1 - \lambda \nabla^{2} } \right)\overline{D}_{i,i} = 0\,\, \hfill \\ \end{gathered}$$
(7)

Substituting Eq. (6) into Eq. (7) to attain Eq. (8):

$$\begin{gathered} \sigma_{ij,j} + \left( {1 - \lambda \nabla^{2} } \right)f_{i} = \left( {1 - \lambda \nabla^{2} } \right)\rho \ddot{u}_{i} \hfill \\ D_{i,i} \, = \,0 \hfill \\ \end{gathered}$$
(8)

Applying the principle of virtual displacement and virtual electric potential, the integration form of Eq. (8) is expressed by

$$\begin{aligned}&\int\limits_{V} {\sigma_{ij,j} \delta u_{i} {\text{d}}} V + \int\limits_{V} {D_{i,i} \delta \Phi_{i} {\text{d}}} V + \left( {1 - \lambda \nabla^{2} } \right)f_{i} \delta u_{i} = \int\limits_{V} {\left( {1 - \lambda \nabla^{2} } \right)\rho \ddot{u}_{i} \delta u_{i} {\text{d}}} V \end{aligned}$$
(9)

where \(\delta u_{i} ,\,\,\delta \Phi_{i}\) are the virtual displacement vector and the virtual electric potential vector, respectively.

Applying the integration by parts and divergence theorem for the left-hand side of Eq. (9), it can be represented by

$$\begin{gathered} \int_{V} {\sigma_{ij,j} } \delta u_{i} {\text{d}}V = - \int_{V} {\sigma_{ij} } \delta u_{i,j} {\text{d}}V + \int_{{\Gamma_{g} }} {\sigma_{ij} } n_{i} \delta u_{i} {\text{d}}\Gamma_{g} \hfill \\ \int\limits_{V} {D_{i,i} \delta \Phi_{i} {\text{d}}} V = - \int\limits_{V} {D_{i} \delta \Phi_{i,i} {\text{d}}} V + \int\limits_{{\Gamma_{g} }} {D_{i} n_{i} \delta \Phi_{i} {\text{d}}} \Gamma_{g} \hfill \\ \end{gathered}$$
(10)

where \(\Gamma_{g}\) is the Neumann boundary.

Substituting Eq. (10) into Eq. (9) and ignoring the integrated components on the boundary \(\Gamma_{g}\), the final integration form of equilibrium equation is formulated as

$$\begin{aligned}& \int\limits_{V} {\sigma_{ij} \delta u_{i,j} {\text{d}}} V + \int\limits_{V} {D_{i} \delta \Phi_{i,i} {\text{d}}} V + \int\limits_{V} {\left( {1 - \lambda \nabla^{2} } \right)\rho \ddot{u}_{i} \delta u_{i} {\text{d}}} V= \int\limits_{V} {\left( {1 - \lambda \nabla^{2} } \right)f_{i} \delta u_{i} {\text{d}}} V \end{aligned}$$
(11)

By replacing \(\delta u_{i,j} = \delta \varepsilon_{ij}\) and \(\delta {\Phi }_{i,i} = - \delta {\rm E}_{i}\), Eq. (11) is rewritten as

$$\int\limits_{V} {\delta \left( {\varepsilon_{ij} } \right)^{T} \sigma_{ij} {\text{d}}} V - \int\limits_{V} {\delta \left( {E_{i} } \right)^{T} D_{i} {\text{d}}} V + \int\limits_{V} {\left( {1 - \lambda \nabla^{2} } \right)\rho \delta \left( {u_{i} } \right)^{T} \ddot{u}_{i} {\text{d}}} V = \int\limits_{V} {\left( {1 - \lambda \nabla^{2} } \right)\delta \left( {u_{i} } \right)^{T} f_{i} {\text{d}}} V$$
(12)

For the easy representation, Eq. (12) can be expressed by

$$\delta U + \delta K - \delta W = 0$$
(13)

where

$$\begin{aligned} \delta U = & \int_{V} {\delta \left( {\varepsilon_{ij} } \right)^{T} \sigma_{ij} } {\text{d}}V - \int_{V} {\delta \left( {{\rm E}_{i} } \right)^{T} D_{i} } {\text{d}}V \\ \delta K = & \int_{V} {\left( {1 - \lambda \nabla^{2} } \right)\delta \left( {u_{i} } \right)^{T} \rho \ddot{u}_{i} {\text{d}}V} \\ \delta W = & \int_{V} {\left( {1 - \lambda \nabla^{2} } \right)\delta \left( {u_{i} } \right)^{T} f_{i} {\text{d}}V} \\ \end{aligned}$$
(14)

in which δU, δK and δW represent the virtual strain energy, virtual kinetic energy, and virtual external work, respectively.

3.2 Displacement field

Through the generalized higher-order shear deformation theory, the displacement field is defined

$$\begin{gathered} u(x,y,z) = u_{0} (x,y) - z\frac{\partial w(x,y)}{{\partial x}} + f(z)\beta_{x} (x,y) \hfill \\ v(x,y,z) = v_{0} (x,y) - z\frac{\partial w(x,y)}{{\partial y}} + f(z)\beta_{y} (x,y) \hfill \\ w(x,y,z) = w_{0}(x,y) \hfill \\ \end{gathered}$$
(15)

where \(u_{0}\),\(v_{0}\),\(w\),\(\beta_{x}\) and \(\, \beta_{y}\) are variables of displacement; \(f(z) = z - 4z^{3} /3h^{2}\) is a continuous function.

Strain components can be displayed as

$$\begin{gathered} {{\varvec{\upvarepsilon}}}^{b} = \left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } & {\varepsilon_{yy} } & {\gamma_{xy} } \\ \end{array} } \right\}^{{\text{T}}} = {{\varvec{\upvarepsilon}}}_{1} + z{{\varvec{\upvarepsilon}}}_{2} + f(z){{\varvec{\upvarepsilon}}}_{3} \hfill \\ {{\varvec{\upgamma}}} = \left\{ {\begin{array}{*{20}c} {\gamma_{xz} } & {\gamma_{yz} } \\ \end{array} } \right\}^{{\text{T}}} {\mathbf{ = }}f^{\prime}(z){{\varvec{\upvarepsilon}}}_{s} \hfill \\ \end{gathered}$$
(16)

where f’(z) is the derivative of the function f(z) and

$$\begin{aligned} &{\mathbf{\varepsilon }}_{1} = \left[ {\begin{array}{*{20}c} {u_{{0,x}} } \\ {v_{{0,y}} } \\ {u_{{0,y}} + v_{{0,x}} } \\ \end{array} } \right]\,\,;\,\,{\mathbf{\varepsilon }}_{2} = - \left[ {\begin{array}{*{20}c} {w_{{0,xx}} } \\ {w_{{0,yy}} } \\ {2w_{{0,xy}} } \\ \end{array} } \right]\,\,\,\,\, \hfill \\ & {\mathbf{\varepsilon }}_{3} = \left[ {\begin{array}{*{20}c} {\beta _{{x,x}} } \\ {\beta _{{y,y}} } \\ {\beta _{{x,y}} + \beta _{{y,x}} } \\ \end{array} } \right]\,\,;\,\,\,{\mathbf{\varepsilon }}_{s} = \left[ {\begin{array}{*{20}c} {\beta _{x} } \\ {\beta _{y} } \\ \end{array} } \right] \hfill \\ \end{aligned}$$
(17)

The FGPP nanoplate is performed under an initial electric voltage V0 on the top and bottom surfaces. According to the Maxwell's formula, the electric potential is given as

$$\Phi \left( {x,y,z} \right) = g(z)\phi \left( {x,y} \right) + \tfrac{2z}{h}V_{0}$$
(18)

where \(\phi \left( {x,y} \right)\) is the electric potential; \(g(z) = - {\text{cos}}\left( {\tfrac{\pi z}{h}} \right)\). The electric fields are defined as

$$\begin{gathered} E_{x} = - \Phi_{,x} = - g(z)\phi_{,x} \hfill \\ E_{y} = - \Phi_{,y} = - g(z)\phi_{,y} \hfill \\ E_{z} = - \Phi_{,z} = - g^{\prime}(z)\phi - \tfrac{{2V_{0} }}{h} \hfill \\ \end{gathered}$$
(19)

For easy numerical computations, the local stress and local electric displacement in Eq. (12) can be rewritten by an explicit form as

$$\begin{aligned} \underbrace {{\left\{ {\begin{array}{*{20}c} {\sigma_{xx} } \\ {\sigma_{yy} } \\ {\sigma_{xy} } \\ \end{array} } \right\}}}_{{{{\varvec{\upsigma}}}^{b} }} = & \underbrace {{\left[ {\begin{array}{*{20}c} {\tilde{C}_{11} } & {\tilde{C}_{12} } & 0 \\ {\tilde{C}_{12} } & {\tilde{C}_{22} } & 0 \\ 0 & 0 & {\tilde{C}_{66} } \\ \end{array} } \right]}}_{{{\mathbf{C}}^{b} }}\underbrace {{\left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\varepsilon_{yy} } \\ {\varepsilon_{xy} } \\ \end{array} } \right\}}}_{{{{\varvec{\upvarepsilon}}}^{b} }} - \underbrace {{\left[ {\begin{array}{*{20}c} 0 & 0 & {\tilde{e}_{31} } \\ 0 & 0 & {\tilde{e}_{32} } \\ 0 & 0 & 0 \\ \end{array} } \right]}}_{{{\mathbf{C}}_{e}^{b} }}\underbrace {{\left\{ {\begin{array}{*{20}c} 0 \\ 0 \\ {E_{z} } \\ \end{array} } \right\}}}_{{{\mathbf{E}}^{b} }} = {\mathbf{C}}^{b} {{\varvec{\upvarepsilon}}}^{b} - {\mathbf{C}}_{e}^{b} {\mathbf{E}}^{b} \\ \underbrace {{\left\{ {\begin{array}{*{20}c} {\sigma_{xz} } \\ {\sigma_{yz} } \\ \end{array} } \right\}}}_{{{{\varvec{\uptau}}}^{s} }} = & \underbrace {{\left[ {\begin{array}{*{20}c} {\tilde{C}_{55} } & 0 \\ 0 & {\tilde{C}_{44} } \\ \end{array} } \right]}}_{{{\mathbf{C}}^{s} }}\underbrace {{\left\{ {\begin{array}{*{20}c} {\gamma_{xz} } \\ {\gamma_{yz} } \\ \end{array} } \right\}}}_{{{\varvec{\upgamma}}}} - \underbrace {{\left[ {\begin{array}{*{20}c} {\tilde{e}_{15} } & 0 \\ 0 & {\tilde{e}_{24} } \\ \end{array} } \right]}}_{{{\mathbf{C}}_{e}^{s} }}\underbrace {{\left\{ {\begin{array}{*{20}c} {E_{x} } \\ {E_{y} } \\ \end{array} } \right\}}}_{{{\mathbf{E}}^{s} }} = {\mathbf{C}}^{s} {\mathbf{\gamma - C}}_{c}^{s} {\mathbf{E}}^{s} \\ \underbrace {{\left\{ {\begin{array}{*{20}c} {D_{x} } \\ {D_{y} } \\ \end{array} } \right\}}}_{{{\mathbf{D}}^{p} }} = & \left[ {\begin{array}{*{20}c} {\tilde{e}_{15} } & 0 \\ 0 & {\tilde{e}_{24} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\gamma_{xz} } \\ {\gamma_{yz} } \\ \end{array} } \right\} + \underbrace {{\left[ {\begin{array}{*{20}c} {\tilde{k}_{11} } & 0 \\ 0 & {\tilde{k}_{22} } \\ \end{array} } \right]}}_{{{\mathbf{C}}_{k}^{s} }}\left\{ {\begin{array}{*{20}c} {E_{x} } \\ {E_{y} } \\ \end{array} } \right\} = {\mathbf{C}}_{e}^{s} {{\varvec{\upgamma}}} + {\mathbf{C}}_{k}^{s} {\mathbf{E}}^{s} \\ D_{z} = & \tilde{e}_{31} \varepsilon_{x} + \tilde{e}_{32} \varepsilon_{y} + \tilde{k}_{33} E_{z} \\ \end{aligned}$$
(20)

where

$$\begin{gathered} \tilde{C}_{{11}}^{{}} = {\mkern 1mu} C_{{11}}^{{}} - \frac{{C_{{13}}^{2} }}{{C_{{33}}^{{}} }};\,\tilde{C}_{{12}}^{{}} = C_{{12}}^{{}} - \frac{{C_{{13}}^{2} }}{{C_{{33}}^{{}} }};\,\,{\text{ }}\tilde{C}_{{66}}^{{}} = C_{{66}}^{{}} \hfill \\ \tilde{C}_{{55}}^{{}} = C_{{55}}^{{}} \,\,;\,\,\,\tilde{C}_{{44}}^{{}} = C_{{44}}^{{}} \hfill \\ \tilde{e}_{{31}} = e_{{31}} - \frac{{e_{{13}} e_{{33}} }}{{C_{{33}} }};\,\,\tilde{e}_{{15}} = e_{{15}} ;\,\,\,\tilde{k}_{{11}} = k_{{11}} \hfill \\ \tilde{k}_{{33}} = k_{{33}} + \frac{{e_{{33}}^{2} }}{{C_{{33}} }}\, \hfill \\ \varepsilon _{x} = \varepsilon _{{1x}} + z\varepsilon _{{2x}} + f(z)\varepsilon _{{3x}} \hfill \\ \varepsilon _{y} = \varepsilon _{{1y}} + z\varepsilon _{{2y}} + f(z)\varepsilon _{{3y}} \hfill \\ \end{gathered}$$
(21)

in which \(C_{11} ,\,\,C_{12} ,\,\,C_{33} ,\,\,C_{66} ,\,\,e_{31} ,\,\,e_{33} ,\,\,k_{31} ,\,\,k_{33}\) are material constants defined in Eq. (1) or Eq. (2) and

$$\begin{gathered} \,\varepsilon_{1x} = u_{0,x} \,\,\,;\,\,\,\varepsilon_{2x} = - w_{,xx} \,\,\,;\,\,\,\varepsilon_{3x} = \beta_{x,x} \hfill \\ \varepsilon_{1y} = v_{0,y} \,\,\,;\,\,\,\varepsilon_{2y} = - w_{,yy} \,\,\,;\,\,\,\varepsilon_{3y} = \beta_{y,y} \hfill \\ \end{gathered}$$
(22)

The virtual strain energy is formulated by inserting Eqs. (16) and (20) into Eq. (14)

$$\begin{aligned} \delta U = & \int_{\Omega } {\left( {\left( {\delta {\mathbf{\bar{\varepsilon }}}^{b} } \right)^{T} {\mathbf{\bar{C}}}^{b} {\mathbf{\bar{\varepsilon }}}^{b} - \left( {\delta {\mathbf{\bar{\varepsilon }}}^{b} } \right)^{T} {\mathbf{\bar{C}}}_{e}^{b} {\mathbf{\bar{E}}}^{b} } \right){\text{d}}\Omega } \\ & + \int_{\Omega } {\left( {\left( {\delta {\mathbf{\varepsilon }}_{s} } \right)^{T} {\mathbf{\bar{C}}}^{s} {\mathbf{\varepsilon }}_{s} - \left( {\delta {\mathbf{\varepsilon }}_{s} } \right)^{T} {\mathbf{\bar{C}}}_{e}^{s} {\mathbf{\bar{E}}}^{s} } \right){\text{d}}\Omega } \\ & - \int_{\Omega } {\left( {\left( {\delta {\mathbf{\bar{E}}}^{s} } \right)^{T} {\mathbf{\bar{C}}}_{e}^{s} {\mathbf{\varepsilon }}_{s} + \left( {\delta {\mathbf{\bar{E}}}^{s} } \right)^{T} {\mathbf{\bar{C}}}_{k}^{s} {\mathbf{\bar{E}}}^{s} } \right){\text{d}}\Omega } \\ & - \int\limits_{\Omega } {\left( {\delta \bar{E}_{z} } \right)^{T} \left( {{\mathbf{\bar{C}}}_{{e31}}^{{}} {\mathbf{\bar{\varepsilon }}}_{x} + {\mathbf{\bar{C}}}_{{e32}}^{{}} {\mathbf{\bar{\varepsilon }}}_{y} + \bar{C}_{{k33}}^{{}} \bar{E}_{z} } \right)} {\text{d}}\Omega \\ \end{aligned}$$
(23)

where

$$\begin{gathered} {\mathbf{\bar{\varepsilon }}}^{b} = \left[ {\begin{array}{*{20}c} {{\mathbf{\varepsilon }}_{1} } \\ {{\mathbf{\varepsilon }}_{2} } \\ {{\mathbf{\varepsilon }}_{3} } \\ \end{array} } \right];\,\,{\mathbf{\bar{\varepsilon }}}_{x} = \left[ {\begin{array}{*{20}c} {\varepsilon _{{1x}} } \\ {\varepsilon _{{2x}} } \\ {\varepsilon _{{3x}} } \\ \end{array} } \right];\,\,\,{\mathbf{\bar{\varepsilon }}}_{y} = \left[ {\begin{array}{*{20}c} {\varepsilon _{{1y}} } \\ {\varepsilon _{{2y}} } \\ {\varepsilon _{{3y}} } \\ \end{array} } \right]; \hfill \\ {\mathbf{\bar{C}}}^{b} = \left[ {\begin{array}{*{20}c} {{\mathbf{A}}^{b} } & {{\mathbf{B}}^{b} } & {{\mathbf{E}}^{b} } \\ {{\mathbf{B}}^{b} } & {{\mathbf{D}}^{b} } & {{\mathbf{F}}^{b} } \\ {{\mathbf{E}}^{b} } & {{\mathbf{F}}^{b} } & {{\mathbf{H}}^{b} } \\ \end{array} } \right];\,{\mathbf{\bar{E}}}^{b} = - \left[ {\begin{array}{*{20}c} 0 \\ 0 \\ \phi \\ \end{array} } \right]\,\,\,;\,{\mathbf{\bar{E}}}^{s} = - \left[ {\begin{array}{*{20}c} {\phi _{{,x}} } \\ {\phi _{{,y}} } \\ \end{array} } \right]\,\,; \hfill \\ \,\left( {{\mathbf{A}}^{b} ,{\mathbf{B}}^{b} ,{\mathbf{D}}^{b} ,{\mathbf{E}}^{b} ,{\mathbf{F}}^{b} ,{\mathbf{H}}^{b} } \right) \hfill \\ = \,\,\int_{{ - h/2}}^{{h/2}} {\left( {1,z,z^{2} ,f(z),zf(z),f^{2} (z)} \right)} {\mathbf{C}}^{b} {\text{d}}z \hfill \\ {\mathbf{\bar{C}}}_{e}^{b} = \left[ {\begin{array}{*{20}c} {{\mathbf{\bar{C}}}_{e}^{{b1}} } & {{\mathbf{\bar{C}}}_{e}^{{b2}} } & {{\mathbf{\bar{C}}}_{e}^{{b3}} } \\ \end{array} } \right];\,\, \hfill \\ \left( {{\mathbf{\bar{C}}}_{e}^{{b1}} ,{\mathbf{\bar{C}}}_{e}^{{b2}} ,{\mathbf{\bar{C}}}_{e}^{{b3}} } \right) = \,\int_{{ - h/2}}^{{h/2}} {\left( {g^{\prime}(z),zg^{\prime}(z),f(z)g^{\prime}(z)} \right)} {\mathbf{C}}_{e}^{b} {\text{d}}z; \hfill \\ {\mathbf{\bar{C}}}^{s} = \int_{{ - h/2}}^{{h/2}} {\left( {f^{\prime}(z)} \right)^{2} } {\mathbf{C}}^{s} {\text{d}}z;\,\,\,\, \hfill \\ {\mathbf{\bar{C}}}_{e}^{s} = \int_{{ - h/2}}^{{h/2}} {g(z)\left( {f^{\prime}(z)} \right)^{2} } {\mathbf{C}}_{e}^{s} {\text{d}}z;\,\,\,\,{\mathbf{\bar{C}}}_{k}^{s} = \int_{{ - h/2}}^{{h/2}} {g\left( z \right){\mathbf{C}}_{k}^{s} } {\text{d}}z; \hfill \\ {\mathbf{\bar{C}}}_{{e31}}^{{}} = \left[ {\begin{array}{*{20}c} {{\mathbf{\bar{C}}}_{{e31}}^{1} } & {{\mathbf{\bar{C}}}_{{e31}}^{2} } & {{\mathbf{\bar{C}}}_{{e31}}^{3} } \\ \end{array} } \right]\,;\,\, \hfill \\ \left( {{\mathbf{\bar{C}}}_{{e31}}^{1} ,{\mathbf{\bar{C}}}_{{e31}}^{2} ,{\mathbf{\bar{C}}}_{{e31}}^{3} } \right) = \,\int_{{ - h/2}}^{{h/2}} {\left( {g^{\prime}(z),zg^{\prime}(z),f(z)g^{\prime}(z)} \right)} \tilde{e}_{{31}} {\text{d}}z; \hfill \\ {\mathbf{\bar{C}}}_{{e32}}^{{}} = \left[ {\begin{array}{*{20}c} {{\mathbf{\bar{C}}}_{{e32}}^{1} } & {{\mathbf{\bar{C}}}_{{e32}}^{2} } & {{\mathbf{\bar{C}}}_{{e32}}^{3} } \\ \end{array} } \right]\,;\,\, \hfill \\ \left( {{\mathbf{\bar{C}}}_{{e32}}^{1} ,{\mathbf{\bar{C}}}_{{e32}}^{2} ,{\mathbf{\bar{C}}}_{{e32}}^{3} } \right) = \,\int_{{ - h/2}}^{{h/2}} {\left( {g^{\prime}(z),zg^{\prime}(z),f(z)g^{\prime}(z)} \right)} \tilde{e}_{{32}} {\text{d}}z; \hfill \\ \bar{C}_{{k33}}^{{}} = \,\int_{{ - h/2}}^{{h/2}} {\left( {g^{\prime}(z)} \right)^{2} \tilde{k}_{{33}} {\text{d}}z} \hfill \\ \end{gathered}$$
(24)

Similarly, the virtual kinetic energy is described as

$$\delta K = \int_{\Omega } {\left( {1 - \lambda \nabla^{2} } \right)\left( {\delta {\mathbf{u}}} \right)^{T} {\mathbf{I}}_{m} {\mathbf{\ddot{u}}}{\text{d}}\Omega } \,$$
(25)

where

$$\begin{gathered} {\mathbf{u}} = \left\{ {\begin{array}{*{20}c} {{\mathbf{u}}^{1} } \\ {{\mathbf{u}}^{2} } \\ {{\mathbf{u}}^{3} } \\ \end{array} } \right\}\,\,;\,\,{\mathbf{u}}^{1} = \left\{ {\begin{array}{*{20}c} {u_{0} } \\ {v_{0} } \\ w \\ \end{array} } \right\};\,{\text{ }}\,{\mathbf{u}}^{2} = - \left\{ {\begin{array}{*{20}c} {w_{{0,x}} } \\ {w_{{0,y}} } \\ 0 \\ \end{array} } \right\}{\text{ }} \hfill \\ {\text{ }}{\mathbf{u}}^{3} = \left\{ {\begin{array}{*{20}c} {\beta _{x} } \\ {\beta _{y} } \\ 0 \\ \end{array} } \right\}\,\,\,;\,\,\,{\mathbf{I}}_{m} = \left[ {\begin{array}{*{20}c} {{\mathbf{I}}_{1} } & {{\mathbf{I}}_{2} } & {{\mathbf{I}}_{4} } \\ {{\mathbf{I}}_{2} } & {{\mathbf{I}}_{3} } & {{\mathbf{I}}_{5} } \\ {{\mathbf{I}}_{4} } & {{\mathbf{I}}_{5} } & {{\mathbf{I}}_{6} } \\ \end{array} } \right] \hfill \\ \end{gathered}$$
(26)

in which

$$\left( {{\mathbf{I}}_{1} ,{\mathbf{I}}_{2} ,{\mathbf{I}}_{3} ,{\mathbf{I}}_{4} ,{\mathbf{I}}_{5} ,{\mathbf{I}}_{6} } \right) = \int\limits_{ - h/2}^{h/2} {\rho (z)\left( {1,z,z^{{2}} ,f(z),zf(z),f^{2} (z)} \right)} \left[ {\begin{array}{*{20}c} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \\ \end{array} } \right]{\text{d}}z$$
(27)

The virtual external work subjected to an external transverse load (f) can be expressed as follows

$$\delta W = \int_{\Omega } {\left( {1 - \lambda \nabla^{2} } \right)\delta w^{T} f{\text{d}}\Omega }$$
(28)

In this research, the FGPP nanoplate is additionally considered the pre-buckling in-plane load by the electrical field, the variation of potential energy is expressed as

$$\begin{aligned} \delta V = & - h\int_{\Omega } {\left( {1 - \lambda \nabla^{2} } \right)\delta \left\{ {\begin{array}{*{20}c} {w_{0,x} } \\ {w_{0,y} } \\ \end{array} } \right\}^{{\text{T}}} \left[ {\begin{array}{*{20}c} {N_{x}^{0} } & 0 \\ 0 & {N_{y}^{0} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {w_{0,x} } \\ {w_{0,y} } \\ \end{array} } \right\}{\text{d}}\Omega } \;{\text{or}} \\ \delta V = & - h\int_{\Omega } {\left( {1 - \lambda \nabla^{2} } \right)\delta \left( {{\mathbf{B}}^{g} } \right)^{{\text{T}}} \left[ {\begin{array}{*{20}c} {N_{x}^{0} } & 0 \\ 0 & {N_{y}^{0} } \\ \end{array} } \right]{\mathbf{B}}^{g} {\text{d}}\Omega } \\ \end{aligned}$$
(29)

where \(N_{x}^{0} = N_{x}^{mech} + N_{x}^{elec}\) and \(N_{y}^{0} = N_{y}^{mech} + N_{y}^{elec}\); in which \(N_{x}^{mech}\) and \(N_{y}^{mech}\) are in-plane mechanical loads, whereas electrical components are \(N_{x}^{elec} = N_{y}^{elec} = 2e_{31}^{p} V_{0}\).

Finally, Eq. (13) can be rewritten by

$$\delta U + \delta K - \delta W - \delta V = 0$$
(30)

3.3 NURBS formulation for the FGPP nanoplate

The displacement field of the FGPP nanoplate is defined as

$${\mathbf{u}}^{h} = \sum\limits_{I = 1}^{m \times n} {\left[ {\begin{array}{*{20}c} {R_{I} } & 0 & 0 & 0 & 0 \\ 0 & {R_{I} } & 0 & 0 & 0 \\ 0 & 0 & {R_{I} } & 0 & 0 \\ 0 & 0 & 0 & {R_{I} } & 0 \\ 0 & 0 & 0 & 0 & {R_{I} } \\ \end{array} } \right]} \left\{ {\begin{array}{*{20}c} {u_{0I} } \\ {v_{0I} } \\ {w_{0I} } \\ {\beta_{xI} } \\ {\beta_{yI} } \\ \end{array} } \right\}{\mathbf{ = }}\sum\limits_{I = 1}^{m \times n} {{\mathbf{R}}_{I} } {\mathbf{d}}_{I}$$
(31)

where m × n is the number of basis functions,\(R_{I}\) is a matrix of NURBS basis function (Hughes et al. 2005), \({\mathbf{d}}_{I} = \left\{ {\begin{array}{*{20}c} {u_{0I} } & {v_{0I} } & {w_{0I} } & {\beta_{xI} } & {\beta_{yI} } \\ \end{array} } \right\}{\kern 1pt} {\kern 1pt}^{T}\) are degrees of freedom of the control point I.

Substituting Eq. (31) into Eq. (17) to form of Eq. (32):

$${\overline{\mathbf{\varepsilon }}}^{b} = \left\{ {\begin{array}{*{20}c} {{{\varvec{\upvarepsilon}}}_{1} } & {{{\varvec{\upvarepsilon}}}_{2} } & {{{\varvec{\upvarepsilon}}}_{3} } \\ \end{array} } \right\}^{T} = \sum\limits_{I = 1}^{{m{\text{x}}n}} {\left\{ {\begin{array}{*{20}c} {{\mathbf{B}}_{I}^{1} } & {{\mathbf{B}}_{I}^{2} } & {{\mathbf{B}}_{I}^{3} } \\ \end{array} } \right\}^{T} {\mathbf{d}}_{I} } = \sum\limits_{I = 1}^{{m{\text{x}}n}} {{\mathbf{B}}_{I}^{b} {\mathbf{d}}_{I} } ;\;{\overline{\mathbf{\varepsilon }}}_{s} = \sum\limits_{I = 1}^{{m{\text{x}}n}} {{\mathbf{B}}_{I}^{s} {\mathbf{d}}_{I} }$$
(32)

where

$$\begin{gathered} {\mathbf{B}}_{I}^{1} = \left[ {\begin{array}{*{20}c} {R_{I,x} } & 0 & 0 & 0 & 0 \\ 0 & {R_{I,y} } & 0 & 0 & 0 \\ {R_{I,y} } & {R_{I,x} } & 0 & 0 & 0 \\ \end{array} } \right]; {\mathbf{B}}_{I}^{2} = - \left[ {\begin{array}{*{20}c} 0 & 0 & {R_{I,xx} } & 0 & 0 \\ 0 & 0 & {R_{I,yy} } & 0 & 0 \\ 0 & 0 & {2R_{I,xy} } & 0 & 0 \\ \end{array} } \right] \hfill \\ {\mathbf{B}}_{I}^{3} = \left[ {\begin{array}{*{20}c} 0 & 0 & 0 & {R_{I,x} } & 0 \\ 0 & 0 & 0 & 0 & {R_{I,y} } \\ 0 & 0 & 0 & {R_{I,y} } & {R_{I,x} } \\ \end{array} } \right];{\mathbf{B}}_{I}^{s} = \left[ {\begin{array}{*{20}c} 0 & 0 & 0 & {R_{I} } & 0 \\ 0 & 0 & 0 & 0 & {R_{I} } \\ \end{array} } \right] \hfill \\ \end{gathered}$$
(33)

Substituting Eq. (31) into Eq. (26) to form of Eq. (34):

$${\mathbf{u}} = \left\{ {\begin{array}{*{20}c} {{\mathbf{u}}^{1} } & {{\mathbf{u}}^{2} } & {{\mathbf{u}}^{3} } \\ \end{array} } \right\}^{T} = \sum\limits_{I = 1}^{{m{\text{x}}n}} {\left\{ {\begin{array}{*{20}c} {{\mathbf{N}}_{I}^{1} } & {{\mathbf{N}}_{I}^{2} } & {{\mathbf{N}}_{I}^{3} } \\ \end{array} } \right\}^{T} {\mathbf{q}}_{I} } = \sum\limits_{I = 1}^{{m{\text{x}}n}} {{\overline{\mathbf{N}}}_{I}^{{}} {\mathbf{q}}_{I} }$$
(34)

where

$${\mathbf{N}}_{I}^{1} = \left[ {\begin{array}{*{20}c} {R_{I} } & 0 & 0 & 0 & 0 \\ 0 & {R_{I} } & 0 & 0 & 0 \\ 0 & 0 & {R_{I} } & 0 & 0 \\ \end{array} } \right];{\mathbf{N}}_{I}^{2} = - \left[ {\begin{array}{*{20}c} 0 & 0 & {R_{I,x} } & 0 & 0 \\ 0 & 0 & {R_{I,y} } & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 \\ \end{array} } \right]{\mathbf{;N}}_{I}^{3} = \left[ {\begin{array}{*{20}c} 0 & 0 & 0 & {R_{I} } & 0 \\ 0 & 0 & 0 & 0 & {R_{I} } \\ 0 & 0 & 0 & 0 & 0 \\ \end{array} } \right]$$
(35)

Substituting Eq. (31) into Eq. (29),\({\mathbf{B}}^{g}\) can be expressed as

$${\mathbf{B}}^{g} = \left[ {\begin{array}{*{20}c} 0 & 0 & {R_{I,x} } & 0 & 0 \\ 0 & 0 & {R_{I,y} } & 0 & 0 \\ \end{array} } \right]$$
(36)

The electric potential can also be approximated using a NURBS basis function as follows

$$\phi = \sum\limits_{I = 1}^{m \times n} {R_{I} } \phi_{I}$$
(37)

where \(\phi_{I}\) is the degree of freedom of the electric potential at the control point I.

Substituting Eqs. (31), (37) into Eqs. (22), (24), we have

$$\begin{gathered} {\mathbf{\bar{E}}}^{b} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{\phi I}}^{b} } \phi _{I} \,;\,\,\,{\mathbf{\bar{E}}}^{s} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{\phi I}}^{s} } \phi _{I} ;\,\,\,\bar{E}_{z} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{zI}} } \phi _{I} \hfill \\ \;\,{\mathbf{\bar{\varepsilon }}}_{x} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{xI}}^{{}} } {\mathbf{d}}_{I} ;\,\,\,\varepsilon _{{1x}} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{xI}}^{1} } {\mathbf{d}}_{I} ;\;\varepsilon _{{3x}} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{xI}}^{3} } {\mathbf{d}}_{I} \hfill \\ \,\varepsilon _{{2x}} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{xI}}^{2} } {\mathbf{d}}_{I} ;\;\,{\mathbf{\bar{\varepsilon }}}_{y} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{yI}}^{{}} } {\mathbf{d}}_{I} ;\;\varepsilon _{{1y}} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{yI}}^{1} } {\mathbf{d}}_{I} \hfill \\ \varepsilon _{{2y}} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{yI}}^{2} } {\mathbf{d}}_{I} ;\varepsilon _{{3y}} = \sum\limits_{{I = 1}}^{{m \times n}} {{\mathbf{B}}_{{yI}}^{3} } {\mathbf{d}}_{I} \hfill \\ \end{gathered}$$
(38)

where

$$\begin{gathered} {\mathbf{B}}_{{\phi I}}^{b} = \,\, - \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} 0 \\ 0 \\ {R_{I} } \\ \end{array} } \right]} \,;\,\,\,{\mathbf{B}}_{{\phi I}}^{s} = \,\, - \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} {R_{{I,x}} } \\ {R_{{I,y}} } \\ \end{array} } \right]} \,\, \hfill \\ {\mathbf{B}}_{{zI}} = \,\, - \sum\limits_{{I = 1}}^{{m \times n}} {R_{I} } \,;\,\,\,{\mathbf{B}}_{{xI}}^{{}} = \left[ {\begin{array}{*{20}c} {{\mathbf{B}}_{{xI}}^{1} } \\ {{\mathbf{B}}_{{xI}}^{2} } \\ {{\mathbf{B}}_{{xI}}^{3} } \\ \end{array} } \right]\,;\,\,\,\,\,{\mathbf{B}}_{{yI}}^{{}} = \left[ {\begin{array}{*{20}c} {{\mathbf{B}}_{{yI}}^{1} } \\ {{\mathbf{B}}_{{yI}}^{2} } \\ {{\mathbf{B}}_{{yI}}^{3} } \\ \end{array} } \right] \hfill \\ {\mathbf{B}}_{{xI}}^{1} = \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} {R_{{I,x}} } & 0 & 0 & 0 & 0 \\ \end{array} } \right]} \,\, \hfill \\ {\mathbf{B}}_{{xI}}^{2} = - \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} 0 & 0 & {R_{{I,xx}} } & 0 & 0 \\ \end{array} } \right]} ;\,{\mathbf{B}}_{{xI}}^{3} = \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} 0 & 0 & 0 & {R_{{I,x}} } & 0 \\ \end{array} } \right]} \, \hfill \\ {\mathbf{B}}_{{yI}}^{1} = \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} 0 & {R_{{I,y}} } & 0 & 0 & 0 \\ \end{array} } \right]} \hfill \\ {\mathbf{B}}_{{yI}}^{2} = - \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} 0 & 0 & {R_{{I,yy}} } & 0 & 0 \\ \end{array} } \right]} ;\;{\mathbf{B}}_{{yI}}^{3} = \sum\limits_{{I = 1}}^{{m \times n}} {\left[ {\begin{array}{*{20}c} 0 & 0 & 0 & 0 & {R_{{I,y}} } \\ \end{array} } \right]} \, \hfill \\ \end{gathered}$$
(39)

The weak form of the FGPP nanoplates can be taken by substituting Eqs. (32)-(36), (38)-(39) into Eq. (30)

$$\left[ {\begin{array}{*{20}c} {\mathbf{M}} & {0} \\ {\mathbf{0}} & 0 \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {{\mathbf{\ddot{d}}}} \\ {\ddot{\phi }} \\ \end{array} } \right] + \left[ {\begin{array}{*{20}c} {{\mathbf{K}}_{uu} } & {{\mathbf{K}}_{u\phi } } \\ {{\mathbf{K}}_{\phi u} } & {{\mathbf{K}}_{\phi \phi } } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\mathbf{d}} \\ \phi \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {\mathbf{f}} \\ {\mathbf{0}} \\ \end{array} } \right]$$
(40)

where

$$\begin{gathered} {\mathbf{K}}_{uu} = {\mathbf{K}}_{1} {\mathbf{ + K}}_{3} - {\mathbf{K}}_{w} \,;\,\,\,\,\,\,{\mathbf{K}}_{u\phi } = - \left( {{\mathbf{K}}_{5} + {\mathbf{K}}_{71} + {\mathbf{K}}_{72} } \right) \hfill \\ {\mathbf{K}}_{\phi u} = - \left( {{\mathbf{K}}_{2} + {\mathbf{K}}_{4} } \right) \, ; \, {\mathbf{K}}_{\phi \phi } = - \left( {{\mathbf{K}}_{6} + {\mathbf{K}}{}_{73}} \right) \hfill \\ {\mathbf{M}} = \int_{\Omega } {\left( {1 - \lambda \nabla^{2} } \right){\overline{\mathbf{N}}}_{{}}^{T} {\mathbf{I}}_{m} {\overline{\mathbf{N}}}{\text{d}}\Omega } \,\,;\,\,\,{\mathbf{f}} = \int_{\Omega } {\left( {{\mathbf{B}}^{w} - \lambda \nabla^{2} {\mathbf{B}}^{w} } \right)f{\text{d}}\Omega } \,\,\,\,\,\,\,\,\,\, \hfill \\ \end{gathered}$$
(41)

in which

$$\begin{gathered} {\mathbf{K}}_{1} = \int_{\Omega } {\left( {{\mathbf{B}}^{b} } \right)^{T} {\overline{\mathbf{C}}}^{b} {\mathbf{B}}^{b} {\text{d}}\Omega } \,\,;\,\,{\mathbf{K}}_{2} = \int_{\Omega } {\left( {{\mathbf{B}}_{\phi }^{b} } \right)^{T} {\overline{\mathbf{C}}}_{e}^{b} {\mathbf{B}}^{b} {\text{d}}\Omega } \,\,;\,\,\,\,{\mathbf{K}}_{3} = \int_{\Omega } {\left( {{\mathbf{B}}^{s} } \right)^{T} {\overline{\mathbf{C}}}^{s} {\mathbf{B}}^{s} {\text{d}}\Omega } \,\,\,\, \hfill \\ {\mathbf{K}}_{4} = \int_{\Omega } {\left( {{\mathbf{B}}_{\phi }^{s} } \right)^{T} {\overline{\mathbf{C}}}_{e}^{s} {\mathbf{B}}^{s} {\text{d}}\Omega } \,\,;\,\,{\mathbf{K}}_{5} = \int_{\Omega } {\left( {{\mathbf{B}}^{s} } \right)^{T} {\overline{\mathbf{C}}}_{e}^{s} {\mathbf{B}}_{\phi }^{s} {\text{d}}\Omega } \,\,;\,\,{\mathbf{K}}_{6} = \int_{\Omega } {\left( {{\mathbf{B}}_{\phi }^{s} } \right)^{T} {\overline{\mathbf{C}}}_{k}^{s} {\mathbf{B}}_{\phi }^{s} {\text{d}}\Omega } \,\,\, \hfill \\ {\mathbf{K}}_{71} = \int_{\Omega } {\left( {{\mathbf{B}}_{x} } \right)^{T} {\overline{\mathbf{C}}}_{e31}^{{}} {\mathbf{B}}_{z} {\text{d}}\Omega } \,\,;\,\,\,{\mathbf{K}}_{72} = \int_{\Omega } {\left( {{\mathbf{B}}_{y} } \right)^{T} {\overline{\mathbf{C}}}_{e32}^{{}} {\mathbf{B}}_{z} {\text{d}}\Omega } \,\,;\,\,\,{\mathbf{K}}_{73} = \int_{\Omega } {\left( {{\mathbf{B}}_{z} } \right)^{T} \overline{C}_{k33}^{{}} {\mathbf{B}}_{z} {\text{d}}\Omega } \hfill \\ {\mathbf{K}}_{w} = \int_{\Omega } {\left( {1 - \lambda \nabla^{2} } \right)\left( {{\mathbf{B}}^{g} } \right)^{T} \left[ {\begin{array}{*{20}c} {N_{x}^{0} } & 0 \\ 0 & {N_{y}^{0} } \\ \end{array} } \right]{\mathbf{B}}^{g} {\text{d}}\Omega } ; \, {\mathbf{B}}^{w} = \left[ {\begin{array}{*{20}c} 0 & 0 & {R_{I} } & 0 & 0 \\ \end{array} } \right]^{T} \hfill \\ \end{gathered}$$
(42)

Substituting the second line of Eq. (40) into the first line, we have a shortened form as follows

$${\mathbf{M\ddot{d}}} + \left( {{\mathbf{K}}_{uu} - {\mathbf{K}}_{u\phi } {\mathbf{K}}_{{_{\phi \phi } }}^{ - 1} {\mathbf{K}}_{\phi u} } \right){\mathbf{d}} = {\mathbf{f}}$$
(43)

The principal equation of the free vibration problem is provided as follows

$$\left( {{\mathbf{K}} - \omega^{2} {\mathbf{M}}} \right){\mathbf{d}} = {\mathbf{0}}$$
(44)

In which the global stiffness matrix \({\mathbf{K}}\) is taken as

$${\mathbf{K}} = {\mathbf{K}}_{uu} - {\mathbf{K}}_{u\phi } {\mathbf{K}}_{{_{\phi \phi } }}^{ - 1} {\mathbf{K}}_{\phi u}$$
(45)

4 Numerical examples

This section verifies the current findings and introduces new results. Table 1 outlines the material properties of piezoelectric. A mesh composed of 11 × 11 cubic Non-Uniform Rational B-Splines elements is utilized. The boundary conditions for each edge can either be clamped (C), free (F), or simply supported (S).

Table 1 Material properties of piezoelectric
Full size table

4.1 Square nanoplates

We now consider a square nanoplate with a length of a and thickness of h. The non-dimensional natural frequency \(\tilde{\omega } = \omega a^{2} /h\sqrt {\left( {\rho /c_{11} } \right)}_{PZT - 4}\) is being used for analysis purposes. Table 2 shows the first non-dimensional frequency of the entirely simply supported (SSSS) FGPP nanoplate for different values of input voltage and power law index. The results obtained in this research are consistent with those presented in Ref. (Barati et al. 2017) which used an analytical solution and a refined four-variable plate theory. However, the values obtained in our study are slightly larger than the reference values due to the use of different theories.

Table 2 The lowest non-dimensional natural frequency of the SSSS FGPP-II nanoplate with \(\alpha = 0.2\), \(\lambda = 0\), a/h = 100
Full size table

The following section investigates the effect of the nonlocal parameter on the first frequency of a fully clamped (CCCC) FGPP nanoplate, as shown in Table 3. The results indicate that the natural frequency of the plate decreases as the nonlocal parameter increases. Additionally, an increase in the power law exponent leads to a decrease in the natural frequency. It is worth noting that the natural frequency of the FGPP-I nanoplate is smaller than that of the FGPP-II nanoplate. Table 4 presents the impact of the external electric voltage on the first natural frequency of a SSSS FGPP-II nanoplate. The results show that an increase in the external electric voltage results in a decrease in the frequency of the nanoplate.

Table 3 The effect of the nonlocal parameter on the first natural frequency of a CCCC FGPP nanoplate with \(V_{0}\) = 0, a/h = 100
Full size table
Table 4 The effect of external electric voltage on the first natural frequency of a SSSS FGPP-II nanoplate with a/h = 20, n = 5
Full size table

In addition, Table 5 lists the first six natural frequencies of a SSSS FGPP-I nanoplate. The results show that the natural frequencies decrease as the power law exponent increases and increase as the porous volume fraction increases. The effect of different boundary conditions on the natural frequency of the nanoplate is presented in Table 6. As demonstrated in the table, the highest and lowest frequencies are obtained for the CCCC and SSSS nanoplates, respectively. Figure 2 illustrates the first six mode shapes of the SCFS FGPP-I square nanoplate.

Table 5 The first six natural frequencies of a SSSS FGPP-I nanoplate with a/h = 100, λ = 2, V0 = 0
Full size table
Table 6 The first natural frequency of a FGPP nanoplate with \(\alpha = 0.3\), n = 3, a/h = 50
Full size table
Fig. 2
figure 2

The first six mode shapes of the SCFS FGPP-I nanoplate with \(\alpha = 0.3\), \(\lambda = 3\), a/h = 50, n = 3, V0 = 300

Full size image

4.2 Circular nanoplates

A FGPP circular nanoplate with radius R and thickness h is considered in this section. The non-dimensional natural frequency \(\tilde{\omega } = 4\omega R^{2} /h\sqrt {\left( {\rho /c_{11} } \right)}_{PZT - 4}\) is used. The effect of the nonlocal parameter and external electric voltage on the first natural frequency of the simply supported circular FGPP nanoplate is shown in Table 7. The finding is that the frequency decreases with increasing of the nonlocal parameter and external electric voltage, respectively.

Table 7 The first natural frequency of a simply supported circular FGPP nanoplate with \(\alpha = 0.3\), R/h = 20
Full size table

Next, the influence of different boundary conditions on the first natural frequency of a circular FGPP nanoplate is shown in Table 8. Again, we can see that the natural frequency of the FGPP-I nanoplate is smaller than that of the FGPP-II nanoplate. And, the natural frequency of the nanoplates decreases with a rise of the nonlocal parameter and external electric voltage. Finally, the lowest six mode shapes of a fully clamped circular FGPP nanoplate are also plotted in Fig. 3.

Table 8 The effect of different boundary conditions on the first natural frequency of a circular FGPP nanoplate with \(\alpha = 0.1\), R/h = 20, n = 0.5
Full size table
Fig. 3
figure 3

The first six mode shapes of the fully clamped circular FGPP-I nanoplate with \(\alpha = 0.1\), \(\lambda = 4\), R/h = 20, n = 0.5, \(V_{0}\) = 500

Full size image

5 Conclusions

This paper presented the size-dependent analysis of the functionally graded piezoelectric porous nanoplates using higher-order shear deformation theory, the nonlocal elasticity and isogeometric analysis. Material properties of the nanoplate made of two piezoelectric materials including PZT-4 and PZT-5H are performed by using the modified power-law function. Two porous distributions through the thickness direction are employed. The Eringen’s nonlocal elasticity is used to simulate the size-dependent effects. Based on the study results and the established formulations, some remarkable conclusions can be listed as follows:

  • Isogeometric analysis of porous-dependent FG piezoelectric porous nanoplates is extended and developed in this study.

  • The combination of the Eringen’s nonlocal elasticity theory and IGA is a simply, suitable, effective and robust method to calculate and simulate behaviors of the FG piezoelectric porous nanoplates.

  • The frequency of the nanoplates decreases with increasing of the nonlocal parameter and external electric voltage.

  • The frequency of the FGPP-I nanoplate is smaller than that of the FGPP-II nanoplate.

  • The frequency decreases and increases with increasing of the law exponent index and porous volume fraction factor, respectively.

Finally, these findings shed light on the behavior of smart functionally graded piezoelectric porous nanoplates and contribute to a more comprehensive understanding of the implications for future research in this field.