Characterizing monotone games

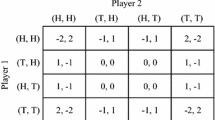
Abstract

Solution concepts in games of strategic heterogeneity (GSH), which include games of strategic complements as a special case, have been shown to possess very useful properties, such as the existence of highest and lowest serially undominated strategies, and the equivalence of the stability of equilibria and dominance solvability. The main result of this paper gives necessary and sufficient conditions for when a very general class of games, referred to as games of mixed heterogeneity, can be transformed into GSH in such a way so that these properties are preserved, allowing us to draw the same strong conclusions about solution sets in games that are not originally GSH. This is achieved by reversing the orders on the actions spaces of a given subset of players. Our second main result shows, rather surprisingly, that under mild conditions on the underlying ordering of action spaces, the reversal of orders is the only way in which such a transformation can be achieved. Applications to aggregate games, market games, and crime networks are given.

1 Introduction

Solution sets in games in which players’ payoffs satisfy certain monotonicity conditions have been shown to possess very useful properties. Milgrom and Shannon (1994) show that in games of strategic complements (GSC), where each agent best responds in a monotone increasing way to an increase in opponents’ strategies, there exist largest and smallest serially undominated strategies $a^{*}$ and $a_{*}$ which are also Nash equilibria, and that the limits of all adaptive learning processes eventually fall within the interval defined by $[a_{*}, a^{*}]$. Thus, if a unique serially undominated strategy ${\hat{a}}$ exists, it can be guaranteed to be a globally stable Nash equilibrium in the sense that any adaptive learning processes starting from any initial strategy will converge to it. In fact, as Milgrom and Roberts (1990) show, dominance solvability is equivalent to global stability. Roy and Sabarwal (2012) show that the same can be said for games of strategic substitutes (GSS), where players best respond in a monotone decreasing manner, with the exception that $a^{*}$ and $a_{*}$ need not be Nash equilibria. Barthel and Hoffmann (2019) generalize both GSC and GSS by defining games of strategic heterogeneity (GSH), where players may best respond either increasingly or decreasingly to an increase in the joint action choice of opponents, and show that these same aforementioned properties of solution sets hold in general GSH as well.

One sensible and alternative way to generalize the notions of GSC and GSS would be to allow each player to best respond either increasingly or decreasingly to an increase in each individual opponent’s actions, as opposed to the joint action choice of opponents, as in GSH. Notice, however, that such a formulation, which we term games of mixed heterogeneity (GMH), is even more general than the notion of GSH, and quite a bit more complex in that not only may the joint best response correspondence fail to be monotonic, but the best response correspondence of each individual agent may fail to be monotonic as well. Hence, it is unclear that the standard tools of monotonic analysis can be applied to GMH in order to guarantee nice properties of solution sets. The main contribution of this paper bridges this gap by providing both necessary and sufficient conditions for when the notions of GSH and GMH “coincide,” in the sense that a GMH may be viewed as GSH in such as way so that the order properties of solution sets guaranteed in GSH may be guaranteed in a GMH as well.

Our paper first considers transformations which employ the simple approach of “reversing” the order on the strategy spaces of some subset of players. Note that the strategy of transforming a game by reversing orders is not new in the literature on monotone games. Milgrom and Roberts (1990) show that a 2-firm oil exploration game can be transformed into a GSC by reversing one of the firm’s strategy spaces, whereas Amir (1996) shows that Cournot duopolies, which are GSS, can be transformed into a GSC in the same way. Roy and Sabarwal (2012), however, pointed out the limitations of this approach by constructing a three-player GSS possessing no Nash equilibria. Because the existence of equilibria is independent of an underlying order, and GSC always possess equilibria, it follows that not all GSS can be transformed into GSC. An important question then remains as to what properties an initial underlying game must possess in order to be transformed into a GSH in this way.

Theorem 1 provides a conclusive answer to this question by giving necessary and sufficient conditions for when a GMH can be transformed into a GSH through a reversal of orders. We provide similar necessary and sufficient conditions for games to be specifically transformed into GSC or GSS. Other authors have considered various ways in which an order can be constructed so that a game satisfies the definition of a GSC or GSS, ensuring that the order properties of solution sets in GSH are preserved with respect to some original order is a more complicated matter. For example, suppose we consider a market game ${\mathcal {G}}$ where firms must choose some level of output or price $x \in [0, {\hat{x}}] \in \mathbb {R}$, where $[0, {\hat{x}}]$ inherits the natural order $\succeq$ on $\mathbb {R}$. Suppose that an analyst constructs an alternative ordering $\succeq ^{*}$ on action spaces, so that ${\mathcal {G}}$ satisfies the definition of a GSH. Then, by the above discussion, it is clear that under $\succeq ^{*}$, an interval $[a_{*}, a^{*}]$ can be constructed which contains all serially undominated strategies, where $a^{*}$ and $a_{*}$ are themselves serially undominated, and which contains the limits of all adaptive learning processes. However, this does not guarantee that such an interval can be constructed in ${\mathcal {G}}$ under the natural order $\succeq$ on $\mathbb {R}$. Furthermore, because the notion of an adaptive dynamic also relies on how an order is defined, it is not guaranteed that a globally stable equilibrium under $\succeq ^{*}$ remains so under $\succeq$, and vice versa. However, Proposition 1 ensures that if a GMH can be transformed into a GSH by means of the reversal of the orders, then the set of solutions in a GMH can be guaranteed to possess all of the order properties inherent in a GSH.

A natural question then arises as to what other orderings can be constructed which transform a GMH into a GSH. Theorem 2, our second main contribution, shows that, under very general conditions, transforming a GMH into a GSH can $\textit{only}$ be done by some reversal of orders. Specifically, if one hopes to transform a strict GMH into a GSH or vice versa, then the only orders which can accomplish this while not increasing or decreasing the number or ordered pairs are those in which each player has either their original order, or their reversed order. This encompasses many scenarios, such as when the original and transformed orders are complete orders. This result, along with our previous discussion regarding the preservation of order properties of solution concepts, serves to justify our focus on searching for transformations through order reversals.

Our approach is most similar to Cao et al. (2018), who show that under some conditions, a GSC or any game where players have either increasing or decreasing differences with respect to opponents’ actions can be embedded into a larger GSS through the reversal of orders on strategy spaces, such that the set of Nash equilibria of the original game is a projection of the set of Nash equilibria of the embedding GSS. They conclude that the existence of such transformations show that GSS are more fundamental than GSC. However, in order to achieve this, they introduce so-called bridge players, who serve to reverse the strategic relationship between players in the original game. Thus, the original game and its embedding are fundamentally different games, with potentially different utility functions and an expanded set of players. Echenique (2004) takes a similar approach, but instead gives conditions under which a game, which may not have a previously defined order on action spaces, may be endowed with an order so that it satisfies the definition of a GSC. Here it is shown that for games with two or more equilibria, an order may be derived in which the game may be seen as a GSC. This result is very interesting in terms of addressing how generally one can describe payoffs in a given strategic scenario as exhibiting strategic complementarities under some order. However, notice that the existence of such an order can be guaranteed only with prior information about the equilibrium set, and that it need not adhere to any intuitive notion of how actions should be ordered.

In contrast to Cao et al. (2018) and Echenique (2004), who are interested in finding orders under which a game can be viewed as a GSS or GSC, respectively, we are interested in conditions under which a game’s solution set possesses the properties of more general GSH under some initial order, such as the natural order $\succeq$ on $\mathbb {R}$ discussed above. We give conditions under which the existence of an order that transforms ${\mathcal {G}}$ into a GSH allows us to draw conclusions about the set of solutions with respect to the original order $\succeq$. In particular, with respect to $\succeq$, there exist highest and lowest serially undominated strategies $a^{*}$ and $a_{*}$ whose interval $[a_{*}, a^{*}]$ contains all other serially undominated strategies, and the limits of all adaptive dynamics fall within $[a_{*}, a^{*}]$. Hence, ${\mathcal {G}}$ is dominance solvable if and only if it is globally stable.

This paper is organized as follows: Sect. 2 gives the relevant definitions and establishes necessary preliminary results. Section 3 states our main result (Theorem 1). Section 4 states our second main result (Theorem 2), which shows that under mild conditions, one can only hope to transform a GMH into a GSH with some reversal of orders. Section 5 concludes with supporting examples.

2 Model and definitions

A set X is called partially ordered if there exists a binary relation $\succeq$ on $X \times X$ which is reflexive, transitive, and anti-symmetric.¹ We call a partially ordered set a lattice if for each pair of elements $x,y \in X$, the supremum and infimum are contained in X, which we denote by $\mathrm{sup}_{\succeq }(x,y)$ and $\mathrm{inf}_{\succeq }(x,y)$, respectively. X is a complete lattice if for each non-empty subset $S \subset X$, we have that the supremum and infimum of S are contained in X, which we denote by $\mathrm{sup}_{\succeq }(S)$ and $\mathrm{inf}_{\succeq }(S)$, respectively. Finally, given a set X with an order $\succeq$, the order interval topology on X is that topology which takes all order intervals $[ a, b] \subset X$ as a sub-basis for closed sets.

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ denote a strategic form game, where ${\mathcal {I}}=\{1,2,\dots ,N\}$ is the set of N players. For an action space ${\mathcal {A}}= \mathop {\prod }\nolimits _{i \in {\mathcal {I}}}{\mathcal {A}}_{i}$, we let $\succeq =(\succeq _{i})_{i \in {\mathcal {I}}}$ describe a partial order $\succeq _{i}$ on ${\mathcal {A}}_{i}$ for each player i. We will abuse notation and allow $\succeq$ to represent the product order on ${\mathcal {A}}$. Also, for any player $i \in {\mathcal {I}}$, we will allow $\succeq _{-i}$ be the corresponding product order on ${\mathcal {A}}_{-i}= \mathop {\prod }\nolimits _{j \not = i}{\mathcal {A}}_{j}$. Throughout the paper, we will assume that for each $i \in {\mathcal {I}}$, $({\mathcal {A}}_{i}, \succeq _{i})$ is a complete lattice. Moreover, for each $i \in {\mathcal {I}}$, $\pi _{i}$ is continuous in a (in the order interval topology), and quasisupermodular in $a_{i}$.²

The literature on monotone games furthermore assumes that each player responds in a monotone way to the joint action of all opponents. Well-known results in this literature establish that player i’s best response is increasing (decreasing) in the joint action of opponents if $\pi _i$ satisfies the single-crossing property (SCP) (resp. decreasing SCP).³ If for all $i \in {\mathcal {I}}$, $\pi _{i}$ satisfies the SCP (resp. decreasing SCP) in $(a_{i}, a_{-i})$, a game ${\mathcal {G}}$ is called a game of strategic complements (GSC) (resp. game of strategic substitutes (GSS)).⁴ If for each $i \in {\mathcal {I}}$, $\pi _{i}$ satisfies either the SCP in $(a_{i}, a_{-i})$, or decreasing SCP in $(a_{i}, a_{-i})$, then ${\mathcal {G}}$ is also called a game of strategic heterogeneity (GSH).

In this paper, we will consider games that are very similar to those in the standard literature on monotone games, with the exception that we only require that each player has such a monotone relationship with each of her opponents individually as opposed to a monotone relationship with the joint action of opponents.⁵ To that end, we will assume that $\pi _{i}$ satisfies the pairwise SCP⁶ (respectively, pairwise decreasing SCP)⁷ with respect to $(a_{i}, a_{j})$ instead of the standard SCP with respect to $(a_i, a_{-i})$. With this in mind, we have the following definition:

Definition 1

Consider ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$.

• 1. If for each $i,j \in {\mathcal {I}}$, $\pi _{i}$ satisfies either the pairwise single-crossing property (SCP) in $(a_{i}, a_{j})$, or the pairwise decreasing SCP in $(a_{i}, a_{j})$, ${\mathcal {G}}$ is a game of mixed heterogeneity (GMH).

• 2. If for each $i \in {\mathcal {I}}$, $\pi _{i}$ satisfies either the pairwise SCP in $(a_{i}, a_{j})$ for all $j \not =i$, or the pairwise decreasing SCP in $(a_{i}, a_{j})$ for all $j \not =i$, then ${\mathcal {G}}$ is also called a game of strategic pairwise heterogeneity (GSPH).

Given an order $\succeq _{i}$ for some player $i \in {\mathcal {I}}$, define ${\widetilde{\succeq }}_{i}$ on ${\mathcal {A}}_{i}$ as, for each $x,y \in {\mathcal {A}}_{i}$, $x\,\,{\widetilde{\succeq }}_{i}\,\,y$ if and only if $y \succeq _{i} x$. That is, ${\widetilde{\succeq }}_{i}$ is the reversed order of $\succeq _{i}$. We will then explore the following question: Given a GMH $\mathcal {G}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$, which conditions are necessary and sufficient to guarantee the existence of some ${\hat{\succeq }}=(\hat{\succeq _{i}})_{i \in {\mathcal {I}}}$ such that $\mathcal {G}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, {\hat{\succeq }}\}$ is a GSC, GSS, or general GSH, where for each player i, we have that ${\hat{\succeq }}_{i}$ is equal to either $\succeq _{i}$ or ${\widetilde{\succeq }}_{i}$?

In order to address this question, we first establish that reversing the orders of a set of players in a GMH so that it becomes a GSPH is enough to conclude that it is a GSH as well. Cao et al. (2018) show that part (3) of Lemma 1 for the case of increasing and decreasing differences. For the sake of completeness, we provide a proof for the ordinal case as well.

Lemma 1

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ be a GMH. Consider the GMH given by ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, {\hat{\succeq }} \}$, where for each player i, ${\hat{\succeq }}_{i}$ is equal to either $\succeq _{i}$ or ${\widetilde{\succeq }}_{i}$.

• 1. For each $i \in {\mathcal {I}}$, if $({\mathcal {A}}_{i}, \succeq _{i})$ is a complete lattice, then $({\mathcal {A}}_{i}, {\widetilde{\succeq }}_{i})$ is a complete lattice. Furthermore, if $\pi _{i}$ satisfies quasisupermodularity under the order $\succeq _{i}$, it does so as well under the order ${\widetilde{\succeq }}_{i}$.

• 2. For each $i \in {\mathcal {I}}$, if $\pi _{i}$ is continuous in actions under the order interval topology resulting from $\succeq$, then it is continuous in actions under the order interval topology resulting from ${\hat{\succeq }}$.

• 3. For each $i \in {\mathcal {I}}$, if $\pi _{i}$ satisfies the pairwise (decreasing) SCP in $(a_{i}, a_{j})$ for each $j \not =i$, then $\pi _{i}$ satisfies the (decreasing) SCP in $(a_{i}, a_{-i})$, where ${\mathcal {A}}_{-i}$ is endowed with the product order $\succeq _{-i}$.

Proof

See “Appendix.” $\square$

Therefore, if an analyst hopes to reverse some collection of the $\succeq _{i}$ so that a GMH can be seen as a GSH, it is sufficient (and equivalent) to transform it into a GSPH.

We now discuss the properties that a GMH inherits given the existence of some order reversal which transforms it into a GSH. First, given a game ${\mathcal {G}}$, and player $i \in {\mathcal {I}}$, consider some subset of actions $Z_{-i} \subset {\mathcal {A}}_{-i}$. We then define the set

$$\begin{aligned} U_{i}(Z_{-i}) = \{a_{i} \in {\mathcal {A}}_{i} \mid \forall a'_{i} \in {\mathcal {A}}_{i}, \, \exists a_{-i} \in Z_{-i}, \, \pi _{i}(a_{i}, a_{-i}) \ge \pi _{i}(a'_{i}, a_{-i}) \} \end{aligned}$$

as player i’s undominated responses to $Z_{-i}$. For $Z \subset {\mathcal {A}}$, let $Z_{-i}$ be the projection of Z onto ${\mathcal {A}}_{-i}$. Then $U(Z)=(U_{i}(Z_{-i}))_{i \in {\mathcal {I}}}$ is defined as the set of undominated responses to Z. Consider the following process:

• 1. $Z^{0}=U({\mathcal {A}})$.

• 2. For all $n \ge 1$, $Z^{n}=U(Z^{n-1})$.

We then call $S= \mathop \cap \nolimits _{n \ge 0} Z^{n}$ the set of $\mathbf serially undominated strategies$ in ${\mathcal {G}}$.

For some sequence of actions $(a^{n})^{\infty }_{n=0}$, define

$$\begin{aligned} {\mathcal {P}}(T,t)=\{a^{n} \mid t > n \ge T \} \end{aligned}$$

as the history of play between time periods T and t. We then say that $(a^{n})^{\infty }_{n=0}$ is an $\mathbf adaptive dynamic$ if for each $T \ge 0$ there exists some $T' \ge 0$, such that for each $t \ge T'$,

$$\begin{aligned} a^{t} \in [\mathrm{inf}_{\succeq }U(\widehat{{\mathcal {P}}(T,t)}), \mathrm{sup}_{\succeq }U(\widehat{{\mathcal {P}}(T,t)})], \end{aligned}$$

where $\widehat{{\mathcal {P}}(T,t)}=[\mathrm{inf}_{\succeq }{\mathcal {P}}(T,t), \, \mathrm{sup}_{\succeq }{\mathcal {P}}(T,t)]$. The class of adaptive learning processes include learning rules such as best response dynamics and fictitious play, among many others. Notice that the definition of an adaptive dynamic relies on the underlying order in the game, as it depends on the relevant notion of an order interval. Also, we will call a Nash equilibrium ${\hat{a}} \in {\mathcal {A}}$ $\mathbf globally stable$ as long as every adaptive dynamic $(a^{n})^{\infty }_{n=0}$ which is non-constant converges to ${\hat{a}}$.⁸

The next proposition, due to Barthel and Hoffmann (2019), highlights why transforming a game into a GSH is of interest. In particular, we see that given a game ${\mathcal {G}}$ and an original order $\succeq =(\succeq _{i})_{i \in {\mathcal {I}}}$, then the existence of some reversal of orders ${\hat{\succeq }}=(\hat{\succeq _{i}})_{i \in {\mathcal {I}}}$ under which ${\mathcal {G}}$ is a GSH allows us to draw strong conclusions about solution concepts in ${\mathcal {G}}$ with respect to the original ordering $\succeq =(\succeq _{i})_{i \in {\mathcal {I}}}$. The proof of this statement requires some slight modifications for our setting, which are given in “Appendix.”

Proposition 1

(Barthel and Hoffmann) Let ${\mathcal {G}}$ be a GMH under $\succeq =(\succeq _{i})_{i \in {\mathcal {I}}}$. Suppose that ${\mathcal {G}}$ is a GSH under some ${\hat{\succeq }}=(\hat{\succeq _{i}})_{i \in {\mathcal {I}}}$, where for each player $i \in {\mathcal {I}}$, we have that either ${\hat{\succeq }}_{i}=\succeq _{i}$ or ${\hat{\succeq }}_{i}={\widetilde{\succeq }}_{i}$. Then, with respect to the original ordering $\succeq =(\succeq _{i})_{i \in {\mathcal {I}}}$,

• 1. There exist upper and lower serially undominated strategies $a^{*}$ and $a_{*}$ such that the limit of all adaptive learning processes falls within the interval defined by $[a_{*}, a^{*}]$.

• 2. The following are equivalent statements:

  • (a) ${\mathcal {G}}$ is dominance solvable.

  • (b) $a^{*}=a_{*}$.

  • (c) There exists a unique, globally stable Nash equilibrium ${\hat{a}}$.

• 3. If ${\mathcal {G}}$ exhibits more than one Nash equilibrium, then no Nash equilibrium is globally stable.

Proof

See “Appendix.” $\square$

The following example highlights the main concepts that have been discussed thus far.

Example 1

As an example, consider the following three-player game, where each player $i \in \{1,2,3\}$ has the strategy space ${\mathcal {A}}_{i}=\{L,H\}$.

Suppose that the ${\mathcal {A}}_{i}$ are endowed with orders $\succeq _{i}$ such that $H\succeq _{i}L$ for $i=1,2$, and $L\succeq _{3}H$ for Player 3. It is straightforward to check that utility for Players 1 and 2 satisfy the decreasing SCP between each others’ actions, and that each satisfy the SCP with respect to Player 3’s action. Also, the utility given for Player 3 satisfies the SCP in each of her opponents’ actions. Hence, this game is a GMH.

Now suppose that the order on Player 3’s strategy space is reversed, so that ${\mathcal {A}}_{3}$ is endowed with order ${\widetilde{\succeq }}_{3}$, where $H\,\,{\widetilde{\succeq }}_{3}\,\,L$. Keeping the orders on ${\mathcal {A}}_{1}$ and ${\mathcal {A}}_{2}$ the same as before, we see that utility for each player satisfies the decreasing SCP with respect to each of her individual opponents. By Lemma 1, we may conclude that the game is a GSS. Furthermore, as (H, L, L) is the unique, serially undominated strategy, we may conclude from Proposition 1 that it is also globally stable when the original orders are considered.

3 Main results

We now come to the main results of the paper. Having established the notions of GMH and GSH, we must first properly define the process of transforming one into another. To this end, let $\mathbb {R}^{N \times N}$ be the set of real-valued, $N \times N$ square matrices. It will be useful to make the following definition:

Definition 2

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ be a GMH. Then the strategic matrix associated with ${\mathcal {G}}$ is the $N \times N$ matrix ${\mathcal {M}}_{{\mathcal {G}}} \in \mathbb {R}^{N \times N}$ whose $(j,i)\mathrm{th}$ entry $m_{j,i}$ is given by the following:

• 1. For all $i \in {\mathcal {I}}$, $m_{i,i}=0$.

• 2. For all $i,j \in {\mathcal {I}}$, $i \not =j$, $m_{j,i}=1$ if $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$.

• 3. For all $i,j \in {\mathcal {I}}$, $i \not =j$, $m_{j,i}=-1$ if $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$.

Thus, ${\mathcal {M}}_{{\mathcal {G}}}$ fully determines the pairwise strategic relationships between each pair of players, so that the $i\mathrm{th}$ column describes how player i best responds to an increase in the strategy from each of her opponents. By convention, we set each $m_{i,i}=0$, as player i does not best respond to an action taken by herself. For example, let $1_{N \times N} \in \mathbb {R}^{N \times N}$ be the $N \times N$ matrix whose every entry is 1, $I_{N \times N} \in \mathbb {R}^{N \times N}$ be the identity matrix, and $Q \in \mathbb {R}^{N \times N}$ be the matrix whose off-diagonal elements are zero, but whose diagonal elements $q_{i,i}$ are either 1 or $-1$. Consider the matrix

$$\begin{aligned} J=(1_{N \times N}-I_{N \times N})Q. \end{aligned}$$

(1)

Then,

• 1. If $q_{i,i}=1$ for each $i \in {\mathcal {I}}$, then J is the strategic matrix ${\mathcal {M}}_{{\mathcal {G}}}$ associated with some game of strategic complements ${\mathcal {G}}$.

• 2. If $q_{i,i}=-1$ for each $i \in {\mathcal {I}}$, then J is the strategic matrix ${\mathcal {M}}_{{\mathcal {G}}}$ associated with some game of strategic substitutes ${\mathcal {G}}$.

• 3. If $q_{i,i}=1$ or $q_{i,i}=-1$ for each $i \in {\mathcal {I}}$, then J is the strategic matrix ${\mathcal {M}}_{{\mathcal {G}}}$ associated with some game of strategic heterogeneity ${\mathcal {G}}$.

The next lemma describes how the strategic matrix associated with a game ${\mathcal {G}}$ changes after the orders of any subset of players $S \subset {\mathcal {I}}$ are reversed.

Lemma 2

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ be a GMH, and let ${\mathcal {M}}_{{\mathcal {G}}}$ be the corresponding strategic matrix associated with a game ${\mathcal {G}}$, with common entry $m_{j,i}$. Let $S \subset {\mathcal {I}}$ be any non-empty subset of players, and consider the game $\hat{{\mathcal {G}}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, {\hat{\succeq }} \}$, where ${\hat{\succeq }}=(\hat{\succeq _{i}})_{i \in {\mathcal {I}}}$ is such that for all $i \in S$, ${\hat{\succeq }}_{i}={\widetilde{\succeq }}_{i}$, and for all $i \notin S$, ${\hat{\succeq }}_{i}=\succeq _{i}$. For each $A \in \mathbb {R}^{N \times N}$ with common element $a_{i,j}$, let $T_{S}: \mathbb {R}^{N \times N} \rightarrow \mathbb {R}^{N \times N}$ be the transformation described by

$$\begin{aligned} T_{S}(A)_{j,i}={\left\{ \begin{array}{ll} a_{j,i} &{} \quad i \in S, j \in S \\ a_{j,i} &{} \quad i \notin S, j \notin S \\ -a_{j,i} &{} \quad i \in S, j \notin S \\ -a_{j,i} &{} \quad i \notin S, j \in S. \\ \end{array}\right. } \end{aligned}$$

(2)

Then, if ${\mathcal {M}}_{\hat{{\mathcal {G}}}}$ is the strategic matrix associated with $\hat{{\mathcal {G}}}$, we have that $T_{S}({\mathcal {M}}_{{\mathcal {G}}})={\mathcal {M}}_{\hat{{\mathcal {G}}}}$.

Proof

See “Appendix.” $\square$

In view of Lemmas 1 and 2, we have reduced our problem of finding an ordering ${\hat{\succeq }}$ under which a GMH may be written as a GSH to one of the findings, an appropriate $S \subset {\mathcal {I}}$, so that the corresponding $T_{S}$ transforms our original strategic matrix into one that represents a GSH, as in Eq. (2). The next definition formalizes this notion:

Definition 3

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ be a GMH, and let ${\mathcal {M}}_{{\mathcal {G}}}$ be the corresponding strategic matrix associated with a game ${\mathcal {G}}$. Then ${\mathcal {G}}$ can be transformed into a GSH if there exists some non-empty $S \subset {\mathcal {I}}$ such that $S \not = {\mathcal {I}}$, and

$$\begin{aligned} T_{S}({\mathcal {M}}_{{\mathcal {G}}})=J, \end{aligned}$$

where J is the strategic matrix ${\mathcal {M}}_{\hat{{\mathcal {G}}}}$ of some GSH. We similarly define the cases of when ${\mathcal {G}}$ can be transformed more specifically into GSC or GSS.

It is easy to verify that when $S={\mathcal {I}}$, $T_{S}(A)=A$ for any matrix A. Hence, requiring that $S \subset {\mathcal {I}}$ be non-empty and not equal to ${\mathcal {I}}$ itself simply rules out accounting for transforming GSH into themselves. Obviously, this is with no loss of generality. We now come to our first result, which gives necessary and sufficient condition for when a GMH can be transformed into a GSH.

Theorem 1

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ be a GMH, such that ${\mathcal {G}}$ is not a GSH. Then ${\mathcal {G}}$ can be transformed into a GSH if and only if ${\mathcal {I}}$ can be partitioned into two non-empty subsets ${\mathcal {I}}'$ and ${\mathcal {I}}''$ such that

• 1. For all $i \in {\mathcal {I}}'$, $\pi _{i}$ satisfies either the pairwise SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}'/ \{i\}$, or satisfies the pairwise decreasing SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}'/ \{i\}$.

• 2. For all $i \in {\mathcal {I}}''$, $\pi _{i}$ satisfies either the pairwise SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}''/ \{i\}$, or satisfies the pairwise decreasing SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}''/ \{i\}$.

• 3. For all $i \in {\mathcal {I}}$, if $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$ for all $j \in {\mathcal {I}}'/ \{i\}$, then $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$ for all $j \in {\mathcal {I}}''/ \{i\}$. If $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$ for all $j \in {\mathcal {I}}'/ \{i\}$, then $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$ for all $j \in {\mathcal {I}}''/ \{i\}$.

Proof

Given in “Appendix.” $\square$

Because GSC can be guaranteed to possess upper and lower Nash equilibria, it is often of particular interest to know when a game can be transformed into a GSC, which is addressed in our next result. Interestingly, Cao et al. (2018) allude to the “only if” part concerning transforming a GSH into a GSC in Remark 2 of their paper. Proposition 2 thus serves as an extension to this observation by providing both necessary and sufficient conditions for such a transformation in the case of both GSC and GSS.

Proposition 2

Let ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ be a GMH, such that ${\mathcal {G}}$ is not a GSC. Then ${\mathcal {G}}$ can be transformed into a GSC if and only if ${\mathcal {I}}$ can be partitioned into two non-empty subsets ${\mathcal {I}}'$ and ${\mathcal {I}}''$ such that

• 1. For all $i,j \in {\mathcal {I}}'$ such that $i \not =j$, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$.

• 2. For all $i,j \in {\mathcal {I}}''$ such that $i \not =j$, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$.

• 3. For all $i \in {\mathcal {I}}'$ and $j \in {\mathcal {I}}''$, $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$.

Likewise, if ${\mathcal {G}}$ is such that ${\mathcal {G}}$ is not a GSS, then ${\mathcal {G}}$ can be transformed into a GSS if and only if ${\mathcal {I}}$ can be partitioned into two non-empty subsets ${\mathcal {I}}'$ and ${\mathcal {I}}''$ such that

• 1. For all $i,j \in {\mathcal {I}}'$ such that $i \not =j$, $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$.

• 2. For all $i,j \in {\mathcal {I}}''$ such that $i \not =j$, $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$.

• 3. For all $i \in {\mathcal {I}}'$ and $j \in {\mathcal {I}}''$, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$.

Proof

Similar to that of Theorem 1, and given in “Appendix.” $\square$

The next corollary gives necessary and sufficient conditions for transforming games that are initially a GSH into either a GSC or GSS. More importantly, it shows that, as long as there are more than two players, then if a GMH can be transformed into a GSH that is not a GSC (respectively, a GSS), then one cannot hope to find an alternative transformation which transforms it into such.

Corollary 1

Let ${\mathcal {G}}$ be a GSH that is not a GSC. Then ${\mathcal {G}}$ can be transformed into a GSC if and only if it is a two-player GSS. Likewise, if ${\mathcal {G}}$ is a GSH that is not a GSS, then ${\mathcal {G}}$ can be transformed into a GSS if and only if it is a two-player GSC.

Proof

See “Appendix.” $\square$

4 Considering other orders

In light of the results of the previous section, it is natural to ask whether, given a game ${\mathcal {G}}$ which is a GMH under $\succeq$, other possible orders can be found which transform ${\mathcal {G}}$ into a GSH besides reversing some combination of the original $\succeq _{i}$. In this section, we show that in many settings, the answer is surprisingly no. To this end, we first consider the class of GSH under which player’s utility satisfies increasing or decreasing differences, as opposed to the ordinal SCP or decreasing SCP considered in the previous section. Recall that in a set with a partial order $\succeq$, two elements x and y are said to be strictly ordered under $\succeq$ if and only if $x \succeq y$ and $x \not = y$. Hence, any two elements are either unordered, strictly ordered, or equal.

Definition 4

A tuple ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$ is said to be a game of mixed heterogeneity with differences (GMHD) if we have the following:

• For each $i,j \in {\mathcal {I}}$, $\pi _{i}$ satisfies either pairwise increasing differences in $(a_{i}, a_{j})$,⁹ or pairwise decreasing differences in $(a_{i}, a_{j})$.¹⁰

Furthermore, if for all $i \in {\mathcal {I}}$, $\pi _{i}$ satisfies either increasing differences in $(a_{i}, a_{-i})$, or decreasing differences in $(a_{i}, a_{-i})$,¹¹ then ${\mathcal {G}}$ is called a game of strategic heterogeneity with differences (GSHD).

Lastly, we define the strict versions of the above games by requiring that the inequalities in the above requirements hold strictly for strictly ordered pairs of actions. In these cases, we say either strict GMHD or strict GSHD.

Recall from the previous section that given reflexive, transitive, and anti-symmetric orders $\succeq =(\succeq _{i})_{i \in {\mathcal {I}}}$ on strategy spaces, we called ${\hat{\succeq }}=({\hat{\succeq }}_{i})_{i \in {\mathcal {I}}}$ a reversed order of $\succeq$ if, for some $S \subset {\mathcal {I}}$, we have that ${\hat{\succeq }}_{i}={\widetilde{\succeq }}_{i}$ for each $i \in S$, and ${\hat{\succeq }}_{i}=\succeq _{i}$ for each $i \in {\mathcal {I}}/S$. Thus, given $\succeq$, we will let the set $R^{\succeq }$ denote the collection of all possible reversed orders of $\succeq$. Also, given $\succeq _{i}$ on ${\mathcal {A}}_{i}$, we will denote by ${\mathcal {A}}^{\succeq }_{i}$ as those pairs of actions $(a_{i},a'_{i}) \in {\mathcal {A}}_{i}$ which are ordered under $\succeq _{i}$. More precisely,

$$\begin{aligned} {\mathcal {A}}^{\succeq }_{i}=\{ (a_{i},a'_{i}) \in {\mathcal {A}}_{i} \times {\mathcal {A}}_{i} \mid a_{i} \succeq _{i} a'_{i}, \,\, or\,\, a'_{i} \succeq _{i} a_{i}\}. \end{aligned}$$

Lastly, for a game ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$, we let ${\mathcal {G}}^{*}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq ^{*} \}$ be that game with the same elements as ${\mathcal {G}}$, but with another arbitrary ordering on strategy spaces $\succeq ^{*}=(\succeq ^{*}_{i})_{i \in {\mathcal {I}}}$. In what follows, we will consider only those $\succeq ^{*}$ such that the $\succeq ^{*}_{i}$ are reflexive, transitive, and anti-symmetric as well.

Theorem 2 shows that given a strict GMHD ${\mathcal {G}}$ (respectively, GMHD) with ordering $\succeq$, then any other ordering $\succeq ^{*}$ which has the same collection of ordered pairs as $\succeq$ and which transforms ${\mathcal {G}}$ into a GSHD (respectively, strict GSHD) must be such that each $\succeq _{i}^{*}$ is either the original order $\succeq _{i}$, or the reversed order ${\widetilde{\succeq }}_{i}$.

Theorem 2

Consider two games ${\mathcal {G}}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq \}$, and

${\mathcal {G}}^{*}= \{{\mathcal {I}}, ({\mathcal {A}}_{i}, {\pi }_{i})_{i \in {\mathcal {I}}}, \succeq ^{*} \}$. Suppose that for each player $i \in {\mathcal {I}}$, ${\mathcal {A}}^{\succeq }_{i}={\mathcal {A}}^{\succeq ^{*}}_{i}$, and that each ${\mathcal {A}}_{i}$ has at least one strictly ordered pair. Then,

• 1. If ${\mathcal {G}}$ is a GMHD, and ${\mathcal {G}}^{*}$ is a strict GSHD, then $\succeq ^{*} \in R^{\succeq }$.

• 2. If ${\mathcal {G}}$ is a strict GMHD, and ${\mathcal {G}}^{*}$ is a GSHD, then $\succeq ^{*} \in R^{\succeq }$.

Proof

Given in “Appendix.” $\square$

It is apparent from the above proof that the requirements in Theorem 2 can be weakened even more, in that instead of requiring either strict increasing or decreasing differences among all players, we only require that for each player j, there exists some other player i so that $\pi _{i}$ exhibits strict increasing or decreasing differences in $(a_{i},a_{j})$. Also, note that the requirement that ${\mathcal {A}}^{\succeq }_{i}={\mathcal {A}}^{\succeq ^{*}}_{i}$ is automatically satisfied when $\succeq _{i}$ and $\succeq ^{*}_{i}$ are complete orders.

It is a very natural question to ask whether the results of Theorem 2 can be extended to ordinal games that satisfy the (decreasing) SCP instead of (decreasing) increasing differences. We will address this questions in the following section in Example 6.

5 Applications

We now present some examples. We will make use of the following observations on a slightly more general version of the aggregative games defined in Acemoglu and Jensen (2013), so that player i’s payoff is given by

$$\begin{aligned} u_i = F_i(g(a), v_{i}((a_i)), \end{aligned}$$

where $g: A \rightarrow \mathbb {R}^k$ is a k-dimensional aggregator function, and $v_{i}:A_{i} \rightarrow \mathbb {R}$ is increasing. As in Acemoglu and Jensen (2013) will assume for $i = 1, \dots , k$, each component function $g_i$ is additively separable, and assume further that each $F_{i}$ is separable in the $g_{i}$, so that $\frac{\partial ^2 F_i}{\partial g_m \partial g_l} = 0$ for each $l \not =m$.

For each player i and j, where $i\not =j$, we can then write

$$\begin{aligned} \frac{\partial ^2 u_i}{\partial a_i \partial a_j} = \sum _{z=1}^{k} \frac{\partial g_z}{\partial a_j} D_{i,z}, \end{aligned}$$

(3)

where

$$\begin{aligned} D_{i,z} = \frac{\partial ^2 F_i}{\partial g^2_z} \cdot \frac{\partial g_z}{\partial a_i} + \frac{\partial ^2 F_i}{\partial v \partial g_z} \cdot \frac{\partial v}{\partial a_i}. \end{aligned}$$

Players i and j then have pairwise increasing differences in $(a_i, a_j)$ if $\frac{\partial g_z}{\partial a_j}$ and $D_{i,z}$ have the same signs for all z and pairwise decreasing differences in $(a_i, a_j)$ if $\frac{\partial g_z}{\partial a_j}$ and $D_{i,z}$ have opposite signs for all z. We will apply this observation in the following examples.

Example 2

(Cournot–Bertrand oligopoly) Consider an N firm Cournot–Bertrand oligopoly, where $\mathcal {I^\prime } \subset {\mathcal {I}}$ are those firms who compete in quantity, and $\mathcal {I^{\prime \prime }}\subset {\mathcal {I}}$ are those firms who compete in price. We will assume that there exists at least one quantity competitor and at least one price competitor to focus on mixed oligopolies. We will denote the number of quantity competitors by m. Let $Q_{i}(\cdot )$ and $P_i( \cdot )$ denote firm i’s demand and inverse demand functions in terms of each firm’s strategic variable, respectively, which we assume are twice differentiable.¹² For example, suppose as in Matsumoto and Szidarovszky (2009) that for each $i \in {\mathcal {I}}$ market demand is given by¹³

$$\begin{aligned} P_i = \alpha - q_i -\gamma \sum _{j \ne i} q_j, \end{aligned}$$

where $\alpha >0$ and $-1< \gamma <1$ with $\gamma \ne 0$. That is, the goods are (imperfect) substitutes for $0<\gamma <1$ and (imperfect) complements for $-1< \gamma < 0$. Expressing demand and inverse demand in terms of each firm’s strategic variable results in, for each $i \in \mathcal {I^\prime }$,

$$\begin{aligned} P_{i}=A-ba_{i}-c\sum _{j \in \mathcal {I^\prime }, j \not =i} a_j + d\sum _{j \in \mathcal {I^{\prime \prime }}} a_j, \end{aligned}$$

where

$$\begin{aligned} A&=\frac{(1-\gamma )\alpha }{1+(N-m-1)\gamma },&b&=\frac{(1-\gamma )(1+(N-m)\gamma )}{1+(N-m-1)\gamma }, \\ c&=\frac{\gamma (1-\gamma )}{1+(N-m-1)\gamma },&d&=\frac{\gamma }{1+(N-m-1)\gamma }, \end{aligned}$$

and for each $i \in \mathcal {I^{\prime \prime }}$,

$$\begin{aligned} Q_{i}={\hat{A}}-{\hat{b}}a_{i}-{\hat{c}}\sum _{j \in \mathcal {I^\prime }} a_j + {\hat{d}}\sum _{j \in \mathcal {I^{\prime \prime }}, j \not =i} a_j, \end{aligned}$$

where

$$\begin{aligned} {\hat{A}}&=\frac{(1-\gamma )\alpha }{1+(N-m-1)\gamma },&{\hat{b}}&=\frac{1+(N-m-2)\gamma }{(1-\gamma )(1+(N-m-1)\gamma )}, \\ {\hat{c}}&=\frac{\gamma (1-\gamma )}{(1-\gamma )(1+(N-m-1)\gamma )},&{\hat{d}}&=\frac{\gamma }{(1-\gamma )(1+(N-m-1)\gamma )}. \end{aligned}$$

Demand functions satisfy the law of demand with a positive intercept when $A, {\hat{A}}, b, {\hat{b}} >0$, which holds for $-\frac{1}{N-m}< \gamma < 1$.

Then for all $i \in {\mathcal {I}}'$, define $g_i = A - P_i(a)$ and for all $i \in {\mathcal {I}}''$, let $g_i = {\hat{A}} - Q_i(a)$, which are aggregator functions as described above. Notice that profits for each quantity competitor $i \in \mathcal {I^\prime }$ can be written as

$$\begin{aligned} \pi _i = P_i(g_{i}(a))a_{i} - C_i(a_{i}), \end{aligned}$$

and for each price competitor $i \in \mathcal {I^{\prime \prime }}$,

$$\begin{aligned} \pi _i = Q_i(g_{i}(a))a_{i}- C_i( g_{i}(a)), \end{aligned}$$

where we assume that each $C_{i}$ is convex.

In order to apply Eq. 3, first note that for each $i \in {\mathcal {I}}$, $D_{i,i}=-1$, and for each $i, i' \in \mathcal {I^\prime }$ and $j, j' \in \mathcal {I^{\prime \prime }}$ such that $i \not =i'$ and $j \not =j'$,

$$\begin{aligned}&\mathrm{sgn}\left( \frac{\mathrm{d}g_{i}}{\mathrm{d}a_{i'}}\right) = \mathrm{sgn}\left( \frac{\mathrm{d}g_{j}}{\mathrm{d}a_{i'}}\right) = \mathrm{sgn}(\gamma ),\\&\mathrm{sgn}\left( \frac{\mathrm{d}g_{i}}{\mathrm{d}a_{j'}}\right) = \mathrm{sgn} \left( \frac{\mathrm{d}g_{j}}{\mathrm{d}a_{j'}}\right) = -\mathrm{sgn}(\gamma ). \end{aligned}$$

Then, we have by Eq. 3 that

$$\begin{aligned} \mathrm{sgn}\left( \frac{\partial ^2 \pi _i}{\partial a_{i'} \partial a_i}\right)&=\mathrm{sgn}\left( \frac{\mathrm{d}g_{i}}{\mathrm{d}a_{i'}} \cdot D_{i,i}\right) =-\mathrm{sgn}(\gamma ), \\ \mathrm{sgn}\left( \frac{\partial ^2 \pi _i}{\partial a_{j} \partial a_i}\right)&=\mathrm{sgn}\left( \frac{\mathrm{d}g_{i}}{\mathrm{d}a_{j}} \cdot D_{i,i}\right) =\mathrm{sgn}(\gamma ), \\ \mathrm{sgn}\left( \frac{\partial ^2 \pi _j}{\partial a_{j'} \partial a_j}\right)&=\mathrm{sgn}\left( \frac{\mathrm{d}g_{j}}{\mathrm{d}a_{j'}} \cdot D_{j,j}\right) =\mathrm{sgn}(\gamma ), \\ \mathrm{sgn}\left( \frac{\partial ^2 \pi _j}{\partial a_{i} \partial a_j}\right)&=\mathrm{sgn}\left( \frac{\mathrm{d}g_{j}}{\mathrm{d}a_{i}} \cdot D_{j,j}\right) = -\mathrm{sgn}(\gamma ). \end{aligned}$$

Therefore, for $-\frac{1}{N-m}< \gamma < 1$, this game is not a GSH, since for $0<\gamma <1$, players in $\mathcal {I^{\prime }}$ share the decreasing SCP for all other $i' \in {\mathcal {I}}'$, but the SCP with each $j \in \mathcal {I^{\prime \prime }}$. Likewise, each player $j \in {\mathcal {I}}''$ satisfies the SCP for all other $j' \in {\mathcal {I}}''$, but the decreasing SCP with each $i \in {\mathcal {I}}'$. The opposite is the case for $-\frac{1}{N-m}< \gamma < 0$. However, notice that by Theorem 1, we can use ${\mathcal {I}}'$ and ${\mathcal {I}}''$ as partitioning elements of ${\mathcal {I}}$ to conclude that the game can always be transformed into a GSH for the case when products are gross substitutes, as well as for some degree of gross complementarity.

Furthermore, if the four inequalities on the cross-partials hold strictly, then the Cournot–Bertrand oligopoly is a strict GMHD. Thus, by Theorem 2, any complete order $\succeq ^{*}$ which transforms either game into a GSHD must be such that for each i, $\succeq _{i}^{*}$ is either the original order $\succeq _{i}$, or the reversed order ${\widetilde{\succeq }}_{i}$.

Example 3

(Oligopolies with substitutes and complements) In this example, we consider Cournot or Bertrand markets in which some firms produce goods which are complement goods with some competitors, and substitute goods with others. First, consider the following N firm Cournot oligopoly from Amir and Jin (2001), where players $i \in {\mathcal {I}}' \subset {\mathcal {I}}$ have substitutes with all other players $j \ne i$ and players $i \in {\mathcal {I}}'' \subset {\mathcal {I}}$ have complements with all other players $j \ne i$. That is, for $i \in {\mathcal {I}}'$, $\frac{\partial P_j}{\partial q_i} <0$ and for all $i \in {\mathcal {I}}''$, $\frac{\partial P_j}{\partial q_i} >0$, where $P_j(\cdot )$ denotes firm j’s twice differentiable inverse demand function. Following Singh and Vives (1984) as a concrete example, assume firm i’s inverse demand function can be written as

$$\begin{aligned} P_i = \alpha _i - a_i - \gamma \sum _{j \in {\mathcal {I}}', j \ne i} a_j + \delta \sum _{j \in {\mathcal {I}}'', j \ne i} a_j, \end{aligned}$$

where $a_i$ denotes firm i’s quantity, and $\alpha _i, \gamma , \delta >0$.

Thus, we have that for all i, $P_i = \alpha _i - g_i$, where

$$\begin{aligned} g_i = a_i - \gamma \sum _{j \in {\mathcal {I}}', j \ne i} a_j + \delta \sum _{j \in {\mathcal {I}}'', j \ne i} a_j. \end{aligned}$$

Thus, profits for each firm i can be written as

$$\begin{aligned} \pi _i = F_i(g(a), a_{i}) = P_i(g_{i}(a))a_{i} - C_i(a_{i}), \end{aligned}$$

where we assume that each $C_i$ is convex. Following Eq. 3, we have that for each i,

$$\begin{aligned} D_{i,i}=P_i^{\prime \prime } \cdot \frac{\partial g_{i}}{\partial a_i}+P_i^{\prime } \le 0. \end{aligned}$$

Moreover, for each $i, i' \in \mathcal {I^\prime }$ and $j, j' \in \mathcal {I^{\prime \prime }}$ such that $i \not =i'$ and $j \not =j'$, we have that

$$\begin{aligned} \frac{\partial ^2 \pi _i}{\partial a_{i'} \partial a_i}&=\frac{\mathrm{d}g_{i}}{\mathrm{d}a_{i'}} \cdot D_{i,i} \ge 0,&\frac{\partial ^2 \pi _i}{\partial a_{j} \partial a_i}&=\frac{\mathrm{d}g_{i}}{\mathrm{d}a_{j}} \cdot D_{i,i} \le 0, \\ \frac{\partial ^2 \pi _j}{\partial a_{j'} \partial a_j}&=\frac{\mathrm{d}g_{j}}{\mathrm{d}a_{j'}} \cdot D_{j,j} \le 0,&\frac{\partial ^2 \pi _j}{\partial a_{i} \partial a_j}&=\frac{\mathrm{d}g_{j}}{\mathrm{d}a_{i}} \cdot D_{j,j} \ge 0. \end{aligned}$$

Note that this game is not originally a GSH, as each player $i \in {\mathcal {I}}'$ shares the SCP for all other $i' \in {\mathcal {I}}'$, but the decreasing SCP with each $j \in \mathcal {I^{\prime \prime }}$ and each player $j \in {\mathcal {I}}''$ satisfies the decreasing SCP for all other $j' \in {\mathcal {I}}''$, but the SCP with each $i \in {\mathcal {I}}'$. However, as we have shown, we can use ${\mathcal {I}}'$ and ${\mathcal {I}}''$ as partitioning elements of ${\mathcal {I}}$ to conclude that this game can be transformed into a GSH as well. A similar argument can be made when all firms are price competitors.

Example 4

(Resource conservation) Consider a situation where each player i in some group of players $\mathcal {I^\prime } \subset {\mathcal {I}}$ chooses to extract some amount $a_{i}$ of a natural resource to use as an input in order to compete in quantity competition among the other members of $\mathcal {I^\prime }$, while each player j in a separate group $\mathcal {I^{\prime \prime }} \subset {\mathcal {I}}$ purchases an amount $a_{j}$ of the natural resource in order to preserve it. For example, players in group $\mathcal {I^\prime }$ may be loggers who wish to purchase trees to process, while players in group $\mathcal {I^{\prime \prime }}$ may be conservationists. We assume that each player $i \in {\mathcal {I}}$ derives a positive benefit which is increasing in the amount of natural resource present. To this end, define $g_{1}:A \rightarrow \mathbb {R}$ by $g_1(a) = \sum _{j \in \mathcal {I^{\prime \prime }}} a_j - \sum _{j \in \mathcal {I^{\prime }}} a_j.$ and let $P_{i}(g_{1}(a))$ be the benefit of player i derived from the amount of natural resource present, where it is assumed that each $P_{i}$ is positive, increasing, and $\frac{\partial ^2 P_{i}}{\partial g_1^2}\le 0$, where equality holds only when $g_{1}=0$. For each player $i \in \mathcal {I^{\prime \prime }}$, we write $C_{i}(a_{i})$ as the cost of purchasing natural resources. Furthermore, define $g_{2}:A \rightarrow \mathbb {R}$ by $g_2(a) = \sum _{i \in \mathcal {I^{\prime }}} a_j.$ Then, for each player $i \in \mathcal {I^{\prime }}$, we write $\pi _{i}(g_{2}(a), a_{i})$ as player i’s profit from extracting resource amount $a_{i}$ and competing in quantity competition among opponents in $\mathcal {I^{\prime }}$. Hence, defining $g:A \rightarrow \mathbb {R}^{2}$ as $g(a)=(g_{1}(a), g_{2}(a))$, utility for each player $i \in \mathcal {I^{\prime \prime }}$ is defined as

$$\begin{aligned} u_{i}(a)=F_{i}(g(a), v_{i}(a_{i}))=P_{i}(g_{1}(a))-C_{i}(a_{i}), \end{aligned}$$

whereas utility for each player $i \in \mathcal {I^\prime }$ can be expressed as

$$\begin{aligned} u_{i}(a)=F_{i}(g(a), v_{i}(a_{i}))=P_{i}(g_{1}(a))+\pi _{i}(g_{2}(a), a_{i}). \end{aligned}$$

Notice that the appearance of $P_{i}(g_{1})$ in the utility of each player prevents this game from being a GSH: For each player $i \in \mathcal {I^{\prime \prime }}$, we have that as long as $g_{1}>0$, $\frac{\partial ^2 \pi _{i}}{\partial a_{j}\partial a_{i}}> 0$ for each $j' \in \mathcal {I^{\prime \prime }}$, but $\frac{\partial ^2 \pi _{i}}{\partial a_{j}\partial a_{i}}< 0$ for each $j' \in \mathcal {I^{\prime }}$. More specifically, let $j,j' \in \mathcal {I^{\prime }}$ and $i,i' \in \mathcal {I^{\prime \prime }}$ be such that $i \not =i'$, and $j \not =j'$. Then, as defined in Eq. (3), we have that

$$\begin{aligned} D_{j,1}&= \frac{\partial ^2 P_l}{\partial g_1^2} \cdot \frac{\partial g_1}{\partial a_j} \ge 0,&D_{j,2}&= \frac{\partial ^2 \pi _l}{\partial g_2^2} \cdot \frac{\partial g_2}{\partial a_l} + \frac{\partial ^2 \pi _l}{\partial v \partial g_2} \cdot \frac{\partial v}{\partial a_l} \le 0, \\ D_{i,1}&=\frac{\partial ^2 P_c}{\partial g_1^2} \cdot \frac{\partial g_1}{\partial a_c} \le 0,&D_{i,2}&= 0. \end{aligned}$$

Hence, because $\frac{\mathrm{d}g_{1}}{\mathrm{d}a_{i}}=\frac{\mathrm{d}g_{2}}{\mathrm{d}a_{j}}>0$, $\frac{\mathrm{d}g_{1}}{\mathrm{d}a_{j}}<0$, and $\frac{\mathrm{d}g_{2}}{\mathrm{d}a_{i}}=0$, we have by Eq. 3 that

$$\begin{aligned} \frac{\partial ^2 u_i}{\partial a_{i'} \partial a_i}&=\frac{\mathrm{d}g_{1}}{\mathrm{d}a_{i}} \cdot D_{i,1} \le 0,&\frac{\partial ^2 u_i}{\partial a_{j} \partial a_i}&=\frac{\mathrm{d}g_{1}}{\mathrm{d}a_{1}} \cdot D_{i,1} \ge 0, \\ \frac{\partial ^2 u_j}{\partial a_{j'} \partial a_j}&=\frac{\mathrm{d}g_{1}}{\mathrm{d}a_{j'}} \cdot D_{j,1}+\frac{\mathrm{d}g_{2}}{\mathrm{d}a_{j'}} \cdot D_{j,2} \le 0,&\frac{\partial ^2 u_i}{\partial a_{i} \partial a_j}&=\frac{\mathrm{d}g_{1}}{\mathrm{d}a_{1}} \cdot D_{j,1} \ge 0. \end{aligned}$$

Therefore, we see that if we partition ${\mathcal {I}}$ into $\mathcal {I^\prime }$ and $\mathcal {I^{\prime \prime }}$, each member in each groups exhibits the pairwise deceasing SCP with other members of their own group and the pairwise SCP with members of the opposite group. Thus, by Proposition 2, this game can be transformed into a GSS.

Example 5

(Crime and social networks) Consider the model of Bramoullé et al. (2014), which consists of N criminals, where each criminal $i=1,\dots ,N$ chooses their crime level $a_i$ from some closed interval ${\mathcal {A}}_i\subset \mathbb {R}$. Crime is assumed to have a constant marginal cost of $c>0$. Also, returns from crime are decreasing in the overall crime level, as criminals compete among themselves for territory, resources, etc. Moreover, if criminal i and j are partners, criminal i’s cost of committing a crime decreases as criminal j engages in more crime, which models potential peer effects. Let $g_{i,j} \in \{0,1\}$ be such that $g_{i,j}=1$ if player i and j are partners, and 0 otherwise. Criminal i’s payoff is given by

$$\begin{aligned} \pi _i(a_i,a_{-i})=a_i\left( 1-\alpha \underset{j\in N}{\sum }a_j \right) -ca_i \left( 1- \phi \underset{j \in N}{\sum }g_{ij}a_j \right) , \end{aligned}$$

where $\alpha >0$ denotes the impact of crime and $\phi >0$ accounts for the impact of each partner’s level of crime. Note that

$$\begin{aligned} \frac{\partial ^2 \pi _{i}}{\partial a_i \partial a_j} ={\left\{ \begin{array}{ll} -\alpha &{} \quad \mathrm{if} \,\, g_{i,j}=0 \\ -\alpha +c \phi &{}\quad \mathrm{if} \,\, g_{i,j}=1.\\ \end{array}\right. } \end{aligned}$$

Because $\alpha >0$, we have that each $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$ for all criminals $j \not =i$ who are not partners with i. Hence, we have the following three cases:

• 1. If $\alpha >c \phi$, then each $\pi _{i}$ also satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$ for all criminals $j \not =i$ who are partners with i. Hence, by Lemma 1, the game is a GSS.

• 2. If $\alpha <c \phi$ and for each i and j, $g_{i,j}=g_{j,i}$, then $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$ for all criminals $j \not =i$ who are partners with i. Hence, by the discussion above, this game is a GMH, but not a GSH. However, notice that we can partition ${\mathcal {I}}$ into ${\mathcal {I}}'$ and ${\mathcal {I}}''$, where all criminals in a partitioning element are partners with one another. Hence, for each player i, $\pi _{i}$ exhibits the pairwise SCP $(a_{i}, a_{j})$ for all criminals $j \not =i$ who are in the same partitioning element as i, and the pairwise decreasing SCP in $(a_{i}, a_{j})$ for all criminals $j \not =i$ who are in the opposite partitioning element. By Proposition 2, this game can then be transformed into a GSC.

• 3. Suppose $\alpha <c \phi$ and that we have two types of criminals such that for all $j \ne i$, $g_{i,j}=1$ for each $i \in {\mathcal {I}}'$ and for all $j \ne i$, $g_{i,j}=0$ for each $i \in {\mathcal {I}}''$.¹⁴ Then $\pi _i$ satisfies the pairwise SCP in $(a_i, a_j)$ for all $j \ne i$ for all $i \in {\mathcal {I}}'$ and $\pi _i$ satisfies the pairwise decreasing SCP in $(a_i,a_j)$ for all $j \ne i$ for all $i \in {\mathcal {I}}''$. Intuitively, we have one type of criminal who is partners with everybody else and hence affects everybody’s optimal crime levels positively and another type of criminal who is not partners with anybody else and hence affects everybody’s optimal crime levels negatively. Similarly to the Cournot–Bertrand oligopoly example above, this game is a GMH, but not a GSH. However, with the given partition of ${\mathcal {I}}'$ and ${\mathcal {I}}''$, by Theorem 1, this game can be transformed into a GSH.

Hence, by Proposition 1, we know that there exist upper and lower serially undominated strategies, and they are dominance solvable if and only if there exists a unique equilibrium that is globally stable. Furthermore, in the case of when $\alpha <c \phi$, these upper and lower strategies can be guaranteed to be Nash equilibria.

Moreover, notice that this game a strict GMHD as stated. Thus, by Theorem 2, any complete order $\succeq ^{*}$ which transforms either game into a GSHD must be such that for each i, $\succeq _{i}^{*}$ is either the original order $\succeq _{i}$, or the reversed order ${\widetilde{\succeq }}_{i}$.

Example 6

It is a very natural question to ask whether the results of Theorem 2 can be extended to ordinal games that satisfy the (decreasing) SCP instead of (decreasing) increasing differences. To that end, consider the following example below:

Player 1’s action space is given by ${\mathcal {A}}_1 = \{L, H\}$ with $H \succeq _1 L$, while ${\mathcal {A}}_2 = \{L, M, H\}$ with $H \succeq _2 M \succeq _2 L$. It is straightforward to verify that payoffs for Players 1 and 2 satisfy the strict SCP and hence this game is a strict (ordinal) GSC. Now consider the order $\succeq ^*$, where $\succeq ^*_1 = \succeq _1$ and $\succeq ^*_2 = {\widetilde{\succeq }}_2$, which reverses Player 2’s strategy space. Hence, we now have $H \succeq ^*_1 L$ and $L \succeq ^*_2 M \succeq ^*_2 H$. Under this new ordering, both players’ payoffs now satisfy the decreasing SCP, and hence, this game is now a (ordinal) GSS. However, reversing one player’s strategy space is not the only order that allows us to transform the original GSC to a GSS. To see this, consider the order ${\hat{\succeq }} = ({\hat{\succeq }}_1, {\hat{\succeq }}_2)$ such that $H {\hat{\succeq }}_1 L$ and $M {\hat{\succeq }}_2 H {\hat{\succeq }}_2 L$. We can now verify that both players’ payoff functions satisfy the decreasing SCP, but not decreasing differences. This example illustrates that if we weaken the assumptions in Theorem 2 to their ordinal counterparts, there may exist other orders besides the reversal of some players’ strategy spaces that can transform a GSC into a GSS. The exact nature of these other orders that allow us to transform ordinal games remains an open question for further research.

Appendix

1.1 Proof of Lemma 1

Proof

It suffices to show that these hold when $\hat{\succeq _{i}}={\widetilde{\succeq }}_{i}$, as the result is immediate if $\hat{\succeq _{i}}=\succeq _{i}$. For part 1, let $i \in {\mathcal {I}}$. Let $S \subset {\mathcal {A}}_{i}$. Let $z \in {\mathcal {A}}_{i}$ be such that for all $s \in S$, $z \,\, {\widetilde{\succeq }}_{i} \,\, s$. Then for all $s \in S$, $s \succeq _{i} z$. Hence, $\mathrm{inf}_{\succeq _{i}}(S) \succeq _{i} z$, so that $z \,\, {\widetilde{\succeq }}_{i} \,\, \mathrm{inf}_{\succeq _{i}}(S)$. Since $\mathrm{inf}_{\succeq _{i}}(S) \,\, {\widetilde{\succeq }}_{i} \,\, s$ for all $s \in S$, this shows that a supremum of S exists under ${\widetilde{\succeq }}_{i}$, is in the set ${\mathcal {A}}_{i}$, and is equal to $\mathrm{inf}_{\succeq _{i}}(S)$. We can likewise show that $\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(S)$ exists and is equal to $\mathrm{sup}_{\succeq _{i}}(S)$.

We now show that $\pi _{i}$ still satisfies quasisupermodularity under ${\widetilde{\succeq }}_{i}$. We will suppress the $a_{-i}$ notion in $\pi _{i}$ for simplicity. Suppose that $x,y \in {\mathcal {A}}_{i}$ are such that $\pi _{i}(x) \ge \pi _{i}(\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y))$. Suppose by way of contradiction that $\pi _{i}(y)>\pi _{i}(\mathrm{sup}_{{\widetilde{\succeq }}_{i}}(x,y))$. Then, by the above observation, since $\mathrm{inf}_{{\succeq }_{i}}(x,y)=\mathrm{sup}_{{\widetilde{\succeq }}_{i}}(x,y)$, we have that $\pi _{i}(y)>\pi _{i}(\mathrm{inf}_{{\succeq }_{i}}(x,y))$. By quasisupermodularity under $\succeq _{i}$, this implies that $\pi _{i}(\mathrm{sup}_{{\succeq }_{i}}(x,y))>\pi (x)$. Once again, since $\mathrm{sup}_{{\succeq }_{i}}(x,y)=\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y)$, this implies $\pi _{i}(\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y))>\pi _{i}(x)$, a contradiction. Hence, $\pi _{i}(x) \ge \pi _{i}(\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y)) \Rightarrow \pi _{i}(\mathrm{sup}_{{\widetilde{\succeq }}_{i}}(x,y))\ge \pi _{i}(y)$.

Lastly, suppose that $x,y \in {\mathcal {A}}_{i}$ are such that $\pi _{i}(x)>\pi _{i}(\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y))$. Suppose by way of contradiction that $\pi _{i}(y) \ge \pi _{i}(\mathrm{sup}_{{\widetilde{\succeq }}_{i}}(x,y))$. Then, by the above observation, since $\mathrm{inf}_{{\succeq }_{i}}(x,y)=\mathrm{sup}_{{\widetilde{\succeq }}_{i}}(x,y)$, we have that $\pi _{i}(y)\ge \pi _{i}(\mathrm{inf}_{{\succeq }_{i}}(x,y))$. By quasisupermodularity under $\succeq _{i}$, this implies that

$\pi _{i}(\mathrm{sup}_{{\succeq }_{i}}(x,y)) \ge \pi _{i}(x)$. Once again, since $\mathrm{sup}_{{\succeq }_{i}}(x,y)=\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y)$, this implies $\pi _{i}(\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y)) \ge \pi _{i}(x)$, a contradiction. We therefore have that $\pi _{i}(x)>\pi _{i}(\mathrm{inf}_{{\widetilde{\succeq }}_{i}}(x,y)) \Rightarrow \pi _{i}(\mathrm{sup}_{{\widetilde{\succeq }}_{i}}(x,y))>\pi _{i}(y)$. This establishes quasisupermodularity under ${\widetilde{\succeq }}_{i}$.

To prove part 2, note that the interval topology on ${\mathcal {A}}$ is the topology whose closed sets are generated by the sub-basis of order intervals [a, b]. After reversing the orders of any collection of players, the set [a, b] under $\succeq$ will equal the order interval $[a',b']$ under ${\hat{\succeq }}$, where $a'_{i}=a_{i}$ and $b'_{i}=b_{i}$ if $\hat{\succeq _{i}}=\succeq _{i}$, and $a'_{i}=b_{i}$ and $b'_{i}=a_{i}$ if $\hat{\succeq _{i}}={\widetilde{\succeq }}_{i}$. Thus, both topologies will have the same closed and hence open sets, so that both topologies coincide.

To prove part 3, suppose that $i \in {\mathcal {I}}$ is such that for all $i \not =j$, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$. Endow ${\mathcal {A}}_{-i}$ with the product order $\succeq _{-i}$, and suppose that for $a'_{i}, a_{i} \in {\mathcal {A}}_{i}$ and $a'_{-i}, a_{-i} \in {\mathcal {A}}_{-i}$ such that $a'_{i} \succeq _{i} a_{i}$ and $a'_{-i} \succeq _{-i} a_{-i}$,

$$\begin{aligned} \pi _{i}(a'_{i}, a_{-i}) \ge \pi _{i}(a_{i}, a_{-i}). \end{aligned}$$

By the definition of the product order, $a'_{-i} \succeq _{-i} a_{-i}$ implies that for each $j \not =i$, $a'_{j} \succeq _{j} a_{j}$. Thus, because $\pi _{i}$ satisfies the pairwise SCP in each $(a_{i}, a_{j})$, we have that

$$\begin{aligned} \pi _{i}(a'_{i}, a'_{j}, a_{-i,j}) \ge \pi _{i}(a_{i}, a'_{j}, a_{-i,j}). \end{aligned}$$

This process can be repeated successively, so that we may conclude

$$\begin{aligned} \pi _{i}(a'_{i}, a'_{-i}) \ge \pi _{i}(a_{i}, a'_{-i}). \end{aligned}$$

Showing that $\pi _{i}(a'_{i}, a_{-i})>\pi _{i}(a_{i}, a_{-i}) \Rightarrow \pi _{i}(a'_{i}, a'_{-i})>\pi _{i}(a_{i}, a'_{-i})$ follows similarly, as do the cases for when $\pi _{i}$ satisfies the pairwise decreasing SCP. $\square$

1.2 Proof of Proposition 1

Proof

We apply Theorem 2 in Barthel and Hoffmann (2019) (BH) with one modification. First, for each player i, we must find a monotonic homeomorphism

$$\begin{aligned} f_{i}:({\mathcal {A}}_{i}, \succeq _{i}) \rightarrow (\widehat{{\mathcal {A}}}_{i}, {\widehat{\succeq }}_{i}). \end{aligned}$$

In the context of Proposition 1, notice that if for each player i, $f_{i}(a_{i})=a_{i}$ and $\widehat{{\mathcal {A}}}_{i}={\mathcal {A}}_{i}$, then $f_{i}$ is a monotone increasing or decreasing homeomorphism if we define ${\widehat{\succeq }}_{i}$ to be either $\succeq _{i}$ or the flipped order ${\widetilde{\succeq }}_{i}$ of $\succeq _{i}$, respectively.

Notice that in Theorem 2 in BH, it is assumed that the ${\mathcal {A}}_{i}$ are linearly ordered, which is used in two instances. We show that this assumption is not necessary in our setting. First, BH show that if $y_{i}$ and $z_{i}$ define upper and lower serially undominated strategies for player i, they continue to do so after the game is transformed into a GSH through the $f_{i}$. But notice that this holds automatically in our setting: If ${\widetilde{\succeq }}_{i}=\mathcal {\succeq }_{i}$, the result is immediate. Now suppose that ${\widehat{\succeq }}_{i}=\widetilde{\mathcal {\succeq }}_{i}$, and for all serially undominated strategies $a_{i} \in {\mathcal {A}}_{i}$, $z_{i} \succeq _{i} a_{i} \succeq _{i} y_{i}$. Then, by the definition of ${\widetilde{\succeq }}_{i}$, it is immediate that $y_{i} \succeq _{i} a_{i} \succeq _{i} z_{i}$. Hence, linearity is not required in our setting.

Second, BH use linearity to show that the $f_{i}$ preserve lattice operations.¹⁵ However, this is straightforward to check by the definition of ${\widetilde{\succeq }}_{i}$. Once again, the assumption of linearity is not necessary.

The last statement of Proposition 1 follows from Corollary 1 in BH. $\square$

1.3 Proof of Lemma 2

Proof

Let $S \subset {\mathcal {I}}$ be given. We show one case; the rest can be shown similarly. Suppose that $i \in S$, and $j \notin S$, and that $m_{j,i}=-1$, so that $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$ under $\succeq$. We claim that under ${\hat{\succeq }}$, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$. To that end, let $a'_{i}\,\, {\widetilde{\succeq }}_{i}\,\, a_{i}$, $a'_{j}\,\, {\widetilde{\succeq }}_{j} \,\,a_{j}$, and $a_{-i,j} \in {\mathcal {A}}_{-i,j}$ be given, and suppose that

$$\begin{aligned} \pi _{i}(a'_{i}, a_{j}, a_{-i,j}) \ge \pi _{i}(a_{i}, a_{j}, a_{-i,j}), \end{aligned}$$

and suppose by way of contradiction that

$$\begin{aligned} \pi _{i}(a_{i}, a'_{j}, a_{-i,j})>\pi _{i}(a'_{i}, a'_{j}, a_{-i,j}). \end{aligned}$$

Because $a'_{i}\,\, {\widetilde{\succeq }}_{i}\,\, a_{i}$, $a'_{j}\,\, {\widetilde{\succeq }}_{j} \,\,a_{j}$, then by definition, $a_{i}\succeq _{i} a'_{i}$ and $a'_{j} \succeq _{j} a_{j}$. Thus, by the contrapositive statement of $\pi _{i}$ satisfying the pairwise decreasing SCP under $\succeq$, we have that

$$\begin{aligned} \pi _{i}(a_{i}, a'_{j}, a_{-i,j})>\pi _{i}(a'_{i}, a'_{j}, a_{-i,j}) \Rightarrow \pi _{i}(a_{i}, a_{j}, a_{-i,j})>\pi _{i}(a'_{i}, a_{j}, a_{-i,j}), \end{aligned}$$

a contradiction.

Now suppose that

$$\begin{aligned} \pi _{i}(a'_{i}, a_{j}, a_{-i,j}) > \pi _{i}(a_{i}, a_{j}, a_{-i,j}), \end{aligned}$$

and by way of contradiction, suppose that

$$\begin{aligned} \pi _{i}(a_{i}, a'_{j}, a_{-i,j}) \ge \pi _{i}(a'_{i}, a'_{j}, a_{-i,j}). \end{aligned}$$

Once again, because $a_{i}\succeq _{i} a'_{i}$ and $a'_{j} \succeq _{j} a_{j}$, a direct application of the pairwise decreasing SCP yields

$$\begin{aligned} \pi _{i}(a_{i}, a_{j}, a_{-i,j})\ge \pi _{i}(a'_{i}, a_{j}, a_{-i,j}), \end{aligned}$$

a contradiction. Thus, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$ under ${\hat{\succeq }}$, so that if ${\mathcal {M}}_{\hat{{\mathcal {G}}}}$ has common element ${\hat{m}}_{j,i}$, then ${\hat{m}}_{j,i}=1=-m_{j,i}=T_{S}({\mathcal {M}}_{{\mathcal {G}}})_{j,i}$. The other cases follow similarly, completing the proof. $\square$

1.4 Proof of Theorem 1

Proof

First, suppose that ${\mathcal {G}}$ can be transformed into a GSH, so that for some non-empty $S \subset {\mathcal {I}}$, we have that

$$\begin{aligned} T_{S}({\mathcal {M}}_{{\mathcal {G}}})=J, \end{aligned}$$

where J is such that $J_{j,i}=0$ for all $i=j$, and for all $j\not =i$, $J_{j,i}=J_{i}$, where $J_{i}$ is either equal to 1 or $-1$. It is straightforward to check that for any matrix $A \in \mathbb {R}^{N \times N}$, and any $S \subset {\mathcal {I}}$, $T_{S}(T_{S}(A))=A$. Thus, one more application of $T_{S}$ to the equation above yields

$$\begin{aligned} {\mathcal {M}}_{{\mathcal {G}}}=T_{S}(J). \end{aligned}$$

Define ${\mathcal {I}}'=S$, and ${\mathcal {I}}''={\mathcal {I}}/S$. By the definition of $T_{S}$, we have that the diagonal elements of ${\mathcal {M}}_{{\mathcal {G}}}$ are zero, and that every off-diagonal element $m_{j,i}$ can be described as

$$\begin{aligned} m_{j,i}={\left\{ \begin{array}{ll} J_{i} &{} \quad i \in {\mathcal {I}}', j \in {\mathcal {I}}' \\ J_{i} &{} \quad i \in {\mathcal {I}}'', j \in {\mathcal {I}}'' \\ -J_{i} &{} \quad i \in {\mathcal {I}}', j \in {\mathcal {I}}'' \\ -J_{i} &{} \quad i \in {\mathcal {I}}'', j \in {\mathcal {I}}'. \\ \end{array}\right. } \end{aligned}$$

(4)

Suppose $i \in {\mathcal {I}}'$. Then, by Eq. (4), for all other $j \in {\mathcal {I}}'/\{i\}$, $m_{j,i}=J_{i}$. Hence, regardless of whether $J_{i}$ is equal either to 1 or $-1$, we see that for all $j \in {\mathcal {I}}'/\{i\}$, $\pi _{i}$ satisfies either the pairwise SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}'/ \{i\}$ or the pairwise decreasing SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}'/ \{i\}$. Also, for the same $i \in {\mathcal {I}}'$, by Eq. (4) we have that $m_{j,i}=-J_{i}$ for all $j \in {\mathcal {I}}''$. Hence, regardless of whether $J_{i}$ is equal to 1 or $-1$, we see that for all $j \in {\mathcal {I}}''$, $\pi _{i}$ satisfies either the pairwise SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}''$ or the pairwise decreasing SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}''$. Lastly, we see that for all $j \in {\mathcal {I}}'/\{i\}$ and all $j' \in {\mathcal {I}}''$, $m_{j,i}=-m_{j',i}$. Because the same arguments can be made for $i \in {\mathcal {I}}''$, we have proved the first implication.

Conversely, suppose that ${\mathcal {I}}$ can be partitioned into ${\mathcal {I}}'$ and ${\mathcal {I}}''$ in the manner described in the statement of the hypothesis, so that a typical off-diagonal element $m_{j,i}$ of ${\mathcal {M}}_{{\mathcal {G}}}$ can be described by Eq. (4), where all diagonal elements are zero. Then, by the definition of $T_{{\mathcal {I}}'}$, a typical off-diagonal element $T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})_{j,i}$ of $T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})$ can be written as

$$\begin{aligned} T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})_{j,i}={\left\{ \begin{array}{ll} J_{i} &{} \quad i \in {\mathcal {I}}', j \in {\mathcal {I}}' \\ J_{i} &{} \quad i \notin {\mathcal {I}}', j \notin {\mathcal {I}}' \\ J_{i} &{} \quad i \in {\mathcal {I}}', j \notin {\mathcal {I}}' \\ J_{i} &{} \quad i \notin {\mathcal {I}}', j \in {\mathcal {I}}'. \\ \end{array}\right. } \end{aligned}$$

That is, for all $i \in {\mathcal {I}}$, and all $j \in {\mathcal {I}}'/ \{i\}$, $T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})_{j,i}=J_{i}$, so that $\pi _{i}$ satisfies the pairwise SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}/ \{i\}$, or the pairwise decreasing SCP $(a_{i}, a_{j})$ for each $j \in {\mathcal {I}}/ \{i\}$. Because ${\mathcal {I}}'$ is non-empty and not equal to ${\mathcal {I}}$ by assumption, this completes the proof. $\square$

1.5 Proof of Proposition 2

Proof

For part 1, first suppose that ${\mathcal {G}}$ can be transformed into a GSC, so that for some $S \subset {\mathcal {I}}$, we have that

$$\begin{aligned} T_{S}({\mathcal {M}}_{{\mathcal {G}}})=J, \end{aligned}$$

where J is such that $J_{j,i}=1$ for all $j \not =i$, and $J_{j,i}=0$ for all $j=i$. As previously mentioned, for any matrix $A \in \mathbb {R}^{N \times N}$, and any $S \subset {\mathcal {I}}$, we have that $T_{S}(T_{S}(A))=A$. Thus, one more application of $T_{S}$ to the equation above yields

$$\begin{aligned} {\mathcal {M}}_{{\mathcal {G}}}=T_{S}(J). \end{aligned}$$

Define ${\mathcal {I}}'=S$, and ${\mathcal {I}}''={\mathcal {I}}/S$. By the definition of $T_{S}$, we have that the diagonal elements of ${\mathcal {M}}_{{\mathcal {G}}}$ are zero, and that every off-diagonal element $m_{j,i}$ can be described as

$$\begin{aligned} m_{j,i}={\left\{ \begin{array}{ll} 1 &{} \quad i \in {\mathcal {I}}', j \in {\mathcal {I}}' \\ 1 &{} \quad i \in {\mathcal {I}}'', j \in {\mathcal {I}}'' \\ -1 &{} \quad i \in {\mathcal {I}}', j \in {\mathcal {I}}'' \\ -1 &{} \quad i \in {\mathcal {I}}'', j \in {\mathcal {I}}', \\ \end{array}\right. } \end{aligned}$$

(5)

proving the first direction.

Conversely, suppose that ${\mathcal {I}}$ can be partitioned into ${\mathcal {I}}'$ and ${\mathcal {I}}''$ in the manner described in the statement of the proposition, so that a typical off-diagonal element $m_{j,i}$ of ${\mathcal {M}}_{{\mathcal {G}}}$ can be described by Eq. (5), where all diagonal elements are zero. Then, by the definition of $T_{{\mathcal {I}}'}$, a typical off-diagonal element $T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})_{j,i}$ of $T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})$ can be written as

$$\begin{aligned} T_{{\mathcal {I}}'}({\mathcal {M}}_{{\mathcal {G}}})_{j,i}={\left\{ \begin{array}{ll} 1 &{} \quad i \in {\mathcal {I}}', j \in {\mathcal {I}}' \\ 1 &{} \quad i \notin {\mathcal {I}}', j \notin {\mathcal {I}}' \\ 1 &{} \quad i \in {\mathcal {I}}', j \notin {\mathcal {I}}' \\ 1 &{} \quad i \notin {\mathcal {I}}', j \in {\mathcal {I}}'. \\ \end{array}\right. } \end{aligned}$$

Because ${\mathcal {I}}'$ is non-empty and not equal to ${\mathcal {I}}$, this gives the result. A similar argument proves part 2.$\square$

1.6 Proof of Corollary 1

Proof

We prove the first statement, where the other follows by similar arguments. First, suppose that $N=2$, and that ${\mathcal {G}}$ can be transformed into a GSC. If, for some player i, $\pi _{i}$ satisfies the SCP in $(a_{i}, a_{j})$ for $j \not =i$, then no non-trivial partitioning of ${\mathcal {I}}$ satisfying the requirements of Proposition 2 can be established, a contradiction. Hence, each $\pi _{i}$ satisfies the decreasing SCP in $(a_{i}, a_{j})$, for $j \not =i$. The converse holds immediately by flipping the order on the strategy space of one of the two players.

Now suppose that $N>2$ and that ${\mathcal {G}}$ can be transformed into a GSC. First observe that since ${\mathcal {G}}$ is a GSH, ${\mathcal {I}}$ can be partitioned into two sets ${\mathcal {I}}_{C}$ and ${\mathcal {I}}_{S}$ such that for each $i \in {\mathcal {I}}_{C}$, $\pi _{i}$ satisfies the pairwise SCP in $(a_{i}, a_{j})$, for $j \not =i$, and for each $i \in {\mathcal {I}}_{S}$, $\pi _{i}$ satisfies the pairwise decreasing SCP in $(a_{i}, a_{j})$, for $j \not =i$. If ${\mathcal {I}}_{C}={\mathcal {I}}$, the fact that ${\mathcal {G}}$ is not a GSC to begin with is contradicted. If ${\mathcal {I}}_{S}={\mathcal {I}}$, then it is immediate that no partitioning of ${\mathcal {I}}$ satisfying the requirements of Proposition 2 can be established. Thus, both ${\mathcal {I}}_{C}$ and ${\mathcal {I}}_{S}$ are non-empty and not equal to ${\mathcal {I}}$. Consider any two $i \in {\mathcal {I}}_{C}$ and $j \in {\mathcal {I}}_{S}$. Notice that in any non-trivial partitioning $\{ {\mathcal {I}}', {\mathcal {I}}''\}$ of ${\mathcal {I}}$, the requirements of Proposition 2 are contradicted whether i and j are put in the same partitioning elements, or separate partitioning elements, establishing the result. $\square$

1.7 Proof of Theorem 2

Proof

Because for each j, $\succeq _{j}$ and $\succeq ^{*}_{j}$ are partial orders, it follows from ${\mathcal {A}}^{\succeq ^{*}}_{j}={\mathcal {A}}^{\succeq }_{j}$ that any two distinct elements in ${\mathcal {A}}_{j}$ are either unordered under both $\succeq _{j}$ and $\succeq ^{*}_{j}$, or strictly ordered under both $\succeq _{j}$ and $\succeq ^{*}_{j}$. Therefore, we must only concentrate on pairs of elements which are strictly ordered.

To that end, notice that the result holds trivially if for each player j, ${\mathcal {A}}_{j}$ has only one ordered pair. Suppose that for some player j, ${\mathcal {A}}_{j}$ has more than one ordered pair, and consider any other opponent $i \not =j$. In order to prove part 1, first consider the case where in the game ${\mathcal {G}}$, $\pi _{i}$ exhibits pairwise increasing differences in $(a_{i}, a_{j})$ under $\succeq$, and strict pairwise decreasing differences in $(a_{i}, a_{j})$ under $\succeq ^{*}$. Let $a_{-i,j} \in {\mathcal {A}}_{-i,j}$ be given, and consider $a'_{i} \succeq ^{*}_{i} a_{i}$ and $a'_{j} \succeq ^{*}_{j} a_{j}$ such that $a'_{i} \not = a_{i}$, $a'_{j} \not = a_{j}$. Then, by the definition of strict pairwise decreasing differences, we have that

$$\begin{aligned} \pi _{i}(a_{i}, a'_{j}, a_{-i,j})-\pi _{i}(a'_{i}, a'_{j}, a_{-i,j})>\pi _{i}(a_{i}, a_{j}, a_{-i,j})-\pi _{i}(a'_{i}, a_{j}, a_{-i,j}). \end{aligned}$$

(6)

Because $a'_{i} \succeq ^{*}_{i} a_{i}$, we have that $(a_{i}, a'_{i}) \in {\mathcal {A}}^{\succeq ^{*}}_{i}={\mathcal {A}}^{\succeq }_{i}$, so that $a'_{i} \succeq _{i} a_{i}$ or $a_{i} \succeq _{i} a'_{i}$. Suppose $a'_{i} \succeq _{i} a_{i}$ without loss of generality. Likewise, $a'_{j} \succeq _{j} a_{j}$ or $a_{j} \succeq _{j} a'_{j}$. Suppose that $a'_{j} \succeq _{j} a_{j}$. Notice that Eq. (6) can be re-arranged to give

$$\begin{aligned} \pi _{i}(a'_{i}, a_{j}, a_{-i,j})-\pi _{i}(a_{i}, a_{j}, a_{-i,j}) > \pi _{i}(a'_{i}, a'_{j}, a_{-i,j})-\pi _{i}(a_{i}, a'_{j}, a_{-i,j}). \end{aligned}$$

However, this contradicts the fact that $\pi _{i}$ exhibits pairwise increasing differences in $(a_{i}, a_{j})$ under $\succeq$, which would require

$$\begin{aligned} \pi _{i}(a'_{i}, a'_{j}, a_{-i,j})-\pi _{i}(a_{i}, a'_{j}, a_{-i,j}) \ge \pi _{i}(a'_{i}, a_{j}, a_{-i,j})-\pi _{i}(a_{i}, a_{j}, a_{-i,j}). \end{aligned}$$

Therefore, we must have that $a_{j} \succeq _{j} a'_{j}$. Hence, we can conclude that for all $a'_{j},a_{j} \in {\mathcal {A}}_{j}$ such that $a'_{j} \not = a_{j}$, $a'_{j} \succeq ^{*}_{j} a_{j} \Rightarrow a_{j} \succeq _{j} a'_{j}$.¹⁶

We now show that for all $a'_{j},a_{j} \in {\mathcal {A}}_{j}$ such that $a'_{j} \not = a_{j}$, $a'_{j} \succeq _{j} a_{j} \Rightarrow a_{j} \succeq ^{*}_{j} a'_{j}$. Suppose that $a'_{j} \not = a_{j}$ and $a'_{j} \succeq _{j} a_{j}$, but it is not the case that $a_{j} \succeq ^{*}_{j} a'_{j}$. Because $(a_{j}, a'_{j}) \in {\mathcal {A}}^{\succeq }_{j}={\mathcal {A}}^{\succeq ^{*}}_{j}$, then if $a_{j} \succeq ^{*}_{j} a'_{j}$ is not true, we must have $a'_{j} \succeq ^{*}_{j} a_{j}$. However, by the above result, this implies $a_{j} \succeq _{j} a'_{j}$. But since $a'_{j} \not = a_{j}$, this contradicts $a'_{j} \succeq _{j} a_{j}$, giving the result. Because this argument can be done for any player j to conclude that either $\succeq ^{*}_{j}={\widetilde{\succeq }}_{j}$ or $\succeq ^{*}_{j}=\succeq _{j}$, we have that $\succeq ^{*} \in R^{\succeq }$.

The case of when $\pi _{i}$ exhibits either pairwise increasing or decreasing differences in $(a_{i}, a_{j})$ under $\succeq$, and strict increasing or decreasing differences in $(a_{i}, a_{j})$ under $\succeq ^{*}$, can be done similarly. This proves part (1). The case of when ${\mathcal {G}}$ is a strict GMHD and ${\mathcal {G}}^{*}$ is a GSHD can be done similarly, proving part (2). $\square$