Introduction

As the use of time-dependent composite materials increases in industries, the use of viscoelastic theories has been increasing recently. Due to the damping property of viscoelastic materials, the dynamic analysis of viscoelastic plates subjected to harmonic load is one of the most interesting problems in the structural vibration field. Vibration control is needed to control noise in engineering systems and to reduce vibration levels. Wang and Tsai [1] employed the finite element method to analyze the quasi-static and dynamic responses of linear viscoelastic plates with constant Poisson’s ratio. Ilyasov and Akoz [2] considered the static and dynamic behavior of simply supported viscoelastic triangular plates subjected to static and dynamic loads employing the Boltzmann–Volterra principle. Eshmatov [3] studied the geometrically nonlinear vibration and dynamic stability analysis of viscoelastic orthotropic rectangular plates based on the Kirchhoff–Love hypothesis and Reissner–Mindlin generalized theory. Abdoun et al. [4] investigated the asymptotic numerical method for the forced harmonic vibration analysis of viscoelastic structures. Gupta et al. [5] presented the vibration analysis of clamped viscoelastic rectangular plates with varying thickness, linearly in one and parabolically in other direction. Li and Cheng [6] studied the dynamic behavior of viscoelastic plates by considering higher-order shear effects and finite deformations. Shariyat [7] investigated the free vibration and dynamic buckling analyses of viscoelastic composite sandwich plates subjected to thermomechanical loads based on the double-superposition global–local theory. Mahmoudkhani et al. [8] considered the free and forced vibration analysis of sandwich plates with thick viscoelastic cores under wide-band random excitation. Temel and Sahan [9] studied the transient behavior of orthotropic viscoelastic thick plates under dynamic loads using the Laplace transformation. Amabili [10] investigated the nonlinear vibration analysis of viscoelastic rectangular plates using the Von Karman assumptions and Kelvin–Voigt solids. Wan and Zheng [11] considered the vibration and damping analysis of the five-layered constrained damping plates based on the theory of Donnell using the complex constant model. Khadem Moshir et al. [12] studied the free vibration analysis of moderately thick annular viscoelastic plates based on the perturbation technique. Amabili [13] presented the nonlinear vibration analysis of viscoelastic rectangular plates derivation from viscoelasticity and experimental validation. Balasubramanian et al. [14] studied the geometrically nonlinear vibration analysis of rubber viscoelastic rectangular plates with fixed edges and identified the increase of damping with the vibration amplitude, experimentally and numerically. Zhou and Wang [15] studied the transverse vibration and dynamic stability of the axially moving viscoelastic plates based on the Kelvin–Voigt model. Rouzegar and Davoudi [16] investigated the forced vibration analysis of smart laminated viscoelastic composite plates integrated with a piezoelectric layer using the RPT finite element approach. Silva et al. [17] presented the uncertainty propagation and numerical evaluation for the nonlinear dynamic analysis of viscoelastic sandwich plates by performing the Karhunen–Loeve expansion technique. Sofiyev et al. [18] considered the free vibration and dynamic stability analyses of functionally graded viscoelastic plates subjected to compressive load and resting on elastic foundations. Ojha and Dwivedy [19] studied the dynamic analysis of a three-layered sandwich plate with thin composite layers and LPRE-based viscoelastic core. Amabili et al. [20] considered the nonlinear vibrations and nonlinear damping of fractional viscoelastic rectangular plates using a fractional linear solid model, experimentally and numerically. Zamani [21] investigated the free vibration analysis of viscoelastic plates employing single-term Bubnov–Galerkin, least squares, and point collocation methods. Jafari and Azhari [22] presented the free vibration analysis of moderately thick viscoelastic plates based on the free vibration analysis of elastic plates. Jafari [23] investigated the non-harmonic resonance of Mindlin viscoelastic plates subjected to time-dependent decreasing exponential transversal distributed loads. Jafari and Azhari [24] studied the dynamic stability analysis of moderately thick viscoelastic plates subjected to constant and harmonic in-plane compression based on the free vibration analysis of elastic plates.

Due to the difficulty and complexity of the equations, there are few articles on the forced vibration analysis of moderately thick viscoelastic plates. Also, there are not any closed-form solutions for the total dynamic responses of Mindlin viscoelastic plates subjected to harmonic transversal load and in-plane compression, simultaneously. The present paper investigates the forced vibration analysis of moderately thick viscoelastic plates under harmonic transversal and constant in-plane compressive loads, simultaneously. The excitation frequency of the harmonic load is investigated as equal to the natural frequency. The stress–strain relation is written based on the Boltzmann superposition principle with constant bulk modulus for linear viscoelastic materials. The displacement field is approximated using the separation of variables technique by the product of a known geometrical function and an unknown time function. The Laplace transform is employed to convert equations from the time domain to the Laplace domain. The simple hp cloud method is employed for discretization. Calculating the natural and viscous damping frequencies, geometry and mass matrices, stiffness matrix in the Laplace–Carson domain, and finding the best values to replace the Laplace parameter, the unknown coefficients of the time function are determined. Finally, a closed-form solution is extracted while the transient, steady-state and total dynamic responses of Mindlin viscoelastic plates under out-of-plane and in-plane loadings are explicitly formulated in the time domain for the first time.

This paper is organized as follows: The extraction of equations of forced vibration analysis of moderately thick viscoelastic plates is described in Sect. 2. The numerical results are presented in Sect. 3. Section 4 presents the conclusions. The extraction of equations of forced vibration analysis of Bernoulli viscoelastic beams is described in Appendix 1.

Governing Equations

Kinematic Relations

The time-dependent displacement field is defined using the first-order shear deformation theory as:

$$\left\{\begin{array}{c}u\left(x\text{,}y\text{,}z\text{,}t\right)\\ v\left(x\text{,}y\text{,}z\text{,}t\right)\\ w\left(x\text{,}y\text{,}z\text{,}t\right)\end{array}\right\}=\left\{\begin{array}{c}{u}_{0}(x\text{,}y\text{,}t)-z{\theta }_{x}(x\text{,}y\text{,}t)\\ {v}_{0}(x\text{,}y\text{,}t)-z{\theta }_{y}(x\text{,}y\text{,}t)\\ w\left(x\text{,}y\text{,}t\right)\end{array}\right\},$$
(1)

in which \({\theta }_{x}\) and \({\theta }_{y}\) denote the rotations around \(y\) and \(x\) axes, respectively. \({u}_{0}\) and \({v}_{0}\) are the in-plane displacements and \(w\left(x\text{,}y\text{,}t\right)\) is an out-of-plane displacement of the middle surface.

According to the small deformation theory, the in-plane and out-of-plane solutions of a plate are uncoupled [25]. So, assuming the in-plane compressions to be less than the critical buckling load, the in-plane displacements of the middle surface can be removed for out-of-plane analysis.

Omitting the in-plane displacements, the strain–displacement relations can be given as follows:

$${\varvec{\upvarepsilon}}\left(x\text{,}y\text{,}z\text{,}t\right)=\left\{\begin{array}{c}-z{\theta }_{x\text{,}x}\\ -z{\theta }_{y\text{,}y}\\ -z({\theta }_{x\text{,}y}+{\theta }_{y\text{,}x})\\ {w}_{\text{,}x}-{\theta }_{x}\\ {w}_{\text{,}y}-{\theta }_{y}\end{array}\right\}.$$
(2)

Constitutive Equations

According to the Boltzmann superposition principle, the stress–strain relations of a linear viscoelasticity can be written as [26]:

$${\varvec{\upsigma}}\left(x\text{,}y\text{,}z\text{,}t\right)=\mathbf{C}\left(t\right){\varvec{\upvarepsilon}}\left(x\text{,}y\text{,}z\text{,}0\right)+{\int }_{0}^{t}\mathbf{C}\left(t-\tau \right)\dot{{\varvec{\upvarepsilon}}}\left(x\text{,}y\text{,}z\text{,}\tau \right)d\tau ,$$
(3)

where \(\mathbf{C}(t)\) is the time-dependent relaxed modulus tensor, \(\dot{{\varvec{\upvarepsilon}}}(t)=\partial {\varvec{\upvarepsilon}}/\partial t\), and the stress vector \({\varvec{\upsigma}}\left(x\text{,}y\text{,}z\text{,}t\right)\) is defined as:

$${\varvec{\upsigma}}\left(x\text{,}y\text{,}z\text{,}t\right)={\langle \begin{array}{cc}\begin{array}{ccc}{\upsigma }_{x}& {\upsigma }_{y}& {\tau }_{xy}\end{array}& \begin{array}{cc}{\tau }_{xz}& {\tau }_{yz}\end{array}\end{array}\rangle }^{T}.$$
(4)

For isotropic materials, Eqs. (5–6) are always valid:

$$K\left(t\right)=\frac{E(t)}{3(1-2\upnu \left(t\right))},$$
(5)
$$G\left(t\right)= \frac{E\left(t\right)}{2\left(1+\upnu \left(t\right)\right)},$$
(6)

where \(K\left(t\right)\), \(G\left(t\right)\) and \(E\left(t\right)\) are the bulk, shear, and elastic moduli and \(\upnu \left(t\right)\) is the Poisson ratio.

Assuming the constant bulk modulus:

$$K\left(t\right)=K .$$
(7)

Substituting Eqs. (5) and (7) into Eq. (6), Eq. (8) is obtained:

$$G\left(t\right)=\frac{3K}{2} \frac{(1-2\upnu \left(t\right))}{(1+\upnu \left(t\right))}={G}_{0}\eta \left(t\right), {G}_{0}=\frac{3K}{2}, \eta \left(t\right)=\frac{\left(1-2\upnu \left(t\right)\right)}{(1+\upnu \left(t\right))},$$
(8)

where the dimensionless relaxation function of a viscoelastic material can be defined by three coefficients as follows [22, 24, 27]:

$$\eta \left(t\right)={c}_{1}+{c}_{2}\mathit{exp}\left(-\uplambda t\right),\uplambda =1/{t}_{s}.$$
(9)

\({c}_{1}\) and \({c}_{2}\) are constant parameters and \({t}_{s}\) is the relaxation time of a viscoelastic material.

Substituting Eqs. (7–9) into Eqs. (5–6), the time-dependent elastic modulus and the time-dependent Poisson ratio can be given as:

$$E\left(t\right)=\frac{9K\eta \left(t\right)}{2+\eta \left(t\right)},$$
(10)
$$\upnu \left(t\right)=\frac{1-\eta \left(t\right)}{2+\eta \left(t\right)}.$$
(11)

Equation of Motion

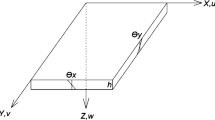
The equilibrium equation of a moderately thick viscoelastic plate subjected to harmonic transversal loading \(q\left(t\right)\), and in-plane compressive forces \({N}_{x}\) and \({N}_{y}\), as illustrated in Fig. 1, can be stated as:

Fig. 1
figure 1

A viscoelastic plate subjected to harmonic transversal load and in-plane compressions

Full size image
$$\delta U-\delta {V}_{1}-\delta {V}_{2}-\delta T=0.$$
(12)

The variations of the strain, \(\delta U\), potentials, \(\delta {V}_{1}\) and \(\delta {V}_{2}\), and kinetic, \(\delta T\), energies are written as:

$$\delta U={\int }_{V}\left({{\varvec{\upvarepsilon}}\left(0\right)}^{T}\mathbf{C}\left(t\right)+{\int }_{0}^{t}{\dot{{\varvec{\upvarepsilon}}}\left(\tau \right)}^{T}\mathbf{C}\left(t-\tau \right)d\tau \right)\delta {\varvec{\upvarepsilon}}dV,$$
(13)
$$\delta {V}_{1}={\int }_{A}\left[{N}_{x}\frac{\partial w}{\partial x}\frac{\partial \delta w}{\partial x}+{N}_{y}\frac{\partial w}{\partial y}\frac{\partial \delta w}{\partial y}\right]dA,$$
(14)
$$\delta {V}_{2}={\int }_{A}q\left(t\right)\delta wdA,$$
(15)
$$\delta T=-{\int }_{V}\rho \left[\frac{{\partial }^{2}u}{\partial {t}^{2}}\delta u+\frac{{\partial }^{2}v}{\partial {t}^{2}}\delta v+\frac{{\partial }^{2}w}{\partial {t}^{2}}\delta w\right]dV,$$
(16)

where \(\rho \) is the plate density.

Separation of Variables

The displacement vector can be approximated using the separation of variables method as follows:

$$\mathbf{u}\left(x\text{,}y\text{,}t\right)=\left\{\begin{array}{c}w\left(x\text{,}y\text{,}t\right)\\ {\theta }_{x}\left(x\text{,}y\text{,}t\right)\\ {\theta }_{y}\left(x\text{,}y\text{,}t\right)\end{array}\right\}=\left\{\begin{array}{c}w\left(x\text{,}y,t=0\right)\\ {\theta }_{x}\left(x\text{,}y,t=0\right)\\ {\theta }_{y}\left(x\text{,}y,t=0\right)\end{array}\right\}F\left(t\right)={\mathbf{u}}^{0}F\left(t\right), {\mathbf{u}}^{0}=\mathbf{u}\left(x\text{,}y\text{,}t=0\right).$$
(17)

The variation and the rate of displacement vector can be given as:

$$\delta \mathbf{u}\left(x\text{,}y\text{,}t\right)={\mathbf{u}}^{0}\delta F,$$
(18)
$$\dot{\mathbf{u}}\left(x\text{,}y\text{,}t\right)={\mathbf{u}}^{0}\dot{F}\left(t\right).$$
(19)

Using Eqs. (17–19), the strain vector, the variation of strain vector, the rate of strain vector, and the variation of out-of-plane displacement are obtained as:

$${\varvec{\upvarepsilon}}\left(x\text{,}y\text{,}z\text{,}t\right)={\varvec{\upvarepsilon}}\left(x\text{,}y\text{,}z\text{,}t=0\right)F\left(t\right)={{\varvec{\upvarepsilon}}}^{0}F\left(t\right),$$
(20)
$$\delta {\varvec{\upvarepsilon}}={{\varvec{\upvarepsilon}}}^{0}\delta F,$$
(21)
$$\dot{{\varvec{\upvarepsilon}}}={{\varvec{\upvarepsilon}}}^{0}\dot{F}(t),$$
(22)
$$\delta w={w}^{0}\delta F.$$
(23)

Discretization

There are several numerical methods for discretizing equations in the space domain. In this study, the simple hp cloud method is utilized, [27].

The displacement vector can be discretized as:

$${\mathbf{u}}^{0}=\mathbf{N}{\mathbf{U}}^{0}, {\mathbf{U}}^{0}=\left[\begin{array}{ccc}{{\mathbf{U}}_{1}}^{T}& {{\mathbf{U}}_{2}}^{T}& ..\end{array} \begin{array}{cc}..& {{\mathbf{U}}_{N}}^{T}\end{array}\right],$$
(24)

in which:

$${\mathbf{U}}_{i}={\langle \begin{array}{ccc}{w}_{i}& {\theta }_{{x}_{i}}& {\theta }_{{y}_{i}}\end{array}\rangle }^{T}$$
(25)

and \(\mathbf{N}\) is the vector of basis functions. More details exist in Appendix 2.

Substituting Eqs. (17–25) into Eqs. (13–16), Eqs. (26–29) are derived:

$$\delta U={\int }_{V}{{\mathbf{U}}^{0}}^{T}{\mathbf{B}}^{T}F(0)\mathbf{C}\left(t\right)\mathbf{B}{\mathbf{U}}^{0}\delta FdV+{\int }_{V}{\int }_{0}^{t}{{\mathbf{U}}^{0}}^{T}{\mathbf{B}}^{T}\dot{F}(\tau )\mathbf{C}\left(t-\tau \right)d\tau \mathbf{B}{\mathbf{U}}^{0}\delta FdV,$$
(26)
$$\delta {V}_{1}={\int }_{A}{{\mathbf{U}}^{0}}^{T}{\mathbf{B}}_{G}^{T}F(t){\mathbf{N}}_{p}{\mathbf{B}}_{G}{\mathbf{U}}^{0}\delta FdA,$$
(27)
$$\delta {V}_{2}={\int }_{A}q(t){\mathbf{N}}_{w}{\mathbf{U}}^{0}\delta FdA,$$
(28)
$$\delta T=-{\int }_{V}{{\mathbf{U}}^{0}}^{T}{\mathbf{N}}^{T}{\mathbf{C}}_{m}\ddot{F}\left(t\right)\mathbf{N}{\mathbf{U}}^{0}\delta F\mathrm{d}V.$$
(29)

The strain–displacement transformation matrices \(\mathbf{B}\) and \({\mathbf{B}}_{G}\) are introduced in Sect. 2.9 and the matrix of in-plane compressive forces \({\mathbf{N}}_{p}\) is defined as follows:

$${\mathbf{N}}_{p}=\left[\begin{array}{cc}{N}_{x}& 0\\ 0& {N}_{y}\end{array}\right]={\alpha }_{1}{N}_{cr}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right], {k}_{1}={N}_{y}/{N}_{x}\text{,} 0\le {\alpha }_{1}<1.$$
(30)

\({N}_{cr}\) is the critical compressive load of a viscoelastic plate at time zero and \({\alpha }_{1}\) is an arbitrary constant coefficient.

Harmonic Transversal Load

For Mindlin viscoelastic plates, \(\Omega \) is defined as the fundamental natural frequency calculated by the free vibration analysis of moderately thick viscoelastic plates at time zero [22]. If the plate is subjected to in-plane compression too, the natural frequency \({\omega }_{0}\) is decreased \({\omega }_{0}\cong\Omega \sqrt{1-{\alpha }_{1}}\),\(0\le {\alpha }_{1}<1\), [24]. In this paper, the plate is subjected to harmonic transversal load \(q\left(t\right)=q\mathrm{sin}{\omega }_{0}t\) and in-plane compressions, simultaneously. In other word, the excitation frequency is considered as equal to the natural frequency and the time-dependent behavior of the viscoelastic plate is studied.

Integrating over the Thickness

By integrating over the thickness of the plate, Eq. (26) can be rewritten as:

$$\delta U={\int }_{A}{{\mathbf{U}}^{0}}^{T}{\mathbf{B}}^{T}F(0)\mathbf{D}\left(t\right)\mathbf{B}{\mathbf{U}}^{0}dA\delta F+{\int }_{A}{\int }_{0}^{t}{{\mathbf{U}}^{0}}^{T}{\mathbf{B}}^{T}\dot{F}(\tau )\mathbf{D}\left(t-\tau \right)d\tau \mathbf{B}{\mathbf{U}}^{0}dA\delta F,$$
(31)

in which the time-dependent effective modulus tensor can be stated as:

$$\mathbf{D}\left(t\right)=\left[\begin{array}{cc}\frac{E\left(t\right){h}^{3}}{12\left(1-{\nu \left(t\right)}^{2}\right)}\left[ \begin{array}{ccc}1& \nu \left(t\right)& 0\\ \nu \left(t\right)& 1& 0\\ 0& 0& \frac{1-\nu \left(t\right)}{2}\end{array}\right]& 0\\ 0& \frac{E\left(t\right)hk}{2\left(1+\nu \left(t\right)\right)}\left[\begin{array}{cc} 1& 0\\ 0& 1\end{array}\right]\end{array}\right],$$
(32)

\(h\) is the plate thickness and \(k\) is the shear correction factor of the first-order shear deformation theory.

Also, by integrating over the thickness of the plate, Eq. (29) can be stated as:

$$\delta T=-{\int }_{A}{{\mathbf{U}}^{0}}^{T}{\mathbf{N}}^{T}\ddot{F}\left(t\right){\mathbf{D}}_{m}\mathbf{N}{\mathbf{U}}^{0}dA\delta F,$$
(33)

where the mass density matrix, \({\mathbf{D}}_{m}\), can be expressed as:

$${\mathbf{D}}_{m}=\rho \left[\begin{array}{ccc}h& 0& 0\\ 0& \frac{{h}^{3}}{12}& 0\\ 0& 0& \frac{{h}^{3}}{12}\end{array}\right].$$
(34)

Substituting Eqs. (27–28, 31, 33) into Eq. (12), Eq. (35) is derived:

$$\left\{{\int }_{A}{{\mathrm{U}}^{0}}^{T}{\mathrm{B}}^{T}\left(F\left(0\right)\mathrm{D}\left(t\right)+{\int }_{0}^{t}\dot{F}(\tau )\mathrm{D}\left(t-\tau \right)d\tau \right){\mathrm{BU}}^{0}dA-{\alpha }_{1}{N}_{cr}{\int }_{A}{{\mathrm{U}}^{0}}^{T}{\mathrm{B}}_{G}^{T} F\left(t\right)\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathrm{B}}_{G}{\mathrm{U}}^{0}dA+{\int }_{A}{{\mathrm{U}}^{0}}^{T}{\mathrm{N}}^{T}\ddot{F}(t){\mathrm{D}}_{m}{\mathrm{NU}}^{0}dA\right\}\delta F=\mathit{sin}{\omega }_{0}t{\int }_{A}q{\mathrm{N}}_{w}{\mathrm{U}}^{0}dA\delta F$$
(35)

Removing \(\delta F\) from Eq. (35), Eq. (36) is derived:

$${{\mathbf{U}}^{0}}^{T}\left\{{\int }_{A}{\mathbf{B}}^{T}\left(F\left(0\right)\mathbf{D}\left(t\right)+{\int }_{0}^{t}\dot{F}(\tau )\mathbf{D}\left(t-\tau \right)d\tau \right)\mathbf{B}dA\right\}{\mathbf{U}}^{0}-{\alpha }_{1}{N}_{cr}F\left(t\right){{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}_{G}^{T}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathbf{B}}_{G}dA\right){\mathbf{U}}^{0}+\ddot{F}\left(t\right){{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{N}}^{T}{\mathbf{D}}_{m}\mathbf{N}\mathrm{d}A\right){\mathbf{U}}^{0}=\mathrm{sin}{\omega }_{0}t\left({\int }_{A}q{\mathbf{N}}_{w}dA\right){\mathbf{U}}^{0}.$$
(36)

Transforming to Laplace Domain

Utilizing the Laplace transform, the convolution integral of Eq. (36) can be simplified as follows:

$${{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}^{T}{F}^{*}s{\mathbf{D}}^{*}\mathbf{B}dA\right){\mathbf{U}}^{0}-{\alpha }_{1}{N}_{cr}{F}^{*}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}_{G}^{T}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathbf{B}}_{G}dA\right){\mathbf{U}}^{0}+{\ddot{F}}^{*}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{N}}^{T}{\mathbf{D}}_{m}\mathbf{N}\mathrm{d}A\right){\mathbf{U}}^{0}={\left(\mathrm{sin}{\omega }_{0}t\right)}^{*}\left({\int }_{A}q{\mathbf{N}}_{w}dA\right){\mathbf{U}}^{0},$$
(37)

in which \({F}^{*}\), \({\mathbf{D}}^{\mathbf{*}}\), \({\ddot{F}}^{*}\) and \({\left(\mathrm{sin}{\omega }_{0}t\right)}^{*}\) are the Laplace transformation of \(F(t)\), \(\mathbf{D}(t)\), \(\ddot{F}\left(t\right)\) and \(\mathrm{sin}{\omega }_{0}t\), respectively.

Steady-State Response

The time function is approximated as follows:

$$F\left(t\right)=A\mathit{sin}{\omega }_{0}t+B\mathit{cos}{\omega }_{0}t.$$
(38)

Hence, Eqs. (39–40) are derived:

$$\ddot{F}\left(t\right)=-A{{\omega }_{0}}^{2}\mathit{sin}{\omega }_{0}t-B{{\omega }_{0}}^{2}\mathit{cos}{\omega }_{0}t=-{{\omega }_{0}}^{2}F\left(t\right),$$
(39)
$${\ddot{F}}^{*}=-{{\omega }_{0}}^{2}{F}^{*}.$$
(40)

Inserting Eq. (40) into Eq. (37), Eq. (41) is obtained:

$${F}^{*}\left\{{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}^{T}\overline{\mathbf{D}}\mathbf{B }dA\right){\mathbf{U}}^{0}-{\alpha }_{1}{N}_{cr}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}_{G}^{T}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathbf{B}}_{G}dA\right){\mathbf{U}}^{0}-{{\omega }_{0}}^{2}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{N}}^{T}{\mathbf{D}}_{m}\mathbf{N}\mathrm{d}A\right){\mathbf{U}}^{0}\right\}={\left(\mathrm{sin}{\omega }_{0}t\right)}^{*}\left({\int }_{A}q{\mathbf{N}}_{w}dA\right){\mathbf{U}}^{0}, \overline{\mathbf{D} }=s{\mathbf{D}}^{*}.$$
(41)

On the other hand:

$${F}^{*}=\frac{A{\omega }_{0}+Bs}{{s}^{2}+{{\omega }_{0}}^{2}}, {\left(\mathrm{sin}{\omega }_{0}t\right)}^{*}=\frac{{\omega }_{0}}{{s}^{2}+{{\omega }_{0}}^{2}}.$$
(42)

Substituting Eqs. (42) into Eq. (41), Eq. (43) is derived:

$$\frac{A{\omega }_{0}+Bs}{{s}^{2}+{{\omega }_{0}}^{2}}\left\{{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}^{T}\overline{\mathbf{D}}\mathbf{B }dA\right){\mathbf{U}}^{0}-{\alpha }_{1}{N}_{cr}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}_{G}^{T}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathbf{B}}_{G}dA\right){\mathbf{U}}^{0}-{{\omega }_{0}}^{2}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{N}}^{T}{\mathbf{D}}_{m}\mathbf{N}\mathrm{d}A\right){\mathbf{U}}^{0}\right\}=\frac{{\omega }_{0}}{{s}^{2}+{{\omega }_{0}}^{2}}{\int }_{A}q{\mathbf{N}}_{w}dA{\mathbf{U}}^{0}.$$
(43)

Equation (43) can be rewritten as follows:

$$\left(A{\omega }_{0}+Bs\right)\left\{{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}^{T}\overline{\mathbf{D}}\mathbf{B }dA\right){\mathbf{U}}^{0}-{\alpha }_{1}{N}_{cr}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}_{G}^{T}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathbf{B}}_{G}dA\right){\mathbf{U}}^{0}-{{\omega }_{0}}^{2}{{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{N}}^{T}{\mathbf{D}}_{m}\mathbf{N}\mathrm{d}A\right){\mathbf{U}}^{0}\right\}={\omega }_{0}\left({\int }_{A}q{\mathbf{N}}_{w}dA\right){\mathbf{U}}^{0}.$$
(44)

Defining the stiffness matrix in the Laplace–Carson domain \(\overline{\mathbf{K} }\), geometry matrix \({\mathbf{K}}_{G}\), mass matrix \({\mathbf{K}}_{m}\), and load vector \(\mathbf{R}\) as follows:

$$\overline{\mathbf{K} }={\int }_{A}{\mathbf{B}}^{T}\overline{\mathbf{D}}\mathbf{B }dA=\left({\int }_{A}{\mathbf{B}}_{b}^{T}{\overline{\mathbf{D}} }_{b}{\mathbf{B}}_{b}dA+{\int }_{A}{\mathbf{B}}_{s}^{T}{\overline{\mathbf{D}} }_{s}{\mathbf{B}}_{s}dA\right), \overline{K }={{\mathbf{U}}^{0}}^{T}\overline{\mathbf{K}}{\mathbf{U} }^{0},$$
(45)
$${\mathbf{K}}_{G}={\int }_{A}{\mathbf{B}}_{G}^{T}\left[\begin{array}{cc}1& 0\\ 0& {k}_{1}\end{array}\right]{\mathbf{B}}_{G}dA, {K}_{G}={{\mathbf{U}}^{0}}^{T}{\mathbf{K}}_{G}{\mathbf{U}}^{0},$$
(46)
$${\mathbf{K}}_{m}={\int }_{A}{\mathbf{N}}^{T}{\mathbf{D}}_{m}\mathbf{N}dA, {K}_{m}={{\mathbf{U}}^{0}}^{T}{\mathbf{K}}_{m}{\mathbf{U}}^{0},$$
(47)
$$\mathbf{R}={\int }_{A}q{\mathbf{N}}_{w}dA, R=\mathbf{R}{\mathbf{U}}^{0},$$
(48)

in which:

$${\mathbf{B}}_{b}^{i}=\left[\begin{array}{ccc}0& -{\mathbf{N}}_{\text{,}x}^{i}& 0\\ 0& 0& -{\mathbf{N}}_{\text{,}y}^{i}\\ 0& -{\mathbf{N}}_{\text{,}y}^{i}& -{\mathbf{N}}_{\text{,}x}^{i}\end{array}\right]\text{, }{\mathbf{B}}_{s}^{i}=\left[\begin{array}{ccc}{\mathbf{N}}_{\text{,}x}^{i}& -{\mathbf{N}}^{i}& 0\\ {\mathbf{N}}_{\text{,}y}^{i}& 0& -{\mathbf{N}}^{i}\end{array}\right], {\mathbf{B}}_{G}^{i}=\left[\begin{array}{ccc}{\mathbf{N}}_{\text{,}x}^{i}& 0& 0\\ {\mathbf{N}}_{\text{,}y}^{i}& 0& 0\end{array}\right],$$
(49)
$${\overline{\mathbf{D}} }_{b}={h}^{3}/12\left[ \begin{array}{ccc}3K\overline{\eta }\frac{\left(2+\overline{\eta }\right)}{\left(1+2\overline{\eta }\right)}& 3K\overline{\eta }\frac{\left(1-\overline{\eta }\right)}{\left(1+2\overline{\eta }\right)}& 0\\ 3K\overline{\eta }\frac{\left(1-\overline{\eta }\right)}{\left(1+2\overline{\eta }\right)}& 3K\overline{\eta }\frac{\left(2+\overline{\eta }\right)}{\left(1+2\overline{\eta }\right)}& 0\\ 0& 0& 3/2K\overline{\eta }\end{array}\right],$$
(50)
$${\overline{\mathbf{D}} }_{s}=hk\left[\begin{array}{cc} 3/2K\overline{\eta }& 0\\ 0& 3/2K\overline{\eta }\end{array}\right], \overline{\eta }=s{\int }_{0}^{\infty }\eta (t){e}^{-st}dt={c}_{1}+\frac{{c}_{2}s}{s+\lambda }.$$
(51)

Equation (44) can be rewritten as follows:

$$\left(A{\omega }_{0}+Bs\right)\left(\overline{K }-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}\right)={\omega }_{0}R.$$
(52)

Satisfying Eq. (52) at \(s=0\), Eq. (53) is obtained:

$$A{\omega }_{0}\left({\overline{K} }_{s=0}-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}\right)={\omega }_{0}R.$$
(53)

Consequently, the coefficients of the time function are determined as follows:

$$\mathrm{A}=\frac{R}{{\overline{K} }_{s=0}-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}, {\overline{K} }_{s=0}={{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}^{T}\overline{\mathbf{D} }\left(s=0\right)\mathbf{B}dA\right){\mathbf{U}}^{0},$$
(54)
$$B=\frac{{\omega }_{0}\left(\frac{R}{\left(\overline{K }-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}\right)}-A\right)}{s}, \forall s>0.$$
(55)

It is noted that for calculating \(B\), although Eq. (55) is satisfied for every \(s>0\), our experience with the numerical solution of Eq. (87), which is related to the Bernoulli viscoelastic beam (Please study the Appendix 1), showed that the best value for \(s\) is \(s\approx \lambda \). Thus, Eq. (56) is obtained:

$$B=\frac{{\omega }_{0}}{\lambda }\left(\frac{R}{\left({\overline{K} }_{s=\lambda }-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}\right)}-\frac{R}{{\overline{K} }_{s=0}-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}\right), {\overline{K} }_{s=\lambda }={{\mathbf{U}}^{0}}^{T}\left({\int }_{A}{\mathbf{B}}^{T}\overline{\mathbf{D} }\left(s=\lambda \right)\mathbf{B}dA\right){\mathbf{U}}^{0}.$$
(56)

Finally, the steady-state response of a Mindlin viscoelastic plate can be expressed as follows:

$$\mathrm{u}\left(x\text{,}y\text{,}t\right)={\mathrm{u}}^{0}\left(\frac{R}{{\overline{K} }_{s=0}-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}\mathit{sin}{\omega }_{0}t+\frac{{\omega }_{0}}{\lambda }\left(\frac{R}{{\overline{K} }_{s=\lambda }-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}-\frac{R}{{\overline{K} }_{s=0}-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}\right)\mathit{cos}{\omega }_{0}t\right),$$
(57)

where \({\mathbf{u}}^{0}=\mathbf{u}\left(x\text{,}y\text{,}t=0\right)\) is easily calculated utilizing the elastic bending analysis at time zero.

Transient Response

The transient response of a Mindlin viscoelastic plate can be expressed as follows [24]:

$$\mathrm{u}\left(x\text{,}y\text{,}t\right)={\mathrm{u}}^{0}J\left(t\right), J\left(t\right)={e}^{-{\alpha }_{0}t}\left(C\mathrm{sin}{\omega }_{0}t+D\mathrm{cos}{\omega }_{0}t\right),$$
(58)

where \({\alpha }_{0}\) is the viscous damping frequency. \({\alpha }_{0}\) and \({\omega }_{0}\) are calculated by solving Eq. (59) as follows:

$$\mathit{det}\left({s}_{0}{\mathrm{K}}^{*}+{{s}_{0}}^{2}{\mathrm{K}}_{m}-{N}_{cr}{\alpha }_{1}{\mathrm{K}}_{G}\right)=0, {s}_{0}=i{\omega }_{0}-{\alpha }_{0}, {\omega }_{0}\cong\Omega \sqrt{1-{\alpha }_{1}.}$$
(59)

Total Dynamic Response

Thus, the total dynamic response can be written as:

$$\mathrm{u}\left(x\text{,}y\text{,}t\right)={\mathrm{u}}^{0}\left(F\left(t\right)+ J\left(t\right)\right)={\mathrm{u}}^{0}\left[A\mathit{sin}{\omega }_{0}t+B\mathit{cos}{\omega }_{0}t+{e}^{-{\alpha }_{0}t}\left(C\mathit{sin}{\omega }_{0}t+D\mathit{cos}{\omega }_{0}t\right)\right].$$
(60)

\(C\) and \(D\) can be calculated using the initial conditions at time zero:

$$w\left(x\text{,}y, t=0\right)=0\Rightarrow D=-B,$$
(61)
$$\dot{w}\left(x\text{,}y, t=0\right)=0\Rightarrow C=-B\frac{{\alpha }_{0}}{{\omega }_{0}}-A\cong -A, \frac{{\alpha }_{0}}{{\omega }_{0}}\ll 1.$$
(62)

In other words, the transient response can be rewritten as:

$$\mathbf{u}\left(x\text{,}y\text{,}t\right)=-{\mathbf{u}}^{0}{e}^{-{\alpha }_{0}t}\left(A\mathrm{sin}{\omega }_{0}t+B\mathrm{cos}{\omega }_{0}t\right).$$
(63)

Finally, the total nonlinear dynamic response of Mindlin viscoelastic plates subjected to harmonic transversal load \(q\left(t\right)=q\mathrm{sin}{\omega }_{0}t\) and in-plane compression \({N}_{x}={\alpha }_{1}{N}_{cr}\) can be expressed as:

$$\mathbf{u}\left(x\text{,}y\text{,}t\right)={\mathbf{u}}^{0}H\left(t\right), H\left(t\right)=\left(1-{e}^{-{\alpha }_{0}t}\right)\left(A\mathrm{sin}{\omega }_{0}t+B\mathrm{cos}{\omega }_{0}t\right).$$
(64)

Equation (64) indicates that, due to the damping property of viscoelastic materials, the transient response is damped and the total response converges to the steady-state response.

Numerical Results

In this section, the forced vibration analysis of viscoelastic plates subjected to harmonic transversal load and in-plane compression with different material properties are considered. The unknown coefficients of the total response, \(A\), \(B\), \({\omega }_{0}\) and \({\alpha }_{0}\), are determined using Matlab programming. The geometrical and material properties are taken as \(h/a=0.1,K=3\times {10}^{7}N/{m}^{2}, k=5/6\),\(\rho =7800Kg/{m}^{2}\),\({c}_{1}+{c}_{2}=1\) and \(q={10}^{5}N/{m}^{2}\). Also, for spatial discretization, distribution of 25 nodes on the domain of the square plate is considered.

For calculating \(B\), our experience with the numerical solution of Eq. (87), which is related to the Bernoulli viscoelastic beam, showed that the best value for \(s\) is \(s\approx \lambda \). Therefore, in the range \(s\approx \lambda \), several values are selected and the average of the obtained values for \(B\) is calculated.

Verification

Table 1 considers the effect of relaxation function parameter, \({c}_{1}\), on the time responses of moderately thick viscoelastic plates.

Table 1 The coefficients of the time function of simply supported Mindlin viscoelastic square plates with different materials (\({t}_{s}=1s, {\alpha }_{1}=0\),\(H\left(t\right)=\left(1-{e}^{-{\alpha }_{0}t}\right)\left(A\mathit{sin}{\omega }_{0}t+B\mathit{cos}{\omega }_{0}t\right)\))
Full size table

As the results show, by increasing \({c}_{1}\) which means the material tends to elasticity, the absolute value of the coefficients \(A\) and \(B\) are increased and the responses tend to the resonance behavior. So that, the maximum value of the time function corresponding to \({c}_{1}=0.95\) is approximately 55 times of the maximum value of the time function related to \({c}_{1}=0.1\).

Effect of Material Properties

Table 2 investigates the effect of relaxation function parameter, \({c}_{1}\), on the time responses of moderately thick viscoelastic plates.

Table 2 The coefficients of the time function of simply supported Mindlin viscoelastic square plates with different materials (\({t}_{s}=1s, {\alpha }_{1}=0.5, H\left(t\right)=\left(1-{e}^{-{\alpha }_{0}t}\right)\left(A\mathit{sin}{\omega }_{0}t+B\mathit{cos}{\omega }_{0}t\right)\))
Full size table

Figure 2 shows the steady-state responses of Mindlin viscoelastic square plates with simply supported boundary conditions subjected to harmonic transversal loading and in-plane compression.

Fig. 2
figure 2

Steady-state responses of simply supported moderately thick viscoelastic square plates (\(h/L=0.1\), \({\alpha }_{1}=0.5\))

Full size image

The results show that, by increasing \({c}_{1}\), the absolute value of the coefficients \(A\) and \(B\) are increased and the responses tend to the resonance behavior.

Table 3 investigates the effect of relaxation time, \({t}_{s}\), on the time responses of moderately thick viscoelastic plates.

Table 3 The coefficients of the time function of simply supported Mindlin viscoelastic square plates with different relaxation times (\({c}_{1}=0.1, {\alpha }_{1}=0.5,{\omega }_{0}=12.9\))
Full size table

The results show that the absolute value of \(B\) is linearly related to the relaxation time, so that \(B/\left({\omega }_{0}/\lambda \right)\) does not change by changing the relaxation time. Also, \(A\) does not change by changing the relaxation time.

Tables 4–5 consider the effect of bulk modulus and mass density on the time responses of moderately thick viscoelastic plates.

Table 4 The coefficients of the time function of simply supported Mindlin viscoelastic square plates with different bulk moduli (\({c}_{1}=0.1, {\alpha }_{1}=0.5, {t}_{s}=1s\))
Full size table
Table 5 The coefficients of the time function of simply supported Mindlin viscoelastic square plates with different mass densities (\({c}_{1}=0.1, {\alpha }_{1}=0.5, {t}_{s}=1s\))
Full size table

As the results illustrate \(B/\left({\omega }_{0}/\lambda \right)\) and \(A\) do not change by changing the bulk modulus.

As the results show, the absolute value of \(B\) is decreased by increasing mass density. But \(B/\left({\omega }_{0}/\lambda \right)\) and \(A\) do not change by changing the mass density.

Effect of Loading

Tables 6, 7 consider the effect of in-plane compression and transversal loading on the time responses of moderately thick viscoelastic plates.

Table 6 The coefficients of the time function of simply supported Mindlin viscoelastic square plates subjected to different in-plane compressions (\({c}_{1}=0.1, {t}_{s}=1s\))
Full size table
Table 7 The coefficients of the time function of a simply supported Mindlin viscoelastic square plate subjected to different transversal loadings (\({c}_{1}=0.1, {\alpha }_{1}=0.5, {t}_{s}=1s\))
Full size table

As the results illustrate, by increasing the in-plane compression, the absolute value of \(B\) is decreased. But \(B/\left({\omega }_{0}/\lambda \right)\) and \(A\) are almost constant.

The results show that \(A\) and \(B\) do not change by changing the value of the distributed load.

Total Dynamic Responses

Employing Eq. (64), Figs. 3, 4, 5, 6 show the transient, steady-state and total dynamic responses of Mindlin viscoelastic square plates with simply supported boundary conditions subjected to harmonic transversal loading and in-plane compression.

Fig. 3
figure 3

Transient \(J\left(t\right)\), steady-state \(F\left(t\right)\), and total dynamic \(H(t)\) responses of a simply supported moderately thick viscoelastic square plate under harmonic transversal loading (\(h/L=0.1\), \({c}_{1}=0.1\), \({\alpha }_{1}=0\), \({\alpha }_{0}=0.29\), \({\omega }_{0}=18.18\))

Full size image
Fig. 4
figure 4

Transient \(J\left(t\right)\), steady-state \(F\left(t\right)\), and total dynamic \(H(t)\) responses of a simply supported moderately thick viscoelastic square plate under harmonic transversal loading and in-plane compression (\(h/L=0.1\), \({c}_{1}=0.1\), \({\alpha }_{1}=0.5\), \({\alpha }_{0}=0.57\), \({\omega }_{0}=12.9\))

Full size image
Fig. 5
figure 5

Transient \(J\left(t\right)\), steady-state \(F\left(t\right)\), and total dynamic \(H(t)\) responses of a simply supported moderately thick viscoelastic square plate under harmonic transversal loading (\(h/L=0.1\), \({c}_{1}=0.5\), \({\alpha }_{1}=0\), \({\alpha }_{0}=0.16\), \({\omega }_{0}=18.18\))

Full size image
Fig. 6
figure 6

Transient \(J\left(t\right)\), steady-state \(F\left(t\right)\), and total dynamic \(H(t)\) responses of a simply supported moderately thick viscoelastic square plate under harmonic transversal loading and in-plane compression (\(h/L=0.1\), \({c}_{1}=0.5\), \({\alpha }_{1}=0.5\), \({\alpha }_{0}=0.31\), \({\omega }_{0}=12.9\))

Full size image

The results indicate that, due to the damping property of viscoelastic materials, the transient responses are damped and the total responses converge to the steady-state responses.

Conclusions

In this research, a novel formulation was introduced while the transient, steady-state and total dynamic responses of Mindlin viscoelastic plates under out-of-plane and in-plane loadings are explicitly formulated in the time domain for the first time.

The results show that the total dynamic responses of Mindlin viscoelastic plates subjected to harmonic transversal load \(q\left(t\right)=q\mathrm{sin}{\omega }_{0}t\), the excitation frequency is considered as equal to the natural frequency, and in-plane compression \({N}_{x}={\alpha }_{1}{N}_{cr}\) can be expressed as \(\mathrm{u}={\mathrm{u}}^{0}\left(1-{e}^{-{\alpha }_{0}t}\right)\left(A\mathit{sin}{\omega }_{0}t+B\mathit{cos}{\omega }_{0}t\right)\) in which \(A=\frac{R}{{\overline{K} }_{s=0}-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}\), and \(B=\frac{{\omega }_{0}}{\lambda }\left(\frac{R}{{\overline{K} }_{s=\lambda }-{\alpha }_{1}{N}_{cr}{K}_{G}-{{\omega }_{0}}^{2}{K}_{m}}-A\right)\) and \({\mathrm{u}}^{0}\) is calculated using the elastic bending analysis at time zero.

Also, the results indicate that the absolute value of \(B\) is linearly related to the relaxation time. Besides, \(A\) and \(B/\left({\omega }_{0}/\lambda \right)\) do not change by changing the relaxation time, bulk modulus, density, and transversal load.

The results show that by increasing \({c}_{1}\) which means the material tends to elasticity, the responses tend to the resonance behavior.