1 Introduction

There is no pure elastic material in nature and most of the materials have viscoelastic properties to some extent. The time-dependent behavior and damping effect are two interesting characteristics of viscoelastic materials. The superior properties of piezoelectric materials such as their coupled electromechanical properties, vacuum capability and working at very low temperatures, large power generation and quick response make them be frequently used as actuators and sensors in different smart structures. Using the piezoelectric layers in laminated viscoelastic plates can lead to a structure that has both intrinsic viscoelastic damping properties and damping due to the piezoelectric layer’s electromechanical effects. Such a structure can have excellent function in controlling the excited vibrations.

There are many investigations on different analysis of plate structures bonded with piezoelectric layers. Mallik and Ray [1] performed the static analysis of laminated composite plate integrated with an actuator layer using a finite element approach based on the first-order shear deformation plate theory (FSDT). The structure consists of a cross-ply or angle-ply laminated substrate attached with a piezoelectric fiber-reinforced composite (PFRC) layer. They [2] also presented an exact solution for the bending analysis of the mentioned smart composite structure. Topdar et al. [3], using the finite element method, determined the free vibration characteristics of hybrid plates consisting of laminated composite plate or sandwich plate with surface-bonded or embedded piezoelectric layers. Bending, free vibration and forced vibration analysis of functionally graded piezoelectric (FGP) plates was investigated by Behjat et al. [4]. Governing equations were obtained by considering the FSDT assumptions and Hamilton’s principle and they were solved by the finite element method. The material of the structure was varied from PZT4 (PZT stands for lead zirconate titanate) to PZT5H through the plate thickness and the plate was subjected to electrical and mechanical loadings. Loja et al. [5] utilized the B-spline finite strip method to determine the bending and free vibration behavior of functionally graded (FG) plates bonded with piezoelectric layers. The FG material properties were obtained by Mori–Tanaka scheme and the results were compared with experimental tests. Sreehari et al. [6] investigated the bending and buckling analysis of laminated composite plates integrated with piezoelectric layers bonded to the top and bottom faces using a finite element approach based on the inverse hyperbolic shear deformation theory. They also examined the effect of internal flaws on the deflection and critical buckling load of the structure. A finite element formulation for dynamic analysis of the smart sandwich plate was developed by Araujo et al. [7]. The structure was composed of a soft core and two laminated composite faces integrated with two piezoelectric layers at the top and bottom surfaces. Considering a layerwise approach, the displacement field of the core plate and two face layers was assumed based on the higher-order shear deformation theory (HSDT) and the FSDT, respectively. Selim et al. [8] employed the element-free IMLS-Ritz method for active vibration control of FG plates integrated with piezoelectric layers. Their formulation was based on the HSDT and at each node, seven mechanical degrees of freedom and one electrical degree of freedom were considered. A finite element approach based on the four-variable refined plate theory was developed for bending analysis of functionally graded and laminated composite plates integrated with the piezoelectric layer by Rouzegar and Abbasi [9, 10]. Abad and Rouzegar [11] performed the free vibration analysis of an FG plate integrated with piezoelectric layers using the spectral element method. Based on the Mindlin plate theory assumptions and utilizing Maxwell’s relation and Hamilton’s principle the governing equations were obtained for a levy-type plate. The equations were converted to the frequency domain using the fast Fourier transform and a closed-form solution was proposed for them. The accuracy of the presented approach was confirmed by solving several benchmark problems.

The mechanical behaviors of plate structure with viscoelastic material properties have been investigated by many researchers. Wang and Tsai [12] developed a finite element formulation based on the Mindlin plate theory assumptions for the quasi-static and dynamic analysis of linear viscoelastic plates. They changed the integral form of governing equations to several algebraic equations through the finite difference approach in order to reduce the computational cost. Zenkour [13] performed the buckling analysis of the fiber-reinforced laminated composite plate under different in-plane loading conditions. He employed different plate theories such as classical, first-order and sinusoidal shear deformation theories and compared the obtained results together. Also, he [14] proposed a closed-form solution for thermo-viscoelastic analysis of fiber-reinforced composite plates subjected to non-uniform temperature distribution based on a refined plate theory. Eshmatov [15] performed the nonlinear vibration and dynamic stability analysis of rectangular viscoelastic plates using the quadrature numerical method. He employed the classical and first-order shear deformation theories and considered the effect of rotary inertia, too. Abdoun et al. [16] conducted the forced vibration analysis of the viscoelastic beam and plate structure under harmonic excitation. A general formulation that can be implemented to various viscoelastic models was presented and the governing equations were solved by the finite element method. Moita et al. [17] developed an efficient and simple finite element formulation to optimize the damping properties of a multi-layered sandwich plate consisting of a viscoelastic core and two elastic face layers. The fundamental natural frequencies of the structure were obtained by solving an eigenvalue problem and then using the gradient-based algorithm, an optimization process was performed to find the maximum modal loss factor. The cylindrical bending analysis of an angle-ply piezoelectric laminated plate with viscoelastic interfaces was performed by Yan et al. [18] using the state-space method. It was assumed that the bonding between piezoelectric layers has weak (or high) electrically conducting behavior and its viscoelastic properties were defined by the Kelvin–Voigt law. Hosseini et al. [19] presented an analytical nonlinear solution to determine the natural frequencies and dynamic response of a cantilever viscoelastic beam integrated with several piezoelectric patches. The piezoelectric patches, acting as the actuators, were bonded on the top surface of the beam and the hybrid beam was rested on a nonlinear elastic foundation. The Euler–Bernoulli and Kelvin–Voigt models were used for beam theory and viscoelastic material behavior. Using the Kirchhoff and Donnell assumptions, the free vibration analysis a multilayered viscoelastic plate with constrained damping properties was investigated by Wan et al. [20]. The obtained equations were solved using an improved transfer matrix method. The effect of number, thickness, and sequence of layers was studied on the results and the conditions resulting in the optimum frequency were determined. Rouzegar and Gholami [21] employed the dynamic relaxation method for bending analysis of a viscoelastic plate. The material properties were defined by the Prony series and the governing equations based on two-variable refined plate theory were obtained using the principle of virtual work. The finite difference method was utilized for solving the equations. Considering various loading conditions, the creep and recovery responses of isotropic plates were determined and a parametric study was performed, too. Amoushahi [22] performed the bending and buckling analysis of thick viscoelastic plates using the finite strip method. Polynomial and trigonometric functions were used in transverse and longitudinal directions. The formulation was based on the FSDT and the material properties of the viscoelastic plate were described using the Prony series. Moita et al. [23] employed the finite element method for vibration analysis of a pure FG plate and a sandwich plate consisting of a viscoelastic core and two FG faces. The FG faces and core layer were modeled based on the classical plate theory (CPT) and the third-order shear deformation theory (TSDT), respectively. The natural frequencies were obtained from dynamic analyses of the structures in the frequency domain. Also, the dynamic response of the systems under steady-state harmonic motion was determined. Several benchmark problems were solved by the presented approach and the obtained results were examined.

There are few studies on vibration analysis of smart viscoelastic structures. The free vibration analysis of laminated viscoelastic composite panels integrated with piezoelectric actuator patches was performed by Luis et al. [24]. Employing the direct multi-search solver, a multi-objective optimization process was performed to determine the optimal distribution of the patches. The design variables were the position and number of piezoelectric patches and the goals were minimizing the required patches and maximizing the fundamental modal loss factor and natural frequency. The objective functions were validated by the finite element method.

Developing a finite element (FE) formulation using a refined plate theory (RPT) for the viscoelastic plate integrated with a piezoelectric layer could be mentioned as a novelty in the present study. Furthermore, employing the four-variable refined plate theory in the FE formulation, which is a new, efficient and simple HSDT and predicts a parabolic variation for transverse shear stresses across the plate thickness, is an improvement in comparison with FSDT and other conventional HSDTs. For this purpose, a finite element MATLAB code is developed based on the four-variable RPT and a 4-node rectangular plate element with 36 degrees of freedom (DOFs) at each node is introduced. The effects of the piezoelasticity and viscoelasticity on the damping behavior of dynamic responses are investigated, too. Though there is no active vibration control strategy in this research, the vibration can be passively controlled by the piezoelectric layer. This layer, which is attached to the top surface of the structure, plays a key role in controlling the vibration of the plate.

2 Theoretical formulation

2.1 Geometry

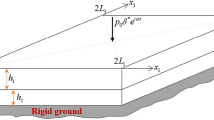
This research deals with the forced vibration analysis of rectangular viscoelastic laminated plates integrated with a piezoelectric layer. The piezoelectric layer which is made of PFRC material acts as an actuator and is attached on the top surface of the plate. As illustrated in Fig. 1, the length and width of the hybrid plate is a and b, and the thickness of the viscoelastic laminate and the PFRC layer is h and hp, respectively. A Cartesian coordinate system is considered at the corner of the middle plane of the plate.

Fig. 1
figure 1

Geometry of viscoelastic laminated plate integrated with a PFRC layer

Full size image

2.2 Constitutive equations

The stress–strain relation for linear viscoelastic plate according to the Boltzmann superposition principle is described as:

$$\sigma (t )= E ( 0 )\varepsilon (t )- \int_{0}^{t} {\frac{\text{d}}{{{\text{d}}k}}[E (t - k )\varepsilon (k ) ] {\text{d}}k} ,$$
(1)

which means that the stress in the specific time (t) would be obtained by the summation behaviors of the plate from the initial time until t. It is assumed that each lamina of the cross-ply substrate is a fiber-reinforced composite and has an orthotropic property. Elastic behavior is considered in the direction of fibers and viscoelastic behavior is considered in other directions due to the polymeric characteristics of the matrix. The Prony-series is utilized for describing the relaxation module of viscoelastic material:

$$E (t )= E_{0} + \sum\limits_{m = 1}^{{M_{t} }} {E_{m} {\text{e}}^{{ - \alpha_{m} t}} } ,$$
(2)

in which \({1 \mathord{\left/ {\vphantom {1 {\alpha_{m} }}} \right. \kern-0pt} {\alpha_{m} }}\) is called the retardation time.

As it was shown in Fig. 1, the main structure is a laminated plate which consists of several viscoelastic orthotropic laminas. The constitutive equations for each viscoelastic layer (for instance nl) are introduced as below:

$$\begin{aligned} \left\{ \begin{aligned} \sigma_{x} \hfill \\ \sigma_{y} \hfill \\ \tau_{xy} \hfill \\ \end{aligned} \right\}^{(nl)} = & \left[ {\begin{array}{*{20}l} {\bar{Q}_{11} (0)} & {\bar{Q}_{12} (0)} & {\bar{Q}_{16} (0)} \\ {\bar{Q}_{12} (0)} & {\bar{Q}_{22} (0)} & {\bar{Q}_{26} (0)} \\ {\bar{Q}_{16} (0)} & {\bar{Q}_{26} (0)} & {\bar{Q}_{66} (0)} \\ \end{array} } \right]^{(nl)} \left\{ \begin{aligned} \varepsilon_{x} (x,y,t) \hfill \\ \varepsilon_{y} (x,y,t) \hfill \\ \varepsilon_{xy} (x,y,t) \hfill \\ \end{aligned} \right\} \\ & - \int_{0}^{t} {\left[ {\begin{array}{*{20}l} {\dot{\bar{Q}}_{11} (t - k)} & {\dot{\bar{Q}}_{12} (t - k)} & {\dot{\bar{Q}}_{16} (t - k)} \\ {\dot{\bar{Q}}_{12} (t - k)} & {\dot{\bar{Q}}_{22} (t - k)} & {\dot{\bar{Q}}_{26} (t - k)} \\ {\dot{\bar{Q}}_{16} (t - k)} & {\dot{\bar{Q}}_{26} (t - k)} & {\dot{\bar{Q}}_{66} (t - k)} \\ \end{array} } \right]}^{(nl)} \left\{ \begin{aligned} \varepsilon_{x} (x,y,k) \hfill \\ \varepsilon_{y} (x,y,k) \hfill \\ \varepsilon_{xy} (x,y,k) \hfill \\ \end{aligned} \right\}{\text{d}}k, \\ \left\{ \begin{aligned} \tau_{yz} \hfill \\ \tau_{xz} \hfill \\ \end{aligned} \right\}^{(nl)} = & \left[ {\begin{array}{*{20}l} {\bar{Q}_{44} (0)} & {\bar{Q}_{45} (0)} \\ {\bar{Q}_{45} (0)} & {\bar{Q}_{55} (0)} \\ \end{array} } \right]^{(nl)} \left\{ \begin{aligned} \gamma_{yz} (x,y,t) \hfill \\ \gamma_{xz} (x,y,t) \hfill \\ \end{aligned} \right\} \\ & - \int_{0}^{t} {\left[ {\begin{array}{*{20}l} {\dot{\bar{Q}}_{44} (t - k)} & {\dot{\bar{Q}}_{45} (t - k)} \\ {\dot{\bar{Q}}_{45} (t - k)} & {\dot{\bar{Q}}_{55} (t - k)} \\ \end{array} } \right]^{(nl)} } \left\{ \begin{aligned} \gamma_{yz} (x,y,k) \hfill \\ \gamma_{xz} (x,y,k) \hfill \\ \end{aligned} \right\}{\text{d}}k, \\ \end{aligned}$$
(3)

where \(\dot{\bar{Q}}\) and \(\bar{Q}\) is the transformed viscose coefficient and transformed elastic coefficient in the reference coordinate system, respectively. The viscose and elastic coefficients in the local coordinate system are defined in Eqs. (4) and (5), respectively:

$$\begin{array}{*{20}l} {Q_{11} (0) = \frac{{E_{1} (0)}}{{1 - \upsilon_{12} \upsilon_{21} }},} \hfill & {Q_{12} (0) = \frac{{\upsilon_{12} E_{2} (0)}}{{1 - \upsilon_{12} \upsilon_{21} }},} \hfill & {Q_{22} (0) = \frac{{E_{2} (0)}}{{1 - \upsilon_{12} \upsilon_{21} }},} \hfill \\ {Q_{44} (0) = G_{23} (0),} \hfill & {Q_{55} (0) = G_{13} (0),} \hfill & {Q_{66} (0) = G_{12} (0),} \hfill \\ \end{array}$$
(4)
$$\begin{array}{*{20}l} {\dot{Q}_{11} (t - k) = \frac{{\dot{E}_{1} (t - k)}}{{1 - \upsilon_{12} \upsilon_{21} }},} \hfill & {\dot{Q}_{12} (t - k) = \frac{{\upsilon_{12} \dot{E}_{2} (t - k)}}{{1 - \upsilon_{12} \upsilon_{21} }},} \hfill \\ {\dot{Q}_{22} (t - k) = \frac{{\dot{E}_{2} (t - k)}}{{1 - \upsilon_{12} \upsilon_{21} }},} \hfill & {\dot{Q}_{44} (t - k) = \dot{G}_{23} (t - k),} \hfill \\ {\dot{Q}_{55} (t - k) = \dot{G}_{13} (t - k),} \hfill & {\dot{Q}_{66} (t - k) = \dot{G}_{12} (t - k),} \hfill \\ \end{array}$$
(5)

where \(E_{1}\) and \(E_{2}\) are elastic modules, \(G_{12}\), \(G_{13}\) and \(G_{23}\) are shear modules, and \(\upsilon_{12}\), \(\upsilon_{21}\) are the Poisson’s ratio in the principal directions.

Based on the rotation angle between the reference and local coordinate system, which is depicted by \(\theta\), the transformed coefficients are:

$$\begin{array}{*{20}l} {\bar{Q}_{11} = Q_{11} \,\cos^{4} \theta + 2\,(Q_{12} + 2Q_{66} )\,\sin^{2} \theta \,\cos^{2} \theta + Q_{22} \sin^{4} \theta ,} \hfill \\ {\bar{Q}_{12} = \,(Q_{11} + Q_{22} - 4Q_{66} )\,\sin^{2} \theta \,\cos^{2} \theta + Q_{12} \left( {\sin^{4} \theta + \cos^{4} \theta } \right),} \hfill \\ {\bar{Q}_{22} = Q_{11} \,\sin^{4} \theta + 2\,(Q_{12} + 2Q_{66} )\,\sin^{2} \theta \,\cos^{2} \theta + Q_{22} \cos^{4} \theta ,} \hfill \\ {\bar{Q}_{16} = (Q_{11} - Q_{12} - 2Q_{66} )\,\sin \theta \,\cos^{3} \theta + (Q_{12} - Q_{22} + 2Q_{66} )\sin^{3} \theta \,\cos \theta ,} \hfill \\ {\bar{Q}_{26} = (Q_{11} - Q_{12} - 2Q_{66} )\,\sin^{3} \theta \,\cos \theta + (Q_{12} - Q_{22} + 2Q_{66} )\sin \theta \,\cos^{3} \theta ,} \hfill \\ {\bar{Q}_{66} = (Q_{11} + Q_{22} - 2Q_{12} - 2Q_{66} )\,\sin^{2} \theta \,\cos^{2} \theta + Q_{66} \left( {\sin^{4} \theta + \cos^{4} \theta } \right),} \hfill \\ {\bar{Q}_{44} = Q_{44} \,\cos^{2} \theta + Q_{55} \,\sin^{2} \theta ,} \hfill \\ {\bar{Q}_{45} = (Q_{55} \, - Q_{44} \,)\sin \theta \,\cos \theta ,} \hfill \\ {\bar{Q}_{55} = Q_{55} \,\cos^{2} \theta + Q_{44} \,\sin^{2} \theta .} \hfill \\ \end{array}$$
(6)

The same relations can be written for \(\dot{\bar{Q}}\) components.

On the other hand, the coupled electro-mechanical constitutive equations for a piezoelectric layer are stated by:

$$\begin{aligned} \varvec{d} & = \varvec{e}^{\text{T}} \{ \varepsilon \} + \varvec{\eta \varXi }, \\ \{ \sigma \} & = [Q]\{ \varepsilon \} - \varvec{e\varXi }, \\ \end{aligned}$$
(7)

where \(\varvec{d}\), \(\varvec{e}\), \(\varvec{\eta}\), \([Q]\) and \(\varvec{\varXi}\) are electrical displacement vector, piezoelectric constant matrix, dielectric constant matrix, stress-reduced stiffness matrix, and electrical field intensity vector, respectively. The electric field components are defined as:

$$\varXi_{i} = - \phi_{,i} \,\,\,\,\,\,\,\,i = 1,2,3.$$
(8)

Due to the small thickness of the piezoelectric layer, the electrostatic potential, \(\phi\), is considered to be linear in z-direction:

$$\phi (x,y,z) = (z - \frac{h}{2})\frac{{\phi_{0} (x,y)}}{{h_{p} }}\,\,\,,\,\,\,\,\,\,\,\,\,\frac{h}{2} \le z \le \frac{h}{2} + h_{p} .$$
(9)

The elastic coefficients Qij for the PFRC layer in the principal material directions can be obtained as:

$$\begin{aligned}\begin{array}{*{20}l} {Q_{11} = \frac{{E_{1} }}{{1 - v_{12} v_{21} }},} \hfill\\ \\ {Q_{12} = \frac{{v_{12} E_{2} }}{{1 - v_{12} v_{21} }},}\\ \hfill \\ {Q_{22} = \frac{{E_{2} }}{{1 - v_{12} v_{21} }},}\\ \hfill \\ {Q_{44} = G_{23} ,}\\ \hfill \\ {Q_{55} = G_{13} ,}\\ \hfill \\ {Q_{66} = G_{12} } \hfill \\ \end{array}\end{aligned}.$$
(10)

According to the structure of the PFRC layer, the effective piezoelectric and dielectric constant matrices can be defined as:

$$\varvec{e} = \left[ {\begin{array}{*{20}l} 0 \hfill & 0 \hfill & {e_{31} } \hfill \\ 0 \hfill & 0 \hfill & {e_{32} } \hfill \\ 0 \hfill & 0 \hfill & {e_{33} } \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & {e_{24} } \hfill & 0 \hfill \\ {e_{15} } \hfill & 0 \hfill & 0 \hfill \\ \end{array} } \right],\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\varvec{\eta}= \left[ {\begin{array}{*{20}l} {\eta_{11} } \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & {\eta_{22} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {\eta_{33} } \hfill \\ \end{array} } \right].$$
(11)

2.3 Four-variable refined plate theory

As the name indicates, this theory includes four parameters by which the displacement components are introduced as:

$$\begin{aligned} u(x,y,z) & = u_{0} (x,y) - z\frac{{\partial w_{b} }}{\partial x} + z\left[ {\frac{1}{4} - \frac{5}{3}\left( {\frac{z}{{h_{t} }}} \right)^{2} } \right]\frac{{\partial w_{s} }}{\partial x}, \\ v(x,y,z) & = v_{0} (x,y) - z\frac{{\partial w_{b} }}{\partial y} + z\left[ {\frac{1}{4} - \frac{5}{3}\left( {\frac{z}{{h_{t} }}} \right)^{2} } \right]\frac{{\partial w_{s} }}{\partial y}, \\ w(x,y) & = w_{b} (x,y) + w_{s} (x,y), \\ \end{aligned}$$
(12)

where \(h_{t} = h + h_{p}\), \(u_{0}\) and \(v_{0}\) are the in-plane displacements of mid-plane in x and y direction, and \(w_{b}\) and \(w_{s}\) are the bending and shear components of transverse displacement, respectively. Regarding the displacement field defined in Eq. (12), the strain components are obtained as:

$$\begin{aligned} \left\{ \begin{aligned} \varepsilon_{x} \hfill \\ \varepsilon_{y} \hfill \\ \varepsilon_{xy} \hfill \\ \end{aligned} \right\} & = \left\{ \begin{aligned} \frac{{\partial u_{0} }}{\partial x} \\ \frac{{\partial v_{0} }}{\partial y} \\ \frac{{\partial u_{0} }}{\partial y} + \frac{{\partial v_{0} }}{\partial x} \\ \end{aligned} \right\} + z\left\{ \begin{aligned} - \frac{{\partial^{2} w_{b} }}{{\partial x^{2} }} \\ \, - \,\frac{{\partial^{2} w_{b} }}{{\partial y^{2} }} \\ - 2\frac{{\partial^{2} w_{b} }}{\partial x\partial y} \\ \end{aligned} \right\} + f\left\{ \begin{aligned} - \frac{{\partial^{2} w_{s} }}{{\partial x^{2} }} \\ - \frac{{\partial^{2} w_{s} }}{{\partial y^{2} }} \\ - 2\frac{{\partial^{2} w_{s} }}{\partial x\partial y} \\ \end{aligned} \right\}, \\ \left\{ \begin{aligned} \gamma_{yz} \hfill \\ \gamma_{xz} \hfill \\ \end{aligned} \right\} & = g\left\{ \begin{aligned} \frac{{\partial w_{s} }}{\partial y} \\ \frac{{\partial w_{s} }}{\partial x} \\ \end{aligned} \right\}, \\ \varepsilon_{z} & = 0, \\ \end{aligned}$$
(13)

where

$$\begin{aligned} f & = - \frac{1}{4}z + \frac{5}{3}z\left( {\frac{z}{{h_{t} }}} \right)^{2} , \\ g & = \frac{5}{4} - 5\left( {\frac{z}{{h_{t} }}} \right)^{2} . \\ \end{aligned}$$
(14)

2.4 Governing equation

Hamilton’s principle is employed to derive the governing equation:

$$\int_{{t_{1} }}^{{t_{2} }} { \, \delta \, (T - \varPi )} \,{\text{d}}t{ = 0,}$$
(15)

where \(T\) and \(\varPi\) describes the kinetic energy and the total potential energy, respectively which are defined as below:

$$T = \,\frac{1}{2}\,\sum\limits_{nl = 1}^{N + 1} {\int\limits_{V} {\rho^{k} \,\left[ {(\dot{u}_{0} - z\frac{{\partial \dot{w}_{b} }}{\partial x} - f\frac{{\partial \dot{w}_{s} }}{\partial x})^{2} + (\dot{v}_{0} - z\frac{{\partial \dot{w}_{b} }}{\partial y} - f\frac{{\partial \dot{w}_{s} }}{\partial y})^{2} + (\dot{w}_{b} + \dot{w}_{s} )^{2} } \right]} \,{\text{d}}V} ,$$
(16)
$$\varPi = \frac{1}{2}\left[ {\sum\limits_{nl = 1}^{N + 1} {\int\limits_{V} {(\sigma_{ij}^{k} \,\varepsilon_{ij}^{k} } } )\,{\text{d}}V - \int\limits_{V} {(d_{i} \,\varXi_{i} } )\,{\text{d}}V} \right] - \int\limits_{{\varOmega_{e} }} {q\,(w_{b} + w_{s} )} \,{\text{d}}A,$$
(17)

where V and \(\varOmega_{e}\) are volume and middle plane area of the plate and q is the external mechanical load applied in the normal direction. Substituting the stresses and strains in terms of displacement components into Eqs. (15)–(17) and setting the variation of the total energy with respect to the independent variables equal to zero leads to the weak form governing equations. The vector form of these equations can be written as:

$$\begin{gathered} \int\limits_{{\varOmega _{e} }} {\left\{ {\left( {{\varvec{D}}_{{\mathbf{2}}} \delta w_{b} } \right)^{{\text{T}}} \left\{ \begin{gathered} {\varvec{D}}(0)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (t)} \right) - \int_{0}^{t} {[\dot{\varvec{D}}(t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{D}}^{\varvec{s}} (0)({\varvec{D}}_{{\mathbf{2}}} w_{s} (t)) - \int_{0}^{t} {[\dot{\varvec{D}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{s} (k)} \right)]{\text{d}}k} \hfill \\ - {\varvec{B}}(0)({\varvec{D}}_{{\mathbf{3}}} u_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{B}}(t - k)\left( {{\varvec{D}}_{{\mathbf{3}}} u_{0} (k)} \right)]{\text{d}}k} \hfill \\ - {\varvec{B}}(0)({\varvec{D}}_{{\mathbf{4}}} v_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{B}}(t - k)\left( {{\varvec{D}}_{{\mathbf{4}}} v_{0} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{e}}^{{{\mathbf{1}}{\varvec{xp}}}} (\phi _{0} ) + {\varvec{e}}^{{{\mathbf{1}}{\varvec{yp}}}} (\phi _{0} ) \hfill \\ \end{gathered} \right\}} \right.} \hfill \\ + \left( {{\varvec{D}}_{{\mathbf{2}}} \delta w_{s} } \right)^{{\text{T}}} \left\{ \begin{gathered} {\varvec{D}}^{\varvec{s}} (0)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (t)} \right) - \int_{0}^{t} {[\dot{\varvec{D}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{H}}^{\varvec{s}} (0)({\varvec{D}}_{{\mathbf{2}}} w_{s} (t)) - \int_{0}^{t} {[\dot{\varvec{H}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{s} (k)} \right)]{\text{d}}k} \hfill \\ - {\varvec{B}}^{\varvec{s}} (0)({\varvec{D}}_{{\mathbf{3}}} u_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{B}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{3}}} u_{0} (k)} \right)]{\text{d}}k} \hfill \\ - {\varvec{B}}^{\varvec{s}} (0)({\varvec{D}}_{{\mathbf{4}}} v_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{B}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{4}}} v_{0} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{e}}^{{{\mathbf{2}}{\varvec{xp}}}} (\phi _{0} ) + {\varvec{e}}^{{{\mathbf{2}}{\varvec{yp}}}} (\phi _{0} ) \hfill \\ \end{gathered} \right\} \hfill \\ + ({\varvec{D}}_{{\mathbf{1}}} \delta w_{s} )^{{\text{T}}} \left\{ {({\varvec{A}}^{\varvec{s}} (0)\left( {{\varvec{D}}_{{\mathbf{1}}} w_{s} (t)} \right) - \int_{0}^{t} {[\dot{\varvec{A}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{1}}} w_{s} (k)} \right)} ]} \right\}{\text{d}}k \hfill \\ + \left( {{\varvec{D}}_{{\mathbf{3}}} \delta u_{0} } \right)^{{\text{T}}} \left\{ \begin{gathered} - {\varvec{B}}(0)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (t)} \right) - \int_{0}^{t} {[\dot{\varvec{B}}(t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (k)} \right)]{\text{d}}k} \hfill \\ - {\varvec{B}}^{\varvec{s}} (0)({\varvec{D}}_{{\mathbf{2}}} w_{s} (t)) - \int_{0}^{t} {[\dot{\varvec{B}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{s} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{A}}(0)({\varvec{D}}_{{\mathbf{3}}} u_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{A}}(t - k)\left( {{\varvec{D}}_{{\mathbf{3}}} u_{0} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{A}}(0)({\varvec{D}}_{{\mathbf{4}}} v_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{A}}(t - k)\left( {{\varvec{D}}_{{\mathbf{4}}} v_{0} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{e}}^{{{\mathbf{3}}{\varvec{xp}}}} (\phi _{0} ) \hfill \\ \end{gathered} \right\} \hfill \\ \end{gathered}$$
(18a)
$$\begin{gathered} + \left( {{\varvec{D}}_{{\mathbf{4}}} \delta v_{0} } \right)^{{\text{T}}} \left. {\left\{ \begin{gathered} - {\varvec{B}}(0)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (t)} \right) - \int_{0}^{t} {[\dot{\varvec{B}}(t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{b} (k)} \right)]{\text{d}}k} \hfill \\ - {\varvec{B}}^{\varvec{s}} (0)({\varvec{D}}_{{\mathbf{2}}} w_{s} (t)) - \int_{0}^{t} {[\dot{\varvec{B}}^{\varvec{s}} (t - k)\left( {{\varvec{D}}_{{\mathbf{2}}} w_{s} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{A}}(0)({\varvec{D}}_{{\mathbf{3}}} u_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{A}}(t - k)\left( {{\varvec{D}}_{{\mathbf{3}}} u_{0} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{A}}(0)({\varvec{D}}_{{\mathbf{4}}} v_{0} (t)) - \int_{0}^{t} {[\dot{\varvec{A}}(t - k)\left( {{\varvec{D}}_{{\mathbf{4}}} v_{0} (k)} \right)]{\text{d}}k} \hfill \\ + {\varvec{e}}^{{{\mathbf{3}}{\varvec{yp}}}} (\phi _{0} ) \hfill \\ \end{gathered} \right\}} \right\}{\text{d}}x{\text{d}}y \hfill \\ - \int\limits_{{\varOmega_{e} }} {\left[ {\delta \left( {w_{b} + w_{s} } \right)^{{\text{T}}} q} \right]} {\text{d}}x{\text{d}}y \hfill \\ + \int\limits_{{\varOmega _{e} }} {\left\{ \begin{gathered} \left( {{\varvec{D}}_{{\mathbf{1}}} \delta w_{b} } \right)^{{\text{T}}} \left\{ {I_{2} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{b} } \right) + I_{4} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{s} } \right) - I_{1} \left[ {\left( {{\varvec{D}}_{{\mathbf{5}}} \ddot{u}_{0} } \right) + \left( {{\varvec{D}}_{{\mathbf{6}}} \ddot{v}_{0} } \right)} \right]} \right\} \hfill \\ + \left( {{\varvec{D}}_{{\mathbf{1}}} \delta w_{s} } \right)^{{\text{T}}} \left\{ {I_{4} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{b} } \right) + I_{5} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{s} } \right) - I_{3} \left[ {\left( {{\varvec{D}}_{{\mathbf{5}}} \ddot{u}_{0} } \right) + \left( {{\varvec{D}}_{{\mathbf{6}}} \ddot{v}_{0} } \right)} \right]} \right\} \hfill \\ + \left( {\delta w_{b} } \right)^{{\text{T}}} \left[ {I_{0} \left( {\ddot{w}_{b} } \right) + I_{0} \left( {\ddot{w}_{s} } \right)} \right] + \left( {\delta w_{s} } \right)^{{\text{T}}} \left[ {I_{0} \left( {\ddot{w}_{b} } \right) + I_{0} \left( {\ddot{w}_{s} } \right)} \right] \hfill \\ + \left( {{\varvec{D}}_{{\mathbf{5}}} \delta u_{0} } \right)^{{\text{T}}} \left\{ {I_{0} \left[ {\left( {{\varvec{D}}_{{\mathbf{5}}} \ddot{u}_{0} } \right) + \left( {{\varvec{D}}_{{\mathbf{6}}} \ddot{v}_{0} } \right)} \right] - I_{1} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{b} } \right) - I_{3} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{s} } \right)} \right\} \hfill \\ + \left( {{\varvec{D}}_{{\mathbf{6}}} \,\delta v_{0} } \right)^{{\text{T}}} \left\{ {I_{0} \left[ {\left( {{\varvec{D}}_{{\mathbf{5}}} \ddot{u}_{0} } \right) + \left( {{\varvec{D}}_{{\mathbf{6}}} \ddot{v}_{0} } \right)} \right] - I_{1} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{b} } \right) - I_{3} \left( {{\varvec{D}}_{{\mathbf{1}}} \ddot{w}_{s} } \right)} \right\} \hfill \\ \end{gathered} \right\}{\text{d}}x{\text{d}}y} = 0, \hfill \\ \end{gathered}$$
(18b)

where

$$\begin{aligned} \begin{array}{*{20}l} {\varvec{A} = \left[ {\begin{array}{*{20}l} {A_{11} } & {A_{12} } & 0 \\ {A_{12} } & {A_{22} } & 0 \\ 0 & 0 & {A_{66} } \\ \end{array} } \right],} \hfill & {\varvec{A}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {A_{11}^{s} } & {A_{12}^{s} } & 0 \\ {A_{12}^{s} } & {A_{22}^{s} } & 0 \\ 0 & 0 & {\dot{A}_{66}^{s} } \\ \end{array} } \right],} \hfill & {\varvec{D} = \left[ {\begin{array}{*{20}l} {D_{11} } & {D_{12} } & 0 \\ {D_{12} } & {D_{22} } & 0 \\ 0 & 0 & {D_{66} } \\ \end{array} } \right],} \hfill \\ {\varvec{B}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {B_{11}^{s} } & {B_{12}^{s} } & 0 \\ {B_{12}^{s} } & {B_{22}^{s} } & 0 \\ 0 & 0 & {B_{66}^{s} } \\ \end{array} } \right],} \hfill & {\varvec{B} = \left[ {\begin{array}{*{20}l} {B_{11} } & {B_{12} } & 0 \\ {B_{12} } & {B_{22} } & 0 \\ 0 & 0 & {B_{66} } \\ \end{array} } \right],} \hfill & {\varvec{D}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {B_{11}^{s} } & {B_{11}^{s} } & 0 \\ {B_{11}^{s} } & {B_{11}^{s} } & 0 \\ 0 & 0 & {B_{11}^{s} } \\ \end{array} } \right],} \hfill \\ \end{array} \hfill \\ \begin{array}{*{20}l} {\varvec{H}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {H_{11}^{s} } & {H_{12}^{s} } & 0 \\ {H_{12}^{s} } & {H_{22}^{s} } & 0 \\ 0 & 0 & {H_{66}^{s} } \\ \end{array} } \right],} \hfill & {} \hfill & {} \hfill \\ \end{array} \hfill \\ \begin{array}{*{20}l} {\dot{\varvec{A}} = \left[ {\begin{array}{*{20}l} {\dot{A}_{11} } & {\dot{A}_{12} } & 0 \\ {\dot{A}_{12} } & {\dot{A}_{22} } & 0 \\ 0 & 0 & {\dot{A}_{66} } \\ \end{array} } \right],} \hfill & {\dot{\varvec{A}}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {\dot{A}_{11}^{s} } & {\dot{A}_{12}^{s} } & 0 \\ {\dot{A}_{12}^{s} } & {\dot{A}_{22}^{s} } & 0 \\ 0 & 0 & {\dot{A}_{66}^{s} } \\ \end{array} } \right],} \hfill & {\dot{\varvec{D}} = \left[ {\begin{array}{*{20}l} {\dot{D}_{11} } & {\dot{D}_{12} } & 0 \\ {\dot{D}_{12} } & {\dot{D}_{22} } & 0 \\ 0 & 0 & {\dot{D}_{66} } \\ \end{array} } \right],} \hfill \\ {\dot{\varvec{B}}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {\dot{B}_{11}^{s} } & {\dot{B}_{12}^{s} } & 0 \\ {\dot{B}_{12}^{s} } & {\dot{B}_{22}^{s} } & 0 \\ 0 & 0 & {\dot{B}_{66}^{s} } \\ \end{array} } \right],} \hfill & {\dot{\varvec{B}} = \left[ {\begin{array}{*{20}l} {\dot{B}_{11} } & {\dot{B}_{12} } & 0 \\ {\dot{B}_{12} } & {\dot{B}_{22} } & 0 \\ 0 & 0 & {\dot{B}_{66} } \\ \end{array} } \right],} \hfill & {\dot{\varvec{D}}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {\dot{B}_{11}^{s} } & {\dot{B}_{11}^{s} } & 0 \\ {\dot{B}_{11}^{s} } & {\dot{B}_{11}^{s} } & 0 \\ 0 & 0 & {\dot{B}_{11}^{s} } \\ \end{array} } \right],} \hfill \\ \end{array} \hfill \\ \begin{array}{*{20}l} {\dot{\varvec{H}}^{\varvec{s}} = \left[ {\begin{array}{*{20}l} {\dot{H}_{11}^{s} } & {\dot{H}_{12}^{s} } & 0 \\ {\dot{H}_{12}^{s} } & {\dot{H}_{22}^{s} } & 0 \\ 0 & 0 & {\dot{H}_{66}^{s} } \\ \end{array} } \right],} \hfill & {} \hfill & {} \hfill \\ \end{array} \hfill \\ \end{aligned}$$
(19)

in which

$$\begin{aligned} \left( {A_{ij} ,D_{ij} ,D_{ij}^{s} ,H_{ij}^{s} ,B_{ij}^{s} ,B_{ij} } \right) & = \sum\limits_{nl = 1}^{N + 1} {\int\limits_{{z_{k} }}^{{z_{k + 1} }} {(\overline{Q}_{ij} )(1,z^{2} ,zf,f^{2} ,f,z){\text{d}}z,\quad i,j = 1,2,6} } , \\ A_{ij}^{s} & = \sum\limits_{nl = 1}^{N + 1} {\int\limits_{{z_{k} }}^{{z_{k + 1} }} {(\overline{Q}_{ij} )g^{2} {\text{d}}z,} } \quad i,j = 4,5, \\ \left( {\dot{A}_{ij} ,\dot{D}_{ij} ,\dot{D}_{ij}^{s} ,\dot{H}_{ij}^{s} ,\dot{B}_{ij}^{s} ,\dot{B}_{ij} } \right) & = \sum\limits_{nl = 1}^{N + 1} {\int\limits_{{z_{k} }}^{{z_{k + 1} }} {(\dot{\bar{Q}}_{ij} )(1,z^{2} ,zf,f^{2} ,f,z){\text{d}}z,\quad i,j = 1,2,6} } , \\ \dot{A}_{ij}^{s} & = \sum\limits_{nl = 1}^{N + 1} {\int\limits_{{z_{k} }}^{{z_{k + 1} }} {(\dot{\bar{Q}}_{ij} )g^{2} {\text{d}}z} } ,\quad i,j = 4,5, \\ \left( {I_{0} ,I_{1} ,I_{2} ,I_{3} ,I_{4} ,I_{5} } \right) & = \sum\limits_{nl = 1}^{N + 1} {\int\limits_{{z_{k} }}^{{z_{k + 1} }} {\rho^{k} } } \,(1,z,z^{2} ,f,zf,f^{2} )\text{d}z, \\ \end{aligned}$$
(20)

and

$$\begin{aligned} \begin{array}{*{20}l} {\varvec{e}^{{{\mathbf{1}}\varvec{xp}}} = \left[ {\begin{array}{*{20}l} {e_{1}^{1xp} } \\ 0 \\ 0 \\ \end{array} } \right],} & {\varvec{e}^{{{\mathbf{2}}\varvec{xp}}} = \left[ {\begin{array}{*{20}l} {e_{1}^{2xp} } \\ 0 \\ 0 \\ \end{array} } \right],} & {\varvec{e}^{{{\mathbf{3}}\varvec{xp}}} = \left[ {\begin{array}{*{20}l} {e_{1}^{3xp} } \\ 0 \\ 0 \\ \end{array} } \right]} \\ \end{array} , \hfill \\ \begin{array}{*{20}l} {\varvec{e}^{{{\mathbf{1}}\varvec{yp}}} = \left[ {\begin{array}{*{20}l} 0 \\ {e_{1}^{1yp} } \\ 0 \\ \end{array} } \right],} & {\varvec{e}^{{{\mathbf{2}}\varvec{yp}}} = \left[ {\begin{array}{*{20}l} 0 \\ {e_{1}^{2yp} } \\ 0 \\ \end{array} } \right],} & {\varvec{e}^{{{\mathbf{3}}\varvec{yp}}} = \left[ {\begin{array}{*{20}l} 0 \\ {e_{1}^{3yp} } \\ 0 \\ \end{array} } \right]} \\ \end{array} , \hfill \\ \end{aligned}$$
(21)

where

$$\begin{array}{*{20}l} {e_{1}^{1xp} = \int\limits_{{\frac{h}{2}}}^{{\frac{h}{2} + h_{p} }} {e_{31} \frac{z}{{h_{p} }}} {\text{d}}z,} \hfill & {e_{1}^{2xp} = \int\limits_{{\frac{h}{2}}}^{{\frac{h}{2} + h_{p} }} {e_{31} \frac{f}{{h_{p} }}} {\text{d}}z,} \hfill & {e_{1}^{3xp} = \int\limits_{{\frac{h}{2}}}^{{\frac{h}{2} + h_{p} }} { - e_{31} \frac{1}{{h_{p} }}} {\text{d}}z,} \hfill \\ {e_{1}^{1yp} = \int\limits_{{\frac{h}{2}}}^{{\frac{h}{2} + h_{p} }} {e_{32} \frac{z}{{h_{p} }}} {\text{d}}z,} \hfill & {e_{1}^{2yp} = \int\limits_{{\frac{h}{2}}}^{{\frac{h}{2} + h_{p} }} {e_{32} \frac{f}{{h_{p} }}} {\text{d}}z,} \hfill & {e_{1}^{3yp} = \int\limits_{{\frac{h}{2}}}^{{\frac{h}{2} + h_{p} }} { - e_{32} \frac{1}{{h_{p} }}} {\text{d}}z,} \hfill \\ \end{array}$$
(22)

and finally, the vectors \(\varvec{D}_{{\mathbf{1}}}\) to \(\varvec{D}_{{\mathbf{6}}}\) are defined as below:

$$\varvec{D}_{{\mathbf{1}}} = \left\{ \begin{aligned} \frac{\partial }{\partial x} \hfill \\ \frac{\partial }{\partial y} \hfill \\ \end{aligned} \right\},\,\varvec{D}_{{\mathbf{2}}} = \left\{ \begin{aligned} \,\,\,\frac{{\partial^{2} }}{{\partial x^{2} }} \hfill \\ \,\,\,\frac{{\partial^{2} }}{{\partial y^{2} }} \hfill \\ 2\frac{{\partial^{2} }}{\partial x\partial y} \hfill \\ \end{aligned} \right\},\,\varvec{D}_{{\mathbf{3}}} = \left\{ \begin{aligned} \frac{\partial }{\partial x} \hfill \\ \,\,0 \hfill \\ \frac{\partial }{\partial y} \hfill \\ \end{aligned} \right\},\,\varvec{D}_{{\mathbf{4}}} = \left\{ {\begin{array}{*{20}l} 0 \\ {\frac{\partial }{\partial y}} \\ {\frac{\partial }{\partial x}} \\ \end{array} } \right\},\,\varvec{D}_{{\mathbf{5}}} = \left\{ \begin{aligned} 1 \hfill \\ 0 \hfill \\ \end{aligned} \right\},\,\varvec{D}_{{\mathbf{6}}} = \left\{ \begin{aligned} 0 \hfill \\ 1 \hfill \\ \end{aligned} \right\}.$$
(23)

2.5 Element design

The domain of the variables is discretized by a 4-node rectangular element. According to the degree of the finite element differential equations, the in-plane displacements and electrostatic potential require the C0 continuity while the bending and shear deflections have to satisfy the C1 continuity over the element domain. Hence, the Lagrangian linear interpolation function (\(\psi_{i}\)) and the non-conforming Hermit cubic interpolation function (\(\kappa_{i}\)) are utilized for these two types of variables:

$$\begin{aligned} & \psi_{i} = \frac{1}{4}\left( {1 + \xi \xi_{i} } \right)\left( {1 + \lambda \lambda_{i} } \right), \\ & \kappa_{i} = \frac{1}{8}\left( {1 + \xi \xi_{i} } \right)\left( {1 + \lambda \lambda_{i} } \right)\left\{ \begin{array}{*{20}c} \left( {2 + \xi \xi_{i} + \lambda \lambda_{i} - \xi^{2} - \lambda^{2} } \right) \hfill \\ r\lambda_{i} \left( {1 - \lambda^{2} } \right) \hfill \\ - p\xi_{i} \left( {1 - \xi^{2} } \right) \hfill \\ \end{array} \right\}, \\ & \begin{array}{*{20}l} {\xi = \frac{{2\left( {x - x_{c} } \right)}}{p},} & {\lambda = \frac{{2\left( {y - y_{c} } \right)}}{r},} \\ \end{array} \\ \end{aligned}$$
(24)

where \((x, \, y)\) is the global coordinate system, \((\xi , \, \lambda )\) is the generalized coordinate system, \(x_{c}\) and \(y_{c}\) are the coordinates of the midpoint of the element, and p and r is the length and width of the element, respectively. Generally, the developed rectangular element has 36 DOFs (including 32 displacement DOFs and 4 electrostatic potential DOFs) as below

$$\Delta \varvec{u}_{\text{0}} = \left[ {\begin{array}{*{20}l} {u_{0}^{1} } \\ {u_{0}^{2} } \\ {u_{0}^{3} } \\ {u_{0}^{4} } \\ \end{array} } \right]_{4} ,\,\Delta \varvec{v}_{\text{0}} = \left[ {\begin{array}{*{20}l} {v_{0}^{1} } \\ {v_{0}^{2} } \\ {v_{0}^{3} } \\ {v_{0}^{4} } \\ \end{array} } \right]_{4} ,\,\Delta \varvec{w}_{\varvec{b}} = \left[ {\begin{array}{*{20}l} {w_{b}^{1} } \\ {\left( {\frac{{\partial w_{b} }}{\partial y}} \right)^{1} } \\ {\left( { - \frac{{\partial w_{b} }}{\partial x}} \right)^{1} } \\ \ldots \\ {\left( { - \frac{{\partial w_{b} }}{\partial x}} \right)^{4} } \\ \end{array} } \right]_{12} ,\,\Delta \varvec{w}_{\varvec{s}} = \left[ {\begin{array}{*{20}l} {w_{s}^{1} } \\ {\left( {\frac{{\partial w_{s} }}{\partial y}} \right)^{1} } \\ {\left( { - \frac{{\partial w_{s} }}{\partial x}} \right)^{1} } \\ \ldots \\ {\left( { - \frac{{\partial w_{s} }}{\partial x}} \right)^{4} } \\ \end{array} } \right]_{12} ,\,\Delta \varvec{\varphi }_{0} = \left[ {\begin{array}{*{20}l} {\varphi_{0}^{1} } \\ {\varphi_{0}^{2} } \\ {\varphi_{0}^{3} } \\ {\varphi_{0}^{4} } \\ \end{array} } \right]_{4} ,$$
(25)

where \(\Delta \varvec{u}_{\text{0}}\) and \(\Delta \varvec{v}_{\text{0}}\) are the in-plane nodal DOF vectors, \(\Delta \varvec{w}_{\varvec{b}}\) and \(\Delta \varvec{w}_{\varvec{s}}\) are the bending and shear DOF vectors and \(\Delta \varvec{\varphi }_{\text{0}}\) is the electrostatic potential DOF vector. The discretized form of the in-plane displacement \(u_{0}\) and \(v_{0}\), bending and shear components of transverse displacement, \(w_{b}\) and \(w_{s}\), and also the electrostatic potential over the element domain are expressed by:

$$\begin{aligned} & u_{0} (x,y) = \sum\limits_{j = 1}^{n} {\Delta u_{j}^{0} } \psi_{j} (x,y) = \varvec{n}^{\text{T}} \Delta \varvec{u}_{\text{0}} , \\ & v_{0} (x,y) = \sum\limits_{j = 1}^{n} {\Delta v_{j}^{0} } \psi_{j} (x,y) = \varvec{n}^{\text{T}} \Delta \varvec{v}_{\text{0}} , \\ & w_{b} (x,y) = \sum\limits_{j = 1}^{n} {\Delta w_{j}^{b} } \kappa_{j} (x,y) = \varvec{N}^{\text{T}} \Delta \varvec{w}_{\varvec{b}} , \\ & w_{s} (x,y) = \sum\limits_{j = 1}^{n} {\Delta w_{j}^{s} } \kappa_{j} (x,y) = \varvec{N}^{\text{T}} \Delta \varvec{w}_{\varvec{s}} , \\ & \phi_{0} (x,y) = \sum\limits_{j = 1}^{n} {\Delta \phi_{j}^{0} } \psi_{j} (x,y) = \varvec{n}^{\text{T}} \Delta \varvec{\varphi }_{\text{0}} , \\ \end{aligned}$$
(26)

where N and n are the shape function vectors for the transverse and in-plane displacement, respectively. The finite element equations based on the four-variable refined plate for the forced vibration analysis are obtained by substituting Eq. (25) in Eq. (17)

$$\begin{aligned} & \left[ {\begin{array}{*{20}l} {\varvec{M}_{{{\mathbf{11}}}} } & {\varvec{M}_{{{\mathbf{12}}}} } & {\varvec{M}_{{{\mathbf{13}}}} } & {\varvec{M}_{{{\mathbf{14}}}} } \\ {\varvec{M}_{{{\mathbf{21}}}} } & {\varvec{M}_{{{\mathbf{22}}}} } & {\varvec{M}_{{{\mathbf{23}}}} } & {\varvec{M}_{{{\mathbf{24}}}} } \\ {\varvec{M}_{{{\mathbf{31}}}} } & {\varvec{M}_{{{\mathbf{32}}}} } & {\varvec{M}_{{{\mathbf{33}}}} } & {\varvec{M}_{{{\mathbf{34}}}} } \\ {\varvec{M}_{{{\mathbf{41}}}} } & {\varvec{M}_{{{\mathbf{42}}}} } & {\varvec{M}_{{{\mathbf{43}}}} } & {\varvec{M}_{{{\mathbf{44}}}} } \\ \end{array} } \right]\left\{ \begin{aligned} \Delta \varvec{\ddot{u}}_{\text{0}} \hfill \\ \Delta \varvec{\ddot{v}}_{\text{0}} \hfill \\ \Delta \varvec{\ddot{w}}_{\varvec{b}} \hfill \\ \Delta \varvec{\ddot{w}}_{\varvec{s}} \hfill \\ \end{aligned} \right\} + \left[ {\begin{array}{*{20}l} {\varvec{K}_{{{\mathbf{11}}}} } & {\varvec{K}_{{{\mathbf{12}}}} } & {\varvec{K}_{{{\mathbf{13}}}} } & {\varvec{K}_{{{\mathbf{14}}}} } \\ {\varvec{K}_{{{\mathbf{21}}}} } & {\varvec{K}_{{{\mathbf{22}}}} } & {\varvec{K}_{{{\mathbf{23}}}} } & {\varvec{K}_{{{\mathbf{24}}}} } \\ {\varvec{K}_{{{\mathbf{31}}}} } & {\varvec{K}_{{{\mathbf{32}}}} } & {\varvec{K}_{{{\mathbf{33}}}} } & {\varvec{K}_{{{\mathbf{34}}}} } \\ {\varvec{K}_{{{\mathbf{41}}}} } & {\varvec{K}_{{{\mathbf{42}}}} } & {\varvec{K}_{{{\mathbf{43}}}} } & {\varvec{K}_{{{\mathbf{44}}}} } \\ \end{array} } \right]\left\{ \begin{aligned} \Delta \varvec{u}_{\text{0}} \hfill \\ \Delta \varvec{v}_{\text{0}} \hfill \\ \Delta \varvec{w}_{\varvec{b}} \hfill \\ \Delta \varvec{w}_{\varvec{s}} \hfill \\ \end{aligned} \right\} \\ & - \left[ {\begin{array}{*{20}l} {\dot{\varvec{K}}_{{{\mathbf{11}}}} } & {\dot{\varvec{K}}_{{{\mathbf{12}}}} } & {\dot{\varvec{K}}_{{{\mathbf{13}}}} } & {\dot{\varvec{K}}_{{{\mathbf{14}}}} } \\ {\dot{\varvec{K}}_{{{\mathbf{21}}}} } & {\dot{\varvec{K}}_{{{\mathbf{22}}}} } & {\dot{\varvec{K}}_{{{\mathbf{23}}}} } & {\dot{\varvec{K}}_{{{\mathbf{24}}}} } \\ {\dot{\varvec{K}}_{{{\mathbf{31}}}} } & {\dot{\varvec{K}}_{{{\mathbf{32}}}} } & {\dot{\varvec{K}}_{{{\mathbf{33}}}} } & {\dot{\varvec{K}}_{{{\mathbf{34}}}} } \\ {\dot{\varvec{K}}_{{{\mathbf{41}}}} } & {\dot{\varvec{K}}_{{{\mathbf{42}}}} } & {\dot{\varvec{K}}_{{{\mathbf{43}}}} } & {\dot{\varvec{K}}_{{{\mathbf{44}}}} } \\ \end{array} } \right]\left\{ \begin{aligned} \Delta \varvec{u}_{\text{0}} \hfill \\ \Delta \varvec{v}_{\text{0}} \hfill \\ \Delta \varvec{w}_{\varvec{b}} \hfill \\ \Delta \varvec{w}_{\varvec{s}} \hfill \\ \end{aligned} \right\} = \left\{ \begin{aligned} \varvec{K}^{{{\mathbf{3}}\varvec{p}}} \,\Delta \varvec{\varphi }_{\text{0}} \hfill \\ \varvec{K}^{{{\mathbf{4}}\varvec{p}}} \,\Delta \varvec{\varphi }_{\text{0}} \hfill \\ \varvec{K}^{{{\mathbf{1}}\varvec{p}}} \,\Delta \varvec{\varphi }_{\text{0}} \hfill \\ \varvec{K}^{{{\mathbf{2}}\varvec{p}}} \Delta \varvec{\varphi }_{\text{0}} \hfill \\ \end{aligned} \right\} + \left\{ \begin{aligned} 0 \hfill \\ 0 \hfill \\ \varvec{F}_{\varvec{q}} \hfill \\ \varvec{F}_{\varvec{q}} \hfill \\ \end{aligned} \right\}, \\ \end{aligned}$$
(27)

while the components of the matrixes are expressed in Appendix A.

2.6 Newmark method

Following the \(M\ddot{u} + C\dot{u} + Ku = F\) shape for general dynamic problems, \(u\), \(\dot{u}\) and \(\ddot{u}\) in each time step is represented for displacement, velocity and acceleration, respectively. Based on the Newmark method, the displacement and velocity are expanded by the Taylor series

$$\begin{aligned} & u(t) = u(t - \Delta t) + \Delta t\dot{u}(t - \Delta t) + \frac{{\Delta t^{2} }}{2}\ddot{u}(t - \Delta t) + \beta \Delta t^{3} \dddot u, \\ & \dot{u}(t) = \dot{u}(t - \Delta t) + \Delta t\ddot{u}(t - \Delta t) + \gamma \Delta t^{2} \dddot u, \\ \end{aligned}$$
(28)

in which \(\gamma\) and \(\beta\) are the Newmark constants. Assuming linear acceleration during the time we will have

$$\dddot u = \frac{{\left[ {\ddot{u}(t) - \ddot{u}(t - \Delta t)} \right]}}{\Delta t}.$$
(29)

So, the final form of the Newmark method can be defined as

$$\begin{aligned} & M_{eff} \ddot{u}_{n + 1} = F_{eff} , \\ & M_{eff} = M + \gamma \Delta tC + \beta \Delta t^{2} K, \\ & F_{eff} = F_{n + 1} - C\left[ {\dot{u}_{n} + (1 - \gamma )\Delta t\ddot{u}_{n} } \right] - K\left[ {u_{n} + \Delta t\dot{u}_{n} + \frac{1}{2}\Delta t^{2} (1 - 2\beta )\ddot{u}_{n} } \right]. \\ \end{aligned}$$
(30)

By substituting \(\ddot{u}_{n + 1}\) calculated from Eq. (29), into Eq. (27), displacement and velocity in time \(n + 1\) will be determined. By selecting different values for \(\gamma\) and \(\beta\), several methods with various features are obtained. In this study, the constant average acceleration method is employed in which \(\gamma\) and \(\beta\) is 0.5 and 0.25, respectively.

3 Numerical results and discussion

As mentioned, this research deals with the dynamic analysis of viscoelastic laminated plates integrated with a piezoelectric layer subjected to different electrical and mechanical loads. A finite element code based on the four-variable refined plate theory is developed. In order to validate the introduced approach, two benchmark problems are solved and the results of the present FE code are compared with those existing in the literature. Since there is no similar study for dynamic analysis of smart viscoelastic laminated plate in the literature, a problem is solved to prove the accuracy of the code in dynamic analysis and another is considered to confirm the performance of the method in simulating the smart structures.

3.1 Benchmark problems

First, a simply-supported three-layered [0/90/0] square elastic plate with a length of 180 mm, integrated with two polyvinylidene fluoride (PVDF) piezoelectric layers at the top and bottom surfaces is considered. The piezoelectric layers are activated by a sinusoidal electrical voltage, \(Voltage = V_{0} \sin ({{\uppi x} \mathord{\left/ {\vphantom {{\uppi x} a}} \right. \kern-0pt} a})\sin ({{\uppi y} \mathord{\left/ {\vphantom {{\uppi y} a}} \right. \kern-0pt} a})\sin (\omega t)\). The thickness of each piezoelectric layer and the core substrate is 0.1 mm and 6 mm, respectively. The material properties of each substrate lamina and the piezoelectric layer are indicated in Eqs. (30) and (31), respectively.

$$\begin{array}{*{20}l} {{{E_{1} } \mathord{\left/ {\vphantom {{E_{1} } {E_{2} = 25,}}} \right. \kern-0pt} {E_{2} = 25,}}} \hfill & {E_{2} = E_{3} = 21.0 \, \text{GPa,}} \hfill \\ {G_{12} = G_{13} = 0.5E_{2} ,} \hfill & {G_{23} = 0.2E_{2} ,} \hfill \\ {\upsilon_{12} = \upsilon_{13} = \upsilon_{23} = 0.25,} \hfill & {\rho = 800\;{{\text{kg}} \mathord{\left/ {\vphantom {{\text{kg}} {\text{m}^{3} }}} \right. \kern-0pt} {\text{m}^{3} }},} \hfill \\ \end{array}$$
(31)
$$\begin{array}{*{20}l} {E = 2.0 \, \text{GPa,}} \hfill & {\upsilon = 0.25,} \hfill \\ {\rho = 100{{ \, \text{kg}} \mathord{\left/ {\vphantom {{ \, \text{kg}} {\text{m}^{3} }}} \right. \kern-0pt} {\text{m}^{3} }},} \hfill & {e_{31} = e_{32} = 0.046 \, {\text{C} \mathord{\left/ {\vphantom {\text{C} {\text{m}^{2} }}} \right. \kern-0pt} {\text{m}^{2} }},} \hfill \\ {e_{15} = e_{24} = e_{33} = 0.0.} \hfill & {} \hfill \\ \end{array}$$
(32)

The normalized central deflection of the plate is defined as:

$$w_{n} = w({a \mathord{\left/ {\vphantom {a {2,}}} \right. \kern-0pt} {2,}}{b \mathord{\left/ {\vphantom {b {2,}}} \right. \kern-0pt} {2,}}0)\frac{{E_{2} }}{{V_{0} e_{31} }}.$$
(33)

After the mesh convergence study, it was found that considering 10 × 10 elements for the plate sides is sufficient to ensure converged results for the dynamic analysis. In Fig. 2, considering \(V_{0} = 100 \, \text{V}\), \(\omega = 100 \, {{\text{rad}} \mathord{\left/ {\vphantom {{\text{rad}} \text{s}}} \right. \kern-0pt} \text{s}}\) and \({a \mathord{\left/ {\vphantom {a {h = 30}}} \right. \kern-0pt} {h = 30}}\), the obtained normalized central of the structure is compared with the results reported by Ref. [25]. As observed, the present results are in good agreement with those obtained by layer-wise plate theory.

Fig. 2
figure 2

Normalized central deflection of the three-layered laminate [0/90/0] integrated with two PVDF layers subjected to a sinusoidal voltage

Full size image

In the second benchmark problem, the dynamic response of a simply-supported viscoelastic plate under uniformed normal pressure (\(q = 10 \, {\text{N} \mathord{\left/ {\vphantom {\text{N} {\text{m}^{\text{2}} }}} \right. \kern-0pt} {\text{m}^{\text{2}} }}\)) is investigated. The time-variation of loading is rectangular-pulse which means that it starts from t = 0 and remains constant during the time. The relaxation module and other geometrical parameters are defined in Eq. (34):

$$\begin{array}{*{20}l} {a = b = 10 \, \text{m,}} \hfill \\ {h = 1 \, \text{m,}} \hfill \\ {E(t) = 1.96 \times 10^{7} + 7.84 \times 10^{7} \text{e}^{{{{ - t} \mathord{\left/ {\vphantom {{ - t} {2.24}}} \right. \kern-0pt} {2.24}}}} \, \text{Pa}\text{.}} \hfill \\ \end{array}$$
(34)

The variation of central deflection of the plate is compared with the existing results [12] in Fig. 3. As seen, the obtained results are well matched with those of Ref. [12].

Fig. 3
figure 3

Variation of the central deflection of the simply supported square plate under uniform mechanical load

Full size image

Two investigated benchmark problems proved the capability of the presented approach in simulating the dynamic behavior of viscoelastic plate and laminate integrated with piezoelectric layers. In the following sections, the dynamic behavior of viscoelastic laminated plate with a piezoelectric actuator layer under various mechanical and electrical loadings is investigated. First, the spatial and temporal convergence study is presented and then, by solving several examples the effect of various parameters on the results is investigated.

3.2 Spatial and temporal convergence study

Consider a square three-layer cross-ply viscoelastic laminate \([0/90/0]\) with the length and thickness of 1 m and 0.01 m which is integrated with a PFRC layer with the thickness of \(250 \times 10^{ - 6} \, \text{m}\) on the top surface. The fully simply supported hybrid structure is subjected to sinusoidally-distributed mechanical and electrical loads with maximum values of 30 N/m2 and − 150 V as shown in Figs. 4 and 5. These loads start from t = 0 and remain constant through the time. The mechanical properties of the laminas and mechanical and electrical properties of the PFRC layer are listed in Eqs. (35) and (36), respectively.

Fig. 4
figure 4

Distribution of sinusoidal mechanical load applied on the plate in the x and y direction

Full size image
Fig. 5
figure 5

Distribution of sinusoidal electrical load applied on the plate in the x and y direction

Full size image
$$\begin{array}{*{20}l} {E_{1} = 9.8 \times 10^{7} \, \text{Pa},\quad \upsilon_{12} = 0.25,} \hfill \\ {E_{2} (t) = (1.96 + 7.84{\text{e}}^{ - 2t} + {\text{e}}^{{{{ - t} \mathord{\left/ {\vphantom {{ - t} 2}} \right. \kern-0pt} 2}}} ) \times 10^{5} \, \text{Pa,}} \hfill \\ {G_{12} (t) = G_{13} (t) = (0.98 + 3.92{\text{e}}^{ - 2t} + 0.5{\text{e}}^{{{{ - t} \mathord{\left/ {\vphantom {{ - t} 2}} \right. \kern-0pt} 2}}} ) \times 10^{5} \, \text{Pa,}} \hfill \\ {G_{23} (t) = (0.784 + 3.136{\text{e}}^{ - 2t} + 0.4{\text{e}}^{{{{ - t} \mathord{\left/ {\vphantom {{ - t} 2}} \right. \kern-0pt} 2}}} ) \times 10^{5} \, \text{Pa,}} \hfill \\ {\rho = 1000{\text{ kg/m}}^{ 3} ,} \hfill \\ \end{array}$$
(35)
$$\begin{array}{*{20}l} {C_{11} = 32.6 \times \text{10}^{9} \, \text{Pa},\quad C_{12} = C_{21} = 4.3 \times \text{10}^{9} \, \text{Pa,}} \hfill \\ {C_{13} = C_{31} = 4.76 \times \text{10}^{9} \, \text{Pa},\quad C_{22} = C_{33} = 7.2 \times \text{10}^{9} \, \text{Pa,}} \hfill \\ {C_{23} = 3.58 \times \text{10}^{9} \, \text{Pa},\quad C_{44} = 1.05 \times \text{10}^{9} \, \text{Pa,}} \hfill \\ {C_{55} = C_{66} = 1.29 \times \text{10}^{9} \, \text{Pa},\quad e_{31} = - 6.76 \, {\text{C} \mathord{\left/ {\vphantom {\text{C} {m^{2} }}} \right. \kern-0pt} {m^{2} }},} \hfill \\ {\rho = 3640{\text{ kg/m}}^{ 3} .} \hfill \\ \end{array}$$
(36)

In order to determine the proper mesh size and time step, two convergence studies are performed and the related results are illustrated in Figs. 6 and 7. In Fig. 6 the effect of the number of elements is investigated. As seen by increasing the number of elements per plate edge (ne) the results converge and finally the mesh structure 10 × 10 seems to be sufficient. For dynamic analysis, the solution is advanced in time and the adequate time step must be determined. The effect of time step size is investigated in Fig. 7. As seen by decreasing the time step size the diagram becomes smoother and \(\Delta t = 0.01 \, \text{s}\) is small enough to present accurate and acceptable results. It should be mentioned that for all examples (previous two benchmark problems and subsequent examples) the results are presented after satisfying both special and temporal convergence criteria.

Fig. 6
figure 6

Spatial convergence study by considering the different number of elements in the plate sides for the smart viscoelastic laminated plate under sinusoidal electrical and mechanical loads

Full size image
Fig. 7
figure 7

Temporal convergence study by considering different time step sizes for the smart viscoelastic laminated plate under sinusoidal electrical and mechanical loads

Full size image

3.3 Parametric studies

The force vibration behavior of elastic, viscoelastic and smart viscoelastic plates is compared in Fig. 8. Mechanical properties of the viscoelastic plate (shown in Eq. (31)) at t = 0 is assumed for the elastic plate. Both elastic and viscoelastic are subjected to the sinusoidal mechanical load which starts at t = 0 and remains constant through the time (rectangular-pulse loading). As seen both plates are vibrating with the same frequency but the damping effect of viscoelasticity gradually decreases the amplitude of the response. By adding the piezoelectric layer and applying the sinusoidal voltage (which starts at t = 0 and remains constant through the time) to this layer the amplitude of the vibration is reduced impressively. It shows the convenient electromechanical effect which can control the amplitude of the structural vibration. Nevertheless, the vibration frequency increases by attaching the piezoelectric layer to the laminate. The effect of the piezoelectric layer on the vibrational behavior of the structure can be controlled by changing the applied voltage. In general, viscoelasticity shows a passive vibration control and, using the piezoelectricity effect the vibration can be controlled actively.

Fig. 8
figure 8

Comparison of the forced vibration behavior of elastic, viscoelastic and smart viscoelastic plates

Full size image

3.4 Harmonic excitation

The prescribed smart viscoelastic laminated plate \([0/90/0/\text{p}]\) is considered under the action of sinusoidally-distributed mechanical and electrical loads with a maximum value of 30 N/m2 and − 150 V occurred at the plate center. It is assumed that the temporal variation of the loadings is harmonic with different frequencies. The fundamental natural frequency of the structure is calculated as 2.67 Hz. The dynamic response of the system when the frequency of mechanical and electrical excitation is taken as 0.04 Hz is illustrated in Fig. 9. According to this figure, at the start of the diagram, the dynamic response is a composition of two harmonic waves: excitation vibration and natural vibration. The fast Fourier transform (FFT) diagram shows the frequency of these two waves in the dynamic response. After a while, the viscoelastic properties of the material damp down the natural vibration of the structure and just the excited vibration of the system due to the applied loading remains.

Fig. 9
figure 9

a Vibration behavior of the smart viscoelastic laminate under harmonic mechanical and electrical excitation, b FFT diagram

Full size image

Now, the frequency of the harmonic mechanical and electrical excitations is considered equal to the fundamental natural frequency. In this case, if the plate behavior was elastic, the deflection would increase dramatically and the resonance phenomena would take place. The dynamic response of the smart viscoelastic structure is illustrated in Fig. 10. As observed, due to the damping property of viscoelastic materials, as time goes on, the rate of increase in deflection is controlled and the response amplitude does not exceed a specified range. The FFT diagram illustrated in Fig. 10 shows that the frequency of dynamic response is just the fundamental natural frequency, as expected.

Fig. 10
figure 10

a Vibration behavior of the smart viscoelastic laminate under harmonic mechanical and electrical excitation with a frequency equal to the fundamental natural frequency (resonance condition), b FFT diagram

Full size image

When the frequency of harmonic excitation is very close to the natural frequency of the system, the beating phenomenon occurs. Knowing that the natural frequency is 2.67 Hz, the frequency of excitation is considered as 2.55 Hz and the obtained dynamic response is illustrated in Fig. 11. As observed the beating behavior happens but the maximum amplitude of beats has a descending trend which is related to damping property of viscoelastic material. In the FFT diagram, both natural frequency and excitation frequency are observed.

Fig. 11
figure 11

a Vibration behavior of the smart viscoelastic laminate under harmonic mechanical and electrical excitation with frequency closed to the fundamental natural frequency (beating condition), b FFT diagram

Full size image

In Eq. (2) the retardation time (\({1 \mathord{\left/ {\vphantom {1 {\alpha_{m} }}} \right. \kern-0pt} {\alpha_{m} }}\)) was defined as the inverse of the coefficient of exponential argument in the Prony series. According to Eqs. (2) and (35) two terms of the Prony series (\(\alpha_{1} = 2\) and \(\alpha_{2} = 0.5\)) is considered in this study. The effect of first retardation time (\(1/\alpha_{1}\)) on the dynamic response of the smart viscoelastic laminate is investigated. The structure is considered under harmonic excitation in resonance condition. As illustrated in Fig. 12, by increasing \(\alpha_{1}\), the stabilization time of the vibration is reduced.

Fig. 12
figure 12

Effect of the inverse of retardation time on the dynamic response of the smart viscoelastic laminate under resonance condition

Full size image

As mentioned before beside the passive control of excited vibration due to the viscoelastic properties of the laminate, the dynamic response of the structure can be actively controlled by applying the electrical voltage to the piezoelectric actuator. The previous smart viscoelastic laminate is considered under harmonic excitation in resonance condition and the effect of the voltage applied to the PFRC layer is investigated. As observed in Fig. 13, by applying − 300 V to the PFRC layer the amplitude of vibration decreases significantly in comparison to the condition that no voltage is applied which means that the induced deflections due to electrical load diminish a portion of mechanical deflections. When the applied voltage is +300 V, the induced electrical deflection amplifies the mechanical deflections.

Fig. 13
figure 13

Effect of the electrical voltage applied to the PFRC layer on the dynamic response of smart viscoelastic laminate under resonance condition

Full size image

In Fig. 14 the effect of boundary condition on the dynamic response of prescribed smart viscoelastic laminated plate is investigated. The structure is subjected to sinusoidally-distributed mechanical and electrical loadings which start at t = 0 and remain constant through the time (rectangular-pulse loading). SSSS, SSCC, and CCCC are stood for fully simply supported, simply supported-clamped and fully clamped plates, respectively. As seen, the SSSS plate by the least constraint possesses the highest deflection and the amplitude of vibration decreases dramatically by increasing the constraints in the SSCC plate. Following that, the CCCC plate presents the lowest deflection, as expected. The frequency of vibration has a reverse trend which means that by increasing the constraint on the plate boundaries and consequently increment of the plate stiffness, the frequency increases, too.

Fig. 14
figure 14

Effect of boundary condition on the central vibration of the smart viscoelastic laminate plate

Full size image

The effect of the thickness to side ratio on the dynamic response of the simply supported smart viscoelastic laminate is investigated in Fig. 15. The loadings are similar to the previous problem. The results are obtained for three different thicknesses to side ratio of 0.01, 0.015 and 0.02. By increasing this ratio and consequently increasing the plate thickness (the plate side is kept constant), both stiffness and mass of structure increase but the amount of increase in stiffness is higher than mass and thus as observed by increasing the thickness ratio the response frequency increases, too. By increasing the thickness ratio the response amplitude decreases due to increment of the plate stiffness.

Fig. 15
figure 15

Effect of thickness to side ratio on the central vibration of the smart viscoelastic laminate plate

Full size image

4 Conclusion

In this study, a finite element formulation based on the four-variable refined plate theory was presented for forced vibration analysis of cross-ply viscoelastic laminates integrated with a piezoelectric actuator. By comparing the obtained results for several benchmark problems existing in the literature, the accuracy and performance of the presented method were verified. For all investigated problems by performing the spatial and temporal convergence studies, the proper element size and time step were determined.

The behavior of elastic, viscoelastic and smart viscoelastic plate was compared and it was concluded that the viscoelastic property, like a vibration passive control, causes a damping effect on the dynamic response of the plate. Integrating the structure with a piezoelectric actuator gives us this possibility to control the mechanical vibration actively by applying an appropriate electrical voltage to the PFRC layer.

The forced vibration behavior of the smart viscoelastic plate under the action of harmonic mechanical and electrical excitations was investigated in three conditions. First, the frequency of excitations was taken different from the fundamental natural frequency of the structure. In this case, the dynamic response of the system was a composition of two harmonic waves with excitation and natural frequencies but because of the damping effect of viscoelasticity the natural response of the system disappeared after a while and the system just vibrated according to the excitation conditions. When the frequency of excitations was considered equal to the natural frequency, the resonance phenomena occurred. Thought an unstable behavior is expected in resonance condition of an elastic body, in this case, the viscoelastic property prevented plate to vibrate unlimited and the deflection was controlled in a specific range. And finally, when the frequency of excitation was kept much closed to the natural frequency, beating phenomena occurred and again because of the viscoelastic properties of the material, a damping behavior was observed.

The effect of some other parameters was also investigated. It was observed that by increasing retardation time the damping behavior of viscoelasticity increases. The effect of the amount of applied voltage to the PFRC layer was investigated and it was observed that due to the amount and sign of applied voltage the vibration amplitude may increase or decrease. The effect of the type of boundary condition on the dynamic response of the system was studied, too. The SSSS plate by the most and the CCCC plate by the least deflection demonstrated that increasing constraints on the plate edges decreased the vibration amplitude and increased the vibration frequency. Finally, the effect of thickness to side ratio on the plate vibration was studied and results were gained for three different thicknesses. As expected, by increasing the thickness, the stiffness increased and consequently, the vibration amplitude decreased. Also, the mass of structure increased by increasing the thickness but the amount of increase of stiffness is more than mass and therefore it was observed that by increasing the plate thickness the response frequency increases, too.