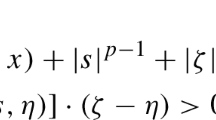On nonlinear parabolic equations with singular lower order term

Abstract

In this paper we study existence and regularity results for solution to a nonlinear and singular parabolic problem. The model is

$$\begin{aligned} \left\{ \begin{array}{lll} \dfrac{\partial u}{\partial t}-\text{ div }((a(x,t)+|u|^{q})\nabla u)=\frac{f}{u^{\gamma }} &{} \text{ in } &{} Q,\\ u(x,t)=0 &{} \text{ on } &{} \Gamma ,\\ u(x,0)=u_{0}(x) &{} \text{ in } &{} \varOmega , \end{array} \right. \end{aligned}$$

where $\varOmega$ is a bounded open subset of $\mathbb {R}^{N},$ $N\ge 2,$ Q is the cylinder $\varOmega \times (0,T),$ $T>0,$ $\Gamma$ the lateral surface $\partial \varOmega \times (0,T),$ $q>0,$ $\gamma >0,$ and f is non-negative function belonging to some Lebesgue space $L^{m}(Q),$ $m\ge 1$ and $u_{0}\in L^{\infty }(\varOmega )$ such that

$$\begin{aligned} \forall \; \omega \subset \subset \varOmega ,\; \exists \; D_{\omega }>0: \; u_{0}\ge D_{\omega }\;\; \text{ in }\; \omega . \end{aligned}$$

1 Introduction

In this work we prove existence and regularity results for a class of nonlinear singular parabolic equations. More precisely, we are interested in the following nonlinear problem

$$\begin{aligned} \left\{ \begin{array}{lll} \dfrac{\partial u}{\partial t}-\text{ div }((a(x,t)+|u|^{q})\nabla u)=\frac{f}{u^{\gamma }} &{} \text{ in } &{} Q,\\ u(x,t)=0 &{} \text{ on } &{} \Gamma ,\\ u(x,0)=u_{0}(x) &{} \text{ in } &{} \varOmega , \end{array} \right. \end{aligned}$$

(1)

where $\varOmega$ is a bounded open subset of $\mathbb {R}^{N},$ $N\ge 2,$ Q is the cylinder $\varOmega \times (0,T),$ $T>0,$ $\Gamma$ the lateral surface $\partial \varOmega \times (0,T),$ $q>0,$ $\gamma >0,$ and f is non-negative function which belongs to some Lebesgue space $L^{m}(Q),$ $m\ge 1,$ the data $u_{0}$ satisfies

$$\begin{aligned} u_{0}\in L^{\infty }(\varOmega )\; \text{ and }\; \forall \; \omega \subset \subset \varOmega ,\; \exists \; D_{\omega }>0:\; u_{0}\ge D_{\omega }\; \text{ in }\; \omega . \end{aligned}$$

(2)

Moreover a(x, t) is a measurable function satisfying

$$\begin{aligned} 0<\alpha \le a(x,t)\le \beta \;\; a.e.\; Q; \end{aligned}$$

(3)

where $\alpha ,\; \beta$ are fixed real numbers.

The interest in problem as (1) started in [12] in connection with the study of thermo-conductivity ($u^{\gamma }$ represented the resistivity the material), and later in the study of signal transmission and in the theory of non-Newtonian pseudo-plastic fluids, see [13, 20, 22].

If $\gamma =0$ many works have appeared concerning the existence and regularity of elliptic equations. Boccardo In [5] has been studied the existence and regularity results of quasi linear elliptic problem

$$\begin{aligned} \left\{ \begin{array}{lll} -\text{ div }((a(x)+|u|^{q})\nabla u)+b(x)u|u|^{p-2}|\nabla u|^{2}=f(x) &{} \text{ in } &{} \varOmega ,\\ u=0 &{} \text{ on } &{} \partial \varOmega , \end{array} \right. \end{aligned}$$

where a(x), b(x) are measurable bounded functions, $p, q\ge 0$ and $0\le f\in L^{m}(\varOmega ),$ 1 $\le$ m $\le \frac{N}{2},$ see also [19]. In the case parabolic the authors in [18] has been studied the existence and regularity results of nonlinear problems

$$\begin{aligned} \left\{ \begin{array}{lll} \frac{\partial u}{\partial t}-\text{ div }((a(x,t)+|u|^{q})\nabla u)+b(x,t)u|u|^{p-1}|\nabla u|^{2}=f &{} \text{ in } &{} Q,\\ u=0 &{} \text{ on } &{} \partial \varOmega ,\\ u(t=0)=0 &{} \text{ in } &{} \varOmega , \end{array} \right. \end{aligned}$$

where a(x, t), b(x, t) are measurable positive bounded functions, $p,q>0$ and f belongs to $L^{m}(Q)$ for some $m\ge 1.$ If $q=0,$ then the operator $A(x,t,\xi )=b(x,t)\xi$ existing in [14] and [8]($p=2$) is linear coercive, monotone and satisfying the growth condition $|A(x,t,\xi )|\le C(d(x,t)+|\xi |)$ with C a positive constant and $d\in L^{2}(Q),$ we highlight that our case ($q>0$) the required growth of $A(x,t,s,\xi )=(a(x,t)+s^{q})\xi$ is more general, handling growths greater then linear case (see also [3, 10, 15, 28]).

In the elliptic framework and when $\gamma >0$ a rich amount of research has been conducted to prove the existence of solution to singular problems, see [25, 26]. For example Boccardo and Orsina in [6] proved the existence and regularity results to problem

$$\begin{aligned} \left\{ \begin{array}{lll} -\Delta u=\frac{f(x)}{u^{\gamma }} &{} \text{ in } &{} \varOmega ,\\ u>0 &{} \text{ in } &{} \varOmega ,\\ u=0 &{} \text{ on } &{} \partial \varOmega , \end{array} \right. \end{aligned}$$

where $\gamma >0$ and f is a nonnegative function belonging to $L^{m}(Q), m\ge 1.$ In the same concept the authors in [23] proved the existence of solution to problem

$$\begin{aligned} \left\{ \begin{array}{lll} -\Delta u=\frac{f(x)}{u^{\gamma }}+\mu &{} \text{ in } &{} \varOmega ,\\ u>0 &{} \text{ in } &{} \varOmega ,\\ u=0 &{} \text{ on } &{} \partial \varOmega , \end{array} \right. \end{aligned}$$

with $\gamma >0, f$ is a nonnegative function on $\varOmega ,$ and $\mu$ is a nonnegative bounded Radon measures on $\varOmega .$ Hence Charkaoui and Alaa [7] established the existence of weak periodic solution to singular parabolic problems

$$\begin{aligned} \left\{ \begin{array}{lll} \frac{\partial u}{\partial t}-\Delta u=\frac{f(x)}{u^{\gamma }} &{} \text{ in } &{} Q,\\ u=0 &{} \text{ on } &{} \Gamma ,\\ u(.,0)=u(.,T) &{} \text{ in } &{} \varOmega , \end{array} \right. \end{aligned}$$

with $\gamma >0$ and f is a nonnegative integrable function periodic in time with period T. Let us observe that we refer to [8, 9, 11, 17, 24] for more details on singular parabolic problems.

If $\gamma =0$ and $q=0,$ the problem (1) has been studied in [14]. When $q=0$ and $\gamma >0,$ the existence and regularity results of problem (1) has been obtained in [8]. The aim of this paper to prove the existence and regularity of solutions of problem (1) depending on the summability of the datum f and the parameters $q, \gamma >0.$ As we will see, our growth assumption on the function $a(x,t)+|u|^{q}$ has a regularization effect on the solution u and its gradient $\nabla u,$ allowing in some cases to have finite energy solution (i.e $u\in L^{2}(0,T;\, H_{0}^{1}(\varOmega )$) even if $f\in L^{1}(Q).$

Notation. Hereafter, we will make use of two truncation functions $T_{k}$ and $G_{k}:$ for every $k\ge 0$ and $s\in \mathbb {R},$ let

$$\begin{aligned} T_{k}(s)=\min (k,\max (r,-k)),\;\;\; G_{k}(s)=s-T_{k}(s). \end{aligned}$$

We will denote with $\rho ^{*}=\frac{\rho N}{N-\rho }$ the Sobolev conjugate of $1\le \rho <N.$

For the sake of simplicity we will use when referring to the integrals the following notation

$$\begin{aligned} \int _{Q}f=\int _{0}^{T}\int _{\varOmega }f=\int _{Q}fdxdt. \end{aligned}$$

Finally, throughout this paper, C will indicate any positive constant which depends only on the data and whose value change from line to line and, some times in the same line.

Our aim is to prove the existence of weak solutions to problem (1). Here is the definition of solution we will consider.

Definition 1

If $\gamma \le 1,$ a solution of (1) is a function $u\in L^{1}(0,T;W_{0}^{1,1}(\varOmega ))$   such that 

$$\begin{aligned}&\forall \omega \subset \subset \varOmega \,\,\; \exists \; C_{\omega }>0: u\ge C_{\omega } \,\,in\,\,\; \omega \times (0,T), \end{aligned}$$

(4)

$$\begin{aligned}&(a(x,t)+u^{q})\nabla u\in L^{1}(0,T;L_{loc}^{1}(\varOmega )), \end{aligned}$$

(5)

and

$$\begin{aligned} -\int _{\varOmega }u_{0}(x)\varphi (x,0)-\int _{0}^{T}\int _{\varOmega }u\frac{\partial \varphi }{\partial t}+\int _{0}^{T}\int _{\varOmega }(a(x,t)+u^{q})\nabla u\nabla \varphi =\int _{0}^{T}\int _{\varOmega }\frac{f\varphi }{u^{\gamma }}, \end{aligned}$$

(6)

$\forall \varphi \in C_{c}^{1}(\varOmega \times [0,T)).$

If $\gamma >1,$ a solution of problem (1) is a function $u\in L^{2}(0,T; H_{loc}^{1}(\varOmega )),$ $u^{r}\in L^{1}(0,T;\, W_{0}^{1,1}(\varOmega )),$ for some $r>1$ and u satisfying (4)–(6).

Now we give a consequence of the Gagliardo–Nirenberg inequality, see [21].

Lemma 1

Let v be a function in $L^{p}(0,T;\, W_{0}^{1,p}(\varOmega ))\cap L^{\infty }(0,T;\, L^{s}(\varOmega )),$ with $p\ge 1, s\ge 1.$ Then $v\in L^{\sigma }(Q)$ with $\sigma =p\frac{N+s}{N}$ and

$$\begin{aligned} \int _{Q}|v|^{\sigma }\le C||v||_{L^{\infty }(0,T:L^{s}(\varOmega ))}^{\frac{sp}{N}}\int _{Q}|\nabla v|^{p}. \end{aligned}$$

2 The approximation scheme

Let f be a non-negative measurable function which belongs to some Lebesgue space, let $n\in \mathbb {N},$ $f_{n}=\frac{f}{1+\frac{1}{n}f},$ and let us consider the following approximation of problem (1)

$$\begin{aligned} \left\{ \begin{array}{lll} \dfrac{\partial u_{n}}{\partial t}-\text{ div }((a(x,t)+u_{n}^{q})\nabla u_{n})=\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }} &{}\text{ in }&{} Q,\\ u_{n}(x,t)=0 &{}\text{ on }&{} \Gamma ,\\ u_{n}(x,0)=u_{0}(x) &{}\text{ in }&{} \varOmega . \end{array} \right. \end{aligned}$$

(7)

Lemma 2

The problem (7) has a non-negative solution $u_{n}\in L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\infty }(Q).$

Proof

Let $k,n\in \mathbb {N},$ be fixed $v\in L^{2}(Q)$ and define $w:=S(v)$ to be the unique solution of (see [16])

$$\begin{aligned} \left\{ \begin{array}{lll} \dfrac{\partial w}{\partial t}-\text{ div }((a(x,t)+|T_{k}(v)|^{q})\nabla w)=\frac{f_{n}}{(|v|+\frac{1}{n})^{\gamma }} &{}\text{ in }&{} Q,\\ w=0 &{}\text{ on }&{} \Gamma ,\\ w(x,0)=u_{0}(x) &{}\text{ in }&{} \varOmega . \end{array} \right. \end{aligned}$$

Using w as test function by (3) and dropping the non-negative terms, we have

$$\begin{aligned} \alpha \int _{Q}|\nabla w|^{2}\le n^{\gamma +1}\int _{Q}|w|+\frac{1}{2}\int _{\varOmega }u_{0}^{2}, \end{aligned}$$

an application of Poincaré inequality on the left hand side and Hölder inequality on the right hand side and the fact that $u_{0}\in L^{\infty }(\varOmega )$ yields

$$\begin{aligned} \int _{Q}|w|^{2}\le Cn^{\gamma +1}\left( \int _{Q}|w|^{2}\right) ^{\frac{1}{2}}+\frac{1}{2}||u_{0}||_{L^{2}(\varOmega )}^{2}, \end{aligned}$$

this by Young inequality with $\epsilon$, implies that

$$\int _{Q}|w|^{2}\le M,$$

where M is a positive constant independent of v. So that the ball of radius M is invariant under S.

$\bullet$ Now we prove that S is continuous.

Let us choose a sequence $v_{n}\rightarrow v$ strongly in $L^{2}(Q);$ then by Lebesgue convergence Theorem :

$$\begin{aligned} \frac{f_{n}}{(|v_{n}|+\frac{1}{n})^{\gamma }}\rightarrow \frac{f_{n}}{(|v|+\frac{1}{n})^{\gamma }}\;\; \text{ in } \; L^{2}(Q), \end{aligned}$$

and the uniqueness of solution for linear problem yields that $w_{r}=S(v_{r})$

$\rightarrow w=S(v)$ strongly in $L^{2}(Q).$ Therefore, we proved that S is continuous.

As we proved before, we have that:

$$\begin{aligned} \int _{Q}|\nabla S(v)|^{2}\le C(n,\gamma , ||u_{0}||_{L^{2}(\varOmega )}),\; \text{ for } \text{ every }\;\;v\in L^{2}(Q). \end{aligned}$$

Then, S(v) is relatively compact in $L^{2}(Q),$ and by Shauder’s fixed point Theorem, there exist $u_{n,k}\in L^{2}(0,T;H_{0}^{1}(\varOmega ))$ such that $S(u_{n,k})=u_{n,k}$ for each n, k fixed. Moreover, $u_{n,k}\in L^{\infty }(Q),$ for all $k,n\in \mathbb {N}.$ Indeed, for $h\ge 1$ fixed, using $G_{h}(u_{n,k})$ as test function, we obtain, since   $u_{n,k}+\frac{1}{n}\ge h\ge 1$ on $\{u_{n,k}\ge h\}$

$$\begin{aligned} \frac{1}{2}\int _{\varOmega }|G_{h}(u_{n,k})|^{2}+\alpha \int _{Q}|\nabla G_{h}(u_{n,k})|^{2}\le \int _{Q}f_{n}G_{h}(u_{n,k})+\frac{1}{2}\int _{\varOmega }u_{0}^{2}. \end{aligned}$$

From now, we can follow the standard technique used for the non-singular case in [1] to get $u_{n,k}\in L^{\infty }(Q).$ Furthermore, the estimate of $u_{n,k}\in L^{\infty }(Q)$ is independent from $k\in \mathbb {N},$ then for k large enough and for n fixed, $u_{n}\in L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\infty }(Q)$ is the solution of the following approximate problem

$$\begin{aligned} \left\{ \begin{array}{lll} \dfrac{\partial u_{n}}{\partial t}-\text{ div }((a(x,t)+u_{n}^{q})\nabla u_{n})=\frac{f_{n}}{(|u_{n}|+\frac{1}{n})^{\gamma }} &{}\text{ in }&{} Q,\\ u_{n}(x,t)=0 &{}\text{ on }&{} \Gamma ,\\ u_{n}(x,0)=u_{0}(x) &{}\text{ in }&{} \varOmega . \end{array} \right. \end{aligned}$$

Since $\frac{f_{n}}{(|u_{n}|+\frac{1}{n})^{\gamma }} \ge 0.$ The maximum principle implies that $u_{n}\ge 0,$ and this concludes the proof. $\square$

Lemma 3

Let $u_{n}$ be a solution of (7). Then for every $\omega \subset \subset \varOmega$ there exists  $C_{\omega }>0$ independent on n such that    $u_{n}\ge C_{\omega } \,\, in\,\, \omega \times (0,T),\; \forall n\in \mathbb {N}.$

Proof

Define for $s\ge 0$ the function

$$\begin{aligned}\psi _{\delta }(s)= \left\{ \begin{array}{lll} 1 &{}if&{} 0\le s\le 1,\\ \frac{1}{\delta }(1+\delta -s) &{}if&{} 1\le s\le \delta +1,\\ 0 &{}if&{} s>\delta +1. \end{array} \right. \end{aligned}$$

We choose $\psi _{\delta }(u_{n})\varphi$ as test function in (7) with $\varphi \in L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\infty }(\varOmega ),$ $\varphi \ge 0$ then we have

$$\begin{aligned}&\int _{0}^{T}\int _{\varOmega }\frac{\partial u_{n}}{\partial t}\psi (u_{n})\varphi +\int _{Q}(a(x,t)+u_{n}^{q})\nabla u_{n}\nabla \varphi \psi _{\delta }(u_{n})\\&\quad =\frac{1}{\delta }\int _{\{1\le u_{n}\le \delta +1\}}(a(x,t)+u_{n}^{q})|\nabla u_{n}|^{2}\varphi +\int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}\psi _{\delta }(u_{n})\varphi , \end{aligned}$$

thus, dropping the non-negative term $\frac{1}{\delta }\int _{\{1\le u_{n}\le \delta +1\}}(a(x,t)+u_{n}^{q})|\nabla u_{n}|^{2}\varphi ,$ and letting $\delta$ goes to zero, we obtain

$$\begin{aligned}&\int _{0}^{T}\int _{\varOmega }\frac{\partial u_{n}}{\partial t}\chi _{\{0\le u_{n}<1\}}\varphi +\int _{Q}(a(x,t)+u_{n}^{q})\nabla u_{n}\cdot \nabla \varphi \chi _{\{0\le u_{n}<1\}}\\&\quad \ge \int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}\varphi \chi _{\{0\le u_{n}<1\}}. \end{aligned}$$

Then for the last inequality we can write as follows

$$\begin{aligned}&\int _{0}^{T}\int _{\varOmega }\frac{\partial T_{1}(u_{n})}{\partial t}\varphi +\int _{Q}(a(x,t)+T_{1}(u_{n})^{q})\nabla T_{1}(u_{n})\nabla \varphi \\&\quad \ge \int _{Q}\frac{f}{2^{\gamma }(1+f)}\varphi \chi _{\{0\le u_{n}<1\}}, \end{aligned}$$

for all $0 \le \varphi \in L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\infty }(Q) .$ Since $\frac{f}{2^{\gamma }(1+f)} \chi _{\{0\le u_{n}<1\}}$ not identically zero and $\alpha \le a(x,t)+T_{1}(u_{n})^{q}\le \beta +1,$ then we have

$$\begin{aligned} \int _{0}^{T}\int _{\varOmega }\frac{\partial T_{1}(u_{n})}{\partial t}\varphi +(\beta +1)\int _{Q}\nabla T_{1}(u_{n}).\nabla \varphi \ge 0. \end{aligned}$$

(8)

This yields that $v_{n}=T_{1}(u_{n})$ is a weak solution of the variational inequality

$$\begin{aligned} \left\{ \begin{array}{lll} \frac{1}{\beta +1}\frac{\partial v_{n}}{\partial t}-\triangle v_{n} \ge 0 &{} \text{ in } &{} Q,\\ v_{n}(x,t)=0 &{} \text{ on } &{} \Gamma ,\\ v_{n}(x,0)=T_{1}(u_{0}(x)) &{} \text{ in } &{} \varOmega , \end{array} \right. \end{aligned}$$

where $v_{n}=T_{1}(u_{n}).$ We are going to prove that

$$\begin{aligned} \forall \; \omega \subset \subset \varOmega ,\; \exists \; C_{\omega }>0:\; \; v_{n}(x,t)\ge C_{\omega }\; \text{ in }\;\; \omega \times (0,T),\; \forall n\in \mathbb {N}. \end{aligned}$$

(9)

Let $w_{n}$ be the solution of the following problem

$$\begin{aligned} \left\{ \begin{array}{lll} \frac{1}{\beta +1}\frac{\partial w_{n}}{\partial t}-\triangle w_{n} = 0 &{} \text{ in } &{} Q,\\ w_{n}(x,t)=0 &{} \text{ on } &{} \Gamma ,\\ w_{n}(x,0)=v_{n}(x,0) &{} \text{ in } &{} \varOmega . \end{array} \right. \end{aligned}$$

(10)

From (8) $v_{n}$ is a supersolution of (10), we have $v_{n}\ge w_{n},$ so that we only have to prove that

$$\begin{aligned} \forall \; \omega \subset \subset \varOmega ,\; \exists \; C_{\omega }>0:\; \; w_{n}(x,t)\ge C_{\omega }\; \text{ in }\;\; \omega \times (0,T),\; \forall n\in \mathbb {N}. \end{aligned}$$

(11)

Since by (2)

$$\begin{aligned} \forall \; \omega \subset \subset \varOmega ,\; \exists \; d_{\omega }>0:\; \; w_{n}(x,0)=v_{n}(x,0)\ge d_{\omega }\; \text{ in }\;\; \omega \times (0,T),\; \forall n\in \mathbb {N}. \end{aligned}$$

(12)

For the rest of the proof we can argue as Boccardo, Orsina and Porzio in [4] (see pp $414-416$), we deduce that there exists $C_{\omega }>0$ such that $w_{n}\ge C_{\omega }$ in $\omega \times (0,T),\; \forall \omega \subset \subset \varOmega ,$ since $v_{n}\ge w_{n},$ then $T_{1}(u_{n})=v_{n}\ge C_{\omega }$ in $\omega \times (0,T),\; \forall \omega \subset \subset \varOmega .$ As $u_{n}\ge T_{1}(u_{n})=v_{n},$ then we obtain

$$u_{n}\ge C_{\omega }\; \text{ in }\; \omega \times (0,T),\;\; \forall \omega \subset \subset \varOmega ,\; \forall n\in \mathbb {N}.$$

$\square$

3 A priori estimates and main results

3.1 $\underline{\hbox {Case }\gamma <1}$

Lemma 4

Let $u_{n}$ be a solution of (7), with $\gamma <1$ and $q>1-\gamma .$ Assume that $f\in L^{1}(Q),$ then $u_{n}$ is bounded in $L^{2}(0,T;H_{0}^{1}(\varOmega )).$

Proof

For n fixed, we choose $\epsilon <\frac{1}{n}$ and using $\phi (u_{n})=((u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma })\times (1-(1+u_{n})^{1-(q+\gamma )})$ as test function, then we have

$$\begin{aligned}&\int _{\varOmega }\Psi (u_{n}(x,t))+\gamma \int _{Q}(u_{n}+\epsilon )^{\gamma -1}(1-(1+u_{n})^{1-(q+\gamma )})(a(x,t)+u_{n}^{q})|\nabla u_{n}|^{2} \nonumber \\&\quad +(q+\gamma -1)\int _{Q}((u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma })(a(x,t)+u_{n}^{q})\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}\nonumber \\&\qquad =\int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma })(1-(1+u_{n})^{1-(q+\gamma )}) +\int _{\varOmega }\Psi (u_{0}), \end{aligned}$$

(13)

where $\Psi (s)=\displaystyle \int _{0}^{s}\phi (\ell )d\ell .$ Dropping the first and second non-negative terms in the left hand side of (13), since $u_{0}\in L^{\infty }(\varOmega )$ and using (3), $\epsilon <\frac{1}{n}$ we have

$$\begin{aligned}&(q+\gamma -1)\int _{Q}((u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma })(a(x,t)+u_{n}^{q})\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}\nonumber \\&\quad \le \int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((u_{n}+\frac{1}{n})^{\gamma }-\epsilon ^{\gamma })(1-(1+u_{n})^{1-(q+\gamma )})\le \int _{Q}f +C, \end{aligned}$$

(14)

and passing to the limit on $\epsilon ,$ we get

$$\begin{aligned} \int _{Q} (\alpha u_{n}^{\gamma }+u_{n}^{q+\gamma })\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}\le C\int _{Q}f +C. \end{aligned}$$

(15)

By working in $\{u_{n}\ge 1\},$ we have

$$\begin{aligned} \int _{\{u_{n}\ge 1\}}(\alpha +u_{n}^{q+\gamma })\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}\le \int _{Q}(\alpha u_{n}^{\gamma }+u_{n}^{q+\gamma })\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}, \end{aligned}$$

then it follows from (15) that

$$\begin{aligned} \frac{\min (\alpha , 1)}{2^{q+\gamma -1}}\int _{\{u_{n}\ge 1\}}|\nabla u_{n}|^{2}\le \min (\alpha ,1)\int _{\{u_{n}\ge 1\}}\frac{1+u_{n}^{q+\gamma }}{(1+u_{n})^{q+\gamma }}|\nabla u_{n}|^{2}\le C\int _{Q}f +C. \end{aligned}$$

we can deduce that

$$\begin{aligned} \int _{\{u_{n}\ge 1\}}|\nabla u_{n}|^{2}\le C. \end{aligned}$$

(16)

Now, we choose $(T_{k}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma }$ as a test function with $\epsilon <\frac{1}{n}$ in (7), by (3) and dropping the nonnegative terms, we get

$$\begin{aligned} \alpha \int _{Q}\frac{|\nabla T_{k}(u_{n})|^{2}}{(T_{k}(u_{n})+\epsilon )^{1-\gamma }}&\le \int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((T_{k}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma })\\&\quad +\frac{1}{\gamma +1}\int _{\varOmega }(T_{k}(u_{0})+\epsilon )^{\gamma +1}-\epsilon ^{\gamma }\int _{\varOmega }u_{0} \le \int _{Q}f\\&\quad +\frac{1}{\gamma +1}\int _{\varOmega }(T_{k}(u_{0})+\epsilon )^{\gamma +1}-\epsilon ^{\gamma }\int _{\varOmega }u_{0}. \end{aligned}$$

Therefore

$$\begin{aligned} \int _{Q}|\nabla T_{k}(u_{n})|^{2}&=\int _{Q}\frac{|\nabla T_{k}(u_{n})|^{2}}{(T_{k}(u_{n})+\epsilon )^{1-\gamma }}(T_{k}(u_{n})+\epsilon )^{1-\gamma }\\&\le (k+\epsilon )^{1-\gamma } \int _{Q}\frac{|\nabla T_{k}(u_{n})|^{2}}{(T_{k}(u_{n})+\epsilon )^{1-\gamma }}\\&\le (k+\epsilon )^{1-\gamma }\left[ \int _{Q}f+\frac{1}{\gamma +1}\int _{\varOmega }(T_{k}(u_{0})+\epsilon )^{\gamma +1}-\epsilon ^{\gamma }\int _{\varOmega }u_{0}\right] . \end{aligned}$$

By the fact that $u_{0}\in L^{\infty }(\varOmega )$ and letting $\epsilon$ goes to zero, implies that

$$\begin{aligned} \int _{Q}|\nabla T_{k}(u_{n})|^{2}\le Ck^{1-\gamma }. \end{aligned}$$

(17)

Combining (16) and (17) we obtain

$$\begin{aligned} \int _{Q}|\nabla u_{n}|^{2}=\int _{\{u_{n}\ge 1\}}|\nabla u_{n}|^{2}+\int _{\{u_{n}\le 1\}}|\nabla u_{n}|^{2}\le C. \end{aligned}$$

Hence by last inequality we deduce that $u_{n}$ is bounded in $L^{2}(0,T;H_{0}^{1}(\varOmega ))$ with respect to n. $\square$

Lemma 5

Let $u_{n}$ be a solution of problem (7), with $\gamma <1$ and $q\le 1-\gamma .$ Suppose that f belongs to $L^{1}(Q),$ then $u_{n}$ is bounded in $L^{r}(0,T;W_{0}^{1,r}(\varOmega ));$ with $r=\frac{N(q+\gamma +1)}{N-(1-(q+\gamma ))}.$

Proof

For n fixed, we choose $\epsilon <\frac{1}{n}$ and using $\psi (u_{n})=(u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma }$ as test function in (7), we obtain

$$\begin{aligned} \int _{\varOmega }\Psi (u_{n}(x,t))+\gamma \int _{Q}(a(x,t)+u_{n}^{q})(u_{n}+\epsilon )^{\gamma -1}|\nabla u_{n}|^{2}\\ =\int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma }) +\int _{\varOmega }\Psi (u_{0}), \end{aligned}$$

where $\Psi (s)=\displaystyle \int _{0}^{s}\psi (\ell )d\ell .$ By removing the first nonnegative terms and using (3), $u_{0}\in L^{\infty }(\varOmega ),$ since $q\le 1-\gamma<1, \epsilon<\frac{1}{n}<1$ and by the fact that

$$\begin{aligned} \min (\alpha , 1)(u_{n}+\epsilon )^{q}\le \min (\alpha , 1)(u_{n}+1)^{q}\le \min (\alpha , 1)(1+u_{n}^{q})\le \alpha +u_{n}^{q}\le a(x,t)+u_{n}^{q}, \end{aligned}$$

we have

$$\begin{aligned} \gamma \min (\alpha ,1)\int _{Q}(u_{n}+\epsilon )^{q+\gamma -1}|\nabla u_{n}|^{2}\le &\, {} \gamma \int _{Q}(\alpha +u_{n}^{q})(u_{n}+1)^{\gamma -1}|\nabla u_{n}|^{2}\\\le &\, {} \int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((u_{n}+\epsilon )^{\gamma }-\epsilon ^{\gamma })\le \int _{Q}f +C. \end{aligned}$$

If $q=1-\gamma ,$ then $u_{n}$ is bounded in $L^{2}(0,T; H_{0}^{1}(\varOmega ))$ with respect to n.

If $q<1-\gamma ,$ then applying Sobolev inequality, we have

$$\begin{aligned} \left( \int _{Q}((u_{n}+\epsilon )^{\frac{q+\gamma +1}{2}}-\epsilon ^{\frac{q+\gamma +1}{2}})^{2^{*}}\right) ^{\frac{2}{2^{*}}}\le C\int _{Q}|\nabla (u_{n}+\epsilon )^{\frac{q+\gamma +1}{2}}|^{2}\le C\int _{Q}f +C, \end{aligned}$$

(18)

letting $\epsilon \rightarrow 0,$ then (18) implies

$$\begin{aligned} \int _{Q}u_{n}^{\frac{2^{*}(q+\gamma +1)}{2}}\le C. \end{aligned}$$

(19)

Therefore, $u_{n}$ is bounded in $L^{\frac{N(q+1+\gamma )}{N-2}}(Q)$ with respect to n.

Now, if $r<2$ as in the statement of Lemma 5, we have by the Hölder inequality

$$\begin{aligned} \int _{Q}|\nabla u_{n}|^{r}&=\int _{Q}\frac{|\nabla u_{n}|^{r}}{(u_{n}+\epsilon )^{(1-(q+\gamma ))\frac{r}{2}}}(u_{n}+\epsilon )^{(1-(q+\gamma ))\frac{r}{2}}\\&\le \left( \int _{Q}\frac{|\nabla u_{n}|^{2}}{(u_{n}+\epsilon )^{1-(q+\gamma )}}\right) ^{\frac{r}{2}}\left( \int _{Q}(u_{n}+\epsilon )^{(1-(q+\gamma ))\frac{r}{2-r}}\right) ^{1-\frac{r}{2}}\\&\le C\left( \int _{Q}(u_{n}+\epsilon )^{(1-(q+\gamma ))\frac{r}{2-r}}\right) ^{1-\frac{r}{2}}. \end{aligned}$$

Thanks to (19), the value of r is such that $\frac{(1-(q+\gamma ))r}{2-r}=\frac{N(q+\gamma +1)}{N-2},$ so that the right hand side of the above inequality is bounded, and then

$$\begin{aligned} \int _{Q}|\nabla u_{n}|^{r}\le M, \end{aligned}$$

(20)

where M is a positive constant independent of n. Then $u_{n}$ is bounded in $L^{r}(0,T;$ $W_{0}^{1,r}(\varOmega ))$ with respect to n,  with $r=\frac{N(q+\gamma +1)}{N-(1-(q+\gamma ))}$ as desired. $\square$

Remark 1

As consequence of both Lemmas 4 and 5, there exists a sub-sequence (not relabeled) and a function u such that $u_{n}$ converge weakly to u in $L^{r}(0,T;W_{0}^{1,r}$ $(\varOmega ))$ (with $r=\frac{N(q+1+\gamma )}{N-(1-(q+\gamma ))}$ ) and almost everywhere in Q as $n\rightarrow \infty .$

In the next lemma we give an estimate of $u_{n}^{q}|\nabla u_{n}|$ in $L^{\rho }(Q)$ for any $\rho <\frac{N}{N-1}.$

Lemma 6

Let $u_{n}$ be a solution of problem (7), with $\gamma <1.$ Suppose that $f\in L^{1}(Q),$ then $u_{n}^{q}|\nabla u_{n}|$ is bounded in $L^{\rho }(Q)$ for every $\rho <\frac{N}{N-1}.$

Proof

For n fixed, we choose $\epsilon <\frac{1}{n}$ and we take as test function $\psi (u_{n})=((T_{1}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma })(1-(1+u_{n})^{1-\lambda }),$ with $\lambda >1,$ we have

$$\begin{aligned} &\int _{\varOmega }\Psi (u_{n}(x,t))+\gamma \int _{Q}(T_{1}(u_{n})+\epsilon )^{\gamma -1}(1-(1+u_{n})^{1-\lambda })(a(x,t)+u_{n}^{q})|\nabla T_{1}(u_{n})|^{2}\\&\quad +(\lambda -1)\int _{Q}(T_{1}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma })(a(x,t)+u_{}^{q})\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{\lambda }}\\&\qquad =\int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((T_{1}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma })(1-(1+u_{n})^{1-\lambda })+\int _{\varOmega }\Psi (u_{0}), \end{aligned}$$

(21)

where $\Psi (s)=\displaystyle \int _{0}^{s}\psi (\sigma )d\sigma .$

In the following, we ignore the first and second non-negative terms in the left hand side of (21), using (3) and the fact that $\alpha +u_{n}^{q}\ge c_{0}(1+u_{n})^{q}$ yield

$$\begin{aligned} &(\lambda -1)c_{0}\int _{Q}((T_{1}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma })(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\\&\quad \le \int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}((T_{1}(u_{n})+\epsilon )^{\gamma }-\epsilon ^{\gamma })(1-(1+u_{n})^{1-\lambda })+\int _{\varOmega }\Psi (u_{0}). \end{aligned}$$

(22)

Letting $\epsilon$ goes to zero and using the fact that $u_{0}\in L^{\infty }(\varOmega )$, then (22) becomes

$$\begin{aligned} \int _{\{u_{n}\ge 1\}}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le \int _{Q}T_{1}^{\gamma }(u_{n})(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le C\int _{Q}f+C. \end{aligned}$$

(23)

Combining (17) and (23) lead to

$$\begin{aligned} \int _{Q}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}= &\, {} \int _{\{u_{n}\ge 1\}}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\\&+\int _{\{u_{n}\le 1\}}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le C. \end{aligned}$$

Now, let $\rho =\frac{N(2+q-\lambda )}{N(q+1)-(\lambda +q)}$ and using the previous result together with Hölder inequality, we have

$$\begin{aligned} \int _{Q}u_{n}^{q\rho }|\nabla u_{n}|^{\rho }\le \int _{Q}(1+u_{n})^{\frac{\rho (q+\lambda )}{2}}\frac{|\nabla u_{n}|^{\rho }}{(1+u_{n})^{\frac{\rho (\lambda -q)}{2}}}\le C\left( \int _{Q}(1+u_{n})^{\frac{\rho (q+\lambda )}{2-\rho }}\right) ^{\frac{2-\rho }{2}}, \end{aligned}$$

and by Sobolev inequality, we get

$$\begin{aligned} \left( \int _{Q}u_{n}^{\rho ^{*}(q+1)}\right) ^{\frac{\rho }{\rho ^{*}}}\le C\left( \int _{Q}u_{n}^{\frac{\rho (q+\lambda )}{2-\rho }}\right) ^{\frac{2-\rho }{2}}, \end{aligned}$$

the previous choice of $\rho$ implies that $\rho ^{*}(q+1)=\rho (q+\lambda )/(2-\rho ),$ and since $\lambda >1,$ we obtain an estimate of $u_{n}^{q}|\nabla u_{n}|$ in $L^{\rho }(Q)$ for every $\rho <N/(N-1),$ as desired. In order to pass to the limit in the approximate equations, the almost everywhere convergence of the $\nabla u_{n}$ to $\nabla u$ is required, this result will be proved following the same techniques as in [2] (see also [19]). $\square$

Lemma 7

The sequence $\{\nabla u_{n}\}$ converges to $\nabla u$ a.e. in Q.

Proof

Let $\varphi \in C_{c}^{1}(\varOmega ), \varphi \ge 0$ independent of $t\in [0,T]$ $\varphi \equiv 1$ on $w=Supp \varphi \subset \subset \varOmega$ and using $T_{h}(u_{n}-T_{k}(u))\varphi$ as a test function in (7)

$$\begin{aligned}&\int _{0}^{T}\int _{\varOmega }\frac{\partial u_{n}}{\partial t}T_{h}(u_{n}-T_{k}(u))\varphi +\int _{0}^{T}\int _{\varOmega }(a(x,t)+u_{n}^{q})\varphi \nabla u_{n}\nabla T_{h}(u_{n}-T_{k}(u))\nonumber \\&\qquad +\int _{0}^{T}\int _{\varOmega }(a(x,t)+u_{n}^{q})T_{h}(u_{n}-T_{k}(u))\nabla u_{n}\nabla \varphi \nonumber \\&\quad =\int _{0}^{T}\int _{\varOmega }\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}T_{h}(u_{n}-T_{k}(u))\varphi . \end{aligned}$$

(24)

Since $w=Supp \varphi \subset \subset \varOmega$ and by Lemma 3 we have $u_{n}\ge C_{Supp\varphi },$ then we the above equality becomes

$$\begin{aligned} &\frac{1}{2}\int _{\varOmega }T_{h}^{2}(u_{n}-T_{k}(u))\varphi +\int _{0}^{T}\int _{\varOmega }(a(x,t)+u_{n}^{q})|\nabla T_{h}(u_{n}-T_{k}(u))|^{2}\varphi \\&\quad \le Ch||\nabla \varphi ||_{L^{\infty }} +h||\varphi ||_{L^{\infty }}\frac{1}{C_{Supp\varphi }^{\gamma }}\int _{0}^{T}\int _{Supp\varphi }f+\frac{1}{2}\int _{\varOmega }T_{h}^{2}(u_{0}-T_{k}(u_{0}))\varphi \\&\qquad -\int _{0}^{T}\int _{\varOmega }(a(x,t)+u_{n}^{q})\nabla T_{h}(u)\nabla T_{h}(u_{n}-T_{k}(u))\varphi , \end{aligned}$$

(25)

by removing the first non-negative term, we obtain

$$\begin{aligned} &\int _{0}^{T}\int _{\varOmega }(a(x,t)+u_{n}^{q})|\nabla T_{h}(u_{n}-T_{k}(u))|^{2}\varphi \\&\quad \le Ch||\nabla \varphi ||_{L^{\infty }} +h||\varphi ||_{L^{\infty }}\frac{1}{C_{Supp\varphi }^{\gamma }}\int _{0}^{T}\int _{Supp\varphi }f+\frac{1}{2}h^{2}\text{ meas }(\varOmega ) \\&\qquad -\int _{0}^{T}\int _{\varOmega }(a(x,t)+u_{n}^{q})\nabla T_{h}(u)\nabla T_{h}(u_{n}-T_{k}(u))\varphi . \end{aligned}$$

(26)

Since $\nabla T_{h}(u_{n}-T_{k}(u))\ne 0$ (which implies that $u_{n}\le h+k$), we can easily to pass the limit as n tends to $\infty ,$ thanks to Remark 1, in the right hand side of the above inequality, so that

$$\begin{aligned} \alpha \limsup _{n\rightarrow \infty }\int _{0}^{T}\int _{\varOmega }|\nabla T_{h}(u_{n}-T_{k}(u))|^{2}\varphi \le Ch. \end{aligned}$$

(27)

Let now s be such that $s<r<2,$ where r is in the statement of Lemma 5

$$\begin{aligned} \int _{0}^{T}\int _{w}|\nabla u_{n}-\nabla u|^{s}&\le \int _{0}^{T}\int _{\varOmega }|\nabla u_{n}-\nabla u|^{s}\varphi \\&=\int _{\{|u_{n}-u|\le h, u\le k\}} |\nabla u_{n}-\nabla u|^{s}\varphi +\int _{\{|u_{n}-u|\le h, u> k\}} |\nabla u_{n}-\nabla u|^{s}\varphi \\&\quad +\int _{\{|u_{n}-u|> h\}} |\nabla u_{n}-\nabla u|^{s}\varphi . \end{aligned}$$

(28)

From (20), we have

$$\begin{aligned} \int _{0}^{T}\int _{\varOmega }|\nabla u_{n}-\nabla u|^{s}\varphi&\le \int _{0}^{T}\int _{\varOmega }|\nabla T_{h}(u_{n}-T_{k}(u))|^{s}\varphi \\&\quad +||\varphi ||_{L^{\infty }}\left( 2^{s}M^{s} (meas\{u>k\})^{1-\frac{s}{r}}+2^{s}M^{s}(meas\{|u_{n}-u|>h\})^{1-\frac{s}{r}}\right) . \end{aligned}$$

(29)

Thus, combining (27) and (28), we obtain for every $h>0$ and every $k>0$

$$\begin{aligned} \limsup _{n\rightarrow \infty }\int _{0}^{T}\int _{\varOmega }|\nabla u_{n}-\nabla u|^{s}\varphi \le \left( \frac{2h}{\alpha }\int _{0}^{T}\int _{\varOmega }\right) ^{\frac{s}{2}}||\varphi ||_{L^{\infty }} meas(Q)^{1-\frac{s}{2}}\\ +||\varphi ||_{L^{\infty }}2^{s}M^{s}(meas\{u>k\})^{1-\frac{s}{r}}. \end{aligned}$$

(30)

Letting h tends to zero and k tends to infinity, we finally that

$$\begin{aligned} \limsup _{n\rightarrow \infty }\int _{0}^{T}\int _{\varOmega }|\nabla u_{n}-\nabla u|^{s}\varphi =0, \quad \forall s<2. \end{aligned}$$

Therefore, up to sub sequence, $\{\nabla u_{n}\}$ converges to $\nabla u$ a.e., and Lemma 7 is completely proved. $\square$

Now we are in position to prove our existence result given by

Theorem 1

Let $\gamma <1$ and f be nonnegative function in $L^{1}(Q),$ then there exists a nonnegative solution u of problem (1) in the sense of Definition 1. Moreover, u belong to $L^{2}(0,T;H_{0}^{1}(\varOmega ))$ if $q>1-\gamma$ and it belongs to $L^{r}(0,T;W_{0}^{1,r}(\varOmega ))$ (with r as in the statement of Lemma 5) if $q\le 1-\gamma .$

Proof

As we have already said (see Remark 1 ), there exists a function $u\in L^{r}(0,T;\, W_{0}^{1,r}(\varOmega )),$ such that $u_{n}$ converges weakly to u in $L^{r}(0,T;\, W_{0}^{1,r}(\varOmega )).$

By Lemma 3, we have $\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}$ is bounded in $L^{1}(0,T;\, L^{1}_{loc}(\varOmega ))$ and Lemma 6 gives $(a(x,t)+u_{n}^{q})|\nabla u_{n}|$ is bounded in $L^{\rho }(Q),$ $\rho<\frac{N}{N-1}<2$ then ${\text {div}}((a(x,t)+u_{n}^{q})\nabla u_{n})$ is bounded $L^{\rho ^{\prime }}(Q)\subset L^{2}(Q)\subset L^{2}(0,T;\, H^{-1}(\varOmega )),$ then we deduce $\{\frac{\partial u_{n}}{\partial t}\}_{n}$ is bounded in $L^{1}(0,T;\, L^{1}_{loc}(\varOmega ))+L^{2}(0,T;\,H^{-1}(\varOmega )),$ using compactness argument in [27], we deduce that

$$\begin{aligned} u_{n}\longrightarrow u \,\,\,\, \;\; \text{ strongly } \text{ in }\,\,\, L^{1}(Q). \end{aligned}$$

(31)

On the other hand, Lemmas 6, 7 and Remark 1 imply that the sequence $u_{n}^{q}|\nabla u_{n}|$ converges weakly to $u^{q}|\nabla u|$ in $L^{\rho }(Q)$ for every $\rho <\frac{N}{N-1}.$ Hence for every $\varphi \in C^{1}_{c}(\varOmega \times [0,T))$

$$\begin{aligned} \lim _{n\rightarrow \infty }\int _{Q}(a(x,t)+u_{n}^{q})\nabla u_{n}\cdot \nabla \varphi =\int _{Q}(a(x,t)+u^{q})\nabla u\cdot \nabla \varphi . \end{aligned}$$

(32)

For the limit of the right hand of (7). Let $w=\{\varphi \ne 0\},$ then by Lemma 3, one has, for every $\varphi \in C^{1}_{c}(\varOmega \times [0,T))$

$$\begin{aligned} \left| \frac{f_{n}\varphi }{(u_{n}+\frac{1}{n})^{\gamma }}\right| \le \frac{||\varphi ||_{L^{\infty }}}{C_{w}^{\gamma }}f, \end{aligned}$$

(33)

then by Remark 1, (33) and dominated convergence theorem, we get

$$\begin{aligned} \frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}\longrightarrow \frac{f}{u^{\gamma }} \;\;\;\,\,\, \text{ strongly } \text{ in }\,\,\, L_{loc}^{1}(Q). \end{aligned}$$

(34)

Let $\varphi \in C_{c}^{1}(\varOmega \times [0,T))$ as test function in (7), by (31), (32), (33), (34) and letting $n\rightarrow +\infty ,$ we obtain

$$\begin{aligned} -\int _{\varOmega }u_{0}(x)\varphi (x,0)-\int _{Q}u\frac{\partial \varphi }{\partial t}+\int _{Q}(a(x,t)+u^{q})\nabla u.\nabla \varphi =\int _{Q}\frac{f}{u^{\gamma }}\varphi . \end{aligned}$$

(35)

Hence, we conclude that the solution u satisfies the conditions (4), (5) and (6) of Definition 1, so that the proof of Theorem 1 is now completed. $\square$

3.2 $\underline{\hbox {Case} \gamma =1}$

Lemma 8

Let $u_{n}$ be a solution of problem (7), with $\gamma =1.$ Suppose that f belongs to $L^{1}(Q).$ Then $u_{n}$ is bounded in $L^{\infty }(0,T;\,L^{2}(\varOmega ))\cap L^{2}(0,T;\,H_{0}^{1}(\varOmega ))\cap L^{\frac{N(q+2)}{N-2}}(Q).$

Proof

we use $u_{n}\chi _{(0,t)}$ as test function in (7) and by (3), we obtain

$$\begin{aligned} \frac{1}{2}\int _{\varOmega }|u_{n}(x,t)|^{2}+\alpha \int _{0}^{t}\int _{\varOmega }|\nabla u_{n}|^{2}+\int _{0}^{t}\int _{\varOmega }u_{n}^{q}|\nabla u_{n}|^{2}\le \int _{0}^{t}\int _{\varOmega }f_{n}+\frac{1}{2}\int _{\varOmega }u_{0}^{2}, \end{aligned}$$

as $f_{n}\le f$ and $u_{0}\in L^{\infty }(\varOmega ),$ passing to supremum for $t\in (0,T)$ in the above estimate, we get

$$\begin{aligned} &\frac{1}{2}||u_{n}||_{L^{\infty }(0,T; L^{2}(\varOmega ))}+\alpha \int _{Q}|\nabla u_{n}|^{2}+\int _{Q}u_{n}^{q}|\nabla u_{n}|^{2}\\&\quad \le \int _{Q}f+\frac{1}{2}||u_{0}||_{L^{2}(\varOmega )}^{2}\le C. \end{aligned}$$

(36)

This implies that

$$\begin{aligned} ||u_{n}||_{L^{\infty }(0,T;\, L^{2}(\varOmega ))}\le C \text{ and } ||u_{n}||_{L^{2}(0,T;\, H_{0}^{1}(\varOmega ))}\le C. \end{aligned}$$

(37)

In the other hand by Sobolev embedding Theorem and from (36), we can get

$$\begin{aligned} \int _{Q}u_{n}^{\frac{(q+2)2^{*}}{2}}\le \frac{4S}{(q+2)^{2}}\int _{Q}|\nabla u_{n}^{\frac{q+2}{2}}|^{2}\le \int _{Q}f+\frac{1}{2}||u_{0}||_{L^{2}(\varOmega )}^{2}\le C. \end{aligned}$$

where S the constant of Sobolev embedding, hence the above estimate implies that the boundedness of $u_{n}$ in $L^{\frac{N(q+2)}{N-2}}(Q)$ with respect to n. Then the proof of Lemma 8 is completed. $\square$

Lemma 9

Let $u_{n}$ be a solution of problem (7), with $\gamma =1.$ Suppose that $f\in L^{1}(Q),$ then $u_{n}^{q}|\nabla u_{n}|$ is bounded in $L^{\rho }(Q)$ for every $\rho <N/(N-1).$

Proof

We take $\varphi (u_{n})=T_{1}(u_{n})(1-(1+u_{n})^{1-\lambda }),$ with $\lambda >1,$ as test function in (7), we obtain

$$\begin{aligned}&\displaystyle \int _{\varOmega }\psi (u_{n})+\gamma \displaystyle \int _{Q}T_{1}(u_{n})(1-(1+u_{n})^{1-\lambda })(a(x,t)+u_{n}^{q})|\nabla T_{1}(u_{n})|^{2}\\&\quad +(\lambda -1)\displaystyle \int _{Q}T_{1}(u_{n})(a(x,t)+u_{n}^{q})\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{\lambda }}\\&\qquad =\displaystyle \int _{Q}\frac{f_{n}}{u_{n}+\frac{1}{n}}T_{1}(u_{n})(1-(1+u_{n})^{1-\lambda })+\int _{\varOmega }\psi (u_{0}), \end{aligned}$$

where $\psi (s)=\int _{0}^{s}\varphi (\ell )d\ell .$ Dropping the non-negative terms, from (3) and by the fact that $u_{0}\in L^{\infty }(\varOmega ),$ $\alpha +u_{n}^{q}\ge c_{0}(1+u_{n})^{q},$ we have

$$\int _{Q}T_{1}(u_{n})(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le C\int _{Q}f+C.$$

By working in the set $\{u_{n}\ge 1\}$ and using the above estimate, we get

$$\begin{aligned} \int _{\{u_{n}\ge 1\}}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le \int _{Q}T_{1}(u_{n})^{\gamma }(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le C\int _{Q}f+C. \end{aligned}$$

(38)

The inequality (37) with (38), yields

$$\begin{aligned} \int _{Q}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}&= \int _{\{u_{n}\ge 1\}}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\\&\quad +\int _{\{u_{n}<1 \}}(1+u_{n})^{q-\lambda }|\nabla u_{n}|^{2}\le C. \end{aligned}$$

(39)

Now let us fix $\rho =\frac{N(2+q-\lambda )}{N(q+1)-(\lambda +q)},$ by Hölder’s inequality and (39), we have

$$\begin{aligned} \int _{Q}u_{n}^{q\rho }|\nabla u_{n}|^{\rho }=\int _{Q}\frac{|\nabla u_{n}|^{\rho }}{(1+u_{n})^{\frac{\rho (\lambda -q)}{2}}}(1+u_{n})^{\frac{\rho (\lambda +q)}{2}} \le C\left( \int _{Q}(1+u_{n})^{\frac{\rho (q+\lambda )}{2-\rho }}\right) ^{\frac{2-\rho }{2}}, \end{aligned}$$

applying Sobolev inequality and using the above estimate, we deduce

$$\begin{aligned} \left( \int _{Q}u_{n}^{\rho ^{*}(q+1)} \right) ^{\frac{\rho }{\rho ^{*}}}\le C\left( \int _{Q}u_{n}^{\frac{\rho (q+\lambda )}{2-\rho }}\right) ^{\frac{2-\rho }{2}}. \end{aligned}$$

The previous choice of $\rho$ implies that $\rho ^{*}(q+1)=\frac{\rho (q+\lambda )}{2-\rho },$ and since $\lambda >1,$ we obtain an estimate of $u_{n}^{q}|\nabla u_{n}|$ in $L^{\rho }(Q)$ for every $\rho <N/(N-1).$ $\square$

Theorem 2

Let $\gamma =1$ and f be a function in $L^{1}(Q).$ Then there exists a solution u in $L^{\infty }(0,T;\,L^{2}(\varOmega ))\cap L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\frac{N(q+2)}{N-2}}(Q)$ of problem (1) in the sense of Definition 1.

Proof

By Lemmas 3, 7, 8 and 9, the proof of Theorem 2 is identical to the one of Theorem 1. $\square$

3.3 The strongly singular case γ > 1

In this case we do not have an estimate on $u_{n}$ in $L^{2}(0,T;\, H_{0}^{1}(\varOmega )),$ but we can prove that $u_{n}$ is bounded in $L^{2}(0,T;\, H_{loc}^{1}(\varOmega ))$ such that $u^{\frac{q+\gamma +1}{2}} \in L^{2}(0,T;\, H_{0}^{1}(\varOmega )).$

Lemma 10

Let $u_{n}$ be a solution of the problem (7), with $\gamma >1.$ Suppose that f belongs to $L^{1}(Q),$ then $u_{n}^{\frac{q+\gamma +1}{2}}$ is bounded in $L^{2}(0,T;\, H_{0}^{1}(\varOmega )),$ and $u_{n}$ is bounded in $L^{2}(0,T;\, H_{loc}^{1}(\varOmega ))\cap L^{\infty }(0,T;\, L^{\gamma +1}(\varOmega )).$ Moreover if $q\le \gamma -1,$ then $u_{n}^{q}|\nabla u_{n}|$ is bounded in $L^{2}(w\times (0,T))$ for every $w\subset \subset \varOmega .$

Proof

Choosing $u_{n}^{\gamma }\chi _{(0,t)},$ as test function in (7) with ($0< t\le T).$ Since $0\le \frac{u_{n}^{\gamma }}{(u_{n}+\frac{1}{n})^{\gamma }}\le 1,$ recalling that (3), the fact that $0\le f_{n}\le f$ and the dropping the non-negative term, we have;

$$\begin{aligned}&\frac{1}{\gamma +1}\int _{\varOmega }u_{n}(x,t)^{\gamma +1}+\gamma \int _{0}^{t}\int _{\varOmega }u_{n}^{q+\gamma -1}|\nabla u_{n}|^{2}\\&\quad \le \int _{0}^{t}\int _{\varOmega }\frac{f_{n}u_{n}^{\gamma }}{(u_{n}+\frac{1}{n})^{\gamma }}+\frac{1}{\gamma +1}\int _{\varOmega }u_{0}^{\gamma +1}\le \int _{0}^{t}\int _{\varOmega }f+\frac{1}{\gamma +1}\int _{\varOmega }u_{0}^{\gamma +1}. \end{aligned}$$

Since $u_{0}\in L^{\infty }(\varOmega )$ and passing to supremum in $t\in [0,T],$ we obtain

$$\begin{aligned} \frac{1}{\gamma +1}||u_{n}||_{L^{\infty }(0,T;L^{\gamma +1}(\varOmega ))}+\gamma \int _{Q}u_{n}^{q+\gamma -1}|\nabla u_{n}|^{2}\le \int _{Q}f+\frac{1}{\gamma +1}||u_{0}||_{L^{\gamma +1}(\varOmega )}^{\gamma +1}, \end{aligned}$$

(40)

then we get

$$\begin{aligned} \frac{4}{(q+\gamma +1)^{2}}\int _{Q}|\nabla u_{n}^{\frac{q+\gamma +1}{2}}|^{2}=\int _{Q}u_{n}^{q+\gamma -1}|\nabla u_{n}|^{2}\le \int _{Q}f+\frac{1}{\gamma +1}||u_{0}||_{L^{\gamma +1}(\varOmega )}^{\gamma +1}, \end{aligned}$$

hence

$$\begin{aligned} \int _{Q}|\nabla u_{n}^{\frac{q+\gamma +1}{2}}|^{2}\le C. \end{aligned}$$

The last inequality and (40), imply that $u_{n}^{\frac{q+\gamma +1}{2}}$ is bounded in $L^{2}(0,T;H_{0}^{1}(\varOmega ))$ and $u_{n}$ is bounded in $L^{\infty }(0,T;L^{\gamma +1}(\varOmega ))$ with respect to n. We choose now

$\varphi (u_{n})=u_{n}^{\gamma }(1-(1+u_{n})^{1-(q+\gamma )})$ as test function, dropping the non-negative terms, from (3), we have

$$\begin{aligned} (q+\gamma -1)\int _{Q}u_{n}^{\gamma }(\alpha +u_{n}^{q})\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}\le &\, {} \int _{Q}\frac{f_{n}u_{n}^{\gamma }}{(u_{n}+\frac{1}{n})^{\gamma }}+\int _{\varOmega }\Psi (u_{0})\\\le &\, {} \int _{Q}f+\int _{\varOmega }\Psi (u_{0}), \end{aligned}$$

where $\Psi (s)=\int _{0}^{s}\varphi (\ell )d\ell .$ By working in the set $\{u_{n}\ge 1\}$ and the fact that $u_{0}\in L^{\infty }(\varOmega )$, we get

$$\begin{aligned} \int _{\{u_{n}\ge 1\}}(\alpha +u_{n}^{q+\gamma })\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{q+\gamma }}\le &\, {} \int _{Q}(\alpha u_{n}^{\gamma }+u_{n}^{q+\gamma }) \frac{|\nabla u_{n}|^{2}}{(u_{n}+1)^{q+\gamma }}\\\le &\, {} \int _{Q}f+C, \end{aligned}$$

the above estimate implies

$$\begin{aligned}&\frac{\min (\alpha ,1)}{2^{q+\gamma -1}}\int _{\{u_{n} \ge 1\}}|\nabla u_{n}|^{2}\le \min (\alpha ,1)\int _{\{u_{n}\ge 1\}}\frac{1+u_{n}^{q+\gamma }}{(1+u_{n})^{q+\gamma }}|\nabla u_{n}|^{2}\\&\quad \le C\int _{Q}f+C, \end{aligned}$$

then we get

$$\begin{aligned} \int _{\{u_{n}\ge 1\}}|\nabla u_{n}|^{2}\le C. \end{aligned}$$

(41)

Now we take $(T_{k}(u_{n}))^{\gamma }$ as test function in (7), by (3), Lemma 3 and the fact that $\frac{T_{k}(u_{n})^{\gamma }}{(u_{n}+\frac{1}{n})^{\gamma }}\le \frac{u_{n}^{\gamma }}{(u_{n}+\frac{1}{n})^{\gamma }}\le 1,$ $u_{0}\in L^{\infty }(\varOmega )$ and dropping the nonnegative terms, we obtain

$$\begin{aligned} \alpha C_{w}^{\gamma -1}\int _{0}^{T}\int _{w}|\nabla T_{k}(u_{n})|^{2}\le &\, {} \alpha \int _{Q}T_{k}(u_{n})^{\gamma -1}|\nabla T_{k}(u_{n})|^{2}\\\le &\, {} \int _{Q}f+\frac{1}{\gamma +1}\int _{\varOmega }T_{k}(u_{0})^{\gamma +1} \le \int _{Q}f+\frac{1}{\gamma +1}||u_{0}||_{L^{\gamma +1}(\varOmega )}^{\gamma +1}, \end{aligned}$$

then we get that

$$\begin{aligned} \int _{0}^{T}\int _{w}|\nabla T_{k}(u_{n})|^{2}\le C\;\;\;\; \forall w\subset \subset \varOmega . \end{aligned}$$

(42)

Combining (41) and (42), we can deduce that

$$\begin{aligned} \int _{0}^{T}\int _{w}|\nabla u_{n}|^{2}\le \int _{0}^{T}\int _{w\cap \{u_{n}\ge 1\}}|\nabla u_{n}|^{2} +\int _{0}^{T}\int _{w}|\nabla T_{1}(u_{n})|^{2}\le C \end{aligned}$$

(43)

$\forall w\subset \subset \varOmega ,$ so that $u_{n}$ is bounded in $L^{2}(0,T, H_{loc}^{1}(\varOmega ))$ is achieved. Now going back to (40), we have

$$\begin{aligned} \int _{\{u_{n}\ge 1\}} u_{n}^{q+\gamma -1}|\nabla u_{n}|^{2}\le \int _{Q}u_{n}^{q+\gamma -1}|\nabla u_{n}|^{2} \le \frac{1}{\gamma }\int _{Q}f+\frac{1}{\gamma (\gamma +1)}||u_{0}||_{L^{\gamma +1}(\varOmega )}^{\gamma +1}. \end{aligned}$$

Then we obtain since $2q\le q+\gamma -1$

$$\begin{aligned} \int _{0}^{T}\int _{w}u_{n}^{2q}|\nabla u_{n}|^{2}&\le \int _{0}^{T}\int _{w\cap \{u_{n}\ge 1\}}u_{n}^{q+\gamma -1}|\nabla u_{n}|^{2}\\&\quad +\int _{0}^{T}\int _{w}|\nabla T_{1}(u_{n})|^{2}\le C,\;\;\;\; \forall w\subset \subset \varOmega , \end{aligned}$$

(44)

then the last inequality implies that $u_{n}^{q}|\nabla u_{n}|$ is bounded in $L^{2}(w\times (0,T))$ for every $w\subset \subset \varOmega .$ $\square$

Remark 2

We note that by virtue of Lemma 10 we easily deduce the almost everywhere convergence of $\nabla u_{n}$ to $\nabla u$ following exactly the same proofs as the one of Lemma 7.

Theorem 3

Let $\gamma >1, q\le \gamma -1$ and f be a nonnegative function in $L^{1}(Q).$ Then there exists a nonnegative solution $u\in L^{2}(0,T; H^{1}_{loc}(\varOmega ))$ of problem (1) in the sense of Definition 1. Moreover $u^{\frac{q+\gamma +1}{2}}\in L^{2}(0,T; H_{0}^{1}(\varOmega )).$

Proof

Thanks to Lemmas 3, 7, 10, the proof of Theorem 3 is identical to the one of Theorem 1. $\square$

4 Regularity results

In this section we study the regularity results of solution of problem (1) depending on $q, \gamma >0$ and the summability of f.

Theorem 4

Let $\gamma <1,$ f be a nonnegative function in $L^{m}(Q),$ $1<m<\frac{N}{2}+1.$ Then the solution found in Theorem 1, satisfies the following summabilities:

• (i) If $\frac{2(N+2-q)}{N(q+\gamma +1)+2(2-q)}\le m<\frac{N}{2}+1,$ $q\le 1-\gamma$ then u belongs to $L^{2}(0,T;\, H_{0}^{1}(\varOmega ))\cap L^{\sigma }(Q),$ where

  $$\begin{aligned} \sigma =m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}. \end{aligned}$$

• (ii) If $1<m< \frac{2(N+2-q)}{N(q+\gamma +1)+2(2-q)},$ $q>1-\gamma$ then u belongs to $L^{r}(0,T;\, W_{0}^{1,r}(\varOmega ))\cap L^{\sigma }(Q),$ where

  $$\begin{aligned} r=m\frac{N(q+\gamma +1)+2(\gamma +1)}{N+2-m(1-\gamma )+q(m-1)},\;\;\; \sigma =m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}. \end{aligned}$$

Proof

Let $u_{n}$ be a solution of (7) given by Lemma 2, such that $u_{n}$ converges to a solution of (1). We choose $\varphi (u_{n})=((u_{n}+1)^{\lambda }-1)\chi _{(0,t)},\;\; (\lambda >0)$ as test function in (7), we have

$$\begin{aligned}&\int _{\varOmega }\Psi (u_{n}(x,t))+\lambda \int _{0}^{t}\int _{\varOmega }(1+u_{n})^{\lambda -1}(a(x,t)+u_{n}^{q})|\nabla u_{n}|^{2}\\&\quad \le C\int _{0}^{t}\int _{\varOmega }|f_{n}|u_{n}^{\lambda -\gamma }+\int _{\varOmega }\Psi (u_{0}), \end{aligned}$$

where $\Psi (s)=\displaystyle \int _{0}^{s}\varphi (\ell )d\ell .$ From the condition (3) and the fact that $u_{0}\in L^{\infty }(\varOmega ),\; c_{0}(1+u_{n})^{q}\le \alpha + u_{n}^{q},$ and applying Hölder’s inequality, we obtain

$$\begin{aligned} \int _{\varOmega }\Psi (u_{n}(x,t))&+\lambda c_{0}\int _{0}^{t}\int _{\varOmega }(1+u_{n})^{\lambda +q-1}|\nabla u_{n}|^{2}\\&\quad \le C \left( \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C. \end{aligned}$$

(45)

By the definition of $\Psi (s)$ and $\varphi (s),$ if $\gamma \le 1-q\le \lambda ,$ we can write

$$\begin{aligned} \Psi (s)\ge \frac{|s|^{\lambda +1}}{\lambda +1} \quad \forall s\in \mathbb {R}. \end{aligned}$$

From the above estimate and some simplification the inequality (45), we can estimate as follows

$$\begin{aligned}&\frac{1}{\lambda +1}\int _{\varOmega }[|u_{n}(x,t)|^{\frac{\lambda +q+1}{2}}]^{\frac{2(\lambda +1)}{\lambda +q+1}}+\frac{4\lambda c_{0}}{(\lambda +q+1)^{2}}\int _{0}^{t}\int _{\varOmega }|\nabla u_{n}^{\frac{\lambda +q+1}{2}}|^{2}\\ {}&\quad \le C \left( \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C. \end{aligned}$$

Now passing to supremum for $t\in [0,T],$ we get

$$\begin{aligned} \frac{1}{\lambda +1}|||u_{n}|^{\frac{\lambda +q+1}{2}}||^{\frac{2(\lambda +1)}{\lambda +q+1}}_{L^{\infty }(0,T;L^{\frac{2(\lambda +1)}{\lambda +q+1}}(\varOmega ))}&+\frac{4\lambda c_{0}}{(\lambda +q+1)^{2}}\int _{Q}|\nabla u_{n}^{\frac{\lambda +q+1}{2}}|^{2}\\&\quad \le C \left( \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C. \end{aligned}$$

(46)

By Lemma 1 (where $v=u_{n}^{\frac{\lambda +q+1}{2}}, \; s=\frac{2(\lambda +1)}{\lambda +q+1},\; p=2$), (46), we have

$$\begin{aligned} \displaystyle \int _{Q}[|u_{n}|^{\frac{\lambda +q+1}{2}}]^{2\frac{N+\frac{2(\lambda +1)}{\lambda +q+1}}{N}}\le &\, {} \left( |||u_{n}|^{\frac{\lambda +q+1}{2}}||^{\frac{2(\lambda +1)}{\lambda +q+1}}_{L^{\infty }(0,T;L^{\frac{2(\lambda +1)}{\lambda +q+1}}(\varOmega ))}\right) ^{\frac{2}{N}} \displaystyle \int _{Q}|\nabla u_{n}^{\frac{\lambda +q+1}{2}}|^{2}\\\le &\, {} \left[ C\left( \displaystyle \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C\right] ^{\frac{2}{N}+1}\\\le &\, {} C\left( \displaystyle \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{(\frac{2}{N}+1)\frac{1}{m^{\prime }}}+C, \end{aligned}$$

then, we can obtain

$$\begin{aligned} \int _{Q}|u_{n}|^{\frac{N(\lambda +q+1)+2(\lambda +1)}{N}}\le C\left( \displaystyle \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{(\frac{2}{N}+1)\frac{1}{m^{\prime }}}+C. \end{aligned}$$

(47)

Now choosing $\lambda$ such that

$$\begin{aligned} \sigma =\frac{N(\lambda +q+1)+2(\lambda +1)}{N}=(\lambda -\gamma )m^{\prime }, \end{aligned}$$

(48)

then implies that

$$\begin{aligned} \lambda =\frac{N(q+1)+2+N\gamma m^{\prime }}{Nm^{\prime }-N-2},\;\;\; \sigma =m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}. \end{aligned}$$

By virtue of $m<\frac{N}{2}+1,$ then $(\frac{2}{N}+1)\frac{1}{m^{\prime }}<1,$ and combining (47) and (48) with Young’s inequality, we obtain

$$\begin{aligned} \int _{Q}|u_{n}|^{\sigma }\le C. \end{aligned}$$

(49)

The condition $m\ge \frac{2(N+2-q)}{N(q+\gamma +1)+2(2-q)},$ ensure that $\lambda \ge 1-q\ge \gamma$ and going back to (45), from (48) and (49), we have

$$\begin{aligned} \int _{Q}|\nabla u_{n}|^{2}&\le \int _{Q}(1+u_{n})^{\lambda +q-1}|\nabla u_{n}|^{2}\\&\le C\left( \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C\le C\left( \int _{Q}u_{n}^{\sigma }\right) ^{\frac{1}{m^{\prime }}}+C\le C. \end{aligned}$$

(50)

The estimate (49) and (50), implies that $u_{n}$ is bounded in $L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\sigma }(Q)$ with respect to n,  so $u \in L^{2}(0,T;H_{0}^{1}(\varOmega ))\cap L^{\sigma }(Q).$ Hence the proof of (i) is desired.

Now we prove (ii)

If $\gamma \le \lambda <1-q,$ by definition $\varphi (s),\; \Psi (s),$ we can get

$$\begin{aligned} \Psi (s)\ge C|s|^{\lambda +1}-C, \end{aligned}$$

from the last inequality and going back to (45), we have

$$\begin{aligned}&C\int _{\varOmega }|u_{n}(x,t)|^{\lambda +1}+\lambda c_{0}\int _{0}^{t}\int _{\varOmega }\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{1-\lambda -q}}\\&\quad \le C\left( \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+\int _{\varOmega }\Psi (u_{0})+ Cmeas(\varOmega ), \end{aligned}$$

by the fact that $u_{0}\in L^{\infty }(\varOmega )$ and passing to supremum for $t\in [0,T],$ then we get

$$\begin{aligned} C||u_{n}||^{\lambda +1}_{L^{\infty }(0,T;L^{\lambda +1}(\varOmega ))}&+\lambda c_{0}\int _{Q}\frac{|\nabla u_{n}|^{2}}{(1+u_{n})^{1-\lambda -q}}\\&\quad \le C\left( \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+ C. \end{aligned} $$

(51)

Let $\delta \le 2,$ applying Hölder’s inequality, we have

$$\begin{aligned} \displaystyle \int _{Q}|\nabla u_{n}|^{\delta }&=\int _{Q}\frac{|\nabla u_{n} |^{\delta }}{(1+u_{n})^{\frac{\delta (1-\lambda -q)}{2}}}(1+u_{n})^{\frac{\delta (1-\lambda -q)}{2}}\\&\le \left( \displaystyle \int _{Q}\frac{|\nabla u_{n}|^{2}}{(u_{n}+1)^{1-\lambda -q}}\right) ^{\frac{\delta }{2}}\left( \displaystyle \int _{Q}(1+u_{n})^{\frac{\delta (1-\lambda -q)}{2-\delta }}\right) ^{\frac{2-\delta }{2}}\\&\le C\left( 1+\displaystyle \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{\delta }{2m^{\prime }}}\left( 1+\displaystyle \int _{Q}u_{n}^{\frac{\delta (1-\lambda -q)}{2-\delta }}\right) ^{\frac{2-\delta }{2}}. \end{aligned}$$

(52)

Applying Lemma 1 (where $v=u_{n},\; s=\lambda +1,\; p=\delta$) we get

$$\begin{aligned} \displaystyle \int _{Q}u_{n}^{\frac{\delta (N+\lambda +1)}{N}}&\le ||u_{n}||^{\frac{\delta (\lambda +1)}{N}}_{L^{\infty }(0,T;\,L^{\lambda +1}(\varOmega ))}\int _{Q}|\nabla u_{n}|^{\delta }\\&\le C\left( 1+\displaystyle \int _{Q}u_{n}^{(\lambda -\gamma )m^{\prime }}\right) ^{\frac{\delta }{Nm^{\prime }}+\frac{\delta }{2m^{\prime }}} \left( 1+\displaystyle \int _{Q}u_{n}^{\frac{\delta (1-\lambda -q)}{2-\delta }}\right) ^{\frac{2-\delta }{2}}. \end{aligned}$$

(53)

Let choose $\lambda$ such that

$$\begin{aligned} \sigma =\frac{\delta (N+\lambda +1)}{N}=(\lambda -\gamma )m^{\prime }=\frac{\delta (1-\lambda -q)}{2-\delta }, \end{aligned}$$

(54)

then we deduce

$$\begin{aligned} \lambda =\frac{N(q+1)+2+N\gamma m^{\prime }}{Nm^{\prime }-N-2},\;\; \; \sigma = m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}. \end{aligned}$$

$$\begin{aligned} r=m\frac{N(q+\gamma +1)+2(\gamma +1)}{N+2-m(1-\gamma )+q(m-1)}. \end{aligned}$$

From (54), the inequality (53), becomes

$$\begin{aligned} \int _{Q}u_{n}^{\sigma }\le C\left( 1+\int _{Q}u_{n}^{\sigma }\right) ^{\frac{\delta }{Nm^{\prime }}+\frac{\delta }{2m^{\prime }}+\frac{2-\delta }{2}}. \end{aligned}$$

By virtue of $m<\frac{2(N+2-q)}{N(q+\gamma +1)+2(2-q)},$ ensure that $\frac{\delta }{Nm^{\prime }}+\frac{\delta }{2m^{\prime }}+\frac{2-\delta }{2} <1,$ then applying Young’s inequality we can deduce that

$$\begin{aligned} \int _{Q}u_{n}^{\sigma }\le C. \end{aligned}$$

(55)

We combine (54) and (55) in (52), yields

$$\begin{aligned} \int _{Q}|\nabla u_{n}|^{\delta }\le C. \end{aligned}$$

(56)

Two last inequalities proved that the sequence $u_{n}$ is bounded in $L^{\delta }(0,T; W_{0}^{1,\delta }(\varOmega ))$ $\cap L^{\sigma }(Q),$ and so $u\in L^{\delta }(0,T; W_{0}^{1,\delta }(\varOmega )) \cap L^{\sigma }(Q).$ $\square$

Theorem 5

Let $\gamma =1,$ f be a nonnegative function in $L^{m}(Q),$ $1\le m<\frac{N}{2}+1.$ Then the solution found in Theorem 2, satisfy the following summability $u\in L^{2}(0,T; H_{0}^{1}(\varOmega ))\cap L^{\sigma }(Q)$ with $\sigma =\frac{m(N(q+2)+4)}{N-2m+2}.$

Proof

Let $u_{n}$ be a solution of (7) given by Lemma 2, such that $u_{n}$ converges to a solution of (1). Choosing $u_{n}^{\lambda }\chi _{(0,t)}$ as test function, with $\lambda \ge 1,$ using (3) and applying Hölder’s inequality, we have

$$\begin{aligned}&\frac{1}{\lambda +1}\int _{\varOmega }|u_{n}(x,t)|^{\lambda +1}+\lambda \int _{0}^{t}\int _{\varOmega }(\alpha + u_{n}^{q})u_{n}^{\lambda -1}|\nabla u_{n}|^{2}\\&\quad \le C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+\frac{1}{\lambda +1}\int _{\varOmega }u_{0}^{\lambda +1}, \end{aligned}$$

thanks to $u_{0}\in L^{\infty }(\varOmega )$ and dropping the nonnegative term, we get

$$\begin{aligned}&\frac{1}{\lambda +1}\int _{\varOmega }|u_{n}(x,t)|^{\lambda +1}+\lambda \int _{0}^{t}\int _{\varOmega } u_{n}^{\lambda +q-1}|\nabla u_{n}|^{2}\\&\quad \le C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+\frac{1}{\lambda +1}||u_{0}||_{L^{\lambda +1}(\varOmega )}^{\lambda +1} \le C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C, \end{aligned}$$

by simple simplification the above estimate becomes

$$\begin{aligned}&\frac{1}{\lambda +1}\int _{\varOmega }[|u_{n}(x,t)|^{\frac{\lambda +q+1}{2}}]^{\frac{2(\lambda +1)}{\lambda +q+1}} +\frac{4\lambda }{(\lambda +q+1)^{2}}\int _{0}^{t}\int _{\varOmega }|\nabla u_{n}^{\frac{\lambda +q+1}{2}}|^{2}\\&\quad \le C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C. \end{aligned}$$

Passing to supremum in $t\in [0,T],$ then we obtain

$$\begin{aligned} &\frac{1}{\lambda +1}||u_{n}^{\frac{\lambda +q+1}{2}}||^{\frac{2(\lambda +1)}{\lambda +q+1}}_{L^{\infty }(0,T; L^{\frac{2(\lambda +1)}{\lambda +q+1}}(\varOmega ))}+\frac{4\lambda }{(\lambda +q+1)^{2}}\int _{Q}|\nabla u_{n}^{\frac{\lambda +q+1}{2}}|^{2}\\&\quad \le C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C. \end{aligned}$$

(57)

By Lemma 1 (where $v=u_{n}^{\frac{\lambda +q+1}{2}},\; s=\frac{2(\lambda +1)}{\lambda +q+1}, \; p=2$ ), we use the same proof as before, we get

$$\begin{aligned} \int _{Q}|u_{n}|^{\frac{N(\lambda +q+1)+2(\lambda +1)}{N}}\le \left[ C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}}+C\right] ^{\frac{2}{N}+1} \\ \le C\left( \int _{Q}u_{n}^{(\lambda -1)m^{\prime }}\right) ^{\frac{1}{m^{\prime }}(\frac{2}{N}+1)}+C. \end{aligned}$$

(58)

Choosing $\lambda$ such that

$$\begin{aligned} \sigma =\frac{N(\lambda +q+1)+2(\lambda +1)}{N}=(\lambda -1)m^{\prime }, \end{aligned}$$

(59)

then

$$\begin{aligned} \lambda =\frac{N(q+1)+2+Nm^{\prime }}{Nm^{\prime }-N-2},\;\; \sigma =\frac{m(N(q+2)+4)}{N-2m+2}. \end{aligned}$$

Thanks to (59) and (58), implies that

$$\begin{aligned} \int _{Q}|u_{n}|^{\sigma }\le C\left( \int _{Q}|u_{n}|^{\sigma }\right) ^{\frac{1}{m^{\prime }}(\frac{2}{N}+1)}+C. \end{aligned}$$

The condition $m<\frac{N}{2}+1$ ensure that $\frac{1}{m^{\prime }}(\frac{2}{N}+1)<1$ and $\lambda \ge 1$ implies that $m\ge 1,$ and using Young’s inequality in the above estimate gives

$$\begin{aligned} \int _{Q}|u_{n}|^{\sigma }\le C, \end{aligned}$$

(60)

then we deduce that $u_{n}$ is bounded in $L^{\sigma }(Q)$ and so u belong to $L^{\sigma }(Q).$ $\square$

Theorem 6

Let $\gamma>1, q>\gamma -1$ and f be a nonnegative function in $L^{m}(Q), m>1.$ Then there exists a solution u of problem (1) such that if

$\max (1, \frac{(N+2)(2q-\gamma +1)}{N(q+\gamma +1)+4(q+1)})<m<\frac{N}{2}+1,$ then u belong to $L^{\sigma }(Q)$ with

$$\begin{aligned} \sigma =m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}. \end{aligned}$$

Proof

We will take $u_{n}^{\lambda }\chi _{(0,t)} (\lambda > 1)$ as test function in (7), as in the case $\gamma =1$ we will follow the proof of Theorem 5, repeating the same passage in order to arrive to the inequality

$$\begin{aligned} \int _{Q}|u_{n}|^{\frac{N(\lambda +q+1)+2(\lambda +1)}{N}}\le C\left( \int _{Q}|u_{n}|^{(\lambda -\gamma )m^{\prime }} \right) ^{\frac{1}{m^{\prime }}(\frac{2}{N}+1)}+C. \end{aligned}$$

(61)

We now choose $\lambda$ such that

$$\begin{aligned} \sigma = \frac{N(\lambda +q+1)+2(\lambda +1)}{N}=(\lambda -\gamma )m^{\prime }, \end{aligned}$$

(62)

i.e $\lambda =\frac{N(q+1)+2+N\gamma m^{\prime }}{Nm^{\prime }-N-2},\;\; \sigma =m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}.$ Combining (61) and (62), implies that

$$\begin{aligned} \int _{Q}|u_{n}|^{\sigma }\le C\left( \int _{Q}|u_{n}|^{\sigma } \right) ^{\frac{1}{m^{\prime }}(\frac{2}{N}+1)}+C , \end{aligned}$$

by virtue of $m<\frac{N}{2}+1,$ then we have $\frac{1}{m^{\prime }}(\frac{2}{N}+1)<1$ and $\lambda > 1$ ensure that $m>1,$ then by Young’s inequality, we get

$$\begin{aligned} \int _{Q}|u_{n}|^{\sigma }\le C. \end{aligned}$$

(63)

Hence from (63) it follows that $u_{n}$ is bounded in $L^{\sigma }(Q)$ so that $u\in L^{\sigma }(Q).$ Next we testing (7) by $u_{n}^{\gamma }T_{1}(u_{n}-T_{k}(u_{n})),$ we have

$$\begin{aligned} &\int _{Q}\frac{\partial u_{n}}{\partial t}u_{n}^{\gamma }T_{1}(u_{n}-T_{k}(u_{n}))+\gamma \int _{Q}u_{n}^{\gamma -1}(a(x,t)+u_{n}^{q})|\nabla u_{n}|^{2}T_{1}(u_{n}-T_{k}(u_{n}))\\&\quad +\int _{Q\cap \{k\le u_{n}\le k+1\}}u_{n}^{\gamma }(a(x,t)+u_{n}^{q})|\nabla u_{n}|^{2}=\int _{Q}\frac{f_{n}}{(u_{n}+\frac{1}{n})^{\gamma }}u_{n}^{\gamma }T_{1}(u_{n}-T_{k}(u_{n})). \end{aligned}$$

(64)

Dropping the first and second nonnegative terms in the left hand side of (64) and using the assumption (3), we obtain

$$\begin{aligned} \int _{Q\cap \{u_{n}\ge k\}}u_{n}^{\gamma }|\nabla u_{n}|^{2}\le \frac{1}{\gamma \alpha }\int _{Q\cap \{u_{n}\ge k\}}f+C. \end{aligned}$$

(65)

Thus, thanks to the estimate (65), implies that

$$\begin{aligned} \displaystyle \int _{Q\cap \{u_{n}> k\}}u_{n}^{q}|\nabla u_{n}|&\le \left( \displaystyle \int _{Q\cap \{u_{n}> k\}} u_{n}^{2q-\gamma +1}\right) ^{\frac{1}{2}}\left( \displaystyle \int _{Q\cap \{u_{n}> k\}}u_{n}^{\gamma -1}|\nabla u_{n}|^{2}\right) ^{\frac{1}{2}}\\&\le \left( \displaystyle \int _{Q\cap \{u_{n}> k\}} u_{n}^{2q-\gamma +1}\right) ^{\frac{1}{2}}\left( \frac{1}{\gamma \alpha }\displaystyle \int _{Q\cap \{u_{n}> k\}}f+C\right) ^{\frac{1}{2}}. \end{aligned}$$

Since $u_{n}$ is bounded in $L^{\sigma }(Q),$ then $2q-\gamma +1\le \sigma$ is equivalent to $m\ge \frac{(N+2)(2q-\gamma +1)}{N(q+\gamma +1)+4(q+1)},$ hence we get

$$\begin{aligned} \int _{Q\cap \{u_{n}> k\}}u_{n}^{q}|\nabla u_{n}|\le C\left( \frac{1}{\gamma \alpha }\displaystyle \int _{Q\cap \{u_{n}> k\}}f\right) ^{\frac{1}{2}}. \end{aligned}$$

(66)

Now let $\varphi \in C_{c}^{1}(\varOmega \times [0,T)),\; \varphi \equiv 1$ on $w\times (0,T),\; w\subset \subset \varOmega ,$ and E be a measurable subset of Q,  from (66) and Lemma 10, we can get

$$\begin{aligned}&\displaystyle \int _{E\cap \{w\times (0,T)\}}u_{n}^{q}|\nabla u_{n}|\le \displaystyle \int _{E}u_{n}^{q}|\nabla u_{n}|\varphi \le \displaystyle \int _{Q\cap \{u_{n}> k\}}u_{n}^{q}|\nabla u_{n}|\varphi +k^{q}\displaystyle \int _{E}|\nabla u_{n}|\varphi \\&\quad \le C||\varphi ||_{L^{\infty }}\left( \displaystyle \int _{Q\cap \{u_{n}> k\}}f+C \right) ^{\frac{1}{2}}+||\varphi ||_{L^{\infty }}k^{q}meas(E) ^{\frac{1}{2}}\left( \displaystyle \int _{w\times (0,T)}|\nabla u_{n}|^{2}\right) ^{\frac{1}{2}}. \end{aligned}$$

Taking the limit as $\text{ meas }(E)$ tends to zero, k tend to infinity and since $u_{n}^{q}|\nabla u_{n}|$ converge to $u^{q}|\nabla u|$ almost everywhere, we easily verify thanks to Vitali’s Theorem that

$$\begin{aligned} u_{n}^{q}|\nabla u_{n}|\rightarrow u^{q}|\nabla u|\quad \;\; \text{ strongly } \text{ in } \;\; L^{1}(0,T; L^{1}_{loc}(\varOmega )). \end{aligned}$$

(67)

Therefore, putting together (67), Lemma 3 and Lemma 10, we conclude the proof of Theorem 6. $\square$

Theorem 7

Let $\gamma >1, q\le \gamma -1$ and f be a non-negative function in $L^{m}(Q), 1<m<\frac{N}{2}+1.$ Then the solution found in Theorem3, satisfies the following summability, $u\in L^{\sigma }(Q),$ with $\sigma =m\frac{N(q+\gamma +1)+2(\gamma +1)}{N-2m+2}.$

Proof

The proof of Theorem 7 is similar to proof of item (i) of Theorem 4. $\square$