Establishment of EOQ (Economic Order Quantity) Model for Spoilage Products and Power Demand Under Permissible Delay in Payments

Abstract

The demand for a service is the amount of it that a consumer will purchase or will be ready to purchase at different prices at a given moment of time. An EOQ model for spoilage commodities and power demand under trade credits is established. Mathematical model is established to obtain optimal ordering policies for policies for retailer under two different cases. In this model buyer who purchases the commodities enjoy a fixed period offered by his/her vendor. We show that total profit function is concave with respect to time. We then provide for finding maximum profit. Numerical examples are provided of the optimal solution to find order quantity and total profit. Sensitivity analysis of the key parameters is presented to validate the model.

Introduction

In high tech business transaction industries found that they can get more advantages by establishing steady and long term relationship between retailer and supplier. Thus, it is a powerful and incremental tool to get more profit. Therefore inventory model is an excellent model for both seller and buyer. In traditional Economic Order Quantity (EOQ) models, it is assumed that the demand rates of commodities be either constant or time induced.But in actual practice it may be stock-sensitive. In recent days, changeable demand is attracting the researchers due to maintaining inventory very crucial.

Every item in the universe deteriorates over time. While the rate of deterioration of some items may be small. Therefore, the effect of deterioration cannot ignore in the study of inventory policy, otherwise it will cause inaccurate results. Jaggi and Aggarwal [1] established the economic ordering policies considering discounted cash flow approach. Jaggi et al. [2] established an EOQ of deteriorating items under inflation. Ghare and Schrader [3] first proposed an inventory model with constant deterioration rate over a finite planning horizon. Covert and Philip [4] generalized a model considering variable deterioration rate. Researchers like: Holler and Mak [5], Datta and Pal [6], Dye et al. [7], Philip [8], Chakrabarty and Chaudhuri [9], Giri and Chaudhuri [10], Deb and Chaudhuri [11], Chung and Ting [12], Hariga [13], Hariga and Benkherouf [14], Jalan and Chaudhuri [15], Roy [16].

At present in high tech business transaction, supplier offers their buyers a fixed period with interest in between the credit period. Two benefits are produced in case of permissible delay in payment: (1) it motivates more buyers that consider it a price discount and (2) it is applicable an alternative discount price. Shinn et al. [17] provided an EOQ model considering quantity discount for freight charges. Teng et al. [18] developed an EOQ model with progressive demand. Khanra et al. [19] focused an EOQ model for deteriorating items with time-sensitive demand under permissible delay in payments. Tripathi [20] established “EOQ model for optimal payment time for a retailer with exponential demand under permitted credit period by the whole seller”. Tripathi and Mishra [21] presented an inventory model for deteriorating items with inventory sensitive demand. Shah [22] considered a stochastic EOQ model under trade credits. Several related papers can be seen in Chung [23], Jamal et al. [24], Hwang and Shinn [25], Chung and Teng [26], Chung and Liao [27], Ouyang et al. [28] etc.

In the classical EOQ model demand is always constant. In actual practice, it is in dynamic stage and may not always constant. Demand expresses the functional relational ship between price and quantity demanded. Price of an item is the most important factor affecting the demand for a commodity. Generally, demand for an item increases, when its price falls. In the same way, if the price increases the demand will fall. Variation in the price of a commodity may result in the change of demand for that commodity. Demand may occur due to factors other than price. Silver and Meal [29] first considered a generalization of the inventory model for the case of a varying demand. Jalan and Chaudhuri [30] established inventory model considering exponentially time varying demand pattern. Ghosh and Chaudhuri [31] presented EOQ models considering time- quadratic demand rate. Soni and Shah [32] presented an EOQ model retailer when customer demand is stock-induced and when supplier offers two progressive credit periods. Tripathi and Singh [33] developed EOQ model for inventory-induced demand rate. Soni [34] established inventory model for optimal replenishment policies for spoilage products with stock-sensitive demand. Hou [35] derived a model for deteriorating items with stock-dependent consumption rate and shortages under inflation and time discounting. Padmanabhan and Vrat [36] pointed out inventory models for perishable commodities for stock-dependent selling rate. The notable researchs were addressed by Kim et al. [37], Noh [38], Cheikhrouhou et al. [39]. Sarkar [40, 41], Kang [42] Sarkar and Saren [43], Sarkar et al. [44,45,46], Shin et al [47], Tayyab and Sarkar [48], Sett et al. [49], Datta and Pal [50], Dye and Ouyang [51], Taleizadeh et al. [52], Tripathi [53] and others (Table 1).

Table 1 Major characteristics of inventory models of different authors is this research field including this work

The problem of determining the total profit with stock- dependent demand (power demand pattern) is addressed in this paper in a manner that reflects realistic situation. Thus this model has a new managerial insight that helps a manufacturing system to generate maximum profit. The rest of the paper is designed as follows: in “Notation and Assumptions” section fundamental notations and assumption are provided. In “Mathematical Formulation” section, Mathematical model is shown. Optimal solution is discussed in “Determination of Optimal Solution” section followed by solution algorithm. Numerical examples and sensitivity analysis are given in “Solution Algorithm and Numerical Examples” sections respectively. Conclusions are made in the last section.

Notation and Assumptions

Notations

$c, p,\,\mathrm{and}\,h$ :

Unit purchase, selling and holding cost (Rs./unit/year)

$\theta$ :

Constant deterioration rate of an item (0 $\le$ $\theta$ $\le$ 1)

A :

Replenishment cost of item (Rs./order)

$D\{I(t)\}=\alpha \{I(t)\}^{\beta }$ :

Demand rate which is inventory dependent $\alpha$ > 0, 0 $\le$ $\beta$ $\le$ 1, where $\alpha$ is initial demand (for $\beta$ = 0)

M :

Permissible delay period (in years)

T :

Time interval between (in years)

$I_{c}$ :

Interest charges/unit/year (in Rs.)

$I_{d}$ :

Interest earned unit/year (in Rs.) ($I_{c}>I_{d})$

I(t) :

Inventory level at instant t

Q :

Lot-size (in units)

RC :

Replenishment cost/unit time (in Rs.)

DC :

Deterioration cost (in Rs.)

SR :

Sales revenue (Rs./year)

${IP}_{1 }\,\mathrm{and}\,{IP}_{2}$ :

Interest payable (Rs./unit/year for case I and II respectively

${IE}_{1}\,\mathrm{and}\,{IE}_{2 }$ :

Interest earned (Rs./unit/year for case I and II respectively

$Z_{1}{(T)},\,\mathrm{and}\,Z_{2}(T)$ :

Total variable profit (in Rs.) for case I and II respectively

$T_{1}^{*}\,\mathrm{and}\,T_{2}^{*}$ :

Optimal T for case I and II

$Z_{1}^{*}(T_{1}^{*})\,\mathrm{and}\,Z_{2}^{*}(T_{2}^{*})$ :

Optimal $Z_{1}(T)\,\mathrm{and}\, Z_{2}(T)$ respectively

Assumptions

• (i) Deterioration rate is constant and 0 $\le$ $\theta$ $\le$ 1 per unit time.

• (ii) Demand rate is inventory dependent of the item.

• (iii) Selling price is greater than purchase cost ($p>c)$.

• (iv) Inventory is considered for single item.

Mathematical Formulation

The rate of decrease of inventory I(t) at time t is:

$$\begin{aligned} \frac{dI(t)}{dt}=-\theta I(t)-D\{I(t)\},\quad {0}\le \hbox {t}\le \hbox { T} \end{aligned}$$

(1)

The solution of (1) with the condition I(T) = 0, is

$$\begin{aligned} \hbox {I(t)=}\left( {\alpha /\theta } \right) ^{1/{(1-\beta )}}\left\{ {e^{\theta (1-\beta )(T-t)}-1} \right\} ^{1/{(1-\beta )}} \end{aligned}$$

(2)

The order quantity Q is

$$\begin{aligned}&\hbox {Q = }\left( {\alpha /\theta } \right) ^{1/{(1-\beta )}}\left\{ {e^{\theta (1-\beta )T}-1} \right\} ^{1/{(1-\beta )}} \end{aligned}$$

(3)

$$\begin{aligned}&\hbox {The replenishment cost } {RC}~=~A \end{aligned}$$

(4)

$$\begin{aligned}&\hbox {The sales revenue } SR=\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )} \hbox { (see appendix A}_{3})\nonumber \\ \end{aligned}$$

(5)

$$\begin{aligned}&\hbox {Total demand during one cycle is} =\frac{\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )} \end{aligned}$$

(6)

$$\begin{aligned}&\hbox {Number of deteriorated units}~=~Q-\int \limits _0^T {\alpha \{I(t)\}^{\beta }dt}\nonumber \\&\qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad =\frac{\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}\theta (1-\beta )T}{(2-\beta )} \end{aligned}$$

(7)

$$\begin{aligned}&\hbox {Deteriorated cost } ({DC}), \hbox { in } [0, T] \hbox { is } =\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )} \end{aligned}$$

(8)

$$\begin{aligned}&\hbox {The holding cost } ({HC}), \hbox {during } [0, T] \hbox { is }\nonumber \\&\quad HC =h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} \end{aligned}$$

(9)

The following two cases may arise depending on credit period

Case I: T ${\varvec{>}}$ M

In this case, the cycle time is greater than credit period (M), the interest is payable during (T–M), the interest payable in [0, T] is:

$$\begin{aligned} IP_1 =cI_c \int \limits _M^T {I(t)dt} =cI_c \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )(T-M)} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta (T-M)}{2(3-2\beta )}} \right\} \end{aligned}$$

(10)

The interest earned (IE$_{1})$ in between [0, T] is:

$$\begin{aligned} IE_1 =\frac{pI_d \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{2-\beta }\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} \end{aligned}$$

(11)

The total variable profit/ unit time is:

$$\begin{aligned} Z_1 (T)= & {} \frac{1}{T}\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. \nonumber \\&\quad -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad -\,h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} \nonumber \\&\quad -\,cI_c \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )(T-M)} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta (T-M)}{2(3-2\beta )}} \right\} \nonumber \\&\quad \left. {+\,\frac{pI_d \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{2-\beta }\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} } \right] \end{aligned}$$

(12)

Case II: T ${\varvec{\le }}$ M

In this, case, the cycle time (T) is less than credit period (M) customer earns interest on the sales revenue and no interest is payable (i.e. IP$_{2}$ = 0). Therefore, interest earned in [0, M] is:

$$\begin{aligned} pI_d \int \limits _0^T {t.D(t)dt+pI_d (M-T)\int \limits _0^T {D(t)dt} } \end{aligned}$$

(13)

Therefore, the interest earned IE$_{2}$ is:

$$\begin{aligned} IE_2= & {} pI_d \left[ \frac{\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{(2-\beta )}\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} \right. \nonumber \\&\left. +\frac{(M-T)\left\{ {\alpha (1-\beta )T} \right\} ^{1/{(1-\beta )}}(4-2\beta +\theta \beta T)}{2(2-\beta )} \right] \end{aligned}$$

(14)

The total variable profit/cycle is:

$$\begin{aligned} Z_2 (T)= & {} \frac{1}{T}\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. \nonumber \\&\quad -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad \left. -\,{h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} } \right] \nonumber \\&\quad +\,\frac{pI_d }{T}\left[ \frac{\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{(2-\beta )}\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} \right. \nonumber \\&\quad \left. +\,\frac{(M-T)\left\{ {\alpha (1-\beta )T} \right\} ^{1/{(1-\beta )}}(4-2\beta +\theta \beta T)}{2(2-\beta )} \right] \end{aligned}$$

(15)

Case III: Let ${\varvec{T}}~{\varvec{=}~{\varvec{M}}}$

At $T~=~M$, $Z_{1}(T)$ and $Z_{2}(T)$ are equal i .e. $Z_{1}(T)~=~Z_{2}(T)$. Substituting $T~=~M$ in Eqs. (12) or (16), we get

$$\begin{aligned} Z_3 (T)= & {} \frac{1}{T}\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. \nonumber \\&\quad -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad \left. -\,{h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} } \right] \nonumber \\&\quad +\,\frac{pI_d }{T}\left[ {\frac{\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{(2-\beta )}\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} } \right] \end{aligned}$$

(16)

Or

$$\begin{aligned} Z_3 (M)= & {} \frac{1}{M}\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta M)}{2(2-\beta )}} \right. \nonumber \\&\quad -\,A-\frac{c\theta (1-\beta )M\{\alpha (1-\beta )M\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad \left. -\,{h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )M} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta M}{2(3-2\beta )}} \right\} } \right] \nonumber \\&\quad +\,\frac{pI_d }{T}\left[ {\frac{\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )M} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{(2-\beta )}\left\{ {1+\frac{\beta \theta M}{2(3-2\beta )}} \right\} } \right] \end{aligned}$$

(17)

Determination of Optimal Solution

Differentiating $Z_{1}(T)$ and $Z_{2}(T)$ from Eqs. (12) and (15) with respect to T, we get

$$\begin{aligned} \frac{dZ_1 (T)}{dT}= & {} -\frac{1}{T^{2}}\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. \nonumber \\&\quad -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad -\,h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} \nonumber \\&\quad -\,cI_c \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )(T-M)} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta (T-M)}{2(3-2\beta )}} \right\} \nonumber \\&\quad \left. {+\,\frac{pI_d \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{2-\beta }\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} } \right] \nonumber \\&\quad +\,\frac{\alpha ^{1/{1-\beta }}}{2T}\left[ {p\left\{ {(1-\beta )T} \right\} ^{\beta /{1-\beta }}(2+\beta \theta T)} \right. \nonumber \\&\quad -\,\{(1-\beta )T\}^{1/{1-\beta }}\left\{ {2c\theta +h(2+\theta T)-\frac{pI_d (4-2\beta +\theta T)}{2-\beta }} \right\} \nonumber \\&\quad \left. -\,cI_c \{(1-\beta )(T-M)\}^{1/{1-\beta }}\left\{ {2+\theta (T-M)} \right\} \right] \end{aligned}$$

(18)

$$\begin{aligned} \frac{dZ_2 (T)}{dT}= & {} -\frac{1}{T^{2}}\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. \nonumber \\&\quad -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad -\,\left. {h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} } \right] \nonumber \\&\quad -\,\frac{pI_d }{T^{2}}\left[ {\frac{\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{(2-\beta )}\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} } \right. \nonumber \\&\quad \left. {+\,\frac{(M-T)\left\{ {\alpha (1-\beta )T} \right\} ^{1/{(1-\beta )}}(4-2\beta +\theta \beta T)}{2(2-\beta )}} \right] \nonumber \\&\quad +\,\frac{\alpha ^{1/{1-\beta }}}{2T}\left[ {p\left\{ {(1-\beta )T} \right\} } \right. ^{\beta /{(1-\beta )}}(2+\beta \theta T)\nonumber \\&\quad -\,\left\{ {(1-\beta )T} \right\} ^{1/{(1-\beta )}}\left\{ {2c\theta +h(2+\theta T)-\frac{pI_d (4-2\beta +\theta T)}{2-\beta }} \right\} \nonumber \\&\quad +\,\frac{pI_d \left\{ {(1-\beta )T} \right\} ^{\beta /{(1-\beta )}}}{(2-\beta )}\left\{ \left( {\beta T+M-2T} \right) (4-2\beta +\beta \theta T)\right. \nonumber \\&\quad \left. \left. +\,(1-\beta )\beta \theta (M-T) \right\} \right] \end{aligned}$$

(19)

The main aim is to find the maximum value of profit. The maximum value of $Z_{i}(T)$ for given $T~=~T_{i}^{*}, i~=~1,2$, are obtained by solving $\frac{dZ_i (T)}{dT}=0$ for T,  provided $\frac{d^{2}Z_i (T)}{dT^{2}}<0$, (see “Appendix”).

Putting $\frac{dZ_i (T)}{dT}=0$, i = 1,2, from Eqs. (18) and (19), we obtain

$$\begin{aligned}&\alpha ^{1/{1-\beta }}T\left[ {p\left\{ {(1-\beta )T} \right\} ^{\beta /{1-\beta }}(2+\beta \theta T)} -\{(1-\beta )T\}^{1/{1-\beta }}\right. \nonumber \\&\qquad \left. \left\{ {2c\theta +h(2+\theta T)-\frac{pI_d (4-2\beta +\theta T)}{2-\beta }} \right\} \right. \nonumber \\&\quad \left. -\,{cI_c \{(1-\beta )(T-M)\}^{1/{1-\beta }}\left\{ {2+\theta (T-M)} \right\} } \right] \nonumber \\&\quad -\,2\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad -\,h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} \nonumber \\&\quad -\,cI_c \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )(T-M)} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta (T-M)}{2(3-2\beta )}} \right\} \nonumber \\&\quad \left. {+\frac{pI_d \alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{2-\beta }\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} } \right] =0 \end{aligned}$$

(20)

$$\begin{aligned}&\alpha ^{1/{1-\beta }}T\left[ {p\left\{ {(1-\beta )T} \right\} } \right. ^{1/{(1-\beta )}}(2+\beta \theta T)-\left\{ {(1-\beta )T} \right\} ^{1/{(1-\beta )}}\nonumber \\&\qquad \left\{ {2c\theta +h(2+\theta T)-\frac{pI_d (4-2\beta +\theta T)}{2-\beta }} \right\} \nonumber \\&\quad +\,\left. {\frac{pI_d \left\{ {(1-\beta )T} \right\} ^{\beta /{(1-\beta )}}}{(1-\beta )(2-\beta )}\left\{ {\left( {\beta T+M-2T} \right) (4-2\beta +\beta \theta T)+(1-\beta )\beta \theta (M-T)} \right\} } \right] \nonumber \\&\quad -\,2\left[ {\frac{p\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}(4-2\beta +\beta \theta T)}{2(2-\beta )}} \right. -\,A-\frac{c\theta (1-\beta )T\{\alpha (1-\beta )T\}^{1/{(1-\beta )}}}{(2-\beta )}\nonumber \\&\quad -\,\left. {h\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}\left\{ {\frac{1}{2-\beta }+\frac{\theta T}{2(3-2\beta )}} \right\} } \right] \nonumber \\&\quad -\,2pI_d \left[ {\frac{\alpha ^{1/{(1-\beta )}}\left\{ {(1-\beta )T} \right\} ^{{(2-\beta )}/{(1-\beta )}}}{(2-\beta )}\left\{ {1+\frac{\beta \theta T}{2(3-2\beta )}} \right\} } \right. \nonumber \\&\quad \left. {+\,\frac{(M-T)\left\{ {\alpha (1-\beta )T} \right\} ^{1/{(1-\beta )}}(4-2\beta +\theta \beta T)}{2(2-\beta )}} \right] ~=~0. \end{aligned}$$

(21)

The following algorithm is established to obtain the $Q^{*}$ and $Z^{*}(T^{*})$.

Solution Algorithm

Step 1 :

: Initialize the parameters

Step 2 :

: Find $T_{1}^{*}$ from Eq. (21), if $T_{1}^{*}~>~M$, calculate $Z_{1}^{*}(T_{1}^{*})$ from Eq. (12)

Step 3 :

: Find $T_{2}^{*}$ from Eq. (21), if $T_{2}^{*}~\le ~M$, calculate $Z_{2}^{*}(T_{2}^{*})$ from Eq. (15)

Step 4 :

: If $T_{1}^{*}~>~M$, and $T_{2}^{*}~\le ~M$ is satisfied, compare $Z_{1}^{*}(T_{1}^{*})$, $Z_{2}^{*}(T_{2}^{*})$ and obtain the maximum profit.

Step 5 :

: If $\hbox {T}_{1}^{*}$ > M is satisfied and $T_{2}^{*}~>~M$ is not satisfied, then $Z_{1}^{*}(T_{1}^{*})$ is the maximum profit

Step 6 :

: If $\hbox {T}_{1}^{*}<M$ is not satisfied and $T_{2}^{*}~\le ~M$ is satisfied, then $Z_{2}^{*}(T_{2}^{*})$ is the maximum profit

Step 7 :

: Compare $Q (T_{1}^{*})$ and $Q (T_{2}^{*})$ for corresponding maximum profit

The following examples are given to validate the above algorithm:

Numerical Examples

Following three examples discussed below cover all three cases. The numerical data is taken from the previous literature survey:

Example 1

In this inventory system, let us take $\alpha$ = 1000 units/ year, $\beta$ = 0.5, c = 40 units/year, A = Rs. 200/ order, $I_{c}$ = 0.15/ year, $I_{d}$ = 0.13/year, h = Rs. 150/year, p = Rs.50/units, $\theta$ = 0.20 and M = 0.35 year.

Putting these values in (20) and solving for T, we get $T_{1}^{*}$ = 0.483832 year, corresponding $Z_{1}(T_{1}^{*})$ = Rs. $3.08245\,\times \,10^{6}$ and $Q(T_{1}^{*})$ = 61381.1 units.

Also, substituting these above parameter values is (21), and solving for T, we obtain $T_{2}^{*}$ = 0.42826 year, corresponding $Z_{2}(T_{2}^{*})$ = Rs. $2.97985\,\times \,10^{6}$ and $Q(T_{2}^{*})$ = 46156.4 units.

Here $T_{2}^{*}~>~M$, which contradicts the assumption of case II, thus only case I holds as $T_{1}^{*}~>~M$. Therefore, $Z_{1}(T_{1}^{*})$ = Rs. $3.08245\,\times \,10^{6}$, in which $T_{1}^{*}$ = 0.483832 year and $Q(T_{1}^{*})$ = 61381.1 units.

Example 2

Let us consider $\alpha$ = 1000 units, $\beta$ = 0.5, c = 40, A = 200, $I_{c}$ = 0.15, $I_{d}~$= 0.13, h = . 230, p = 50, $\theta$ = 0.20 and M = 0.30 year in appropriate units.

Putting these values in (20) and solving for T, we get, $T_{1}^{*}$ = 0.31971 year, $Z_{1}(T_{1}^{*})$ = Rs. $2.01991\,\times \,10^{6}$ and $Q(T_{1}^{*})$ = 26377.1 units.

Also, substituting these above parameter values is Eq. (21), and solving for T, we obtain $T_{2}^{*}~$= 0.296152 year, corresponding $Z_{2}(T_{2}^{*})$ = Rs. $2.01066\,\times \,10^{6}$ and $Q(T_{2}^{*})$ = 22580.7 units.

Here $T_{1}^{*}~>~M$, and $T_{2}^{*}~\le ~M$, both cases are satisfied. Since $Z_{1}(T_{1}^{*})~>~Z_{2}(T_{2}^{*}),$therefore, the $Z_{1}(T_{1}^{*})$ = Rs. $2.01991\,\times \,10^{6}$, in which the maximum, cycle time is $T_{1}^{*}$ = 0.31971 year and optimal $Q(T_{1}^{*})$ = 26377.1 units.

Example 3

Let us consider $\alpha$ = 1000, $\beta$ = 0.5, c = 40, A = 200, $I_{c}$ = 0.15, $I_{d}$ = 0.13, h = 250, p = 50, $\theta$ = 0.20 and M = 0.30 year, in appropriate units.

Substituting these values in Eq. (20) and solving for T, we get, $T_{1}^{*}$ = 0.294609 year, corresponding $Z_{1}(T_{1}^{*})$ = Rs. $1.85942\,\times \,10^{6}$ and $Q(T_{1}^{*})$ = 22342.6 units.

Also, substituting these above parameter values is Eq. (21), and solving for T,  we obtain $T_{2}^{*}~$= 0.276562 year, corresponding $Z_{2}(T_{2}^{*})$ = Rs. $1.86301\,\times \,10^{6}$ and $Q(T_{2}^{*})$ = 19654.1 units.

Here $T_{1}^{*}<M$, which contradicts the assumption of case I, thus only case II holds as $T_{2}^{*}<M$. Therefore, the $Z_{2}(T_{2}^{*})$ = Rs. $1.86301\,\times \,10^{6}$, in which the maximum, cycle time is $T_{2}^{*}$ = 0.276562 year and the optimal $Q(T_{2}^{*})$ = 19654.1 units.

Example 4

Let us consider $\alpha$ = 1000, $\beta$ = 0.5, c = 40, A = 200, $I_{c}$ = 0.15, $I_{d}$ = 0.13, h = 150, p = 50, $\theta$ = 0.20 and $T~=~M$ in appropriate units.

Substituting these values in Eqs. (20) or (21) and solving for Tor M, we get $T_{1}^{*} = T_{2}^{*}= M^{*}$ = 0.48572 year, which is the case III. Thus the maximum average profit is $Z(M^{*})$ = Rs. 3.08497 x10$^{6}$, in which optimal cycle time is $T^{*}$ = 0.48572 year and optimal $Q(T^{*})$ = 61880.6 units.

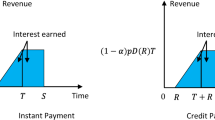
The following Figs. 1 and 2 are given for case I and II respectively

Fig. 1

Graph of $Z_{1}(T)$ with $T~>~M$ ($M=0.35$ years)

Fig. 2

Graph of $Z_{2}(T)$ with $T<M$ ($M=0.35$ years)

Sensitivity Analysis

Sensitivity analysis is established for case I, considering the rest parameters at their original values as in Example 1.

Sensitivity analysis for case II: Sensitivity analysis is established considering the numerical data as in Example 2.

Table 2 Variation of $T_1^{*}$ , $Q(T_1^*)$ and $Z_{1}(T_1^*)$ with $\alpha , c, p, M, h$ and A

Table 3 Variation of $T_2^{*}$ , $Q(T_2^*)$ and $Z_{2}(T_2^*)$ with $\alpha , c, p, M, h$ and A

Based on Tables 2 and 3, following inferences can be made:

• (i) We see that if initial demand $\upalpha$, unit selling price (p) and credit period (M), will increase, total profit will also increase. It means that $Z_{1}(T_{1}^{*})$ and $Z_{2}(T_{2}^{*})$ are quite sensitive to change in $\upalpha$, and $T_{1}^{*}$, $T_{2}^{*}$ are moderately sensitive with $\alpha$, p, and M.

• (ii) We observe that if, unit holding cost (h), unit purchase cost (c), replenishment cost (A) will increase, total profit $Z_{1}(T_{1}^{*})$ and $Z_{2}(T_{2}^{*})$ will decrease. It means that $Z_{1}(T_{1}^{*})$ and $Z_{2}(T_{2}^{*})$ are sensitive with h, c and A, and $T_{1}^{*}$ is approximately insensitive to change in h and A.

Conclusion

This model is based on inventory dependent demand. Most of the EOQ models are considered that demand rate remain constant. If $\beta$ = 0, the demand becomes constant. However, at present, the demand rate of items increases during growth of production process. In this paper, we have provided an EOQ model for spoilage commodities trade credits. An algorithm is discussed to obtain the order quantity and total profit. Numerical examples are given to illustrate the applicability solution algorithm. Sensitivity analysis has been discussed with variation of several key parameters. Several managerial phenomena have also pointed out:

• Increase in, initial demand, unit selling price, and credit period, will lead increase in total profit.

• Increase in unit holding cost, unit purchase cost and replenishment cost will cause decrease in total profit.

Appendices

Appendix A$_{1}$

We first to prove the Appendix $A_{1}$ for following Lemma 1.

Lemma 1

Let $H(T)=\frac{\phi (T)}{T}$, where $\phi (T)$is continuous and twice differentiable function of T, then the maximum value of H(T) exists at $T~=~T^{*}$, if $\frac{1}{T}\frac{d^{2}\phi (T)}{dT^{2}}<0$, at $T~=~T^{*}.$

Proof

$$\begin{aligned} \hbox {We have } H(T)=\frac{\phi (T)}{T} \end{aligned}$$

(i)

Differentiating (i) w.r.t. T, we have

$$\begin{aligned} \frac{dH(T)}{dT}=\frac{1}{T^{2}}\left\{ {T\frac{d\phi (T)}{dT}-\phi (T)} \right\} \end{aligned}$$

(ii)

The necessary condition for extremum is $\frac{dH(T)}{dT}=0$, from (ii) we get

$$\begin{aligned} T\frac{d\phi (T)}{dT}-\phi (T)=0 \end{aligned}$$

(iii)

Let Eq. (iii) is satisfied at $T~=~T^{*}$.

Again differentiating (ii) we get

$$\begin{aligned} \frac{d^{2}H(T)}{dT^{2}}=\frac{1}{T^{3}}\left\{ {T^{2}\frac{d^{2}\phi (T)}{dT^{2}}+2\left( {T\frac{d\phi (T)}{dT}-\phi (T)} \right) } \right\} \end{aligned}$$

(iv)

But at $T~=~T^{*}$, $T\frac{d\phi (T)}{dT}-\phi (T)=0$

From (iv), we get $\frac{dH(T)}{dT}=\frac{1}{T}\frac{d^{2}\phi (T)}{dT^{2}}$

The sufficient condition for maximum value of H(T) is $\frac{d^{2}H(T)}{dT^{2}}<0$.

Hence we have proved the Lemma. $\square$

We have

$$\begin{aligned} \frac{d^{2}Z_1 (T)}{dT^{2}}= & {} \frac{1}{T}\left( {\frac{d^{2}SR}{dT^{2}}-\frac{d^{2}RC}{dT^{2}}-\frac{d^{2}CD}{dT^{2}}-\frac{d^{2}HC}{dT^{2}}-\frac{d^{2}IP_1 }{dT^{2}}+\frac{d^{2}IE_1 }{dT^{2}}} \right) \nonumber \\= & {} \frac{\left\{ {\alpha (1-\beta )} \right\} ^{1/{1-\beta }}T^{{(3\beta -2)}/{(1-\beta )}}}{1-\beta }\nonumber \\&\quad \left[ \frac{p\left\{ {\beta (2+\theta T)+I_d T(1-\beta )(2+\beta \theta T)} \right\} }{2(1-\beta )}\left( {1+I_d T} \right) \right. \nonumber \\&\quad -\,c\theta T- \frac{h}{2}\left( {2+2\theta T-\beta \theta T} \right) \nonumber \\&-\,\left. {\frac{cI_c }{2}\left( {1-\frac{M}{T}} \right) ^{\beta /{(1-\beta )}}T\left\{ {2+(2-\beta )\theta (T-M)} \right\} } \right] \nonumber \\&\quad \frac{d^{2}Z_1 (T)}{dT^{2}}<0, \hbox {if}\nonumber \\&\quad (1-\beta )\left[ 2c\theta T+h\left( {2+2\theta T-\beta \theta T} \right) \right. \nonumber \\&\quad +\,\left. cI_c \left( {1-\frac{M}{T}} \right) ^{\beta /{(1-\beta )}}T\left\{ {2+(2-\beta )\theta (T-M)} \right\} \right] .\nonumber \\&\quad -\,p\left\{ {\beta \left( {2+\theta T} \right) I_d T(1-\beta )(2+\beta \theta T)} \right\} >0 \end{aligned}$$

Appendix A$_{2}$

We have $Z_2 (T)=\frac{1}{T}\left( {SR-RC-DC-HC+IE_2 } \right)$

For maximum or minimum of $Z_{2}(T), \frac{dZ_2 (T)}{dT}=0$, if $T~=~T_{2}^{*}$ be maximum value of $Z_{2}(T),$ then at $T~=$ $T_{2}^{*}$, we have

$$\begin{aligned}&\hbox {We have }\frac{d^{2}Z_2 (T)}{dT^{2}}=\frac{1}{T}\left( {\frac{d^{2}SR}{dT^{2}}-\frac{d^{2}RC}{dT^{2}}-\frac{d^{2}CD}{dT^{2}}-\frac{d^{2}HC}{dT^{2}}+\frac{d^{2}IE_2 }{dT^{2}}} \right) \\&=\frac{\left\{ {\alpha (1-\beta )} \right\} ^{1/{1-\beta }}T^{{(3\beta -2)}/{(1-\beta )}}}{1-\beta }\left[ \frac{p\left\{ {\beta (2+\theta T)+I_d T(1-\beta )(2+\beta \theta T)} \right\} }{2(1-\beta )}\left( {1+I_d T} \right) \right. \\&\quad -\,c\theta T- \frac{h}{2}\left( {2+2\theta T-\beta \theta T} \right) \\&\quad +\,\left. {\left\{ {\frac{\beta }{(1-\beta )}\left( {4-2\beta +\theta \beta T} \right) +2(\beta \theta M-2\beta \theta T-4+2\beta )T-2(1-\beta )\theta T^{2}} \right\} } \right] \\&\quad \frac{d^{2}Z_2 (T)}{dT^{2}}<0, \hbox { if}\\&\quad (1-\beta )\left\{ {2c\theta T+h\left( {2+2\theta T-\beta \theta T} \right) } \right\} -p\left\{ {\beta \left( {2+\theta T} \right) I_d T(1-\beta )(2+\beta \theta T)} \right\} \\&\quad -\, (1-\beta )\left\{ \frac{\beta }{(1-\beta )}\left( {4-2\beta +\theta \beta T} \right) +\,2(\beta \theta M-2\beta \theta T-4+2\beta )T-2(1-\beta )\theta T^{2} \right\} >0 \\ \end{aligned}$$

Appendix A $_{3}$

It is very difficult to handle the total profit function and its elements for finding closed form optimal solution. Truncated Taylor’s series expansions are considered for exponential terms to find closed form optimal solution. For low deterioration rate $e^{\theta t}\approx 1+\theta t+\frac{(\theta t)^{2}}{2}$etc. Note that this approximation is valid only for $\theta t<1$.

Using the above approximation in (3), we get

$$\begin{aligned} I(t)= & {} \left( {\frac{\alpha }{\theta }} \right) ^{1/{1-\beta }}\left\{ {\theta (1-\beta )(T-t)+\frac{\theta ^{2}(1-\beta )^{2}(T-t)^{2}}{2}} \right\} ^{1/{1-\beta }}\\= & {} \left\{ {\alpha (1-\beta )} \right\} ^{1/{1-\beta }}(T-t)\left\{ {1+\frac{\theta (1-\beta )(T-t)}{2}} \right\} ^{1/{1-\beta }}\\= & {} \left\{ {\alpha (1-\beta )} \right\} ^{1/{1-\beta }}(T-t)\left\{ {1+\frac{\theta (T-t)}{2}} \right\} , \qquad (\hbox {by Binomial Theorem})\qquad (\mathbf{A}_{31})\\ SR= & {} \hbox { p}\int \limits _{\mathrm{0}}^{\mathrm{T}} D\{I(t)\}dt=p\int \limits _0^T \alpha \{T(t)\}^{\beta }dt\\= & {} p\alpha \int \limits _0^T {\{\alpha (1-\beta )\}^{\beta /{(1-\beta )}}(T-t)^{\beta /{(1-\beta )}}\left\{ {1+\frac{\theta (T-t)}{2}} \right\} } ^{\beta }dt \qquad (\mathbf{by A}_{31})\\ SR= & {} \hbox { p}\alpha ^{{\mathrm{1}}/{(1-\beta )}}(1-\beta )^{\beta /{1-\beta }}\int \limits _0^T {(T-t)^{\beta /{(1-\beta )}}\left\{ {1+\frac{\theta \beta (T-t)}{2}} \right\} } dt\\&\quad (\hbox {by Binomial Theorem})\\= & {} \frac{\hbox {p}\left\{ {\alpha (1-\beta )T} \right\} ^{{\mathrm{1}}/{(1-\beta )}}(4-2\beta +\theta \beta T)}{2(2-\beta )} \end{aligned}$$

Similarly we can calculate the remaining elements of the total profit.