Modeling of the effects of weakness planes in rock masses on the stability of tunnels using an equivalent continuum and damage model

Abstract

This paper proposes an equivalent continuum model to describe the mechanical behavior of transversely isotropic rocks. In this model, the transverse isotropy of deformation and strength was achieved based on the Mohr–Coulomb and maximum tensile-stress criteria, and the damage was captured by adopting a statistical damage evolution rule. The application of the model is verified through numerical simulation of conventional triaxial tests. The model is then used to reveal the non-uniform mechanical response of the surrounding rock and the secondary lining for a tunnel situated in a weak layered rock mass. The results show that: (1) The proposed model can capture the transverse isotropy in deformation and strength of rocks, and the proposed damage formulation can represent the deterioration and degree of failure of rocks; (2) The fracturing pattern, failure strength and stress–strain curves obtained from the proposed model agree well with test results for three typical rocks with different directional variations in strength; (3) The damage distribution based on the proposed model can identify the failure of layered rock mass; and (4) The damage zones of the surrounding rock and the loads on the secondary lining after tunnel excavation show distinctly asymmetric behavior, that is, the damaged zones are concentrated in the tunnel direction normal to the weak planes, and the positive bending moment and larger axial force are parallel and vertical to the weak planes, respectively.

1 Introduction

Layered structures due to bedding plane, foliation, stratification and fissuring bring directional anisotropy in the physical and mechanical behaviors of transversely isotropic rocks [29, 44]. In turn, anisotropy affects the failure patterns of rocks in almost all major engineering projects involving foundations, slopes or underground excavations [2, 17, 33, 57]. Thus, it is of vital importance to evaluate the mechanical behavior of transversely isotropic rocks.

Numerous compression tests have been conducted to study the strength anisotropy for transversely isotropic rocks, including sedimentary rocks, such as sandstone [14, 37, 58], limestone [12, 47], Marl [12], Coal [12], mudstone [43] and argillite [45]; metamorphic rocks, such as shale [3,4,5,6, 12, 21, 22, 27, 35, 47], phyllite [8, 30, 42, 53], slate [10, 20, 30, 47], schist [1, 18, 34] and gneiss [5]; igneous rocks, such as orthoquartzite [30]; and synthetic rocks [56].

Typically, strength anisotropy of transversely isotropic rocks shows three distinct features (Fig. 1): (1) the directional variations in strength can be classified into “U” type, “shoulder” type or “wavy” undulatory type, and the “U” type is the most common one among the three types [41] (Fig. 1a); (2) The maximum strength occurs at θ = 0° or 90° and mostly at θ = 90°, where θ is the angle between the main loading direction and the orientation of the weak planes. The minimum strength appears at θ = 30°–45°; and (3) The degree of anisotropy (the ratio between maximum and minimum strength) decreases as the confining pressure increases in most cases and the strength types may change due to the confining effect. As for fracturing, the pattern changes according to the direction of loading with respect to the orientation of the weakness planes (Fig. 1b): (1) failure propagates through the rock matrix, i.e., the non-sliding model; (2) across and along weak planes, i.e., the composite model; and (3) along the weak planes, i.e., the sliding model. The non-sliding model becomes more dominant with the increase in the confining pressure.

Fig. 1

Mechanical behavior of transversely isotropic rocks: a three different types of the directional variations of strength (Ramamurthy [41]); b failure patterns for transversely isotropic rocks

In numerical simulations, the representation of the layered structures in the rock mass can be made implicitly (i.e., continuum-based methods [39, 40]) or explicitly (i.e., discontinuum-based methods). The commonly used discontinuous approaches are block or particle discrete element method (DEM) and discontinuous deformation analysis (DDA) method. In these techniques, the geometry and the behavior of the weak planes can be described explicitly. Thus, they are suitable to be adopted to study the anisotropic mechanisms of rocks from a mesoscopic view, such as the initiation, propagation and coalescence of cracks. For example, based on the Particle Flow Code [24, 50], Gao et al. [19], Park and Min [36], Duan and Kwok [16] and Xia and Zeng [51] adopted: (1) a set of parallel continuous smooth joint contacts to represent the weak planes, and (2) the parallel bonded contacts to simulate the rock matrix to reveal the mechanical behaviors of transversely isotropic rocks with different micro-structures and micro-parameters. Based on the platform of Universal Distinct Element Code [25], the rock matrix is characterized by a plastic constitutive law and weak planes are described by explicit joints by Debecker [15] and Tan et al. [46] to study the fracturing features of transversely isotropic rocks.

The use of discontinuum-based methods is limited when dealing with complex and large-scale engineering applications due to greater computational requirements and longer computation times compared to continuum-based methods. Thus, some continuum-based constitutive models, mostly based on the equivalent continuum methods (i.e., the effect of weak planes is diffused into each rock matrix element and each element can describe both the failure of rock matrix and weak planes), have been put forward to address this issue. For example, Zhou et al. [59] proposed an enhanced equivalent continuum model for layered rock mass incorporating bedding structure and stress dependence. Xu et al. [55] introduced the yield approach index into a transversely isotropic elastic–plastic model to estimate the yield degree of layered rock mass. Ismael and Konietzky [23] put forward a constitutive model for inherently anisotropic rocks based on the Hoek and Brown failure criterion. These models have several features such as: (1) the elastic matrix and the strength are transversely isotropic; (2) the yielding behavior can be non-linear and (3) the maximum tensile-stress criterion is introduced in the rock matrix and the weak planes.

For transversely isotropic rocks, significant strain-softening behavior in the post-failure stage has been observed [34, 35, 58]. Thus, an ideal model for layered rock mass should also be able to describe the post-failure behavior. Numerous models have been proposed to describe the strength-weakening effect in the strain-softening stage for isotropic rocks. Examples are: (1) the use of linear strength reduction with the growth of strain in the softening stage and constant strength in the residual stage [13, 31, 48]; and (2) the use of damage evolution function to describe the deterioration process of the rock due to micro-fracture propagation [28, 38, 52]. However, to the authors’ knowledge, research regarding the damage-induced strain-softening behavior of transversely isotropic rocks remains quite limited [10, 11, 55]. In these papers, a scalar anisotropy parameter, which is related to stress invariants and structure orientation tensors, was adopted to reflect the anisotropic behavior of rocks, and scalar internal variable was introduced to account for the material softening. These models have a strong theoretical basis and can retain mathematical rigor. However, the formulations of such models are complex compared to other phenomenological models, which limits their applications in complex engineering problems to some extent. Thus, it is essential to put forward a constitutive model which can describe the strain-softening and damage behavior of transversely isotropic rocks containing weakness planes and deploy such model in practical engineering application.

In this article, a constitutive model is developed to provide a direct representation of the anisotropic and damage behavior of rocks. The aims of the present study are to: (1) Establish an elastic–plastic model to reflect the transverse isotropy in rock deformation and strength based on the Mohr–Coulomb and maximum tensile-stress failure criteria; (2) Introduce a statistical damage evolution rule into the elastic–plastic model to describe the damage and strain-softening behavior of rocks and (3) Demonstrate the use of the model in engineering applications by implementing the model in the computer code FLAC3D [26] to study the non-uniform mechanical response of the surrounding rock and the secondary lining in tunnels situated in a weak layered rock mass.

2 Mechanical properties of transversely isotropic rocks

A summary of test results of transversely isotropic rocks from the literature is given in Table 1, which indicates that the “U” type rocks account for about 71.2% of the total samples, and the proportion of the “wavy” type rocks is the least with magnitude of 5.1%. The following two indices are adopted to evaluate the degree of transverse strength anisotropy:

$${k}_{1}=\frac{{\sigma }_{max}}{{\sigma }_{min}}$$

(1)

$${k}_{2}=\frac{{\sigma }_{0}}{{\sigma }_{90}}$$

(2)

where σ_max, σ_min, σ₀ and σ₉₀ (in MPa) are the maximum strength, the minimum strength and the strength at θ = 0° and θ = 90°, respectively.

Table 1 Results of triaxial compression test on transversely isotropic rocks

Based on the test results of nine kinds of shale specimens, Cheng et al. [7] obtained four types of curves regarding the relation between k₁ and confining pressures. Five types of k₁ ~ confining pressure curves and three types of k₂ ~ confining pressure curves have been classified by Xu [54] based on the test results in the existing literature. Thus, the number of rocks is increased to 39 to validate the former classification (Table 1). The statistical analysis shows that the former classification has general applicability. Specifically, three trends about the changing trends of k₁ with the increase of the confining pressure can be found (Fig. 2): (1) Trend T_k1-1: k₁ undergoes a slight decrease of less than one with the increase of the confining pressure; (2) Trend T_k1-2: k₁ shows a decrease of larger than one with the increase of the confining pressure; and (3) Trend T_k1-3: k₁ remains nearly constant over the entire interval.

Fig. 2

The changing trends of k₁ with the increase of confining pressure: a trend T_k1-1; b trend T_k1-2; c trend T_k1-3

In addition, three trends can be categorized regarding the changing trends of k₂ with the increase of the confining pressure (Fig. 3): (1) Trend T_k2-1: k₂ undergoes a slight increase of less than one with the increase of the confining pressure; (2) Trend T_k2-2: k₂ shows a slight decrease less than one with the increase of the confining pressure, and (3) Trend T_k2-3: k₂ remains nearly constant over the entire interval.

Fig. 3

The changing trends of k₂ with the increase of confining pressure: a trend T_k2-1; b trend T_k2-2; c trend T_k2-3

3 An equivalent continuum model for the transversely isotropic rocks

This section presents the formulation of the equivalent continuum model for the transversely isotropic rocks that considers experimental observations discussed above.

3.1 Basic assumptions

The equivalent continuum model meets the following assumptions: (1) The transversely isotropic rock comprises rock matrix and weak planes and the influence of weak planes is diffused into the rock matrix. In addition, the rock matrix or the weak plane is described with its own plastic criterion; (2) The elastic deformation of rocks obeys transverse isotropy, that is, the elastic properties of rocks in all directions are the same along the plane parallel to the weak planes but different perpendicular to the weak planes; (3) The rock matrix and weak planes follow the same damage evolution law.

3.2 Elastic behavior

For a transversely isotropic rock, its mechanical response is isotropic at the x’y’ plane which is parallel to the weak planes. The coordinate systems are shown in Fig. 4. The elastic behavior of rock in the local coordinate system is:

$$[\sigma^{{\prime}} ] = [C^{{\prime}} ][\varepsilon^{{\prime}} ]$$

(3)

where $[\sigma^{{\prime}}$, $[\varepsilon^{{\prime}} ]$ and $[C^{{\prime}} ]$ are the stress, strain and stiffness matrix in the local coordinate system, respectively. The stiffness matrix $[C^{{\prime}} ]$ (MPa) is expressed as follows:

$$[C^{{\prime}} ] = \left[ {\begin{array}{*{20}c} {C_{11}^{{\prime}} } & {C_{12}^{{\prime}} } & {C_{13}^{{\prime}} } & 0 & 0 & 0 \\ {C_{21}^{{\prime}} } & {C_{22}^{{\prime}} } & {C_{23}^{{\prime}} } & 0 & 0 & 0 \\ {C_{31}^{{\prime}} } & {C_{32}^{{\prime}} } & {C_{33}^{{\prime}} } & 0 & 0 & 0 \\ 0 & 0 & 0 & {C_{44}^{{\prime}} } & 0 & 0 \\ 0 & 0 & 0 & 0 & {C_{55}^{{\prime}} } & 0 \\ 0 & 0 & 0 & 0 & 0 & {C_{66}^{{\prime}} } \\ \end{array} } \right]$$

(4)

where $C_{11}^{{\prime}} = C_{22}^{{\prime}} = \frac{{E_{1} (1 - nv_{2}^{2} )}}{{(1 + v_{1} )(1 - v_{1} - 2nv_{2}^{2} )}}$; $C_{12}^{{\prime}} = C_{21}^{{\prime}} = \frac{{E_{1} (v_{1} + nv_{2}^{2} )}}{{(1 + v_{1} )(1 - v_{1} - 2nv_{2}^{2} )}}$; $n = \frac{{E_{1} }}{{E_{3} }}$; $C_{13}^{{\prime}} = C_{31}^{{\prime}} = C_{23}^{{\prime}} = C_{32}^{{\prime}} = \frac{{E_{1} v_{2} }}{{1 - v_{1} - 2nv_{2}^{2} }}$; $C_{33}^{{\prime}} = \frac{{E_{3} (1 - v_{1} )}}{{1 - v_{1} - 2nv_{2}^{2} }}$; $C_{44}^{{\prime}} = C_{55}^{{\prime}} = \frac{{E_{1} }}{{1 + v_{1} }}$; $C_{66}^{{\prime}} = \frac{{E_{1} E_{3} }}{{E_{1} (1 + 2v_{1} ) + E_{3} }}$; E₁ and v₁ are the elastic modulus (MPa) and Poisson’s ratio in the plane of isotropy, respectively; E₃ and v₂ are the elastic modulus (MPa) and Poisson’s ratio in the direction normal to the plane of isotropy, respectively.

Fig. 4

Coordinate systems of the weak planes and the global reference axes

The elastic behavior of rock in the global coordinate system is

$$[\sigma ] = [C][\varepsilon ]$$

(5)

where $[\sigma ]$, $[\varepsilon ]$ and $[C]$ are the elastic stress (in MPa), strain and stiffness matrix in the global coordinate system, respectively. The formulation of the stiffness matrix [C] is shown in “Appendix I”.

3.3 Plastic behavior

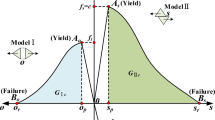
The undamaged Mohr–Coulomb criterion with a tension cut-off was adopted to describe the plastic behavior of the rock matrix (Fig. 5a):

$$f^{{\text{s}}} = \sigma_{1} - \sigma_{3} N_{\varphi } + 2c\sqrt {N_{\varphi } }$$

(6)

$$f^{{\text{t}}} = \sigma_{{\text{t}}} - \sigma_{3}$$

(7)

where f^s is the shear yield criterion, f^t is the tensile yield criterion, N_φ = (1 + sinφ)/(1 − sinφ), c, φ and σ_t are cohesion (MPa), friction angle (°) and tensile strength (MPa) of the rock matrix, respectively.

Fig. 5

Yield criterion: a rock matrix; b weak planes (after Itasca [26])

The corresponding potential functions are:

$$g^{{\text{s}}} = \sigma_{1} - \sigma_{3} N_{\psi }$$

(8)

$$g^{{\text{t}}} = - \sigma_{3}$$

(9)

where g^s is the shear potential function, g^t is the tensile potential function, N_ψ = (1 + sinψ)/(1 − sinψ) and ψ is the dilation angle (°).

The Mohr–Coulomb criterion with a tension cut-off was also adopted to describe the plastic behavior of the weak planes (Fig. 5b):

$$f_{{\text{j}}}^{{\text{s}}} = \tau - \sigma_{{\text{n}}} \tan \varphi_{{\text{j}}} - c_{{\text{j}}}$$

(10)

$$f_{{\text{j}}}^{{\text{t}}} = \sigma_{{\text{n}}} - \sigma_{{\text{j}}}^{{\text{t}}} ;\,\,\sigma_{{{\text{jmax}}}}^{{\text{t}}} = {\raise0.7ex\hbox{${c_{{\text{j}}} }$} \!\mathord{\left/ {\vphantom {{c_{{\text{j}}} } {\tan \varphi_{{\text{j}}} }}}\right.\kern-\nulldelimiterspace} \!\lower0.7ex\hbox{${\tan \varphi_{{\text{j}}} }$}}$$

(11)

where $f_{{\text{j}}}^{{\text{s}}}$ is the shear yield criterion, $f_{{\text{j}}}^{{\text{t}}}$ is the tensile yield criterion, c_j, φ_j and σ_j^t are cohesion (Pa), friction angle (°) and tensile strength (Pa) of weak planes, respectively, σ^t_jmax is the maximum tensile strength for a weak plane with nonzero friction angle (Pa) and σ_n is the normal stress (Pa) on the weak plane.

The corresponding potential functions are as follows:

$$g_{{\text{j}}}^{{\text{s}}} = \tau - \sigma_{{\text{n}}} \tan \psi_{{\text{j}}}$$

(12)

$$g_{{\text{j}}}^{{\text{t}}} = - \sigma_{{\text{n}}}$$

(13)

where g_j^s is the shear potential function, g_j^t is the tensile potential function and Ψ_j is the dilation angle of weak planes (°).

3.4 Damage behavior

The damaged plastic behavior can be obtained by introducing a damage formulation into the Mohr–Coulomb criterion in Sect. 3.3. The damage evolution law based on Weibull distribution [49] is:

$$D_{t} = 1{ - }\exp \left( {{ - }\left( {\frac{\varepsilon }{\alpha }} \right)^{m} } \right)$$

(14)

ε is the plastic strain related to the failure pattern of the rocks.

The damage index is defined as:

$$D = {1}{-}D_{t}$$

(15)

Thus, D corresponds to the probability of survival of rocks, with D = 1 corresponding to an undamaged material, and D = 0 to a fully damaged material. As rock can undergo either tensile or shear failure, the plastic strain ε, which controls the degree of damage, is defined as follows [26]:

$$\varepsilon { = }\left\{ {\begin{array}{*{20}c} {\frac{2}{3}\sqrt {\left[ {(\varepsilon_{1}^{p} - \varepsilon_{2}^{p} )^{2} + (\varepsilon_{2}^{p} - \varepsilon_{3}^{p} )^{2} + (\varepsilon_{3}^{p} - \varepsilon_{1}^{p} )^{2} } \right]} \;{\text{ for shear failure}}} \\ {\varepsilon_{3}^{{\text{p}}} \quad {\text{for tensile failure}}} \\ \end{array} } \right.$$

(16)

For simplicity, the same damage magnitude D is applied to the evolutions of the cohesion, friction and tensile strength of rocks. Thus, all the plastic parameters vary with the equivalent plastic strains:

$$c_{d} = \, Dc,\varphi_{{\text{d}}} = D\varphi ,\sigma_{{{\text{td}}}} = D\sigma_{{\text{t}}}$$

(17)

$$\it c_{jd} = \, Dc_{j} ,\varphi_{{{\text{jd}}}} = D\varphi_{{\text{j}}} ,\sigma^{{\text{j}}}_{{{\text{td}}}} = D\sigma^{{\text{j}}}_{{\text{t}}}$$

(18)

where c_d, φ_d and σ_td are the cohesion (Pa), friction angle (°) and tensile strength (MPa) of the rock matrix after damage, respectively, c_jd, φ_jd and σ^j_td are the cohesion (MPa), friction angle (°) and tensile strength (Pa) of the weak planes after damage, respectively.

The implementation of the equivalent continuum model for transversely isotropic rocks into the explicit finite different code FLAC3D is presented in the “Appendix II”. In the implementation, during a time increment, trial stresses are first calculated; then, a determination is made whether the rock mass will behave only elastically, or in addition to elastic deformation, plastic deformations will occur in the rock matrix, weakness planes or both. The trial stresses are initially obtained assuming elastic loading. If the calculated stress state does not agree with the mode of yielding, then an iteration is performed until there is agreement with the trial and final stresses for a given time increment.

4 Model calibration

As it can be seen, there exists many parameters for the proposed model. Thus, a precise and rapid method determining these parameters is important. The procedures in the calibration of the parameters (Fig. 6) are outlined as follows [16]:

• 1. Determine the deformation parameters in the plane of isotropy (E₁ and v₁) based on the elastic modulus of a specimen with θ = 0° obtained from the test;

• 2. Determine the deformation parameters in the direction normal to the plane of isotropy (E₃ and v₂) based on the elastic modulus of a specimen with θ = 90° obtained from the test. The other separate equivalent deformation parameter G₁₃ (MPa) is obtained from the equation [32]:

  $$G_{13} = E_{1} E_{3} /(E_{1} (1 + 2v_{2} ) + E_{3} )$$

  (19)

• 3. Determine the rock matrix strength parameters (c, φ, σ_t and ψ) and the damage parameters (α and m) of the rock matrix based on the test result regarding the stress–strain of the specimen with θ = 90°.

• 4. Calibrate the strength of the weak planes (c_j, φ_j, σ^j_t and ψ_j) to fit the strength versus θ curve.

Fig. 6

Model calibration process

Following the above calibration steps, the parameters of three typical rocks with “U” shape (Jiujiang slate, Chen et al. [10]), “shoulder” shape (Longmaxi shale, He et al. [22]) and “wavy” shape (Pittsburgh shale, Cheng et al. [6]) failure trends were calibrated. A cylindrical specimen with diameter of 50 mm and height of 100 mm was built, as shown in Fig. 7. The vertical deformation at the bottom of the specimen was fixed, and the constant vertical displacement at 1 × 10^–6 m/step was applied at the top of the specimen for triaxial compression tests. The calibrated parameters are listed in Table 2. Good agreement between numerical simulation and test results in strength and the variation of anisotropic indexes with the increasing confining pressure can be found in Fig. 8.

Fig. 7

FLAC3D discretization of a triaxial compression test specimen

Table 2 Model rock parameters used in FLAC3D

Fig. 8

Comparison of strengths from numerical and test results: a Jiujiang slate (Chen et al. [10]); b Longmaxi shale (He et al. [22]); c Pittsburgh shale (Cheng et al. [6])

The numerical simulation and test results regarding the fracture patterns (the localized damaged zones for numerical simulation) and stress–strain curves of Jiujiang slate with different confining pressures are shown in Figs. 9 and 10. Note that the predicted failure patterns were identified by the distribution of damage index, and the damage distribution with a magnitude being less than 0.25 is suitable for identifying rock mass failure. Broadly speaking, several fracturing surfaces along the weak planes occur when rock breaks for θ = 0°, and one fracturing surface along the weak plane occurs when rock breaks for θ = 15°–75°, and one oblique fracturing surface passing through the specimen occurs when rock breaks for θ = 90°. Figure 10 shows that the simulated stress–strain curves agreed well with the test results, even for the post-failure stages, which indicate that the proposed model can describe the anisotropy in the deformation and strength, and the strain-softening behaviors of transversely isotropic rocks.

Fig. 9

Comparison of fracture patterns between test results (Chen et al. [10]) and numerical simulation for Jiujiang slate: a σ₃ = 5 MPa; b σ₃ = 10 MPa; c σ₃ = 15 MPa. Notes: The highlighted regions of the simulated results indicate the fracturing planes with the damage index being less than 0.25

Fig. 10

The stress–strain curves of Jiujiang slate from experiments (Chen et al. [10]) and model simulations: a σ₃ = 5 MPa; b σ₃ = 10 MPa; c σ₃ = 15 MPa

In addition, the modelling results also show that the transverse isotropy of rocks is affected by the confining stress. The reason is that, although the initial yield and plastic potential functions are linear and the plastic parameters do not depend on the confining stress, the yield and plastic potential functions will become non-linear, and the plastic parameters will become strain-dependent after the occurrence of damage. Thus, the confining stress dependency in transverse isotropy can also be reflected in this model.

5 Application to tunneling

5.1 Background of the project

The Wenchuan–Maerkang Highway [9] is situated in the northwestern part of Sichuan province, China. Metamorphic soft rocks are widely distributed in this region due to the strong uplift and extrusion. The Zhegu mountain tunnel [53] is two-way, four-lane, separated tunnel with width of 13.4 m and height of 10.5 m. The length and maximum buried depth of Zhegu mountain tunnel is 8784 m and 1340 m, respectively. Figure 11a reveals that the tunnel passes through strata consisting of carbonaceous phyllite, carbonaceous slate and metamorphic sandstone. The foliation planes of the carbonaceous phyllite are extremely developed, and the dip angles are about 0°–60°. The supporting structures are shown in Fig. 11b. Due to the influence of the weak planes, non-uniform large deformations, such as the invasion of rock mass, cracking of shotcrete, and distortion of the steel arches, appear frequently when the tunnel passes through the phyllite stratum [9].

Fig. 11

Zhegu mountain tunnel: a longitudinal profile (Chen et al. [9]); b supporting structures; c layout of sensors

5.2 Field measurements

Pressure cells, steel strain gauges and concrete strain gauges were installed to measure the rock pressure born by the secondary lining, the strain of the steel arch and the strain of the secondary lining, respectively (Fig. 11c). The inner forces of the steel arch and secondary lining can be calculated as follows:

$$M = \frac{W}{2}E(\varepsilon_{i} - \varepsilon_{e} )$$

(20)

$$N = \frac{A}{2}E(\varepsilon_{{\text{i}}} + \varepsilon_{{\text{e}}} )$$

(21)

where M and N are the bending moment (N·m) and axial force (N), respectively, A, E and W are the area (m²), elastic modulus (MPa) and bending stiffness (m³), respectively, ε_i and ε_e are the strains of the inner and external surfaces respectively.

For the section of Zhegu mountain tunnel with β (β is the inclination angle of weak planes) of 30° or 60°, the rock pressure and the inner forces were both asymmetrically distributed (Fig. 12). Specifically, the maximum rock pressure occurs near the left spandrel. The bending moment at the left spandrel and right spring line regions are positive with maximum value near the left spandrel, while that at the remaining regions are negative with maximum value near the right spandrel. The distribution of the axial force had an elliptical shape, with the maximum values near the left spring line and right spandrel.

Fig. 12

Measurement results: a rock pressure (unit: MPa); b bending moment of steel arch (unit: kN m); c axial force of steel arch (unit: kN); d bending moment of secondary lining (unit: kN·m); e axial force of secondary lining (unit: kN)

5.3 Numerical model

The Zhegu mountain tunnel is modeled using FLAC3D with a finite difference mesh with dimensions of 80 m × 80 m × 40 m (in the x, z and y dimensions, respectively), and the results of section at y = 20 m is chosen to study the mechanical behavior of the surrounding rock and secondary lining after tunnel excavation, as shown in Fig. 13. The rock bolt, steel arch0 and shotcrete were represented by a cable element, a beam element, and solid element with the Mohr–Coulomb failure criterion, respectively. The parameters for the different elements are presented in Tables 3, 4 and 5. The secondary lining was represented by the elastic shell elements with E_s of 30 GPa and ν_s of 0.22. The phyllite was simulated using the proposed model, and its parameters are listed in Table 2.

Fig. 13

Numerical model: a surrounding rock; b supporting structures

Table 3 Rock bolt and grout parameters used in FLAC3D

Table 4 Steel arch parameters used in FLAC3D

Table 5 Shotcrete parameters used in FLAC3D

The following values were used in parametric studies of the response of the tunnel:

• 1. Lateral pressure coefficient: λ = σ_y/σ_x = 0.5 (σ_x = 20 MPa, σ_y = 10 MPa), λ = 1 (σ_x = σ_y = 20 MPa), λ = 2 (σ_x = 10 MPa, σ_y = 20 MPa).

• 2. Inclination angle β: 0°, 30°, 45°, 60° and 90°.

• 3. Ratio between the stiffness of weak planes and that of rock matrix: 0.3, 0.55 and 0.8.

• 4. Ratio between the strength of weak planes and that of rock matrix: 0.4, 0.6 and 0.8.

5.4 Simulation results

The main results of the numerical simulations in terms of the different parameters are presented and discussed below.

• (1) Effect of the lateral to vertical stress ratio

Taking β = 45° as an example, the damage zones of surrounding rock and the inner forces of secondary lining are shown in Fig. 14. The following conclusions can be drawn:

• 1. For λ = 0.5, the damage of the surrounding rock is evident in the region from the left spandrel to the right spandrel, and from the invert to the right haunch. For λ = 1, the damage zones occur at the region from the left haunch to the crown, and from the invert to the right haunch. For λ = 2, the damage zones happen in the region from the left haunch to the crown, and from the right spring line to the right spandrel. It can be concluded that the damage zones occur in two particular ranges in which the tangent of the tunnel contour was parallel to the direction of the weak planes, and the connecting line of the damage zones (the red line in Fig. 14a) is nearly normal to the weak planes for λ = 1, while it deflected to the direction of minor principal stress to a certain extent for the non-isotropic geo-stress field. The influence of geo-stress field on the damage zone of surrounding rock may be attributed to the reason that weak planes tend to slide downward along the planes, while the difference of principal stress in vertical and horizontal directions hinders or promotes this movement when λ = 0.5 and λ = 1, respectively.

• 2. For λ = 0.5, 1 and 2, the bending moment of the secondary lining at the regions of 75°–150° and 270°–330°, 85°–165° and 280°–350°, and 105°–180° and 305°–25° is positive, respectively, while that at the remaining regions are negative. The regions of the positive bending moment are related to the damage zones of the surrounding rock, and the maximum bending moment appears at the section where the connecting line of the damage zones intersects the tunnel contour.

• 3. The axial force is positive (i.e., compression) along the tunnel perimeter with an oval shape, and the maximum value appears at the sections supported by the surrounding rock with few damages, e.g., at 30° and 180°, 60° and 210°, and 85° and 235° for λ = 0.5, 1 and 2, respectively.

• (2) Effect of weak plane inclination

The damage zones of surrounding rock and the inner forces of secondary lining for different inclination angles (λ = 1) are shown in Fig. 15. The following conclusions can be drawn:

• 1. The damage region in the surrounding rock is greatly influenced by the inclination angle of the weak planes, and it shows similar distributed features for each angle, that is, the damaged zones are concentrated in certain regions which extend from the tunnel in the direction normal to the weak planes.

• 2. For θ = 0° or 90° (the weak planes are horizontally or vertically aligned), the inner forces are nearly symmetrically distributed due to the uniform geo-stress and symmetry of the surrounding rock. Specially, for θ = 0°, the maximum axial force appears at the haunch and it is much larger than that at the crown and the invert, while the bending moment is relatively large at the crown and the invert due to the damage of the surrounding rock in these regions. For θ = 90°, the maximum axial force occurs at the crown and the invert, and the minimum one appears at the haunch. The bending moment is positive in the region near the left and right haunch, and the invert, and it is negative in the remaining region.

• 3. For θ = 30° or 60°, the distributed features of inner forces are similar to those for θ = 45°.

• 4. As the inclination angle increases, the shapes of the inner forces for the secondary lining change gradually. Increasing the angle from 0 to 90º results in a maximum axial force moving from the haunch to the spandrel, and eventually to the crown. On the other hand, the maximum of the positive bending moment eventually moved from the crown to the haunch.

• (3) Effect of the stiffness and strength of weak planes

Fig. 14

Effect of the lateral pressure coefficient: a damage zones of surrounding rock; b bending moment of secondary lining; c axial force of secondary lining

Fig. 15

Effect of inclination angles of weak planes: a damage zones of surrounding rock; b bending moment of secondary lining; c axial force of secondary lining

The mechanical responses of surrounding rock and the inner forces of secondary lining after tunnel excavation for different stiffness or strength of weak planes (λ = 1, β = 45°) are shown in Figs. 16, 17. The following conclusions are obtained:

• 1. With the increase in the stiffness or strength of weak planes, the damage regions of surrounding rock get smaller and their damage degrees decrease. While the distributed features remain the same, e.g., the damaged zones are concentrated in certain regions which extend from the tunnel in the direction normal to the weak planes for each case.

• 2. The inner forces get smaller with the increase in the stiffness or strength of weak planes, while their distributed features are similar. The bending moment at the left spandrel and right spring line region are positive with maximum value near the left spandrel, while that at the remaining regions are negative with maximum value near the right spandrel. The distribution of the axial force shows an elliptical shape, with the maximum values near the left spring line and right spandrel.

Fig. 16

Effect of the stiffness of weak planes: a damage zones of surrounding rock; b bending moment of secondary lining; c axial force of secondary lining

Fig. 17

Effect of the strength of weak planes: a damage zones of surrounding rock; b bending moment of secondary lining; c axial force of secondary lining

The above phenomena indicate that the surrounding rock becomes weaker with the decrease in stiffness and strength of weak planes. Thus, shear sliding and tensile splitting are more likely to emerge on weak planes. The relative movement between fractured weak planes introduces more damaged zones in rock matrix, which result in a larger deformation of surrounding rock and larger inner force of supporting structures as consequence.

6 Conclusion

In this study, an equivalent continuum model for transversely isotropic rocks incorporating nonlinear damage effects was presented. The advantages of this proposed model are as follows: (1) it can describe the strain-softening behavior of rocks by introducing a statistical damage evolution rule, and (2) it can be adopted to evaluate the safety of practical engineering using a damage index, which is more effective than the plastic zone distribution in identifying the failure of layered rock mass. The validity of the model was verified through the numerical simulation of the conventional triaxial tests. The model was then used to study the non-uniform mechanical responses of the surrounding rock and the secondary lining for a tunnel situated in rock containing weak planes. The main conclusions from the study are summarized as follows:

• 1. The proposed mode can capture the transverse isotropy in deformation and strength of rocks, and the damage index can represent the failure and fracturing of rocks containing weak planes.

• 2. The triaxial compression simulation results from three typical rocks with different directional variations in strength indicate that the fracturing pattern, the failure strength and the stress–strain curves obtained from the proposed model agreed well with the test results. In addition, damage distribution based on the proposed model is effective to identify the failure of layered rock mass.

• 3. The damaged zones of the surrounding rock after tunnel excavation are concentrated in certain regions which extend from the tunnel in the direction normal to the weak planes for each inclination angle, and the connecting line of the damage zones is nearly normal to the weak planes for λ = 1, while it deflected to the direction of minor principal stress to a certain extent for non-isotropic geo-stress field.

• 4. The distribution of inner forces of the secondary lining shows distinctly asymmetric behaviors, that is, the positive bending moment or larger axial force mainly distributes in the regions where the tangent of tunnel contour is parallel or vertical to the weak planes, respectively.

• 5. The damage regions of surrounding rock get smaller and their damage degrees decrease, and the inner forces get smaller with the increase in the stiffness or strength of weak planes.

Appendices

Appendix I: Formulation of stiffness matrix in the global coordinate

The stiffness matrix in the global coordinate $[C]$ is expressed as

$$[C] = [Q][C^{{\prime}} ][Q]^{T}$$

(I.1)

where $[Q]$ is the transformation matrix defined as follows:

$$\begin{aligned}&[Q] \\=& \left[ {\begin{array}{*{20}c} {l_{1}^{2} } & {m_{1}^{2} } & {n_{1}^{2} } & {2m_{1} n_{1} } & {2n_{1} l_{1} } & {2l_{1} m_{1} } \\ {l_{2}^{2} } & {m_{2}^{2} } & {n_{2}^{2} } & {2m_{2} n_{2} } & {2n_{2} l_{2} } & {2l_{2} m_{2} } \\ {l_{3}^{2} } & {m_{3}^{2} } & {n_{3}^{2} } & {2m_{3} n_{3} } & {2n_{3} l_{3} } & {2l_{3} m_{3} } \\ {l_{2} l_{3} } & {m_{2} m_{3} } & {n_{2} n_{3} } & {m_{2} n_{3} + m_{3} n_{2} } & {n_{2} l_{3} + n_{3} l_{2} } & {l_{2} m_{3} + l_{3} m_{2} } \\ {l_{3} l_{1} } & {m_{3} m_{1} } & {n_{3} n_{1} } & {m_{3} n_{1} + m_{1} n_{3} } & {n_{3} l_{1} + n_{1} l_{3} } & {l_{3} m_{1} + l_{1} m_{3} } \\ {l_{1} l_{2} } & {m_{1} m_{2} } & {n_{1} n_{2} } & {m_{1} n_{2} + m_{2} n_{1} } & {n_{1} l_{2} + n_{2} l_{1} } & {l_{1} m_{2} + l_{2} m_{1} } \\ \end{array} } \right] \end{aligned}$$

(I.2)

where l_i, m_i and n_i (i = 1,2,3) are the directional cosines of axis x^’, y^’ and z^’, respectively. The matrix of directional cosines [R] is

$$[R] = \left[ {\begin{array}{*{20}c} {l_{1} } & {m_{1} } & {n_{1} } \\ {l_{2} } & {m_{2} } & {n_{2} } \\ {l_{3} } & {m_{3} } & {n_{3} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {\sin \alpha \cos \beta } & {\cos \alpha \cos \beta } & { - \sin \beta } \\ { - \cos \alpha } & {\sin \alpha } & 0 \\ {\sin \alpha \sin \beta } & {\cos \alpha \sin \beta } & {\cos \beta } \\ \end{array} } \right]$$

(I.3)

where α and β are the dip direction and dip angle of weak planes, respectively.

Thus, final form of the stiffness matrix [C] is:

$$[C] = \left[ {\begin{array}{*{20}c} {C_{11} } & {C_{12} } & {C_{13} } & {C_{14} } & {C_{15} } & {C_{16} } \\ {C_{21} } & {C_{22} } & {C_{23} } & {C_{24} } & {C_{25} } & {C_{26} } \\ {C_{31} } & {C_{32} } & {C_{33} } & {C_{34} } & {C_{35} } & {C_{36} } \\ {C_{41} } & {C_{42} } & {C_{43} } & {C_{44} } & {C_{45} } & {C_{46} } \\ {C_{51} } & {C_{52} } & {C_{53} } & {C_{54} } & {C_{55} } & {C_{56} } \\ {C_{61} } & {C_{62} } & {C_{63} } & {C_{64} } & {C_{65} } & {C_{66} } \\ \end{array} } \right]$$

(I.4)

where the elements of matrix [C] can be calculated based on Eqs. 4, I.2 and I.3.

Appendix II: implementation of the proposed constitutive model in FLAC 3D

The three-dimensional explicit finite difference implementation of the proposed model are outlined in this Appendix.

• 1. Deformations of the rock matrix

The function h(σ₁, σ₃) = 0, which is represented by the diagonal between the strength envelopes of f^s = 0 and f^t = 0 in the principal stress plane (Fig. 

Fig. 18

Determination of yielding type: a definition of h and domains used in determining yield type of the rock matrix; b definition of h_j and the domains used in determining yield type of the weak planes (after Itasca [26])

18a), is defined to determine the model of yielding of rock matrix:

$$h = \sigma_{3} - D\sigma_{t} + \alpha^{p} (\sigma_{1} - \sigma^{p} )$$

(II.1)

where

$$\alpha^{p} = \sqrt {1 + N_{\varphi d}^{2} } + N_{\varphi d}$$

(II.2)

$$\sigma^{p} = D\sigma_{{\text{t}}} N_{\varphi d} - 2Dc\sqrt {N_{\varphi d} }$$

(II.3)

$$N_{\varphi d} = \left( {{1} + {\sin}\left( {D\varphi } \right)} \right)/\left( {{1} - {\sin}\left( {D\varphi } \right)} \right)$$

(II.4)

If the stress state falls within domain 1, then shear failure occurs, and the new stress is revised using the flow rule derived from g^s. If the stress falls within domain 2, then tensile failure occurs, and the new stress is re-calculated adopting the flow rule derived from g^t.

For shear failure, partial differentiation of Eq. 8 with damage yields

$$\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \sigma_{x} }} = 1,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \sigma_{y} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \sigma_{z} }} = - N_{\psi } ,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \tau_{xy} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \tau_{yz} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \tau_{xz} }} = 0$$

(II.5)

Thus, the expressions of the new stress are

$$\sigma_{x}^{N} = \hat{\sigma }_{x}^{I} - \lambda^{*} (C_{11} - C_{13} N_{\psi } )\quad \sigma_{y}^{N} = \hat{\sigma }_{y}^{I} - \lambda^{*} (C_{21} - C_{23} N_{\psi } )$$

$$\sigma_{z}^{N} = \hat{\sigma }_{z}^{I} - \lambda^{*} (C_{31} - C_{33} N_{\psi } )\quad \sigma_{yz}^{N} = \hat{\sigma }_{yz}^{I} - \lambda^{*} (C_{41} - C_{43} N_{\psi } )$$

(II.6)

$$\sigma_{xz}^{N} = \hat{\sigma }_{xz}^{I} - \lambda^{*} (C_{51} - C_{53} N_{\psi } )\quad \sigma_{xy}^{N} = \hat{\sigma }_{xy}^{I} - \lambda^{*} (C_{61} - C_{63} N_{\psi } )$$

where

$$\lambda^{*} = \frac{{f^{{\text{s}}} = \sigma_{1} - \sigma_{3} N_{\varphi d} + 2Dc\sqrt {N_{\varphi d} } }}{{(C_{11} - C_{13} )N_{\psi } - (C_{31} - C_{33} N_{\psi } )N_{\varphi d} }}$$

(II.7)

For tensile failure, partial differentiation of Eq. 9 with damage gives

$$\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \sigma_{x} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \sigma_{y} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \sigma_{z} }} = - 1,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \tau_{xy} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \tau_{yz} }} = 0,\frac{{\partial {\text{g}}^{{\text{s}}} }}{{\partial \tau_{xz} }} = 0$$

(II.8)

Thus, the expressions of the new stress are

$$\sigma_{x}^{N} = \hat{\sigma }_{x}^{I} - \lambda^{*} C_{13} \quad \sigma_{y}^{N} = \hat{\sigma }_{y}^{I} - \lambda^{*} C_{23}$$

$$\sigma_{z}^{N} = \hat{\sigma }_{z}^{I} - \lambda^{*} C_{33} \quad \sigma_{yz}^{N} = \hat{\sigma }_{yz}^{I} - \lambda^{*} C_{43}$$

(II.9)

$$\sigma_{xz}^{N} = \hat{\sigma }_{xz}^{I} - \lambda^{*} C_{53} \quad \sigma_{xy}^{N} = \hat{\sigma }_{xy}^{I} - \lambda^{*} C_{63}$$

where

$$\lambda^{*} = \frac{{\sigma_{td} - \sigma_{t3} }}{{(C_{11} - C_{13} )N_{\psi } - (C_{31} - C_{33} N_{\psi } )N_{\varphi d} }}$$

(II.10)

• 2. Deformations of the weak planes

The function h_j(σ₁, σ₃) = 0, which is represented by the diagonal between the strength envelope of f_j^s = 0 and f_j^t = 0 in the principal stress plane (Fig. 18b), is defined:

$$h_{{\text{j}}} = \tau - \tau_{{\text{j}}}^{{\text{p}}} - \alpha_{{\text{j}}}^{{\text{p}}} (\sigma_{{\text{n}}} - \sigma_{{\text{j}}}^{{\text{t}}} )$$

(II.11)

where

$$\tau_{{\text{j}}}^{{\text{p}}} = Dc_{{\text{j}}} - \tan (D\varphi_{{\text{j}}} )D\sigma_{{\text{j}}}^{{\text{t}}}$$

(II.12)

$$\alpha_{{\text{j}}}^{{\text{p}}} = \sqrt {1 + \tan (D\varphi_{{\text{j}}}^{{}} )^{2} } - \tan (D\varphi_{{\text{j}}} )$$

(II.13)

If the stress falls within domain 1, then shear failure occurs, and the new stress is revised using the flow rule derived from g_j^s. If the stress falls within domain 2, then tensile failure occurs, and the new stress is re-calculated adopting the flow rule derived from g_j^t.

For shear failure, partial differentiation of Eq. 12 yields

$$\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{s}}} }}{{\partial \sigma_{{x^{{\prime}} }} }} = 0,\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{s}}} }}{{\partial \sigma_{{y^{{\prime}} }} }} = 0,\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{s}}} }}{{\partial \sigma_{{z^{{\prime}} }} }} = \tan \psi_{j} ,\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{s}}} }}{\partial \tau } = 1$$

(II.14)

Thus, the expressions of the new stress are

$$\left\{ \begin{gathered} \sigma_{{x^{{\prime}} }}^{N} = \sigma_{{x^{{\prime}} }}^{I} - \lambda^{s} C_{13} \tan \psi_{{\text{j}}} \hfill \\ \sigma_{{y^{{\prime}} }}^{N} = \sigma_{{y^{{\prime}} }}^{I} - \lambda^{s} C_{13} \tan \psi_{{\text{j}}} \hfill \\ \sigma_{{z^{{\prime}} }}^{N} = \sigma_{{z^{{\prime}} }}^{I} - \lambda^{s} C_{33} \tan \psi_{{\text{j}}} \hfill \\ \tau^{N} = \tau^{I} - 2\lambda^{s} C_{44} \hfill \\ \end{gathered} \right..$$

(II.15)

where

$$\lambda^{{\text{s}}} { = }\frac{{\tau - \sigma_{{\text{n}}} \tan (D\varphi_{{\text{j}}} ) - Dc_{{\text{j}}} }}{{2C_{44} + C_{33} \tan \psi_{j} \tan (D\varphi_{j} )}}$$

(II.16)

For tensile failure, partial differentiation of Eq. 13 gives

$$\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{t}}} }}{{\partial \sigma_{{x^{{\prime}} }} }} = 0,\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{t}}} }}{{\partial \sigma_{{y^{{\prime}} }} }} = 0,\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{t}}} }}{{\partial \sigma_{{z^{{\prime}} }} }} = 1,\frac{{\partial {\text{g}}_{{\text{j}}}^{{\text{t}}} }}{\partial \tau } = 0$$

(II.17)

Thus, the expressions of the new and updated stresses are

$$\left\{ \begin{gathered} \sigma_{{x^{{\prime}} }}^{N} = \sigma_{{x^{{\prime}} }}^{I} - \lambda^{t} C_{13} \hfill \\ \sigma_{{y^{{\prime}} }}^{N} = \sigma_{{y^{{\prime}} }}^{I} - \lambda^{t} C_{13} \hfill \\ \sigma_{{z^{{\prime}} }}^{N} = \sigma_{{z^{{\prime}} }}^{I} - \lambda^{t} C_{33} \hfill \\ \end{gathered} \right.$$

(II.18)

where

$$\lambda^{t} = \frac{{\sigma_{{\text{n}}} - D\sigma_{{\text{j}}}^{{\text{t}}} }}{{C_{33} }}$$

(II.19)

The flowchart of the implementation of the proposed model is shown in Fig. 

Fig. 19

Flowchart of the implementation of the proposed model

19. The main steps are as follows:

• 1. Assume that the rock behaves elastically, and the trial stresses in global coordinate are initially obtained.

• 2. Judge the yielding state of the rock matrix element based on the M-C criterion and modify its stress and strain if it yields. In addition, the plastic parameters of the element will be updated based on the damage evolutional law.

• 3. Convert the stress tensor from the global coordinate to the local coordinate and judge the yielding state of the weak plane element based on the M-C criterion and modify its stress and strain if it yields. In addition, the plastic parameters of the element will be updated based on the damage evolutional law.

• 4. Steps 1–3 are repeated until convergence of calculation is reached.