Heat Transport in an Anisotropic Porous Medium Saturated with Variable Viscosity Liquid Under G-jitter and Internal Heating Effects

Abstract

Thermo-rheological effect of temperature-dependent viscous fluid saturating a porous medium has been studied in the presence of imposed time periodic gravity field and internal heat source. Weak nonlinear stability analysis has been performed by using the power series expansion in terms of the amplitude of gravity modulation, which is considered to be small. Nusselt number is calculated numerically using Ginzburg–Landau equation. The nonlinear effects of thermo-mechanical anisotropies, internal heat source parameter, Vadász number, thermo-rheological parameter and amplitude of gravity modulation have been obtained and depicted graphically. Streamlines and isotherms have been drawn for different times. Comparisons have been made between various physical systems.

1 Introduction

Rayleigh–Bénard convection in porous media commonly known as Horton–Rogers–Lapwood convection has attracted many researchers and become a major part of research in fluid dynamics in recent times. The study finds its applications in wide range of science and engineering problems such as in geophysics, metallurgy, solidification of polymeric liquids, geothermal energy extraction, oil recovery process, nuclear waste disposal and earth’s mantle convection. Documented work in this area are well collected and reviewed by Nield and Bejan (2012), Kaviany (1995), Vafai (2000, 2005), Straughan (2004), Ingham and Pop (2005) and Vadász (2008).

Temperature-dependent viscosity fluid gives rise to variation in top and bottom structures and referred as a non-Boussinesq effect. Nonlinear energy stability theory has been derived by Richardson and Straughan (1993) for the problem of convection in porous medium when the viscosity depends on the temperature for vanishingly small initial data thresholds. Payne et al. (1999) derived unconditional nonlinear stability for temperature-sensitive fluid in porous media. Qin and Chadam (1996), Nield (1996), Holzbecher (1998), Rees et al. (2002), Siddheshwar and Chan (2004), Vanishree and Siddheshwar (2010) and Siddheshwar and Vanishree (2012) studied the effects of variable viscosity on convection problems in a porous medium.

There are large number of practical situations in which convection is driven by internal heat source. Due to internal heating of earth there is a temperature gradient between the interior and the exterior of the earth’s crust which causes convection in earth crust. Further the applications of internal heat source may be found in radioactive decay of unstable isotopes, metal waste form development for spent nuclear fuel and weak exothermic reaction which can take place within porous materials. Moreover, internal heat source is the main energy source of celestial bodies which is generated by radioactive decay and nuclear reaction. Research article related to internal heat source in porous media are given by Tveitereid (1977), Bejan (1978), Haajizadeh et al. (1984), Rionero and Straughan (1990), Rao and Wang (1991), Parthiban and Patil (1997), Herron (2001), Khalili and Huettel (2002), Joshi et al. (2006), Gaikwad et al. (2009), Bhadauria et al. (2011, 2013a, b), Bhadauria (2012) and Altawallbeh et al. (2013).

The gravity modulation of the system leads to the variable coefficients in the governing equations of thermal instability in porous media and involves the vertical time-periodic vibrations of the system. This leads to the appearance of a modified gravity, collinear with actual gravity, in the form of a time-periodic gravity field perturbation and is known as gravity modulation or g-jitter in literature. Research article related to gravity modulation are provided by Malashetty and Padmavathi (1997), Rees and Pop (2000, 2001, 2003), Malashetty and Basavaraj (2002), Govender (2004, 2005a, b), Kuznetsov (2005, 2006a, b), Siddhavaram and Homsy (2006), Strong (2008a, b), Razi et al. (2009), Saravanan and Purusothaman (2009), Siddheshwar and Vanishree (2012a), Saravanan and Arunkumar (2010), Saravanan and Sivakumar (2010, 2011); Siddheshwar and Bhadauria (2012b) and Bhadauria et al. (2012a, b, 2013a).

In most of the investigations, porous medium is assumed to be isotropic; however, for geological and pedological process rarely it forms isotropic media, as is usually assumed in transport studies. Processes such as sedimentation, compaction, frost action and reorientation of the solid matrix are responsible for the creation of anisotropic natural porous media. Anisotropic can also be a characteristic of artificial porous like pelleting used in chemical engineering process and fibre materials used in insulating purposes. Some of the articles related to anisotropic porous media are: Epherre (1975), Kvernvold and Tyvand (1979), Nisen and Storesletten (1990), Tyvand and Storesletten (1991), Degan et al. (1995), Nield and Kuznetsov (2003, 2007), Govender (2006, 2007), Malashetty and Heera (2006), Malashetty and Swamy (2007), Simmons et al. (2010), Bhadauria et al. (2013a, b) and Altawallbeh et al. (2013).

Thus anisotropy in porous media, which may be due to the preferential orientation or asymmetric geometry of porous matrix or fibres, is encountered in many systems in industry and nature. In context of the present problem, it is of particular interest in the study of extraction of metals from ores wherein a mushy layer is formed during solidification of a metallic alloy. The quality and structure of the resulting solid can be controlled by influencing the transport process. Since internal heating or gravity modulation or a combination of both is an effective mechanism of suppressing or advancing the thermoconvective flow, these mechanisms individually or collectively can be used as effective means of influencing the quality and structure of the resulting solid. It can be noticed from the literature for variable viscosity above, the works on thermal instability discussed earlier address only the onset of convection or deal with heat transport in the absence of internal heat generation or gravity modulation. To the best of authors’ knowledge, till date no nonlinear study is available that investigates the combined effect of internal heating of fluid/porous layer and gravity modulation under variable viscosity. It is with these motives that a weakly nonlinear analysis of thermal instability in a variable viscosity fluid-saturated anisotropic porous medium has been made under gravity modulation and the effects of internal heating and variable viscosity parameters have been investigated.

2 Governing Equations

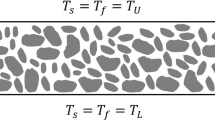
We consider an infinitely extended horizontal anisotropic porous layer saturated by variable viscosity Newtonian fluid with temperature-dependent viscosity confined between the planes $z = 0$ and $z = d$, which is heated from below. We choose Cartesian frame of reference as, origin at the lower boundary and the z-axis vertically upward direction. The gravity force is acting in vertically downward direction, we consider only free–free boundaries. It is assumed that the mechanical properties and thermal properties in x and y-directions are same. A uniform adverse temperature gradient $\Delta T/d$ is maintained between the surfaces. Further the density variation is considered under Boussinesq approximation. The governing Eqs. under above considerations are given by

$$\begin{aligned} \nabla . \mathbf{q }&= 0,\end{aligned}$$

(1)

$$\begin{aligned} \frac{\rho _0}{\phi }\frac{\partial \mathbf q }{\partial t}&= -\nabla p+\rho \mathbf g (t)-\mu K. \mathbf q ,\end{aligned}$$

(2)

$$\begin{aligned} \gamma \frac{\partial T}{\partial t}+(\mathbf q .\nabla )T&= \nabla .\left( \kappa _T.\nabla T\right) +Q\left( T-T_0\right) ,\end{aligned}$$

(3)

$$\begin{aligned} \rho&= \rho _0\left[ 1-\beta _{\mathrm{T}}\left( T-T_0\right) \right] ,\end{aligned}$$

(4)

$$\begin{aligned} \mathbf g (t)&= g_0\left[ 1+\epsilon ^2a_1\text{ Cos }(\varOmega _0 t)\right] \hat{k} ,\end{aligned}$$

(5)

$$\begin{aligned} \mu (T)&= \frac{\mu _0}{1+\epsilon ^2\delta _0\left( T-T_0\right) }, \end{aligned}$$

(6)

where q is velocity, $\phi$ is porosity of the matrix, $p$ is the pressure, $\mathbf g$ is the acceleration due to gravity, $\mu$ is viscosity, $\rho$ is density and $T$ represent temperature. $K=K_{x}^{-1}{ii}+K_{x}^{-1}{jj} +K_{z}^{-1}{kk}$ is the inverse of the permeability tensor, $\kappa _T=\kappa _{Tx}{ii}+\kappa _{Tx}{jj} +\kappa _{Tz}{kk}$ is the thermal diffusivity tensor, $\rho _0$ is reference density, $g_0$ is mean gravity, $a_1$ is amplitude of gravity modulation, $\varOmega _0$ is the frequency and $\epsilon$ is the quantity that indicates smallness in order of magnitude of modulation and $t$ is time. Furthermore $\beta _{T }$ is thermal volume expansion coefficient and $\gamma =\frac{(\rho c)_{\mathrm{m}}}{(\rho c)_{\mathrm{f}}}$ is the heat capacity ratio. Introducing the stream function $\psi$ and eliminating the pressure term and then nondimensionalizing the resultant equations using the substitution

$$\begin{aligned} (x,y,z)=\left( x^*,y^*,z^*\right) d, t=t^*\left( \gamma d^2/\kappa _{Tz}\right) , \psi =(\kappa _{\text{ Tz }})\psi ^*\quad \text{ and } \quad T=(\Delta \text{ T })T^*, \end{aligned}$$

(7)

the nondimensionlized Eqs. 2 and 3 are:

$$\begin{aligned}&\frac{1}{{Va}}\frac{\partial \left( \nabla ^2 \psi \right) }{\partial t} =-Ra_{\mathrm{T}} \left( 1+\epsilon ^2a_1\text{ Cos }(\varOmega _0 t)\right) \frac{\partial T}{\partial x} -{\bar{\mu }}(T) \left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) \psi \nonumber \\&\qquad \qquad \qquad \qquad \quad -\left( \frac{\partial {\bar{\mu }}}{\partial x}\frac{\partial \psi }{\partial x}+\frac{1}{\xi }\frac{\partial {\bar{\mu }}}{\partial z}\frac{\partial \psi }{\partial z}\right) , \end{aligned}$$

(8)

$$\begin{aligned} \frac{\partial T}{\partial t}-\left( \eta \frac{\partial ^2}{\partial x^2}+\frac{\partial ^2}{\partial z^2}+R_{\mathrm{i}}\right) T=\frac{\partial (\psi ,T)}{\partial (x,z)}, \end{aligned}$$

(9)

where ${\bar{\mu }}(T)=\frac{1}{1+\epsilon ^2V\left( T-T_0\right) }$ and the appearance of $\epsilon ^2$ indicates that the viscosity variation is weak as $\epsilon$ is small quantity. ${Va}=\frac{\nu d^2}{K_z\kappa _{Tz}}$ Vadász number or Darcy–Prandtl number, $Ra_{\mathrm{T}}=\frac{\beta _{\mathrm{T}} g_{0} {\Delta T} d K_z}{\nu \kappa _{Tz}}$ is thermal Rayleigh number, $\xi =\frac{K_x}{K_z}$ is mechanical anisotropy parameter, $\eta =\frac{\kappa _{Tx}}{\kappa _{Tz}}$ is thermal anisotropy parameter, $R_{\mathrm{i}}=\frac{Q d^2}{\kappa _{Tz}}$ is internal heat source parameter and $V=\delta _0 {\Delta T}$ thermo-rheological parameter. For simplicity $\gamma$ and $\phi$ are taken unity in the present problem. The boundary condition for solving Eqs. 8 and 9 are

$$\begin{aligned} \psi&= 0\quad \text{ and } \quad T=1 \text{ at } z=0\end{aligned}$$

(10)

$$\begin{aligned} \psi&= 0\quad \text{ and } \quad T=0 \text{ at } z=1 \end{aligned}$$

(11)

The conduction profile is given by

$$\begin{aligned} \psi _{\mathrm{b}}=0\quad \text{ and } \quad T_{\mathrm{b}}(z)=\frac{\text{ Sin }\sqrt{R_{\mathrm{i}}}(1-z)}{\text{ Sin }\sqrt{R_{\mathrm{i}}}} \end{aligned}$$

(12)

Using ${\bar{\mu }}={\bar{\mu }}\left( T_{\mathrm{b}}\right)$ Eq. 8 reduces to

$$\begin{aligned} \frac{1}{{Va}}\frac{\partial \left( \nabla ^2\psi \right) }{\partial t}=-Ra_{\mathrm{T}} \left( 1+\epsilon ^2a_1\text{ Cos }(\varOmega _0 t)\right) \frac{\partial T}{\partial x} -{\bar{\mu }}(T) \left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) \psi -\frac{1}{\xi }\frac{\partial {\bar{\mu }}}{\partial z}\frac{\partial \psi }{\partial z},\nonumber \\ \end{aligned}$$

(13)

where ${\bar{\mu }}\left( T_{\mathrm{b}}\right) =\frac{\mu ^{\prime }}{\mu _0}=\frac{\text{ Sin }\sqrt{R_{\mathrm{i}}}}{\text{ Sin }\sqrt{R_{\mathrm{i}}}+\epsilon ^2V \text{ Sin }\sqrt{R_{\mathrm{i}}}(1-z)} \text{ and } \varOmega _0^*=\frac{\varOmega _0d^2}{\kappa _{{Tz}}}$.

We impose finite amplitude perturbations on the basic quiescent state given by Eq. 12 as

$$\begin{aligned} \psi =\varPsi \quad \text{ and } \quad T=\frac{\text{ Sin }\sqrt{R_{\mathrm{i}}}(1-z)}{\text{ Sin }\sqrt{R_{\mathrm{i}}}}+\varTheta . \end{aligned}$$

(14)

Substituting the above expressions 14 in Eqs. 13 and 9 we have

$$\begin{aligned}&\frac{1}{{Va}}\frac{\partial \left( \nabla ^2\varPsi \right) }{\partial t}=-Ra_{\mathrm{T}} \left( 1+\epsilon ^2a_1\text{ Cos }\left( \varOmega _0^* t\right) \right) \frac{\partial \varTheta }{\partial x} -\frac{\text{ Sin }\sqrt{R_{\mathrm{i}}}}{\text{ Sin }\sqrt{R_{\mathrm{i}}}+\epsilon ^2V \text{ Sin }\sqrt{R_{\mathrm{i}}}(1-z)}\nonumber \\&\quad \qquad \qquad \qquad \quad \quad .\left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) \varPsi -\frac{\epsilon ^2 V\sqrt{R_{\mathrm{i}}} \text{ Cos }\sqrt{R_{\mathrm{i}}} (1-z) \text{ Sin }\sqrt{R_{\mathrm{i}}}}{\xi \left( \text{ Sin }\sqrt{R_{\mathrm{i}}}+ \epsilon ^2 V \text{ Sin }\sqrt{R_{\mathrm{i}}} (1-z)\right) ^2}\frac{\partial \varPsi }{\partial z}, \end{aligned}$$

(15)

$$\begin{aligned} -\frac{\text{ dT }_{\mathrm{b}}}{\text{ dz }}\frac{\partial \varPsi }{\partial x}+\frac{\partial \varTheta }{\partial t}-\left( \eta \frac{\partial ^2}{\partial x^2}+\frac{\partial ^2}{\partial z^2}+R_{\mathrm{i}}\right) \varTheta&= \frac{\partial (\varPsi ,\varTheta )}{\partial (x,z)} \end{aligned}$$

(16)

Boundary conditions to solve Eqs. 15 and 16 are

$$\begin{aligned} \varPsi&= 0 \quad \text{ and } \quad \varTheta =0 \text{ at } z=0,\end{aligned}$$

(17)

$$\begin{aligned} \varPsi&= 0 \quad \text{ and } \quad \varTheta =0 \text{ at } z=1. \end{aligned}$$

(18)

We now introduce the following asymptotic expansion

$$\begin{aligned} Ra_{\mathrm{T}}&= R_{\mathrm{0c}}+\epsilon ^2 R_2+\epsilon ^4R_4+\text{... },\end{aligned}$$

(19)

$$\begin{aligned} \varPsi&= \epsilon \varPsi _1+\epsilon ^2\varPsi _2+\epsilon ^3\varPsi _3+\text{... },\end{aligned}$$

(20)

$$\begin{aligned} \varTheta&= \epsilon \varTheta _1+\epsilon ^2\varTheta _2+\epsilon ^3\varTheta _3+\text{... }, \end{aligned}$$

(21)

where $R_{\mathrm{0c}}$ is the critical value of the Rayleigh number at which the onset of convection takes place in the absence of gravity modulation.

We now assume the variation of time only at the slow time scale $\tau = \epsilon ^2 t$ and arranging the systems at different order of $\epsilon$.

At the lowest order, we have

$$\begin{aligned} \left( \begin{array}{cc} \left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) &{} R_{\mathrm{0c}}\frac{\partial }{\partial x} \\ -\frac{\text{ dT }_{\mathrm{b}}}{\text{ dz }}\frac{\partial }{\partial x} &{} -\left( \eta \frac{\partial ^2}{\partial x^2}+\frac{\partial ^2}{\partial z^2}+R_{\mathrm{i}}\right) \end{array} \right) \left( \begin{array}{c} \varPsi _1 \\ \varTheta _1 \end{array} \right) =0, \end{aligned}$$

(22)

Solution at the lowest order is given by

$$\begin{aligned} \varPsi _1&= A[\tau ] \text{ Sin }\left( k_{\mathrm{c}} x\right) \text{ Sin }(\pi z),\end{aligned}$$

(23)

$$\begin{aligned} \varTheta _1&= \frac{4\pi ^2k_{\mathrm{c}}}{\left( \delta _{2}^2-R_{\mathrm{i}}\right) \left( R_{\mathrm{i}}-4\pi ^2\right) }A[\tau ] \text{ Cos }\left( k_{\mathrm{c}} x\right) \text{ Sin }(\pi z), \end{aligned}$$

(24)

where $\delta ^2=k_{\mathrm{c}}^2+\pi ^2,\delta _{1}^2=k_{\mathrm{c}}^2+\frac{\pi ^2}{\xi } \text{ and } \delta _{2}^2=\eta k_{\mathrm{c}}^2+\pi ^2.$

The critical value of the Rayleigh number and the corresponding wave number for the onset of stationary convection is calculated numerically and the expression for Rayleigh number is given by:

$$\begin{aligned} R_{\mathrm{0c}}&= \frac{\delta _1^2 \left( \delta _{2}^2-R_{\mathrm{i}}\right) \left( 4 \pi ^2-R_{\mathrm{i}}\right) }{4\pi ^2 k_{\mathrm{c}}^2 },\end{aligned}$$

(25)

$$\begin{aligned} k_{\mathrm{c}}&= \left( \frac{\pi ^2\left( \pi ^2-R_{\mathrm{i}}\right) }{\xi \eta }\right) ^{\frac{1}{4}}. \end{aligned}$$

(26)

3 Amplitude Equation and Heat Transport for Stationary Instability

At the second order, we have

$$\begin{aligned} \left( \begin{array}{cc} \left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) &{} R_{\mathrm{0c}}\frac{\partial }{\partial x} \\ -\frac{\text{ dT }_{\mathrm{b}}}{\text{ dz }}\frac{\partial }{\partial x} &{} -\left( \eta \frac{\partial ^2}{\partial x^2}+\frac{\partial ^2}{\partial z^2}+R_{\mathrm{i}}\right) \end{array} \right) \left( \begin{array}{c} \varPsi _2 \\ \varTheta _2 \end{array} \right) =\left( \begin{array}{c} R_{21} \\ R_{22} \end{array} \right) , \end{aligned}$$

(27)

where

$$\begin{aligned} R_{21}&= 0,\end{aligned}$$

(28)

$$\begin{aligned} R_{22}&= \frac{2\pi ^3k_{c}^2}{\left( \delta _{2}^2-R_{\mathrm{i}}\right) \left( R_{\mathrm{i}}-4\pi ^2\right) }A[\tau ]^2 \text{ Sin }(2\pi z). \end{aligned}$$

(29)

The second order solution subject to the boundary conditions (17 and 18) is given by

$$\begin{aligned} \varPsi _2&= 0,\end{aligned}$$

(30)

$$\begin{aligned} \varTheta _2&= -\frac{2\pi ^3k_{c}^2}{\left( \delta _{2}^2-R_{\mathrm{i}}\right) \left( 4\pi ^2-R_{\mathrm{i}}\right) ^2}A[\tau ]^2 \text{ Sin }(2\pi z). \end{aligned}$$

(31)

The horizontally averaged Nusselt number, $Nu$, for stationary mode of convection (the mode considered in this problem) is given by :

$$\begin{aligned} Nu(\tau )=1+\frac{\left[ \frac{k_{\mathrm{c}}}{2\pi }\int _0^{\frac{2\pi }{k_{\mathrm{c}}}} \left( \frac{\partial \varTheta _2}{\partial z}\right) \, dx\right] _{z=0}}{\left[ \frac{k_{\mathrm{c}}}{2\pi }\int _0^{\frac{2\pi }{k_{\mathrm{c}}}} \left( \frac{\partial T_{\mathrm{b}}}{\partial z}\right) \, dx\right] _{z=0}} \end{aligned}$$

(32)

One can notice here that the gravity modulation is effective at $O(\epsilon ^2)$ and affects $Nu(\tau )$ through $A[\tau ]$ as shown next. Substituting expression of $\varTheta _2$ in the above expression (Eq. 32) and simplifying, we get

$$\begin{aligned} Nu(\tau )=1+\frac{\left( 4\pi ^4k_{\mathrm{c}}^2\text{ Sin }\sqrt{R_{\mathrm{i}}}\right) }{\left( \sqrt{R_{\mathrm{i}}} \text{ Cos }\sqrt{R_{\mathrm{i}}}\left( \delta _{2}^2-{R_{\mathrm{i}}}\right) \left( {R_{\mathrm{i}}}-4\pi ^2\right) ^2\right) }(A[\tau ])^2. \end{aligned}$$

(33)

At the third order, we have

$$\begin{aligned} \left( \begin{array}{cc} \left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) &{} R_{\mathrm{0c}}\frac{\partial }{\partial x} \\ -\frac{\text{ dT }_{\mathrm{b}}}{\text{ d }z}\frac{\partial }{\partial x} &{} -\left( \eta \frac{\partial ^2}{\partial x^2}+\frac{\partial ^2}{\partial z^2}+R_{\mathrm{i}}\right) \end{array} \right) \left( \begin{array}{c} \varPsi _3 \\ \varTheta _3 \end{array} \right) =\left( \begin{array}{c} R_{31} \\ R_{32} \end{array} \right) , \end{aligned}$$

(34)

where

$$\begin{aligned} R_{31}&= -\text{ Sin }^2\sqrt{R_{\mathrm{i}}} \frac{1}{{{Va}}}\frac{\partial }{\partial \tau }\left( \nabla ^2 \varPsi _1\right) -V \text{ Sin }\sqrt{R_{\mathrm{i}}} \text{ Sin }\sqrt{R_{\mathrm{i}}} (1-z)\left( \frac{\partial ^2}{\partial x^2}+\frac{1}{\xi }\frac{\partial ^2}{\partial z^2}\right) \varPsi _1\nonumber \\&-\sqrt{R_{\mathrm{i}}} \text{ Sin }\sqrt{R_{\mathrm{i}}} \text{ Cos }\sqrt{R_{\mathrm{i}}} (1-z)\frac{V}{\xi }\frac{\partial \varPsi _1}{\partial z}\nonumber \\&-\left( R_{\mathrm{0c}}\left( 2 V \text{ Sin }\sqrt{R_{\mathrm{i}}} \text{ Sin } \sqrt{R_{\mathrm{i}}} (1\!-\!z)\!+\!a_1\text{ Cos }(\varOmega \tau )\text{ Sin }^2\sqrt{R_{\mathrm{i}}}\right) \!+\!R_2 \text{ Sin }^2\sqrt{R_{\mathrm{i}}}\right) \frac{\partial \varTheta _1}{\partial x},\nonumber \\ \end{aligned}$$

(35)

$$\begin{aligned} R_{32}=\frac{\partial \varPsi _1}{\partial x}\frac{\partial \varTheta _2}{\partial z}-\frac{\partial \varTheta _1}{\partial \tau } \end{aligned}$$

(36)

and $\varOmega =\frac{\varOmega _0^*}{\epsilon ^2}=\frac{\varOmega _0d^2}{\epsilon ^2 \kappa _{Tz}}$.

Substitute the value of $\varPsi _1,\varTheta _1 \text{ and } \varTheta _2$ in the above equations to get the expressions of $R_{31}, R_{32}$.

Applying the solvability condition for the existence of third order solution, we get the non-autonomous Ginzburg–Landau equation with time periodic coefficients in the form

$$\begin{aligned} A_1 A^{\prime }[\tau ]+A_2 A[\tau ]+A_3(A[\tau ])^3=0, \end{aligned}$$

(37)

where

$$\begin{aligned} A_1&= \left( \frac{\delta ^2\text{ Sin }^2\sqrt{R_{\mathrm{i}}}}{{Va} \delta _1^2}+\frac{1}{\left( \delta _2^2-R_{\mathrm{i}}\right) }\right) ,\\ A_2&= -\left( \frac{R_2}{R_{\mathrm{0c}}}+a_1\text{ Cos }(\varOmega \tau )\right) \text{ Sin }^2\sqrt{R_{\mathrm{i}}}-V\left( \frac{4\pi ^2\left( -1+\text{ Cos }\sqrt{R_{\mathrm{i}}}\right) }{\sqrt{R_{\mathrm{i}}}\left( R_{\mathrm{i}}-4 \pi ^2\right) }\right) \text{ Sin }\sqrt{R_{\mathrm{i}}}\\&-\frac{2\pi ^2 V \left( -1+\text{ Cos }\sqrt{R_{\mathrm{i}}}\right) }{\xi \left( 4 \pi ^2-R_{\mathrm{i}}\right) \delta _1^2}\sqrt{R_{\mathrm{i}}} \text{ Sin }\sqrt{R_{\mathrm{i}}} \end{aligned}$$

and $A_3=\dfrac{\pi ^2 k_{\mathrm{c}}^2 }{2\left( \delta _2^2-R_{\mathrm{i}}\right) \left( 4 \pi ^2-R_{\mathrm{i}}\right) }.$

The Ginzburg–Landau equation given by (37) is a Bernoulli equation and obtaining the analytical solution is difficult due to its non-autonomous nature. Therefore, it has been solved numerically by the in-built function NDSolve of Mathematica 7.0, subject to the initial condition $A[0]=a_0$, where $a_0$ is the chosen initial amplitude of convection. In our calculations, we may assume $R_2= R_0$ to keep the parameters to the minimum.

In case of unmodulated porous medium, the above Ginzburg–Landau equation takes the form:

$$\begin{aligned} A_1 A_u^{\prime }[\tau ]-K_1 A_u[\tau ]+A_3(A_u[\tau ])^3=0, \end{aligned}$$

(38)

where $A_u[\tau ]$ is amplitude of convection for unmodulated case and

$$\begin{aligned} K_1&= \left( \frac{R_2}{R_{\mathrm{0c}}}\right) \text{ Sin }^2\sqrt{R_{\mathrm{i}}}+V\left( \frac{4\pi ^2\left( -1+\text{ Cos }\sqrt{R_{\mathrm{i}}}\right) }{\sqrt{R_{\mathrm{i}}}\left( R_{\mathrm{i}}-4 \pi ^2\right) }\right) \text{ Sin }\sqrt{R_{\mathrm{i}}}\\&+\frac{2\pi ^2 V \left( -1+\text{ Cos }\sqrt{R_{\mathrm{i}}}\right) }{\xi \left( 4 \pi ^2-R_{\mathrm{i}}\right) \delta _1^2}\sqrt{R_{\mathrm{i}}} \text{ Sin }\sqrt{R_{\mathrm{i}}}. \end{aligned}$$

Solution of Eq. 38 is given by

$$\begin{aligned} A_u[\tau ]=\frac{1}{\sqrt{\left( \frac{A_3}{2K_1}+C_1\text{ Exp }\left[ -\frac{2 K_1 }{A_1}\tau \right] \right) }}, \end{aligned}$$

(39)

where $C_1$ is a parameter that can be found by using suitable initial condition. The Nusselt number in this case can be calculated from Eq. 33 using $A_u[\tau ]$ in place of $A[\tau ]$.

4 Results and Discussions

We perform weak nonlinear analysis in the presence of internal heat source and gravity modulation for temperature-dependent viscosity fluid-saturated anisotropic porous media, considering Darcy model. The work of Nield (1996) has been used for the thermo-rheological relationship of temperature-dependent viscosity of the fluid. We investigated the effects of internal heat source, gravity modulation and thermo-rheological parameter on heat transport. The effect of gravity modulation is considered to be of order $O(\epsilon ^2)$ so as to provide us only small amplitude vibrations. Such an assumption will help us in obtaining the amplitude equation of convection in a rather simple and elegant manner and is much easier to obtain than in the case of the Lorenz model.

Before writing the discussion of the results, we enlighten some features of the following aspects of the problem:

• 1. The need for nonlinear stability analysis,

• 2. The relation of the problem to a real application and

• 3. The selection of all dimensionless parameters used in computations.

If one wants to quantify heat transfer, which linear stability analysis is unable to do, this problem needs to perform the nonlinear analysis and hence the importance.

External regulation of convection is important in the study of convection in porous media. The objective of this article is to consider internal heat source, gravity modulation and temperature-dependent viscosity variation for either enhancing or inhibiting the convective heat transport as is required by a real application.

The parameters that appeared in this article and affect heat transfer are $\xi , \eta , V, {Va}, R_{\mathrm{i}}, \varOmega , a_1$. The first four parameters are related to the fluid and the structure of the porous medium, and the last three concern external mechanisms of controlling convection.

Vadász (1998) pointed out that there are some modern porous medium applications, such as mushy layer in solidification of binary alloys and fractured porous medium, where the value of ${Va}$ may be considered to be of unity order; therefore, the time-derivative term in the present study has been retained. Further this is the reason that we have kept the values of ${Va}$ around one in our calculations, and retained the local acceleration term $\frac{1}{{Va}}\frac{\partial q}{\partial t}$.

The values of $R_{i}$ are considered to be moderate so that it will not affect the effect of gravity modulation on the system by dominating it otherwise. The values of $a_{1}$ are considered to be between $0$ and $0.5$, since we are studying the effect of small amplitude modulation on heat transport. Further, as the effect of low frequencies on the onset of convection as well as on the heat transport is maximum, the modulation of gravity is assumed to be of low frequency. Further the value of thermo-rheological parameter, $V$ is also considered to be small. (Fig. 1)

Fig. 1

Physical configuration of the problem

The values of $Nu(\tau )$ are obtained numerically from the expressions of $Nu(\tau )$ (Eq. 33) by using the numerical value of amplitude of convection obtained from the Ginzburg–Landau equation. We used the values to plot the curve for $Nu(\tau )$ versus slow time variation $\tau$ and presented in the Figs. 2, 3, 4, 5, 6, 7 and 8. A close observation of Eq. 33 in conjunction with Eq. 37 reveals that $Nu(\tau )$ depends on thermal and mechanical anisotropy, internal heat source parameter $R_{\mathrm{i}}$, Vadász number ${Va}$, thermo-rheological parameter $V$ and the amplitude of g-jitter $a_1$.

From the Figs. 2, 3, 4, 5, 6, 7 and 8, it is observed that when $\tau$ is very small, the value of $Nu(\tau )$ is 1 thus showing that initially heat transport is due to conduction only. However, as time passes, heat transport across the porous medium increases, which shows that convective regime is in place. The convective regime remains oscillatory for further elapse of time. As particular cases of the present study, we have drawn graphs for isotropic cases also. Following results have been found from Figs. 2, 3, 4, 5, 6, 7 and 8 for heat transport:

• 1. $[Nu]_{V=0}<[Nu]_{V \ne 0}$

• 2. $[Nu]_{R_{\mathrm{i}}=1}<[Nu]_{R_{\mathrm{i}}=1.2}<[Nu]_{R_{\mathrm{i}}=1.4}$

• 3. $[Nu]_{\xi =1.5}<[Nu]_{\xi =1.0}<[Nu]_{\xi =0.5}$

• 4. $[Nu]_{\eta =0.5}<[Nu]_{\eta =1.0}<[Nu]_{\eta =1.5}$

• 5. $[Nu]_{{Va}=0.5}<[Nu]_{{Va}=1.0}<[Nu]_{{Va}=1.5}$

• 6. $[Nu]_{a_1=0.3}<[Nu]_{a_1=0.4}<[Nu]_{a_1=0.5}$

From Fig. 2 we find that the effect of variable viscosity parameter $V$ on thermal instability is to destabilize the system as heat transport increases on increasing the value of $V$. From Fig. 3, we find that the effect of internal heating on thermal instability is destabilizing, as heat transport increases on increasing $R_{i}$. The heat transport is more at higher values of $R_{i}$. This confirms the results obtained most recently by Bhadauria et al. (2011, 2013a, b), Bhadauria (2012) and Altawallbeh et al. (2013). In Fig. 4, we observe that an increment in $\xi$ decreases heat transport, thus suppresses the convection. When $\xi$ increases, then either $K_{x}$ increases or $K_{z}$ decreases, and so in both the cases fluid flow through porous medium decreases in vertical direction in comparison to the flow in horizontal direction. This delays the convection, and thus decreases the heat transport across the porous media. However, from Fig. 5, the effect of thermal anisotropy $\eta$ is found to be opposite to that of mechanical anisotropy $\xi$, compatible with the results of Epherre (1975), Kuznetsov and Nield (2008) and Bhadauria (2012), obtained for unmodulated case.

Fig. 2

Variation of Nusselt number with time for different values of V

Fig. 3

Variation of Nusselt number with time for different values of $R_{\mathrm{i}}$

Fig. 4

Variation of Nusselt number with time for different values of $\xi$

Fig. 5

Variation of Nusselt number with time for different values of $\eta$

Fig. 6

Variation of Nusselt number with time for different values of Va

Fig. 7

Variation of Nusselt number with time for different values of $a_1$

Fig. 8

Variation of Nusselt number with time for different values of $\varOmega$

From Fig. 6, the effect of Vadász number ${Va}$ on the system is destabilizing as heat transport increases on increasing its value. This result is compatible with the result of Vadász (1998) obtained for rotating porous medium. The effect of amplitude of modulation $a_{1}$ on $Nu$ is depicted in Fig. 7. From the figure, we find that the effect of increasing the amplitude of gravity modulation is to increase the heat transport, thus advancing the convection. In Fig. 8, we observe that an increment in $\varOmega$ decreases the magnitude of $Nu$, and shortens the wavelength of oscillations. As the frequency of modulation increases from 2 to 10, the magnitude of $Nu$ decreases, and so is the modulation effect. When the value of $\varOmega$ is increased further, we find that at $\varOmega =50$, the effect of gravity modulation on thermal instability disappears altogether. This result agrees with that of linear studies of Wen-Mei (1997), Malashetty and Padmavathi (1997) and Malashetty and Basavaraja (2005), where at higher frequencies, the shift in critical Rayleigh number due to gravity modulation becomes almost zero.

In Fig. 9, we have shown comparison between the analytical solution of unmodulated case and the numerical solution of the problem at hand. We observe that the value of Nusselt number for unmodulated case is qualitatively similar to that of Bhadauria (2012), however, more than in the modulated case.

Fig. 9

With g-jitter and without g-jitter

Variation of stream lines and isotherms at different instants of time is shown graphically in Figs. 10 and 11, respectively. From the Figs. 10a–f, it is clear that the magnitudes of stream lines increase as time increase. Figure 11a–f shows the variation of isotherms at different instants of time. It is found from the graphs that initially isotherms are flat and parallel, thus heat transport is due to conduction only. However, as time increases, isotherms form contours, showing convective regime is in place. Further, it is also clear from the Figs. 10 and 11 that after reaching certain instant there is no change in the magnitude of stream lines and isotherms, thus showing the steady state.

Fig. 10

Variation of stream lines with time a $\tau =0.1$ b $\tau =1.0$ c $\tau =2.0$ d $\tau =3.0$ e $\tau =4.0$ f $\tau =6.0$ $\eta =0.5, \xi =0.5, V=0.1, {Va}=1, a_1=0.3, \varOmega =2, R_{\mathrm{i}}=1, \epsilon =0.5$

Fig. 11

Variation of isotherms with time a $\tau =0.1$ b $\tau =1.0$ c $\tau =2.0$ d $\tau =3.0$ e $\tau =4.0$ f $\tau =6.0 \eta =0.5, \xi =0.5, V=0.1, {Va}=1, a_1=0.3, \varOmega =2, R_{\mathrm{i}}=1, \epsilon =0.5$

5 Conclusions

We perform a weak nonlinear stability analysis to study the combined effect of internal heat source, gravity modulation and temperature-dependent viscosity on the heat transfer in an infinite horizontal anisotropic porous medium saturated with temperature sensitive fluid using the Ginzburg–Landau equation. The porous medium is closely packed, and heated from below. The following conclusions have been made from our analysis, for increasing values of parameter:

• 1. On increasing the value of thermo-rheological parameter $V$, the heat transfer increases.

• 2. An increment in internal heat source parameter $R_{\mathrm{i}}$ increases the heat transport across the porous medium, thus destabilizes the system.

• 3. Mechanical anisotropic parameter $\xi$ has stabilizing effect on the system as heat transport decreases on increasing the value of $\xi$.

• 4. Thermal anisotropic parameter $\eta$ has opposite effect on heat transport in comparison of $\xi$.

• 5. An increment in Vadász number ${Va}$ increases the heat transport, thus having destabilizing effect on the system.

• 6. On increasing the amplitude of modulation $a_1$, heat transport in porous medium increases.

• 7. On increasing the value of frequency of gravity modulation $\varOmega$, the amplitude of modulation of heat transfer decreases. Effect of gravity modulation becomes negligible at higher values of $\varOmega$.

• 8. Magnitude of streamlines increases with passes of time.

• 9. Initially isotherms are flat due to conduction state, become contour showing convective regime.