Linear and Nonlinear Double Diffusive Convection in a Couple Stress Fluid Saturated Anisotropic Porous Layer with Soret Effect and Internal Heat Source

Abstract

In this paper, we analyze the effect of internal heating and the Soret effect on linear and nonlinear double diffusive convection in a couple stress fluid saturated anisotropic porous layer, heated and salted from below. Linear stability analysis has been performed by using normal mode technique and nonlinear analysis is carried out using a truncated Fourier series. The modified Darcy model, which includes the time-derivative term, has been employed in the momentum equation. The effects of the Vadasz number, the anisotropic parameter, the Soret parameter, the couple stress parameter, the solute Rayleigh number, the internal heat source parameter, the Lewis number, the Darcy–Prandtl number, and normalized porosity on the stationary and oscillatory are shown graphically. Also, heat and mass transports are calculated in terms of the Nusselt number and the Sherwood number and shown graphically.

1 Introduction

Studies of double diffusive convection in porous media play a significant role in many areas, such as the petroleum industry, solidification of binary mixtures, and migration of solutes in water-saturated soils. Other examples include geophysics systems, crystal growth, electrochemistry, the migration of moisture through air contained in fibrous insulation, the Earth’s oceans, and magma chambers. The problem of double diffusive convection in a porous media has been presented by Ingham and Pop [1], Nield and Bejan [2], Vafai [3, 4], and Vadasz [5]. The study was continued by Poulikakos [6], Trevison and Bejan [7], and Momou [8] among others. The first study of double diffusive convection in porous media was mainly concerned with linear stability analysis and was performed by Nield [9].

The growing importance of non-Newtonian fluids with suspended particles in modern technology and industries makes the investigation of such fluids desirable. These fluids are applied in the extrusion of polymer fluids in industry, exotic suspensions, fluid film lubrication, solidification of liquid crystals, cooling of metallic plates in baths, and colloidal and suspension solutions. Non-Newtonian stress fluids have specific features, such as the polar effect. The theory of polar fluids and related theories are models for fluids whose microstructure is mechanically significant. The theory for couple stress fluid was proposed by Stokes [10]; it is a simpler polar fluid theory, that shows all the important features and effects of such fluids that occur inside a deforming continuum. The stabilizing effect of the couple stress parameter is reported in the works of Sharma and Thakur [11], who investigated thermal instability in an electrically conducting couple stress fluid with a magnetic field. Sunil et al. [12] studied the effect of suspended particles on double diffusive convection in a couple stress fluid-saturated porous medium, Sharma and Sharma [13] investigated the effect of suspended particles on couple stress fluid, heated from below, in the presence of rotation and a magnetic field. Malashetty et al. [14] performed an analytical study of linear and nonlinear double diffusive convection with the Soret effect in couple stress liquids. Gaikwad and Kamble [15] analyzed the linear stability of double diffusive convection in a horizontal, sparsely packed, rotating, anisotropic porous layer in the presence of the Soret effect. Malashetty and Kollur [16] investigated the onset of double diffusive convection in an anisotropic porous layer saturated with couple stress fluid. Shivakumara et al. [17] analyzed the linear and nonlinear stability of double diffusive convection in a couple stress fluid-saturated porous layer.

In the study of double diffusive convection in the Soret effect, in some of the important areas of application in engineering, including geophysics, oil reservoirs, and groundwater, researchers have developed a great interest in these type of flows. In the presence of cross diffusion two transport properties are produced: the Soret effect and the Dufour effect. The Soret effect describes the tendency of a solute to diffuse under the influence of a temperature gradient. There are only a few studies available on double diffusive convection in the presence of the Soret effect. The diffusion material is heated unevenly. A mixture of gases or a solution is caused by the presence of temperature gradient in the system. The effect was described by Swiss scientist J. Soret, who was the first to study thermodiffusion (1879). Hurle and Jakeman argue that the liquid mixture, the Dufour term, is indeed small, and thus the Dufour effect will be negligible when compared with the Soret effect. They conducted an experimental and theoretical study of Soret-driven thermosolutal convection in a binary fluid mixture [18]. Malashetty et al. [19] performed an analytical study of linear and nonlinear double diffusive convection with the Soret effect in couple stress liquids. Rudraiah and Malashetty [20] discussed double diffusive convection in a porous medium in the presence of the Soret and Dufour effects. Bahloul et al. [21] studied double diffusive convection and Soret-induced convection in a shallow horizontal porous layer analytically and numerically. Malashetty and Biradar [22] carried out an analytical study of linear and nonlinear double diffusive convection in a fluid-saturated porous layer with Soret and Dufour effects. Also in another study, Bhadauria and Hashim et al. [23] performed linear and nonlinear double diffusive convection in a saturated anisotropic porous layer with couple stress fluid. Hill [25] showed linear and nonlinear double diffusive convection in a saturated anisotropic porous layer with a Soret effect and an internal heat source. Bhadauria et al. [26] studied effect of internal heating on double diffusive convection in a couple stress fluid saturated anisotropic porous medium. A study concerning an internal heat source in porous media was provided by Tveitereid [24], who performed thermal convection in a horizontal porous layer with internal heat sources. Srivastava et al. [27] performed linear and nonlinear analyses of double diffusive convection in a porous layer with a concentration-based internal heat source. Bhadauria [28], Horton and Rogers [29], and Lapwood [30] studied the effect of internal heating on double diffusive convection in a couple stress fluid-saturated anisotropic porous medium. Govender [31] showed that the Coriolis effect on the stability of centrifugally driven convection in a rotating anisotropic porous layer is subject to gravity. Kapil [32] performed at the onset of convection in a dusty couple stress fluid with variable gravity through a porous medium in hydromagnetics.

The aim of this chapter was to study the Soret effect and an internal heat source with a couple stress fluid. However, in the present study, stability analysis of the Soret and internal heating effect on double diffusive convection in an anisotropic porous layer with a couple stress fluid was performed.

1.1 Nomenclature

Table 1

2 Mathematical Formulation

We consider an infinitely extended horizontal plane at z=0 and z=d a fluid-saturated porous medium, which is heated from below and cooled from above. The Darcy model has been employed in the momentum equation. Further, an internal heat source term has been included in the energy equation. A cartesian frame of reference is chosen in such a way that the origin lies on the lower plane and the z-axis is vertical upward. An adverse temperature gradient is applied across the porous layer and the lower and upper planes are kept at temperatures T ₀ +  ΔT and T ₀, with a concentration S ₀ +  ΔS and S ₀ respectively. The physical configuration of the model is reported in the Figure 1. The governing equations are given below

$$\displaystyle \begin{aligned} \left\{ \begin{array}{l} \nabla.\vec{q}=0,\\ \\ \frac{\rho_0}{\varepsilon}(\frac{\partial q}{\partial t})=-\nabla p+\rho g-\frac{1}{K}(\mu-\mu_c\nabla^2)\vec{q},\\ \\ \gamma\frac{\partial T}{\partial t}+(\vec{q}.\nabla)T=\nabla(\kappa_{Tz}. \nabla T)+Q(T-T_0),\\ \\ \varepsilon\frac{\partial S}{\partial t}+(\vec{q}.\nabla)S=\kappa_s\nabla^2S+D\nabla^2T,\\ \\ \rho=\rho_0[1-\beta_T(T-T_0)+\beta_S(S-S_0)]\\ \\ \end{array} \right. \end{aligned} $$

(1)

where the physical variables have their usual meanings as given in the nomenclature. The externally imposed thermal and solutal boundary conditions are given by

$$\displaystyle \begin{aligned} \left\{ \begin{array}{l} T=T_0+\Delta T , at z=0 and T=T_0 , at z=d,\\ S=S_0+\Delta S , at z=0 and S=S_0 , at z=d,\\ \end{array} \right. \end{aligned} $$

(2)

Fig. 1

Physical configuration of the problem

3 Basic State

In this state, the velocity, pressure, temperature, and density profiles are given by

$$\displaystyle \begin{aligned} \vec{q_b}=0,p=p_b(z),T=T_b(z),S=S_b(z),\rho=\rho_b(z). \end{aligned} $$

(3)

Substituting Equation (3) in Equation (1), we obtain the following relations:

$$\displaystyle \begin{aligned} \frac{ dp_b}{dz}=-\rho_bg, \end{aligned} $$

(4)

$$\displaystyle \begin{aligned} \kappa_T\frac{d^2(T_b-T_0)}{dz^2}+Q(T_b-T_0)=0, \end{aligned} $$

(5)

$$\displaystyle \begin{aligned} K_s\frac{d^2S_b}{dz^2}+D \frac{d^2T_b}{dz^2}=0, \end{aligned} $$

(6)

$$\displaystyle \begin{aligned} \rho_b=\rho_0[1-\beta_T(T_b-T_0)+\beta_S(S_b-T_0)]. \end{aligned} $$

(7)

The solution of equation (5), subject to the boundary conditions (2), is given by

$$\displaystyle \begin{aligned} T_b=T_0+\Delta T\frac{\sin{}( \big(\sqrt{\frac{Qd^2}{\kappa_T}\big)}(1-\frac{z}{d}))}{\sin\big(\sqrt{\frac{Qd^2}{\kappa_T}}\big)}. \end{aligned} $$

(8)

The solution of equation (6), subject to the boundary conditions (2),

$$\displaystyle \begin{aligned} S_b=S_0+(\Delta S+\frac{D \Delta T}{K_s})(1-\frac{z}{d})-\frac{D \Delta T}{K_s} \frac{\sin{}( \big(\sqrt{\frac{Qd^2}{\kappa_T}\big)}(1-\frac{z}{d}))}{\sin\big(\sqrt{\frac{Qd^2}{\kappa_T}}\big)} \end{aligned} $$

(9)

Now, we superimpose finite amplitude perturbations on the basic state in the form:

$$\displaystyle \begin{aligned} \vec{q}=q_b+q',T=T_b+T',p=p_b+p',S=S_b+S',\rho=\rho_b+\rho', \end{aligned} $$

(10)

Infinitesimal perturbation was applied to the basic state of the system and then the pressure term was eliminated by taking the curl twice of Equation (1). The resulting equations were nondimensional using the following transformations:

$$\displaystyle \begin{aligned} (x,y,z)=(x^*,y^*,z^*)d, t=t^*(\frac{\gamma d^2}{\kappa_{Tz}}), \end{aligned} $$

(11)

$$\displaystyle \begin{aligned} (u,v,w)=(u^*,v^*,w^*)(\frac{\kappa _{Tz}}{d}),T=(\Delta T)T^*,S=(\Delta S)S^* \end{aligned}$$

T _b, S _b in dimensionless forms are given

$$\displaystyle \begin{aligned} T_b=\frac{\sin\sqrt{R_i}(1-z)}{\sin\sqrt{R_i}} , \end{aligned} $$

(12)

$$\displaystyle \begin{aligned} S_b=\frac{S_r L_e Ra_T\sin{}(\sqrt{R_i}(1-z))}{Ra_S \sin\sqrt{R_i}}-(\frac{S_r L_e Ra_T}{Ra_S}+1)(1-z) \end{aligned}$$

to obtain nondimensional equation (on dropping the asterisks for simplicity), and use the stream function $u=\frac {\partial \psi }{\partial z}$, $w=-\frac {\partial \psi }{\partial x}$

$$\displaystyle \begin{aligned} \frac{1}{V_a}\frac{\partial}{\partial t}\nabla^2_{1} \psi+(\frac{\partial^2}{\partial x^2}+\frac{1}{\xi}\frac{\partial^2}{\partial z^2})(1-C \nabla^2_{1})\psi= Ra_T\frac{\partial T}{\partial x}-Ra_{S} \frac{\partial S}{\partial x}=0 \end{aligned} $$

(13)

$$\displaystyle \begin{aligned} \Big[\frac{\partial}{\partial t}-\frac{\partial^2}{\partial z^2}-\eta\frac{\partial^2}{\partial x^2}-R_i\Big]T-f(z)\frac{\partial \psi}{\partial x}-\frac{\partial (\psi,T)}{\partial(x, z)}=0 \end{aligned} $$

(14)

$$\displaystyle \begin{aligned} \Big[\frac{\partial}{\partial t}-\frac{1}{L_e}(\frac{\partial^2}{\partial z^2}+\frac{\partial^2}{\partial x^2})\Big]S-S_r\frac{Ra_T}{Ra_S}\nabla^2 T-b(z)\frac{\partial \psi}{\partial x}-\frac{\partial (\psi,S)}{\partial(x, z)}=0 \end{aligned} $$

(15)

where $V_a=\frac {\varepsilon P_r}{D_a}$ is Vadasz number, $Ra_{T}=\frac {\beta _Tg\Delta TK_z d}{\nu \kappa _{Tz}}$ is the thermal Rayleigh number, $Ra_{S}=\frac {\beta _Sg\Delta SK_z d}{\nu \kappa _{Tz}}$ is the solute Rayleigh number, $R_i=\frac {Qd^2}{\kappa _{Tz}}$ is the internal heat source parameter, $C=\frac {\mu _C}{\mu d^2}$ is the couple stress fluid, $L_e=\frac {\kappa _{Tz}}{\kappa _S}$ is the Lewis number, and $\chi =\frac {\varepsilon }{\gamma }$ is normalized porosity. The above system will be solved by considering stress-free and isothermal boundary conditions as given below:

$$\displaystyle \begin{aligned} w=\frac{\partial^2w}{\partial z^2}=T=S=0 on z=0, z=1. \end{aligned} $$

(16)

4 Linear Stability Analysis

To study linear stability analysis according to solving the eigenvalue problem defined by Equations (13)–(15) subject to the boundary condition by Equations (5), (6), using time-dependent periodic disturbance in the horizontal plane:

$$\displaystyle \begin{aligned} (w,T,S)=(W,\Theta,\phi)exp[i(l x+m y)+\sigma t] \end{aligned} $$

(17)

where l, m are horizontal wave number and σ = σ _r + iσ _j the growth rate. Substituting Equation (17) into the linearized equations (13)–(15), we obtain

$$\displaystyle \begin{aligned} \Big[\frac{\sigma}{V_a}\delta^2+\delta^2_1(1-C \delta^2)\Big]W+a Ra_T\Theta-a Ra_S\phi=0 \end{aligned} $$

(18)

$$\displaystyle \begin{aligned}{}[\sigma+\eta_1-R_i]\Theta - 2a F W=0 \end{aligned} $$

(19)

$$\displaystyle \begin{aligned}{}[\sigma+\frac{\delta^2}{L_e}]\phi -2a B W+S_r\delta^2\frac{Ra_T}{Ra_S}\Theta =0. \end{aligned} $$

(20)

Where D = d∕dz  and  a ² = l ² + m ². The boundary conditions are (17). Now read

W= D ² W =  Θ = ϕ = 0  at  z = 0, 1:

We assume that the solutions of equations (13)–(15) satisfying the boundary conditions (17),

(W(z), Θ(z), ϕ(z)) = (W ₀, Θ₀, ϕ ₀)sinnπz    (n = 1, 2, 3, …)

in the form of the thermal Rayleigh number can be obtained as

$$\displaystyle \begin{aligned} Ra_T=\frac{Ri-(\sigma+\eta_1)}{2a^2 F}\Big[\frac{(\delta^2+L_e\sigma)(\frac{\sigma}{V_a}\delta^2+\delta^2_1(1-C\delta^2))-2 a^2B L_e Ra_S}{\sigma+\delta^2+\delta^2 S_r L_e}\Big] \end{aligned} $$

(21)

where a ² = l ² + m ², δ ² = π ² + a ², $\delta ^2_1=\frac {\pi ^2}{\xi }+a^2$, η ₁ = π ² + ηa ², F=$\int _{0}^{1}\frac {dT_b}{dz} sin^2(\pi z) dz$, B= $\int _{0}^{1}\frac {dS_b}{dz} sin^2(\pi z) dz$, η is a representative viscosity of the fluid. The growth rateσ is in general a complex quantity such that σ = σ + iσ _i. The system with σ _r <0 is always stable, whereas for σ _r >0 it will become unstable. For the neutral stability state σ _r = 0.

4.1 Stationary State

The values of the thermal Rayleigh number and the corresponding wave number of the system for a stationary mode of convection are given below:

$$\displaystyle \begin{aligned} Ra_T^{st}=\frac{Ri-\eta_1}{2a^2 F}\Big[\frac{\delta^2 \delta^2_1(1-C\delta^2)-2a^2B Ra_S L_e}{\delta^2(1+L_e S_r)}\Big], \end{aligned} $$

(22)

It is important to note the critical wave number $a= a_c^{St}$, which is the result given by Malashetty et al. [19]. For single component fluid, Ra _S = 0, i.e., in the absence of a solute Rayleigh number, Equation (22) gives

$$\displaystyle \begin{aligned} Ra_T^{st}=\frac{(Ri-\eta_1)\delta^2_1(1-C\delta^2)}{2a^2 F(1+L_e S_r)}. \end{aligned} $$

(23)

For the system without internal heating, i.e., R _i = 0,F=-1/2, we get

$$\displaystyle \begin{aligned} Ra_T^{st}=\frac{(\eta_1)\delta^2_1(1-C\delta^2)}{a^2 (1+L_e S_r)} \end{aligned} $$

(24)

which is the one obtained by Shivakumara et al. [17]. When C = 0 (i.e., Newtonian fluid case), Eq. (3.11) reduces to

$$\displaystyle \begin{aligned} Ra_T^{st}=\frac{(\pi^2+\eta^2 a^2)(a^2+\frac{\pi^2}{\xi})}{a^2(1+L_e S_r)} \end{aligned} $$

(25)

In the case of no Soret effect

$$\displaystyle \begin{aligned} Ra_T^{st}=\frac{(\pi^2+\eta^2 a^2)(a^2+\frac{\pi^2}{\xi})}{a^2} \end{aligned} $$

(26)

Lastly, in the case of isotropic porous medium, put η = ξ = 1

$$\displaystyle \begin{aligned} Ra_T^{st}=\Big(\frac{\pi^2+a^2}{a}\Big)^2 \end{aligned} $$

(27)

which has the critical value $Ra_c^{St} = 4\pi ^2$ for $a_c^St = \pi ^2$ and which are the classical results obtained by Horton and Rogers [29] and Lapwood [30].

4.2 Oscillatory State

For the corresponding wave number of the system for the oscillatory mode of convection, we now set σ = iσ _i in Equation (21) and clear the complex quantities from the denominator, to obtain

$$\displaystyle \begin{aligned}Ra_T^{osc} = \Delta_1 + i\sigma_i\Delta_2.\end{aligned}$$

$$\displaystyle \begin{aligned} \Delta_1=\frac{1}{2a^2 F}\frac{A_1 B_1+\sigma^2 A_2 B_2}{B_1^2+\sigma^2B_2^2}\end{aligned} $$

(28)

$$\displaystyle \begin{aligned} \Delta_2=\frac{1}{2a^2 F}\frac{A_2 B_1- A_1 B_2}{B_1^2+\sigma^2B_2^2}, \end{aligned} $$

(29)

where, $A_1= (R_i-\eta _1)(\delta ^2 \delta ^2_1(1-C\delta ^2)-\frac {\sigma ^2}{V_a}L_e \delta ^2)+\sigma ^2(L_e \delta ^2_1(1-C\delta ^2)+\frac {\delta ^4}{V_a})-(R_i-\eta _1)Ra_S 2a^2B L_e$,

$A_2= (R_i-\eta _1)(L_e \delta ^2_1(1-C\delta ^2)+\frac {\delta ^4}{V_a})-\delta ^2 \delta ^2_1(1-C\delta ^2)+\frac {\sigma ^2}{V_a}L_e\delta ^2+Ra_S 2a^2B L_e$

B ₁ = δ ²(1 + S _r L _e)

B ₂ = 1

For oscillatory onset Δ₂ = 0 and (σ _i ≠ 0), where σ is the oscillatory frequency, which is not given for brevity.

We have the necessary expression for the oscillatory Rayleigh number as:

$$\displaystyle \begin{aligned} Ra_T^{osc}=\Delta_1. \end{aligned} $$

(30)

5 Nonlinear Stability Analysis

In this section, we study the nonlinear stability analysis using a minimal truncated Fourier series. For simplicity, we consider only two-dimensional rolls, so that all the physical quantities are independent of y. Consider the stream function ψ such that $u=\frac {\partial \psi }{\partial z}$, $w=\frac {-\partial \psi }{\partial x}$, then taking curl to eliminate the pressure term from Equation (1) and then the resulting nondimensional equations by using transformation given by Equation (11) and the following equation

$$\displaystyle \begin{aligned} \Big(\frac{1}{V_{a}}\frac{\partial}{\partial t}\nabla1^2\psi+(\frac{\partial^2}{\partial x^2}+\frac{1}{\chi}\frac{\partial ^2}{\partial z^2})(1-C\nabla1^2)\psi\Big)+Ra_{T}\frac{\partial T}{\partial x}-Ra_{S}\frac{\partial S}{\partial x}=0, \end{aligned} $$

(31)

$$\displaystyle \begin{aligned} \Big(\frac{\partial}{\partial t}-\frac{\partial^2}{\partial z^2}-\eta\frac{\partial^2}{\partial x^2}-R_i\Big)T-f(z)\frac{\partial \psi}{\partial x}-\frac{\partial(\psi ,T)}{\partial(x,z)}=0, \end{aligned} $$

(32)

$$\displaystyle \begin{aligned} \Big[\frac{\partial}{\partial t}-\frac{1}{L_e}(\frac{\partial ^2}{\partial z ^2}+\frac{\partial ^2}{\partial x^2})\Big]S-\frac{\partial \psi}{\partial x} b(z)-\frac{\partial(\psi ,S)}{\partial(x,z)}-S_r\frac{Ra_T}{Ra_S}\nabla^2 T=0 \end{aligned} $$

(33)

It should be noted that the effect of nonlinearity is to distort the temperature and concentration fields through the interaction of ψ and T, ψ, and S. As a result, a component of the form sin(2πz) will be generated, where V is zonal velocity induced by rotation. A minimal Fourier series that describes the finite amplitude convection is given by

$$\displaystyle \begin{aligned} \psi = A_1(t) sin(ax)sin(\pi z),\end{aligned} $$

(34)

$$\displaystyle \begin{aligned} T = B_1(t)cos(ax)sin(\pi z) + B_2(t)sin(2 \pi z),\end{aligned} $$

(35)

$$\displaystyle \begin{aligned} S = C_1(t)cos(ax)sin(\pi z) + C_2(t)sin(2 \pi z), \end{aligned} $$

(36)

where the amplitudes A ₁(t), B ₁(t), B ₂(t), C ₁(t), C ₂(t) are functions of time and are to be determined. Substituting the above expressions in Equations (31)–(33) and equating the like terms, the following set of nonlinear autonomous differential equations were obtained

$$\displaystyle \begin{aligned} \frac{d A_1(t)}{dt}=\frac{-V_{a}}{\delta^2}(\delta1^2 (1+C\delta^2)A_1+a Ra_{T}B_1-a Ra_{S}C_1) \end{aligned} $$

(37)

$$\displaystyle \begin{aligned} \frac{d B_1(t)}{dt}= 2 a F A_1-\pi a A_1 B_2+(R_i-\eta_1) B_1 \end{aligned} $$

(38)

$$\displaystyle \begin{aligned} \frac{d B_2(t)}{dt}=\frac{\pi a}{2}A_1 B_1+(R_i-4\pi^2)B_2 \end{aligned} $$

(39)

$$\displaystyle \begin{aligned} \frac{d C_1(t)}{dt}=2 a B A_1-\delta^2 S_r \frac{Ra_T}{Ra_S}B_1-\delta^2 \frac{1 }{L_e}C_1-\pi a A_1 C_2 \end{aligned} $$

(40)

$$\displaystyle \begin{aligned} \frac{d C_2(t)}{dt}= \pi \frac{a}{2} A_1 C_1-4\pi^2 S_r \frac{Ra_T}{Ra_S}B_2- \frac{4 \pi^2 }{L_e}C_2 \end{aligned} $$

(41)

where A = 1 + 4cπ ². The numerical method was used to solve the above nonlinear differential equation to find the amplitudes.

5.1 Steady Finite Amplitude Convection

For steady-state finite amplitude convection we have to set the left-hand side of the Equations (37)–(41) to zero.

$$\displaystyle \begin{aligned} (\delta1^2 (1+C\delta^2)A_1+a Ra_{T}B_1-a Ra_{S}C_1)=0\end{aligned} $$

(42)

$$\displaystyle \begin{aligned} 2 a F A_1-\pi a A_1 B_2+(R_i-\eta_1) B_1=0\end{aligned} $$

(43)

$$\displaystyle \begin{aligned} \frac{\pi a}{2}A_1 B_1+(R_i-4\pi^2)B_2=0\end{aligned} $$

(44)

$$\displaystyle \begin{aligned} 2 a B A_1-\delta^2 S_r \frac{Ra_T}{Ra_S}B_1-\delta^2 \frac{1 }{L_e}C_1-\pi a A_1 C_2=0\end{aligned} $$

(45)

$$\displaystyle \begin{aligned} \pi \frac{a}{2} A_1 C_1-4\pi^2 S_r \frac{Ra_T}{Ra_S}B_2- \frac{4 \pi^2 }{L_e}C_2=0 \end{aligned} $$

(46)

on solving for the amplitudes in terms of A ₁, we obtain

$$\displaystyle \begin{aligned} B_1=\frac{4a F(z)(4\pi^2-R_i)A_1}{a^2 A_1^2\pi^2-8\pi^2 R_i+2 R_i^2+8\pi^2\eta_1-2R_i\eta}, \end{aligned}$$

$$\displaystyle \begin{aligned} B_2=\frac{2 a^2 F(z)\pi A_1^2}{a^2 A_1^2\pi^2-8\pi^2 R_i+2 R_i^2+8\pi^2\eta_1-2R_i\eta}, \end{aligned}$$

$$\displaystyle \begin{aligned} C_1=\dfrac{\begin{array}{l}16 \left(\-8 A_1 B L_e \pi^2 Ra_S R_i a + 2 A_1 B L_e Ra_S Ri^2 a +A_1^3 B L_e \pi^2 Ra_S a^3\right.\\ \left.+A_1^3 F L_e^2 \pi^2 Ra_T s a^3 - 8 A_1 F L_e \pi^2 Ra_T S_r a \delta^2 +2 A_1 F L_e Ra_T R_i S_r a \delta^2\right.\\ \left. +8 A_1 B L_e \pi^2 Ra_S a \eta -2 A_1 B L_e Ra_S R_i a \eta_1\right)\end{array}}{Ra_S (A_1^2 L_e^2 a^2 + 8 \delta^2) (-8 \pi^2 R_i + 2 R_i^2 +A_1^2 \pi^2 a^2 + 8 \pi^2 \eta_1 - 2 R_i \eta_1)}, \end{aligned}$$

$$\displaystyle \begin{aligned} C_2=\dfrac{\begin{array}{l}2 \left (\-8 A_1^2 B L_e^2 \pi^2 Ra_S R_i a^2 +2 A_1^2 B L_e^2 Ra_S R_i^2 a^2 +A_1^4 B L_e^2 \pi^2 Ra_S a^4\right. \\ \left. -8 A_1^2 F L_e \pi^2 Ra_T S a^2 \delta^2 -8 A_1^2 F L_e^2 \pi^2 Ra_T S a^2 \delta^2 +2 A_1^2 F L_e^2 Ra_T R_i S a^2 \delta^2\right. \\ \left. +8 A_1^2 B L_e^2 \pi^2 Ra_S a^2 \eta_1 -2 A_1^2 B L_e^2 Ra_S R_i a^2 \eta_1\right)\end{array}}{Ra_S \pi (A_1^2 L_e^2 a^2 + 8\delta^2) (-8 \pi^2 R_i + 2 R_i^2 +A_1^2 \pi^2 a^2 + 8 \pi^2 \eta_1 - 2 R_i \eta_1)}. \end{aligned}$$

To solve the above equation, a quadratic equation in $\frac {A_1^2}{8}$ is given by

a ₀ x ² + a ₁ x + a ₂ = 0

where x=$\frac {A_1^2}{8}$,

$a_0=L_e^2 a^4 \pi ^2 \delta _1^2 Ra_S(1+C \delta ^2)$

$a_1= \frac {1}{4}\delta _1^2 Ra_S(1+C \delta ^2)(R_i-\eta _1)L_e^2 a^2(R_i-4\pi ^2)-\frac {1}{2}(R_i-4\pi ^2)F Ra_T Ra_S L_e^2 a^4-2L_ea^4\pi ^2 Ra_S(B+L_e F S_r)+a^2 \pi ^2\delta ^2\delta _1^2Ra_S(1+C\delta ^2)$

$a_2=\frac {(R_i-4\pi ^2)}{4}(\delta ^2\delta _1^2Ra_S(1+C\delta ^2)(R_i-\eta _1)-2L_ea^2BRa_S(R_i-\eta _1)-2a^2\delta ^2FRa_S(L_eS_r+Ra_T))$

The required root of the above equation is

$x=\frac {-a_1+\sqrt {a_1^2-4a_o a_2}}{2 a_0}$

5.2 Steady Heat and Mass Transport

In the study of this type of problem, quantification of heat and mass transport is very important in porous media. Let Nu and Sh be noted as the rate of heat and mass transport per unit for the fluid phase.

The Nusselt number and Sherwood number are defined by

$$\displaystyle \begin{aligned} Nu=1+\Big[\frac{\int_0^{2\pi/a} \frac{\partial T}{\partial z} dx,}{\int_0^{2\pi/a} \frac{\partial T_b}{\partial z} dx,}\Big]_{z=0} \end{aligned} $$

(47)

$$\displaystyle \begin{aligned} Sh=1+\Big[\frac{\int_0^{2\pi/a} \frac{\partial S}{\partial z} dx,}{\int_0^{2\pi/a} \frac{\partial S_b}{\partial z} dx,}\Big]_{z=0} \end{aligned} $$

(48)

substituting the value of T, T _b,S, and S _b in Equations (47)–(48),

$$\displaystyle \begin{aligned} Nu=1-\frac{2\pi B_2}{\sqrt{R_i}\cot\sqrt{R_i}}, \end{aligned} $$

(49)

$$\displaystyle \begin{aligned} Sh=1-\frac{2\pi C_2Ra_S \sin\sqrt{R_i}}{-S_r Ra_T \cos\sqrt{R_i}\sqrt{R_i}+\sin\sqrt{R_i}Ra_S+\sin\sqrt{R_i}S_r Ra_T} \end{aligned}$$

substituting B ₂, C ₂ of Equations (??) into (49) gives

$$\displaystyle \begin{aligned} Nu,\ \ Sh \end{aligned} $$

(50)

6 Results and Discussion

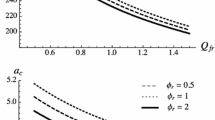
This chapter investigates the combined effect of internal heating and the Soret effect on stationary and oscillatory convection in a anisotropic porous medium with couple stress fluid. In this section, we discuss the effects of the parameters in the governing equations on the onset of double diffusive convection numerically and graphically. The stationary and oscillatory expressions for different values of the parameters such as the Vadasz number, the couple stress parameter, the solute Rayleigh number, the mechanical anisotropic parameter, and the thermal anisotropic parameter are computed, and the results are depicted in the figures. The neutral stability curves in the (Ra _T,a) plane for various parameter values are shown in Figure 2a–e. We fixed the values for the parameters as Va = 5, C = 2, Ra _S = 100, L _e = 20, ξ = .5, η = .5, S _r = .05, and R _i = 2, except for the varying parameter. The effect of the Vadasz number Ta on the neutral curves is shown in Figure 2. We find that for fixed values of all other parameters, the minimum value of the Rayleigh number for the oscillatory mode increases as a function of increasing Va, indicating that the effect of the Vadasz number is to stabilize the system. In addition, the critical wave number increases with increasing Va. We observed that by increasing the value of internal heat source R _i, the mechanical anisotropic parameter ξ decreased the stationary and oscillatory Rayleigh number, which means that the internal heat source R _i, mechanical anisotropic parameter ξ destabilized. Figure 2 depicts the effect of the couple stress parameter C on the neutral stability curves. We find that with an increase in the value of the couple stress parameter, the value of the Rayleigh number for both stationary and oscillatory mode is enhanced, indicating that it stabilizes the onset of double diffusive convection and depicts the effect of the solute Rayleigh number Ra _S on the stability curve for stationary and oscillatory convection. We show that the effect of increasing Ra _S is to decrease the value of the Rayleigh number for stationary and oscillatory convection and the corresponding wave number. Thus, the solute Rayleigh number becomes unstable. We also show that the effect of an increasing Lewis number L _e and the thermal anisotropic parameter η is to increase the value of the Rayleigh number for stationary convection and decrease the value of oscillatory convection. With regard to the corresponding wave number, we found it unstable for the stationary and stable for the oscillatory modes. We find that Figures 2 and 3 show that an increase in the value of the Soret parameter S _r decreases the Rayleigh number, indicating that the Soret parameter destabilizes the onset of stationary and oscillatory convection.

Fig. 2

Stationary neutral stability curves for the different values of (a), (b), (c), (d), (e), (f), (g)

Fig. 3

Oscillatory neutral stability curves for the different values of (a), (b), (c), (d), (e), (f), (g), (h)

We use the parameter in a graph of the Nusselt and Sherwood number C = 2, Ra _S = 20, L _e = 2, ξ = .5, η = .5, S _r = .05, and R _i = 2, and Figures 4a and 5c show that an increase in the value of the internal Rayleigh number R _i decreases the rate of heat and increases mass transfer. We note that the effect of increasing the solute Rayleigh number Ra _S and the thermal anisotropic parameter η is to increase the value of the Nusselt number N _u and the Sherwood number S _h, thus reducing the heat and mass transfer. In Figures 4b and 5a, it can be found that with an increase in the value of the Soret parameter S _r, the mechanical anisotropic parameter ξ and then the value of the Nusselt number N _u and the Sherwood number S _h decrease; thus, the heat and mass transfer across the porous layer also decrease.

Fig. 4

Variation of Nu with Rat for different values of parameters

Fig. 5

Variation of Sh with Rat for different values of parameters

7 Conclusions

The Soret effect and the internal heating effect on double diffusive convection in a anisotropic porous medium saturated with a couple stress fluid that is heated and salted from below was investigated using linear and nonlinear stability analysis. The linear analysis is carried out using the normal mode technique. The following conclusions were drawn:

• 1) The Vadasz number Va has a stabilizing effect on oscillatory convection.

• 2) The internal heat parameter R _i, the solute Rayleigh number Ra _s, the Soret parameter S _r, and the mechanical anisotropic parameter ξ destabilize the system in the stationary and oscillatory modes.

• 3) The couple stress fluid C has a stabilizing effect on both the stationary and the oscillatory convection.

• 4) The normalized porosity parameter η and the Lewis number L _e have a destabilizing effect in the case of stationary and opposite oscillatory convection.

• 5) With the increasing value of the mechanical anisotropic parameter ξ, the Soret parameter S _r then increases the value of the Nusselt number N _u, i.e., increasing heat transfer, but increasing the value of the internal Rayleigh number R _i, and the normalized porosity parameter η and the solutal Rayleigh number Ra _S decrease the value of the Nusselt number N _u.

• 6) Mass transfer S _h increases with the increasing value of the internal Rayleigh number R _i, the mechanical anisotropic parameter ξ, the Soret parameter S _r, and decreases with the normalized porosity parameter η and the solutal Rayleigh number Ra _S.