1 Introduction

Due to their outstanding material features like large deformation, light weight, low cost, flexibility, chemical and biological compatibility [1–3], dielectric elastormers (DEs) have been receiving increasing interest for applications in electromechanical transducers, artificial muscles, adaptive optical elements, soft robots and electromechanical resonators [4–8]. In general, the DE membrane consists of a soft elastomeric membrane sandwiched between two compliant electrodes. As reported in the literature, the DE material is mostly expected to perform under dynamic situations in applications, e.g., pumps, loudspeakers [9, 10] and vibrotactile display for mobile applications [11].

In the past decade, it has been reported that the material behavior of DEs is strongly nonlinear in both geometry and constitutive relations [12]. Some early studies were concerned with the nonlinear modeling of DEs in the static case [13, 14]. Some of the work has focused on the quasi-static deformation [15] by neglecting the effect of inertia.

However, a DE is often subject to transient, harmonic forces and/or time-dependent voltages in practical applications. For example, when a DE performs as an electromechanical actuator, time-dependent external loadings are inevitable. In such a case, the inertia may play a significant role in the performance of dynamic applications. Indeed, the dynamic behavior of DEs has been frequently observed in experiments when investigating, for example, frequency tuning [16], acoustic actuator [17] and vibrotactile displays for mobile applications [11]. Therefore, the dynamical problem of DEs is fast becoming a new paradigm in nonlinear dynamics due to the fact that a number of issues related to time-dependent behaviors of DEs are still unsolved. Perhaps one of the most important new features compared with other conventional materials is that the DEs are usually subject to extremely large deformation. This implies that such a dynamical system is strongly nonlinear, and hence, the available analytical methods for analyzing the nonlinear dynamical behavior of DEs are very limited.

Before presenting the objective of this work, it is instructive to review some of the notable contributions on the dynamic analysis of DE membranes. Zhu et al. [18, 19] studied the resonant behavior of a pre-stretched DE membrane and the nonlinear vibrations of a DE balloon. They reported that for a DE balloon subject to a combination of static pressure and static voltage, the balloon may reach a state of equilibrium. When the voltage is sinusoidal, the balloon resonates at multiple frequencies of excitation. Yong et al. [20] have analyzed the dynamic responses of a thick-walled DE spherical shell. They found that the spherical shell may be destroyed as the mean voltage exceeds the critical mean voltage. It was also shown that the spherical shell may undergo a nonlinear quasi-periodic oscillation. Li et al. [21] explored the electromechanical behavior of a tunable pure shear DE-based resonator, showing that when actuating the resonator, there is a safe operation range of system parameters for failure prevention. Xu et al. [12] developed a theoretical model for studying the dynamics of a DE-based actuator with stretching deformation. The equation of motion for the DE-based actuator was obtained by the Euler-Lagrange equation. Soares et al. [22] proposed a mathematical model for describing the nonlinear dynamics of a radially pre-stretched hyperelastic annular membrane with finite deformations. By analytically and numerically solving the linearized equations of motion, the vibration modes and frequencies of the hyperelastic membrane were obtained, and these normal modes were then used together with the Galerkin’s approach to obtain reduced order models for the nonlinear dynamical system. Sheng et al. [23] used the Euler-Lagrange equation to characterize the influence of temperature, excitation frequency and viscoelasticity on the dynamic electromechanical deformation and stability of viscoelastic dielectrics. Li et al. [24] presented an analysis of the nonlinear dynamics of a DE as an electromechanical resonator configured as a pure shear actuator. They have detected chaotic behavior when the DE membrane is connected with another elastic membrane oscillating simultaneously. Sheng et al. [25] have constructed a dynamic model of DE actuator by using thermodynamics in which the Gent model was used to describe the extension limit of the DE. Recently, Zhou et al. [26] considered the viscoelastic effect to investigate the frequency tuning of a DE membrane resonator. It was found that not only the applied voltage changes the natural frequency of the resonator, but also the inelastic deformation contributes to frequency tuning. It was also concluded that the electrical loading rate may influence the actuation process of the DE resonator, while it has little effect on the final steady frequency tuned by the prescribed voltage within the safety range. Zhang et al. [27] have studied the dynamic performance of a viscoelastic DE under the conditions of equal-biaxial force, uniaxial force and pure shear state, respectively. It was observed that for the boundary conditions of an equal-biaxial force or pure shear state, the DE membrane may undergo a nonlinear quasi-periodic vibration; for a DE membrane subject to a uniaxial force, however, an aperiodic motion would occur. Very recently, Zhu [28] studied the dynamic instability of a membrane of DE. It was demonstrated that pre-stretches can improve DE-based actuators’ capabilities to resist dynamic instability. Interestingly, it was shown that the critical deformation at the onset of dynamic instability can be much larger than that at the onset of static instability.

The linear and nonlinear dynamics of DE-based beams have also been studied by several investigators in the past years. Feng et al. [3] developed an analytical model to discuss the dynamic characteristics of a DE-based microbeam resonator. The resonant frequencies of the resonator were derived using Raleigh’s method for small amplitude vibrations. It was found that high voltage is beneficial for improving the sensitivity of the resonator. However, high voltage may put the resonator at the risk of mechanical instability. Afterward, Feng et al. [29] further investigated the dynamic performance of DE-based microbeam resonators with the consideration of gas damping under large amplitude vibrations. It was shown that the initial pre-stretching stress and the ambient pressure can significantly alter the resonant frequency of the resonator.

Although the above studies have attempted to investigate the dynamical behavior of DE membranes or beams, the literature on the nonlinear dynamics of this type of structures is relatively limited. Many issues related to dynamic responses are unsolved yet, as discussed by Li et al. [24]. Indeed, a further understanding on the fundamental/basic dynamical behavior of DE membranes is expected to be useful and helpful for designs and applications of DE membranes. Consequently, this motivates the current work.

In this study, we present a theoretical and numerical study aiming at exploring the nonlinear oscillations of a DE membrane undergoing in-plane stretching. The width of the DE membrane is clamped and mounted on a pair of slide tracks (see Fig. 1). To analyze the basic dynamics of the DE membrane, it is assumed that the possible voltage across the membrane is absent. In particular, the nonlinear free oscillation of the DE membrane without external time-dependent forces is investigated, as well as the forced oscillation due to external harmonic forces, detailedly showing the dynamic responses of the DE membrane system.

Fig. 1
figure 1

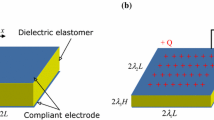
a Reference state: the DE membrane has dimensions \(L_{1}, L_{2}\) and \(L_{3}\), without any external forces; b mounted state: the DE membrane is stretched and fixed to a rigid frame and a pair of slide tracks; c spring-constrained state; and d excited state: the DE membrane is subjected to boundary force stretching

Full size image

2 Problem formulation

The analytical model of DE membranes will be described in this section. To focus on the fundamental/basic dynamics of DE membranes, the present study is concerned with the nonlinear dynamic responses of a DE membrane undergoing homogeneous deformation with in-plane stretch only. As discussed by several researchers [24, 30], in targeting application of vibration resonators, the natural rubber qualifies as ideal for its fast response within the elastic range, large stress and little viscoelastic dissipation; thus, we only focus on elastic deformations in the modeling.

In this study, the dynamic responses of DE membranes without electric loading will be investigated, although much work has been devoted to some aspects of DE membranes subject to electric loading. Obviously, a first better understanding on the basic dynamics of DE membranes without electric loading is necessary and significant in order to further study the nonlinear responses of DE membranes excited by electric loading.

The DE membrane under consideration is shown in Fig. 1. As plotted in Fig. 1a, the DE membrane in its reference state has initial dimensions of length \(L_{1}\), width \(L_{2}\) and thickness \(L_{3}\). Suppose that the membrane is incompressible, of density \(\rho \). It is worthwhile to point out that in practice, the membrane of a dielectric elastomer is generally sandwiched between two electrodes. For the dielectric elastomer to deform substantially, the electrodes are made of an even softer substance, with mechanical stiffness much lower than that of the dielectric elastomer. Therefore, the current work assumes the electrodes to be of negligible mechanical stiffness. The width of the membrane is devised to be fixed using rigid clamps and then mounted on a pair of slide tracks, as shown in Fig. 1b. Since the membrane elongates only in length, such a deformation mode is well defined as pure shear [31].

In some cases, the membrane may be subjected to an in-plane mechanical force. In Fig. 1c, several springs (linear or nonlinear) stretch the membrane to keep it in tension. Such a spring force may be viewed as a mechanical force which usually provides tensile stretching. Here, we initially introduce a force P representing the mechanical force (see Fig. 1d). In the following analysis, the three models shown in Fig. 1b–d will be separately considered.

To describe the stretching behavior of the DE membrane, we define \(\lambda _{i}\) \((i=1,2,3)\) as scaling factors for the three directions. It is seen in Fig. 1b–d that \(\lambda _{2 }= 1\) is prescribed and \(\lambda _{3 }= 1/(\lambda _{1}\lambda _{2})\). For simplicity, we denote \(\lambda =\lambda _{1}\) in the following analysis.

For a DE membrane subjected to electric loading, the dynamic behavior of the DE membrane is characterized by the density of the Helmholtz free energy as a function of the two independent variables, \(W(\lambda , D)\), where D is the electric displacement of the membrane. In this work, however, we focus on the dynamic response of the DE membrane without any electric loadings. Thus, the free energy density function is only associated with the stretch ratio of \(\lambda \).

To derive the formulation of the free energy density function, we employ the neo-Hookean model for characterization of the hyperelasticity in the DE membrane. Of course, various strain energy models are available to describe the mechanical behavior of DE membrane, e.g., Gent model and neo-Hookean model. In this work, the DE membrane is supposed to be composed of natural rubber as its key component, with a stretch ratio below 5 and no significant stiffening. Thus, the neo-Hookean model is adequate for characterization [24, 32].

Then, according to the neo-Hookean model, the free energy density function may be written as [24]

$$\begin{aligned} W(\lambda )=\frac{\mu }{2}\left( {\lambda ^{2}+\lambda _2^2 +\lambda ^{-2}\lambda _2^{-2} -3} \right) \end{aligned}$$
(1)

in which \(\mu \) is the shear modulus of the elastomer.

For an oscillating DE membrane, the inertia force in every material element of its length is given by [24]

$$\begin{aligned} F_i =-\frac{\hbox {d}^{2}\lambda }{\hbox {d}t^{2}}\rho xL_2 L_3 \hbox {d}x \end{aligned}$$
(2)

Thus, the total work done by the inertial force may be obtained by integrating \(F_{i}\) along the length, i.e.,

$$\begin{aligned} -\rho L_2 L_3 \frac{\hbox {d}^{2}\lambda }{\hbox {d}t^{2}}\delta \lambda \int _0^{L_1 } {x^{\hbox {2}}\hbox {d}x} =-\frac{\rho L_1^3 L_2 L_3 }{3}\frac{\hbox {d}^{2}\lambda }{\hbox {d}t^{2}}\delta \lambda \end{aligned}$$
(3)

According to the variation principle, the variation in the free energy of the DE membrane should equal to the work done jointly by the mechanical force and inertia force; thus

$$\begin{aligned} L_1 L_2 L_3 \delta W=PL_1 \delta \lambda -\frac{\rho L_1^3 L_2 L_3 }{3}\frac{\hbox {d}^{2}\lambda }{\hbox {d}t^{2}}\delta \lambda \end{aligned}$$
(4)

or

$$\begin{aligned} \frac{\partial W}{\partial \lambda }=\frac{P}{L_2 L_3 }-\frac{\rho L_1^2 }{3}\frac{\hbox {d}^{2}\lambda }{\hbox {d}t^{2}} \end{aligned}$$
(5)

Substituting Eq. (1) into Eq. (5), one obtains

$$\begin{aligned} \frac{\hbox {d}^{2}\lambda }{\hbox {d}t^{2}}+\frac{3\mu }{\rho L_1^2 }\left( {\lambda -\lambda ^{-3}\lambda _2^{-2} } \right) -\frac{3P}{\rho L_1^2 L_2 L_3 }=0 \end{aligned}$$
(6)

Defining the following two dimensionless parameters

$$\begin{aligned} T=t\sqrt{\frac{3\mu }{\rho L_1^2 }},\quad F=\frac{1}{\mu L_2 L_3 }P \end{aligned}$$
(7)

Eq. (6) may be rewritten as

$$\begin{aligned} \frac{\hbox {d}^{2}\lambda }{\hbox {d}T^{2}}+g\left( \lambda \right) =0 \end{aligned}$$
(8)

where \(g(\lambda )=(\lambda -\lambda ^{-3}\lambda _2^{-2} )-F\).

For the DE membrane system shown in Fig. 1b, c, Eq. (8) governs the free oscillations of the DE membrane since F does not explicitly contain a time-dependent component in these two cases. For the system plotted in Fig. 1d, however, forced oscillations may occur if the external force F has a time-dependent harmonic component superposed on a constant value.

3 Free oscillation

The performance of the DE membrane may be affected by the mechanical boundary conditions of the system. In static deformation, the strain in the DE membrane with a fully fixed frame rises to over 100 % in terms of in-plane extension before breakdown [33]. However, when the frame is replaced by a mechanical weight, the maximum strain may be up to 380 % [31]. In dynamic deformation, it was also reported that the dynamic performances of DE membranes may vary greatly due to the change of boundary conditions.

In the analysis of free oscillations, two types of boundary conditions will be considered: (1) the DE membrane has a free end shown in Fig. 1b and (2) the movable end of the DE membrane is constrained by linear/nonlinear springs.

3.1 Dynamic response of a DE membrane without constrained springs

We first analyze the dynamics of the DE membrane shown in Fig. 1b. In such a case, the equation of motion, Eq. (8), may be reduced to

$$\begin{aligned} \frac{\hbox {d}^{2}\lambda }{\hbox {d}T^{2}}+\lambda -\lambda ^{-3}=0 \end{aligned}$$
(9)

It is clear that the natural frequency of the linearized system of Eq. (9) is equal to 1. Solving Eq. (9), with a set of selected initial conditions of stretch ratio, the nonlinear oscillation of the DE membrane may be obtained in the time domain. In the following calculations, the initial velocity of stretch ratio is fixed to be 0.

In the calculations, it is found that the initial length of the membrane may affect the oscillation of the system. We plot a bifurcation diagram in Fig. 2 to show the detailed effect of initial stretch ratio on the oscillation amplitudes of the membrane. It is seen from this figure that the membrane is usually undergoing periodic motions when the initial stretch ratio \(\lambda (0)\) is successively increased. When \(\lambda (0)=1\), the membrane would keep static with \(\lambda (T)=1\) since the static equilibrium is \(\lambda _{s}=1\). For sufficiently small or large value of \(\lambda (0)\), the oscillations are periodic with high oscillation amplitude, as may be observed in Fig. 2.

Fig. 2
figure 2

Bifurcation diagram when the initial stretch ratio is varied

Full size image

The phase portrait, time history diagram and power spectra (PS) diagram for two typical values of \(\lambda (0)\) are presented in Fig. 3. Of particular interest is that the oscillation frequency for \(\lambda (0)=0.5\) is equal to that for \(\lambda (0)=4.5\), although their oscillation amplitudes are different.

Fig. 3
figure 3

Nonlinear responses of the DE membrane for two different initial stretch ratios: \(\lambda (0)=0.5\) or 4.5; a phase portrait, b time history diagram and c power spectra diagram

Full size image

It is also observed from Fig. 3c that the dimensionless oscillation radian frequency is \(\omega = 2\pi f \approx 2\) (f is the frequency of the system), at which the maximum amplitude appears. The nonlinear oscillation frequency is much higher than the natural frequency. Actually, the oscillation frequency may be analytically calculated. The dimensionless oscillation frequency takes the form

$$\begin{aligned} \omega ^{2}=\left. {\frac{\partial g(\lambda )}{\partial \lambda }} \right| _{\lambda =\lambda _s } \end{aligned}$$
(10)

at the static equilibrium \(\lambda _s \). Thus, one may solve Eq. (10) by using \(g(\lambda )=\lambda -\lambda ^{-3}\) and obtains

$$\begin{aligned} \omega =\left( {1+3\lambda _s^{-4} } \right) ^{1/2} \end{aligned}$$
(11)

For the DE membrane shown in Fig. 1b, it is obvious that \(\lambda _s =1\), thus leading to \(\omega =2\).

3.2 Dynamic response of a DE membrane with constrained linear/nonlinear springs

In this subsection, we will focus on the dynamic response of a DE membrane with constrained linear or nonlinear springs. As illustrated in Fig. 1c, several springs stretch the membrane to keep it in tension. Denoting the stiffness of the linear spring by \(k_{1}\) and the stiffness of the cubic spring by \(k_{3}\), the variable P in Eq. (6) may be given by

$$\begin{aligned} P=k_1 \left[ {d-(\lambda -1)L_1 } \right] \end{aligned}$$
(12)

for a linear spring case and

$$\begin{aligned} P=k_3 \left[ {d-(\lambda -1)L_1 } \right] ^{3} \end{aligned}$$
(13)

for a cubic spring case. In Eqs. (12) and (13), d is the initial elongation strain of the springs. In this paper, the value of d is selected from the literature and defined by \(L_{1}/d =0.1\) [24].

Typical results of the bifurcation diagrams for the linear spring case and cubic spring case are plotted in Fig. 4a, b, respectively. It is immediately seen that these two bifurcation diagrams are similar as that shown in Fig. 2 for a DE membrane without springs. However, the static equilibrium positions in Fig. 4a, b are found to be \(\lambda _s \approx 1.36\) and \(\lambda _s \approx 2.17\), respectively, which are different from that shown in Fig. 2. These two static equilibria may be also analytically predicted.

Fig. 4
figure 4

Bifurcation diagrams when the initial stretch ratio is varied; a linear spring with \(k_{1}=1\), and b cubic spring with \(k_{3}=3\)

Full size image

Since the response of the DE membrane is periodic, during an oscillation period there is a minimum value and a maximum value of \(\lambda (T)\). For example, when \(k_{1}=1\) and \(\lambda (0)=0.5\), the minimum value of \(\lambda (T)\) is found to be \(\lambda _{\min } =0.5\) and the maximum value of \(\lambda (T)\) is equal to \(\lambda _{\max } =2.95\). As may be expected, the minimum and maximum values of \(\lambda (T)\) are related to several system parameters, i.e., \(\lambda (0), k_{1}\) and \(k_{3}\). Typical results for six sets of system parameters are shown in Figs. 5 and 6. From these two plots, it is readily figured out that when \(\lambda (0)\) is smaller than the static equilibrium value, the minimum value of \(\lambda (T)\) would be equal to \(\lambda (0)\) (see, for example, the result of \(k_{1}=6\) shown in Fig. 5c and the result of \(k_{3}=15\) shown in Fig. 6c). If, however, the initial stretch ratio \(\lambda (0)\) is larger than the static equilibrium value, the maximum value of \(\lambda (T)\) would be equal to \(\lambda (0)\) (see, for example, the result of \(k_{1}=2\) shown in Fig. 5c and the result of \(k_{3}=10\) shown in Fig. 6c).

Fig. 5
figure 5

Phase portraits of the nonlinear responses of the DE membrane for various \(k_{1}\) and \(\lambda (0)\); a \(\lambda (0)=0.5\), b \(\lambda (0)=1\), and c \(\lambda (0)=4\)

Full size image
Fig. 6
figure 6

Phase portraits of the nonlinear responses of the DE membrane for various \(k_{3}\) and \(\lambda (0)\); a \(\lambda (0)=0.5\), b \(\lambda (0)=1\), and c \(\lambda (0)=4\)

Full size image

4 Forced oscillation

This section presents the nonlinear forced oscillation of the DE membrane by means of the bifurcation diagram, phase portraits, time history diagrams and PS diagrams. For that purpose, we write the equation of motion as

$$\begin{aligned} \frac{\hbox {d}^{2}\lambda }{\hbox {d}T^{2}}+\lambda -\lambda ^{-3}=f_0 \left[ {1+a\sin (\varOmega T)} \right] \end{aligned}$$
(14)

in which \(f_{0}\) is the forcing amplitude, \(\varOmega \) is the forcing frequency and a is generally small.

The effect of forcing frequency on the dynamical behavior of the system will be examined. Throughout the numerical simulation, f and a are chosen as \(f_{0}=1\) and \(a=0.2\). The initial stretch ratio is fixed to be \(\lambda (0)=1.5\).

The bifurcation diagram of the dynamic responses of the DE membrane is depicted in Fig. 7. This plot illustrates that as the excitation frequency is increased gradually from \(\varOmega =0\), the responses of the DE membrane dramatically evolve. At \(\varOmega \approx 0.65, 1.55\) or 2.7, the maximum amplitudes of the oscillation change sharply. Indeed, in the vicinity of each value of these three excitation frequencies, the oscillation traces display much difference when \(\varOmega \) is gradually increased. In Fig. 7, the three excitation frequencies (\(\varOmega \approx 0.675, 1.55\) and 2.7) at which three peak amplitudes occur are associated with the resonances of the nonlinear dynamical system. It may be readily found that the natural frequency of the linearized system of Eq. (14) is about \(\omega _{0} \approx 1.35\). At about \(\varOmega = 0.675\) and 2.7, the super-harmonic and sub-harmonic resonances of the system would occur. The peak amplitude at about \(\varOmega = 1.55\) (slightly larger than \(\omega _{0})\) is due to the occurrence of the primary resonance of system (14). When the sub-harmonic, super-harmonic or primary resonances are excited, the amplitude of the elastomer becomes relatively large.

Fig. 7
figure 7

Bifurcation diagram when the excitation frequency is varied and \(\lambda (0)=1.5, f_{0}=1\) and \(a=0.2\)

Full size image

To better understand the dynamic responses of the system, several phase portraits, time history diagrams and PS diagrams are plotted in Fig. 8 for various excitation frequencies. It is clearly seen from this figure that the oscillations of the system are quasi-periodic, which are significantly different from those of the DE membrane without any harmonic forces.

Fig. 8
figure 8

Nonlinear forced responses of the DE membrane for various \(\varOmega \) when \(\lambda (0)=1.5, f_{0}=1\) and \(a=0.2\); a \(\varOmega =0.7\), b \(\varOmega =1.5\), c \(\varOmega =2.5\), and d \(\varOmega =4.5\)

Full size image

In each PS diagram of Fig. 8, it may be found that there is a certain peak amplitude at which the frequency is proximately equal to the excitation frequency. For example, in Fig. 8c, the PS curve contains a peak amplitude with \(\varOmega =2.5\), which is the same as the excitation frequency. However, each PS curve in Fig. 8 also contains many other frequencies at which peak amplitudes occur. From a nonlinear dynamics point of view, it is difficult to theoretically predict the values of these frequencies in such a strongly nonlinear system.

5 Conclusions

The nonlinear oscillations of a DE membrane undergoing in-plane stretching have been investigated in this paper numerically, based on the neo-Hookean model for characterization of the hyperelasticity. The width of the membrane is set to be fixed using rigid clamps and then mounted on a pair of slide tracks. The nonlinear differential equation of motion was derived based on energy consideration.

Examining the nonlinear free oscillations of the system revealed that the effect of initial stretch ratio on the amplitude of periodic oscillations is remarkable. The nonlinear oscillation frequency is much higher than the linear natural frequency of the system. Moreover, the static equilibrium of the system may be changed in the presence of linear/or nonlinear springs. In the case of forced oscillation of the system with harmonic excitations, it is found that the DE membrane is usually undergoing quasi-periodic oscillations. This work is envisaged to be helpful for the design and applications of soft structures based on DE membranes.