1 Introduction

We all know that a quantum computer (QC) will be more efficient than a classical computer in solving some certain problems. The elementary unit of quantum information in a QC is the quantum bit (qubit), and a variety of physical systems have been suggested as qubits. Due to the knowledge of their theoretical and experimental properties and the existence of an industrial base for semiconductor processing, semiconducting quantum dots as a promising candidate for scalable quantum information processing have attracted wide attention for more than a decade. However, the physical implementation of a quantum register requires a high degree of the quantum regime isolation from the environment. If not, decoherence will lead to loss of quantum information [1, 2]. Therefore, the decoherence dynamics attributed to the entanglement of the qubit system with its surrounding environment has become the central issue in the investigation of quantum information processing [3–8].

In the real world, the work of the experiment about quantum qubits is performed at finite temperature. However, quantum systems are very frail and the temperature destroys the quantum coherence of the stored information [9], a process called decoherence. So that the temperature effects on quantum systems for information processing should be investigated. One of the most prominent issues facing investigation of quantum decoherence in QDs qubit is the influence of the temperature on the QDs qubit. We have studied the temperature effects of the parabolic linear bound potential quantum dot qubit and the parabolic quantum dot qubit in the electric field in our earlier papers [10–12], and the effects of the temperature on the coherence time of a PQD qubit and a RbCl parabolic QD qubit have been researched in our other papers [13–15] and the paper of Xiao and Wang [16].

In this paper, using the variational method of the Pekar type, the temperature influences on the parabolic quantum dot are studied in the magnetic field under the condition of electric–LO-phonon strong coupling. The paper is arranged as follows. In Sect. 2, we first analyze the system and introduce the general Hamiltonian of an electric–LO-phonon system in the magnetic field and then obtain the relations of the oscillation period of the electron in the superposition state of the ground state and the first-excited state to the temperature, the magnetic field, the electron–LO-phonon coupling constant and the confinement length, respectively. In Sect. 3, we demonstrate the numerical results in detail. Sect. 4 presents our conclusions.

2 Theoretical Model

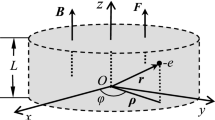
We consider a system where the electrons are bounded by the parabolic potential and the magnetic field. The electrons are much more confined in one direction (taken as the Z direction) than in other two directions. Therefore, we shall only take the effect of electron and LO-phonon on the QDs qubit into account assuming that the electrons move in the X–Y plane. The Hamiltonian of an electron–phonon system in the presence of the static uniform magnetic field which is along the z direction \({{\varvec{B}}}=(0,0,B)\) and described by a vector potential in the Landau gauge \({{\varvec{A}}}=B({-y}/2,x/2,0)\) can be written as follows:

$$\begin{aligned} H={\left( {{{\varvec{p}}}+e{{\varvec{A}}}} \right) ^{2}}/{2m^{*}}+{m^{*}\omega _0^2 \rho ^{2}}/2+\sum _{{\varvec{q}}} {\hbar \omega _\mathrm{{LO}} b_{{\varvec{q}}}^+ b_{{\varvec{q}}} } +\sum _{{\varvec{q}}} {\left( {V_q e^{i{{\varvec{q}}}\cdot {{\varvec{r}}}}b_{{\varvec{q}}} +h\cdot c} \right) },\nonumber \\ \end{aligned}$$
(1)

where \(m^{*}\) is the band mass of electron. \({{\varvec{p}}}\) is the momentum operator of the electron. \({{\varvec{\rho }} }\) is the two-dimensional coordinate vector, and \(\omega _0 \) is the confinement constant. \({m^{{*}}\omega _0^2 \rho ^{2}}/2\) is the parabolic confining potential in a single QD. \(\omega _\mathrm{{LO}} \) is the frequency of the longitudinal optical vibrational mode of the lattice oscillation. \(b_q^+ \left( {b_q } \right) \) is the creation (annihilation) operator of bulk LO-phonon with the wave vector \({q}({q}_{{//}}, q_\bot )\). \({{\varvec{r}}}=\left( {\mathbf{\rho },z} \right) \) is the coordinate of the electron. e is the electron charge, and

$$\begin{aligned} V_q= & {} \left( {{i\hbar \omega _\mathrm{{LO}} }/q} \right) \left( {\hbar /{2m^{{*}}\omega _\mathrm{{LO}} }} \right) ^{1/4}\left( {{4\pi \alpha }/V} \right) ^{1/2}, \end{aligned}$$
(2)
$$\begin{aligned} \alpha= & {} \left( {{e^{2}}/{2\hbar \omega _\mathrm{{LO}} }} \right) \left( {{2m^{{*}}\omega _\mathrm{{LO}} }/\hbar } \right) ^{1/2}\left( {1/{\varepsilon _\infty }-1/{\varepsilon _0 }} \right) , \end{aligned}$$
(3)

where \(\alpha \) is the dimensionless number that plays the role of an electron–LO-phonon coupling constant for the lattice–electron interaction. V is the volume of the crystal. \(\varepsilon _\infty \) and \(\varepsilon _0 \) are the high-frequency and vacuum dielectric constant, respectively.

Using the Fourier expansion and the LLP transformation, we have

$$\begin{aligned} {H}'=U^{-1}HU. \end{aligned}$$
(4)

2.1 Two-level Systems of Electron–Phonon

Suppose the Gaussian function approximation is valid in the ground state and the first-excited state of electron–phonon system by the method of the Pekar type. We choose the trial wave functions of the electron–phonon system in the ground and the first-excited state [17] as

$$\begin{aligned} |\phi _{e-p}\rangle= & {} \left[ \lambda \hbox {exp}(-\lambda ^{2} \rho ^{2}/2)/{\sqrt{\pi }} \right] | \xi (z) \rangle | 0_\mathrm{{ph}}\rangle , \end{aligned}$$
(5)
$$\begin{aligned} |\phi _{e-p}\rangle ^{\prime }= & {} \left[ \lambda ^{2} \rho \, \hbox {exp}(-\lambda ^{2} \rho ^{2}/2) \hbox {exp} (\pm i\varphi )/ {\sqrt{\pi }} \right] | \xi (z) \rangle | 0_\mathrm{{ph}}\rangle . \end{aligned}$$
(6)

Using \(l_0 ,\theta \,and \,\omega _c\) to denote \(\left( {\hbar /{m^{{*}}\omega _0 }} \right) ^{1/2},{\omega _c }/{\omega _\mathrm{{LO}} }\) and \({eB}/{m^{{*}}}\) respectively, choosing the polaron unit \(\left( {\hbar =2m^{{*}}=\omega _\mathrm{{LO}} =1} \right) \), we find the ground state energy and the first-excited state energy of the electron as the following forms

$$\begin{aligned} E_{0}= & {} \lambda ^{2}_{0}+(1+\theta ^{2}/16)/\lambda ^{2}_{0} l^{4}_{0} -\alpha \lambda _{0} (2\pi )^{1/2}/2, \end{aligned}$$
(7)
$$\begin{aligned} E_{1}= & {} 2\lambda ^{2}_{0}+(1+\theta ^{2}/16)/\lambda ^{2}_{0} l^{4}_{0} -11 \alpha \lambda _{0} (2\pi )^{1/2}/32, \end{aligned}$$
(8)

where \(l_0\) is the confinement length and \(\theta \) is the cyclotron frequency parameter [18–21].

We can obtain \(\lambda _{0}\) by the variational method, and then we can get the eigenlevel and the eigenwave function. Thus, we obtain the two-level system needed by a single qubit.

2.2 Probability Density

The time evolution of the quantum state of the electron in this system can be written as

$$\begin{aligned} \psi _{01} ={\left[ {\phi _0 \left( \rho \right) \exp \left( {-iE_0 t/\hbar } \right) } \right] }/{\sqrt{2}}+{\left[ {\phi _1 \left( \rho \right) \exp \left( {-iE_1 t/\hbar } \right) } \right] }/{\sqrt{2}}. \end{aligned}$$
(9)

Based on Eqs. (7)–(9), we can present the probability density in the following form

$$\begin{aligned} Q(\rho ,t)= & {} [\left| {\phi _0 (\rho )} \right| ^{2}+\left| {\phi _1 (\rho )} \right| ^{2}+\phi ^{{*}}_0 (\rho )\phi _1 (\rho )\exp (i\omega _{01} t)\nonumber \\&+\,\phi _0 (\rho )\phi ^{{*}}_1 (\rho )\exp (-i\omega _{01} t)]/2, \end{aligned}$$
(10)

where \(\phi _0 (\rho ) \,and \, \phi _1 (\rho )\) of Eqs. (9) and (10) are the ground state and the first-excited state, respectively. And

$$\begin{aligned} \omega _{01}=(E_{1}-E_{0})/\hbar \end{aligned}$$
(11)

then the period of oscillation is

$$\begin{aligned} T_0 ={2\pi }/{\omega _{01} }={2\pi }/{\left[ {\lambda _0^2 +{\left( {1+{\theta ^{2}}/{16}} \right) }/{\lambda _0^2 l_0^4 }+{5\left( {2\pi } \right) ^{1/2}\alpha \lambda _0 }/{32}} \right] }. \end{aligned}$$
(12)

The mean number of optical phonons of the superposition state around the electron in parabolic quantum dot is

$$\begin{aligned} N_{q}=\left\langle \psi _{01}|U^{-1} {\mathop {\sum }_{q}} b^{+}_{q} b_{q} U| \psi _{01}\right\rangle =27(2\pi )^{1/2} \alpha \lambda _{0}/64. \end{aligned}$$
(13)

2.3 Temperature Influences

At a finite temperature, the electron–phonon system is no longer in the ground state entirely. The lattice vibrations excite not only the real phonon but also the electron in a parabolic potential. In this case, the statistical average value of the electron–phonon system in various states can describe the properties of the bound polaron. According to quantum statistics, the statistical average number of optical phonons can be described as

$$\begin{aligned} {\bar{N}}_{q}=\left[ \hbox {exp}(\hbar \omega _\mathrm{{LO}}/k_\mathrm{B} T)-1\right] ^{-1}, \end{aligned}$$
(14)

where \(k_\mathrm{B}\) is the Boltzmann constant.

Through Eqs. (13) and (14) self-consistent calculation, we can obtain the relationship of the variational parameter \(\lambda _0\) with the temperature T. From Eqs. (7), (8) and (12), we can obtain the relation of \(T_{0}\) to \(\lambda _0 , T\).

3 Results and Discussions

The influences of the temperature on the period of oscillation, which are extracted from a numerical evaluation, are shown in Figs. 1, 2 and 3.

Fig. 1
figure 1

a The period of oscillation as a function of the temperature and the cyclotron frequency parameter. b The period of oscillation as a function of the cyclotron frequency parameter at different temperatures

Full size image

Figure 1a illustrates the period of oscillation as a function of the temperature and the cyclotron frequency parameter when the electron is in the superposition state of \(\psi _{01}\) for the electron–LO-phonon coupling constant \(\alpha =6\) and the confinement length \(l_{0}=0.5\). It is shown that the period of oscillation is increasing with increasing temperature which is in agreement with the results of the paper [12]. The reason is that, with the increment of temperature, the velocity for thermal motion of the electron and the phonon increases so that the electron will interact with more phonons. However, the contribution that the increment of the velocity of the electron causes the probability of the electron in the superposition state to increase is relatively strong. And the contribution from the electron interacting with more phonons to destruct the superposition state is relatively weak. Therefore, the electron lifetime on the superposition state is prolonged and the period of oscillation increases with the increase in temperature. Figure 1a also shows that the period of oscillation slowly decreases with the cyclotron frequency parameter increasing at any temperatures (Fig. 1b is clearer). Due to the existence of the magnetic field, the ground and the first-excited state energies increase and the effect on the first-excited state energy is greater than on the ground state energy. As a result, the energy spacing between the ground and the first-excited states increases and the period of oscillation decreases [17]. The decrease of the period of oscillation will inevitably lead to the process of decoherence quicken. It is very harmful to store information in the QD qubit. Meanwhile, as is shown in Fig. 1b, at a finite temperature, the cyclotron frequency parameter does not impact the dependence of the period of oscillation on the temperature.

Fig. 2
figure 2

The period of oscillation as a function of the temperature and the electron–LO-phonon coupling constant for different cyclotron frequency parameters

Full size image

Figure 2a and b depict the period of oscillation as a function of the temperature and the electron–LO-phonon coupling constant for the confinement length \(l_{0}=0.5\) and different cyclotron frequency parameters \(\theta = {1,} {2.5}\). They are shown, when the cyclotron frequency parameter \(\theta \) is 1 or 2.5, that the period of oscillation increases with the increase in temperature. The reason is the same as discussed above. Figure 2 also depicts, when the cyclotron frequency parameter \(\theta \) is 1 or 2.5, that the period of oscillation decreases with the increase in electron–LO-phonon coupling constant. This is because the coupling constant of the electron–phonon interaction is weaker in the first-excited state than that in the ground state, then the energy spacing increases with the increasing coupling constant. The increase in the energy spacing causes a reduction in the period of oscillation [11, 12, 22]. Furthermore, Fig. 2 reveals that the period of oscillation decreases with the increase in cyclotron frequency parameter as in Fig. 1. Besides, Fig. 2 also means that the cyclotron frequency parameter does not change the relations of the period of oscillation with the temperature and the electron–LO-phonon coupling at finite temperature (can compare Fig. 2 with Fig. 2 in Refs. [11, 12]).

Fig. 3
figure 3

The period of oscillation as a function of the temperature and the confinement length for different cyclotron frequency parameters

Full size image

Figure 3a and b describe the period of oscillation as a function of the temperature and the confinement length for the electron–LO-phonon coupling constant \(\alpha =6\) and different cyclotron frequency parameters \(\theta = 1, 2.5\). As is seen in Fig.3, at different cyclotron frequency parameters, the period of oscillation increases with the confinement length increasing at any temperature. For the same reason as in Fig. 2, the period of oscillation fluctuates because of the increase in temperature and the period of oscillation decreases with the increase in cyclotron frequency parameter as in Fig. 1. From another point of view, when the temperature is high and the confinement length is strong, the period of oscillation rising is faster with the temperature increasing than when the temperature is low and the confinement length is weak. This is because the strong confinement length means the lattice thermal vibrations to become strong and it makes the numbers of phonon around electron decrease sharply. At the same time, Fig. 3 also shows that the cyclotron frequency parameter does not make the relations of the period of oscillation with the temperature and the confinement length change (can compare Fig. 3 with Fig. 4 in Refs. [11, 12]).

The period of oscillation of the probability density is one of crucial quantities to describe the life time of the qubit [14]. It has been demonstrated that the longer the period of oscillation is, the longer the life time of the qubit is [14, 16, 23]. The above results indicate that the period of oscillation increases in superposition state of electron in a QD with the increment of temperature when the magnetic field is existent. And our earlier studies show the probability density of electron and the period of oscillation are all affected by the temperature due to the existence of the parabolic linear bound potential, the electric field and the Coulomb bound potential [10–12]. A qubit cannot be independent of environment and must interact with the heat bath. As a result, the interaction generally destroys the superposition state of a qubit. However, the period of oscillation increases, in other words, the lifetime of a qubit increases. So the process of decoherence is slower. It is very useful to store information where the QD is made as its elementary unit.

4 Conclusions

Using the Pekar variational method, the influences of the temperature on the parabolic quantum dot qubit in the magnetic field have been investigated. From a numerical evaluation, we have shown that the period of oscillation decreases with the magnetic field at any temperature. Meanwhile, at lower magnetic field or higher magnetic field, our results show: (1) the period of oscillation increases with the increasing temperature; (2) the period of oscillation decreases with the increase in electron–LO-phonon coupling constant when the temperature is lower or higher; (3) the period of oscillation increases with the confinement length when the temperature is lower or higher. In addition, our work shows the advantage of the method that we used in this paper is the simplicity of the design of the computation process.