1 Introduction

Ever since the formulation of Einstein-Maxwell field equations, the relativists have been proposing different models of immensely gravitating astrophysical objects by considering the distinct nature of matter or radiation (energy-momentum tensor) present in them. Einstein-Maxwell field equations have more importance over Einstein field equations due to following rationale justifications:

  • The presence of some charge may avert the catastrophic gravitational collapse by counter balancing the gravitational attraction by the electric repulsion in addition to the pressure gradient.

  • The inclusion of charge inhibits the growth of space time curvature which has a great role to avoid singularities (Ivanov, 2002; de Felice and Fang, 1995).

  • Bonnor (1965), pointed out that a dust distribution of arbitrarily large mass and small radius can remain in equilibrium against the pull of gravity by a repulsive force produced by a small amount of charge.

  • The solutions of Einstein-Maxwell equations are useful to study the cosmic matter.

  • The charge dust models and electromagnetic mass models are providing some clue about the structure of electron (Bijalwan et al. 2011) and Lepton model (Kiess 2013).

  • Several solutions which do not satisfy some or all the conditions for well behaved nature can be renewed into well behaved nature by charging them.

Thus it is desirable to study the insinuations of Einstein-Maxwell field equations with reference to the general relativistic prediction of gravitational collapse. For this purpose charged fluid ball models are required. The external field of such ball is to be matched with Reissner-Nordstrom solution. The solutions of Einstein-Maxwell field equations successfully explain the characteristics of massive objects like neutron star, quark star or other super-dense object. Further, these stars are specified in terms of their masses and densities:

  1. (a)

    A Neutron Star has surface density ρ b =2×1014 g/cm3 (Brecher and Caporaso, 1976) or 2.7×1014 g/cm3 (Astashenok et al. 2013) and mass 1.4 M ⊙–2.9 M ⊙. However, Astashenok et al. (2013) established that f(R) models with realistic equation of state of neutron star has upper limit Mass 2.0 M ⊙ and minimal radius close to 9 km.

  2. (b)

    A Strange Quark Star has surface density ρ b =4.6888×1014 g/cm3 (Fatema and Murad 2013; Zdunik 2000) and possible maximum mass 2 M ⊙. However, Dong et al. (2013) established that due to presence of half skyrmions in the dense baryonic matter the stable Strange Quark Star can have upper mass limit 2.4 M ⊙.

2 Conditions for well behaved solution

For well behaved nature of the solution in isotropic coordinates, the following conditions should be satisfied:

  1. (i)

    The solution should be free from physical and geometrical singularities i.e. finite and positive values of central pressure, central density and non zero positive values of e ω and e ν.

  2. (ii)

    The solution should have positive and monotonically decreasing expressions for pressure and density (p and ρ) with the increase of r. The solution should have positive value of ratio of pressure-density and less than 1 (weak energy condition) and less than 1/3 (strong energy condition) throughout within the star, monotonically decreasing as well.

  3. (iii)

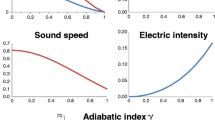
    The causality condition should be obeyed i.e. velocity of sound should be less than that of light throughout the model. In addition to the above the velocity of sound should be decreasing towards the surface i.e. \(\frac{d}{dr} ( \frac{dp}{d\rho} ) < 0\) or \(( \frac{d^{2}p}{d\rho^{2}} ) > 0\) for 0≤r≤r b i.e. the velocity of sound is increasing with the increase of density. In this context it is worth mentioning that the equation of state at ultra-high distribution, has the property that the sound speed is decreasing outwards (Canuto and Lodenquai 1975).

  4. (iv)

    \(\frac{p}{\rho} \le\frac{dp}{d\rho}\), everywhere within the ball. \(\gamma=\frac{d\log_{e}P}{d\log_{e} \rho} = \frac{\rho}{p} \frac{dp}{d\rho} \Rightarrow \frac{dp}{d\rho} = \gamma\frac{p}{\rho}\), for realistic matter γ≥1.

  5. (v)

    The red shift z should be positive, finite and monotonically decreasing in nature with the increase of r.

  6. (vi)

    Electric intensity E, such that E(r=0)=0, is taken to be monotonically increasing.

Under these conditions, we have to assume the one of the gravitational potential components and electric intensity in such a way that the field equation (8) can be integrated and solution should be well behaved.

Keeping in view this aspect, several authors obtained the parametric class of exact solutions Das et al. (2011), Pant et al. (2011a, 2011b), Pant and Negi (2012), Kiess (2012), Gupta and Maurya (2011), Pant (2011a, 2011b), Murad and Fatema (2013), Takisa and Maharaj (2013) etc. These coupled solutions are well behaved with some positive values of charge parameter K and completely describe the interior of the super-dense astrophysical object with charged matter. However, the works of Pant (2011a, 2011b), Kiess (2012), Gupta and Maurya (2011) and Murad and Fatema (2013) are well behaved with their neutral counterparts. Most of the findings are in curvature coordinates. In this paper we present a new class of solution of Einstein-Maxwell field equations which is well behaved in isotropic coordinates by motivation of Ivanov (2012) and Das et al. (2011).

3 Field equations in isotropic coordinates

We consider the static and spherically symmetric metric in isotropic co-ordinates

$$ ds^{2} = - e^{\omega} \bigl[dr^{2} + r^{2} \bigl(d\theta^{2} + \sin^{2}\theta d \phi^{2} \bigr) \bigr] + c^{2}e^{\nu} dt^{2} $$
(1)

where, ω and ν are functions of r.

Einstein-Maxwell field equations of gravitation for a non empty space-time are

$$\begin{aligned} R_{j}^{i} - \frac{1}{2}R\delta_{j}^{i} =& - \frac{8\pi G}{c^{4}}T_{j}^{i} \\ =& - \frac{8\pi G}{c^{4}}\biggl[\bigl(p + \rho c^{2} \bigr)v^{i}v_{j} - p\delta_{j}^{i} \\ &{} + \frac{1}{4\pi} \biggl( - F^{im}F_{jm} + \frac{1}{4}\delta_{j}^{i}F_{mn}F^{mn} \biggr)\biggr] \end{aligned}$$
(2)

where R ij is Ricci tensor, T ij is energy-momentum tensor, R the scalar curvature and F jm is the electromagnetic field tensor.

Where p denotes the pressure distribution, ρ the density distribution and v i the velocity vector, satisfying the relation

$$ g_{ij}v^{i}v^{j} = 1 $$
(3)

Since the field is static, therefore

$$ v^{1} = v^{2} = v^{3} = 0\quad \mbox{and}\quad v^{4} = \frac{1}{\sqrt{g_{44}}} $$
(4)

Thus we find that for the metric (1) under these conditions and for matter distributions with isotropic pressure the field equation (2) reduces to the following (Das et al. 2011)

$$\begin{aligned} &{\frac{8\pi G}{c^{4}}p = e^{ - \omega} \biggl[\frac{(\omega^{\prime})^{2}}{4} + \frac{\omega^{\prime}}{r} + \frac{\omega^{\prime}\nu^{\prime}}{2} + \frac{\nu^{\prime}}{r} \biggr] + \frac{q^{2}}{r^{4}} } \end{aligned}$$
(5)
$$\begin{aligned} &{\frac{8\pi G}{c^{4}}p = e^{ - \omega} \biggl[\frac{\omega^{\prime\prime}}{2} + \frac{\nu^{\prime\prime}}{2} + \frac{(\nu^{\prime})^{2}}{4} + \frac{\omega^{\prime}}{2r} + \frac{\nu^{\prime}}{2r} \biggr] - \frac{q^{2}}{r^{4}} } \end{aligned}$$
(6)
$$\begin{aligned} &{\frac{8\pi G}{c^{2}}\rho= - e^{ - \omega} \biggl[ \omega^{\prime\prime} + \frac{(\omega^{\prime})^{2}}{4} + \frac{2\omega^{\prime}}{r} \biggr] - \frac{q^{2}}{r^{4}} } \end{aligned}$$
(7)

where, prime (′) denotes differentiation with respect to r. From Eqs. (5) and (6) we obtain following differential equation in ω and ν.

$$\begin{aligned} &{e^{ - \omega} \biggl(\omega^{\prime\prime} + \nu^{\prime\prime} + \frac{(\nu^{\prime})^{2}}{2} - \frac{(\omega^{\prime})^{2}}{2} - \omega^{\prime} \nu^{\prime} - \frac{\omega^{\prime}}{r} - \frac{\nu^{\prime}}{r} \biggr) - 4 \frac{q^{2}}{r^{4}} } \\ &{\quad = 0 } \end{aligned}$$
(8)

Our task is to explore the solutions of Eq. (8) and obtain the fluid parameters p and ρ from Eqs. (5) and (7). To solve the above equation we consider a seed solution as Murad and Pant (2013) and the electric intensity E of the following form:

$$ \frac{E^{2}}{C} = \frac{q^{2}}{Cr^{4}} = \frac{KCr^{2} ( 1 + Cr^{2} )^{\frac{ - 8}{5}}}{B^{2}} $$
(9)

where K is a positive constant. The electric intensity is so assumed that the model is physically significant and well behaved i.e. E remains regular and positive throughout the sphere. In addition, E vanishes at the center of the star and increases towards the boundary.

4 Boundary conditions in isotropic coordinates

For exploring the boundary conditions, we use the principle that the metric coefficients g ij and their first derivatives g ij,k in interior solution (I) as well as in exterior solution (E) are continuous upto and on the boundary B. The continuity of metric coefficients g ij of I and B on the boundary is known first fundamental form. The continuity of derivatives of metric coefficients g ij of I and B on the boundary is known second fundamental form.

The exterior field of a spherically symmetric static charged fluid distribution is described by Reissner-Nordstrom metric given by

$$\begin{aligned} ds^{2} =& \biggl( 1 - \frac{2GM}{c^{2}R} + \frac{q^{2}}{R^{2}} \biggr) c^{2} dt^{2} \\ &{} - \biggl( 1 - \frac{2GM}{c^{2} R} + \frac{q^{2}}{R^{2}} \biggr)^{ - 1}dR^{2} - R^{2}d \theta^{2} \\ &{} - R^{2} \sin^{2}\theta d \phi^{2} \end{aligned}$$
(10)

where M is the mass of the ball as determined by the external observer and R is the radial coordinate of the exterior region.

Since Reissner-Nordstrom metric (10) is considered as the exterior solution, thus we shall arrive at the following conclusions by matching first and second fundamental forms:

$$\begin{aligned} &{e^{\nu_{b}}= \biggl[1 - 2\frac{GM}{c^{2}R_{b}} + \frac{q_{b}^{2}}{R_{b}^{2}} \biggr] } \end{aligned}$$
(11)
$$\begin{aligned} &{R_{b} = r_{b}\cdot e^{\frac{\omega_{b}}{2}}\quad \mbox{and}\quad q\ (\mathrm{at}\ r = r_b)= q_{b} } \end{aligned}$$
(12)
$$\begin{aligned} &{\frac{1}{2} \biggl( \omega^{\prime} + \frac{2}{r} \biggr)_{b} r_{b} = \biggl( 1 - 2\frac{GM}{c^{2}R_{b}} + \frac{q_{b}^{2}}{R_{b}^{2}} \biggr)^{1/2} } \end{aligned}$$
(13)
$$\begin{aligned} &{\frac{1}{2} \bigl( \nu^{\prime} \bigr)_{b} r_{b} = \biggl(\frac{GM}{c^{2}R_{b}} - \frac{q_{b}^{2}}{R_{b}^{2}} \biggr) \biggl( 1 - 2\frac{GM}{c^{2}R_{b}} + \frac{q_{b}^{2}}{R_{b}^{2}} \biggr)^{-1/2} } \end{aligned}$$
(14)

Equations (11) to (14) are four conditions, known as boundary conditions in isotropic coordinates. Moreover, (12) and (14) are equivalent to zero pressure of the interior solution on the boundary.

5 A new class of solution

Equation (8) is solved by assuming the seed solution as a particular member of Murad and Pant (2013) and the charge q in such a manner that the solution can be obtained and physically viable. Thus we have,

$$\begin{aligned} \begin{aligned} &e^{\omega/2} = B \bigl( 1 + Cr^{2} \bigr)^{-1/5},\qquad x = Cr^{2},\\ &y = \frac{d\nu}{dx}\quad \mathrm{and}\quad q^{2} = \frac{K}{B^{2}C}x^{3}(1 + x)^{- 8/5} \end{aligned} \end{aligned}$$
(15)

On substituting the above in Eq. (8), we get the following Riccati differential equation in y,

$$ \frac{dy}{dx} + \frac{2}{5}\frac{1}{(1 + x)}y + \frac{1}{2} y^{2} = - \frac{8}{25}\frac{1}{ ( 1 + x )^{2}} + \frac{K}{(1 + x)^{2}} $$
(16)

which yields the following solution,

$$ e^{\nu/2} = \frac{ \{ 1 + A ( 1 + Cr^{2} )^{\frac{2S}{10}} \} ( 1 + Cr^{2} )^{\frac{3 - S}{10}}}{B^{2}} $$
(17)

where A, B, C and K are arbitrary constants and

$$ S = \sqrt{50K - 7} $$
(18)

S is imaginary for K<0.14.

The expressions for density and pressure are given by

$$\begin{aligned} &{\frac{8 \pi G \rho}{c^{2}} = \frac{ C}{25B^{2} f^{16}} \bigl( 60 + 16C r^{2} - 25KCr^{2} \bigr) } \end{aligned}$$
(19)
$$\begin{aligned} &{ \begin{aligned}[b] \frac{8\pi Gp}{c^{4}} &= \frac{C}{25B^{2}f^{16}\{ 1 + Af^{2S}\}} \bigl[ Cr^{2}\bigl[Af^{2S}(25K + 2 + 6S) \\ &\quad{} + (25K + 2 - 6S)\bigr] \\ &\quad{} + 10\bigl[Af^{2S}(1 + S) + (1 - S)\bigr] \bigr] \end{aligned} } \end{aligned}$$
(20)

where

$$ f = \bigl(1 + Cr^{2} \bigr)^{1/10}. $$
(21)

6 Properties of the new solution

The central values of pressure and density are given by

$$\begin{aligned} \biggl( \frac{8 \pi G p}{c^{4}} \biggr)_{r = 0} =& \frac{2C}{5B^{2} (1 + A)} \bigl[ (1 + A) + S(A - 1) \bigr] \end{aligned}$$
(22)
$$\begin{aligned} \biggl( \frac{8 \pi G \rho}{c^{2}} \biggr)_{r = 0} =& \frac{12 C}{5B^{2}} \end{aligned}$$
(23)

The central values of pressure and density will be non zero positive definite, if the following conditions will be satisfied.

$$ A > (S-1)/(S+1),\qquad C > 0. $$
(24)

In view of (19) and (20) the ratio of pressure-density is given by

$$\begin{aligned} \frac{P}{\rho c^{2}} =& \frac{Cr^{2}[f^{2S}A(25K + 2 + 6S) + (25K + 2 - 6S)]}{(1 + Af^{2S})[60 + 16Cr^{2} - 25KCr^{2}]} \\ &{}+\frac{ 10[f^{2S}A(1 + S) + (1 - S)]}{(1 + Af^{2S})[60 + 16Cr^{2} - 25KCr^{2}]} \end{aligned}$$
(25)

Subjecting the condition that positive value of ratio of pressure-density and less than 1 at the centre i.e. \(\frac{p_{0}}{\rho_{0} c^{2}} \le1\) which leads to the following inequality,

$$ \biggl\{ \frac{P}{\rho c^{2}} \biggr\} _{r = 0} = \frac{(1 + A) + SA - S}{6(1 + A)} = \frac{1}{6} - \frac{S(1 - A)}{6(1 + A)} $$
(26)

All the values of A which satisfy Eq. (24), will also lead to the condition\(\frac{p_{0}}{\rho_{0} c^{2}} \le1\).

Differentiating (20) with respect to r, we get

$$\begin{aligned} \frac{8\pi G}{c^{4}}\frac{dp}{dr} =& \frac{C^{2}r}{125B^{2} \{ 1 + Af^{2S} \}^{2}f^{26}} \bigl[ - 6Cr^{2} \bigl[A^{2}f^{4S} \\ &\times{}\{ 25K + 2 + 6S\} +\{ 25K + 2 - 6S\} \\ &{} + 2Af^{2S}\bigl(25K + 2 - 2S^{2}\bigr)\bigr] \\ &{}+ 10\bigl[A^{2}f^{4S}\{ 25K - 14 - 10S\} \\ &{} + (25K - 14 + 10S) \\ &{} + 2Af^{2S}\bigl(25K - 14 + 2S^{2}\bigr)\bigr]\bigr] \end{aligned}$$
(27)

Thus it is found that extrema of p occur at the centre i.e.

$$ p' = 0 \quad \Rightarrow\quad r = 0\quad\mbox{and}\quad \frac{8 \pi G}{c^{4}} \bigl( p'' \bigr)_{r = 0} = - ve $$
(28)

Thus the expression of right hand side of Eq. (28) is negative for all values of A satisfying condition (24), showing thereby that the pressure (p) is maximum at the centre and monotonically decreasing.

Now differentiating Eq. (19) with respect to r we get

$$\begin{aligned} \frac{8\pi G}{c^{2}}\frac{d\rho}{dr} =& \frac{C^{2}r}{125B^{2}f^{26}} \bigl[ - 800 - 250K - 96Cr^{2} \\ &{} + 150KCr^{2} \bigr] \end{aligned}$$
(29)

Thus the extrema of ρ occur at the centre if

$$\begin{aligned} &{\rho' = 0 \quad \Rightarrow\quad r = 0 } \\ &{\frac{8 \pi G}{c^{2}} \bigl( \rho'' \bigr)_{r = 0} = \frac{( - 800 - 250K)C^{2}}{ 125B^{2}} } \end{aligned}$$
(30)

Thus, the expression of right hand side of (30) is negative showing thereby that the density ρ is maximum at the centre and monotonically decreasing.

The square of adiabatic sound speed at the centre, \(\frac{1}{c^{2}} ( \frac{dp}{d\rho} )_{r = 0}\), is given by

$$\begin{aligned} &{\frac{1}{c^{2}} \biggl( \frac{dp}{d\rho} \biggr)_{r = 0} }\\ &{\quad = \frac{ ( 25K - 14)(1 + A)^{2} + 10S + 4AS^{2} - 10SA^{2} }{( - 80 - 25K)(1 + A)^{2}} } \\ &{\quad <1 \quad \mbox{and}\ \mathrm{positive}} \end{aligned}$$
(31)

The causality condition is obeyed at the centre for all values of constants satisfying condition (24).

Due to cumbersome expressions of (25) and (31), the trend of pressure-density ratio and adiabatic sound speed is studied analytically after applying the boundary conditions.

Applying the boundary conditions from (11) to (14), we get the values of the arbitrary constants in terms of Schwarzschild parameters \(u = \frac{GM}{c^{2}R_{b}}\) and radius of the star R b .

$$\begin{aligned} &{C = \frac{5(1 - d)}{(5d - 3) r_{b}^{2}} > 0\quad \mbox{for}\ u \le\frac{8}{25} + \frac{q_{b}^{2}}{2R_{b}^{2}} } \end{aligned}$$
(32)
$$\begin{aligned} &{A=\frac{5(u-\frac{q^2_b}{R^2_b}) (1+Cr^2_b)^{\frac{(3-S)}{10}}- (3-S)dCr^2_b (1+Cr^2_b)^{\frac{-7-S}{10}}}{(3+S)dCr^2_b(1+Cr^2_b)^{\frac{-7+S}{10}} -5(u-\frac{q^2_b}{R^2_b})(1+Cr^2_b)^{\frac{3+S}{10}}} }\\ \end{aligned}$$
(33)
$$\begin{aligned} &{B = \sqrt{\frac{(1 + Cr_{b}^{2})^{\frac{3 - S}{10}} + A(1 + Cr_{b}^{2})^{\frac{3 + S}{10}}}{d}} } \end{aligned}$$
(34)

Where we define a new parameter called as Reissner-Nordstrom parameter ‘d’ given by

$$ d = \sqrt{1 - 2u + \frac{q_{b}^{2}}{R_{b}^{2}}} $$
(35)

Whose value lies between 0.6<d<1 for \(Cr_{b}^{2} > 0\).

Surface density is given by

$$ \frac{8 \pi G}{c^{2}} \rho_{b} R_{b}^{2} = \frac{Cr_{b}^{2}[60 + 16Cr_{b}^{2} - 25KCr_{b}^{2}]}{25(1 + Cr_{b}^{2})^{2}} $$
(36)

Central red-shift is given by

$$ Z_{0} = \biggl[ \frac{B^{2}}{1 + A} - 1 \biggr] $$
(37)

The surface red shift is given by

$$ Z_{b} = \bigl[e^{ -\frac{\nu_{b}}{2}} - 1 \bigr] $$
(38)

7 Discussion and conclusion

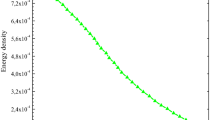
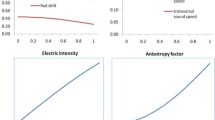
In view of Tables 1 and 2 and Figs. 1 and 2 it has been observed that all the physical parameters (p, ρ, \(\frac{p}{\rho c^{2}}\), \(\frac{dp}{d\rho}\), z, γ and E) are positive at the centre and within the limit of realistic equation of state and the conditions for well behaved nature are satisfied. Our solution is well behaved for the all values of K satisfying the inequalities 0.141≤K≤0.15999. However, corresponding to any value of K<0.141, there exist no value of u for which the nature of adiabatic sound speed is monotonically decreasing from centre to pressure free interface and for K>0.15999, the pressure is negative.

Fig. 1
figure 1

The variation of p, ρ, \(\frac{p}{\rho c^{2}}\), Z, \(\frac{1}{c^{2}} ( \frac{dp}{d\rho} )\), γ, E etc. from centre to surface for K=0.15 is shown in the following graphs

Full size image
Fig. 2
figure 2

The variation of p, ρ, \(\frac{p}{\rho c^{2}}\), Z, \(\frac{1}{c^{2}}(\frac{dp}{d\rho})\), γ, E etc. from centre to surface for K=0.159999 is shown in the following graphs

Full size image
Table 1 The march of pressure, density, pressure-density ratio, square of adiabatic speed of sound, γ, red shift and electric field intensity within the ball corresponding to K=0.15 and u=0.238
Full size table
Table 2 The march of pressure, density, pressure-density ratio, square of adiabatic speed of sound, γ, red shift and electric field intensity within the ball corresponding to K max =0.159999 for which u max =0.259
Full size table

In Table 3, we present the neutron star modeling based on the particular solution; corresponding to K=0.15, u=0.238 and by assuming the surface density ρ b =2.7×1014 g/cm3 the maximum mass of neutron star M=1.966 M ⊙ and radius R=12.23 km and by assuming the surface density ρ b =2×1014 g/cm3 the resulting well behaved solution has a maximum mass of neutron M=2.284 M ⊙ and radius R=14.21 km.

Table 3 The variation of maximum mass of neutron star and corresponding radius R b with u for K=0.15
Full size table

In Table 4, we present a quark star modeling based on the particular solution discussed above by assuming surface density; ρ b =4.6888×1014 g/cm3. Corresponding to K max =0.159999 for which, u max =0.259, the resulting well behaved solution has a maximum mass M=1.618 M ⊙ and radius R=9.263 km. The robustness of our result is that it matches with the recent discoveries.

Table 4 By assuming the surface density ρ b =4.6888×1014 g/cm3, the variation of maximum mass of Quark star, corresponding radius R b , surface red shift and the surface electric field with u for K=0.159999
Full size table