Abstract
We prove some results on the existence and uniqueness of solutions to a recently derived nonlinear model for the ocean flow in arctic gyres. We derive an equivalent formulation of the problem in the form of an integral equation on a semi-infinite interval and then use fixed point techniques. We also obtain some stability result.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
The combined forces of gravity and Coriolis (due to Earth’s rotation), triggered by the wind stress, drive circulating ocean currents, called gyres, in which the ocean flow adjusts to these two major forces acting on them so that those forces balance one another—see the discussion in [10, 11]. Gyres are constrained by land masses, with dimensions nearly those of ocean basins, and, since the Coriolis effect deflects winds to the right in the Northern Hemisphere and to the left in the Southern Hemisphere, this results in the deflection of gyres to the right in the Northern Hemisphere (clockwise rotation) and to the left in the Southern Hemisphere (counterclockwise rotation). These geophysical flows are dominantly horizontal, with horizontal velocities typically of the order of 0.01 m/s [6] and about a factor \(10^4\) larger than the vertical velocities [14]. The importance of these slow flows, which are a dominant factor in the circulation of ocean water around the entire planet, is due to their extent, of the order of \(10^6\) km\(^2\) in the case of the North Pacific gyre. There are gyres in all of the world’s seven major ocean regions (North Atlantic, South Atlantic, Indian, North Pacific, South Pacific, Arctic, Antarctic), each presenting some specific flow pattern, mostly dependent on its location north and south of the equator—gyres do not occur at the Equator [1]. Indeed, the Coriolis effect is not present at the Equator due to the vanishing of the meridional component of the Coriolis force, which implies that the Equator works as a fictitious natural boundary, facilitating azimuthal flow propagation (for details we refer to the discussions in [3, 4]).
Recently, a model of the general motion of a gyre in spherical coordinates as a shallow-water flow on a rotating sphere was obtained (see the discussion in [6]), in which the gyre motion is described in terms of a stream function by the solution of an elliptic boundary value problem. In particular, this applies to the gyre problem in the northern part of the Arctic Ocean (above 84\(^\circ \)N), where permanent ice, with thickness exceeding 2 m, floats on the ocean, slowly rotating clockwise, roughly centered on the North Pole, cf. the discussion in [16]. By means of the stereographic projection, the formulation of the model in spherical coordinates can be transformed into a planar elliptic boundary value problem (see [6]) which, neglecting azimuthal variation, can be transformed into a second-order differential equation on a semi-infinite interval, constrained by some asymptotic conditions (see [2]). The linear setting was recently analyzed in detail in [2], where also some explicit solutions were obtained. The aim of the present paper is to investigate the problem of an arctic gyre motion which depends on the polar angle in the nonlinear setting. The approach consists in deriving a suitable integral formulation for the problem and using fixed point techniques to prove the existence and uniqueness of solutions. We also derive some stability results. When particularized to the linear case, the results that we present recover those obtained in [2], so that our method provides an extension of the linear theory developed in [2]. We point out that the pursued analysis applies only to the polar region in the Northern hemisphere: while the North Pole is located in the middle of the Arctic Ocean, the South Pole is terrestrial, being located in Antarctica, a continent that is completely encircled by the eastward moving Antarctic Circumpolar Current (see the discussion in [5]). Note also that while surface waves of various types interact with the Antarctic Circumpolar Current (see [7]) and some of these appear to be important for the climate (for example, the Antarctic Circumpolar Wave, which moves eastward with the prevailing currents—see[12, 15]), the sea ice at the surface of the Arctic Ocean presents only very small elastic deformations (see [13]) which, while difficult to analyze and compute, are negligible at the geophysical scales appropriate to study the gyre motion.
2 Preliminary considerations
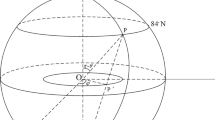
Let us introduce the model for gyres derived in [6]. Considering spherical coordinates, with \(\theta \in [0,\pi )\) the polar angle (with \(\theta =0\) corresponding to the South Pole, so that \(\theta -\pi /2\) is the conventional angle of latitude) and \(\varphi \in [0,2 \pi \)) the azimuthal angle i.e. the angle of longitude, the horizontal flow on the spherical Earth, corresponding to a gyre, has azimuthal and polar velocity components given by
respectively, where \(\psi (\theta ,\varphi )\) represents the stream function. Writing
where \(\varPsi \) is associated with the vorticity of the underlying motion of the ocean (relative to the Earth’s surface and not driven by the rotation of the Earth), the governing equation is
The vorticity of the flow comprises two distinct components: the spin vorticity, \(2\omega \,\cos \theta \), due to the rotation of the Earth, and the oceanic vorticity, \(F(\varPsi -\omega \,\cos \theta )\), due to the motion of the ocean. The latter is coupled to the Earth’s rotation, the total vorticity of the ocean flow being the sum of these two components. While the spin vorticity is completely prescribed, that associated with the movement of the ocean may be chosen to represent a particular gyre. In particular, the flow field is irrotational if the oceanic vorticity vanishes, that is, \(F \equiv 0\).
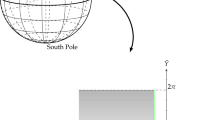
In terms of the stereographic projection (we refer to the discussions in [9] for the physical motivation and a historical background to the stereographic projections) from the South Pole to the equatorial plane, given by
where \((r,\phi )\) are the polar coordinates in the Earth’s equatorial plane, the ocean flow in the gyre is modeled by the semilinear elliptic equation
in terms of the unknown \(\psi (x,y)\). For specified \(\omega \) and F, one has to solve Eq. (2) in a given planar region \({\mathcal O}\), with Dirichlet boundary data
where \(u_0\) is the value that the stream function attains on boundary \(\partial {\mathcal O}\) of \({\mathcal O}\), which corresponds therefore to the streamline \(\psi =u_0\). The planar region \({\mathcal O}\) corresponds, in view of the stereographic projection (1), to the ocean region in which the gyre is located, with the arctic setting corresponding to the planar region \({\mathcal O}=\{r:\ 0 \le r < r_0\}\), for a suitable \(r_0>0\). Since the physically relevant values of \(\theta \) are in the interval \([14\pi /15,\pi ]\), with \(\theta =\pi \) corresponding to the North Pole, we see that \(\hbox {cotan}(\theta /2) < 1/e^2\) and therefore \(r_0 \le 1/e^2\). An arctic gyre in which the flow velocity presents no azimuthal variations is provided by radially symmetric solutions \(\psi =\psi (r)\) of the problem (2)–(3). Setting \(t_0=-\ln (r_0) \ge 2\), by changing variables according to \(r=e^{-t}\) and
we transform (2)–(3) to the second-order ordinary differential equation
with the constraint
expressing the fact that the circle of latitude \(r=r_0\) is a streamline. Indeed, note that (1) yields
as well as the fact that in the gyre region the azimuthal velocity component vanishes. Physically relevant solutions of the problem (4)–(5) are therefore obtained if the asymptotic conditions
hold for some constant \(\psi _0\), which is the value of the stream function \(\psi \) at the North Pole. The second asymptotic condition in (7) expresses the fact that the flow is stagnant at the North Pole, which is the gyre’s center.
3 Main result
The considerations made in [2, 6] provide some explicit solutions to the Eq. (4) with the asymptotic behavior specified in (7) for linear functions \(F(u)=au+b\), where \(a,\, b \in {\mathbb R}\) are constants. The aim of the present paper is to investigate the case of nonlinear vorticity functions F.
Note that if u(t) is a solution to (4), satisfying (5), then integration of (4) on \([t,\infty )\) yields
which, integrated on \([t,\infty )\), leads to
in view of the first condition in (7). The integral equation (9) is a convenient way to compress (4) and (7). Indeed, assume that \(u: [t_0,\infty ) \rightarrow {\mathbb R}\) is a continuous function satisfying (9) and \(\lim \limits _{t \rightarrow \infty } u(t)=\psi _0\). Then, differentiation of (9) yields (8) and the second condition in (7) follows since, by l’Hospital’s rule,
due to the fact that u is bounded on \( [t_0,\infty )\). Furthermore, differentiation of (8) yields (4). As for (5), this is satisfied provided that we have the freedom to assign the value of \(u_0\) and this can be done since the specific value of \(u_0\) has no bearing on the underlying physics.
We now prove an existence and uniqueness result for the solution to the integral equation (9).
Theorem 1
Assume that \(F: {\mathbb R} \rightarrow {\mathbb R}\) is Lipschitz continuous: there exists a constant \(M>0\) such that
Then, for every \(\psi _0 \in {\mathbb R}\), Eq. (9) has a unique continuous solution \(u: [t_0,\infty ) \rightarrow {\mathbb R}\) satisfying \(\lim \limits _{t \rightarrow \infty } u(t)=\psi _0\).
Proof
Choose \(T_0 \ge t_0\) such that \(\cosh (T_0)>M\). On the Banach space X of all continuous and bounded functions \(u: [T_0,\infty ) \rightarrow {\mathbb R}\), endowed with the supremum norm \(\Vert u \Vert =\sup \limits _{t \ge T_0} \{ |u(t)|\}\), consider the operator
Note that the inequality
confirms that \({\mathcal F}: X \rightarrow X\). For any \(u,\, v \in X\), we have that
since \(\sinh (s) \ge s\) for \(s \ge 0\). Consequently, the map \({\mathcal F}\) is a contraction on X. By the contraction principle, \({\mathcal F}\) will have a unique fixed point (see [8]). This fixed point is the unique solution to (9) on \([T_0,\infty )\). If \(T_0=t_0\), we are done, while if \(T_0>t_0\), then we note that \(u(T_0)\) and \(u'(T_0)\) are determined. Taking into account that (4) follows from (9), the uniqueness of solutions for (4) if F is Lipschitz ensures that we have a unique backward continuation of u(t) to \([t_0,\infty )\); note that the linear growth rate of F (see Remark 2) prevents blow-up in finite time, so that we may extend the solution from \([T_0,\infty )\) to the maximal possible time-interval, which is \([t_0,\infty )\).\(\Box \)
Remark 1
Examples of nonlinear functions satisfying the hypotheses of Theorem 1 are
for some real constants a and b with \(a \ne 0\).
Remark 2
Choosing \(v=0\) in the Lipschitz condition in Theorem 1 yields
so that all such functions are sublinear.
While the exact values of \(u_0\) and \(\psi _0\) are not physically relevant, they cannot be assigned arbitrarily: the fact that the problem (4)–(5)–(7) appears to be overdetermined hints at an interdependence between \(u_0\) and \(\psi _0\). To elucidate this, we show that the solution provided by Theorem 1 is stable (in the supremum norm) with respect to variations of \(\psi _0\). In particular, this shows that \(u_0\) depends continuously on \(\psi _0\).
Theorem 2
The solution obtained in Theorem 1 is stable (in the supremum norm) with respect to variations of \(\psi _0\).
Proof
Let u(t) and \(\tilde{u}(t)\) be two solutions of Eq. (9) with \(\lim \limits _{t \rightarrow \infty } u(t)=\psi _0\) and \(\lim \limits _{t \rightarrow \infty } \tilde{u}(t)=\tilde{\psi }_0\), respectively. Then, choosing \(T_0 \ge t_0\) such that
and defining \(\Vert u \Vert =\sup \limits _{t \ge T_0} \{ |u(t)|\}\), we have
since
so that
Using now (8) and (12), we obtain that
and therefore
Since clearly \(| u(T_0) -\tilde{u} (T_0)|\le \Vert u -\tilde{u} \Vert \), the above inequality in combination with (12) yields
If \({T_{0}}={t_{0}}\), then the proof is complete. If \({T_{0}} >{t_{0}}\), we see that \(u(t_0)\) is the value obtained by evaluating the backward flow of the differential equation (4) at \(t=t_0\), starting with data \(u(T_0)\) and \(u'(T_0)\) at \(t=T_0\). In other words, if u(t) solves (4), we set
to see that
with initial data
We now use the fact that the general solution of the linear second-order differential equation
has the form
where \(A,\, B \in {\mathbb R}\) are constants (see [2]), to infer that the general solution of the linear second-order differential equation
has the form
It is convenient to express this fact by noticing that
where
is a fundamental matrix for the linear system
which is equivalent to (16). Writing (14)–(15) in the form
with initial data
the variation of constants formula yields
for \(0 \le s \le T_0-t_0\). Therefore,
for \(0 \le s \le T_0-t_0\). Setting \(\tilde{v}(s)=\tilde{u}(T_0-s)\) for \(0 \le s \le T_0-t_0\), we deduce that \(\tilde{v}(s)\) satisfies an integral equation analogous to (17), so that
for \(0 \le s \le T_0-t_0\). Therefore,
for \(0 \le s \le T_0-t_0\), where
Taking (13) into account, we deduce that
for \(0 \le s \le T_0-t_0\). Gronwall’s inequality (see [8]) now yields
for \(0 \le s \le T_0-t_0\). Evaluating the above relation at \(s=T_0-t\), we get
for \(t_0 \le t \le T_0\). In combination with (12), the above inequality proves the claimed stable dependence (in the supremum norm) of the solution determined in Theorem 1, with respect to variations of \(\psi _0\).\(\Box \)
References
Apel, J.R.: Principles of Ocean Physics. Academic Press, London (1987)
Chu, J.: On a differential equation arising in geophysics. Monatsh. Math. (2017). doi:10.1007/s00605-017-1087-1
Constantin, A., Johnson, R.S.: The dynamics of waves interacting with the Equatorial Undercurrent. Geophys. Astrophys. Fluid Dyn. 109, 311–358 (2015)
Constantin, A., Johnson, R.S.: An exact, steady, purely azimuthal equatorial flow with a free surface. J. Phys. Oceanogr. 46, 1935–1945 (2016)
Constantin, A., Johnson, R.S.: An exact, steady, purely azimuthal flow as a model for the Antarctic circumpolar current. J. Phys. Oceanogr. 46, 3585–3594 (2016)
Constantin, A., Johnson, R.S.: Large gyres as a shallow-water asymptotic solution of Euler’s equation in spherical coordinates. Proc. R. Soc. Lond. A 473, 20170063 (2017)
Constantin, A., Monismith, S.G.: Gerstner waves in the presence of mean currents and rotation. J. Fluid Mech. 820, 511–528 (2017)
Coppel, W.A.: Stability and Asymptotic Behavior of Differential Equations. D. C. Heath and Co., Boston (1965)
Daners, D.: The Mercator and stereographic projections, and many in between. Am. Math. Mon. 119, 199–210 (2012)
Gabler, R.E., Petersen, J.F., Trapasso, L.M.: Essentials of Physical Geography. Thomson Brooks/Cole, Belmont (2007)
Garrison, T.: Essentials of Oceanography. National Geographic Society, Stamford (2014)
Jacobs, G.A., Mitchell, J.L.: Ocean circulation variations associated with the Antarctic Circumpolar Wave. Geophys. Res. Lett. 23, 2947–2950 (1996)
Squire, V.A.: Of ocean waves and sea-ice revisited. Cold Reg. Sci. Technol. 49, 110–133 (2007)
Viudez, A., Dritschel, D.G.: Vertical velocity in mesoscale geophysical flows. J. Fluid Mech. 483, 199–223 (2015)
White, W.B., Peterson, R.G.: An Antarctic circumpolar wave in surface pressure, temperature and sea-ice extent. Nature 380, 699–702 (1996)
Wilkinson, T., Wiken, E., Bezaury-Creel, J., Hourigan, T., Agardy, T., Herrmann, H., Janishevski, L., Madden, C., Morgan, L., Padilla, M.: Marine Ecoregions of North America, p. 200. Commission for Environmental Cooperation, Montreal (2009)
Acknowledgements
This work was supported by the National Natural Science Foundation of China (Grant No. 11671118).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Chu, J. On a nonlinear model for arctic gyres. Annali di Matematica 197, 651–659 (2018). https://doi.org/10.1007/s10231-017-0696-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10231-017-0696-6