Introduction

Direct observation of the processes governing explosive volcanic eruptions is difficult, if not impossible. Pyroclasts represent quenched fragments of magma and often preserve abundant bubbles (in melt) in the form of vesicles (in rock). These vesicles provide an indirect record of magma ascent conditions. A significant body of work has been aimed at using bubble number density and size distributions to constrain rates of eruptive magma ascent and the timing of gas exsolution (e.g., Mangan et al. 1993; Cashman and Mangan 1994; Polacci et al. 2003; Burgisser and Gardner 2005; Proussevitch et al. 2007). Vesicle shape is another manifestation of magma ascent conditions, in particular bubble growth, coalescence, and shearing (e.g., Klug and Cashman 1996; Mangan and Cashmann 1996; Polacci et al. 2003; Rust et al. 2003; Okumura et al. 2008; Wright and Weinberg 2009), and can therefore provide a valuable complement to conventional studies of pyroclast textures.

Here, we study vesicle shapes in pyroclasts from fall deposits of seven explosive eruptions, comprising six different eruptive styles, including the enigmatic Plinian eruptions of basaltic magma, for which the cause for high explosive intensity has been controversial (e.g., Walker et al. 1984; Coltelli et al. 1998; Houghton et al. 2004; Sable et al. 2006, 2009; Costantini et al. 2009; Goepfert and Gardner 2010). We are primarily interested in the relationship between bubble growth, as a consequence of magma decompression, and vesicle shape. We therefore restrict our analysis to samples with vesicles that are not significantly affected by shear deformation, that is samples where the median elongation is small (\(\le 0.35\), e.g., Rust and Manga 2002a; Rust et al. 2003). We interpret vesicle shapes within the context of recent work on the relationship between the “structure” of expanding bubbly fluids, that is bubble shapes, and capillary number (Koerner 2008). By estimating the capillary number, Ca, (see also Table 1 for notations) through bubble growth modeling, we relate the overpressure of bubbles, which is proportional to the energy needed to initiate and sustain magma fragmentation (Mueller et al. 2008), to vesicle shapes in the analyzed pyroclasts.

Table 1 Symbols used in this paper and their units with description
Full size table

Eruptions studied

In order to relate vesicle shapes and capillary number, we analyzed pyroclasts from fall deposits of well-constrained eruptions over a wide range of eruption styles and intensities (Table 2), as well as compositions (Table 3). They are (1) the basaltic 1959 high-fountaining eruption at Kilauea Iki, Hawai‘i (Stovall et al. 2011), (2) a basaltic Strombolian eruption at Stromboli, Italy in 2002 (Lautze and Houghton 2007), (3) a typical Vulcanian eruption at Soufrière Hills, Montserrat in 1997 (Druitt et al. 2002), (4) the dacitic–rhyolitic 1912 Plinian eruption of Novarupta, Alaska (Adams et al. 2006), (5) the 1.8 ka ultraplinian eruption in Taupo, New Zealand (Houghton et al. 2010), and two basaltic Plinian eruptions (6) Mt. Etna, Italy in 122 BC (Sable et al. 2006) and (7) Mt. Tarawera, New Zealand in 1886 (Sable et al. 2009). For each eruption, we analyze vesicle shapes of a modal sample (Table 4) that is representative of the given deposit in terms of composition, vesicle size distribution, vesicle number density, and qualitative appearance of vesicle shapes.

Table 2 Eruption parameters and microtextural characteristics of pyroclasts from the eruptions studied
Full size table
Table 3 Oxide concentrations in weight percent. Oxide concentrations of major element compositions are reported on a volatile-free basis
Full size table
Table 4 Summary of vesicle shape analysis
Full size table

Quantifying vesicle shapes

Shape parameter, \(\Omega \)

Our focus in this study is to quantify the shapes of vesicles, with an emphasis on distinguishing shapes produced by the coalescence of two or more vesicles. To identify a suitable metric, we compared the values of shape parameters for various hypothetical vesicle shapes. As illustrated in Fig. 1a, elongation, defined as \((\lambda _{\mathrm {l}}-\lambda _{\mathrm {s}})/(\lambda _{\mathrm {l}}+\lambda _{\mathrm {s}})\) (e.g., Rust and Manga 2002a; Rust et al. 2003), and circularity, the ratio of cross-sectional area and surface area (e.g., Russ and Dehoff 2000), are significantly affected by bubbles that are elongated due to shear deformation. Here, \(\lambda _{\mathrm {l}}\) and \(\lambda _{\mathrm {s}}\) are the semi-long and semi-short axes of the best-fit ellipse, respectively. In contrast, the shape parameter,

$$ \Omega = \frac{A}{\pi \lambda_{\mathrm{l}} \lambda_{\mathrm{s}}} , $$
(1)

defined as “regularity” by Shea et al. (2010), is not affected by vesicle elongation but is sensitive to vesicles with complex margins. Figure 1a illustrates that only vesicles with complex margins have values of \(\Omega <1\). We therefore use \(\Omega \) as a metric to differentiate vesicles that preserve shapes due to unrelaxed coalescence events from more spheroidal vesicles. Because \(\Omega \) is a rather sensitive measure of vesicle shape, small deviation from \(\Omega =1\) can represent significant complexity in vesicle shapes. It is therefore convenient to show \((1-\Omega )\) together with \(\Omega \) (Fig. 1b).

Fig. 1
figure 1

a Comparison of different shape factors—circularity, elongation, and \(\Omega \) (see text for formulations) for six hypothetical vesicle shapes. From left to right, the vesicles represent a spherical vesicle, an elongated (sheared) vesicle with an aspect ratio of approximately 3:1, an elongated (sheared) vesicle with an aspect ratio of approximately 8:1, a vesicle formed by the coalescence of two bubbles, a vesicle formed by the coalescence of three bubbles, and a complexly shaped vesicle formed due to the coalescence of many bubbles. Among the different shape factors, \(\Omega \) is not affected by vesicle elongation and represents a robust metric for vesicles with complexly shaped margins, that is convolutely shaped vesicles. b Illustrating \((1-\Omega )\) for hypothetical vesicle shapes. Blue curve is for vesicles that are identical to the red ones, with somewhat more gradual changes in curvature of the vesicle perimeter. As illustrated, the measure, \((1- \Omega )\), increases with increasing complexity of vesicle shapes

Full size image

For each of the considered eruptions, \(\Omega \) was calculated for vesicles with cross-sectional areas that are equivalent to circular areas with diameters ranging from 10 to 100 \(\mu \)m. This size range includes median vesicle size range observed in pyroclasts for all of the eruptions studied. Vesicle shapes are analyzed using a fixed magnification to ensure the same pixel resolution throughout. Thus, vesicle shapes were analyzed on all the vesicles imaged at a scale of 1 \(\mu \)m/px (\(\times {100}\) magnification) in backscattered electron images from a given thin section, previously used for the study of vesicle size distributions (Tables 2 and 4; Adams et al. 2006; Sable et al. 2006, 2009; Lautze and Houghton 2007; Giachetti et al. 2010; Houghton et al. 2010; Stovall et al. 2011). In these studies, the original, grayscale, SEM images were transformed into binary images using Adobe® Photoshop and Scion Image (Scion Corporation, USA) or ImageJ (Schneider et al. 2012) software. In these binary images, vesicles are black and solid phases are white. Manual editing of the images was required to rebuild vesicle walls that were thought to have broken during thin sample preparation and to remove flaws, such as grinding compound. In addition, thin bubble walls were often lost during image acquisition or during the conversion from grayscale to binary and also had to be redrawn. The original images had been positioned to avoid areas with large crystals. In this study, we analyzed these binary images. The reader is referred to the corresponding papers for more details concerning the acquisition and processing of the SEM images used herein.

Measured vesicle shapes

The cumulative frequency distribution of \(\Omega \) and \((1-\Omega )\) for the seven eruptions studied is shown in Fig. 2a, b, respectively. Although the minimum value of \(\Omega \) measured for all the eruptions is 0.27, we show from \(\Omega = 0.5\) as values smaller than this have a very low frequency of occurrence. Figure 2a shows that vesicles in pyroclasts from Strombolian and Hawaiian fire-fountain eruptions have distinctly higher values of \(\Omega \) than vesicles in pyroclasts from the other eruptions. This distinction is more pronounced in Fig. 2b. Under “Results and discussion” we will provide an interpretation of these results.

Fig. 2
figure 2

Cumulative frequency distributions of a \(\Omega \) and b (\(1-\Omega \)) for the seven eruptions studied. \(\Omega \) is calculated for vesicles with an equivalent diameter between 10 and 100 \(\mu \)m for the samples listed in Table 4. The values of \(\Omega \) are shown for \(\Omega \ge 0.5\) as the frequency of occurrence of smaller values of \(\Omega \) is close to 0. Measured minimum value of \(\Omega \) is 0.27 for all the eruptions. Vesicles in Hawaiian and Strombolian pyroclasts have distinctly higher values of \(\Omega \) than all other eruptions. This distinction is more pronounced in b

Full size image

Capillary number, Ca

The capillary number represents the balance of viscous and capillary stresses. The latter tends to restore deformed bubbles toward spherical shape, whereas the former is the consequence of fluid motions that deform bubbles (Stone 1994). The capillary number can be expressed as the product of dynamic viscosity and flow velocity, divided by surface tension. This requires a judicious choice of flow velocity and is typically associated with an externally imposed shear flow (Taylor 1932, 1934; Rallison 1984; Stone 1994; Rust and Manga 2002a, b; Rust et al. 2003). An alternate possibility is the “expansion velocity,” \(v_{\mathrm {e}}\), of a bubbly fluid (Koerner 2008), defined as the velocity at which the surface of a bubbly fluid or foam is expanding upon bubble growth (Namiki and Manga 2006; Koerner 2008). For an unconfined fluid with bubbles of average size R, at a volume fraction \(\phi \), the expansion velocity is

$$ v_{\mathrm{e}} = \phi^{2/3} \frac{dR}{dt} = \phi^{2/3} v_{R}, $$
(2)

where t denotes time. The balance between viscous and capillary stresses, expressed in terms of \(v_{\mathrm {e}}\), thus gives

$$ \textnormal{Ca} = \frac{\eta v_{\mathrm{e}}}{ \sigma}, $$
(3)

where \(\eta \) is the viscosity of the fluid phase and \(\sigma \) is surface tension.

Koerner (2008) provides an analysis of the evolving structure of expanding bubbly fluids in relation to capillary number. Material rearrangement, which is necessary during expansion of a highly vesicular bubbly fluid, results in bubble coalescence. At the same time, capillary stresses cause coalesced bubbles to relax toward a “regular” shape (e.g., Gardner 2007). For \(\textnormal {Ca}\ll 1\), the capillary velocity exceeds the expansion velocity (\(\sigma /\eta \gg v_{\mathrm {e}}\)) and bubble shapes minimize changes in curvature of the interface between bubble and surrounding liquid (Fig. 3). For \(\textnormal {Ca}\gg 1\), the speed at which bubbles relax toward a regular shape is reduced and remnants of coalescence events become more persistent (Koerner 2008). By estimating capillary number from modeling of magma expansion for the different eruptions, and by comparing it to vesicle shapes in pyroclasts from these eruptions with vesicularity \(\phi > \approx 0.6\), we show that vesicle shapes in pyroclasts bear a relationship to Ca. Because of the dependence of capillary number on the average rate of bubble growth, \(v_{R}\), vesicle shapes may provide a quantitative record of magma ascent conditions for some time \(\tau \) prior to magma quenching, approximately equal to the viscous time scale, \(\eta /\Delta P\), where \(\Delta P = P_{\mathrm {g}}-P_{\mathrm {m}}\) is the pressure difference between gas pressure inside the bubble, \(P_{\mathrm {g}}\), and the ambient pressure, \(P_{\mathrm {m}}\).

Fig. 3
figure 3

Schematic diagram illustrating the dependence of \(\Omega \) on capillary number, Ca. During magma ascent, bubbles grow and coalesce with each other. Coalesced bubbles relax due to capillary forces while bubble growth continues. If bubble shape relaxation rate exceeds bubble growth rate (Ca \(\ll 1\)), coalesced bubbles relax back to spherical shape (\(\Omega \approx 1\)). On the other hand, for Ca\(\gg 1\), bubble shape relaxation becomes slower and remnants of coalescence events become more persistent, leading to a more complex geometry (\(\Omega <1\))

Full size image

Bubble overpressure

During magma ascent, the decline of confining pressure permits dissolved volatiles to form bubbles of supercritical fluid, due to a decrease in pressure-dependent volatile solubility. If bubble growth is limited by viscosity, \(P_{\mathrm {m}}\) may decrease at a faster rate than \(P_{\mathrm {g}}\). Consequently, bubbles can become pressurized with respect to the surrounding melt (e.g., Sparks 1978; Gonnermann and Manga 2007). Rapid expansion and/or fragmentation of these pressurized bubbles drives Hawaiian, Vulcanian, sub-Plinian, Plinian, and ultraplinian explosions (e.g., McBirney 1970; Alidibirov 1994; Dingwell 1996; Zhang 1999; Spieler et al. 2004; Koyaguchi 2005). In contrast, during Strombolian explosions much larger bubbles rise independently through relatively low viscosity magma (Vergniolle and Mangan 2000; Parfitt 2004), carrying upward with them magma with smaller bubbles. The large bubbles, also referred to as gas slugs, may be pressurized as they reach the surface (e.g., Ripepe 2001; Pistolesi et al. 2011) and their rupture is thought to constitute Strombolian explosions (Walker 1973; Blackburn et al. 1976; Vergniolle and Mangan 2000).

A relationship between vesicle shape and bubble overpressure, \(\Delta P\), may exist through the relationship of capillary number and bubble shape and the dependence of capillary number on \(v_{R}\). This dependence may be determined from the momentum balance for an idealized spherical bubble (e.g., Scriven 1959; Arefmanesh and Advani 1991; Proussevitch et al. 1993)

$$ \Delta P = P_{g} - P_{m} = \frac{2 \sigma}{R} + \frac{4 \eta_{\mathrm{e}}}{R} v_{R}, $$
(4)

where \(\eta _{\mathrm {e}}\) is the effective viscosity, accounting for the radially variable \({\rm H}_{2}{\rm O}\)-dependent viscosity (Lensky et al. 2001). Expressing Eq. (4) in terms of capillary number gives

$$ \Delta P = \frac{2 \sigma}{R} ( 1 + 2\, \phi^{-2/3} \textnormal{Ca} ). $$
(5)

Because \(\Delta P\) is proportional to the potential energy capable of initiating and sustaining magma fragmentation (Mueller et al. 2008), we hypothesize that vesicle shapes in pyroclasts produced during magma fragmentation in explosive volcanic eruptions may provide an indirect manifestation of eruption intensity through the relationship between vesicle shape, capillary number, and \(\Delta P\).

Bubble growth modeling

To estimate capillary numbers, we calculate the expansion velocity, \(\phi ^{2/3} v_{R}\), using a model for diffusive bubble growth. We assume that the pyroclasts represent parcels of magma that were carried to the surface from an initial depth equal to the \({\rm H}_{2}{\rm O}\) saturation depth for the given eruption. We model a single representative bubble of initial radius of \(R=10^{-6}\; {\rm m} \) and final radius \(R \approx 25\; {\rm{\mu}}{\rm m}\), consistent within the 10 to 100 μm range in vesicle diameters considered for vesicle shape analysis. Although somewhat larger than the size of a critical bubble nucleus, this choice of initial bubble radius does not significantly affect the model results.

The bubble growth model is based on established formulations (e.g., Amon and Denson 1984; Arefmanesh and Advani 1991; Proussevitch et al. 1993) and \({\rm H}_{2}{\rm O}\) is the sole volatile phase considered, with a solubility based on Dixon (1997) and Liu et al. (2005) for basaltic and silicic eruptions, respectively. The model couples Eq. 4 with an equation for mass balance,

$$ \frac{d}{dt}(\rho_{\mathrm{g}} R^{3}) = 3 r^{2} \rho_{\mathrm{m}} D \left(\frac{\delta c}{\delta r}\ \right)_{r = R}, $$
(6)

and an equation for the diffusion of \({\rm H}_{2}{\rm O}\),

$$ \frac{\delta c}{\delta t} + v_{r} \frac{\delta c}{\delta r}= \frac{1}{r^{2}} \frac{\delta c}{\delta r} \left(D \, r^{2} \frac{\delta c}{\delta r} \right). $$
(7)

Here, r is the radial coordinate, \(v_{r}\) is the radial velocity at radius r, and \(\rho _{\mathrm {g}}\) is the density of the exsolved \({\rm H}_{2}{\rm O}\), which depends on \(P_{\mathrm {g}}\) and is calculated using a modified Redlich–Kwong equation of state (Kerrick and Jacobs 1981). c is the mass fraction of dissolved \({\rm H}_{2}{\rm O}\) and D is its diffusivity, which depends on c and temperature. D is based on Eq. (18) of Zhang et al. (2007) for the basaltic eruptions and on Zhang and Behrens (2000) for the silicic eruptions. \(c_{R}\) is the \({\rm H}_{2}{\rm O}\) concentration at the melt–vapor interface and depends on the solubility at \(P_{\mathrm {g}}\). The effective viscosity, \(\eta _{\mathrm {e}}\), is based on the formulation of Lensky et al. (2001), using the melt viscosity, \(\eta \), which depends on melt composition, temperature, and dissolved \({\rm H}_{2}{\rm O}\) (Hui and Zhang 2007). For basaltic Plinian eruptions, \(\eta _{\mathrm {e}}\) includes the effect of microlites on viscosity. For simplicity, we assume a constant value of \(\sigma =0.05\) N \({\rm m}^{-1}\). The variability in \(\sigma \) is probably less than a factor of 2 (e.g., Gardner and Ketcham 2011 and references therein) and does not affect model results significantly (Gonnermann and Houghton 2012).

The bubble growth calculation is coupled to a model for magma ascent within the conduit, from which we obtain the decompression rate, \(dP_{\mathrm {m}}/dt\). We assume a uniform distribution of bubbles and that all bubbles are carried to the surface within the ascending magma. Consequently, the ascent and growth of bubbles are modeled in a Lagrangian frame of reference (e.g., Proussevitch and Sahagian 2005), with conduit flow formulated in an explicit manner and bubble growth semi-implicitly, similar to Proussevitch et al. (1993).

Magma ascent modeling

Modeling the Plinian, ultraplinian, and Hawaiian eruptions

For Plinian, ultraplinian, and Hawaiian eruptions, \(dP_{\mathrm {m}}/dt\) is calculated using a conduit flow model that assumes one-dimensional flow of melt with suspended bubbles that are carried passively with the ascending magma. We model this bubbly flow up to the point where magma fragmentation is predicted by the empirical formulation of Mueller et al. (2008), assuming a characteristic magma permeability of \(k \sim 10^{-12}\) \({\rm m}^{2}\). The resultant fragmentation threshold is defined by

$$ \Delta P = \Delta P_{f} = \frac{2.4}{\phi} \, \textnormal{MPa}. $$
(8)

We do not model the flow of magma above the fragmentation depth but instead use the analytical method of Koyaguchi (2005) to estimate exit pressure at the vent, \(P_{\textnormal {vent}}\) (Table 5).

Table 5 Parameters for the model calculations
Full size table

For the Hawaiian eruption, there are no feasible solutions that would predict brittle magma fragmentation. This is consistent with the view of hydrodynamical fragmentation during these eruptions (e.g., Namiki and Manga 2008; Houghton and Gonnermann 2008). Accordingly, we model bubbly flow up to the surface (Parfitt 2004), assuming an exit pressure of \(10^{5}\) Pa at the vent.

We assume isothermal flow with a constant discharge rate, Q, in a conduit of constant radius a. The rate of change in \(P_{\mathrm {m}}\) is calculated in the conventional manner for one-dimensional flow in a cylindrical vertical conduit (e.g., Wilson 1980; Dobran 1992; Mastin 2002; Proussevitch and Sahagian 2005)

$$ \frac{dP_{\mathrm{m}}}{dt} = - \rho g u - f_{\mathrm{m}}\frac{\rho u^{3}}{4a}. $$
(9)

Here, g is the acceleration due to gravity and \(u = dz/dt = Q / (\pi a^{2} \rho )\) is the magma ascent velocity, with z denoting the vertical coordinate. \(\rho = \rho _{\mathrm {m}}(1 - \phi )\) is magma density, \(\rho _{\mathrm {m}}\) is the melt density, which is assumed to be constant, and \(\phi = R^{3}/S^{3}\) is the volume fraction of vesicles, where S is the half distance between two bubbles. \(f_{\mathrm {m}} = 64 / \textnormal {Re}\ + f_{0}\) is the friction factor for pipe flow, with \(f_{0} = 0.02\) and Reynolds number, Re = \(\rho u a / \eta \) (e.g., Wilson 1980). We find that values of \(f_{0}\) corresponding to wall roughnesses of approximately 0.1-1 % of the conduit diameter do not significantly affect the results, especially given uncertainties in a and Q. For a given Q, a and initial H\(_{2}\)O (Tables 2, 3, and 5), we solve Eqs. (4), (6), (7), and (9) to estimate Ca for these eruptions.

Modeling the Strombolian eruption

For the Strombolian sample, we assume that the sample represents a parcel of magma that was passively carried to the surface by an ascending gas slug or agglomeration of large gas bubbles, consistent with the conceptual model of Lautze and Houghton (2005, 2007). The resultant value of \(dP_{\mathrm {m}}/dt\) is based on the rate of change in magma-static pressure for ascent velocities of Strombolian gas slugs (Seyfried and Freundt 2000)

$$ u_{\textnormal{str}} = 0.345 \sqrt{2 a g}. $$
(10)

Neglecting frictional pressure losses, we use

$$ \frac{dP_{\mathrm{m}}}{dt} = - \rho g u_{\textnormal{str}}, $$
(11)

with a conduit radius of 4 m, a likely lower bound (Pistolesi et al. 2011). We explored conduit radii between 2 and 10 m (Parfitt 2004; Pistolesi et al. 2011) and found that it does not change the overall model results. Given \(u_{\textnormal {str}}\), we solve the coupled Eqs. (4), (6), (7), and (11) to estimate Ca.

Modeling the Vulcanian eruption

Soufrière Hills volcano experienced 88 Vulcanian explosions in 1997, each of which discharged approximately \(3 \times 10^{5}\;{\rm m}^{3}\) of magma (dry rock equivalent), about one-third forming fallout and two-third forming pyroclastic flows (Druitt et al. 2002). Each explosion started by the rupture of a plug of dense and degassed magma (Druitt et al. 2002; Spieler et al. 2004; Burgisser et al. 2010; Burgisser et al. 2011). Triggered decompression caused the fragmentation and eruption of the conduit contents down to a maximum depth of about 2.5–3.5 km (Burgisser et al. 2011) within a time increment of about 10–100 s (Druitt et al. 2002; Melnik and Sparks 2002). Based on matrix-glass water contents of a representative suite of pyroclasts, and taking into account the pre-, syn-, and post-explosive vesiculation processes of a typical 1997 Vulcanian explosion of Soufrière Hills volcano (Giachetti et al. 2010), Burgisser et al. (2010) determined that the pre-explosive pressure of the deepest magma ejected during an explosion is approximately 80 MPa, leading to an average decompression rate of about 1–10 MPa \({\rm s}^{-1}\), consistent with estimates by Giachetti et al. (2010). Given \(dP_{\mathrm {m}}/dt\), as well as initial \({\rm H}_{2}{\rm O}\) content, we solve Eqs. (4), (6), and (7) to estimate Ca.

Modeling the basaltic Plinian eruptions

Pyroclasts from both basaltic Plinian eruptions (Etna, 122 BC and Tarawera, 1886) contain approximately 60–90 % plagioclase microlites within the groundmass that surrounds the vesicles (Sable et al. 2006, 2009). We account for the effect of microlites on magma viscosity using the formulation of Costa et al. (2009), parameterized to fit the viscosities of silicate melts containing plagioclase crystals of similar shape, size, and volume fractions as in the Etna and Tarawera samples (Picard et al. 2011). As shown in Fig. 4a, the relative viscosity, \(\eta _{\mathrm {r}}\), which is the viscosity of the crystal-bearing melt divided by the crystal-free melt viscosity, increases rapidly with the volume fraction of plagioclase microlites, \(\phi _{x}\), for values of \(\phi _{x} > 0.3\) (Picard et al. 2011).

Fig. 4
figure 4

a Relative viscosity, \(\eta _{\mathrm {r}}\), as a function of crystal volume fraction, \(\phi _{x}\). The values of \(\eta _{\mathrm {r}}\) are calculated using equations \(\eta _{\mathrm {r}} = (1+\phi ^{\delta })/(1-F)^{B\phi _*}\) and \(F = (1-\xi ) {\mathrm {erf}}((\sqrt \pi \phi (1+\phi ^{\gamma }))/(2(1-\xi )))\) of Costa et al. (2009) with fitting parameters \(\delta =8\), \(\gamma =6\), \(B=2.5\), and \(\phi _*=0.29\). b Modeled crystal volume fraction, \(\phi _{x}\) (Eq. 12), as a function of time for \(I \, G^{3} = 10^{-8}\) s\(^{-4}\) and \(k_{\mathrm {v}} = 4 \pi /3\). c Corresponding relative viscosity, \(\eta _{\mathrm {r}}\), as a function of time. The measured values of \(\eta _{\mathrm {r}}\) for silicic melt with plagioclase microcrystals (Picard et al. 2011) are shown as open circles at model times corresponding to the values of \(\phi _{x}\) in b

Full size image

It is uncertain at precisely what depth and over what time interval the microlites formed during either eruptions. Although Sable et al. (2006) and Sable et al. (2009) inferred that the microlites found in both Etna and Tarawera samples formed syn-eruptively, as a consequence of undercooling during \({\rm H}_{2}{\rm O}\) exsolution (Hammer 2008), post-eruptive overprinting can obscure syn-eruptive crystallization (Szramek et al. 2006, 2010; Szramek 2010). Furthermore, some microlite crystallization may have occurred during a short time interval prior to eruption (Szramek 2010). We therefore explored a wide range of crystallization rates in our modeling of both eruptions, using the Avrami equation (e.g., Cashman 1993; Marsh 1998; Pupier 2008, and references therein)

$$ \phi_{x} = 1 - \exp(-k_{\mathrm{v}} I G^{3} t^{4} ). $$
(12)

Here, I is the crystal nucleation rate, G is the crystal growth rate, t is the time from the onset of crystallization, and \(k_{\mathrm {v}}\) is a shape factor, for simplicity assumed to be \(k_{\mathrm {v}}=4\pi /3\) (Marsh 1998). We explored a wide range of values, with \(10^{-9} \, \textnormal {s}^{-4} \le (I \, G^{3}) \le 10^{-3} \, \textnormal {s}^{-4}\). If crystallization was too early, model simulations predicted magma fragmentation at values of \(\phi \) that are significantly smaller than those found in the basaltic Plinian clasts. If crystallization was too late, no magma fragmentation was predicted. However, for a range of crystallization rates (\(7.5 \le (I \, G^{3}) \le 8.5\) s\(^{-4}\)), model results predict magma fragmentation, together with values of \(\phi \) and R that are consistent with those measured in the basaltic Plinian samples. These values of \((I\,G^{3})\) are also well within the range of results from plagioclase crystallization experiments (Burkhard 2005; Hammer 2008; Brugger and Hammer 2010). The resultant change in \(\phi _{x}\) and \(\eta _{\mathrm {r}}\), with respect to time after the onset of crystallization, is shown in Fig. 4b, c for a representative case.

Results and discussion

Figure 2 indicates that vesicles in pyroclasts from Strombolian and Hawaiian eruptions have distinctly larger values of \(\Omega \) than the other eruptions. Vesicle shapes formed during ascent within the conduit may be modified after fragmentation by bubble growth (e.g., Thomas and Sparks 1992; Kaminsky and Jaupart 1997) and shape relaxation due to capillary forces (e.g., Klug and Cashman 1996). However, for silicic and for microlite-rich basaltic magmas, post-fragmentation bubble growth should be of limited extent, due to permeable outgassing (e.g., Rust and Cashmann 2011; Gonnermann and Houghton 2012). Moreover, the characteristic time scale for shape relaxation, \(\tau _{\textnormal {relaxation}}\), would need to be much shorter than the characteristic quenching time, \(\tau _{\textnormal {quenching}}\). To evaluate the effect of post-fragmentation shape relaxation, we estimate \(\tau _{\textnormal {quenching}} \sim 100\) s for centimeter-size pyroclasts following the approach of Thomas and Sparks (1992). For Vulcanian, Plinian, ultraplinian, and basaltic Plinian eruptions, \(\tau _{\textnormal {relaxation}} = \eta R/\sigma \sim 10^{3} \; \textnormal {to} \; 10^{5} \, \textnormal {s} \, \gg \tau _{\textnormal {quenching}}\) (assuming that the viscosity in the case of the basaltic Plinian magmas accounts for the effect of microlites). Consequently, post-fragmentation shape relaxation should be negligible. For the less viscous Hawaiian and Strombolian basalt magmas, \(\tau _{\textnormal {relaxation}} \sim 10^{-2} \; \textnormal {to} \; 10^{-3} \, \textnormal {s} \, \ll \tau _{\textnormal {quenching}}\) and bubbles are likely to undergo post-fragmentation shape relaxation. Therefore, the measured values of \(\Omega \) for the Hawaiian and Strombolian samples cannot irrevocably be attributed to bubble shapes during magma ascent within the conduit.

Although vesicular volcanic rocks that have undergone vesicle collapse due to open-system degassing can also exhibit complex vesicle shapes (e.g., Adams et al. 2006; Wright et al. 2009), none of the samples analyzed herein have undergone noticeable vesicle collapse nor vesicle elongation due to shear. Volcanic rocks that have undergone vesicle collapse, such as volcanic dome samples, may qualitatively exhibit a range of vesicle shapes, including large elongation and complexly shaped vesicle margins (e.g., Wright and Weinberg 2009). Textures of such samples, if they have small values of \(\Omega \), should in principle also be indicative of large capillary numbers, either associated with processes that are not a consequence of decompression-driven bubble growth (e.g., due to large strain rates associated with bubble collapse at high viscosities) or perhaps as a consequence of preserving some textural remnants of bubble growth (e.g., small strain rates at high viscosity). Consequently, the relationship between \(\Omega \), capillary number, \(\Delta P\), and fragmentation suggested herein only holds for pyroclastic samples associated with explosive eruptions that have not undergone bubble collapse. For these cases, we therefore suggest that \(\Omega \) can be related to Ca, through modeling of the expansion velocity, \(v_{\mathrm {e}}\) (Koerner 2008).

Figure 5 shows a representative model result for each eruption as a graph of bubble radius, R, overpressure, \(\Delta P\), and capillary number as a function of dimensionless pressure, defined as \(\hat {P} = (P_{\mathrm {m}}-P_{\textnormal {frag}})/(P_{\textnormal {initial}}-P_{\textnormal {frag}})\). Note that in the case of Stromboli and Kilauea Iki, the fragmentation threshold is not reached and \(P_{\textnormal {frag}}\) is assumed as the vent pressure of \(10^{5}\) Pa. We chose dimensionless \(\hat {P}\) as the ordinate, as opposed to dimensional \(P_{\mathrm {m}}\) or depth, because \(\hat {P}\) facilitates an easier comparison between the individual eruptions. We refer the reader to Table 5 for the different values of \(P_{\textnormal {initial}}\), \(P_{\textnormal {frag}}\), \(z_{\textnormal {initial}}\), and fragmentation depth. Although we find from a parametric analysis that the details of the plotted curves will change somewhat within the range of realistic parameter values (Table 2), neither their characteristic shape nor the predicted values of capillary number and \(\Delta P\) will be significantly affected.

Fig. 5
figure 5

Plots of dimensionless pressure as a function of a bubble radius, R, b overpressure, \(\Delta P\), and c capillary number, Ca. Dimensionless pressure, \(\hat {P}\) is defined as \((P_{\mathrm {m}}-P_{\textnormal {frag}})/(P_{\textnormal {initial}}-P_{\textnormal {frag}})\) (Table 5) for Vulcanian, Plinian, basaltic Plinian, and ultraplinian eruptions, whereas \(\hat {P} = P_{\mathrm {m}}/P_{\mathrm {initial}}\) in the case of Stromboli and Kilauea Iki eruptions. Modeled final bubble radii are 20–40 \(\mu \)m, consistent with vesicle shape quantification. Values of modeled final capillary number obtained for Hawaiian and Strombolian eruptions are smaller than those obtained for Vulcanian, Plinian, and ultraplinian eruptions

Full size image

As magma ascends toward the surface, \(P_{\mathrm {m}}\) decreases and water diffuses into growing bubbles. Because the viscosity of silicic magmas depends strongly on the concentration of dissolved \({\rm H}_{2}{\rm O}\), the increasing viscosity will retard bubble growth and allow the buildup of bubble overpressure, \(\Delta P\). At the same time, the capillary number will increase to values \(\gg {1}\). Although the viscosity of basaltic melt is much less affected by \({\rm H}_{2}{\rm O}\) exsolution, the resultant undercooling may induce significant crystallization, as suggested for Etna and Tarawera basaltic Plinian eruptions (Sable et al. 2006, 2009). The microlites found in Etna and Tarawera samples must have produced a significant increase in viscosity (see Fig. 4a; Picard et al. 2011); however, the timing of microlite formation is somewhat uncertain (Szramek et al. 2006, 2010; Goepfert and Gardner 2010; Szramek 2010).

Here, we show through combined bubble growth and conduit flow modeling that the basaltic Plinian eruptions of Etna and Tarawera can be explained by syn-eruptive crystallization, buildup of overpressure, and brittle fragmentation. Both, pre- and syn-eruptive crystallization are consistent with vesicle shapes (\(\Omega <1\) and \(\textnormal {Ca}\gg 1\)). However, conduit flow modeling indicates that the viscosity of the microlite-rich magma would require magma fragmentation within a short distance of the pre-eruptive reservoir, as the large magma viscosity would otherwise inhibit magma ascent to the surface. Regardless, the model results demonstrate that the increase in viscosity due to microlites will result in \(\textnormal {Ca}\gg 1\) for these eruptions, consistent with \(\Omega <1\). Therefore, our results support the hypothesis that basaltic Plinian eruptions were, like their silicic counterparts, associated with overpressure and brittle magma fragmentation as a consequence of high viscosity (Sable et al. 2006, 2009; Houghton and Gonnermann 2008).

The relationships of Ω and predicted capillary number for the different eruptions are shown in Fig. 6. All the silicic eruptions, which presumably were associated with brittle fragmentation, have larger values of (1−Ω) (i.e., smaller values of Ω) than the Strombolian and Hawaiian style eruptions, as well as \(\textnormal {Ca}\gg 1\). This is also the case for the basaltic Plinian eruptions. In contrast, for the Hawaiian and Strombolian eruptions, our model results indicate that \(\textnormal {Ca}\ll 1\), with insufficient overpressure for brittle fragmentation, thus consistent with the work of Namiki and Manga (2008). Although our model results imply that bubble shapes for the Hawaiian and Strombolian eruptions should have had values of \(\Omega \approx 1\) during magma ascent within the conduit, this cannot be established unequivocally because of post-fragmentation shape relaxation.

Fig. 6
figure 6

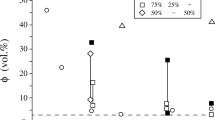
Measured median \((1-\Omega )\) versus calculated capillary number, Ca, for the clasts analyzed herein (Table 4), with images thereof shown as insets (the scale bar at the bottom left of each image is 100 \(\mu \)m in length). Capillary numbers represent the values at magma fragmentation for Vulcanian, Plinian and ultraplinian eruptions, or at \(P_{\mathrm {m}} = 10^{5}\) Pa for Hawaiian and Strombolian eruptions. A clear distinction exists between Hawaiian and Strombolian eruptions (smaller (\(1-\Omega \)) and \(\textnormal {Ca}\ll 1\)), and the other eruptions (larger (\(1-\Omega \)) and \(\textnormal {Ca}\gg 1\)). This distinct dichotomy in \(\Omega \) and Ca is mirrored in inferred fragmentation style, with brittle fragmentation of magma occurring in Vulcanian, Plinian, basaltic Plinian, and ultraplinian eruptions

Full size image

Conclusions

Vesicle shapes in pyroclasts from fall deposits of a wide range of explosive volcanic eruptions were quantified using the shape parameter \(\Omega \). When compared to estimates of capillary number, based on the expansion velocity obtained from bubble growth modeling, we find a clear distinction of Hawaiian and Strombolian eruptions (\(\Omega \approx 1\), \(\textnormal {Ca}\ll 1\)) versus eruptions of higher intensity (\(\Omega <1\), \(\textnormal {Ca}\gg 1\)), such as Vulcanian, Plinian, and ultraplinian. Importantly, basaltic Plinian eruptions are distinctly different in \(\Omega \) and in capillary number from Strombolian and Hawaiian eruptions. This suggests that the presence of abundant plagioclase microlites resulted in a sufficient increase in viscosity to result in overpressure for brittle fragmentation during the basaltic Plinian eruptions, akin to their silicic counterparts, which do not contain large abundances of microlites.