1 Introduction

The atmosphere on the rotating earth is subjected to the gravity and Coriolis force fields. The large-scale atmospheric motion at the middle-high latitudes is thus quasi-horizontal, hydrostatic equilibrium, quasi-geostrophic equilibrium and quasi-horizontal non-divergent (Rossby 1939; Charney 1947). After introducing hydrostatic equilibrium, geostrophic equilibrium and the representation of two dimensional (2D) stream function and potential function for velocity into the equations of atmospheric motions with the appropriate approximations (Holton 2004), the resulting quasi-geostrophic theories have become the theoretical foundation of weather forecast. It also consists of the major components of the theory of atmospheric dynamics (Tao et al. 2012), allowing us to understand the physical mechanisms of the generation and evolution of large-scale atmospheric motions at middle-high latitudes (Zhou et al. 2013). However, the atmospheric motions at the tropics is constrained by the angular momentum conservation and can easily excite the vertical motions (Palmén and Alaka 1952; Oort and Peixóto 1983), exhibiting the overturning Hadley and Walker circulation (Hartmann 1994). Thus, quasi-geostrophic theories are not appropriate for the atmospheric motions at low latitudes, leading to two subjects in the modern theory of atmosphere, i.e., middle-high latitudes and low latitudes atmospheric dynamics (Holton 2004). Nevertheless, such split breaks the integrity of the global atmospheric motion, resulting in insufficient understanding of the interactions between the atmospheric motions at low latitudes and that at middle-high latitudes (Gu and Philander 1997; Liu and Yang 2003; Ding et al. 2011; Lau et al. 2012). Therefore, it becomes an important topic of studying the interaction characteristics between middle-high latitudes and low latitudes atmospheric circulations (Ferranti et al. 1990; Kiladis and Feldstein 1994).

As we mentioned earlier, the Rossby wave is the major component of the atmospheric motions at the middle-high latitudes (Rossby 1939). It is one of the most important discoveries in modern atmospheric dynamics. There exist ridges and troughs and high and low pressure systems developed by the perturbation of the zonal atmospheric motions. Their centers and strength reflect the characteristics of the atmospheric circulations in the corresponding region. At the low latitudes on the other hand, the motion is dominated by the Hadley and Walker circulations within the meridional and zonal planes respectively. Hadley circulation is considered as one of the most important circulations that affect the balance of the incoming and outgoing physical quantities in the global atmospheric circulations (Oort and Peixóto 1983; Trenberth and Solomon 1994; Bowman and Cohen 1997; Dima and Wallace 2003), while the Walker circulation is considered as a component of the ENSO (El Niño Southern Oscillation) phenomenon in tropical ocean and atmosphere (Julian and Chervin 1978; Kousky et al. 1984; Bayr et al. 2014). The above three major circulations are the most important global large-scale circulations. Their movement and evolution can greatly affect the global atmospheric circulation and the thermal and water exchange between high and low latitudes and between ocean and continent, and thus impact the formation and evolution of weather and climate. Based on these important features of atmospheric circulations, we propose the mathematical definitions of three dimensional (3D) horizontal, meridional and zonal circulations which can be considered as the global generalization of the Rossby wave at the middle-high latitudes and Hadley and Walker circulations at the low latitudes. We propose a novel method of 3D circulation decomposition that can describe the actual atmosphere from a global-wide perspective (Xu 2001; Hu 2006, 2008; Liu et al. 2008), i.e., the global atmospheric circulation is decomposed into three orthogonal horizontal, meridional and zonal circulations, which is called the three-pattern decomposition model. The new dynamical equations of the large-scale three-pattern circulations are then established by applying the decomposition model to the primitive equations of atmosphere at the planetary-scale. This leads to a novel theory of a complete description of large-scale atmospheric circulations and is potentially useful to uncover the mechanisms of the complicated interaction between the circulations at middle-high latitudes and low latitudes. With appropriate simplification of the new set of the dynamical equations, the mechanisms for the evolution and interaction of the large-scale three-pattern circulations with the global warming can be revealed.

The rest of the paper is organized as follows. In Sect. 2, we define the 3D horizontal, meridional and zonal circulations based on the traditional definitions of the large-scale circulations. In Sect. 3, we then express the actual global atmospheric circulation as the superposition of the three-pattern circulations, which forms the foundations of the three-pattern decomposition of global atmospheric circulation. We provide the theorems of the sufficient and necessary conditions for such decomposition to ensure its physical validity. To test our theory, we input the NCEP/NCAR reanalysis data into our model to obtain the climate characteristics of the horizontal, meridional and zonal circulations in Sect. 4. Section 5 is the conclusion part.

2 Definition of large-scale three-pattern circulations

Rossby wave dominates the middle-high latitudes motions which are usually quasi-horizontal and quasi-geostrophic, while Hadley and Walker circulations in the vertical direction reside in the low latitudes. These circulations can be generalized to the globe, e.g., Rossby wave to the low latitudes and Hadley and Walker to the middle-high latitudes. Such generalized circulations are called horizontal, meridional and zonal circulations. In this section, we will offer the mathematical definitions of those three orthogonal circulations.

2.1 Coordinate system

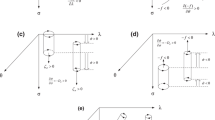
We usually use the spherical \(p\)-coordinate system to describe the global atmospheric motion based on the thin layer approximation and hydrostatic equilibrium. The latitude variable in the spherical \(p\)-coordinate system is usually replaced by colatitude. If we use \(\vec{i},\vec{j}\) and \(\vec{k}\) to represent the three unit vector in the spherical \(p\)-coordinate system, then \(\vec{i}\) is pointing from west to east along the latitude circles, \(\vec{j}\) is from north to south along the longitudinal direction, and \(\vec{k}\) is pointing from the earth surface to center. The three vectors \(\vec{i},\vec{j}\) and \(\vec{k}\) constitute a local right-hand orthogonal coordinate system in which any point in space is denoted by \((\lambda ,\theta ,p)\) where \(\lambda\) represents the longitude, \(\theta\) is the colatitude and \(p\) is the atmospheric pressure. The three coordinate elements in the spherical \(p\)-coordinate system are:

$$\delta x = a\sin \theta \delta \lambda ,\quad \delta y = a\delta \theta ,\quad \delta p = \delta p,$$
(2.1)

where \(a\) is the earth radius. The corresponding velocity fields are:

$$u = a\sin \theta \frac{d\lambda }{dt},\quad v = a\frac{d\theta }{dt},\quad \omega = \frac{dp}{dt}.$$
(2.2)

Note that the unit in Eq. (2.2) for the horizontal velocity field is \({\text{m}}\,{\text{s}}^{ - 1}\), while the vertical velocity field has a unit of \({\text{Pa}}\,{\text{s}}^{ - 1}\). In order to resolve this unit inconsistency in calculating the 3D vorticity vector, we introduced the spherical \(\sigma\)-coordinate system, e.g., let \(\sigma = \frac{{p - p_{0} }}{{P_{s} - p_{0} }}\) where \(p_{0}\) is the pressure at the top of the atmosphere and \(P_{s}\) is the pressure at the earth surface. We can assume \(P_{s} = 1000\,{\text{hPa}}\) and \(p_{0} = 0\,{\text{Pa}}\), and the coordinate elements become

$$\delta x^{{\prime }} = \frac{\delta x}{a},\quad \delta y^{{\prime }} = \frac{\delta y}{a},\quad \delta \sigma = \frac{\delta p}{{P_{s} }},$$
(2.3)

and the velocity fields in the spherical \(p\)-coordinate system are transformed into

$$u^{{\prime }} = \frac{u}{a},\quad v^{{\prime }} = \frac{v}{a},\quad \dot{\sigma } = \frac{\omega }{{P_{s} }}.$$
(2.4)

The velocity fields (\(u^{{\prime }} ,v^{{\prime }} ,\dot{\sigma }\)) in Eq. (2.4) now has the same unit of \({\text{s}}^{ - 1}\). The 3D vorticity vector of the velocity field \(\vec{V}^{{\prime }} = u^{{\prime }} \vec{i} + v^{{\prime }} \vec{j} + \dot{\sigma }\vec{k}\) in the spherical \(\sigma\)-coordinate system then is

$$\nabla \times \vec{V}^{{\prime }} = \vec{i}\left( {\frac{{\partial \dot{\sigma }}}{\partial \theta } - \frac{{\partial v^{{\prime }} }}{\partial \sigma }} \right) + \vec{j}\left( {\frac{{\partial u^{{\prime }} }}{\partial \sigma } - \frac{1}{\sin \theta }\frac{{\partial \dot{\sigma }}}{\partial \lambda }} \right) + \vec{k}\left( {\frac{1}{\sin \theta }\frac{{\partial v^{{\prime }} }}{\partial \lambda } - \frac{1}{\sin \theta }\frac{{\partial (u^{{\prime }} \sin \theta )}}{\partial \theta }} \right),$$
(2.5)

the magnitude and direction of which represent the rotation of \(\vec{V}^{\prime}\) at any point. Using Eq. (2.4) and the continuity equation in the spherical \(p\)-coordinate system, we can easily obtain the continuity equation of the velocity fields in the spherical \(\sigma\)-coordinate system:

$$\frac{1}{\sin \theta }\frac{{\partial u^{{\prime }} }}{\partial \lambda } + \frac{1}{\sin \theta }\frac{{\partial (v^{{\prime }} \sin \theta )}}{\partial \theta } + \frac{{\partial \dot{\sigma }}}{\partial \sigma } = 0.$$
(2.6)

Hereafter, all of our discussions about the velocity fields (\(u^{{\prime }} ,v^{{\prime }} ,\dot{\sigma }\)) are carried out in the spherical \(\sigma\)-coordinate system. Note that any physical quantities can be easily transformed back using Eq. (2.4) into expressions of (\(u,v,\omega\)) in the spherical \(p\)-coordinate system.

2.2 Horizontal circulation

Based on the characteristics of the Rossby wave, we can assume that the horizontal circulation has zero vertical velocity and the horizontal velocity is however having the baroclinic structure, i.e., at any time instant \(t\), the horizontal velocity is varying with height as the following

$$\vec{V}_{R}^{{\prime }} (\lambda ,\theta ,\sigma ) = \vec{i}u_{R}^{{\prime }} (\lambda ,\theta ,\sigma ) + \vec{j}v_{R}^{{\prime }} (\lambda ,\theta ,\sigma ),$$
(2.7)

which satisfies the continuity equation based on Eq. (2.6):

$$\frac{1}{\sin \theta }\frac{{\partial u_{R}^{{\prime}} }}{\partial \lambda } + \frac{1}{\sin \theta }\frac{{\partial (\sin \theta v_{R}^{{\prime }} )}}{\partial \theta } = 0.$$
(2.8)

The streamlines are usually used to directly represent the motion of fluid. For the horizontal circulation, \(\vec{V}_{R}^{{{{\prime }} }}\) is parallel to the streamline element \(d\vec{S} = \vec{i}\sin \theta d\lambda + \vec{j}d\theta + \vec{k}d\sigma\). We then must have

$$v_{R}^{\prime } \sin \theta d\lambda - u_{R}^{\prime} \,d\theta = 0,\quad d\sigma = 0.$$
(2.9)

According to the continuity Eq. (2.8), Eq. (2.9) can be expressed as the total derivative of some differentiable function \(R(\lambda ,\theta ,\sigma )\) for any \(\sigma \in [0,1]\):

$$dR(\lambda ,\theta ,\sigma ) = \frac{\partial R}{\partial \lambda }d\lambda + \frac{\partial R}{\partial \theta }d\theta = v_{R}^{{\prime }} \sin \theta d\lambda - u_{R}^{{\prime }} \,d\theta = 0,$$
(2.10)

thus

$$u_{R}^{{\prime }} = - \frac{\partial R}{\partial \theta },\quad v_{R}^{{\prime }} = \frac{1}{\sin \theta }\frac{\partial R}{\partial \lambda }.$$
(2.11)

Since \(\sigma \in [0,1]\) can be arbitrary in Eq. (2.10), \(R(\lambda ,\theta ,\sigma ) = c\) actually represents a 3D surface. For given constants \(c\) and \(\sigma\), \(R(\lambda ,\theta ,\sigma ) = c\) characterizes the distribution of streamlines for \(\vec{V}_{R}^{{\prime }}\) at time instant \(t\) on the plane with constant \(\sigma\). The direction of the tangent on streamlines at any point must be parallel to \(\vec{V}_{R}^{{\prime }}\), and the density of streamlines determines the magnitude of \(\vec{V}_{R}^{{\prime }}\). We thus call \(R(\lambda ,\theta ,\sigma )\) the stream function of the horizontal circulation and the components of \(\vec{V}_{R}^{{\prime }}\) are expressed by \(R(\lambda ,\theta ,\sigma )\) in Eq. (2.11). The number of the required physical quantities about the velocity field \(\vec{V}_{R}^{{\prime }}\) is reduced by introducing the stream function \(R\). It also provides the intuitive physical picture of the fluid motion.

Note that \(u_{R}^{{\prime }}\) and \(v_{R}^{{\prime }}\) depend on \(\sigma\) in Eq. (2.9), thus the stream function \(R\) cannot be fully determined by a simple integration of Eq. (2.10). This is due to the fact that for any differentiable function \(f(\sigma )\) the sum \(R(\lambda ,\theta ,\sigma ) + f(\sigma )\) also satisfies Eq. (2.10). We will resolve this issue in the next section.

2.3 Meridional circulation

We can assume that the meridional circulation has zero zonal wind. We further assume that its meridional wind and vertical velocity can vary with the longitude. At time instant \(t\), it can be represented as:

$$\vec{V}_{H}^{{\prime }} (\lambda ,\theta ,\sigma ) = \vec{j}v_{H}^{{\prime }} (\lambda ,\theta ,\sigma ) + \vec{k}\dot{\sigma }_{H} (\lambda ,\theta ,\sigma ),$$
(2.12)

satisfying the continuity equation according to Eq. (2.6):

$$\frac{1}{\sin \theta }\frac{{\partial (\sin \theta v_{H}^{{\prime }} )}}{\partial \theta } + \frac{{\partial \dot{\sigma }_{H} }}{\partial \sigma } = 0.$$
(2.13)

Similarly, the velocity field \(\vec{V}_{H}^{{\prime }}\) for the meridional circulation should be parallel to the streamline element \(d\vec{S}\):

$$\dot{\sigma }_{H} d\theta - v_{H}^{\prime } d\sigma = 0,\quad d\lambda = 0,$$
(2.14)

which is called the streamline equation for the velocity field \(\vec{V}_{H}^{{\prime }}\). Using the continuity Eq. (2.13), for any given \(\lambda \in [0,2\pi ]\), Eq. (2.14) can be expressed as the total derivative of some differentiable function \(\hat{H}(\lambda ,\theta ,\sigma )\) after multiplying both sides of Eq. (2.14) with \(\sin \theta\):

$$d\hat{H}(\lambda ,\theta ,\sigma ) = \sin \theta \dot{\sigma }_{H} d\theta - \sin \theta v_{H}^{{\prime }} d\sigma = 0.$$
(2.15)

In order to remove the factor \(\sin \theta\), we can let \(\hat{H}(\lambda ,\theta ,\sigma ) = \sin \theta H(\lambda ,\theta ,\sigma )\). Then Eq. (2.15) becomes

$$d(\sin \theta H) = \frac{\partial (\sin \theta H)}{\partial \theta }d\theta + \sin \theta \frac{\partial H}{\partial \sigma }d\sigma = \sin \theta \dot{\sigma }_{H} d\theta - \sin \theta v_{H}^{{\prime }} d\sigma = 0.$$
(2.16)

Thus

$$v_{H}^{{\prime }} = - \frac{\partial H}{\partial \sigma },\quad \dot{\sigma }_{H} = \frac{1}{\sin \theta }\frac{\partial (\sin \theta H)}{\partial \theta }.$$
(2.17)

We call \(H(\lambda ,\theta ,\sigma )\) the stream function of the meridional circulation with which the velocity field \(\vec{V}_{H}^{{\prime }}\) can be expressed in Eq. (2.17). Similarly, \(\hat{H}(\lambda ,\theta ,\sigma )\) in Eq. (2.15) is fixed up to a differentiable function \(f(\lambda )\). Thus, \(H\) cannot be uniquely determined by simply integrating Eq. (2.16).

2.4 Zonal circulation

We assume that the zonal circulation has zero meridional wind. Its zonal wind and vertical velocity can vary with the latitude. At the time instant \(t\), the zonal circulation can be represented as

$$\vec{V}_{W}^{{\prime }} (\lambda ,\theta ,\sigma ) = \vec{i}u_{W}^{{\prime }} (\lambda ,\theta ,\sigma ) + \vec{k}\dot{\sigma }_{W} (\lambda ,\theta ,\sigma ),$$
(2.18)

which satisfies the continuity equation according to Eq. (2.6):

$$\frac{1}{\sin \theta }\frac{{\partial u_{W}^{{\prime }} }}{\partial \lambda } + \frac{{\partial \dot{\sigma }_{W} }}{\partial \sigma } = 0.$$
(2.19)

Similarly, \(\vec{V}_{W}^{{\prime }}\) is parallel to the element \(d\vec{S}\) along the streamline of the velocity field \(\vec{V}_{W}^{{\prime }}\) for the zonal circulation:

$$u_{W}^{{\prime }} d\sigma - \dot{\sigma }_{W} \sin \theta d\lambda = 0,\quad d\theta = 0,$$
(2.20)

which is the streamline equation for \(\vec{V}_{W}^{{\prime }}\). According to the continuity Eq. (2.19), for any given \(\theta \in [0,\pi ]\), the first equation in Eq. (2.20) can be expressed in a total differential form of a differentiable function \(W(\lambda ,\theta ,\sigma )\):

$$dW(\lambda ,\theta ,\sigma ) = \frac{\partial W}{\partial \lambda }d\lambda + \frac{\partial W}{\partial \sigma }d\sigma = u_{W}^{{\prime }} d\sigma - \dot{\sigma }_{W} \sin \theta d\lambda = 0,$$
(2.21)

thus

$$u_{W}^{{\prime }} = \frac{\partial W}{\partial \sigma },\quad \dot{\sigma }_{W} = - \frac{1}{\sin \theta }\frac{\partial W}{\partial \lambda }.$$
(2.22)

The velocity field \(\vec{V}_{W}^{{\prime }}\) for the zonal circulation can be expressed in terms of \(W(\lambda ,\theta ,\sigma )\) using Eq. (2.22). We call \(W(\lambda ,\theta ,\sigma )\) the stream function of the zonal circulation. It also cannot be uniquely determined by a simple integration of Eq. (2.21).

3 The three-pattern decomposition of global atmospheric circulation

Circulation decomposition is commonly used in atmosphere sciences, e.g., the stream function and velocity potential function decomposition in 2D fluid dynamics are successfully applied to the quasi-horizontal and quasi-geostrophic atmospheric dynamics at the middle-high latitudes and generate many important theoretical results and applications. However, there exists strong vertical motion at the low latitudes. A study of the 3D motions of atmosphere is then required to investigate the phenomena such as intertropical convergence zone (ITCZ), monsoon and tropical cyclone. Therefore, the traditional 2D decomposition in fluid dynamics is not appropriate to study the global atmosphere. A novel method of 3D decomposition will be introduced in this section.

For large-scale motions where the small-scale effect can be neglected, we can assume that the global atmospheric circulation can actually be expressed as the superposition of the horizontal, meridional and zonal circulations defined above. In other words, for any given velocity field \(\vec{V}^{{\prime }} = \vec{i}u^{\prime }(\lambda ,\theta ,\sigma ) + \vec{j}v^{\prime }(\lambda ,\theta ,\sigma ) + \vec{k}\dot{\sigma }(\lambda ,\theta ,\sigma )\), we have

$$\vec{V}^{\prime } = \vec{V}_{H}^{{\prime }} + \vec{V}_{W}^{{\prime }} + \vec{V}_{R}^{{\prime }} ,$$
(3.1)

with its components being expressed as

$$u^{{\prime }} = u_{W}^{{\prime }} + u_{R}^{{\prime }} ,\quad v^{{\prime }} = v_{H}^{{\prime }} + v_{R}^{{\prime }} ,\quad \dot{\sigma } = \dot{\sigma }_{W} + \dot{\sigma }_{H} .$$
(3.2)

Using the stream functions in Eqs. (2.11), (2.17) and (2.22), we arrive at

$$\left\{ {\begin{array}{*{20}l} {\frac{\partial W}{\partial \sigma } - \frac{\partial R}{\partial \theta } = u^{{\prime }} ,} \hfill \\ \\ {\frac{1}{\sin \theta }\frac{\partial R}{\partial \lambda } - \frac{\partial H}{\partial \sigma } = v^{\prime },} \hfill \\ \\ {\frac{1}{\sin \theta }\frac{\partial (\sin \theta H)}{\partial \theta } - \frac{1}{\sin \theta }\frac{\partial W}{\partial \lambda } = \dot{\sigma }.} \hfill \\ \end{array} } \right.$$
(3.3)

We then can determine the velocity fields \(\vec{V}_{H}^{{\prime }}\), \(\vec{V}_{W}^{{\prime }}\) and \(\vec{V}_{R}^{{\prime }}\) using Eqs. (2.11), (2.17) and (2.22) and furthermore the original velocity field using Eq. (3.3) if the stream functions \(H,W,R\) are known. On the other hand, the velocity fields \(\vec{V}_{H}^{{\prime }}\), \(\vec{V}_{W}^{{\prime }}\) and \(\vec{V}_{R}^{{\prime }}\) can be obtained by calculating the stream functions \(H,W,R\). We call the velocity fields \(\vec{V}_{H}^{{\prime }}\), \(\vec{V}_{W}^{{\prime }}\) and \(\vec{V}_{R}^{{\prime }}\) satisfying Eq. (3.1) or Eq. (3.3) the three-pattern decomposition of the original velocity field \(\vec{V}^{\prime }\).

3.1 Theorems of the three-pattern decomposition

We can conveniently denote \(\vec{A} = H\vec{i} + W\vec{j} + R\vec{k}\). According to the definition of 3D vorticity vector in Eq. (2.5), Eq. (3.3) is equivalent to

$$- \nabla \times \vec{A} = \vec{V}^{{\prime }} .$$
(3.4)

Therefore, the vector \(\vec{A}\) satisfying Eq. (3.4) is called the 3D stream function vector for the velocity field \(\vec{V}^{{\prime }}\). Note that for any given \(\vec{V}^{\prime}\) in Eq. (3.4), there are infinitely many \(\vec{A}\) satisfying Eq. (3.4). We have the following definition and theorems to pick up the correct \(\vec{A}\).

Definition 1

For a given velocity field \(\vec{V}^{{\prime }}\), if there exists a unique 3D stream function vector \(\vec{A} = H\vec{i} + W\vec{j} + R\vec{k}\) such that \(- \nabla \times \vec{A} = \vec{V}^{{\prime }}\), the decomposition of \(\vec{V}^{{\prime }}\) into \(\vec{V}_{H}^{{\prime }} ,\vec{V}_{W}^{{\prime }} ,\vec{V}_{R}^{{\prime }}\) through Eqs. (2.11), (2.17) and (2.22), called the three-pattern decomposition of global atmospheric circulation, is said to be appropriate.

Theorem 1

The sufficient and necessary conditions for an appropriate three-pattern decomposition of global atmospheric circulation are: a zero velocity field \(\vec{V}^{{\prime }}\) (zero magnitude) can only be decomposed into three zero velocity fields \(\vec{V}_{H}^{{\prime }} ,\vec{V}_{W}^{{\prime }} ,\vec{V}_{R}^{{\prime }}\) , i.e., it is not allowed to decompose zero field into the sum of two fields with equal magnitude and opposite direction.

Theorem 2

For \(\sigma = 1\) , we have \(H = W = 0,\frac{\partial R}{\partial \sigma } = 0.\) Then the sufficient and necessary conditions that a zero field \(\vec{V}^{{\prime }}\) is decomposed into three zero fields \(\vec{V}_{H}^{{\prime }} ,\vec{V}_{W}^{{\prime }} ,\vec{V}_{R}^{{\prime }}\) are such that the 3D stream function vector \(\vec{A}\) satisfies

$$\nabla \cdot \vec{A} = \frac{1}{\sin \theta }\frac{\partial H}{\partial \lambda } + \frac{1}{\sin \theta }\frac{\partial (W\sin \theta )}{\partial \theta } + \frac{\partial R}{\partial \sigma } = 0.$$
(3.5)

Theorems 1 and 2 indicate that our proposed three-pattern decomposition of global atmospheric circulation is physically significant. Theorem 1 guarantees that our decomposition method will yield a unique result, while Theorem 2 provides the sufficient and necessary conditions. The proof of Theorem 1 is obvious, and the proof of Theorem 2 is provided in “Appendix”.

Note that when \(p = P_{s}\), the velocity fields in the spherical \(p\)-coordinate system have the boundary conditions of \(\omega = 0\), \(\frac{\partial u}{\partial p} = 0\), \(\frac{\partial v}{\partial p} = 0\). Thus, when \(\sigma = 1\), we have \(\dot{\sigma } = 0,\) \(\frac{{\partial u^{\prime}}}{\partial \sigma } = 0,\) \(\frac{{\partial v^{\prime}}}{\partial \sigma } = 0\). Using Eq. (3.3), the stream functions \(H,W,R\) in Theorem 2 require the boundary conditions of \(H = W = 0,\frac{\partial R}{\partial \sigma } = 0\), which is of physical significance.

3.2 The three-pattern decomposition model

According to the theorems in Sect. 3.1, at fixed time \(t\), the three-pattern decomposition model in the spherical \(\sigma\)-coordinate system for the global atmospheric circulation can be expressed as:

$$\left\{ {\begin{array}{*{20}l} {\frac{\partial W}{\partial \sigma } - \frac{\partial R}{\partial \theta } = u^{{\prime }} ,} \hfill \\ \\ {\frac{1}{\sin \theta }\frac{\partial R}{\partial \lambda } - \frac{\partial H}{\partial \sigma } = v^{{\prime }} ,} \hfill \\ \\ {\frac{1}{\sin \theta }\frac{\partial (\sin \theta H)}{\partial \theta } - \frac{1}{\sin \theta }\frac{\partial W}{\partial \lambda } = \dot{\sigma },} \hfill \\ \\ {\frac{1}{\sin \theta }\frac{\partial H}{\partial \lambda } + \frac{1}{\sin \theta }\frac{\partial (W\sin \theta )}{\partial \theta } + \frac{\partial R}{\partial \sigma } = 0,} \hfill \\ \end{array} } \right.$$
(3.6)

or in a compactly equivalent form

$$\left\{ {\begin{array}{*{20}l} { - \nabla \times \vec{A} = \vec{V}^{{\prime }} ,} \hfill \\ {\nabla \cdot \vec{A} = 0.} \hfill \\ \end{array} } \right.$$
(3.7)

Using Theorem 2, applying vorticity operation on the first equation in Eq. (3.7) and fitting the second equation of Eq. (3.7) into it, we can obtain the following three boundary value problems

$$\left\{ {\begin{array}{*{20}l} {\varDelta R = \frac{1}{\sin \theta }\frac{{\partial v^{{\prime }} }}{\partial \lambda } - \frac{1}{\sin \theta }\frac{{\partial (u^{{\prime }} \sin \theta )}}{\partial \theta },\quad (\lambda ,\theta ,\sigma ) \in \varOmega ,} \hfill \\ {\frac{\partial R}{\partial n}|_{\partial \varOmega } = \frac{\partial R}{\partial \sigma }|_{\sigma = 1} = 0,} \hfill \\ \end{array} } \right.$$
(3.8)
$$\left\{ {\begin{array}{*{20}l} {\frac{\partial H}{\partial \sigma } = \frac{1}{\sin \theta }\frac{\partial R}{\partial \lambda } - v^{{\prime }} ,\quad (\lambda ,\theta ,\sigma ) \in \varOmega ,} \hfill \\ {H|_{\partial \varOmega } = H|_{\sigma = 1} = 0,} \hfill \\ \end{array} } \right.$$
(3.9)
$$\left\{ {\begin{array}{*{20}l} {\frac{\partial W}{\partial \sigma } = \frac{\partial R}{\partial \theta } + u^{{\prime }} ,\quad (\lambda ,\theta ,\sigma ) \in \varOmega ,} \hfill \\ {W|_{\partial \varOmega } = W|_{\sigma = 1} = 0,} \hfill \\ \end{array} } \right.$$
(3.10)

where \(\varOmega\) is a sphere with \(\lambda\) and \(\theta\) being the longitude and colatitude respectively and radius of \(\sigma = 1\), \(\partial \varOmega\) is the sphere surface, \(n\) is the unit outer normal vector of \(\partial \varOmega\). The differential operator \(\varDelta = \frac{1}{{\sin^{2} \theta }}\frac{{\partial^{2} }}{{\partial \lambda^{2} }} + \frac{1}{\sin \theta }\frac{\partial }{\partial \theta }(\sin \theta \frac{\partial }{\partial \theta }) + \frac{{\partial^{2} }}{{\partial \sigma^{2} }}\) is the 3D Laplacian in the spherical \(\sigma\)-coordinate system.

Note that if stream function \(R\) satisfying Eq. (3.8) is known, \(H,W\) can be expressed as

$$W = \int_{1}^{\sigma } {\left( {\frac{\partial R}{\partial \theta } + u^{{\prime }} } \right)d\sigma } ,$$
(3.11)
$$H = \int_{1}^{\sigma } {\left( {\frac{1}{\sin \theta }\frac{\partial R}{\partial \lambda } - v^{{\prime }} } \right)d\sigma } ,$$
(3.12)

Therefore, solving Eq. (3.8) will help us to obtain all of the 3D stream functions \(H,W,R\) defined in Sect. 2. The problem of (3.8)–(3.10) are called the definite solution problems corresponding to the three-pattern decomposition model (3.6) or (3.7) for global large-scale atmospheric circulation. The existing mathematical theorems (Taylor 1999) can be used to prove the existence and uniqueness of the solutions to the definite solution problems (3.8)–(3.10).

4 The climate characteristics of three-pattern circulations

As we discussed above, the three-pattern decomposition of global atmospheric circulation will yield unique results of physical significance. In this section, we use the NCEP/NCAR reanalysis data to verify our models. The NCEP/NCAR data returns the monthly mean of zonal wind \(u\), meridional wind \(v\) and vertical velocity \(\omega\) at isobaric surface, with a spatial resolution of \(2.5^{0} \times 2.5^{0}\) from January, 1948 to December, 2011. We firstly fit the zonal wind \(u\) and meridional wind \(v\) into the definite solution problem (3.8)–(3.10) to obtain the monthly mean stream functions \(R,H,W\) for the period from January, 1948 to December, 2011. We then obtain the numerical results of the monthly mean of the horizontal circulation \(\vec{V}_{R}\) [components \(u_{R}\) and \(v_{R}\) are computed by Eqs. (2.4) and (2.11)], meridional circulation \(\vec{V}_{H}\) [components \(v_{H}\) and \(\omega_{H}\) are computed by Eqs. (2.4) and (2.17)], zonal circulation \(\vec{V}_{W}\) [components \(u_{W}\) and \(\omega_{W}\) are computed by Eqs. (2.4) and (2.22)] also for the period from January, 1948 to December, 2011. Note that we use the transformation Eq. (2.4) to convert the results into that in the \(p\)-coordinate system. Therefore, the horizontal velocities \(u_{R} ,u_{W} ,v_{R} ,v_{H}\) have the unit of \(m\,s^{ - 1}\), while the vertical velocities \(\omega_{H} ,\omega_{W}\) have the unit of \({\text{Pa}}\,{\text{s}}^{ - 1}\). The stream functions \(R,H,W\) have the same unit \(s^{ - 1}\) as that in the \(\sigma\)-coordinate system. The unit for the calculated stream functions \(R,H,W\) is \(10^{ - 6} \,{\text{s}}^{ - 1}\).

To expose the difference between the stream functions \(R,H,W\) derived from the three-pattern decomposition model with the traditional ones, we provide the definitions of \(\psi_{R}\), \(\psi_{H}\) (Oort and Yienger 1996), \(\psi_{W}\) (Yu and Boer 2002; Yu and Zwiers 2010):

$$\varDelta_{2} \psi_{R} = \zeta_{z} ,$$
(4.1)
$$\psi_{H} = \frac{2\pi a\cos \varphi }{g}\int_{0}^{p} {[v]dp} ,$$
(4.2)
$$\psi_{W} = \frac{2\pi a}{g}\int_{0}^{p} {u_{div} dp} ,$$
(4.3)

where \(\psi_{R}\), \(\psi_{H}\) and \(\psi_{W}\) represent the traditional stream functions of the horizontal, meridional and zonal circulation respectively, \(\varDelta_{2} = \frac{1}{{a^{2} \cos^{2} \varphi }}\frac{{\partial^{2} }}{{\partial \lambda^{2} }} + \frac{1}{{a^{2} \cos \varphi }}\frac{\partial }{\partial \varphi }(\cos \varphi \frac{\partial }{\partial \varphi })\) is the 2D Laplacian in the spherical coordinate system, \(\zeta_{z} = \frac{1}{a\cos \varphi }\frac{\partial v}{\partial \lambda } - \frac{1}{a\cos \varphi }\frac{\partial (u\cos \varphi )}{\partial \varphi }\) is the vertical vorticity, \(a\) is the radius of earth, \(g\) is the gravitational acceleration, \(\varphi\) is the latitude, \(p\) is the atmospheric pressure, \(u_{div}\) is the zonal divergent wind and \([v]\) is the zonal average of meridional wind. According to Eqs. (2.4), (2.8) and (2.11), \(u_{R} = au_{R}^{{\prime }}\) and \(v_{R} = av_{R}^{{\prime }}\) are the horizontal vortex winds which are non-divergent. Therefore, according to Eq. (2.11), we have

$$u_{R}^{{\prime }} = \frac{{u_{R} }}{a} = \frac{{u_{rot} }}{a} = - \frac{\partial R}{\partial \theta },\quad v_{R}^{{\prime }} = \frac{{v_{R} }}{a} = \frac{{v_{rot} }}{a} = \frac{1}{\sin \theta }\frac{\partial R}{\partial \lambda },$$
(4.4)

where \(u_{rot}\) and \(v_{rot}\) are the zonal and meridional vortex wind, respectively. Using Eq. (2.4), and inserting Eqs. (4.4) to (3.11) and (3.12), we have

$$H = \int_{1}^{\sigma } {\left( {\frac{{v_{rot} }}{a} - \frac{v}{a}} \right)d\sigma } = - \frac{1}{a}\int_{1}^{\sigma } {v_{div} d\sigma } ,$$
(4.5)
$$W = \int_{1}^{\sigma } {\left( { - \frac{{u_{rot} }}{a} + \frac{u}{a}} \right)d\sigma } = \frac{1}{a}\int_{1}^{\sigma } {u_{div} d\sigma } .$$
(4.6)

A comparison of Eqs. (4.1) and (3.8) indicates that the horizontal stream function \(R\) is calculated from the whole layer of horizontal vortex wind. \(R\) at the isobaric surface is resulted from the interaction between horizontal vortex winds at different layers in the vertical direction. However, \(\psi_{R}\) from Eq. (4.1) is the 2D stream function from a particular layer of 2D horizontal vortex wind. Similarly, from a comparison of Eqs. (4.2) and (4.5), or (4.3) and (4.6), \(H\) and \(W\) are 3D functions while the traditional stream functions \(\psi_{H}\) and \(\psi_{W}\) are 2D functions in spatial coordinate. They have similar mathematical forms, except a difference of factors \(- \frac{{2\pi a^{2} }}{g}\) (the minus sign is due that the vectors \(\vec{j}\) corresponding to latitude \(\varphi\) and colatitude \(\theta\) are opposite) and \(\frac{{2\pi a^{2} \cos \varphi }}{g}\). Therefore, the traditional 2D stream functions are specific to the 3D stream functions we proposed.

4.1 Global distribution characteristics of stream functions

4.1.1 Horizontal circulation

The horizontal circulation can be considered as the global generalization of the Rossby wave that usually dominates the middle-high latitudes. The climatological-mean of the stream function \(R\) in January and July are shown in Fig. 1a, b, which indicates that there exists a low pressure center surrounding the North Pole in summer, winter and the transition seasons (plots in the transition seasons are not provided). There also exist oscillations along the latitudinal circles around the low pressure center. In the southern hemisphere, the stream function \(R\) is smooth, and the oscillations are far less obvious than that in the northern hemisphere.

Fig. 1
figure 1

The climatological-mean of stream function \(R\) in a January and b July (1948–2011), c and d are the same as a and b but here for the traditional stream function \(\psi_{R}\) calculated from NCEP/NCAR reanalysis data by Eq. (4.1). The unit of \(R\) is 10−6 s−1, and the unit of \(\psi_{R}\) is 108 m2 s−1

Full size image

There are three major troughs at middle latitude of northern hemisphere in winter (Fig. 1a), located at the east coast of the Asia continent (corresponding to East Asia major trough), the east coast of American continent (corresponding to American trough) and the east part of Europe. The last one is the weakest. At July in summer, the horizontal circulation at the lower troposphere exhibits obvious characteristics (Fig. 1b). Firstly, the major trough at eastern Europe extends to Arabia peninsula and the Indian subcontinent near 15°N. Secondly, the horizontal circulation is a high pressure system of anticyclone type in the Pacific Ocean, the Atlantic Ocean and the tropical western Indian Ocean, it is also a high pressure system of anticyclone type in the Pacific Ocean and South American continent near 15°S. These results indicate that the stream function \(R\) defined in this study can reveal the major characteristics of the large-scale horizontal circulations at not only the middle-high latitudes, but also the low latitude tropical regions. This result is significant for studying the global evolution of horizontal circulations, and especially for investigating the interaction between the horizontal circulation at middle-high latitudes and the vertical circulations at low latitudes.

Comparing \(R\) and \(\psi_{R}\), the mean state of the stream function \(R\) shown in Fig. 1a, b agree quite well with the traditional stream function \(\psi_{R}\) (see Fig. 1c, d), which confirms the validity of our three-pattern decomposition model.

4.1.2 Meridional circulation

The meridional circulation can be considered as the global generalization of the Hadley circulation. The global climatological-mean zonal mean of the stream function \(H\) in January and July is shown in Fig. 2a, b, and its global distribution characteristics are shown in Fig. 3. Figure 2c, d shows the distribution of traditional mass stream function \(\psi_{H}\) for January and July. Comparing Fig. 2a–d, we find that the pattern of the stream function \(H\) and \(\psi_{H}\) is almost identical. For both of them, in the northern hemisphere, the meridional circulation with moving upwards (downwards) at low latitude and downwards (upwards) at high latitude happens in the region where the stream function \(H\) or \(\psi_{H}\) is positive (negative), while it is the opposite in the southern hemisphere. Figure 2 indicates that there exist three meridional circulations in both the northern and southern hemispheres, i.e., the traditional Hadley circulation, Ferrel circulation and the Polar circulation. In January, the Hadley circulation in the northern hemisphere is the strongest, moving upwards at the equator and southern tropical areas and downwards at the northern subtropical latitudes, but it is weak in the southern hemisphere, covering a small region. In July, the Hadley circulation is weak in the northern hemisphere with shrinking covered region, but it is strongest in the southern hemisphere, moving upwards at the equator and northern tropical areas and downwards at the southern subtropical latitudes. In addition, the centers of the meridional circulations are shifting to south or north for different seasons and their intensity also strongly depends on the seasons.

Fig. 2
figure 2

The climatological-mean zonal mean of stream function \(H\) in a January and b July (1948–2011), c and d are the same as a and b but here for traditional mass stream function \(\psi_{H}\) calculated from the NCEP/NCAR reanalysis data by Eq. (4.2). The unit of \(H\) is 10−6 s−1, and the unit of \(\psi_{H}\) is 109 kg s−1

Full size image
Fig. 3
figure 3

The climatological-mean of stream function \(H\) in a January and b July (1948–2011), where the unit of \(H\) is 10−6 s−1

Full size image

In Fig. 3, there are four major meridional circulations in winter located at the regions of European continent, east coast of Asia continent, north America and the north Atlantic Ocean in the areas above 30°N. In winter at the tropical regions, the meridional circulation is of Hadley type, moving upwards at low latitude and downwards at high latitude, which is the opposite in the regions of east Pacific Ocean and Atlantic Ocean. In summer, the intensity of the meridional circulation in the northern hemisphere decreases and it is shifting towards north; while at the regions of east Pacific Ocean the Hadley type circulation is originated from the meridional circulation moving in the opposite direction in winter. These results indicate that the meridional circulation is strongly dependent on the season for both the low latitudes and middle-high latitudes.

Compared to the traditional way of zonal mean (Fig. 2c, d), the global distribution of the meridional circulations is more obviously displayed in Fig. 3. An obvious feature is that the meridional circulation is stronger in winter and occupying larger area than that in summer. There exist a few large closed centers which exhibit interval distribution depending on the direction of circulations, probably due to the difference of thermodynamics between oceans and continents in the corresponding regions. The above analysis demonstrates that the stream function \(H\) can reveal the evolution characteristics of local Hadley circulations more appropriately than the traditional analysis, offering new routes to study the local Hadley circulations.

4.1.3 Zonal circulation

The zonal circulation can be considered as the global generalization of the Walker circulation. The climatological-mean meridional-mean of the stream function \(W\) in January and July between 10°N and 10°S is shown in Fig. 4a, b, while its global distribution is shown in Fig. 5. Figure 4c, d shows the distribution of traditional mass stream function \(\psi_{W}\) between 10°N and 10°S for January and July. Comparing Fig. 4a–d, we find that the pattern of \(W\) and \(\psi_{W}\) is almost identical. For both of them, the zonal circulation is moving upwards in the east and downwards in the west of the eastern hemisphere (called anti-Walker type) in the region where the stream function \(W\) or \(\psi_{W}\) is negative while it is moving upwards in the west and downwards in the east of the western hemisphere in the region where the stream function \(W\) or \(\psi_{W}\) is positive (called Walker type).

Fig. 4
figure 4

The climatological-mean meridional-mean of stream function \(W\) between 10°N and 10°S in a January and b July (1948–2011), c and d are the same as a and b but here for traditional mass stream function \(\psi_{W}\) calculated from the NCEP/NCAR reanalysis data by Eq. (4.3). The unit of \(W\) is 10−6 s−1, and the unit of \(\psi_{W}\) is 1011 kg s−1

Full size image
Fig. 5
figure 5

The climatological-mean of stream function \(W\) in a January and b July (1948–2011), where the unit of \(W\) is 10−6 s−1

Full size image

There exist a few zonal circulations in all seasons near the equator shown in Fig. 4. Among them, the one with upwards movement at the west pacific ocean and downwards movement at east Pacific Ocean is usually called Walker circulation; the one with upwards movement at Indonesia and west Pacific Ocean and downwards moment at Africa and west Indian Ocean is called anti-Walker circulation. Note that the zonal circulation around the equator strongly depends on seasons, as shown in Fig. 4. In January, the Walker circulation across the Pacific Ocean around the equator is the strongest and the zonal circulation in South America and Atlantic Ocean is also strong, while it is weak in Africa and Indian Ocean. In July, the Walker circulation in the Pacific Ocean near the equator is weakened while its strength increases in Africa and Indian Ocean with the circulation center moving towards west.

The global distribution of the zonal circulations shown in Fig. 5 is more thorough compared to the result from the traditional meridional averaging (Fig. 4c, d). The zonal circulations mainly exist at low latitudes between 30°N and 30°S in January (Fig. 5). Except the Walker circulation in the east Pacific Ocean around the equator, there are two anti-Walker circulations between the equator and 30°S. One is the zonal circulation across the whole eastern hemisphere centered at Indonesia, Indian Ocean and Africa continent, while the other one is centered in the South America. The region between 15°N and 30°N is dominated by the Walker type circulation, with the strongest one centered at the east coast of China. In July, the zonal circulations shift towards north at the area north of the equator. The Walker circulations in the Pacific Ocean near the equator increase the covering area. The anti-Walker circulations in Indonesia and Indian Ocean shrink the covering area and enhance the strength.

4.2 Global distribution of kinetic energy

In Sect. 4.1, the climate characteristics of the stream functions \(R,H,W\) are in qualitative agreement with the observations. This is an evidence that our generalizations of the traditional Rossby circulation, Hadley circulation and Walker circulation and the three-pattern decomposition model are appropriate. The physical nature of the three-pattern circulations is still not fully understood, as compared to the traditional circulations. We will provide an interpretation based on the calculation of the kinetic energies of those circulations from the NCEP/NCAR reanalysis data.

The annual mean of the kinetic energy for three-pattern circulations is shown in Fig. 6. The formula for the kinetic energy of the horizontal circulation in Fig. 6a, the meridional circulation in Fig. 6b and the zonal circulation in Fig. 6c are

$$E_{a} = \frac{1}{2}(u_{R}^{2} + v_{R}^{2} ),$$
(4.7)
$$E_{b} = \frac{1}{2}(v_{H}^{2} + w_{H}^{2} ),$$
(4.8)

and

$$E_{c} = \frac{1}{2}(u_{W}^{2} + w_{W}^{2} ),$$
(4.9)

respectively, where \(w_{H} = - \frac{{\omega_{H} }}{\rho g}\) and \(w_{W} = - \frac{{\omega_{W} }}{\rho g}\), representing the vertical velocity for the meridional and zonal circulations respectively. The kinetic energy for the traditional circulations are calculated from the zonal wind \(u\), meridional wind \(v\) and vertical velocity \(\omega\) from the NCEP/NCAR reanalysis data, shown in Fig. 6d–f. The formula for the kinetic energy in Fig. 6d–f are

$$E_{d} = \frac{1}{2}(u^{2} + v^{2} ),$$
(4.10)
$$E_{e} = \frac{1}{2}(v^{2} + w^{2} ),$$
(4.11)

and

$$E_{f} = \frac{1}{2}(u^{2} + w^{2} ),$$
(4.12)

respectively, where \(w = - \frac{\omega }{\rho g}\) is the vertical velocity. The kinetic energy calculated from Eqs. (4.10) to (4.12) in Fig. 6d–f exhibits only the Rossby wave type characteristics, not for Walker or Hadley circulations. However, the kinetic energy from Eqs. (4.7) to (4.9) using the six velocity fields \(u_{R}\), \(v_{R}\), \(v_{H}\), \(\omega_{H}\), \(u_{W}\), \(\omega_{W}\) from the three-pattern decomposition model shown in Fig. 6a–c can reveal the major characteristics of the horizontal, meridional and zonal circulations. The annual mean kinetic energy of the horizontal circulation is mainly located at middle-high latitudes, shown in Fig. 6a. Except at low latitudes, the kinetic energy of the meridional circulation is also located at high latitudes, shown in Fig. 6b. The kinetic energy for the zonal circulation is mostly distributed in the Indian Ocean and east Pacific Ocean at low latitudes, shown in Fig. 6c. The kinetic energy for the meridional and zonal circulations is much less than that of the horizontal circulation, with that of the meridional circulation being the smallest.

Fig. 6
figure 6

The climate characteristics of the annual mean of the kinetic energy (500 hPa, 1948–2011) (The unit is \({\text{m}}^{2} \,{\text{s}}^{ - 2} \,1\,{\text{kg}}\), a–c are plotted by the three-pattern decomposition model, while d–f are plotted by the NCEP/NCAR reanalysis data.)

Full size image

In addition, we also study the variance of the monthly and annual mean kinetic energy for the three-pattern circulations and the ratio between the variance and kinetic energy. We conclude that the variance of the kinetic energy of the horizontal circulation concentrates at middle-high latitudes for either the monthly or annual mean, however, the ratio between the variance and the kinetic energy shows the opposite behavior, i.e., larger ratio at low latitudes than that at middle-high latitudes. This indicates that the ratio between the variance and kinetic energy is a strong signal, though the horizontal circulation itself at low latitudes is weaker than middle-high latitudes. Therefore, to study the abnormal behavior of the large-scale circulations at low latitude, the role played by the horizontal circulation should not be overlooked.

5 Conclusions and discussions

The atmosphere around the rotating earth is restricted by thermal equilibrium at middle-high latitudes and by the angular momentum conservation at tropical areas, which requires that the earth atmosphere is quasi-horizontal and quasi-geostrophic and is of Rossby type circulation at middle-high latitudes, while it is overturning and is of Hadley and Walker type circulations at the tropical areas. In order to study the 3D characteristics of the large-scale circulations both at middle-high latitudes and low latitudes, we firstly generalize the concepts of the Rossby wave at middle-high latitude and the Hadley and Walker circulations at low latitudes and quantitatively define the horizontal, meridional and zonal circulations. We then decompose the global atmospheric circulations into these three orthogonal circulations and establish the three-pattern decomposition model for the global circulations. We also provide the theorems for such decomposition to ensure its physical validity. By inputting the NCEP/NCAR reanalysis data into the three-pattern decomposition model, we obtain the climate characteristics of the three-pattern circulations which demonstrate that our decomposition model agrees well with the observations. Furthermore, the characteristics of kinetic energy of the three-pattern circulations in this paper indicate that our model is more accurate to capture the major features of global 3D circulations than the traditional circulation definitions.

The three-pattern decomposition of global atmospheric circulation introduced in this paper can only be applied to 3D atmospheric circulations. For the first time, it decomposes the global circulation into three orthogonal ones on the horizontal, meridional and zonal planes. This provides new methods to study the complicated interactions between all these circulations and establishes novel theoretical foundations to investigate the climate change and extreme weather caused by the evolution of the large-scale circulations. In addition, the three-pattern decomposition model can describe the major characteristics of the local circulations, offering new opportunities to study the atmospheric circulations. Nonetheless, we need to point out fitting the velocity fields \(u,v,\omega\) into Eq. (2.5) will yield inconsistent result, since the unit of \(u,v\) is \({\text{m}}\,{\text{s}}^{ - 1}\) while the unit of \(\omega\) is \({\text{Pa}}\,{\text{s}}^{ - 1}\) and they cannot be added together. To overcome this difficulty, we introduce the \(\sigma\)-coordinate system, i.e., \(\sigma = \frac{{p - p_{0} }}{{P_{s} - p_{0} }}\), where \(P_{s} = 1000\,{\text{hPa}}\) and \(p_{0} = 0\,{\text{Pa}},\) so that the velocity fields \(u^{{\prime }} ,v^{{\prime }} ,\dot{\sigma }\) in the coordinate transformation Eq. (2.4) have the same unit of \({\text{s}}^{ - 1}\). Note that \(P_{s} = 1000\,{\text{hPa}}\) means that we neglect the effect of the earth’s topographic features in our decomposition model. Thus, we should use the velocity fields \(u,v\) and \(\omega\), which include the effect of earth’s topography, in the three-pattern decomposition model when we need considering the earth’s topographic features.

This paper and its sequel present the complete theory of the three-pattern decomposition of global atmospheric circulation. The detailed diagnosis and analysis about the three-pattern circulations will be discussed in a separate paper.