1 Introduction

Quantum entanglement has garnered significant attention for its pivotal role in the realm of quantum information and computation. It offers a plethora of promising applications, including quantum teleportation [1], quantum remote preparation [2, 3], quantum key distribution [4], and algorithms for quantum computations [5]. As a pure quantum correlation [6, 7], quantum entanglement stands as the fundamental distinction between quantum and classical physics [8]. In the context of the quantum ground state in condensed matter physics, it becomes imperative to explore the correlation between quantum entanglement and quantum phase transitions (QPT). These transitions typically occurr at zero temperature or under extreme cryogenic conditions, where thermal fluctuations freeze [9,10,11,12], rendering them of paramount importance.

Recently, considerable attention has been devoted to exploring the relationship between quantum entanglement and QPT in Heisenberg spin models. Specifically, researchers have investigated the bipartite entanglement of the system, leveraging the quantum renormalization-group (QRG) method [13,14,15,16,17,18]. This method serves as a refined analytic tool, providing insights into the phase analysis of the model, even for scenarios beyond exact solutions. Consequently, QRG stands out as an effective methodology for investigating the quantum behavior associated with the QPT [19,20,21,22]. The versatility of the QRG method extends to efficiently revealing the dynamical properties of multi-body systems. Furthermore, it serves as a potent numerical tool for studying the properties of ground and low-lying states within the framework of low-dimensional lattice models. Notably, the implementation of the QRG method has proven instrumental in detecting scaling behaviors and nonanalytic features near critical points in various physical systems [23,24,25,26,27]. In this work, our focus will be on uncovering QPT through the utilization of multipartite entanglement, leveraging this effective method in spin models.

On the other hand, spin models can be enhanced by incorporating a magnetic term known as the Dzyaloshinskii–Moriya (DM) interaction. Importantly, a quantum spin model, being a type of multipartite system, holds promise for constructing quantum computer. Concurrently, the DM interaction, stemming from spin-orbit coupling, consistently influences the ground state structure in the quantum spin model and plays a pivotal role in QPT. The DM interaction was originally proposed by Dzyaloshinskii and Moriya about half a century ago [28, 29], arising from spin-orbit coupling. Recent studies have reported Ising and XXZ models with the DM interaction, denoted by the form \(\overrightarrow{D} \cdot (\overrightarrow{{S_i}} \times \overrightarrow{{S_j}} )\) for two spins \(\overrightarrow{{S_i}}\) and \(\overrightarrow{{S_j}}\), as cited in references [30,31,32,33,34,35].

For a quantum system, critical points can be categorized into two phases: the spin-fluid phase and the Néel phase. It has been observed that the DM interaction can induce quantum fluctuations, leading to a phase transition in the spin model. The DM interaction is fundamentally crucial for observing ferromagnetism, as investigated in reference [36, 37]. Of course, the most straightforward approach to studying the effect of staggered DM interaction on the system’s ground state is to gauge out the DM interaction [38]. However, it is worth noting that detecting the nonanalytic behavior of order parameters near critical points in a quantum spin system is challenging without the application of a sophisticated formulation scheme. In this context, the QRG method has proven to be a valuable and convenient tool for the rigorous treatment of QPT. Highly regarded examples [30, 31, 39,40,41,42] dealing with nonanalytic behaviors near critical points for numerous systems are available. These advancements open up the possibility of utilizing the QRG method for describing multipartite entanglement in QPT. Consequently, the dynamics of multipartite entanglement emerge as a crucial topic. Notably, multipartite entanglement has found applications in detecting QPT [43,44,45,46,47]. It is only natural for us to employ multipartite entanglement to investigate quantum critical properties in the vicinity of the critical points of spin systems.

Motivated by the considerations mentioned above, the primary focus of our work is a dedicated exploration of both global entanglement (GE) [48, 49] and tripartite negativity [50, 51] as indicators of multipartite entanglement in multipartite systems, alongside an in-depth investigation of QPT in the XY model incorporating the DM interaction, utilizing the QRG method. Our inquiry leads us to discern both stable and unstable fixed points within the system. Remarkably, we observe that as the strength of the DM interaction intensifies, the phase transition point becomes dynamically variable. Notably, both GE and tripartite negativity tend to converge toward two distinct fixed values corresponding to the different phases of the system as the number of the QRG iterations increases. Additionally, our investigation yields insights into the nonanalytic phenomenon and scaling behavior of the first derivative for this system precisely at the critical point, establishing a significant relationship with the correlation length.

The structure of this paper is outlined as follows: In Sect. 2, we present the introduction of the QRG spin-1/2 XY model with the DM interaction. The investigation into the dynamical properties of renormalization multipartite entanglement is undertaken in Sect. 3. And then the Sect. 4 delves into a detailed disclosure of the scaling behavior and nonanalytic phenomena. Lastly, a brief conclusion is provided in the final section of the text.

2 The XY model

The Hamiltonian for the one-dimensional anisotropic spin-1/2 XY model with staggered Dzyaloshinskii-Moriya interaction on a periodic chain of N sites is expressed as

$$\begin{aligned} \begin{aligned} \begin{array}{l} {H_0} = \frac{J}{4}\sum \limits _{i = 1}^N {[(1 + \Delta )\sigma _i^x\sigma _{i + 1}^x + (1 - \Delta )\sigma _i^y\sigma _{i + 1}^y} \mathrm{{ }}\\ \mathrm{{ }} + {( - 1)^i}D(\sigma _i^x\sigma _{i + 1}^y - \sigma _i^y\sigma _{i + 1}^x)], \end{array} \end{aligned} \end{aligned}$$
(1)

where J is the nearest exchange coupling constant,\(\Delta\) is the anisotropy parameter, D is the strength of z component of the DM interaction, and \(\sigma _i^{x,y}\) are Pauli matrices at site i. Notably, the model becomes the isotropic XX model when \(\Delta = D = 0\), falls within the Ising universality class when \(0 < \Delta \le 1\).

The ground states of this model are doubly degenerate, providing a useful basis for constructing a projection operator and quantifying entanglement. The self-similarity of the Hamiltonian after each QRG step is crucial, necessitating the division of the spin chain into three-site blocks. The degenerate ground states are represented by

$$\begin{aligned}{} & {} \left| {{\psi _0}} \right\rangle = \frac{{\sqrt{2(1 + {D^2})} }}{{2q}}\left[ {\frac{{(1 - Di)\Delta }}{{1 + {D^2}}}\left| { \uparrow \downarrow \uparrow } \right\rangle } \right. \nonumber \\{} & {} \quad \mathrm{{ }}\left. { - \frac{{\sqrt{2} q(1 - Di)}}{{2(1 + {D^2})}}\left| { \uparrow \uparrow \downarrow } \right\rangle - \frac{{\sqrt{2} q(1 - Di)}}{{2(1 + {D^2})}}\left| { \downarrow \uparrow \uparrow } \right\rangle + \left| { \downarrow \downarrow \downarrow } \right\rangle } \right] , \end{aligned}$$
(2)
$$\begin{aligned}{} & {} \quad \left| {\psi _0'} \right\rangle = \frac{1}{2}\left( {\left| { \downarrow \downarrow \uparrow } \right\rangle + \left| { \uparrow \downarrow \downarrow } \right\rangle } \right) \nonumber \\{} & {} \quad - \frac{{\sqrt{2} (1 + {D^2})}}{{2(1 + Di)q}}\left| { \uparrow \uparrow \uparrow } \right\rangle - \frac{{\sqrt{2} \Delta }}{{2q}}\left| { \downarrow \uparrow \downarrow } \right\rangle , \end{aligned}$$
(3)

here, \(\left| \downarrow \right\rangle\) and \(\left| \uparrow \right\rangle\) are the basis vectors of \({\sigma ^z}\) in the spin-1/2 representation, and \(q = \sqrt{1 + {D^2} + {\Delta ^2}}\). The effective Hamiltonian can be given by [51,52,53]

$$\begin{aligned} \begin{aligned} \begin{array}{l} {H^{eff}} = \frac{{J'}}{4}\sum \limits _{k = 1}^{{N / 3}} {[(1 + \Delta ')\sigma _k^x\sigma _{k + 1}^x} \\ - (1 - \Delta ')\sigma _k^y\sigma _{k + 1}^y + D'(\sigma _k^x\sigma _{k + 1}^y + \sigma _k^y\sigma _{k + 1}^x)] \end{array} \end{aligned} \end{aligned}$$
(4)

where the iterative relationships governing the effective parameters are

$$\begin{aligned} \begin{aligned} \begin{array}{l} J' = \frac{{1 + {D^2} + 3{\Delta ^2}}}{{2{q^2}}}J,\mathrm{{ }}\\ \Delta ' = \frac{{3\Delta + 3{D^2}\Delta + {\Delta ^3}}}{{1 + {D^2} + 3{\Delta ^2}}},\mathrm{{ }}D' = - D. \end{array} \end{aligned} \end{aligned}$$
(5)

Due to the antisymmetric property of the DM interaction \({\overrightarrow{D} _{i,j}}\mathrm{{ = }} - {\overrightarrow{D} _{j,i}}\)), the stable and unstable fixed points can be determined by solving the equation \(\Delta ' = \Delta\). The stable fixed points correspond to \(\Delta \mathrm{{ = }}\sqrt{1\mathrm{{ + }}{D^2}}\) and \(\Delta \rightarrow \infty\), aligning with distinct phases of the system. The unstable fixed point is \(\Delta = 0\), which is indicate the phase transition point. Thus, it essentially divides the system into the spin-fluid phase ( \(\Delta = 0\) and \(\Delta \rightarrow \infty\)) and the Néel phase (\(0< \left| \Delta \right| < \sqrt{1 + {D^2}}\)).

3 Renormalization of multipartite entanglement

The global entanglement is introduced by Meyer and Wallach [54] as a measure to quantify multipartite entanglement. Specifically, for a given state \(\left| \varphi \right\rangle\) describing an N-body system, the GE can be expressed as [48]

$$\begin{aligned} \begin{aligned} GE\left( {\left| \varphi \right\rangle } \right) = 2\left[ {1 - \frac{1}{N}\sum \limits _{i = 1}^N {\mathrm{{Tr}}(\rho _i^2)} } \right] , \end{aligned} \end{aligned}$$
(6)

where \({\rho _i}\) represents a reduced density matrix traced over all parties except the i-th one, and the GE is restricted to the scope of \(0 \le GE \le 1\). Importantly, \(GE\left( {\left| \varphi \right\rangle } \right) = 0\) if and only if \(\left| \varphi \right\rangle\) is a product state, and \(GE\left( {\left| \varphi \right\rangle } \right) = 1\) if the systemic state is a maximally entangled pure one.

Furthermore, negativity serves as another computable measure of entanglement. Vidal and Werner introduced bipartite negativity as the first measure to quantify the entanglement of any bipartite state [55]. Related to the Peres-Horodecki criterion, the definition of bipartite negativity can be expressed as [50]

$$\begin{aligned} \begin{aligned} {N_{12}}\left( {{\rho _{AB}}} \right) = 2\sum \limits _i {\mathrm{{max}}} \left( 0, - {\lambda _i}\right) , \end{aligned} \end{aligned}$$
(7)

where \({\lambda _i}\) are the negative eigenvalues of the partial transpose \({\rho ^{TA}}\) of the total state \({\rho }\) respect to the subsystem A. We can extend this definition to a tripartite system (e.g., \({\rho _{ABC}}\) ) by taking a geometric average of all appropriately bipartite systems, resulting in the tripartite negativity [50, 51]

$$\begin{aligned} \begin{aligned} {N_{123}}\left( {\rho _{ABC}}\right) = \mathrm{{ }}{\left\{ {{N_{12}}\left( {\rho _{A|BC}}\right) {N_{12}}\left( {\rho _{B|AC}}\right) {N_{12}}\left( {\rho _{C|AB}}\right) } \right\} ^{1/3}}, \end{aligned} \end{aligned}$$
(8)

where \({\rho _{I\left| {JK} \right. }}\) is the density matrix of the bipartition \(I-JK\). \({N_{12}}({\rho _{I\left| {JK} \right. }})\) is the bipartite negativity of \({\rho _{I\left| {JK} \right. }}\) (defined by Eq. (7)). In this work, we utilize both GE and tripartite negativity as the measurements of the ground-state multipartite entanglement to demonstrate how the multipartite entanglement reveals quantum critical point in the Heisenberg spin chain.

Later on, we consider one of the degenerate ground states. The density matrix of a ground state can be expressed as follows

$$\begin{aligned} \begin{aligned} \rho = \left| {{\psi _0}} \right\rangle \langle {\psi _0}|, \end{aligned} \end{aligned}$$
(9)

where \(\left| {{\psi _0}} \right\rangle\) is defined as in Eq. (2). Substituting \(\left| {\psi _0'} \right\rangle\) into the density matrix yields the same result. Consequently, we can determine the non-zero elements with respect to the density matrix \(\rho\) of the ground state \(\left| {{\psi _0}} \right\rangle\), as shown below

$$\begin{aligned} \begin{aligned} \begin{array}{l} {\rho _{22}} = {{{\rho _{25}} = {\rho _{52}} = {\rho _{55}} = 1} / 4},\mathrm{{ }}\\ {\rho _{23}} = {\rho _{32}} = {\rho _{35}} = {\rho _{53}} = \frac{{ - \Delta }}{{2\sqrt{2} q}},\\ {\rho _{28}} = {\rho _{58}} = \frac{{ - (1 + Di)}}{{2\sqrt{2} q}},\mathrm{{ }}{\rho _{38}} = \frac{{\Delta (1 + Di)}}{{2{q^2}}},\mathrm{{ }}\\ {\rho _{83}} = \frac{{\Delta (1 - Di)}}{{2{q^2}}},\mathrm{{ }}{\rho _{82}} = {\rho _{85}} = \frac{{Di - 1}}{{2\sqrt{2} q}},\mathrm{{ }}{\rho _{88}} = \frac{{\left( 1 + {D^2}\right) }}{{2{q^2}}}. \end{array} \end{aligned} \end{aligned}$$
(10)

By utilizing Eqs. (6) and (8), the expression for the GE and tripartite negativity can be derived

$$\begin{aligned} \begin{aligned} \begin{array}{l} GE = \frac{{10{q^4} - 3 - 3{D^4} - 2{\Delta ^2} - 3{\Delta ^4}}}{{6{q^4}}}\\ - \frac{{2{q^2}\left( {1 + {\Delta ^2}} \right) + 2{D^2}\left( {3 + {q^2} + {\Delta ^2}} \right) }}{{6{q^4}}} \end{array} \end{aligned} \end{aligned}$$
(11)

and

$$\begin{aligned} \begin{aligned} {N_{123}} = {\left\{ {\frac{{\left( {2 + 2{D^2} + {q^2}} \right) \sqrt{1 + {D^2} + {\Delta ^2}} \left( {{q^2} + 2{\Delta ^2}} \right) }}{{4{q^5}}}} \right\} ^{\frac{1}{3}}}, \end{aligned} \end{aligned}$$
(12)

respectively.

Fig. 1
figure 1

The GE (left) and tripartite negativity (right) as a function of anisotropy for different values of the strength of the DM interaction, respectively

Full size image
Fig. 2
figure 2

The GE (left) and tripartite negativity (right) as a function of the DM interaction in terms of the QRG steps in the XY model at a fixed value for \(\Delta = \sqrt{2}\), respectively

Full size image

Analysis of Fig. 1 reveals a correlation between multipartite entanglements (GE and tripartite negativity) and the strength of the DM interaction and anisotropy parameters. Moreover, both GE and tripartite negativity increase with the strength of the DM interaction when \(\left| \Delta \right|\) is relatively large, indicating that the DM interaction enhances GE and tripartite negativity within this range. Conversely, both GE and tripartite negativity decrease with increasing DM interaction strength D when \(\left| \Delta \right|\) is relatively small, emphasizing that the effect of the anisotropic parameter on multipartite entanglement surpasses that of the DM interaction in this situation. Multipartite entanglements reach the minimum value when the anisotropy parameter is equal to zero, and then the anisotropy parameter can grow GE, however, as GE reaches its maximum, the anisotropy parameter weakens GE. Additionally, it is also noteworthy that multipartite entanglements remain constant when the anisotropy parameter is equal to zero, i.e., \(\Delta =0\), signifying a transition from an anisotropic XY model to an isotropic XX model in the Hamiltonian. Compared to the GE, tripartite negativity is less affected by the DM interaction and anisotropy parameter, indicating that tripartite negativity exhibits greater robustness. Furthermore, the graph illustrates that the variation trend of bight become gently with increasing DM interaction strength D. Thus, one can infer that the DM interaction can enhance multipartite entanglements when \(\Delta > \sqrt{1 + {D^2}}\).

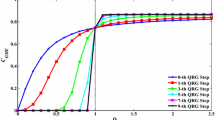
Additionally, we depict the relationship between multipartite entanglements and the DM interaction with an increasing number of the QRG iterations for \(\Delta = \sqrt{2}\) in Fig. 2. The figure indicates that multipartite entanglements increase with increasing DM interaction as \(0< D < 1\), while they decrease for \(D > 1\). Overall, GE reduces with increasing DM interaction, eventually reaching a minimum value. Notably, the attenuation of multipartite entanglements slows down with the increase in the number of QRG iterations, hinting that at \(\Delta = \sqrt{2}\), the variations in DM interaction cannot induce a quantum phase transition. This observation is further supported by the derivative of GE with respect to the DM interaction, as depicted in Fig. 7.

Fig. 3
figure 3

The GE versus \(\Delta\) for different the QRG iterations at a \(D = 0\) and b \(D = 1\). (The inset of diagram shows that the relations between GE and \(\Delta\), when \(\Delta\) ranges from \(- \sqrt{2}\) to \(\sqrt{2}\).) The tripartite negativity versus \(\Delta\) for different the QRG iterations at a \(D = 0\) and b \(D = 1\)

Full size image
Fig. 4
figure 4

(Color online) First derivative of the GE: a \(dGE/d\Delta\) and b dGE/dD, in the parameter space (\(D,\mathrm{{ }}\Delta\)) for a three-site model, respectively. The transition solid lines \(\Delta =0\) and \(\left| \Delta \right| = \sqrt{1 + {D^2}}\) divide the whole space into several parts presenting the different phase in (a)

Full size image

Let us delve into examining the impact of the anisotropic parameter on systemic entanglement with varying the QRG iterations, assuming a fixed DM interaction. In this scenario, the fixed DM interaction strength are set at two constant values, i.e., \(D = 0\) and \(D = 1\), respectively. Specifically, the relationship between multipartite entanglements (GE and tripartite negativity) and the anisotropy parameter \(\Delta\) is illustrated in Fig. 3. The graph reveals that multipartite entanglements initially increase to a maximum and then decrease to a certain value as the anisotropy parameter \(\Delta\) increases. If the number of QRG iterations is sufficiently large, both GE and tripartite negativity tend to approach 1. Furthermore, the analysis demonstrates that as the number of QRG iteration increases, multipartite entanglement of the model is divided into three partitions: (i) Multipartite entanglement is reach a minimum at the point of \(\Delta =0\). (ii) The system transitions to the Néel phase as \(\left| \Delta \right| < \sqrt{2}\), and multipartite entanglement rapidly rises from the minimum value to 1 with the increasing \(\left| \Delta \right|\). (iii) For \(\left| \Delta \right| > \sqrt{2}\), the system corresponds to the spin-fluid phase, and multipartite entanglement gradually decreases from the maximum value to a specific level. Notably, when \(\Delta =0\) and \(D=0\), where the system shifts from an anisotropic XY model to an isotropic XX model. Crucially, one can realize that the system becomes apparent that a quantum phase transition occurs at \(\Delta =0\). Thus, the QRG iteration results on \(dGE/d\Delta\) align with the iterated results on GE, indicating that \(\Delta =0\) rather than \(\Delta = \pm \sqrt{2}\) is the critical phase transition point, and \(\Delta = \pm \sqrt{2}\) can be termed the critical pseudo-phase transition point. This critical point \(\Delta =0\) is consistent with the derived critical point from Fig. 4. Additionally, the stable fixed points are obtained from the infinity of the derivative \(dGE/d\Delta\), as depicted in Fig. 4. Moreover, the color mutation positions represent the transition points, and the transition solid lines \(\Delta =0\) and \(\left| \Delta \right| = \sqrt{1 + {D^2}}\) divide the whole space into several parts presenting the different phase, with corresponding labels in Fig. 4a.

4 Scaling behaviors and nonanalytic phenomenon

For simplicity, we choose global entanglement as the measure of multipartite entanglement to characterize nonanalytic behavior. Our explicit emphasis is on the dynamics of the three-block global entanglement in the one-dimensional spin-1/2 Heisenberg XY model with DM interaction. We aim to elucidate the occurrence of nonanalytic behavior and the absence of a characteristic length scale at the intersection point. This observation is particularly noteworthy as it signifies the manifestation of scaling behavior in the global entanglement around the critical point.

Fig. 5
figure 5

First derivative of the GE \(dGE/d\Delta\) as a function of the anisotropic parameter \(\Delta\) when the number of the QRG iterations increasing, while the \(0< \Delta < \sqrt{2}\) shown in (a) and the \(\Delta > \sqrt{2}\) shown in (b)

Full size image
Fig. 6
figure 6

a, b The logarithm of the absolute value of maximum or minimum, \(\ln \left( {{{\left| {\mathrm{{d}}GE/d\Delta } \right| }_{\max }}} \right)\) and versus the logarithm of chain size \(\ln (N)\), respectively. c, d The logarithm of \(\left| {{\Delta _{\max }} - {\Delta _\mathrm{{c}}}} \right|\) and \(\left| {{\Delta _{\min }} - {\Delta _\mathrm{{c}}}} \right|\) versus the logarithm of chain size N, respectively

Full size image
Fig. 7
figure 7

First derivative of GE dGE/dD versus the DM parameter D in case of different QRG iterations as \(\Delta = \sqrt{2}\)

Full size image

To deepen our investigation, we scrutinize the first derivative of the GE, which provides insight into its nonanalytic behavior at the critical point. This analysis, showcased in Fig. 5, explicitly presents the first derivative of GE with respect to the anisotropy parameter \(\Delta\) for \(D = 1\). Evidently, as the QRG iteration approaches infinity, each plot demonstrates distinct maximum and minimum values, indicating a second-order QPT in the model. Notably, the inset of the diagram reveals a gradual approach to zero for the minimum values as the system size increases. This compellingly establishes the equivalence of properties for \(\Delta = 0\) and \(\Delta \rightarrow \infty\). Further, conducting a meticulous analysis, we unveil the intricate behavior of GE in the one-dimensional XY model with DM interaction. Examining the scaling behavior, we observe a linear correlation between the maximum \(y = {\left| {dGE/d\Delta } \right| _{\max }} \sim {N^a}\) with \(a = 0.86\), and the minimum \(y = {\left| {dGE/d\Delta } \right| _{\min }} \sim {N^b}\) with \(b =-0.95\) of the system as \(D=1\) (as show in Fig. 6a, b). Notably, the positions (i.e., \({\Delta _{\max }}\) and \({\Delta _{\min }}\)) of the maximum and the minimum for \(dGE/d\Delta\) gradually converge towards the critical point with an increase in QRG iterations, as shown in Fig. 6c, d, respectively. Numerical calculations affirm exponents of are \({\Delta _c} - {\Delta _{\max }} = {N^{ - 0.084}}\) and \({\Delta _{\min }} - {\Delta _c} = {N^{0.84}}\), respectively. Thus, the results validate the singular behavior of the global entanglement and the scaling dynamics of the system, aligning with the universality inherent in the model. The outcomes affirm that implementing QRG for multipartite entanglement effectively captures the critical characteristics of the XY model. In summary, we can assert that the derivatives of the GE not only reveal scaling behavior at the critical point but also unveil scaling properties at the stable fixed points. Exploring variations in the DM interaction values, we can similarly trace the evolution of the GE concerning the anisotropy parameter.

Fig. 8
figure 8

a The logarithm of the absolute value of minimum, \(\ln \left( {{{\left| {dGE/dD} \right| }_{\min }}} \right)\) versus the logarithm of chain size \(\ln (N)\). b The logarithm of \(\left| {{D_{\min }} - {D_\mathrm{{c}}}} \right|\) versus the logarithm of chain size N

Full size image

Following that, we further explore the analysis of the first derivative of GE concerning D for \(\Delta =\sqrt{2}\), as depicted in Fig. 7. With an escalation in the number of the QRG iterations, the minimum of the derivative dGE/dD (the position of the minimum of dGE/dD is \({D_{\min }}\)) increases. As the system size approaches infinity, dGE/dD tends toward zero. Moreover, we delve into the scaling behavior of \(y = {\left| {dGE/dD} \right| _{\min }}\) in relation to the system size N for \(\Delta = \sqrt{2}\). The linear relationship is elucidated by \({\left| {dGE/dD} \right| _{\min }}\sim {N^{ - 1.0023}}\) in Fig. 8a. Additionally, the position \({D_{\min }}\) of the minimum of dGE/dD gradually expands with an increase in the QRG iterations, as illustrated in Fig. 8b. It is substantiated that the exponent is \({D_{\min }} - {D_c} = {N^{0.784}}\). It is pertinent to mention that this exponent is correlated with the correlation length exponent close to the critical point, precisely the reciprocal of the correlation exponent.

5 Conclusions

In this work, we have investigated the interplay between multipartite entanglement (both GE and tripartite negativity) and QPT in the spin-1/2 XY model with DM interaction, employing the QRG method. The results reveal that both the anisotropy parameters and the DM interaction exert significant influences on the phase diagrams. Notably, both GE and tripartite negativity approach 1 with an increase in QRG iterations. Besides, when the anisotropy parameter is relatively large, multipartite entanglement becomes increasingly dependent on the DM interaction. Significantly, with an escalation in the number of QRG iterations, the multipartite entanglement manifests itself into two distinct phases: The Spin-fluid phase and the Néel phase. In the Néel phase, the range of values widens as the DM interaction becomes larger. Moreover, at the critical point \(\Delta = 0\), multipartite entanglement effectively demonstrates a quantum phase transition in this model, and the critical behavior is elucidated through the first derivative of the GE of the block (shown in Fig. 4 for details). Additionally, our analysis elucidates the scaling behavior, illustrating how the critical point of this model is approached with an increase in the system’s size. We observe that the critical exponent is correlated with the length exponent near the critical point. Compared to tripartite negativity, GE is more strongly affected by the DM interaction, highlighting the greater robustness of tripartite negativity. Moreover, our in-depth examination indicates a gradual decrease in GE as the system size becomes larger, ultimately converging to a fixed value.

These findings provide compelling evidence that the QRG implementation of multipartite entanglement adeptly captures the critical behavior of the XY model. The derivatives of GE not only unveil scaling behavior at the critical point but also disclose scaling properties at stable fixed points. A similar investigation into the evolution of GE concerning the anisotropy parameter can be applied for other DM interaction strength. Thereby, we believe that studying the behavior of the multipartite entanglement via the method of the QRG could help to observe the quantum critical properties in Heisenberg spin models. In conclusion, our work showcases the nuanced dynamics of quantum entanglement within the spin-1/2 XY model with DM interaction, providing a comprehensive understanding of quantum critical properties. The QRG implementation of multipartite entanglement emerges as a powerful tool for unveiling the intricacies of QPT in Heisenberg spin models. We are confident that our research significantly contributes to the understanding of multipartite entanglement dynamics in condensed matter systems.