Keywords

1 Introduction

Single-layer graphene sheet proffered astounding properties in the area of electronics, bio-medical, electrical, mechanical, and all these are directed to find multitudinous application [1,2,3]. Graphene has extraordinary properties in terms of application compared to other material like phosphorene nanoribbons [4]. In the recent material innovation, graphene has immerged as the strongest material in the field of material engineering as it possesses interesting and extraordinary properties [5, 6]. It is thin too and lightweight with tremendous electrical, optical, and thermal properties which made it versatile material in the application of various fields, such as electronics, aviation, sensors, solar panels, etc. [7,8,9]. Graphene has being become interesting material due to its profound properties like thermal conductivity as 5000 W/mK [10], explored surface area as 2630 m2/g [11], mobility of electron at room temperature as 250,000 cm2/Vs [12], and reasonable electrical conductivity. Graphene has carbon arrangement in a single layer with honeycomb structure which is said hexagons [13].

There have been done many researches on single-layer graphene sheet from 1970 to date which included a study of transverse vibration of graphene [14], torsional vibration, dynamic analysis on graphene [15] sheet. Further research was extended with different boundary conditions and with or without masses attached to graphene sheets to cultivate comprehension of behaviors of graphene sheets against various conditions [16]. There are many researches were completed too regarding various boundary conditions like clamped-pinned-free without mass boundary condition, clamped-pinned with masses boundary condition [17], and a combination of two. One predominant aspect of graphene is observed that to date; there has been very less research completed on configuration modification and effect of variation of configuration on natural frequency and other parameters [18, 19].

2 Modeling Approach

2.1 Analytical Approach

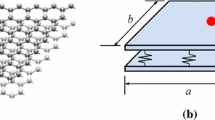
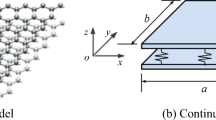
In this study, single phase of the modeling approach is adopted for graphene sheets without defect and zigzag atomic arrangement configuration is employed. It is assumed that graphene is a kind of rectangular plate and so that classical thin plate theory said Kirchoff theory can be applied to it, as graphene has single layer atom arrangement [20]. All the assumptions are taken as same as used in classical plate theory, and finally equilibrium approach has been applied to the rectangular plate. There are various solution methods that are available for getting the equation of motion of rectangular plate but one predominant method is the equilibrium approach to obtain the equation of motion. Here, displacement of graphene is considered in transverse direction, and solution is obtained by recalling Blevin’s solution for natural frequency [21, 22]. Considering the equilibrium approach in ‘Z’ direction, the equation of motion can be obtained as, [22] (Tables 1 and 2).

$$D\left( {\frac{{\partial^{4} w}}{{\partial^{4} x}} + { }2{ }\frac{{\partial^{4} w}}{{\partial^{2} x \times \partial^{2} y}} + \frac{{\partial^{4} w}}{{\partial^{4} y}}} \right) + { }\rho h\frac{{\partial^{2} w}}{{\partial t^{2} }} = 0$$
(1)
Table 1 Properties of graphene
Full size table
Table 2 Dimensions of graphene
Full size table

Solution of the above equation of motion (free vibration) is expressed as below:

$$\begin{aligned} W\left( {x,y} \right) \, & = {\text{ A1 sin}}\alpha x{\text{sin}}\beta y + {\text{ A2 sin}}\alpha x{\text{cos}}\beta y + {\text{ A3 cos}}\alpha x{\text{sin}}\beta y + {\text{ A4 cos}}\alpha x{\text{cos}}\beta y \hfill \\& + {\text{ A5 sinh}}\theta x{\text{sinh}}\emptyset y + {\text{ A6 sinh}}\theta x{\text{cosh}}\emptyset y + {\text{ A7 cosh}}\theta x{\text{sinh}}\emptyset y \hfill \\& + {\text{ A8 cosh}}\theta x{\text{cosh}}\emptyset y \hfill \\ \end{aligned}$$

where, λ2 = α2 + β2 = θ2 + Ø2.

Blevins had formulated a solution for the various boundary conditions for the above equation so recalling the solution of Blevins for finding natural frequency of plate is expressed as, [21, 22].

$$\omega_{ij} = \sqrt {\left\{ {\frac{{\pi^{4} D}}{{\alpha^{4} h\rho }}} \right\}\left\{ {G_{\text{x}}^{4} + G_{\text{y}}^{4} \left( \frac{a}{b} \right)^{4} + 2\left( \frac{a}{b} \right)^{4} \left[ {\vartheta H_{\text{x}} H_{\text{y}} + \left( {1 - \vartheta } \right)J_{\text{x}} J_{\text{y}} } \right]} \right\}}$$
(2)

where \({\text{D Flexural Density}} = \frac{{Eh^{3} }}{{12\left( {1 - \vartheta^{2} } \right)}}\), Gx, Hx, Jx, Gy, Hy, Jy = constants depending on BCs.

For bridged boundary condition (FFCC),

$$\begin{aligned} \omega_{11} {\text{ as}}:G_{{\text{x}}} = { 1}.{5}0{6},H_{{\text{x}}} = { 1}.{248},J_{{\text{x}}} = { 1}.{248},G_{{\text{y}}} = \, 0,H_{{\text{y}}} = \, 0,J_{{\text{y}}} = \, 0 \hfill \\ \omega_{12} {\text{ as}}:G_{{\text{x}}} = { 2}.{5},H_{{\text{x}}} = { 4}.{658},J_{{\text{x}}} = { 4}.{658},G_{{\text{y}}} = \, 0,H_{{\text{y}}} = \, 0,J_{{\text{y}}} = \, 0. \hfill \\ \end{aligned}$$

Sample calculation of (5,5) armchair configuration of rapheme is formulated as:

$${\text{Flexural rigidity }}\left( D \right) = \frac{{Eh^{3} }}{{12\left( {1 - \vartheta^{2} } \right)}} = \frac{{10^{12} \times \left( {3.45 \times 10^{ - 10} } \right)^{3} }}{{12\left( {1 - 0.456^{2} } \right)}} = 4.3203 \times 10^{ - 18} {\text{ N}}.{\text{m}}$$
$$\begin{aligned} \omega_{11} &= \sqrt {\left\{ {\frac{{{\uppi }^{4} \times 4.3203 \times 10^{ - 18} }}{{\left( {10^{ - 9} } \right)^{4} \times 1161 \times 1.7 \times 10 - 10}}} \right\}\left\{ {\begin{array}{*{20}c} {1.506^{4} + 0^{4} \left( {\frac{{10^{ - 9} }}{{2.1315 \times 10^{ - 9} }}} \right)^{4} + 2\left( {\frac{{10^{ - 9} }}{{2.1315 \times 10^{ - 9} }}} \right)^{4} } \\ { \times \left[ {0.456 \times 1.248 \times 0 + \left( {1 - 0.456} \right)1.248 \times 0} \right]} \\ \end{array} } \right\}} \hfill \\& = {6}{\text{.62}} \times {10}^{{{12}}} {\text{ Hz}} \hfill \\ \end{aligned}$$
$$\begin{aligned} \omega_{12} &= \sqrt {\left\{ {\frac{{\pi^{4} \times 4.3203 \times 10^{ - 18} }}{{\left( {10^{ - 9} } \right)^{4} \times 1161 \times 1.7 \times 10 - 10}}} \right\}\left\{ {\begin{array}{*{20}c} {2.5^{4} + 0^{4} \left( {\frac{{10^{ - 9} }}{{2.1315 \times 10^{ - 9} }}} \right)^{4} + 2\left( {\frac{{10^{ - 9} }}{{2.1315 \times 10^{ - 9} }}} \right)^{4} } \\ { \times \left[ {0.456 \times 4.658 \times 0 + \left( {1 - 0.456} \right)1.248 \times 0} \right]} \\ \end{array} } \right\}} \hfill \\& = {6}.{73} \times {1}0^{{{12}}} {\text{Hz}} \hfill \\ \end{aligned}$$

So from the above calculation similar procedure can be adopted for cantilever boundary condition (FFFC) and results can be compared.

2.2 Finite Element Analysis

Simulation of continuum approach of rapheme sheet for frequency analysis of (10,0), (14,0), and (18,0) zigzag configuration with various lengths have been carried out in ANSYS workbench shown in Fig. 1. Dimensions of configuration were taken 10 nm × 21.315 Å with a width of 170 pm which became the diameter of carbon atom. But CAD software is enabled to generate this size of object and so that in ANSYS modeler, input dimensions were set as 0.001 × 0.0021315 µm and thus 2D rectangular rapheme sheet was extruded by 0.00017 µm.

Fig. 1
figure 1

Continuum solid model of single-layer graphene sheet along with boundary conditions

Full size image

2.3 Finite Element Analysis (Space Frame Approach)

In the previous calculation, graphene sheet is considered as a continuous plate for getting analytical solution of natural frequency but in the actual scenario, it is a single layer carbon sheet possesses a hexagonal lattice structure [23, 24].

We can validate the continuum approach by executing simulation in ANSYS APDL. For creating a space frame model in ANSYS APDL, coordinates of carbon atoms are generated in software and for that, each coordinate works as a key point in the active plane of ANSYS APDL. After getting all key points in ANSYS APDL, they were connected by line for obtaining lattice structure.

3 Result and Discussion

Three approaches to analyzing graphene sheet have given reasonable results with minor differences in the natural frequency in separate boundary conditions. Total three simulations of zigzag configurations graphene sheets were executed as an analytical solution, in Ansys Workbench and as a space frame approach in ANSYS APDL. Moreover, length of the graphene sheet has been set as 23.391, 47.995, 72.603, 97.213, 121.822 A for all approaches which are shown in Tables 3 and 4 for two different boundary conditions as, bridged boundary condition and cantilever boundary condition. Using Nano Modeler software, coordinates of space frame model have been generated using different lengths of graphene sheet and regarding that length, width of graphene sheet has been set by software. After creating successfully three configurations of zigzag atomic arrangement, all coordinates were exported in ANSYS APDL to generate the require size of graphene sheet and which is shown in Fig. 2. In bridged boundary conditions, two edges of graphene sheet are fixed and two edges are set free to deform whereas in cantilever condition, one edge is fixed and three edges are allowed to deform.

Table 3 Comparison of natural frequency (bridge boundary condition)
Full size table
Table 4 Comparison of natural frequency (cantilever boundary condition)
Full size table
Fig. 2
figure 2

Space frame model of zigzag (10,0) modeled using FEM package ANSYS

Full size image

As we know that natural frequency is affected by mass of the material and stiffness of the material, increment in length of the graphene sheet added the mass of carbon atoms in the sheet. This added mass resulted in change in natural frequency as per Fig. 3 and in Fig. 3, we can see that added mass decreases natural frequency of graphene sheet. In the bridged boundary condition, (18,0) configuration shows lower frequency at small length of sheet, whereas (14,0) shows higher frequency but ultimately as length of sheet increased, natural frequency matches to same value as in all configuration.

Fig. 3
figure 3

Variation of natural frequency with respect to length of SLGS in Zigzag atomic structures, for bridged boundary conditions

Full size image
Fig. 4
figure 4

Variation of natural frequency with respect to length of SLGS in Zigzag atomic structures, for cantilevered boundary conditions

Full size image

In cantilever boundary condition, Fig. 4 shows changes in natural frequency phenomenon completely due to free edges from three sides. As in this case results obtained in space frame approaches similar to boundary conditions but little higher natural frequency is shown due to motion allow from three sides. Less mass of carbon atoms shows higher frequency in (18,0) configuration whereas (10,0) shows lower natural frequency in the same approach for less mass of graphene sheet.

4 Conclusion

In the study of natural frequency of single-layer graphene sheet with different length phenomenon considering different configuration (10,0), (14,0), (18,0); it is observed that there is a little variation between analytical, FEA, and SFA approaches as ranges from 1 to 6%. In bridge boundary conditions, small size of SLGS resulted in higher natural frequency around 2.0E12 Hz as shown in (14,0) configuration whereas increment in length of SLGS, decreases natural frequency. On the contrary, in cantilever boundary condition, shortest length of SLGS gave very low-frequency compare to bridge boundary condition while considering any atomic structure. Increasing length of graphene sheet resulted in increment in width too but key sight of result can be seen from the Fig. 5 that after certain length of graphene sheet, minor variation is observed in natural frequency as stability can be achieved due to addition of mass does not affect too much. But one predominant data was observed regarding two boundary conditions that there is a huge variation in natural frequency for short length of graphene sheet so it is suggested that for some application where too much variation is not required, then length of graphene sheet can be kept higher for avoiding variation in frequency.

Fig. 5
figure 5

Variation of natural frequency against length of SLGS, comparison between bridged [(10,0)(B), (14,0)(B), (18,0)(B)] & cantilevered [(10,0)(C), (14,0)(C), (18,0)(C)] boundary conditions

Full size image