Thermogradient Dimensional Stabilization of eddential Cross-Sections of the Carrying Structure of an Autonomous Object

Abstract

The operating efficiency of autonomous objects of different purposes (underwater vehicles, spacecraft, etc.) substantially depends on the quality of the information received from heat-releasing apparatuses arranged on a carrying structure of the autonomous object. The thermal deformation of the carrying framework is caused by the irregularity of the temperature field due to a heat-releasing of devices, unsteady heat exchange with the ambient, and other reasons. In this case, the thermal deformation of the supporting framework, caused by the non-uniformity of the temperature field due to the heat dissipation of devices, unsteady heat transfer with the environment, and other reasons, is an important component of the complex distortion of information, especially optical measuring devices due to the displacement of the optical axis, focal length, etc., under the influence of a thermal gradient. Different systems of automatic thermal-stabilization are applied in order to decrease said distortions. The chapter is devoted to the mathematical modeling of thermal processes in the supporting framework during its automatic thermal gradient stabilization.

1 Introduction

Most devices arranged on a carrying structure of an autonomous object emits heat when operating, and it may cause thermal deformation, which, in turn, negatively affects the operation’s efficiency of the information-measuring system comprising optical equipment [1,2,3]. The problem of stabilization of the average value of the temperature distribution as well as the reduction of its irregularity in order to exclude thermogradient instability of the carrying framework shape is of current interest. The irregularity of the temperature distribution can be significantly reduced by arranging additional controlled heat-releasing elements (means of thermal gradient stabilization—hereinafter referred to as MTGS) and/or using a liquid cooling system. To analyze the operating mode of the heat-releasing devices and MTGS, to synthesize effective stabilization systems for the temperature distribution of the carrying framework, a mathematical model of its (framework’s) temperature state is required.

2 Problem Statement

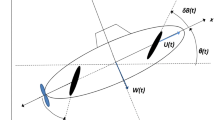
The carrying framework of the information-measuring system of the autonomous object is considered. The said framework has a shape of a rectangular prism (plate) and is subject to the influence of various thermal deformation loads. The plate (Fig. 1a) is cooled under the effect from the environment (the boundary condition of the third kind is BC-3) and is heated at the points of contact with the heat-releasing measuring devices: Pr1-Pr4, Pru1-Pru4, C1, h1, C2, h2, Pra, Prb, operating according to the cyclograms shown in Figs. 2, 3, 4, 5, 6 and 7 [4, 5] for one typical cycle with period t₁ = 30,000 s, and being controlled by MTGS: St1-St9, S1-S9. The basic initial data required for the calculations are presented in Tables 1 and 2.

Fig. 1

Carrying framework: a Arrangement of heat-releasing devices b Arrangement of control points

Fig. 2

Cyclogram of changing in heat-releasing power of devices Pr1-Pr4

Fig. 3

Cyclogram of changing in heat-releasing power of devices Pru1-Pru4

Fig. 4

Cyclogram of changing in heat-releasing power of devices Pra-Prb

Fig. 5

Cyclogram of changing in heat-releasing power of devices C1, C2

Fig. 6

Cyclogram of changing in heat-releasing power of devices h1

Fig. 7

Cyclogram of changing in heat-releasing power of devices h2

Table 1 Basic parameters

Table 2 Nominal power of heat-releasing measuring devices

The coordinates of the centers of the heat-releasing devices. Central MTGS on the plate’s side $x = 0$: St1, St2, St3, St4, St5. Central MTGS on the plate’s side $x = R_{1}$: S1, S2, S3, S4, S5. Diagonal MTGS on the plate’s side $x = 0:$ St6, St7, St3, St8, St9. Diagonal MTGS on the plate’s side $x = R_{1}$: S6, S7, S3, S8, S9. Heat-releasing measuring devices on the plate’s side $x = 0$: Pru1, Pru2, Pru3, Pru4. Heat-releasing measuring devices on the plate’s side $x = R_{1}$: Pr1, Pr2, Pr3, Pr4. Heat-releasing measuring devices on the plate’s side $y = 0$: Pra, Prb. Heat-releasing measuring devices on the plate’s side $z = 0$: h1, C1. Heat-releasing measuring devices on the plate’s side $z = R_{3}$: h2, C2.

The temperature field $\theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )$ of the plate is described by the three-dimensional thermal conductivity equation [6,7,8,9,10]:

$$\begin{aligned} & \frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{\partial \phi } = \frac{{\partial^{2} \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{x}^{2} }} \\ & \quad + \frac{{\partial^{2} \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{y}^{2} }} + \frac{{\partial^{2} \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{z}^{2} }}, \\ & \quad 0 < l_{x} < R_{1} \cdot R_{2}^{ - 1} ,\,\,0 < l_{y} < 1,\,\,0 < l_{z} < R_{3} \cdot R_{2}^{ - 1} ,\phi \in (0;\infty ), \\ \end{aligned}$$

(1)

by relative units: $y \cdot R_{2}^{ - 1} = l_{y} ,\,\,x \cdot R_{2}^{ - 1} = l_{x} ,\,\,z \cdot R_{2}^{ - 1} = l_{z} ,$ wherein $\phi = \frac{at}{{R_{2}^{2} }}$—Fourier number (« non-dimensional time »), $a = \frac{\lambda }{c\rho }$—thermal diffusivity, $\begin{aligned} \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi ) = (T_{pl} (x,y,z,t) - T^{ * } ) \cdot T^{ * - 1} , \hfill \\ \hfill \\ \end{aligned}$ $T^{*} = T_{sred} = const$—basic temperature, °C.

Initial condition:

$$\theta_{pl} (l_{x} ,l_{y} ,l_{z} ,0) = (T_{{_{pl} }}^{0} - T^{*} ) \cdot T^{* - 1} ,$$

(2)

wherein $T_{pl}^{0} = const$—initial temperature of the plate, °C.

Boundary conditions:

$$\left. {\frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{x} }}} \right|_{{l_{x} = 0}} = Q_{pl}^{lx0} (l_{y} ,l_{z} ,\phi ),$$

(3)

$$\left. {\frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{x} }}} \right|_{{l_{x} = R_{1} \cdot R_{2}^{ - 1} }} = Q_{pl}^{lx1} (l_{y} ,l_{z} ,\phi ),$$

(4)

$$\left. {\frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{y} }}} \right|_{{l_{y} = 0}} = Q_{pl}^{ly0} (l_{x} ,l_{z} ,\phi ),$$

(5)

$$\left. {\frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{y} }}} \right|_{{l_{y} = 1}} = Q_{pl}^{ly1} (l_{x} ,l_{z} ,\phi ),$$

(6)

$$\left. {\frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{z} }}} \right|_{{l_{z} = 0}} = Q_{pl}^{lz0} (l_{x} ,l_{y} ,\phi ),$$

(7)

$$\left. {\frac{{\partial \theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{z} }}} \right|_{{l_{z} = R_{3} \cdot R_{2}^{ - 1} }} = Q_{pl}^{lz1} (l_{x} ,l_{y} ,\phi ).$$

(8)

Here $\theta_{pl}^{lx0} (l_{y} ,l_{z} ,\phi ),$ $\theta_{pl}^{lx1} (l_{y} ,l_{z} ,\phi ),$ $\theta_{pl}^{ly0} (l_{x} ,l_{z} ,\phi ),$ $\theta_{pl}^{ly1} (l_{x} ,l_{z} ,\phi ),$ $\theta_{pl}^{lz0} (l_{x} ,l_{y} ,\phi ),$ $\theta_{pl}^{lz1} (l_{x} ,l_{y} ,\phi )$—summarized heat flow on the corresponding edges of the framework:

$$Q_{pl}^{lx0} (l_{y} ,l_{z} ,\phi ) = q_{p} (\phi ) - \sum\limits_{i = 1}^{N} {q_{{}}^{i} } \cdot V_{l \times 0}^{i} (l_{y} ,l_{z} ),$$

(9)

$$Q_{pl}^{lx1} (l_{y} ,l_{z} ,\phi ) = - q_{p} (\phi ) + \sum\limits_{i = 1}^{N} {q_{l \times 1}^{i} } \cdot V_{l \times 1}^{i} (l_{y} ,l_{z} ),$$

(10)

$$Q_{pl}^{ly0} (l_{x} ,l_{z} ,\phi ) = q_{p} (\phi ) - \sum\limits_{i = 1}^{M} {q_{ly0}^{i} } \cdot V_{ly0}^{i} (l_{x} ,l_{z} ),$$

(11)

$$Q_{pl}^{ly1} (l_{x} ,l_{z} ,\phi ) = - q_{p} (\phi ) + \sum\limits_{i = 1}^{M} {q_{ly1}^{i} } \cdot V_{ly1}^{i} (l_{x} ,l_{z} ),$$

(12)

$$Q_{pl}^{lz0} (l_{x} ,l_{y} ,\phi ) = q_{p} (\phi ) - \sum\limits_{i = 1}^{K} {q_{lz0}^{i} } \cdot V_{lz0}^{i} (l_{x} ,l_{y} ),$$

(13)

$$Q_{pl}^{lz1} (l_{x} ,l_{y} ,\phi ) = - q_{p} (\phi ) + \sum\limits_{1 = 1}^{K} {q_{lz1}^{i} } \cdot V_{lz1}^{i} (l_{x} ,l_{y} ).$$

(14)

$q_{p} (\phi )$—flows of heat losses from the surface, which are assumed to be the same on all surfaces of the plate; $q_{lx0}^{i} ,$ $q_{lx1}^{i}$—flows from the heat sources arranged on the edges $l_{x} = 0$ and $l_{x} = R_{1} \cdot R_{2}^{ - 1}$ accordingly, $q_{ly0}^{i} ,$ $q_{ly1}^{i}$—flows from the heat sources arranged on the edges $l_{y} = 0$ and $l_{y} = 1$ accordingly, $q_{lz0}^{i} ,$ $q_{lz1}^{i}$—flows from the heat sources arranged on the edges $l_{z} = 0$ and $l_{z} = R_{3} \cdot R_{2}^{ - 1}$ accordingly, wherein the intensity of flows from the heat sources is defined as a ratio of heat emission power P of the corresponding device to its area S of contact with the plate surface $q^{i} = P \cdot S^{ - 1}$; $V_{lx0}^{i} (l_{y} ,l_{z} ),$ $V_{lx1}^{i} (l_{y} ,l_{z} )$—functions determining the location of the devices on the edges $l_{x} = 0$ and $l_{x} = R_{1} \cdot R_{2}^{ - 1}$ accordingly, $V_{ly0}^{i} (l_{x} ,l_{z} ),$ $V_{ly1}^{i} (l_{x} ,l_{z} )$—functions determining the location of the devices on the edges $l_{y} = 0$ and $l_{y} = 1$ accordingly, $V_{lz0}^{i} (l_{x} ,l_{y} ),$ $V_{lz1}^{i} (l_{x} ,l_{y} )$—functions determining the location of the devices on the edges $l_{z} = 0$ and $l_{z} = R_{3} \cdot R_{2}^{ - 1}$ accordingly.

The temperature distribution in the plate is determined by solving auxiliary three-dimensional boundary value problem (1)–(8) according to the following algorithm:

• 1. Solving $\theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi )$ three-dimensional boundary value problem (1)–(8) is considered as the product of $\theta_{pl} (l_{x} ,\phi ),$ $\theta_{pl} (l_{y} ,\phi )$ and $\theta_{pl} (l_{z} ,\phi )$:

  $$\theta_{pl} (l_{x} ,l_{y} ,l_{z} ,\phi ) = \theta_{pl} (l_{x} ,\phi ) \cdot \theta_{pl} (l_{y} ,\phi ) \cdot \theta_{pl} (l_{z} ,\phi )$$

  solving the corresponding boundary value problems [11, 12] under condition $q_{lx}^{i} = 0,\,\,q_{ly}^{i} = 0,\,\,q_{lz}^{i} = 0,$ influence thereof is reflected by the summation of said solving and corresponding solving of three-dimensional boundary value problem with the boundary condition of the second kind (BC-2).

• 2. The convective nature of the heat exchange of the plate with the environment (BC-3) is reflected by the equivalent model for BC-2.

  Figure 8 shows proposed in the paper [13] structural representation of one-dimensional third boundary value problem BC-3 for $\theta_{pl}^{0} = 0$ by transfer functions BC-2 $W_{lx0} (l_{x} ,p),\,\,W_{lx1} (l_{x} ,p)$ accordingly for the one $l_{x} = 0$ and for the other $l_{x} = R_{1} \cdot R_{2}^{ - 1}$ side of the plate.

  Fig. 8

  

  The framework of one-dimensional auxiliary boundary value problem $\theta_{pl} (l_{x} ,\phi )$ by the Laplace transforms $\theta_{pl} (l_{x} ,p)$

  Here $Bi = \alpha \cdot R_{2} \cdot \lambda^{ - 1}$—Biot number. In the current example $\theta_{sred} (\phi ) = (T_{sred} (t) - T^{*} ) \cdot T^{* - 1} = 0.$

  The corresponding transforms $\theta_{pl} (l_{y} ,p),$ $\theta_{pl} (l_{z} ,p)$ of temperature distributions $\theta_{pl} (l_{y} ,\phi ),$ $\theta_{pl} (l_{z} ,\phi )$ is determined in the same way.

• 3. The thermal effect from the heat-releasing devices and MTGS is determined independently, and then taken into account in the general problem (1)–(8) by summation of the corresponding components of the temperature field.

3 Interaction with the Environment

One-dimensional boundary value problems with BC-2 for $\theta_{pl} (l_{x} ,\phi ),$ $\theta_{pl} (l_{y} ,\phi ),$ $\theta_{pl} (l_{z} ,\phi )$ used in the scheme (Fig. 8) are the following:

$$\left\{ {\begin{array}{*{20}l} {\frac{{\partial \theta_{pl} (l_{x} ,\phi )}}{\partial \phi } = \frac{{\partial^{2} \theta_{pl} (l_{x} ,\phi )}}{{\partial l_{x}^{2} }},\,0 < l_{x} < R_{1} \cdot R_{2}^{ - 1} ,\phi \in (0;\infty ),\,\,\,\,} \\ {\theta_{pl} (l_{x} ,0) = \theta_{pl}^{0} ,0 \le l_{x} \le R_{1} \cdot R_{2}^{ - 1} ,\phi \in (0;\infty ),\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {\left. {\frac{{\partial \theta_{pl} (l_{x} ,\phi )}}{{\partial l_{x} }}} \right|_{lx = 0} = q_{p} (\phi ),\left. {\frac{{\partial \theta_{pl} (l_{x} ,\phi )}}{{\partial l_{x} }}} \right|_{{lx = R_{1} \cdot R_{2}^{ - 1} }} = - q_{p} (\phi ).\,} \\ \end{array} } \right.\begin{array}{*{20}c} {\kern 1pt} \\ \, \\ \end{array}$$

(15)

$$\left\{ {\begin{array}{*{20}l} {\frac{{\partial \theta_{pl} (l_{y} ,\phi )}}{\partial \phi } = \frac{{\partial^{2} \theta_{pl} (l_{y} ,\phi )}}{{\partial l_{y}^{2} }},\,0 < l_{y} < 1,\phi \in (0;\infty ),} \\ {\theta_{pl} (l_{y} ,0) = \theta_{pl}^{0} ,0 \le l_{y} \le 1,\phi \in (0;\infty ),} \\ {\left. {\frac{{\partial \theta_{pl} (l_{y} ,\phi )}}{{\partial l_{y} }}} \right|_{ly = 0} = q_{p} (\phi ),\left. {\frac{{\partial \theta_{pl} (l_{y} ,\phi )}}{{\partial l_{y} }}} \right|_{ly = 1} = - q_{p} (\phi ).} \\ \end{array} } \right.$$

(16)

$$\left\{ {\begin{array}{*{20}l} {\frac{{\partial \theta_{pl} (l_{z} ,\phi )}}{\partial \phi } = \frac{{\partial^{2} \theta_{pl} (l_{z} ,\phi )}}{{\partial l_{z}^{2} }},0 < l_{z} < R_{3} \cdot R_{2}^{ - 1} ,\phi \in (0;\infty ),\,\,} \\ {\theta_{pl} (l_{z} ,0) = \theta_{pl}^{0} ,0 \le l_{z} \le R_{3} \cdot R_{2}^{ - 1} ,\phi \in (0;\infty ),\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {\left. {\frac{{\partial \theta_{pl} (l_{z} ,\phi )}}{{\partial l_{z} }}} \right|_{lz = 0} = q_{p} (\phi ),\left. {\frac{{\partial \theta_{pl} (l_{z} ,\phi )}}{{\partial l_{z} }}} \right|_{{lz = R_{3} \cdot R_{2}^{ - 1} }} = - q_{p} (\phi ).} \\ \end{array} } \right.\begin{array}{*{20}c} {\kern 1pt} \\ \, \\ \end{array}$$

(17)

In order to solve said boundary value problems a finite integral transformations method is applied [13]:

$$\bar{\theta }_{pl} (\mu_{n} ,\tau ) = \int\limits_{0}^{{R_{1} \cdot R_{2}^{ - 1} }} {\theta_{pl} (l_{x} ,\phi )\varphi_{n} } (\mu_{n} ,l_{x} )r(l_{x} )dl_{x} ,$$

(18)

$$\bar{\theta }_{pl} (\psi_{m} ,\tau ) = \int\limits_{0}^{1} {\theta_{pl} (l_{y} ,\phi )\varphi_{m} } (\psi_{m} ,l_{y} )r(l_{y} )dl_{y} ,$$

(19)

$$\bar{\theta }_{pl} (\gamma_{k} ,\tau ) = \int\limits_{0}^{{R_{3} \cdot R_{2}^{ - 1} }} {\theta_{pl} (l_{z} ,\phi )\varphi_{k} } (\gamma_{k} ,l_{z} )r(l_{z} )dl_{z} ,$$

(20)

wherein $\bar{\theta }_{pl} (\mu_{n} ,\psi_{m} ,\gamma_{k} ,\tau )$—transform of the original function; $r$—weight function (according to current problems $r = 1$); $\mu_{n} ,\psi_{m} ,\gamma_{k}$—proper numbers:

$$\begin{aligned} \mu _{n} & = \frac{{\pi nR_{2} }}{{R_{1} }},{\mkern 1mu} {\mkern 1mu} n = 0,1,2, \ldots ;{\mkern 1mu} {\mkern 1mu} \psi _{m} = \pi m,{\mkern 1mu} {\mkern 1mu} m = 0,1,2, \ldots ; \\ \gamma _{k} & = \frac{{\pi kR_{2} }}{{R_{3} }},{\mkern 1mu} {\mkern 1mu} k = 0,1,2, \ldots . \\ \end{aligned}$$

The normalized system of proper functions in one-dimensional problems has the form:

$$\varphi_{n} (\mu_{n} ,l_{x} ) = \frac{1}{{E_{n} }}\varphi_{n}^{*} (\mu_{n} ,l_{x} ) = \,\frac{1}{{E_{n} }}\cos (\pi n\frac{{R_{2} l_{x} }}{{R_{1} }})\,,n = 0,1,2, \ldots ,$$

$$\varphi_{m} (\psi_{m} ,l_{y} ) = \frac{1}{{E_{m} }}\varphi_{m}^{*} (\psi_{m} ,l_{y} ) = \,\frac{1}{{E_{m} }}\cos (\pi ml_{y} )\,,m = 0,1,2, \ldots ,$$

$$\varphi_{k} (\gamma_{k} ,l_{z} ) = \frac{1}{{E_{k} }}\varphi_{k}^{*} (\gamma_{k} ,l_{z} ) = \,\frac{1}{{E_{k} }}\cos (\pi k\frac{{R_{2} l_{z} }}{{R_{3} }})\,,k = 0,1,2, \ldots ,$$

Normalizing constants:

$$E_{n} = \sqrt {\int_{0}^{{R_{1} \cdot R_{2}^{ - 1} }} {\left[ {\varphi_{n}^{*} (\mu_{n} ,l_{x} )} \right]^{2} r(l_{x} )} dl_{x} } = \left\{ {\begin{array}{*{20}l} {\sqrt {R_{1} \cdot R_{2}^{ - 1} } ,n = 0} \\ {\sqrt {R_{1} \cdot (2R_{2} )^{ - 1} } ,n = 1,2,3 \ldots } \\ \end{array} } \right.,$$

$$E_{m} = \left\{ {\begin{array}{*{20}c} {1,m = 0\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {\sqrt {0.5} ,m = 1,2,3 \ldots } \\ \end{array} } \right.,E_{k} = \left\{ {\begin{array}{*{20}l} {\sqrt {R_{3} \cdot R_{2}^{ - 1} } ,k = 0} \\ {\sqrt {R_{3} \cdot (2R_{2} )^{ - 1} } ,k = 1,2,3 \ldots } \\ \end{array} } \right..$$

Solving the boundary value problems (15)–(17) for BC-2 $\theta_{pl} (l_{x} ,\phi ),$ $\theta_{pl} (l_{y} ,\phi ),$ $\theta_{pl} (l_{z} ,\phi )$ is the following:

$$\begin{aligned} \theta_{pl} (l_{x} ,\phi ) & = \sum\limits_{n = 0}^{\infty } {\varphi (\mu_{n} ,l_{x} )\int\limits_{0}^{\phi } R } (\mu_{n} ,\tau )G_{n}^{*} (\mu_{n} ,\phi - \tau )d\tau \\ & \quad + \sum\limits_{n = 0}^{\infty } {\varphi (\mu_{n} ,l_{x} )\int\limits_{0}^{{R_{1} \cdot R_{2}^{ - 1} }} {\theta_{pl}^{0} } } G_{n}^{*} (\mu_{n} ,\phi )\varphi (\mu_{n} ,\xi_{lx} )d\xi_{lx} \\ \end{aligned}$$

(21)

$$\begin{aligned} \theta_{pl} (l_{y} ,\phi ) & = \sum\limits_{m = 0}^{\infty } {\varphi (\psi_{m} ,l_{y} )\int\limits_{0}^{\phi } R } (\psi_{m} ,\tau )G_{m}^{*} (\psi_{m} ,\phi - \tau )d\tau \\ & \quad + \sum\limits_{m = 0}^{\infty } {\varphi (\psi_{m} ,l_{y} )\int\limits_{0}^{1} {\theta_{pl}^{0} } } G_{m}^{*} (\psi_{m} ,\phi )\varphi (\psi_{m} ,\xi_{ly} )d\xi_{ly} \\ \end{aligned}$$

(22)

$$\begin{aligned} \theta_{pl} (l_{z} ,\phi ) & = \sum\limits_{k = 0}^{\infty } {\varphi (\gamma_{k} ,l_{z} )\int\limits_{0}^{\phi } R } (\gamma_{k} ,\tau )G_{k}^{*} (\gamma_{k} ,\phi - \tau )d\tau \\ & \quad + \sum\limits_{k = 0}^{\infty } {\varphi (\gamma_{k} ,l_{z} )\int\limits_{0}^{{R_{3} \cdot R_{2}^{ - 1} }} {\theta_{pl}^{0} } } G_{k}^{*} (\gamma_{k} ,\phi )\varphi (\gamma_{k} ,\xi_{lz} )d\xi_{lz} \\ \end{aligned}$$

(23)

wherein  $R(\mu_{n} ,\phi ) = R_{1} (\mu_{n} ,\phi ) - R_{0} (\mu_{n} ,\phi ),\,$ $R(\psi_{m} ,\phi ) = R_{1} (\psi_{m} ,\phi ) - R_{0} (\psi_{m} ,\phi ),\,$ $R(\gamma_{k} ,\phi ) = R_{1} (\gamma_{k} ,\phi ) - R_{0} (\gamma_{k} ,\phi )$—functions determined by the boundary conditions: $R_{0} (\mu_{n} ,\phi ) = q_{p} (\phi )\varphi_{n} (\mu_{n} ,0),\,n = 0,1,2,3 \ldots ,$

$$R_{0} (\psi_{m} ,\phi ) = q_{p} (\phi )\varphi_{m} (\psi_{m} ,0),\,m = 0,1,2,3 \ldots ,$$

$$R_{0} (\gamma_{k} ,\phi ) = q_{p} (\phi )\varphi_{k} (\gamma_{k} ,0),\,k = 0,1,2,3 \ldots ,$$

$R_{1} (\mu_{n} ,\phi ) = - q_{p} (\phi )\varphi_{n} (\mu_{n} ,R_{1} \cdot R_{2}^{ - 1} ),\,R_{1} (\psi_{m} ,\phi ) = - q_{p} (\phi )\varphi_{m} (\psi_{m} ,1),\,$ $R_{1} (\gamma_{k} ,\phi ) = - q_{p} (\phi )\varphi_{k} (\gamma_{k} ,R_{3} \cdot R_{2}^{ - 1} ).$ $G_{n}^{*} ,\,\,G_{m}^{*} ,\,\,G_{k}^{*}$—time components of the Green’s function:

$$G_{n}^{*} (\mu_{n} ,\phi ) = e( - \mu_{n}^{2} \phi ) = e( - R_{2}^{2} \pi^{2} n^{2} (R_{1}^{2} )^{ - 1} \phi ),\,\,\,n = 0,1,2 \ldots ,$$

(24)

$$G_{m}^{*} (\psi_{m} ,\phi ) = e( - \psi_{m}^{2} \phi ) = e( - \pi^{2} m^{2} \phi ),\,\,\,m = 0,1,2 \ldots ,$$

(25)

$$G_{k}^{*} (\gamma_{k} ,\phi ) = e( - \gamma_{k}^{2} \phi ) = e( - R_{2}^{2} \pi^{2} k^{2} (R_{3}^{2} )^{ - 1} \phi ),\,\,\,k = 0,1,2 \ldots ,$$

(26)

Transfer functions (TF) used in the scheme (Fig. 8) for one-dimensional boundary value problem with BC-2 along $l_{x} ,l_{y} ,l_{z}$ and being the Laplace transforms of the corresponding Green’s functions have the following form:

$$\begin{aligned} W_{lx} (l_{x} ,p) & = R_{2} \cdot (R_{1} p)^{ - 1} + 2R_{2} \cdot R_{1}^{ - 1} \sum\limits_{n = 1}^{\infty } {\cos (\pi nR_{2} l_{x} \cdot R_{1}^{ - 1} )} \\ & \quad \times \cos (\pi nR_{2} \xi_{lx} R_{1}^{ - 1} )R_{1}^{2} (R_{2}^{2} \pi^{2} n^{2} )^{ - 1} (R_{1}^{2} (R_{2}^{2} \pi^{2} n^{2} )^{ - 1} p + 1)^{ - 1} \\ \end{aligned}$$

(27)

$$\begin{aligned} W_{ly} (l_{y} ,p) & = p^{ - 1} + 2\sum\limits_{m = 1}^{\infty } {\cos (\pi ml_{y} )\cos (\pi m\xi_{ly} )} \\ & \quad \times (\pi^{2} m^{2} )^{ - 1} ((\pi^{2} m^{2} )^{ - 1} p + 1)^{ - 1} \\ \end{aligned}$$

(28)

$$\begin{aligned} W_{lz} (l_{z} ,p) & = R_{2} \cdot (R_{3} p)^{ - 1} + 2R_{2} R_{3}^{ - 1} \sum\limits_{k = 1}^{\infty } {\cos (\pi kR_{2} l_{z} R_{3}^{ - 1} )} \\ & \quad \times \cos (\pi kR_{2} \xi_{lz} R_{3}^{ - 1} )R_{3}^{2} (R_{2}^{2} \pi^{2} k^{2} )^{ - 1} (R_{3}^{2} (R_{2}^{2} \pi^{2} k^{2} )^{ - 1} p + 1)^{ - 1} \\ \end{aligned}$$

(29)

wherein $\xi$—corresponding spatial coordinate of the heat source arrangement, said heat source is considered as a control (disturbing) action. A design model for solving additional boundary value problems (15)–(17) under the condition $q_{lx}^{i} = 0,\,\,q_{ly}^{i} = 0,\,\,q_{lz}^{i} = 0$ may be created by dependencies (27)–(29) within using the scheme according to Fig. 8 in the MATLAB software.

4 Temperature Effect from the Heat Sources

The areas of contact of the heat sources with the plate surfaces $l_{x} = 0,$ $l_{x} = R_{1} \cdot R_{2}^{ - 1}$ along the corresponding coordinates are designated in Fig. 9: $A_{lx0} (0,A_{lx0}^{y} ,A_{lx0}^{z} ),$ $B_{lx0} (0,B_{lx0}^{y} ,B_{lx0}^{z} ),$ $C_{lx0} (0,C_{lx0}^{y} ,C_{lx0}^{z} ),$ $D_{lx0} (0,D_{lx0}^{y} ,D_{lx0}^{z} );$ $A_{lx1} (R_{1} \cdot R_{2}^{ - 1} ,A_{lx1}^{y} ,A_{lx1}^{z} ),$ $B_{lx1} (R_{1} \cdot R_{2}^{ - 1} ,B_{lx1}^{y} ,B_{lx1}^{z} ),$ $C_{lx1} (R_{1} \cdot R_{2}^{ - 1} ,C_{lx1}^{y} ,C_{lx1}^{z} ),$ $D_{lx1} (R_{1} \cdot R_{2}^{ - 1} ,D_{lx1}^{y} ,D_{lx1}^{z} ).$

Fig. 9

General designation of the heat sources coordinates when $l_{x}$ fixed

The boundary value problem for the temperature component $\theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )$ of the heat sources effect is as follows:

$$\begin{aligned} \frac{{\partial \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{\partial \phi } & = \frac{{\partial^{2} \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{x}^{2} }} + \frac{{\partial^{2} \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{y}^{2} }} \\ & \quad + \frac{{\partial^{2} \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{z}^{2} }},0 \\ & \quad< l_{x} < \frac{{R_{1} }}{{R_{2} }},\,\,0 < l_{y} < 1,\,\,0 < l_{z} < \frac{{R_{3} }}{{R_{2} }},\phi \in (0;\phi_{k} ). \\ \end{aligned}$$

(30)

Initial conditions: $\theta_{ist} (l_{x} ,l_{y} ,l_{z} ,0) = 0.$ Boundary conditions along axis $l_{x}$:

$$\begin{array}{*{20}l} {\left. {\frac{{\partial \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{x} }}} \right|_{lx = 0} = - \sum\nolimits_{i = 0}^{\infty } {q_{lx0}^{i} \left[ {1(l_{y} - A_{lx0}^{y} ) - 1(l_{y} - B_{lx0}^{y} )} \right]} \cdot } \\ { \cdot \left[ {1(l_{z} - A_{lx0}^{z} ) - 1(l_{z} - D_{lx0}^{z} )} \right],} \\ \end{array}$$

(31)

$$\begin{array}{*{20}l} {\left. {\frac{{\partial \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi )}}{{\partial l_{x} }}} \right|_{{lx = R_{1} \cdot R_{2}^{ - 1} }} = \sum\nolimits_{i = 0}^{\infty } {q_{lx1}^{i} \left[ {1(l_{y} - A_{lx1}^{y} ) - 1(l_{y} - B_{lx1}^{y} )} \right]} \cdot } \\ { \cdot \left[ {1(l_{z} - A_{lx1}^{z} ) - 1(l_{z} - D_{lx1}^{z} )} \right],} \\ \end{array}$$

(32)

Boundary conditions along with the coordinates $l_{y}$ and $l_{z}$—formalized similarly. The components of the temperature distribution in the plate under the heat sources effect is determined by the corresponding convolution with the Green’s function $G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )$ [14]:

$$\theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi ) = \theta_{ist}^{lx} (l_{x} ,l_{y} ,l_{z} ,\phi ) + \theta_{ist}^{ly} (l_{x} ,l_{y} ,l_{z} ,\phi ) + \theta_{ist}^{lz} (l_{x} ,l_{y} ,l_{z} ,\phi ),$$

(33)

$$\begin{aligned} \theta_{ist} (l_{x} ,l_{y} ,l_{z} ,\phi ) & = \\ & \quad - \sum\limits_{{l_{x} = 0}} {\int\limits_{{\phi_{1} }}^{{\phi_{2} }} {\int\limits_{{A_{lx0}^{z} }}^{{D_{lx0}^{z} }} {\int\limits_{{A_{lx0}^{y} }}^{{B_{lx0}^{y} }} {q_{lx0}^{i} G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )d\xi_{ly} d\xi_{lz} d\tau } } } } \\ & \quad + \sum\limits_{{l_{x} = R_{1} \cdot R_{2}^{ - 1} }} {\int\limits_{{\phi_{3} }}^{{\phi_{4} }} {\int\limits_{{A_{lx1}^{z} }}^{{D_{lx1}^{z} }} {\int\limits_{{A_{lx1}^{y} }}^{{B_{lx1}^{y} }} {q_{lx1}^{i} G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )d\xi_{ly} d\xi_{lz} d\tau } } } } \\ & \quad - \sum\limits_{{l_{y} = 0}} {\int\limits_{{\phi_{1} }}^{{\phi_{2} }} {\int\limits_{{A_{ly0}^{x} }}^{{D_{ly0}^{x} }} {\int\limits_{{A_{ly0}^{z} }}^{{B_{ly0}^{z} }} {q_{ly0}^{i} G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )d\xi_{lz} d\xi_{lx} d\tau } } } } \\ & \quad + \sum\limits_{{l_{y} = 1}} {\int\limits_{{\phi_{3} }}^{{\phi_{4} }} {\int\limits_{{A_{ly1}^{x} }}^{{D_{ly1}^{x} }} {\int\limits_{{A_{ly1}^{z} }}^{{B_{ly1}^{z} }} {q_{ly1}^{i} G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )d\xi_{lz} d\xi_{lx} d\tau } } } } \\ & \quad - \sum\limits_{{l_{z} = 0}} {\int\limits_{{\phi_{1} }}^{{\phi_{2} }} {\int\limits_{{A_{lz0}^{x} }}^{{D_{lz0}^{x} }} {\int\limits_{{A_{lz0}^{y} }}^{{B_{lz0}^{y} }} {q_{lz0}^{i} G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )d\xi_{ly} d\xi_{lx} d\tau } } } } \\ & \quad + \sum\limits_{{l_{z} = R_{3} \cdot R_{2}^{ - 1} }} {\int\limits_{{\phi_{3} }}^{{\phi_{4} }} {\int\limits_{{A_{lz1}^{x} }}^{{D_{lz1}^{x} }} {\int\limits_{{A_{lz1}^{y} }}^{{B_{lz1}^{y} }} {q_{lz1}^{i} G_{ist} (l_{x} ,\xi_{lx} ,l_{y} ,\xi_{ly} ,l_{z} ,\xi_{lz} ,\phi - \tau )d\xi_{ly} d\xi_{lx} d\tau .} } } } \\ \end{aligned}$$

(34)

wherein

$$\begin{aligned} G_{{ist}} (l_{x} ,\xi _{{lx}} ,l_{y} ,\xi _{{ly}} ,l_{z} ,\xi _{{lz}} ,\phi - \tau ) & = \sum\limits_{{n = 0}}^{\infty } {G_{n}^{*} (\mu _{n} ,\phi - \tau )\varphi _{n} (\mu _{n} ,l_{x} )} \\ & \quad \times \varphi _{n} (\mu _{n} ,\xi _{{lx}} )r(\xi _{{lx}} ) \cdot \sum\limits_{{m = 0}}^{\infty } {G_{m}^{*} (\psi _{m} ,\phi - \tau )\varphi _{m} (\psi _{m} ,l_{y} )} \\ & \quad \times \varphi _{m} (\psi _{m} ,\xi _{{ly}} )r(\xi _{{ly}} )\sum\limits_{{k = 0}}^{\infty } {G_{k}^{*} (\gamma _{k} ,\phi - \tau )\varphi _{k} (\gamma _{k} ,l_{z} )} \\ & \quad \times \varphi _{k} (\gamma _{k} ,\xi _{{lz}} )r(\xi _{{lz}} ). \\ \end{aligned}$$

5 Result of Modeling

The mathematical model created in the MATLAB software on the basis of the obtained relations [15, 16] allows us to carry out the approximate synthesis of the controller, analysis of the corresponding control system stability and etc. A finite—element numerical modeling in the ANSYS software [17,18,19] is applied to problem solving more precisely.

We consider here the task of stabilizing the temperature in the critical cross-section of the carrying framework of the autonomous object (Fig. 1b) along the line from Uz1 to Uz5 by controlling the diagonal MTGS St6, St7, St3, St8, St9 on the one side of the prism and S6, S7, S3, S8, S9 on the other side thereof. The results of modeling the reaction of the temperature distribution in the carrying framework to the effect of the heat sources during t = 30,000 s in the ANSYS software are reflected in Fig. 10.

Fig. 10

Finite-element modeling of the carrying framework temperature in the Ansys software (t = 30,000): a Without MTGS effect b When diagonal MTGS effect, wherein MTGS arranged along the line from Uz1 to Uz5

Figure 10b shows the temperature fields of the prism under the effect of diagonal MTGS St6, S6, St9, S9 with the power of 8 W.

The temperature graphs of the corresponding cross-section line are presented in Fig. 11, wherein the vertical axis $T_{pl} ,\,^{^\circ } {\text{C}}$ represents temperature values, and the horizontal axis D, m represents a diagonal cross-section from Uz1 to Uz5.

Fig. 11

Graphs of temperature distribution for t = 30,000 s: a Along diagonal cross section from Uz1 to Uz5 without MTGS b Along diagonal cross section from Uz1 to Uz5 when MTGS effect

The results of the operation of the automatic temperature stabilization system in the critical node Uz1 being critical for providing the dimensional stabilization along the line of cross-section from Uz1 to Uz5 are subject to consideration. The closest heat sources influence Uz1 in a most substantial way: from the measuring devices in nodes Pr3, Pru3 being controlled by MTGS St6, S6, as well as the convective heat exchange with the environment.

The algorithm of control of MTGS St6 and S6 effecting on the point of control Uz1 is implemented by the proportional—integral (PI) law of control (Figs. 12, 13).

Fig. 12

Scheme of modeling a temperature control system of the critical node of the carrying framework in the MATLAB software: Tsr—the predetermined temperature in the node Uz1, Alfa—the unit of determination of the heat exchange coefficient, α; WS6, WSt6, WPr3,WPru3, Wk—transfer functions determining the temperature in the node Uz1 when MTSG S6, St6, measuring devices Pr3, Pru3 and convective heat exchange effect, PID Controller—unit of modelling PI-controller (without derivative term)

Fig. 13

Result of modeling the automatic temperature stabilization system in the node in the MATLAB software: 1 Temperature in the node Uz1 under the automatic control, °C; 2 Temperature in the node Uz1 without control, °C; 3 Power of MTGS generated at the PI-controller output (control for MTGS), W; 4 Cyclogram of changing in heat-releasing power of the measuring devices, W; 5 Convectional heat losses, °C; a Portion of temperature distribution under the automatic control, °C

There is an automatically formed the changing in the power of MTGS St6 and S6 in deviation from the average value of the MTGS power at the PI-controller output.

6 Conclusions

There have been presented an argument for the problem of automatic stabilization of temperature distribution in the carrying framework of the autonomous object as the effective means for decreasing the temperature component in the error of the information-measuring system arranged on the said framework.

There has been provided a method of mathematical modeling of the temperature distribution in the carrying frameworks of autonomous objects, said method comprises approximate mathematical modeling in the MATLAB software and more precise modeling in the ANSYS software.

By analytical structural-parametric identification, there have been obtained the transfer functions of the temperature distribution in the carrying framework for implementation in an approximate mathematical model in MATLAB software and controller synthesis.

Modeling of the automatic temperature stabilization system in the node of the critical cross-section of the supporting framework has confirmed its high efficiency.