Surprising solutions for some challenging problems arising from boundary layer theory by a new technique: the homotopy contraction mapping technique (HCMT)

Abstract

In this work, the homotopy structure $(1 - p)L\,[f] = - p\,N\,[f]$ is considered to solve some newly developed nonlinear problems in fluid dynamics. In summary, deformation in homotopy series solutions occurs from the initial trial function to the actual solution. The main challenging part in many similarity equations associated with the boundary layer theory is to identify a certain quantity of the exact solution such as $f^{{\prime \prime }} (0)$. This quantity is initially unknown and is automatically guessed through the zeroth function. Therefore, it makes sense that in the previous homotopy series solutions one would need at least another parameter i.e. the controlling parameter ($\hbar$) to handle the convergence through the so-called $\hbar$-curves analysis and eventually get an approximate value for $f^{{\prime \prime }} (0)$. Here it is accounted the basic homotopy structure with No controlling parameter ($\hbar$) and it is shown for the 1^st time that the zeroth order solution in homotopy series may be potential to contain some certain quantities of the exact solution, here is to be $f^{{\prime \prime }} (0)$; i.e. $f_{0}^{{\prime \prime }} (0)$ could be $f^{{\prime \prime }} (0)$. This hypothesis is checked through a theorem, being totally linked to the Fixed Point Property (FPP), a topological invariant; i.e. being preserved by any homeomorphism. The theorem enables us Not only to check the validity of the hypothesis, but also to extract the value of the quantity as the unique description of the homotopy series solutions truncated at any order of approximation. The technique is initially introduced in a simple and straightforward manner and then it is applied to some fluid mechanical problems to be further compared with traditional homotopy analysis method, homotopy perturbation method and Numerical solutions. The outcomes indicated an excellent improvement by the present approach as only a few series terms were accounted to obtain highly accurate solutions.

1 Introduction

HAM in individual as described by Liao (e.g. [1,2,3,4,5,6,7,8]) contains several degrees of freedom; the choice for the initial guess (zeroth function, $f_{0}$), the auxiliary linear operator (written as: $L[f]$), the controlling parameter ($\hbar$) and possibly additional homotopy functions. Indeed, as indicated by Liao himself in his numerous published papers (e.g. see [1,2,3,4,5,6,7,8]), these freedoms give the technique an excellent maneuverability to variety of nonlinear problems in a convenient manner. Later on, HPM is systematically described by He (e.g. [9,10,11,12,13,14,15]), in particular fixing the so-called controlling parameter (asserting that such a parameter has been heuristically introduced by Liao) to suggest (as He was convinced with) a more compact algorithm. P.N. that, as argued by Liao [8], one still needs more rigorous mathematical proofs to distinguish HPM from HAM by the so-called No-secular terms rules; and hence, HPM may not be considered as a more compact algorithm compared to HAM without this proof.

It should be mentioned that the controlling parameter is fixed in HPM and hence, the so-called No-secular condition is somewhat responsible for the convergence of homotopy terms; and without this condition, there would be No control on the convergence and therefore, HPM would become a subset of HAM (see [8, 12] to follow the arguments by Liao and He respectively about HPM).

In the present introduction, it is not intended to judge HPM and only the summary of some outcomes of this algorithm is placed to shortly review the literature.

As shown in many references such as [9,10,11,12,13,14,15,16,17,18,19,20,21], if the No-secular term condition is successfully applied, the solution for the nonlinearity can be described in parametric forms (upon having any parameter in the problem) and free from the appearance of controlling parameter which needs further analysis through $\hbar$-curves for the convergence issue.

Reviewing the above cited papers with regard to HPM, authors have mostly sought ways to ease obtaining higher orders of approximation mainly on the basis of parameter expansion methods (as a glance, parameter expansion method is a technique to somewhat manipulate the linear operator with additional parameters; enabling one to remove further secular terms occurring in higher orders of approximation) together with repeating the same rule (the condition of No-secular terms); and in this respect, functionalizing freedoms within the successful and easily-iterative system of HAM has been less sought.

Parameter expansion method has been practiced previously by some authors and it appears that this technique is an advancement in HPM (e.g. see [16,17,18,19,20,21]); however, in most cases, dealing with complicated systems is the inherent drawback of this technique to obtain higher orders of approximation (e.g. see [18, 21]). In addition, in some cases, this technique may reveal conditional solution and/or the solution by this technique may Not be unique and it is Not even clear that the additional solutions are trivial or Not (e.g. see [21]).

By this research, the present author mainly wishes to introduce an open and masked topic in homotopy series analysis.

In homotopy, deformation occurs from the initial guess (zeroth series term) to the actual solution. Suppose that the trial function contains some certain target quantities of the exact solution, here is to be $f^{{\prime \prime }} (0)$; i.e. $f_{0}^{{\prime \prime }} (0)$ is supposed to be $f^{{\prime \prime }} (0)$; and hence, the induction that ‘the summation of this quantity generated due to the rest of the series terms should be zero’ is immediate. Indeed, this is an extension of the quote, initially postulated by Liao where he defines the initial guess in such a way satisfying the boundary conditions and hence, the boundary conditions for the subsequent linear equations are zero; P.N. that the boundary conditions are the certain quantities of the exact solution (see e.g. [1,2,3,4,5,6,7,8]).

By developing a theorem, it is shown that the above hypothesis is totally linked to the Fixed Point Property (FPP). The theorem Not only assesses the validity of the hypothesis, but also gives the value of the target quantity, being regarded as the unique description of the homotopy series solution at any order of approximation.

In the following, the new approach is systematically defined. Later, the 3-D Magnetohydrodynamics (MHD) flow due to an exponentially stretching surface and the 2-D flow of Upper Convected Maxwell (UCM) fluids over a linearly stretching sheet are solved via various analytic and numeric techniques. Examples illustratively show that the proposed approach is extremely straightforward, highly accurate and promising.

2 Methodology

2.1 The homotopy contraction mapping technique (HCMT)

The basic idea is to hypothesize that the leading term (series zeroth term) is potential to contain any certain quantity of the exact solution (such as $f^{{\prime \prime }} (0)$; i.e. $f_{0}^{{\prime \prime }} (0)$ could be $f^{{\prime \prime }} (0)$). This hypothesis is checked in a detailed manner through a theorem as comes later (denoted as Theorem 2 in this paper).

For this purpose it is initially referred to the following theorem.

Theorem 1

(Banach’s Contraction Principle) Let $(X,d)$ represent a complete metric space and $f:\,\,X \to X$ be a contraction, i.e. there exists $k \in (0,1)$ such that for each $x,y \in X:\,d(fx,fy) \le kd(x,y)$. Then, $f$ has a unique fixed point in $X$, say $x^{*}$ and for each $x_{0} \in X$ the infinite sequence of function composition converges to this fixed point $\left\{ {f^{n \to \infty } x_{0} } \right\} = fofof \ldots fx_{0} = x^{*}$ [22]. For more information regarding the proof of the theorem and further extensions interested readers are referred to the in-depth study of Ref. [23,24,25,26,27,28].

Definitions

Consider a nonlinear problem in a general form as: ${\text{N}}[u(r)] = 0,\,r \in \varOmega$ and the boundary conditions as: $B\left( {u,\frac{\partial u}{\partial n}} \right) = 0,\;r \in \varGamma$.

Where $N$ is a general differential operator, $u(r)$ is a solution defined in $r \in \varOmega$, $B$ is a boundary condition operator and $\varGamma$ is a boundary of domain $\varOmega$.

Consider the homotopy $(1 - p)L[u(r),\alpha ] = - pN\;[u(r)]$; with $L$ being an auxiliary linear operator, $p$ the embedding artificial parameter ($p \in [0,1]$) and $\alpha$, an initially unknown parameter. P.N. that $L$ is arbitrary, e.g. $L[( \cdot ),\alpha ] = \frac{{\partial^{n} ( \cdot )}}{{\partial r^{n} }} + \alpha \frac{{\partial^{m} ( \cdot )}}{{\partial r^{m} }}$. In accordance with the above homotopy set-up, the initial solution is linked to $p = 0$ and $p = 1$ recovers the actual solution and hence, on using the standard expansion $u(r) = u_{0} (r) + pu_{1} (r) + p^{2} u_{2} (r) + \cdots$ the solution is described as $u = \sum\nolimits_{i = 0}^{n \to \infty } {u_{i} }$. P.N., as dictated by Liao, the expansion method in HAM is normally Taylor method; however, as suggested by Sajid et al. [29], Taylor method works equivalently as the standard expansion method denoted above.

Lemma

Let $\zeta^{*,m}$ be the unique description of a certain quantity of the exact solution (say $\zeta$; e.g. $\zeta = f^{{\prime \prime }} (0)$) after the mth order of approximation generated due to the homotopy construction defined above, and $\zeta_{0}^{*}$ the corresponding zeroth guess; i.e. $\sum\nolimits_{i = 0}^{m} {\zeta_{i}^{*} = \zeta^{*,m} }$; then, $\zeta^{*,m} = \zeta_{0}^{*}$ holds if, $\exists \varLambda = \left\{ {\zeta_{0} |\sum\nolimits_{i = 0}^{m \to \infty } {\zeta_{i} = \zeta } } \right\}:\zeta \in \varLambda$.

Proof

The homotopy series is convergent on $\varLambda$ implying the existence of a unique series solution for the nonlinearity. It follows that there exists a unique description of the solution for the mth order of approximation, i.e. $\zeta^{*,m}$, satisfying $\zeta^{\,*,m \to \infty } = \zeta$, and $\zeta^{*,m}$ has No dependency on $\zeta_{0}$. With $\zeta = \zeta^{\,*,m \to \infty } \in \varLambda$, we can write $\zeta_{0} = \zeta^{\,*,m \to \infty }$; by induction, this also holds for $\zeta^{*,m}$ i.e. $\zeta^{\,*,m} \in \varLambda$ and we can write $\zeta_{0} = \zeta^{\,*,m}$. Since $\zeta^{*,m}$ is unique, it follows that $\zeta_{0}$ is also unique, say $\zeta_{0}^{*}$. □

Theorem 2

Let $T_{m} \left( {\zeta_{0} } \right) = \sum\limits_{i = 0}^{m} {\zeta_{i} }$ be a mapping function.

If $T_{m \ge 1}^{n \to \infty } \zeta_{0} = T_{m} oT_{m} oT_{m} o \ldots T_{m} \left( {\zeta_{0} } \right) = C_{m}$ holds for some $\zeta_{0}$, then $\zeta = C_{m \to \infty } \in \varLambda$ and $C_{m} = \zeta^{*,m}$ is the non-trivial real solution of $\sum\nolimits_{i = 1}^{m} {\zeta_{i} = 0}$.

Proof

Let the condition holds true. Suppose that $T_{m}$ is invertible in a neighborhood of $\zeta^{*,m}$. This gives $T_{m} \left( {C_{m} } \right) = C_{m}$. Since $C_{m}$ is the unique fixed point of $T_{m}$, then by the lemma $C_{m} = \zeta^{*,m}$ and it is the non-trivial real solution of $\sum\nolimits_{i = 1}^{m} {\zeta_{i} = 0}$. It follows that $\zeta = C_{m \to \infty } \in \varLambda$. □

Remark

In fact, for the mth order of approximation by the homotopy construction defined earlier, whatever $\zeta_{0}$ is, it converges to the fixed value $\zeta^{*,m}$ through the recursive process $\zeta_{0,n + 1} = T_{m} \left( {\zeta_{0,\,n} } \right),\,n = 0,1,2, \ldots ,:\zeta_{0,n \to \infty } = \zeta^{*,m} = C_{m}$; meaning that the homotopy structure associated with the linear operator is self-corrector for $\zeta$. Therefore, at this stage it is understandable that the strategy is indeed an insight into HAM preventing from unnecessary extra series terms and providing an optimized solution.

2.2 Ex. 1: the 3-D MHD flow due to an exponentially stretching surface

The basic equations and boundary conditions for such a flow can be found in several recent studies such as [30,31,32,33,34,35]:

$$ f^{\prime \prime \prime } (\eta ) + \left( {f(\eta ) + g(\eta )} \right)f^{\prime \prime } (\eta ) - 2\left( {f^{\prime } (\eta ) + g^{\prime } (\eta )} \right)f^{\prime } (\eta ) - Mf^{\prime } (\eta ) = 0 $$

(1)

$$ g^{{{\prime \prime \prime }}} (\eta ) + \left( {f(\eta ) + g(\eta )} \right)g^{{{\prime \prime }}} (\eta ) - 2\left( {f^{{\prime }} (\eta ) + g^{{\prime }} (\eta )} \right)g^{{\prime }} (\eta ) - Mg^{{\prime }} (\eta ) = 0 $$

(2)

Along with the following boundary conditions:

$$ f(0) = 0,\quad f^{{\prime }} (0) = 1,\quad f^{{\prime }} (\infty ) = 0,\quad g(0) = 0,\quad g^{{\prime }} (0) = c,\quad g^{{\prime }} (\infty ) = 0 $$

(3)

In Eqs. 1–3, $M$ is the magnetic parameter and $c$ is the stretching ratio. Moreover, $c = 0$ corresponds to the 2-D version, whilst $c = 1$ represents the axisymmetric flow.

Unlike the 3-D linearly stretching sheet flow, the above coupled system can be simply broken down (the system is scalable by $g(\eta ) = cf(\eta )$), a point which seems to be untapped in the literature. It can be easily shown that the above system is convertible to:

$$ f^{{{\prime \prime \prime }}} (\eta ) + (c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) - Mf^{{\prime }} (\eta ) = 0 $$

(4)

$$ f(0) = 0,\quad f^{{\prime }} (0) = 1,\quad f^{{\prime }} (\infty ) = 0 $$

(5)

$$ g(\eta ) = cf(\eta ) $$

(6)

Therefore, it is sufficient to solve Eq. 4. P.N. that the solutions by the above scheme only reveal one possible class of solutions for this nonlinearity; however, it will be compared that these solutions are accurately those already established by other researchers employing numerical analysis and also agree well with the numerical analysis presented in this paper.

First assume $M = 0$:

$$ f^{{{\prime \prime \prime }}} (\eta ) + (c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) = 0 $$

(7)

Suppose that $\bar{f}(\eta )$ is the solution for the 2-D version of the above equation, then if the general solution is sought in the form of $A\bar{f}(B\eta )$, one reaches:

$$ f(\eta ) = \frac{1}{{\sqrt {c + 1} }}\bar{f}\left( {\sqrt {c + 1} \,\eta } \right) $$

(8)

Equation 8 is a consistent solution. Therefore, in the absence of magnetic effect, one should only solve the 2-D version of the flow:

$$ \bar{f}^{{{\prime \prime \prime }}} (\eta ) + \bar{f}(\eta )\bar{f}^{{{\prime \prime }}} (\eta ) - 2\bar{f}^{{{\prime }2}} (\eta ) = 0 $$

(9)

$$ \bar{f}(0) = 0,\quad \bar{f}^{{\prime }} (0) = 1,\quad \bar{f}^{{\prime }} (\infty ) = 0 $$

(10)

Therefore, for 3-D flow due to an exponentially stretching surface:

$$ f^{{{\prime \prime }}} (0) = \bar{f}^{{{\prime \prime }}} (0)\sqrt {c + 1} $$

(11)

$$ g^{{{\prime \prime }}} (0) = \bar{f}^{{{\prime \prime }}} (0)\,c\sqrt {c + 1} $$

(12)

The same fundamental tricks are also applicable to 3-D flow due to a nonlinearly stretching surface (linearly stretching surface is an exception); however, for the sake of the scope of the present study further analysis in this respect is deferred to the future studies.

For $M \ne 0$ Eq. 4, in its original form, is preserved and is to be solved via several methods.

2.3 Solution by homotopy analysis method (HAM)

The auxiliary linear operator and the initial guess are chosen as (see e.g. [36,37,38,39,40] to see how HAM may work):

$$ f_{0} (\eta ) = 1 - e^{ - \eta } $$

(13)

$$ L(f) = f^{{{\prime \prime \prime }}} (\eta ) - f^{{\prime }} (\eta ) $$

(14)

Principally, the problem satisfies:

$$ (1 - p)L[\bar{f}(\eta ,p) - f_{0} (\eta )] = p\hbar N[\bar{f}(\eta ,p)] $$

(15)

with

$$ \bar{f}(0,p) = 0,\quad \bar{f}^{{\prime }} (0,p) = 1,\quad \bar{f}(\infty ,p) = 0 $$

(16)

In Eq. 15, the nonlinear operator is:

$$ N[\bar{f}] = \frac{{\partial^{3} \bar{f}}}{{\partial \eta^{3} }} + (c + 1)\bar{f}(\eta ,p)\frac{{\partial^{2} \bar{f}}}{{\partial \eta^{2} }} - 2(c + 1)\left( {\frac{{\partial \bar{f}}}{\partial \eta }} \right)^{2} - M\frac{{\partial \bar{f}}}{\partial \eta } $$

(17)

In addition, $\hbar$ is the so-called controlling parameter and $p \in [0,1]$ is the homotopy embedding parameter. Clearly:

$$ \bar{f}(\eta ,0) = f_{0} (\eta ),\quad \bar{f}(\eta ,1) = f(\eta ) $$

(18)

When the embedding parameter $p$ deforms from 0 to 1, the initial guess $f_{0} (\eta )$ approaches $f(\eta )$ (P.N. that this is an artificial topological parameter; and No value between 0 and 1 appears in the HAM calculation process).

Upon using the straightforward expansion technique (Taylor method):

$$ \bar{f}(\eta ,p) = f_{0} (\eta ) + \sum\limits_{m = 1}^{\infty } {f_{m} (\eta )p^{m} } $$

(19)

where

$$ f_{m} (\eta ) = \frac{1}{m!}\frac{{\partial^{m} \bar{f}}}{{\partial p^{m} }}\left| {_{p = 0} } \right. $$

(20)

Since the series converge for $p = 1$:

$$ f(\eta ) = f_{0} (\eta ) + \sum\limits_{m = 1}^{\infty } {f_{m} (\eta )} $$

(21)

For the mth-order deformation:

$$ L[f_{m} (\eta ) - \chi_{m} f_{m - 1} (\eta )] = \hbar \Re_{m} (\eta ) $$

(22)

with the boundary conditions:

$$ f_{m} (0) = f_{m}^{\prime } (0) = f_{m}^{\prime } (\infty ) = 0 $$

(23)

where

$$ \chi_{m} = \left\{ {\begin{array}{*{20}l} 0 \hfill & {m \le 1} \hfill \\ 1 \hfill & {m > 2} \hfill \\ \end{array} } \right. $$

(24)

$$ \Re_{m} (\eta ) = f_{m - 1}^{{\prime }} (\eta ) + (c + 1)\sum\limits_{k = 0}^{m - 1} {\left\{ {\left( {f_{m - 1 - k} (\eta )} \right)f_{k}^{{\prime }} (\eta )} \right\}} - 2(c + 1)\sum\limits_{k = 0}^{m - 1} {\left\{ {\left( {f_{m - 1 - k}^{{\prime }} (\eta )} \right)f_{k}^{{\prime }} (\eta )} \right\} - Mf_{m - 1}^{{\prime }} (\eta )} $$

(25)

The above system was solved by developing a symbolic code in MATLAB.

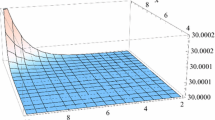
Figure 1 represents the so-called $\hbar$-curves in different order of approximations for the 2-D case ($c = 0$) with $M = 0$. The algorithm was truncated at the 15th order of approximation and it was firmly confirmed $f^{{\prime \prime }} (0) = - 1.2818$ (As a comparison, Liu et al. [30] have reported, employing numerical analysis, that for this case $f^{{\prime \prime }} (0) = - 1.28180856$). Hence, e.g. for $c = 0.5$ and $c = 1$ we obtain, from Eq. 10 and 11, $f^{{\prime \prime }} (0) = - 1. 5 6 9 9$ (Liu et al. [30]: $f^{{\prime \prime }} (0) = - 1.5698884$), $g^{{\prime \prime }} (0) = - 0. 7 8 4 9$ (Liu et al. [30]: $g^{{\prime \prime }} (0) = - 0.78494423$) and $f^{{\prime \prime }} (0) = - 1. 8 1 2 7$ (Liu et al. [30]: $f^{{\prime \prime }} (0) = - 1.8127510$), $g^{{\prime \prime }} (0) = - 1. 8 1 2 7$ (Liu et al. [30]: $g^{{\prime \prime }} (0) = - 1.8127510$) respectively.

Fig. 1

$\hbar$-curves in different orders of approximation for $c = 0$, $M = 0$: X-axis and Y-axis are $\hbar$ and $f^{{{\prime \prime }}} (0)$ respectively

Employing $\hbar$-curves analysis for other cases, we could obtain reliable solutions for this problem.

For other cases, having $c \ne 0$ and $M \ne 0$, Table 1 is provided to indicate the behavior of the significant engineering quantity of interest, being secured to the 4^th decimal place.

Table 1 $f^{{{\prime \prime }}} (0)$

2.4 Solution by 1st-order homotopy perturbation method (HPM)

To indicate the basic idea of this method, the following form for a nonlinear equation is considered (see e.g. [41]):

$$ A(U) - f(r) = 0,\quad r \in \varOmega $$

(26)

Boundary conditions are defined as:

$$ B\left( {u,\frac{\partial u}{\partial n}} \right) = 0,\quad r \in \varGamma $$

(27)

In above, $A$ is a general function operator, $B$ is a boundary condition operator and $\varGamma$ is a boundary of domain $\varOmega$ and $f(r)$ is a known function.

The general operator in Eq. 26 may be decomposed into a linear and a nonlinear operator as:

$$ L(U) + N(U) - f(r) = 0,\quad r \in \varOmega $$

(28)

On using the homotopy $U(r,p):\varOmega \times [0,1] \to R$ satisfying:

$$ H(U,p) = (1 - p)\left( {L(U) - L(U_{0} )} \right) + p\left( {A(U) - f(r)} \right) = 0,\quad p \in [0,1],\quad r \in \varOmega $$

(29)

or

$$ H(U,p) = \left( {L(U) - L(U_{0} )} \right) + p\left( {L(U_{0} ) + N(U) - f(r)} \right) = 0 $$

(30)

In above, $p$ is an embedding artificial parameter (with the same deformation property as dictated in HAM) and $U_{0}$ is an initial approximate solution to Eq. 26. The following properties can be deduced:

$$ H(U,0) = \left( {L(U) - L(U_{0} )} \right) = 0 $$

(31)

$$ H(U,1) = \left( {A(U) - f(r)} \right) = 0 $$

(32)

According to HPM, we normally employ the standard expansion as:

$$ U = U_{0} + pU_{1} + p^{2} U_{2} + p^{3} U_{3} + p^{4} U_{4} + \cdots $$

(33)

On choosing $p = 1$, the solution of Eq. 26 can be approximated as:

$$ U = U_{0} + U_{1} + U_{2} + U_{3} + U_{4} + \cdots $$

(34)

As it can be seen, the HPM is quite similar to HAM with $\hbar = - 1$; however, as indicated by He (see e.g. [9,10,11,12,13,14,15]), HPM can be distinguished from HAM by e.g. implementing the No-secular term rule as being initially proposed by Lighthill [42], that any term in the series occurring in the solution should be No more singular than the preceding term; however, as discussed in the introduction section, in order to present HPM as a completely distinguished nonlinear solver, one still needs more mathematical proofs.

In the following we apply HPM for the present nonlinear example.

Let us set up the following homotopy equation:

$$ (1 - p)L[f(\eta )] = - pN[f(\eta )] $$

(35)

The operators are assumed as:

$$ L[f(\eta )] = f^{{{\prime \prime \prime }}} (\eta ) - \beta \,^{2} f^{{\prime }} (\eta ) $$

(36)

$$ N[f(\eta )] = f^{{{\prime \prime \prime }}} (\eta ) + (c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) - Mf^{{\prime }} (\eta ) $$

(37)

Therefore, one reaches:

$$ f^{{{\prime \prime \prime }}} (\eta ) - \beta^{2} f^{{\prime }} (\eta ) + p\left( {(c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) - Mf^{{\prime }} (\eta ) + \beta^{2} f^{{\prime }} (\eta )} \right) = 0,\quad \beta > 0 $$

(38)

Taking into accounting the standard expansion, $f(\eta )$ is described as:

$$ f(\eta ) = f_{0} (\eta ) + f_{1} (\eta )p + f_{2} (\eta )p^{2} + \cdots $$

(39)

Since, at $p = 1$ the original system is recovered, the solution is:

$$ f(\eta ) = f_{0} (\eta ) + f_{1} (\eta ) + f_{2} (\eta ) + \cdots $$

(40)

On substituting Eq. 39 into Eq. 38 and collecting terms with like powers of $p$, the following zeroth and 1st-order systems are obtained:

$$ \begin{aligned} & p^{0} :\,f_{0}^{{{\prime \prime \prime }}} \,\,(\eta ) - \beta^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{0} (0) = 0,\quad f_{0}^{{\prime }} (0) = 1,\quad f_{0}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(41)

$$ \begin{aligned} & p^{1} :\,f_{1}^{{{\prime \prime \prime }}} (\eta ) - \beta^{2} f_{1}^{{\prime }} (\eta ) + \left( {(c + 1)f_{0} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) - 2(c + 1)f_{0}^{{{\prime }2}} (\eta ) - Mf_{0}^{{\prime }} (\eta ) + \beta^{2} f_{0}^{{\prime }} (\eta )} \right) = 0 \\ & f_{1} (0) = 0,\quad f_{1}^{{\prime }} (0) = 0,\quad f_{1}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(42)

Solution to Eq. 41 reads:

$$ f_{0} (\eta ) = \frac{1}{\beta }\left( {1 - e^{ - \beta \eta } } \right),\quad \beta > 0 $$

(43)

Equation 42 gives:

$$ f_{1} (\eta ) = e^{( - \beta \eta )} \left( {\frac{3M + 5c + 5}{{6\beta^{3} }} - \frac{1}{2\beta }} \right) - e^{( - 2\beta \eta )} \left( {\frac{c + 1}{{6\beta^{3} }}} \right) + \eta \,e^{( - \beta \eta )} \left( {\frac{M + c + 1}{{2\beta^{2} }} - \frac{1}{2}} \right)\, + \frac{1}{2\beta } - \frac{2c + M + 2}{{3\beta^{3} }} $$

(44)

The no-secular terms condition requires:

$$ \beta = \sqrt {c + M + 1} $$

(45)

Obviously, in the 1st-order HPM, once the secular terms (such as $\eta^{n} e^{( - m\eta )}$) are removed, the control is lost on the appearance of these terms in higher orders of approximation. Therefore, it is obtained with ease:

$$ f(\eta ) = f_{0} (\eta ) + f_{1} (\eta ) $$

(46)

$$ f^{{{\prime \prime }}} (0) = f_{0}^{{{\prime \prime }}} (0) + f_{1}^{{\prime }} (0) = - \frac{3M + 4c + 4}{{3\sqrt {M + c + 1} }} $$

(47)

The results will be compared later.

2.5 Solution by 2nd-order homotopy perturbation method (HPM)

Employing the parameter expansion method (see e.g. [16,17,18,19,20,21]), the operators in Eq. 35 are defined as:

$$ L[f(\eta )] = f^{{{\prime \prime \prime }}} (\eta ) + \left( { - \beta_{0}^{2} + p\beta_{1} } \right)f^{{\prime }} (\eta ) $$

(48)

$$ N[f(\eta )] = f^{{{\prime \prime \prime }}} (\eta ) + (c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) - Mf^{{\prime }} (\eta ) $$

(49)

By substitution:

$$ f^{{{\prime \prime \prime }}} (\eta ) + \left( { - \beta_{0}^{2} + p\beta_{1} } \right)f^{{\prime }} (\eta ) + p\left( {(c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) - Mf^{{\prime }} (\eta ) - \left( { - \beta_{0}^{2} + p\beta_{1} } \right)f^{{\prime }} (\eta )} \right) = 0 $$

(50)

The following zeroth, 1st and 2nd order systems are then immediate:

$$ \begin{aligned} & p^{0} :\,f_{0}^{{{\prime \prime \prime }}} \,\,(\eta ) - \beta_{0}^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{0} (0) = 0,\quad f_{0}^{{\prime }} (0) = 1,\quad f_{0}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(51)

$$ \begin{aligned} & p^{1} :\,f_{1}^{{{\prime \prime \prime }}} (\eta ) - \beta_{0}^{2} f_{1}^{{\prime }} (\eta ) + \left( {(c + 1)f_{0} (\eta )f_{0}^{{\prime }} (\eta ) - 2(c + 1)f_{0}^{{{\prime }2}} (\eta ) - Mf_{0}^{{\prime }} (\eta ) + \left( {\beta_{0}^{2} + \beta_{1} } \right)f_{0}^{{\prime }} (\eta )} \right) = 0 \\ & f_{1} (0) = 0,\quad f_{1}^{{\prime }} (0) = 0,\quad f_{1}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(52)

$$ \begin{aligned} & p^{2} :\,f_{2}^{{{\prime \prime \prime }}} (\eta ) - \beta_{0}^{2} f_{2}^{{\prime }} (\eta ) + \left( \begin{array}{l} (c + 1)\left( {f_{0} (\eta )f_{1}^{{{\prime \prime }}} (\eta ) + f_{1} (\eta )f_{0}^{{{\prime \prime }}} (\eta )} \right) - 2(c + 1)\left( {2f_{0}^{{\prime }} (\eta )f_{1}^{{\prime }} (\eta )} \right) - Mf_{1}^{{\prime }} (\eta ) \hfill \\ + \left( {\beta_{0}^{2} + \beta_{1} } \right)f_{1}^{{\prime }} (\eta ) - \beta_{1} \,f_{0}^{{\prime }} (\eta ) \hfill \\ \end{array} \right) = 0 \\ & f_{2} (0) = 0,\quad f_{2}^{{\prime }} (0) = 0,\quad f_{2}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(53)

Therefore, one reaches:

$$ f_{0} (\eta ) = \frac{1}{{\beta_{0} }}\left( {1 - e^{{ - \beta_{0} \eta }} } \right),\quad \beta_{0} > 0 $$

(54)

$$ \begin{aligned} f_{1} (\eta ) & = e^{{( - \beta_{0} \eta )}} \left( {\frac{{5 + 5c + 3M - 3\beta_{1} }}{{6\beta_{0}^{3} }} - \frac{1}{{2\beta_{0} }}} \right) - e^{{( - 2\beta_{0} \eta )}} \left( {\frac{c + 1}{{6\beta_{0}^{3} }}} \right) + \eta \,e^{{( - \beta_{0} \eta )}} \left( {\frac{ - 1}{2} + \frac{{1 + c + M - \beta_{1} }}{{2\beta_{0}^{2} }}} \right) \\ & \quad + \left( {\frac{{3\beta_{1} - 3M - 4c - 4}}{{6\beta_{0}^{3} }} + \frac{1}{{2\beta_{0} }}} \right) \\ \end{aligned} $$

(55)

$$ \begin{aligned} f_{2} (\eta ) & = -\, \eta^{2} e^{{( - \beta_{0} \eta )}} \frac{{\left( { - \beta_{0}^{2} + M - \beta_{1} + c + 1} \right)^{2} }}{{8\beta_{0}^{3} }} - e^{{( - 3\beta_{0} \eta )}} \frac{{\left( {c + 1} \right)^{2} }}{{48\beta_{0}^{5} }} + e^{{( - 2\beta_{0} \eta )}} \frac{{\left( {c + 1} \right)\left( { - 9\beta_{0}^{2} + 9M - 9\beta_{1} + 11c + 11} \right)}}{{36\beta_{0}^{5} }} \\ & \quad + \frac{1}{{72\beta_{0}^{5} }}\left( {K_{1} } \right) - \frac{{\eta \,e^{{( - \beta_{0} \eta )}} }}{{24\beta_{0}^{4} }}\left( {K_{2} } \right) - \frac{{e^{{( - \beta_{0} \eta )}} }}{{144\beta_{0}^{5} }}\left( {K_{3} } \right) + \eta \,e^{{( - 2\beta_{0} \eta )}} \frac{{\left( {c + 1} \right)\left( { - \beta_{0}^{2} + M - \beta_{1} + c + 1} \right)}}{{6\beta_{0}^{4} }} \\ K_{1} & = 27M^{2} - 54M\beta_{1} - 54M\beta_{0}^{2} + 72Mc + 72M + 27\beta_{1}^{2} + 18\beta_{1} \beta_{0}^{2} - 72\beta_{1} c - 72\beta_{1} + 27\beta_{0}^{4} - 72\beta_{0}^{2} c \\ & \quad - 72\beta_{0}^{2} + 52c^{2} + 104c + 52 \\ K_{2} & = 9M^{2} - 18M\beta_{1} - 18M\beta_{0}^{2} + 22Mc + 22M + 9\beta_{1}^{2} + 6\beta_{1} \beta_{0}^{2} - 22\beta_{1} c - 22\beta_{1} + 9\beta_{0}^{4} - 22\beta_{0}^{2} c \\ & \quad - 22\beta_{0}^{2} + 15c^{2} + 30c + 15 \\ K_{3} & = 54M^{2} - 108M\beta_{1} - 108M\beta_{0}^{2} + 180Mc + 180M + 54\beta_{1}^{2} + 36\beta_{1} \beta_{0}^{2} - 180\beta_{1} c - 180\beta_{1} \\ & \quad + 54\beta_{0}^{4} - 180\beta_{0}^{2} c - 180\beta_{0}^{2} + 145c^{2} + 290c + 145 \\ \end{aligned} $$

(56)

Removing the secular terms requires:

$$ \beta_{0} = \sqrt {\left( {\frac{3K - 1}{6}} \right) + \sqrt {\left( {\frac{3K - 1}{6}} \right)^{2} + \frac{{\left( { - 22 - 44M + 44K(c + 1) - 44Mc + 36MK - 30c^{2} - 18K^{2} - 60c - 18M^{2} } \right)}}{24}} } $$

(57)

where

$$ K = c + 1 + M,\quad \beta_{1} = K - \beta_{0}^{2} $$

(58)

The approximate solution reads:

$$ f(\eta ) = f_{0} (\eta ) + f_{1} (\eta ) + f_{2} (\eta ) $$

(59)

Finally, the engineering quantity of interest is obtained as:

$$ f^{{{\prime \prime }}} (0) = f_{0}^{{{\prime \prime }}} (0) + f_{1}^{{{\prime \prime }}} (0) + f_{2}^{{{\prime \prime }}} (0) = \frac{{\left( {\begin{array}{*{20}c} {9M^{2} - 18M\beta_{1} - 54M\beta_{0}^{2} + 30Mc + 30M + 9\beta_{1}^{2} + 18\beta_{1} \beta_{0}^{2} - 30\beta_{1} c} \\ { - 30\beta_{1} - 27\beta_{0}^{4} - 90\beta_{0}^{2} c - 90\beta_{0}^{2} + 26c^{2} + 52c + 26} \\ \end{array} } \right)}}{{72\beta_{0}^{3} }} $$

(60)

2.6 Solution by the homotopy contraction mapping technique (HCMT)

Let us take $\zeta = f^{{{\prime \prime }}} (0)$. The basic homotopy structure is:

$$ (1 - p)L\,[f,\alpha ] = - p\,N\,[f] $$

(61)

We choose:

$$ L\,[f,\alpha ] = \frac{{\partial^{3} f}}{{\partial \eta^{3} }} - \alpha^{2} \frac{\partial f}{\partial \eta } $$

(62)

$$ N[f(\eta )] = f^{{{\prime \prime \prime }}} (\eta ) + (c + 1)f(\eta )f^{{{\prime \prime }}} (\eta ) - 2(c + 1)f^{{{\prime }2}} (\eta ) - Mf^{{\prime }} (\eta ) $$

(63)

Substituting Eqs. 63 and 62 into Eq. 61 and further employing the standard expansion technique and collecting terms with like power of $p$ (or Taylor method and in HAM) the following systems are reduced:

$$ \begin{aligned} & p^{0} :\,f_{0}^{{{\prime \prime \prime }}} (\eta ) - \alpha^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{0} (0) = 0,\quad f_{0}^{{\prime }} (0) = 1,\quad f_{0}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(64)

$$ \begin{aligned} & p^{1} :\,f_{1}^{{{\prime \prime \prime }}} (\eta ) - \alpha^{2} f_{1}^{{\prime }} (\eta ) + \left( {(c + 1)f_{0} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) - 2(c + 1)f_{0}^{{{\prime }2}} (\eta ) - Mf_{0}^{{\prime }} (\eta ) + \alpha^{2} f_{0}^{{\prime }} (\eta )} \right) = 0 \\ & f_{1} (0) = 0,\quad f_{1}^{{\prime }} (0) = 0,\quad f_{1}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(65)

$$ \begin{aligned} & p^{2} :\,f_{2}^{{{\prime \prime \prime }}} (\eta ) - \alpha^{2} f_{2}^{{\prime }} (\eta ) + \left( {(c + 1)\left( {f_{1} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) + f_{0} (\eta )f_{1}^{{{\prime \prime }}} (\eta )} \right) - 2(c + 1)\left( {2f_{0}^{{\prime }} (\eta )f_{1}^{{\prime }} (\eta )} \right) - Mf_{1}^{{\prime }} (\eta ) + \alpha^{2} f_{1}^{{\prime }} (\eta )} \right) = 0 \\ & f_{2} (0) = 0,\quad f_{2}^{{\prime }} (0) = 0,\quad f_{2}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(66)

and so on (P.N. that the higher deformation equations are exactly those in the equivalent HAM system).

The technique is to apply the following mapping scheme (see Theorem 2) with

$$ T_{m} \left( {\zeta_{0} } \right) = \sum\limits_{i = 0}^{m} {\zeta_{i} } :T_{m \ge 1}^{n \to \infty } \zeta_{0} = T_{m} oT_{m} oT_{m} o \ldots T_{m} \left( {\zeta_{0} } \right) = \zeta^{*,m} = C_{m} $$

(67)

or

$$ \zeta_{0,n + 1} = T_{m} \left( {\zeta_{0,\,n} } \right),\quad n = 0,1,2, \ldots ,:\zeta_{0,\,n \to \infty } = \zeta^{*,m} = C_{m} $$

(68)

$\zeta_{0} \left( { = f_{0}^{{{\prime \prime }}} (0)} \right),\zeta_{1} ,\zeta_{2} , \ldots$ are functions of $\alpha$; hence, if there exist some $\zeta_{0} = g(\alpha )$ convergent to a certain value through (67) or (68), the value is regarded as the fixed point of $T_{m}$ and is the unique description of the series solution for $\zeta = f^{{{\prime \prime }}} (0)$ at the mth order of truncation; if not, the zeroth term/leading term is Not potential to contain the target quantity $\zeta = f^{{{\prime \prime }}} (0)$. In this situation, the linear operator and/or the target quantity should change.

First assume that the series is truncated at the 1st order:

$$ f_{0}^{{{\prime \prime }}} (0) = - \alpha $$

(69)

$$ \sum\limits_{i = 0}^{1} {f_{i}^{{{\prime \prime }}} (0)} = f_{0}^{{{\prime \prime }}} (0) + f_{1}^{{{\prime \prime }}} (0) = \chi_{1} \left( {f_{0}^{{{\prime \prime }}} (0)} \right) = \frac{{\left( {3f_{0}^{{{\prime \prime }}} (0)^{2} + 3M + 5c + 5} \right)}}{{6f_{0}^{{{\prime \prime }}} (0)}} $$

(70)

Therefore, the contraction mapping is checked through:

$$ T_{1}^{n \to \infty } f_{0}^{\prime \prime } (0) = T_{1} oT_{1} oT_{1} o \ldots T_{1} \left( {f_{0}^{\prime \prime } (0)} \right) = f_{0}^{\prime \prime *,1} (0) = C_{1} $$

(71)

or

$$ f_{0,n + 1}^{\prime \prime } (0) = T_{1} \left( {f_{0,n}^{\prime \prime } (0)} \right),\quad n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{\prime \prime } (0) = f^{\prime \prime *,1} (0) = C_{1} $$

(72)

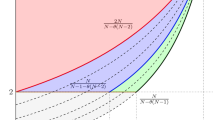
In this particular example, it can be simply checked that (71) is convergent for any arbitrary choice for $f_{0}^{\prime \prime } (0)$ (see Fig. 2). Moreover, for the 1st order of truncation, it is possible to establish an analytic relation for $f^{\prime \prime } (0)$ since $\sum\nolimits_{i = 1}^{m} {\zeta_{i} = 0}$ is solvable:

$$ \sum\limits_{i = 1}^{1} {f_{i}^{\prime \prime } (0)} = f_{1}^{\prime \prime } (0) = \frac{{\left( { - 3f_{0}^{\prime \prime } (0)^{2} + 3M + 5c + 5} \right)}}{{6f_{0}^{\prime \prime } (0)}} = 0 \Rightarrow f_{0}^{\prime \prime } (0) = - \sqrt {\frac{3M + 5c + 5}{3}} $$

(73)

Therefore, for the 1st order solution:

$$ f^{\prime \prime *,1} (0) = - \sqrt {\frac{3M + 5c + 5}{3}} $$

(74)

As a comparison, one may check that for the case with $c = M = 0$ ($f^{\prime \prime } (0) = - 1.2818$), Eq. 74 gives: $f^{\prime \prime } (0) = - \sqrt {\frac{5}{3}} \approx - 1. 2 9 0 9 9$ which is much better than the 1st order HPM (Eq. 47 shows $f^{\prime \prime } (0) = - \frac{4}{3} = - 1. {\bar{\text{3}}}$). Further it is notable that this accuracy is close to the 2nd order HPM (Eq. 60 gives: $f^{\prime \prime } (0) \approx - 1.2752$).

Fig. 2

$f_{0,n + 1}^{\prime \prime } (0) = T_{1} \left( {f_{0,n}^{{{\prime \prime }}} (0)} \right),\;n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{{{\prime \prime }}} (0) = f^{{{\prime \prime }*,1}} (0) = C_{1}$, X-axis is the iteration ($n$): $c = M = 0$

In order to increase the accuracy, 5th order of approximation was computed. For this:

$$ T_{5}^{n \to \infty } f_{0}^{\prime \prime } (0) = T_{5} oT_{5} oT_{5} o \ldots T_{5} \left( {f_{0}^{\prime \prime } (0)} \right) = f^{\prime \prime *,5} (0) = C_{5} $$

(75)

or

$$ f_{0,n + 1}^{\prime \prime } (0) = T_{5} \left( {f_{0,n}^{\prime \prime } (0)} \right),\quad n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{\prime \prime } (0) = f^{\prime \prime *,5} (0) = C_{5} $$

(76)

It was computed that:

$$ T_{5} \left( {f_{0}^{\prime \prime } (0)} \right) = \overbrace {{\frac{{ - P\left( {f_{0}^{\prime \prime } (0)} \right)}}{{108864000f_{0}^{\prime \prime } (0)^{9} }}}}^{{\sum\nolimits_{i = 1}^{5} {f_{i}^{\prime \prime } (0)} }} + f_{0}^{\prime \prime } (0) $$

(77)

with

$$ P\left( {f_{0}^{\prime \prime } (0)} \right) = A_{0} + A_{1} f_{0}^{\prime \prime } (0)^{2} + A_{2} f_{0}^{\prime \prime } (0)^{4} + A_{3} f_{0}^{\prime \prime } (0)^{6} + A_{4} f_{0}^{\prime \prime } (0)^{8} + A_{5} f_{0}^{\prime \prime } (0)^{10} $$

(78)

$$ A_{0} = - \left( {\begin{array}{*{20}c} {2976750M^{5} + 24806250M^{4} (1 + c) + 85995000M^{3} (1 + c)^{2} } \\ { + 151814250M^{2} (1 + c)^{3} + 135329670M(1 + c)^{4} + 48539791(1 + c)^{5} } \\ \end{array} } \right) $$

(79)

$$ A_{1} = \left( {\begin{array}{*{20}c} {173995290(1 + c)^{4} + 19136250M^{4} + 127575000M^{3} (1 + c)} \\ { + 331695000M^{2} (1 + c)^{2} + 390379500M(1 + c)^{3} } \\ \end{array} } \right) $$

(80)

$$ A_{2} = - \left( {\begin{array}{*{20}c} {53581500M^{3} + 267907500M^{2} (1 + c) + 464373000M(1 + c)^{2} } \\ { + 273265650(1 + c)^{3} } \\ \end{array} } \right) $$

(81)

$$ A_{3} = \left( {89302500M^{2} + 297675000M(1 + c) + 257985000(1 + c)^{2} } \right) $$

(82)

$$ A_{4} = - \left( {133953750M + 223256250(1 + c)} \right) $$

(83)

$$ A_{5} = \left( { - 26790750 + 108864000} \right) $$

(84)

The fixed point is one of the real polynomial roots ($\sum\nolimits_{i = 1}^{5} {f_{i}^{\prime \prime } (0) = 0 \Rightarrow P\left( {f_{0}^{\prime \prime } (0)} \right) = 0}$) which certainly shows itself through the recursive process (see Fig. 3). By analysis, 5th order of approximation in each certain values of $c$ and $M$ gives solutions in which the maximum deviation, compared to the high-order HAM results, Does Not exceed 0.03%.

Fig. 3

$f_{0,n + 1}^{\prime \prime } (0) = T_{5} \left( {f_{0,n}^{\prime \prime } (0)} \right),\,n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{\prime \prime } (0) = f^{\prime \prime *,5} (0) = C_{5}$, X-axis is the iteration ($n$): $c = M = 0$

2.7 A perturbation solution valid for large $M$

Introducing the new variable $\xi = \sqrt M \eta$ Eq. 4 and the associated boundary conditions become:

$$ \begin{aligned} & f^{{{\prime \prime \prime }}} (\xi ) - f^{{\prime }} (\xi ) + \varepsilon \left( {(c + 1)f(\xi )f^{{{\prime \prime }}} (\xi ) - 2(c + 1)f^{{{\prime }2}} (\xi )} \right) = 0 \\ & f(0) = 0,\quad f^{{\prime }} (0) = \frac{1}{\sqrt M },\quad f^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(85)

Let us choose:

$$ \varepsilon = \frac{1}{\sqrt M }:M \to \infty \Rightarrow \varepsilon \to 0 $$

(86)

Employing the standard expansion $f(\xi ) = f_{0} (\xi ) + \varepsilon f_{1} (\xi ) + \varepsilon^{2} f_{2} (\xi ) + \cdots$ the following zeroth and 1st order systems can be obtained:

$$ \begin{aligned} & f_{0}^{{{\prime \prime \prime }}} (\xi ) - f_{0}^{{\prime }} (\xi ) = 0 \\ & f_{0} (0) = 0,\quad f_{0}^{{\prime }} (0) = \frac{1}{\sqrt M },\quad f_{0}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(87)

$$ \begin{aligned} & f_{1}^{{{\prime \prime \prime }}} (\xi ) - f_{1}^{{\prime }} (\xi ) + \left( {(c + 1)f_{0} (\xi )f_{0}^{{{\prime \prime }}} (\xi ) - 2(c + 1)f_{0}^{{{\prime 2}}} (\xi )} \right) = 0 \\ & f_{1} (0) = 0,\quad f_{1}^{{\prime }} (0) = 0,\quad f_{1}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(88)

By solution:

$$ f(\xi ) \approx f_{0} (\xi ) + \varepsilon f_{1} (\xi ) = \frac{1}{\sqrt M }\left( {\left( {1 - e^{ - \xi } } \right) + \frac{5(c + 1)}{6M}e^{ - \xi } - \frac{2(c + 1)}{3M} + \frac{(c + 1)}{2M}\xi e^{ - \xi } - \frac{(c + 1)}{6M}e^{ - 2\xi } } \right) $$

(89)

Retrieving the original variable it is obtained with ease:

$$ f^{{{\prime \prime }}} (0) = - \sqrt M - \frac{5(c + 1)}{6\sqrt M } $$

(90)

2.8 Numerical solutions

Equation 4 is initially considered in the following form:

$$ F = f(\eta ) $$

(91)

$$ F^{{\prime }} = Y $$

(92)

$$ Y^{{\prime }} = Z $$

(93)

$$ Z^{{\prime }} + (c + 1)FZ - 2(c + 1)\,Y^{2} - MY = 0 $$

(94)

$$ F(0) = 0,\quad Y(0) = 1,\quad Z(0) = \alpha $$

(95)

$\alpha$ is initially missing; hence, a shooting procedure is required to treat the problem as an IVP. Besides, one of the basic challenges to obtain much more accurate results is to deal with the boundary condition as $\eta \to \infty$, namely, $\eta_{\infty }$ (usually a trial and error procedure is followed to handle this situation). In fact, changing the involved parameters will affect the boundary layer thickness; i.e. the real value of $\left| {F^{{\prime }} (\eta_{\infty } )} \right|$ in a fixed $\eta_{\infty }$ (e.g. $\eta_{\infty } = 10$) is highly dependent on $c$ and $M$, expressing rather drastic behaviors. Therefore, in order to retain the same representation of the real solution at the infinity while changing the engaged parameters and searching for a suitable zeroth correspondence ($\alpha$), $\eta_{\infty }$ (with a fixed error tolerance) or the error tolerance (with a fixed $\eta_{\infty }$) should also change. Here, it is suggested using the 1st-order HPM results and following the former scenario to handle the uncertainties. In the present study, on using Eqs. 43–45, the following scheme was considered with $\left| {F^{{\prime }} (\eta_{\infty } )} \right| = 10^{ - 6}$:

$$ \eta_{\infty } = \frac{1}{{\left( {1 + c + M} \right)}}Ln\left[ {\frac{{\left( {2 + 2c + 3M} \right)}}{{3\left( {1 + c + M} \right)\left( {\left| {F^{{\prime }} (\eta_{\infty } )} \right|} \right)}}} \right] $$

(96)

5th order Runge–Kutta was employed for discretization purpose. An iterative shooting procedure (to the 5th decimal place) was then pursued.

Table 2 shows a comparison between the methods.

Table 2 Comparison of the applied methods for $-\, f^{{{\prime \prime }}} (0)$ where $c = 0.5$

2.9 Ex. 2: The 2-D flow of UCM fluid over a linearly stretching sheet

The basic equations for such a flow can be found in several studies e.g. [43,44,45,46,47,48]:

$$ f^{{{\prime \prime \prime }}} (\eta ) + f(\eta )f^{{{\prime \prime }}} (\eta ) - f^{{\prime }} (\eta )^{2} + \beta \left( {2f(\eta )f^{{\prime }} (\eta )f^{{{\prime \prime }}} (\eta ) - f(\eta )^{2} f^{{{\prime \prime \prime }}} (\eta )} \right) = 0 $$

(97)

The associated boundary conditions are:

$$ f(0) = 0,\quad f^{{\prime }} (0) = 1,\quad f^{{\prime }} (\infty ) = 0 $$

(98)

In above, $\beta$ is Deborah number.

2.10 Solution by homotopy analysis method (HAM)

The auxiliary linear operator and the initial guess are chosen as before:

$$ f_{0} (\eta ) = 1 - e^{ - \eta } $$

(99)

$$ L(f) = f^{{{\prime \prime \prime }}} (\eta ) - f^{{\prime }} (\eta ) $$

(100)

Following Liao (see e.g. [1,2,3,4,5,6,7,8]), the homotopy is constructed as:

$$ (1 - p)L[\bar{f}(\eta ,p) - f_{0} (\eta )] = p\hbar N[\bar{f}(\eta ,p)] $$

(101)

with

$$ \bar{f}(0,p) = 0,\quad \bar{f}^{{\prime }} (0,p) = 1,\quad \bar{f}(\infty ,p) = 0 $$

(102)

In above, the nonlinear operator is:

$$ N[\bar{f}(\eta ,p)] = \frac{{\partial^{3} \bar{f}}}{{\partial \eta^{3} }} + \bar{f}(\eta ,p)\frac{{\partial^{2} \bar{f}}}{{\partial \eta^{2} }} - \left( {\frac{{\partial \bar{f}}}{\partial \eta }} \right)^{2} + \beta \left( {2\bar{f}(\eta ,p)\left( {\frac{{\partial \bar{f}}}{\partial \eta }} \right)\frac{{\partial^{2} \bar{f}}}{{\partial \eta^{2} }} - \bar{f}(\eta ,p)^{2} \frac{{\partial^{3} \bar{f}}}{{\partial \eta^{3} }}} \right) $$

(103)

Following the same procedure, as in the previous problem, for the mth-order deformation one obtains:

$$ L[f_{m} (\eta ) - \chi_{m} f_{m - 1} (\eta )] = \hbar \Re_{m} (\eta ) $$

(104)

With the boundary conditions:

$$ f_{m} (0) = f_{m}^{{\prime }} (0) = f_{m}^{{\prime }} (\infty ) = 0 $$

(105)

where

$$ \chi_{m} = \left\{ {\begin{array}{*{20}l} 0 \hfill & {m \le 1} \hfill \\ 1 \hfill & {m > 2} \hfill \\ \end{array} } \right. $$

(106)

$$ \begin{aligned} \Re_{m} (\eta ) & = f_{m - 1}^{{{\prime \prime \prime }}} (\eta ) + \sum\limits_{k = 0}^{m - 1} {\left\{ {\left( {f_{m - 1 - k} (\eta )} \right)f_{k}^{{\prime }} (\eta )} \right\}} - \sum\limits_{k = 0}^{m - 1} {\left\{ {\left( {f_{m - 1 - k}^{{\prime }} (\eta )} \right)f_{k}^{{\prime }} (\eta )} \right\}} \\ & \quad + \beta \sum\limits_{k = 0}^{m - 1} {\left\{ {\left( {f_{m - 1 - k} (\eta )} \right)\sum\limits_{l = 0}^{k} {\left\{ {2f_{k - 1}^{{\prime }} (\eta )f_{l}^{{{\prime \prime }}} (\eta ) - f_{k - l} (\eta )f_{l}^{{{\prime \prime }}} (\eta )} \right\}} } \right\}} \\ \end{aligned} $$

(107)

Figures 4 and 5 show the behavior of the so-called $\hbar$-curves for 2 different stages. In addition, Table 3 provides the behavior of this significant quantity of interest in various stages.

Fig. 4

$\hbar$-curve for $\beta = 1/10$ in 10th order of approximation: X-axis and Y-axis are $\hbar$ and $f^{{{\prime \prime }}} (0)$ respectively

Fig. 5

$\hbar$-curve for $\beta = 1$ in 10th order of approximation: X-axis and Y-axis are $\hbar$ and $f^{{{\prime \prime }}} (0)$ respectively

Table 3 $f^{{{\prime \prime }}} (0)$ in different stages, secured to the 4th decimal place after 15th-order of approximation

Table 4 Comparison of the applied methods for $- f^{{{\prime \prime }}} (0)$

2.11 Solution by 1st-order homotopy perturbation method (HPM)

Let us consider the following classic homotopy transformation:

$$ f^{{{\prime \prime \prime }}} (\eta ) - k^{2} f^{{\prime }} (\eta ) + p\left( {f(\eta )f^{{{\prime \prime }}} (\eta ) - f^{{\prime }} (\eta )^{2} + \beta \left( {2f(\eta )f^{{\prime }} (\eta )f^{{{\prime \prime }}} (\eta ) - f(\eta )^{2} f^{{{\prime \prime \prime }}} (\eta )} \right) + k^{2} f^{{\prime }} (\eta )} \right) = 0,\quad k > 0 $$

(108)

With the standard expansion:

$$ f(\eta ) = f_{0} (\eta ) + pf_{1} (\eta ) + p^{2} f_{2} (\eta ) + \cdots $$

(109)

The following zeroth and 1st order systems are then immediate:

$$ \begin{aligned} & f_{0}^{{{\prime \prime \prime }}} (\eta ) - k^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{0} (0) = 0,\quad f_{0}^{{\prime }} (0) = 1,\quad f^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(110)

$$ \begin{aligned} & f_{1}^{{{\prime \prime \prime }}} (\eta ) - k^{2} f_{1}^{{\prime }} (\eta ) + f_{0} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) - f_{0}^{{\prime }} (\eta )^{2} + \beta \left( {2f_{0} (\eta )f_{0}^{{\prime }} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) - f_{0} (\eta )^{2} f_{0}^{{{\prime \prime \prime }}} (\eta )} \right) + k^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{1} (0) = f_{1}^{{\prime }} (0) = f_{1}^{{{\prime \prime }}} (\infty ) = 0 \\ \end{aligned} $$

(111)

Upon solving the above systems and removing the secular terms it is eventually obtained with ease:

$$ f^{{{\prime \prime }}} (0) = f_{0}^{{{\prime \prime }}} (0) + f_{1}^{{{\prime \prime }}} (0) = - \frac{4 + 3\beta }{{4\sqrt {1 + \beta } }} $$

(112)

2.12 Solution by HCMT

Consider the basic homotopy structure $(1 - p)L[f,\alpha ] = - pN[f]$ with $L\,[f,\alpha ] = \frac{{\partial^{3} f}}{{\partial \eta^{3} }} - \alpha^{2} \frac{\partial f}{\partial \eta }$ and $N[f] = f^{{{\prime \prime \prime }}} + ff^{{{\prime \prime }}} - f^{{{\prime }2}} + \beta \left( {2ff^{{\prime }} f^{{{\prime \prime }}} - f^{2} f^{{{\prime \prime \prime }}} } \right)$.

Employing the standard expansion $f = f_{0} + pf_{1} + p^{2} f_{2} + \cdots$ it is obtained:

$$ \begin{aligned} & f_{0}^{{{\prime \prime \prime }}} (\eta ) - \alpha^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{0} (0) = 0,\quad f_{0}^{{\prime }} (0) = 1,\quad f^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(113)

$$ \begin{aligned} & f_{1}^{{{\prime \prime \prime }}} (\eta ) - \alpha^{2} f_{1}^{{\prime }} (\eta ) + f_{0} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) - f_{0}^{{\prime }} (\eta )^{2} + \beta \left( {2f_{0} (\eta )f_{0}^{{\prime }} (\eta )f_{0}^{{{\prime \prime }}} (\eta ) - f_{0} (\eta )^{2} f_{0}^{{{\prime \prime }}} (\eta )} \right) + \alpha^{2} f_{0}^{{\prime }} (\eta ) = 0 \\ & f_{1} (0) = f_{1}^{{\prime }} (0) = f_{1}^{{\prime }} (\infty ) = 0 \\ \end{aligned} $$

(114)

and so on.

The contraction mapping is checked through:

$$ T_{m \ge 1}^{n \to \infty } \zeta_{0} = T_{m} oT_{m} oT_{m} o \ldots T_{m} \left( {\zeta_{0} } \right) = \zeta^{*,m} = C_{m} $$

(115)

or:

$$ \zeta_{0,n + 1} = T_{m} \left( {\zeta_{0,\,n} } \right),\quad n = 0,1,2, \ldots ,:\zeta_{0,n \to \infty } = \zeta^{*,m} = C_{m} $$

(116)

Let us choose $\zeta = f^{{{\prime \prime }}} (0)$ and the 1st order approximation reads:

$$ f_{0}^{{{\prime \prime }}} (0) = - \alpha $$

(117)

$$ \sum\limits_{i = 0}^{1} {f_{i}^{\prime \prime } (0)} = f_{0}^{\prime \prime } (0) + f_{1}^{\prime \prime } (0) = T_{1} \left( {f_{0}^{{{\prime \prime }}} (0)} \right) = \frac{{\left( {2f_{0}^{{{\prime \prime }}} (0)^{2} + \beta + 2} \right)}}{{4f_{0}^{{{\prime \prime }}} (0)}} $$

(118)

Therefore:

$$ T_{1}^{n \to \infty } f_{0}^{{{\prime \prime }}} (0) = T_{1} oT_{1} oT_{1} o \ldots T_{1} \left( {f_{0}^{{{\prime \prime }}} (0)} \right) = f^{{{\prime \prime }*,1}} (0) = C_{1} $$

(119)

or

$$ f_{0,n + 1}^{\prime \prime } (0) = T_{1} \left( {f_{0,n}^{{{\prime \prime }}} (0)} \right),\quad n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{{{\prime \prime }}} (0) = f^{{{\prime \prime }*,1}} (0) = C_{1} $$

(120)

Figure 6 shows the contraction mapping behavior.

Fig. 6

$f_{0,n + 1}^{\prime \prime } (0) = T_{1} \left( {f_{0,n}^{{{\prime \prime }}} (0)} \right),\;n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{{{\prime \prime }}} (0) = f^{{{\prime \prime }*,1}} (0) = C_{1}$, X-axis is the iteration ($n$): $\beta = 1/2$

By Theorem 2, since the 1st order system is solvable:

$$ \sum\limits_{i = 1}^{1} {f_{i}^{{{\prime \prime }}} (0)} = f_{1}^{{{\prime \prime }}} (0) = \frac{{\left( { - 2f_{0}^{{{\prime \prime }}} (0)^{2} + \beta + 2} \right)}}{{4f_{0}^{{{\prime \prime }}} (0)}} = 0 \Rightarrow f_{0}^{{{\prime \prime }}} (0) = f^{{{\prime \prime }*,1}} (0) = - \sqrt {\frac{\beta + 2}{2}} $$

(121)

In order to increase the accuracy, 4th order of approximation was computed. For this:

$$ T_{4}^{n \to \infty } f_{0}^{{{\prime \prime }}} (0) = T_{4} oT_{4} oT_{4} o \ldots T_{4} \left( {f_{0}^{{{\prime \prime }}} (0)} \right) = f^{{{\prime \prime }*,4}} (0) = C_{4} $$

(122)

or

$$ f_{0,n + 1}^{{{\prime \prime }}} (0) = T_{4} \left( {f_{0,n}^{{{\prime \prime }}} (0)} \right),\quad n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{{{\prime \prime }}} (0) = f^{{{\prime \prime }*,4}} (0) = C_{4} $$

(123)

It was computed that:

$$ T_{4} \left( {f_{0}^{{{\prime \prime }}} (0)} \right) = \overbrace {{\frac{{ - P(f_{0}^{{{\prime \prime }}} (0))}}{{1016064000f_{0}^{{{\prime \prime }}} (0)^{7} }}}}^{{\sum\nolimits_{i = 1}^{4} {f_{i}^{{{\prime \prime }}} (0)} }} + f_{0}^{{{\prime \prime }}} (0) $$

(124)

with

$$ P\left( {f_{0}^{{{\prime \prime }}} (0)} \right) = A_{0} + A_{1} f_{0}^{{{\prime \prime }}} (0)^{2} + A_{2} f_{0}^{{{\prime \prime }}} (0)^{4} + A_{3} f_{0}^{{{\prime \prime }}} (0)^{6} + A_{4} f_{0}^{{{\prime \prime }}} (0)^{8} $$

(125)

where

$$ A_{0} = - \left( {3928575\beta^{4} + 12315190\beta^{3} - 11915364\beta^{2} - 59037048\beta - 39690000} \right) $$

(126)

$$ A_{1} = \left( {14553000\beta^{3} - 52955280\beta^{2} - 271902960\beta - 222264000} \right) $$

(127)

$$ A_{2} = \left( {64827000\beta^{2} + 500094000\beta + 555660000} \right) $$

(128)

$$ A_{3} = - \left( {555660000\beta + 1111320000} \right) $$

(129)

$$ A_{4} = - 277830000 + 1016064000 $$

(130)

The fixed point is one of the real polynomial roots ($\sum\nolimits_{i = 1}^{4} {f_{i}^{{{\prime \prime }}} (0) = 0 \Rightarrow P\left( {f_{0}^{{{\prime \prime }}} (0)} \right) = 0}$) which certainly shows itself through the recursive process (see Fig. 7). By analysis, 4th order of approximation in each given value of $\beta$ shows solutions in which the maximum deviation (up to $\beta = 5$), compared to the high-order HAM results, Does Not exceed 0.1%; e.g. for $\beta = 5$ it was computed by HAM after handling the controlling parameter and from the 25th-order of approximation that $f^{{{\prime \prime }}} (0) = - 1.9424$, whist the new technique shows $f^{{{\prime \prime }}} (0) = - 1.9443$ which is indeed a good match, still below 0.1% error.

Fig. 7

$f_{0,n + 1}^{\prime \prime } (0) = T_{4} \left( {f_{0,n}^{{{\prime \prime }}} (0)} \right),\,n = 0,1,2, \ldots ,:f_{0,n \to \infty }^{{{\prime \prime }}} (0) = f^{{{\prime \prime }*,4}} (0) = C_{4}$, X-axis is the iteration ($n$): $\beta = 1/2$

2.13 Numerical solutions

Equation 97 is initially expressed as:

$$ F = f(\eta ) $$

(131)

$$ F^{{\prime }} = Y $$

(132)

$$ Y^{{\prime }} = Z $$

(133)

$$ \left( {1 - F^{2} \beta } \right)Z^{{\prime }} + \left( {1 + 2\beta \,\,Y} \right)FZ - Y^{2} = 0 $$

(134)

$$ F(0) = 0,\quad Y(0) = 1,\quad Z(0) = \alpha $$

(135)

Following the instruction given in the previous section, from the 1st-order HPM solution the following scheme with $\left| {F^{\prime}(\eta_{\infty } )} \right| = 10^{ - 6}$ is considered:

$$ \eta_{\infty } = \frac{1}{{\sqrt {\beta + 1} }}Ln\left[ {\frac{(8 + 9\beta )}{{8(\beta + 1)\left| {F^{\prime}(\eta_{\infty } )} \right|}}} \right] $$

(136)

5th order Runge–Kutta was employed for discretization purpose. An iterative shooting procedure (to the 5th decimal place) was then pursued.

Table 4 shows a comparison between the methods. It should be also noted that for $\beta = 0$, Eq. 97 shows a well-known closed form solution in the form of $f(\eta ) = 1 - e^{ - \eta }$; having No secular terms, a point which provides advantage for HPM in such a nonlinear problem, as well as serving as a suitable initial guess in HAM. Therefore, the nonlinearity seems to be quite simpler that the previous one.

3 Conclusion

The aim of the present work was to account the easily-iterative HAM system to be further analyzed for some novel and untapped insights. As a gist, the insight was that the zeroth order term may be potential to contain a certain quantity of the exact solution such as $f^{{{\prime \prime }}} (0)$ that is normally sought. Through examples we saw that $f_{0}^{{{\prime \prime }}} (0) = - \alpha$. The new insight simply states that $f_{0}^{{{\prime \prime }}} (0)$ could be $f^{{{\prime \prime }}} (0)$ truncated at any homotopy series order; i.e. $f^{{{\prime \prime }*,m}} (0)$. This hypothesis is checked through Theorem 2 and $f^{{{\prime \prime }}} (0)$ is extracted which is regarded as the unique description of the homotopy series solution for $f^{{{\prime \prime }}} (0)$ in an order of approximation.

It was shown that unique description of the homotopy series solutions is simply behind a topological feature, the Fixed Point Property, indicating that the homotopy is self-corrector for some certain quantities of the exact solution (here the target quantity was $f^{{{\prime \prime }}} (0)$). Examples were provided revealing that the present approach is indeed promising.