The Integral Manifolds of the 4 Body Problem with Equal Masses: Bifurcations at Infinity

Abstract

In the N-body problem, it is classical that there are conserved quantities of center of mass, linear momentum, angular momentum and energy. The level sets $\mathfrak {M}(c,h)$ of these conserved quantities are parameterized by the angular momentum c and the energy h, and are known as the integral manifolds. A long-standing goal has been to identify the bifurcation values, especially the bifurcation values of energy for fixed non-zero angular momentum, and to describe the integral manifolds at the regular values. Alain Albouy identified two categories of singular values of energy: those corresponding to bifurcations at relative equilibria; and those corresponding to “bifurcations at infinity”, and demonstrated that these are the only possible bifurcation values. This work examines the bifurcations for the four body problem with equal masses. There are four singular values corresponding to bifurcations at infinity. To establish that the topology of the integral manifolds changes at each of these values, and to describe the manifolds at the regular values of energy, the homology groups of the integral manifolds are computed for the five energy regions on either side of the singular values. The homology group calculations establish that all four energy levels are indeed bifurcation values, and allows some of the global properties of the integral manifolds to be explored. A companion paper will provide the corresponding analysis of the bifurcations at relative equilibria for the four-body problem with equal masses.

1 Introduction

This work continues the investigation of the integral manifolds of the spatial N-body problem. The integral manifolds are the level sets of the classical conserved quantities of energy, angular momentum, linear momentum and center of mass. In the spatial problem, they form a family of $(6N - 10)$-dimensional manifolds. Their structure depends on the values $m_1, \ldots m_N$ of the masses, the angular momentum $\vec {c} \in \mathbb {R}^3$ and energy h. Typically, the dependence on the masses is not displayed explicitly, and the integral manifolds are viewed as a parameterized family $\mathfrak {M}(c,h)$.

The integral manifolds for $c = 0$ have been characterized in [3]. The focus of this work is on $c\ne 0$. The N-body problem admits a rotational symmetry. This allows the system to be rotated so that the angular momentum vector is oriented along $\hat{k}$. Once that orientation is fixed, the $SO_3$ symmetry reduces to an $SO_2$-symmetry of rotations about the z-axis. The equations of motion and the conserved quantities are all preserved by rotation, so there are well-defined dynamics on the reduced integral manifold $\mathfrak {M}_R(c,h) = \mathfrak {M}(c,h)/SO_2$.

In addition to the rotational symmetry, a global rescaling can be applied, mapping $\mathfrak {M}(c,h)$ homeomorphically to $\mathfrak {M}(\frac{c}{\sqrt{\lambda }}, \lambda h)$. That is, the topology of $\mathfrak {M}(c,h)$ depends only on the one-parameter family $\nu = c^2 h$. In this work, we will view c as fixed at a non-zero value, so that $\mathfrak {M}(c,h)$ can be viewed as a one-parameter family of energy level sets on a manifold of fixed angular momentum, center of mass and center of linear momentum.

In this setting, the questions of interest for the global behavior are:

• Identify the bifurcation values of h—the values at which the topology of $\mathfrak {M}(c,h)$ and $\mathfrak {M}_R(c,h)$ change.

• At the regular values of h between those bifurcation values, describe $\mathfrak {M}(c,h)$ and $\mathfrak {M}_R(c,h)$.

• Use that global description to provide insights into the global dynamics of the N-body problem.

The starting point for this program is [1]. There, Albouy produced necessary conditions for an energy level h to be a bifurcation value for $\mathfrak {M}(c,h)$. Those conditions are formulated in detail in Sect. 2. At the moment, it suffices to take note of two observations:

• As the angular momentum manifolds are not compact, singular values can occur either as critical values of H restricted to the angular momentum manifold; or as limiting values of sequences that are unbounded on the angular momentum manifold, where the gradient of H along the angular momentum manifold tends to zero. The former are referred to as “finite bifurcations”, the latter as “bifurcations at infinity”¹

• For a given set of masses $m_1, \ldots m_N$, there is an alogorithm that identifies all singular values. That algorithm requires knowledge of the full set of planar central configurations, both for that set of masses and for all of its subsets.

At present, there are no results on sufficient conditions analogous to Albouy’s necessary condition. That is, there is no formula or algorithm that produces a set of energy levels that are guaranteed to be bifurcation values. Instead, a brute force approach has been taken. Given an energy level $h_0$ that meets Albouy’s necessary condition, we consider $h_-< h_0 < h_+$ such that $h_0$ is the only candidate value in the interval $[h_-, h_+]$. Then calculate a topological invariant such as the homology groups at $h_-$ and $h_+$. If those topological invariants are different, then the integral manifolds underwent bifurcation at $h_0$.

While a change in any topological invariant is sufficient to detect bifurcation, computing the homology groups speaks to the next goal as well, by providing a description of sorts of the global structure of the manifolds. Moreover, methods such as Morse theory have a long tradition of deriving insights into global dynamics from homological information. While the non-compactness of $\mathfrak {M}(c,h)$ and $\mathfrak {M}_R(c,h)$ limits the opportunity to apply such techniques, [6, 8] allow some dynamical information to be obtained.

An important limiting factor in this work is the need to identify all of the planar central configurations. At present, the identification of complete set of central configurations has only been rigorously established for three arbitrary masses, or four, five, six or seven equal masses [2, 10]. The case of three arbitrary masses has been analyzed in [5], with a correction for the case of positive energy provided in [6]. The need for a correction arose from our incomplete understanding of the complexities generated by the behavior at the collinear configurations.

Those complexities motivated the development in [9] of a blow-up construction $\mathcal {B}$ of the configuration space. By adapting the methods of [5, 13] to this blow-up, the complexities at collinear were controlled, and formulae describing $H_*(\mathfrak {M}(c,h))$ and $H_*(\mathfrak {M}_R(c,h))$ were developed.

The obvious situation to apply the reduction formulae is that of the four-body problem with equal masses. The current work is the first step in doing so. Albouy’s algorithm for identifying singular values produces the set of energy levels shown in Table 1. The multiplicity refers to the number of $SO_2$-orbits of central configurations that differ by a permutation of the masses.

Table 1 Singular values of energy for four equal masses

These eight values, together with $h = 0$, define ten energy regions. These will be denoted

$$\begin{aligned}\begin{array}{ccccccccccccccc} \text {P} &{} = &{} (0, \infty ) &{} \text {I} &{} = &{} (h_1, 0) &{} \text {{II}} &{} = &{} (h_2, h_1) &{} \text {{III}} &{} = &{} (h_3, h_2) &{} \text {{IV}} &{} = &{} (h_4, h_3) \\ \text {V} &{} = &{} (h_5, h_4) &{} \text {{VI}} &{} = &{} (h_6, h_5) &{} \text {V{II}} &{} = &{} (h_7, h_6) &{} \text {V{III}} &{} = &{} (h_8, h_7) &{} \text {IX} &{} = &{} (-\infty , h_8) \\ \end{array}\end{aligned}$$

For four equal masses, it happens to be the case that all of the bifurcations at infinity occur first, then all of the finite bifurcations. This is not true in general, but in this instance, it allows us to divide the singular values of Table 1 fall naturally into three groups: $h_0 = 0$, the singular values at infinity $h_1, \ldots h_4$ and the singular values corresponding to relative equilibria $h_5, \ldots h_8$. The homology groups of $\mathfrak {M}(c,h)$ and $\mathfrak {M}_R(c,h)$ for $h >0$ were identified in [4]; those of Region I were identified in [9], which in turn established that $h_0 = 0$ is a bifurcation value. This work focuses on the evolution of the integral manifolds from Region I to Region V, passing through the four bifurcations at infinity. The analysis of the finite bifurcations, from Region V to Region IX, will be addressed in a separate companion work. In the present work, the results for Region I are used to bootstrap region by region through regions II–V. The results can be tabulated as follows.

Theorem 1.0.1

The spatial integral manifolds $\mathfrak {M}(c,h)$ for four equal masses with non-zero angular momentum, in the energy range $h > h_5$, have the following homology groups:

$$\begin{aligned} \begin{array}{|c||c|c|c|c|c|c|c|c|c|c|c|c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 &{} 7 &{} 8 &{} 9 &{} 10 &{} 11 &{} 12 \\ \hline P &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^6 &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ \hline I &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{11} &{} 0 &{} \mathbb {Z}^{6} \\ \hline {II} &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} \mathbb {Z}^{13} &{} \mathbb {Z}^{30} &{} \mathbb {Z}^{11} &{} 0 &{} \mathbb {Z}^{6} \\ \hline {III} &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} \mathbb {Z}^{7} &{} \mathbb {Z}^{18} &{} \mathbb {Z}^{5} &{} 0 &{} \mathbb {Z}^{6} \\ \hline {IV} &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} \mathbb {Z}^{20} &{} \mathbb {Z}^{14} &{} 0 &{} \mathbb {Z}^{6} \\ \hline V &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} \mathbb {Z} &{} \mathbb {Z}^{6} &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{6} \\ \hline \end{array}\end{aligned}$$

and the reduced integral manifolds $\mathfrak {M}_R(c,h)$ have homology groups

$$\begin{aligned}\begin{array}{|c||c|c|c|c|c|c|c|c|c|c|c|c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 &{} 7 &{} 8 &{} 9 &{} 10 &{} 11 &{} 12 \\ \hline P &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^7 &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ \hline I &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} \mathbb {Z}^{23} &{} 0 &{} \mathbb {Z}^{17} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline {II} &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} \mathbb {Z}^{13} &{} \mathbb {Z}^{17} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline {III} &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} \mathbb {Z}^{7} &{} \mathbb {Z}^{11} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline {IV} &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} 0 &{} \mathbb {Z}^{20} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline V &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} 0 &{} \mathbb {Z} &{} \mathbb {Z}^{5} &{} \mathbb {Z}^{6} &{} 0 \\ \hline \end{array}\end{aligned}$$

Corollary 1.0.1

For four equal masses and non-zero angular momentum, for $h > h_5 = -18 - 8 \sqrt{2}$, the bifurcation values of energy are $h_0$, $h_1$, $h_2$, $h_3$, $h_4$, with both the integral manifold $\mathfrak {M}(c,h)$ and reduced integral manifold $\mathfrak {M}_R(c,h)$ changing their homotopy type at those values, and at no others.

In particular, for four equal masses, the “bifurcations at infinity” detected by Albouy’s algorithm, are indeed bifurcation values. This relies only on the observation that, at each of the energy levels $h_i$, $i = 1, \ldots 4$, at least one homology group $H_k(\mathfrak {M}(c,h))$ and at least one homology group $H_k(\mathfrak {M}_R(c,h))$ change their values. We can go beyond that, and make use of the specific values in the table to draw conclusions about the integral manifolds.

Corollary 1.0.2

For all h in regions I–V, the following hold:

• The reduced integral manifold $\mathfrak {M}_R(c,h)$ does not admit a geodesic flow.

• The flow on the reduced integral manifold $\mathfrak {M}_R(c,h)$ does not admit a global cross section.

• The full integral manifold $\mathfrak {M}(c,h)$ is an orientable $S^1$-bundle over $\mathfrak {M}_R(c,h)$, but does not admit a product structure $\mathfrak {M}_R(c,h) \times S^1$.

Proof

These are negative conclusions, asserting that something does not happen. Each conclusion follows from the failure of a necessary homological condition.

In order for a $(2n-1)$-manifold $\mathcal {P}$ to admit a geodesic flow structure, it must first admit the topological structure as the unit tangent bundle of an n-manifold. In [8], it was shown that, if the $(2n-1)$-manifold $\mathcal {P}$ is non-compact and orientable, with torsion-free homology, then a necessary condition to admit such a topological structure is that $H_{n-1}(\mathcal {P}) \ne 0$. Applying this to the 13-dimensional non-compact orientable manifold $\mathfrak {M}_R(c,h)$, we see that its homology is torsion-free, with $H_6(\mathfrak {M}_R(c,h)) = 0$.

Similarly, it was shown in [6] that, for $\mathfrak {M}_R(c,h)$ to admit a global cross-section and has finitely-generated homology, then the Euler characteristic must satisfy $\chi (\mathfrak {M}_R(c,h)) = 0$. From the table, we see that in each region, $\chi (\mathfrak {M}_R(c,h)) \ne 0$.

Finally, if $\mathfrak {M}(c,h)$ admitted a product structure as $\mathfrak {M}_R(c,h) \times S^1$, then $H_*(\mathfrak {M}(c,h)) \cong H_*(\mathfrak {M}_R(c,h))\otimes H_*(S^1)$. This clearly fails to hold in any region. $\square$

Beyond those specific negative conclusions, the table displays patterns that are suggestive of additional structural issues. The most obvious of these is that the changes in the homology groups occur only in dimensions $7 \le k \le 11$ for the full integral manifolds, and dimensions $7 \le k \le 10$ for the reduced manifolds. There are no changes in dimensions $0 \le k \le 6$. This signals that the changes in the structure of the manifold have more to do with the changes in the structure of momentum fibers over the set of allowable positions, rather than being generated by changes in the set of allowable positions. The most striking of these changes was noted in [9]: for positive energy, the momentum fibers are hyperplanes; as h passes from positive to negative; these fold over to form spheres. This is reflected in the homology, with non-trivial homology appearing in dimensions 7–12.

The forthcoming companion work will show that there is a different pattern of changes in the homology groups for regions VI–IX. Those changes in the homology groups confirm that the set of allowable positions undergoes changes at the bifurcations at relative equilibria. This points up the different nature of the bifurcations at infinity, in comparison to the bifurcations at relative equilibria.

This work takes [1, 9] as its starting point. The framework established by those works is briefly summarized in Sect. 2. That framework reduces to the study of a real-valued function D on a space $\mathcal {B}$ associated with the 8-dimensional mass ellipsoid, with the homology groups of $\mathfrak {M}(c,h)$ and $\mathfrak {M}_R(c,h)$ computed from super-level sets of D on $\mathcal {B}$, together with various subspaces of those super-level sets. Almost all of the information we will need about that function and sets will associate with either the collision set $\Delta$ or the set of collinear configurations $\mathcal {C}$. Section 3 explores in detail those aspects, and prepares the way for the calculation of the homology groups by translating that analysis into topological terms. The homological calculations, which bootstrap off of those from [9, § 8], are in Sect. 4.

2 Singular Values and Level Sets of Energy

This section summarizes the results of [1, 9] that provide the framework for the current analysis. As noted, Albouy’s work in [1] identifies the singular values of energy on level set of angular momentum, center of mass and linear momentum. In the intervals between those singular values, [9] provides a reduction formula for computing the homology of the integral manifolds. Section 2.1 introduces the framework, while Sects. 2.3 and 2.2 review the core results from [1, 9] required for the present work.

2.1 Integral Manifolds

The approach to analyzing the integral manifolds follows the decomposition approach deployed in [5, 9, 13]. As that approach is described in detail in those works, we will only sketch it here. As we are examining the 4-body problem with equal masses, the masses are all set to $m_i = 1$. Except for the identification of central configurations (where the assumption of equal masses is critical), the assumption of equal masses does not play a role in the analysis.

Let $\vec {x}_1, \vec {x}_2, \vec {x}_3, \vec {x}_4 \in \mathbb {R}^3$ denote the positions of the four particles, and let $\vec {y}_i = \frac{d \vec {x}_i}{d t} \in \mathbb {R}^3$ be the corresponding velocities. There are four well-known constants of motion: center of mass; linear momentum; angular momentum and energy, as well as a rotational symmetry.

$$\begin{aligned} \sum _{i=1}^4 \vec {x}_i&= \vec {0} \quad \text{ Center } \text{ of } \text{ Mass } \end{aligned}$$

(2.1.1a)

$$\begin{aligned} \sum _{i=1}^4 \vec {y}_i&= \vec {0} \quad \text{ Linear } \text{ Momentum } \end{aligned}$$

(2.1.1b)

$$\begin{aligned} \sum _{i=1}^4 \vec {x}_i \times \vec {y}_i&= \vec {c} \quad \text{ Angular } \text{ Momentum } \end{aligned}$$

(2.1.1c)

$$\begin{aligned} \frac{1}{2} \sum _{i=1}^{4} |\vec {y}_i|^2 - U&= h \quad \text{ Energy } \end{aligned}$$

(2.1.1d)

where U is the self potential

$$\begin{aligned} U = \sum _{i<j} \frac{1}{ \parallel \vec {x}_{i} - \vec {x}_{j} \parallel }. \end{aligned}$$

(2.1.2)

The potential function is undefined at collisions (i.e. when $\vec {x}_i = \vec {x}_j$ for $i \ne j$), so the state space for the spatial four-body problem is $\mathbb {R}^{12}\setminus \Delta \times \mathbb {R}^{12}$, where

$$\begin{aligned}\Delta = \left\{ (\vec {x}_1, \vec {x}_2, \vec {x}_3, \vec {x}_4) \in \mathbb {R}^{12} | \vec {x}_i = \vec {x}_j \; \exists \; i \ne j \right\} \end{aligned}$$

is the collision set.

When $\vec {c} = \vec {0}$, there is an $SO_3$ symmetry. We will focus on the case of non-zero angular momentum, which has $\vec {c}$ as a preferred direction, and $SO_2$ symmetry under rotations around $\vec {c}$. There is no loss of generality in assuming that $\vec {c} = c\hat{k}$. The spatial integral manifold is defined formally as

$$\begin{aligned} \mathfrak {M}(c,h) = \left\{ (\vec {x},\vec {y}) \in \mathbb {R}^{3N}\setminus \Delta \times \mathbb {R}^{3N} | \; \text{ equations } \; 2.1.1 \; \text{ hold } \right\} \end{aligned}$$

When $\vec {c} = \vec {0}$, the reduced integral manifold is defined as $\mathfrak {M}_R(c,h) = \mathfrak {M}(c,h)/SO_ 3$, while for $\vec {c} \ne \vec {0}$, $\mathfrak {M}_R(c,h) = \mathfrak {M}(c,h)/SO_ 2$ For the spatial problem with non-zero angular momentum, there are ten integrals and the spatial integral manifolds are 14 dimensional spaces, while $\mathfrak {M}_R(c,h)$ is 13-dimensional.

With the angular momentum vector oriented along $\hat{k}$, the planar N-body problem can be embedded in the spatial problem by setting all $x_{i3} = y_{i3} = 0$. It is a simple calculation to see that this planar submanifold is invariant under the equations of motion, and the underlying plane $x_3 = 0$ is often referred to as the invariant plane. The planar integral manifold is the subset

$$\begin{aligned}\mathfrak {m}(c,h) = \left\{ (\vec {x},\vec {y}) \in \mathfrak {M}(c,h) | x_{i3} = y_{i3} = 0 \; \forall \; i = 1,\ldots ,N \right\} . \end{aligned}$$

This is invariant under the $SO_2$ action, so there is a well-defined reduced planar manifold $\mathfrak {m}_R(c,h)$. The planar 4-body manifold has dimension 9, while $\mathfrak {m}_R(c,h)$ is 8-dimensional.

While the integral manifolds present themselves as parameterized by c and h, for non-zero angular momentum, all manifolds with $\nu = h c^2$ constant are diffeomorphic. We will usually think of holding c fixed and and treating this as a one-parameter family parameterized by h. That is, integral manifolds $\mathfrak {M}(c,h)$ are level sets of H on the angular momentum manifold

$$\begin{aligned}\mathfrak {A}(\vec {c}) = \left\{ (\vec {x},\vec {y}) \in \mathbb {R}^{3N} \times \mathbb {R}^{3N} | \; \text{ equations } \; 2.1.1a - 2.1.1c \; \text{ hold } \right\} .\end{aligned}$$

The analysis of the integral manifolds proceeds through projection onto the configuration spaces. The spatial configuration space is

$$\begin{aligned}\mathcal {S} = \left\{ (\vec {x}_1,\ldots , \vec {x}_N) \in \mathbb {R}^{12}\setminus \Delta \; | \; \sum _{i} \vec {x}_i = \vec {0}, \sum _{i} \vec {x}_i^2 = 1 \right\} \end{aligned}$$

The spatial configuration space has various subspaces that will be of interest to us. The planar configuration space

$$\begin{aligned}\mathcal {P} = \left\{ (\vec {x}_1, \vec {x}_2, \vec {x}_3, \vec {x}_4) \in \mathbb {R}^{8}\setminus \Delta \; | \; \sum _{i} \vec {x}_i = \vec {0}, \sum _{i} \vec {x}_i^2 = 1 \right\} \end{aligned}$$

can also be defined by $\mathcal {P} = \left\{ (\vec {x}_1, \vec {x}_2, \vec {x}_3, \vec {x}_4) \in \mathcal {S} \; | \; x_{i3} = 0 \; \forall i\right\}$

The collinear configuration space

$$\begin{aligned}\mathcal {C} = \left\{ (\vec {x}_1, \vec {x}_2, \vec {x}_3, \vec {x}_4) \in \mathcal {S} \; | \; \exists \, x_0 \in S^2 \ni : x_{i} = \lambda _i x_0 \; \forall i \right\} \end{aligned}$$

consists of all configurations with all of the particles lying on a single line. Note that we do not assume collinear configurations to lie in the invarinant plane. The set of collinear configurations that lie in the invariant plane, $\mathcal {C}_0 = \mathcal {C} \cap \mathcal {P}$, will be of particular interest to us.

For these or any other $Z \subset \mathcal {S}$, we will denote $\Delta \cap Z$ by $\Delta _U$.

The spatial configuration space $\mathcal {S}$ is a dense open subset of the sphere $S^8$, while the planar configuration space is a dense open subset of a 5-sphere. These spaces clearly admit rotational symmetries, and have the obvious corresponding reduced quotient spaces $\mathcal {S}_R = \mathcal {S}/SO_2$ and $\mathcal {P}_R = \mathcal {P}/SO_2$.

To remain consistent with the notation in previous works, the sets $\mathcal {C}$ and $\mathcal {C}_0$ do not contain collisions. However, in the present work, we will want to consider sets that contain both collisions and non-collisional collinear configurations, and to be more explicit about the inclusion or exclusion of collisions. We will therefore make frequent use of the full set of collinear configurations (including collisions) along a single line L:

$$\begin{aligned}\mathcal {C}_L \cong \left\{ (x_1, x_2, x_3, x_4) \in \mathbb {R}^4 | \sum _{i} x_i = 0, \; \sum _{i} x_i^2 = 1\right\} .\end{aligned}$$

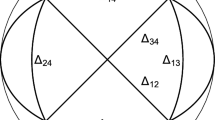
This set, which contains collisions, is clearly homeomorphic to $S^2$. The collision set $\Delta _L = \Delta \cap \mathcal {C}_L$ consists of six circles $\Delta _{ijL}$, one for each binary intersection $x_i = x_j$. These meet in three pairs of double binary points $\Delta _{ij,klL}$ and four pairs of triple collisions $\Delta _{ijk}$, as shown in Fig. 1. The union of the six circles forms a graph with 14 vertices and 36 edges, so $\chi (\Delta _L) = -22$. The complement $\mathcal {C}_L \setminus \Delta _L$ is homeomorphic to 24 open disks.

Fig. 1

The collision set in $\mathcal {C}_L$

The full set of collinear configurations $\mathcal {C} \cup \Delta _{\mathcal {C}}$, including collisions, fibers over $\mathbb {R}P^2$ with fiber $\mathcal {C}_L$, and similarly, $\mathcal {C}_0 \cup \Delta _{\mathcal {C}_0}$ fibers over $\mathbb {R}P^1$ with fiber $\mathcal {C}_L$.

Let $\Theta :\mathfrak {M}(c,h)) \rightarrow \mathcal {S}$ be the projection $(\vec {x}, \vec {y}) \mapsto \frac{1}{\left| |\vec {x}\right| |} \vec {x}$. The projection is equivariant with respect to the $SO_2$ symmetries, so there is a well-defined projection $\theta :\mathfrak {H}_R \rightarrow \mathcal {S}_R$. The configuration spaces are defined as the images of $\Theta$ in $\mathcal {S}$:

$$\begin{aligned}\begin{array}{llll} \text{ Spatial } \text{ Configurations } &{} \mathfrak {K}(c,h) &{} = &{} \left\{ \vec {x} \in \mathcal {S} | \; (\lambda \vec {x}, \vec {y}) \in \mathfrak {M}(c,h) \; \exists \; \lambda> 0, \vec {y} \in \mathbb {R}^{12} \right\} \\ \text{ Planar } \text{ Configurations } &{} &{} &{} \left\{ \vec {x} \in \mathcal {P} | \; (\lambda \vec {x}, \vec {y}) \in \mathfrak {m}(c,h) \; \exists \; \lambda > 0, \vec {y} \in \mathbb {R}^8 \right\} \\ \text{ Collinear } \text{ Configurations } &{} &{} &{} \mathfrak {K} \cap \mathcal {C}. \end{array}\end{aligned}$$

There is a commutative diagram

and a corresponding planar diagram.

At this point, we introduce a shift in notation, to reflect a shift in perspective. The definitions of the integral manifolds and related spaces were formulated in terms of the positions $\vec {x}_i = (x_{i1}, x_{i2}, x_{i3})$ and velocities $\vec {y}_i = (y_{i1}, y_{i2}, y_{i3})$ of the individual particles. Moving forward, we will focus instead on the component vectors $q_j = (x_{1j}, x_{2j}, x_{3j}, x_{4j})$ consisting of the projections of $\vec {x}$ onto the $x-$, $y-$ and $z-$axis. The notation for configurations in $\mathcal {S}$ will be

$$\begin{aligned} \mathcal {S} = \{ (q_1, q_2, q_3) \in \mathbb {R}^{12} | (1,1,1,1)\cdot q_i = 0, q_1^2 + q_2^2 + q_3^3 = 0 \}\end{aligned}$$

with $\mathcal {P}$ consisting of configurations with $q_3 = 0$, and $\mathcal {C}_L$ viewed as configurations with $q_2 = q_3 = 0$. Note that, in this notation, rotations in $SO_3$ intertwine $q_1$, $q_2$ and $q_3$. Similarly, the angular momentum constraint now takes the form $J(q) p = \vec {c}$, where

$$\begin{aligned}J(q) = \left[ \begin{array}{ccc} 0 &{} -q_3^T &{} q_2^T \\ q_3^T &{} 0 &{} -q_1^T \\ -q_2^t &{} q_1^T &{} 0 \end{array} \right] \end{aligned}$$

2.2 The Reduction Framework

As noted, [9] describes a process for reducing the calculation of $H_*(\mathfrak {M})$ to calculations on the mass ellipsoid $\mathcal {S}$. Or, nearly so. The reduction process encounters irregularities at the collinear configurations. First, it is a simple observation that the only collinear configurations that satisfy the angular momentum constraint are those in the invariant plane: $\mathfrak {K} \cap \mathcal {C} \subset \mathcal {C}_0$. Second, at collinear points in the invariant plane, the matrix J(q) that describes the angular momentum constraint becomes degenerate and the dimension of the fiber $\Theta ^{-1}(q)$ increases from $3N-6$ to $3N-5$. Finally, we will see that as a key function that carries informatin about the angular momentum contraint is discontinuous at $\mathcal {C}_0$. These are resolved by deleting $\mathcal {C}\setminus \mathcal {C}_0$ from the configuration space, then introducing a blow-up construction at $\mathcal {C}_0$ that resolves the discontinuities.

The projection $\Theta :\mathfrak {M} \rightarrow \mathcal {S}$ naturally invites a description of $\mathfrak {M}(c,h)$ in terms of the image $\mathfrak {K}(c,h)$ and the pre-images $\Theta ^{-1}(q)$. Examination of $\Theta$ shows that this description can be encoded via the potential function U(q) and a function $Y:\mathcal {S} \rightarrow \mathbb {R}^+$ that measures the square of the distance from the origin to the affine space $J(q)p = c \hat{k}$. For negative energy, we find that $q \in \mathfrak {K}(c,h)$ if and only if $U^2(q) + 2h Y(q) \ge 0$. We therefore define

$$\begin{aligned} D(q) = \frac{U^2(q)}{Y(q)}, \end{aligned}$$

(2.2.1)

so that $\mathfrak {K}(c,h) = \{ q \in \mathcal {S} | D(q) \ge - 2 h\}$. The pre-image $\Theta ^{-1}(q)$ consists of a single point for when $D(q) = -2h$ and is a sphere when $D(q) > -2h$.

While the properties of the potential function have been extensively studied, the function Y does not occupy the same central role, so there has been less occasion to record its properties. For fixed angular momentum $c \hat{k}$ and position vector q, Y(q) is defined by $Y(q) = \min \{ p^2 | J(q) p = c \hat{k} \}$. There are a variety of ways to express this:

• If $q = (q_1, q_2, q_3)$ is non-collinear, then the moment of inertia tensor

  $$\begin{aligned}I(q) = \left[ \begin{array}{ccc} q_2^2 + q_3^2 &{} - q_1 \cdot q_2 &{} - q_1 \cdot q_3 \\ - q_1 \cdot q_2 &{} q_1^2 + q_3^2 &{} - q_2 \cdot q_3 \\ - q_1 \cdot q_3 &{} - q_2 \cdot q_3 &{} q_1^2 + q_2^2 \end{array} \right] \end{aligned}$$

  is invertible, and $Y(q) = \hat{k} I^{-1}(q) \hat{k}$ is the $3-3$ entry of $I^{-1}(q)$.

• If q has $q_1 \cdot q_2 = q_1 \cdot q_3 = q_2 \cdot q_3 = 0$ and $q_1^2 \ge q_2^2 \ge q_3^2$, we refer to q as a standard configuration. For standard configurations,

  $$\begin{aligned}I(r)= \left[ \begin{array}{ccc} q_2^2 + q_3^2 &{} 0 &{} 0 \\ 0 &{} q_1^2 + q_3^2 &{} 0 \\ 0 &{} 0 &{} q_1^2 + q_2^2 \end{array} \right] \end{aligned}$$

  and $Y(q) = \frac{c^2}{q_1^2 + q_2^2}$. In particular, if q lies in the invariant plane (i.e. $q_3 = 0$), then $Y(q) = \frac{c^2}{q ^2 }$ and $D(q) =\frac{q^2 U^2(q) }{c^2}$.

• An arbitrary position vector q is a rotation of a standard configuration: there is a standard configuration $r = (r_1, r_2, r_3)$ and an $R \in SO_3$ acts component-wise on each $(r_{1i}, r_{2i}, r_{3i})$ so that $q = R r$. Then $I(q) = R I(r) R^T$, so $I^{-1}(q) = R I^{-1}(r) R^T$ and $Y(q) = \hat{k}^T R I^{-1}(r) R^T \hat{k}$.

• I(q) is positive semi-definite with non-negative eigenvalues $\alpha _1(q) \le \alpha _2(q) \le \alpha _3(q)$ that are invariant under rotation. At a standard configuration, $\alpha _1(q) = r_2^2 + r_3^2$, $\alpha _2(q) = r_1^2 + r_3^2$ and $\alpha _3(q) = r_1^2 + r_2^2$. If $v = (v_1, v_2, v_3) \in S^3$ has $R v = \hat{k}$ (or alternatively, $R^T \hat{k} = v$), then

  $$\begin{aligned}Y(q) = \frac{c^2 v_1}{\alpha _1(q)} + \frac{c^2 v_2}{\alpha _2(q)} + \frac{c^2 v_3}{\alpha _3(q)}\end{aligned}$$

  This formulation of Y displays the dependence on the shape of the configuration through the eigenvalues $\alpha _1(q)$, $\alpha _2(q)$, $\alpha _3(q)$ and the dependence on the orientation through the components of the vector $v_1$, $v_2$, $v_3$.

While the characterization of the integral manifolds in terms of the super-level sets of a single function on a sphere has a certain elegance to it, there are some complexities within this formulation. Implicit in the definition of D(q) is that both U(q) and Y(q) must be defined at q. The potential function is undefined at collisions, and the function Y is undefined for collinear configurations that do not lie in the invariant plane. The domain of definition is therefore not the full mass ellipsoid $\mathcal {S}$, but rather the dense subset

$$\begin{aligned}\mathcal {S}_0 = \mathcal {S} \setminus \left( \Delta \cup (\mathcal {C}\setminus \mathcal {C}_0)\right) \end{aligned}$$

An added complexity is that Y, and hence D, is discontinuous at $\mathcal {C}_0$. Related to that discontinuity, for points q with $D(q) > -2h$, the pre-image $\Theta ^{-1}(q)$ in $\mathfrak {M}(c,h)$ is the sphere $S^{6}$ for non-collinear configurations, while for collinear configurations, the pre-image is $S^{7}$.

Those complexities are addressed by introducing a blow-up construction. The space $\mathcal {B}$ is formed from $\mathcal {S}_0$ by replacing each collinear configuration in the invariant plane $q \in \mathcal {C}_0$ with a set of the form $S^{4}\setminus S^0$. As $\mathcal {S}$ has dimension 8 and $\mathcal {C}_0$ has dimension 3, the 4-sphere can be viewed as the sphere of directions normal to $\mathcal {C}_0$ in $\mathcal {S}$. The deleted antipodal points correspond to the direction of approach to q from $\mathcal {C}\setminus \mathcal {C}_0$. Removing those directions of approach reflects the fact that collinear configurations outside of the invariant plane are excluded. The punctured sphere attached at $q \in \mathcal {C}_0$ is denoted $\mathcal {B}_0(q)$. The union of those sets is $\mathcal {B}_0$ and the space resulting from attaching $\mathcal {B}_0$ to $\mathcal {S}_0 \setminus C_0$ is $\mathcal {B}$.

While somewhat awkward, this blow-up set proves to be precisely what is needed to define Y continuously. The intuition is that, if the deleted antipodal points in the 4-sphere attached at $q_0 \in \mathcal {C}_0$ are viewed as the poles and the corresponding $S^{3}$ as the equator, then it is the latitude that measures the proximity to the “forbidden” collinear configurations that are not orthogonal to the angular momentum vector. This captures the different limiting values of Y(q) as $q \rightarrow q_0$. This allows Y to be extended continuously to $\mathcal {B}$. We can clearly extend U to $\mathcal {B}$ by assigning value U(q) at every point in $\mathcal {B}_0(q)$, and so extend D continuously to $\mathcal {B}$.

Moreover, when the blow-up $\varrho :\mathcal {B} \rightarrow \mathcal {S}$ is lifted to produce $\mathfrak {N}(c,h) \rightarrow \mathfrak {M}(c,h)$, the discontinuity in the dimension of the fiber is eliminated. The projection $\Theta :\mathfrak {N}(c,h) \rightarrow \mathcal {B}$ then has the properties that $\Theta (\mathfrak {N}(c,h)) = \{ q \in \mathcal {B} | D(q) \ge -2h\}$, with $\Theta ^{-1}(q) \cong S^{3N-6}$ for all q with $D(q) > -2h$ and $\Theta ^{-1}(q)$ collapsing to a single point when $D(q) > -2h$.

The impact on homology groups of the pull-back from $\mathfrak {M}(c,h)$ to $\mathfrak {N}(c,h)$ and projection onto $\mathcal {B}$ can be traced. The pull-back requires us to distinguish behavior at the collinear blow-up set, while the projection distinguishes behavior on $\{ D(q) = -2h\}$ vs. $\{ D(q) > -2h\}$. In the end, the following subsets of $\mathcal {B}$ are found to play a role in computing the homology groups of $\mathfrak {M}(c,h)$ and $\mathfrak {M}_R(c,h)$:

$$\begin{aligned}\begin{array}{lll} \mathfrak {B}(d) &{} = &{} \{ q \in \mathcal {B} | D(q) \ge d \} \\ \partial \mathfrak {B}(d) &{} = &{} \{ q \in \mathcal {B} | D(q) = d \} \\ \mathfrak {B}_0(d) &{} = &{} \{ q \in \mathcal {B}_0 | D(q) \ge d \} \\ \partial \mathfrak {B}^+(d) &{} = &{} \partial \mathfrak {B}(d) \cup \mathfrak {B}_0(d) \\ \end{array}\end{aligned}$$

These sets will emerge as our primary objects of study. For $N = 4$, the homology groups of $H_*(\mathfrak {M})$ are given by

$$\begin{aligned}H_{k}\left( \mathfrak {M} \right) \cong {\left\{ \begin{array}{ll} \text {im}\left( j_*:H_3(\mathfrak {B}) \rightarrow H_3(\mathfrak {B}, \mathfrak {B}_0)\right) &{} k = 3 \\ H_{k}\left( \mathfrak {B}, \mathfrak {B}_0 \right) \oplus H_{k-6}\left( \mathfrak {B}, \partial \mathfrak {B}^+ \right) &{} k= 4, 5, 6, 10, 11, 12\\ H_{k}\left( \mathfrak {B} \right) \oplus H_{k-6}\left( \mathfrak {B}, \partial \mathfrak {B} \right) &{} k = 0, 1, 2, 7, 8 , 9, k > 12 \\ \end{array}\right. }\end{aligned}$$

All of these constructions are invariant under the $SO_2$ rotation around the z-axis (i.e. around $\vec {c}$). The homology groups of $H_*(\mathfrak {M}_R)$ are given by

$$\begin{aligned}H_{k}\left( \mathfrak {M}_R \right) \cong {\left\{ \begin{array}{ll} H_{k}\left( \mathfrak {B}_R, \mathfrak {B}_{R0} \right) \oplus H_{k-6}\left( \mathfrak {B}_R, \partial \mathfrak {B}_R^+ \right) &{} k = 3, 4, 5, 10, 11 \\ H_{k}\left( \mathfrak {B}_R \right) \oplus H_{k-6}\left( \mathfrak {B}_R, \partial \mathfrak {B}_R \right) &{} \text {otherwise} \end{array}\right. }\end{aligned}$$

2.3 Singular Values of Energy on the Angular Momentum Manifold

Albouy [1] provides necessary conditions for an energy level h to be a bifurcation value of $H\mid _{\mathfrak {A}}$. As a smooth function on a non-compact manifold, bifurcation values of the level sets of H on $\mathfrak {A}$ can occur only at singular values of $H\mid _{\mathfrak {A}}$, which can arise in one of two ways. The most straightforward is $h = H(q,p)$ for a critical point (q, p). Critical point occur when $\nabla H(q,p) = \lambda _x \nabla C_x(q,p) + \lambda _y \nabla C_y(q,p) + \lambda _z \nabla C_z(q,p)$ for some $\Lambda = (\lambda _x, \lambda _y, \lambda _z)$, and are well-known to correspond to relative equilibria: planar central configurations uniformly rotating around the center of mass. If $\mathfrak {A}$ were compact, those would be the only singular values. However, $\mathfrak {A}$ has two sources of non-compactness: the collision set has been deleted, and neither positions nor momenta are bounded. Albouy demonstrated that the former does not generate singular values of H, but the latter can. Namely, singular values also occur when there exists a sequence $\left\{ (q_n, p_n) \right\} \subset \mathfrak {A}$ and sequence $\Lambda _n = (\lambda _{nx}, \lambda _{ny}, \lambda _{nz})$ such that $\nabla H(q_n, p_n) - \lambda _{nx} \nabla C_x(q_n, p_n) - \lambda _{ny} \nabla C_y(q_n, p_n) - \lambda _{nz} \nabla C_z(q_n, p_n)$ tends to zero and $H(q_n, p_n)$ tends to a finite limit $h_s$. Albouy refers to such a sequence as a horizontal critical sequence and produces necessary and sufficient conditions for a value $h_s$ to be associated with a horizontal critical sequence. In these sequences, the positions diverge to infinity, so the resulting bifurcation values are referred to as bifurcations at infinity.

Albouy demonstrated that, for every energy level $h_s$ associated with a bifurcation at infinity, a particularly elegant and illuminating set of model horizontal critical sequences exist. In these model sequences, the particles are partitioned into clusters. Particles in a cluster are positioned at a common height along the z-axis, with $x-y$ positions forming a planar central configuration. Each non-trivival cluster is given $x-y$ momentum to form a relative equilibrium, while singletons are given zero $x-y$ momentum, and all particles are given zero z-momentum. The $x-y$ momenta of the non-trivial clusters can be adjusted so that all rotate at the same speed, and so that the sum of their angular momentum equals the prescribed value c. Finally, the z-positions are allowed to diverge, so that the spacing between each pair of clusters diverges to infinity. In the limit, as the clusters diverge from each other, each cluster experiences only its own potential, which allows each cluster to satisfy

$$\begin{aligned}\nabla H(q_{\sigma n}, p_{\sigma n}) - \lambda _{\sigma nx} \nabla C_x(q_{\sigma n}, p_{\sigma n}) - \lambda _{\sigma ny} \nabla C_y(q_{\sigma n}, p_{\sigma n}) - \lambda _{\sigma nz} \nabla C_z(q_{\sigma n}, p_{\sigma n}) \rightarrow 0 \end{aligned}$$

for some choice $(\lambda _{\sigma nx}, \lambda _{\sigma ny}, \lambda _{\sigma nz})$. Setting $p_{nz} = 0$ for all particles allows $\lambda _{\sigma nx} = \lambda _{\sigma ny} = 0$, while the synchronization of the momenta allows a common value of $\lambda _{\sigma nz}$ to be chosen for all clusters.

Not only do these model sequences illuminate the behavior of the bifurcations of the energy function on the angular momentum manifold, they also provide a useful heuristic for understanding the subject at hand, namely, the corresponding bifurcations of the function D on the configuration space. Without loss, a model sequence has the form $\{(q_{10}, q_{20}, n q_{30}, -\lambda _z q_{20}, \lambda _z q_{10}, 0)\}$ so its the projection onto the configuration space has the form $\frac{1}{\sqrt{q_{10}^2 + q_{20}^2 + n^2 q_{30}^2}}(q_{10}, q_{20}, n q_{30})$. As $n \rightarrow \infty$, this limits to $(0, 0, \frac{q_{30}}{|q_{30}|})$. In particular, all of the particles in a sub-cluster limit to the same point $(0, 0, \frac{q_{3\sigma }}{|q_3|})$. That is, the projections of the model sequences limit to collinear configurations oriented along the z-axis, with all particles in a sub-cluster limiting to mutual collision. We might describe as “vertical collisions”. We will see that it is precisely the set of vertical collisions where the topological changes associated with these singular values occur.

There is another heuristic that reinforces this focus. Non-compactness of the configuration space occurs as a result of the deletion of the collision set $\Delta$ (where U is undefined) and the deletion of collinear configurations $\mathcal {C}\setminus \mathcal {C}_0$ outside of the invariant plane (where Y is undefined). In order for a sequence $q_n$ to produce a finite non-zero singular value for $D= \frac{U^2}{Y}$, both $U^2$ and Y must diverge to infinity. That is, the sequence must limit to a point in $\Delta \cap (\mathcal {C} \setminus \mathcal {C}_0)$. We will see below that Y is monotone decreasing as configurations are rotated towards the z-axis, so it is at the vertical collisions that bifurcations occur.

Returning to Albouy’s analysis, the result is that, for non-zero angular momentum c, the singular values of $H\mid _{\mathfrak {A}}$ can be identified by the following algorithm:

• 1. Identify all planar central configurations of the N-body problem with masses $m_1, \ldots m_N$.

• 2. For each N-body central configuration q, normalized so that $q^2 = 1$, the corresponding singular value is $H = -\frac{1}{2}p^2 = -\frac{U^2(q)}{2c^2}$.

• 3. The set of finite singular values of H is the set of values $-\frac{U^2(q)}{2c^2}$ as q varies over all planar central configurations with $q^2 = 1$.

• 4. Identify all planar central configurations for all subsets of the masses $m_{i_1}, \ldots , m_{i_K}$.

• 5. Take all possible non-trivial partitions of the index set $\left\{ 1, \ldots , N\right\}$.

• 6. For any non-trivial partition $\sigma _1, \ldots \sigma _l$, associate with each non-trivial cluster $\sigma _j = \left\{ i_1, \ldots i_k \right\}$ in the partition a planar central configuration $q_{\sigma _j}$ of the k-body problem with masses $m_{i_1}, \ldots , m_{i_k}$. Normalize each $q_{\sigma _j} = 1$, and let $U_{\sigma _j}(q_{\sigma _j})$ denote the potential energy of the configuration within the k-body problem.

• 7. If $\sigma _1, \ldots \sigma _l$ are the non-trivial clusters of a partition, and $q_{\sigma _1}, \ldots q_{\sigma _l}$ are the corresponding normalized planar central configurations, then the resulting singular value is $H = \frac{-1}{2c^2}\left( \sum _j U_{\sigma _j}^{\frac{2}{3}}(q_{\sigma _j}) \right) ^3$.

• 8. The set of singular values at infinity of H is the set of values $\frac{-1}{2c^2}\left( \sum _j U_{\sigma _j}^{\frac{2}{3}}(q_{\sigma _j}) \right) ^3$, ranging over all possible non-trivial partitions of the masses $m_1, \ldots , m_N$ and all possible planar central configurations associated with the non-trivial clusters of those partitions.

Applying the algorithm to four equal masses, there are four planar central configurations: the square, isosceles, equilateral and collinear configurations. For sub-clusters with three equal masses, there are two central configurations: the equilateral and collinear configurations. For sub-clusters with two equal masses, there is only the collinear configuration. This produces the following cases:

• Six distinct partitions into a two-body cluster and two trivial clusters. All occur at the same energy level $h_1$.

• Three distinct partitions into two two-body clusters, with one collinear configuration for each partition. All occur at the same energy level $h_2$. This is the only instance in which two non-trivial clusters occur.

• Eight distinct partitions into a three-body cluster and a trivial cluster, with the three masses forming a Lagrange configuration. All occur at the same energy level $h_3$.

• Twelve distinct partitions into a three-body cluster and a trivial cluster, with the three-body cluster forming an Euler configuration. All occur at the same energy level $h_4$.

• Six square configurations, corresponding the six cyclical orderings of the masses, which are all distinct relative to the preferred direction of the angular momentum vector, but all occurring at the same energy level $h_5$.

• Twenty-four isosceles configurations, corresponding to distinct ordering of the masses. All occur at the same energy level $h_6$.

• Eight equilateral triangle configurations, corresponding to the four choices for the center mass and two cyclical orderings of the remaining three masses. All occur at the same energy level $h_7$.

• Twelve distinct collinear central configurations (corresponding to the 24 possible orderings of the masses, modulo the rotational symmetry that identifies pairs whose orderings are reversed). All occur at the same energy level $h_8$.

Note that, except for the case of $h_2$, there is only one non-trivial cluster, with one central configuration $q_i$. With $q_i$ normalized to $q_i^2 = 1$, then the corresponding singular energy level is $h_i = -\frac{1}{2 c^2} U_0^2(q_i)$. At $h_2$, where we have two binary clusters, $h_2 = -\frac{1}{2 c^2}(2U^{\frac{2}{3}}_0(q_i))^3 = -\frac{4}{ c^2}U^{2}_0(q_i)$. This produces the results displayed in Table 1. Properly speaking, for given non-zero angular momentum c, it is the quotient of the values in the table by $c^2$ that are the singular values. Also, while it is clearly redundant to list both $h_i$ and $\delta _i = -2 h_i$, we list both for the convenience of the reader: on the one hand, it is the values of $h_i$ that are shown to be the bifurcation values of the integral manifolds; on the other hand, Sect. 3 will show that all of the calculational effort will be organized around a function D on the collision manifold whose singular values are $-2 h_i$. Finally, note energy level for the isosceles configurations is incorrectly stated in [7, 9]. This impacts the ordering of the bifurcation levels, reversing the order of the isosceles and equilateral bifurcation levels.

3 Topology of the Level Sets

With the problem reduced to studying the level sets and super-level sets of D, we now turn to a closer study of the function, with the goal of translating analysis of it into the topological information needed to compute the homology groups of interest. The first step will be to demonstrate that for d sufficiently small, all of the changes to the topology lie within an identifiable neighborhood of the collinear configurations. The approach established in [9] identifies a neighborhood $\mathcal {T}(\mathcal {C}_L)$ and a useful parameterization of it. In this section, we briefly review that framework, establish the key properties of the function D, and show that, for the relevant parameter range, essentially all of the necessary information is captured in the limiting behavior of D approaching collinear configurations oriented along the z-axis.

Section 3.1 establishes a parameterization of a neighborhood of the collinear configurations, and Sect. 3.2 reviews the dependence of D on those parameters in $\mathcal {T}(\mathcal {C}_L)$. One new aspect, explored in Sect. 3.4, will be a closer examination of the limiting behavior of D as $t \rightarrow 0$ along the set of vertical configurations $\mathcal {T}^1(\mathcal {C}_L)$. The main result, Proposition 3.5.1 is established in Sect. 3.5. The proposition shows that the key information needed will be the limiting behavior of D approaching , which in turn will depend significantly on the structure of the collision set. That structure is summarized in Sect. 3.3.

3.1 Coordinates Near Collinear

We will see that, for the energy regime we are studying, all of the interesting behavior occurs in a neighborhood of the collinear configurations. We will also see that the most consequential behavior occurs near collinear configurations in the invariant plane, and near collinear configurations oriented along the z-axis (resp. collinear configurations perpendicular to, or parallel to, the angular momentum vector $\vec {c}$). The rotational symmetry around the z-axis allows us to focus the former on collinear configurations that lie along the x-axis.

To develop the needed neighborhoods, for each $(q_1, 0, 0) \in \mathcal {C}_L$, we first define

$$\begin{aligned}\mathcal {T}^0(q_0, \tau ) = \varrho ^{-1}\left( \left\{ (\sqrt{1 - t^2} q_1, t q_2, t q_3) \mid q_2 \cdot q_0 = q_3 \cdot q_0 = 0, q_2^2 + q_3^2 = 1, t \le \tau \right\} \right) \end{aligned}$$

Note that in $\mathcal {B}$, each distinct $(0, q_2, q_3)$ limits to a distinct $\lim _{t \rightarrow 0}(\sqrt{1 - t^2} q_1, t q_2, t q_3)$. These form a 3-sphere that can be viewed as the equator of $\mathfrak {B}_0(q_1)$. To emphasize the dependence on t, we will denote a point $(\sqrt{1 - t^2} q_0, t q_2, t q_3)$ as q(t). If $R(\phi )$ is the rotation by $\phi$ around the y-axis, define

$$\begin{aligned}\mathcal {T}(q_0, \tau ) = \left\{ R(\phi ) q(t) \mid -\frac{\pi }{2}< \phi < \frac{\pi }{2}, q(t) \in \mathcal {T}^0(q_0, \tau ) \right\} .\end{aligned}$$

and

$$\begin{aligned}\mathcal {T}^1(q_0, \tau ) = \left\{ R\left( \frac{\pi }{2}\right) q(t) \mid q(t) \in \mathcal {T}^0(q_0, \tau ) \right\} \end{aligned}$$

The final step is to rotate the set $\mathcal {T}(q_0, \tau )$ around the z-axis. We will not have as much need to explicitly display these rotations, and so do not introduce notation to record them.

We will find it advantageous to focus on the range $0 \le \tau \le \frac{1}{2}$. We therefore define $\mathcal {T}(q_0) = \bigcup _{\tau = 0}^{\frac{1}{2}} \mathcal {T}(q_0, \tau )$. As the limiting behavior as $t \rightarrow 0$ will be of interest to us for all $\phi$ (especially $\phi = \frac{\pi }{2}$), we single out the limit sets

$$\begin{aligned}\mathcal {X}^{0}(q_0) = \mathcal {T}^0(q_0, 0), \; \mathcal {X}^1(q_0) = \mathcal {T}^1(q_0, 0), \; \mathcal {X}(q_0) = \mathcal {T}(q_0, 0).\end{aligned}$$

There is an obvious projection $\xi :\mathcal {T}(q) \rightarrow \mathcal {X}(q)$.

The set $\mathcal {T}(\mathcal {C}_L)$ represents a blow-up construction, similar to, but distinct from, the blow-up construction of $\mathcal {B}$ described in Sect. 2. The two constructions differ in structure, and are deployed to serve different purposes. The blow-up construction $\mathfrak {B}$ is focused solely on collinear configurations in the invariant plane $\mathcal {C}_0$, as those are the only collinear points that actually occur in the integral manifolds. The blow-up at those points resolves the discontinuity in D at those collinear points. On the other hand, the blow-up $\mathcal {X}$ is, in part, an extension of the coordinate system of $\mathcal {T}(\mathcal {C}_L)$ near collinear, and in part, a convenient tool for describing the changes in topology of the level sets $\partial \mathfrak {B}(d)$.

Each is structured to support its role. Away from collinear, the two differ only in that $\mathcal {T}(\Delta _L)$ is defined and includes collision points, while $\mathcal {B}$ has been constructed with all collisions deleted. At collinear, we can compare $\mathcal {X}(\mathcal {C}_L)$ to $\mathcal {B}_0$. Here too, the former contains collisions which the latter does not. Further, only $\mathcal {X}^0(\mathcal {C}_L\setminus \Delta _L)$ is a subset of $\mathcal {B}$. The rest of $\mathcal {X}(\mathcal {C}_L)$ is not part of the construction of $\mathcal {B}$. On the other hand, at $q_0 \in \mathcal {C}_L\setminus \Delta _L$, we noted above that the set $\mathcal {X}^0(q_0) \cong S^3$ sits as the equator in $\mathcal {B}_0(q_0) \cong S^4 \setminus S^0$. All of these differences illustrate that $\mathcal {T}(\mathcal {C})$ should be considered to be a distinct blow-up construction from $\mathcal {B}$. Care will need to be taken when translating into $\mathcal {B}_0$ the results at $\mathcal {X}^0(\mathcal {C}_L)$ derived from analysis on $\mathcal {T}(\mathcal {C}_L)$. Fortunately, those results are straightforward. We will therefore not seek to integrate $\mathcal {T}$ and $\mathcal {B}$ into a single blow-up construction.

In this work, the focus will be on the behavior of D on $\mathcal {T}(\mathcal {C}_L)$, with the rotational symmetry around the z-axis used to extend the results. To support this approach, we first clarify the extent to which this parameterization provides well-defined co-ordinates. Sufficient for our purposes, we note:

Lemma 3.1.1

Viewed as a map of $S^2 \times S^3 \times (0,1] \times [-\frac{\pi }{2}, \frac{\pi }{2}] \rightarrow \mathcal {S}\setminus \mathcal {C}$, the parameterization of $\mathcal {T}(\mathcal {C}_L)$ is one-to-one on $S^2 \times S^3 \times (0,\frac{1}{2}] \times [-\frac{\pi }{2}, \frac{\pi }{2}]$, except that the rotations by $\phi = \frac{\pi }{2}$ and $\phi = -\frac{\pi }{2}$ fully overlap. When rotations about the z-axis are applied, $S^1 \times \mathcal {T}(\mathcal {C}_L, \sqrt{\frac{2}{3}})$ maps onto $\mathcal {S} \setminus \mathcal {C}$.

Proof

Injectivity on $\mathcal {T}(\mathcal {C}_L)$ was demonstrated in [9, Lemma 8.1]. To establish surjectivity, note that for any configuration q, there is a rotation R so that the component vectors $r_i = (Rq)_i$ are orthogonal. Without loss of generality, the rotation can be chosen so that $r_1^2 \ge r_2^2, r_3^2$, hence $\tau ^2 = r_1^2 \ge \frac{1}{3}$. Setting $q_1 = \frac{1}{\tau }r_1$ and $(q_2, q_3) = \frac{1}{\sqrt{1-\tau ^2}}(r_1, r_2)$, we have $q_1\cdot q_2 = q_1\cdot q_3 = 0$ and $r = (\tau q_1, \sqrt{1 - \tau ^2} q_2, \sqrt{1 - \tau ^2} q_3)$ $\square$

3.2 Local Behavior on $\mathcal {T}(\mathcal {C}_L)$

The next step is to describe the behavior of Y and D in the neighborhood $\mathcal {T}(\mathcal {C}_L)$. This will allow us to first establish that, for the parameter range $0< d < \frac{27}{2c^2}$ , all of $\mathcal {B} \setminus \mathcal {T}(\mathcal {C}_L) \subset D^{-1}((d, \infty )) = \text {int}( \mathfrak {B}(d))$, and second, describe $\partial \mathfrak {B}(d) = D^{-1}(d)$ with sufficient precision.

Lemma 3.2.1

On $\mathcal {T}(\mathcal {C}_L)$, Y(q) depends only on t, $\phi$, $\rho ^2 = q_2^2$ and $\theta$, defined by $q_2 \cdot q_3^2 = \rho ^2 (1 - \rho ^2) \cos ^2(\theta )$. Namely,

$$\begin{aligned}Y(\rho , \theta , t, \phi ) = \frac{c^2 \sin ^2(\phi )}{t^2} + \frac{c^2 \cos ^2(\phi )(1-t^2 \rho ^2)}{1 - t^2 + t^4 \rho ^2 (1-\rho ^2) \sin ^2(\theta )}.\end{aligned}$$

Proof

For $q \in \mathcal {T}^0(\mathcal {C}_L)$, we can appeal the identification of Y(q) as the $3-3$ entry of the inverse of the moment of inertia matrix. For such q, we have $q_1 \cdot q_2 = q_1 \cdot q_3 = 0$, $q_1^2 = 1 - t^2$, $q_2^2 = t^2 \rho ^2$, $q_3^2 = t^2 (1-\rho ^2)$ and $q_2 \cdot q_3 = t^2 \rho \sqrt{1- \rho ^2} \cos (\theta )$, so

$$\begin{aligned}MI(q) = \left[ \begin{array}{ccc} t^2 &{} 0 &{} 0 \\ 0 &{} 1-t^2 + t^2(1-\rho ^2) &{} - t^2 \rho \sqrt{1- \rho ^2} \cos (\theta ) \\ 0 &{} -t^2 \rho \sqrt{1- \rho ^2} \cos (\theta ) &{} 1-t^2 + t^2 \rho ^2 \end{array} \right] \end{aligned}$$

A straightforward computation of the inverse yields the formula for $Y(\rho , \theta , t, 0)$. Similarly, at $\phi = \pm \frac{\pi }{2}$, the roles of $q_1$ and $q_3$ are reversed, so $q_1 \cdot q_3 = q_2 \cdot q_3 = 0$ and $q_1^2 + q_2^2 = t^2$, so $Y(\rho , \theta , t, \pm \frac{pi}{2}) = \frac{c^2}{t^2}$. Finally, as a configuration is carried by a rotation $\phi$ in the $x-z$ plane from $\mathcal {T}^0(\mathcal {C}_L)$ to $\mathcal {T}^1(\mathcal {C}_L)$, the rotation carries $\hat{k}$ to $(\sin (\phi ), 0, \cos (\phi ))$,

To interpolate values for $0< |\phi | < \frac{\pi }{2}$, we make use of another formulation of Y. As noted in [9, 13], if $\alpha _1(q)$, $\alpha _2(q)$, $\alpha _3(q)$ are the eigenvalues of MI(q), with q obtained from standard configuration r by rotation matrix R, so that $q = R r$, denote the image of $\hat{k}$ under $R^T$ by $R^T \hat{k} = (\nu _1, \nu _2, \nu _3)$. Then

$$\begin{aligned}Y(q) = \frac{c^2 \nu _1}{\alpha _1(q)} + \frac{c^2 \nu _2}{\alpha _2(q)} + \frac{c^2 \nu _3}{\alpha _3(q)}\end{aligned}$$

If we begin with standard configuration $r = (r_1, r_2, r_3)$ and apply $y-z$ rotation $R_0$ to obtain $q = (r_1, q_2, q_3)$, then in turn apply rotation $R(\phi )$ to rotate by $\phi$ in the $x-z$ plane, then $R_0^T \hat{k} = (0, -\sin (\psi ), \cos (\psi ) )$ for some $\psi$, while $(R(\phi ) R_0)^T\hat{k} = (-\sin (\phi ), -\cos (\phi ) \sin (\psi ), \cos (\phi ) \cos (\psi ))$. That is, $Y(R(\phi )q) = \sin ^2(\phi ) Y(R(\frac{\pi }{2}) q)+ \cos ^2(\phi ) Y(q)$. $\square$

Our first application of this expression is to restrict our attention to $\mathcal {T}(\mathcal {C}_L)$, as the boundary set $\partial \mathfrak {B}(d)$ is contained there for $0 < d \le \frac{27}{2c^2}$.

Lemma 3.2.2

For every $0< d < \frac{27}{2c^2}$, $\partial \mathfrak {B}(d) = D^{-1}(d)$ is contained in the rotation of $\mathcal {T}(\mathcal {C}_L)$ around the z-axis.

Proof

We observed in 3.1.1 that every $q \in \mathcal {S}\setminus \mathcal {C}$ is a rotation of some $q'(t) \in \mathcal {T}(\mathcal {C}_L, t)$ for some $0 < t \le \sqrt{\frac{2}{3}}$ . It therefore suffices to show that $D(q, \theta , t, \phi ) > \frac{27}{2c^2}$ for $\frac{1}{2} < t \le \sqrt{\frac{2}{3}}$. The global minimum of U(q) is achieved at the tetrahedral configuration, with $U_{\min } = 3\sqrt{6}$. We therefore need only show that $Y(\rho , \theta , t, \phi ) < 4 c^2$ for $\frac{1}{2} < t \le \sqrt{\frac{2}{3}}$.

As a function of $\theta$, Y is maximized when $\theta = 0$. Then, as a function of $\rho$, $Y(\rho , 0, t, \phi )$ is maximized at $\rho = 0$. $Y(0, 0, t, \phi ) = \frac{c^2 \sin ^2(\phi )}{t^2} + \frac{c^2 \cos ^2(\phi )}{1-t^2}$. Optimizing this function on $\frac{1}{2} \le t \le \sqrt{\frac{2}{3}}$, $0 \le \phi \le \frac{\pi }{2}$, the expression achieves a maximum of $4 c^2$ at $t = \frac{1}{2}$, $\phi = \frac{\pi }{2}$. $\square$

We next characterize the behavior of $\partial \mathfrak {B}(d)$ on $\mathcal {T}(\mathcal {C}_L)$.

Lemma 3.2.3

On $\mathcal {T}(\mathcal {C}_L)$, $D(q, t, \phi )$ is a decreasing function of $| \phi |$. On $\mathcal {T}^1(\mathcal {C}_L)$, $D(q, t, \frac{\pi }{2}) = \frac{ t^2 U^2(q(t))}{c^2}$ is monotone increasing in t. At $t = \frac{1}{2}$, $D(q,t,\phi ) \ge \frac{27}{2c^2}$ for all q, and on $\mathcal {T}^0(\mathcal {C}_L)$, (i.e. $\phi = 0$), $D(q,t,\phi ) \ge \frac{81}{2c^2}$ for all q and t.

Proof

The proof that $D(q, t, \frac{\pi }{2})$ is monotone decreasing in $| \phi |$, and that $D(q, t, \frac{\pi }{2})$ is monotone increasing in t, were given in [9, Lemma 8.4].

At $t = \frac{1}{2}$, Y is an function with $| \phi |$, and at $\phi = \pm \frac{\pi }{2}$, $Y(q,\frac{1}{2},\frac{\pm \pi }{2}) = \frac{c^2}{t^2} = 4 c^2$. When $\phi = 0$,

$$\begin{aligned}Y(q(t)) = \frac{ ( 1 - t^2 \rho ^2) c^2}{1 - t^2 + t^4 \rho ^2 (1 - \rho ^2) \sin ^2(\theta )} \le \frac{( 1 - t^2 \rho ^2) c^2}{1 - t^2 } \le \frac{ c^2 }{1 - t^2 } \le \frac{4 c^2}{3}.\end{aligned}$$

$\square$

3.3 The Collision Set

It is natural to expect the structure of the collision set $\Delta$ to play a significant role in determining the homology of the integral manifolds as $h \rightarrow -\infty$. Section 3.4 will show that the collision set also plays a direct role in the low-energy bifurcations at infinity. It is therefore an important preliminary to lay out the structure of the collision set and its intersection with $\mathcal {T}(\mathcal {C}_L)$. As a starting point, we register the following observations (see Fig. 1).

Lemma 3.3.1

For four masses, the collision set $\Delta$ in the mass ellipsoid $\mathcal {S}$ has the following properties:

• The full collision set $\Delta$ is the union of the six binary collision sets

  $$\begin{aligned}\Delta _{ij} = \{ (x_1, x_2, x_3, x_4) \in \mathcal {S} | x_i = x_j \}\end{aligned}$$

• Each $\Delta _{ij} \cong S^{5}$. Each $\Delta _{ij}$ intersects $\mathcal {C}_L$ in a circle, with $\Delta _{ijC} \cong SO_3$.

• Intersections of binary collision sets have two forms: there are four triple collision sets $\Delta _{ijk}$ with $x_i = x_j = x_k$ for three distinct indices i, j, k; and three double binary collision sets $\Delta _{ij,kl}$ with $x_i = x_j$ and $x_k = x_l$ for distinct indices i, j, k, l.

• All intersections of binary collision sets $\Delta _{ijk}$ and $\Delta _{ij,kl}$ are collinear and homeomorphic to $S^2$. Each intersects $\mathcal {C}_L$ in a pair of antipodal points.

• Total collision does not occur in $\mathcal {S}$.

• Denote the complement in $\Delta _{ij}$ of the triple collision points and double binary collision points by $\Delta _{ij}^o$. Each $\Delta _{ij}$ intersects two triple collision sets and one double binary collision set, so $Delta_{ij}^o \cong S^5\setminus (\sqcup _{3} S^2)$ and has the homology of $S^2 \times (S^2 \vee S^2)$. Each $\Delta _{ijL}$ contains four triple collision points and two double binary collision points, which divide $\Delta _{ijL}^o$ into six disjoint intervals.

Those observations are independent of the parameterization $\mathcal {T}(\mathcal {C})$. In that parameterization, it is clear that $\mathcal {T}(\mathcal {C}) \cap \Delta _{\mathcal {S}} \subset \mathcal {T}(\Delta _L)$. Further, if $q(t) \in \mathcal {T}(q_0,t) \cap \Delta$, then for all $\tau$, $q(\tau ) \in \mathcal {T}(q_0, \tau )$, so $\mathcal {T}(\mathcal {C}_L) \cap \Delta \cong (\mathcal {T}(\Delta _L, \tau ) \cap \Delta ) \times \mathbb {I}$. We will abuse notation and refer to $(\mathcal {T}(\Delta _L, \tau ) \cap \Delta )$ as $\mathcal {X}(\Delta _L) \cap \Delta$. As this is independent of orientation, we will further focus on the description of $\mathcal {X}^1(\Delta _L) \cap \Delta$ and the complements $\mathcal {X}(\Delta _L) \setminus \Delta$.

Lemma 3.3.2

For $q_0 \in \Delta _{L}$, we have the following descriptions:

• If $q_0 \in \Delta _{ijL}^o$, then $\mathcal {X}^1(q_0) \cap \Delta \subset \Delta _{ij}^o$ and $\mathcal {X}^1(q_0) \cap \Delta \cong S^1$, with $\mathcal {X}^1(q_0) \setminus \Delta \cong S^3 \setminus S^1 \cong S^1 \times D^2$

• If $q_0 \in \Delta _{ij,klL}$, then $\mathcal {X}^1(q_0) \cap \Delta \subset \Delta _{ij} \cup \Delta _{kl}$ and $\mathcal {X}^1(q_0) \cap \Delta \cong \bigsqcup _{2} S^1$, with $\mathcal {X}^1(q_0) \cap \Delta \cong S^3 \setminus \bigsqcup _{2} S^1 \cong T^2 \times (-1,1)$

• If $q_0 \in \Delta _{ijkL}$, then $\mathcal {X}^1(q_0) \cap \Delta \subset \Delta _{ij} \cup \Delta _{ik} \cup \Delta _{jk}$ and $\mathcal {X}^1(q_0) \cap \Delta \cong \bigsqcup _{3} S^1$, with $\mathcal {X}^1(q_0) \cap \Delta \cong S^3 \setminus \bigsqcup _{3} S^1 \cong S^1 \times (S^2\setminus \{ 3 pts.\}$

Integrating this pointwise description across $\mathcal {X}^1(\mathcal {C}) \cong S^2 \times S^3$, the collision set consists of six tori over the the six circles in $\Delta _L$. While the circles $\Delta _{ijL}$ intersect, the tori lying over them do not.

3.4 Limiting Behavior of D on $\mathcal {X}^1(\mathcal {C}_L)$

We next consider the limiting behavior of D on $\mathcal {X}^1(\mathcal {C}_L)$. On $\mathcal {T}^1(\mathcal {C}_L)$, $D(q, t) =\frac{ t^2}{c^2} U^2(q(t)) =\frac{1}{c^2} U^2(\frac{q(t)}{t})$, Configurations in $\mathcal {T}^1(\mathcal {C}_L)$ have the form $(t q_1, t q_2, \sqrt{1-t^2}q_3)$ with $q_1\cdot q_3 = q_2 \cdot q_3 = 0$. Observe that $\frac{q(t)}{t} = (q_1, q_2,\frac{\sqrt{1 - t^2}}{t} q_3)$, with $\frac{\sqrt{1 - t^2}}{t} \rightarrow \infty$ as $t \rightarrow 0$. If $q_{3i} \ne q_{3j}$, then as $t \rightarrow 0$, $r_{ij}(t) \approx \frac{\sqrt{1-t^2}}{t}|q_{3i} - q_{3j}|$ and $\frac{1}{r_{ij}} \rightarrow 0$. On the other hand, if $q_{3i} = q_{3j}$, then $r_{ij} = \sqrt{(q_{1i}-q_{1j})^2 + (q_{2i}-q_{2j})^2}$. If we partition the particle indices, with i and j in the same partition if $q_{3i} = q_{3j}$, let $q_\sigma = (q_{1i_i}, \ldots q_{1i_k},q_{2i_i}, \ldots q_{2i_k})$ for $i \in \sigma$, let $U_\sigma (q_\sigma )$ denote the internal potential $U_\sigma (q_\sigma ) = \sum _{i,j \in \sigma , i \ne j} \frac{1}{\sqrt{(q_{1i}-q_{1j})^2 + (q_{2i}-q_{2j})^2}}$ and let $U_0(q) = \sum _\sigma U_\sigma (q_\sigma )$. Then

$$\begin{aligned}LD(q) = \frac{1}{c^2}U_0^2(q) = {\left\{ \begin{array}{ll} 0 &{} q_3 \not \in \Delta \\ \frac{1}{c^2}\left( \frac{1}{\sqrt{(q_{1i} - q_{1j})^2 + (q_{2i} - q_{2j})^2}} \right) ^2 &{} q_3 \in \Delta _{ij} \\ \frac{1}{c^2}\left( \frac{1}{\sqrt{(q_{1i} - q_{1j})^2 + (q_{2i} - q_{2j})^2}} + \frac{1}{\sqrt{(q_{1k} - q_{1l})^2 + (q_{2k} - q_{2l})^2}}\right) ^2 &{} q_3 \in \Delta _{ij,kl} \\ \frac{1}{c^2}\left( \frac{1}{\sqrt{(q_{1i} - q_{1j})^2 + (q_{2i} - q_{2j})^2}} + \frac{1}{\sqrt{(q_{1i} - q_{1k})^2 + (q_{2i} - q_{2k})^2}} \right. \\ \quad \left. + \frac{1}{\sqrt{(q_{1j} - q_{1k})^2 + (q_{2j} - q_{2k})^2}}\right) ^2 &{} q_3 \in \Delta _{ijk} \\ \end{array}\right. }\end{aligned}$$

Lemma 3.4.1

The limiting value of $D(q, t, \pm \frac{\pi }{2})$ as $t \rightarrow 0$ is

$$\begin{aligned} LD(q) = \lim _{t\rightarrow 0} D(q,t,\frac{\pi }{2}) = \frac{1}{c^2} U^2_0(q)\end{aligned}$$

It is defined for $q \in \mathcal {X}^1(\mathcal {C}_L) \setminus \Delta$ and is upper semi-continuous. For $0< | \phi | < \frac{\pi }{2}$, the limiting value of $D(q, t, \phi )$ as $t \rightarrow 0$ is $\frac{LD(q)}{\sin ^2 (\phi )}$.

Proof

As we have noted, rotating by $R(\phi )$ does not change $U(R(\phi )q)$, so for $0< | \phi | < \frac{\pi }{2}$,

$$\begin{aligned}\begin{array}{lll} D(q,t,\phi ) &{} = &{} \frac{U^2(R(\phi )q)}{Y(q,t,\phi )} = \frac{U^2(q)}{Y(q,t,\phi )} \\ &{} = &{} \frac{ U^2(q(t))}{c^2}\left( \frac{\sin ^2(\phi )}{t^2} + \frac{\cos ^2(\phi ) (1-t^2 \rho ^2) }{(1 - t^2 + t^4 \rho ^2 (1-\rho ^2) \sin ^2 (\theta )}\right) ^{-1} \\ &{} = &{} \frac{t^2}{c^2} U^2(q,t) \frac{1 - t^2 + t^4 \rho ^2 (1-\rho ^2) \sin ^2(\theta )}{t^2 (1 - t^2 \rho ^2) \cos ^2(\phi ) + (1 - t^2 + t^4 \rho ^2 (1 - \rho ^2) sin^2(\theta ))) Sin^2(\phi ))^2} \\ &{} = &{}D(q,t,\frac{\pi }{2}) \frac{1 - t^2 + t^4 \rho ^2 (1-\rho ^2) \sin ^2(\theta )}{t^2 (1 - t^2 \rho ^2) \cos ^2(\phi ) + (1 - t^2 + t^4 \rho ^2 (1 - \rho ^2)\sin ^2(\theta ))) \sin ^2(\phi ))^2} \\ \end{array}\end{aligned}$$

As $t \rightarrow 0$, the quantity multiplying $D(q,t,\frac{\pi }{2})$ limits to $\csc ^{2}(\phi )$, as required. $\square$

The sublevel sets of LD on $\mathcal {X}(\mathcal {C}_L)$ will be key objects of study. We will denote these sets as

$$\begin{aligned} X(d) = \{ q \in \mathcal {X}^1(\mathcal {C}_L) \setminus \Delta | LD(q) \le d \}.\end{aligned}$$

As $LD \equiv 0$ on $\mathcal {X}^1(\mathcal {C}_L\setminus \Delta _L)$, the set $\bigsqcup _{24} D^2 \times S^3 \cong \mathcal {X}^1(\mathcal {C}_L\setminus \Delta _L) \subset X(d)$ for all d. The interest is in the behavior of LD on $\mathcal {X}^1(\Delta _L)\setminus \Delta$. For each $q \in \Delta _L$, the domain of LD(q) is the set $\mathcal {X}^1(q)\setminus \Delta \cong S^3 \setminus \Delta _S$. The elements of interest are as follows:

Lemma 3.4.2

For $q \in \Delta _L$, the structures of the sets $\mathcal {X}^1(q)$ and the behavior of LD on those sets are:

$$\begin{aligned}\begin{array}{|c|c|c|c|c|c|c|} \hline {q} &{} {\Delta \cap \mathcal {X}^1(q)} &{} {\mathcal {X}^1(q) \setminus \Delta } &{} \text {critical} &{} \text {critical } &{} \text {level} &{}{X(d) \cap \mathcal {X}^1(q)} \\ &{} &{} &{} \text {points} &{} \text { values} &{} \text {sets} &{} \\ \hline \Delta _{ij}^o &{} S^1 &{} S^1 \times D^2 &{} S^1 &{} \frac{1}{2 c^2} &{} T^2 &{} S^1\times \bar{D}^2 \\ \hline \Delta _{ij,kl} &{} S^1 \times S^0 &{} T^2 \times (-1,1) &{} T^2 &{} \frac{4}{c^2} &{} S^0 \times T^2 &{} T^2 \times \mathbb {I} \\ \hline { \Delta _{ijk}} &{}{\bigsqcup _3 S^1} &{} { (D^2 \setminus \text {2 pts.}) \times S^1} &{} S^0 \times S^1 &{} \frac{9}{c^2} &{} S^0 \times T^2 &{} S^0 \times S^1 \times \bar{D}^2 \\ &{} &{} &{} \bigsqcup _{3} S^1 &{} \frac{25}{2 c^2} &{} \bigsqcup _3 \times T^2 &{} S^3 \setminus (\bigsqcup _3 S^1 \times D^2) \\ \hline \end{array}\end{aligned}$$

Proof

First consider $q_3 \in \Delta _{12L}$ with $q_{31} = q_{32}$ and $q_{33}$ and $q_{34}$ distinct. It is convenient to consider 1/LD(q) on $\mathcal {X}^1(q_0)$. This is the quadratic $(q_{11} - q_{12})^2 + (q_{21} - q_{22})^2$ subject to the constraints $q_3 \cdot q_1 = q_3 \cdot q_2 = 0$, $(1,1,1,1)\cdot q_1 = (1,1,1,1)\cdot q_2 = 0$ and $q_1^2 + q_2^2 = 1$. These constraints reduce to an elliptical constraint on $q_{11}, q_{12}, q_{21}, q_{22}$. Via Lagrange multipliers, we find that the only critical points of $\frac{1}{LD}$ are the maxima $1/LD = 2$ at $(q_{11}, - q_{11}, q_{21}, -q_{21})$ and minima of 0 at $(q_{11}, q_{11}, q_{21}, q_{21})$. Each of these describes a circle in $S^3$. If we view $S^3$ as the join $S^1*S^1$, these can be viewed as the two end circles. That is, LD achieves its minimum of $\frac{1}{2c^2}$ when $q_1 = (q_{11},-q_{11},0,0)$, $q_2 = (q_{21}, -q_{21}, 0, 0)$ with $2 q_{11}^2 + 2 q_{21}^2 = 1$, has level sets $LD^{-1}(d) \cong S^1 \times S^1$ for all $d > \frac{1}{2}$, and diverges to infinity when $q_{11} = q_{12}$ and $q_{21} = q_{22}$. For all $d > \frac{1}{2c^2}$, the sublevel sets $LD^{-1}([0,d] \cong S^1 \times \bar{D}^2$.

For $q_3 \in \Delta _{12,34L}$, the only values possible for $q_3$ are $\pm \frac{1}{2}(1,1,-1,-1)$, so the constraints on $(q_1, q_2)$ restrict to $q_1 = (q_{11}, -q_{11},-q_{14}, q_{14})$ and $q_2 = (q_{21}, -q_{21},-q_{24}, q_{24})$ with $2(q_{11}^2 + q_{14}^2+q_{21}^2 + q_{24}^2 = 1$. On this set,

$$\begin{aligned}LD(q) = \frac{1}{c^2}\left( \frac{1}{\sqrt{4q_{11}^2 + 4q_{21}^2}} + \frac{1}{\sqrt{4q_{14}^2 + 4q_{24}^2}}\right) ^2\end{aligned}$$

Critical points occur when

$$\begin{aligned}\begin{array}{lll} \nabla LD(q) &{} = &{}-\frac{8}{c^2} \left( \frac{1}{\sqrt{4q_{11}^2 + 4q_{21}^2}} + \frac{1}{\sqrt{4q_{14}^2 + 4q_{24}^2}}\right) \\ &{}&{}\left( \frac{ q_{11}}{(4q_{11}^2 + 4q_{21}^2)^{3/2}}, \frac{ q_{14}}{(4q_{14}^2 + 4q_{24}^2)^{3/2}}, \frac{ q_{22}}{(4q_{11}^2 + 4q_{21}^2)^{3/2}}, \frac{ q_{24}}{(4q_{21}^2 + 4q_{24}^2)^{3/2}}\right) \end{array}\end{aligned}$$

is a multiple of $(q_{11}, q_{14}, q_{21}, q_{24})$. This occurs when $q_{11}^2 + q_{14}^2 = q_{21}^2 + q_{24}^2$. Combining this with the constraint $2 (q_{11}^2 + q_{14}^2 + q_{21}^2 + q_{24}^2) = 1$, we see $q_{11}^2 + q_{14}^2 = q_{21}^2 + q_{24}^2 = \frac{1}{4}$ and $LD(q,0) = \frac{4}{c^2}$. At the other end of the spectrum, if either $q_{11}^2 + q_{21}^2$ or $q_{14}^2 + q_{24}^2$ goes to zero, then LD diverges to infinity. If we view $S^3$ as the join $S^1*S^1$, then LD takes on its minimum value of $\frac{4}{c^2}$ at the equator $S^1 \times S^1$, and grows monotonically as one moves from the equator to the ends. Thus, for $d > \frac{4}{c^2}$, the level set $\{ LD(q) = d\}$ is homeomorphic to $S^0 \times S^1 \times S^1$, and the sublevel set $\{ LD(q) \le d\}$ is homeomorphic to $T^2 \times \mathbb {I}$.

Finally, for $q_3 \in \Delta _{123}$ and $\Delta _{124}$, we have more structure. The only values for $q_3$ are $\pm \frac{1}{2\sqrt{3}}(1,1,1,-3)$, so the constraints on $(q_1, q_2)$ restrict to $q_{14} = q_{24} = 0$. That is, for $q_1 = (q_{11}, q_{12}, q_{13},0)$ and $q_2 = (q_{21}, q_{22}, q_{23}, 0)$, we have

$$\begin{aligned}LD(q)= & {} \frac{1}{c^2}\left( \frac{1}{\sqrt{(q_{11} - q_{12})^2 + (q_{21} - q_{22})^2}} + \frac{1}{\sqrt{(q_{11} - q_{13})^2 + (q_{21} - q_{23})^2}} \right. \\&\left. + \frac{1}{\sqrt{(q_{12} - q_{13})^2 + (q_{22} - q_{23})^2}}\right) ^2 \end{aligned}$$

subject to the constraints $\sum _{j=1}^3 q_{ij} = 0$ and $q_1^2 + q_2^2= 1$. That is, this is exactly the same as the three-body planar potential, whose behavior on $S^3$ is well-known: the function achieves a minimum at the two circles of equilateral configurations, undergoes bifurcation at the three circles of Euler configurations, and diverges to infinity at the three circles of binary collision. These occur at the values indicated. The sublevel sets below $d = \frac{25}{2c^2}$ consist of two copies of $S^1 \times \bar{D}^2$. At $\frac{25}{2c^2}$, these touch at three copies of $S^1$, leaving complement consisting of three copies of$S^1 \times D^2$, which shrink to the three circles of collision configurations. $\square$

Note that the critical values of LD correspond to the infinite singular values of Albouy’s algorithm, with the singular points of LD corresponding to the projections of Albouy’s horizontal critical sequences.

The next question to consider is the manner in which these sets fit together. As q varies along $\Delta _{ijL}$, the intersections of $\mathcal {X}^1(q)$ with the level sets $D^{-1}(d)$, sublevel sets X(d) and the collision set $\Delta$ are homeomorphic to $T^2$, $S^1 \times \bar{D}^2$ and $S^1$ respectively. It is clear that they vary continuously with q, and have well-defined (and homeomorphic) limit sets as q approaches $\Delta _{ij,klL}$ or $\Delta _{ijkL}$.

In $\Delta _L$, the points $\Delta _{ij,kl}$ are the intersections of $\Delta _{ij}$ and $\Delta _{kl}$, so there are two axes of approach to $q_0 \in \Delta _{ij,klL}$ within $\Delta _L$, namely along $\Delta _{ijL}$ and along $\Delta _{klL}$. For q along each of those directions of approach, the level sets $D^{-1}(d)\cap \mathcal {X}^1(q)$, sublevel sets $X(d) \cap \mathcal {X}^1(q)$ and collision set $\Delta \cap \mathcal {X}^1(q)$ are homeomorphic to $T^2$, $S^1 \times \bar{D}^2$ and $S^1$ respectively. For $d > 4$, the corresponding level sets, sublevel sets and collision set in $\mathcal {X}^1(q)$ consist of two disjoint copies of $T^2$, $S^1 \times \bar{D}^2$ and $S^1$ respectively.

For $q \in \Delta _{ijkL} \cup \Delta _{ij,klL}$, define

$$\begin{aligned}\begin{array}{lll} \Delta _{ij}(q) &{} = &{} \text {cl}(\Delta \cap \mathcal {X}^1(\Delta ^o_{ijL})) \cap \mathcal {X}^1(q) \\ X_{ij}(d, q) &{} = &{} \text {cl}(X(d) \cap \mathcal {X}^1(\Delta ^o_{ijL})) \cap \mathcal {X}^1(q) \\ \end{array}\end{aligned}$$

The sets $\Delta _{ij}(q)$ are the limiting sets in $\mathcal {X}^1(q)$ of the collision sets lying over $\Delta ^o_{ij}$; similarly, the sets $X_{ij}(d,q)$ are the limiting sets in $\mathcal {X}^1(q)$ of the level sets of D over $\Delta ^o_{ij}$. If $q \in \Delta _{ij,klL}$, then there are two limit sets $\Delta _{ij}(q)$ and $\Delta _{kl}(q)$, $X_{ij}(q)$ and $X_{kl}(q)$. If $q \in \Delta _{ij,klL}$, then there are three limit sets $\Delta _{ij}(q)$, $\Delta _{ik}(q)$, $\Delta _{jk}(q)$ and $X_{ij}(q)$, $X_{ik}(q)$, $X_{jk}(q)$. While collision sets limit to collision sets, the discontinuity of D at collision means that the sets $X_{ij}(d,q)$ are distinct from $D^{-1}(d) \cap \mathcal {X}^1(d)$. The sets have the following descriptions:

Lemma 3.4.3

• (1) At $q \in \Delta _{ij,kl}$, the structures are:

  • (a) $\Delta \cap \mathcal {X}^1(q) = \Delta _{ij}(q) \sqcup \Delta _{kl}(q)$, with $\Delta _{ij}(q) \cong \Delta _{kl}(q) \cong S^1$.

  • (b) For all $d \ge \frac{1}{2c^2}$, $X_{ij}(d, q) \cong X_{kl}(d, q) \cong \bar{D}^2 \times S^1$.

  • (c) For all d, $(X(d) \cap \mathcal {X}^1(q)) \subset X_{ij}(d, q) \cap X_{kl}(d, q)$, with the inclusion a homotopy equivalence.

  • (d) For $d < \frac{4}{c^2}$, $X_{ij}(d, q) \cap X_{kl}(d, q) = \emptyset$, while for $d > \frac{4}{c^2}$, $X_{ij}(d, q) \cap X_{kl}(d, q) \cong T^2 \times \mathbb {I}$.

• (2) At $q \in \Delta _{ijk}$, the structures are:

  • (a) $\Delta \cap \mathcal {X}^1(q) = \Delta _{ij}(q) \sqcup \Delta _{ik}(q) \sqcup \Delta _{jk}(q)$, with $\Delta _{ij}(q) \cong \Delta _{ik}(q) \cong \Delta _{jk}(q) \cong S^1$.

  • (b) For all $d \ge \frac{1}{2c^2}$, $X_{ij}(d, q) \cong X_{ik}(d, q) \cong X_{jk}(d, q) \cong \bar{D}^2 \times S^1$.

  • (c)
    $$\begin{aligned}X{ijk}(d,q)= & {} X_{ij}(d, q) \cap X_{ik}(d, q) \cap X_{jk}(d, q) \\\cong & {} {\left\{ \begin{array}{ll} \emptyset &{} d< \frac{1}{c^2} \\ \bar{D}^2 \times S^1 \times S^0 &{} \frac{1}{c^2}< d<\frac{2}{c^2} \\ (S^2 \setminus \bigsqcup _3 D^2) \times S^1 &{} \frac{2}{c^2} < d \end{array}\right. }\end{aligned}$$
  • (d)
    $$\begin{aligned}X(d) \cap \mathcal {X}^1(q) \cong {\left\{ \begin{array}{ll} \emptyset &{} d< \frac{9}{c^2} \\ \bar{D}^2 \times S^1 \times S^0 &{} \frac{9}{c^2}< d< \frac{25}{2c^2} \\ (S^2 \setminus \bigsqcup _3 D^2) \times S^1 &{} \frac{25}{22c^2} < d \end{array}\right. }\end{aligned}$$

  • (e) For all d, $(X(d) \cap \mathcal {X}^1(q)) \subset X_{ijk}(d, q)$, with the inclusion a homotopy equivalence for $\frac{25}{2} < d$. For $9< d < \frac{25}{2}$, the induced map $\iota _*:H_*(X(d) \cap \mathcal {X}^1(q)) \rightarrow H_*(X_{ijk}(d, q))$ is $H_*(S^0)\otimes H_*(S^1) \rightarrow H_*(S^1 \vee S^1)\otimes H_*(S^1)$, with the map on the second factor an isomorphism.

Proof

As a representative case of $\Delta _{ij,kl}$, consider $q_0 = (\frac{1}{2},\frac{1}{2},-\frac{1}{2},-\frac{1}{2}) \in \Delta _{12,34}$. Then

$$\begin{aligned}\mathcal {X}^1(q_0) = \{ (q_1, q_2) \in \mathcal {S}^8 | q_{i1}+q_{i2} = q_{i3}+q_{i4} = 0 \}\end{aligned}$$

If we consider $q \in \Delta _{12}^o$ approaching $q_0$, the limiting value of LD on $\mathcal {X}^1(q)$ is $LD_{12}(q) = \frac{1}{4c^2(q_{11}^2 + q_{21}^2)}$, while the limiting value of LD when approaching along $\Delta _{34}^o$ is $LD_{34}(q) = \frac{1}{4c^2(q_{13}^2 + q_{23}^2)}$ and the actual value of LD on $\mathcal {X}^1(q)$ is $LD(q) = \left( \sqrt{LD_{12}(q)}+ \sqrt{LD_{34}(q)}\right) ^2.$ The results from Lemma 3.4.2 apply to $LD_{12}(q)$ and $LD_{34}(q)$, with $LD_{12}(q)$ growing from $\frac{1}{2c^2}$ to $\infty$ and $LD_{34}(q)$ declining from $\infty$ to $\frac{1}{2c^2}$ as $q_{11}^2 + q_{21}^2$ declines from $\frac{1}{2c^2}$ to 0. The behavior of the level sets of $LD_{12}$, $LD_{34}$ and LD follow immediately.

As a representative case of $\Delta _{ijk}$, consider $q_0 = (\frac{1}{2\sqrt{3}},\frac{1}{2\sqrt{3}},\frac{1}{2\sqrt{3}},-\frac{\sqrt{3}}{2}) \in \Delta _{123}$. Then $\mathcal {X}^1(q_0) = \{ (q_1, q_2) \in S^7 | q_{14} = q_{24} = 0, q_{11}+q_{12}+q_{13} = 0, q_{21}+q_{22}+q_{23} = 0 \}$, on which the three directions of approach, $\Delta _{12}^o$, $\Delta _{13}^o$ and $\Delta _{23}^o$ generate limiting functions

$$\begin{aligned}\begin{array}{ccc} LD_{12}(q) &{} = &{} \frac{1}{c^2} \frac{1}{(q_{11} - q_{12})^2 + (q_{21}-q_{22})^2} \\ LD_{13}(q) &{} = &{} \frac{1}{c^2} \frac{1}{(q_{11} - q_{13})^2 + (q_{21}-q_{23})^2} \\ LD_{23}(q) &{} = &{} \frac{1}{c^2} \frac{1}{(q_{12} - q_{13})^2 + (q_{22}-q_{23})^2} \end{array}\end{aligned}$$

while

$$\begin{aligned}LD(q) = \left( \sqrt{LD_{12}(q)}+ \sqrt{LD_{13}(q)} + \sqrt{LD_{23}(q)}\right) ^2\end{aligned}$$

We have observed that $\mathcal {X}^1(q_0) \cong S^3$ corresponds to the planar 3-body problem with equal masses. There is a rotational symmetry, which allows us to focus on the behavior on the quotient space $S^3/S^1 \cong S^2$. We can view a point in $S^2$ as the set of oriented triangles with the sum of the squares of the side lengths equal to 3. The poles correspond to the Lagrange configurations and the equator to the collinear configurations. The equator is divided into three intervals by the three collision points, with the collinear central configurations antipodal to them.

The three functions $LD_{ij}(q)$ are the reciprocals of the squares of the side-lengths, while LD is the square of the sum of the reciprocals of the side lengths. The function $LD_{ij}(q)$ has minimum value of $\frac{1}{2c^2}$ at the central configuration with particle k lying between particles i and j, growing monotonically as one moves along the equator towards the ij collision point, with circular level sets.

The triple intersections $LD_{12}^{-1}([0,d]) \cap LD_{13}^{-1}([0,d]) \cap LD_{23}^{-1}([0,d])$ are empty for $d < \frac{1}{c^2}$, form two disks around the two Lagrange points for $\frac{1}{c^2}< d < \frac{2}{c^2}$, and have the form $S^2 \setminus \bigsqcup _3 D^2$ for $\frac{2}{c^2} < d$. We have already observed the same structure for $LD^{-1}([0,d])$, with corresponding bifurcation values $d = \frac{9}{c^2}$ and $d = \frac{25}{2c^2}$. As at $\Delta _{12,34}$, the set $LD^{-1}([0,d])$ is a proper subset of the intersection of the sets $LD_{ij}^{-1}([0,d])$. In particular, for $d > \frac{25}{2c^2}$, the inclusion of $LD^{-1}([0,d])$ into the intersection is a homotopy equivalence, while for $\frac{9}{c^2}< d < \frac{25}{2c^2}$ we have

The $S^1$ bundle over $S^2 \setminus \bigsqcup _3 D^2 \simeq S^1 \vee S^1$ is the restriction of $S^1 \times S^3 \rightarrow S^2$, and so is orientable. The spectral sequence of the bundle collapses, hence the homology groups are those of the product $H_*(S^1 \vee S^1) \otimes H_*(S^1)$. $\square$

3.5 Structure of $\mathfrak {B}(d)$ and $\partial \mathfrak {B}(d)$

We are now ready to formulate the main step in the translation of analysis to topology.

Proposition 3.5.1

For every $0< d < \frac{27}{2c^2}$, the super-level sets $\mathfrak {B}(d) = D^{-1}([d,\infty ))$ and their subsets $\partial \mathfrak {B}(d) = D^{-1}(d)$, $\mathfrak {B}_0(d) = \mathfrak {B}(d) \cap \mathcal {B}_0$ and $\partial \mathfrak {B}_0^+(d) = \partial \mathfrak {B}(d) \cup \mathfrak {B}_0(d)$ have the following properties:

• $\mathfrak {B}(d)$ is a strong deformation retraction of $S^8 \setminus (\mathcal {C} \cup \Delta )$.

• The intersection $\partial \mathfrak {B}_0(d) = \partial \mathfrak {B}(d) \cap \mathcal {B}_0$ is homeomorphic to twelve copies of $D^2 \times S^1 \times S^3 \times S^0$, while $\mathfrak {B}_0(d)$ is homeomorphic to twelve copies of $D^2 \times S^1 \times S^3 \times \mathbb {I}$.

• There is a homotopy equivalence $X(d) \rightarrow \partial \mathfrak {B}(d)$. The set $\partial \mathfrak {B}(d) \subset \mathcal {T}(\mathcal {C}_L)$ has a strong deformation retraction onto $\partial \mathfrak {B}(d) \cap \mathcal {T}^1(\mathcal {C}_L)$, which is in turn homeomorphic to $X(d) \subset \mathcal {X}^1(\mathcal {C}_L).$

• The inclusion $\partial \mathfrak {B}_0(d) \rightarrow \partial \mathfrak {B}(d)$ determines $\partial \mathfrak {B}^+(d)$.

The key step in establishing these results is to describe $\partial \mathfrak {B}(d)$ as the graph of a function $\phi = f(q, t)$, as suggested by Fig. 2. To do so, we make use of the monotonicity of D, first with respect to t on $\mathcal {T}^1(\mathcal {C}_L)$, then with respect to $\phi$ on $\mathcal {T}(\mathcal {C}_L)$. The first allows us to identify the set on which the function is defined, and the second defines the function.

First, for $0 < d \le \frac{27}{2c^2}$, define $\tau :X(d) \rightarrow [0, \frac{1}{2}]$ implicitly by $D(q, \tau _d(q), \frac{\pi }{2}) = d$. The monotonicity of $D(q,t,\frac{\pi }{2})$, together with the observation that $D(q,\frac{1}{2},\frac{\pi }{2}) \ge \frac{27}{2c^2}$, guarantees that this is well-defined. For q and d with $d > LD(q)$, the continuity of D on $\mathcal {T}^1(\mathcal {C}_L)\setminus \Delta$ allows us to invoke the implicit function theorem to show that $\tau$ is continuous. Likewise, if $LD(q_0) = d$ (which can occur only occur when $q_0 \in \mathcal {X}^1(\Delta _L)$), then as $q \rightarrow q_0$, the continuity of D for $t > 0$ implies that $\tau (q) \rightarrow 0$. Next, we set $\mathcal {Q}(d) = \{ q(t) \in \mathcal {T}(\mathcal {C}_L) | t \le \tau _d(R(\frac{\pi }{2})q) \}$ and define $f_d:\mathcal {Q}(d) \rightarrow (0, \frac{\pi }{2}]$ implicitly by $D(q, t, f(q,t)) = d$. As $\frac{\partial D}{\partial \phi } < 0$ for $0< t \le \frac{1}{2}, 0 < \phi \le \frac{\pi }{2}$, a similar argument shows that $f_d(q, t)$ is defined and continuous.

Fig. 2

$\partial \mathfrak {B} \cap \mathcal {T}(q_1)$

This construction allows us to complete the proof of Proposition 3.5.1. For $\mathfrak {B}_0(d)$ and $\partial \mathfrak {B}_0(d)$, the behavior of D on $\mathcal {B}$ has already been established: for $q_1 \in \mathcal {C}_L\setminus \Delta _L$, the set $\mathcal {B}_0(q_1) \cong S^3 \times \mathbb {I}$ consists of directions of approach $(q_2, q_3)$ with $q_1 \cdot q_2 = 0$, normalized with $q_2^2 + q_3^2 = 1$. On this set, $D(q_1, q_2, q_3) = U^2(q_0) \frac{1 - (q_1 \cdot q_3)^2}{c^2}$, so for every $q_1$, $\mathfrak {B}_0(d) \cap \mathcal {B}_0(q_1) \cong S^3 \times \mathbb {I}$, while $\partial \mathfrak {B}_0(d) \cap \mathcal {B}_0(q_1) \cong S^3 \times S^0$

To fit this together with the description of $\partial \mathfrak {B}(d)$ as the graph of $\phi = f(q,t)$, we consider the limiting behavior of f as $t \rightarrow 0$. If $q(t) \in \mathcal {T}(\mathcal {C}_L \setminus \Delta _L)$, then $\lim _{t\rightarrow 0} f(q,t) = 0$, while for $q(t) \in \mathcal {T}(\Delta _L)$, $LD(q, \frac{\pi }{2}) > 0$. If $LD(q, \frac{\pi }{2}) > d$, then the ray $\{ q(t) \} \cap \mathcal {Q}(d) = \emptyset$ and f is not defined for any (q, t). On the other hand if $0< LD(q, \frac{\pi }{2}) < d$ and there exists $0< \phi _0 < \frac{\pi }{2}$ such that $LD(q, \phi _0) = \frac{LD(q, \frac{\pi }{2})}{\sin ^2(\phi _0)} = d$, so $\lim _{t\rightarrow 0} f(t) = \phi _0 > 0$.

This shows that $\partial \mathfrak {B}_0(d)$, when viewed as the limit of $\partial \mathfrak {B}(d)$, is the limit only of points in $\partial \mathfrak {B}(d) \cap \mathcal {T}(\mathcal {C}_L \setminus \Delta _L)$. Points in $\partial \mathfrak {B}(d) \cap \mathcal {T}(\Delta _L)$ do not limit to $\mathfrak {B}_0$. Instead, they limit to $\mathcal {C}\setminus \mathcal {C}_0$, and so have no limit in $\mathfrak {B}(d)$. For points $q_1 \in \mathcal {C}_L \setminus \Delta _L$, as $t \rightarrow 0$ and $f_d(t) \rightarrow 0$, then in $\mathcal {B}$, $(q_1,q_2, q_3, t, f_d(q_2, q_3, t))$ limits to $(q_1, q_2, q_3 + a q_1) \in \mathcal {B}_0$, with $a^2 = \frac{ U^2(q_0) -c^2 d}{U^2(q_0)}$. As this holds for all $q_1 \in \mathcal {C}_L\setminus \Delta _L \cong \bigsqcup _{24}D^2$, and for the full set of directions of approach $S^3$, the limiting set for $\mathcal {T}(\mathcal {C}_L)$ is homeomorphic to $\bigsqcup _{24}D^2 \times S^3 \times S^0.$

To globalize this, consider the rotation of $\mathcal {B}_0$ around the z-axis. This rotation identifies pairs of antipodal components, and does so consistently identifying the the components of $\partial \mathfrak {B}_0(d)$. That is, as the rotation through $\pi$ carries $\mathfrak {B}_0(\pm q_1)$ to $\mathfrak {B}_0(\mp q_1)$, the identification of $\{q_2, q_3\} \times [-q_1 \cdot q_3, q_1\cdot q_3]$ with $\{-q_2, -q_3\} \times [q_1 \cdot -q_3, -q_1\cdot -q_3]$ is orientation-preserving, so the identification of the two sets of the form $S^3 \times \mathbb {I}$ preserves the boundary components, forming $S^3 \times S^1 \times \mathbb {I}$, rather than the product of $S^3$ with a Möbius band.

To complete the proof of Proposition 3.5.1, define $\chi _d:X(d) \rightarrow \partial \mathfrak {B}(d) \cap \mathcal {T}^1(\mathcal {C}_L)$ by $\chi _d(q) = (q, \tau _d(q))$. Then for $d_1 < d_2$, let

$$\begin{aligned}\mathfrak {B}(d_1, d_2) = D^{-1}([d_1, d_2])\end{aligned}$$

and define $\beta :\mathfrak {B}(d_1, d_2) \rightarrow \partial \mathfrak {B}(d_1)$ by setting

$$\begin{aligned}\beta (q,t,\phi ) = {\left\{ \begin{array}{ll} (q, t \frac{\tau _{d_2(q)}}{\tau _{d_1}(q)}, f_{d_2}(q, t \frac{\tau _{d_2}(q)}{\tau _{d_1}(q)})) &{} (q,t,\phi ) = (q, t, f_{d_1}(q,t)) \in \partial \mathcal {B}(d), \tau _{d_1}(q) >0 \\ (q,0,\frac{\pi }{2}) &{} \tau _{d_1}(q) = 0 \end{array}\right. }.\end{aligned}$$

The proof of Proposition 3.5.1 then follows from the following lemma:

Lemma 3.5.1

If $0< d_1< d_2 < \frac{27}{2}$ are regular values of D, then there is a commutative diagram of embeddings

The maps $\chi _{d_i}$ are homeomorphisms and the inclusion maps $\iota _{d_i}:\partial \mathfrak {B}(d_i) \cap \mathcal {T}^1(\mathcal {C}_L) \rightarrow \mathfrak {B}(d_1, d_2)$ are homotopy equivalences. The inclusion $\partial \mathfrak {B}(d_2) \rightarrow \mathfrak {B}(d_1, d_2)$ is a homotopy equivalence with inverse $\beta$.

Proof

We have already noted that, for every $q \in X(d)$, there is a continuous $\tau _d(q)$ with $D(q, \tau _d(d)) = d$. The inverse of $\tau$ is the restriction of the projection of $\mathcal {T}^1(\mathcal {C}_L) \rightarrow \mathcal {X}^1(\mathcal {C}_L)$, which is clearly continuous. The homotopy from $\beta$ to the identity map on $\mathfrak {B}(d_1, d_2)$ is simply

$$\begin{aligned}&H(q,t,\phi ,s)\\&\quad ={\left\{ \begin{array}{ll} (q, t (1+s(\frac{\tau _{d_2}(q)}{\tau _{d_1}(q)}-1)),&{} \\ \quad f_{d_2}(q, t (1+s(\frac{\tau _{d_2}(q)}{\tau _{d_1}(q)}-1))) &{} (q,t,\phi ) = (q, t, f_{d_1}(q,t)) \in \partial \mathcal {B}(d_1), \tau _{d_1}(q) >0 \\ (q,0,\frac{\pi }{2}) &{} \tau _{d_1}(q) = 0 \end{array}\right. }.\end{aligned}$$

A similar homotopy homotopy equivalence from $\partial \mathfrak {B}(d)$ to $\partial \mathfrak {B}(d) \cap \mathcal {T}^1(\mathcal {C}_L)$ is defined by $G(q,t,f(q,t)) = (q, t (1-s) + s \tau _d(q), f(q, t (1-s) + s \tau _d(q)))$. Note that, while $\partial \mathfrak {B}(d)$ limits to $\partial \mathfrak {B}_0(d)$ from both $\phi > 0$ and $\phi < 0$, for each component of $\mathfrak {C}_L \setminus \Delta$, the limits from $\phi < 0$ and $\phi > 0$ are the two distinct components of $\partial \mathfrak {B}_0(d)$, so there is no discontinuity encountered. $\square$

With $\beta$ established as a strong deformation retraction of $\mathfrak {B}(d_1, d_2)$, it extends to a strong deformation retraction of $\mathfrak {B}(d_1)$ to $\mathfrak {B}(d_2)$.

Finally, note that the construction in Lemma 3.5.1 allows us to view X(d) as a subset of $\partial \mathfrak {B}(d)$ and hence $\mathfrak {B}(d)$ whenever convenient, and indeed to view $X(d_1)$ as a subset of $\partial \mathfrak {B}(d_2)$ and $\mathfrak {B}(d_2)$. We will make frequent use of this in Sect. 4, where we will typically suppress the map notation and treat $\chi$ and $\beta$ as inclusions.

4 Homological Calculations

The sets that contribute to the homological calculations have now been characterized in topological terms, which now allows us to turn to the computation of the relevant homology groups. The homology formulae for $H_*(\mathfrak {M})$ and $H_*(\mathfrak {M}_R)$ require the identification of $H_*(\mathfrak {B}(d))$ and the pairs $H_*(\mathfrak {B}(d), \mathfrak {B}_0(d))$, $H_*(\mathfrak {B}(d), \partial \mathfrak {B}(d))$ and $H_*(\mathfrak {B}(d), \partial \mathfrak {B}^+(d))$, as well as the corresponding elements for the reduced spaces. These calculations will be leveraged by the following observations:

• The homology groups for Region I were computed in [9, §8.2]. Taking these as a starting point, we will bootstrap from one region to the next.

• To obtain results that include Region V, we need to compute the homology groups for some $\frac{25}{2c^2}< d_5 < \frac{36 + 16\sqrt{2}}{c^2}$. To leverage the results of Sect. 3, we will focus on the parameter range $\frac{25}{2c^2}< d_5 < \frac{27}{2c^2}$. Limiting our focus on this interval allowed us to confine all of the changes in topology within $\mathcal {T}(\mathcal {C})$. As we know that $H_*(\mathfrak {M}(c,h))$ and $H_*(\mathfrak {M}_R(c,h))$ do not change across Region V, there is no loss in doing so.

• We know from Proposition 3.5.1 that $H_*(\mathfrak {B}(d))$ and $H_*(\mathfrak {B}(d), \mathfrak {B}_0(d))$ are constant for $0< d < \frac{27}{2c^2}$, so the values computed in [9, §8.2] for Region I extend across Regions II - V. This allows us to focus on computing $H_*(\mathfrak {B}(d), \partial \mathfrak {B}(d))$ and $H_*(\mathfrak {B}(d), \partial \mathfrak {B}^+(d))$ in regions II - V.

• The set $\partial \mathfrak {B}^+(d)$ is formed from $\partial \mathfrak {B}(d)$ by attaching the set $\partial \mathfrak {B}_0(d)$, whose homotopy type is unchanged from Region I to Region V. So it is only the changes in $\mathcal {B}_0(d)$, as detected by $\iota _*:\mathcal {B}_0(d) \rightarrow \mathcal {B}(d)$, that influence change in $H_*(\mathfrak {B}(d), \partial \mathfrak {B}^+(d))$.

• The homology formulae provided at the end of Sect. 2.2 include the somewhat awkward statement that $H_3(\mathfrak {M}(c,h))$ is computed as the image $\text {im}(j_*:H_3(\mathfrak {B}(d)) \rightarrow H_3(\mathfrak {B}(d), \mathfrak {B}_0(d))$. In [9], it was shown that in Region I, $H_2(\mathfrak {B}_0(d_1)) = 0$, which implies that this map is surjective and hence $H_3(\mathfrak {M}(c,h)) \cong H_3(\mathfrak {B}(d), \mathfrak {B}_0(d))$. As neither $H_3(\mathfrak {B}(d))$ nor $H_3(\mathfrak {B}(d), \mathfrak {B}_0(d))$ change as d varies from Region I to Region V, this implies that $\text {im}(j_*)$ remains unchanged across these regions. The homology formula simplifies to

  $$\begin{aligned}H_{k}\left( \mathfrak {M} \right) \cong {\left\{ \begin{array}{ll} H_{k}\left( \mathfrak {B}, \mathfrak {B}_0 \right) \oplus H_{k-6}\left( \mathfrak {B}, \partial \mathfrak {B}^+ \right) &{} k= 3, 4, 5, 6, 10, 11, 12\\ H_{k}\left( \mathfrak {B} \right) \oplus H_{k-6}\left( \mathfrak {B}, \partial \mathfrak {B} \right) &{} k = 0, 1, 2, 7, 8 , 9, k > 12 \\ \end{array}\right. }\end{aligned}$$

  with the values of $H_*(\mathfrak {B}(d))$ and $H_*(\mathfrak {B}(d), \mathfrak {B}_0(d))$ those already computed for Region I.

• The key to the homology calculations is the structure of $\partial \mathcal {B}(d).$ Lemma 3.5.1 shows that $\partial \mathcal {B}(d) \simeq X(d)$, the sublevel set of LD, so our computations will be driven by analyzing the behavior of $LD(q_0)$ on the set $\mathcal {X}^1(\mathcal {C})$.

4.1 Homological Preliminaries

Before entering in to the homological calculations, we introduce two ingredients that will be useful in those calculations: the homology group calculations for Region I from [9, §8.2] and a braid diagram relating the groups from Region I to the corresponding groups for regions II–V.

The homology groups of $\mathfrak {B}(d_1)$, relevant subspaces and corresponding pairs of spaces are

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1) ) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{6} &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{6} &{} 0 \\ \hline (\mathfrak {B}(d_1),\partial \mathfrak {B}^+_0(d_1)) &{} 0 &{}\mathbb {Z}^{11} &{} \mathbb {Z}_2^5 &{} \mathbb {Z}^6 &{} \mathbb {Z}^{11} &{} 0 &{} \mathbb {Z}^{6}\\ \hline (\mathfrak {B}(d_1), \mathfrak {B}_0 (d_1)) &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{18} &{} 0 \\ \hline (\mathfrak {B}_0(d_1), \partial \mathfrak {B}_0(d_1) ) &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{12} &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{12} &{} 0 \\ \hline \mathfrak {B}(d_1) &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{6} &{} \mathbb {Z}\oplus \mathbb {Z}_2^{5} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline \partial \mathfrak {B}(d_1) &{} \mathbb {Z}^{24} &{} 0 &{} 0 &{} \mathbb {Z}^{24} &{} 0 &{} 0 &{} 0 \\ \hline \partial \mathfrak {B}^+(d_1) &{} \mathbb {Z}^{12} &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{12} &{} 0 &{} \mathbb {Z}^{12} &{} 0 \\ \hline \mathfrak {B}_0(d_1) &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{12} &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{12} &{} 0 &{} 0 \\ \hline \partial \mathfrak {B}_0(d_1) &{} \mathbb {Z}^{24} &{} \mathbb {Z}^{24} &{} 0 &{} \mathbb {Z}^{24} &{} \mathbb {Z}^{24} &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

For the reduced spaces, the homology groups of $\mathfrak {B}_R(d_1)$, relevant subspaces and corresponding pairs of spaces are

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (\mathfrak {B}_R, \partial \mathfrak {B}_R ) &{} 0 &{}\mathbb {Z}^{23} &{} 0 &{} \mathbb {Z}^{17} &{} \mathbb {Z}^{6} &{} 0 &{} 0 \\ \hline (\mathfrak {B}_R, \partial \mathfrak {B}_R^+) &{} 0 &{}\mathbb {Z}^{11} &{} 0 &{}\mathbb {Z}^{17} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline (\mathfrak {B}_R, \mathfrak {B}_{R0} ) &{} 0 &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{18} &{} 0 &{} 0 \\ \hline (\mathfrak {B}_{R0}, \partial \mathfrak {B}_{R0} ) &{} 0 &{} \mathbb {Z}^{12} &{} 0 &{} 0 &{} \mathbb {Z}^{12} &{} 0 &{} 0 \\ \hline \mathfrak {B}_R &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} 0 \\ \hline \partial \mathfrak {B}_R &{} \mathbb {Z}^{24} &{} 0 &{} \mathbb {Z}^{24} &{} 0 &{} 0 &{} 0 &{} 0 \\ \hline \partial \mathfrak {B}_R^+ &{} \mathbb {Z}^{12} &{} 0 &{} \mathbb {Z}^{24} &{} 0 &{} \mathbb {Z}^{12}&{} 0 &{} 0 \\ \hline \mathfrak {B}_{R0} &{} \mathbb {Z}^{12} &{} 0 &{} 0 &{} \mathbb {Z}^{12} &{} 0 &{} 0 &{} 0 \\ \hline \partial \mathfrak {B}_{R0} &{} \mathbb {Z}^{24} &{} 0 &{} 0 &{} \mathbb {Z}^{24} &{} 0 &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

Almost all of the homology maps are uniquely determined by these groups. The only two that are not immediately obvious are that $\iota _{+*}:H_2(\partial \mathfrak {B}^+(d_1)) \rightarrow H_2(\mathfrak {B}(d_1))$ has cokernel $\mathbb {Z}_2^5$ and $\iota _{+*}: H_3(\partial \mathfrak {B}^+(d_1)) \rightarrow H_3(\mathfrak {B}(d_1))$ is surjective.

The strategy for computing $H_*(\mathfrak {B}(d_i), \partial \mathfrak {B}(d_i))$ and $H_*(\mathfrak {B}(d_i), \partial \mathfrak {B}^+(d_i))$ in Regions II - V will be to relate these unknown quantities for $\frac{1}{2c^2}< d_i < \frac{27}{2c^2}$ to the corresponding quantities for $0< d_1 < \frac{1}{2c^2}$ in Region I. To do so, consider the inter-woven exact sequences of the triples

$$\begin{aligned}\begin{array}{ccc} (\mathfrak {B}(d_1), \mathfrak {B}(d_1,d_i), \partial \mathfrak {B}(d_1)) &{} &{} (\mathfrak {B}(d_1), \mathfrak {B}^+(d_1,d_i), \partial \mathfrak {B}^+(d_1))\\ (\mathfrak {B}(d_1), \mathfrak {B}^+(d_1,d_i), \mathfrak {B}(d_1,d_i)) &{} &{} (\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1), \partial \mathfrak {B}(d_1))\\ \end{array}\end{aligned}$$

where $\mathfrak {B}(d_1, d_i) = D^{-1}([d_1, d_i])$ and $\mathfrak {B}^+(d_1,d_i) = \mathfrak {B}_0(d_1) \cup \partial \mathfrak {B}(d_1,d_i)$.

These are linked in a two-dimensional array of long exact sequences. The array consists of $3 \times 3$ blocks containing the $H_k$ entries and their inclusion-induced maps, with the $k+1$ block occurring both above and to the left of the k block, and the boundary maps linking the blocks both horizontally and vertically.

Note that the maps $\delta _+$ and $\partial _2$ in the lower left corner are the same as the maps $\delta _+$ and $\partial _2$ in the upper right corner. To simplify the presentation, we will display only the middle three rows, with the understanding that there are boundary maps that emanate from the bottom row and map to the top row, three entries to the right.

We observed in Lemma 3.5.1 that $\partial \mathfrak {B}(d_i) \rightarrow \mathfrak {B}(d_1, d_i)$ is a homotopy equivalence. The same argument shows that $\partial \mathfrak {B}^+(d_i) \rightarrow \mathfrak {B}^+(d_1, d_i)$ is likewise a homotopy equivalence. In addition to these equivalencies, there are a number of additional relationships between these sets:

• The homotopy equivalences imply $H_k(\mathfrak {B}(d_1), \mathfrak {B}(d_1, d_i)) \cong H_ k(\mathfrak {B}(d_i), \partial \mathfrak {B}(d_i))$ and $H_k(\mathfrak {B}(d_1), \mathfrak {B}^+(d_1,d_i)) \cong H_ k(\mathfrak {B}(d_i), \partial \mathfrak {B}^+(d_i))$

• By excision, $H_k(\partial \mathfrak {B}(d_1, d_i), \partial \mathfrak {B}(d_1)) \cong H_k(\partial \mathfrak {B}^+(d_1,d_i), \partial \mathfrak {B}^+(d_1))$

• On the other hand, from Lemma 3.5.1, $H_k(\partial \mathfrak {B}(d_1, d_i), \partial \mathfrak {B}(d_1)) \cong H_k(X(d_i), X(d_1))$.

• By excision, $H_k(\mathfrak {B}_0(d_1), \partial \mathfrak {B}_0(d_1)) \cong H_k(\mathfrak {B}^+(d_1), \partial \mathfrak {B}(d_1))$, while the homotopy equivalences together with excision yield $H_k(\mathfrak {B}_0(d_i), \partial \mathfrak {B}_0(d_i)) \cong H_k(\partial \mathfrak {B}^+(d_1,d_i), \partial \mathfrak {B}(d_1,d_i))$

• As part of Proposition 3.5.1, we have observed that $H_k(\mathfrak {B}_0(d_1), \partial \mathfrak {B}_0(d_1)) \cong H_k(\mathfrak {B}_0(d_i), \partial \mathfrak {B}_0(d_i))$

Inserting all of this information into the commutative diagram, we arrive at Table 2, which we refer to as the unreduced braid diagram. Note that the vertical exact sequences step over three columns at a time. A representative boundary map is illustrated.

Table 2 Braid Diagram for unreduced spaces

Note that the middle row of the braid, relating $H_*(\mathfrak {B}_0(d_1), \partial \mathfrak {B}_0(d_1))$, $H_*(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1))$ and $H_*(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1))$, is known, with the following groups and maps.

$$\begin{aligned}\begin{array}{ccccccc} k &{} H_k(\mathfrak {B}_0(d_1),\partial \mathfrak {B}_0(d_1)) &{} \rightarrow &{} H_k(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1) ) &{} \rightarrow &{} H_k(\mathfrak {B}(d_1),\partial \mathfrak {B}^+(d_1)) &{} \rightarrow \\ 6 &{} 0 &{} &{} 0 &{} \rightarrow &{} \mathbb {Z}^{6} &{} \rightarrowtail \\ 5 &{} \mathbb {Z}^{12} &{} \twoheadrightarrow &{} \mathbb {Z}^{6} &{} \rightarrow &{} 0 &{} \\ 4 &{} \mathbb {Z}^{12} &{} \rightarrowtail &{} \mathbb {Z}^{23} &{} \twoheadrightarrow &{} \mathbb {Z}^{11} &{} \rightarrow \\ 3 &{} 0 &{} &{} 0 &{} \rightarrow &{} \mathbb {Z}^6 &{} \rightarrowtail \\ 2 &{} \mathbb {Z}^{12} &{} \rightarrow &{} \mathbb {Z}^{6} &{} \twoheadrightarrow &{} \mathbb {Z}_2^5 &{} {\mathop {\rightarrow }\limits ^{0}} \\ 1 &{} \mathbb {Z}^{12} &{} \rightarrowtail &{} \mathbb {Z}^{23} &{} \twoheadrightarrow &{}\mathbb {Z}^{11} \\ 0 &{} 0 &{} &{} 0 &{} &{} 0 \\ \end{array}\end{aligned}$$

All of this applies to the reduced spaces as well, yielding a reduced braid diagram analogue of Table 2 with all of the spaces replaced by their quotients under the $S^1$ action. The corresponding long exact sequence for $H_*(\mathfrak {B}_{R0}(d_1), \partial \mathfrak {B}_{R0}(d_1))$, $H_*(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ and $H_*(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R^+(d_1))$ is

$$\begin{aligned}\begin{array}{ccccccc} k &{} H_k(\mathfrak {B}_{R0}(d_1),\partial \mathfrak {B}_{R0}(d_1)) &{} \rightarrow &{} H_k(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1) ) &{} \rightarrow &{} H_k(\mathfrak {B}_R(d_1),\partial \mathfrak {B}_R^+(d_1)) &{} \rightarrow \\ 6 &{} 0 &{} &{} 0 &{} &{} 0 &{} \\ 5 &{} 0 &{} &{} 0 &{} &{} \mathbb {Z}^{6} &{} \rightarrowtail \\ 4 &{} \mathbb {Z}^{12} &{} \twoheadrightarrow &{} \mathbb {Z}^{6} &{} &{} 0 &{} \\ 3 &{} 0 &{} &{} \mathbb {Z}^{17} &{} {\mathop {\rightarrow }\limits ^{\cong }} &{} \mathbb {Z}^{17} &{} \\ 2 &{} 0 &{} &{} 0 &{} &{} 0 &{} \\ 1 &{} \mathbb {Z}^{12} &{} \rightarrowtail &{} \mathbb {Z}^{23} &{} \twoheadrightarrow &{}\mathbb {Z}^{11} \\ 0 &{} 0 &{} &{} 0 &{} &{} 0 \\ \end{array}\end{aligned}$$

In order to fully leverage these “reduced” and “unreduced” diagrams, they can be connected via the Gysin sequence. If

$$\begin{aligned} \rightarrow H_k(A) \rightarrow H_k(B) \rightarrow H_k(C) \rightarrow \end{aligned}$$

is any long exact sequence of objects that admit an orientable $S^1$ action (e.g. either the homology groups of single spaces or pairs), then there is a braid diagram as shown in Table 3, with columns forming long exact sequences, linked by boundary maps that step three columns to the right.

Table 3 Gysin braid diagram

4.2 Region II

With all of this in place, we begin the step-by-step process of computing the homology groups for Region II. This will set the pattern that we will use for Regions III, IV and V as well: we first compute $H_*(X(d_2), X(d_1))$ and $H_*(X_R(d_2), X_R(d_1))$, then use those results to compute $H_*(\mathcal {B}(d_2), \partial \mathfrak {B}(d_2))$ and $H_*(\mathcal {B}_R(d_2), \partial \mathfrak {B}_R(d_2))$, then finally compute $H_*(\mathcal {B}(d_2), \partial \mathfrak {B}^+(d_2))$ and $H_*(\mathcal {B}(d_2), \partial \mathfrak {B}^+(d_2))$.

Proposition 4.2.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{1}{2c^2}< d_2 < \frac{4}{c^2}$, we have the following homology groups

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_2), X(d_1)) &{} 0 &{} \mathbb {Z}^{36} &{} \mathbb {Z}^{36} &{} 0 &{} 0 &{} 0 &{} 0\\ \hline (\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2) ) &{} 0 &{} 0 &{} \mathbb {Z}^{13} &{} \mathbb {Z}^{30} &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{6} &{} 0 \\ \hline (\mathfrak {B}(d_2),\partial \mathfrak {B}^+(d_2)) &{} 0 &{} 0 &{} \mathbb {Z}^{25} &{} \mathbb {Z}^{42} &{} \mathbb {Z}^{11} &{} 0 &{} \mathbb {Z}^{6}\\ \hline (X_R(d_2), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{36} &{} 0 &{} 0 &{} 0 &{} 0 &{} 0\\ \hline (\mathfrak {B}_R(d_2), \partial \mathfrak {B}_R(d_2) ) &{} 0 &{} 0 &{} \mathbb {Z}^{13} &{} \mathbb {Z}^{17} &{} \mathbb {Z}^{6} &{} 0 &{} 0 \\ \hline (\mathfrak {B}_R(d_2),\partial \mathfrak {B}_R^+(d_2)) &{} 0 &{} 0 &{} \mathbb {Z}^{25} &{} \mathbb {Z}^{17} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline \end{array}\end{aligned}$$

Proof

The computation of $H_*(X(d_2), X(d_1))$ is straightforward. From the discussion in Sect. 3.4, we know that $X(d_2)$ is formed by attaching 36 copies of $(-1, 1) \times S^1 \times D^2$, with each attaching to two copies of $D^2 \times S^3$. Each copy of $(-1, 1) \times S^1 \times D^2$ attaches to each copy of $D^2 \times S^3$ along the entirety of $(-1, 1) \times S^1 \times D^2$. By excision, $H_k(X(d_2), X(d_1)) \cong \bigoplus _{36} H_k(S^3 \cup _{S^1 \times D^2} S^3 , S^3 \sqcup S^3) \cong \oplus _{36} H_{k-1}(S^1)$. Similarly, $X_R(d_2)$ attaches 36 copies of $(-1, 1) \times D^2$, with each attaching to two copies of $D^2 \times S^2$ attaching to each along the entirety of $(-1, 1) \times D^2$, yielding $H_k(X_R(d_2), X_R(d_1)) \cong \oplus _{36} H_{k-1}(D^2)$.

To compute $H_*(\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2))$, the first step is to establish that the map $H_1(X_R(d_2), X_R(d_1)) \rightarrow H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$, which maps from $\mathbb {Z}^{36}$ to $\mathbb {Z}^{23}$, is surjective. To verify this, consider the mapping of pairs $(X_R(d_2), X_R(d_1)) \rightarrow (\mathfrak {B}_R(d_2), X_R(d_1))$. This generates commutative diagram

whose values are

This diagram shows that $H_1(X_R(d_2), X_R(d_1)) \rightarrow H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ is surjective.

With that as the starting point, we can work our way through the Gysin braid diagram of triple $(\mathfrak {B}(d_1), \mathfrak {B}(d_1, d_2),\mathfrak {B}(d_1))$ to obtain the values for $H_*(\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2))$ and $H_*(\mathfrak {B}_R(d_2), \partial \mathfrak {B}_R(d_2))$. For example, the surjectivity of $H_1(X_R(d_2), X_R(d_1)) \rightarrow H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ implies the surjectivity of $H_1(X(d_2), X(d_1)) \rightarrow H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1))$, which allows us to conclude that $H_2(\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2)) \rightarrow H_2(\mathfrak {B}_R(d_2), \partial \mathfrak {B}_R(d_2))$ is an isomorphism $\mathbb {Z}^{13} \rightarrow \mathbb {Z}^{13}$. In dimensions $k > 3$, the maps $H_k(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \rightarrow H_k(\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2))$ are flanked by zeros, and so are isomorphisms. In dimension 3, the Gysin braid diagram shows

When the known values are inserted, this yields

which produces the values for $H_3(\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2))$ and $H_3(\mathfrak {B}_R(d_2), \partial \mathfrak {B}_R(d_2))$.

Once $H_*(\mathfrak {B}(d_2), \partial \mathfrak {B}(d_2))$ and $H_*(\mathfrak {B}_R(d_2), \partial \mathfrak {B}_R(d_2))$ are computed, the values for $H_*(\mathfrak {B}_R(d_2), \partial \mathfrak {B}^+_R(d_2))$ follow immediately from the reduced braid for Region II. Finally, the Gysin braid of the triple $(\mathfrak {B}(d_1), \mathfrak {B}^+(d_1, d_2),\mathfrak {B}^+(d_1))$ provides the values for $H_*(\mathfrak {B}(d_2), \partial \mathfrak {B}^+(d_2))$ in the same manner. $\square$

4.3 Region III

The calculations for Region III follow along the same lines. Direct calculations of $H_*(X(d_3))$ and $H_*(X(d_3), X(d_1))$ and the corresponding reduced spaces are required, as are computations of the inclusion-induced maps $H_*(X_R(d_3), X_R(d_1)) \rightarrow H_*(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$. Once those calculations are carried out in Lemma 4.3.1, the same sequence of diagram-chasing yields the following results.

Proposition 4.3.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{4}{c^2}< d_3 < \frac{9}{c^2}$, we have the following homology groups

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_3), X(d_1)) &{} 0 &{} \mathbb {Z}^{30} &{} \mathbb {Z}^{24} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} 0\\ \hline (\mathfrak {B}(d_3),\partial \mathfrak {B}(d_3) ) &{} 0 &{} 0 &{} \mathbb {Z}^7 &{} \mathbb {Z}^{18} &{} \mathbb {Z}^{17} &{} \mathbb {Z}^{6} &{} 0 \\ \hline (\mathfrak {B}(d_3),\partial \mathfrak {B}^+(d_3)) &{} 0 &{} 0 &{} \mathbb {Z}^{19} &{} \mathbb {Z}^{30} &{} \mathbb {Z}^{5} &{} 0 &{} \mathbb {Z}^{6}\\ \hline (X_R(d_3), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{30} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} 0 &{} 0\\ \hline (\mathfrak {B}_R(d_3), \partial \mathfrak {B}_R(d_3) ) &{} 0 &{} 0 &{} \mathbb {Z}^{7} &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{6} &{} 0 &{} 0 \\ \hline (\mathfrak {B}_R(d_3),\partial \mathfrak {B}_R^+(d_3)) &{} 0 &{} 0 &{} \mathbb {Z}^{19} &{} \mathbb {Z}^{11} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline \end{array}\end{aligned}$$

Proof

The arguments follow the same pattern as for Region II: inserting the known values, including the information provided in Lemma 4.3.1, into the Gysin braid of the triple $(\mathfrak {B}(d_1), \mathfrak {B}(d_1, d_3), \mathfrak {B}(d_1))$ provides the values for $H_*(\mathfrak {B}(d_3), \partial \mathfrak {B}(d_3))$ and $H_*(\mathfrak {B}_R(d_3), \partial \mathfrak {B}_R(d_3))$. Next, the reduced braid diagram is used to compute $H_*(\mathfrak {B}_R(d_3), \partial \mathfrak {B}_R^+(d_3))$, and finally, the Gysin braid of the triple $(\mathfrak {B}(d_1), \mathfrak {B}(d_1, d_3), \mathfrak {B}(d_1))$ computes $H_*(\mathfrak {B}(d_3), \partial \mathfrak {B}^+(d_3))$ $\square$

We focus then on the computations for $(X(d_3), X(d_1))$.

Lemma 4.3.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{4}{c^2}< d_3 < \frac{9}{c^2}$, the homology groups of $X(d_3)$ and $(X(d_3), X(d_1))$ and their reduced spaces are

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_3), X(d_1)) &{} 0 &{} \mathbb {Z}^{30} &{} \mathbb {Z}^{24} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} 0\\ \hline X(d_3) &{} \mathbb {Z} &{} \mathbb {Z}^7 &{} \mathbb {Z}^{24} &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 \\ \hline (X_R(d_3), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{30} &{} 0 &{} \mathbb {Z}^{6} &{} 0 &{} 0 &{} 0 \\ \hline X_R(d_3) &{} \mathbb {Z} &{} \mathbb {Z}^7 &{} \mathbb {Z}^{18} &{} 0 &{} 0 &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

The inclusion-induced map $H_1(X_R(d_3), X_R(d_1)) \rightarrow H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ is surjective and $H_3(X_R(d_3), X_R(d_1)) \rightarrow H_3(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ is injective.

Proof

From Lemma 3.4.3, we can observe that $X(d_3)$ is built from $X(d_1) \cong \bigsqcup _{24} D^2 \times S^3$ via the following attachments:

• In $\Delta _L$, there are twelve open intervals $\Delta _{ijL}^o$ that connect two triple collision points $\Delta _{ijk}$ and $\Delta _{ijl}$. Along each of the open intervals, a set of the form $(-1, 1) \times (\bar{D}^2 \times S^1)$ is attached.

• In $\Delta _L$, each of the six double binary collision point $q_0 = \Delta _{ij,kl}$ has four open intervals that emanate from it: two in $\Delta _{ijL}^o$ and two in $\Delta _{klL}^o$, while over $q_0$ itself, a set of the form $T^2 \times \mathbb {I}$ is added.

For each of these eighteen components of $X(d_3)\setminus X(d_1)$, take the projection onto $C_L$ and take disjoint neighborhoods in $C_L\setminus \bigsqcup \Delta _{ijk}$ around each, with each neighborhood homeomorphic to $D^2$. Finally, take the pre-images in $\mathcal {X}^1(\mathcal {C}_L)$ and denote the intersection with $(X(d_3), X(d_1))$ by $(Z_3, Z_1)$. The components of $(Z_3, Z_1)$ are

• Twelve copies of

  $$\begin{aligned}(A_3, A_1) = (-1,1) \times \left( (-1,1) \times S^3 \setminus (\{0\} \times (D^2 \times S^1)), ((-1,1) \setminus \{0\}) \times S^3 \right) \end{aligned}$$

• Six copies of

  $$\begin{aligned}&(B_3, B_1) = \left( (-1, 1)^2 S^3 \setminus (((-1,1) \times \{0\} \times D^2 \times S^1) \cup ((\{0\} \times (-1,1) \times D^2 \times S^1), \right. \\&\left. ((-1,1)\setminus \{0\})\times ((-1,1)\setminus \{0\}) \times S^3 \right) \end{aligned}$$

That is, each of these components of $Z_3$ is formed from $(-1,1)^2 \times S^3$ by deleting thickened tubes $(-1, 1) \times D^2 \times S^1$, one along each of the axes of $(-1, 1)^2$. The two tubes both pass through $S^3 \times \{(0,0)\}$. They do not intersect there, and their complement in $S^3$ is homeomorphic to $T^2 \times \mathbb {I}$.

Figure 3 illustrates the constructions. Figure 3a illustrates the structure of $( B_{3R}, B_{1R})$, with the lines indicating the deleted tubes. Figure 3b suggests the projections of the pairs onto $\mathcal {C}_L$. The components of $(A_3, A_1)$ actually run the full length of the open intervals $\Delta _{ij}^o$, and the sets $A_1$ and $B_1$ are not bounded away from $\Delta$. However, the subsets indicated are homotopy-equivalent to the actual sets.

Fig. 3

The pairs $(A_3, A_1)$ and $(B_3, B_1)$

By excision, $H_*(Z_3, Z_1) \cong H_*(X(d_3), X(d_1))$. It is straightforward that $H_*(A_3, A_1) \cong H_*(\mathbb {I}, S^0) \otimes H_*(S^1)$. To compute $H_*(B_3, B_1)$, let $B_{31}$ and $B_{32}$ be the two sets formed from $(-1,1)^2 \times S^3$ when each of the thickened tubes are removed one at a time, and let $B_{11}$, $B_{12}$ be the corresponding intersections with $Z_1$. Each has $H_*(B_{3i}, B_{1i}) \cong H_*(S^3 \cup _{D^2 \times S^1} \cup S^3, S^3 \sqcup S^3).$ The intersection $(B_{31} \cap B_{32}, B_{11} \cap B_{12}) = (B_3, B_1)$, while the union $(B_{31} \cup B_{32}, B_{11} \cup B_{12}) \cong ( (-1,1)^2 \times S^3, ((-1,1)^2\setminus \{(0,0)\}) \times S^3)$. All of this construction is invariant under the $S^1$ rotation on $S^3$, so comparable reduced spaces can be formed. The Gysin braid diagram of the Mayer-Vietoris sequence for $(B_{31} , B_{11})$ and $(B_{32}, B_{12})$ shows that

$$\begin{aligned}\begin{array}{|c||c|c|c|c|c|c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline H_k(B_3, B_1) &{} 0 &{} \mathbb {Z}^3 &{} \mathbb {Z}^2 &{} 0 &{} \mathbb {Z} &{} 0 &{} 0 \\ \hline H_k(B_{R3}, B_{R1}) &{} 0 &{} \mathbb {Z}^3 &{} 0 &{} \mathbb {Z} &{} 0 &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

Note that $Z_1 \simeq \bigsqcup _{48} S^3$ and $Z_{R1} \simeq \bigsqcup _{48} S^2$ have the obvious 2 : 1 mapping to $X_1 \simeq \bigsqcup _{24} S^3$ and $X_{R1} \simeq \bigsqcup _{24} S^2$. The values for $X(d_3)$ and $X_R(d_3)$ follow from the Gysin braid of the exact sequence of the pair $(X(d_3), X(d_1))$.

Finally, in the commutative diagram of the pairs $(X_R(d_3), X_R(d_1)) \rightarrow (\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$, we see

and

from which the injectivityof $\iota _{3*}$ and surjectivity of $\iota _{1*}$ follow. $\square$

4.4 Region IV

As d passes from Region III to Region IV, the only changes are at the eight points of the form $\Delta _{ijk}$, where sets of the form $S^0 \times D^2 \times S^1$ emerge. As d passes from Region IV to Region V, the changes are likewise at these eight points, where the sets coalesce to form $S^3 \setminus \bigsqcup _3 (D^3 \times S^1)$. As with Regions II and III, we we will focus on the pairs $(X(d_4), X(d_1))$ and $(X_R(d_4), X_R(d_1))$ and derive all of the other values from there. In this instance, accessing the information on $(X(d_4), X(d_1))$ will be obtained by first studying the pair $(X(d_4), X(d_3)$.

Proposition 4.4.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{9}{c^2}< d_4 < \frac{25}{2c^2}$, we have the following homology groups

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_4), X(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{26} &{} \mathbb {Z}^{9} &{} \mathbb {Z}^{6} &{} 0 &{} 0\\ \hline (\mathfrak {B}(d_4),\partial \mathfrak {B}(d_4) ) &{} 0 &{} 0 &{} 0 &{} \mathbb {Z}^{20} &{} \mathbb {Z}^{26} &{} \mathbb {Z}^{6} &{} 0 \\ \hline (\mathfrak {B}(d_4),\partial \mathfrak {B}^+(d_4)) &{} 0 &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{32} &{} \mathbb {Z}^{14} &{} 0 &{} \mathbb {Z}^{6}\\ \hline (X_R(d_4), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{9} &{} \mathbb {Z}^{6} &{} 0 &{} 0 &{} 0\\ \hline (\mathfrak {B}_R(d_4), \partial \mathfrak {B}_R(d_4) ) &{} 0 &{} 0 &{} 0 &{} \mathbb {Z}^{20} &{} \mathbb {Z}^{6} &{} 0 &{} 0 \\ \hline (\mathfrak {B}_R(d_4),\partial \mathfrak {B}_R^+(d_4)) &{} 0 &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{20} &{} 0 &{} \mathbb {Z}^{6} &{} 0 \\ \hline \end{array}\end{aligned}$$

Lemma 4.4.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{9}{c^2}< d_4 < \frac{25}{2c^2}$, the homology groups of $X(d_4)$ and $(X(d_4), X(d_1))$ and their reduced spaces are

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_4), X(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{26} &{} \mathbb {Z}^{9} &{} \mathbb {Z}^{6} &{} 0 &{} 0\\ \hline X(d_4) &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{26} &{} \mathbb {Z}^{27} &{} 0 &{} 0 &{} 0 \\ \hline (X_R(d_4), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^{9} &{} \mathbb {Z}^{6} &{} 0 &{} 0 &{} 0 \\ \hline X_R(d_4) &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{27} &{} 0 &{} 0 &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

The inclusion-induced maps from $H_*(X(d_4), X(d_1))$ and $H_*(X_R(d_4), X_R(d_1))$ have the following properties:

• The maps

  $$\begin{aligned}\begin{array}{ccccc} H_2(X(d_4), X(d_1)) &{} \rightarrow &{} H_2(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) &{} \rightarrow &{} H_2(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1)) \\ H_1(X(d_4), X(d_1)) &{} \rightarrow &{} H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) &{} \rightarrow &{} H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1)) \\ H_1(X_R(d_4), X_R(d_1)) &{} \rightarrow &{} H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1)) &{} \rightarrow &{} H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R^+(d_1)) \\ \end{array}\end{aligned}$$

  are all surjective

• The maps

  $$\begin{aligned} H_3(X(d_4), X(d_1)) \rightarrow H_3(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \rightarrow H_3(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1))\end{aligned}$$

  are trivial.

• The maps

  $$\begin{aligned}\begin{array}{c} H_4(X(d_4), X(d_1)) \rightarrow H_4(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \\ H_4(X(d_4), X(d_1)) \rightarrow H_4(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1)) \\ H_3(X_R(d_4), X_R(d_1)) \rightarrow H_3(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1)){\mathop {\rightarrow }\limits ^{\cong }} H_3(\mathfrak {B}_R(d_1), \partial \mathfrak {B}^+_R(d_1)) \end{array}\end{aligned}$$

  are all injective with torsion-free cokernels.

Proof

We develop the results for $X(d_4)$ and $(X(d_4), X(d_1))$ by leveraging the relationship between $X(d_4)$ and $X(d_3)$, as they differ only at the eight triple collision points in $\Delta _L$. Take a disk $U_q$ in $\mathcal {C}_L$ around each of eight triple collision points q in $\Delta _L$. Over each, form $N_{3q} = (U_q \times S^3) \cap X(d_3)$ and $N_{4q} = (U_q \times S^3) \cap X(d_4)$, and let $(N_4, N_3) = \bigsqcup _8 (N_{4q}, N_{3q}).$ By excision, $H_k(X(d_4),X(d_3)) \cong H_k( N_4, N_3) = \bigoplus _8 H_k( N_{4q}, N_{3q}).$ As $X(d_3)$ and $X(d_4)$ are homotopic outside of $N_4$, excision also yields $H_*(X(d_4), N_4) \cong H_*(X(d_3), N_3).$

The two sets $N_{3q}$ and $N_{4q}$ differ only over q itself. Traversing the boundary of the disk, $N_{3q}$ intersects six points in $\Delta _L$ (two each in $\Delta _{ij}^o$, $\Delta _{ik}^o$ and $\Delta _{jk}^o$). At each of these points, the pre-image in $N_{4q}$ and $N_{3q}$ has the form $\bar{D}^2 \times S^1$, while on the six intervals created by the intersection with the binary collisions, the pre-image in both is all of $S^3$. Over the collision point q itself, the pre-image in $N_{4q}$ is homeomorphic to $D^2 \times S^1 \times S^0$, while the pre-image in $N_{3q}$ is empty. The alternating pattern of $S^3$ and $D^2 \times S^1$ yields $H_*(N_{3q}) \cong (\mathbb {Z}, \mathbb {Z}, \mathbb {Z}^6, \mathbb {Z}^6, 0, \ldots )$.

$N_{4q}$ is formed from $N_{3q}$ by attaching two copies of $D^2 \times D^2 \times S^1$ along $S^1 \times D^2 \times S^1$, so $H_*(N_{4q}, N_{3q}) \cong \bigoplus _2 H_*(D^2, S^1) \otimes H(S^1)$. The exact sequence of the pair $(N_{4q}, N_{3q})$ is

$$\begin{aligned}0 \rightarrow \mathbb {Z}^{6} \rightarrow H_3(N_{4q}) \rightarrow \mathbb {Z}^{2} \rightarrow \mathbb {Z}^{6} \rightarrow H_2(N_{4q}) \rightarrow \mathbb {Z}^{2} \rightarrow \mathbb {Z}^{1} \rightarrow H_1(N_{4q}) \rightarrow 0 \end{aligned}$$

In dimension $k = 1$, the map $\mathbb {Z}^4 \rightarrow \mathbb {Z}^3$ is surjective, so $H_1(N_4) = 0$. In dimension $k = 2$, each of the generators of $\mathbb {Z}^2$ maps to the sum of the generators of $\mathbb {Z}^6$, so the map has rank 1 with torsion-free cokernel. This implies $H_k(N_{4q}) = (\mathbb {Z}, 0, \mathbb {Z}^6, \mathbb {Z}^7, 0, \ldots )$.

The corresponding reduced spaces follow parallel arguments: Each $N_{R3q}$ is homotopic to a bouquet of six 2-spheres attached to a circle, and each $N_{R4q}$ is formed by attaching two copies of $\bar{D}^4$ along disjoint copies of $\bar{D}^2 \times S^1$. This yields $H_*(N_{R3q}) \cong ( \mathbb {Z}, \mathbb {Z}, \mathbb {Z}^6, 0, \ldots )$, $H_*(N_{R4q}, N_{R3q}) \cong \bigoplus _2 H_*(D^2, S^1)$ and $H_*(N_{R4q}) \cong (\mathbb {Z}, 0, \mathbb {Z}^7, 0, \ldots )$.

We next show that $\iota :H_*(N_3) \rightarrow H_*(X(d_3))$ and $\iota _R:H_*(N_{R3}) \rightarrow H_*(X_R(d_3))$ are surjective. Take $N_1 = N_3 \cap X(d_1)$ and $N_{R1} = N_{R3} \cap X_R(d_1)$. Each $N_{1q}$ (resp. $N_{R1q}$) consists of six copies $S^3$ (resp. $S^2$), so $H_3(N_1) \cong H_2(N_{R1}) \cong \mathbb {Z}^{48}$, with obvious 2 : 1 mappings to $H_3(X(d_1))$ and $H_2(X_{R}(d_1))$. We also observe that $H_3(N_1) \rightarrow H_3(N_3)$ and $H_2(N_{R1}) \rightarrow H_2(X_{R3})$ are isomorphisms. Since $H_3(X(d_3), X(d_1)) = H_2(X_R(d_3), X_R(d_1)) = 0$, the maps $H_3(X(d_1)) \rightarrow H_3(X(d_3))$ and $H_2(X_R(d_1)) \rightarrow H_2(X_R(d_3))$ are surjective, so $H_3(N_3) \rightarrow H_3(X(d_3))$ and $H_2(N_{R3}) \rightarrow H_2(X_R(d_3))$ are surjective.

$H_1(N_3) \cong H_1(N_{R3}) \cong \mathbb {Z}^8$ are both generated by circles in $\mathcal {C}_L$ around the eight triple collision points. Similarly,

$$\begin{aligned}H_1(X(d_3)) \cong H_1(X_{R}(d_3)) \cong H_1(\mathcal {C}_L \setminus \bigsqcup \Delta _{ijk}) \cong H_1(S^2 \setminus \{8 pts.\}) \cong \mathbb {Z}^7,\end{aligned}$$

with each of these circles essential, but the sum adding to zero. That is, both $H_1(N_3) \rightarrow H_1(X(d_3))$ and $H_1(N_{R3}) \rightarrow H_1(X_R(d_3))$ are surjective.

Finally, $H_2(N_3) \cong H_2(N_3, N_1) \cong \bigoplus _{8} H_2(N_{3q}, N_{1q}) \cong \mathbb {Z}^{48}$ has six generators associated with each triple collision point q. More precisely, there is one generator associated with each direction of approach to q along $\Delta _{ij}^o$. From the proof of Lemma 4.3.1, $H_2(X(d_3)) \cong H_2(X(d_3), X(d_1)) \cong \mathbb {Z}^{24}$ has one generator associated with each of the 12 pairs of the form $(A_3, A_1)$ and two generators associated with each of the six pairs of the form $(B_3, B_1)$.

Fig. 4

Mapping of $(N_{3},N_1)$ to $(A_3, A_1)$ and $(B_3, B_1)$

As Fig. 4 illustrates, each pair $(A_3, A_1)$ has two sets $(N_{3q}, N_{1q})$ that map to it, while each pair $(B_3, B_1)$ has four sets $(N_{3q}, N_{1q})$. The maps from $H_2(N_{3q}, N_{1q})$ to $H_2(A_3, A_1)$ clearly take generator to generator. Examining the inclusion into sets of the form $(B_3, B_1)$ more closely, the generators of each $H_2(B_{3t}, B_{1t})$ correspond to the generators of $H_2(B_{31b}, B_{11b})$ and $H_2(B_{32t}, B_{12t})$. Each direction of approach from a triple collision point q to a double binary point b maps the corresponding generator of $H_2(N_{3q}, N_{1q})$ onto the appropriately-aligned generator $H_2(B_{3ib}, B_{1ib})$. Each $H_2(B_{3ib}, B_{1ib})$ has two directions of approach for which the corresponding generator of $H_2(N_{3q}, N_{1q})$ maps onto it, so every generator of $H_*(X(d_3), X(d_1))$ has two generators of $H_*(N_3, N_1)$ that map onto it.

With surjectivity established, we have the following homology group values

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline H_k(X(d_4), N_4) \cong H_*(X(d_3), N_3)&{} 0 &{} \mathbb {Z}^{7} &{} \mathbb {Z} &{} \mathbb {Z}^{24} &{} \mathbb {Z}^{30} &{} 0 &{} 0\\ \hline H_k(X_R(d_4), N_{R4}) \cong H_*(X_R(d_3), N_{R3}) &{} 0 &{} \mathbb {Z}^{7} &{} \mathbb {Z} &{} \mathbb {Z}^{30} &{} 0 &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

The sets $N_{4}$ and $X(d_3)$ are (up to homotopy equivalence) a decomposition of $X(d_4)$ with intersection $N_{3}$. Inserting the known values for the groups $H_*(N_3)$, $H_*(N_4)$, $H_*(X(d_3))$ and the maps $H_*(N_3) \rightarrow H_*(X(d_3))$ and $H_*(N_4) \rightarrow H_*(X(d_4))$ (and the corresponding reduced spaces) into the Gysin braid of the Mayer-Vietoris sequence, we obtain the values for $H_*(X(d_4))$ and $H_*(X_R(d_4))$.

There is then a braid of reduced spaces that intertwines the exact sequences of the pairs $(X_R(d_4), X_R(d_3))$, $(X_R(d_4), X_R(d_1))$, $(X_R(d_3), X_R(d_1))$ and the exact sequence of the triple $(X_R(d_4), X_R(d_3)), X_R(d_1))$. Inserting the known values into this braid diagram yields the values for $H_*(X_R(d_4), X_R(d_1))$. The Gysin braid of the pair $(X_4, X_1)$ provides the values for $H_*(X(d_4), X(d_1))$.

Turning to the inclusion-induced maps $H_*(X(d_4), X(d_1)) \rightarrow H_*(\mathfrak {B}(d_4), \partial \mathfrak {B}(d_1))$ and $H_*(X(d_4), X(d_1)) \rightarrow H_*(\mathfrak {B}(d_4), \partial \mathfrak {B}^+(d_1))$, we leverage a variety of existing results. First, from Sect. 4.1, we observe that $H_k(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \rightarrow H_k(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1))$ and $H_k(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \rightarrow H_k(\mathfrak {B}_R(d_1), \partial \mathfrak {B}(d_1))$ are surjective for $k = 1, 2$, that $H_3(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) = 0$, and that $H_3(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \rightarrow H_3(\mathfrak {B}_R(d_1), \partial \mathfrak {B}(d_1))$ is an isomorphism. Next, note that the comparable maps from $(X(d_3), X(d_1))$ and $(X_R(d_3), X_R(d_1))$ factor through $(X(d_4), X(d_1))$ and $(X_R(d_4), X_R(d_1))$. Moreover, from the triples $(X(d_4), X(d_3), X(d_1))$ and $(X_R(d_4), X_R(d_3), X_R(d_1))$, we observe that $H_k(X(d_3), X(d_1)) \rightarrow H_k(X(d_4), X(d_1))$ is surjective for $k=1$, injective for $k = 4$. Similarly, $H_k(X_R(d_3), X_R(d_1)) \rightarrow H_k(X_R(d_4), X_R(d_1))$ is surjective for $k=1$ and injective for $k = 3$.

The values in Lemma  show that $H_3(X_R(d_3), X_R(d_1)) \rightarrow H_3(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ and $H_4(X(d_3), X(d_1)) \rightarrow H_3(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1))$ are injective with torsion-free kernels, and that $H_1(X_R(d_3), X_R(d_1)) \rightarrow H_1(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1))$ and $H_1(X(d_3), X(d_1)) \rightarrow H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1))$ are surjective. Combining this with the information above, we know (for example) that the composition

$$\begin{aligned}\begin{array}{rcl}H_1(X(d_3), X(d_1)) \twoheadrightarrow H_1(X(d_4), X(d_1)) &{} \rightarrow &{} H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1)) \\ &{} \twoheadrightarrow &{} H_1(X(d_4), X(d_1)) \rightarrow H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1)) \end{array}\end{aligned}$$

is surjective, which implies that the maps from $H_1(X(d_4), X(d_1))$ to both $H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1))$ and $H_1(\mathfrak {B}(d_1), \partial \mathfrak {B}^+(d_1))$ are surjective. This approach establishes all of the assertions about the inclusion-induced maps except for $H_2(X(d_4), X(d_1)) \rightarrow H_2(\mathfrak {B}(d_1), \partial \mathfrak {B}(d_1))$. The surjectivity of this map follows from the Gysin braid diagram of the triple $(\mathfrak {B}(d_1), \mathfrak {B}(d_1, d_4), \mathfrak {B}(d_1))$. $\square$

4.5 Region V

Finally, we turn to region V. As discussed above, while the results are valid for $\frac{25}{2c^2} \le d_5 \le \frac{36 + 16\sqrt{2}}{c^2}$, for convenience, we will focus our analysis on the range $\frac{25}{2c^2} \le d_5 \le \frac{27}{2c^2}$.

Proposition 4.5.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{25}{2c^2}< d_5 < \frac{27}{2c^2}$, we have the following homology groups

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_5), X(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^7 &{} \mathbb {Z}^{6}&{} \mathbb {Z}^{22} &{} 0 &{} 0\\ \hline (\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5)) &{} 0 &{} 0 &{} 0 &{} \mathbb {Z} &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{11} &{} 0 \\ \hline (\mathfrak {B}(d_5), \partial \mathfrak {B}^+(d_5)) &{} 0 &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z}^{13} &{} \mathbb {Z}^{6} &{} \mathbb {Z}^{11} &{} \mathbb {Z}^{6} \\ \hline (X_R(d_5), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^1 &{} \mathbb {Z}^{22}&{} 0 &{} 0 &{} 0\\ \hline (\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R(d_5)) &{} 0 &{} 0 &{} 0 &{} \mathbb {Z} &{} \mathbb {Z}^{11} &{} 0 &{} 0 \\ \hline (\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R^+(d_5)) &{} 0 &{} 0 &{} \mathbb {Z}^{12} &{} \mathbb {Z} &{} \mathbb {Z}^{5} &{} \mathbb {Z}^{6} &{} 0 \\ \hline \end{array}\end{aligned}$$

As with the previous regions, we first compute $H_*(X(d_5))$ and $H_5(X_R(d_5))$, then $H_*(\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5))$ and $H_*(\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R(d_5))$, then finally $H_*(\mathfrak {B}(d_5), \partial \mathfrak {B}^+(d_5))$ and $H_*(\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R^+(d_5))$.

Lemma 4.5.1

For $0< d_1 < \frac{1}{2c^2}$ and $\frac{25}{2c^2}< d_5 < \frac{27}{2c^2}$, the homology groups of $X(d_5)$ and $(X(d_5), X(d_1))$ and their reduced spaces are

$$\begin{aligned}\begin{array}{|c|| c| c| c| c| c| c|c|} \hline k &{} 0 &{} 1 &{} 2 &{} 3 &{} 4 &{} 5 &{} 6 \\ \hline (X(d_5), X(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^7 &{} \mathbb {Z}^{6}&{} \mathbb {Z}^{22} &{} 0 &{} 0\\ \hline X(d_5) &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{7} &{} \mathbb {Z}^{13} &{} \mathbb {Z}^{5} &{} 0 &{} 0 \\ \hline (X_R(d_5), X_R(d_1)) &{} 0 &{} \mathbb {Z}^{23} &{} \mathbb {Z}^1 &{} \mathbb {Z}^{22}&{} 0 &{} 0 &{} 0\\ \hline X_R(d_5) &{} \mathbb {Z} &{} 0 &{} \mathbb {Z}^{8} &{} \mathbb {Z}^{5} &{} 0 &{} 0 &{} 0 \\ \hline \end{array}\end{aligned}$$

Proof

We combine the proofs of the lemma and proposition, beginning with the computation of $H_*(X_R(d_5))$

To compute $H_*(X_{R}(d_5))$, we draw on the characterization of Lemma 3.4.3 that $\mathcal {X}(\mathcal {C}_L)$ can be viewed as $S^2 \times S^2$ with six disjoint solid tori $S^1 \times D^2$ deleted. For each, the $S^1$ component corresponds to $\Delta _{ijL} \subset \mathcal {C}_L$, while the $D^2$ component corresponds to the disk around $\Delta _{ij}$ in $\mathcal {X}(q)$ for $q\in \Delta _{ij}$. If we denote the closure of the complement by $Y_R(d_5)$, then the pair $(Y_R(d_5), \partial Y_R(d_5)) \cong \bigsqcup _6 ( \mathbb {I} \times D^2 \times S^1, \partial \mathbb {I} \times D^2) \times S^1) \cong \bigsqcup _6 S^1 \times (D^3, S^2)$.

If we consider the braid diagram of the pairs $(\mathcal {X}_R(\mathcal {C}_L), X_{R}(d_5))$ and $(\mathcal {X}_R(\mathcal {C}_L), Y_{R}(d_5))$, we see that $H_*(\mathcal {X}_R(\mathcal {C}_L), X_{R}(d_5)) \cong H_*(Y_R(d_5), \partial Y_R(d_5)) \cong \bigoplus _6 H_*(S^1) \otimes H_*(D^3, S^2)$, while $H_*(X_R(d_5), \partial Y_R(d_5)) \cong H_*(\mathcal {X}_R(\mathcal {C}_L), Y_{R}(d_5)) \cong H_*(S^2 \times S^2, \bigsqcup _6 S^1)$. From the braid diagram, we can immediately conclude that $H_k(X_R(d_5)) = 0$ for $k \ne 0, 2, 3$. In dimensions 2 and 3, the exact sequence of the pair $(\mathcal {X}_R(\mathcal {C}_L), X_{R}(d_5))$ yields

$$\begin{aligned}0 \rightarrow \mathbb {Z} \rightarrow \mathbb {Z}^6 \rightarrow H_3(X_R(d_5)) \rightarrow 0 \rightarrow \mathbb {Z}^6 \rightarrow H_2(X_R(d_5)) \rightarrow \mathbb {Z}^2 \rightarrow 0\end{aligned}$$

from which the values for $H_*(X_R(d_5))$ follow.

To compute $H_*(X(d_5))$, we first exploit the corresponding construction in $\mathcal {X}(\mathcal {C}_L)$. That is, $\mathcal {X}(\mathcal {C}_L) \cong S^2 \times S^3$, while $H_*(\mathcal {X}(\mathcal {C}_L), X(d_5)) \cong H_*(Y(d_5), \partial Y(d_5)) \cong \bigoplus _6 H_*(T^2) \otimes H_*(D^3, S^2)$. From the exact sequence of the pair $(\mathcal {X}(\mathcal {C}_L), X(d_5))$, we see that $H_1(X(d_5)) = 0$. All of the other values of $H_*(X(d_5))$ then follow immediately from the Gysin sequence for $X(d_5)$.

The next step is to compute $H_*(X(d_5), X(d_1))$ and $H_*(X_R(d_5), X_R(d_1))$ from the Gysin braid diagram of the pair $(X(d_5), X(d_1))$. This diagram is completely determined by the map $\iota _R:H_2(X_R(d_1)) \rightarrow H_2(X_R(d_5))$. We will show that $\iota _R$ has rank 7 and torsion-free cokernel. It will also be useful to note that the same argument will show that $H_2(X_R(d_5)) \rightarrow H_2(\mathfrak {B}_R(d_5))$ is surjective, which will be the key piece of information needed to compute $H_*(\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5))$ and $H_*(\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R(d_5))$.

To establish both of these, consider $H_2(X_R(d_5)) \rightarrow H_2(\mathfrak {B}_R(d_5))$. We have seen that $H_2(\mathfrak {B}_R(d_1), \partial \mathfrak {B}_R(d_1)) = 0$, so $H_2(X_R(d_1)) \rightarrow H_2(\mathfrak {B}_R(d_1)) \cong \mathbb {Z}^7$ is surjective. Since $H_2(X_R(d_1)) \rightarrow H_2(\mathfrak {B}_R(d_1))$ factors as $H_2(X_R(d_1)) \rightarrow H_2(X_R(d_5)) \rightarrow H_2(\mathfrak {B}_R(d_5))$, it follows that $H_2(X_R(d_5)) \rightarrow H_2(\mathfrak {B}_R(d_5))$ is surjective and that $H_2(X_R(d_1)) \rightarrow H_2(X_R(d_5))$, which maps from $\mathbb {Z}^{24}$ to $\mathbb {Z}^8$, has rank of at least 7.

To see that $H_2(X_R(d_1)) \rightarrow H_2(X_R(d_5))$ has rank 7 and cokernel $\mathbb {Z}$, we revisit the construction in Sect.  of the neighborhoods $U_{q}$ in $\mathcal {C}_L$ around the eight triple collision points in $\Delta _L$. The sets $N_{5q} = X(d_5) \cap (U_q \times S^3)$ can be viewed as a subset of $D^2 \times S^2$, formed by deleting three disjoint tubes $(\Delta _{ij} \cap D^2) \times D_{ij}^2$, with $D_{ij}^2$ a neighborhood around $\Delta _{ij}$ in $\mathcal {X}_R(q)$. That is, $N_{R5q}$ has the homotopy type of $\mathbb {I} \times S^2$ with $\{0\} \times \bigsqcup _{3} D_{ij}^2$ deleted, which has homology groups $H_*(N_{R5q}) \cong (\mathbb {Z}, 0, \mathbb {Z}^4, 0, 0, \ldots )$ with one generator of $H_2(N_{R5q})$ corresponding to a neighborhood of each of the deletions in $\mathcal {X}_R(q)$, and the fourth generator corresponding to $\mathcal {X}_R(p)$ for any point in $U_q \setminus \Delta _L$. In particular, $H_1(N_{R5}) = 0$.

Now, consider the commutative diagram of the mapping of pairs $(X_R(d_1), N_{R1}) \rightarrow (X_R(d_5), N_{R5})$. We noted in Sect.  that $H_*(X_R(d_4), N_{R4}) \cong H_*(X_R(d_3), N_{R3})$. By exactly the same argument, $H_*(X_R(d_5), N_{R5}) \cong H_*(X_R(d_3), N_{R3})$, and in particular, $H_2(X_R(d_4), N_{R4}) \cong H_2(X_R(d_3), N_{R3}) \cong \mathbb {Z}$. On the other hand, $X_R(d_1) \cong \bigsqcup _{24} D^2 \times S^2$, while $N_{R1} \cong \bigsqcup _{48} D^2 \times S^2$, with each component of $X_{R}(d_1)$ containing two components of $N_{R1}$. That is, $H_2(X_{R}(d_1), N_{R1}) = 0$. Finally, noting that $H_1(N_{R5}) = 0$, the commutative diagram of the mapping of pairs $(X_R(d_1), N_{R1}) \rightarrow (X_R(d_5), N_{R5})$ yields

That is, $\iota _R$ must map into the kernel of $H_2(X_R(d_5)) \cong \mathbb {Z}^8 \twoheadrightarrow \mathbb {Z}$, so $\iota _R$ has rank at most 7. The maps $H_2(N_{R1}) \rightarrow H_2(N_{R4})$ and $H_2(N_{R4}) \rightarrow H_2(N_{R5})$ have torsion-free cokernels, so their composition $H_2(N_{R1}) \rightarrow H_2(N_{R5})$ and $H_2(N_{R1}) \rightarrow H_2(X_{R}(d_5))$ likewise have torsion-free cokernel. The surjectivity of $H_2(N_{R1}) \rightarrow H_2(X_{R}(d_1))$ then implies that $H_2(X_{R}(d_1)) \rightarrow H_2(X_{R}(d_5))$ has torsion-free cokernel.

With the behavior of $\iota _R$ established inserting the known values into the Gysin braid of $(X(d_5), X(d_1))$ determines both $H_*(X(d_5), X(d_1))$ and $H_*(X_R(d_5), X_R(d_1))$. The Gysin braid of the pair $(\mathfrak {B}_R(d_5), X_R(d_5))$ is determined by the surjectivity of $H_2(\mathfrak {B}_R(d_5)) \rightarrow H_2(X_R(d_5))$

We next use the reduced braid diagram to compute $H_*(\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R^+(d_5))$. The bottom row (i.e. the exact sequence of the triple $(\mathfrak {B}_R(d_5), \mathfrak {B}^+(d_1,d_5), \mathfrak {B}(d_1, d_5))$) immediately yields $H_k(\mathfrak {B}_R(d_5), \partial \mathfrak {B}_R^+(d_5))$ for all values except $k = 4, 5$, and these are determined by the third column (i.e. the exact sequence of the triple $(\mathfrak {B}_R(d_5), \mathfrak {B}^+(d_1,d_5), \partial \mathfrak {B}^+(d_1))$).

Finally, the values for $H_*(\mathfrak {B}(d_5), \partial \mathfrak {B}^+(d_5))$ are obtained from the Gysin braid diagram of the triple $(\mathfrak {B}(d_5), \mathfrak {B}^+(d_1, d_5), \mathfrak {B}^+(d_1))$. Inserting the known values into the exact sequence of the triple (i.e. the middle row of the braid diagram) immediately yields the values for all $H_k(\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5))$ except $k = 3, 4, 5$. For those, we focus on the following portion of the diagram

Since the map $\mathbb {Z} \rightarrow \mathbb {Z}$ is surjective, it is an isomorphism, which in turn forces the maps $\mathbb {Z}^{22} \rightarrow \mathbb {Z}^{17}$ and $\mathbb {Z}^{22} \rightarrow \mathbb {Z}^{11}$ to be surjective. That in turn yields $H_5(\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5)) \cong \mathbb {Z}^{11}$ and $H_4(\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5)) \cong \mathbb {Z}^{6}$. They Gysin sequnece of $(\mathfrak {B}(d_5), \partial \mathfrak {B}(d_5))$ then yields the final value needed. $\square$