1 Introduction

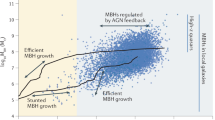
Black hole growth is believed to be powered by gas accretion and actively accreting black holes are detected as optically bright quasars. Optically bright quasars powered by accretion onto supermassive black holes (SMBHs) are now detected at the earliest times, \(z \sim 7\) when the Universe was barely 6 % of its current age [49] down to more recent epochs (\(z \sim 1\); [40]). In fact, populations of optical quasars have now been detected at \(z > 6\) (e.g., [27]) in the Sloan Digital Sky Survey (SDSS). Therefore, the mass build-up of SMBHs is likely to have commenced at extremely high redshifts (\(z > 10\)). The observed luminosities of these high redshift quasars in turn imply extrtemely large black hole masses \(M_\mathrm{BH} > 10^9\,\mathbf{M_{\odot } }\). Assembling such large black holes so early on within the currently accepted cold dark matter structure formation paradigm poses a real challenge for models, specially if the starting point is from remnants of the first generation of metal free stars as seeds, with \(M_0 \sim 10^{1-2}\,M_{\odot }\). One explanation to accomplish this rapid early growth has invoked bumper (super-Eddington) accretion rates for brief periods of time [70]. Alternatively, it has been suggested that the formation of more massive, rarer black hole seeds ab-initio through direct collapse of self-gravitating pre-galactic gas disks at high redshifts might also solve the problem [7, 8, 12, 44–46]. These two assumptions coupled with merger triggered accretion episodes that produce growth spurts appears to somewhat alleviate the problem of building up super-massive black hole masses to the required values by \(z = 6\) for these rare objects. However many open questions still remain on how the first black holes formed and evolved.

Observations provide only moderate constraints on theoretical models at the highest redshifts due to the scarce number of objects that are accessible optically and in the X-ray [27, 49, 67]. At this juncture only a handful of quasars have been detected at \(z > 6.5\) and these tend to be the brightest ones skewing our understanding as they constitute only the tip of the iceberg. Recently, however, a more complete census of the accreting black hole population at \(1<z<4.5\) has become available from 58,000 broad-line quasars (BLQSOs) in the SDSS [40]. This sample has provided an observationally determined black hole mass function at these epochs. These data bridge a critical time gap and enable comparison of competing theoretical models as they cover about one-third of the age of the universe including the critical time span when the bulk of the stars form. This data-set is also extremely valuable in unraveling the connection if any between star formation and black hole growth. Observed correlations like the local \(M_\mathrm{bh} - \sigma \) relation (the mass of the central black hole in a galaxy appears to be strongly correlated to the velocity dispersion of the stars in the inner regions) suggest that these activities in galactic nuclei might be linked. This data-set has been immensely powerful as the derived accretion rates from this spectral sample were used by Kelly et al. [40] to report that most BLQSOs are radiating very sub-optimally, and at nowhere near or at the Eddington limit. And if at all BLQSOs exceed the Eddington limit, it is for a very short period of time. So what the data suggests is that there is a large population of extremely massive black holes at every epoch in the universe from the earliest times to \(z \sim 1\). Reproducing the massive end of the black hole mass function through cosmic time is proving to really test the mettle of current theoretical models. Even models that successfully explain the \(z>6\) monsters more often than not under-produce the massive black holes needed at later times to account for the observed BLQSOs [52].

2 A few basic conceptual definitions in the black hole growth paradigm

The assembly of BH mass in the Universe has been tracked using optical quasar activity. The current phenomenological approach to understanding the assembly of SMBHs involves optical data from both high and low redshifts. These data are used as a starting point to construct a consistent picture that fits within the larger framework of the growth and evolution of structure in the Universe (more details can be found in reviews by Volonteri et al. [73] and Natarajan [51] and references therein). The luminosities of these high redshift quasars imply black hole masses \(M_\mathrm{bh} > 10^9\,M_{\odot }\).

Some of the basic definitions and assumptions that are useful in understanding the accretion paradigm are provided below. Quasars are active black holes that are powered by accretion of gas. A small fraction of the rest mass energy of the accreted gas is emittted as radiation. This luminosity is typically measured in units of the Eddington luminosity which for a black of mass \(M_\mathrm{bh}\) is defined to be

$$\begin{aligned} L_\mathrm{Edd} = \frac{4 \,\pi \,G\,c\,{m_p}\,M_\mathrm{bh}}{\sigma _T}, \end{aligned}$$
(1)

where \(m_p\) is the proton mass and \({}_T\) is the Thomson scattering cross-section. The bolometric luminosity (integrated over all wavelengths) of the accreting black hole is given by:

$$\begin{aligned} L_\mathrm{bol} = \epsilon \,{\dot{M}}\,c^2, \end{aligned}$$
(2)

where \(\dot{M}\) is the mass accretion rate and \(\epsilon \) (typically assumed to be 10 %) is the radiative efficiency factor. The Eddington rate is defined to be the mass accretion rate for which a black hole with radiative efficiency \(\epsilon = 0.1\) has the Eddington luminosity,

$$\begin{aligned} {\dot{M}_\mathrm{Edd}}\, =\, \frac{L_\mathrm{Edd}}{0.1\,c^2}\, =\, 2.2\,\left( \frac{M_\mathrm{bh}}{10^8\,M_{\odot }}\right) \,\,M_{\odot }\,\mathrm{year^{-1}} \end{aligned}$$
(3)

The dimensionless rate \({\dot{m}}\) is simply the accretion rate measured in units of the Eddington rate, \({\dot{m}} = {\dot{M}} / {\dot{M}_\mathrm{Edd}}\) This definition of the Eddington rate applies in the case of accretion onto a black hole from a thin accretion disk whose viscosity \(\nu = \alpha \,c_s\,H\) is defined in terms of the dimensionless parameter \(\alpha \), the sound speed \(c_s\), and the disk scale height \(H\). The mass growth rate of a black hole accreting at \({\dot{M}_\mathrm{Edd}}\) is exponential with an e-folding timescale

$$\begin{aligned} t_\mathrm{Salpeter} = 4.5 \times 10^7\,\,\mathrm{years} \end{aligned}$$

the Salpeter time. For Eddington accretion, this is the only characteristic time-scale in the problem and challenges arise when the age of the universe is comparable to the Salpeter time-scale.

The mass build-up of black holes over cosmic time is understood and computed in the context of the standard paradigm for formation of structure in the universe. As the local demography of black holes suggests that they are hosted in pretty much every galactic nucleus, the mass assembly of the black hole and the stellar component of the host galaxy are assumed to be correlated. When such a correlation is set-up and how it evolves with time are some of the open questions that are currently driving all modeling attempts. Current modeling is grounded in the framework of the standard scenario of structure formation in a cold dark matter, dark energy dominated universe that involves the growth of structure via gravitational amplification of small perturbations in a CDM universe—a model that has independent validation, most recently from Wilkinson Microwave Anisotropy Probe (WMAP) measurements of the anisotropies in the cosmic microwave background [26]. Structure formation is tracked over cosmic time by keeping a census of the number of collapsed dark matter halos of a given mass that form; these provide the sites for harboring black holes. The computation of the mass function of dark matter halos is done using either the Press–Schechter [55] or the extended Press–Schechter theory [43], or Monte-Carlo realizations of merger trees [38, 69] or, in some cases, directly from cosmological N-body simulations [19].

In particular Volonteri et al. [69] have presented a detailed merger-tree based scenario to trace the growth of black holes from the earliest epochs to the present day that we adopted in the work presented here. Monte-Carlo merger trees are created for present day halos and propagated back in time to a redshift of \(\sim \)20. With the merging history thus determined, the initial halos at \(z \sim 20\) are then populated with seed black holes.

3 Seed formation models

To track the mass assembly history of black holes in the Universe, we need to start with an initial population of seeds at high redshift. In the standard picture, the assumption is that the remnants of the massive first stars (Pop III stars) likely provide the earliest seeds in the range of 50–100\(\,M_{\odot }\). However, whether the first stars were indeed this massive has been called to question from the latest round of recent higher resolution simulation results where fragmentation occurs ubiquitously [17, 30, 68]. Besides these simulations also find that now lighter remnants that are a few solar masses at most also get ejected from the gas-rich nuclei of dark matter halos where they form due to many-body interactions as the first stars appear to form in clusters. An alternate model for the formation of massive seeds from the direct collapse of pre-galactic disks has been investigated by Lodato and Natarajan [44, 45]. In these models, there is a limited mass range of halos with a further narrow range in appropriate angular momentum properties (spins) that are able to form seeds. However, contrary to the Pop III case, massive seeds with \(M\approx 10^5\hbox {--}10^6M_{\odot }\) can form at high redshift (\(z>15\)), when the intergalactic medium has not been significantly enriched by metals as reported in [44, 45], where more details of this seeding model can be found. In this scenario, the development of non-axisymmetric spiral structures drives mass infall and gas accumulation in a pre-galactic disc with primordial composition. The central mass accumulation that provides an upper limit to the SMBH seed mass that can form is given by:

$$\begin{aligned} M_\mathrm{BH}= m_\mathrm{d}M_\mathrm{halo}\left[ 1-\sqrt{\frac{8\lambda }{m_\mathrm{d}Q_\mathrm{c}}\left( \frac{j_\mathrm{d}}{m_\mathrm{d}}\right) \left( \frac{T_\mathrm{gas}}{T_\mathrm{vir}}\right) ^{1/2}}\right] \end{aligned}$$
(4)

for

$$\begin{aligned} \lambda <\lambda _\mathrm{max}=m_\mathrm{d}Q_\mathrm{c}/8(m_\mathrm{d}/j_\mathrm{d}) (T_\mathrm{vir}/T_\mathrm{gas})^{1/2} \end{aligned}$$
(5)

and \(M_\mathrm{BH}=0\) otherwise. Here \(\lambda _\mathrm{max}\) is the maximum halo spin parameter (that is defined as the ratio between gravitational and rotational support in the pre-galactic disk) for which the disc is gravitationally unstable, \(m_d\) is the gas fraction that participates in the infall and \(Q_\mathrm{c}\) is the dynamical Toomre stability parameter. The efficiency of SMBH formation is strongly dependent on the Toomre parameter \(Q_\mathrm{c}\), which sets the frequency of formation, and consequently the number density of SMBH seeds. We set \(Q_\mathrm{c}=2\) (the intermediate efficiency massive seed model) as described in [71] for the purposes of comparison with observations presented here.

The efficiency of the seed assembly process ceases at large halo masses, where the disc undergoes fragmentation instead. This occurs when the virial temperature exceeds a critical value \(T_\mathrm{max}\), given by:

$$\begin{aligned} \frac{T_\mathrm{max}}{T_\mathrm{gas}}=\left( \frac{4\alpha _\mathrm{c}}{m_\mathrm{d}}\frac{1}{1+M_\mathrm{BH}/m_\mathrm{d}M_\mathrm{halo}}\right) ^{2/3}, \end{aligned}$$
(6)

where \(\alpha _\mathrm{c}\approx 0.06\) is a dimensionless parameter measuring the critical gravitational torque above which the disc fragments [56].

To summarize the seeding model, every dark matter halo that can seed an initial massive black hole is characterized by its mass \(M\) (or virial temperature \(T_\mathrm{vir}\)) and by its spin parameter \(\lambda \). The gas has a temperature \(T_\mathrm{gas}=5000\)K. If \(\lambda <\lambda _\mathrm{max}\) (see Eq. 5) and \(T_\mathrm{vir}<T_\mathrm{max}\) (Eq. 6), then we assume that a seed BH of mass \(M_\mathrm{BH}\) given by Eq. (4) forms in the center. The remaining relevant parameters are set to \(m_\mathrm{d}=j_\mathrm{d}=0.05\), \(\alpha _\mathrm{c}=0.06\) and here we consider in detail the \(Q_\mathrm{c}=2\) case.

In the massive seed model, SMBHs form (i) only in halos within a narrow range of virial temperatures (\(10^4\hbox { K} < T_{vir}<1.4\times 10^4\) K), hence, halo velocity dispersion (\(\sigma \simeq 15\,\mathrm{km\,s}^{-1} \)), and (ii) for a given virial temperature all seed masses below \(m_d\,M\) modulo the spin parameter of the halo are allowed (see Eqs. 1 and 3). The mass function of initial massive black hole seeds that form from this direct collapse process peaks at \(10^5M_{\odot }\), with a steep drop at about \(3\times 10^6M_{\odot }\). Furthermore in recent work Agarwal et al. [2] demonstrate that the sites where these direct-collapse black holes (DCBHs) can form, star formation is initially inhibited due to the photo-dissociating effect of Lyman-Werner radiation on molecular hydrogen (which is the primarily coolant for gas available at these early times). We refer the reader to [45, 51] for a more detailed discussion of the mass function of seed black holes. Here we stress that given points (i) and (ii) above the initial seeds do not satisfy the locally empiricially determined \(M_\mathrm{BH} -\sigma \) relation, in fact the seed masses are not correlated with \(\sigma \), rather they are correlated with the initial angular momentum of the gas in the parent dark matter halo. Therefore in the massive seed models, we start with no initial correlations between the black hole and its immediate vicinity.

Both scenarios for black hole seed formation rely on zero metallicity gas. However, as we track the mass build-up of black holes over time, metals from generations of forming stars will pollute the pristine gas. We model the evolution of metallicity as per a physically motivated numerical implementation of Scannapieco et al. [59] where metal enrichment occurs via pair-instability supernovae winds, by following the expansion of spherical outflows into the Hubble flow. They compute the co-moving radius, at a given redshift, of an outflow from a population of supernovae that exploded at an earlier time. Using a modification of the Press–Schechter technique, they compute the bivariate mass function of two halos of arbitrary mass and collapse redshift, initially separated by a given co-moving distance. From this function they calculate the number density of supernovae host halos at a given co-moving distance from a ‘recipient’ halo of a given mass \(M_h\) that form at a given redshift \(z\). By integrating over this function, one can calculate the probability that a halo of mass \(M_h\) forms from metal-free gas at a redshift \(z\). In our work and that of other modelers, Monte-Carlo realizations of the merging histories of dark matter halos over cosmic time are created. Therefore every present day halo can be traced back to its progenitors at all earlier redshifts. When a halo forms in our merger tree we calculate the probability that it is metal-free (hence, it can form Pop III stars) and determine if conducive conditions are satisfied.

Every halo entering this merger tree structure is then assigned a spin parameter drawn randomly from the log-normal distribution in \(\lambda _\mathrm{spin}\) found in numerical simulations to mimic the variation in angular momentum content of the baryonic gas in these halos, with mean \(\bar{\lambda }_\mathrm{spin}=0.05\) and standard deviation \(\sigma _\lambda =0.5\) [16]. We assume that the spin parameter of a halo is not modified by its merger history, as no consensus exists on this issue at the present time.

4 Evolving black hole seeds over cosmic time

To illustrate how theoretical models of black hole growth are compared to observational data, we describe various models derived using a range of input assumptions. The data-set that will be compared to here are those presented from the SDSS in Kelly et al. We evolve the population of SMBH seeds according to simple models of self-regulation with the host dark matter halo. The main features of the models have been discussed elsewhere [72]. We summarize below the relevant assumptions. SMBHs in galaxies undergoing a major merger (i.e., the two halos having a mass ratio \(>\)1:10) accrete mass and become active. Each SMBH accretes an amount of mass, \(\varDelta M=9\times 10^7(\sigma /200\,\mathrm{km\,s^{-1}})^{4.24}M_{\odot }\), where \(\sigma \) is the velocity dispersion after the merger. This relationship scales with the \(M_\mathrm{BH}-\sigma \) relation, as it is seen today [31]:

$$\begin{aligned} M_\mathrm{BH}=1.3\times 10^8 \left( \frac{\sigma }{200\,\mathrm{km\,s^{-1}}} \right) ^{4.24} M_{\odot }, \end{aligned}$$
(7)

the normalization in \(\varDelta M\) was chosen to take into account the contribution of SMBH–SMBH mergers, without exceeding the mass given by the \(M_\mathrm{BH}-\sigma \) relation.

We link the correlation between the black hole mass and the central stellar velocity dispersion of the host with the empirical correlation between the central stellar velocity dispersion and the asymptotic circular velocity as \(\sigma =v_\mathrm{c}/\root \of {2}\)) of galaxies [28]. The latter is a measure of the total mass of the dark matter halo of the host galaxy. We calculate the circular velocity from the mass of the host halo and its redshift. With all these ingredients in hand, we can now trace the accretion history of a population of black holes over cosmic time. Flowing back through the merger history of the dark matter halos that host these black holes, we now assume a variety of accretion modes (accretion rates) that appropriately span parameter space for various independent model assumptions. This enables us to compare accretion histories for several sets of input assumptions for the theoretical model (two kinds of initial seeds) with observational data.

The rate at which mass is accreted scales with the Eddington rate for the SMBH, and we set either a fixed Eddington ratio of \(f_\mathrm{Edd}=1\) (for Pop III seeds), \(f_\mathrm{Edd}=0.3\) (for massive seeds), or an accretion rate derived from the distribution derived by Merloni and Heinz [47] (we apply this model to massive seeds only) for our investigation. The empirical distribution of Eddington ratios derived by Merloni and Heinz [47] (MH08 thereafter) is fit by a function in \(\log (L_\mathrm{bol}/L_\mathrm{Edd})\). The fitting function of the Eddington ratio distribution as a function of SMBH mass and redshift, is computed in 10 redshift intervals (from \(z=0\) to \(z=5\)) for 4 different mass bins (\(6 < \log (M_\mathrm{BH}/M_{\odot })< 7, 7 < \log (M_\mathrm{BH}/M_{\odot }) < 8, 8 < \log (M_\mathrm{BH}/M_{\odot }) < 9, 9 < \log (M_\mathrm{BH}/M_{\odot }) < 10\)), and then fit with an analytic function which is the sum of a Schechter function and a log-normal. The Eddington ratio distributions are then normalized to unity at every given mass and redshift. Here we present the comparison of three models denoted as PopIII-Edd, Massive-MH and Massive-subEdd respectively with the data-set of Kelly et. al. Note that in the model names used here the first part refers to the kind of seed and the second part refers to the kind of accretion history assumed. Therefore the model PopIII-Edd refers to: initial seeds from Pop III remnants that are always accreting at the Eddington rate; model Massive-MH refers to initial massive seeds formed from direct collapse accreting with Eddington ratios drawn from the MH08 distribution and the model Massive-subEdd: initial massive seeds accreting \(0.3X\) Eddington at all times.

In the Massive-MH model the accretion rate is not limited to the Eddington rate and mildly super-Eddington accretion rates (up to \(f_\mathrm{Edd}\sim 10\)) are possible and allowed as per MH08. For all three scenarios considered here, accretion starts after a dynamical timescale and lasts until the SMBH, of initial mass \(M_\mathrm{in}\), has accreted \(\varDelta M\). The lifetime of an AGN therefore depends on how much mass it accretes during each episode:

$$\begin{aligned} t_\mathrm{AGN}=\frac{t_\mathrm{Edd}}{f_\mathrm{Edd}} \frac{\epsilon }{1-\epsilon }\ln (M_\mathrm{fin}/M_\mathrm{in}), \end{aligned}$$
(8)

where \(\epsilon \) is the radiative efficiency (\(\epsilon \simeq 0.1\)), \(t_\mathrm{Edd}=0.45\) Gyr and \(M_\mathrm{fin}=\min [(M_\mathrm{in}+\varDelta M),1.3\times 10^8 (\sigma /200 \,\mathrm{k\,ms^{-1}})^{4.24}M_{\odot }]\).

We further assume that, when two galaxies hosting SMBHs merge, the SMBHs themselves merge within the merger timescale of the host halos, which is a plausible assumption for SMBH binaries formed after gas-rich galaxy mergers [22]. We adopt the relations suggested by Taffoni et al. [65] for the merger timescale. Black holes are allowed to accrete during the merging process if the timescale for accretion, corresponding to the sum of the dynamical timescale and \(t_\mathrm{AGN}\), is longer than the merger timescale.

As outlined before, in propagating the seeds it is assumed that accretion episodes and therefore growth spurts are triggered only by major mergers. Major mergers refers to cases in which the mass ratio of the two interacting dark matter halos is 1:4 or higher. We find that in a merger-driven scenario for SMBH growth the most biased galaxies at every epoch host the most massive SMBHs that are most likely to populate the \(M_\mathrm{BH} -\sigma \) relation. Lower mass SMBHs (below \(10^6\,M_{\odot }\)) are instead off the relation at \(z = 4\) and even at \(z = 2\). These baseline results are independent of the seeding mechanism. In the initial massive seeds scenario, most of the SMBH seeds start out well above the \(z=0 M_\mathrm{bh} - \sigma \), that is, they are ‘overmassive’ compared to the local relation. Seeds form only in halos within a narrow range of velocity dispersion (\(\sigma \simeq 15\,\mathrm{km\,s}^{-1} \), see Eqs. 1 and 3. The SMBH mass corresponding to \(\sigma \simeq 15\,\mathrm{km\,s}^{-1}\), according to the local \(M_\mathrm{BH} -\sigma \) relation, would be \(\sim \!\! 3\times 10^3 M_{\odot }\). The MF instead peaks at \(10^5M_{\odot }\) [45]. As time elapses, all halos are bound to grow in mass by mergers. The lowest mass halos, though, experience mostly minor mergers, that do not trigger accretion episodes, and hence do not grow the SMBHs. The evolution of these systems can be described by a shift towards the right of the \(M_\mathrm{bh} - \sigma \) relation: \(\sigma \) increases, but \(M_\mathrm{BH}\) stays roughly constant.

5 Confronting models with observations

Kelly et al. [40] derive an estimate of the black hole mass function (BHMF) of BLQSOs correcting for incompleteness and statistical uncertainties from a sample of 9886 quasars at \(1< z < 4.5\) from the SDSS. They find ‘downsizing’ of BHs in BLQSOs, i.e. the peak of the number density shifts to higher redshift with increasing, with black hole mass peaking at \(z \sim 2\). They report that as a function of back hole mass and Eddington ratio, the SDSS at \(z >1\) is highly incomplete at \(M_\mathrm{BH} \le 10^9 M_{\odot }\) and \(L/L_\mathrm{Edd} < 0.5\). The lower limit on the lifetime of a single BLQSO phase was estimated to be \(>\!\!150 \pm 15\) Myr, with a maximum black hole mass of \(\sim \!\!3 \times 10^{10} M_{\odot }\). Kelly et al. [40] also find that the Eddington ratio distribution peaks at \(L/L_\mathrm{Edd} \sim 0.05\) with a small dispersion implying that most BLQSOs are radiating nowhere near or at the Eddington limit. From their estimated lifetime and Eddington ratio distributions they infer that most massive black holes spend a significant amount of time growing in an earlier obscured phase consistent with models of self-regulated growth.

We focused our modeling efforts on four redshifts of interest (\(z=1.25, z=2, z=3.25, z=4.25\), to match the redshift bins in K10). At each redshift our models provide us with a sample of all SMBHs present at that particular cosmic time, and of the SMBHs that are actively accreting. From the SMBH mass and its Eddington ratio, \(f_\mathrm{Edd}\), we can derive their bolometric luminosity: \(\log (L_\mathrm{bol}/\mathrm{erg}\,\mathrm{s}^{-1}=38.11+\log (M_\mathrm{BH}/M_{\odot })+\log (f_\mathrm{Edd})\). We apply a bolometric correction of 4.3 (K10) and select only quasars that are more luminous than the minimum luminosity determined by the flux limit described in Richards et al. [57] (the parent sample of K10). Finally, we assume that the fraction of unobscured quasars is 20 %, based on La Franca et al. [42]. We do not here apply explicitly the evolutionary model of La Franca et al. [42], where the fraction of obscured quasars depends on both redshift and luminosity, as the redshift range we are interested is beyond those explored by La Franca et al. [42], but we note that when we apply their evolutionary model in the redshift range \(z=1\hbox {--}3\) we obtain consistent results. Recently Fiore et al. [29] have also derived and published BHMFs for the entire active SMBH population as opposed to just the BLQSOs (as done by Kelly et al. [40]) and as a consequence of which their mass functions are slightly higher than those reported in K10.

We then compare the observationally derived BHMFs of luminous (\(>\)10\(^{45}\hbox {--}10^{46}\) erg/s) BLQSOs at \(1<z<4.5\) drawn from the SDSS presented in [40], with models of merger driven BH growth in the context of standard hierarchical structure formation models described in the previous section. In the models, we explore two distinct black hole seeding prescriptions at the highest redshifts: “light seeds”—remnants of Population III stars and “massive seeds” that form from the direct collapse of pre-galactic disks. The subsequent merger triggered mass build-up of the black hole population is tracked over cosmic time under the assumption of a fixed accretion rate as well as rates drawn from the distribution derived by Merloni and Heinz [47]. Four model snapshots at \(z=1.25, z=2, z=3.25, z=4.25\) are compared to the SDSS derived BHMFs of BLQSOs. The results of the detailed comparisons are shown in Figs. 1, 2, 3 and 4. We find that the light seed models fall short of reproducing the observationally derived mass function of BLQSOs at \(M_\mathrm{BH}>10^9 M_{\odot }\) throughout the redshift range; the massive seed models with a fixed accretion rate of \(0.3\,Edd\), or with accretion rates drawn from the Merloni and Heinz distribution provide the best fit to the current observational data at \(z>2\), although they overestimate the high-mass end of the mass function at lower redshifts. At low redshifts, a drastic drop in the accretion rate is observed and this is explained as arising due to the diminished gas supply available due to consumption by star formation or changes in the geometry of the inner feeding regions. Therefore, the over-estimate at the high mass end of the black hole mass function for the massive seed models can be easily be modified, as the accretion rate is likely significantly lower at these epochs than what we assume. For the Merloni and Heinz [47] model, examining the Eddington ratio distributions \(f_{Edd}\), we find that they are almost uniformly sampled from \(f_{Edd}=10^{-2}-1\) at \(z\simeq 1\), while at high redshift, current observations suggest accretion rates close to Eddington, if not mildly super-Eddington, at least for these extremely luminous quasars (comparison shown in Fig. 5). Our key findings are that the duty cycle of SMBHs powering BLQSOs increases with increasing redshift for all models and models with Pop III remnants as black hole seeds are unable to fit the observationally derived BHMFs for BLQSOs, lending support for the massive seeding model (the results of the comparison are plotted in Fig. 6).

Fig. 1
figure 1

The derived mass function (MF) of SMBHs at \(z=4.25\). The upper shaded curve in all three panels is the MF for all SMBHs including active and inactive ones. The lower (darker) shaded curve in all three panels is the MF for SMBHs that can be identified as BLQSOs. The dashed curve in all three panels is the mass function of all SMBHs from MH08. The solid curve is the MF of BLQSOs from K10. The three panels refer to the models: PopIII-Edd (uppermost panel), Massive-MH (middle panel), Massive-subEdd (bottom panel)

Full size image
Fig. 2
figure 2

The derived MF of SMBHs at \(z=3.25\). The upper shaded curve in all three panels is the MF for all SMBHs including active and inactive ones. The lower (darker) shaded curve in all three panels is the MF for SMBHs that can be identified as BLQSOs. The dashed curve in all three panels is the MF of all SMBHs from MH08. The solid curve is the MF of BLQSOs from K10. The three panels refer to the models: PopIII-Edd (uppermost panel), Massive-MH (middle panel), Massive-subEdd (bottom panel)

Full size image
Fig. 3
figure 3

The derived MF of SMBHs at \(z=2\). The upper shaded curve in all three panels is the MF for all SMBHs including active and inactive ones. The lower (darker) shaded curve in all three panels is the MF for SMBHs that can be identified as BLQSOs. The dashed curve in all three panels is the MF of all SMBHs from MH08. The solid curve is the MF of BLQSOs from K10. The three panels refer to the models: PopIII-Edd (uppermost panel), Massive-MH (middle panel), Massive-subEdd (bottom panel)

Full size image
Fig. 4
figure 4

The derived MF of SMBHs at \(z=1.25\). The upper shaded curve in all three panels is the MF for all SMBHs including active and inactive ones. The lower (darker) shaded curve in all three panels is the MF for SMBHs that can be identified as BLQSOs. The dashed curve in all three panels is the MF of all SMBHs from MH08. The solid curve is the MF of BLQSOs from K10. The three panels refer to the models: PopIII-Edd (uppermost panel), Massive-MH (middle panel), Massive-subEdd (bottom panel)

Full size image
Fig. 5
figure 5

Comparison of observational data and massive seeding model for the Eddington ratio distribution for BLQSOs. The model with uncertainties is shown in the shaded region and the data from [40] are plotted as red crosses (color figure online)

Full size image
Fig. 6
figure 6

Comparison of observational data and models for the duty-cycle of BLQSOs. The models with uncertainties are plotted as the shaded regions and the data from [40] are plotted as red crosses (color figure online)

Full size image

6 Challenging key model assumptions

As shown above, with the growing wealth of data that spans a significant portion of the age of the universe, current theoretical models are unable to satisfactorily reproduce observations. The inability to match the mass function of the most massive black holes at every epoch, not just at the highest redshifts appears to be an endemic failure of these models. This calls into question the various assumptions that are adopted in this scheme to understand the assembly history of black holes. Now we scrutinize in greater detail some of the key assumptions in this modeling procedure.

7 Could mergers not be the key players? Is there any evidence for other growth mechanisms?

Theoretical approaches often couple major mergers of galaxies to growth spurts for the central black hole, as mergers facilitate gas delivery to the galactic nucleus. Furthermore, in most models including those shown here the mass gain during accretion episodes triggered by major mergers is capped due strong coupling or feedback from the growing black hole to the galactic nucleus. This limiting of growth is invoked in order to match and not exceed the local \(M_\mathrm{BH} - \sigma \) relation [72] and to match the observed quenched star formation in massive galaxies [64]. While this highly efficient form of feedback quenches star formation as needed, it also suppresses the mass growth of the most massive black holes. Feedback shuts down MBH accretion over timescales of tens of millions of years, causing interrupted, rather than continuous growth. To account for the presence of high mass black holes inferred from the data at all redshifts, this coupling between black hole growth and star formation needs to be significantly weaker than currently presumed.

Indeed, it is suggested that feedback is likely suppressed in the presence of cosmic flows of cold gas, while the MBHs are allowed to accrete at slightly super-Eddington rates to assemble the monsters [21]. This result is however based on cosmological simulations at kpc resolution that are far from resolving the impact of feedback on the relevant small scales. Dubois et al. [24], using higher resolution zoomed-in simulations, find that feedback from the accreting MBH affects the very structure of cold flows making MBH feeding less efficient. The conclusions of these studies confirm that making \(M_\mathrm{bh}>10^9\,\mathbf{M_{\odot } }\) black holes with appropriate feedback is challenging regardless of the technique. In fact, black holes may simply not be the dominant source of feedback for the most massive galaxies at early times. There also seems to be evidence that, while quasar activity is merger-driven, this is definitely not the case for most of the AGN that constitute the bulk of the population [13, 23, 50]. There are several hints that non-merger processes, such as turbulence driven inflows and secular mechanisms, may enable long-lived gas flows that trickle steadily triggering AGN, and perhaps be the dominant feeding mechanism at low redshifts, \(z \simeq 1\hbox {--}2\). Theoretical support to this view is provided by simulations of isolated, gas rich galaxies. Gravitational torques sustain strong turbulence that can in turn supply gas at high rates to nuclear regions. Therefore gas supply to galactic nuclei, right down to the central pc can be maintained by these instabilities without requiring mergers [36].

8 Does slow and steady actually win the mass build-up race?

If instead of mergers we explore the notion of steady mass build up (not necessarily triggered by mergers), we obtain constraints on the average Eddington rate when matching to data. Adopting an optimistic approach, and letting MBHs accrete unimpeded, the simplest question one may ask is: what is the minimum average accretion rate that is needed to grow a BH to \(M_\mathrm{bh}>10^9\,\mathbf{M_{\odot } }\) by \(z=4\) and \(z=3\)? Scaling the accretion rate in units of the Eddington luminosity, we have \(L_\mathrm{Edd}=M_{BH}c^2/t_\mathrm{Edd}\), where \(t_\mathrm{Edd}=\frac{\sigma _T \,c}{4\pi \,G\,m_p}= 0.45\) Gyr, where \(c\) is the speed of light, \(\sigma _T\) is the Thomson cross section, and \(m_p\) is the proton mass. Therefore, if the inflow rate of mass is \(\dot{M}_{in}\), and \(\dot{M}\) is the mass that goes into increasing the BH mass, then:

$$\begin{aligned} L=\epsilon \, \dot{M}_\mathrm{in} c^2= f_\mathrm{Edd} L_\mathrm{Edd} c^2, \end{aligned}$$
(9)

where \(f_\mathrm{Edd}\) is the Eddington ratio, the incremental mass gain is given by \(dM = (1-\epsilon ) dM_{in}\), and \(\epsilon \simeq 0.1\) is the efficiency of conversion of rest-mass into energy. The time-averaged Eddington ratio of an MBH with initial mass \(M_0\) to grow to a final mass \(M_{BH}(z)\) by redshift \(z\) within the Hubble time redshift \(z\) is given by:

$$\begin{aligned} \langle f_\mathrm{Edd} \rangle _t=\frac{t_\mathrm{Edd}}{t_\mathrm{Hubble}(z)} \frac{\epsilon }{1-\epsilon }\ln \left( \frac{M_\mathrm{BH}}{M_0}\right) . \end{aligned}$$
(10)

As shown in Fig. 7, to grow a ‘light’ seed (\(\sim \!\!10^2\,\mathbf{M_{\odot } }\)) to, say, \(5\times 10^9\,\mathbf{M_{\odot } }\) by \(z=3\), when the age of the Universe is 2.19 Gyr, it needs to accrete continuously at \(\langle f_\mathrm{Edd}\rangle _t=0.40 \), and at \(\langle f_\mathrm{Edd}\rangle _t=0.56 \) if it must grow by \(z=4\), when the Universe was 1.57 Gyr old. The constraints for ‘massive’ seeds (\(\sim \!\! 10^5\,\mathbf{M_{\odot } }\)) are slightly less stringent: \(\langle f_\mathrm{Edd}\rangle _t=0.34 \) at \(z=4\), and \(\langle f_\mathrm{Edd}\rangle _t=0.25\) at \(z=3\). In other words, these MBHs must accrete at about a third to half of the Eddington rate for the entire time, or at the Eddington rate for about a third to a half their life in spite of feedback effects. This seems at odds with how strongly feedback affects low-mass seeds in the first galaxies [48, 54].

Fig. 7
figure 7

Time-averaged Eddington ratio that an MBH with initial mass \(M_0\) requires to grow to a final mass \(M_{BH}=5\times 10^{9}\,\mathbf{M_{\odot } }\) within the Hubble time at a given redshift \(z=3; 4; 6; 7\) (bottom to top). This clearly illustrates the challenge of assembling the most massive black holes at every epoch and the model fine-tuning that is needed to do so

Full size image

We note here that recent simulation results of the formation and evolution of the first metal-free stars find that the initial mass function of these objects may not be as top-heavy as originally thought. Copious fragmentation is now found in these simulations, thus lowering the masses of the remnants further to \(\sim \!\!1 \hbox {--}10\,\mathbf{M_{\odot } }\) [30, 37]. Besides, with a multiplicity of these low black hole seed masses now forming, easy ejection due to many-body encounters is inevitable and they rarely remain in gas-rich regions and, in fact, do not grow appreciably even by \(z \sim 8\) [3]. So more massive seeds are favored to match the brightest detected quasars at \(z > 6\) as they require a less stringent growth path (see also [32]). For instance, Eq. 10 shows that a \(5\times 10^9\,\mathbf{M_{\odot } }\) MBH at \(z=7\) requires \(M_0>10^3\,\mathbf{M_{\odot } }\) if \(\langle f_\mathrm{Edd}\rangle _t=1\), i.e., if it grows exactly at the Eddington rate for the whole age of the Universe at \(z=7\).

As estimated by Salvaterra et al. [58] such steady growth however is not required of the entire MBH population but only of the most massive BHs. Soltan’s argument [62] constrains the average accretion rate of the high-z MBH population, as a whole. Equation 10 can be recast in terms of the black hole mass density, \(\rho \), as:

$$\begin{aligned} \dot{\rho }= \frac{\langle f_\mathrm{Edd}\rangle _\mathrm{t,all}}{t_\mathrm{Edd}} \frac{1-\epsilon }{\epsilon } \rho +\dot{\rho }_\mathrm{form}, \end{aligned}$$
(11)

where \(\langle f_\mathrm{Edd}\rangle _{t,all}\) is a mean Eddington ratio (here the mean is over the whole population and over time), and \(\dot{\rho }_{form}\) is a source term that accounts for MBH formation. A simple approximation is one wherein all seeds are assumed to have similar masses and to form co-evally (\(\dot{\rho }_\mathrm{form}=0\). In principle such an approximation is always valid once one choses as starting time for the integration a time, \(t_0>0\), to be when all MBHs have formed already giving a total density \(\rho _0\)). This renders the equation easily integrable to obtain the total accreted mass density, \(\rho _\mathrm{acc}\), and provides insight on the growth requirements without further assumptions of a functional form for \(\dot{\rho }_\mathrm{form}\):

$$\begin{aligned} \rho _\mathrm{acc}(t)=\rho _0 \left[ \exp \left( \langle f_\mathrm{Edd}\rangle _{t,all} \frac{t}{t_{Edd}} \frac{1-\epsilon }{\epsilon } \right) -1 \right] . \end{aligned}$$
(12)

In Fig. 8, we show the constraints on \(\rho _\mathrm{acc}\) obtained by Salvaterra et al. [58] from the cosmic X-ray background. The maximum accreted mass density, \(\rho _\mathrm{acc}\) of all BHs, is related to the unresolved intensity observed at 1.5 keV, as this is due to the integrated emission of undetected sources at \(z>5\). In Fig. 8, we also include results from stacking analyses that look for AGN contribution in galaxies at \(z>6\) [14, 29, 67, 74]. This figure highlights the conundrum. For plausible values of \(\rho _0\simeq 10\hbox {--}1000\hbox { M}_\odot \hbox { Mpc}^{-3}\) (e.g., [18, 71]), the average Eddington rate \(\langle f_\mathrm{Edd}\rangle _{t,all}\) for the whole population must be less than 0.1–0.3 at \(z>5\) [58], and less than 0.05–0.1 by \(z=0\), to match today’s MBH mass density as shown in Fig. 2. So while some MBHs must, and can, grow, most of them must have their growth stunted. What is salient is that the growth of the most massive black holes cannot be preferentially stunted, as exemplified by Fig. 1 and discussion therein.

Fig. 8
figure 8

Example evolution of the initial MBH mass density, \(\rho _0\) (in units of \(\hbox {M}_\odot \hbox { Mpc}^{-3}\)) and average \(\langle f_\mathrm{Edd}\rangle _{t,all}\) for the whole population in order to match observational constraints. Ignoring obscuration, the mass density must be below the upper limits and the shaded area on the right-hand side of the figure, and within the hatched area on the left-hand side

Full size image

Of course, these constraints cannot account the possibility of obscured accretion—so unless \(\sim \!\!30\,\%\) of the mass growth and that too preferentially of the most massive MBHs at all epochs occurs in the obscured mode, obscuration cannot solve the conundrum of simultaneously explaining the \(z >6\) QSOs and the lower redshift BLQSOs. However, since observationally high redshift galaxies seem to be UV-bright, this possibility will require nuclear obscuration for the most massive accreting black holes at the earliest epochs [11, 35].

On the other hand, brief bouts of super-Eddington phases may occur that ease the constraints (e.g., [6, 70]). While often the Eddington limit is considered insurmountable, care should be taken to consider when it is not [1]. When the gas supply rate is super-critical, the excess radiation can be trapped, as the infall speed of the gas is larger than the diffusion speed of the radiation [75]. In this case the emergent luminosity is still Eddington limited [4, 5], and yet the growth rate can exceed the Eddington-limited value by a factor of a few [39, 41]. The growth rate can in principle surpass the critical Eddington limit by several orders of magnitude if the efficiency of converting mass into radiation becomes very low. When radiation is trapped and advected inward, the flow may adjust so that the material plunges in from an orbit with small binding energy [53]. The lower the radiative efficiency, \(\epsilon \), the faster the black hole grows, as, while a factor \(\epsilon \) goes into luminosity, a factor of (\(1-\epsilon \)) goes into feeding the black hole. As noted by Abramowicz [1] in this condition the luminosity, in Eddington units, depends logarithmically rather than linearly on the accretion rate (also in Eddington units), thus allowing for highly super-Eddington accretion rates, while the emergent luminosity is only super-Eddington by a small factor.

9 Discussion and conclusions

As we have illustrated above, in order to build a self-consistent picture of black hole growth that explains observations over cosmic history, several of our current input theoretical assumptions appear to be invalid. For instance, the coupling of star formation and black fueling and growth that is adopted (referred to as feedback processes) needs to be highly inefficient for some MBHs. Or a sub-population of MBHs needs to be in gas-rich sites where they can continually accrete. In other words current models appear to prematurely curtail black hole growth causing the deficit in the production of the most massive black holes at all epochs. In event of unperturbed accretion from a rich gas reservoir, an average rate of \(\langle 0.5\,\mathrm{Edd}\,\rangle \) is required throughout cosmic history. The simple models of feedback implemented thus far appear to be inadequate and far too simplistic ([9, 20, 33, 63] for alternate modeling approaches see for example: [66]; or [34]). In terms of physical models, feedback is required to be extremely inefficient in galaxies that harbor the most massive black holes, thereby allowing for more copious accretion in these sites to enable growth. However, these are the very galaxies that also require rapid shutdown of star formation to match observational data. Clearly a new feedback process is required for these massive galaxies that host the most massive black holes. One possibility is that feedback in massive galaxies at high redshift is dominated by kinetic (‘radio-mode’), rather than thermal (‘quasar-mode’) energy input [15, 61]. Theoretical support for this possibility is provided by the study of Dubois et al. [24] where they find that ‘radio-mode’ feedback produces larger black hole growth than ‘quasar-mode’ feedback. This in turn suggests that numerical simulations ought to explore including this kind of feedback at high accretion rates too, as many radio-loud quasars exist that appear to accrete close to the Eddington limit. Alternatively gravitational torques might drive strong turbulence facilitating very efficient angular momentum transport and therefore enable extremely high and steady black hole feeding rates at high redshifts.

It is clear that the abundance and luminosity functions of quasars at the high mass/luminosity end even at lower redshifts requires an additional pathway to modulate the growth of MBHs—likely a more steady mode. Recent theoretical work suggests that cold gas flows are likely ubiquitous in universe, although observational evidence for these remains scant at the moment. Recent high resolution simulations find that cold flows reinforced by the motion of satellites streaming in along the filaments are long-lived and can provide preferentially low angular momentum cold gas to feed galactic centers [10, 25]. Numerically at least, the sustenance of a steady gas flow appears plausible and this might well supply the long-lived accretion mode needed to grow the most massive black holes at all epochs in addition to merger activated growth spurts. Of course the accretion flow itself is not resolved on sub-parsec scales even in the state-of-the-art simulations. Even with gas flows into galaxy discs on kpc scales by cold flows, global instabilities (e.g., bars within bars) on smaller parsec scales as originally suggested by Shlosman and Begelman [60] are needed to deliver gas to the nuclear regions. Bournaud et al. [10] also find that these flows have to be an integral part of the growth picture. If cold flows are indeed implicated, then non-axisymmetric structures like bars that help funnel gas down to the center from larger scales need to operate ubiquitously and at all times in the universe. Bars in these nuclear regions would be hard to detect and characterize observationally, however this is a clear observational prediction that can be tested in the near future with ALMA data of the inner regions of galaxies. Such detections could strongly support the theoretical picture of dense, gas flows permeating and essentially holding the key to the solution of the black hole feeding problem.

One of the key discriminants between the massive and light black hole seed models and the concomitant accretion histories of these initial seeds to late times are the gravitational waves that expected to arise from the mergers of black holes. The gravitational wave signal expected depends on the mass ratio of the merging holes and encodes a lot of additional physical information on the merger process like the spin and the eccentricity of the black hole binary prior to merger. Detection of gravitatonal waves from merging black holes will offer the ultimate test of whether mergers drive the black hole growth process or if they are inconsequential. For the supermassive black holes considered here future experiments like LISA will provide the data to disentangle various models. Other reviewers at this conference have tackled the production of gravitational wave from merging supermassive binaries and their observational signatures.

It is patently obvious that our current rather simple picture for understanding the mass assembly history of black holes needs to be revised. And what appeared to be plausible and exceptional fixes needed to explain the highest mass black holes at the earliest epochs turn out to be essential ingredients required at all epochs to explain the mass functions of growing black holes. Therefore, in order to explain the accretion history of black holes given observations of the brightest quasars from \(z = 1\) to and beyond \(z = 6\), we require most, if not all, of the following elements: massive initial black hole seeds (with masses of \(10^4\)–\(10^5\,\mathbf{M_{\odot } }\)); brief episodes of super-Eddington accretion; highly inefficient feedback from the most massive accreting black holes to sustain growth; and a very efficient means of losing angular momentum via a variety of stellar dynamical and gas processes to bridge the final gap in feeding black holes in galactic nuclei with ease to provide a steady trickle of gas throughout cosmic time.