Instability of a family of examples of harmonic maps

Abstract

The radial map u(x) $=$ $\frac{x}{\Vert x\Vert }$ is a well-known example of a harmonic map from ${\mathbb {R}}^m\,-\,\{0\}$ into the spheres ${\mathbb {S}}^{m-1}$ with a point singularity at x $=$ 0. In Nakauchi (Examples Counterexamples 3:100107, 2023), the author constructed recursively a family of harmonic maps $u^{(n)}$ into ${\mathbb {S}}^{m^n-1}$ with a point singularity at the origin $(n = 1,\,2,\ldots )$, such that $u^{(1)}$ is the above radial map. It is known that for m $\ge$ 3, the radial map $u^{(1)}$ is not only stable as a harmonic map but also a minimizer of the energy of harmonic maps. In this paper, we show that for n $\ge$ 2, $u^{(n)}$ may be unstable as a harmonic map. Indeed we prove that under the assumption n > ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$ $(m \ge 3$, $n \ge 2)$, the map $u^{(n)}$ is unstable as a harmonic map. It is remarkable that they are unstable and our result gives many examples of unstable harmonic maps into the spheres with a point singularity at the origin.

1 Introduction

The radial map u, defined by u(x) $=$ $\frac{x}{\Vert x\Vert }$, is a well-known example of a harmonic map with a point singularity at x $=$ 0 from the m-dimensional Euclidean space except the origin ${\mathbb {R}}^m$ − $\{0\}$ into the $(m-1)$-dimensional sphere ${\mathbb {S}}^{m-1}$ in ${\mathbb {R}}^{m}$ (m is a positive integer). Several studies are given for this special example of harmonic maps ([1, 5, 6, 8], etc. See [2, 3] for harmonic maps.).

In [9], the author introduced a family of harmonic maps $u^{(n)}$ $(n = 1,2,\ldots )$ from ${\mathbb {R}}^m$ − $\{0\}$ into spheres of higher dimension, with a point singularity of a polynomial order of degree n at x $=$ 0, such that $u^{(1)}$ is the above radial map:

Theorem A

([9]). For any positive integers m, n with m $\ge$ n, there exists a harmonic map

such that

• (1) $u^{(n)}$ is a smooth harmonic map, i.e., it satisfies the harmonic map equation

  $$\begin{aligned} \bigtriangleup u^{(n)}\ + \Vert Du^{(n)}\Vert ^2\,u^{(n)}\ = \ 0\,. \end{aligned}$$

• (2) Each component of $u^{(n)}(x)$ is a polynomial of $y_1,\ldots ,y_m$ of degree n, where

  $$\begin{aligned} y_i\ =\ \frac{x_i}{\Vert x\Vert }\ \ \ (i = 1,\ldots ,m). \end{aligned}$$

  More precisely the component $u^{(n)}_{i_1\ldots i_n}(x)$ is a polynomial of $y_{i_1},\ldots ,y_{i_n}$ of degree n, Therefore, $u^{(n)}$ has a point singularity of the polynomial of degree n at x $=$ 0 .

• (3) (the energy density)

  $$\begin{aligned} \Vert D u^{(n)}\Vert ^2 \ = \ \frac{n (n+m-2)}{\Vert x\Vert ^2} \end{aligned}$$

• (4) (the initial map is the radial one)

  $$\begin{aligned} u^{(1)}(x)\ = \ \frac{x}{\Vert x\Vert } \end{aligned}$$

Theorem A gives a harmonic map with a point singularity of a polynomial of various general order, and recovers our previous paper [7] and Fujioka’s paper [4].

For any fixed integer m, this family of examples is constructed recursively with respect to n $(\le \,m)$ by the following defining equalities:

$$\begin{aligned} \qquad \quad u^{(1)}_{i_1}(x)= & {} \frac{x_{i_1}}{\Vert x\Vert } \end{aligned}$$

(1.1)

$$\begin{aligned} u^{(n)}_{i_1\,\ldots \, i_n}(x)= & {} C_{m,n} \left( \frac{x_{i_n}}{\Vert x\Vert }\, u^{(n-1)}_{i_1\,\ldots \, i_{n-1}}(x) \ - \ \frac{1}{n+m-3}\, \Vert x\Vert D_{i_n}u^{(n-1)}_{i_1\ldots i_{n-1}}(x) \right) \ \ \,(n \ge 2)\nonumber \\ \end{aligned}$$

(1.2)

where $D_i$ denotes the derivative with respect to $x_i$ , i.e.,

$$\begin{aligned} D_i\ = \ \frac{\partial }{\partial x_i} \end{aligned}$$

and

$$\begin{aligned} C_{m,n}\ = \ \sqrt{\frac{n+m-3}{2n+m-4}}. \end{aligned}$$

(1.3)

It is known that for m $\ge$ 3, the radial map $u^{(1)}$ is not only stable as a harmonic map but also a minimizer of the energy of harmonic maps ([6]). In this paper, we show that for $n \ge 2$, $u^{(n)}$ may be unstable as a harmonic map. Indeed we prove that for any integer n $\ge$ ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$ ($m \ge 3$, $n \ge 2$), the map $u^{(n)}$ is unstable as a harmonic map.

Main Theorem. Let $m \ge 3$ and $n \ge 2$. For n $\ge$ ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$, the map $u^{(n)}$ is unstable as a harmonic map.

Main Theorem gives many examples of unstable harmonic maps into the spheres with a point singularity at the origin. For example, in the case of m $=$ 3 and n $=$ 2, Main Theorem implies that the map

such that

$$\begin{aligned} u^{(2)}(x)\, = \sqrt{\frac{3}{2}} \left( \frac{x_1^2}{\Vert x\Vert ^2} - \frac{1}{3},\,\frac{x_1x_2}{\Vert x\Vert ^2},\,\frac{x_1x_3}{\Vert x\Vert ^2},\, \frac{x_2x_1}{\Vert x\Vert ^2},\,\frac{x_2^2}{\Vert x\Vert ^2} - \frac{1}{3},\,\frac{x_2x_3}{\Vert x\Vert ^2},\, \frac{x_3x_1}{\Vert x\Vert ^2},\,\frac{x_3x_2}{\Vert x\Vert ^2},\,\frac{x_3^2}{\Vert x\Vert ^2} - \frac{1}{3} \right) \end{aligned}$$

is an unstable harmonic map.

In Sect. 2, we recall basic concepts on stability. In Sect. 3, we give preliminary facts to prove our Main Theorem. We prove Main Theorem in Sect. 4.

2 Basic concepts on stability

In this section, we recall basic facts on harmonic maps, especially the stability of harmonic maps.

Let $(M,\,g)$, $(N,\,h)$ be Riemannian manifolds without boundary and let u be a smooth map from M into N. We know the $\textrm{L}^2$-energy

$$\begin{aligned} E (u)\ =&{} \int _M \left\| du\right\| ^2 dv_g \end{aligned}$$

where

$$\begin{aligned} \begin{array}{lcl} du &{}\quad : &{} \quad \text {the differential map of }u \\ dv_g &{}\quad : &{} \quad \text {the volume form on } (M,\,g). \end{array} \end{aligned}$$

We call it the energy or the energy functional. A map u is harmonic if it is stationary for the energy E(  ), where u is stationary for the energy E(  ) if the first variation of the energy E(  )

$$\begin{aligned} (\delta E)(u)(X) \,=\, \frac{d}{dt} E(u_t) \bigg |_{t=0} \end{aligned}$$

vanishes for any variation $u_t$ of u with compact support such that $u_0$ $=$ u, and X $=$ ${\displaystyle dU\!\!\left. \left( \frac{\partial }{\partial t}\right) \right| _{t=0}}$ is the variation vector field with U(t, x) $=$ $u_t(x)$. In other words, it satisfies the Euler–Lagrange equation for the energy $E(\ )$, i.e., the harmonic map equation:

$$\begin{aligned} \sum _{i=1}^m \big (\nabla _{e_i}du\big ) (e_i)\ = \ 0 \ \ \ \ \ \Big (\,\text{ i.e., }\ \ \text {tr}\,\big (\nabla du\big )\ = \ 0 \ \Big )\end{aligned}$$

where $e_i$ $(i = 1,\ldots ,m)$ is a local orthonormal frame on M, and $\nabla$ denotes the connection on the bundle $TM \otimes f^{-1}TN$. A harmonic map u is unstable (resp. stable) if the second variation

$$\begin{aligned} (\delta ^2E)(u)(X)\ = \ \frac{d^2}{dt^2} E(u_t) \bigg |_{t=0} \end{aligned}$$

is negative (resp. nonnegative) for some (resp. any) variation $u_t$ with compact support.

In our situation such as M $=$ ${\mathbb {R}}^m\,-\,\{0\}$ and N $=$ ${\mathbb {S}}^n$ $\subset$ ${\mathbb {R}}^{n+1}$, we can write u as a map

$$\begin{aligned} x =(x_1,\ldots ,x_m)\ \ \rightarrow \ \ u(x) = \big (u_1(x),\ldots ,u_{n+1}(x)\big ). \end{aligned}$$

Take any function $\varphi$ $\in$ $\textrm{C}^\infty ({\mathbb {R}}^m\,-\,\{0\},\,{\mathbb {R}}^{n+1})$ with compact support. Consider the variation $u_t$ of u with the variation function $\varphi$:

$$\begin{aligned} u_t(x)\ = \ \frac{u(x) + t \varphi (x)}{\Vert u(x) + t \varphi (x)\Vert }. \end{aligned}$$

We can see

$$\begin{aligned} \left. \frac{\partial }{\partial t} u_t(x)\right| _{t=0}\ = \ \varphi (x) \ - \ \big (\varphi (x)\,\cdot \,u(x) \big ) u(x), \end{aligned}$$

where $\,\cdot \,$ denotes the inner product on ${\mathbb {R}}^{n+1}$. Then, we have the first variation

$$\begin{aligned} (\delta E)(u)(\varphi )= & {} \frac{d}{dt} E(u_t) \bigg |_{t=0} \nonumber \\= & {} \int _{{\mathbb {R}}^m\,-\,\{0\}} \Big ( \langle Du,\,D \varphi \rangle \ - \ \Vert Du\Vert \,u\,\cdot \,\varphi \Big )\,dx \end{aligned}$$

(2.1)

for any variation function $\varphi$ $\in$ $\textrm{C}^\infty ({\mathbb {R}}^m\,-\,\{0\},\,{\mathbb {R}}^{n+1})$ with compact support, where ${\displaystyle Du\ =\ \left( \frac{\partial u_j}{\partial x_i}\right) \!\!{\frac{}{}}_{ \begin{array}{l} {\scriptscriptstyle 1 \le i \le m } \\ {\scriptscriptstyle 1 \le j \le n+1 } \end{array} } }$ and $dx = dx_1 \ldots dx_m$. Then, we know

We see the second variation

$$\begin{aligned} (\delta ^2E)(u)(\varphi )= & {} \frac{d^2}{dt^2} E(u_t) \bigg |_{t=0} \nonumber \\= & {} 2 \int _{{\mathbb {R}}^m\,-\,\{0\}}\Big (\Vert D\varphi \Vert ^2\,-\,\Vert Du\Vert ^2 \Vert \varphi \Vert ^2\Big )\,dx \end{aligned}$$

(2.2)

for any variation function $\varphi$ $\in$ $\textrm{C}^\infty ({\mathbb {R}}^m\,-\,\{0\},\,{\mathbb {R}}^{n+1})$ with compact support satisfying the orthogonality condition

$$\begin{aligned} \varphi \,\cdot \,u\ =\ 0. \end{aligned}$$

For a harmonic map u, we have the definition of instability:

In the proof of Main Theorem, we give a special variation function $\varphi$ with compact support, given by (4.6) later. For this variation function $\varphi$, we calculate the second variation $(\delta ^2E)(u)(\varphi )$ and prove that it is negative.

3 Preliminaries

In this section, we give preliminary facts for our proof of Main Theorem. We introduce the following two basic quantities. They play an important role in our proofs. See [7] and [9] for their details.

Two quantities

$$\begin{aligned} \begin{array}{llcl} y \,=\, \big (y_i\big )_{1 \le i \le m}\quad \,\, : &{} \ \ y_i &{}\! = &{} \! {\displaystyle \frac{x_i}{\Vert x\Vert }} \\ &{} &{} &{} \\ a \,=\, \big (a_{ij}\big )_{1 \le i,j \le m} \,: &{} \ \ a_{ij} &{}\! = &{} \! {\displaystyle \delta _{ij} - \frac{x_ix_j}{\Vert x\Vert ^2} \ = \ \delta _{ij} - y_iy_j } \end{array} \end{aligned}$$

These two quantities $y_i$ and $a_{ij}$ satisfy the following conditions:

Lemma 1

• (1) ${\displaystyle \sum _{i=1}^m y_i^2\,= \, 1} \ \ \ \big (i.e., \Vert y\Vert = 1\,\big )$

• (2) ${\displaystyle \sum _{i=1}^m a_{ii} \,= \, m-1 } \ \ \ \big (i.e., \text {tr}\,a\,= \, m-1\,\big )$

• (3) ${\displaystyle D_iy_j \,= \, \frac{1}{\Vert x\Vert }a_{ij} } \ \ \ \Big (i.e., {\displaystyle Dy\,= \, \frac{1}{\Vert x\Vert }\,a}\,\Big )$

We omit the proof of Lemma 1, because Lemma 1 follows from the definitions of $y_i$ and $a_{ij}$ with simple calculations.

In this paper, we use the following properties of $u^{(n)}_{i_1\,\ldots \, i_n}$.

Lemma 2

$$\begin{aligned} \sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} u^{(n)}_{i_1\ldots i_n} \ = \ 0 \end{aligned}$$

(3.1)

for n $\ge$ 2.

Proof

We use the induction. We first prove (3.1) for n $=$ 2. Equality (1.2) for n $=$ 2 implies

$$\begin{aligned}{} & {} u^{(2)}_{i_1i_2} {\mathop {=}\limits ^{(1.2)}} C_{m,2} \left( y_{i_2} u^{(1)}_{i_1} \ - \ \frac{1}{m-1}\, \Vert x\Vert D_{i_2}u^{(1)}_{i_1} \right) \\{} & {} {\mathop {=}\limits ^{ (1.1),\,\text {Lemma}1 (3) }} C_{m,2} \left( y_{i_1} y_{i_2} \ - \ \frac{1}{m-1}\, a_{i_1i_2} \right) \end{aligned}$$

Then,

$$\begin{aligned} \sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} u^{(2)}_{i_1i_2}= & {} C_{m,2} \left( \Vert y\Vert ^2 \ - \ \frac{1}{m-1}\, \sum _{i = 1}^m a_{i i} \right) \\ {}&{\mathop {=}\limits ^{\text{ Lemma }\,1 (1),(2)}}&\ \ 0\end{aligned}$$

Thus, we have (3.1) for n $=$ 2.

We assume that (3.1) holds for n $=$ $k-1$ $(k \ge 3)$, i.e.,

$$\begin{aligned} \sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} u^{(k-1)}_{i_1\ldots i_{k-1}} \ = \ 0. \end{aligned}$$

(3.2)

Then for n $=$ k, we have from (1.2)

$$\begin{aligned}&{\sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} u^{(k)}_{i_1\,\ldots \, i_k} } \\ {}&{\mathop {=}\limits ^{(1.2)}} C_{m,k} \sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} \left( y_{i_k} u^{(k-1)}_{i_1\,\ldots \, i_{k-1}} \ - \ \frac{1}{k+m-3}\, \Vert x\Vert D_{i_k}u^{(k-1)}_{i_1\,\ldots \, i_{k-1}} \right) \\ {}&= C_{m,k} \left\{ y_{i_k}\! \sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} u^{(k-1)}_{i_1\,\ldots \, i_{k-1}} \ - \ \frac{1}{k+m-3}\, \Vert x\Vert D_{i_k} \left( \sum _{i_1,\,i_2 = 1}^m \delta _{i_1 i_2} u^{(k-1)}_{i_1\,\ldots \, i_{k-1}} \right) \right\} \\ {}&{\mathop {=}\limits ^{(3.2)}} \quad 0. \end{aligned}$$

by the induction assumption (3.2). We have (3.1) for n $=$ k. Thus, (3.1) holds for any n $\ge$ 2. $\square$

4 Instability of u

In this section, we prove the following result on the instability of u.

Main Theorem. Let $m \ge 3$ and $n \ge 2$. For n $\ge$ ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$, the map $u^{(n)}$ is unstable as a harmonic map.

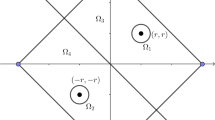
Let $B_r$ denotes the open ball of radius r in ${\mathbb {R}}^m$ centered at the origin:

$$\begin{aligned} B_r\ = \ \big \{\ x \in {\mathbb {R}}^m\ |\ \,\Vert x\Vert \ < \ r\ \big \}. \end{aligned}$$

Take positive real numbers a, b, c and d satisfying a < b < c < d. Let $\eta _{_{\scriptstyle 0}}(x)$ be a Lipschitz continuous function on ${\mathbb {R}}^m\,-\,\{0\}$ given by

$$\begin{aligned} \eta _{_{\scriptstyle 0}}(x)\ = \ \left\{ \begin{array}{ccl} 0 &{} \text{ on } &{} B_{a}\,-\,\{0\} \\ {} &{} &{} \\ {\displaystyle \frac{\Vert x\Vert - a}{(b-a)}} &{} \text{ on } &{}{} B_{b}\,-\,B_{a} \\ {} &{} &{} \\ 1 &{} \text{ on } &{} B_{c}\,-\,B_{b} \\ {} &{} &{} \\ {\displaystyle \frac{d-\Vert x\Vert }{d-c} } &{} \text{ on } &{} B_{d}\,-\,B_{c} \\ {} &{} &{} \\ 0 &{}{} \text{ on } &{} {\mathbb {R}}^m\,-\,B_{d} \end{array} \right. \end{aligned}$$

(4.1)

Take a sufficiently small positive number $\varepsilon$ which is determined later. Let $\eta (x)$ be a smooth cutoff function, approximating $\eta _{_{\scriptstyle 0}}(x)$, satisfying the following four conditions:

$$\begin{aligned}{} & {} \eta (x)\ \left\{ \begin{array}{lcc} = 0 &{} \text {on} &{} B_{a}\,-\,\{0\} \\ \in [0,\,1] &{} \text {on} &{} B_{b}\,-\,B_{a} \\ = 1 &{} \text {on} &{} B_{c}\,-\,B_{b} \\ \in [0,\,1] &{} \text {on} &{} B_{d}\,-\,B_{c} \\ = 0 &{} \text {on} &{} {\mathbb {R}}^m\,-\,B_{d} \end{array} \right. \end{aligned}$$

(4.2)

$$\begin{aligned}{} & {} |\eta (x) - \eta _{_{\scriptstyle 0}}(x)|\ < \ \varepsilon \ \ \text {for}\ \forall x \in {\mathbb {R}}^m\,-\,\{0\} \end{aligned}$$

(4.3)

$$\begin{aligned}{} & {} \Vert D \eta \Vert \ \le \ \frac{1+\varepsilon }{(b-a)} \ \ \ \text {on}\ \ B_{b}\,-\,B_{a} \end{aligned}$$

(4.4)

$$\begin{aligned}{} & {} \Vert D \eta \Vert \ \le \ \frac{1+\varepsilon }{(d-c)} \ \ \ \text {on}\ \ B_{d}\,-\,B_{c}. \end{aligned}$$

(4.5)

Note that the support of $\eta (x)$ is compact since $\eta (x)$ $=$ 0 outside $B_{d}\,-\,B_{a}$.

For simplicity, we set u  :  $=$ $u^{(n)}$. Take the variation function $\varphi$ with compact support, for $n \ge 2$, defined by

$$\begin{aligned} \varphi _{i_1\ldots i_n}(x)\ \ = \ \left\{ \begin{array}{ll} \eta (x)\,\delta _{i_1 i_2} &{} (n = 2) \\ \eta (x)\,\delta _{i_1 i_2} y_{i_3}\ldots y_{i_n} &{} (n \ge 3) \end{array} \right. \end{aligned}$$

(4.6)

for $x \in {\mathbb {R}}^m\,-\,\{0\}$. Note that the condition “$n \ge 2$” is necessary for this variation $\varphi$ $=$ $(\varphi _{i_1 \ldots i_n})_{_{1 \le i_1,\ldots ,i_n \le m}}$, since $\delta _{i_1 i_2}$ in the definition of $\varphi _{i_1\,\ldots \,i_n}$ requires two indices $i_1$ and $i_2$.

We can easily check the following three properties:

Lemma 3

• (1) ${\displaystyle \varphi \,\cdot \,u \ = \ 0 }$

• (2) ${\displaystyle \Vert \varphi \Vert ^2 \ = \ m \eta ^2 }$

• (3) ${\displaystyle \Vert D\varphi \Vert ^2 \ = \ m \Vert D \eta \Vert ^2 }$

Proof

• (1:) We have

  $$\begin{aligned} \varphi \,\cdot \,u= & {} \sum _{i_1,\,\ldots \, ,\,i_n=1}^m \varphi _{i_1\,\ldots \, i_n} u_{i_1\,\ldots \, i_n}^{(n)} \\ {}&{\mathop {=}\limits ^{(4.6)}}&\left\{ \begin{array}{ll} {\displaystyle \eta (x)\,\sum _{i_1,\,i_2=1}^m \delta _{i_1 i_2}u^{(2)}_{i_1 i_2} } &{}{} (n = 2) \\ {\displaystyle \eta (x)\,\sum _{i_3,\,\ldots \,,\,i_n=1}^m \left( \sum _{i_1,\,i_2=1}^m \delta _{i_1 i_2} u^{(n)}_{i_1\,\ldots \, i_n}\right) y_{i_3}\ldots y_{i_n} } &{}{} (n \ge 3) \end{array} \right. \\ {}&{\mathop {=}\limits ^{ \scriptscriptstyle \text{ Lemma }\,2 }}&\ \ 0\end{aligned}$$

• (2:) We get

  $$\begin{aligned} \Vert \varphi \Vert ^2= & {} \sum _{i_1,\,\ldots \, ,\,i_n=1}^m \big (\varphi _{i_1,\,\ldots \, i_n}\big )^2 \\ {}&{\mathop {=}\limits ^{(4.6)}}&\left\{ \begin{array}{ll} {\displaystyle \eta ^2 \sum _{i_1,\,i_2=1}^m \delta _{i_1 i_2}^2\, } &{}{} (n = 2) \\ {\displaystyle \eta ^2 \sum _{i_1,\,i_2=1}^m \delta _{i_1 i_2}^2\, \sum _{i_3=1}^m y_{i_3}^2\ldots \sum _{i_n=1}^m y_{i_n}^2 } &{}{} (n \ge 3) \end{array} \right. \\ {}&{\mathop {=}\limits ^{\scriptscriptstyle \text{ Lemma }\,1(1)}}&\ \ m \eta ^2 \nonumber \end{aligned}$$

• (3:) We see

  $$\begin{aligned}{} & {} \Vert D \varphi \Vert ^2 \,\, {\mathop {=}\limits ^{(4.6)}} \left\{ \begin{array}{ll} {\displaystyle \Vert D \eta \Vert ^2 \sum _{i_1,\,i_2=1}^m \delta _{i_1 i_2}^2\, } &{} (n = 2) \\ {\displaystyle \Vert D \eta \Vert ^2 \sum _{i_1,\,i_2=1}^m \delta _{i_1 i_2}^2\, \sum _{i_3=1}^m y_{i_3}^2\ldots \sum _{i_n=1}^m y_{i_n}^2 } &{} (n \ge 3) \end{array} \right. \\{} & {} \quad \quad \quad \; {\mathop {=}\limits ^{\scriptscriptstyle \text {Lemma}\,1(1)}} m \Vert D \eta \Vert ^2 \end{aligned}$$

Thus, we have Lemma 3. $\square$

By Theorem A (3), we have

$$\begin{aligned} \Vert Du\Vert ^2= & {} \frac{n(n+m-2)}{\Vert x\Vert ^2}. \end{aligned}$$

(4.7)

Take the variation

$$\begin{aligned} u_t(x)\ = \ \frac{u(x) + t \varphi (x)}{\Vert u(x) + t \varphi (x)\Vert }. \end{aligned}$$

The support of this variation is compact, since it is contained in the closure of $B_{d}\,-\,B_{a}$. Then, we have the second variation

$$\begin{aligned}&{ (\delta ^2E)(u)(\varphi ) \ = \ \frac{d^2}{dt^2} E(u_t) \bigg |_{t=0} } \nonumber \\&{\mathop {=}\limits ^{{\mathop {\text{ Lemma }\,3\,(1) }\limits ^{\scriptstyle (2.2)\, \text{ with }\ \ \ \ \ \ }} }} 2 \int _{{\mathbb {R}}^m\,-\,\{0\}}\Big (\Vert D\varphi \Vert ^2\,-\,\Vert Du\Vert ^2 \Vert \varphi \Vert ^2\Big )\,dx_1\ldots dx_m. \nonumber \\&{\mathop {=}\limits ^{ \text{ Lemma }\,3\,(2),(3) }} 2m \int _{{\mathbb {R}}^m\,-\,\{0\}}\Big (\Vert D\eta \Vert ^2\,-\,\frac{n(n+m-2)}{\Vert x\Vert ^2} \,\eta ^2 \Big )\,dx_1\ldots dx_m \nonumber \\&\qquad \quad \!\! = 2m \int _{B_{b}\,-\,B_{a}}\Big (\Vert D\eta \Vert ^2\,-\,\frac{n(n+m-2)}{\Vert x\Vert ^2} \,\eta ^2 \Big )\,dx_1\ldots dx_m \nonumber \\&\qquad \qquad \ + \ 2m \int _{B_{c}\,-\,B_{b}}\Big (\Vert D\eta \Vert ^2\,-\,\frac{n(n+m-2)}{\Vert x\Vert ^2} \,\eta ^2 \Big )\,dx_1\ldots dx_m \nonumber \\&\qquad \qquad \ + \ 2m \int _{B_{d}\,-\,B_{c}}\Big (\Vert D\eta \Vert ^2\,-\,\frac{n(n+m-2)}{\Vert x\Vert ^2} \,\eta ^2 \Big )\,dx_1\ldots dx_m \nonumber \\ {}&\qquad \quad \!\! =\ : \text {I}_1 \ + \ \text {I}_2 \ + \ \text {I}_3. \end{aligned}$$

(4.8)

Since $\eta = 1$ on $B_{c}\,-\,B_{b}$, we have

$$\begin{aligned}{} & {} \textrm{I}_2 {\mathop {=}\limits ^{(4.2)}} \quad -\ 2m \int _{B_{c}\,-\,B_{b}} \frac{n(n+m-2)}{\Vert x\Vert ^2}\,dx_1\ldots dx_m \ <\ 0. \end{aligned}$$

(4.9)

To estimate $\textrm{I}_1$, we note

$$\begin{aligned} \eta (x)^2 \ \ge \ \big (\max \,\{\eta _{_{\scriptstyle 0}}(x)\,-\,\varepsilon ,\,0\}\big )^2 \end{aligned}$$

(4.10)

since $\eta (x)$ $\ge$ $\eta _{_{\scriptstyle 0}}(x)\,-\,\varepsilon$ by (4.3). On $B_{b}\,-\,B_{a}$, we see

$$\begin{aligned} \eta _{_{\scriptstyle 0}}(x)\ \ge \ \varepsilon \ \ \ \Leftrightarrow \ \ \ \frac{\Vert x\Vert - a}{b - a}\ \ge \ \varepsilon \ \ \ \Leftrightarrow \ \ \ \Vert x\Vert \ \ge \ a + \varepsilon (b - a) \end{aligned}$$

and hence

$$\begin{aligned}{} & {} \eta _{_{\scriptstyle 0}}(x)\ \ge \ \varepsilon \,\,\, \text {on} \,\,\, B_{b}\,-\,B_{( a + \varepsilon (b - a))} \end{aligned}$$

(4.11)

$$\begin{aligned}{} & {} \eta _{_{\scriptstyle 0}}(x)\ \le \ \varepsilon \,\,\,\text {on} \,\,\, B_{( a + \varepsilon (b - a))}\,-\,B_{a}. \end{aligned}$$

(4.12)

Thus from (4.10), (4.11) and (4.12), we have

$$\begin{aligned} {}&{} \eta (x)^2 \ \ge \ (\eta _{_{\scriptstyle 0}}(x)\,-\,\varepsilon )^2 \ \ \ \text{ on } \ \ B_{b}\,-\,B_{( a + \varepsilon (b - a))} \end{aligned}$$

(4.13)

$$\begin{aligned} {}&{} \eta (x)^2 \ \ge \ (\eta _{_{\scriptstyle 0}}(x)\,-\,\varepsilon )^2 \ \ \ \text{ on } \ \ B_{b}\,-\,B_{( a + \varepsilon (b - a))} \end{aligned}$$

(4.14)

Then, we have

$$\begin{aligned} \text {I}_1 {\mathop {\le }\limits ^{(4.4)}}&2m \int _{B_{b}\,-\,B_{a}}\frac{ (1+\varepsilon )^2}{(b-a)^2} \,dx_1\ldots dx_m - 2m \int _{B_{b}\,-\,B_{a}} \frac{n(n+m-2)}{\Vert x\Vert ^2}\,\eta ^2\,dx_1\ldots dx_m \nonumber \\ {\mathop {\le }\limits ^{(4.13),(4.14)}}&\frac{2m (1+\varepsilon )^2}{(b-a)^2} \int _{B_{b}\,-\,B_{a}}\,dx_1\ldots dx_m \nonumber \\&- \ 2nm(n+m-2) \int _{B_{b}\,-\,B_{( a + \varepsilon (b - a))}}\ \frac{1}{\Vert x\Vert ^2}\,(\eta _0 - \varepsilon )^2\,dx_1\ldots dx_m \nonumber \\ {\mathop {=}\limits ^{(4.1)}}&\frac{2m (1+\varepsilon )^2}{(b-a)^2} \int _{B_{b}\,-\,B_{a}}\,dx_1\ldots dx_m \nonumber \\&- \ 2nm(n+m-2) \int _{B_{b}\,-\,B_{( a + \varepsilon (b - a))} }\ \frac{1}{\Vert x\Vert ^2}\left( \frac{\Vert x\Vert -a}{b-a} - \varepsilon \right) ^2 \,dx_1\ldots dx_m \nonumber \\ =&\frac{2m (1+\varepsilon )^2}{(b-a)^2} \int _{B_{b}\,-\,B_{a}}\,dx_1\ldots dx_m \nonumber \\&- \frac{2nm(n\!+\!m\!-\!2)}{(b-a)^2} \int _{B_{b}\,-\,B_{( a + \varepsilon (b - a))} } \frac{1}{\Vert x\Vert ^2}\Big (\Vert x\Vert -\! \big (a \!+\! \varepsilon (b - a)\big ) \Big )^2 \,dx_1\ldots dx_m\nonumber \\ \end{aligned}$$

(4.15)

Using the polar coordinate in ${\mathbb {R}}^m\,-\,\{0\}$, we have

$$\begin{aligned}&{ \text{ the } \text{ right } \text{ hand } \text{ side } \text{ of } (4.15)} \nonumber \\ {}= & {} \frac{2m (1+\varepsilon )^2}{(b-a)^2} \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \int _{a}^{b}\rho ^{m-1}d\rho \nonumber \\ {}{} & {} \qquad - \ \frac{2nm(n+m-2)}{(b-a)^2} \text {Vol}\,({\mathbb {S}}^{m-1})\nonumber \\ {}{} & {} \qquad \qquad \times \int _{( a + \varepsilon (b - a))}^{b} \frac{1}{\rho ^2} \Big (\rho \,-\,\big ( a + \varepsilon (b - a)\big ) \Big )^2 \,\rho ^{m-1}d\rho \nonumber \\ {}= & {} \frac{2m }{(b-a)^2} \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \Bigg [ (1 + \varepsilon )^2 \int _{a}^{b}\rho ^{m-1}d\rho \nonumber \\ {}{} & {} \qquad - \ n(n+m-2)\, \bigg \{ \int _{( a + \varepsilon (b - a))}^{b} \rho ^{m-1}d\rho \nonumber \\ {}{} & {} \qquad \qquad \ \ - \ 2\big ( a + \varepsilon (b - a)\big ) \int _{( a + \varepsilon (b - a))}^{b} \rho ^{m-2}d\rho \nonumber \\ {}{} & {} \qquad \qquad \qquad \ \ + \ \big ( a + \varepsilon (b - a)\big )^2 \int _{( a + \varepsilon (b - a))}^{b} \rho ^{m-3}d\rho \bigg \} \Bigg ] \nonumber \\ {}= & {} \frac{2m }{(b-a)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \Bigg [ \frac{(1+\varepsilon )^2}{m}(b^m-a^m) \nonumber \\ {}{} & {} \quad -\,n(n+m-2) \bigg \{ \frac{1}{m}\Big (b^m - \big ( a + \varepsilon (b - a)\big )^m\Big ) \nonumber \\ {}{} & {} \qquad \ - \ \frac{2}{m-1}\,\big ( a + \varepsilon (b - a)\big )\,\Big (b^{m-1}-\big ( a + \varepsilon (b - a)\big )^{m-1}\Big ) \nonumber \\ {}{} & {} \qquad \qquad \ + \ \frac{1}{m-2}\,\big ( a + \varepsilon (b - a)\big )^2\,\Big (b^{m-2}-\big ( a + \varepsilon (b - a)\big )^{m-2}\Big )\, \bigg \} \Bigg ] \nonumber \\ {}= & {} \frac{2m}{(b-a)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \Bigg [ \frac{ (1+\varepsilon )^2}{m}(b^m-a^m) \nonumber \\ {}{} & {} \qquad \ - \ n(n+m-2)\, \bigg \{ \frac{1}{m}\,b^2 \ - \ \frac{2}{m-1}\,b \big ( a + \varepsilon (b - a)\big ) \nonumber \\ {}{} & {} \qquad \qquad \qquad \qquad \qquad \qquad \qquad \ + \ \frac{1}{m-2}\,\big ( a + \varepsilon (b - a)\big )^2 \bigg \}\, b^{m-2} \nonumber \\ {}{} & {} \qquad \ + \ n(n+m-2)\, \left( \frac{1}{m} \,-\, \frac{2}{m-1} \,+\, \frac{1}{m-2} \right) \big ( a + \varepsilon (b - a)\big )^m \Bigg ] \nonumber \\ {}&{\mathop {=}\limits ^{ {\mathop {\scriptscriptstyle \text{ for }\,A=b\,\text{ and } \,B=a+\varepsilon (b-a) }\limits ^{ {\mathop { \text{ which } \text{ is } \text{ given } \text{ later, } }\limits ^{ \text{ Lemma }\,4\,(1)\,\text{ and }\,(2), }} }} }}&\ \ \ \ \ \ \frac{2m }{(b-a)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\,\left[ \frac{(1+\varepsilon )^2}{m}(b^m-a^m) \right. \nonumber \\ {}{} & {} \qquad \ - \ \frac{n(n+m-2)}{m(m-1)(m-2)}\, \bigg \{ (1-\varepsilon )^2 m(m-1) (b-a)^2 \nonumber \\ {}{} & {} \qquad \qquad \ - \ 2(1 - \varepsilon ) m b (b-a) \ + \ 2b^2 \bigg \}\, b^{m-2} \nonumber \\ {}{} & {} \qquad \qquad \qquad \left. \ + \ \frac{2n(n+m-2)}{m(m-1)(m-2)}\, \big ( a + \varepsilon (b - a)\big )^m \right] . \end{aligned}$$

(4.16)

In the last equality, we use Lemma 4 (1) and (2), given later, for A $=$ b and B $=$ $a+\varepsilon (b-a)$. Thus from (4.15) and (4.16), we have

$$\begin{aligned} {}&{} \text {I}_1 \ \le \ \frac{2m }{(b-a)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \left[ \frac{(1+\varepsilon )^2}{m}(b^m-a^m) \right. \nonumber \\ {}&{} \quad \,-\,\frac{n(n+m-2)}{m(m-1)(m-2)}\, \bigg \{ (1-\varepsilon )^2 m(m-1) (b-a)^2 - \ 2(1 - \varepsilon ) m b (b-a) \ + \ 2b^2 \bigg \}\, b^{m-2} \nonumber \\{}&{} \qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \left. \ + \ \frac{2n(n+m-2)}{m(m-1)(m-2)}\, \big ( a + \varepsilon (b - a)\big )^m \right] . \end{aligned}$$

(4.17)

Take a large positive numbers $\alpha$ and we set b $=$ $(\alpha + 1) a$. We know $(\alpha + 1)^m \ = \ \alpha ^m \,+\, O(\alpha ^{m-1})$, where $O(\ )$ denotes Landau’s symbol, i.e., $O(\alpha ^\ell )$ is a term satisfying ${\displaystyle \frac{O(\alpha ^{\ell })}{\alpha ^{\ell }}}$ is bounded as $\alpha$ $\rightarrow$ $\infty$. Then, (4.17) implies

$$\begin{aligned} \text {I}_1\le & {} 2 m\, \text {Vol}\,({\mathbb {S}}^{m-1}) \Bigg [ \frac{(1+\varepsilon )^2}{m}\,\frac{(\alpha + 1)^m - 1}{\alpha ^2}\,a^{m-2} \nonumber \\ {}{} & {} \qquad \,-\,\frac{n(n+m-2)}{m(m-1)(m-2)}\, \bigg \{ (1-\varepsilon )^2 m(m-1) \nonumber \\ {}{} & {} \qquad \qquad \qquad \ - \ 2(1 - \varepsilon ) m \,\frac{\alpha + 1}{\alpha } \ + \ \frac{2(\alpha + 1)^2}{\alpha ^2} \bigg \}\,(\alpha + 1)^{m-2}\,a^{m-2} \nonumber \\ {}{} & {} \qquad \qquad \qquad \qquad \qquad \qquad \ \ \ \ + \ \frac{2n(n+m-2)}{m(m-1)(m-2)}\, \frac{\big ( 1 + \varepsilon \alpha \big )^m}{\alpha ^2}\,a^{m-2} \Bigg ] \nonumber \\ {}= & {} 2m\, \text {Vol}\,({\mathbb {S}}^{m-1})\, a^{m-2} \Bigg [ \frac{(1+\varepsilon )^2}{m}\,\alpha ^{m-2} \nonumber \\ {}{} & {} \qquad \,-\,\frac{n(n+m-2)}{m(m-1)(m-2)}\, \bigg \{ (1-\varepsilon )^2 m(m-1) \ - \ 2(1 - \varepsilon ) m \ + \ 2\bigg \}\,\alpha ^{m-2} \nonumber \\ {}{} & {} \qquad \qquad \qquad \qquad \quad \ + \ \frac{2n(n+m-2)}{m(m-1)(m-2)}\, \frac{\big ( 1 + \varepsilon \alpha \big )^m}{\alpha ^2} \ + \ O\big (\alpha ^{m-3}\big )\, \Bigg ]. \end{aligned}$$

(4.18)

Take a sufficiently small positive number $\varepsilon$ such that

$$\begin{aligned} \varepsilon \ <\ \frac{1}{\alpha }. \end{aligned}$$

(4.19)

Then, $\varepsilon \alpha ^{m-2}$ $=$ $O\big (\alpha ^{m-3}\big )$ and $\varepsilon ^2 \alpha ^{m-2}$ $=$ $O\big (\alpha ^{m-4}\big )$, we see

$$\begin{aligned}{} & {} \frac{(1+\varepsilon )^2}{m}\,\alpha ^{m-2} \ = \ \frac{1}{m}\,\alpha ^{m-2} \ + \ O\big (\alpha ^{m-3}\big ), \\{} & {} \bigg \{ (1 - \varepsilon )^2 m (m-1) \ - \ 2(1 - \varepsilon ) m \ + \ 2 \bigg \}\,\alpha ^{m-2} \\= & {} \bigg \{ m (m-1) \ - \ 2m \ + \ 2 \bigg \}\,\alpha ^{m-2} \ + \ O\big (\alpha ^{m-3}\big ) \\= & {} (m-1)(m-2)\,\alpha ^{m-2} \ + \ O\big (\alpha ^{m-3}\big ) \end{aligned}$$

and

$$\begin{aligned} \frac{2n(n+m-2)}{m(m-1)(m-2)}\, \frac{\big ( 1 + \varepsilon \alpha \big )^m}{\alpha ^2}= & {} O\big (\alpha ^{-2}\big ). \end{aligned}$$

Then for sufficiently large $\alpha$, we have

$$\begin{aligned} \text {I}_1\\le & {} \ 2m\, \text {Vol}\,({\mathbb {S}}^{m-1})\, a^{m-2} \Bigg \{ \left( \frac{1}{m}\ - \ \frac{n(n+m-2)}{m} \right) \,\alpha ^{m-2} \ + \ O\big (\alpha ^{m-3}\big )\, \Bigg \} \nonumber \\ {}= & {} \ -\,2 \text {Vol}\,({\mathbb {S}}^{m-1})\, a^{m-2} \Bigg \{ \bigg ( (n^2 - 1)\ + \ (m-2)n \bigg )\,\alpha ^{m-2} \ + \ O\big (\alpha ^{m-3}\big )\, \Bigg \} \nonumber \\ {}< & {} \ 0,\end{aligned}$$

(4.20)

since the assumptions m $\ge$ 3 and n $\ge$ 2 imply $(n^2-1)$ $\,+\,$ $(m-2)n$ $\ge$ 5 > 0.

Similarly on $B_{d}\,-\,B_{c}$, we see

$$\begin{aligned} \eta _{_{\scriptstyle 0}}(x)\ \ge \ \varepsilon \ \ \ \Leftrightarrow \ \ \ \frac{d - \Vert x\Vert }{d-c}\ \ge \ \varepsilon \ \ \ \Leftrightarrow \ \ \ \Vert x\Vert \ \le \ d - \varepsilon (d-c) \end{aligned}$$

and hence

$$\begin{aligned} {}&{} \eta (x)^2 \ \ge \ (\eta _{_{\scriptstyle 0}}(x)\,-\,\varepsilon )^2 \ \ \ \text{ on } \ \ B_{( d - \varepsilon (d-c))}\,-\,B_{c} \end{aligned}$$

(4.21)

$$\begin{aligned} {}&{} \eta (x)^2 \ \ge \ 0 \ \ \qquad \qquad \qquad \ \ \, \text{ on }\ \ B_{d} \,-\,B_{( d - \varepsilon (d-c))}. \end{aligned}$$

(4.22)

and we have

$$\begin{aligned} \text {I}_3&{\mathop {\le }\limits ^{ {\mathop {\scriptstyle \text{ and }\,(4.22) }\limits ^{\scriptstyle (4.5),(4.21) }} }}&2m \int _{B_{d}\,-\,B_{c}} \frac{(1+\varepsilon )^2}{(d-c)^2} \,dx_1\ldots dx_m \nonumber \\ {}{} & {} \qquad - \ 2m \int _{B_{( d - \varepsilon (d-c))}\ \ \ \,-\,B_{c}} \frac{n(n+m-2)}{\Vert x\Vert ^2}\, (\eta _{_{\scriptstyle 0}}\,-\,\varepsilon )^2 dx_1\ldots dx_m \nonumber \\ {}&{\mathop {=}\limits ^{(4.1)}}&\frac{2m (1+\varepsilon )^2}{(d-c)^2}\, \int _{B_{d}\,-\,B_{c}}\,dx_1\ldots dx_m \nonumber \\ {}{} & {} \qquad - \ 2nm(n+m-2)\, \int _{B_{( d - \varepsilon (d-c))}\ \ \ \,-\,B_{c}} \frac{1}{\Vert x\Vert ^2} \left( \frac{d - \Vert x\Vert }{d-c}\,-\,\varepsilon \right) ^2 \,dx_1\ldots dx_m \nonumber \\ {}= & {} \frac{2m (1+\varepsilon )^2}{(d-c)^2}\, \int _{B_{d}\,-\,B_{c}}\,dx_1\ldots dx_m \nonumber \\ {}{} & {} \qquad - \ \frac{2nm(n+m-2)}{(d-c)^2}\nonumber \\ {}{} & {} \qquad \qquad \times \int _{B_{( d - \varepsilon (d-c))}\ \ \ -\,B_{c}} \frac{1}{\Vert x\Vert ^2} \Big (\big ( d - \varepsilon (d - c)\big ) \,-\, \Vert x\Vert \Big )^2 \,dx_1\ldots dx_m \nonumber \\ {}= & {} \frac{2m (1+\varepsilon )^2}{(d-c)^2} \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \int _{c}^{d}\rho ^{m-1}d\rho \nonumber \\ {}{} & {} \qquad - \ \frac{2nm(n+m-2)}{(d-c)^2} \text {Vol}\,({\mathbb {S}}^{m-1}) \nonumber \\ {}{} & {} \qquad \qquad \times \int _{c}^{( d - \varepsilon (d - c))} \frac{1}{\rho ^2}\Big (\big ( d - \varepsilon (d - c)\big ) \,-\, \rho \Big )^2 \,\rho ^{m-1}d\rho \nonumber \\ {}= & {} \frac{2m}{(d-c)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\, \Bigg [ \frac{(1+\varepsilon )^2}{m}(d^m-c^m) \nonumber \\ {}{} & {} \qquad - \ n(n+m-2)\left\{ \frac{1}{m}\Big (\big ( d - \varepsilon (d-c)\big )^m - c^m\Big ) \right. \nonumber \\ {}{} & {} \qquad \qquad - \ \frac{2}{m-1}\Big (\big ( d - \varepsilon (d-c)\big )^{m-1}-c^{m-1}\Big )\,\big (d - \varepsilon (d-c)\big ) \nonumber \\ {}{} & {} \qquad \qquad \qquad \left. \left. + \ \frac{1}{m-2}\Big (\big ( d - \varepsilon (d-c)\big )^{m-2}-c^{m-2}\Big )\,\big (d - \varepsilon (d-c)\big )^2 \right\} \right] \nonumber \\ {}= & {} \frac{2m}{(d-c)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\,\Bigg [ \frac{ (1+\varepsilon )^2}{m}(d^m-c^m) \nonumber \\ {}{} & {} \qquad - \ n(n+m-2)\, \bigg ( \frac{1}{m} \,-\, \frac{2}{m-1} \,+\, \frac{1}{m-2} \bigg ) \big ( d - \varepsilon (d - c)\big )^m \nonumber \\ {}{} & {} \qquad \qquad + \ n(n+m-2)\, \bigg \{ \frac{1}{m}\,c^2 \ - \ \frac{2}{m-1}\,c \big ( d - \varepsilon (d - c)\big ) \nonumber \\ {}{} & {} \qquad \qquad \qquad + \ \frac{1}{m-2}\,\big ( d - \varepsilon (d - c)\big )^2 \bigg \}\, c^{m-2}\, \Bigg ] \nonumber \\ {}&{\mathop {=}\limits ^{ {\mathop {\scriptscriptstyle \text{ for }\,A=c\,\text{ and }\,B=d-\varepsilon (d-c) }\limits ^{ {\mathop { \text{ which } \text{ is } \text{ given } \text{ later, } }\limits ^{ \text{ Lemma }4\,(1)\,\text{ and }\,(2), }} }} }}&\ \ \ \ \frac{2m}{(d-c)^2 } \, \text {Vol}\,({\mathbb {S}}^{m-1})\,\Bigg [ \frac{(1 + \varepsilon )^2}{m}(d^m-c^m) \nonumber \\ {}{} & {} \qquad - \ \frac{2n(n+m-2)}{m(m-1)(m-2)}\, \big ( d - \varepsilon (d-c)\big )^m \nonumber \\ {}{} & {} \qquad \qquad \ + \ \frac{n(n+m-2)}{m(m-1)(m-2)}\, \bigg \{ (1 - \varepsilon )^2 m (m-1) (d-c)^2 \nonumber \\ {}{} & {} \qquad \qquad \qquad + \ 2(1 - \varepsilon ) m c (d-c) \ + \ 2c^2 \bigg \}\, c^{m-2}\, \Bigg ] \end{aligned}$$

(4.23)

Take a large positive number $\beta$ and let $\varepsilon$ be sufficiently small satisfying $\varepsilon$ < ${\displaystyle \frac{1}{\beta }}$. We set d $=$ $(\beta + 1) c$. Then, (4.23) implies

$$\begin{aligned} \text {I}_3 \ {}\le & {} \ 2m\, \text {Vol}\,({\mathbb {S}}^{m-1})\, c^{m-2} \Bigg \{ \frac{1}{m}\,\beta ^{m-2} \ - \ \frac{2n(n+m-2)}{m(m-1)(m-2)}\,\beta ^{m-2} \ + \ O\big (\beta ^{m-3}\big ) \bigg \} \\ {}= & {} \ -\ \frac{2}{(m-1)(m-2)}\,\text {Vol}\,({\mathbb {S}}^{m-1})\, c^{m-2} \\ {}{} & {} \qquad \times \,\Bigg \{ \bigg ( 2n^2\ + \ 2(m-2)n -(m-1)(m-2) \ \bigg )\,\beta ^{m-2} \ + \ O\big (\beta ^{m-3}\big ) \Bigg \}.\end{aligned}$$

Then using Lemma 5 mentioned later, we have

$$\begin{aligned} \textrm{I}_3\ <\ 0 \quad \text {for} \quad n\ \ge \ \frac{\sqrt{3}-1}{2}\,(m-1) \end{aligned}$$

(4.24)

for sufficiently large number $\beta$. Thus by (4.9), (4.20) and (4.24), we conclude

$$\begin{aligned} (\delta ^2E)(u)(\varphi )\ < \ 0 \end{aligned}$$

and we finish the proof of our Main Theorem. $\square$

We give here the following two lemmas which are used in the proof of Main Theorem. Lemma 4 is easy to prove and then we omit the proof. We give a proof of Lemma 5 only.

Lemma 4

• (1) ${\displaystyle \frac{1}{m}\ - \ \frac{2}{m-1}\ + \ \frac{1}{m-2} \ = \ \frac{2}{m(m-1)(m-2)} }$

• (2) ${\displaystyle \frac{1}{m}\,A^2\ - \ \frac{2}{m-1}\,AB\ + \ \frac{1}{m-2}\,B^2 }$ $\displaystyle \ = \ \frac{1}{m(m-1)(m-2)} \bigg \{ m (m-1) (A-B)^2 \ - \ 2mA(A-B) \ + \ 2A^2 \bigg \}$

Lemma 5

If x $\ge$ ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$, then we have

$$\begin{aligned} 2x^2\ + 2(m-2)x\ - \ (m-1)(m-2)\ >\ 0. \end{aligned}$$

Proof of Lemma 5

Let $\omega$ $=$ ${\displaystyle \frac{\sqrt{3}-1}{2}}$ and we note

$$\begin{aligned} 2 \omega ^2\ + \ 2\omega \ -\ 1\ = \ 0. \end{aligned}$$

(4.25)

Let

$$\begin{aligned} f(x)\ = \ 2x^2\ + 2(m-2)x\ - \ (m-1)(m-2) \end{aligned}$$

and then we have

$$\begin{aligned} f'(x)\ = \ 4x\ + \ 2(m-2)\ = \ 2(2x+m-2)\ >\ 0 \end{aligned}$$

for any x > 0. Therefore, f(x) is monotone increase on $\{x > 0\}$ and we have

$$\begin{aligned} f(x) \ \ge \ f\left( \frac{\sqrt{3}-1}{2}\,(m-1)\right) \end{aligned}$$

for any x $\ge$ ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$. The right hand side of this inequality is:

$$\begin{aligned} f\left( \frac{\sqrt{3}-1}{2}\,(m-1)\right)= & {} f\big (\,(m-1)\omega \big ) \\= & {} 2(m-1)^2 \omega ^2\,+\,2(m-1)(m-2)\omega \,-\,(m-1)(m-2) \\= & {} (m-1)(m-2)\big (2\omega ^2\,+\,2\omega \,-\,1\big ) \ + \ 2(m-1) \omega ^2 \\&{\mathop {=}\limits ^{(4.25)}}&2(m-1) \omega ^2 \ > \ 0. \end{aligned}$$

Thus, we have f(x) > 0. $\square$

At the end of this paper, we give two remarks on Main Theorem.

Remark 1

Though the map $u^{(n)}$ in Main Theorem has a singularity at x $=$ 0, it is a weakly harmonic map from ${\mathbb {R}}^m$ $(m \ge 3)$, where

Here, $\textrm{L}_{\text {loc}}^{1,2}\big ({\mathbb {R}}^m,\,{\mathbb {S}}^{m^n-1}\big )$ denotes the Sobolev space of ${\mathbb {S}}^{m^n-1}$-valued functions u on ${\mathbb {R}}^m$ such that both u and the weak derivative Du are in $\textrm{L}^2$ on any compact subset K of ${\mathbb {R}}^m$. The fact that $u^{(n)}$ is a weakly harmonic map from ${\mathbb {R}}^m$ $(m \ge 3)$ follows from the finiteness of the local energy near x $=$ 0, i.e.,

$$\begin{aligned} \int _{B_r}\Vert Du^{(n)}\Vert ^2\,dx \ = \ n(n+m-2)\textrm{Vol}\,({\mathbb {S}}^{m-1}) \int _0^r \rho ^{m-3}d\rho \ < \ \infty \quad (r > 0) \end{aligned}$$

for any m $\ge$ 3, by the condition (3) in Theorem A. Then, Main Theorem implies that $u^{(n)}$ is an unstable weakly harmonic map from ${\mathbb {R}}^m$. Furthermore rescaling radially, we can obtain an unstable weakly harmonic map $\tilde{u}^{(n)}$ from $B_1$. Indeed, we take a large radius R > 0 satisfying that the support of the variation function $\varphi$ in our proof is contained in $B_R$, and then we define

$$\begin{aligned} \tilde{u}^{(n)}\, \ B_1 \ \rightarrow \ {\mathbb {S}}^{m^n-1} \ \ \text {s.t.} \ \ \tilde{u}^{(n)}(x)= & {} u^{(n)}(Rx) \end{aligned}$$

which is an unstable weakly harmonic map.

Remark 2

As we have seen, our proof of Main Theorem needs only the quadratic inequality $2n^2$ $+$ $2(m-2)n$ − $(m-1)(m-2)$ $\ge$ 0 with respect to n in Lemma 5, and therefore, we may assume the weaker condition

$$\begin{aligned} n \ {}\ge & {} \ \frac{-\,(m-2)\ +\ \sqrt{(m-2)^2\,+\,2(m-1)(m-2)}}{2} \\ {}= & {} \ \frac{(m-1)(m-2)}{\sqrt{(m-2)(3m-4)}\ + \ m-2} \end{aligned}$$

in place of the assumption n $\ge$ ${\displaystyle \frac{\sqrt{3}-1}{2}\,(m-1)}$.