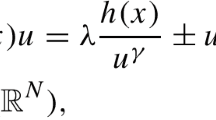Stability of singular solutions to the b-family of equations

Abstract

In this paper, we first construct some explicit solutions to the b-family of equations, which will become unbounded in a finite time. Then, we investigate the asymptotic stability of the aforementioned singular solutions of the b-family of equations in the Sobolev space $H^s$ with $s>\frac{7}{2}$. It is also interesting to point out that this stability highly depends on the values of parameter b, that is, $b\in (-1,2]$. The proof is based on the detailed analysis on the estimates of the perturbed solutions and the properties of the corresponding linear operators.

1 Introduction and main results

1.1 Introduction

In this paper, we consider the following b-family of equations (b-equation)

$$\begin{aligned} u_t-u_{xxt}+(b+1)uu_x-bu_xu_{xx}-uu_{xxx}=0, \end{aligned}$$

(1.1)

and the initial datum is given by

$$\begin{aligned} u(0,x)=u_0(x). \end{aligned}$$

(1.2)

Here b is a real parameter, and u(x, t) is a horizontal velocity. The b-equation was introduced originally by Degasperis, Holm and Hone [16, 17] (see also Holm and Staley [29, 30]) and can be derived as the family of asymptotically equivalent shallow water wave equations [18, 19]. Note that the b-equation can be rewritten in the following nonlocal form

$$\begin{aligned} u_t+uu_x+\partial _x(1-\partial _x^2)^{-1}\left( \frac{b}{2}u^2+\frac{3-b}{2}(u_x)^2\right) =0, \end{aligned}$$

(1.3)

where the nonlocal term represents a balance between the dispersion and nonlinearity. The equation (1.3) can be seen as a dispersive perturbation of the famous Burgers equation $u_t+uu_x=0$.

We note that if we take $b =2$ and $b=3$, then the b-equation (1.1) reduces into two integrable members, i.e., the Camassa–Holm (CH) equation [5] and the Degasperis-Procesi (DP) equation [18], respectively. Moreover, for any $b\in \mathbb {R}$, the b-equation (1.1) possesses the following peakon traveling wave solutions

$$\begin{aligned} u(x,t)=ce^{-|x-ct|}, \end{aligned}$$

where c is a constant. In fact, this equation possesses n-peakons like

$$\begin{aligned} u(x,t)=\sum _{j=1}^np_j(t)e^{-|x-q_j(t)|}, \end{aligned}$$

where the positions $q_j$ and the momenta $p_j$ satisfy a system of ODEs [35].

The well-posedness of (1.1) in the Sobolev space $H^s$ with $s>3/2$ has been proved extensively (see for instance Escher et al. [20], Escher and Yin [21], Zhang and Yin [37], Grayshan [24] and Himonas and Holliman [28]). For the ill-posedness of b-equation (1.1), Himonas et al. [27] showed that it is ill-posed in $H^s$ when $s<\frac{3}{2}$ and $b>1$ on both the torus and the line. On the other hand, whenever $b<1$, Novruzov [35] established the ill-posedness for (1.1) by constructing some peakon-antipeakon solutions. However, the results in [35] can hold by adding the b-equation an additional dispersion term $k(\text {sign}(u) (u-u_{xx})_x$. To the best of our knowledge, if one removes this term, the ill-posedness of the b-equation (1.1) is still not clear. For the remaining critical case $s=\frac{3}{2}$, Guo et al. pointed out that the b-equation with $1<b\le 3$ is ill-posed in the critical Sobolev space $H^{\frac{3}{2}}$ on both the torus and the line (see Remark 1.2 in [25]). Besides the above results, there is many other literatures about the well-posedness theory, traveling wave solutions, stability of the solution map, unique continuation and other analytic and geometric properties of the b-equation, CH-equation and DP-equation (see [3, 4, 6, 10,11,12] and the references therein). Here we would like to mention that there are also some interesting works on the Lipschitz metrics for nonlinear wave equations or Novikov equation and other shallow water wave equations [2, 7, 9].

Recently, Li et al. [33] studied the wave-breaking mechanism and dynamical behavior of solutions near the explicit self-similar singularity for the two component Camassa–Holm equations. In 2019, Li and Yan [34] considered the dynamical behavior of solutions near explicit self-similar singularity for a class of nonlinear shallow water models including the Camassa–Holm equation, the dispersive rod equation. They showed that the constructed explicit self-similar solutions for the Dullin-Gottwald-Holm equation, the Camassa–Holm equation and the dispersive rod equation are asymptotic stable, but for the Korteweg-de Vries equation and the Benjamin-Bona-Mahony equation being unstable. Gao and Chen [23] also studied the stability problem of special solutions for the Dullin-Gottwald-Holm equation. For the stability of solitary wave solutions to the shallow water wave equations, we refer the readers to the works [13,14,15, 26] and the references therein.

It is well-known that wave breaking phenomena often happens for shallow water wave equations. For example, the b-equation admits multipeakon solutions. Moreover, as pointed out most recently by Barnes and Hone [1] the b-family of equations admits the Burgers “ramp and cliffs" solutions for $-1<b<1$, in particular the following similarity ramp solution (see (1.19) in [1])

$$\begin{aligned} u(t,x)=\frac{x}{(b+1)t}. \end{aligned}$$

(1.4)

Beyond the ramp (1.4), the scaling similarity solutions of the b-equation are extensively investigated and are related to an autonomous third-order ODE [1]. We remark here that in Sect. 2, for our purpose we shall derive the following explicit singular solutions to the b-equation

$$\begin{aligned} \bar{u}(t,x)=\frac{1}{1+b}\cdot \frac{c-x}{T-t}, \end{aligned}$$

(1.5)

where $T>0, c$ are arbitrary constants. We mention that the work of Holm and Staley [29, 30] presented a numerical study of the solutions of b-equation (1.1) for different values of b. More precisely, they pointed out that there are three distinct parameter regimes separated by $b=1$ and $b=-1$, that is, peakon regime for $b>1$, ramp-cliff regime for $-1<b<1$ and lefton regime for $b<-1$. At the same time, there have been some recent literatures on the (spectral) stability/instability of the peakon solutions to the b-equation (1.1), see for example Charalampidis, Parker, Kevrekidis et al. [8], Lafortune and Pelinovsky [32]. However, it seems that the stability for ramp solutions (1.4), or (1.5) has not been proven before.

In this paper, we shall focus on the ramp solutions (1.5) to the b-equation and prove the asymptotic stability of these solutions by employing the Banach fixed point theorem and the analysis on the perturbed solutions. The most novelty is that our results depend on the parameter b in the equation (1.1). More precisely, the stability of the explicit solutions can persist only for $b\in (-1,2]$ including the corresponding results for the CH-equation. On the contrary, if $b\notin (-1,2]$, the stability for these explicit solutions remains unsolved.

1.2 Main results

We provide the stability of the above singular ramp solutions (1.5) for the b-equation (1.1).

Theorem 1.1

Assume that $-1<b\le 2, s>\frac{7}{2}$. Let $\varepsilon >0$ is a sufficiently small parameter. Then, the solution (2.4) of the b-equation (1.1) is asymptotically stable, that is, whenever the initial data $u_0(x)$ satisfies

$$\begin{aligned} \Vert u_0(\cdot )-\bar{u}(0,\cdot )\Vert _{H^{s+1}}<\varepsilon , \end{aligned}$$

(1.6)

then there exists a solution u of (1.1) such that

$$\begin{aligned} \lim _{t\rightarrow T^-}\Vert u(t,\cdot )-\bar{u}(\cdot )\Vert _{H^s}=0,\quad (t,x)\in (0,T)\times \mathbb {R}. \end{aligned}$$

Remark 1.1

When b = 2, the b-equation (1.1) becomes the celebrated Camassa–Holm equation. This means that the results in Theorem 1.2 also hold for the Camassa–Holm equation.

Remark 1.2

The condition (1.6) for the initial data implies that $H^{s+1}(\mathbb {R})$ norm of $u_0(x)-\frac{c-x}{(1+b)T}$ is sufficiently small and $u_0(x)$ approaches to the linear function $\frac{c-x}{(1+b)T}$ as $|x|\rightarrow \infty$. In addition, by the Sobolev embedding theorem [22] $H^s(\mathbb {R}^n)\hookrightarrow C^r(\mathbb {R}^n)$ for $s>r+\frac{n}{2}$, we know that $u_0(x)$ is actually smooth in $C^4(\mathbb {R})$.

In this paper, we denote the usual norms of Lebesgue space $L^2(\mathbb {R})$ and Sobolev space $H^s(\mathbb {R})$ by $\Vert \cdot \Vert _{L^2(\mathbb {R})}$ and $\Vert \cdot \Vert _{H^s(\mathbb {R})}$, respectively. For brevity, we often use the notations $L^2$ and $H^s$, instead of $L^2(\mathbb {R})$, $H^s(\mathbb {R})$. In the meantime, we use $*$ to represent the convolution. The symbol [A, B] denotes the commutator of two linear operators A, B. $D({\mathcal {L}})$ stands for the domain of the operator ${\mathcal {L}}$.

The rest of this paper is organized as follows. In Sect. 2, we construct the explicit singular solutions for the b-equation (1.1). Section 3 is devoted to studying the stability behavior of solutions near the derived explicit singular solutions and to proving Theorem 1.1.

2 Explicit solutions

In this section, we are going to find the following solutions

$$\begin{aligned} u(t,x)=\sum _{j=0}^na_j(t)x^j, \end{aligned}$$

where $a_j(t)\,(j=0,1,\cdots ,n)$ are to be determined. Substituting the above formula into (1.1) and letting $a_j=0 \,(j\ge 2)$ gives the following solutions

$$\begin{aligned} \bar{u}(t,x)=\frac{x+c_2}{(1+b)t-c_1}, \end{aligned}$$

(2.1)

where $c_1, c_2$ are arbitrary constants. Here we have used the homogeneous balance principle. Throughout this paper, we assume that

$$\begin{aligned} \frac{2-b}{1+b}\ge 0,\quad \text {i.e.,}\quad -1<b\le 2. \end{aligned}$$

(2.2)

Since we only have interest in the singular solutions, so we let $c_1=(1+b)T,c_2=-c$. Then, (2.1) becomes

$$\begin{aligned} \bar{u}(t,x)=\frac{1}{1+b}\cdot \frac{c-x}{T-t}, \end{aligned}$$

(2.3)

where $T>0, c$ are constants. Thus, as a conclusion, we obtain an existence result of solutions to the b-equation (1.1).

Theorem 2.1

Assume $T>0$ be the maximal existence time of the solution. Then, the b-equation has the following singular solutions

$$\begin{aligned} \bar{u}(t,x)=\frac{1}{1+b}\cdot \frac{c-x}{T-t}, \end{aligned}$$

(2.4)

where c is an arbitrary constant.

Remark 2.1

We know that

$$\begin{aligned} \partial _x\bar{u}(t,x)=-\frac{1}{1+b}\cdot \frac{1}{T-t}\rightarrow -\infty , \end{aligned}$$

as t tends to T from below. This implies the solutions (2.4) are indeed the singular solutions to the b-family of equations (1.1).

3 Proof of main results

In this section, we consider stability of the explicit solution (2.3) for the b-equation (1.1). Let

$$\begin{aligned} u(t,x)=v(t,x)+\bar{u}(t,x), \end{aligned}$$

(3.1)

where $\bar{u}(t,x)$ is given in (2.3). Substituting (3.1) into (1.1) leads to the following dissipative quasilinear equation with singular coefficients

$$\begin{aligned}{} & {} v_t-v_{xxt}+(b+1)vv_x-bv_xv_{xx}-vv_{xxx}\nonumber \\{} & {} \quad -\frac{1}{1+b}\cdot \frac{c-x}{T-t}v_{xxx}+\frac{b}{1+b}\cdot \frac{1}{T-t}v_{xx}+\frac{c-x}{T-t}v_x\nonumber \\{} & {} \quad -\frac{1}{T-t}v=0,\;\;\forall \;(t,x)\in (0,T)\times \mathbb {R}, \end{aligned}$$

(3.2)

and the initial condition is

$$\begin{aligned} v(0,x)=u_0(x)-\frac{1}{1+b}\cdot \frac{c-x}{T},\;\;\forall x\in \mathbb {R}. \end{aligned}$$

(3.3)

Due to the presence of singular terms in (3.2), we now introduce the coordinates in the form

$$\begin{aligned} \tau =-\log (T-t),\quad y=\frac{x}{T-t}. \end{aligned}$$

Then, we denote the solution $v(t,x)=\phi (\tau ,y)$. A direct computation shows that

$$\begin{aligned} v_t=e^{\tau }(\phi _{\tau }+y\phi _{y}), \quad v_x=e^{\tau }\phi _y,\quad v_{xx}=e^{2\tau }\phi _{yy}, \end{aligned}$$

and

$$\begin{aligned} v_{xxx}=e^{3\tau }\phi _{yyy},\quad v_{txx}=e^{3\tau }(\phi _{\tau yy}+2\phi _{yy}+y\phi _{yyy}). \end{aligned}$$

Based on the above identities, the equation (3.2) can be transformed to

$$\begin{aligned}{} & {} \left( \phi -e^{2\tau }\phi _{yy}\right) _{\tau }-ye^{2\tau }\phi _{yy}+\frac{1}{1+b}e^{2\tau }\left( y-ce^{\tau }\right) \phi _{yyy} -\phi \nonumber \\{} & {} \quad +\frac{b}{1+b}e^{2\tau }\phi _{yy}+(1+b)\phi \phi _y+ce^{\tau }\phi _y =e^{2\tau }\left( b\phi _y\phi _{yy}+\phi \phi _{yyy}\right) . \end{aligned}$$

(3.4)

Furthermore, we introduce the variables $z=ye^{-\tau }, \psi (\tau ,z)=e^{-\tau }\phi$. Noting that

$$\begin{aligned} \phi _{\tau }=e^{\tau }\psi +e^{\tau }\psi _{\tau }-ze^{\tau }\psi _z,\;\phi _y=\psi _z,\;\phi _{yy}=e^{-\tau }\psi _{zz},\; \phi _{yyy}=e^{-2\tau }\psi _{zzz}, \end{aligned}$$

and

$$\begin{aligned} \phi _{\tau y }=\psi _{\tau z}-z\psi _{zz},\; \phi _{\tau yy}=e^{-\tau }\left( \psi _{\tau zz}-\psi _{zz}-z\psi _{zzz}\right) , \end{aligned}$$

we can rewrite (3.4) as follows

$$\begin{aligned}{} & {} \psi _{\tau }-\psi _{\tau zz}-\frac{1}{1+b}\psi _{zz}-\frac{c+bz}{1+b}\psi _{zzz}+c\psi _z +(b+1)\psi \psi _z=b\psi _z\psi _{zz}+\psi \psi _{zzz}.\nonumber \\ \end{aligned}$$

(3.5)

Recall the operator $\Lambda =(1-\partial _z^2)^{1/2}$ and we know that $\Lambda ^{-2}f=p*f$ for any $f\in L^2(\mathbb {R})$, where $p(z)=\frac{1}{2} e^{-|z|}$ is the fundamental solution to the equation $(1-\partial _z^2)p=\delta$. Then, it would be convenient to introduce a new unknown $w=(1-\partial _z^2)\psi$, which implies that

$$\begin{aligned} \psi =\Lambda ^{-2}w=p*w. \end{aligned}$$

It is easy to see that

$$\begin{aligned} (p*w)_{zz}=p*w-w,\quad \psi _{zzz}=(p*w)_z-w_z. \end{aligned}$$

As a consequence, the equation (3.5) can be written by in the non-local form

$$\begin{aligned}{} & {} w_{\tau }+\frac{1}{1+b}w+\frac{c+bz}{1+b}w_z-\frac{1}{1+b}p*w+\frac{b(c-z)}{1+b}(p*w)_z\nonumber \\{} & {} \qquad +(1+b)(p*w)(p*w)_z\nonumber \\{} & {} \quad =b(p*w)_z(p*w-w)+(p*w)\Big ((p*w)_z-w_z\Big ). \end{aligned}$$

(3.6)

We need to derive the corresponding initial condition for w. Note the relations

$$\begin{aligned} v(t,x)=e^{\tau }\psi (\tau ,z),\quad \tau =-\log (T-t), \quad z=ye^{-\tau }=\frac{x}{T-t}(T-t)=x, \end{aligned}$$

we have

$$\begin{aligned} v(0,x)=\frac{1}{T}\psi (-\log T,x),\quad v_{xx}(0,x)=\frac{1}{T}\psi _{zz}(-\log T,x) \end{aligned}$$

and

$$\begin{aligned} w(-\log T,z)= & {} \psi (-\log T,z)-\psi _{zz}(-\log T,z)\\= & {} T\left[ u_0(x)-u_0''(x)-\frac{1}{1+b}\cdot \frac{c-x}{T}\right] . \end{aligned}$$

Hence, it suggests to let $t'=\tau +\log T$ and the initial condition for w can be given as

$$\begin{aligned} w(0,z)=T[u_0(z)-u_0''(z)]-\frac{c-z}{1+b}\equiv w_0(z). \end{aligned}$$

(3.7)

The boundary conditions for w are

$$\begin{aligned} \lim _{|z|\rightarrow +\infty }w(\tau ,z)=0,\quad \lim _{|z|\rightarrow +\infty }w_z(\tau ,z)=0. \end{aligned}$$

(3.8)

While, the equation (3.6) can be rewritten in the form

$$\begin{aligned}{} & {} w_{t'}+\frac{1}{1+b}w+\frac{c+bz}{1+b}w_z-\frac{1}{1+b}p*w+\frac{b(c-z)}{1+b}(p*w)_z\nonumber \\{} & {} \quad +(1+b)(p*w)(p*w)_z =b(p*w)_z(p*w-w)+(p*w)\Big ((p*w)_z-w_z\Big ). \end{aligned}$$

For simplicity, we still denote $t'$ by $\tau$ and have the equation in the form (3.6).

We introduce a commutator estimate which can be found in [31].

Lemma 3.1

Let $s>0$. Then it holds that

$$\begin{aligned} \Vert [\Lambda ^s,u]v\Vert _{L^2}\le C\Big (\Vert \partial _xu\Vert _{L^{\infty }}\Vert \Lambda ^{s-1}v\Vert _{L^2} +\Vert \Lambda ^su\Vert _{L^2}\Vert v\Vert _{L^{\infty }}\Big ), \end{aligned}$$

(3.9)

where positive constant C depending only on s.

Now we are ready to derive some a priori estimates for the solution of perturbation equations (3.6), (3.7) and (3.8). Applying $\Lambda ^s$ with $s>0$ at the both sides of (3.6) yields

$$\begin{aligned}{} & {} (\Lambda ^sw)_{\tau }+\frac{1}{1+b}\Lambda ^sw+\Lambda ^s\left( \frac{c+bz}{1+b}w_z\right) -\frac{1}{1+b}\Lambda ^s(p*w) \nonumber \\{} & {} \qquad +\Lambda ^s\left[ \frac{b(c-z)}{1+b}(p*w)_z\right] \nonumber \\{} & {} \qquad +(1+b)\Lambda ^s[(p*w)(p*w)_z]\nonumber \\{} & {} \quad =b\Lambda ^s[(p*w)_z(p*w-w)]+\Lambda ^s\left[ (p*w)\Big ((p*w)_z-w_z\Big )\right] . \end{aligned}$$

(3.10)

Lemma 3.2

Let $s>\frac{7}{2}$ and the Assumption (2.2) holds. Then, we have

$$\begin{aligned} \Vert w\Vert _{H^s}\le C \exp \left( -\frac{2-b}{2(1+b)}\tau \right) \Vert w_0\Vert _{H^s}, \end{aligned}$$

(3.11)

where C is a positive constant depending on s and b.

Proof

Taking $L^2$-inner product with (3.10) by $\Lambda ^sw$ gives

$$\begin{aligned}{} & {} \frac{1}{2}\frac{d}{d\tau }\Vert w\Vert ^2_{H^s}+\frac{1}{1+b}\Vert w\Vert ^2_{H^s}+\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s\left( \frac{c+bz}{1+b}w_z\right) dz\nonumber \\{} & {} \qquad -\frac{1}{1+b}\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s(p*w)dz +\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s\left[ \frac{b(c-z)}{1+b}(p*w)_z\right] dz\nonumber \\{} & {} \qquad +(1+b)\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s[(p*w)(p*w)_z]dz\nonumber \\{} & {} \quad =b\,\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s[(p*w)_z(p*w-w)]dz\nonumber \\{} & {} \qquad +\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s\left[ (p*w)\Big ((p*w)_z-w_z\Big )\right] dz. \end{aligned}$$

(3.12)

Next we shall estimate each term in (3.12). First, by the integration by parts, we have

$$\begin{aligned} \int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s\left( \frac{c+bz}{1+b}w_z\right) dz= & {} \int _{\mathbb {R}}\left( \frac{c+bz}{1+b}w_z\right) \Lambda ^{2s}wdz\\= & {} -\frac{b}{1+b}\int _{\mathbb {R}}\left( \Lambda ^{s}w\right) ^2dz -\int _{\mathbb {R}}\frac{c+bz}{1+b}(\Lambda ^{s}w)_z\Lambda ^swdz\\= & {} -\frac{b}{1+b}\Vert w\Vert ^2_{H^s}-\frac{1}{2}\int _{\mathbb {R}}\frac{c+bz}{1+b}\left[ (\Lambda ^{s}w)^2\right] _zdz\\= & {} -\frac{b}{1+b}\Vert w\Vert ^2_{H^s}+\frac{1}{2}\cdot \frac{b}{1+b}\Vert w\Vert ^2_{H^s}\\= & {} -\frac{1}{2}\cdot \frac{b}{1+b}\Vert w\Vert ^2_{H^s}. \end{aligned}$$

It is easy to see that

$$\begin{aligned} \int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s(p*w)dz=\Vert w\Vert ^2_{H^{s-1}}. \end{aligned}$$

Still by the integration by parts, we have

$$\begin{aligned} \int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s\left[ \frac{b(c-z)}{1+b}(p*w)_z\right] dz= & {} \int _{\mathbb {R}}\frac{b(c-z)}{1+b}\Lambda ^{2s}w\cdot (p*w)_zdz\\= & {} \frac{b}{1+b}\Vert w\Vert ^2_{H^{s-1}}\\{} & {} -\frac{1}{2}\int _{\mathbb {R}}\frac{b(c-z)}{1+b}\left[ (\Lambda ^{s-1}w)^2\right] _zdz\\= & {} \frac{b}{1+b}\Vert w\Vert ^2_{H^{s-1}}-\frac{1}{2}\cdot \frac{b}{1+b}\Vert w\Vert ^2_{H^{s-1}}\\= & {} \frac{1}{2}\cdot \frac{b}{1+b}\Vert w\Vert ^2_{H^{s-1}}. \end{aligned}$$

In addition, we have

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s[(p*w)(p*w)_z]dz\\{} & {} \quad =\frac{1}{2}\int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s[(p*w)^2]_zdz\\{} & {} \quad =-\frac{1}{2}\int _{\mathbb {R}}w_z\Lambda ^{2s}(p*w)^2dz=-\frac{1}{2}\int _{\mathbb {R}}w_z\Big (\Lambda ^{s-2}w\Big )^2dz\\{} & {} \quad \le \frac{1}{2}\Vert w_z\Vert _{L^{\infty }}\Vert w\Vert ^2_{H^{s-2}}\le \frac{1}{2}\Vert w\Vert ^3_{H^{s-2}}, \end{aligned}$$

since we have assumed $s>\frac{7}{2}$. Here we have made use of the embedding result $H^{s-1}(\mathbb {R})\subset L^{\infty }(\mathbb {R})$ with $s>\frac{3}{2}$.

By using the commutator estimate (3.9), the Cauchy-Schwartz inequality and the embedding $H^{s-1}(\mathbb {R})\subset L^{\infty }(\mathbb {R})$ with $s>\frac{3}{2}$, we have

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s[(p*w)_z(p*w-w)]dz\\{} & {} \quad =\int _{\mathbb {R}}\Lambda ^sw\cdot \Big ([\Lambda ^s,\;p*w-w](p*w)_z+(p*w-w)\Lambda ^s(p*w)_z\Big )dz\\{} & {} \quad =\int _{\mathbb {R}}\Lambda ^sw\cdot [\Lambda ^s,\;p*w-w](p*w)_zdz+\int _{\mathbb {R}}\Lambda ^sw\cdot (p*w-w)\Lambda ^s(p*w)_zdz\\{} & {} \quad \le C\Big (\Vert (p*w-w)_z\Vert _{L^{\infty }}\Vert \Lambda ^{s-1}(p*w)_z\Vert _{L^2}\\{} & {} \qquad +\Vert \Lambda ^s(p*w-w)\Vert _{L^2}\Vert (p*w)_z\Vert _{L^{\infty }}\Big )\Vert w\Vert _{H^s}\\{} & {} \qquad +\Big (\Vert p*w-w\Vert _{L^{\infty }}+\Vert (p*w-w)_z\Vert _{L^{\infty }}\Big )\Vert w\Vert ^2_{H^s}\\{} & {} \quad \le C\Vert w\Vert ^3_{H^s}, \end{aligned}$$

where C is a positive constant depending on s. Here we have utilized the following facts

$$\begin{aligned} \Vert (p*w-w)_z\Vert _{L^{\infty }},\quad \Vert (p*w)_z\Vert _{L^{\infty }},\quad \Vert p*w-w\Vert _{L^{\infty }}\le C\Vert w\Vert _{H^s}, \quad s>\frac{3}{2}, \end{aligned}$$

which are valid due to $\Vert p*w\Vert _{L^{\infty }}\le \Vert w\Vert _{L^{\infty }}$ and the inequality $H^{s-1}(\mathbb {R})\subset L^{\infty }(\mathbb {R})$ with $s>\frac{3}{2}$.

In a similar way, we have

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\Lambda ^sw\cdot \Lambda ^s\left[ (p*w)\Big ((p*w)_z-w_z\Big )\right] dz\\{} & {} \quad =\int _{\mathbb {R}}\Lambda ^sw\cdot \Big ([\Lambda ^s,\;p*w]\Big ((p*w)_z-w_z\Big )+(p*w)\Lambda ^s\Big ((p*w)_z-w_z\Big )\Big )dz\\{} & {} \quad \le C\Big [\Vert (p*w)_z\Vert _{L^{\infty }}\Vert \Lambda ^{s-1}\Big ((p*w)_z-w_z\Big )\Vert _{L^2}\\{} & {} \qquad +\Vert \Lambda ^s(p*w)\Vert _{L^2}\Vert (p*w)_z-w_z\Vert _{L^{\infty }}\Big ]\Vert w\Vert _{H^s}\\{} & {} \qquad +\Vert p*w\Vert _{L^{\infty }}\Vert w\Vert ^2_{H^s}\\{} & {} \quad \le C\Vert w\Vert ^3_{H^s}, \end{aligned}$$

where C is a positive constant depending on s.

Combining the above analysis yields

$$\begin{aligned} \frac{1}{2}\frac{d}{d\tau }\Vert w\Vert ^2_{H^s}+\frac{2-b}{2(1+b)}\Vert w\Vert ^2_{H^s}+\frac{b-2}{2(1+b)}\Vert w\Vert ^2_{H^{s-1}}\le \frac{1+b}{2}\Vert w\Vert ^3_{H^{s-2}}+C\Vert w\Vert ^3_{H^s}, \end{aligned}$$

and thus,

$$\begin{aligned} \frac{d}{d\tau }\Vert w\Vert ^2_{H^s}+\frac{2-b}{1+b}\Vert w\Vert ^2_{H^s}\le C\Vert w\Vert ^3_{H^s}, \end{aligned}$$

(3.13)

where C is a positive constant depending on s and b. Equation (3.13) is a Bernoulli-type differential inequality. A direct analysis shows that

$$\begin{aligned} -\frac{d}{d\tau }\left( \frac{1}{\Vert w\Vert _{H^s}}\right) +\frac{2-b}{2(1+b)}\frac{1}{\Vert w\Vert _{H^s}}\le C, \end{aligned}$$

which implies that

$$\begin{aligned} \Vert w\Vert _{H^s}\le C \exp \left( -\frac{2-b}{2(1+b)}\tau \right) \Vert w_0\Vert _{H^s}, \end{aligned}$$

where C is a positive constant depending on s and b. The proof is completed. $\square$

Now we are going to study the global well-posedness for (3.6) with the initial data (3.7) and the boundary condition (3.8). By introduce the following linear operator

$$\begin{aligned} {\mathcal {L}}[w]:=-\frac{1}{1+b}w-\frac{c+bz}{1+b}w_z-\frac{1}{1+b}p*w-\frac{b(c-z)}{1+b}(p*w)_z, \end{aligned}$$

(3.14)

we can rewrite the equation (3.6) as

$$\begin{aligned} w_{\tau }={\mathcal {L}}[w]+f(w), \end{aligned}$$

(3.15)

where the nonlinear term f(w) is given by

$$\begin{aligned} f(w):=&\,-(1+b)(p*w)(p*w)_z+b(p*w)_z(p*w-w)\nonumber \\&\, +(p*w)\Big ((p*w)_z-w_z\Big ). \end{aligned}$$

(3.16)

By the definition of operator ${\mathcal {L}}$, we can see that

Lemma 3.3

Assume $s>\frac{7}{2}$. Then, it holds that

$$\begin{aligned} {\mathcal {L}}[w]\in H^s,\quad \forall w\in {\mathcal {D}}({\mathcal {L}}). \end{aligned}$$

Moreover, ${\mathcal {L}}$ is closed and densely defined in $H^s$.

Lemma 3.4

Assume $s>\frac{7}{2}$. Then the linear operator ${\mathcal {L}}$ in (3.14) is dissipative in $H^s$.

Proof

It suffices to prove that

$$\begin{aligned} \langle {\mathcal {L}}[w],\;w \rangle _{H^s}\le 0. \end{aligned}$$

(3.17)

By the previous computation, we have

$$\begin{aligned} \int _{\mathbb {R}}\left( \Lambda ^s{\mathcal {L}}[w]\right) \Lambda ^swdz= & {} -\frac{1}{1+b}\Vert w\Vert ^2_{H^s} -\int _{\mathbb {R}}\Lambda ^sw\Lambda ^s\left( \frac{c+bz}{1+b}w_z\right) dz\nonumber \\{} & {} -\frac{1}{1+b}\int _{\mathbb {R}}\Lambda ^sw\Lambda ^s(p*w)dz\nonumber \\{} & {} -\int _{\mathbb {R}}\Lambda ^sw\Lambda ^s\left( \frac{b(c-z)}{1+b}(p*w)_z\right) dz\nonumber \\= & {} -\frac{1}{1+b}\Vert w\Vert ^2_{H^s}-\frac{b}{2(1+b)}\Vert w\Vert ^2_{H^s}-\frac{1}{1+b}\Vert w\Vert ^2_{H^{s-1}}\nonumber \\{} & {} -\frac{b}{2(1+b)}\Vert w\Vert ^2_{H^s}\nonumber \\= & {} -\Vert w\Vert ^2_{H^s}-\frac{1}{1+b}\Vert w\Vert ^2_{H^{s-1}}<0. \end{aligned}$$

(3.18)

Thus, the proof is completed. $\square$

Lemma 3.5

Assume $s>\frac{7}{2}$. The operator ${\mathcal {L}}$ in (3.14) is invertible in $H^s$ and can generate a $C_0$-semigroup $\{\textbf{S}(t)\}_{t\ge 0}$ in $H^s$.

Proof

Step 1 We prove the operator ${\mathcal {L}}$ is invertible. We only need to verify that ${\mathcal {L}}$ is injective and surjective. Let $w\in \mathcal {D(L)}$ such that ${\mathcal {L}}[w]=0$. Then, it follows that

$$\begin{aligned} {-}\frac{1}{1+b}\Lambda ^sw{-}\Lambda ^s\left( \frac{c+bz}{1+b}w_z\right) {-}\frac{1}{1+b}\Lambda ^s(p*w){-}\Lambda ^s\left( \frac{b(c-z)}{1{+}b}(p*w)_z\right) {=}0. \end{aligned}$$

Multiplying $\Lambda ^sw$ on the both sides of the above equation and integrating it over $\mathbb {R}$ leads to

$$\begin{aligned} -\Vert w\Vert ^2_{H^s}-\frac{1}{1+b}\Vert w\Vert ^2_{H^{s-1}}=0, \end{aligned}$$

which implies that $w=0$ since the boundary condition (3.8). This means the operator ${\mathcal {L}}$ is injective.

Now we show that ${\mathcal {L}}$ is surjective. Indeed, for any $h\in H^s$, we assume

$$\begin{aligned} {\mathcal {L}}[w]=h. \end{aligned}$$

Then, applying $\Lambda ^s$ to the above equation and multiplying it by $\Lambda ^sw$ and integrating over $\mathbb {R}$ gives

$$\begin{aligned} \Vert w\Vert ^2_{H^s}+\frac{1}{1+b}\Vert w\Vert ^2_{H^{s-1}}=-\int _{\mathbb {R}}\Lambda ^sh\cdot \Lambda ^swdz, \end{aligned}$$

which furthermore we have

$$\begin{aligned} \Vert w\Vert _{H^s}\le C\Vert h\Vert _{H^s}. \end{aligned}$$

Thus, by the standard theory of elliptic partial differential equations, there exists a unique weak solution $w\in H^s$. Moreover, for this solution if $h\in H^s$, then we have $w\in H^{s+1}$. This implies that ${\mathcal {L}}$ is surjective.

Step 2 By the Lumer-Philips theorem (cf. Chapter 1 in [36]), we can find that the operator ${\mathcal {L}}$ generates a $C_0$-semigroup $\{\textbf{S}(t)\}_{t\ge 0}$ in $H^s$. The proof is finished. $\square$

Combining the results in Lemmas 3.3-3.5 yields the following conclusions.

Proposition 3.1

Let $s>\frac{7}{2}$. The Cauchy problem

$$\begin{aligned} \frac{d}{d\tau }w={\mathcal {L}}[w],\quad w(0)=w_0, \end{aligned}$$

with the zero boundary condition admits a unique solution

$$\begin{aligned} w(\tau )=\textbf{S}(\tau )w_0, \end{aligned}$$

where the initial data $w_0$ is given in (3.7).

In the sequel, we consider the nonlinear problem (3.16). By making use of the Duhamel’s principle and Proposition 3.1, we are able to write (3.16) in an abstract form.

$$\begin{aligned} w(\tau )=\textbf{S}(\tau )w_0+\int _{0}^{\tau }{} \textbf{S}(\tau -t)f(w(t))dt. \end{aligned}$$

As usual, to prove the above integral equation has a solution, one can consider the closed ball in $H^s$ as follows.

$$\begin{aligned} B_{\varepsilon }=\Big \{w\in H^s:\quad \Vert w\Vert <\varepsilon ,\quad s>\frac{7}{2}\Big \}, \end{aligned}$$

where $\varepsilon >0$ is a small constant.

Define the map

$$\begin{aligned} {\mathcal {T}}w(\tau )=\textbf{S}(\tau )w_0+\int _{0}^{\tau }{} \textbf{S}(\tau -t)f(w(t))dt. \end{aligned}$$

Now we aim to show that the map ${\mathcal {T}}$ has a fixed point in $B_{\varepsilon }$ for some $\varepsilon <1$. Here we shall employ the Banach fixed point theorem.

First, we recall the following well-known results [31].

Lemma 3.6

The space $H^s\cap L^{\infty }$ with $s>0$ is an algebra. Moreover, it holds that

$$\begin{aligned} \Vert uv\Vert _{H^s}\le C\left( \Vert u\Vert _{L^{\infty }}\Vert v\Vert _{H^s}+\Vert u\Vert _{H^s}\Vert v\Vert _{L^{\infty }}\right) , \end{aligned}$$

where C is a positive constant depending upon s.

Next we have the following lemma.

Lemma 3.7

Let $s>\frac{7}{2}$. Assume that $\Vert w_0\Vert _{H^{s+1}}<\varepsilon$. Then ${\mathcal {T}}$ maps $B_{\varepsilon }$ into $B_{\varepsilon }$. Moreover, the map ${\mathcal {T}}$ is a contractive mapping.

Proof

Based on Lemma 3.6, we have

$$\begin{aligned} \Vert f(w)\Vert _{H^s}\le & {} (1+b)\Vert (p*w)(p*w)_z\Vert _{H^s}+|b|\cdot \Vert (p*w)_z(p*w-w)\Vert _{H^s}\\{} & {} \quad +\Vert (p*w)\Big ((p*w)_z-w_z\Big )\Vert _{H^s}\\\le & {} (1{+}b)\Vert (p*w)\Vert _{H^s}\Vert (p*w)_z\Vert _{L^{\infty }}{+}|b|\cdot \Vert (p*w)_z\Vert _{L^{\infty }}\Vert p*w{-}w\Vert _{H^s}\\{} & {} \quad +\Vert (p*w)\Vert _{H^s}\Vert (p*w)_z-w_z\Vert _{L^{\infty }}. \end{aligned}$$

Bearing in mind that $H^{s-2}\subset L^{\infty }$ with $s>\frac{7}{2}$ and Lemma 3.2, we conclude that

$$\begin{aligned} \Vert f(w)\Vert _{H^s}\le C\Vert w\Vert ^2_{H^s}\le C\Vert w_0\Vert ^2_{H^s}\le C\varepsilon ^2<\varepsilon , \end{aligned}$$

which implies the map ${\mathcal {T}}$ is indeed a self-mapping on $B_{\varepsilon }$.

Next we shall prove the mapping ${\mathcal {T}}$ is contractive. Suppose $w, \tilde{w}\in B_{\varepsilon }$. By Lemma 3.6 and the embedding inequality $H^s\subset L^{\infty }$, we have

$$\begin{aligned}{} & {} \Vert f(w)-f(\tilde{w})\Vert \\{} & {} \quad =\left\| -(1+b)(p*w)(p*w)_z+b(p*w)_z(p*w-w)+(p*w)\Big ((p*w)_z-w_z\Big )\right. \\{} & {} \qquad \left. +(1+b)(p*\tilde{w})(p*\tilde{w})_z-b(p*\tilde{w})_z(p*\tilde{w}-\tilde{w})-(p*\tilde{w})\Big ((p*\tilde{w})_z-\tilde{w}_z\Big ) \right\| _{H^s}\\{} & {} \quad \le C\Big \{\Vert (p*w)(p*(w-\tilde{w}))_z\Vert _{H^s}+\Vert (p*(w-\tilde{w}))(p*\tilde{w})_z\Vert _{H^s}\\{} & {} \qquad +\Vert (p*(w-\tilde{w}))_z(p*w-w)\Vert _{H^s}+\Vert (p*\tilde{w})_z(p*(w-\tilde{w}))-(w-\tilde{w})))\Vert _{H^s}\\{} & {} \qquad +\Vert (p*(w-\tilde{w}))\Big ((p*w)_z-w_z\Big )\Vert _{H^s}\\{} & {} \qquad +\Vert (p*\tilde{w})\Big ((p*(w-\tilde{w}))_z-(w-\tilde{w})_z\Big )\Vert _{H^s}\Big \}\\{} & {} \quad \le C\Big \{\Vert p*w\Vert _{L^{\infty }}\Vert (p*(w-\tilde{w}))_z\Vert _{H^s}+\Vert (p*(w-\tilde{w}))\Vert _{H^s}\Vert (p*\tilde{w})_z\Vert _{L^{\infty }}\\{} & {} \qquad +\Vert (p*(w-\tilde{w}))_z\Vert _{H^s}\Vert (p*w-w)\Vert _{L^{\infty }}\\{} & {} \qquad +\Vert (p*\tilde{w})_z\Vert _{L^{\infty }}\Vert (p*(w-\tilde{w}))-(w-\tilde{w})))\Vert _{H^s}\\{} & {} \qquad +\Vert (p*(w-\tilde{w}))\Vert _{H^s}\Vert \Big ((p*w)_z-w_z\Big )\Vert _{L^{\infty }}\\{} & {} \qquad +\Vert (p*\tilde{w})\Vert _{L^{\infty }}\Vert \Big ((p*(w-\tilde{w}))_z-(w-\tilde{w})_z\Big )\Vert _{H^s}\Big \}\\{} & {} \quad \le C\varepsilon \Vert w-\tilde{w}\Vert _{H^s}. \end{aligned}$$

Then, it follows that

$$\begin{aligned} \Vert {\mathcal {T}}w-{\mathcal {T}}\tilde{w}\Vert _{H^s}= & {} \Vert \int _0^{\tau }\textbf{S}(\tau -t)[f(w)-f(\tilde{w})]dt\Vert _{H^s}\\\le & {} \int _0^{\tau }\Vert \textbf{S}(\tau -t)[f(w)-f(\tilde{w})]\Vert _{H^s}dt\\\le & {} C\varepsilon \Vert w-\tilde{w}\Vert _{H^s}<\Vert w-\tilde{w}\Vert _{H^s}, \end{aligned}$$

provided that $\varepsilon >0$ is sufficiently small. This means that ${\mathcal {T}}$ is a contractive mapping. $\square$

Now we have the following existence results.

Proposition 3.2

Assume $s>\frac{7}{2}$ and $-1<b\le 2$. Let $\varepsilon$ be a positive and sufficiently small constant. Then, it holds that

• (i) if the initial data $\Vert w_0\Vert _{H^{s+1}}<\varepsilon$, then there is a unique solution $w\in B_{\varepsilon }$ to the nonlinear problem (3.6), (3.7) and (3.8);

• (ii) there admits a global solution $\phi (\tau ,y)\in H^s$ to the equation (3.5) with the initial data (3.7) and boundary condition (3.8). In addition, if the initial data $\Vert \phi _0\Vert _{H^{s+1}}<\varepsilon$, then it holds that

  $$\begin{aligned} \Vert \phi \Vert _{H^s}\le C \varepsilon \exp \left( -\frac{4+b}{2(1+b)}\tau \right) , \end{aligned}$$

  where C is a positive constant and may depends on b.

Proof

• (i) By Lemma 3.7 and the Banach fixed point theorem, we find that the map ${\mathcal {T}}$ has a fixed point in the set $B_{\varepsilon }$, which is exactly the solution of (3.6) with the initial-boundary conditions (3.7), (3.8).

• (ii) By the conclusion in (i), we have the solution $\phi (\tau , y)$ to (3.4) satisfying

  $$\begin{aligned} \phi (\tau ,y)=e^{\tau }\psi (\tau ,y)=e^{\tau }\Big [p*w(\tau ,e^{-\tau }z)\Big ], \end{aligned}$$

  which implies that

  $$\begin{aligned} \phi _{yy}=(p*w)_{zz}e^{-\tau }=e^{-\tau }(p*w-w). \end{aligned}$$

  As a consequence, by Lemma 3.2 we have

  $$\begin{aligned}\Vert \phi _{yy}\Vert _{H^{s-2}}\le e^{-\tau }\Vert p*w-w\Vert _{H^{s-2}}\le C e^{-\tau }\Vert w\Vert _{H^{s}}\le C\varepsilon \exp \left( -\frac{4+b}{2(1+b)}\tau \right) , \end{aligned}$$

  where we used $\Vert w_0\Vert _{H^s}\le \varepsilon$. Note that the constant C may depend on the parameter b.

$\square$

Finally, by Proposition 3.2 we can obtain the results in Theorem 1.1 (see also [23] for similar discussions). Thus, the proof of Theorem 1.1 is completed.