Analytical Study for Stress Wave Interaction with Rock Joints Having Unequally Close–Open Behavior

Abstract

Stress wave interaction with rock joints during wave propagation is usually dependent on the dynamic response of the joints. During wave propagation, joints may be closed and open under the effects of the stress wave and the in situ stress. A joint in nature can only resist load during close process. In this paper, the close and open behaviors of rock joints are considered to be different. The joints are assumed to be linearly elastic in close status but turn into free surfaces in open status. Wave propagation equation across joints with unequally close–open behavior is first derived and expressed as a time-differential form based on the displacement discontinuity method. SHPB test recording is then adopted to verify the present approach, which is also compared with the results from existing methods for joints with equally close–open behavior. Next, analysis is conduced for wave propagation across a single joint and a set of parallel joints with unequally close–open behavior, respectively. From the analysis, effects of unequally close–open behavior of a joint on wave propagation and the dynamic response of the joint are studied finally.

1 Introduction

It is well known that a natural rock mass usually contains discontinuities, ranging over several orders of magnitudes, from micro-cracks to large-scale joints, fractures and faults. Due to their universality in a rock mass, rock joints not only govern the mechanical behavior of the rock mass but also effect the wave propagation in the rock mass. Studying the interaction of stress wave and rock joints is crucial to evaluate the stability and damage of rock structures, such as underground caverns, rock slopes and foundations under dynamic loads.

During wave propagation process, the two sides of a joint have relative deformation, such as opening, closure and slip under normal and shear stresses (Barton 1973; Brown and Scholz 1986; Daehnke and Rossmanith 1997). By conducting model tests and comparing the test results with those from numerical calculation, Fourney et al. (1997) found that open gaps affected the wave character not only in its amplitude but also in the spectral content. Recently, Wang et al. (2014) analyzed the closing process of an open joint under blast-induced waves and investigated the transmitted energy. In the studies, the two sides of the joints were taken into account as free surfaces to reflect stress waves impinging on the joints when joints are open.

The normal mechanical behavior of a joint is the main factor to influence P-wave propagation in jointed rock masses. Since a rock joint is not able to sustain tensile stresses, the normal property of the joint was generally described as the relation between its closure and pressure on it. For example, Goodman (1976) proposed a joint closure–stress equation by measuring mated and non-mated joints. Bandis et al. (1983) observed non-linear closurestress curves (i.e. B–B model) by measuring the difference of the displacements for the two sides of natural, unfilled joints with different degrees of weathering and roughness. Pyrak-Nolte (1988) measured the closure of natural joints and the corresponding seismic wave transmission across the joints, and derived the closurepressure relation.

Among analytical methods, the displacement discontinuity method (DDM) (Miller 1977; Schoenberg 1980) was used commonly for wave propagation across rock joints. For example, Pyrak-Nolte et al. (1990) derived the close-form solutions for a harmonic incidence across a linearly elastic rock joint. Coupling with the characteristic line theory (Ewing et al. 1957; Bedford and Drumheller 1994), Zhao and Cai (2001) calculated the transmission coefficient of incident longitudinal (P-) waves across a single nonlinear rock joint. Later, Zhao et al. (2006a, b) developed this coupling method to derive a wave propagation equation across linear and non-linear joints, respectively. With a transmission line formula, the scattering matrix method (SMM) (Aki and Richards 2002; Perino et al. 2010) was coupled with the DDM to study harmonic wave propagation across a set of parallel joints. As another expression of the scattering matrix method, the recursive method was modified with the DDM by Zhu et al. (2012) to fast calculate wave propagation across rock joints filled with viscoelastic medium, when the joints and rocks have similar mechanical properties and spatial configuration. Zhu et al. (2011) improved the DDM to analyze the effect of viscoelastic behavior of filled joints on seismic wave propagation. Li et al. (2012, 2013) proposed a time domain recursive method coupled with the DDM to analyze the obliquely incident wave propagation across a set of parallel rock joints with linear or nonlinear property. In the above studies, the close and open mechanical properties of a joint were considered to be equal, that is, closurestress relation of joints from the tests was also adopted to describe the joint-opening mechanical behavior.

Underground rock masses are generally in compressive state due to in situ stress, which causes joints usually closed. Many investigations have shown that the in situ stress is related to the complex geological conditions and varies with the depth of rock masses (Haimson et al. 2003; Zhao et al. 2005; Liu et al. 2014). The in situ stress is comparatively low in some cases, such as slope engineering and shallow depths. When an incident wave impinges on rock joints, the interaction between stress waves and joints creates new stress field around the joints and may cause joint close or open deformation.

The study is motivated by the need to better understand the effects of close and open behaviors of joints on wave propagation and the dynamic response of the joints. During P-wave propagation across a jointed rock mass, joints may have close or open deformation. The joints are assumed to be linearly elastic in close process and become free surfaces once joints open. After the brief introduction of the method of characteristic, wave propagation equation is first established. Comparison is consequently conducted between SHPB test recordings and analytical results which are obtained from the existing and present approaches for joints having equally and unequally close–open behavior, respectively. The entity of the error between the joints with equally and unequally close–open behaviors is analyzed. The role of the relevant parameters, such as in situ stress, joint spacing and joint number, on the transmitted wave is finally studied.

2 Theoretical Formulations

2.1 Problem Description

Assume there is a set of parallel joints in a linearly elastic, homogeneous and isotropic rock. When a plane P-wave impinges on one joint, both reflection and transmission usually take place from the two sides of the joint. Meanwhile, multiple reflected waves propagating in two opposite directions are induced from the joints. During wave propagation, the closure and opening of joints may occur, as shown in Fig. 1. In the close process, the two sides of the joint begin to contact and interact, as shown in Fig. 1a. This interaction is dependent on the close behavior of the joint. When the joint is open, the two sides are separated from each other and become as two free surfaces, as shown in Fig. 1b. At this moment, any waves are reflected at all from the joint sides (i.e. the free surfaces). This study is to investigate the interaction between stress wave and rock joints with unequally open and close mechanical behaviors.

Fig. 1

Schematic view of the joint close–open process during wave propagation

2.2 Wave Propagation Equation

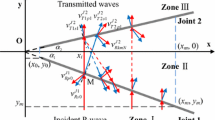
For one-dimensional wave propagation in a continuous medium, there are two types of characteristics: left-running and right-running characteristics (Ewing et al. 1957; Bedford and Drumheller 1994). Later, Zhao et al. (2006) proposed a similar triangle-shape characteristic method (see Fig. 2) to analyze wave propagation across a set of parallel joints for which normal property is linearly elastic. In Fig. 2, the conjunction points a and b are located at integral values of ${1 \mathord{\left/ {\vphantom {1 {(C\Delta t)}}} \right. \kern-0pt} {(C\Delta t)}}$ along the x axes and ${1 \mathord{\left/ {\vphantom {1 {\Delta t}}} \right. \kern-0pt} {\Delta t}}$ along the t in the x–t plane, so are the points a′ and c, where $\Delta t$ is the time interval and C is the P-wave propagation velocity in one continuous medium. The wave impendence of P-wave is denoted as z, and z = ρC, where ρ is the density of the medium. According to the similar triangle-shape characteristic method, the two relations between the particle velocities and the normal stresses along two characteristics can be expressed as

$$zv^{ - } \left( {x_{n} ,t_{j + 1} } \right) + \sigma^{ - } \left( {x_{n} ,t_{j + 1} } \right) = zv^{ + } \left( {x_{n - 1} ,t_{j} } \right) + \sigma^{ + } \left( {x_{n - 1} ,t_{j} } \right),{\text{along right-running characteristic line }} ab$$

(1)

$$zv^{ + } \left( {x_{n} ,t_{j + 1} } \right) - \sigma^{ + } \left( {x_{n} ,t_{j + 1} } \right) = zv^{ - } \left( {x_{n + 1} ,t_{j} } \right) - \sigma^{ - } \left( {x_{n + 1} ,t_{j} } \right),{\text{along left-running characteristic line}}\;a^{\prime}c$$

(2)

where $v^{ - } \left( {x_{n} ,t_{j + 1} } \right)$ and $v^{ + } \left( {x_{n} ,t_{j + 1} } \right)$ are the particle velocities at time $t_{j + 1}$ before and after the interface at $x_{n}$, respectively; $\sigma^{ - } \left( {x_{n} ,t_{j + 1} } \right)$ and $\sigma^{ + } \left( {x_{n} ,t_{j + 1} } \right)$ are the normal stresses at time $t_{j + 1}$ before and after the interface at $x_{n}$, respectively; j = [1, J], n = [2, N], and J and N are the time steps and the number of interfaces, respectively.

Fig. 2

Schematic view of the characteristics on x–t plane

When the method of characteristic was adopted to analyze P-wave propagation, the rock mass with a set of parallel joints was also divided with finite interfaces with number N and equal space $C\Delta t$ (Zhao et al. 2006b). The interfaces could be discontinuities, i.e. joins, or continuities, i.e. welded interfaces.

• 1. When the interface is a joint.

  In this case, the points a and a′ are situated on the two sides of the joint. The distance between points a and a′ represents the initial thickness of the joint, which is very small if compared to the wavelength of an incident wave and so is ignored in the present study.

  When an incident P-wave impinges normally on a set of parallel joints, the joints may be open and closed. If the relatively normal displacement of two sides of one joint is denoted as $\Delta u$, there is

  $$u^{ - } \left( {x_{n} ,t_{j} } \right) - u^{ + } \left( {x_{n} ,t_{j} } \right) = \Delta u\left( {x_{n} ,t_{j} } \right)$$

  (3)

  where $u^{ - }$ and $u^{ + }$ are the displacements of the two sides of the joint. The initial closure of a joint is denoted as $\Delta u_{0}$ under the effect of in situ stress $\sigma_{0}$ and $\Delta u_{0} = {{\sigma_{0} } \mathord{\left/ {\vphantom {{\sigma_{0} } k}} \right. \kern-0pt} k}$, where k is the normal stiffness of the joints and the in situ stress is supposed to be normal on the joints. In this paper, we consider the compressive stress to be positive.

  During wave propagation process, there are two possible deformation modes for one joint, i.e. closed mode when $\Delta u > - \Delta u_{0}$ and open mode when $\Delta u \le - \Delta u_{0}$, as shown in Fig. 1. The symbol $- \Delta u_{0}$ denotes the critical opening of joints.

  • Mode I: Closed mode when $\Delta u > - \Delta u_{0}$

    When one joint is closed, i.e. $u^{ - } - u^{ + } > - \Delta u_{0}$, the normal deformations of the joint are elastic and the joint becomes a displacement discontinuous boundary. The displacement discontinuity method (DDM) is adopted in the paper to describe the boundary. In the DDM, the stresses before and after the joint are considered to be continuous while the displacement before and after the joint are discontinuous. When the method of characteristic was adopted, Zhao et al. (2006b) derived the mathematical expression for wave propagation across a set of parallel joints of which the compressive property is equal to their tensile property.

    When the stress at time $t_{j + 1}$ on the joint was expressed as

    $$\sigma \left( {x_{n} ,t_{j + 1} } \right) = \sigma \left( {x_{n} ,t_{j} } \right) + k\Delta t\left[ {v^{ - } \left( {x_{n} ,t_{j} } \right) - v^{ + } \left( {x_{n} ,t_{j} } \right)} \right],$$

    (4)

    the particle velocities $v^{ - } \left( {x_{n} ,t_{j + 1} } \right)$ and $v^{ + } \left( {x_{n} ,t_{j + 1} } \right)$ before and after any joints with linearly elastic behavior were expressed as the following form (Li et al. 2010),

    $$v^{ - } \left( {x_{n} ,t_{j + 1} } \right) = v^{ + } \left( {x_{n - 1} ,t_{j} } \right) + \frac{1}{z}\left[ {\sigma \left( {x_{n - 1} ,t_{j} } \right) - \sigma \left( {x_{n} ,t_{j + 1} } \right)} \right]$$

    (5)
    $$v^{ + } \left( {x_{n} ,t_{j + 1} } \right) = v^{ - } \left( {x_{n + 1} ,t_{j} } \right) + \frac{1}{z}\left[ { - \sigma \left( {x_{n + 1} ,t_{j} } \right) + \sigma \left( {x_{n} ,t_{j + 1} } \right)} \right]$$

    (6)

    where $\sigma$ denotes the normal stress on the joint; $\Delta t$ denotes the time interval.

  • Mode II: Open mode when $\Delta u \le - \Delta u_{0}$When one joint is open, i.e. $u^{ - } - u^{ + } \le - \Delta u_{0}$, the normal deformation of the joint is not elastic any more, and then the two sides of the joint become free surfaces. For this case, the stress on each side is zero,

    $$\sigma^{ - } \left( {x_{n} ,t_{j} } \right) = \sigma^{ + } \left( {x_{n} ,t_{j} } \right) = 0$$

    (7)

    According to the right- and left-running characteristics expressed mathematically as Eqs. (1) and (2), the particle velocities before and after the joint can be written as

    $$v^{ - } \left( {x_{n} ,t_{j + 1} } \right) = v^{ + } \left( {x_{n - 1} ,t_{j} } \right) + \frac{1}{z}\sigma^{ + } \left( {x_{n - 1} ,t_{j} } \right)$$

    (8)
    $$v^{ + } \left( {x_{n} ,t_{j + 1} } \right) = v^{ - } \left( {x_{n + 1} ,t_{j} } \right) - \frac{1}{z}\sigma^{ - } \left( {x_{n + 1} ,t_{j} } \right)$$

    (9)

    Equations (8) and (9) mean that the particle velocities for the points a and a′ on the left- and right-sides of an open joint can be calculated from the dynamic responses of points b and c, respectively.

• 2. When the interface is continuous.

  For this case, the points a and a′ are overlapped or welded, which results in continuous physical variables before and after the interface, such as the stress and displacement. If the displacement shown as

  $$u^{ - } \left( {x_{n} ,t_{j} } \right) = u^{ + } \left( {x_{n} ,t_{j} } \right) = u\left( {x_{n} ,t_{j} } \right)$$

  (10)

  is differential to time t, the stress and the particle velocity of this continuous interface can be deduced from Eqs. (1) and (2) and expressed as

  $$v\left( {x_{n} ,t_{j + 1} } \right) = \frac{1}{2}\left[ {v^{ + } \left( {x_{n - 1} ,t_{j} } \right) + v^{ - } \left( {x_{n + 1} ,t_{j} } \right)} \right] + \frac{1}{2z}\left[ {\sigma \left( {x_{n - 1} ,t_{j} } \right) - \sigma \left( {x_{n + 1} ,t_{j} } \right)} \right]$$

  (11)
  $$\sigma \left( {x_{n} ,t_{j + 1} } \right) = \frac{z}{2}\left[ {v^{ + } \left( {x_{n - 1} ,t_{j} } \right) - v^{ - } \left( {x_{n + 1} ,t_{j} } \right)} \right] + \frac{1}{2}\left[ {\sigma \left( {x_{n - 1} ,t_{j} } \right) + \sigma \left( {x_{n + 1} ,t_{j} } \right)} \right]$$

  (12)

  The joint number is denoted as JN and the joint spacing S between two adjacent joints is divided into m layers. If the 1st and the JNth joints are assumed to be located at the 2nd and the Nth interfaces, respectively, there is N = (JN − 1)m + 2. If the incident wave is applied on the 1st layer and denoted as $v^{ + } (x_{1} ,t)$, the stress $\sigma (x_{1} ,t)$ on the 1st interface is equal to $\rho Cv^{ + } (x_{1} ,t)$. The particle before the 1st interface is assumed to be not disturbed, that is, $v^{ - } (x_{1} ,t) = 0$. When the initial conditions of $v^{ - } (x_{1} ,0)$, $v^{ + } (x_{1} ,0)$ and $\sigma (x_{i} ,0)$ (i = [2, N]) are known, wave propagation across the rock mass and the dynamic response of the joints can be calculated from above differential equations. For a joint interface, the stress on the joint and the particle velocities before and after the joint can be obtained from Eqs. (4) to (6) if the joint is closed or from Eqs. (7) to (9) when the joint is open. For a non-joint interface (i.e. continuous interface), the stress and the particle velocities at the interface can be obtained from Eqs. (11) and (12). Hence, the transmitted wave after the JNth joint is calculated and written as

  $$v_{\text{T}} (t_{j} ) = v^{ + } \left( {x_{N} ,t_{j} } \right)$$

  (13)

  and the incident wave before the 1st joint is

  $$v_{R} (t_{j} ) = v^{ - } \left( {x_{2} ,t_{j} } \right) - v_{I} \left( {t_{j} - \Delta t} \right)$$

  (14)

  where $v_{\text{I}} (t_{j} )$ is the incident wave impinging on the 1st interface.

3 Verification

In this section, verification is carried out by comparing the theoretical solution from the above derivation and the test results. The theoretical results are also compared with those from the existing method where the dynamic close and open behaviors of joints are assumed to be the same.

The test was conducted by Chen et al. (2015) using the modified SHPB experimental equipment to investigate the effect of joints with rough surface on wave propagation. Figure 3 shows the schematic view of the test equipment and the specimen with 36 % contact area ratio. The input and output bars were both made of norite with length of 1.5 m and square cross section of 40 × 40 mm. The density of the norite is 2960 kg/m³, and the Young’s modulus is 63.6 GPa. The specimens sandwiched between the input and output bars were also made of norite. The main cross section of each specimen was identical to those of the input and output bars. One surface of each specimen was flat and the other surface in contact with the output bar was sawn to shape a number of notches and columns, as shown in Fig. 3b, c. The artificial joint was formed from the notches and columns of each specimen. The specific stiffness of joint is defined as the average gradient of the closure–pressure curve for each joint. The test result (Chen et al. 2015) shows that the closurepressure curve for the joint with notch depth 1 mm and contact area ratio of 36 % is almost straight and the specific stiffness of the joint is about 110 GPa/m. No initial pressure was applied on the equipment, that is, $\sigma_{0} = 0$.

Fig. 3

SHPB test fro wave propagation across an artificial joint with rough surface

For one incident strain wave recorded in the input bar during the test, we can calculate the transmitted strain wave from Eqs. (4) to (9) and (13) when divided by the wave propagation velocity C in norite. The joint stiffness k is adopted as 110 GPa/m from the tests for the artificial joint which is 1 mm in thickness and 36 % in contact area ratio. The incident and transmitted waves recorded from the test are shown in Fig. 4, where the incident one has an obviously compressive portion with a tiny tensile tail. Based on the present approach for joints with different close and open behaviors and the existing method (Zhao and Cai 2001) for joints with the same close and open behaviors, the transmitted waves are calculated and shown in Fig. 4, too. By comparison, we can see that the transmitted waveform based on the present approach is closer to the test recording. Since there is no initial pressure on the two surfaces of each test sample during the test, the critical opening of joints $\Delta u_{0}$ is zero and the transmitted wave calculated from the present approach only has a compressive portion, while the transmitted wave from the existing method includes compressive and tensile portions.

Fig. 4

Comparison of the transmitted waves from theoretical methods and the test

4 Wave Propagation Across a Single Joint or a Set of Parallel Joints

In this section, an incident P-wave impinging on a single joint or a set of parallel joints is analyzed based on the wave propagation equation derived in Sect. 2. In the following analysis, some basic parameters for the joint and rock are chosen from the typical properties of the Bukit Timah granite of Singapore (Zhao 1996): the normal compressive stiffness k is 2.0 GPa/m, the rock mass density $\rho$ is 2650 kg/m³, and the P-wave velocity C is 5840 m/s.

The in situ stresses $\sigma_{0}$ are assumed to be 0.2zA, 0.5zA and 1.0zA, respectively. The incident waves are assumed as a one-cycle sinusoidal waveform, i.e.

$$v_{I} = \left\{ {\begin{array}{*{20}c} {A\sin (\omega t),} & {t = 0 - {1 \mathord{\left/ {\vphantom {1 {f_{0} }}} \right. \kern-0pt} {f_{0} }}} \\ {0,} & {t > {1 \mathord{\left/ {\vphantom {1 {f_{0} }}} \right. \kern-0pt} {f_{0} }}} \\ \end{array} } \right.$$

(15)

where A is the amplitude of the incident waves and assumed to be 1.0 m/s, $\omega = 2\pi f_{0}$ and $f_{0}$ = 50 Hz.

4.1 Wave Propagation Across a Single Joint

When an incident P-wave with the form of Eq. (15) impinges normally on one joint with unequally close–open behavior, the particle velocities before and after the joint can be obtained from Eqs. (4) to (6) when the joint is closed and from Eqs. (8) and (9) when the joint is open. The transmitted and reflected waves across the joint are then obtained from Eqs. (13) and (14). Figure 5a, b show the wave transmission and reflection when the in situ stresses $\sigma_{0}$ are 0.2zA and 0.5zA, respectively. If the joint has equally close–open dynamic behavior, Eqs. (4–6) are only needed to calculate the particle velocities before and after the joint. The corresponding transmitted and reflected waves are shown in Fig. 5c.

Fig. 5

Wave propagation normally across a joint with equal and unequal close–open behaviors

We can see from Fig. 5 that the first positive parts of the transmitted waveforms are identical in compressive stress state when the stress wave propagates across a joint with equally or unequally close–open behavior. We also observed from Figs. 2b and 5a that the negative parts of the transmitted waveforms are cut off at the particle velocities of 0.2 m/s for $\sigma_{0}$ = 0.2zA and 0.5 m/s for $\sigma_{0}$ = 0.5zA, respectively, and the reflected waveforms are influenced thence. We can conclude that the cut-off line segment occurs at tensile stress state with value of ${{\sigma_{0} } \mathord{\left/ {\vphantom {{\sigma_{0} } {zA}}} \right. \kern-0pt} {zA}}$. The cut-off waveform of the transmitted waves across a joint with unequally close–open behavior indicates that the joint begins to be open and fails to resist the initial pressure. When the in situ stress is 1.0zA, we also calculate wave propagation across the joint with unequally close–open behavior and find that the transmitted and reflected waves are respectively identical to those for joints with equally close–open behavior, as shown in Fig. 5c. This indicates that for $\sigma_{0}$ = 1.0zA, joint appears no open and two sides of the joint are kept in interaction during wave propagation process.

When the difference between the particle velocities of the two sides of the joint is integrated with regard to time t, the relatively normal displacement of the joint is obtained and shown in Fig. 6a. The corresponding normal stress on the joint during wave propagation can be found in Fig. 6b. Figure 6a, b shows that the negative parts of the normal stress curve are cut off when the negative displacement is greater than the critical value of opening joints, i.e. 0.2zA/k and 0.5zA/k. When the in situ stress $\sigma_{0}$ is 1.0zA, the critical value of opening joints is 1.0zA/k, which is a bit greater than the minimum amplitude of the normal displacement. Hence, the curves for the time history of the normal stress on the joints with equally and unequally close–open behaviors are identical for $\sigma_{0}$ being 1.0zA.

Fig. 6

Interaction between stress wave and rock joint during wave propagation across one single joint

4.2 Wave Propagation Across Two Parallel Joints

Based on the derivation shown in Sect. 2, the transmitted waves are calculated and shown in Fig. 7 for wave propagation across two parallel joints with different spacings and in situ stresses. The symbol λ denotes the wavelength of the incident wave and equals to ${C \mathord{\left/ {\vphantom {C {f_{0} }}} \right. \kern-0pt} {f_{0} }}$. Figure 7 shows that for $\sigma_{0}$ = 0.2zA some cut-off lines with value of 0.2 m/s appear at the negative parts of the transmitted waves when the joint spacing denoted as S varies from 1/20λ to 2/5λ. For $\sigma_{0}$ = 0.5zA and S being 1/20λ, 1/10λ and 1/5λ, respectively, there are also some cut-off lines at the negative parts of the transmitted waves and the value of the line equals to 0.5 m/s. When the in situ stress is 0.5zA and joint spacing is 2/5λ, the minimum amplitude of the transmitted wave is less than ${{\sigma_{0} } \mathord{\left/ {\vphantom {{\sigma_{0} } {zA}}} \right. \kern-0pt} {zA}}$ and then there is no cut-off line. Meanwhile, we observe from Fig. 7 that the maximum amplitude of the transmitted waves decreases with increasing joint spacing, and the minimum amplitude of the transmitted waves is not affected by the joint spacing but limited by the ratio of the in situ stress to the maximum momentum of the incident wave when the joint is open.

Fig. 7

Wave propagation across two parallel joints with unequally close–open behavior

To analyze the dynamic response of joints during wave propagation, the relatively normal displacements of the two joints are calculated and shown in Fig. 7, when the in situ stress is 0.5zA. Joint opening state occurs once the negative value of the normal displacement is greater than the critical opening value of joints. It is then observed from Fig. 8 that which joint is open is related to the joint spacing S. When S is 1/20λ, the 1st joint is closed but the 2nd joint is open, and vice versa for S = 2/5λ. When S is 1/10λ or 1/5λ, both joints have opening deformation. Additionally, we find from Figs. 7 and 8 that the cut-off line of the transmitted wave is related to the dynamic response of the 2nd joint. In other words, the occurrence of the cut-off line of the transmitted wave indicates that there must be an opening deformation of the 2nd joint. Even though there is no cut-off line of the transmitted wave, the 1st joint is possibly open, which can be found in Fig. 8d.

Fig. 8

The joint displacement during wave propagation across two parallel joints with unequally close–open behavior

From the above, spectral analysis is then carried out and shown in Fig. 9. It can be seen from the figure that the dominant frequencies for $\sigma_{0}$ = 0.5zA and 1.0zA are quite close to each other, while the dominant frequency for $\sigma_{0}$ = 0.2zA is obviously less than the two others for $\sigma_{0}$ = 0.5zA and 1.0zA, when the joint spacing is given. This indicates that lower in situ stress is more possible to cause joint open and fill out transmitted waves with higher frequency. It is also observed that for the three in situ stresses, the dominant frequency decreases when the joint spacing varies from 1/10λ to 2/5λ. For $\sigma_{0}$ = 0.5zA and 1.0zA, the dominant frequencies are around 50 Hz for S = 1/10λ, 35 Hz for S = 1/5λ and 25 Hz for S = 2/5λ.

Fig. 9

Amplitude spectra of the transmitted waves across two parallel joints with unequally close–open behavior

4.3 Wave Propagation Across a Set of Parallel Joints

Figure 10 shows the transmitted wave across a set of parallel joints when the joint number varies from 5, 8 to 11 and the joint spacing is 1/20λ. For a given joint number, the main compressive portions of the transmitted waves for the three in situ stresses are identical. In addition, the maximum amplitudes are about 0.69, 0.65 and 0.6 for the joint number varying from 5, 8 to 11, respectively, which shows that the maximum amplitude of the transmitted wave is not affected by the in situ stress but influenced by the joint number when the joint spacing is given. This indicates that the stress wave attenuates gradually in amplitude with increasing joint number when the joint spacing is 1/20λ.

Fig. 10

Transmitted waves across a set of parallel joints with unequal close–open behavior (joint spacing = 1/20$\lambda$)

By observing the negative parts of the transmitted waves, we find that there is no cut-off line for $\sigma_{0}$ = 1.0zA, while for $\sigma_{0}$ = 0.2zA and 0.5zA the cut-off lines occur and the values are equal to 0.2 and 0.5 m/s, respectively. The phenomena indicate that the number of joints in a rock mass does not influence the minimum amplitude of the transmitted wave if joints are open. In summary, the minimum amplitude of the transmitted wave across opening joints is related to the in situ stress and limited by ${{\sigma_{0} } \mathord{\left/ {\vphantom {{\sigma_{0} } {zA}}} \right. \kern-0pt} {zA}}$.

The above analysis shows that joints with equally and unequally close–open behaviors result in transmitted waves with different waveforms and different dynamic responses of the joints. For the joints with equally and unequally close–open behaviors, the error is equal to $1- {{\sigma_{0} } \mathord{\left/ {\vphantom {{\sigma_{0} } {zA}}} \right. \kern-0pt} {zA}}$, which indicates that the entity of the error is due to the in situ stress and the maximum momentum of the incident wave.

5 Conclusions

The effects of different close and open deformation properties of joints on wave propagation and the dynamic response of the joints are studied in the paper. During wave propagation across a jointed rock mass, the redistribution of the stress on the rock joints may cause joint deformation. In this study, joints are assumed to be linearly elastic in close deformation and become free surfaces once joints are open, which is different from the joints assumed to have the same linearly elastic behavior in both close and open deformations. Wave propagation equation is derived for wave propagation across one single joint and a set of parallel joints, when the close and open deformation properties of one joint are different. By comparison, it is found that the calculation considering joint open failure is more close to the test results, which proves that the present approach is effective. By analyzing the interaction between stress wave and a single joint, wave propagation across joints with unequally close–open behavior is different from that for joints with equally close–open behavior.

During stress wave propagation across one single joint with unequally close–open behavior, the cut-off line appears in the transmitted wave when the joint opening deformation is big enough to resist the closure of joints caused by in situ stress. The in situ stress affects not only the transmitted and reflected waves but also the joint dynamic response, such as the relative deformation of one joint. For the lower in situ stress, the opening deformation of joint is more obvious and the joint is more possible to filter out stress waves with higher frequency.

During wave propagation across a set of parallel joints, the opening of any joint results in wave attenuated more in both amplitude and frequency. If joints are open, the number of joints in a rock mass and the joint spacing only affect the maximum amplitude of the transmitted wave, which is related to the joint closure. However, the joint number and spacing do not influence the minimum amplitude of the transmitted wave, when joints are open and fail to resist tension. The minimum amplitude of the transmitted wave across open joints is limited by ${{\sigma_{0} } \mathord{\left/ {\vphantom {{\sigma_{0} } {zA}}} \right. \kern-0pt} {zA}}$. The error between the two dynamic behaviors of a joint is related to the ratio of the in situ stress to the momentum of the incident wave. Therefore, for the slope engineering and shallow depths where the in situ stress is low, joints become more possible to open during wave propagation and the unequally close–open property of a joint should be taken into account for study.

In the above study, the joint compressive behavior is assumed to be linearly elastic. The present approach can also be extended to analyze wave propagation across rock joints of which compressive properties are nonlinearly elastic.