1 Introduction

Quantum teleportation is one of the most fascinating protocols predicted by quantum mechanics [1]. It is a critical ingredient for quantum communication and quantum computation networks [2,3,4]. Quantum teleportation is a faithful transfer of quantum states between two distant parties which have established prior entanglement and can communicate classically. In the past two decades, quantum teleportation has attracted considerable attentions and been studied both theoretically and experimentally [5,6,7]. However, in many scenarios, one does not need to teleport the whole quantum state, but rather the information of a particular parameter physically encoded in it. Therefore, there is no need to teleport the full information of the quantum states themselves, but only the relevant parameters that carry these information. In contrast to the quantum state teleportation where the credibility of teleportation is measured by fidelity, the transmission of information that is carried by a physical parameter is usually quantified by quantum Fisher information (QFI) [8, 9]. QFI plays a significant role in the fields of quantum geometry of state spaces [8, 10, 11], quantum information theory [12] and quantum metrology [13, 14]. Particularly, the inverse of QFI characterizes the ultimate achievable precision in parameter estimation [15]. In other words, QFI is used as an estimation tool of parameters that are contained in a quantum system during its evolution [16]. Due to its importance, there are some efforts that have been taken to quantify QFI in different quantum systems.

Recently, some authors have paid attention to quantifying quantum Fisher information in open quantum systems. For example, Zheng et al. [17] investigated the dynamics of QFI for a two-qubit system, where each qubit interacts with its own Markovian environment. Ozaydin [18] has quantified the QFI analytically for the W-state in the presence of different noisy channels. The effect of the Markovian reservoirs on the dynamics of the quantum Fisher information of a two-level system is discussed by You et al. [19]. Quantum Fisher information for a noisy open quantum system and initially prepared in a steady state is quantified by Altinats [20]. In the context of accelerated system, Metwally [21] discussed the possibility of quantifying the teleported by means of quantum Fisher information. Estimating the weight and the phase parameters of pulsed driven qubit via quantum Fisher information, where different pulses are considered [22].

In this context, it is important to mention some applications of quantum Fisher information. However, in the additional of using it as a quantifier of the initial parameters that describe the state, it can be used to calculate the phase sensitivity of systems that provides in quantum technologies, as example it can be used to enhance the imaging process without increasing the illumination power [23]. Since it may be frozen during the evaluation of the quantum system and consequently the information which is encoded in this system, then quantum Fisher information may be used as resource of secure communication [24]. It may be used to process the large amount of data that is needed for quantum computer [25]. Moreover, the quantum Fisher information also quantifies the degree of macroscopicity of a quantum state [26].

In the present work, we use a single atomic-field state, to teleport a two-qubit system. The possibility of estimating the teleported parameters is discussed by the quantum Fisher information of the two-qubit as well as by a single qubit. The effect of the atomic-field parameters on the estimation degree of the teleported parameters are discussed. We show that, it is possible to freeze the quantum Fisher information by controlling the initial state parameters and the atomic- field parameters.

The paper is organized as follows. In Sect. 2, we introduce the suggested model, where an analytical solution in terms of the Bloch vector is given. In Sect. 3, the possibility of teleporting the quantum Fisher information is discussed, where a description of a quantum teleportation protocol of a two-qubit system is reviewed. A mathematical form of the quantum Fisher information is introduced for a single and two-qubit systems. In Sect. 4, the weight and the phase parameters of the teleported state are quantified at the resonance and non-resonance cases, where some numerical calculations are presented. Finally, our results are summarized at Sect. 5.

2 Description of the model

The suggested system is described by Jaynes Cummings model of a single-two level atom interacts with a single cavity mode. In the rotating wave approximation, the Hamiltonian of this system is governed by [27, 28]

$$\begin{aligned} H= \frac{1}{2}\omega _{0}\sigma _{z}+\omega a^{\dagger }a+ g(a|e\rangle \langle g|+ a^{\dagger }|g\rangle \langle e|), \end{aligned}$$

where, the first two terms represent the Hamiltonian of the atom and the cavity mode, respectively, while the third term represents the interaction Hamiltonian. The parameters \(\omega _0\) and \(\omega\) are the frequencies of the atomic system and the cavity mode, respectively, and the operators \(a^{\dagger }(a)\) represent the creation (annihilation) operators of the cavity mode, \(|e \rangle\) and \(|g \rangle\) are the exited and the ground states of the atom, respectively, and g is the constant coupling between the atomic system and the cavity mode.

Let us assume that, the atomic-cavity system is initially prepared in a product state \(\left| \psi _s(0) \right\rangle =\left| \psi _f \right\rangle \otimes \left| \psi _a \right\rangle\)

$$\begin{aligned} |\psi _{{s}}(0)\rangle =\sum _{n=0}^{\infty } P_{n}|n,e\rangle , \end{aligned}$$
(1)

where \(\left| \psi _a(0) \right\rangle =\left| e \right\rangle\) and \(\left| \psi _f(0) \right\rangle =\sum _{0}^{\infty } P_{n}\left| n \right\rangle,\) \(P_{n}=\exp (-\frac{\bar{n}}{2}) \sqrt{\frac{\bar{n}^n}{n!}}\) with \(\bar{n}\) is the mean photons number. For any \(t>0\), the time evoluation of the total system is given by:

$$\begin{aligned} |\psi _{s}(t)\rangle =\mathcal {U}(t)|\psi _{s}(0)\rangle , \end{aligned}$$
(2)

where \(\mathcal {U}(t)\) is the time evolution operator. In the atomic basis \(|e\rangle\) and \(|g\rangle\), the operator \(\mathcal {U}(t)\) may be written as,

$$\begin{aligned} \mathcal {U}(t)=\left( \begin{array}{cc} c(\hat{n}+1,t) &{} d(\hat{n}+1,t)a \\ -a^{\dagger }d^{\dagger }(\hat{n}+1,t) &{} c^{\dagger }(\hat{n},t) \\ \end{array}\right) , \end{aligned}$$
(3)

where,

$$\begin{aligned} c(\hat{n},t)=\, & {} \text {e}^{ i\delta \tau }\left[ \cos \left( \tau \sqrt{\delta ^2+ \hat{n}}\right) -i\frac{\delta }{\sqrt{\delta ^2+ \hat{n}}} \sin \left( \tau \sqrt{\delta ^2+\hat{n}}\right) \right] , \nonumber \\ d(\hat{n},t)=\, & {} -i\frac{ \text {e}^{i\delta \tau }}{\sqrt{\delta ^2+\hat{n}}}\sin \left( \tau \sqrt{\delta ^2+ \hat{n}}\right) , \end{aligned}$$
(4)

and \(\hat{n}=a^{\dagger }a\) is the number operator, \(\tau =gt\) and \(\delta =\Delta /2g\) is the dimensionless detuning. Using (2–4), the time evolution of the atom-field state is given by

$$\begin{aligned} |\psi (t)\rangle=\, & {} \sum _{n=0}^{\infty }\Bigg (\cos \left( \tau \sqrt{\delta ^2+\hat{n}}\right) -i\frac{\delta }{\sqrt{\delta ^2+ \hat{n}}} \sin \left( \tau \sqrt{\delta ^2+\hat{n}}\right) |n,e\rangle \nonumber \\&-i \text {e}^{-i\delta \tau }\frac{\sqrt{n+1}}{\sqrt{\delta ^{2} +(n+1)}}~\sin \left( \tau \sqrt{\delta ^2+\hat{n}}\right) |n+1,g\rangle \Bigg ). \end{aligned}$$
(5)

It is clear that, this state represents the final state of the total system in \(2 \times \infty\)-dimensional space. In this context, we consider the minimum amount of entanglement that generated between the atom and the cavity. So, we have to project this final state in a \(2\times 2\) dimensional subspace, which could be done by a local action only [29]. In the two-dimensional space, the atomic-field state is collapses to be,

$$\begin{aligned} \rho _{af}(t)=\, & {} {\rho }_{11}|n,g\rangle \langle n,g|+{\rho }_{22}|n,e\rangle \langle n,e|+{\rho }_{23}|n,e\rangle \langle n+1,g|+{\rho }_{23}^{*}|n+1,g\rangle \langle n,e|\nonumber \\&+{\rho }_{33}|n+1,g\rangle \langle n+1,g|+{\rho }_{44}|n+1,e\rangle \langle n+1,e|, \end{aligned}$$
(6)

where

$$\begin{aligned} {\rho }_{11}=\, & {} \frac{P_{n-1}^{2}}{\mathcal {N}} \frac{n}{\delta ^2+n}\sin ^{2}\left( \tau \sqrt{\delta ^2+n}\right) ,\nonumber \\ {\rho }_{22}=\, & {} \frac{P_{n}^{2}}{\mathcal {N}} \Big [\cos ^{2}\left( \tau \sqrt{\delta ^2+(n+1)}\right) +\frac{\delta ^{2}}{\delta ^2+(n+1)} \sin ^{2}\left( \tau \sqrt{\delta ^2+(n+1)}\right) \Big ], \nonumber \\ {\rho }_{23}=\, & {} i\frac{P_{n}^{2}\sqrt{n+1} \text {e}^{-i\delta \tau }}{\mathcal {N} \sqrt{\delta ^2+(n+1)}}\sin \left( \tau \sqrt{\delta ^2+(n+1)}\right) \left[ \cos \left( \tau \sqrt{\delta ^2+(n+1)}\right) -i\frac{\delta \sin \left( \tau \sqrt{\delta ^2+(n+1)}\right) }{\sqrt{\delta ^2 +(n+1)}}\right] ,\nonumber \\ \rho _{32}=\, & {} \rho ^*_{23},\nonumber \\ {\rho }_{33}=\, & {} \frac{P_{n}^{2}}{\mathcal {N}} \frac{(n+1)}{\delta ^2+(n+1)}\sin ^{2}(\tau \sqrt{\delta ^2+(n+1)}),\nonumber \\ {\rho }_{44}=\, & {} \frac{P_{n+1}^{2}}{\mathcal {N}} \left[ \cos ^{2}\left( \tau \sqrt{\delta ^2+(n+1)}\right) +\frac{\delta ^2}{\delta ^2+(n+1)}\sin ^{2}\left( \tau \sqrt{\delta ^2+(n+1)}\right) \right] , \end{aligned}$$
(7)

and \(\mathcal {N}\) is the normalization factor is given by,

$$\begin{aligned} \mathcal {N}=\, & {} P_{n}^{2}+\left[ \frac{n}{\delta ^2+n} \sin ^{2}\left( \tau \sqrt{\delta ^2+n}\right) \right] P_{n-1}^2\nonumber \\&+\left[ \cos ^{2}\left( \tau \sqrt{\delta ^2+(n+1)}\right) +\frac{\delta ^2}{\delta ^2+(n+1)}\sin ^{2} \left( \tau \sqrt{\delta ^2+(n+1)}\right) \right] P_{n+1}^{2}. \end{aligned}$$
(8)

3 Teleporting quantum Fisher information

In this section, we quantify the amount of the teleported quantum Fisher information that contained in a two-qubit state, by means of quantum teleportation. We investigate the effect of the filed and atomic parameters on the behavior of the quantum Fisher information.

3.1 Teleportation protocol

In this context, we use a protocol suggested by Cola and Paris [30] to teleport a two-qubit system via a single quantum channel. This protocol based on using a single entangled two-qubit pair, additional qubit introduced by the receiver and the transmission of three classical bits. In our contribution, the users Alice and Bob share the generated partial entangled state Eq. (6). Alice is given unknown state defined by \(\rho _{un}=|\psi _{un}\rangle \langle \psi _{un}|\), where,

$$\begin{aligned} |\psi _{un}\rangle =\cos \theta |10\rangle +\text {e}^{i\varphi /2}\sin \theta |01\rangle , \end{aligned}$$
(9)

with \(0 \le \theta \le \pi\) and \(0 \le \varphi \le 2 \pi\). The aim of Alice is to send this state to Bob using Eq. (6). To implement this process, the users follow Cola and Paris protocol [30]. At the end of this process, Bob will get the state [31]:

$$\begin{aligned} \rho _{\text {Bob}}=\sum _{\mu \nu }P_{\mu \nu }(\sigma _{\mu } \otimes \sigma _{\nu })\rho _{in}(\sigma _{\nu }\otimes \sigma _{\mu }), \end{aligned}$$
(10)

where \(P_{\mu \nu }=Tr[E^{\mu }\rho _{af}]Tr[E^{\nu }\rho _{af}]\), \(\sum _{\mu \nu }P_{\mu \nu }=1\), and \(\sigma _{\mu ,\nu }\) (\(\mu , \nu =0,x,y,z\)) are the three components of the Pauli operators and \(\sigma _{0}\) is the identity operator. The operators \(E^i,~i=1,2,3,4\) are defined as,

$$\begin{aligned} E^{0}=\, & {} |\psi ^{-}\rangle \langle \psi ^{-}|, \quad E^{1}=|\phi ^{-}\rangle \langle \phi ^{-}|, \\ E^{2}=\, & {} |\phi ^{+}\rangle \langle \phi ^{+}|, \quad E^{3}=|\psi ^{+}\rangle \langle \psi ^{+}|, \end{aligned}$$

where \(|\psi ^{\pm }\rangle =\frac{1}{\sqrt{2}}({|01\rangle +|10\rangle })\) and \(|\phi ^{\pm }\rangle =\frac{1}{\sqrt{2}}({|00\rangle +|11\rangle })\) are Bell states [12]. In the two dimensional basis set \(\{|n,g\rangle , |n,e\rangle , |n+1,g\rangle , |n+1,e\rangle \}\), Bob’s state may be written explicitly as,

$$\begin{aligned} \rho _{Bob}=\left( \begin{array}{cccc} \varrho _{11} &{} 0 &{} 0 &{} 0 \\ 0 &{} \varrho _{22} &{} \varrho _{23} &{} 0 \\ 0 &{} \varrho _{32} &{} \varrho _{33} &{} 0 \\ 0 &{} 0 &{} 0 &{} \varrho _{44} \\ \end{array}\right) , \end{aligned}$$
(11)

where,

$$\begin{aligned} \varrho _{11}=\, & {} (\rho _{11}+\rho _{44})(\rho _{22} +\rho _{33})\sin (2\theta )\cos (\varphi /2) ,\nonumber \\ \varrho _{22}=\, & {} (\rho _{23}+\rho _{32})^{2}\sin ^{2}(\theta ), \nonumber \\ \varrho _{23}=\, & {} (\rho _{22}+\rho _{33})^{2}\cos (\theta )\sin (\theta ) \text {e}^{i\varphi /2}+(\rho _{11}+\rho _{44})^{2}\cos (\theta )\sin (\theta ) \text {e}^{-i\varphi /2}, \nonumber \\ \varrho _{32}=\, & {} (\rho _{22}+\rho _{33})^{2}\cos (\theta )\sin (\theta ) \text {e}^{-i\varphi /2}+(\rho _{11}+\rho _{44})^{2}\cos (\theta )\sin (\theta ) \text {e}^{i\varphi /2}, \nonumber \\ \varrho _{33}=\, & {} (\rho _{23}+\rho _{32})^{2}\cos ^{2}(\theta ),\nonumber \\ \varrho _{44}=\, & {} \rho _{11}. \end{aligned}$$
(12)

Now, if we consider the effect of a post partial measurement, Bob gets the teleported state \(\rho _{\text {out}}^{A}\) out whose three Bloch vector components are

$$\begin{aligned} s_{x}=\, & {} \sin (\theta ) \cos (\varphi )[\rho _{22}+\rho _{33})^{2} +(\rho _{11}+\rho _{44})^{2}], \nonumber \\ s_{y}=\, & {} 2 \sin (\varphi )[(\rho _{11}+\rho _{44})^{2} -(\rho _{22}+\rho _{33})^{2}], \nonumber \\ s_{z}=\, & {} -4 \cos (\theta ) [\mathfrak {R}(\rho _{23})]^{2}, \end{aligned}$$
(13)

where \(\rho _{ij}\) given by Eq. (7)

3.2 Fisher information

In the estimation theory, the quantum Fisher information plays a central role. There are some parameters may cannot be quantified directly, so quantum Fisher information can be used to estimate these parameters. QFI is formally generalized from the classical one, and there are several variants of quantum versions of Fisher information, among which the one that based on the symmetric logarithmic derivative operator has been used most widely.

  • Fisher information of two-qubit system

    Assume that we have a two-qubits system which depends on a parameter, \(\kappa\), then the amount of Fisher information that encoded in the parameter \(\kappa\) is defined as [8, 10]

    $$\begin{aligned} {\mathcal{F}}_{\kappa }=Tr[\rho _{\kappa } {{\mathcal{L}}_{\kappa }^{\rho }}] =Tr[(\partial _{\kappa }\rho _{\kappa }) {\mathcal{L}}_{\kappa }], \end{aligned}$$
    (14)

    where \({\mathcal {L}}_{\kappa }\) is the so-called symmetric logarithmic derivative, which is determined by \(\partial _{\kappa }\rho _{\kappa }=({\mathcal{L}}_{\kappa } \rho _{\kappa } +\rho _{\kappa }{\mathcal{L}}_{\kappa })/2\) with \(\partial _{\kappa }=\partial /\partial _{\kappa }\). Making use of the spectrum decomposition, one can diagonalize the matrix as \(\rho _{\kappa }=\sum _{n} \lambda _{n}|\psi _{n}\rangle \langle \psi _{n}|\). And then the QFI can be rewritten as [32]

    $$\begin{aligned} {\mathcal{F}}_{\kappa }=\sum _{n} \frac{(\partial _{\kappa }\lambda _{n})^{2}}{\lambda _{n}}+\sum _{n}\lambda _{n}{\mathcal{F}}_{\kappa ,n} -\sum _{n\ne m} \frac{8\lambda _{n} \lambda _{m}}{\lambda _{m} +\lambda _{n}}|\langle \psi _{n}|\partial _{\kappa }\psi _{m}\rangle |^{2}, \end{aligned}$$
    (15)

    where \({\mathcal{F}}_{\kappa ,n}\) is the QFI for pure state \(|\psi _{n}\rangle\) with the form \(\mathcal{F}_{\kappa ,n} = 4[ \langle \partial _{\kappa } \psi _{n}|\partial _{\kappa } \psi _{n}\rangle -|\langle \psi _{n}|\partial _{\kappa }\psi _{n}\rangle |^{2} ]\).

  • Fisher information of a single-qubit

    It is well known that a single qubit state \(\rho\), may be defined by its Bloch vector as \(\rho =\frac{1}{2}(1+\overrightarrow{s}.\hat{\sigma })\) in the Bloch sphere representation, where \(\overrightarrow{s}=(s_{x}, s_{y}, s_{z})\) is the real Bloch vector and \(\hat{\sigma }=(\hat{\sigma }_{x}, \hat{\sigma }_{y}, \hat{\sigma }_{z} )\) denotes the Pauli matrices. According to Ref. [33], a simple and explicit expression of QFI could be obtained in terms of the Bloch sphere representation \({\mathcal {F}}_{\kappa }\)

    $$\begin{aligned} {\mathcal{F}}_{\kappa } = \left\{ \begin{array}{ll} |\partial _{\kappa } \overrightarrow{s}|^{2} +\frac{(\overrightarrow{s}.\partial _{\kappa } \overrightarrow{s})^{2}}{1-|\overrightarrow{s}|^{2}}, &{} \text{ if } \; |\overrightarrow{s}|<1 \\ |\partial _{\kappa } \overrightarrow{s}|^{2}. &{} \text{ if } \; |\overrightarrow{s}|=1 \end{array}\right. \end{aligned}$$

    From the perspective of parameter estimation, the larger QFI represents the higher estimation precision in general. Hence, how to improve the QFI becomes a key problem to be solved. Making use of quantum resources such as coherence, entanglement and so on, one can find that QFI of quantum systems can provide much more sensitivity than the classical ones.

4 Estimation of the teleported parameters

It is clear that, the initial teleported information is encoded in the weight and phase parameters of the state (9). In the following we estimate these parameters by evaluating the corresponds quantum Fisher information of the initial teleported state.

4.1 Estimating the weight parameter \(\theta\)

We estimate the initial weight parameter using two different methods: the first, when we consider a single qubit is teleported by tracing out the first qubit from the final teleported state. The second by estimating it from the total teleported state. Moreover, we discuss the sensitivity of the estimation degree to the amplitude of the Bloch vector and the purity of the two qubit teleported state. In addition, we investigate the effect of the number of photons inside the cavity on the estimation degree of the weight parameter.

Figure 1a describes the behavior of the quantum Fisher information with respect to the weight parameter \({\mathcal{F}}^q_{\theta }\), using the first method, namely, using the quantum Fisher information that contained in the teleported single qubit. It is assumed that, the initial teleported state is prepared with a phase \(\varphi =\pi\). This means that, \(\left| \psi _{un} \right\rangle =\cos \theta \left| 10 \right\rangle -\sin \theta \left| 01 \right\rangle\) and according to Eq. (13), the final state is polarized in y and z directions, namely \(\overrightarrow{s}=(0,s_y,s_z)\). Moreover, the atomic-field system is initially prepared in a non-resonance case, where we set \(\delta =0.5\).

Fig. 1
figure 1

a The quantum Fisher information \({\mathcal{F}}^q_\theta\), of the single teleported qubit, b the Bloch vector \(\mathcal {S}\) of the single qubit, c \({\mathcal{F}}^t_\theta\) with respect to the total teleported state, d the purity of the teleported state, \(\mathcal {P}\) where, the non-resonance case is considered, with \(\delta =0.5\), \(\varphi =\pi\) and \(n=\bar{n}=2\)

Full size image

The general behavior shows that, the maximum values of Fisher information depend on the initial weight angle \(\theta\) and the dimensionless interaction time \(\tau =gt\). As it is displayed in Fig. 1a, the Fisher information \({\mathcal{F}}^q_{\theta }\) increases gradually to reach its maximum value for the first time at \(\tau =4\). For further values of the interaction time, \({\mathcal{F}}^q_{\theta }\) decreases gradually. However, this behavior is repeated periodically. The effect of the initial weight parameter shows that, Fisher information decreases gradually to its minimum value at \(\theta =\pi /2\). This behavior changes for \(\theta \in [3\pi /4, \pi ]\), where the Fisher information increases to reach its maximum bounds at \(\theta =\pi\).

Figure 1b shows the behavior of the amplitude of the Bloch vector, where the non-resonance case is considered. The behavior of the Bloch vector is similar to that predicated for the quantum Fisher information, namely \(\mathcal {S}\) of the final state of the single qubit is periodically maximized. The lowest bounds of amplitude of the Bloch vector are displayed at \(\theta =\pi /2\), namely the initial state is initially prepared in the state \(\left| \psi _{un} \right\rangle =\text {e}^{i\varphi }\left| 01 \right\rangle\). For larger values of \(\theta\), the amplitude of the Bloch vector increases suddenly to reach its maximum values at \(\theta =\pi\).

In Fig. 1c, we display the behavior of the quantum Fisher information for the total teleported state, Eq. (12), \({\mathcal{F}}^t_{\theta }\). It is clear that, the behavior is similar to that predicated for the single qubit (Fig. 1a), but the maximum bounds are smaller. Moreover, the decay rate is larger than that displayed for the single qubit. In addition, the minimum bounds of \({\mathcal{F}}_\theta ^t\) are displayed around \(\theta =\pi /2\). The periodical time is smaller than that displayed in Fig. 1a for the single qubit.

The purity of the final teleported state is displayed in Fig. 1d, where at \(\tau =0\) and \(\theta =0\), the purity \(\mathcal {P}\) is zero. As \(\theta\) is increased in the interval \([0,~\pi /2]\), the purity increases gradually to reach its maximum values at \(\theta =\pi /2\). For further values of \(\theta\), the purity decreases gradually to completely vanish at \(\theta =\pi\).

From Fig. 1, one may conclude that the possibility of estimating the weight parameter of the teleported state increases as the amplitude of the Bloch vector of the single qubit or the purity of the total teleported state increases. One can estimate the initial weight parameter with large degree by estimating it using the single teleported qubit.

Fig. 2
figure 2

The same as Fig. 1, but \(\varphi =\pi /2\)

Full size image

In Fig. 2, we investigate the behavior of the quantum Fisher information, the amplitude of the Bloch vector and the purity of the total teleported state for different initial state settings, where we set that \(\varphi =\pi /2\). This means that, the initial state is prepared in the state \(\left| \psi _{un} \right\rangle =\cos \theta \left| 10 \right\rangle +\frac{1+i}{\sqrt{2}}\sin \theta \left| 10 \right\rangle\) and the final state is polarized in y and z directions, namely \(\overrightarrow{s}=(0, s_{y}, s_z)\). In Fig. 2a, the behavior of the quantum Fisher information \({\mathcal{F}}^q_\theta\) is displayed, where it displays that the maximum bounds are very large compared with that displayed at \(\phi =\pi\). Moreover, many frozen areas are displayed of the quantum Fisher information. Figure 2b, shows the behavior of the amplitude of the Bloch vector \(\mathcal {S}\) for the single qubit. The general behavior, the upper bounds are smaller than that displayed in Fig. 1b. The maximum bounds are reached periodically. The estimation of the weight parameter by quantifying the Fisher information of the teleported two-qubit state is displayed in Fig. 2c. In general, the possibility of estimating the weight parameter \(\theta\) is smaller than that displayed in Fig. 1c. This indicates that, the initial phase has a clear effect on the estimation degree of the weight parameter. The possibility of freezing the Fisher information increases for this initial state settings. Similar behavior is displayed for the purity, where as it displayed in Fig. 2d, the maximum bounds are smaller than those displayed in Fig. 1d.

Again as the purity of the teleported state increases, the possibility of estimating the weight parameter increases. Moreover, one can freeze the quantum Fisher information by controlling the phase of the initial teleported state.

Fig. 3
figure 3

The quantum Fisher information \({\mathcal{F}}^q_\theta\), of the single teleported qubit, b the Bloch vector \(\mathcal {S}\) of the single qubit, c \({\mathcal{F}}^t_\theta\) with respect to the total teleported state d the purity of the teleported state, \(\mathcal {P}\) where, the resonance case \(\delta =0\) with \(\varphi =\pi\) and \(n=\bar{n}=2\)

Full size image

Figure 3 describes the behavior of the four physical quantities, \({\mathcal{F}}^q_{\theta }, \mathcal {S}, {\mathcal{F}}^t_\theta\) and the purity \(\mathcal {P}\), in the resonance case between the atom and the field, namely we set the dimensionless parameter \(\delta =0\). It is clear that, as it depicted in Fig. 3a, the maximum bounds of \({\mathcal{F}}^q_\theta\) are larger than those displayed in Fig. 1a (non-resonance case). In addition, as the initial weight parameter, \(\theta\) increases, the quantum Fisher information \({\mathcal{F}}^q_\theta\) decreases gradually, where the minimum bounds are displayed at \(\theta \in [\pi /2,3\pi /4]\), while the maximum peaks appear periodically as the \(\tau\) increases. However, the decay rate is smaller than that shown for the non-resonance case. The behavior of the Bloch vector of the teleported state is displayed in Fig. 3b. The behavior is completely different from that displayed in Fig. 1b, where the maximum bounds are displayed at \(\theta =\pi\), while the minimum values are depicted at \(\theta =0,\pi\). In addition, similarly to Fig. 1b, the maximum bounds are displayed periodically at larger values of the dimensionless time \(\tau\). The quantum Fisher information \({\mathcal{F}}^q_{\theta }\) is displayed in Fig. 3c. In general, it behaves similarly to the non-resonance case, but the upper bounds are smaller compared with those displayed in Fig. 1c. In addition, as it is displayed in Fig. 3d, the purity increases as the initial phase \(\varphi\) increases to reach its maximum values at \(\varphi =\pi /2\). However, at further values of \(\varphi \in [\pi /2,\pi ]\), the purity decreases. It is clear that, it shows the same behavior that depicted for the non-resonance case.

Fig. 4
figure 4

The same as Fig. 3 but the initial phase \(\varphi =\pi /2\)

Full size image

In Fig. 4, we display the effect of a different initial phase settings \((\varphi =\pi /2)\) on the behavior of the quantum Fisher information \({\mathcal{F}}^q_\theta\) and \({\mathcal{F}}^t_\theta\). It is clear that, one can not estimate the weight parameter or the estimation degree is almost zero. This means that, the quantum Fisher information is completely frozen. On the other hand, as it is displayed in Fig. 4b, the amplitude of the Bloch vector increases periodically as the dimensionless time increases and at some intervals of time, the telepoted single state turns into a maximum pure state. However, although it is impossible to estimate the teleported weight parameter with larger estimation degree, the teleported state keeps with its local information. In addition, from Fig. 4d, the purity in some interval of time is non-zero and increases as \(\tau\) increases. Again, this indicates that, the teleported two-qubit state has some local information.

From Figs. 1 and 3, one may conclude that, the possibility of estimating the weight parameter \(\theta\) depends on the detuning parameter. In the non-resonance case, the estimation degree of the weight parameter is larger than that displayed for the resonance case. The initial weight settings \(\theta\) has the same effect on resonance and non-resonance case, where the minimum bounds are displayed at \(\theta =\pi /2\). The initial phase may be considered as a decoherence parameter as it displayed from Figs. 2 and 4, where the Fisher information is frozen at different interval time for the non-resonance case. However, the quantum Fishier information is completely frozen for the resonance case.

Fig. 5
figure 5

The same as Fig. 4 but we set \(n=4\) and \(\bar{n}=2\)

Full size image

To investigate the effect of the number of photons on the four physical quantities, we plot Fig. 5, where we set the initial phase \(\varphi =\pi /2\) and the number of photon \(n=4\). It is clear that, the number of photons has no effect on the estimation degree of the weight parameter, where the quantum Fisher information is a almost frozen as it depicted from Fig. 5a, c. On the other hand, the amplitude of the Bloch vector of the single teleported state increases as \(\tau\) increases. Moreover, the initial weight has no effect where, the periodic behavior is displayed between a minimum and maximum bounds. Fig. 5d shows that the purity of the teleported two-qubit state increases periodically as the \(\tau\) increases. Comparing Figs. 4b and 5b, one can see that the larger numbers of photon inside the cavity increases the oscillations number between the maximum and minimum bounds,

From Figs. 4a, c and 5a, c, we can see that, the Fisher information is completely frozen and the estimation degree is almost zero, but the amplitude of the Bloch vector as well as the purity of the teleported two-qubit state are not vanish. This means that, the telepoted state has its own local information. Namely, one can not estimate the parameters which contain all the teleported information.

Fig. 6
figure 6

a, c The quantum Fisher information \({\mathcal{F}}^q_\theta\) and b, d \({\mathcal{F}}^t_\theta\) where \(\varphi =\pi\), \(n=\bar{n}=2\) and \(\delta =0.5,1\) for a, b and c, d, respectively

Full size image

We have shown that, one can freeze the quantum Fisher information by choosing a particular values of the initial phase. However, at some values of the phase, the quantum Fisher information does not completely frozen as it shown at \(\varphi =\pi\). In Fig. 6a, b, we display the frozen region of the quantum Fisher information, \({\mathcal{F}}^q_\theta\) and \({\mathcal{F}}^t_\theta\) respectively, where we set the detuning parameter \(\delta =0.5\). The degree of brightness indicates the estimation degree of the weight parameter. It is clear, that the frozen areas of the quantum Fisher information of the teleported two qubit state are larger than those displayed for the single teleported state. The degree of frozen decreases as the initial weight increases. Figure 6c, d show the effect of the larger detuning parameter \((\delta =1)\), on the frozen areas. It is clear that, the behavior is similar to that displayed at \(\delta =0.5\). However, the upper bounds are smaller than those displayed in Fig. 6a, b. The frozen areas missed their symmetric and the deformation of these areas depicted clearly for \({\mathcal{F}}^t_\theta\).

From Fig. 6, one may conclude that, the possibility of estimating the weight parameter decreases as the initial detuning between the atom and the cavity increases. Moreover, the sizes and the deformation of the frozen areas decrease at larger values of the detuning.

4.2 Estimating the phase parameter \(\varphi\).

In this section, we estimate the initial phase for the teleported single state and the teleported two-qubit state at different initial settings.

Fig. 7
figure 7

a The quantum Fisher information \({\mathcal{F}}^q_\varphi\), of the single teleported qubit, b the Bloch vector \(\mathcal {S}\) of the single qubit, c \({\mathcal{F}}^t_\varphi\) with respect to the total teleported state d the purity of the teleported state, \(\mathcal {P}\) where, the non resonance case, when \(\delta =0.5\), \(\theta =\pi /2\), \(n=2\) and \(\bar{n}=2\)

Full size image

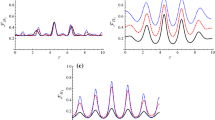
In Fig. 7, we consider the non-resonance case, where we set \(\delta =0.5\). Similarly to the Fisher information with respected to the weight parameter, the upper bounds are displayed periodically and the maximum values are depicted at larger values of the Bloch vector as it is shown from Fig. 7a, b. The phenomenon of the freezing, the sudden changes of the quantum Fisher information \({\mathcal{F}}^q_{\varphi }\) are depicted periodically. The quantum Fisher information as well as the amplitude of the Bloch vector are independent from the initial phase. The estimation degree of the phase parameter using the teleported two-qubit state is smaller than that obtained if one use the teleported single state. Similarly to that displayed of \({\mathcal{F}}^q_\varphi\), the \({\mathcal{F}}^t_\varphi\) is independent of the initial phase as it is displayed from Fig. 7c. The phenomena of the sudden decreasing/increasing are depicted periodically.

Fig. 8
figure 8

a, b The quantum Fisher information \({\mathcal{F}}^q_\varphi\), of the single teleported qubit and c, d represents \({\mathcal{F}}^t_\varphi\) with respect to the total teleported state where, \(\delta =0\), \(\theta =\pi /2, \bar{n}=2\) and a, b \(n=2\), c, d \(n=4\)

Full size image

Figure 8a, b describes the behavior of the quantum Fisher information of the single teleported qubit and the teleported two-qubit system. The general behavior displays that, the Fisher information reaches its maximum values periodically. As it is shown in Fig. 8a, b, the upper bounds of the quantum Fisher information \({\mathcal{F}}^q_\varphi\) and \({\mathcal{F}}^t_\varphi\) are displayed periodically. Moreover, the sudden changes of the quantum Fisher information is displayed. The maximum bounds are depicted at \(\varphi =\pi /2, 3\pi /2\), where the estimation degree of \({\mathcal{F}}^q_\varphi\) is larger than that depicted for \({\mathcal{F}}^t_\varphi\). From these results, one can show that, the possibility of estimating the phase parameter from the single teleported state is larger than that estimated using the teleported two-qubit state. In Fig. 8c, d, we investigate the effect of lager number of photons on the estimation degree, where we set \(n=4\). The behavior is similar to that displayed in Fig. 8a, b. But the number of peaks is larger than that displaced at smaller values of n. The minimum values are displayed around \(\pi /2\) and \(3\pi /2\) for \({\mathcal{F}}^q_\varphi\), while the maximum bounds are shown around \(\pi /2\) and \(3\pi /2\) for \({\mathcal{F}}^t_\varphi\).

From Figs. 7 and 8, one can conclude that, the maximum estimation degree of the phase parameter is displayed in the non-resonance case. The possibility of freezing the quantum Fisher information in the resonance case is larger than that shown for the non-resonance case.

Fig. 9
figure 9

Contour graph for the Fisher information for the single qubit (a, c) and for the two qubit (b, d) with respect to the phase parameter with \(\theta =\pi /2\), \(n=2\), \(\bar{n}=2\) and a, b \(\delta =0.5\), c, d \(\delta =1\)

Full size image
Fig. 10
figure 10

The figures a, c represent the Contour behavior of \({\mathcal{F}}^{q}_\varphi\) and b, d represent \({\mathcal{F}}^{t}_\varphi\) in resonance case, where \(\theta =\pi /2,\bar{n}=2\) and \(n=2\) for a, b and \(n=4\) for c, d

Full size image

In Fig. 9, we investigate the effect of the detuning parameter on the quantum Fisher information. Figure 9 represents a contour graph in the space of \((\tau , \varphi )\) of \({\mathcal{F}}^q_\varphi\) and \({\mathcal{F}}^t_\varphi\) , where we set the initial detuning parameter \(\delta =0.5\). This graph displays the frozen areas of the quantun Fisher information. The dark regions indicate that the estimation degree of the Fisher information is minimum. However, it is clear that, as the interaction time increases, some bright regions are appear, namely, one may estimate the quantum Fisher information with a large degree. From Fig. 9a, b, it is clear that, when \({\mathcal{F}}^q_\varphi\) is estimated with a small degree, the estimation degree of \({\mathcal{F}}^t_\varphi\) is large. Moreover, as the brightness areas appear for \({\mathcal{F}}^q_\varphi\), the darkness regions of \({\mathcal{F}}^t_\varphi\) are predicated. The frozen dark/bright regions appear periodically and independent of the initial phase.

The effect of the larger values of the detuning parameter is displayed in Fig. 9c for \({\mathcal{F}}^q_\varphi\) and Fig. 9d for \({\mathcal{F}}^t_\varphi\), where we set \(\delta =1\). It is clear that, the dark/bright regions appear periodically as \(\tau\) increases. As it displayed from Fig. 9c, the brightness degree of the frozen areas that represents \({\mathcal{F}}^q_\varphi\) increases as \(\tau\) increases, while for \({\mathcal{F}}^t_\varphi\) depends on the initial phase.

Figure 10, describes the frozen regions of the quantum Fisher information in the resonance case. The darkness/brightness degree of the frozen areas, indicates the estimation degree of the quantum Fisher information. These regions appear periodically and the size of these regions decreases as the scaled time increases. The darkness regions, namely, the minimum estimation degree are depicted almost if the initial phase \(\varphi \in [0,\pi /4]\bigcup {[}\pi ,5\pi /4]\), while the most brightness are predicated at \(\varphi \in [\pi /2,3\pi /4]\bigcup [3\pi /2,7\pi /4]\).

However, these results are displayed at smaller values of the photons number inside the cavity, where we set \(n=2\). The effect of a large number of photons on the size of the frozen areas is displayed in Fig. 10c, d, where we set \(n=4\). From Fig. 10c, we can see that the number of the frozen areas increases as the photon number increases. The estimation degree of \({\mathcal{F}}^{q}_\varphi\) increases and \({\mathcal{F}}^{t}_\varphi\) decreases as the scaled interaction time \(\tau\) increases. The frozen bright/dark areas that are depicted for \({\mathcal{F}}^{q,t}_\varphi\) are periodically depicted as \(\tau\) increases. However, the brightness degree of the frozen ares for \({\mathcal{F}}^{q}_\varphi\) does not depend on the initial phase \(\varphi\), while for that displayed for \({\mathcal{F}}^{t}_\varphi\) increases as the initial phase \(\varphi\) increases and the most brightness areas are centered around \(5\pi /4\) and \(7\pi /4\). However, the most dark areas are displayed around \(\varphi \in [0,~\pi /4]\bigcup [\pi , ~5\pi /4]\) for \({\mathcal{F}}^{t}_\varphi\), while for \({\mathcal{F}}^{q}_\varphi\) similarly but periodically with the bright regions.

5 Conclusion

In this contribution, we use a partial entangled state which is generated between a single atom and a single cavity mode, to teleport a two-qubit system between two users; Alice and Bob. The initial parameters of the teleported state; the weight and the phase parameters are estimated, by means of the teleported quantum Fisher information. These parameters are estimated from the teleported two-qubit state and the single qubit state. The relation between the purity of the teleported state and the quantum Fisher information is discussed. The effect of the initial detuning parameter and the number of photons inside the cavity on the estimation degree of the initial telepored parameters is discussed.

Our results show that, the estimation degree of the parameters of the initial teleported state settings, increases as the amplitude of the Bloch vector of the single qubit and the purity of the total teleported state increases. The possibility of estimating the initial weight and the phase parameters by using a single qubit is larger than that displayed for the teleported two-qubit system. It is shown that, by controlling the initial state settings, the quantum Fisher information may be frozen, meanwhile, the teleported state keeps its local information. The frozen degree, dark/bright indicates the estimation degree of the teleported parameters.

The estimation degree depends on the initial parameters of the atomic-field system, where the possibility of estimating the teleported parameter in the resonance case is larger than that depicted for the non-resonance case. The upper and lower bounds of the quantum Fisher information are displayed periodically. The size of the frozen areas decreases as the detuning parameter increases. The frozen areas of quantum Fisher information that are displayed at smaller values of the detuning parameter are regular and symmetric. However, as the detuning parameter increases, the frozen areas are deformed

The effect of the photons number inside the cavity is investigated, where the larger number of photons increases the speed of oscillating the quantum Fisher information between their maximum and minimum bounds. It is shown, as one increases the number of photon inside the cavity, the upper bounds of the quantum Fisher information are larger than that displayed at smaller number of photons. The number of the frozen areas increases at the expense of their sizes.

In conclusion: it is possible to freeze the teleported parameters by controlling the initial atomic-field parameters and meanwhile the teleported state keeps its local information. These results may be useful in the context of secure quantum communication.