Spatiotemporal coherence properties of broadband Gaussian Schell-model beams propagating through atmospheric turbulence

Abstract

The spatiotemporal coherence properties of broadband Gaussian Schell-model (GSM) beams with different spectral bandwidths propagating through atmospheric turbulence are numerically calculated and analyzed. The results show that although the spatial coherence properties of an intermediate-broadband GSM beam almost do not depend on the spectral bandwidth, those of its ultra-broadband counterpart do. The temporal coherence of an ultra-broadband GSM beam not only has radial dependence in the observation plane, but also varies with the increasing propagation distance; however, the same behavior does not hold for an intermediate-broadband GSM beam of which the temporal coherence remains nearly invariable as the radial distance of the observation point or propagation distance changes.

1 Introduction

In the recent years, various types of broadband light sources have been reported, such as Ti:sapphire pulsed lasers, super-luminescent diodes and super-continuum sources [1–5]. Although Ti:sapphire pulsed lasers and super-luminescent diodes generally output optical beams with a spectral bandwidth of several ten nanometers, the light emitted from super-continuum sources can have a spectral bandwidth of several hundred nanometers. The significant development of these light sources has stimulated both experimental and theoretical interest in the propagation of broadband optical fields [3, 6–12].

The propagation-induced coherence evolution of random optical fields is a subject in which many scientists have shown a lot of interest for several decades. Due to the mathematical tractability, the Gaussian Schell-model (GSM) beam has been widely used to analytically describe a beam-like random optical field generated by a spatially partially coherent source. Traditionally, the coherence properties of random optical fields have been treated in either the space–time or space-frequency domain. The space-frequency coherence properties of a GSM beam propagating in free space were analytically characterized in detail by Ref. [13]. More recently, researchers [14–16] have also studied the space-frequency coherence properties of GSM beams passing through atmospheric turbulence. The equal-time complex degree of coherence for broadband GSM array beams passing in free space has been analyzed by Ref. [17], where the effects of atmospheric turbulence on the spatiotemporal coherence properties of GSM beams were not considered, however. The spatiotemporal coherence properties of quasi- and non-monochromatic GSM beams passing in atmospheric turbulence were investigated by Refs [18, 19], respectively. The changes in coherence properties of non-monochromatic optical fields in atmospheric turbulence can play an important role in clarifying some optical correlation phenomena in applications such as interferometry and holography. As mentioned above, existing broadband light sources can emit a beam with a spectral bandwidth varying from several ten to several hundred nanometers. Owing to the wide range of values that the spectral bandwidth of available broadband optical fields can take on, the effects of the spectral bandwidth on the spatiotemporal coherence properties of the fields propagating in atmospheric turbulence should be investigated more carefully than that have been done up to now. One goal of this paper is to better understand the conditions under which the spatial coherence properties in the space–time domain of a broadband optical field have significant dependence on the spectral bandwidth, differing from those of its quasi-monochromatic counterpart. For purposes of description convenience, in this paper, the light with a spectral bandwidth of several ten nanometers will be referred to as the intermediate-broadband optical field, and that with a spectral bandwidth of several hundred nanometers will be called the ultra-broadband one.

It is well known that the free-space propagation enlarges the lateral coherence length of a GSM beam, and, in contrast, the atmospheric turbulence decreases it. Furthermore, the propagation-induced spatiotemporal coupling between the spatial and temporal coherence of a broadband random optical field can generally occur even though the mutual coherence function in the source plane can be factorized into a product of the separable space- and time-dependent parts. Hence, another goal of this paper is to examine the evolution behavior of spatiotemporal coherence properties of a broadband GSM beam propagating from the source to observation plane in atmospheric turbulence.

2 Theoretical formulations

We consider a broadband GSM beam propagating along the positive z axis from the source plane at z = 0 to an observation plane at z = L in atmospheric turbulence. Let V(r, z, t) be the analytic signal representation of the beam-like optical field at the time t and position (r, z), where r denotes a two-dimensional position vector in a plane transverse to the z axis. If we suppose that the field is statistically stationary, at least in the wide sense, the mutual coherence function of the field in the source plane can be written as

$$ \Upgamma^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,\tau ) = \left\langle {V({\mathbf{r}}_{1} ,0,t + \tau )V^{*} ({\mathbf{r}}_{2} ,0,t)} \right\rangle_{s} , $$

(1)

where $\left\langle \cdot \right\rangle_{s}$ denotes an ensemble average over the source statistics, the asterisk represents the complex conjugation. In this work, we consider the case that the field in the source plane is cross-spectrally pure [13], i.e., Г⁽⁰⁾(r ₁, r ₂, τ) takes the form

$$ \Upgamma^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,\tau ) = \Upgamma_{s}^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} )\Upgamma_{t}^{(0)} (\tau ), $$

(2)

where Г ⁽⁰⁾ _s (r ₁, r ₂) and Г ⁽⁰⁾ _t (τ) denote the separable space- and time-dependent parts of the mutual coherence function of the field, respectively. It is well known that the cross-spectral density function and mutual coherence function form a Fourier transform pair [13]

$$ W^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,\omega ) = \frac{1}{2\pi }\int \limits _{ - \infty }^{\infty } {\Upgamma^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,\tau )\exp (i} \omega \tau )d\tau = \Upgamma _{s}^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} )S(\omega ), $$

(3)

where S(ω) is the Fourier transform of Γ ⁽⁰⁾ _t (τ), denoting the spectrum distribution of the field. Here, we use the model spectrum distribution proposed by Turunen [3], which is given by

$$ S(\omega ) = \frac{1}{{\Upgamma (2n)\omega_{0} }}\left( {\frac{2n\omega }{{\omega_{0} }}} \right)^{2n} \exp \left( { - \frac{2n\omega }{{\omega_{0} }}} \right),\omega > \, 0, $$

(4)

where Γ(∙) denotes the Gamma function, n characterizes the spectral bandwidth of the field, and ω ₀ is the peak angular frequency which is associated with the peak wavelength by λ ₀ = 2πc/ω ₀, c is the speed of light. For the model spectrum distribution given by (4), a larger n corresponds to a narrower spectral bandwidth. It should be stressed that ω ₀ in (4) cannot be zero.

For a collimated GSM beam, Г ⁽⁰⁾ _s (r ₁, r ₂) is given by [20]

$$ \Upgamma_{s}^{(0)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ) = \Upgamma_{0} \exp \left( { - \frac{{{r}_{1}^{2} + {r}_{2}^{2} }}{{4\sigma^{2} }} - \frac{{\left| {{\mathbf{r}}_{1} - {\mathbf{r}}_{2} } \right|^{2} }}{{2\delta^{2} }}} \right), $$

(5)

where Γ₀ is a constant independent of both the positions and time, σ characterizes the beam radius, δ is the initial lateral correlation length, r _j  = |r _j | (j = 1, 2). Here both σ and δ are assumed to be the same for all the various frequency components of the beam in the source plane. Based on the extended Huygens–Fresnel principle [21] together with the paraxial approximation [21], the cross-spectral density function of a broadband GSM beam propagating through atmospheric turbulence to an observation plane at z = L is given by [20]

$$ \begin{gathered} W({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,L,\omega ) = \frac{{k^{2} }}{{4{{\uppi}}^{2} L^{2} }}\int {\int \limits_{ - \infty }^{\infty } {{\text{d}}^{2} \rho_{1} \int {\int \limits _{ - \infty }^{\infty } {{\text{d}}^{2} \rho_{2} W^{(0)} ({\varvec{\rho}}_{1} ,{\varvec{\rho}}_{2} ,\omega )} } } } \exp \left( { - ik\frac{{\left| {{\mathbf{r}}_{1} - {\varvec{\rho}}_{1} } \right|^{2} - \left| {{\mathbf{r}}_{2} - {\varvec{\rho}}_{2} } \right|^{2} }}{2L}} \right) \\ \times \left\langle {\exp [\psi^{*} ({\varvec{\rho}}_{1} ,{\mathbf{r}}_{1} ,L,\omega ) + \psi ({\varvec{\rho}}_{2} ,{\mathbf{r}}_{2} ,L,\omega )]} \right\rangle_{m} , \\ \end{gathered} $$

(6)

where k = ω/c is the wavenumber, ψ(ρ _j , r _j , L, ω) is the random part of the complex phase of a spherical wave with the angular frequency ω propagating from the point (ρ _j , 0) to point (r _j , L) in atmospheric turbulence (j = 1, 2), $\left\langle \cdot \right\rangle_{m}$ denotes an ensemble average over the statistics of atmospheric turbulence. Following the procedure of the derivation of (18) presented in Ref. [14], it follows that

$$ \begin{gathered} W({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,L,\omega ) = \frac{{\Upgamma_{0} S(\omega )}}{{[\Updelta (L,k)]^{2} }}\exp \left[ {\frac{{ik{\mathbf{r}}_{s} \cdot {\mathbf{r}}_{d} }}{L} - \left( {\frac{1}{{\rho_{0}^{2} }} + \frac{{k^{2} \sigma^{2} }}{{2L^{2} }}} \right)r_{d}^{2} } \right] \\ \times \exp \left\{ {\frac{{\left| {i{\mathbf{r}}_{s} + [L/(k\rho_{0}^{2} ) - k\sigma^{2} /L] \cdot {\mathbf{r}}_{d} } \right|^{2} }}{{2\sigma^{2} [\Updelta (L,k)]^{2} }}} \right\}, \\ \end{gathered} $$

(7)

where r _s  = (r ₁ + r ₂)/2, r _d  = r ₁−r ₂, r _d  = |r _d |, Δ(L, k) = {1 + L ²[1/(4σ ²) + 1/δ ² + 2/ρ ²₀ ]/(k ² σ ²)}^1/2, ρ ₀ = (0.55C ² _n k ² L)^−3/5 is the spherical-wave lateral coherence radius due to atmospheric turbulence, C ² _n is the refraction-index structure constant characterizing the turbulence strength.

Taking the Fourier transform of (7) leads to

$$ \Upgamma^{(L)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,\tau ) = \int \limits_{0}^{\infty } {W({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,L,\omega )\exp ( - i\omega \tau ){\text{d}}\omega } . $$

(8)

It needs to be pointed out that the fact that the lower limit on the integral in (8) can be written as zero is due to W(r ₁, r ₂, L, ω) = 0, when ω < 0. It is highly nontrivial to perform an analytic evaluation of the integral with respect to ω in (8). Hence, we use numerical integration to determine the integral. The spatiotemporal coherence properties of the field are characterized by the complex degree of coherence

$$ \gamma ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,L,\tau ) = \frac{{\Upgamma^{(L)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{2} ,\tau )}}{{[\Upgamma^{(L)} ({\mathbf{r}}_{1} ,{\mathbf{r}}_{1} ,0)\Upgamma^{(L)} ({\mathbf{r}}_{2} ,{\mathbf{r}}_{2} ,0)]^{1/2} }}. $$

(9)

Based on (7–9), the spatiotemporal coherence properties of a broadband GSM beam propagating through atmospheric turbulence can be analyzed numerically.

3 Numerical calculations and remarks

Now, we numerically determine the spatiotemporal coherence properties of broadband stationary GSM beams propagating in atmospheric turbulence. The beam parameters used in the following calculations are as follows: σ = 1 cm, δ = 0.01σ, and λ ₀ = 800 nm, unless other values are specified in the figure captions. Taking into account both the recent development of broadband light sources and the convenience of comparative analysis, in the calculations the typical value of the parameter n in (4) is specified as 20 or 1,000, corresponding to a spectrum distribution with a full width at half maximum (FWHM) in angular-frequency scale of approximately 0.37ω ₀ or 0.053ω ₀. Note that with n = 20, we generally have an ultra-broadband optical field which can be generated by employing a super-continuum source and with n = 1,000, we essentially consider an intermediate-broadband optical field which can be produced by use of a super-luminescent diode.

3.1 Spatial coherence properties of broadband GSM beams

The modulus of the equal-time complex degree of coherence is a measure of spatial coherence between two points in the observation plane for an optical field. As in Refs. [14, 21], we can define the lateral coherence length L _c of a broadband GSM beam in the plane at z = L by the e ⁻¹ point of |γ(r, –r, L, 0)| in terms of the separation distance between the two symmetric observation points. For a monochromatic GSM beam with the wavenumber k ₀ = 2π/λ ₀ in atmospheric turbulence, Ricklin and Davidson [14] have derived its lateral coherence length ρ _c  = ρ ₀{1 + ρ ²₀ z ² _r /(8σ ² L ²) – [z _r ρ ₀/L–4σ ² L/(ρ ₀ z _r )]²[Δ(L,k ₀)]⁻²/(8σ ²)}^−1/2, where z _r  = 2k ₀ σ ² is independent of the propagation distance L. Figure 1 shows the lateral coherence length of a broadband GSM beam scaled by that of its monochromatic counterpart as a function of the parameter n with various combinations of the parameters C ² _n and L. It needs to be pointed out that in Fig. 1 the value of ρ _c associated with each curve varies with the different combinations of the parameters C ² _n and L. It can be found from Fig. 1 that the lateral coherence length of a broadband GSM beam is always shorter than that of its monochromatic counterpart. Notice that as previously pointed out by Ricklin and Davidson [14], the lateral coherence length of quasi-monochromatic GSM beams is approximately equal to that of their monochromatic counterparts, viz., there exists very little difference in the lateral coherence length between these two beam types. This statement is actually confirmed by Fig. 1 where the value of L _c /ρ _c rises beyond 0.99 as the parameter n increases past 100, that is, the FWHM in angular-frequency scale reduces below 0.17ω ₀, which corresponds to a FWHM in wavelength scale of 133 nm. Hence, the lateral coherence length in the space–time domain of an intermediate-broadband optical field can also be acceptably approximated by that of its monochromatic counterpart. On the other hand, with 1 < n < 100, indeed the ultra-broadband optical fields are considered. In the case of n < 100, it is observed from Fig. 1 that with the same combination of the parameters C ² _n and L, the lateral coherence length of the ultra-broadband GSM beams reduces significantly with the increasing spectral bandwidth, i.e., with the decreasing value of the parameter n. This fact manifests that the spatial coherence properties in the space–time domain of an ultra-broadband GSM beam propagating through atmospheric turbulence depend significantly on the spectral bandwidth, in contrast to those of its quasi-monochromatic counterpart, which are independent of the spectral bandwidth (cf. Ref. [18]).

Fig. 1

The scaled lateral coherence length L _c /ρ _c as a function of the parameter n with various combinations of C ² _n and L. ρ _c is the lateral coherence length of a monochromatic GSM beam with the wavelength λ ₀. σ = 1 cm, δ/σ = 0.01, and λ ₀ = 800 nm

Figure 2 demonstrates the lateral coherence length of both the broadband and monochromatic GSM beams as a function of the normalized propagation distance L/z _r under different conditions of the turbulence strength. It can be observed from Fig. 2 that for both the broadband and monochromatic GSM beams, the lateral coherence length rises initially with the increasing propagation distance until it arrives at its peak value, and then begins to reduce as the propagation distance continues to increase. Furthermore, it can also be found that the propagation distance associated with the peak point of each curve is dependent on the value of C ² _n . Physically, the effects of free-space propagation dominate the changes in the lateral coherence length before it increases to the peak point, and after that the turbulence-induced effects become the domination factor which then causes a continuous decrease in its values. It can be seen from Fig. 2 that with stronger turbulence, the lateral coherence length of a GSM beam with a given parameter n reaches the peak point at a shorter propagation distance. Incidentally, because the lateral coherence length of quasi-monochromatic GSM beams really approximates to that of their monochromatic counterparts, the curves in Fig. 2 for the monochromatic GSM beams can also be viewed as those for their quasi-monochromatic counterparts.

Fig. 2

Lateral coherence length in terms of the normalized propagation distance L/z _r for various GSM beams propagating in atmospheric turbulence. n = ∞ represents a monochromatic GSM beam. σ = 1 cm, δ/σ = 0.01, and λ ₀ = 800 nm

3.2 Temporal coherence properties of broadband GSM beams

The quantity |γ(r, r, L, τ)| is a measure of temporal coherence at the point r in the observation plane at z = L for a broadband GSM beam. Figure 3 illustrates the dependence of temporal coherence of broadband GSM beams upon the radial position in the observation plane with various combinations of the parameters n and δ, where r = (0, ξσΔ(L,k ₀)) and the quantity ξσΔ(L,k ₀) characterizes the radial distance between the observation point and beam axis. It can be seen from Fig. 3a that there exist observable changes in the profile of |γ(r, r, L, τ)| in terms of τ as the observation point r moves from the beam axis, with r = (0, 0), to the beam edge, with r = (0, σΔ(L,k ₀)), and the degree of temporal coherence with a given time delay τ grows as the radial distance increases. Hence, the temporal coherence of ultra-broadband GSM beams in the observation plane has radial dependence, in contrast to that of their quasi-monochromatic counterparts, shown by Ref. [18], being independent of the observation point. According to Fig. 3b–d, where the parameter n is changed from 20 to 1,000 or (and) the parameter δ is changed from 0.01 cm to ∞, it can be found that both the spectral bandwidth and initial lateral correlation length of the beam affect the temporal coherence properties. Comparing the Fig. 3a with b and c with d, it can be observed clearly that for an ultra-broadband GSM beam, increasing the initial lateral correlation length can enhance the degree of temporal coherence and reduce its radial dependence, and, however, for an intermediate-broadband GSM beam, the effects of the initial lateral correlation length on the degree of temporal coherence are nearly unobservable. Theoretically, the coherence time of a broadband beam is roughly inversely proportional to its spectral bandwidth [13]; this law is also manifested in Fig. 3a–d where the degree of temporal coherence with a given τ for n = 1,000 is larger than that for n = 20.

Fig. 3

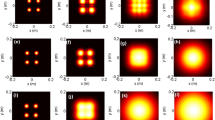
Dependence of temporal coherence of broadband GSM beams upon the radial position in the observation plane with various combinations of n and δ. σ = 1 cm, λ ₀ = 800 nm, C ² _n  = 10⁻¹⁴ m^−2/3, L = 5z _r , and r = (0, ξσΔ(L, k ₀)). a δ/σ = 0.01, n = 20; b δ/σ = ∞, n = 20; c δ/σ = 0.01, n = 1,000; d δ/σ = ∞, n = 1,000

To facilitate the following analysis, a limiting case of the broadband GSM beams with the initial lateral correlation length approaching infinity, i.e., the broadband spatially fully coherent beams, is first considered. The contours of |γ(0, 0, L, τ)| for broadband spatially fully coherent beams are shown in Fig. 4 as functions of the logarithmic normalized propagation distance log(L/z _r ) and time delay τ, where 0 in bold type denotes an on-axis observation point. Note that in Fig. 4, the horizontal interval between the leftmost and rightmost contour lines with a given value of log(L/z _r ) can be used as a simple measure of the profile of |γ(0, 0, L, τ)| in terms of τ with a fixed propagation distance L, viz., a smaller interval means a narrower profile of |γ(0, 0, L, τ)| in terms of τ.

Fig. 4

Contours of the on-axis degree of temporal coherence as functions of the logarithmic normalized propagation distance log(L/z _r ) and time delay τ for broadband spatially fully coherent beams. σ = 1 cm, δ/σ = ∞, and λ ₀ = 800 nm. a n = 20, C ² _n  = 0; b n = 1,000, C ² _n  = 0; c n = 20, C ² _n  = 10⁻¹⁴ m^−2/3; d n = 1,000, C ² _n  = 10⁻¹⁴ m^−2/3

Figure 4a, b indicates the variation of |γ(0, 0, L, τ)| with the increasing propagation distance for two spatially fully coherent beams with different spectral bandwidths propagating in free space. Theoretically, a wider profile of |γ(0, 0, L, τ)| in terms of τ implies a larger coherence time. Based on this statement, it can be seen clearly from Fig. 4a that the coherence time undergoes a minor decreasing transition from a larger value to a smaller one as the propagation distance increases. On the other hand, Fig. 4c, d shows the variation of |γ(0, 0, L, τ)| with the increasing propagation distance for two spatially fully coherent beams with different spectral bandwidths propagating in atmospheric turbulence. It can be clearly observed from Fig. 4c that there is a minor increasing transition of the coherence time from a smaller value to a larger one as the propagation distance increases. Upon comparing Fig. 4a with b, and c with d, it can be found that for an ultra-broadband GSM beam, the propagation-induced changes in the coherence time are relatively conspicuous, and, however, for an intermediate-broadband GSM beam, the coherence time hardly depends on the propagation distance. It is possible to infer from this fact that the temporal coherence of quasi-monochromatic GSM beams will become independent of the propagation distance. In fact, by introducing an approximation of replacing the varying frequency in the term W(∙) of (8) with its mean value, Ref. [18] has drawn a conclusion consistent with this inference.

Furthermore, examination of Fig. 4a, c reveals that the substantial disappearance of the aforementioned decreasing transition in Fig. 4c is due to the reason that the reduction in the coherence time of the beam caused by free-space propagation is compensated by the increase in that induced by atmospheric turbulence. In physics, the variation of the coherence time with the increasing propagation distance is due to the fact that the profile of W(0, 0, L, ω) in terms of ω changes as L increases.

Figure 5 shows the contours of |γ(0, 0, L, τ)| for ultra-broadband GSM beams with various δ. It can be seen from Fig. 5 that the variation of |γ(0, 0, L, τ)| with the increasing propagation distance for ultra-broadband GSM beams depends on the initial lateral correlation length δ. It can be found that the smaller δ is, the greater the separation distance between the aforementioned decreasing and increasing transitions of the coherence time becomes. Hence, the initial degree of spatial coherence of an ultra-broadband GSM beam affects the variations of temporal coherence with the increasing propagation distance. In contrast with Fig. 5, the contours of |γ(0, 0, L, τ)| for intermediate-broadband GSM beams are illustrated in Fig. 6. Comparing Fig. 6a, b with Fig. 5a, c, it can be observed that although the initial lateral correlation length has a significant impact on the variation of |γ(0, 0, L, τ)| with the increasing propagation distance for an ultra-broadband GSM beam, this does not hold for an intermediate-broadband one.

Fig. 5

Contours of the on-axis degree of temporal coherence as functions of the logarithmic normalized propagation distance log(L/z _r ) and time delay τ for broadband GSM beams. σ = 1 cm, λ ₀ = 800 nm, n = 20, and C ² _n  = 10⁻¹⁴ m^−2/3. a δ/σ = 0.01; b δ/σ = 0.05; c δ/σ = 0.1; d δ/σ = 1

Fig. 6

Contours of the on-axis degree of temporal coherence as functions of the logarithmic normalized propagation distance log(L/z _r ) and time delay τ for broadband GSM beams. σ = 1 cm, λ ₀ = 800 nm, n = 1,000, and C ² _n  = 10⁻¹⁴m^−2/3. a δ/σ = 0.01; b δ/σ = 0.1

In the end, we explore the effects of atmospheric turbulence on the variation of |γ(0, 0, L, τ)| with the increasing propagation distance for broadband GSM beams. With C ² _n being specified as 10⁻¹³ m^−2/3 or 0, the contours of |γ(0, 0, L, τ)| are shown in Fig. 7. For contrast, we consider two figure groups: The first consists of Fig. 7a, Fig. 5a and Fig. 7c, and the second Fig. 7b, Fig. 6a and Fig. 7d. By comparing the figures in the first group, it can be seen that the separation distance between the aforementioned decreasing and increasing transitions becomes shorter as atmospheric turbulence grows to be stronger. On the other hand, compared with the first group, the aforesaid transitions become nearly unobservable for the second group due to the much narrower spectral bandwidth.

Fig. 7

Contours of the on-axis degree of temporal coherence as functions of the logarithmic normalized propagation distance log(L/z _r ) and time delay τ for broadband GSM beams. σ = 1 cm, δ/σ = 0.01, and λ ₀ = 800 nm. a n = 20, C ² _n  = 10⁻¹³ m^−2/3; b n = 1,000, C ² _n  = 10⁻¹³ m^−2/3; c n = 20, C ² _n  = 0; d n = 1,000, C ² _n  = 0

4 Conclusions

In this paper, the complex degree of coherence for broadband GSM beams with the model spectrum distribution propagating through atmospheric turbulence has been formulated. Based on it, the spatiotemporal coherence properties of broadband GSM beams passing in atmospheric turbulence have been numerically calculated and analyzed in detail.

On the one hand, similar to the quasi-monochromatic GSM beam, the lateral coherence length of a broadband GSM beam first grows with the increasing propagation distance until it reaches its maximum value, and then decreases as the propagation distance increases further. On the other hand, there exists practically negligible difference in the lateral coherence length between an intermediate-broadband GSM beam and its quasi-monochromatic counterpart in atmospheric turbulence, and, on the contrary, the spatial coherence properties of an ultra-broadband GSM beam in atmospheric turbulence depend significantly on the spectral bandwidth and differ from those of its quasi-monochromatic counterpart.

Different from the intermediate-broadband GSM beam, whose temporal coherence hardly depends on the radial distance of the observation point, the temporal coherence of an ultra-broadband GSM beam in the observation plane has a radial dependence, i.e., the degree of temporal coherence with a given time delay grows as the radial distance of the observation point increases, which can be weakened by increasing the initial lateral correlation length.

The coherence time of an intermediate-broadband GSM beam hardly varies with the increasing propagation distance. However, for an ultra-broadband GSM beam, the coherence time does change with the varying propagation distance, viz., as the propagation distance increases, the coherence time first undergoes a decreasing transition from a larger value to a smaller one, which is caused by free-space propagation and then an increasing transition from a smaller value to a larger one, which is induced by atmospheric turbulence. It has been found that for an ultra-broadband GSM beam, a larger initial lateral correlation length or refraction-index structure constant leads to a shorter separation distance between the decreasing and increasing transitions, and in special cases the decreasing transition can even be substantially compensated by the increasing one so that it becomes nearly invisible.

The obtained results in this paper provide a better insight into the problem with respect to the spatiotemporal coherence properties of broadband GSM beams passing in atmospheric turbulence, and are useful for clarifying optical correlation phenomena of broadband beam-like optical fields in atmospheric turbulence.