On the Existence of Symmetric Bicircular Central Configurations of the 3n-Body Problem

Abstract

In this paper, we consider central configurations of the planar 3n-body problem consisting of n masses at the vertices of a regular n-gon inscribed in a circle of radius r and 2n masses at the vertices of a second (not necessarily regular) concentric 2n-gon inscribed in a circle of radius ar which are symmetric in the sense that the set of positions of the 3n masses and the set of the corresponding masses are invariant under the action of a finite subgroup of O(2). There are two different types of such configurations. In the first type, called regular bicircular central configurations of the 3n-body problem, the second 2n-gon is regular, n of the vertices of the second n-gon are aligned with the vertices of the first regular n-gon and the masses at the vertices of this 2n-gon alternate values. In the second type, called semiregular bicircular central configurations of the 3n-body problem, the second 2n-gon is semiregular and the masses at its vertices are all of them equal. A semiregular 2n-gon has n pair of vertices symmetric by a reflection of an angle $\beta$ with respect to the axis of symmetry of the first regular n-gon. Our aim is to analyze the set of values of the parameter a for the regular 2n-gon and of the parameters $(a,\beta )$ for the semiregular 2n-gon providing symmetric bicircular central configurations. In particular, for all $n\ge 2$ we prove analytically the existence of symmetric bicircular central configurations with a (respectively $(a,\beta )$) satisfying some particular conditions. Using either computer-assisted results or numerical results, we also describe the complete set of values of a (respectively $(a,\beta )$) providing symmetric bicircular central configurations for $n=2,3,4,5$ and we give numerical evidences that the pattern for $n>5$ is the same as the one for $n=5$.

1 Introduction

We consider the planar Newtonian N-body problem

$$\begin{aligned} m_k\,\ddot{\mathbf {q}}_k=-\sum _{j=1,\,j\ne k}^N G\,m_k\,m_j\,\dfrac{\mathbf {q}_k-{\mathbf {q}}_j}{|{\mathbf {q}}_k-{\mathbf {q}}_j|^3}\ , \qquad k=1,\dots ,N , \end{aligned}$$

where ${\mathbf {q}}_k\in {\mathbb {R}}^2$ is the position vector of the point mass $m_k$ in an inertial coordinate system and G is the gravitational constant, which can be taken equal to one by choosing conveniently the unit of time. A configuration of the N bodies is called central if the acceleration vector of each body is proportional to its position vector with respect to the center of mass with the same constant of proportionality. In other words, given $m_1,\dots ,m_N$ a configuration $({\mathbf {q}}_1,\dots ,{\mathbf {q}}_N)$ with ${\mathbf {q}}_i\ne {\mathbf {q}}_j$ for all $i\ne j$ is central if there exists a constant $\lambda$ such that

$$\begin{aligned} \sum _{j=1,\,j\ne k}^N m_j\,\dfrac{{\mathbf {q}}_k-{\mathbf {q}}_j}{|{\mathbf {q}}_k-{\mathbf {q}}_j|^{3}}=\lambda \,({\mathbf {q}}_k-{\mathbf {c}}), \qquad k=1,\dots ,N, \end{aligned}$$

(1)

where ${\mathbf {c}}=\sum _{k=1}^N m_k\,{\mathbf {q}}_k/\sum _{k=1}^N m_k$ is the center of mass. For a classical background on the study of central configurations, see, for instance, (Wintner et al. 1941; Hagihara et al. 1970). In this paper, we deal with symmetric central configurations having the masses at the vertices of two concentric polygons.

A (regular) polygon of n-vertices is usually called a (regular) n-gon. Concentric n-gons are called nested when the vertices of all n-gons are aligned and they are called twisted when the vertices of at least one of the n-gons is rotated by an angle of $\pi /n$ with respect to the other ones.

The simplest central configuration of the planar Newtonian N-body problem consists of N equal masses at the vertices of a regular N-gon. To our knowledge, the first author studying central configurations having the masses at the vertices of two concentric n-gons was Hoppe (1879). In his work, Hope showed that if n equal masses are placed at the vertices of a regular n-gon, then n other equal masses may be placed at the vertices of a second nested/twisted regular n-gon in such a way that the resulting configuration is central (see, for more details, Longley 1907). Since then, several authors have studied central configurations having the masses at the vertices of concentric regular n-gons. Assuming that the masses on each n-gon are equal and masses on different n-gons could be different, Klemperer (1962) gave the relation between the ratio of the masses and the ratio of the radii for two twisted regular n-gons central configurations when $n=2,3,4$. More recently, Moeckel and Simo (1995) proved that for any n and for every ratio of the masses there are exactly two nested regular n-gons central configurations one with the ratios of the sizes of the n-gons less than one and the other with the ratio of the sizes greater than one.

There are also several works studying central configurations with kn masses at the vertices of k concentric n-gons such that all the masses on the same n-gon are equal and masses on different n-gons could be different. These central configurations are called a crown of k rings of n bodies or simply a (k, n)-crown in Barrabés and Cors (2019), but since only few authors use this nomenclature, we will not use it here. Llibre and Mello (2009) proved the existence of central configurations of three twisted regular n-gons with $n=2,3$ and of four twisted regular 2-gons. Corbera et al. (2009) proved the existence of central configurations of p nested regular n-gons for all $p\geqslant 2$ and $n\geqslant 2$ (see also Hénot and Rousseau 2019). Zhao and Chen (2015) proved the existence of central configurations of the $(pn+gn)$-body problem with pn masses at the vertices of p nested regular n-gons and gn masses at the vertices of g twisted regular n-gons. Barrabés and Cors (2019) derived the generic equations of central configurations having kn masses at the vertices of k concentric regular n-gons. They also prove the existence of at least a two twisted regular n-gons central configuration for any mass ratio and for any $n\geqslant 1$, and they give the exact number and location of two twisted n-gons central configurations for $n=3,4$.

Up to here, it is assumed that the masses on each n-gon are equal and masses of different n-gons could be different and that the n-gons are either nested or twisted by an angle of $\pi /n$. Without imposing the condition that the masses on each n-gon are equal, Zhang and Zhou (2003) proved that if the configuration with 2n masses at the vertices of two nested/twisted n-gons is central, then the masses on each n-gon must be equal. Assuming that the n-gons can be twisted by an angle $\theta \in [0,\pi /n]$, Yu and Zhang (2012) proved that if a configuration having n equal masses at the vertices a regular n-gon and n additional masses at the vertices of a second concentric n-gon rotated by an angle of $\theta$ with respect to the first one is central, then the angle $\theta$ must be either 0 or $\pi /n$.

The configurations considered in all the previous papers are highly symmetric. Montaldi (2015), using variational arguments, proved that for every possible symmetry type given by a finite subgroup of O(2) and for any symmetric choice of the masses there is at least a central configuration. In particular, he gives an alternative proof for the existence of nested/twisted regular n-gons central configurations when all the masses on each n-gon are equal and masses of different n-gons could be different. He also proved the existence of symmetric central configurations of concentric polygons that are combinations of nested/twisted regular n-gons and semiregular 2n-gons. Notice that in this last cast the number of masses on each concentric polygon is not necessarily equal. See below for a more precise explanation of Montaldi’s work.

There are some other works studying central configurations with the masses at the vertices of concentric polygons having different number of vertices. Yu and Zhang (2015) proved that if a configuration with n-equal masses at the vertices of a regular n-gon and $\ell$ equal masses at the vertices of a regular $\ell$-gon with a common center is central, then $n=\ell$. Siluszyk (2014) and Siluszyk (2017) studied central configurations consisting of n equal masses at the vertices of a regular n-gon and 2n masses at the vertices of a second concentric 2n-gon with a common center. In particular in Siluszyk (2014), the author found the expressions of the masses in function of the sizes of the n-gons and in Siluszyk (2017), by using computer-assisted methods, she studied the existence of central configurations of this type for $n=2$. Marchesin (2019) proved the existence of central configurations consisting of 4 equal masses at the vertices of an square, 4 equal masses at the vertices of a second concentric square twisted by an angle of $\pi /4$ and 8 equal masses on a third concentric polygon having the vertices at the bisectors of the angles formed by each pair of position vectors of two consecutive masses of the previous two squares.

From now on, a central configuration is symmetric if the set of positions and the set of masses are invariant under the action of a finite subgroup of O(2). Inspired by the works of Siluszyk, Montaldi and Marchesin, in this work we consider symmetric central configurations consisting of n equal masses at the vertices of a regular n-gon and 2n masses (not all them necessarily equal) at the vertices of a second (not necessarily regular) concentric 2n-gon. We call this kind of configurations symmetric bicircular central configurations of the 3n-body problem. Notice that by Yu and Zhang (2015) if the 2n-gon is regular, then the masses at this 2n-gon cannot be all of them equal. In fact, when the 2n-gon is regular the symmetric bicircular central configurations of the 3n-body problem can be thought as the limit case when $a_2\rightarrow a_3$ of the central configurations of the 3n-body problem consisting of n masses equal to $m_1$ at the vertices of a regular n-gon inscribed in a circle of radius $a_1$, n masses equal to $m_2$ at the vertices of a nested regular n-gon inscribed in a circle of radius $a_2$ and n additional masses equal to $m_3$ (with $m_2\ne m_3$ by Yu and Zhang (2015)), at the vertices of a regular n-gon inscribed in a circle of radius $a_3$ twisted by an angle of $\pi /n$ with respect to the previous two. Thus, when the 2n-gon is regular the masses at the vertices of this 2n-gon alternate the values $m_2$ and $m_3$. Using the nomenclature in Barrabés and Cors (2019), the symmetric bicircular central configurations of the 3n-body problem are a (3, n)-crown.

2 Statement of the Problem and of the Main Results

According to Montaldi (2015), we have two different types of symmetric bicircular central configurations of the 3n-body problem. Indeed, in Montaldi (2015) it is proved that a generic symmetric planar central configuration consists of $k_O$ (with $k_O=0$ or $k_O=1$) masses at the origin, $n\cdot k_N$ masses at the vertices of $k_N$ regular nested n-gons, $n\cdot k_T$ masses at the vertices of $k_T$ regular n-gons twisted by an angle of $\pi /n$, and $2n\cdot k_S$ masses at the vertices of $k_S$ nested semiregular 2n-gons, all centered at the origin. Moreover, the masses on each polygon must be equal but masses on different polygons can be different. A semiregular 2n-gon is a symmetric polygon of 2n vertices which is invariant by reflections with respect to all symmetry axis of the regular n-gon.

The symmetric bicircular central configurations of the 3n-body problem does not contain any mass at the origin, so $k_O=0$. Moreover, since in these configurations we have 3n-bodies, $k_N+k_T+2\,k_S=3$. Thus, we have only three possibilities: either $k_N=1$, $k_T=2$ and $k_S=0$, or $k_N=2$, $k_T=1$ and $k_S=0$, or $k_N=1$, $k_T=0$ and $k_S=1$. Since central configurations are invariant under rotations the case $k_N=2$, $k_T=1$ and $k_S=0$ and the case $k_N=1$, $k_T=2$ and $k_S=0$ provide the same configuration up to a rotation. So we have only two different types of symmetric bicircular central configurations of the 3n-body problem, the configurations with $k_N=1$, $k_T=2$ and $k_S=0$ which are called regular bicircular central configurations of the 3n-body problem and the configurations with $k_N=1$, $k_T=0$ and $k_S=1$ which are called semiregular bicircular central configurations of the 3n-body problem.

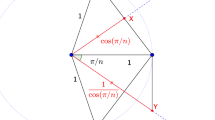
In short, a regular bicircular central configuration of the 3n-body problem consists of n bodies with masses equal to $m_1$ at the vertices of a regular n-gon inscribed in a circle of radius $a_1=r$ and 2n bodies with alternating masses $m_2$ and $m_3$ with $m_2\ne m_3$ at the vertices of a second regular 2n-gon inscribed in a circle of radius $a_2=ar$ with a common center having n vertices aligned with the vertices of the first regular n-gon, see Fig. 1a. Moreover, a semiregular bicircular central configuration of the 3n-body problem consists of n bodies with masses equal to $m_1$ at the vertices of a regular n-gon inscribed in a circle of radius $a_1=r$ and 2n bodies with masses equal to $m_2$ at the vertices of a second concentric semiregular 2n-gon inscribed in a circle of radius $a_2=ar$ having n pair of vertices symmetric by a reflection of angle $\beta$ with respect to the axis of symmetry of the regular n-gon, see Fig. 1b. Without loss of generality, we choose the unit of mass and the unit of length so that $m_1=1$ and $r=1$.

Fig. 1

Examples of bicircular configurations

Notice that the regular bicircular central configurations of the 3n-body problem are the ones studied in Siluszyk (2014) and Siluszyk (2017), but the author in these two works does not analyze whether the expressions of the masses are positive or negative and in case of being positive for which values of the ratios of the radius they are positive and consequently they provide central configurations.

In the first part of the paper (Sect. 3), we go a step further in the study of the regular bicircular central configurations of the 3n-body problem. In particular, we prove analytically for all $n\ge 3$ the existence of central configurations for either sufficiently small values, or sufficiently large values of the ratio of the radius of the circles a and for $n=2$ we prove the existence of central configurations for sufficiently large values of a. Then, using computer-assisted methods we find the complete set of values of a for which there exist regular bicircular central configurations of the 3n-body problem for $n=2,3,4,5$ and we analyze these families of central configurations. Finally, we make a numerical exploration of the families of central configurations of the regular bicircular central configurations of the 3n-body problem for $n=6,...,500$. We remark that the result obtained here for $n=2$ does not coincide with the one given in Siluszyk (2017).

In the second part of the paper (Sect. 4), we study the semiregular bicircular central configurations of the 3n-body problem. In particular, we show that the only values of $\beta$ that can provide central configurations are $\beta \in (\pi /2n,\pi /n)$ and we prove analytically the existence of semiregular bicircular central configurations of the 3n-body problem for all values of $\beta$ in a sufficiently small neighborhood of $\pi /2n$ and of $\pi /n$ for all $n\ge 2$. Then, we make a numerical exploration of the families of semiregular bicircular central configurations of the 3n-body problem for $n=2,\dots ,100$.

Next we give a complete summary of the obtained results.

2.1 Summary of the Results Concerning Regular Bicircular Central Configurations of the 3n-Body Problem

The analytical results that we have obtained for the regular bicircular central configurations of the 3n-body problem are summarized in the following theorem.

Theorem 1

For each $n\geqslant 2$, there exist a nonemptyset D and functions $m_2=m_2(a)$ and $m_3=m_3(a)$ that provide regular bicircular central configurations of the 3n-body problem for all $a\in D$. In particular,

• (a) If $n=2$, then we can assure the existence of central configurations at least for all $a\in (a^*,\infty )$, where $a^*\in (1,\infty )$ is the largest zero of $m_3$. In this configuration, $m_3\rightarrow 0$ and $m_2\rightarrow m_2(a^*)$ when $a\rightarrow {a^*}^+$ and $m_2,m_3\rightarrow \infty$ when $a\rightarrow \infty$.

• (b) If $n\geqslant 3$, then we can assure the existence of central configurations at least for all $a\in (0,a_1^*)\cup (a_2^*,\infty )$, where $a_1^*$ is the minimum between the first positive zero and the pole of $m_2$ and $a_2^*$ is the largest zero of $m_3$. In these configurations, $m_2,m_3\rightarrow 0$ when $a\rightarrow 0^+$, $m_3\rightarrow 0$ and $m_2\rightarrow m_2(a_2^*)$ when $a\rightarrow {a_2^*}^+$, and $m_2,m_3\rightarrow \infty$ when $a\rightarrow \infty$.

The computer-assisted results that we have obtained for the regular bicircular central configurations of the 3n-body problem with $n=2,3,4,5$ are summarized in the following results.

Theorem 2

For $n=2$, we can find functions $m_2=m_2(a)$ and $m_3=m_3(a)$ that provide regular bicircular central configurations of the 6-body problem for all $a\in D=(a_1,a_2)\cup (a^*,\infty )$ where $a_1$ is the pole of $m_2$, $a_2$ is the first zero of $m_2$ and $a^*$ is the largest zero of $m_3$. The approximate values of $a_1$, $a_2$, and $a^*$ are given in Table 1. Moreover, the functions $m_2$ and $m_3$ satisfy the following properties (see Fig. 2):

• (a) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^-$ when $a\rightarrow a_1^+$;

• (b) in the interval $(a_1,a_2)$ the function $m_2$ is decreasing and $m_3$ has a unique critical point at $a=a_0=0.5670013389..$ which is a minimum with $m_3(a_0)=4.7014182338..$. Moreover, $m_2<m_3$;

• (c) $m_2\rightarrow 0$ and $m_3\rightarrow 5.950134407..$ when $a\rightarrow a_2^-$;

• (d) $m_2\rightarrow 1.6921282709..$ and $m_3\rightarrow 0$ when $a\rightarrow {a^*}^+$;

• (e) in the interval $(a^*,\infty )$ both functions $m_2$ and $m_3$ are increasing and $m_2>m_3$;

• (f) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^+$ when $a\rightarrow \infty$.

Table 1 The set D providing regular bicircular central configurations of the 3n–body problem

Theorem 3

For $n=3$, we can find functions $m_2=m_2(a)$ and $m_3=m_3(a)$ that provide regular bicircular central configurations of the 9-body problem for all $a\in D=(0,a_1^*)\cup (a_1,a_2)\cup (a_2^*,\infty )$ where $a_1^*$ is the first zero of $m_2$, $a_1$ is the pole of $m_2$, $a_2$ is the second zero of $m_2(a)$ and $a_2^*$ is the largest zero of $m_3$. The approximate values of $a_1^*$, $a_1$, $a_2$, and $a_2^*$ are given in Table 1. Moreover, the functions $m_2$ and $m_3$ satisfy the following properties (see Fig. 3):

• (a) $m_2,m_3\rightarrow 0$ with $m_2/m_3\rightarrow 1^-$ when $a\rightarrow 0^+$;

• (b) in the interval $(0,a_1^*)$ the function $m_2$ has a unique critical point at $a=a_0=0.1030896914..$ which is a maximum with $m_2(a_0)=0.0003095830..$. Moreover, $m_3$ is increasing and $m_2<m_3$;

• (c) $m_2\rightarrow 0$ and $m_3\rightarrow 0.0060680996..$ when $a\rightarrow {a_1^*}^-$;

• (d) $m_2,m_3\rightarrow \infty$ when $a\rightarrow a_1^+$ with $\lim _{a\rightarrow a_1^+}m_2/m_3=1^-$;

• (e) in the interval $(a_1,a_2)$ the function $m_2$ is decreasing and $m_3$ has a unique critical point at $a=a_3=a_3=0.6096781095..$ which is a minimum with $m_3(a_3)=4.7014182338..$. Moreover, $m_2<m_3$;

• (f) $m_2\rightarrow 0$ and $m_3\rightarrow 4.4805332525..$ when $a\rightarrow a_2^-$;

• (g) $m_2\rightarrow 1.3553872894..$ and $m_3\rightarrow 0$ when $a\rightarrow a_2^*$;

• (h) in the interval $(a_2^*,\infty )$ both functions $m_2$ and $m_3$ are increasing and $m_2>m_3$;

• (i) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^+$ when $a\rightarrow \infty$.

Theorem 4

For $n=4$, we can find functions $m_2=m_2(a)$ and $m_3=m_3(a)$ that provide regular bicircular central configurations of the 12-body problem for all $a\in D= (0,a_1^*)\cup (a_1,a_2)\cup (a_2^*,\infty )$ where $a_1^*$ is the first zero of $m_2$, $a_1$ is the second zero of $m_2$, $a_2$ is the pole of $m_2$ and $a_2^*$ is the largest zero fo $m_3$. The approximate values of $a_1^*$, $a_1$, $a_2$, and $a_2^*$ are given in Table 1. Moreover, the functions $m_2$ and $m_3$ satisfy the following properties (see Fig. 4):

• (a) $m_2,m_3\rightarrow 0$ with $m_2/m_3\rightarrow 1^-$ when $a\rightarrow 0^+$;

• (b) in the interval $(0,a_1^*)$ the function $m_2$ has a unique critical point at $a=a_0=0.2746698699..$ which is a maximum with $m_2(a_0)=0.0085881109..$ and the function $m_3$ is increasing. Moreover, $m_2<m_3$;

• (c) $m_2\rightarrow 0$ and $m_3\rightarrow 0.1238514421..$ when $a\rightarrow {a_1^*}^-$;

• (d) $m_2\rightarrow 0$ and $m_3\rightarrow 2.3831374646..$ when $a\rightarrow a_1^+$;

• (e) in the interval $(a_1,a_2)$ both functions $m_2$ and $m_3$ are increasing and $m_2<m_3$;

• (f) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^-$ when $a\rightarrow a_2^-$;

• (g) $m_2\rightarrow 1.0670996767..$ and $m_3\rightarrow 0$ when $a\rightarrow {a_2^*}^+$;

• (h) in the interval $(a_2^*,\infty )$ both functions $m_2$ and $m_3$ are increasing and $m_2>m_3$;

• (i) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^+$ when $a\rightarrow \infty$.

Theorem 5

For $n=5$, we can find functions $m_2=m_2(a)$ and $m_3=m_3(a)$ that provide regular bicircular central configurations of the 15-body problem for all $a\in D=(0,a_1^*)\cup (a_2^*,\infty )$ where $a_1^*$ is the pole of $m_2$ and $a_2^*$ is the largest zero of $m_3$. The approximate values of $a_1^*$, $a_2$, and $a_2^*$ are given in Table 1. Moreover, the functions $m_2$ and $m_3$ satisfy the following properties (see Fig. 5):

• (a) $m_2,m_3\rightarrow 0$ with $m_2/m_3\rightarrow 1^-$ when $a\rightarrow 0^+$;

• (b) in the interval $(0,a_1^*)$ both functions $m_2$ and $m_3$ are increasing and $m_2<m_3$;

• (c) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^-$ when $a\rightarrow {a_1^*}^-$;

• (d) $m_2\rightarrow 0.8426164718..$ and $m_3\rightarrow 0$ when $a\rightarrow {a_2^*}^+$;

• (e) in the interval $(a_2^*,\infty )$ both functions $m_2$ and $m_3$ are increasing and $m_2>m_3$;

• (f) $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1^+$ when $a\rightarrow \infty$.

The functions $m_2=m_2(a)$ and $m_3=m_3(a)$ in Theorems 1–5 are expressed by the formulas in (4).

After a numerical exploration of the cases $n=6,\dots , 500$, we see that the pattern observed for $n=6,...,500$ is the same as the one observed for $n=5$. So we make the following conjecture.

Conjecture 1

For all $n \ge 5$, there exist functions $m_2=m_2(a)$ and $m_3=m_3(a)$ that provide regular bicircular central configurations of the 3n-body problem for all a in the set $D \subset (0,a_1^*) \cup (a_2^*,\infty )$ where $a_1^*$ is the pole of $m_2$ and $a_2^*$ is the largest zero of $m_3$. Moreover, the functions $m_2$ and $m_3$ are increasing in D, and they satisfy that $m_2<m_3$ when $a \subset (0,a_1^*)$, $m_2>m_3$ when $a \subset (a_2^*,\infty )$, $m_2,m_3\rightarrow 0$ with $m_2/m_3\rightarrow 1$ when $a\rightarrow 0^+$; $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1$ when $a\rightarrow {a_1^*}^-$; $m_3\rightarrow 0$ when $a\rightarrow {a_2^*}^+$; and $m_2,m_3\rightarrow \infty$ with $m_2/m_3\rightarrow 1$ when $a\rightarrow \infty$.

In short, when $n=2$ we have proved analytically (see Theorem 1(a)) the existence of central configurations for all a in the interval $(a^*,\infty )$ where $a^*>1$ is the largest zero of $m_3$. Using computer-assisted results, we prove the existence of an additional interval $(a_1,a_2)$ providing central configurations, where $a_1<1$ is the pole of $m_2$ and $a_2<1$ is a zero of $m_2$ (see Theorem 2).

When $n\ge 3$, we have proved analytically (see Theorem 1(b)) the existence of central configurations for $a\in (0,a_1^*)\cup (a_2^*,\infty )$ where $0<a_1^*<1$ is the minimum between the first positive zero and the pole of $m_2$ and $a_2^*>1$ is the largest zero of $m_3$. For $n=3,4$, using computer-assisted results we have proved the existence of an additional interval $(a_1,a_2)$ with $a_1,a_2<1$ providing central configurations. Moreover, for $n=3$ we have proved that $a_1^*$ is the first positive zero of $m_2$, $a_1$ is the pole of $m_2$ and $a_2$ is the second zero of $m_2$ (see Theorem 3). When $n=4$, we have proved that $a_1^*$ is the first zero of $m_2$, $a_1$ is the second zero of $m_2$ and $a_2$ is the pole of $m_2$ (see Theorem 4). For $n=5$, we have proved using computer-assisted results that the only set of values of a providing central configurations is $(0,a_1^*)\cup (a_2^*,\infty )$; that is, the set given in Theorem 1(b) (see Theorem 5). Finally, after a numerical explorations of the cases $n=6,\dots ,500$ we conclude that for all $n=6,\dots ,500$ that the only set of values of a providing central configurations is the one given in Theorem 1(b).

We must remark that fixed a value of n there are no central configurations of the regular bicircular 3n-body problem with $a\rightarrow 1$. We have observed that as n increases the value $a_1^*$ increases and the value of $a_2^*$ decreases, but we do not know whether $a_1^*\rightarrow {1^-}$ and $a_2^*\rightarrow {1^+}$ when $n\rightarrow \infty$ or on the contrary they could tend to a value different from one. This question remains as an open problem.

On the other hand, as n increases, the values of $m_2$ and $m_3$ are getting closer. We know from results in Yu and Zhang (2015) that $m_2\ne m_3$ because we have two concentric regular n-gons with different number of vertices, but it seems that if $n\rightarrow \infty$ then $m_2\rightarrow m_3$.

2.2 Summary of the Results Concerning Semiregular Bicircular Central Configurations of the 3n-Body Problem

The main analytic results for the semiregular bicircular central configurations of the 3n-body problem are summarized in the following theorem.

Theorem 6

There exist a function $m=m(a,\beta )$ that provides semiregular bicircular central configurations of the 3n-body problem for some values of $a>0$ and $\beta \in (\pi /2n,\pi /n)$. In particular,

• (a) If $n\ge 3$, then for each $\beta$ in a sufficiently small neighborhood of $\pi /n$ there exist at least two central configurations, one with $0<a<1$ and one with $a>1$. Moreover, if $n=2$, then for each $\beta$ in a sufficiently small neighborhood of $\pi /n$ there exists at least one central configuration with $a>1$. The values of m at these central configurations satisfy that $m\rightarrow 0$ as $\beta \rightarrow \pi /n$.

• (b) If $n\ge 3$, then for each $\beta$ in a sufficiently small neighborhood of $\beta =\pi /2n$ there exist at least four central configurations, one with a sufficiently close to the origin, one with $0<a<1$ not necessarily close to the origin, one with a sufficiently large, and one with $a>1$ not necessarily large. Moreover, if $n=2$, then for each $\beta$ in a sufficiently small neighborhood of $\beta =\pi /2n$ there exists at least three central configurations, one with a sufficiently large, one with $a>1$ not necessarily large, and one with $0<a<1$. The values of m at the central configurations with $0<a<1$ not close to the origin and with $a>1$ not being large satisfy that $m\rightarrow \infty$ as $\beta \rightarrow \pi /2n$.

The function $m(a,\beta )$ is given in (16).

After a numerical study of the behavior of the families of semiregular bicircular central configurations of the 3n-body problem for $n=2,3,4$, we get the following numerical results.

Result 1

When $n=2$, we can find continuous functions $\alpha _1(\beta )$ defined for $\beta \in (\pi /4,\pi /2)$, and $\alpha _2(\beta )$ and $\alpha _3(\beta )$ defined for $\beta \in (\pi /4,b^*]$ with $b^*=0.9195936184..$ and a function $m=m(a,\beta )$ such that the following statements hold for the semiregular bicircular central configurations of the 6-body problem (see Fig. 9a).

• (a) If $\beta \in (\pi /4,b^*)$, then $m=m(\beta ,a)$ provides three families of central configurations, one with $a=\alpha _1(\beta )$, one with $a=\alpha _2(\beta )$, and one with $a=\alpha _3(\beta )$.

• (b) If $\beta =b^*$, then $m=m(\beta ,a)$ provides two central configurations, one with $a=\alpha _1(b^*)$ and one with $a=\alpha _2(b^*)=\alpha _3(b^*)$ (the families with $a=\alpha _2(\beta )$ and $a=\alpha _3(\beta )$ coincide at this point).

• (c) If $\beta \in (b^*,\pi /2)$, then $m=m(\beta ,a)$ provides a unique family of central configurations with $a=\alpha _1(\beta )$.

Moreover, $m(\alpha _1(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /2^-$; and $m(\alpha _1(\beta ),\beta ),m(\alpha _2(\beta ),\beta ), m(\alpha _3(\beta ),\beta )\rightarrow \infty$ when $\beta \rightarrow \pi /4^+$.

Result 2

When $n=3$, we can find continuous functions $\alpha _1(\beta )$ and $\alpha _2(\beta )$ defined for $\beta \in (\pi /6,b^*]$, functions $\alpha _1(\beta )$ and $\alpha _2(\beta )$ defined for $\beta \in (\pi /6,\pi /3)$, with $b^*=0.7119233940$.. and a function $m=m(a,\beta )$ such that the following statements hold for the semiregular bicircular central configurations of the 9-body problem (see Fig. 9b).

• (a) If $\beta \in (\pi /6,b^*)$, then $m=m(\beta ,a)$ provides four families of central configurations, one with $a=\alpha _1(\beta )$, one with $a=\alpha _2(\beta )$, one with $a=\alpha _3(\beta )$, and one with $a=\alpha _4(\beta )$.

• (b) If $\beta =b^*$, then $m=m(\beta ,a)$ provides three central configurations, one with $a=\alpha _1(b^*) =\alpha _2(b^*)$ (the families with $a=\alpha _1(\beta )$ and $a=\alpha _2(\beta )$ coincide at this point), one with $\alpha _3(b^*)$, and one with $a=\alpha _4(b^*)$.

• (c) If $\beta \in (b^*,\pi /3)$, then $m=m(\beta ,a)$ provides two family of central configurations, one with $a=\alpha _3(\beta )$ and one with $a=\alpha _4(\beta )$.

Moreover, $m(\alpha _3(\beta ), \beta ),m(\alpha _4(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /3^-$; $m(\alpha _1(\beta ),\beta ),m(\alpha _2(\beta ),\beta ), m(\alpha _3(\beta ),\beta )\rightarrow \infty$ and $m(\alpha _4(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /6^+$.

Result 3

When $n=4$, we can find continuous functions $\alpha _1(\beta )$ and $\alpha _4(\beta )$ defined for $\beta \in (\pi /8,b^*]$, functions $\alpha _2(\beta )$ and $\alpha _3(\beta )$ defined for $\beta \in (\pi /8,\pi /4)$, with $b^*=0.4665964724$.. and a function $m=m(a,\beta )$ such that the following statements hold for the semiregular bicircular central configurations of the 12-body problem (see Fig. 9c).

• (a) If $\beta \in (\pi /8,b^*)$, then $m=m(\beta ,a)$ provides four families of central configurations, one with $a=\alpha _1(\beta )$, one with $a=\alpha _2(\beta )$, one with $a=\alpha _3(\beta )$, and one with $a=\alpha _4(\beta )$.

• (b) If $\beta =b^*$, then $m=m(\beta ,a)$ provides three central configurations, one with $a=\alpha _1(b^*) =\alpha _4(b^*)$ (the families with $a=\alpha _1(\beta )$ and $a=\alpha _4(\beta )$ coincide at this point), one with $\alpha _2(b^*)$ and one with $a=\alpha _3(b^*)$.

• (c) If $\beta \in (b^*,\pi /4)$, then $m=m(\beta ,a)$ provides two family of central configurations, one with $a=\alpha _2(\beta )$ and one with $a=\alpha _3(\beta )$.

Moreover, $m(\alpha _3(\beta ), \beta ),m(\alpha _2(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /4^-$; and $m(\alpha _1(\beta ),\beta ), m(\alpha _2(\beta ), \beta ),m(\alpha _3(\beta ),\beta )\rightarrow \infty$ and $m(\alpha _4(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /8^+$.

We have also studied numerically the families of semiregular bicircular central configurations of the 3n-body for $n=5,\dots ,100$, and we have observed that the behavior is the same as the one for $n=4$. So we state the following conjecture.

Conjecture 2

For all $n \ge 4$, we can find a value $\beta =b^*$ and functions $\alpha _i(\beta )$ for $i=1,2,3,4$ such that

• (a) for $\beta \in (\pi /2n,b^*)$ there exist exactly four different families of semiregular bicircular central configurations, one emanating from a point $(a_1,\pi /2n)$ with $0<a_1<1$ (the family with $a=\alpha _1(\beta )$), one emanating from a point $(a_2,\pi /2n)$ with $a_2>1$ (the family with $a=\alpha _1(\beta )$), one emanating from the point $(\infty ,0)$ (the family with $a=\alpha _3(\beta )$), and one emanating from the point $(0,\pi /2n)$ ($\alpha _4(\beta )$);

• (b) for $\beta =b^*$ there exist exactly three different central configurations, the one with $a=a_1(b^*)=a_4(b^*)$, the one with $a=a_2(b^*)$ and the one with $a=a_3(b^*)$;

• (c) for $\beta \in (b^*,\pi /n)$ there exist exactly two different central configurations, one tending to a point $(a_1^*,\pi /n)$ with $0<a_1^*<1$ when $\beta \rightarrow \pi /n^-$ (the family with $a=a_2(\beta )$) and one tending to a point $(a_2^*,\pi /n)$ with $a_2^*>1$ when $\beta \rightarrow \pi /n^-$ (the family with $a=a_3(\beta )$).

Moreover, the masses associated with these families of central configurations $m=m(a,\beta )$ satisfy that $m(\alpha _3(\beta ),\beta ),m(\alpha _2(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /2n^-$; and $m(\alpha _1(\beta ),\beta ),m(\alpha _2(\beta ),\beta ),m(\alpha _3(\beta ),\beta )\rightarrow \infty$ and $m(\alpha _3(\beta ),\beta )\rightarrow 0$ when $\beta \rightarrow \pi /n^+$.

We note that the relative length of the interval of values of the parameter $\beta$ providing four different families of central configurations gets smaller as n increases. With relative length, we mean relative length with respect to the total length of the interval $(\pi /2n,\pi /n)$.

3 Regular Bicircular Central Configurations of the 3n-Body Problem

3.1 The Equations

As we have seen in the introduction, the regular bicircular central configurations of the 3n-body problem can be thought as the limit case of a (3, n)-crown (see Barrabés and Cors 2019) where two twisted n-gons are inscribed in a circle of the same radius.

From equation (9) in Barrabés and Cors (2019), the equations for central configurations of any (3, n)-crown with n masses equal to $m_1=1$ at the vertices of a first n-gon inscribed in a circle of radius $a_1=1$ and twisted an angle $\varpi _1=0$, n masses equal to $m_2$ at the vertices of a second n-gon inscribed in a circle of radius $a_2$ and twisted an angle $\varpi _2=0$, and n masses equal to $m_3$ at the vertices of a third n-gon inscribed in a circle of radius $a_3$ and twisted an angle $\varpi _3=\pi /n$ are

$$\begin{aligned} \begin{aligned} C_{21}-S_n\,a_2+\left( \frac{S_n}{a_2^2}-a_2\,C_{12}\right) m_2+\Big (C_{23}-a_2\,C_{13}\Big )m_3&=0,\\ C_{31}-S_n\,a_3+\Big (C_{32}-a_3\,C_{12}\Big )m_2+\left( \frac{S_n}{a_3^2}-a_3\,C_{13}\right) m_3&=0, \end{aligned} \end{aligned}$$

(2)

where

$$\begin{aligned} S_n= & {} \sum _{j=1}^{n-1}\frac{1}{4\sin \big (\frac{\pi \,\!j}{n}\big )}= \sum _{j=1}^{n-1}\frac{1-\cos \big (\frac{2\pi \,\!j}{n}\big )}{\Big (2-2\cos \big (\frac{2\pi \,\!j}{n}\big )\Big )^{3/2}}= \sum _{j=1}^{n-1}\frac{1}{2\Big (2-2\cos \big (\frac{2\pi \,\!j}{n}\big )\Big )^{1/2}},\\ C_{k\ell }= & {} C_{k\ell }(a_k,a_\ell )=\sum _{j=1}^n \frac{a_k-a_\ell \cos \big (\varpi _k-\varpi _\ell +\frac{2\pi \,\!j}{n}\big )}{\Big (a_k^2+a_\ell ^2-2a_ka_\ell \cos \big (\varpi _k-\varpi _\ell +\frac{2\pi \,\!j}{n}\big )\Big )^{3/2}}, \end{aligned}$$

for $k\ne \ell$.

Thus, the equations of the regular bicircular central configurations of the 3n-body problem are given by (2) with $a_2=a_3=a$, and they can be written as the linear system in the variables $m_2$, $m_3$

$$\begin{aligned} \left( \begin{array}{cc} a_{11} &{}a_{12}\\ a_{21} &{} a_{22}\end{array}\right) \left( \begin{array}{c} m_2\\ m_3\end{array}\right) =\left( \begin{array}{c} b_1\\ b_2\end{array}\right) , \end{aligned}$$

(3)

where

$$\begin{aligned} \begin{aligned} a_{11}&=\frac{K_1}{a^3}-K_2,\qquad a_{12}=\frac{K_5}{a^3}-K_3,\qquad b_1=K_1-K_4,\\ a_{21}&=\frac{K_5}{a^3}-K_2,\qquad a_{22}=\frac{K_1}{a^3}-K_3,\qquad b_2=K_1-K_6, \end{aligned} \end{aligned}$$

and

$$\begin{aligned} K_1= & {} S_n,\\ K_2= & {} K_2(a)=C_{12}(1,a)=\sum _{j=1}^n\frac{1-a\cos \big (\frac{2\pi \,j}{n}\big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}\big )\Big )^{3/2}},\\ K_3= & {} K_3(a)=C_{13}(1,a)=\sum _{j=1}^n\frac{1-a\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )\Big )^{3/2}},\\ K_4= & {} K_4(a)=\frac{C_{21}(a,1)}{a}=\sum _{j=1}^n\frac{1-1/a\cos \big (\frac{2\pi \,j}{n}\big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}\big )\Big )^{3/2}},\\ K_5= & {} a^2C_{23}(a,a)=a^2C_{32}(a,a)\\= & {} \sum _{j=1}^{n}\frac{1-\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )}{\Big (2-2\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )\Big )^{3/2}}=\sum _{j=1}^{n}\frac{1}{2\Big (2-2\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )\Big )^{1/2}} ,\\ K_6= & {} K_6(a)=\frac{C_{31}(a,1)}{a}=\sum _{j=1}^n\frac{1-1/a\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )\Big )^{3/2}}. \end{aligned}$$

Solving system (3), we get the solution

$$\begin{aligned} \begin{aligned} m_2=m_2(a)&= \frac{a_{22}b_1-a_{12}b_2}{a_{11}a_{22}-a_{12}a_{21}}=\dfrac{a^3\,m_{N,2}}{m_D},\\ m_3=m_3(a)&= \frac{a_{11}b_2-a_{21}b_1}{a_{11}a_{22}-a_{12}a_{21}}=\dfrac{a^3\,m_{N,3}}{m_D} \end{aligned} \end{aligned}$$

(4)

where

$$\begin{aligned} \begin{aligned} m_{N,2}&=K_1^2-K_1K_4(a)-K_1K_5+K_5K_6(a)-a^3K_3(a)(K_6(a)-K_4(a)),\\ m_{N,3}&=K_1^2-K_1K_5+K_4(a)K_5-K_1K_6(a)+a^3K_2(a)(K_6(a)-K_4(a)),\\ m_D&=(K_5-K_1) \varDelta (a), \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \varDelta (a)=-K_1-K_5+a^3(K_2(a)+K_3(a)). \end{aligned}$$

Solution (4) is defined when the denominator $m_D$ is different from zero. By Lemma 3(c) in Appendix 1 $K_5-K_1\ne 0$ and by Lemma 4 in Appendix 1 the function $\varDelta (a)$ has a unique zero and it belongs to the interval (0, 1). So the set where $m_D$ is different from zero is not empty. So in what follows we will assume that $m_D\ne 0$. In fact, this does not seem to be restrictive because, as we will see in Sect. 3.3, we have numerical evidences that there are no solutions of (3) with $m_D=0$ ($m_D$, $m_{N,2}$, and $m_{N,3}$ are not simultaneously 0).

These expressions have been obtained in Siluszyk (2014) by using a different approach.

Note that

$$\begin{aligned} m_3=m_2+\frac{a^3(K_6(a)-K_4(a))}{K_5-K_1}. \end{aligned}$$

(5)

In short, a configuration of the regular bicircular 3n-body problem is central if $m_2$ and $m_3$ are given by (4) and a is so that $m_2,m_3>0$.

3.2 Proof of Theorem 1

The central configurations of the regular bicircular 3n-body problem are given by the solutions of (3) with $m_2,m_3>0$. Let $m_2=m_2(a)$ and $m_3=m_3(a)$ be the solutions of (3) given in (4). We need the following auxiliary result that give some properties of $m_2$ and $m_3$.

Proposition 1

Let $m_2=m_2(a)$ and $m_3=m_3(a)$ be the functions defined in (4). Then, the following statements hold for $n \ge 2$.

• (a) $m_2\rightarrow 0^+$ when $a \rightarrow 0^+$ for $n \ge 3$ and $m_2\rightarrow 0^-$ when $a\rightarrow 0^+$ for $n=2$;

• (b) $m_3\rightarrow 0^+$ when $a\rightarrow 0^+$;

• (c) $m_3\rightarrow -\infty$ when $a\rightarrow 1^+$;

• (d) $m_2\rightarrow \infty$ and $m_3\rightarrow \infty$ when $a\rightarrow \infty$;

• (e) $m_2>m_3$ when $a>1$ and $m_2<m_3$ when $a\in (0,1)$.

The proof of Proposition 1 is given in Appendix 1.

From Proposition 1(a) and (b), for each $n\ge 3$ there exist a sufficiently small interval $I_1=(0,a_1^*)$ such that $m_2,m_3>0$. Since $m_2<m_3$ when $0<a<1$ (see Proposition 1(e)) this value $a_1^*$ corresponds either to a zero of $m_2$ or to a point where the denominator $m_D$ vanishes. In Sect. 3.3 we prove, by using a computer-assisted proof, that $a_1^*$ is a zero of $m_2$ when $n=3,4$ and it is the zero of $m_D$ when $n=5$. Moreover, we give strong numerical evidences that $a_1^*$ is the zero of $m_D$ for $n>5$.

We continue with the proof of Theorem 1. From Proposition 1(d) for each $n\ge 2$, there exists a sufficiently large value $a_2^*>1$ such that $m_2,m_3>0$ for all $a\in I_2=(a_2^*,\infty )$. Using Proposition 1(c) and (d), we get that $m_3$ has at least a zero for $a>1$ and from Lemma 4 in Appendix 1 we obtain that $m_D$ has no zeros for $a>1$. Since $m_2>m_3$ when $a>1$ (see again Proposition 1), and connecting all above comments together we can assure that $a_2^*$ is the largest zero of $m_3$. In Sect. 3.3 using a computer-assisted proof we prove, for $n=2,3,4,5$, that $m_3$ has a unique zero, $a_2^*$, for $a>1$ and we give strong numerical evidences that this also happens for $n>5$.

These arguments, together with Proposition 1, complete the proof of Theorem 1.

3.3 Particular Cases of Regular Bicircular Central Configurations of the 3n-Body Problem

3.3.1 Case $n=2$

Computing the values of $K_i$ with $i=1,\dots ,6$ for $n=2$, we get

$$\begin{aligned} \begin{array}{l} K_1=\dfrac{1}{4}, \qquad K_3(a)=K_6(a)=\dfrac{2}{h_{22}}, \qquad K_5=\dfrac{1}{\sqrt{2}},\\ K_2(a)=\dfrac{1-a}{h_{12}}+\dfrac{1}{(a+1)^2}, \qquad K_4(a)=-\dfrac{1-a}{a\,h_{12}}+\dfrac{1}{a(a+1)^2}, \end{array} \end{aligned}$$

(6)

where $h_{12}=((1-a)^2)^{3/2}$ and $h_{22}=(1+a^2)^{3/2}$. Thus, when $n=2$, the solutions $m_2=m_2(a)$ and $m_3=m_3(a)$ of system (3) with $m_D\ne 0$ are given by (4) with $K_i$ given by (6). Note that in order that these solutions provide central configurations both $m_2$ and $m_3$ have to be positive. Next, we find the set of values of a satisfying these conditions.

Since $a>0$, the possible changes of sign of $m_2$ and $m_3$ are given by the zeroes of $m_{N,2}$ and $m_D$, and $m_{N,3}$ and $m_D$, respectively, see (4). We start computing the zeroes of $m_D$.

First we transform equation $m_D=0$ into a polynomial equation having all the solutions of equation $m_D=0$ and probably new ones in the following way. Dropping the denominators we get the equation

$$\begin{aligned} \begin{aligned} g&=(a+1)^2 \left( 4 a^3 ((a-1)h_{22}-2 h_{12})+\left( 1+2\, \sqrt{2}\right) h_{12} h_{22}\right) \\&\quad -4 a^3 h_{12} h_{22}=0. \end{aligned} \end{aligned}$$

In order to drop the square roots, we consider equation $g=0$ as a polynomial equation in the variables a, $h_{12}$ and $h_{22}$. Then, the zeroes of $m_D$ can be thought as solutions of the polynomial system $g=0$, $e_1=0$ and $e_2=0$ with

$$\begin{aligned} e_1=h_{12}^2-((1-a)^2)^3, \qquad e_2=h_{22}^2-(1+a^2)^3. \end{aligned}$$

We eliminate the variable $h_{12}$ by means of the resultant $R_1=\text{ Res }[g,e_1,h_{12}]$ and the variable $h_{22}$ by means of the resultant $R_2=\text{ Res }[R_1,e_2,h_{22}]$. The resulting polynomial is a polynomial in the variable a with irrational coefficients (the coefficients depend on $\sqrt{2}$) that has all the zeroes of $m_D$ and probably new ones. To avoid irrational coefficients, we introduce a new variable $h_{32}=\sqrt{2}$ and a new equation $e_3=h_{32}^2-2$. We eliminate the variable $h_{32}$ by means of the resultant $R_3=\text{ Res }[R_2,e_3,h_{32}]$ obtaining in this way a polynomial with integer coefficients having all the zeroes of $m_D$ and probably new ones. The obtained polynomial is $(a-1)^8P_{60}(a)$ where $P_{60}(a)$ is given by

$$\begin{aligned}&(45041 a^{28}+471582 a^{26}+1263627 a^{24}+900044 a^{22}+1046137 a^{20}\\&\quad +172866 a^{18}+233227 a^{16}+47784 a^{14}+27723 a^{12}-18750 a^{10}\\&\quad +6329 a^8-564 a^6-693 a^4-98 a^2+49) (4096 a^{32}-2048 a^{31}\\&\quad +32128 a^{30}-12064 a^{29}+176561 a^{28}-42784 a^{27}+495902 a^{26}\\&\quad -50496 a^{25}+1006987 a^{24}+27328 a^{23}+759372 a^{22}+93472 a^{21}\\&\quad +1118393 a^{20}+64800 a^{19}+259394 a^{18}+11904 a^{17}+310283 a^{16}\\&\quad -31104 a^{15}+38184 a^{14}-41696 a^{13}+1675 a^{12}-15072 a^{11}\\&\quad -10814 a^{10}-1344 a^9+3641 a^8-1344 a^7-1204 a^6 \\&\quad +224 a^5-245 a^4+224 a^3-98 a^2+49) \end{aligned}$$

From now on, we will denote by $P_n(a)$ a polynomial of degree n in the variable a. Applying Sturm’s theorem, we get that the polynomial equation $P_{60}(a)=0$ has exactly four real solutions with $a>0$. We solve numerically the equation $P_{60}(a)=0$, and we found the solutions $a=a_1=0.4656636054..$, $a=a_2=d_2=0.5317860740..$, $a=a_3= 0.5390030006..$, $a=a_4=0.5824356327..$. By substituting these solutions into the initial equation $m_D=0$, we see that only the solution $a=d_2$ can be a solution of the initial equation. This can be proved in a more rigorous way by using interval arithmetic (see Tucker 2011). We have used Mathematica’s capability of operating on interval objects to get an interval enclosure of the function $m_D$ in a sufficiently small interval containing the possible solutions of the equation $m_D=0$. We start proving that $a_1\in \mathbf {a_1}=[4656636054/10^{10},4656636055/10^{10}]$ cannot be a solution of the initial equation. Notice that since $a_1<1$ we have that $((1-a_1)^2)^{3/2}=(1-a_1)^3$. Using interval arithmetic, we get

$$\begin{aligned}&1+a_1^2\in [h_{21},h_{22}]=\left[ \tfrac{30421064834853172729}{25\cdot 10^{18}},\tfrac{4867370373949038521}{4\cdot 10^{18}}\right] ,\\&(1-a_1)^3\in \mathbf {h_1}\\&\qquad =\left[ \tfrac{1220490080420246024105505069}{8\cdot 10^{27}},\tfrac{190701575172731 70971924514317}{125\cdot {10^{27}}}\right] . \end{aligned}$$

Moreover, we can see that

$$\begin{aligned} h_{21}^{3/2}\in \left[ \tfrac{13423062258}{10^{10}},\tfrac{13423062259}{10^{10}}\right] , \quad h_{22}^{3/2}\in \left[ \tfrac{13423062259}{10^{10}},\tfrac{13423062260}{10^{10}}\right] , \end{aligned}$$

so $(1+a_1^2)^{3/2}\in \mathbf {h_2}= [13423062258/10^{10},13423062260/10^{10}]$, moreover $\sqrt{2}\in \mathbf {h_3}=[14142135623/10^{10},14142135624/10^{10}]$. By substituting into the expression of $m_D$ the values of a, $((1-a)^2)^{3/2}$, $(1+a^2)^{3/2}$ and $\sqrt{2}$ by $\mathbf {a_1}$, $\mathbf {h_1}$, $\mathbf {h_2}$ and $\mathbf {h_3}$, respectively, and doing interval arithmetic again we get that $m_D\in [-0.1855803641, -0.1855803639]$, so $a_1$ does not satisfy equation $m_D=0$. Repeating this procedure for the remaining solutions, we get that $m_D\in [-0.0000000001,0.0000000004]$ for $a_2\in [5317860740/10^{10},5317860741/10^{10}]$, $m_D\in [0.0271889606,0.0271889613]$ for $a_3\in [5390030006/10^{10},5390030007/10^{10}]$ and $m_D\in [0.2330977572,0.2330977582]$ for $a_4\in [5824356327/10^{10},5824356328/10^{10}]$. This proves that the unique solution of $P_{60}(a)=0$ providing a solution of the initial equations is the solution $a=d_2$.

Using the same procedure, the equation $m_{N,2}=0$ can be transformed into a polynomial equation of the form $(a-1)^8 a^4\,P_{84}(a)=0$ where the polynomial $P_{84}(a)$ has exactly 8 real roots with $a>0$. Among these solutions, only $a=a_{12}=0.6161447847..$ and $a=a_{22}=2.8235222602..$ are solutions of the initial equation $m_{N,2}=0$. Doing it again, we get that $m_{N,3}=0$ can be transformed into a polynomial equation of the form $(a-1)^{16} a^4 \,P_{92}(a)=0$ where the polynomial $P_{92}(a)$ has exactly 8 real roots with $a>0$. Among these solutions only $a=b_{12}=0.5161941182..$ and $a=b_{22}=3.5282322274..$ are solutions of the initial equation $m_{N,3}=0$.

Note that $m_{N,2}$, $m_{N,3}$ and $m_D$ are not simultaneously zero. This means that when $n=2$ there could not be solutions of system (3) with $m_D= 0$.

Finally, we analyze the signs of $m_2$ and $m_3$. By substituting $a=4/10$, $(1-(6/10)^2)^{3/2}\in [12493582352/10^{10}, 12493582353/10^{10}]$ and $\sqrt{2}\in [14142135623/10^{10}, 14142135624/10^{10}]$ into $m_2$ and $m_3$ and doing interval arithmetic again, we get that $m_2\in [-0.3673154049, -0.3673154050]$ and $m_3\in [0.6505307765, 0.6505307766]$, so $m_2$ is negative and $m_3$ is positive in $(0,b_{12})$. Doing the same for $a=6/10$, $a=2$, $a=3$ and $a=4$, we conclude that $m_2>0$ for $a \in (d_2,a_{12})\cup (a_{22},\infty )$ and $m_3>0$ for $a \in (0,b_{12})\cup (d_2,1)\cup (b_{22},\infty )$. So the region where the masses $m_2$ and $m_3$ given in (4) provide central configurations is $a\in (d_2,a_{12})\cup (b_{22},\infty )$, see Fig. 2 for the plots of $m_2$ and $m_3$.

Fig. 2

Plot of the masses $m_2$ (continuous line) and $m_3$ (dashed line) for $n=2$

Examining the properties of the functions $m_2$ and $m_3$, we get that in the interval $(d_2,a_{12})$ the functions $m_2,m_3\rightarrow \infty$ when $a\rightarrow d_2^+$. Since $m_2$ and $m_3$ satisfy the relation (5) and $(K_6(a)-K_4(a))/(K_5-K_1)$ is a finite number for all $a\ne 1$, we can easily see that $\lim _{a\rightarrow d_2^+}m_2/m_3=1$. By computing the derivatives of the functions $m_2$ and $m_3$ and analyzing its zeros as we have done with the zeroes of $m_2$ and $m_3$, we see that the function $m_2$ is decreasing for $a\in (0,d_2)\cup (d_2,1)\cup (1,1.5015204804..)$ and increasing for $a\in (1.5015204804..,\infty )$; and the function $m_3$ is increasing for $a\in (0,0.4812067311..)\cup (a_{c2},1)\cup (1,+\infty )$ with $a_{c2}=0.5670013389..$ and decreasing for $a\in (0.4812067311..,d_2)\cup (d_2,a_{c2})$ (see Fig. 2). Moreover, $m_2\rightarrow 0$ when $a\rightarrow a_{12}^-$, $m_3(a_{c2})=4.7014182338..$, $m_3\rightarrow 5.950134407..$ when $a\rightarrow a_{12}^-$, $m_2\rightarrow 1.6921282709..$ and $m_3\rightarrow 0$ when $a\rightarrow b_{22}^+$. Finally, we get that $m_2,m_3\rightarrow \infty$ when $a\rightarrow \infty$ with $\lim _{a\rightarrow \infty }m_2/m_3=1$ (see Fig. 2 again).

3.3.2 Case $n=3$

When $n=3$, the values of $K_i$ for $i=1,\dots , 6$ are

$$\begin{aligned} \begin{array}{ll} K_1=\dfrac{1}{\sqrt{3}}, \qquad &{} K_5=\dfrac{5}{4},\\ K_2(a)=\dfrac{1-a}{h_{13}}+\dfrac{2+a}{h_{33}}, \qquad &{} K_3(a)=\dfrac{1}{(1+a)^2}+\dfrac{2-a}{h_{23}}, \\ K_4(a)=-\dfrac{1-a}{a\, h_{13}}+\dfrac{1+2a}{a\, h_{33}}, \qquad &{} K_6(a)=\dfrac{1}{a\, (1+a)^2}-\dfrac{1-2a}{a\, h_{23}}, \end{array} \end{aligned}$$

(7)

where $h_{13}=((1-a)^2)^{3/2}$, $h_{23}=(1 - a + a^2)^{3/2}$, and $h_{33}=(1 + a + a^2)^{3/2}$. Thus, when $n=3$, the solutions $m_2=m_2(a)$ and $m_3=m_3(a)$ of system (3) with $m_D\ne 0$ are given by (4) with $K_i$ given by (7). Next we find the values of a for which $m_2=m_2(a)>0$ and $m_3=m_3(a)>0$.

First, we find all the real solutions of equations $m_D=0$, $m_{N,2}=0$ and $m_{N,3}=0$ with $a>0$. Following step by step, the procedure explained in Sect. 3.3.1 by introducing the new variable $h_{43}=\sqrt{3}$ we transform equations $m_D=0$, $m_{N,2}=0$ and $m_{N,3}=0$ into polynomial equations with integer coefficients having the same solutions as the initial equations and probably new ones. Then, we solve numerically the obtained polynomial equations and we check which of their solutions with $a>0$ provide solutions of the corresponding initial equations. The results that we have obtained are summarized in Table 2. Note that $m_{N,2}$, $m_{N,3}$ and $m_D$ are not simultaneously zero. This means that when $n=3$ there could not be solutions of system (3) with $m_D= 0$.

Table 2 Real positive solutions of $m_D=0$, $m_{N,2}=0$, and $m_{N,3}=0$ for $n=3$

Proceeding again as in Sect. 3.3.1, we analyze the signs of $m_2$ and $m_3$ and we get that $m_2>0$ for $a\in (0,a_{13})\cup (d_3,a_{23})\cup (a_{33},\infty )$ and $m_3>0$ for $a\in (0,b_{13})\cup (d_3,1)\cup (b_{23},\infty )$. In short, the masses $m_2$ and $m_3$ given in (4) can provide central configurations when $a\in (0,a_{13})\cup (d_3,a_{23})\cup (b_{23},\infty )$, see Fig. 3 for the plots of $m_2$ and $m_3$.

Fig. 3

Plot of the masses $m_2$ (continuous line) and $m_3$ (dashed line) for $n=3$

Examining the properties of the functions $m_2$ and $m_3$, we get that in the interval $(0,a_{13})$ the functions $m_2,m_3\rightarrow 0$ when $a\rightarrow 0^+$, moreover $\lim _{a\rightarrow 0^+}m_2/m_3=1$; the function $m_2$ is increasing for $a\in (0, {a_{c_1}}_3)$, decreasing for $a\in ({a_{c_1}}_3,a_{13})$, it has a maximum at $a={a_{c_1}}_3=0.1030896914..$ with $m_2({a_{c_1}}_3)=0.0003095830..$, and $m_2\rightarrow 0$ when $a\rightarrow a_{13}^-$; and the function $m_3$ is increasing in $a\in (0,a_{13})$ and $m_3\rightarrow 0.0060680996..$ when $a\rightarrow a_{13}^-$ (see Fig. 3a). In the interval $(d_3,a_{23})$, the functions $m_2,m_3\rightarrow \infty$ when $a\rightarrow d_3^+$ with $\lim _{a\rightarrow d_3^+}m_2/m_3=1$; the function $m_2$ is decreasing and $m_2\rightarrow 0$ when $a\rightarrow a_{23}^-$; the function $m_3$ is decreasing for $a\in (d_3,{a_{c_2}}_3)$, is increasing for $a\in ({a_{c_2}}_3,a_{23})$, it has a minimum at $a={a_{c_2}}_3=0.6096781095..$ with $m_3({a_{c_2}}_3)=4.7014182338..$, and $m_3\rightarrow 4.4805332525..$ when $a\rightarrow a_{23}^-$ (see Fig. 3b). In the interval $(b_{23},\infty )$, both functions $m_2$ and $m_3$ are increasing, $m_2\rightarrow 1.3553872894..$ and $m_3\rightarrow 0$ when $a\rightarrow b_{23}^+$ and $m_2,m_3\rightarrow \infty$ when $a\rightarrow \infty$ with $\lim _{a\rightarrow \infty }m_2/m_3=1$ (see Fig. 3c).

3.3.3 Case $n=4$

When $n=4$, the values of $K_i$ for $i=1,\dots , 6$ are

$$\begin{aligned} \begin{array}{ll} K_1=\dfrac{1}{4}+\dfrac{1}{\sqrt{2}}, \quad &{} K_5=\sqrt{2+\sqrt{2}},\\ K_2(a)=\dfrac{1-a}{h_{14}}+\dfrac{1}{(1+a)^2}+\dfrac{2}{h_{24}}, \quad &{} K_3(a)=\dfrac{2-\sqrt{2} a}{h_{34}}+\dfrac{2+\sqrt{2} a}{h_{44}}, \\ K_4(a)=-\dfrac{1-a}{a\, h_{14}}+\dfrac{1}{a(1+a)^2}+\dfrac{2}{h_{24}}, \quad &{} K_6(a)=\dfrac{-\sqrt{2} +2a}{a h_{34}}+\dfrac{\sqrt{2}+2 a}{a h_{44}}, \end{array} \end{aligned}$$

(8)

where $h_{14}=((1-a)^2)^{3/2}$, $h_{24}= \left( a^2+1\right) ^{3/2}$, $h_{34}=\left( a^2-\sqrt{2} a+1\right) ^{3/2}$ and $h_{44}=\left( a^2+\sqrt{2} a+1\right) ^{3/2}$. Thus, when $n=4$, the solutions $m_2=m_2(a)$ and $m_3=m_3(a)$ of system (3) with $m_D\ne 0$ are given by (4) with $K_i$ given by (8). Next we find the values of a for which $m_2=m_2(a)>0$ and $m_3=m_3(a)>0$.

First, proceeding as in Sects. 3.3.1 and 3.3.2 by introducing the new variables $h_{54}=\sqrt{2}$ and $h_{64}=\sqrt{2+\sqrt{2}}$, we transform equations $m_D=0$, $m_{N,2}=0$ and $m_{N,3}=0$ into polynomial equations with integer coefficients. We find all the real solutions with $a>0$ of these equations, and the results that we have obtained are summarized in Table 3. Note that $m_{N,2}$, $m_{N,3}$ and $m_D$ are not simultaneously zero. This means that when $n=4$ there could not be solutions of system (3) with $m_D= 0$.

Table 3 Real positive solutions of $m_D=0$, $m_{N,2}=0$, and $m_{N,3}=0$ for $n=4$

Next we analyze the signs of $m_2$ and $m_3$ and we see the region where the masses $m_2$ and $m_3$ given in (4) can provide central configurations is $a\in (0,a_{14})\cup (a_{24},d_4)\cup (b_{24},\infty )$. See Fig. 4 for the plots of $m_2$ and $m_3$.

Fig. 4

Plot of the masses $m_2$ (continuous line) and $m_3$ (dashed line) for $n=4$

Finally, we examine the properties of the functions $m_2$ and $m_3$ we get that in the interval $(0,a_{14})$ both functions $m_2,m_3\rightarrow 0$ when $a\rightarrow 0^+$ and moreover $\lim _{a\rightarrow 0^+}m_2/m_3=1$; the function $m_2$ is increasing for $a\in (0, a_{c4})$ and decreasing for $a\in (a_{c4},a_{14})$, it has a maximum at $a=a_{c4}=0.2746698699..$ with $m_2(a_{c4})=0.0085881109..$, and $m_2\rightarrow 0$ when $a\rightarrow a_{14}^-$; and the function $m_3$ is increasing in $a\in (0,a_{14})$ and $m_3\rightarrow 0.1238514421..$ when $a\rightarrow a_{14}^-$ (see Fig. 4a). In the interval $(a_{24},d_4)$ (see Fig. 4b), both functions $m_2$ and $m_3$ are increasing, $m_2,m_3\rightarrow \infty$ when $a\rightarrow d_4^-$ with $\lim _{a\rightarrow d_4^-}m_2/m_3=1$, and $m_2\rightarrow 0$ and $m_3\rightarrow 2.3831374646..$ when $a\rightarrow a_{24}^+$. Finally, in the interval $(b_{24},\infty )$ both functions $m_2$ and $m_3$ are increasing (see Fig. 4d), $m_2\rightarrow 1.0670996767..$ and $m_3\rightarrow 0$ when $a\rightarrow b_{24}^+$ and $m_2,m_3\rightarrow \infty$ when $a\rightarrow \infty$ with $\lim _{a\rightarrow \infty }m_2/m_3=1$.

3.3.4 Case $n=5$

When $n=5$, the values of $K_i$ for $i=1,\dots , 6$ are

$$\begin{aligned} \begin{aligned} K_1&=\sqrt{1+\dfrac{2}{\sqrt{5}}}, \qquad K_5=\dfrac{1}{4}+\sqrt{5},\\ K_2(a)&=\dfrac{1-a}{h_{15}}+\dfrac{\sqrt{2}(4+\eta a)}{h_{45}}+\dfrac{\sqrt{2}(4+\xi a)}{h_{55}}, \\ K_3(a)&=\dfrac{1}{(1+a)^2}+\dfrac{\sqrt{2}(4-\xi a)}{h_{25}}+\dfrac{\sqrt{2}(4-\eta a)}{h_{35}}, \\ K_4(a)&=-\dfrac{1-a}{a h_{15}}+\dfrac{\sqrt{2}(\eta +4a)}{a h_{45}}+\dfrac{\sqrt{2}(\xi +4 a)}{a h_{55}}, \\ K_6(a)&=\dfrac{1}{a(1+a)^2}+\dfrac{\sqrt{2}(4a-\xi )}{a h_{25}}+\dfrac{\sqrt{2}(4a-\eta )}{a h_{35}}, \end{aligned} \end{aligned}$$

(9)

where $h_{15}=((1-a)^2)^{3/2}$, $h_{25}= \left( 2 a^2-a \xi +2\right) ^{3/2}$, $h_{35}= \left( 2 a^2-a \eta +2\right) ^{3/2}$, $h_{45}= \left( 2 a^2+a \eta +2\right) ^{3/2}$, $h_{55}=\left( 2 a^2+a \xi +2\right) ^{3/2}$, $\xi = 1+\sqrt{5}$, and $\eta = 1-\sqrt{5}$. So when $n=5$, the solutions $m_2=m_2(a)$ and $m_3=m_3(a)$ of system (3) with $m_D\ne 0$ are given by (4) with $K_i$ for $i=1,\dots ,6$ given by (9).

We proceed in a similar way than in the cases $n=2,3,4$ to find all the real solutions of equations $m_D=0$, $m_{N,2}=0$ and $m_{N,3}=0$ with $a>0$, but in this case to shorten the computations we consider separately the cases $a>1$ and $0<a<1$ and we eliminate the square root corresponding to $h_{15}$ directly by simplification instead of eliminating it by means of a resultant with respect $h_{15}$. To transform equations $m_D=0$, $m_{N,2}=0$ and $m_{N,3}=0$ into polynomial equations with integer coefficients, we introduce the new variables $h_{65}=\sqrt{2}$, $h_{75}=\sqrt{5}$ and $h_{85}=\sqrt{1+2/h_{75}}$. The results that we have obtained are summarized in Table 4. Note that $m_{N,2}$, $m_{N,3}$ and $m_D$ are not simultaneously zero. This means that when $n=5$ there could not be solutions of system (3) with $m_D= 0$.

Table 4 Real positive solutions of $m_D=0$, $m_{N,2}=0$, and $m_{N,3}=0$ for $n=5$

Analyzing the signs of $m_2$ and $m_3$, we see that the region where the masses $m_2$ and $m_3$ given in (4) can provide central configurations is $a\in (0,d_5)\cup (b_{25},\infty )$, see Fig. 5 for the plots of $m_2$ and $m_3$.

Fig. 5

Plot of the masses $m_2$ (continuous line) and $m_3$ (dashed line) for $n=5$

Examining the properties of the functions $m_2$ and $m_3$ we get that in the interval $(0,d_5)$, $m_2$ and $m_3$ are increasing (see Fig. 5a), $m_2,m_3\rightarrow 0$ when $a\rightarrow 0^+$ with $\lim _{a\rightarrow 0^+}m_2/m_3=1$, and $m_2,m_3\rightarrow \infty$ when $a\rightarrow d_5^-$ with $\lim _{a\rightarrow d_5^-}m_2/m_3=1$. In the interval $(b_{25},\infty )$, $m_2$ and $m_3$ are increasing (see Fig. 5b), $m_2\rightarrow 0.8426164718..$ and $m_3\rightarrow 0$ when $a\rightarrow b_{25}^+$, and $m_2,m_3\rightarrow \infty$ when $a\rightarrow \infty$ with $\lim _{a\rightarrow \infty }m_2/m_3=1$.

3.3.5 Numerical Study for $n>5$

Fig. 6

Plot of the masses $m_2$ (continuous line) and $m_3$ (dashed lien) when $n=6$ in a, $n=10$ in b and $n=20$ in c

Fig. 7

Plot of $d_n$ (the zero of $m_D$) in a and plot of $b_n$ (the zero of $m_{N,3}$ with $a>1$) in b

We have analyzed the behavior of $m_2$ and $m_3$ as a function of a for $n=6,7,\dots ,500$, and we have seen that it is essentially the same as the one for $n=5$ (see Fig. 6 for $n=6,10,20$). More precisely, for all $n=5,\dots ,500$, the denominator $m_D$ has a unique zero $d_n<1$ (the existenc of such zero is proved analytically in Lemma 4(d) in Appendix 1). We have computed numerically the value of $d_n$ for $n=6,\dots ,500$ and we have plotted it in Fig. 7a. Note that $d_n<d_{n+1}<1$ for all $n=6,\dots ,499$. We observe that for all $n=6,\dots ,500$ the functions $m_2$ and $m_3$ are increasing in the interval $(0,d_n)$. Moreover, $m_2<m_3$ in this interval (this has already been proved analytically in Proposition 1(e)), $m_2,m_3\rightarrow 0^+$ when $a\rightarrow 0^+$ (this has already been proved analytically in Proposition 1(a) and (b)) and $m_2,m_3\rightarrow \infty$ when $a\rightarrow d_n^-$. So $0<m_2<m_3$ for all $a\in (0,d_n)$ and therefore the interval $(0,d_n)$ provides central configurations. We also observe that for all $n=6,\dots ,500$ the function $m_2$ is negative in the interval $(d_n,1)$; thus, this interval does not provide central configurations. Finally, we observe that for all $n=6,\dots ,500$, $m_3\rightarrow -\infty$ when $a\rightarrow 1^+$ and $m_3\rightarrow \infty$ when $a\rightarrow \infty$ (this has already been proved analytically in Proposition 1(c) and (d))). Moreover, we observe that $m_3$ is increasing in the interval $(1,\infty )$ for all $n=6,\dots ,500$, and therefore, $m_3$ has a unique zero $b_n$ with $a>1$ (from Proposition 1(c) and (d) again we can prove analytically the existence of at least one zero of $m_3$ with $a>1$, numerically we see that this zero is unique). We have computed numerically the value of $b_n$ for $n=6,\dots ,500$ and we have plotted it in Fig. 7b. We see that $b_n>b_{n+1}>1$ for all $n=6,\dots ,499$. Since $m_2>m_3$ when $a>1$ (this is proved analytically in Proposition 1(e)), the interval $(b_n,\infty )$ provides central configurations.

In short, the set of values of a where the solutions $m_2$ and $m_3$ given by (4) are positive is $a\in (0,d_n)\cup (b_n,\infty )$, where $d_n$ is the zero of $m_D$ and $b_n$ is the zero of the numerator $m_{N,3}$ with $a>1$. In the region $(0,d_n)$, both masses go from zero (when a tends to 0) to infinity (when a tends to $d_n$), whereas in the region $(b_n,\infty )$ the mass $m_3$ goes from 0 (when $a=b_n$) to infinity (when $a\rightarrow \infty$) and the mass $m_2$ goes from the positive value $m_2(b_n)$ to infinity (when $a\rightarrow \infty$). We have computed numerically the value $m_2(b_n)$, and we have plotted it in Fig. 8. We observe that the value of $m_2(b_n)$ tends rapidly to 0 as n increases. For instance, when $n=5$, $m_2(b_n)=0.8426164718..$; when $n=20$, $m_2(b_n)=0.0236101462..$; when $n=40$, $m_2(b_n)=0.0000894392..$; when $n=100$, $m_2(b_n)= 1.1846352161..\times 10^{-11}$. All numerical computations have been done with a minimum of 100 digit precision and we have ensured that all the digits given here are exact.

Fig. 8

Plot of $m_2(b_n)$

We observe that as n increases the difference between $m_2$ and $m_3$ decreases rapidly, see again Fig. 6. Thus, as n increases, the masses $m_2$ and $m_3$ in a regular bicircular central configuration of the 3n-body problem tend to be equal.

4 Semiregular Bicircular Central Configurations of the 3n-Body Problem

4.1 The Equations

Now we consider semiregular bicircular central configurations of the 3n-body problem, which consists of n bodies with masses $m_1=\dots =m_n=1$ at the vertices of a regular n-gon inscribed in a circle of radius 1 and 2n bodies with masses equal $m_{n+1}=\dots =m_{3n}=m$ at the vertices of a semiregular 2n-gon inscribed in a circle of radius a. By using complex coordinates, the positions of the vertices of the initial n-gon can be written as ${\mathbf {q}}_j=e^{i\beta _j}$ with $\beta _j=2\pi j/n$ for $j=1,\dots ,n$ and the vertices of the semiregular 2n-gon situated on the circle of radius a can be written as ${\mathbf {q}}_{j+n}=a e^{i(\beta _j-\beta )}$ and ${\mathbf {q}}_{j+2n}=a e^{i(\beta _j+\beta )}$ with $\beta \in (0,\pi /n)$ and $j=1,\dots ,n$, see Fig. 1b.

It is easy to check that the center of mass of the system is at the origin. Under these hypothesis, the first n equations of (1) become

$$\begin{aligned} \sum _{j=1,j\ne k}^n \dfrac{{\mathbf {q}}_k-{\mathbf {q}}_j}{| {\mathbf {q}}_k-{\mathbf {q}}_j|^3}+m \sum _{j=1}^n \dfrac{{\mathbf {q}}_k-{\mathbf {q}}_{j+n}}{| {\mathbf {q}}_k-{\mathbf {q}}_{j+n}|^3}+ m \sum _{j=1}^n \dfrac{{\mathbf {q}}_k-{\mathbf {q}}_{j+2n}}{|{\mathbf {q}}_k-{\mathbf {q}}_{j+2n}|^3}= \lambda {\mathbf {q}}_k, \end{aligned}$$

(10)

for $k=1,\dots ,n$, the following n equations become

$$\begin{aligned} \begin{aligned}&\sum _{j=1}^n \dfrac{{\mathbf {q}}_{k+n}-{\mathbf {q}}_j}{| {\mathbf {q}}_{k+n}-{\mathbf {q}}_j|^3}+m \sum _{j=1,j\ne k}^n \dfrac{{\mathbf {q}}_{k+n}-{\mathbf {q}}_{j+n}}{| {\mathbf {q}}_{k+n}-{\mathbf {q}}_{j+n}|^3}\\&\quad + m \sum _{j=1}^n \dfrac{{\mathbf {q}}_{k+n}-{\mathbf {q}}_{j+2n}}{|{\mathbf {q}}_{k+n}-{\mathbf {q}}_{j+2n}|^3}= \lambda {\mathbf {q}}_{k+n}, \end{aligned} \end{aligned}$$

(11)

for $k=1,\dots ,n$, and the last n equations of (1) are

$$\begin{aligned} \begin{aligned}&\sum _{j=1}^n \dfrac{{\mathbf {q}}_{k+2n}-{\mathbf {q}}_j}{| {\mathbf {q}}_{k+2n}-{\mathbf {q}}_j|^3}+m \sum _{j=1}^n \dfrac{{\mathbf {q}}_{k+2n}-{\mathbf {q}}_{j+n}}{| {\mathbf {q}}_{k+2n}-{\mathbf {q}}_{j+n}|^3} \\&\quad + m \sum _{j=1,j\ne k}^n \dfrac{{\mathbf {q}}_{k+2n}-{\mathbf {q}}_{j+2n}}{|{\mathbf {q}}_{k+2n}-{\mathbf {q}}_{j+2n}|^3}= \lambda {\mathbf {q}}_{k+2n}, \end{aligned} \end{aligned}$$

(12)

for $k=1,\dots ,n$.

Proceeding in a similar way than in Corbera et al. (2009), we divide the k-th equation of (10) by ${\mathbf {q}}_k$, k-th equation of (11) by ${\mathbf {q}}_{k+n}$ and the k-th equation of (12) by ${\mathbf {q}}_{k+2n}$ and we get system

$$\begin{aligned}&\sum _{j=1,j\ne k}^n \dfrac{1-e^{i(\beta _j-\beta _k)}}{| e^{i\beta _k}-e^{i\beta _j}|^3} +m \sum _{j=1}^n \dfrac{1-a\,e^{i(\beta _j-\beta _k-\beta )}}{| e^{i\beta _k}-a\,e^{i(\beta _j-\beta )}|^3}\nonumber \\&\quad + m \sum _{j=1}^n \dfrac{1-a\,e^{i(\beta _j-\beta _k+\beta )}}{| e^{i\beta _k}-a\,e^{i(\beta _j+\beta )}|^3} =\lambda , \nonumber \\&\quad \sum _{j=1}^n \dfrac{1-1/a\,e^{i(\beta _j-\beta _k+\beta )}}{| a\,e^{i(\beta _k-\beta )}-e^{i\beta _j}|^3}+\frac{m}{a^3} \sum _{j=1,j\ne k}^n \dfrac{1-e^{i(\beta _j-\beta _k)}}{| e^{i(\beta _k-\beta )}-e^{i(\beta _j-\beta )}|^3} \nonumber \\&\quad + \frac{m}{a^3} \sum _{j=1}^n \dfrac{1-e^{i(\beta _j-\beta _k+2\beta )}}{| e^{i(\beta _k-\beta )}-e^{i(\beta _j+\beta )}|^3} =\lambda ,\nonumber \\&\quad \sum _{j=1}^n \dfrac{1-1/a\,e^{i(\beta _j-\beta _k-\beta )}}{| a\,e^{i(\beta _k+\beta )}-e^{i\beta _j}|^3}+\frac{m}{a^3} \sum _{j=1}^n \dfrac{1-e^{i(\beta _j-\beta _k-2\beta )}}{| e^{i(\beta _k+\beta )}-e^{i(\beta _j-\beta )}|^3}\nonumber \\&\quad + \frac{m}{a^3} \sum _{j=1,j\ne k}^n \dfrac{1-e^{i(\beta _j-\beta _k)}}{| e^{i(\beta _k+\beta )}-e^{i(\beta _j+\beta )}|^3} =\lambda , \end{aligned}$$

(13)

for $k=1,\dots ,n$. Here

$$\begin{aligned} \begin{aligned} \left| e^{i\beta _k}-e^{i\beta _j}\right|&=\left| e^{i(\beta _k\pm \beta )}-e^{i(\beta _j\pm \beta )}\right| =\left( 2-2\cos (\beta _j-\beta _k)\right) ^{1/2},\\ \left| e^{i\beta _k}-a\,e^{i(\beta _j\pm \beta )}\right|&=\left( 1+a^2-2a\cos (\beta _j-\beta _k\pm \beta )\right) ^{1/2},\\ \left| a\,e^{i(\beta _k\pm \beta )}-e^{i\beta _j}\right|&=\left( 1+a^2-2a\cos (\beta _j-\beta _k\mp \beta )\right) ^{1/2},\\ \left| e^{i(\beta _k\mp \beta )}-e^{i(\beta _j\pm \beta )}\right|&=\left( 2-2\cos (\beta _j-\beta _k\pm 2\beta )\right) ^{1/2}. \end{aligned} \end{aligned}$$

Since for all $k=1,\dots ,n$, the set $\{\beta _j-\beta _k+\varphi \}_{j=1,\dots ,n}$ modulus $2\pi$ is equal to the set $\{2\pi j/n+\varphi \}_{j=1,\dots ,n}$ for all $\varphi \in {\mathbb {R}}$, the equations of system (13) are independent of k. So it is not restrictive to take $k=n$. After straightforward computations, we can see that system (13) is equivalent to the system

$$\begin{aligned} \begin{aligned}&K_1+m \big (L_2+N_2i \big )+ m \big (L_3+N_3i) =\lambda ,\\&\quad L_4+N_4i+ \frac{m}{a^3} K_1+\frac{m}{a^3}\big (L_5+N_5i\big ) =\lambda ,\\&\quad L_6+N_6i+\frac{m}{a^3} \big (L_7+N_7i\big )+ \frac{m}{a^3} K_1 =\lambda , \end{aligned} \end{aligned}$$

(14)

where $K_1$ is defined as in Sect. 3 (see (3)) and

$$\begin{aligned} L_2= & {} L_2(a,\beta ) =\sum _{j=1}^n\frac{1-a\cos \big (\frac{2\pi \,j}{n}-\beta \big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}-\beta \big )\Big )^{3/2}},\\ N_2= & {} N_2(a,\beta ) =\sum _{j=1}^n\frac{-a\sin \big (\frac{2\pi \,j}{n}-\beta \big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}-\beta \big )\Big )^{3/2}},\\ L_4= & {} L_4(a,\beta ) =\sum _{j=1}^n\frac{1-1/a\cos \big (\frac{2\pi \,j}{n}+\beta \big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}+\beta \big )\Big )^{3/2}},\\ N_4= & {} N_4(a,\beta ) =\sum _{j=1}^n\frac{-1/a\sin \big (\frac{2\pi \,j}{n}+\beta \big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}+\beta \big )\Big )^{3/2}},\\ L_5= & {} L_5(\beta ) =\sum _{j=1}^n\frac{1-\cos \big (\frac{2\pi \,j}{n}+2\beta \big )}{\Big (2-2\cos \big (\frac{2\pi \,j}{n}+2\beta \big )\Big )^{3/2}},\\ N_5= & {} N_5(\beta ) =\sum _{j=1}^n\frac{-\sin \big (\frac{2\pi \,j}{n}+2\beta \big )}{\Big (2-2\cos \big (\frac{2\pi \,j}{n}+2\beta \big )\Big )^{3/2}},\\ L_3= & {} L_3(a,\beta )=L_2(a,-\beta ), \qquad N_3=N_3(a,\beta )=N_2(a,-\beta ),\\ L_6= & {} L_6(a,\beta )=L_4(a,-\beta ), \qquad N_6=N_6(a,\beta )=N_4(a,-\beta ),\\ L_7= & {} L_7(\beta )=L_5(-\beta ), \qquad \qquad N_7=N_7(\beta )=N_5(-\beta ). \end{aligned}$$

Note that

$$\begin{aligned} \sum _{j=1}^{n-1}\frac{-\sin \big (\frac{2\pi \,j}{n}\big )}{\Big (2-2\cos \big (\frac{2\pi \,j}{n}\big )\Big )^{3/2}} =0. \end{aligned}$$

Since for all $\varphi \in {\mathbb {R}}$

$$\begin{aligned} \cos \big (\tfrac{2\pi \,j}{n}-\varphi \big )=\cos \big (\tfrac{2\pi \,(n-j)}{n}+\varphi \big ), \qquad \sin \big (\tfrac{2\pi \,j}{n}-\varphi \big )=-\sin \big (\tfrac{2\pi \,(n-j)}{n}+\varphi \big ), \end{aligned}$$

we see that

$$\begin{aligned} \begin{aligned} L_2(a,\beta )&=L_3(a,\beta ), \quad N_2(a,\beta )=-N_3(a,\beta ), \quad L_4(a,\beta )=L_6, \\ N_4(a,\beta )&=-N_6(a,\beta ), \quad L_5(\beta )=L_7(\beta ), \quad N_5(\beta )=-N_7(\beta ). \end{aligned} \end{aligned}$$

So system (14) is equivalent to system

$$\begin{aligned}&K_1 + 2 m L_2(a,\beta ) =\lambda , \nonumber \\ {}&L_4(a,\beta ) + \frac{m}{a^3}(K_1 + L_5(\beta ))=\lambda , \\ {}&N_4(a,\beta ) + \frac{m}{a^3} N_5(\beta )=0. \nonumber \end{aligned}$$

(15)

Solving the third identity in (15), we get

$$\begin{aligned} m=-a^3 \frac{N_4(a,\beta )}{N_5(\beta )} \end{aligned}$$

(16)

and from the first and second identities in (15), we obtain

$$\begin{aligned} m=a^3 \frac{(K_1 - L_4(a,\beta ))}{K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta )}. \end{aligned}$$

(17)

Therefore, from (16) and (17) we have

$$\begin{aligned} F(a,\beta ):=N_5(\beta )(K_1-L_4(a,\beta )) + N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta ))=0. \end{aligned}$$

In short, a semiregular bicircular configuration of the 3n-body problem is central if $m=m(a,\beta )$ is given by (16) and $a,\beta$ are such that $F(a,\beta )=0$ and $m(a,\beta )>0$.

4.2 Admissible Values of $\beta$

The following proposition provides the range of values of $\beta$ that can provide semiregular bicircular central configurations of the 3n-body problem.

Proposition 2

A necessary condition to have a semiregular bicircular central configuration of the 3n-body problem is that $\beta \in (\pi /2n,\pi /n)$.

Proof

We will see that $m=-a^3 N_4(a,\beta )/N_5(\beta ) >0$ if and only if $\beta \in (\pi /2n,\pi /n)$. We recall that

$$\begin{aligned} N_4(a,\beta ) =\frac{1}{a^2 } \frac{d}{d \beta } \sum _{j=1}^n \frac{1}{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+\beta \big )\Big )^{1/2}} \end{aligned}$$

and that

$$\begin{aligned} N_5(\beta ) =\frac{1}{2} \lim _{a \rightarrow 1} \frac{d}{d \beta } \sum _{j=1}^n \frac{1}{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+2\beta \big )\Big )^{1/2}}. \end{aligned}$$

Consider first the case in which $a \in [0,1)$. In this case, using Proposition 6 in Appendix 1 with $\alpha = 1/2$ and $u=\beta$ and taking derivatives with respect to $\beta$ we get

$$\begin{aligned} \begin{aligned} N_4(a,\beta )&= \frac{n}{a^2 \pi } \int _0^1 \frac{d}{d \beta }\left( \frac{t^{-1/2}}{(1-t)^{1/2}}\,\frac{1}{(1-a^2t)^{1/2}}\,\frac{1-(at)^{2n}}{B_1}\right) dt \\&= \frac{n \sin (n \beta )}{a^2\pi } \int _0^1 \frac{-2 n (at)^n (1-(at)^{2n}) }{t^{1/2} (1-t)^{1/2} (1-a^2 t)^{1/2} B_1^2} \, d t, \end{aligned} \end{aligned}$$

with $B_1=1+(at)^{2n}-2(at)^n \cos (n \beta )$, which is negative for $\beta \in (0,\pi /n)$ because the integrand in the integral is negative. Moreover, using Proposition 6 again with $\alpha = 1/2$ and $u=2\beta$ and taking derivatives with respect to $\beta$, we get

$$\begin{aligned} \begin{aligned} N_5(\beta )&= \frac{1}{2} \lim _{a \rightarrow 1^-} \frac{n}{\pi } \int _0^1 \frac{d}{d \beta }\left( \frac{t^{-1/2}}{(1-t)^{1/2}}\,\frac{1}{(1-a^2t)^{1/2}}\,\frac{1-(at)^{2n}}{B_2}\right) dt \\&= \frac{n\sin (2 n \beta )}{\pi } \lim _{a \rightarrow 1^-} \int _0^1 \frac{-2 n (at)^n (1-(at)^{2n}) }{t^{1/2} (1-t)^{1/2} (1-a^2 t)^{1/2}B_2^2} \, d t, \end{aligned} \end{aligned}$$

with $B_2=1+(at)^{2n}-2(at)^n \cos (2 n \beta )$, which is negative if $\beta \in (0,\pi /2n)$ and positive if $\beta \in (\pi /2n,\pi /n)$ because again the integrand is negative.

Consider now the case $a >1$. In this case using Proposition 7 in Appendix 1 with $\alpha = 1/2$ and $u=\beta$ and taking derivatives with respect to $\beta$, we get that

$$\begin{aligned} \begin{aligned} N_4(a,\beta )&= \frac{n}{a^2\pi } \int _0^1 \frac{d}{d \beta }\left( \frac{a^{2n}-t^{2n}}{t^{1/2} (1-t)^{1/2} (a^2-t)^{1/2} B_3}\right) \, d t \\&= \frac{n \sin (n \beta ) }{a^2\pi } \int _0^1 \frac{-2 n (at)^n (a^{2n}-t^{2n}) }{t^{1/2} (1-t)^{1/2} (a^2-t)^{1/2} B_3^2} \, d t, \end{aligned} \end{aligned}$$

with $B_3=a^{2n} +t^{2n} -2 a^n t^n \cos (n \beta )$, which is negative for $\beta \in (0,\pi /n)$. Moreover, using Proposition 7 again with $\alpha = 1/2$ and $u=2 \beta$ and taking derivatives with respect to $\beta$, we get that

$$\begin{aligned} \begin{aligned} N_5(\beta )&= \frac{1}{2} \lim _{a \rightarrow 1^+} \frac{n}{\pi } \int _0^1 \frac{d}{d \beta }\left( \frac{a^{2n}-t^{2n}}{t^{1/2} (1-t)^{1/2} (a^2-t)^{1/2} B_4}\right) \, d t \\&= \frac{n \sin (2 n \beta )}{\pi } \lim _{a \rightarrow 1^+} \int _0^1 \frac{-2 n (at)^n (a^{2n}-t^{2n}) }{t^{1/2} (1-t)^{1/2} (1-a^2 t)^{1/2} B_4^2} \, d t, \end{aligned} \end{aligned}$$

with $B_4=a^{2n} +t^{2n} -2 a^n t^n \cos (2 n \beta )$, which is negative if $\beta \in (0,\pi /2n)$ because the integrand is negative and positive if $\beta \in (\pi /2n,\pi /n)$, again because the integrand is negative. Therefore, $N_4(a,\beta )$ is negative for $\beta \in (0,\pi /n)$ and $N_5(\beta )$ is negative for $\beta \in (0,\pi /2n)$ and positive for $\beta \in (\pi /2n,\pi /n)$.

In short, $m=-a^3N_4(a,\beta )/N_5(\beta )$ is positive if and only if $\beta \in (\pi /2n,\pi /n)$ and the proposition is proved. $\square$

4.3 Proof of Theorem 6(a)

We study the existence of central configurations of the semiregular bicircular 3n-body problem around $\beta =\pi /n$. For proving Theorem 6(a), we need the following auxiliary proposition concerning the sign of the function $F(a,\beta )$ as $\beta \rightarrow \pi /n$.

Proposition 3

The following holds for ${\bar{F}}(a)=\lim _{\beta \rightarrow \pi /n} F(a,\beta )$.

• (a) ${\bar{F}}(a)>0$ when $a \rightarrow \infty$;

• (b) ${\bar{F}}(a) <0$ when $a\rightarrow 1$;

• (c) ${\bar{F}}(a) > 0$ when $a\rightarrow 0$ and $n\ge 3$ and ${\bar{F}}(a) < 0$ when $a\rightarrow 0$ and $n=2$.

We note that in Proposition 3 we have that ${\bar{F}}(a)$ could be $\pm \infty$.

Proposition 3 is proved in Appendix 2.

Proof of Theorem6(a)

It follows from Proposition 2 that all solutions of $F(a,\beta )=0$ for $\beta \in (\pi /2n,\pi /n)$ satisfy $m>0$; therefore, all solutions of $F(a,\beta )=0$ provide central configurations of the semiregular bicircular 3n-body problem. Notice that the function F is continuous for $a\in (0,\infty )$ and $\beta \in (\pi /2n,\pi /n)$; therefore, the points where the sign of F changes provide always solutions of $F(a,\beta )=0$.

In view of Proposition 3, we have that when $n\ge 3$, fixed a value of $\beta$ in a sufficiently small neighborhood of $\pi /n$, the function F has at least one change of sign with $a>1$ and one change of sign with $0<a<1$. So for each $\beta$ in a sufficiently small neighborhood of $\pi /n$ there are at least two values of a for which $F(a,\beta )=0$, one with $a>1$ and one with $0<a<1$. When $n=2$ the function F has at least one change of sign with $a>1$. So for each $\beta$ in a sufficiently small neighborhood of $\pi /n$, there is at least one value of a with $a>1$ for which $F(a,\beta )=0$.

On the other hand, from the proofs of Lemma 5 and Proposition 3 (see Appendix 2), we have that $\lim _{\beta \rightarrow \pi /n} N_4(a,\beta )=0$ and $\lim _{\beta \rightarrow \pi /n} N_5(\beta )=\infty$, respectively. Therefore, from (16), $m\rightarrow 0$ when $\beta \rightarrow \pi /n$.

$\square$

4.4 Proof of Theorem 6(b)

Now we study the existence of central configurations of the semiregular bicircular 3n-body problem around $\beta =\pi /2n$. For proving Theorem 6(b), we need the following two auxiliary propositions concerning the study of the sign of the function $F(a,\beta )$.

Proposition 4

The following holds for ${\bar{F}}(a)=\lim _{\beta \rightarrow \pi /2n}F(a,\beta )$.

• (a) ${\bar{F}}(a)<0$ when $a \rightarrow 0$;

• (b) ${\bar{F}}(a) <0$ when $a\rightarrow \infty$;

• (c) ${\bar{F}}(a) > 0$ when $a\rightarrow 1$.

Proposition 5

The following statements hold for ${\bar{F}}(\beta )=\lim _{a\rightarrow 0} F(a,\beta )$ and ${\tilde{F}}(\beta )=\lim _{a\rightarrow \infty } F(a,\beta )$.

• (a) For all $\beta \in (\pi /2n,\pi /n)$, we have ${\bar{F}}(\beta )>0$ for $n\ge 3$ and ${\bar{F}}(\beta )<0$ for $n=2$.

• (b) For all $\beta \in (\pi /2n,\pi /n)$ and $n\ge 2$, we have ${\tilde{F}}(\beta )>0$.

We note that in Proposition 5, both ${\bar{F}}(\beta )$ and ${\tilde{F}}(\beta )$ could be $\pm \infty$.

The proof of Propositions 4 and 5 can be found in Appendix 3.

Proof of Theorem 6(b)

As in Theorem 6(a) recall that all solutions of $F(a,\beta )=0$ with $\beta \in (\pi /2n,\pi /n)$ satisfy $m>0$, and therefore, they provide central configurations of the semiregular bicircular 3n-body problem. Moreover, the points where the sign of F changes provide solutions of $F(a,\beta )=0$.

In view of Proposition 4, we have that in a sufficiently small neighborhood of $\beta =\pi /2n$ the function F has at least one change of sign with $a>1$ and one change of sign with $0<a<1$. Therefore, fixed a value of $\beta$ in a sufficiently small neighborhood of $\beta =\pi /2n$, there exist at least two zeros of F, one with $a<1$ and one with $0<a<1$.

In view of Propositions 4 and 5, we have that when $n\ge 3$

$$\begin{aligned} \lim _{a\rightarrow 0}\lim _{\beta \rightarrow \pi /2n} F(a,\beta )<0, \quad \lim _{\beta \rightarrow \pi /2n} \lim _{a\rightarrow 0} F(a,\beta )>0. \end{aligned}$$

Therefore, fixed a value of $\beta$ in a sufficiently small neighborhood of $\pi /2n$, there exists at least one zero of F with a sufficiently close to the origin.

Again, in view of Propositions 4 and 5 we have that for all $n\ge 2$

$$\begin{aligned} \lim _{a\rightarrow \infty }\lim _{\beta \rightarrow \pi /2n} F(a,\beta )<0, \quad \lim _{\beta \rightarrow \pi /2n} \lim _{a\rightarrow \infty } F(a,\beta )>0 \end{aligned}$$

Therefore, fixed a value of $\beta$ in a sufficiently small neighborhood of $\pi /2n$, there exists at least one zero of F with a sufficiently large.

In short, fixed a value of $\beta$ in a sufficiently small neighborhood of $\pi /2n$ there exist at least four zeroes of F when $n\ge 3$, one near $a=0$, one with $0<a<1$ not necessarily small, one with a sufficiently large, and one with $a>1$ not necessarily large.

When $n=2$, there exist at least three solutions of F one with a sufficiently large, one with $a>1$ not necessarily large, and one with $0<a<1$.

On the other hand, in the proof of Lemma 6 (see Appendix 3) we have seen that $\lim _{\beta \rightarrow \pi /2n}N_5(\beta )=0$. Moreover from (4.2), we have that $a\not \in \{0;\infty \}$ then $\lim _{\beta \rightarrow \pi /2n}N_4(a,\beta )\ne 0$. Therefore, from (16) we can guarantee that $m\rightarrow \infty$ at the central configurations coming from the zeroes of F with $0<a<1$ not small and $a>1$ not large.

This completes the proof of Theorem 6(b). $\square$

5 Particular Cases of Semiregular Bicircular Central Configurations of the 3n-Body Problem

When $n= 2$ from Theorem 6(b), we have at least three families (depending on $\beta$) of central configurations in a neighborhood of $\beta =\pi /4$, one emanating from a point $(a_1,\pi /4)$, one emanating from $(a_2,\pi /4)$ with $a_2>1$, and one emanating from $(\infty ,\pi /4)$. From Theorem 6(a), we have at least one family of central configurations in a neighborhood of $\beta =\pi /2$ that emanates from a point $(a_2^*,\pi /2)$ with $a_2^*>1$. They are given by the families of zeroes of F for $n=2$.

We have studied numerically these families of zeroes, and we have obtained the following (see Fig. 9a) the family of central configurations emanating from the point $(a_1,\pi /4)=(0.6240605991..,\pi /4)$ joins the family emanating from $(a_2^*,\pi /2)=(\sqrt{3},\pi /2)$ and the family emanating from $(a_2,\pi /4)=(1.4339374069..,\pi /4)$ joins the family emanating from $(\infty ,\pi /4)$. Moreover, these are the only families of central configurations. In particular, if $\beta \in (\pi /4,b^*)$ with $b^*=0.9195936184..$ the semiregular bicircular 6-body problem has three different central configurations, if $b=b^*$ it has two central configurations and if $\beta >b^*$ it has only one central configuration.

Fig. 9

Solutions of $F(a,\beta )=0$

Fig. 10

Values of m at the families of semiregular bicircular central configurations of the 3n-body problem

When $n\ge 3$ from Theorem 6(a), we have at least four families of central configurations of the semiregular bicircular 3n-body problem in a neighborhood of $\beta =\pi /2n$, one emanating from $(0,2\pi /n)$, one emanating from a point $(a_1,\pi /2n)$ with $a_1\in (0,1)$, one emanating from a point $(a_2,\pi /2n)$ with $a_2>1$ and one emanating from $(\infty ,\pi /2n)$. Moreover, from Theorem 6(b) we have at least two families of central configurations in a neighborhood of $\beta =\pi /n$, one emanating from a point $(a_1^*,\pi /n)$ with $a_1^*\in (0,1)$ and one emanating from a point $(a_2^*,\pi /n)$ with $a_2^*>1$. As above, they are given by the families of zeroes of F.

We have studied numerically these families of zeroes for $n=3,4,5,6$, and we have obtained the following (see again Fig. 9).

When $n=3$, the family of central configurations emanating from the point $(0,\pi /6)$ joins the family emanating from $(a_1^*,\pi /3)=(0.4138879324..,\pi /3)$, the family emanating from $(a_1,\pi /6)=(0.6280478552..,\pi /6)$ joins the family emanating from $(a_2,\pi /6)=(1.1308109202..,\pi /6)$ and the family emanating from $(\infty ,\pi /6)$ joins the family emanating from $(a_2^*,\pi /3)=(1.6197896088..,\pi /3)$. Moreover, these are the only families of central configurations when $n=3$. In particular, if $\beta \in (\pi /6,b^*)$ with $b^*=0.7119233840..$ the semiregular bicircular 9-body problem has four different central configurations, if $b=b^*$ it has three central configurations and if $\beta >b^*$ it has two central configurations.

When $n=4$, the family of central configurations emanating from $(0,\pi /8)$ joins the family emanating from $(a_1,\pi /8)=(0.6351161391..,\pi /8)$, the family emanating from $(a_2,\pi /8)=(1.0636734282..,\pi /8)$ joins the family emanating from $(a_1^*,\pi /4)=(0.697380509..,\pi /4)$ and the family emanating from $(\infty ,\pi /8)$ joins the family emanating from $(a_2^*,\pi /4)=(1.6024084862..,\pi /4)$. Moreover, these are the only families of central configurations when $n=4$. In particular, if $\beta \in (\pi /2n,b^*)$ with $b^*=0.4665964724..$ the semiregular bicircular 12-body problem has four different central configurations, if $b=b^*$ it has three central configurations and if $\beta >b^*$ it has two central configurations. The same behavior occurs for $n=5,\dots , 100$ (see Fig. 9 for $n=5,6$), so we conjecture that this happens for all $n>6$. We note that when $n=5$, $a_1=0.6434495204..$, $a_2=1.0379259369..$, $a_1^*=0.822828699..$, $a_2^*=1.5979217289..$ and $b^*=0.3406546931..$; and when $n=6$, $a_1=0.6515248377..$, $a_2=1.0252694202..$, $a_1^*=0.8843211381..$, $a_2^*=1.5922353553..$ and $b^*=0.2733239284..$.

Finally, we have computed the values of the masses for the families of central configurations with $n=2,3,4$, and we have plotted them in Fig. 10.

Appendices

Appendix 1: Proof of Proposition 1

We state and prove some auxiliary results that will be used in the proof of Proposition 1. We need the following two propositions taken from Bang and Elmabsout (2003).

Proposition 6

(Bang and Elmabsout 2003, Proposition 7) For $0\leqslant a<1$, $\alpha \in (0,1)$ and $u\in [0,2\pi )$, we have

$$\begin{aligned} \begin{aligned}&\sum _{j=1}^n \frac{1}{\big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}+u\big )\big )^\alpha }\\&\quad = \frac{n \,\sin (\pi \alpha )}{\pi }\int _0^1 \frac{t^{\alpha -1}}{(1-t)^\alpha }\,\frac{1}{(1-a^2t)^\alpha }\,\frac{1-(at)^{2n}}{1+(at)^{2n}-2(at)^n\cos (nu)}dt. \end{aligned} \end{aligned}$$

Proposition 7

(Bang and Elmabsout 2003, Proposition 8) For $a>1$, $\alpha \in (0,1)$ and $u\in [0,2\pi )$, we have

$$\begin{aligned} \begin{aligned}&\sum _{j=1}^n \frac{1}{\big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}+u\big )\big )^\alpha }\\&\quad = \frac{n\,\sin (\pi \alpha )}{\pi }\int _0^1 \frac{t^{\alpha -1}}{(1-t)^\alpha }\,\frac{1}{(a^2-t)^\alpha }\,\frac{a^{2n}-t^{2n}}{a^{2n}+t^{2n}-2(at)^n\cos (nu)}dt. \end{aligned} \end{aligned}$$

We need the following auxiliary result.

Lemma 1

Let $u\in {\mathbb {R}}$ and let $\gamma =2\pi \,j/n+u$.

• (a) The following identities hold for all $\ell \in {\mathbb {N}}$

  $$\begin{aligned} \sum _{j=1}^n \cos \left( \frac{2\ell \pi \,j}{n}+u\right) =0, \qquad \sum _{j=1}^n \sin \left( \frac{2\ell \pi \,j}{n}+u\right) =0, \end{aligned}$$

  (18)

  when $n\ge 2$ and $n\ne \ell$.

• (b) For $n\ge 3$, we have

  $$\begin{aligned} \sum _{j=1}^n \cos ^2 \gamma =\frac{n}{2}, \end{aligned}$$

  (19)

  and for $n=2$ we have

  $$\begin{aligned} \sum _{j=1}^2 \cos ^2\big (\pi \,j+u\big ) =2\cos ^2 u. \end{aligned}$$

  (20)

• (c) For all $n\ge 1$, we get

  $$\begin{aligned}\sum _{j=1}^n\frac{a-\cos \gamma }{\Big (1+a^2-2a\cos \gamma \Big )^{3/2}}=-\frac{d}{da}\sum _{j=1}^n\frac{1}{\Big (1+a^2-2a\cos \gamma \Big )^{1/2}}. \end{aligned}$$

• (d) Let

  $$\begin{aligned} L(a,u)=\sum _{j=1}^n\frac{1-1/a\cos \gamma }{\Big (1+a^2-2a\cos \gamma \Big )^{3/2}}. \end{aligned}$$

  We have $L(a,u)=-n/2+O(a)$ when $n\ge 3$ and $L(a,u)=2-6\cos ^2u+O(a)$ when $n=2$.

Proof

Using the sum of the first n terms of a geometric series, we get

$$\begin{aligned} \sum _{j=1}^n e^{i(2\ell \pi j/n+u)}=0, \end{aligned}$$

for all $\ell \in {\mathbb {Z}}$ with $\ell \ne n$ (here $i=\sqrt{-1}$). This proves statement (a). From the formula of the cosinus of twice an angle an applying statement (a) with $\ell =2$ and 2u instead of u, we get

$$\begin{aligned} \sum _{j=1}^n \cos ^2\left( \frac{2\pi \,j}{n}+u\right) =\frac{1}{2}\sum _{j=1}^n \left( \cos \left( 2\Big (\frac{2\pi \,j}{n}+u\Big )\right) +1 \right) =\frac{n}{2}. \end{aligned}$$

This proves statement (b) for $n>2$. Statement (b) for $n=2$ and statement (c) follows from direct computations.

Expanding the function L in Laurent series around $a=0$, we have

$$\begin{aligned} L(a,u)=-\frac{1}{a} \sum _{j=1}^n \cos \big (\frac{2\pi \,j}{n}+u \big ) + \sum _{j=1}^n 1 - 3 \sum _{j=1}^n \cos ^2\big (\frac{2\pi \,j}{n}+u \big ) + O(a). \end{aligned}$$

Then, when $n\ge 3$ in view of (18) with $\ell =1$ together with (19) we obtain

$$\begin{aligned} L(a,u)=n-\frac{3n}{2} + O(a)= -\frac{n}{2} + O(a) \end{aligned}$$

and when $n=2$ in view of (18) with $\ell =1$ and (20) we obtain

$$\begin{aligned} L(a,u)=2-6\cos ^2 u + O(a). \end{aligned}$$

This completes the proof of statement (d).

$\square$

We need the following technical lemma.

Lemma 2

We have $f(v)=(1+v)(1-v^{2n})-4 n v^n (1-v) > 0$ for $n \ge 2$ and $v \in (0,1)$.

Proof

Note that

$$\begin{aligned} \begin{aligned} f(v) =&(1+v)(1-v^n)(1+v^n)-4 n v^n (1-v) \\ =&(1-v)\big ((1+v)(1+v+v^2 +\ldots + v^{n-1})(1+v^n)-4 n v^n\big ) \\ :=&(1-v) g(v) \end{aligned} \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} g(v)&= (1+v)(1+v+v^2 +\ldots + v^{n-1})(1+v^n) -4 n v^n \\&= 1 +2v+2 v^2 + 2 v^3 + \ldots + 2v^{n-1}+ 2 v^n +2 v^n + 2 v^{n+1} +\ldots \\&\quad + 2 v^{2n-1} +v^{2n}-4 n v^n \\&= 1 +2 v +\ldots + 2 v^{n-1} + (2-4n) v^n + 2 v^{n+1} + \ldots + 2 v^{2n-1} + v^{2n}. \end{aligned} \end{aligned}$$

(21)

We see that

$$\begin{aligned} g(1)=0 \quad \text {and} \quad g'(1)=0. \end{aligned}$$

Indeed,

$$\begin{aligned} g(1)=1+ 2 (n-1) + 2-4n + 2 (n-1) +1 = 0, \end{aligned}$$

and since

$$\begin{aligned} \begin{aligned} g'(v)&=2 +4 v + 6v^2 + \ldots + 2(n-1) v^{n-2} + (2-4n)n v^{n-1} \\&\quad + 2 (n+1) v^n +\ldots + 2(2n-1) v^{2n-2} +2 n v^{2n-1}, \end{aligned} \end{aligned}$$

then

$$\begin{aligned} \begin{aligned} g'(1)&=2 (1+2+3 +\ldots + (n-1))+ n (2-4n) \\&\quad + 2 (n+1+n+2 + \ldots + 2n-1) +2 n\\&= 2 \Big (\sum _{j=1}^{2n-1} j - n + (1-2n) n +n \Big ) =2 \Big ( \sum _{j=1}^{2n-1} j + n(1-2n)\Big )=0. \end{aligned} \end{aligned}$$

Therefore,

$$\begin{aligned} g(v)= (1-v)^2 h(v), \quad h(v)=\sum _{j=0}^{2n-2} c_j v^j \end{aligned}$$

for some coefficients $c_j$.

We will show by induction that

$$\begin{aligned} c_j ={\left\{ \begin{array}{ll} (j+1)^2 &{} \text {for }j=0,\ldots , n-1, \\ (2n-j-1)^2 &{} \text {for }j=n,\ldots , 2n-2. \end{array}\right. } \end{aligned}$$

In view of (21), we have that the coefficients $c_j$ satisfy $c_0=1$, $c_1=2 + 2 c_0=4$,

$c_{2n-3} -2 c_{2n-2}=2$ and $c_{2n-2}=1$ (and so $c_{2n-3}= 4).$

We prove by induction the cases for $j=0,\ldots ,n-1$. It is clear for $j=0,1$ and we will prove it for some $2 \le j < n-1$. Note that by the induction hypotheses

$$\begin{aligned} c_{j+1}= -c_{j-1} + 2 c_j +2 = -j^2 +2 j^2 +4 j +2+ 2 = j^2 +4j + 4 = (j+2)^2 \end{aligned}$$

and so the induction is satisfied for $j=0,\ldots ,n-1$. For $j=n$, we have

$$\begin{aligned} c_n = 2 -4 n -(n-1)^2 + 2 n^2 =2 -4 n -n^2 +2n -1 +2 n^2 =n^2-2n +1 =(n-1)^2, \end{aligned}$$

and for $j=n+1$ we have

$$\begin{aligned} c_{n+1} = -c_{n-1}+2c_n+2=-(n-1+1)^2+2(n-1)^2+2=(2n-(n+1)-1)^2. \end{aligned}$$

For $j=n, \ldots , 2n-2$, the induction hypotheses yield $c_j=(2n-j-1)^2$. Note that it is clear for $j=n$ and $j=n+1$ and we will show it for $n+1<j \le 2n-2$. In particular, the cases $j=2n-3$ and $j=2n-2$ are also trivially satisfied. So, we only need to show it for $n+1< j < 2n-3$. By the induction hypotheses for any $n+1< j < 2n-3$, we have

$$\begin{aligned} \begin{aligned} c_j&= 2 -c_{j-2} +2 c_{j-1}= 2 - (2n-(j-2)-1)^2 + 2 (2n-(j-1)-1)^2 \\&= 2-(2n-j+1)^2 +2 (2n-j)^2 =(2n-j-1)^2 \end{aligned} \end{aligned}$$

and the induction hypotheses holds. In short, the lemma is proved. $\square$

The next result concerns properties of the functions $K_i(a)$ introduced in Sect. 3.

Lemma 3

The following statements hold for all $n\ge 2$:

• (a) $K_6(a)>K_4(a)$ for $a\in (0,1)$;

• (b) $K_6(a)<K_4(a)$ for $a>1$;

• (c) $K_5>K_1>0$.

• (d) $a^3K_4(a)=K_2(1/a)$ and $a^3K_6(a)=K_3(1/a)$.

Proof

Note that

$$\begin{aligned} \begin{aligned} aK_6(a)&=\sum _{j=1}^n \frac{a-\cos \big (\frac{2 \pi j}{n} +\frac{\pi }{n} \big )}{\big (1+a^2 -2 a \cos \big (\frac{2 \pi j}{n}+ \frac{\pi }{n} \big )\big )^{3/2}}, \\ aK_4(a)&= \sum _{j=1}^n \frac{a-\cos \big (\frac{2 \pi j}{n} \big )}{\big (1+a^2 -2 a \cos \big (\frac{2 \pi j}{n} \big )\big )^{3/2}}. \end{aligned} \end{aligned}$$

When $a \in (0,1)$, using Lemma 1(c) and Proposition 6 with $\alpha =1/2$ and $u=0$, we get

$$\begin{aligned} \begin{aligned}&a K_4(a)= -\frac{n}{\pi }\int _0^1 \frac{d}{d a}\left( \frac{t^{-1/2}}{(1-t)^{1/2}}\,\frac{1}{(1-a^2t)^{1/2}}\,\frac{1-(at)^{2n}}{1+(at)^{2n}-2(at)^n}\right) dt. \end{aligned} \end{aligned}$$

and using Lemma 1(c) and Proposition 6 with $\alpha =1/2$ and $u=\pi /n$, we get

$$\begin{aligned} \begin{aligned}&a K_6(a)= -\frac{n}{\pi }\int _0^1 \frac{d}{d a}\left( \frac{t^{-1/2}}{(1-t)^{1/2}}\,\frac{1}{(1-a^2t)^{1/2}}\,\frac{1-(at)^{2n}}{1+(at)^{2n}+2(at)^n}\right) dt. \end{aligned} \end{aligned}$$

Hence, for $a \in (0,1)$,

$$\begin{aligned} \begin{aligned} K_6(a) - K_4(a)&= -\frac{n}{\pi a} \int _0^1 \frac{d}{d a} \left( \frac{t^{-1/2}}{(1-t)^{1/2}} \, \frac{1}{(1-a^2 t)^{1/2}} \, \frac{4 (a t)^n}{((at)^{2n}-1)} \right) \, d t \\&= -\frac{n}{\pi a} \int _0^1 \frac{4 (ta)^n (t a^2 (-1+(ta)^{2n}) + n (t a^2 -1)(1+(t a)^{2n}))}{a (1-t)^{1/2} t^{1/2} (1-a^2 t)^{3/2} ((ta)^{2n}-1)^2 }\, d t >0, \end{aligned} \end{aligned}$$

because the integrand is negative for $a \in (0,1)$. Therefore, $K_6(a) > K_4(a)$ for $0< a < 1$ and so statement (a) is proved.

For $a > 1$ using Lemma 1(c) and Proposition 7 with $\alpha =1/2$ and $u=0$, we get

$$\begin{aligned}\begin{aligned}&aK_4(a)=- \frac{n}{\pi }\int _0^1 \frac{d}{d a}\left( \frac{t^{-1/2}}{(1-t)^{1/2}}\,\frac{1}{(a^2-t)^{1/2}}\,\frac{a^{2n}-t^{2n}}{a^{2n}+t^{2n}-2 a^n t^n}\right) dt. \end{aligned} \end{aligned}$$

and using Lemma 1(c) and Proposition 7 with $\alpha =1/2$ and $u=\pi /n$ and taking derivatives with respect to a, we get

$$\begin{aligned}\begin{aligned}&aK_6(a)=- \frac{n}{\pi }\int _0^1 \frac{d}{d a}\left( \frac{t^{-1/2}}{(1-t)^{1/2}}\,\frac{1}{(a^2-t)^{1/2}}\,\frac{a^{2n}-t^{2n}}{a^{2n}+t^{2n}+2 a^n t^n}\right) dt. \end{aligned} \end{aligned}$$

Hence, for $a > 1$,

$$\begin{aligned} \begin{aligned} K_6(a)- K_4(a)&= - \frac{n}{\pi a} \int _0^1 \frac{d}{d a} \left( \frac{t^{-1/2}}{(1-t)^{1/2}} \, \frac{1}{(a^2-t)^{1/2}} \, \frac{4 (a t)^n}{t^{2n}-a^{2n}} \right) \, d t \\&= -\frac{n}{\pi a} \int _0^1 \frac{4 (ta)^n (a^2(a^{2n}-t^{2n})+n(a^2-t)(a^{2n}+t^{2n}))}{a (1-t)^{1/2} t^{1/2} (a^2-t)^{3/2} (t^{2n}-a^{2n})^2 }\, d t <0, \end{aligned} \end{aligned}$$

because the integrand is positive for $a >1$. Therefore, $K_6(a) < K_4(a)$ for $a > 1$ and statement (b) is proved.

To prove statement (c), we proceed as follows. Note that

$$\begin{aligned} \begin{aligned} K_5&= \lim _{a\rightarrow 1} \frac{1}{2}\sum _{j=1}^n \frac{1}{\big (1+a^2 -2 a \cos \big (\frac{2 \pi j}{n} + \frac{\pi }{n}\big )\big )^{1/2}}:=\lim _{a\rightarrow 1} \frac{1}{2}A_0,\\ K_1&= \lim _{a\rightarrow 1}\frac{1}{2} \sum _{j=1}^{n-1} \frac{1}{\big (1+a^2 -2 a \cos \big (\frac{2 \pi j}{n} \big )\big )^{1/2}} \\&= \lim _{a\rightarrow 1} \frac{1}{2} \! \left( \sum _{j=1}^{n} \frac{1}{\big (1+a^2 -2 a \cos \big (\frac{2 \pi j}{n} \big )\big )^{1/2}}\!-\!\sum _{j=1}^1\frac{1}{(1+a^2-2a\cos (2\pi j))^{1/2}}\!\right) \\&:= \lim _{a\rightarrow 1} \frac{1}{2}(A_1-A_2), \end{aligned} \end{aligned}$$

Thus,

$$\begin{aligned} K_5-K_1=\lim _{a\rightarrow 1} \dfrac{1}{2} (A_0-A_1+A_2) \end{aligned}$$

(22)

where $A_0$, $A_1$, $A_2$ are the summations defined above. Applying Proposition 6, we have that $A_0-A_1+A_2$ when $a\in (0,1)$ is given by

$$\begin{aligned} \begin{aligned} A&= \frac{n}{\pi }\int _0^1 \frac{1-(ta)^n}{(1-t)^{1/2} t^{1/2} (1-a^2t)^{1/2}(1+(ta)^n)} \, dt\\&\quad -\frac{n}{\pi }\int _0^1 \frac{1+(ta)^n}{(1-t)^{1/2} t^{1/2} (1-a^2t)^{1/2}(1-(ta)^n)} \, dt \\&\quad + \frac{1}{\pi } \int _0^1 \frac{1+ta}{(1-t)^{1/2} t^{1/2} (1-a^2t)^{1/2}(1-ta)} \, dt\\&= \frac{n}{\pi }\int _0^1 \left( \frac{4 (ta)^n}{-1+(ta)^{2n}} + \frac{1+ta}{n(1-t a)}\right) \frac{1}{(1-t)^{1/2} t^{1/2} (1-a^2 t)^{1/2}} \, d t . \end{aligned} \end{aligned}$$

On the other hand, applying Proposition 7 we have that $A_0-A_1+A_2$ when $a>1$ is given by

$$\begin{aligned} \begin{aligned} {\overline{A}}&= \frac{n}{\pi }\int _0^1 \left( -\frac{4 (ta)^n}{a^{2n}-t^{2n}} + \frac{a+t}{n(a-t)}\right) \frac{1}{(1-t)^{1/2} t^{1/2} (a^2-t)^{1/2}} \, d t . \end{aligned} \end{aligned}$$

After doing the substitution $a\rightarrow 1/a$, the expression $\overline{A}$ can be written as $a\, A$. Thus,

$$\begin{aligned} \lim _{a\rightarrow 1^-} A=\lim _{a\rightarrow 1^+} \overline{A}=\lim _{a\rightarrow 1} A_0-A_1+A_2. \end{aligned}$$

(23)

Now we show that $A>0$. Note that taking $v=ta$, we get

$$\begin{aligned} \frac{4 (ta)^n}{-1+(ta)^{2n}} + \frac{1+ta}{n(1-t a)}=\frac{4 v^n}{-1+v^{2n}} + \frac{1}{n} \, \frac{1+v}{1-v}. \end{aligned}$$

Using Lemma 2, we get that

$$\begin{aligned} \frac{4 v^n}{-1+v^{2n}} + \frac{1}{n} \, \frac{1+v}{1-v} = \frac{(1+v)(1-v^{2n})-4 n v^n(1-v) }{n (1-v)(1-v^{2n})}> 0, \end{aligned}$$

for $v < 1$. So $A>0$ and taking the limit when $a\rightarrow 1^-$ together with (23) and (22) we get $\lim _{a\rightarrow 1^-}A=\lim _{a\rightarrow 1^+}\overline{A}=2(K_5-K_1)>0$ for all $n\ge 2$. Moreover, $K_1$ is positive by definition. So, we have proved statement (c).

Statement (d) follows from direct computations. $\square$

In the following lemma, we provide properties of the function $\varDelta (a)=-K_1-K_5+a^3(K_2(a)+K_3(a))$ that appears in the denominator $m_D$ in (4).

Lemma 4

For all $n\ge 2$, the following statements hold.

• (a) $\varDelta (a)$ is increasing for all $a \in (0,1)$;

• (b) $\varDelta (0)<0$, $\varDelta (a)\rightarrow \infty$ when $a\rightarrow 1^-$ and $\varDelta (a)\rightarrow -\infty$ when $a\rightarrow 1^+$;

• (c) $\varDelta (a)<0$ for all $a>1$;

• (d) $\varDelta (a)$ has a unique zero and it belongs to the interval (0, 1).

Proof

We first note that setting $b=1/a$ we have

$$\begin{aligned} \begin{aligned} a^3 K_2(a)&= \frac{1}{b} \sum _{j=1}^n \frac{b-\cos \big (\frac{2 \pi j}{n}\big )}{\big (1+b^2 -2 b \cos \big (\frac{2 \pi j}{n}\big )\big )^{3/2}} :=\frac{1}{b} {{\bar{K}}}_2(b) ,\\ a^3 K_3(a)&= \frac{1}{b} \sum _{j=1}^n \frac{b-\cos \big (\frac{2 \pi j}{n}+\frac{\pi }{n}\big )}{\big (1+b^2 -2 b \cos \big (\frac{2 \pi j}{n}+\frac{\pi }{n}\big )\big )^{3/2}} :=\frac{1}{b} {{\bar{K}}}_3(b). \end{aligned} \end{aligned}$$

(24)

Let ${\overline{\varDelta }}(b) =(1/b {{\bar{K}}}_2(b) +1/b {{\bar{K}}}_3(b))$. We want to show that for $a\in (0,1)$

$$\begin{aligned} \varDelta '(a)=\left. \frac{d {\overline{\varDelta }}(b)}{db}\right| _{b=1/a}\cdot \frac{db}{da}=\left. ({\overline{\varDelta }}(b))'\right| _{b=1/a}\cdot \left( -\frac{1}{a^2}\right) >0. \end{aligned}$$

So, it is sufficient to show that $\varDelta (b)'<0$ for $b>1$.

Let

$$\begin{aligned} T=\sum _{j=1}^n\frac{1}{\big (1+b^2 -2 b \cos \big (\frac{2 \pi j}{n}\big )\big )^{1/2}}+\sum _{j=1}^n \frac{1}{\big (1+b^2 -2 b \cos \big (\frac{2 \pi j}{n}+\frac{\pi }{n}\big )\big )^{1/2}}. \end{aligned}$$

Using Lemma 1(c), we get that

$$\begin{aligned} {{\bar{K}}}_2(b)+{{\bar{K}}}_3(b)=-dT/db \end{aligned}$$

(25)

and so $({\bar{\varDelta }}(b))' =\frac{1}{b^2} T_1 -\frac{1}{b} T_2$ where

$$\begin{aligned} T_1= \frac{d T}{db}, \quad T_2=\frac{d^2 T}{db^2}. \end{aligned}$$

Since from Proposition 7, we have

$$\begin{aligned} \begin{aligned} T_1&=- \frac{2n}{\pi }\int _0^1 \frac{d}{db} \left( \frac{1}{t^{1/2} (1-t)^{1/2} (b^2 -t)^{1/2}} \, \frac{t^{2n}+b^{2n}}{t^{2n}-b^{2n}}\right) \, d t \ \\&= \frac{n}{\pi }\int _0^1 \frac{1}{t^{1/2} (1-t)^{1/2}} \left( \!\frac{2 b (t^{2n}+b^{2n})}{(b^2-t)^{3/2} (t^{2n}-b^{2n})}\! - \!\frac{8 n t^{2n} b^{2n-1}}{(b^2-t)^{1/2} (t^{2n}-b^{2n})^2}\! \right) d t , \end{aligned} \end{aligned}$$

taking another derivative with respect to b we get

$$\begin{aligned} \begin{aligned} T_2&= \frac{n}{\pi }\int _0^1 \frac{1}{t^{1/2} (1-t)^{1/2}} \left( \frac{-8 n t^{2n} b^{2n-2}((2n-1)t^{2n} + (2n+1)b^{2n})}{(b^2-t)^{1/2} (t^{2n}-b^{2n})^3} \right. \\&\quad \left. +\frac{2 \left( 8 n b^{2 n} \left( b^2-t\right) t^{2 n}+\left( 2 b^2+t\right) \left( b^{4 n}-t^{4 n}\right) \right) }{\left( b^2-t\right) ^{5/2} \left( t^{2 n}-b^{2 n}\right) ^2}\right) \, d t. \end{aligned} \end{aligned}$$

Since $T_1 < 0$ (the integrand is negative) and $T_2 >0$ (the integrand is positive), we readily obtain that $({{\bar{\varDelta }}}(b))' <0$ and so $\varDelta '(a) >0$ for $a \in (0,1)$. In short, statement (a) is proved.

It is clear that $\varDelta (0)=-K_1-K_5<0$, see Lemma 3(c). Moreover,

$$\begin{aligned} \lim _{a \rightarrow 1} K_2(a)= \sum _{j=1}^{n-1} \frac{1-\cos \big (\frac{2 \pi j}{n}\big )}{\big (2 -2 \cos \big (\frac{2 \pi j}{n}\big )\big )^{3/2}} + \lim _{a \rightarrow 1} \frac{1-a}{\big (1+a^2 -2 a \big )^{3/2}}, \end{aligned}$$

so

$$\begin{aligned} \lim _{a \rightarrow 1^-} K_2(a)=\infty \quad \text {and} \quad \lim _{a \rightarrow 1^+} K_2(a)=-\infty . \end{aligned}$$

(26)

Furthermore, $\lim _{a\rightarrow 1} K_3(a)=K_5$ which is different from zero and from infinity. Hence, using (26) we get

$$\begin{aligned} \lim _{a \rightarrow 1^-} \varDelta (a)=\infty \quad \text {and} \quad \lim _{a \rightarrow 1^+} \varDelta (a)=-\infty . \end{aligned}$$

This completes the proof of statement (b).

Now we show that $\varDelta (a) < 0$ for $a > 1$. To do so, we will show that $a^3 K_2(a) +a^3 K_3(a)< 0$. Note that this is sufficient because $-K_1 -K_5 < 0$. Clearly in view of (24) and (25), we have $a^3 K_2(a) + a^3 K_3(a) = \frac{1}{b} ({{\bar{K}}}_2(b) + {{\bar{K}}}_3(b))=-\frac{1}{b}\frac{dT}{db}$ with $b < 1$. So, applying Proposition 6 we get

$$\begin{aligned} \begin{aligned} -\frac{1}{b}\dfrac{dT}{db}&=-\frac{2 n }{\pi b} \int _0^1 \frac{d}{d b} \left( \frac{1}{t^{1/2} (1-t)^{1/2} (1-b^2 t )^{1/2}} \, \frac{1+(bt)^{2n}}{1-(bt)^{2n}}\right) \, d t \\&=-\frac{2 n }{\pi b} \int _0^1 \left( \frac{4 n (t b)^{2n}}{b\, t^{1/2} (1-t)^{1/2} (1-b^2 t )^{1/2}(1-(bt)^{2n})^2}\right. \\&\quad \left. +\frac{ bt(1+(bt)^{2n})}{t^{1/2} (1-t)^{1/2} (1-b^2 t )^{3/2}(1-(bt)^{2n})} \right) \, d t \end{aligned} \end{aligned}$$

and since the integrand is positive we readily have that $a^3 K_2(a) + a^3 K_3(a)< 0$ for $a > 1$ and so $\varDelta (a) < 0$ for $a > 1$. In short, statement (c) is proved.

The proof of statement (d) is a direct consequence of the statements (a)–(c) together with the Bolzano–Cauchy theorem. $\square$

Proof of Proposition 1

We expand $m_2=a^3 m_{N,2}/{m_{D}}$ in Laurent series around $a=0$, see (4). The expansion of $m_D$ around $a=0$ is given by

$$\begin{aligned} m_{D} = (K_5-K_1)(-K_1-K_5 + O(a^3)). \end{aligned}$$

Using Lemma 1(d) with $u=0$ (respectively $u=\pi /n$) to expand $K_4$ (respectively $K_6$) in Laurent series around $a=0$, we get

$$\begin{aligned} K_4(a)=-\frac{n}{2}+O(a) \qquad \text{ and } \qquad K_6(a)=-\frac{n}{2}+O(a) \end{aligned}$$

(27)

when $n\ge 3$ and

$$\begin{aligned} K_4(a)=-4+O(a) \qquad \text{ and } \qquad K_6(a)=2+O(a) \end{aligned}$$

(28)

when $n=2$. Therefore, when $n\ge 3$ we have

$$\begin{aligned} m_2= a^3 \frac{K_1^2 + \frac{n}{2} K_1 -K_ 1 K_5 -\frac{n}{2} K_5}{(K_5-K_1)(-K_1-K_5)} + O(a^4) = a^3 \frac{K_1+ \frac{n}{2} }{K_5+K_1} + O(a^4) \end{aligned}$$

and since $K_1,K_5 > 0$, we obtain $\lim _{a \rightarrow 0^+} m_2 = 0^+$.

When $n=2$, we compute directly the quantities $K_1$ and $K_5$ and we have $K_1=1/4$, $K_5=1/\sqrt{2}$. So expanding $m_3$ in Laurent series around $a=0$, we get

$$\begin{aligned} m_2=- a^3 \Big (\frac{17}{7} + 2\sqrt{2}\Big ) + O(a^4). \end{aligned}$$

Hence, $\lim _{a \rightarrow 0^+} m_2 = 0^-$. This completes the proof of statement (a).

For statement (b), we note that by (5)

$$\begin{aligned} m_3=m_2 + \frac{a^3(K_6(a)-K_4(a))}{K_5-K_1}. \end{aligned}$$

Using the Laurent series of $K_4$ and $K_6$ around $a=0$ given in (27), we get

$$\begin{aligned} \frac{a^3(K_6-K_4(a))}{K_5-K_1} =O(a^4) \end{aligned}$$

when $n\ge 3$ and so

$$\begin{aligned} m_3= a^3 \frac{K_1+ \frac{n}{2} }{K_5+K_1} + O(a^4), \end{aligned}$$

which yields $\lim _{a \rightarrow 0^+} m_3 = 0^+$ when $n\ge 3$.

On the other hand, for $n=2$, using the Laurent series of $K_4$ and $K_6$ around $a=0$ given in (28) and the values of $K_1$ and $K_5$ for $n=2$ computed above, we get

$$\begin{aligned} \frac{a^3(K_6-K_4(a))}{K_5-K_1} =\frac{a^3}{K_5-K_1} (6 + O(a)) \end{aligned}$$

which yields

$$\begin{aligned} m_3= \frac{a^3}{7} (7+34 \sqrt{2}) + O(a^4), \end{aligned}$$

and so $\lim _{a \rightarrow 0^+} m_3 = 0^+.$ This completes the proof of statement (b).

We expand $m_3=a^3 m_{N,3}/m_{D}$ in Laurent series around $a=1$ (with $a > 1$), see (4). Note that

$$\begin{aligned} \begin{aligned} K_2(a)&= K_1 - \frac{1}{(a-1)^{2}}+O(a-1), \quad K_3(a)=K_5 + O(a-1), \\ K_4(a)&=K_1 + \frac{1}{(a-1)^{2}}-\frac{1}{a-1}+1+O(a-1), \quad K_6 =K_5 + O(a-1), \end{aligned} \end{aligned}$$

and $a^3=1+O(a-1)$. Hence,

$$\begin{aligned} m_{N,3} =\frac{1}{(a-1)^4} + O((a-1)^{-3}) \quad \text {and} \quad m_{D} =- \frac{ (K_5-K_1)}{(a-1)^{2}} + O((a-1)^{-1}). \end{aligned}$$

Therefore,

$$\begin{aligned} m_3= -\frac{1}{(a-1)^{2}(K_5-K_1)} + O((a-1)^{-1}). \end{aligned}$$

Since in view of Lemma 3(c) we have $K_5>K_1>0$, then $\lim _{a \rightarrow 1^+} m_3 = -\infty$, which completes the proof of statement (c).

For statement (d), note that taking $b=1/a$ and using Lemma 3(d) we have $K_2(a)=K_2(1/b)=b^3K_4(b)$, $K_3(a)=K_3(1/b)=b^3K_6(b)$, $K_4(a)=K_2(1/a)/a^3=b^3K_2(b)$ and $K_6(a)=K_3(1/a)/a^3=b^3K_3(b)$. Thus, using Lemma  1(d) for $n\ge 3$ we have

$$\begin{aligned} K_4(b)=-\frac{n}{2}+O(b), \quad K_6(b)=-\frac{n}{2}+O(b). \end{aligned}$$

Moreover, expanding in power series around $b=0$, we get

$$\begin{aligned} K_2(b)=n+O(b), \quad K_3(b)=n+O(b). \end{aligned}$$

Hence for $n\ge 3$

$$\begin{aligned} \begin{aligned} K_2(b)&=-\frac{n}{2} b^3+ O(b^4), \qquad K_3(b)=-\frac{n}{2} b^3+ O(b^4),\\ K_4(b)&=nb^3 + O(b^4), \qquad K_6(b)=nb^3+O(b^4), \end{aligned} \end{aligned}$$

(29)

Proceeding in the same way for $n=2$, we get

$$\begin{aligned} \begin{aligned} K_2(b)&=-4b^3+ O(b^4), \qquad K_3(b)=2b^3+ O(b^4),\\ K_4(b)&=2b^3 + O(b^4), \qquad K_6(b)=2b^3+O(b^4). \end{aligned} \end{aligned}$$

(30)

Using (29) and (30), the numerators of $m_2$ and $m_3$ (see (4)) can be written as

$$\begin{aligned} \frac{1}{b^3}(K_1^2-K_1K_5)+O(1), \end{aligned}$$

and the denominators of $m_2$ and $m_3$ (see again (4)) can be written as

$$\begin{aligned} (K_5-K_1)(-K_1-K_5-n)+O(b) \end{aligned}$$

for all $n\ge 2$. Thus, the Laurent expansion of $m_2$ and $m_3$ around $b=0$ becomes (after simplifying $K_5-K_1$)

$$\begin{aligned} \frac{1}{b^3} \frac{K_1}{K_1+K_5+n}+O(b^{-2}). \end{aligned}$$

Then taking into account that in view of Lemma 3(c) we have $K_5>K_1>0$, we conclude that

$$\begin{aligned} \lim _{a\rightarrow \infty } m_2=\lim _{b\rightarrow 0^+} m_2=\infty , \qquad \lim _{a\rightarrow \infty } m_3=\lim _{b\rightarrow 0^+} m_3=\infty . \end{aligned}$$

This proves statement (d).

From Lemma 3(a), we get $K_6-K_4(a)>0$ for $a\in (0,1)$. From Lemma 3(b), we get $K_6-K_4(a)<0$ for $a>1$, and from Lemma 3(c), we get $K_5-K_1>0$. Thus from (5) we get $m_2<m_3$ when $a\in (0,1)$ and $m_2>m_3$ when $a>1$, which proves statement (e). $\square$

Appendix 2: Proof of Proposition 3

We need the following auxiliary lemma.

Lemma 5

Let

$$\begin{aligned} E(a):=\sum _{j=0}^{[n/2]-1} \frac{-2 (1+a^2)\cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )+5 a-a \cos \big (\frac{4\pi \,j}{n}+ \frac{2\pi }{n} \big )}{a\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )\Big )^{5/2}}. \end{aligned}$$

Then

$$\begin{aligned} \begin{aligned}&\lim _{\beta \rightarrow \pi /n} N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta )) =\frac{1}{4} E(a) \end{aligned} \end{aligned}$$

if n is even and

$$\begin{aligned} \begin{aligned}&\lim _{\beta \rightarrow \pi /n} N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta )) =\frac{1}{4} \left( E(a)+\frac{1}{a(1+a)^3}\right) \end{aligned} \end{aligned}$$

if n is odd.

Proof

First note that

$$\begin{aligned} \lim _{\beta \rightarrow \pi /n} N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta )) =0 \end{aligned}$$

because

$$\begin{aligned} \lim _{\beta \rightarrow \pi /n} N_4(a,\beta ) = \sum _{j=1}^n \frac{-1/a \sin \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big ) }{\Big (1+a^2 -2 a\cos \big (\frac{2\pi \,j}{n}+\frac{\pi }{n}\big )\Big )^{3/2}}=0 \end{aligned}$$

and

$$\begin{aligned} \lim _{\beta \rightarrow \pi /n} L_2(a,\beta ) = \sum _{j=1}^n \frac{1-a \cos \big (\frac{2\pi \,j}{n}-\frac{\pi }{n}\big ) }{\Big (1+a^2 -2 a\cos \big (\frac{2\pi \,j}{n}-\frac{\pi }{n}\big )\Big )^{3/2}} \end{aligned}$$

is finite for all $a>0$. On the other hand,

$$\begin{aligned} \begin{aligned} L_5(\beta )&=\left( \sum _{j=0}^{n-2} \frac{1- \cos \big (\frac{2\pi \,j}{n}+2 \beta \big ) }{\Big (2 -2 \cos \big (\frac{2\pi \,j}{n}+2 \beta \big )\Big )^{3/2}}\right) + \frac{1-\cos \big (\frac{2\pi \,(n-1)}{n}+2 \beta \big ) }{\Big (2 -2 \cos \big (\frac{2\pi \,(n-1)}{n}+2 \beta \big )\Big )^{3/2}} \\&:= L_{5,1}(\beta ) + L_{5,2}(\beta ). \end{aligned} \end{aligned}$$

Clearly $\lim _{\beta \rightarrow \pi /n} N_4(a,\beta ) L_{5,1}(\beta )=0$. Therefore, we need to study $\lim _{\beta \rightarrow \pi /n} N_4(a,\beta ) L_{5,2}(\beta )$. Note that expanding $L_{5,2}$ around $\beta =\pi /n$ we have

$$\begin{aligned} L_{5,2}(\beta ) = \frac{1-\cos \big (\frac{2\pi (n-1)}{n}+2 \beta \big ) }{\Big (2 -2 \cos \big (\frac{2\pi (n-1)}{n}+2 \beta \big )\Big )^{3/2}} = \frac{1}{4 \big (\beta -\frac{\pi }{n}\big )} + \frac{1}{24} \big (\beta -\frac{\pi }{n}\big ) + O\Big (\big (\beta -\frac{\pi }{n}\big )^2\Big ). \end{aligned}$$

On the other hand, we can rewrite $N_4(a,\beta )$ as

$$\begin{aligned} \begin{aligned} {\bar{N}}_4(a,\beta )&=\sum _{j=0}^{[n/2]-1} \left( \frac{-1/a \sin \big (\frac{2\pi \,j}{n}+ \beta \big ) }{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+ \beta \big )\Big )^{3/2}}\right. \\&\left. \quad -\frac{1/a \sin \big (\frac{2\pi \,(-j-1)}{n}+ \beta \big ) }{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,(-j-1)}{n}+ \beta \big )\Big )^{3/2}}\right) \end{aligned} \end{aligned}$$

if n is even and as ${\bar{N}}_4(a,\beta )+{\bar{N}}_4^*(a,\beta )$ with

$$\begin{aligned} {\bar{N}}_4^*(a,\beta )= \frac{-1/a \sin \big (\frac{2\pi \,[n/2]}{n}+ \beta \big ) }{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,[n/2]}{n}+ \beta \big )\Big )^{3/2}} \end{aligned}$$

if n is odd. Expanding ${\bar{N}}_4$ around $\beta =\pi /n$, we get

$$\begin{aligned} {\bar{N}}_4(a,\beta ) =E(a) (\beta -\pi /n) + O((\beta -\pi /n)^2), \end{aligned}$$

and expanding ${\bar{N}}_4^*$ also around $\beta =\pi /n$ we get

$$\begin{aligned} {\bar{N}}_4^*(a,\beta )=\frac{1}{a(1+a)^3}(\beta -\pi /n)+ O((\beta -\pi /n)^3). \end{aligned}$$

Thus, expanding $N_4(a,\beta ) L_{5,2}(\beta )$ around $\beta =\pi /n$ we obtain

$$\begin{aligned} N_4(a,\beta ) L_{5,2}(\beta ) = \frac{1}{4} E(a) + O((\beta -\pi /n)) \end{aligned}$$

if n is even and

$$\begin{aligned} N_4(a,\beta ) L_{5,2}(\beta ) = \frac{1}{4} \left( E(a)+\frac{1}{a(1+a)^3}\right) + O((\beta -\pi /n)) \end{aligned}$$

if n is odd. Taking the limit as $\beta \rightarrow \pi /n$ we obtain the result that we wanted to prove. $\square$

Proof of Proposition 3

Note that

$$\begin{aligned} \begin{aligned} \lim _{\beta \rightarrow \pi /n}N_5(\beta )=&\sum _{j=1,j\ne n-1}^n\frac{-\sin \big (\frac{2\pi \,(j+1)}{n}\big )}{\Big (2-2\cos \big (\frac{2\pi \,(j+1)}{n}\big )\Big )^{3/2}}\\&+\lim _{\beta \rightarrow \pi /n} \frac{-\sin \big (\frac{2\pi \,(n-1)}{n}+2\beta \big )}{\Big (2-2\cos \big (\frac{2\pi \,(n-1)}{n}+2\beta \big )\Big )^{3/2}}=\infty . \end{aligned} \end{aligned}$$

In view of Lemma 5, it is clear that the sign of $F(a,\beta )$ as $\beta \rightarrow \pi /n$ is determined by the sign of the difference G given by

$$\begin{aligned} G=\lim _{\beta \rightarrow \pi /n} (K_1-L_4(a,\beta ))= K_1 - {\bar{L}}_4(a), \end{aligned}$$

where

$$\begin{aligned} {\bar{L}}_4(a)=\lim _{\beta \rightarrow \pi /n} L_4(a,\beta )= \sum _{j=1}^{n} \frac{1-1/a \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )}{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )\Big )^{3/2}}. \end{aligned}$$

When $a \rightarrow \infty$, we have

$$\begin{aligned} \lim _{a \rightarrow \infty } G= K_1 -\lim _{a \rightarrow \infty }{\bar{L}}_4(a) =K_1 > 0. \end{aligned}$$

This proves statement (a) of the proposition.

Furthermore, when $a \rightarrow 1$, using Lemma 3(c), we obtain

$$\begin{aligned} \lim _{a \rightarrow 1} G = K_1 - \sum _{j=1}^{n} \frac{1- \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )}{\Big (2-2 \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )\Big )^{3/2}} =K_1 -K_5 < 0. \end{aligned}$$

So, statement (b) is proved.

Using Lemma 1(d) with $u =\pi /n$, the expansion of ${\bar{L}}_4$ around $a=0$ is given by ${\bar{L}}_4(a)= -n/2+ O(a),$ when $n\ge 3$ and ${\bar{L}}_4(a)= 2 + O(a),$ when $n=2$. Hence, when $n\ge 3$ the expansion of G around $a=0$ is $G=K_1 +n/2 + O(a)$ and so $\lim _{a \rightarrow 0} G >0.$ For $n=2$, computing the value of $K_1$, the expansion of G around $a=0$ is $G=1/4 -2 + O(a)$ and so $\lim _{a \rightarrow 0} G =-7/4<0.$ This yields statement (c) of the proposition. $\square$

Appendix 3: Proof of Propositions 4 and 5

We need the following auxiliary lemma.

Lemma 6

We have

$$\begin{aligned} \lim _{\beta \rightarrow \pi /2n} N_5(\beta ) (K_1-L_4(a,\beta ))=0. \end{aligned}$$

Proof

First note that

$$\begin{aligned} \lim _{\beta \rightarrow \pi /2n} L_4(a,\beta )= \sum _{j=1}^{n} \frac{1-1/a \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{2n} \big )}{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{2n} \big )\Big )^{3/2}}, \end{aligned}$$

which is finite for all $a>0$. Moreover, it is easy to see that

$$\begin{aligned} \lim _{\beta \rightarrow \pi /2n}N_5(\beta )= \sum _{j=1}^{n} \frac{- \sin \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )}{\Big (2 -2 \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )\Big )^{3/2}} =0 \end{aligned}$$

and so $\lim _{\beta \rightarrow \pi /2n} N_5(\beta )(K_1-L_4(a,\beta ))=0,$ as we wanted to prove. $\square$

Proof of Proposition 4

In view of Lemma 6 the sign of $F(a,\beta )$ around $\beta =\pi /2n$ is determined by the sign of $N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta ))$ unless $\lim _{\beta \rightarrow \pi /2n} N_4(a,\beta )(K_1-2a^3L_2(a,\beta )+L_5(\beta ))= 0$. From the analysis of the sign of $N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta ))$ around $\beta \rightarrow \pi /2n$ we will see that $\lim _{\beta \rightarrow \pi /2n} N_4(a,\beta )(K_1-2a^3L_2(a,\beta )+L_5(\beta ))\ne 0$.

In view of Proposition 2, we have that $N_4(a,\beta ) < 0$ for $\alpha \in (0,\pi /n)$ and so $N_4(a,\beta ) < 0$. So, we need to study the sign of $K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta )$ as $\beta \rightarrow \pi /2n$.

Hence,

$$\begin{aligned} \lim _{\beta \rightarrow \pi /2n} K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta ) =K_1-2a^3{\bar{L}}_2(a)+ K_5:=H, \end{aligned}$$

where

$$\begin{aligned} {\bar{L}}_2(a)=\lim _{\beta \rightarrow \pi /2n} L_2(a,\beta ) =\sum _{j=1}^n\frac{1-a\cos \big (\frac{2\pi \,j}{n}-\frac{\pi }{2n}\big )}{\Big (1+a^2-2a\cos \big (\frac{2\pi \,j}{n}-\frac{\pi }{2n}\big )\Big )^{3/2}}. \end{aligned}$$

When $a\rightarrow 0$, we have that ${\bar{L}}_2(a) \rightarrow n$. So $\lim _{a \rightarrow 0}H = K_1 +K_5 > 0$ in view of Lemma 3(c). This proves statement (a) of the proposition.

To study the behavior when $a \rightarrow \infty$, we first observe that making the change $b=1/a$ we get $a^3{\bar{L}}_2(a)=L(b,\pi /2n)$ where L is the function defined in Lemma 1(d). Thus, applying Lemma 1(d) with $u=\pi /2n$ and using Lemma 3(c) we have

$$\begin{aligned} \lim _{a \rightarrow \infty } H = K_1 +n + K_5 >0, \end{aligned}$$

when $n\ge 3$ and

$$\begin{aligned} \lim _{a \rightarrow \infty } H = K_1 -2\big (2-6\cos ^2\big (\tfrac{\pi }{4}\big )\big ) + K_5= K_1 +2 + K_5 >0, \end{aligned}$$

when $n=2$. This proves statement (b).

Finally, to prove statement (c) we need to study the sing of H when $a \rightarrow 1$. Note that

$$\begin{aligned} \begin{aligned} \lim _{a \rightarrow 1} H&= \frac{1}{2} \sum _{j=1}^{n-1} \frac{1}{\Big (2 -2 \cos \big (\frac{2\pi \,j}{n} \big )\Big )^{1/2}} -\sum _{j=1}^n \frac{1}{\Big (2 -2 \cos \big (\frac{2\pi \,j}{n}- \frac{\pi }{2n} \big )\Big )^{1/2}} \\&\quad + \frac{1}{2} \sum _{j=1}^n \frac{1}{\Big (2 -2 \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )\Big )^{1/2}}. \end{aligned} \end{aligned}$$

In order to study the sign of $\lim _{a \rightarrow 1} H$, we rewrite $\lim _{a \rightarrow 1} H$ as $\lim _{a\rightarrow 1} H_1$ where

$$\begin{aligned} \begin{aligned} H_1&= \frac{1}{2} \sum _{j=1}^{n} \frac{1}{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n} \big )\Big )^{1/2}} - \frac{1}{2} \sum _{j=1}^1\frac{1}{(1+a^2 -2 a\cos (2\pi j ) )^{1/2} } \\&\quad -\sum _{j=1}^n \frac{1}{\Big (1+a^2 -2a \cos \big (\frac{2\pi \,j}{n}- \frac{\pi }{2n} \big )\Big )^{1/2}} + \frac{1}{2} \sum _{j=1}^n \frac{1}{\Big (1+a^2 -2 a \cos \big (\frac{2\pi \,j}{n}+ \frac{\pi }{n} \big )\Big )^{1/2}}. \end{aligned} \end{aligned}$$

Now applying Proposition 6 with $\alpha =1/2$ and $u=0$; $n=1$, $\alpha =1/2$ and $u=0$; $\alpha =1/2$ and $u=-\pi /2n$; and $\alpha =1/2$ and $u=\pi /n$, respectively, we get

$$\begin{aligned} \begin{aligned} H_1&= \frac{1}{\pi } \int _0^1 \frac{1}{t^{1/2} (1-t)^{1/2} (1-a^2 t)^{1/2}}\left( \frac{4(at)^{2n}n}{1-(at)^{4n}}-\frac{1+at}{2(1-at)}\right) \, d t \end{aligned} \end{aligned}$$

Setting $v=at$ and $N=2n$ and using Lemma 2, we get

$$\begin{aligned} \begin{aligned} \frac{4(at)^{2n}n}{1-(at)^{4n}}-\frac{1+at}{2(1-at)}&=\frac{4v^{N}\frac{N}{2}}{1-v^{2N}}-\frac{1+v}{2(1-v)}\\&= \frac{4v^{N}N (1-v)-(1+v)( 1-v^{2N}) }{(1-v^{2N})2(1-v)}< 0. \end{aligned} \end{aligned}$$

Therefore $\lim _{a\rightarrow 1^-} H_1<0$.

Applying Proposition 7 to $H_1$ with $\alpha =1/2$ and $u=0$; $n=1$, $\alpha =1/2$ and $u=0$; $\alpha =1/2$ and $u=-\pi /2n$; and $\alpha =1/2$ and $u=\pi /n$, respectively, we get

$$\begin{aligned} \begin{aligned} {\bar{H}}_1&= \frac{1}{\pi } \int _0^1 \frac{1}{t^{1/2} (1-t)^{1/2} (a^2-t)^{1/2}}\left( \frac{4(at)^{2n}n}{a^{4n}-t^{4n}}-\frac{a+t}{2(a-t)}\right) \, d t. \end{aligned} \end{aligned}$$

After doing the substitution $a\rightarrow 1/a$, we get that ${\bar{H}}_1$ can be written as $a\, H_1$. Thus, $\lim _{a\rightarrow 1^+} H_1<0$. In short, we have that $H < 0$ when $a\rightarrow 1$ and statement (c) is proved. $\square$

Proof of Proposition 5

We start proving statement (a) for $n=2$. Computing directly the quantities $K_1$, $L_2(a,\beta )$, $L_4(a,\beta )$, $N_4(a,\beta )$, $L_5(\beta )$, and $N_5(\beta )$ for a fixed $\beta$ and expanding F around $a=0$ we get

$$\begin{aligned} F(a,\beta )=-\frac{1}{16} \tan \beta \sec \beta \left( 18 \cos \beta +6 \cos (3 \beta )+17 \cot ^3\beta +7\right) +O(a^2). \end{aligned}$$

So ${\bar{F}}(\beta )<0$ for all $\beta \in (\pi /2n,\pi /n)$ and $n=2$.

Now we will show that for any $\beta \in (\pi /2n,\pi /n)$ and $n\ge 3$ we have ${\bar{F}}(\beta ) >0$. We fix $\beta$ and we expand F around $a=0$. First, expanding $N_4$ around $a=0$, we get

$$\begin{aligned} N_4(a,\beta )= - \frac{1}{a} \sum _{j=1}^n \sin \big (\frac{2\pi \,j}{n}+\beta \big ) - \frac{3}{2} \sum _{j=1}^n \sin \big (\frac{4\pi \,j}{n}+2\beta \big ) + O(a) \end{aligned}$$

and in view of (18) we have $N_4(a,\beta )=O(a)$. So expanding $N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta ))$ around $a=0$ we obtain

$$\begin{aligned} N_4(a,\beta )(K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta )) =O(a). \end{aligned}$$

On the other hand, using Lemma 1(d) with $u=\beta$ and $n\ge 3$, the expansion of $L_4$ around $a=0$ is given by $L_4(a,\beta )=-n/2+O(a)$. Therefore, around $a=0$ we get

$$\begin{aligned} F(a,\beta )= (K_1 + \frac{n}{2}) N_5(\beta ) + O(a). \end{aligned}$$

(31)

The sign of $N_5(\beta )$ for any $\beta \in (0,\pi /n)$ was studied in Proposition 2, and we obtained that $N_5(\beta )$ is negative if $\beta \in (0,\pi /2n)$ and positive if $\beta \in (\pi /2n,\pi /n)$. Therefore, from (31) we have that for any $\beta \in (\pi /2n,\pi /n)$ and $n\ge 3$, ${\bar{F}}(\beta )>0$ which completes the proof of statement (a).

Now we consider the case in which $a \rightarrow \infty$. Fixed $\beta \in (\pi /2n,\pi /n)$ we have that $N_5(\beta )$ is positive. Moreover,

$$\begin{aligned} \lim _{a \rightarrow \infty } L_4(a,\beta )= \lim _{a \rightarrow \infty } N_4(a,\beta )=0. \end{aligned}$$

Making the change $b=1/a$ we get $a^3L_2(a,\beta )=L(b,\beta )$, where L is the function defined in Lemma 1(d). Thus, applying Lemma 1(d) with $u=\beta$, we get that $\lim _{a\rightarrow \infty } a^3 L_2(a,\beta )=\lim _{b\rightarrow 0}-n/2+O(b)$ when $n\ge 3$ and $\lim _{a\rightarrow \infty } a^3 L_2(a,\beta )=\lim _{b\rightarrow 0}2-6\cos ^2(\beta )+O(b)$ when $n=2$. So,

$$\begin{aligned} \begin{aligned}&\lim _{a \rightarrow \infty } (K_1 -2 a^3 L_2(a,\beta ) + L_5(\beta ))N_4(a,\beta )=0 , \\&\quad \lim _{a \rightarrow \infty } N_5(\beta )(K_1-L_4(a,\beta )) = N_5(\beta ) K_1 >0, \end{aligned} \end{aligned}$$

for all $n\ge 2$. In short, for any $\beta \in (\pi /2n,\pi )$ and $n\ge 2$, ${\tilde{F}}(\beta )>0$. This concludes the proof of the proposition. $\square$