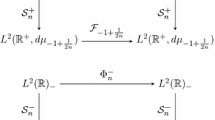Abstract
We indicate how recent results of Grafakos and Teschl (J Fourier Anal Appl 19:167–179, 2013), and Estrada (J Fourier Anal Appl 20:301–320, 2014), relating the Fourier transform of a radial function in \(\mathbb R^n\) and the Fourier transform of the same function in \(\mathbb R^{n+2}\) and \(\mathbb R^{n+1}\), respectively, are located within known results on transplantation for Hankel transforms.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Working with Fourier transforms of radial functions in \(\mathbb R^n\) indispensably leads to Hankel transforms. Indeed, it is well known that the Fourier transform of a radial function in \(\mathbb {R}^n\), \(n \ge 1\), reduces directly to the modified Hankel transform \(H_{\mu }\) of order \(\mu =n/2-1\). Moreover, the radial part of the standard Laplacian in \(\mathbb {R}^n\) is the Bessel operator \(L_\mu =\frac{d^2}{dx^2} + \frac{2\mu +1}{x}\frac{d}{dx}\), \(\mu =n/2-1\), which is the natural ‘Laplacian’ in harmonic analysis associated with \(H_{\mu }\). More precisely, if \(f\) is a radial function on \(\mathbb {R}^n\), \(f(x) = f_0(|x|)\), then \(\mathcal F_nf(x) =H_\mu f_0(|x|)\) and \(\Delta _nf(x)= (L_{\mu }f_0)(|x|)\). Here \(\mathcal F_n\) denotes the Fourier transform on \(\mathbb {R}^n\),
\(\Delta _n=\sum _1^n \partial ^2_j\) is the Laplacian in \(\mathbb {R}^n\), and \(H_\mu \) denotes the modified Hankel transform of order \(\mu \ge -1/2\), the integral transform given by
defined for appropriate functions on \(\mathbb R_+=(0,\infty )\); \(J_\mu \) denotes here the Bessel function of the first kind of order \(\mu \). The Hankel transform possesses the well known properties: \((H_\mu \circ H_\mu )g=g\), and \(\Vert H_\mu g\Vert _{L^2(\mathbb R_+,\,m_\mu )}=\Vert g\Vert _{L^2(\mathbb R_+,\,m_\mu )}\), both identities on appropriate subclass of functions, say, for \(g\in \mathcal S(\mathbb R_+)\), the space of restrictions to \(\mathbb R_+\) of even Schwartz functions on \(\mathbb R\). It is known that \(H_\mu \) is a continuous bijection of \(\mathcal S(\mathbb R_+)\), see e.g. [3], and extends to an isometric isomorphism on \(L^2(\mathbb R_+,\,m_\mu )\).
Now, given a function \(g\in \mathcal S(\mathbb R_+)\), denote \(g_\mu :=H_\mu g\). Then, for \(\mu ,\nu \ge -1/2\),
where \(T^\mu _{\nu }:=H_\mu \circ H_\nu \) is the transplantation operator, see [5, p. 56], which is well defined on \(\mathcal S(\mathbb R_+)\). (A comment: this transplantation operator does not fit into the framework of [4].) Its exact form is known: for \(\nu >\mu \ge -1/2\),
see [5, (3.9)], where \(c_{\nu ,\mu }\) is a constant independent on \(g\) (the proof is based on an integral formula expressing \(J_\mu \) through \(J_\nu \)). In particular,
which immediately shows that \(\frac{d}{dx}g_{\mu }(x)=-c_{\mu +1,\,\mu }\,xg_{\mu +1}(x)\), and this, when specified to \(\mu =n/2-1\), is the formula [2, Theorem 1.1 (1)].
Similarly,
and this, when specified to \(\mu =n/2-1\), is [1, (1.3)], one of the main results of [1]. Iterating this formula \(k\) times and then integrating in polar coordinates brings
which, when specified to \(\mu \!=\!n/2-1\), is [1, (1.4)]; here \(C_{\mu ,\,k}\!=\!\prod _{j=1}^k c_{\mu +j/2,\,\mu +(j-1)/2}\) and \(\omega _{k-1}\) is the surface area of the unit sphere \(S^{k-1}\) in \(\mathbb R^k\).
We remark that the exact form of \(T^\mu _\nu \) is also known when \(\mu >\nu \ge -1/2\):
where \(L_\nu ^\delta \) denotes the \(\delta \)-fractional power of \(L_\nu \) (or rather its natural self-adjoint extension) given spectrally on \(\mathrm{Dom}(L_\nu ^\delta )=\{f\in L^2:(\cdot )^{2\delta }H_\nu f\in L^2\}\) by \(H_\nu (L_\nu ^\delta f)(y)=y^{2\delta }H_\nu f(y)\), see [5, p. 58]. In particular,
Finally, we mention that to keep this note compact we did not specify the exact values of the constants involved. Nevertheless, an inspection shows that the constants appearing in the relevant formulas above are consistent (after taking into account different normalizations of Fourier transforms) with those in [1, (1.2), (1.3), (1.4)]. Perhaps the simplest way to see this consistency is to employ a concrete \(g\), say \(g(x)=\exp (-x^2)\) (it is known that \(g_\mu (x)=2^{-\mu -1}\exp (-x^2/4)\)). Also, to be concise we assumed \(g\in \mathcal S(\mathbb R_+)\), but simple density arguments allow to enlarge applicability of the relevant formulas to more general classes of functions.
References
Estrada, R.: On radial functions and distributions and their Fourier transforms. J. Fourier Anal. Appl. 20, 301–320 (2014)
Grafakos, L., Teschl, G.: On Fourier transforms of radial functions and distributions. J. Fourier Anal. Appl. 19, 167–179 (2013)
Stempak, K.: A note on Zemanian spaces. Extracta Math. 12, 33–40 (1997)
Stempak, K.: Transplantation theorems—a survey. J. Fourier Anal. Appl. 17, 408–430 (2011)
Stempak, K., Trebels, W.: Hankel multipliers and transplantation operators. Studia Math. 126, 51–66 (1997)
Acknowledgments
Research of the second-named author supported by funds of Instytut Matematyki i Informatyki, Politechnika Wrocławska, Project #S30097/I-18.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Arieh Iserles.
Rights and permissions
About this article
Cite this article
Nowak, A., Stempak, K. A Note on Recent Papers by Grafakos and Teschl, and Estrada. J Fourier Anal Appl 20, 1141–1144 (2014). https://doi.org/10.1007/s00041-014-9348-z
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00041-014-9348-z