Investigation of the Extraordinary Phase Transition in Antiferromagnetic Thin Films: Computer Simulation

Abstract

The extraordinary phase transition in antiferromagnetic thin films has been analyzed by computer simulation. The simulation has been performed using the Ising model and the Metropolis algorithm. Epitaxial films with a cubic lattice containing several monoatomic layers have been considered. The condition for the occurrence of surface and extraordinary phase transitions is the difference between the exchange integrals in the bulk of the film and on its surface. It is shown that the surface and extraordinary phase transitions occur in antiferromagnetic thin films containing no less than eight monoatomic layers. The extraordinary phase transition has been investigated for different film thicknesses. It is shown that the magnetic susceptibility near the phase transition line has a logarithmic dependence on the phase-transition temperature. The dependence of the critical indices of the logarithmic phase on the film thickness is obtained.

1 INTRODUCTION

Non-conventional phase transitions make an urgent problem of the condensed-state theory and statistical physics. Such phase transitions can be observed in systems composed of two independent but interacting parts. These systems include materials exhibiting surface magnetism. The phenomenon of surface magnetism consists in the fact that the atomic spin ordering temperature on the surface may differ from the corresponding temperature in the bulk of the system [1–3]. In this case, two phase transitions occur with a decrease in temperature. First, a thin surface layer is ordered, and then (at a lower temperature) the bulk of the system passes to the ordered phase. Surface phase transitions can be observed in both semi-infinite systems with a flat free surface and thin films. Surface phase transitions were experimentally observed in ferromagnetic [1] and antiferromagnetic materials [2, 3].

The phase diagram of a system contains a surface phase transition if the surface magnetic energy exceeds some value [4, 5]. For antiferromagnetic semibounded systems, the surface phase transition occurs when the exchange integral on the surface of a system exceeds the bulk value by a factor of more than 1.55 [6]. For antiferromagnetic thin films, this value depends on the film thickness. At lower ratios of the exchange integrals, a system undergoes a conventional phase transition from the paramagnetic to antiferromagnetic state. The characteristics of a surface phase transition correspond to those of a phase transition in a two-dimensional system. A conventional phase transition belongs to the same universality class as a phase transition in a three-dimensional unbounded system [4, 5]. A transition from a surface-ordered bulk-disordered phase to a completely ordered phase is of great interest. This phase transition is referred to as extraordinary one. The influence of the ordered surface layer results in exotic properties of the extraordinary phase transition [6].

The concept of logarithmic extraordinary phase was proposed for these phase transitions [7, 8]. In this phase, the correlation functions near the Néel point have a logarithmic dependence on the temperature difference rather than a power-law one. The classical O(2) and O(3) models were considered based on this concept [9, 10]. The logarithmic phase was also theoretically predicted for the quantum Heisenberg model with dangling spins [9, 11]. The question of existence of the logarithmic phase was intensely investigated for the classical Heisenberg model [12, 13] and the XY model [14]. The logarithmic phase was revealed in the antiferromagnetic Potts model with three states by computer simulation [15].

In this paper, we report the results of studying the extraordinary phase transition in antiferromagnetic thin films described by the Ising model. The main goal is to determine the possibility of existence of the logarithmic phase in these systems at different values of the surface exchange integral.

2 MODEL

An antiferromagnetic thin film is modeled as a set of monolayers with a cubic lattice. Each atom with the number i corresponds to spin S_i. Thin films are characterized by the shape anisotropy, which is reduced to the presence of the easy-magnetization axis oriented perpendicular to the film plane. Therefore, these films can be described by the Ising model. Each spin S_i can take one of the two values (+1/2 or −1/2). The nearest-neighbor interaction is antiferromagnetic. Surface antiferromagnetism manifests itself when the spin interaction energy on the film surface differs from that in the bulk. The exchange interaction constant in the bulk of the system is denoted as J₀. The exchange interaction constant on the surface of the system is J_s. The surface phase transition is possible when J_s > J₀. It is convenient to perform computer simulation with relative quantities. Therefore, all energy parameters of the system will be calculated in terms of J₀. Let us introduce the ratio of the exchange interaction constants:

$$R = \frac{{{{J}_{s}}}}{{{{J}_{0}}}}.$$

(1)

For this parameter, the inequality R ≥ 1 is satisfied. The computer simulation was performed for different R values. The exchange integral ratio has a critical value R_c, above which (R > R_c) the surface antiferromagnetism is observed. Below this value (R < R_c), there is a conventional phase transition from the paramagnetic to antiferromagnetic phase. At the exchange integral ratio equal to the critical value (R = R_c), the phase diagram contains a tricritical point of a special phase transition. If R > R_c, surface monolayers of the film are first to be ordered with a decrease in temperature. This phase transition occurs at a temperature T_s. The bulk of the film remains in the paramagnetic phase. At a lower temperature T_e (T_e < T_s), the entire volume of the film passes to the antiferromagnetic phase. This transition is referred to as extraordinary one.

The Hamiltonian of the Ising model is calculated as the sum of the energies of spin pairing interactions:

$${\rm H} = {{J}_{0}}\sum\limits_{\left\langle {i,j} \right\rangle } {{{S}_{i}}{{S}_{j}}} + {{J}_{s}}\sum\limits_{\left\langle {i,j} \right\rangle } {{{S}_{i}}{{S}_{j}}} .$$

(2)

In the Hamiltonian, the summation is only over the pairs $\left\langle {i,j} \right\rangle$ of the nearest neighbors. The second term includes only the pairs of spins on the surface. In the first term, at least one spin should be not on the s-urface. In relative units, the Hamiltonian can be w-ritten as

$$\frac{{\rm H}}{{{{J}_{0}}}} = \sum\limits_{\left\langle {i,j} \right\rangle } {{{S}_{i}}{{S}_{j}}} + R\sum\limits_{\left\langle {i,j} \right\rangle } {{{S}_{i}}{{S}_{j}}} .$$

(3)

The temperature t of the system will also be used in the relative form:

$$T = \frac{{{{k}_{{\text{B}}}}t}}{{{{J}_{0}}}},$$

(4)

where k_B is the Boltzmann constant.

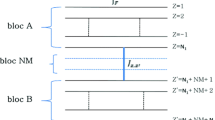
In the computer simulation, the thin film was oriented parallel to the OXY plane. The film surfaces were described by the equations z = 0 and z = D – 1, where D is the film thickness determined by the number of monolayers (ML). The system under study had the sizes of $L \times L \times D$, where L is the number of atoms along the OX and OY axes. Periodic boundary conditions were imposed on the system along the OX and OY axes to analyze the properties of an infinite film.

To study the extraordinary and surface antiferromagnetic phase transition, one should analyze the processes of spin ordering on the surface of the system and in its bulk. Therefore, thin films were considered as three interacting subsystems. Two subsystems were free surfaces (i.e., monolayers bounding the film). The third subsystem was a set of spins in the film bulk without two extreme layers. The first and second subsystems were arranged symmetrically and behaved similarly with a change in the temperature of the system. Therefore, only spin ordering on the surface z = 0 was considered in the computer experiment. Control experiments showed that the thermodynamic functions have the same values for both planes bounding the film.

To describe the processes of spin ordering in the bulk of the system, the antiferromagnetic order parameter m_a was introduced; it was calculated as the difference between magnetizations of two sublattices shifted relative to each other by the lattice constant along the OX, OY, and OZ axes. The first and second sublattices will be referred to as even and odd ones, respectively. Then the antiferromagnetic order parameter is calculated as the difference between two sums normalized to the total number of spins in the system:

$${{m}_{a}} = \frac{{\left( {\sum\limits_{{\text{even}}} {{{S}_{i}}} - \sum\limits_{{\text{odd}}} {{{S}_{i}}} } \right)}}{{{{L}^{2}}\left( {D - 2} \right)}}.$$

(5)

To characterize the surface spin ordering, we introduce a similar antiferromagnetic surface order parameter m_s, which is also equal to the difference between magnetizations of two two-dimensional sublattices:

$${{m}_{s}} = \frac{{\left( {\sum\limits_{{\text{even}\_}S} {{{S}_{i}}} - \sum\limits_{{\text{odd}\_}S} {{{S}_{i}}} } \right)}}{{{{L}^{2}}}},$$

(6)

where even_S is the even sublattice on the film surface and odd_S is the odd sublattice on the film surface.

Both order parameters are zero at random spin directions and unity at antiferromagnetic ordering. The two systems under study undergo a phase transition from the paramagnetic to antiferromagnetic phase with a decrease in temperature. To construct the phase diagram of a thin film, the phase-transition temperatures were investigated separately for the film surface and the bulk at different ratios R of the exchange interaction constants.

The system was studied using the Metropolis algorithm and the finite-size scaling theory [16]. Systems with different linear sizes L were considered within these approaches. The Metropolis algorithm forms different thermodynamic states of the spin system corresponding to a specified temperature. The thermodynamic functions of the system are calculated based on averaging of the system parameters over different thermodynamic configurations. Below, angle brackets $\left\langle \ldots \right\rangle$ are used to denote a certain parameter averaged over the thermodynamic states.

Several parameters were used to describe in detail the phase transitions in thin films.

The magnetic susceptibilities of the sublattices of the system for the bulk (${{\chi }_{a}}$) and surface (${{\chi }_{s}}$) can be written as

$${{\chi }_{a}} = \frac{{\partial {{m}_{a}}}}{{\partial h}} = {{L}^{2}}\left( {D - 2} \right)\left( {\left\langle {m_{a}^{2}} \right\rangle - {{{\left\langle {{{m}_{a}}} \right\rangle }}^{2}}} \right){\text{/}}T,$$

(7)

$${{\chi }_{s}} = \frac{{\partial {{m}_{s}}}}{{\partial h}} = {{L}^{2}}\left( {\left\langle {m_{s}^{2}} \right\rangle - {{{\left\langle {{{m}_{s}}} \right\rangle }}^{2}}} \right){\text{/}}T,$$

(8)

where h is the magnetic field strength.

The fourth-order Binder cumulants for the both order parameters are

$${{U}_{a}} = 1 - \frac{{\left\langle {m_{a}^{4}} \right\rangle }}{{3{{{\left\langle {m_{a}^{2}} \right\rangle }}^{2}}}},$$

(9)

$${{U}_{S}} = 1 - \frac{{\left\langle {m_{S}^{4}} \right\rangle }}{{3{{{\left\langle {m_{S}^{2}} \right\rangle }}^{2}}}}.$$

(10)

The fourth-order Binder cumulants for the system energy have the form

$${{V}_{a}} = 1 - \frac{{\left\langle {E_{a}^{4}} \right\rangle }}{{3{{{\left\langle {E_{a}^{2}} \right\rangle }}^{2}}}},$$

(11)

$${{V}_{S}} = 1 - \frac{{\left\langle {E_{S}^{4}} \right\rangle }}{{3{{{\left\langle {E_{S}^{2}} \right\rangle }}^{2}}}}.$$

(12)

All these three types of thermodynamic parameters can be used to determine the phase-transition temperature. The most accurate value can be obtained using the Binder cumulants U_a and U_s for the order parameters. To this end, one should perform simulation for systems with different linear sizes. The main property of these cumulants is that their values are independent of the size of the system at the phase-transition temperature. Thus, the temperature dependences of the Binder cumulants for systems with different sizes intersect at the same point. This point corresponds to the critical temperature. This property makes it possible to determine the phase-transition temperature with an error less than the temperature step of the algorithm. In addition, the Binder cumulants for the order parameters are sensitive to a change in the phase-transition order. The intersection point of the plots is clearly localized only for second-order phase transitions [16]. For first-order phase transitions, there is no single intersection point.

The method for determining the critical temperature proceeding from the magnetic susceptibility is based on the fact that the susceptibility of the system has a jump at the phase-transition point. This jump manifests itself as a peak in the temperature dependence of the susceptibility.

The temperature dependences of the Binder cumulants V_a and V_s for the energy also contain a peak at the phase-transition point. Note that this peak corresponds to the minimum of the function rather than the maximum (as for the magnetic susceptibility).

3 RESULTS

The computer experiment was carried out for antiferromagnetic films with a thickness from D = 4 ML to D = 16 ML with step ΔD = 2 ML. The linear sizes of the system were varied from L = 16 to L = 64 with step ΔL = 16. The ratio of the exchange interaction constants was varied from R = 1.0 to R = 3.0 with step ΔR = 0.1. The Néel temperature T_N of the antiferromagnetic phase transition in the bulk of the system and the surface phase transition temperature T_s were calculated for all values of the parameters. Afterwards, the phase diagrams of the films with different thicknesses D were constructed at different R values. The phase diagrams depend significantly on the film thickness. This dependence is related to the relative size of the film bulk and the surface layer. Spin ordering in the surface layer affects the spin orientation in the bulk. If the number of spins in the film bulk is larger than that in the surface layer, this effect can be neglected. If the number of spins in the film bulk is comparable with that in the surface layer, this influence becomes significant and may determine the behavior of the system.

For the films with thicknesses D = 4 ML and D = 6 ML, the sizes of the bulk coincide with those of the surface layer (D = 4 ML) or twice as large as the latter (D = 6 ML). As a result, the surface phase transition temperature T_s coincides with the Néel temperature T_N in the bulk (T_s = T_N). These two temperatures are localized quite clearly from the Binder cumulants for the order parameters. For these films, the surface and extraordinary phase transitions are not implemented. Only a conventional phase transition occurs in the system.

For the films with a thickness D ≥ 8, the number of spins in the bulk of the system exceeds significantly that on the surface; therefore, the surface and extraordinary phase transitions are implemented in the system. Note that there is a critical value R_c of the ratio of the exchange interaction constants, below which (R < R_c) only a conventional phase transition is implemented; above this value (R > R_c), the phase transition is divided into the surface and extraordinary transitions. The phase diagram for the film with thickness D = 8 ML is shown in Fig. 1. The phase diagrams for the thicker films have a similar form.

Fig. 1.

Phase diagram for the antiferromagnetic film with thickness D = 8 ML.

Upon cooling, the systems with R > R_c first undergo a phase transition in the surface monolayer at the temperature T_s (surface phase transition) and then a phase transition in the film bulk (extraordinary phase transition) at the lower temperature T_N (T_N < T_s). The difference in the phase-transition temperatures can clearly be seen from the dependences of the surface and bulk antiferromagnetic order parameters. Figure 2 shows the temperature dependences of the surface (m_s) and bulk (m) order parameters for the film with thickness D = 16 ML and the exchange interaction constant ratio R = 2.5.

Fig. 2.

Dependences of the surface (m_s) and bulk (m) order parameters on temperature T at R = 2.5 and D = 16 ML.

This sequence of spin ordering in the system affects the behavior of the thermodynamic functions near the extraordinary phase transition. Ordered surface spins affect positively the ordering processes in the bulk of the system. The two free surfaces act as an external field (related to the order parameter) for spins in the bulk of the system. As is well known, an external field leads to phase-transition diffusion. This diffusion makes it difficult to accurately determine the temperature of the extraordinary phase transition. It can be seen in Fig. 3 that the maxima of the magnetic susceptibility are smoothed, with no pronounced sharp peaks.

Fig. 3.

Dependences of the bulk magnetic susceptibility ${{\chi }_{a}}$ on temperature T at R = 2.5 and D = 14 ML.

The absence of a pronounced second-order phase transition affects the behavior of the Binder cumulants for the order parameter. There is no clearly localized point of intersection of their temperature dependences (Fig. 4).

Fig. 4.

Dependences of the bulk Binder cumulants U on temperature T at R = 2.5 and D = 16 ML.

The same behavior of the Binder cumulants is observed for first-order phase transitions. The phase-transition temperature can be determined from the energy cumulants V. These cumulants have a minimum at the phase transition point (Fig. 5).

Fig. 5.

Dependences of the energy cumulants V on temperature T for the film with thickness D = 16 ML at R = 1.8.

Let us analyze the dependence of the magnetic susceptibility on the temperature of the system. For a conventional phase transition, the magnetic susceptibility near the Néel temperature is approximated by a power-law function and the scaling relation is valid:

$${{\chi }_{a}}\sim {{\left| {T - {{T}_{{\text{N}}}}} \right|}^{{ - \gamma }}}.$$

The dependence of $\ln {{\chi }_{a}}$ on $\ln \left| {T - {{T}_{{\text{N}}}}} \right|$ is linear. The slope of this straight line determines the critical index γ. Now we plot the dependence of the logarithm of the magnetic susceptibility $\ln {{\chi }_{a}}$ on $\ln \left| {T - {{T}_{e}}} \right|$ for the extraordinary phase transition (Fig. 4).

As can be seen in Fig. 4, the plot is nonlinear and the conventional scaling relation is not valid for the extraordinary phase transition. Let us analyze the extraordinary phase transition for the correspondence to the logarithmic scaling relation:

$${{\chi }_{a}}\sim {{\left( {\ln \left| {1 - T{\text{/}}{{T}_{{\text{N}}}}} \right|} \right)}^{{ - q}}},$$

where q is the critical index of magnetic susceptibility at the logarithmic phase transition. In this case, the dependence of $\ln {{\chi }_{a}}$ on $\ln \left( {\ln \left| {1 - T{\text{/}}{{T}_{e}}} \right|} \right)$ should be linear. An example of the corresponding plot for the film with thickness D = 16 ML at R = 2.5 is shown in Fig. 5.

The linearity of the plot in Fig. 5 suggests that the logarithmic scaling relation is valid at the extraordinary phase transition. Similar linear dependences are valid for the films with any thickness D ≥ 8 at any exchange integral ratio R > R_c.

Fig. 6.

Dependence of the logarithm of the magnetic susceptibility $\ln {{\chi }_{a}}$ on $\ln \left| {T - {{T}_{e}}} \right|$ for the extraordinary phase transition in the film with thickness D = 16 ML at R = 2.5.

Fig. 7.

Dependence of the logarithm of the magnetic susceptibility $\ln {{\chi }_{a}}$ on $\ln \left( {\ln \left| {1 - T{\text{/}}{{T}_{e}}} \right|} \right)$ for the extraordinary phase transition in the film with thickness D = 16 ML at R = 2.5.

Calculation of the critical indices q showed that it depends only on the film thickness D and is independent of the exchange integral ratio R. Values of the critical index q at different film thicknesses D are listed in Table 1.

Table 1. Critical index q at different film thicknesses D

As can be seen, the critical index of the magnetic susceptibility increases nonlinearly with an increase in the film thickness.

4 CONCLUSIONS

The computer simulation showed that there is a minimum film thickness, beginning from which the surface and extraordinary phase transitions can be observed. Conventional scaling relations for second-order phase transitions are not valid for the extraordinary phase transition. The behavior of the thermodynamic functions near the temperature of the extraordinary phase transition is described by a logarithmic power-law function rather than a power-law one. This dependence indicates that the logarithmic phase transition is implemented, which was predicted previously for the classical and quantum Heisenberg models [9–11]. The calculations showed that the critical index of the logarithmic phase transition increases with an increase in the film thickness. The logarithmic critical index is independent of the exchange interaction constant ratio (i.e., it remains constant along the entire line of the extraordinary phase transition).