Introduction

Vibration is ubiquitous in engineering applications such as construction machinery, aerospace, vehicles, and precision devices [1]. Adverse vibration may affect the normal function of precision devices and even cause large deformations and damage to the mechanical structure. Therefore, it is necessary to isolate and suppress harmful vibration and control it within a reasonable and acceptable range to avoid large economic losses and resource waste [2].

There is a contradiction between the bearing capacity and vibration isolation performance of traditional linear vibration isolation systems. Normally, when the external excitation frequency is greater than \(\sqrt 2\) times the natural frequency of the system, the linear isolator can work effectively [3,4,5]. Therefore, its performance is superior in high-frequency vibration isolation, but it is difficult to achieve low-frequency vibration isolation. Thus, the nonlinear vibration isolator is proposed to solve the problem of low-frequency vibration isolation [6]. When vibration isolator vibrates near the operating point, its dynamic stiffness is close to 0, this kind of vibration isolator is called QZS vibration isolator. The QZS vibration isolator has high static stiffness and low dynamic stiffness, which can withstand a large mass load and a large range of vibration isolation. It can also solve the problem of low-frequency vibration isolation and large displacement conflict when using a linear vibration isolator [7, 8]. The QZS vibration isolator has sufficient static stiffness to reduce static load and, at the same time, provides low dynamic stiffness near the equilibrium position for vibration isolation [9,10,11]. Such so-called static high stiffness and dynamic low stiffness characteristics enable the QZS isolator to work at a low external excitation frequency [12, 13]. The characteristics of QZS vibration isolator are usually obtained by parallel connections of positive and negative stiffness [14,15,16].

Alabuzhev et al. first proposed the concept of QZS, introduced negative stiffness mechanism into linear vibration isolation system, and defined the QZS theory for the first time [17]. Carrella et al. constructed a new QZS vibration isolation mechanism consisting of three springs using inclined springs as negative stiffness elements [18, 19]. Kovacic et al. adopted preloaded nonlinear spring instead of linear spring to solve the problem of drastic changes in dynamic stiffness after the system deviates from the equilibrium position [20]. Zhou et al. proposed a cam-spring mechanism. The research shows that no matter what the excitation amplitude of the vibration isolation system is the peak transfer rate and initial frequency of the vibration isolation are not higher than that of the corresponding linear isolator, which realizes the structural innovation of the negative stiffness mechanism [21]. Kim et al. proposed a QZS vibration isolator composed of a vertical spring with preload and eight transverse leaf springs with nonlinear buckling characteristics [22]. Dalela et al. used a bistable cosine beam instead of a nonlinear inclined spring as a negative stiffness mechanism to design a vertical vibration isolation system with single degrees of freedom [23]. Zhang et al. designed a new QZS torsional vibration isolator to adapt to load changes by adjusting positive stiffness and synchronously adjusting negative stiffness so that the vibration isolator would always work in the QZS state, aiming at the problem that the existing QZS torsional vibration isolator is only applicable to constant load [24]. In addition, there are negative stiffness mechanisms designed by combining Euler buckling beam [25, 26], air spring [27], composite structure [28,29,30] and bionic structure [31,32,33], etc., which greatly increase the types and number of QZS vibration isolators. However, the design of these vibration isolators still has some defects in compactness and structural stability, which need be further developed.

In this paper, a QZS isolator with a simple and compact structure that can achieve low frequency and heavy load vibration isolation in the horizontal direction is designed. For the realization of QZS, the special structure of plane four-rod mechanism is adopted to realize the horizontal QZS, which has the advantages of simple structure and compact structure compared with the traditional spring structure vibration isolator. In vibration isolation systems, the dual four-rod structure consists of simple basic mechanical elements, rods and hinges that provide reliable QZS near the equilibrium position. In addition, in terms of achieving QZS isolation, the isolator can achieve a wide range of horizontal low-frequency vibration isolation and the load can be borne by two sets of four-rod mechanisms in practical applications. Therefore, the vibration isolator can realize vibration isolation for large loads without increasing the structure of the vibration isolator, which makes it have greater application potential. The dual four-rod isolators offer further improvements in simplicity and compactness compared to previous isolators, and are more suitable for heavy load isolation requirements. In “Conceptual Design and Parameter Optimization”, the conceptual design and physical model of the new dual four-rod vibration isolator are presented, and the parameter design and optimization of QZS are carried out. In “Mechanical analysis of the vibration isolator”, the dynamic model of the new dual four-rod isolator is established, and the theoretical isolation performance of the isolator is simulated. In “Experimental Validation”, experimental platforms are established, and static and dynamic tests are performed. The vibration isolation effect in “Conclusions” is evaluated. Finally, Sect. 5 ends the paper with conclusions and remarks.

Conceptual Design and Parameter Optimization

In this section, the dual four-rod vibration isolator is physically modeled and analyzed, the motion trajectory of the isolator is planned, and the motion trajectory of the isolator working platform is realized as a horizontal quasi-straight line. By optimizing the member center of mass, the isolator equivalent center of mass is realized without deviation near the equilibrium position, which simplifies the motion analysis process. The specific contents are as follows.

Vibration Isolator Structure Design

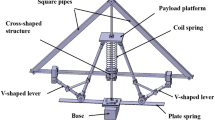
Figure 1 shows the proposed the dual four-rod QZS vibration isolator model. The model of the vibration isolator is a central symmetrical structure. The main structure of the vibration isolator can be divided into three parts. P1 is the first set of parallelogram structure for the vibration isolator, which are hinged to the upper counterweight platform and the lower vibration isolator platform by a long connecting rod. P2 is the second parallelogram structure of the isolator, which is hinged to P1 and the base, respectively. The middle swing rod is hinged with the upper counterweight platform and the lower base, respectively.

Fig. 1
figure 1

Dual four-rod horizontal vibration isolator. a Three-dimensional model of the vibration isolator, b schematic diagram of the unilateral structure

Full size image

The vibration isolator model proposed in this paper is centrosymmetric, and there are many rods with the same function. In order to simplify the calculation, the rods with the same motion are treated as equivalent, where Q1 and P2 are hinged with the base, and the motion track of the upper end of both is an arc, which jointly provides the supporting force of P1. Q1 can be simplified to CD, P2 to OA, and P1 to CB. The connection part between the upper end OF the P2 rod and the P1 rod can be simplified as OF, and the connection part between the counterweight platform and Q1 can be simplified as DE. The simplified equivalent mechanism diagram of the vibration isolator is obtained, as shown in Fig. 2. OD is a part of the base. B is a vibration isolation platform. Assume that the mass of the object to be isolated is M. The lengths of each member are \(OA = l_{1}\), \(CD = l_{3}\), \(OD = l_{4}\), \(AB = d_{1}\), \(AC = d_{2}\), respectively. Rods OA and CD rotate around points O and D, respectively, and rods BC and rods OA and CD are connected by hinges at points A and C, respectively.

Fig. 2
figure 2

Schematic diagram of the vibration isolator mechanism

Full size image

Trajectory Planning of Working Platform

In order to make the movement of the working platform symmetrical with respect to the OD rod and the mechanism can produce a singular increase in the degree of freedom at the central equilibrium position, the length of each rod must satisfy

$$l_{1} + d_{2} = l_{4} + l_{3} .$$
(1)

As shown in Fig. 2, point O is selected as the origin to establish the coordinate system. A and C move in a circle around O and D, respectively. According to the law of circular motion, we can obtain

$$\left\{ {\begin{array}{*{20}c} {(x_{C} - x_{A} )^{2} + (y_{C} - y_{A} )^{2} = d_{2}^{2} } \\ {{\kern 1pt} {\kern 1pt} (x_{C} - x_{D} )^{2} + (y_{C} - y_{D} )^{2} = \left( {l_{3} } \right)^{2} } \\ \end{array} } \right.,$$
(2)

\(\overrightarrow {AB} {\kern 1pt} {\kern 1pt}\) and \({\kern 1pt} {\kern 1pt} \overrightarrow {CA} {\kern 1pt} {\kern 1pt}\) are collinear, and there is \({\kern 1pt} {\kern 1pt} \overrightarrow {AB} = \frac{{d_{1} }}{{d_{2} }}\overrightarrow {CA}\). The relationship equation between point B and points A and C are established as

$$\left\{ {\begin{array}{*{20}c} {x_{B} = \frac{{d_{1} }}{{d_{2} }}(x_{A} - x_{C} ) + x_{A} } \\ {y_{B} = \frac{{d_{1} }}{{d_{2} }}(y_{A} - y_{C} ) + y_{A} } \\ \end{array} } \right..$$
(3)

The coordinates of point B can be obtained by Eqs. (2) and (3) as

$$\left\{ \begin{gathered} x_{B} = l_{1} \cos (\theta ) + \frac{{d_{1} Q}}{{d_{2} }} \hfill \\ y_{B} = l_{1} \sin (\theta ) - \frac{{d_{1} \sqrt {d_{2} + l_{1} \cos (\theta ) - S} \sqrt {d_{2} - l_{1} \cos (\theta ) + S} }}{{d_{2} }} \hfill \\ \end{gathered} \right..$$
(4)

Here,

$$\begin{gathered} Q = l_{1} \cos (\theta ) - \frac{{l_{1}^{3} \cos (\theta ) + {\text{sign}}\left( {\frac{\pi }{2} - \theta } \right)Rl_{4} - d_{2}^{2} l_{1} \cos (\theta ) + l_{1} l_{3}^{2} \cos (\theta )}}{{2l_{1}^{2} + 2l_{4}^{2} - 4l_{1} l_{4} \sin (\theta )}} \hfill \\ {\kern 1pt} + \frac{{l_{1} l_{4}^{2} \cos (\theta ) - {\text{sign}}\left( {\frac{\pi }{2} - \theta } \right)Rl_{1} \sin (\theta ) - 2l_{1}^{2} l_{4} \cos (\theta )\sin (\theta )}}{{2l_{1}^{2} + 2l_{4}^{2} - 4l_{1} l_{4} \sin (\theta )}}, \hfill \\ \end{gathered}$$
$$\begin{gathered} R = (2d_{2}^{2} l_{1}^{2} - l_{1}^{4} - l_{3}^{4} - l_{4}^{4} - d_{2}^{4} + 2d_{2}^{2} l_{3}^{2} + 2d_{2}^{2} l_{4}^{2} + 2l_{1}^{2} l_{3}^{2} - 2l_{1}^{2} l_{4}^{2} + 2l_{3}^{2} l_{4}^{2} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} - \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} 4l_{1}^{2} l_{4}^{2} \sin^{2} (\theta ) + 4l_{1} l_{4}^{3} \sin (\theta ) + 4l_{1}^{3} l_{4} \sin (\theta ) - 4d_{2}^{2} l_{1} l_{4} \sin (\theta ) - 4l_{1} l_{3}^{2} l_{4} \sin (\theta ))^{\frac{1}{2}} , \hfill \\ \end{gathered}$$
$$\begin{gathered} S = \frac{{l_{1}^{3} \cos (\theta ) + {\text{sign}}\left( {\frac{\pi }{2} - \theta } \right)Rl_{4} - d_{2}^{2} l_{1} \cos (\theta ) + l_{1} l_{3}^{2} \cos (\theta )}}{{2l_{1}^{2} + 2l_{4}^{2} - 4l_{1} l_{4} \sin (\theta )}} \hfill \\ {\kern 1pt} + \frac{{l_{1} l_{4}^{2} \cos (\theta ) - {\text{sign}}\left( {\frac{\pi }{2} - \theta } \right)Rl_{1} \sin (\theta ) - 2l_{1}^{2} l_{4} \cos (\theta )\sin (\theta )}}{{2l_{1}^{2} + 2l_{4}^{2} - 4l_{1} l_{4} \sin (\theta )}}. \hfill \\ \end{gathered}$$

Motion Trajectory and QZS Optimization

The motion trajectory of the vibration isolation platform is complicated, and there are many parameters affecting its motion. There are countless groups of rod lengths meeting the requirements when solving the ideal trajectory only through the equation, and all rod lengths can be scaled in the same proportion, and there will be more rod length combinations meeting the accuracy conditions. The coordinate expressions of B and C obtained in the above kinematic analysis are highly nonlinear equations, so it is difficult to search the optimal solution by conventional calculation or gradient method. Artificial fish swarm algorithm (AFSA) is not sensitive to the initial value, has low requirements on the objective function and has the ability to obtain the optimal solution globally.

Motion Trajectory Optimization

The individual state of artificial fish can be represented by vector X = (x1, x2, …, xn), while xi (i = 1, 2, …, n) is an artificial fish in path optimization, and the population size of artificial fish is N. The food concentration at the current position of artificial fish can be expressed as Y = f(x), \(\delta\) is the crowding factor, t is the number of iterations, Step is the moving step length of artificial fish, and Rand (0, 1) is the random number of (0, 1).

This paper mainly adopts the clustering behavior of fish schools to optimize. Assume the number N of artificial fish in the current artificial fish Xi neighborhood and its center position XB. If δYi < YB/N indicates that the center of the artificial fish in the neighborhood is not too crowded and the food concentration is relatively high, then move one unit step toward XB through Eq. (5), otherwise perform foraging behavior:

$$X_{i} (t + 1) = X_{i} (t) + \frac{{X_{B} (t) - X_{i} (t)}}{{\left\| {X_{B} (t) - \left. {X_{i} (t)} \right\|} \right.}} \times {\text{Step}} \times {\text{Rand}}(0,1).$$
(5)

According to the principle of fish swarm algorithm, the angle \({\kern 1pt} {\kern 1pt} \theta {\kern 1pt} {\kern 1pt}\) of connecting rod OA around the base is taken as the independent variable, and the longitudinal expression of point B is set as \({\kern 1pt} {\kern 1pt} f_{y} ({\kern 1pt} {\kern 1pt} \theta {\kern 1pt} {\kern 1pt} ) = y_{B}\). In the whole interval, the motion trajectory of point B is continuously derivable, and \(y_{B}\) obtains the minimum value \({\kern 1pt} {\kern 1pt} y_{\min } = l_{1} - d_{1} {\kern 1pt} {\kern 1pt}\) when the center position is required. The movement range of point B in the abscissa direction is related to the size of the vibration isolation interval. Let the abscissa expression of point B be \({\kern 1pt} {\kern 1pt} f_{x} (\theta ) = x_{B}\), and define the horizontal movement interval as \({\kern 1pt} {\kern 1pt} \left[ { - L_{k} {\kern 1pt} {\kern 1pt} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} L_{k} } \right]\).

According to \({\kern 1pt} {\kern 1pt} f_{y} ({\kern 1pt} \theta {\kern 1pt} ){\kern 1pt} {\kern 1pt}\) monotonicity, minimum value, and \({\kern 1pt} {\kern 1pt} f_{x} ({\kern 1pt} \theta {\kern 1pt} ){\kern 1pt} {\kern 1pt}\) maximum motion interval, the basic constraints can be obtained:

$$\left\{ \begin{gathered} f_{y} (\theta )_{\min } = l_{1} - d_{1} \, ,\theta = \frac{\pi }{2} \hfill \\ f_{y} ^{\prime}(\theta ) \le 0 \, {\kern 1pt} {\kern 1pt} \, ,\theta \in \left[ {\frac{\pi }{2} + \frac{\pi }{20},\frac{\pi }{2}} \right) \hfill \\ f_{y} ^{\prime}(\theta ) \ge 0 \, {\kern 1pt} \, {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} ,\theta \in \left( {\frac{\pi }{2},\frac{\pi }{2} - \frac{\pi }{20}} \right] \hfill \\ f_{x} (\theta )_{\max } \ge L_{k} \, {\kern 1pt} \, {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} ,\theta \in \left( {\frac{\pi }{2},\frac{\pi }{2} - \frac{\pi }{20}} \right] \hfill \\ \end{gathered} \right..$$
(6)

That is, search and obtain the combination of rod length with the minimum fluctuation of the ordinate of point B within the given range of motion, and the corresponding expression is

$$g_{{{\text{opt}}}} (\theta ) \to \min .$$
(7)

Considering the structural size of the vibration isolator and subsequent processing conditions, we define \(l_{1} = 0.35\;{\kern 1pt} {\text{m}}\), the rod length range of \({\kern 1pt} {\kern 1pt} d_{1}\),\({\kern 1pt} {\kern 1pt} l_{3}\),\({\kern 1pt} {\kern 1pt} l_{4} {\kern 1pt} {\kern 1pt}\) is \(\left[ {0.05\;{\text{m,}}\;1\;{\text{m}}} \right]{\kern 1pt} {\kern 1pt}\), the rod length range of \(d_{2}\) is \(\left[ {0.1\;{\text{m, }}\;1.5\;{\text{m}}} \right]{\kern 1pt} {\kern 1pt}\), and the ordinate of the vibration isolation point B allows the maximum. The fluctuation value is set to \(\delta < 0.5\;{\text{mm}}\) and some intervals are discarded in advance.

The search optimization convergence process is shown in Fig. 3. After 1349 optimizations, the target value is within 0.05 to meet the design requirements. For the convenience of subsequent analysis and combined with the processing and assembly conditions, the length of each rod is selected under the condition of satisfying the error accuracy, as shown in Table 1.

Fig. 3
figure 3

Optimizing tracking process curve

Full size image
Table 1 Quasi-straight line optimization results
Full size table

Substitute length data of the optimized pole into the Eq. (4) to select \({\kern 1pt} {\kern 1pt} \theta \in \left[ {\frac{9\pi }{{20}},\frac{11\pi }{{20}}} \right]\), and evenly select 50 values in the interval to draw and draw the motion track points of the working platform, and the fitting curve is \(y{\kern 1pt} (x) = 0.3946x^{4} - 0.1382\). From Fig. 4, it can be seen that the movement trajectory of the working platform in a relatively large range in the horizontal direction is a quasi-straight line.

Fig. 4
figure 4

Motion trajectory fitting curve of the working platform

Full size image

QZS Optimization

The motion trajectory optimization ensures that the motion trajectory of the vibration isolator working platform is quasi-straight line. If the mass of the rod itself is not considered, the system has achieved QZS. However, as the input angle \(\theta\) changes, the accompanying motion of each member will cause its comprehensive center of gravity to change in the vertical direction. For low-frequency vibration isolation, the influence of the load and the gravity generated by its own structure on the total stiffness of the system must be considered. This type of vibration isolator based on the principle of a single pendulum needs to minimize the impact of load mass on the performance of the isolation mechanism, and the change in gravitational potential energy of the isolation mechanism itself during movement. The ideal situation is that the overall height of the center of gravity of the mechanism is always constant.

Rods OA and CD rotate around points O and D, respectively. If the two rods are doubled in the other direction, their center of mass can remain unchanged during the motion. The rod OD is fixed, and its center of mass does not change during the movement. For the rod BC, its motion is a compound motion of rotation and movement, and the trajectory of the center of mass is complex. In order to simplify the problem, taking the center of mass of the base rod OD as the reference object, add counterweights \({\kern 1pt} {\kern 1pt} M_{e} {\kern 1pt} {\kern 1pt}\) and \({\kern 1pt} {\kern 1pt} M_{f}\) to the reverse extension lines of the rods OA and CD, respectively, assuming that the mass of all rods is uniformly distributed and the mass is only related to the length. Let the linear density be \({\kern 1pt} {\kern 1pt} \rho_{0}\), and express the mass at points E and F as

$$\left\{ {\begin{array}{*{20}c} {M_{e} = \rho_{0} l_{e} } \\ {M_{f} = \rho_{0} l_{f} } \\ \end{array} } \right..$$
(8)

Then, the vertical coordinates of the combined equivalent centroid of each active member and counterweight \({\kern 1pt} {\kern 1pt} M_{e} {\kern 1pt} {\kern 1pt}\) and \({\kern 1pt} M_{f}\) is

$$\overline{y} (\theta ) = \frac{1}{2}\frac{{(y_{B} + y_{C} )l_{2} + y_{D} l_{4} + y_{E} l_{5} + y_{F} l_{6} + 2y_{E} l_{e} + 2y_{F} l_{f} }}{{l_{2} + l_{4} + l_{5} + l_{6} + l_{e} + l_{f} }}.$$
(9)

In order to ensure the stability of the vibration isolator system, the comprehensive equivalent centroid height \({\kern 1pt} {\kern 1pt} \overline{h}_{y} {\kern 1pt}\) of the system is the smallest when it is at the center equilibrium position and has a monotonous increase when it deviates from the central position. The constraints of the comprehensive centroid are

$$\left\{ \begin{gathered} \overline{y}(\theta )_{\min } = \overline{h}_{y} \, ,\theta = \frac{\pi }{2} \hfill \\ \overline{y}{\prime} (\theta ) \le 0 \, ,\theta \in \left[ {\frac{\pi }{2} + \frac{\pi }{20},\frac{\pi }{2}} \right) \hfill \\ \overline{y}{\prime} (\theta ) \ge 0 \, ,\theta \in \left( {\frac{\pi }{2},\frac{\pi }{2} - \frac{\pi }{20}} \right] \hfill \\ \end{gathered} \right..$$
(10)

Setting the constraint conditions of each rod as \(l_{5} \in (0,0.16)\), \(l_{6} \in (0,0.35)\), the counterweight mass \(l_{e} \in (0,30)\), \(l_{f} \in (0,30)\), and set the linear density as \(\rho_{0} = 4.83{\text{ kg/m}}\). The design objective equation is

$$h_{{{\text{opt}}}} (\theta ) = \overline{y}(\theta ) - \overline{h}_{y} .$$
(11)

Similarly, AFSA was used to find the optimal solution. After 800 iterations, a stable optimal solution was found at the 301st. The weight optimization results are shown in Table 2.

Table 2 QZS optimization parameter results
Full size table

According to the optimized structural parameters and mass parameters of the counterweight, the mass of the vibration isolation mechanism itself is 24.56 kg. The trajectory of the vibration isolator working platform is curve-fitted to obtain the trajectory equation as \({\kern 1pt} {\kern 1pt} y(x) = 0.3946x^{4} - 0.1382\).

Mechanical Analysis of the Vibration Isolator

In this section, the stiffness of the isolator is analyzed theoretically, and QZS can be obtained near the equilibrium position. The dynamic model of the dual four-rod horizontal vibration isolator is established. The dynamic response of the isolator is solved by polynomial fitting and the harmonic balance method. By studying the influence of different parameters on the displacement transmissibility, the vibration isolation performance of the isolator is studied and explained.

Stiffness Analysis

As shown in Fig. 5, the motion of the vibration isolated object on the working platform is equivalent to the motion along the track with the fitting curve as the contour. The force analysis model of a vibration isolated object, where y(x) is the trajectory equation of the vibration isolated object, \({\kern 1pt} {\kern 1pt} y^{\prime}(x_{i} ,y_{i} ){\kern 1pt} {\kern 1pt}\) is the slope of the tangent line of the center of mass of the vibration isolated object on the trajectory;\({\kern 1pt} {\kern 1pt} \beta {\kern 1pt} {\kern 1pt}\) is the angle between the tangent line and the abscissa, M is the mass of the vibration isolated object, and FN is the orbit pair. The supporting force of the vibration isolated object FH is the horizontal component force of the vibration isolated object.

Fig. 5
figure 5

The force analysis model of a vibration isolated object

Full size image

The initial state of the vibration isolated object is in the static equilibrium position. The centroid position equation of the vibration isolated object is \({\kern 1pt} {\kern 1pt} M_{{(x_{i} ,y_{i} )}}\), and the motion trajectory equation is y(x). According to the motion path of the isolated object, the tangent equation at its centroid is obtained:

$$y(x) = y^{\prime}_{{(x_{i} ,y_{i} )}} (x - x_{i} ) + y_{i} .$$
(12)

The origin of the coordinates is established at the midpoint of the trajectory, the vibration isolated object is on both sides of the ordinate, and the angle \({\kern 1pt} {\kern 1pt} \beta \ge \frac{\pi }{2}{\kern 1pt}\) or \({\kern 1pt} {\kern 1pt} \beta \le \frac{\pi }{2}{\kern 1pt} {\kern 1pt}\) between the tangent equation at the center of mass and the abscissa gives

$$\beta = \arctan \left( {y^{\prime}_{{(x_{i} ,y_{i} )}} {\text{sign}}(x_{i} )} \right).$$
(13)

The horizontal restoring force of the isolating object is given by

$$F = M\sin (\beta )\cos (\beta ).$$
(14)

The horizontal stiffness of the isolating object is given by

$$K = M\left( {\cos^{2} (\beta ) - \sin^{2} (\beta )} \right).$$
(15)

As shown in Fig. 6, select three groups of vibration isolated objects whose masses are M1 = 25 kg, M2 = 30 kg, and M3 = 35 kg, respectively. Drawing force–displacement and stiffness–displacement curves, and observing the graph, it can be seen that the QZS characteristics of vibration isolators with different masses are more obvious the closer they are to the position of 0 within \({\kern 1pt} {\kern 1pt} x \in ( - 0.04{\kern 1pt} {\kern 1pt} {\text{m}},0.04{\kern 1pt} {\kern 1pt} {\text{m}}){\kern 1pt}\). Thus, it can be concluded that the dynamic stiffness of vibration isolator can achieve QZS near the static equilibrium position.

Fig. 6
figure 6

Force–displacement, stiffness–displacement curves. a Force–displacement curve, the horizontal component force value of the vibration isolation platform hardly changes within the horizontal displacement (− 0.04 m, 0.04 m), b the stiffness of the working platform of the isolator is close to 0 near the equilibrium position

Full size image

Dynamic Analysis

The horizontal restoring force obtained after the mechanical analysis of the vibration isolated object is relatively complicated, so the result of Taylor series expansion of the horizontal restoring force is F(x) = (15.5x3 + 0.02x)·M. The external horizontal acceleration excitation on the isolator base is \(- \Lambda \cos (\omega {\kern 1pt} {\kern 1pt} t)\). M is the mass of the vibration isolated object (kg), z is the absolute displacement of the vibration isolation object (m), x is the relative displacement of the vibration isolation object (m), c is the damping coefficient of the system (Ns/m), and F is the level resilience (N).

According to the vibration isolator model, the differential equation of motion of the working position is listed as follows:

$$M{\kern 1pt} \ddot{x} + c\dot{x} + F = M\Lambda \cos \omega t.$$
(16)

Let \(\xi = \frac{c}{{2\omega_{0} M}}\), \(\omega_{0} = \frac{\omega }{\gamma }\), \(\tau = \omega_{0} t\), where \({\kern 1pt} {\kern 1pt} \omega_{0} {\kern 1pt} {\kern 1pt}\) is the natural frequency of the system:

$$\ddot{x} + 2\xi \omega_{0} \dot{x} + \frac{F}{M} = \Lambda \cos \gamma {\kern 1pt} \tau .$$
(17)

Since the horizontal restoring force F is a high-order function of relative displacement x, it is more complicated to use the traditional differential equation to solve it. According to the steady-state response of the system, the ordinary differential equation average method can be used to solve it.

Let the steady-state solution of the system be

$$x(\tau ) = b(\tau )\cos \left( {\gamma \tau + \theta (\tau )} \right),$$
(18)
$$\dot{x}(\tau ) = - b(\tau )\gamma \sin \left( {\gamma \tau + \theta (\tau )} \right).$$
(19)

The establishment of Eq. (19) should meet the following requirement:

$$\dot{b}(\tau )\cos \left( {\gamma \tau + \theta (\tau )} \right) - b(\tau )\dot{\theta }(\tau )\sin \left( {\gamma \tau + \theta (\tau )} \right) = 0.$$
(20)

In order to further solve the relationship between \({\kern 1pt} {\kern 1pt} \dot{b}{\kern 1pt} {\kern 1pt}\) and \({\kern 1pt} {\kern 1pt} \dot{\theta }{\kern 1pt} {\kern 1pt}\) variables, Eq. (20) is sorted out as follows:

$$\left\{ \begin{gathered} - \dot{b}\gamma \sin \left( {\gamma \tau + \theta } \right) = - \frac{{\dot{\theta }b\gamma \sin^{2} \left( {\gamma \tau + \theta } \right)}}{{\cos \left( {\gamma \tau + \theta } \right)}} \hfill \\ - b\gamma \dot{\theta }\cos \left( {\gamma \tau + \theta } \right) = - \frac{{b\gamma \cos^{2} \left( {\gamma \tau + \theta } \right)}}{{\sin \left( {\gamma \tau + \theta } \right)}} \hfill \\ \end{gathered} \right..$$
(21)

Equation (17) can be rewritten as

$$\begin{gathered} - \dot{b}\gamma \sin \left( {\gamma \tau + \theta } \right) - b\gamma^{2} \cos \left( {\gamma \tau + \theta } \right) - b\gamma \dot{\theta }\cos \left( {\gamma \tau + \theta } \right) \hfill \\ - 2b\xi \gamma \sin \left( {\gamma \tau + \theta } \right) + 15.5b^{3} \cos^{3} \left( {\gamma \tau + \theta } \right) + 0.02b\cos \left( {\gamma \tau + \theta } \right) = \Lambda \cos (\gamma \tau ). \hfill \\ \end{gathered}$$
(22)

Simplify Eqs. (21) and (22) to get

$$\left\{ \begin{gathered} \dot{\theta } = - \frac{1}{b\lambda }\Gamma \cos \left( {\gamma \tau + \theta } \right) \hfill \\ \dot{b} = - \frac{1}{\lambda }\Gamma \sin \left( {\gamma \tau + \theta } \right) \hfill \\ \end{gathered} \right..$$
(23)

Here,

$$\begin{gathered} \Gamma = b\gamma^{2} \cos \left( {\gamma \tau + \theta } \right) + 2b\xi \omega_{0} \gamma \sin \left( {\gamma \tau + \theta } \right){\kern 1pt} {\kern 1pt} - 15.5b^{3} \cos^{3} \left( {\gamma \tau + \theta } \right) \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} - 0.02b\cos \left( {\gamma \tau + \theta } \right) + \Lambda \cos (\gamma \tau ). \hfill \\ \end{gathered}$$

Let \({\kern 1pt} {\kern 1pt} \beta = \gamma \tau + \theta {\kern 1pt} {\kern 1pt}\), then

$$\Gamma = b\gamma^{2} \cos \beta + 2b\xi \omega_{0} \gamma \sin \beta - 15.5b^{3} \cos^{3} \beta - 0.02b\cos \beta + \Lambda \cos (\beta - \theta ).$$

In order to simplify the problem, take the average value of \({\kern 1pt} {\kern 1pt} \dot{b}{\kern 1pt} {\kern 1pt}\) and \({\kern 1pt} {\kern 1pt} \dot{\theta }{\kern 1pt} {\kern 1pt}\) within \({\kern 1pt} {\kern 1pt} 2\pi {\kern 1pt} {\kern 1pt}\) to replace its gradually changing real value, and rewrite the Eq. (23) to get

$$\left\{ \begin{gathered} \dot{\theta } = - \frac{1}{2\pi b\gamma }\int\limits_{0}^{2\pi } {\Gamma \cos \beta {\text{d}}\beta } \hfill \\ \dot{b} = - \frac{1}{2\pi \gamma }\int\limits_{0}^{2\pi } {\Gamma \sin \beta {\text{d}}\beta } \hfill \\ \end{gathered} \right..$$
(24)

Solved:

$$\left\{ \begin{gathered} \dot{\theta } = - \frac{1}{2b\gamma }\left( {b\gamma^{2} - 0.02b - \frac{{465b^{3} }}{40} + \Lambda \cos \theta } \right) \hfill \\ \dot{b} = - \frac{1}{2\gamma }\left( {2b\xi \omega_{0} \gamma + \Lambda \sin \theta } \right) \hfill \\ \end{gathered} \right..$$
(25)

Let \(\dot{\theta } = 0{\kern 1pt} {\kern 1pt} ,\dot{b} = 0\) resolve to get

$$\left\{ \begin{gathered} b\gamma^{2} - 0.02b - \frac{{465b^{3} }}{40} + \Lambda \cos \theta = 0 \hfill \\ 2b\xi \omega_{0} \gamma + \Lambda \sin \theta = 0 \hfill \\ \end{gathered} \right..$$
(26)

Organized:

$$\Lambda^{2} = \left( {b\gamma^{2} - 0.02b - \frac{{465b^{3} }}{40}} \right)^{2} + \left( {2b\xi \omega } \right)^{2} ,$$
(27)
$$\cos \theta = \frac{{ - b\gamma^{2} + 0.02b + \frac{{465b^{3} }}{40}}}{\Lambda }.$$
(28)

The absolute displacement of the vibration isolated object is

$$z = \frac{\Lambda }{{\omega^{2} }}\cos \omega \tau + b\cos \left( {\omega \tau + \theta } \right) = \sqrt {b^{2} + \frac{\Lambda }{{\omega^{2} }} + \frac{2\Lambda b}{{\omega^{2} }}\cos \theta } \sin \left( {\omega \tau + \phi } \right).$$
(29)

The absolute bit shift transmissibility is the ratio of the absolute displacement of the isolated object to the amplitude of the excitation displacement of the base:

$$T = \frac{{\parallel z\parallel {\kern 1pt} {\kern 1pt}_{\max } }}{{\parallel y\parallel_{{{\kern 1pt} {\kern 1pt} \max }} }} = \sqrt {1 + \frac{{b^{2} \omega^{4} }}{{\Lambda^{2} }} + \frac{{2b\omega^{2} }}{{\Lambda^{2} }}\left( { - b\gamma^{2} + 0.02b + \frac{{465b^{3} }}{40}} \right)} .$$
(30)

Here,

$$\parallel y\parallel {\kern 1pt}_{\max } = \sqrt {\frac{{\Lambda^{2} }}{{\omega^{4} }}} ,$$
$$\parallel z\parallel {\kern 1pt}_{\max } = \sqrt {b^{2} + \frac{{\Lambda^{2} }}{{\omega^{4} }} + \frac{2b}{{\omega^{2} }}\left( { - b\gamma^{2} + 0.02b + \frac{{465b^{3} }}{40}} \right)} .$$

The displacement transmissibility of the vibration isolation system under different excitation amplitudes is shown in Fig. 7a. Other parameters are set to \({\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} \omega = 1\),\({\kern 1pt} {\kern 1pt} \xi = 0.15\). With the increase of excitation amplitude \(\Lambda\), the peak value of transmissibility shifts to the right, and the peak value gradually increases. The onset frequency at which the isolator begins to isolate vibration has also increased. For example, when \({\kern 1pt} {\kern 1pt} \Lambda {\kern 1pt} {\kern 1pt}\) increases from \({\kern 1pt} {\kern 1pt} \Lambda { = }0.01{\kern 1pt} {\kern 1pt}\) to \({\kern 1pt} {\kern 1pt} \Lambda { = }0.04\), the starting frequency increases from 0.21 to 0.48, which means that the vibration isolation bandwidth becomes narrower. Furthermore, an increase in the excitation amplitude leads to a decrease in the vibration attenuation amplitude even in the isolation zone.

Fig. 7
figure 7

Displacement transmissibility under different conditions

Full size image

Figure 7b shows the displacement transfer rate under different damping ratios when the excitation amplitude \({\kern 1pt} {\kern 1pt} \Lambda {\kern 1pt} {\kern 1pt}\) is 0.04. Increasing the damping ratio significantly reduces the peak transmissibility and suppresses the frequency jump phenomenon. But increasing the damping ratio \({\kern 1pt} {\kern 1pt} \xi {\kern 1pt} {\kern 1pt}\) will reduce the vibration isolation performance of high-frequency vibration. Therefore, the consideration of damping in the vibration isolation system should be determined according to the actual working environment

Simulation Analysis of Vibration Isolation Effect

The relevant kinematic pair constraint information is added to the simulation software and information such as rod density is set according to the optimized quality parameters. The dynamic and static friction factors of the Coulomb friction damping of the rotating pair are set to 0.012 and 0.02, respectively. When the disturbance input \({\kern 1pt} {\kern 1pt} \Lambda \cos (\omega {\kern 1pt} {\kern 1pt} t)\), the simulation results are shown in Fig. 8.

Fig. 8
figure 8

The absolute displacement of the working platform and base when the vibration isolator is excited periodically at different frequencies: a \({\kern 1pt} {\kern 1pt} \omega = 8{\kern 1pt} {\kern 1pt} \pi\), b \({\kern 1pt} {\kern 1pt} \omega = 12{\kern 1pt} \pi\), c \({\kern 1pt} {\kern 1pt} \omega = 16{\kern 1pt} {\kern 1pt} \pi {\kern 1pt} {\kern 1pt}\) when \({\kern 1pt} {\kern 1pt} \Lambda = 0.02\;{\text{m}}\)

Full size image

It can be seen from Fig. 8a that the vibration isolator has good vibration isolation effect under different load masses under the disturbance of the external simple harmonic excitation frequency of 4 Hz, and the greater the mass, the better the vibration isolation effect. It can be seen from Fig. 8b that the vibration amplitude of the vibration isolator is attenuated by 90.2% under the external harmonic excitation frequency of 8 Hz. When the mass of the vibration isolator increases exponentially, the simulation results under the three conditions show that the vibration isolation attenuation does not change proportionally, and the difference in the simulation results is small, which indicates that the vibration isolation performance of the vibration isolator has little correlation with the load mass. Therefore, the vibration isolator can meet the low-frequency horizontal vibration isolation requirements with large external disturbance amplitude.

Experimental Validation

In this section, an experimental model is established to test the force–displacement relationship corresponding to loads of different masses. In addition, the dynamic test is performed to evaluate the vibration isolation performance of the dual four-rod horizontal isolators.

Resilience Test

As shown in Fig. 9, the experimental prototype of the vibration isolator is placed on the horizontal platform, and multiple groups of loads with different masses are selected and fixed on the working platform of the vibration isolator. The tension sensor is used to measure the horizontal restoring force of the load, one end of which is connected to the center of the working platform of the vibration isolator and the other end is fixed on the horizontal platform. The initial position of the load is selected at 0.04 m on the negative axis of the abscissa, and a total of 20 data points are selected within (− 0.04 m, 0.04 m), and the values of the restoring force and corresponding displacement are recorded and the experimental data are plotted in a point diagram. As shown in Fig. 10, the experimental data is in good agreement with the theoretical data, indicating that the experimental prototype of the vibration isolator satisfies the QZS characteristic near the static equilibrium position.

Fig. 9
figure 9

Resilience test. (1) Digital tension gauge (XDS-10), (2) load, (3) working platform, (4) intelligent-L laser sensor (IL-100)

Full size image
Fig. 10
figure 10

Force–displacement diagrams of theoretical and experimental results under different loads: a 5 kg, b 10 kg, c 15 kg

Full size image

Transmissibility Experiment

In order to further verify the frequency response characteristics and transmissibility of the vibration isolator, and evaluate the accuracy of theoretical analysis and dynamic simulation results, this section conducts vibration test experiments on the vibration isolator. The vibration isolation performance test system is shown in Fig. 11. The drive controller (CMba2B04N0N800WNNNYN) outputs control signals to control the movement of the vibration table (CDL40001-00). Two acceleration sensors (AI050) are, respectively, fixed on the base of the vibration isolator and the working platform, one is used to measure the excitation acceleration, and the other is used to measure the response acceleration of the load. A dynamic signal analyzer (JM5936) simultaneously collects signals from two accelerometers and transmits them to a computer (Dell).

Fig. 11
figure 11

Dynamics experiment in which: (1) experimental model of shock isolator, (2) load, (3) ACS motion control, (4) shaking table, (5) acceleration sensor, (6) laser range finder (IL-100), (7) dynamic analyzer, (8) computer

Full size image

The frequency of periodic excitation ranges from 4 to 10 Hz, and the amplitude of each periodic displacement excitation is set to 0.02 m. When the excitation frequencies are, respectively, 4 Hz, 6 Hz, 8 Hz and 10 Hz, the time-domain acceleration results of the base and the working platform are shown in Fig. 12. The acceleration of the base is less than that of the working platform, which means that the vibration of the base is effectively attenuated. The greater the excitation frequency, the greater the attenuation is. Acceleration transmissibility is defined by the ratio of the acceleration amplitude of the working platform to that of the base. For example, when the excitation frequency is 8 Hz, the acceleration of the base of the isolator is 50.52 mm/s2, the acceleration of the working platform is 6.1 mm/s2, and the vibration of the base is attenuated by about 87.9%. The change of acceleration transitivity with excitation frequency under periodic excitation is shown in Fig. 13. The vibration isolator starts to work at \({\kern 1pt} {\kern 1pt} \omega {\kern 1pt} {\kern 1pt} { = 2}{\text{.1}}{\kern 1pt}\) Hz, and with the increase in frequency, the isolation effect becomes more and more obvious. In addition, the experimental transfer rate is in good agreement with the theoretical transfer rate.

Fig. 12
figure 12

Acceleration of platform and base under periodic excitation with different frequencies: a 4 Hz, b 6 Hz, c 8 Hz, d 10 Hz

Full size image
Fig. 13
figure 13

Acceleration transfer rate of the isolator under excitation with a periodic frequency of 1–10 Hz

Full size image

Conclusions

A new dual four-rod horizontal large-amplitude QZS vibration isolator is proposed, which draws on the structural singularity of planar linkage mechanism under special configuration. The vibration isolation performance is improved by realizing the QZS characteristic in the static equilibrium position, and the QZS range is expanded by parameter optimization design. The static analysis of the vibration isolator is carried out. By optimizing the rod length parameters, the motion trajectory of the vibration isolator near the equilibrium position is horizontal quasi-straight line, and the position of the equivalent centroid is stable. After optimization, the stiffness curve increases the QZS range, and the dynamic stiffness is lower in the whole vibration isolation range. Through the analysis of harmonic excitation and transmission rate, the results show that the isolator has lower resonance peak value, lower initial isolation frequency and wider isolation bandwidth. The physical prototype of the isolator is made and the experimental platform is built. The isolation performance of the isolator is evaluated through the isolation performance tests under different frequency external excitation. The results show that the isolator can effectively isolate the external excitation greater than 3 Hz.