Introduction

Modern research focuses on examining nonlinear events across various media and disciplines. There is a growing interest in studying the nonlinear solitary waves in plasmas under diverse physical conditions. Solitons are a stunning and magnificent example of how nonlinear structures appear in nature in both magnetized and unmagnetized plasmas. The solitary wave is an intriguing feature explored experimentally in the atmosphere on earth and in the space laboratory for research. In plasmas made up of some ion species, such as negative ions, electrons, positrons, ion–electron beams, etc., the production of solitary waves is studied using simple or complex models. Initially, solitary waves in plasmas were explored by Korteweg and de Vries [1] and Washimi and Taniuti [2] using basic models. Korteweg-de Vries (KdV) first used the reductive perturbation approach to characterize small amplitude solitary waves. Also, Sagdeev [3] investigated finite but large amplitude solitary waves using the pseudopotential method with full nonlinearity.

The idea of relativistic effects, first proposed by Synge [4] for gases, is also widely used in plasmas. When particle velocities are significantly smaller than the speed of light \(\left( {v < < c} \right)\) but not negligible, the relativistic effect in plasma is considered weakly relativistic. In addition, plasma is regarded as strongly relativistic when the particle velocities are a significant fraction of the speed of light \(\left( {v \approx c} \right)\) or are highly relativistic \(\left( {v > c} \right)\). Many researchers have looked into the possibility of ion-acoustic solitary waves (IASWs) in relativistic plasmas under various physical conditions, including Das and Paul [5], Nejoh [6,7,8], Das et al. [9], Pakira et al. [10], Kuehl and Zhang [11], Malik et al. [12], Chatterjee and Roychoudhury [13], El-Labany and Shaaban [14], Kalita et al. [15], Roychoudhury et al. [16], Singh et al. [17], Gill et al. [18], and Kalita et al. [19, 20]. However, these studies use the relativistic effect and are conducted in magnetized and unmagnetized plasmas. Their properties are said to be substantially impacted by the relativistic effect, ion temperature, and cold-to-hot electron temperature ratio. Since Das and Paul’s original study [5] on the presence of small amplitude IASWs in a weakly relativistic two-fluid plasma made up of massless hot electrons and drifting cold ions, numerous researchers have started into related topics under different conditions in relativistic multi-component plasmas. For instance, KdV-type equations [7, 17, 21] and the Sagdeev pseudopotential approach [22,23,24,25,26] were used to explore the effects of ion temperature and electron inertia on IASWs.

Numerous authors, including Sah and Goswami [27, 28], have also examined the propagation of semirelativistic electron acoustic solitary waves (EASWs). Sahu and Roychoudhary [29] have studied the EASWs in non-magnetized plasma containing ions, hot relativistic electrons, cold relativistic electrons, and relativistic beams. The relativistic effect is shown to limit the region of existence of solitons in the occurrence of relativistic electron beam plasma, utilizing a vortex-like distribution of trapped electrons. Here, \(u_{0e}\) is the initial electron streaming speed, and solitons stop existing when \(u_{0e} /c\) crosses a particular limit. The role of electron inertia is typically disregarded in many relativistic or non-relativistic studies. However, Kalita et al. [30] have considered the effect of electron inertia with the drifting effect in a non-relativistic plasma where the electron’s drift velocity \(v^{\prime}_{e}\) is determined to satisfy \(v^{\prime}_{e} < \,44.72 + M/k_{z}\), (M-Mach number and \(k_{z}\) direction of propagation). In a warm magnetoplasma with initial electron drift motion in the magnetic field, Kalita and Bhatta [31] have explored IASWs. The existence of hump and dip solitons in the parametric domains has been demonstrated. Alternatively, Kuehl and Zhang [11] have considered first the effect of electron inertia in relativistic plasma.

Furthermore, Malik et al. [12] have thought about ion-acoustic solitons in a relativistic plasma of non-drifting electrons and drifting ions at a limited temperature. Due to electron inertia and a limited ion temperature, the ion drift velocity \(u_{0}\) for the weak relativistic effect is restricted. Lee and Choi [32] studied the IASW in a relativistic plasma containing cold ions and hot electrons using totally relativistic two-fluid equations. A two-dimensional ion-acoustic wave propagating obliquely across a dusty plasma with a two-ion-temperature plasma is also governed by the variable-coefficient Zakharov–Kuznetsov equation, which Qu et al. [33] have studied symbolically. During a magnetized ion-beam plasma, IASWs may be generated by positive beam ions, static warm ions, and normal electrons. Additionally, Das [34] has examined similar behaviour in ion-beam plasma. Kalita and Deka [35] have explored hump solitons with low and high amplitudes in a weakly relativistic and magnetized plasma model. Using non-thermal collisional dusty plasma, Sultana [36] has studied the non-Maxwellian j-distributed electrons propagating IASWs. Kamalam and Ghosh [37] have analyzed a plasma model made up of two electrons and warm fluid ions at various temperatures using the Sagdeev pseudopotential approach in the Boltzmann distribution. The Sagdeev pseudopotential approach was used by X. Mushinzimana and F. Nsengiyumva [38] to explore the large amplitude ion-acoustic fast mode solitary waves in a negative ion plasma with kappa electrons. They found a range of parameter values where the two different types of structures can coexist, supporting compressive and rarefactive solitons propagation in this plasma. To explore the non-linear propagation of static large amplitude electromagnetic solitary waves in a magnetized electron–positron plasma, Nooralishahi and Salem [39] adopted the completely relativistic two-fluid hydrodynamic model. Kalita et al. [40] have recently shown that both hump and dip subsonic solitary waves exist based on wave velocities in different propagation directions. Very recently, Almas et al. [41] employed the pseudopotential technique to study the oblique propagation of arbitrary IASWs in magnetized electron–positron-ion plasmas. They have investigated how different plasma configuration parameters, like positron concentration and parallel and perpendicular ion pressure, affect soliton characteristics in the plasma system.

In the present paper, the authors study the non linear properties of IASWs using the Sagdeev potential method by considering the plasma system with relativistic effects on electrons. Non-thermal ions, however, are non-relativistic.

Dynamics of the Motion and Derivation of Sagdeev Potential

Relativistic effects become prominent and alter the nonlinear behaviour of plasmas as electron or ion velocities \(v_{e,i}\) go close to the speed of light \(c\). Due to their low mass, electrons can reach relativistic speeds much more easily than heavier ions. In contrast, relativistic effects are only included in the equations of motion of the electron at a constant temperature \(T_{e}\), not in the equations of motion of the ions. The governing equations in the zx-plane are

$$ \frac{{\partial n_{i} }}{\partial t} + \frac{\partial }{\partial x}\left( {n_{i} v_{ix} } \right) + \frac{\partial }{\partial z}\left( {n_{i} v_{iz} } \right) = 0 $$
(1)
$$ \left( {\frac{\partial }{\partial t} + v_{ix} \frac{\partial }{\partial x} + v_{iz} \frac{\partial }{\partial z}} \right)v_{ix} = - \frac{\partial \phi }{{\partial x}} + v_{iy} $$
(2)
$$ \left( {\frac{\partial }{\partial t} + v_{ix} \frac{\partial }{\partial x} + v_{iz} \frac{\partial }{\partial z}} \right)v_{iy} = - v_{ix} $$
(3)
$$ \left( {\frac{\partial }{\partial t} + v_{ix} \frac{\partial }{\partial x} + v_{iz} \frac{\partial }{\partial z}} \right)v_{iz} = - \frac{\partial \phi }{{\partial z}} $$
(4)

for the ions and

$$ \frac{{\partial n_{e} }}{\partial t} + \frac{\partial }{\partial z}\left( {n_{e} v_{ez} } \right) = 0 $$
(5)
$$ \left( {\frac{\partial }{\partial t} + v_{ez} \frac{\partial }{\partial z}} \right)\gamma_{ez} v_{ez} = \frac{1}{Q}\left( {\frac{\partial \phi }{{\partial z}} - \frac{1}{{n_{e} }}\frac{{\partial n_{e} }}{\partial z}} \right) $$
(6)

for the electrons, where \(\gamma_{ez} = \left\{ {1 - \left( {v_{ez} /c} \right)^{2} } \right\}^{ - 1/2} = 1 + (v_{ez}^{2} /2c^{2} )\) and \(c\) is the speed of light and \(Q\,\left( { = m_{e} /m_{i} } \right)\) is the electron to ion mass ratio. To obtain the set of Eqs. (1) to (6), we normalised the densities using the unperturbed plasma density \(n_{0}\), time using the reciprocal of the ion gyro-frequency \(\Omega_{i}\), space using the ion gyro-radius \(\rho_{s} = C_{s} /\Omega_{i}\), speed using \(C_{s} [ = (T_{e} /m_{i} )^{1/2} ]\), and potential by \(T_{e} /e\).

For a static solution, we consider a frame travelling with the wave defined by

$$ \xi = k_{x} x + k_{z} z - Mt $$
(7)

where \(M =\) Mach number (\(= V/C_{s} =\) pulse speed / ion sound speed), and the direction cosines \(k_{x} ( = \cos \theta )\) and \(k_{z} ( = \sin \theta )\) such that \(k_{x}^{2} + k_{z}^{2} = 1\). For the moving coordinate \(\xi\), we can write down from (7)

$$ \frac{\partial }{\partial x} = k_{x} \frac{\partial }{\partial \xi },\;\frac{\partial }{\partial z} = k_{z} \frac{\partial }{\partial \xi },\;\frac{\partial }{\partial t} = - M\frac{\partial }{\partial \xi } $$

Introducing the additional coordinate \(\xi\) specified in (7), and utilizing the boundary conditions \(v_{ix} = v_{iz} = 0\) at \(n_{i} = 1\) as \(\left| \xi \right| \to \infty\), after integration, Eq. (1) becomes

$$ k_{x} v_{ix} + k_{z} v_{iz} = M\left( {1 - \frac{1}{{n_{i} }}} \right) $$
(8)

Using (7) and (8), Eqs. (2) to (4) can be simplified as

$$ \frac{M}{{n_{i} }}\frac{{\partial v_{ix} }}{\partial \xi } = k_{x} \frac{\partial \phi }{{\partial \xi }} - v_{iy} $$
(9)
$$ \frac{M}{{n_{i} }}\frac{{\partial v_{iy} }}{\partial \xi } = v_{ix} $$
(10)
$$ \frac{M}{{n_{i} }}\frac{{\partial v_{iz} }}{\partial \xi } = k_{z} \frac{\partial \phi }{{\partial \xi }} $$
(11)

Using (7) in (5) and then integrating, we obtain

$$ v_{ez} = \frac{M}{{k_{z} }}\left( {1 - \frac{1}{{n_{e} }}} \right) $$
(12)

In deriving Eq. (12), we employed the boundary conditions \(v_{ez} = 0\) and \(n_{e} = 1\) as \(\left| \xi \right| \to \infty\).

Using the coordinate \(\xi\) and Eq. (12), integrating Eq. (6) once to give the boundary conditions \(\varphi = 0\) at \(n_{e} = 1\) as \(\left| \xi \right| \to \infty\)

$$ n_{e} = e^{\phi } \exp \left[ {\frac{{QM^{2} }}{{2k_{z}^{2} }}\left( {1 - \frac{1}{{n_{e}^{2} }}} \right) + \frac{{QM^{4} }}{{8c^{2} k_{z}^{4} }}\left( {1 - \frac{1}{{n_{e} }}} \right)^{3} \left( {1 + \frac{3}{{n_{e} }}} \right)} \right] $$
(13)

Making use of (13) and the charge neutrality condition \(n_{e} = n_{i} = n\), Eq. (11) can be integrated to acquire

$$ v_{iz} = \frac{{k_{z} }}{M}\left( {n - 1} \right)\left\{ {1 - \frac{{QM^{2} }}{{k_{z}^{2} }}.\frac{1}{n} - \frac{{QM^{4} }}{{2c^{2} k_{z}^{4} }}.\frac{{\left( {n - 1} \right)^{2} }}{{n^{3} }}} \right\} $$
(14)

With the use of (14), we can get from (8)

$$ v_{ix} = \frac{M}{{k_{x} }}\left( {n - 1} \right)\left[ {\frac{1}{n} - \frac{{k_{z}^{2} }}{{M^{2} }}\left\{ {1 - \frac{{QM^{2} }}{{k_{z}^{2} }}.\frac{1}{n} - \frac{{QM^{4} }}{{2c^{2} k_{z}^{4} }}.\frac{{\left( {n - 1} \right)^{2} }}{{n^{3} }}} \right\}} \right] $$
(15)

After entering the value \(v_{ix}\) from (15) into (9), \(v_{iy}\) may be evaluated as

$$ v_{iy} = f\left( n \right)\frac{1}{n}\frac{dn}{{d\xi }} $$
(16)

where \(f\left( n \right) = T_{1} + \frac{{T_{2} }}{{n^{2} }} + \frac{{T_{3} }}{{n^{3} }} + \frac{{T_{4} }}{{n^{4} }}\) with

$$ T_{1} = k_{x} + \frac{{k_{z}^{2} }}{{k_{x} }} $$
$$ T_{2} = - \left\{ {\frac{{M^{2} Qk_{x} }}{{k_{z}^{2} }} + \frac{{3M^{4} Qk_{x} }}{{2c^{2} k_{z}^{4} }} + \frac{{M^{2} \left( {1 + Q} \right)}}{{k_{x} }} + \frac{{3QM^{4} }}{{2c^{2} k_{z}^{2} k_{x} }}} \right\} $$
$$ T_{3} = \frac{{3M^{4} Qk_{x} }}{{c^{2} k_{z}^{4} }} + \frac{{3QM^{4} }}{{c^{2} k_{z}^{2} k_{x} }} $$
$$ T_{4} = - \left\{ {\frac{{3M^{4} Qk_{x} }}{{2c^{2} k_{z}^{4} }} + \frac{{3QM^{4} }}{{2c^{2} k_{z}^{2} k_{x} }}} \right\} $$

In order to get (16), we have to use Eq. (13). From (10), one may get the following expression using the values of \(v_{ix}\) and \(v_{iy}\) from (15) and (16), respectively

$$ \frac{d}{d\xi }\left\{ {f\left( n \right)\frac{1}{n}\frac{dn}{{d\xi }}} \right\} = \frac{1}{{k_{x} }}\left( {n - 1} \right)\left\{ {1 + Q - \frac{{k_{z}^{2} }}{{M^{2} }}.\,n + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}.\,\left( {1 - \frac{1}{n}} \right)^{2} } \right\} $$
(17)

Equation (17) is multiplied by the term in the parenthesis, which is then inserted into the integration process to get the following energy integral involving the Sagdeev potential \(\psi\)

$$ \frac{1}{2}\left( {\frac{dn}{{d\xi }}} \right)^{2} + \psi \left( {n,M,k_{z} } \right) = 0 $$
(18)

where

$$ \psi \left( {n,M,k_{z} } \right) = g\left( n \right)h\left( n \right) $$
(19)

with

$$ g\left( n \right) = \frac{{n^{2} }}{{\left\{ {f\left( n \right)} \right\}^{2} }} $$
(20)

and

$$ \begin{gathered} h\left( n \right) = - \frac{1}{{k_{x} }}\left[ {\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} } \right\}\left( {n - 1} \right) - \left( {\frac{{T_{1} k_{z}^{2} }}{{2M^{2} }}} \right)} \right.\left( {n^{2} - 1} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \left\{ {\frac{{T_{2} k_{z}^{2} }}{{M^{2} }} + \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} } \right\}\log n \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{3} k_{z}^{2} }}{{M^{2} }}} \right\}\left( {\frac{1}{n} - 1} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \frac{1}{2}\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{4} k_{z}^{2} }}{{M^{2} }}} \right\}\left( {\frac{1}{{n^{2} }} - 1} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \frac{1}{3}\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\left( {\frac{1}{{n^{3} }} - 1} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, + \frac{1}{4}\left\{ {\left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\left( {\frac{1}{{n^{4} }} - 1} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \frac{1}{5}\left\{ {\left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} } \right\}\left( {\frac{1}{{n^{5} }} - 1} \right) + \left. {\left( {\frac{{T_{4} QM^{2} }}{{12c^{2} k_{z}^{2} }}} \right)\left( {\frac{1}{{n^{6} }} - 1} \right)} \right] \, \hfill \\ \end{gathered} $$
(21)

and we have used the boundary condition \(\frac{dn}{{d\xi }} = 0\) at \(n = 1\).

Conditions for Solitary Waves to Occur

The characteristics of \(\psi \left( n \right)\) around \(n = 1\) and \(n = N\), where \(N\) is the highest value of \(n\) or the solitary wave pulse’s amplitude, can be used to determine the prerequisites for the existence of localized solitary wave solutions. We are to set the nonlinear dispersion relation \(\psi \left( N \right) = 0\) to obtain the solitary wave pulse’s amplitude “\(N\)” such that

$$ \begin{gathered} \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} } \right\}\left( {N - 1} \right) - \left( {\frac{{T_{1} k_{z}^{2} }}{{2M^{2} }}} \right)\left( {N^{2} - 1} \right)\, - \left\{ {\frac{{T_{2} k_{z}^{2} }}{{M^{2} }} + \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} } \right\}\log N \hfill \\ - \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{3} k_{z}^{2} }}{{M^{2} }}} \right\}\left( {\frac{1}{N} - 1} \right) \hfill \\ - \frac{1}{2}\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{4} k_{z}^{2} }}{{M^{2} }}} \right\}\left( {\frac{1}{{N^{2} }} - 1} \right) \hfill \\ - \frac{1}{3}\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\left( {\frac{1}{{N^{3} }} - 1} \right) \hfill \\ + \frac{1}{4}\left\{ {\left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\left( {\frac{1}{{N^{4} }} - 1} \right) \hfill \\ - \frac{1}{5}\left\{ {\left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} } \right\}\left( {\frac{1}{{N^{5} }} - 1} \right) + \left( {\frac{{T_{4} QM^{2} }}{{12c^{2} k_{z}^{2} }}} \right)\left( {\frac{1}{{N^{6} }} - 1} \right)\, = \,{0 } \hfill \\ \end{gathered} $$
(22)

In addition, the following conditions must exist for solitary waves to exist

$$ \psi \left( 1 \right) = \psi \left( N \right) = \psi^{\prime}\left( 1 \right) = 0 $$
(23)

and

$$ \psi \left( n \right) < 0 $$
(24)

between \(n = 1\) and \(n = N\). Now, to determine the mathematical requirements, we take into consideration

$$ \begin{aligned} h^{\prime}\left( n \right) &= - \frac{1}{{k_{x} }}\left[ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \left( {\frac{{T_{1} k_{z}^{2} }}{{M^{2} }}} \right)} \right.n\, - \left\{ {\frac{{T_{2} k_{z}^{2} }}{{M^{2} }} + \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} } \right\}\frac{1}{n} \\ & \quad + \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{3} k_{z}^{2} }}{{M^{2} }}} \right\}\frac{1}{{n^{2} }}\\ &\quad + \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{4} k_{z}^{2} }}{{M^{2} }}} \right\}\frac{1}{{n^{3} }} \\ &\quad + \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\frac{1}{{n^{4} }} \\ &\quad - \left\{ {\left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\frac{1}{{n^{5} }} \\ &\quad + \left\{ {\left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} } \right\}\frac{1}{{n^{6} }} - \left. {\left( {\frac{{T_{4} QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)\frac{1}{{n^{7} }}} \right]\end{aligned} $$
(25)

and

$$ \begin{gathered} h^{\prime\prime}\left( n \right) = - \frac{1}{{k_{x} }}\left[ { - \frac{{T_{1} k_{z}^{2} }}{{M^{2} }}} \right.\, + \left\{ {\frac{{T_{2} k_{z}^{2} }}{{M^{2} }} + \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} } \right\}\frac{1}{{n^{2} }} \hfill \\ \quad \quad \quad - 2\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{3} k_{z}^{2} }}{{M^{2} }}} \right\}\frac{1}{{n^{3} }} \hfill \\ \hfill \\ \quad \quad \quad - 3\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{1} - \frac{{T_{4} k_{z}^{2} }}{{M^{2} }}} \right\}\frac{1}{{n^{4} }} \hfill \\ \hfill \\ \quad \quad \quad - 4\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\frac{1}{{n^{5} }} \hfill \\ \hfill \\ \quad \quad \quad + 5\left\{ {\left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} + \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{2} } \right\}\frac{1}{{n^{6} }} \hfill \\ \quad \quad \quad - 6\left\{ {\left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{4} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)T_{3} } \right\}\frac{1}{{n^{7} }} + 7\left. {\left( {\frac{{T_{4} QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)\frac{1}{{n^{8} }}} \right] \hfill \\ \end{gathered} $$
(26)

where \(h^{\prime}\left( n \right) = \frac{dh\left( n \right)}{{dn}}\) and \(h^{\prime\prime}\left( n \right) = \frac{{d^{2} h\left( n \right)}}{{dn^{2} }}\).

From Eqs. (21), (25) and (26) it can be seen that at \(n = 1\),

$$ h\left( 1 \right) = 0,\;h^{\prime}\left( 1 \right) = 0\;,h^{\prime\prime}\left( 1 \right) = \frac{{\left( {1 + Q - \frac{{k_{z}^{2} }}{{M^{2} }}} \right)\left( {Q + k_{z}^{2} - \frac{{k_{z}^{2} }}{{M^{2} }}} \right)M^{2} }}{{k_{z}^{2} k_{x}^{2} }} $$
(27)

By using these values and Eq. (20) at \(n = 1\), we obtain

$$ \psi \left( 1 \right) = 0,\;\psi^{\prime}\left( 1 \right) = 0,\;\psi^{\prime\prime}\left( 1 \right) = \frac{{k_{z}^{2} \left( {1 + Q - \frac{{k_{z}^{2} }}{{M^{2} }}} \right)}}{{M^{2} \left( {Q + k_{z}^{2} - \frac{{k_{z}^{2} }}{{M^{2} }}} \right)}} $$
(28)

The relation (22) is derived by taking \(\psi \left( N \right) = g\left( N \right)h\left( N \right) = 0\) for which \(h\left( N \right) = 0\), since \(g\left( N \right) \ne 0\) so that

$$ h^{\prime}\left( N \right) = - \frac{{\left( {N - 1} \right)}}{{k_{x} }}\left( {\frac{1 + Q}{N} - \frac{{k_{z}^{2} }}{{M^{2} }} + \frac{{QM^{2} (N - 1)^{2} }}{{2c^{2} k_{z}^{2} N^{3} }}} \right)\left[ { - \frac{{3QM^{4} \left( {N - 1} \right)^{2} }}{{2N^{4} c^{2} k_{z}^{4} k_{x} }} + \left. {\frac{{\left( {N^{2} - M^{2} } \right)\,k_{z}^{2} - QM^{2} }}{{N^{2} k_{x} k_{z}^{2} }}} \right]} \right. $$
(29-1)
$$ \psi^{\prime}\left( N \right) = \frac{{N^{3} \left( {N - 1} \right)\,\left\{ {2N^{2} c^{2} k_{z}^{2} M^{2} \left( {1 + Q} \right) - 2N^{3} c^{2} k_{z}^{4} + (N - 1)^{2} QM^{4} } \right\}\,k_{z}^{2} }}{{M^{2} \left[ {3QM^{4} \left( {N - 1} \right)^{2} - 2N^{2} c^{2} k_{z}^{2} \left\{ {\left( {N^{2} - M^{2} } \right)\,k_{z}^{2} - M^{2} Q} \right\}} \right]}} $$
(29-2)

The set of requirements (23) are met as a result of (28) and (22). However, by expanding \(\psi \left( n \right)\) in Taylor’s series near \(n \approx 1\) and \(n \approx N\), we have

$$ \psi \left( {n \approx 1} \right) = \,\psi \left( 1 \right) + \left( {n - 1} \right)\psi^{\prime}\left( 1 \right) + \frac{{\left( {n - 1} \right)^{2} }}{2!}\psi^{\prime\prime}\left( 1 \right) + \,\, \cdots $$

and

$$ \psi \left( {n \approx N} \right) = \,\psi \left( N \right) + \left( {n - N} \right)\psi^{\prime}\left( 1 \right) + \frac{{\left( {n - N} \right)^{2} }}{2!}\psi^{\prime\prime}\left( N \right) + \,\,\, \cdots $$

With the help of (28), (22), and (29), it is found

$$ \psi \left( {n \approx 1} \right) = \frac{{\left( {n - 1} \right)^{2} k_{z}^{2} \left( {1 + Q - \frac{{k_{z}^{2} }}{{M^{2} }}} \right)}}{{2M^{2} \left( {Q + k_{z}^{2} - \frac{{k_{z}^{2} }}{{M^{2} }}} \right)}} $$
(30)

This can be precisely reduced to the work of Kalita et al. [30] for \(v^{\prime}_{e} = 0\) in the non-relativistic situation. Since there is no early streaming, there is no relativistic influence when everything is in equilibrium and \(n = 1\), and therefore, the aforementioned requirement is acceptable.

Furthermore

$$ \psi \left( {n \approx N} \right)\, = \,\frac{{N^{3} \left( {N - 1} \right)\left( {n - N} \right)\left\{ {2N^{2} c^{2} k_{z}^{2} M^{2} \left( {1 + Q} \right) - 2N^{3} c^{2} k_{z}^{4} + \left( {N - 1} \right)^{2} QM^{4} } \right\}\,k_{z}^{2} }}{{M^{2} \left[ {3QM^{4} \left( {N - 1} \right)^{2} - 2N^{2} c^{2} k_{z}^{2} \left\{ {\left( {N^{2} - M^{2} } \right)\,k_{z}^{2} - M^{2} Q} \right\}} \right]}} $$
(31)

Finally, the following requirements can be deduced from (30) and (31) for \(\psi \left( n \right) < 0\) between \(n = 1\) and \(n = N\) to describe solitary waves.

near

$$ n = 1,\;1 + Q > \frac{{k_{z}^{2} }}{{M^{2} }} > Q + k_{z}^{2} $$
(32)

near

$$ n = N,\;N > \frac{{M^{2} }}{{k_{z} }},\,\,N < M < 1\;{\text{when}}\;N < 1 $$
(33)

and

$$ N > \frac{{M^{2} }}{{k_{z}^{2} }},\,\,N > M > 1,\;{\text{when}}\,N > 1 $$
(34)

In order to determine the amplitudes of relevant solitons, we must assign proper values to the parameters \(M\) and \(k_{z}\) subject to the constraints (32)–(34). Using these values of the amplitudes, the Sagdeev potential \(\psi \left( n \right)\) from (19) can be displayed to represent the soliton characters including its width \(\Delta\)\(\left( {\Delta = N/\sqrt d } \right)\), \(d\) being its depth for each set of assigned values of \(M\) and \(k_{z}\) for the determination of \(N\).

Results and Discussion

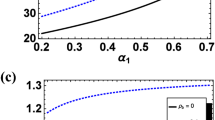
In this investigation it is important to note that solitary waves can be observed in both the situations for \(M < 1\) and \(M > 1\). IASWs with density dip (rarefactive) \(\left( {N < 1} \right)\) are observed when the relativistic effect on electrons in a cold plasma is taken into account. Depending on the particular circumstances and characteristics of the plasma, rarefactive (dip) \(\left( {N < 1} \right)\) and compressive (hump) \(\left( {N > 1} \right)\) solitons can occur in several types of plasmas. The amplitudes (Fig. 1) of the dip soliton are found to increase parabolically with \(k_{z}\) for all \(M = 0.60\) (Blue), \(0.65\) (Red), and \(0.70\) (Yellow). But for \(M > 1\), the amplitude of the dip solitons is noticed to increase rapidly in the lower range of \(k_{z}\) and then decreases slowly in the upper portion of \(k_{z}\) for all \(M = 1.10\) (Blue), \(1.15\) (Red) and \(1.20\) (Yellow) (Fig. 2). Additionally, in Fig. 2, it is seen that the magnitudes of the amplitudes are observed to be higher in comparison to Fig. 1. Figure 3 shows that the amplitudes of the relativistic solitons are found to increase linearly with \(M < 1\) for \(k_{z} = 0.06\)(Blue), \(0.08\)(Red) and \(0.10\) (Yellow). Further, the amplitude of the solitons decreases with the increased value of \(k_{z}\). On the other hand, the widths of the corresponding solitons (Fig. 4) are seen to decrease quickly with \(M < 1\) for different values of \(k_{z} = 0.06\)(Blue), \(0.08\)(Red) and \(0.10\)(Yellow). In this case the magnitude of the widths are higher for higher value of \(k_{z}\). The amplitudes (Fig. 5) of the supersonic dip solitons increase rapidly in the narrow lower regime of \(M > 1\), showing slight declining trend in the upper regime of \(M > 1\) for \(k_{z} = 0.10\)(Blue), \(0.20\)(Red) and \(0.30\)(Yellow). It is evident that as the amplitude grows with the increases of Mach number while the width contracts. The solitons exhibits with a bigger amplitude in the case of smaller \(k_{z}\) because the solitons speed and amplitude are directly correlated, otherwise higher amplitude solitons is noticed away from the magnrtic field. The depth of the Sagdeev pseudopotential \(\psi \left( N \right)\) considerably decreases with soliton amplitude when deviation from the direction of the magnetic field given by \(k_{z} = 0.15\)(Blue), \(0.16\)(Red), \(0.17\)(Yellow) and \(k_{z} = 0.77\)(Blue), \(0.78\)(Red), \(0.79\)(Yellow) (Figs. 6, 7) decreases for fixed \(M\, = 0.20\,( < 1)\) and \(M\, = 1.01\,( > 1)\) respectively. Figures 1b, 2b, 3b, 4b, 5b, 6b and 7b are respectively the demonstration of three dimensional views of 1a, 2a, 3a, 4a, 5a, 6a and 7a. In the investigation of Kalita et al. [40], they have reported that both hump and dip solitons are shown to exist only for \(M < 1\). But, due to consideration of relativistic effects on electrons, only dip soliton appear to exist for both the situations when \(M < 1\) and \(M > 1\), which is a new finding from the ongoing investigation. Nonlinearity is also a fundamental aspect of plasma waves induced by the collective behavior of charged particles and their interactions with electromagnetic fields. Finally, this research ought to be useful in understanding the key characteristics of fully nonlinear IASWs in both space and lab studies involving ions and relativistic thermal electrons.

Fig. 1
figure 1

Amplitudes of the subsonic dip soliton with \(k_{z}\) for \(M = 0.60\) (Blue), \(0.65\) (Red) and \(0.70\) (Yellow) (colour figure online)

Full size image
Fig. 2
figure 2

Amplitudes of the supersonic dip soliton with \(k_{z}\) for \(M = 1.10\) (Blue), \(1.15\) (Red) and \(1.20\) (Yellow) (colour figure online)

Full size image
Fig. 3
figure 3

Amplitudes of the subsonic dip soliton with \(M\) for \(k_{z} = 0.06\) (Blue), \(0.08\) (Red) and \(0.10\) (Yellow) (colour figure online)

Full size image
Fig. 4
figure 4

Variation of widths versus \(M\) for \(k_{z} = 0.06\) (Blue), \(0.08\) (Red) and \(0.10\) (Yellow) (colour figure online)

Full size image
Fig. 5
figure 5

Amplitudes of the supersonic dip soliton with \(M\) for \(k_{z} = 0.10\) (Blue), \(0.20\) (Red) and \(0.30\) (Yellow) (colour figure online)

Full size image
Fig. 6
figure 6

The variation of Sagdeev potential \(\psi \left( N \right)\) vs. \(N\) for \(k_{z} = 0.15\) (Blue), \(0.16\) (Red) and \(0.17\) (Yellow) when \(M\, = \,0.20\) (colour figure online)

Full size image
Fig. 7
figure 7

The variation of Sagdeev potential \(\psi \left( N \right)\) vs. \(N\) for \(k_{z} = 0.77\) (Blue), \(0.78\) (Red) and \(0.79\) (Yellow) when \(M\, = \,1.01\) (colour figure online)

Full size image

Conclusion

IASWs play a crucial role in comprehending space and laboratory plasmas. This study explored the propagation of IASWs in the presence of ions and relativistic electrons. Ions and relativistic electrons within magnetized plasmas are essential constituents of numerous space and astrophysical systems. Analyzing their behaviour and interactions with magnetic fields and other particles is of utmost importance in gaining insights into various phenomena, ranging from Earth’s space weather to the dynamics of distant astrophysical entities. Due to the inclusion of relativistic effects on electrons, only density dip \(\left( {N < 1} \right)\) IASWs existed for both subsonic \(\left( {M < 1} \right)\) and supersonic \(\left( {M > 1} \right)\) situations in the plasma model. It was observed that the amplitudes of the relativistic dip solitons are higher for \(M > 1\) and smaller for \(M < 1\). The present paper can be extended by considering the relativistic effects on both the species electrons and ions.