Introduction

With the rapid development of transportation infrastructure construction, the utilization rate of underground space has significantly increased. The route selection of railway tunnels will inevitably pass through existing buildings. Drilling and blasting are the main methods of mountain tunnel excavation, but the induced blasting vibration adversely affects the surrounding buildings. Therefore, the accurate prediction of rock blasting vibration has important engineering significance to ensure the safety of surrounding buildings (Zheng et al.2021; Alimohammadi et al. 2020; Satvati et al. 2020; Alimohammadi et al. 2019, Zhao et al.2021a).

Most researchers used the peak particle velocity (PPV) as an indicator and conducted research on the response characteristics of blasting vibration. Yu et al. (2021) used similar simulation experiments to study the effect of the joint weakness on the transmission of blasting vibration. The experimental results showed that the number of joints greatly affected the prediction accuracy of blasting vibration. Roy et al. (2020) studied the influence of the total charge on the intensity of blasting vibration. When the maximum charge is used as a fitting parameter, the prediction effect of blasting vibration is good. Zhao et al. (2021b) obtained a new fitting method for tunnel blasting vibration through induction and dimensional analysis. In addition, the analysis method was used to study the attenuation law of blasting vibration energy. A comparison of four prediction models of blasting vibration velocity shows that the prediction effects of the USBM model and Sadofsky equation are similar and can be widely used in subsequent prfediction work (Ongen et al. 2018). Lu et al. (2018) studied the influence of an airborne free surface on the attenuation law of blasting vibration using actual measurements and numerical analysis. In addition, a large number of on-site monitoring technology and experimental verification methods (Dong et al. 2021, 2017; Sengupta et al. 2021; Gao et al. 2019; Wang et al. 2021) have also been applied in the research for the tunnel engineering. Ji et al. (2021) made statistics on the damage characteristics of tunnel surrounding rock through acoustic wave tests, and proposed corresponding control thresholds. Zhong et al. (2012) used the method of combining dimensional analysis with wavelet packet analysis to study the vibration attenuation law of tunnel and open-air slope collaborative blasting.

In summary, most studies predict the attenuation law of blasting vibration through field measurements (Huang et al. 2019; Gou et al. 2020) or numerical simulation (Xu et al. 2019; Yang et al. 2020; Peng et al. 2021). However, there are few theoretical derivation studies on the attenuation law of blasting vibration. The theoretical derivation of blasting vibration is of great significance for comprehensively understanding the transmission of blasting stress waves and accurately predicting the evolution of blasting vibration. Therefore, based on the analytical solution of the wave equation excited by the short column charge, the attenuation model equation of the tunnel blasting vibration is derived. Secondly, the reliability of the improved formula is verified by dimensional analysis. Finally, the two charge expression forms of the two blasting vibration fittings are separately discussed, and the prediction effects of the three fitting methods are compared. The content of this research is dedicated to introducing a new working idea to predict and control blasting vibration in the future and proposes improvements and supplements to traditional prediction methods.

Theoretical analysis of the peak velocity attenuation of blasting vibration

At present, most researchers use the Sadofsky formula (Matidza et al. 2020; Zhao et al. 2021a) or the USBM model (United States Bureau of Mines) (Azimi et al. 2019; Hosseini et al. 2019; Shi et al. 2018; Jayasinghe et al. 2019; Arthur et al. 2020) to fit and analyze the blasting vibration velocity. In addition, other classic models have been used to predict the peak particle velocity.

This empirical formula only considers the influence of the distance from the blast area and the blasting charge on the PPV. It does not reflect the influence of other factors on the PPV, such as charge type, charge radius, drilling radius, blasthole layout, mechanical properties of the medium rock mass, and topographic and geological conditions (Hu et al. 2020; Jiang et al. 2017). Therefore, based on the analytical solution of the wave excited by the short column charge, the attenuation law of blasting vibration in the middle and far fields is studied.

Attenuation law of blasting vibration based on short column charge

When the distance from the blast area is greater than the length of the cylindrical charge and wavelength of the stress wave, Hustrulid et al. (1992) obtained an analytical solution for the low-frequency fluctuations of the short column cavity under instantaneous internal load excitation.

As shown in Fig. 1, assuming that the internal instantaneous excitation load acts on the inner wall of a short cylindrical cavity with a length of dH and a radius of rb, the displacement field can be expressed as:

$${u}_{p}=(\frac{{{r}_{b}}^{2}dH{p}_{0}}{4G{c}_{p}R})[1-(\frac{{c}_{s}}{{c}_{p}}){\mathrm{cos}}^{2}\theta ]\left\{\frac{\partial f[t-\frac{(H-{H}_{1})}{D}-\frac{R}{{c}_{p}}]}{\partial t}\right\}$$
(1)
$${u}_{s}=(\frac{{{r}_{b}}^{2}dH{p}_{0}\mathrm{sin}\theta \mathrm{cos}\theta }{2G{c}_{s}R})\left\{\frac{\partial f[t-\frac{(H-{H}_{1})}{D}-\frac{R}{{c}_{s}}]}{\partial t}\right\}$$
(2)

where up is the displacement caused by the compression wave; us is the displacement caused by the shear wave; rb is the radius of the charge; p0 is the initial pressure of the blasthole; G is the shear modulus of the rock; D is the propagation speed of the detonation wave; R is the distance between the vibration source and the measuring point; t is the time of action; H1 and H2 are the upper and lower limits of the calculation of H; cp and cs are the propagation velocities of the compression wave and shear wave, respectively.

Fig. 1
figure 1

Instantaneous load acts on the inner wall of the short column cavity with a length of dH

Full size image

Then, the radial displacement and vertical displacement excited by the explosion of the short column charge can be expressed as

$${u}_{r}={u}_{p}\mathrm{sin}\theta +{u}_{s}\mathrm{cos}\theta$$
(3)
$${u}_{z}=-{u}_{p}\mathrm{cos}\theta +{u}_{s}\mathrm{sin}\theta$$
(4)

where ur and uz are the radial vertical displacement excited by the instantaneous load.

The velocity field excited by the instantaneous load can be obtained by differential calculation:

$$\left\{\begin{array}{c}{V}_{r}=\frac{\partial {u}_{r}}{\partial t}\\ {V}_{z}=\frac{\partial {u}_{z}}{\partial t}\end{array}\right.$$
(5)

Many scientific studies (Jiang et al. 2012; Ji et al. 2021; Luo et al. 2021) have shown that the S wave is the dominant wave near blasting, which mainly controls the surrounding rock damage caused by blasting construction. The P wave is an important part of the blasting near zone and blasting far zone, and it mainly affects the blasting vibration in the middle and far zones of blasting. However, it is well known that P waves decay faster than S waves. Therefore, the S wave may dominate the vibration in the far region. Based on the above analysis, the common contribution of P wave and S wave to vibration should be considered.

$$\left\{\begin{array}{c}{V}_{p}=\frac{{k}_{1}dH{p}_{0}}{\rho {c}_{p}R}\\ {V}_{s}=\frac{{k}_{2}dH{p}_{0}}{\rho {c}_{\text{s}}R}\end{array}\right.$$
(6)

where ρ is the rock density, and k1 and k2 are undetermined coefficients. The PPV caused by the short column charge is:

$$V={\int }_{H}{\overline{V} }_{p}ds+{\int }_{H}{\overline{V} }_{s}ds$$
(7)

In particular, the result of formula (7) refers to the vector sum of the two velocities.

Simplifying formula (7), we obtain:

$$V=k(\frac{{r}_{b}}{R})(\frac{{p}_{0}}{\rho {c}_{p}})$$
(8)

Since the actual rock mass has viscoelastic and damping properties, the blasting vibration attenuation equation of the actual rock mass can be expressed as:

$$V=k{(\frac{{r}_{b}}{R})}^{\alpha }(\frac{{p}_{0}}{\rho {c}_{p}})$$
(9)

The vibration speed on the blasthole wall can be expressed as:

$${V}_{0}=\frac{{p}_{0}}{\rho {c}_{p}}$$
(10)

Equation (10) can also be expressed as:

$$V=k{(\frac{{r}_{b}}{R})}^{\lambda }{V}_{0}$$
(11)

where k is the site coefficient, λ is the attenuation coefficient, rb is the radius of the cylindrical charge, and R is the distance from the blast area.

Improvement of blasting vibration velocity fitting formula

Equation (11) is indicated to be suitable for single-hole blasting, but it is relatively difficult to obtain engineering parameters in the formula. In addition, multi-hole and multi-stage blasting is often used in tunnel blasting engineering, and single-hole blasting is rare. Therefore, an attempt is made to find an equivalent solution suitable for tunnel blasting.

Determination of equivalent boundary of blasting

According to the difference in stress state, the rock mass near the blasting source can be divided into a crushed zone, fractured zone, and elastic zone. The actual blasting vibration test is often located in the elastic zone. The rock mass in this area can be approximated as an elastic body, while the rock mass in the crushed zone and the fractured zone is seriously damaged by the blast stress wave. However, the boundary of the inelastic zone can be considered the blasting load equivalent boundary (Deng et al. 2020; Irazoqui et al. 2000) to study the propagation law of blasting vibration.

Under the condition of a single blasthole cylindrical charge, the radius of the crushed zone (r1) and radius of the fractured zone (r2) can be expressed as (Yang et al. 2012):

$${r}_{1}={\left(\frac{{{pc}_{p}}^{2}}{5{\sigma }_{c}}\right)}^\frac{1}{2}{\left(\frac{{p}_{0}}{{\sigma }^{*}}\right)}^\frac{1}{4}{r}_{b}$$
(12)
$${r}_{2}={\left(\frac{\mu {p}_{0}}{(1-\mu ){\sigma }_{t}}\right)}^{\frac{1}{\beta }}{r}_{b}$$
(13)

where σc and σt are the dynamic uniaxial compressive strength and dynamic uniaxial tensile strength of the rock mass, respectively; σ* is the dynamic compressive strength of the rock mass under multi-directional stress conditions; β is the propagation attenuation coefficient; μ is Poisson’s ratio of the rock. Generally, the radius of the crushed zone is 3~5 times the radius of the charge, and the radius of the fractured zone is 10~15 times the radius of the charge.

If the interaction between the blastholes is not considered, the detonation of each cutting hole can be approximated as a short cylindrical cavity subjected to internal transient loads in a semi-infinite medium. Therefore, the effective boundary of the cutting section initiation can be approximated as the envelope of the multi-hole blasting fractured zone (Esen et al. 2003; Liang et al. 2013). Non-cutting holes, such as auxiliary holes, penetrate through the axis of adjacent blastholes to destroy the thrown rock, and their void surface can be approximated as an equivalent boundary. Figure 2 is a schematic diagram of the equivalent radius (rd) of the cutting hole of the tunnel blasting.

Fig. 2
figure 2

Schematic diagram of the equivalent boundary of cutting hole blasting

Full size image

Determination of the equivalent load of blasting load

According to the theory of detonation waves C-J of condensed charge, the initial detonation pressure p0 on the blasthole wall can be expressed as:

$${p}_{0}\text{=}\frac{{\rho }_{\text{e}}{D}^{2}}{2\left(\gamma +1\right)}$$
(14)

Under the condition of uncoupling charge, the initial detonation pressure on the blasthole wall is:

$${p}_{0}\text{=}\frac{{\rho }_{\text{e}}{D}^{2}}{2\left(\gamma +1\right)}{\left(\frac{{d}_{a}}{{d}_{b}}\right)}^{2\gamma }$$
(15)

If the uncoupling coefficient is large, the explosion gas expansion must go through two processes.

$$\left\{\begin{array}{l}\begin{array}{cc}\gamma=3&\;\;\;\;\;\;\;\;\;\;\;\;p_0\geq p_e\end{array}\\\begin{array}{cc}\gamma=\upsilon=\frac43&\;\;\;\;p_0<p_e\end{array}\end{array}\right.$$
(16)

where pe is the critical pressure of the explosive gas.

The initial detonation pressure can be expressed as follows:

$${p}_{0}\text{=}{\left(\frac{{\rho }_{\text{e}}{D}^{2}}{2\left(\gamma +1\right)}\right)}^{\frac{\upsilon }{\gamma }}{\left(\frac{{d}_{a}}{{d}_{b}}\right)}^{2\gamma }{{p}_{e}}^{\frac{(\gamma -\upsilon )}{\gamma }}$$
(17)

where ρe is the explosive density, γ is the isentropic coefficient of the explosive, and da and db are the diameters of the cylindrical charge and the blasthole, respectively.

The variation law of the blasting load of a single blasthole with the distance from the blast area is:

$${p}_{d}={p}_{0}{\left(\frac{R}{{r}_{b}}\right)}^{-\xi }$$
(18)

where ζ is the load transmission index; for the crushed zone, ζ=2+μ/1−μ; for the fractured zone, ζ=2−μ/1−μ.

Considering the influence of the group of holes in the cutting section on the equivalent blasting load, η is introduced as the influence coefficient of the blast load to simultaneously initiate multiple holes:

$$\eta =n{\left(\frac{{r}_{2}}{{r}_{d}}\right)}^{2}$$
(19)

where n is the number of blastholes in the cutting section.

For the cutting section with multiple simultaneously detonated blastholes, the equivalent blasting load is:

$${p}_{d}=\eta {p}_{0}{\left(\frac{{r}_{1}}{{r}_{b}}\right)}^{-2-\frac{\mu }{1-\mu }}{\left(\frac{{r}_{2}}{{r}_{1}}\right)}^{-2+\frac{\mu }{1+\mu }}$$
(20)

For noncutting holes, the blasting load is equivalently applied to the plane where the centerline of the blasthole and the axis of the blasthole are located. The equivalent load size is:

$${p}_{d}=\frac{2{r}_{b}{p}_{0}}{s}$$
(21)

where s is the distance between adjacent blastholes.

Then, the improved attenuation equation for the vibration velocity can be written as:

$$\left\{\begin{array}{l}V_0=\frac{p_d}{\rho c_p}\\V=k\left(\frac{r_d}R\right)^\lambda V_0\end{array}\right.$$
(22)

Dimensional analysis

To verify the feasibility of the improved formula (22), a dimensional analysis is used to derive the velocity attenuation equation of tunnel blasting vibration. Among the influencing factors, the rock density (ρ), longitudinal wave propagation velocity (cp), distance from the blast area (R), rock elastic modulus (E), and blasting load equivalent radius (rd) are selected as the main influencing factors. Moreover, cp, R, and ρ are selected as independent dimensions; then:

$$V=\varphi \left(\rho ,{c}_{p},R,{r}_{d},E\right)$$
(23)

According to the π theorem, Eq. (23) can be represented by 3 dimensionless numbers:

$$\left\{\begin{array}{c}{\pi }_{1}=\frac{V}{{c}_{p}}\\ {\pi }_{2}=\frac{{r}_{d}}{R}\\ {\pi }_{3}=\frac{E}{{{c}_{p}}^{2}\rho }\end{array}\right.$$
(24)

Therefore:

$$\frac{V}{{c}_{p}}=\Pi \left\{\frac{E}{{{c}_{p}}^{2}\rho },\left(\frac{{r}_{d}}{R}\right)\right\}$$
(25)

According to the dimensional harmony theorem:

$$\frac{V}{{c}_{p}}=K{\left(\frac{E}{{{c}_{p}}^{2}\rho }\right)}^{\alpha }{\left(\frac{{r}_{d}}{R}\right)}^{\beta }$$
(26)

For a specific tunnel project, E, cp, and ρ remain basically unchanged, so they can be converted into:

$$V=K{\left(\frac{{r}_{d}}{R}\right)}^{\omega }$$
(27)

Equation (27) has the same form as the improved formula (22), which verifies the feasibility of the improved formula.

Project background and monitoring plan

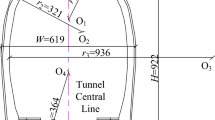
The blasting project of Chong-li Tunnel was used as the engineering background for subsequent research. The main tunnel passes through the existing villages with a minimum vertical clearance of 18 m. The surrounding rock of the tunnel is designed to be grade III. Figure 3 shows the topographic map of the underpass section of the Chong-li Tunnel.

Fig. 3
figure 3

Topographic map of the underpass section of the tunnel

Full size image

Blasting construction plan

According to the surrounding rock grade and geological conditions of the tunnel, the tunnel is driven by full-face blasting, as shown in Fig. 4. Table 1 shows the specific charge of blasting construction. The density of the surrounding rock is 2630 kg/m3, the longitudinal wave velocity is approximately 5500 m/s, the detonation wave velocity is 4500 cm/s, and the explosive density is 1 g/cm3.

Fig. 4
figure 4

Layout of the blasthole

Full size image
Table 1 Specific charge for tunnel blasting
Full size table

Blasting vibration monitoring program

A TC-4850 N blasting vibration tester was used in the on-site monitoring process. The blasting vibration meter is equipped with the three-axis vibration velocity sensor TCS-B3, which can simultaneously collect the blasting vibration velocity in the three directions of X, Y, and Z. As shown in Fig. 5, the five measurement points horizontally adjacent to the tunnel were selected for this monitoring.

Fig. 5
figure 5

Layout of measuring points

Full size image

Regression analysis of on-site measurement results

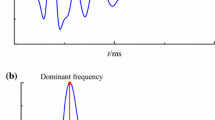
Table 2 is the results of two field experiments. As shown in Fig. 6, the blasting vibration speed excited by each detonator can be clearly distinguished from the waveform time history diagram.

Table 2 Peak blasting vibration velocity (PPV) and related parameters
Full size table
Fig. 6
figure 6

Time-history waveform of the blasting vibration of the experiment

Full size image

Table 2 shows that the vibration velocity in the Z direction is the largest among the three directions. Therefore, the subsequent analysis only focuses on the velocity in the Z direction. In addition, the PPVs corresponding to MS1 and MS9 are the largest. The PPVs corresponding to MS1 of the two blasting constructions are 1.99 cm/s and 2.33 cm/s, respectively. For comparison, the PPVs corresponding to MS9 are 1.58 cm/s and 2.02 cm/s, respectively. To predict the blasting vibration accurately, classification research should be conducted according to the blasthole type.

Regression analysis of PPV

Equation (22) is used in the regression calculation of the blasting vibration velocity, and the fitting results are shown in Fig. 7. It shows that Eq. (22) has a good fitting effect on the PPV induced by different detonator positions, and all correlation coefficients are greater than 0.8.

Fig. 7
figure 7

Fitting of attenuation of blasting vibration particles

Full size image

Figure 7 shows that the same model equation can express the PPV that corresponds to the same type of blastholes. For example, the PPV excited by multiple-level detonators in the auxiliary hole shows a good linear fitting relationship, and the correlation coefficient is as high as 0.8770. Thus, it is feasible to uniformly fit and analyze the vibration velocities induced by the same type of blastholes, which saves calculation time and obtains a blasting vibration attenuation law that is more consistent with reality.

Form of charge of attenuation formula

To verify the superiority of the introduced fitting Eq. (22), a comparative analysis was conducted by introducing a common charge structure. For spherical charge blasting at one time, the charge quantity has the following relationship with the equivalent radius (Liu et al. 2018).

$$Q=\frac{4}{3}{{\pi q\mathrm{r}}_{d}}^{3}$$
(28)

Therefore, Eq. (22) can be rewritten as:

$$\left\{\begin{array}{l}V_0=\frac{p_d}{\rho c_p}\\V=k\left(\frac{\sqrt[3]Q}R\right)^\lambda V_0\end{array}\right.$$
(29)

Similarly, for the columnar charge, Eq. (22) can be rewritten as:

$$\left\{\begin{array}{l}V_0=\frac{p_d}{\rho c_p}\\V=k\left(\frac{\sqrt Q}R\right)^\lambda V_0\end{array}\right.$$
(30)

The regression analysis of the test data is performed using Eqs. (29) and (30). The comparison with the fitting effect of Eq. (22) is summarized in Table 3.

Table 3 Fitting formula of blasting vibration
Full size table

Table 3 shows that all correlation coefficients of Eqs. (29)~(30) are smaller than those of Eq. (22), but the correlation coefficients of cutting holes, peripheral holes, and bottom plate holes are not significantly different. However, for the auxiliary hole, the fitting coefficients obtained by Eqs. (29)~(30) are 0.4976 and 0.4370, respectively, which are much smaller than those obtained by Eq. (22). The above analysis proves that the charge form of the attenuation equation is better in fitting the blasting vibration induced by single-segment detonators, but it is not suitable for analyzing blasting vibrations corresponding to multi-segment detonators. The main reason for this difference is that the initiation of cutting holes, etc. is mainly affected by the maximum charge per delay. However, the auxiliary hole is composed of multiple sections of detonators, which makes the vibration speed that is excited by different levels have obvious differences. If the maximum charge per delay is used as the fitting parameter, it cannot truly reflect the law of blasting vibration.

Conclusion

Based on the analytical solution of the stress wave of the short column charge, the blasting vibration attenuation formula is deduced. Relying on specific blasting projects, a regression analysis was performed, and the following conclusions were obtained:

  1. 1.

    Based on short column charge theory, the concept of equivalent radius is introduced, and the blasting vibration attenuation model equation is theoretically deduced. It is verified through dimensional analysis.

  2. 2.

    The improved model can accurately predict the peak blasting vibration velocity for different detonator positions and apply to the fitting analysis of the PPV for different blasthole types.

  3. 3.

    By comparison, the fitting effect of the two charge forms of the blasting vibration attenuation formula is not as good as the improved formula, which proves that the attenuation model of blasting vibration introduced is suitable for practical engineering.