Limit Cycles Bifurcating of Discontinuous and Continuous Piecewise Differential Systems of Isochronous Cubic Centers with Three Zones

Abstract

We study the maximum number of limit cycles that bifurcate from the periodic annulus of the isochronous cubic centre of discontinuous and continuous piecewise differential systems with three zones formed by the discontinuity set $\Sigma =\{(x,y)\in \mathbb {R}^2: (y=0) \vee (x=0 \wedge y\ge 0)\}$. More precisely, we consider the following perturbed systems

$$\begin{aligned} \dot{x}=-y+x^2y+ \epsilon p_i(x,y),\ \dot{y}= x+xy^2+ \epsilon q_i(x,y),\ i=1,2,3, \end{aligned}$$

where $p_i$ and $q_i$ are polynomials of degree m. Using the averaging theory of first order, we prove that for $m=1, 2, 3$, at most 3, 9 and 15 limit cycles bifurcate from the periodic annulus of the isochronous cubic centre in the discontinuous case, respectively. While for the continuous case, it can appear 1, 5 and 6 limit cycles from the periodic orbits of these centres, respectively. Furthermore, we extend our study when $p_i$ and $q_i$ are homogeneous polynomials with $1\le m \le 3$, obtaining respectively for $m=1, 2, 3$ at most one, seven and at least twelve limit cycles, which bifurcate from the periodic orbits of the isochronous cubic centre.

1 Introduction and Statement of the Main Results

The study of the limit cycles goes back essentially to Poincaré [23, 24] at the end of the nineteenth century. The existence of limit cycles is one of the main problems in the qualitative theory of continuous planar polynomial differential systems and became important in the applications to the real world, since many phenomena are related to its existence. See for instance the Van der Pol oscillator [27, 28] or the Belousov-Zhabotinskii reaction which is a classical reaction of non-equilibrium thermodynamics appearing in a non-linear chemical oscillator, see [3, 29].

On the other hand, the study of the bifurcation of limit cycles of isochronous centers for planar differential systems has been a topic of research for many years. Indeed, several methods are even used to study the problem of isochronicity, which appear in many fields such as physics, chemistry, biology and engineering (for more details see [6, 13]).

The study of this class of systems has been extended to solve the second part of the 16th Hilbert problem for continuous and discontinuous piecewise differential systems, that is, to determine the maximum number of limit cycles. A particular study deals on the bifurcation of limit cycles in planar polynomial vector fields, trying to exhibit the maximum number of limit cycles that the differential polynomial systems can have for some given degree.

In this work, we consider the unperturbed uniform isochronous center of the form

$$\begin{aligned} \dot{x}= -y+x^2y, \quad \dot{y}= x+xy^2. \end{aligned}$$

(1)

The previous system has been studied by many authors, among them we can cite the work of Haihua, Llibre and Torregrosa in [20], when the system (1) is perturbed by polynomials of degree one, and they proved that there are not limit cycles by using the averaging method of first order. While in the case where the perturbed polynomials have degree two, they showed that there exists one limit cycle, and when the polynomial perturbations have degree three, there are three limit cycles, by using the averaging method of first order. Huang and Niu in [16], applying the same method, obtained that from system (1) perturbed by homogeneous polynomials of degree $2n+3$ bifurcates up to $n+2$ limit cycles.

In recent years, the study of system (1) under different polynomial perturbations has also been extended to piecewise differential systems. Among some works, we have Itikawa and Llibre [17], where they study the maximum number of small or medium limit cycles that branch from the periodic orbits of (1), when they are perturbed by cubic polynomials separated by the straight line $y=0$. Obtaining as an upper bound, seven medium limit cycles (one of which bifurcates from a periodic orbit surrounding a center). Also, in [18] the authors proved the existence of at least 12 limit cycles bifurcating from the periodic orbit of the center of system (1) when they are perturbed by cubic polynomials with four zones separated by the axes of coordinates.

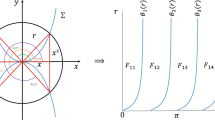
In the present work, our objective is to study the maximum number of limit cycles which bifurcate from the periodic solutions of the uniform isochronous center at the origin given in (1) when it is perturbed by polynomials of degree 1 and 2 inside the class of discontinuous or continuous piecewise differential systems with three zones separated by $\Sigma =\{(x,y)\in \mathbb {R}^2: (y=0) \vee (x=0 \wedge y\ge 0)\}$. We also wish to understand the number of limit cycles in the case where the perturbed polynomials are homogeneous. Additionally, we will treat the case where the piecewise differential systems are continuous, that is, at each point of the discontinuity curve all the perturbed vector fields coincide on the common boundary. The three components of $\mathbb {R}^2 \setminus \Sigma$ are the first quadrant

$$\begin{aligned} R_1=\big \{(x,y)\in \mathbb {R}^2: x\ge 0 \wedge y\ge 0\big \}, \end{aligned}$$

the second quadrant

$$\begin{aligned} R_2=\big \{(x,y)\in \mathbb {R}^2: x\le 0 \wedge y\ge 0\big \}, \end{aligned}$$

and the half-plane

$$\begin{aligned} R_3=\big \{(x,y)\in \mathbb {R}^2: y\le 0\big \}. \end{aligned}$$

Thus, we consider the perturbed system (1) as a discontinuous or continuous piecewise differential system of the form

$$\begin{aligned} Z(x,y)=Z^i(x,y), \quad (x,y)\in R_i, \end{aligned}$$

(2)

with $i=1,2,3$, that is,

$$\begin{aligned} Z^i(x,y)={\left\{ \begin{array}{ll} \dot{x}= -y+x^2 y + \epsilon p_i(x,y),\\ \dot{y}= x+xy^2+\epsilon q_i(x,y),\\ \end{array}\right. } \end{aligned}$$

(3)

where $0\le \epsilon \ll 1$ and

$$\begin{aligned} p_1(x,y)=\displaystyle \sum _{l+j=0}^m a_{lj} x^l y^j,\quad q_1(x,y)=\displaystyle \sum _{l+j=0}^m b_{lj} x^l y^j, \\ p_2(x,y)=\displaystyle \sum _{l+j=0}^m c_{lj} x^l y^j,\quad q_2(x,y)=\displaystyle \sum _{l+j=0}^m d_{lj} x^l y^j, \\ p_3(x,y)=\displaystyle \sum _{l+j=0}^m e_{lj} x^l y^j,\quad q_3(x,y)=\displaystyle \sum _{l+j=0}^m f_{lj} x^l y^j \end{aligned}$$

are polynomials of degree m and $a_{lj},\ b_{lj}, \ c_{lj},\ d_{lj},\ e_{lj}, \ f_{lj}\in \mathbb {R}$.

In this work, by using the averaging method, we obtain the main results as follows.

Theorem 1

For $|\epsilon |\ne 0$ sufficiently small, and using averaging method of first order, we obtain that:

• (a) There are discontinuous piecewise polynomial differential systems (3) for $m=1$ having at most 3 limit cycles which bifurcate from the periodic orbits of the isochronous cubic center (1). Moreover we can find parameters $a_{lj}$, $b_{lj}$, $c_{lj}$, $d_{lj}$, $e_{lj}$, $f_{lj},\ 0\le l+j\le 1$ such that the system (3) has exactly 0, 1, 2 or 3 limit cycles.

• (b) There are discontinuous piecewise polynomial differential systems (3) for $m=2$ having at most 9 limit cycles which bifurcate from the periodic orbits of the isochronous cubic center (1). Moreover we can find parameters $a_{lj}$, $b_{lj}$, $c_{lj}$, $d_{lj}$, $e_{lj}$, $f_{lj},\ 0\le l+j\le 2$ such that the system (3) has exactly 0, 1, 2, 3, 4, 5, 6, 7, 8 or 9 limit cycles.

• (c) There are discontinuous piecewise polynomial differential systems (3) for $m=3$ having at most 15 limit cycles which bifurcate from the periodic orbits of the isochronous cubic center (1).

Corollary 1

Using the averaging theory of first order for $|\epsilon |\ne 0$ sufficiently small, the following statements hold.

• (a) There are discontinuous piecewise polynomial differential systems (3) with $m=1$, homogeneous polynomial perturbations having at most a limit cycle which bifurcate from the periodic orbits of the isochronous cubic center (1);

• (b) There are discontinuous piecewise polynomial differential systems (3) with $m=2$, homogeneous quadratic polynomial perturbations having at most seven limit cycles which bifurcates from the periodic orbits of the isochronous cubic center (1).

• (c) There are discontinuous piecewise polynomial differential systems (3) with $m=3$, homogeneous cubic polynomial perturbations having at least twelve limit cycles which bifurcate from the periodic orbits of the isochronous cubic center (1).

Theorem 2

For $|\epsilon |\ne 0$ sufficiently small, and using the averaging method of first order we obtain that:

• (a) There are continuous piecewise polynomial differential systems (3) for $m=1$ having at most one limit cycle which bifurcate from the periodic orbits of the isochronous cubic center (1).

• (b) There are continuous polynomial differential systems (3) for $m=2$ having at most four limit cycles which bifurcate from the periodic orbits of the isochronous cubic center (1).

• (c) There are continuous polynomial differential systems (3) for $m=3$ having at least six limit cycles which bifurcate from the periodic orbits of the isochronous cubic center (1).

The unperturbed problem in (1) has the first integral $\mathcal {H}(x, y)=(x^2+y^2)/(1-x^2)$, so the origin is a center surrounding by periodic solutions bounded by the line $x=-1$ and $x=1$. In any case, our objective is to find limit cycles in the previous region. In our analysis we use the parametrization of the isochronous given by $x=\frac{r \cos \theta }{\sqrt{r^2 \cos ^2\theta +1}}$ and $y=\frac{r \sin \theta }{\sqrt{r^2 \cos ^2\theta +1}}$, where $0<r<1$ and $\theta \in [0, 2\pi )$. We point out that in the case $m=3$, it is very difficult under our approach to provide an explicit upper bound for the maximum number of limit cycles bifurcating the periodic orbits of the cubic isochronous center for all $r\in (0, 1)$. This is mainly due to the fact that the determinants of the Wronskians are too complicated to determine whether they vanish at the interval (0, 1). However, we find in the discontinuous case at most 15 limit cycles that bifurcate from the periodic orbits of the cubic isochronous center, in a small subinterval (0.72, 1) of (0, 1). While for the homogeneous and continuous case, we provide a lower bound for the number of limit cycles by applying Taylor series around $r=0$.

Theorem 1 and Corollary 1 are shown in Sects. 2 and 2.2, respectively. On the other hand, the results of Theorem 2 are proved in Sect. 3.

2 Proof of Theorem 1

Proof of statement (a) of Theorem 1

To apply Theorem 4 given in the Appendix, we need to write the system (3) in the form (41). In order to do that, consider the first integral $\mathcal {H}(x, y)=(x^2+y^2)/(1-x^2)$ and its corresponding integrating factor $\mu (x, y)=2/(1-x^2)^2$. Note that $h_1=0$, $h_2=1$ and taking $\rho (r,\theta )=r/(1+r^2 \cos ^2\theta )$ for $0<r<1$, $\theta \in [0,2\pi )$ with $x=\frac{r \cos \theta }{\sqrt{r^2 \cos ^2\theta +1}}$ and $y=\frac{r \sin \theta }{\sqrt{r^2 \cos ^2\theta +1}}$, we transform the system (3) for $m=1$ into the form

$$\begin{aligned} \frac{dr}{d\theta }=\epsilon \displaystyle \sum _{j=1}^3 \chi _{\bar{R}_j}F_1^j (\theta ,r)+\mathcal {O}(\epsilon ^2), \end{aligned}$$

(4)

where

$$\begin{aligned} \begin{array}{l} F_1^j(\theta , r)=\frac{\sqrt{r^2 \cos 2 \theta +r^2+2}}{\sqrt{2}} \left( \bar{p}_j(\theta , r) \left( r^2+1\right) \cos \theta +\bar{q}_j(\theta , r) \sin \theta \right) ,\\ \bar{p}_j(\theta , r)=p_j\left( \frac{r \cos \theta }{\sqrt{r^2 \cos ^2\theta +1}}, \frac{r \sin \theta }{\sqrt{r^2 \cos ^2\theta +1}}\right) ,\\ \bar{q}_j(\theta , r)=q_j\left( \frac{r \cos \theta }{\sqrt{r^2 \cos ^2\theta +1}}, \frac{r \sin \theta }{\sqrt{r^2 \cos ^2\theta +1}}\right) , \end{array} \end{aligned}$$

for $j=1,2,3$ the equation (4) satisfies the hypotheses of Theorem 4. The discontinuous differential system (3) satisfies the assumptions of Theorem 3. Therefore, the averaging function is the sum of three definite integrals as follows

$$\begin{aligned} f_1(r)=\int _0^{\pi /2} F_1^1(\theta , r)d\theta +\int _{\pi /2}^{\pi } F_{1}^2(\theta , r)d\theta +\int _{\pi }^{2\pi } F_{1}^3(\theta , r) d\theta , \end{aligned}$$

(5)

where

$$\begin{aligned} F_{1}^1(\theta , r)= & {} \frac{1}{2} \left( a_{01} r^3 \sin 2 \theta +a_{10} r^3 \cos 2 \theta +a_{10} r^3 +a_{00} \sqrt{2} r^2 \cos \theta \sqrt{r^2 \cos 2 \theta +r^2+2} \right. \nonumber \\{} & {} \quad +a_{00}\sqrt{2} \cos \theta \sqrt{r^2 \cos 2 \theta +r^2+2} +a_{01} r \sin 2 \theta +a_{10}r \cos 2 \theta +a_{10}r \nonumber \\{} & {} \quad +\left. b_{00}\sqrt{2} \sin \theta \sqrt{r^2 \cos 2 \theta +r^2+2} +b_{10}r \sin 2 \theta -b_{01}r \cos 2 \theta +b_{01}r \right) ,\nonumber \\ F_{1}^2(\theta , r)= & {} \frac{1}{2} \left( c_{01} r^3 \sin 2 \theta +c_{10} r^3 \cos 2 \theta +c_{10} r^3 +c_{00} \sqrt{2} r^2 \cos \theta \sqrt{r^2 \cos 2 \theta +r^2+2} \right. \nonumber \\{} & {} \quad +c_{00}\sqrt{2} \cos \theta \sqrt{r^2 \cos 2 \theta +r^2+2} +c_{01} r \sin 2 \theta +c_{10}r \cos 2 \theta +c_{10}r \nonumber \\{} & {} \quad +\left. d_{00}\sqrt{2} \sin \theta \sqrt{r^2 \cos 2 \theta +r^2+2} +d_{10}r \sin 2 \theta -d_{01}r \cos 2 \theta +d_{01}r \right) ,\nonumber \\ F_{1}^3(\theta , r)= & {} \frac{1}{2} \left( e_{01} r^3 \sin 2 \theta +e_{10} r^3 \cos 2 \theta +e_{10} r^3 +e_{00} \sqrt{2} r^2 \cos \theta \sqrt{r^2 \cos 2 \theta +r^2+2} \right. \nonumber \\{} & {} \quad +e_{00}\sqrt{2} \cos \theta \sqrt{r^2 \cos 2 \theta +r^2+2} +e_{01} r \sin 2 \theta +e_{10}r \cos 2 \theta +e_{10}r \nonumber \\{} & {} \quad +\left. f_{00}\sqrt{2} \sin \theta \sqrt{r^2 \cos 2 \theta +r^2+2} +f_{10}r \sin 2 \theta -f_{01}r \cos 2 \theta +f_{01}r \right) . \end{aligned}$$

(6)

Using the integrals of Lemma 1 given in Appendix 1 and rearranging conveniently, the averaging function $f_1(r)$ takes the form

$$\begin{aligned} \begin{aligned} f_1(r)=&\dfrac{1}{8 \pi r}\left( \alpha _1 g_1+\alpha _2 g_2+ \alpha _3 g_3+ \alpha _4 g_4+\alpha _5 g_5+ \alpha _6 g_6\right) , \end{aligned} \end{aligned}$$

(7)

with

$$\begin{aligned} \begin{array}{ll} \alpha _1=\left( \pi b_{01}+2 b_{10}+\pi d_{01}-2 d_{10}+2 \pi f_{01}\right) ,&{} \alpha _2=\left( 2 a_{01}+\pi a_{10}-2 c_{01}+\pi c_{10}+2 \pi e_{10}\right) ,\\ \alpha _3=2\left( b_{00}+d_{00}-2 f_{00}\right) , &{}\alpha _4=2\left( b_{00}-f_{00}\right) ,\\ \alpha _5=2 \left( a_{00}-c_{00}\right) , &{}\alpha _6=-2\left( d_{00}-f_{00}\right) , \end{array} \end{aligned}$$

(8)

and

$$\begin{aligned} \begin{array}{ll} g_1=r^2,&{} g_2=\left( r^4+r^2\right) ,\\ g_3= r \sqrt{r^2+1}, &{}g_4= \ln \left( \sqrt{r^2+1}+r\right) ,\\ g_5= \left( r^2+1\right) \left( (r^2+1)\tan ^{-1}(r)+r\right) , &{} g_6= \ln \left( \sqrt{r^2+1}-r\right) . \end{array} \end{aligned}$$

Now observing that $g_6=-g_4$ and $\alpha _3=\alpha _4-\alpha _6$, so we can rewrite the function (7) as

$$\begin{aligned} \begin{aligned} f_1(r)=&\dfrac{1}{8 \pi r}\left( \alpha _1 G_1+ \alpha _2 G_2+ \alpha _3 G_3 + \alpha _4 G_4\right) , \end{aligned} \end{aligned}$$

(9)

where $G_1=g_1$, $G_2=g_2$, $G_3=g_3+g_4$, $G_4=g_5$. The functions $\{G_1, G_2, G_3, G_4\}$ form ECT-systems on (0, 1) (according to Appendix in Sect. 1), because the Wronskians of these functions are

$$\begin{aligned} \small {\begin{aligned} W_1(G_1)&=r^2,\\ W_2(G_1, G_2)&=2r^5,\\ W_3(G_1, G_2, G_3)&=4 r^3 \left( 4 \sinh ^{-1}(r)-\frac{r}{\sqrt{r^2+1}}\right) ,\\ W_4(G_1, G_2, G_3, G_4)&=\frac{8 r \left( 5 r^3-4 \left( 4 r^2+3\right) \tan ^{-1}(r)-4 \left( r^2-3\right) \sqrt{r^2+1} \sinh ^{-1}(r)\right) }{\left( r^2+1\right) ^{3/2}}, \end{aligned}} \end{aligned}$$

and easily $W_j\ne 0$, $j=1,2$ for $r\in (0,1)$. On the other hand, for the functions $W_3$ and $W_4$ the analysis is not direct, but we are going to prove that they are monotonous functions for $0< r < 1$. In fact, first we note that the first derivative of $W_3$ satisfies

$$\begin{aligned} W'_3(r)=48 r^2 \sinh ^{-1}(r)+\frac{4 r^5}{\left( r^2+1\right) ^{3/2}} > 0, \end{aligned}$$

so $W_3(r)$ is increasing and $W_3(0) =0$, thus $W_3(r)> 0$ for all $r \in (0, 1)$. For $W_4$ we obtain that the fifth derivative is

$$\begin{aligned} W_4^{(5)}(r)=-\frac{4 \left( M_1(r)+ M_2(r) \sinh ^{-1}(r)+M_3(r) \tan ^{-1} (r)\right) }{\left( r^2+1\right) ^{3/2}} <0, \end{aligned}$$

where

$$\begin{aligned}\begin{array}{ll} M_1(r)=r \left( 32 r^4+289 r^2+233\right) \sqrt{r^2+1},\\ M_2(r)=4 \left( 96 r^6+190 r^4+105 r^2+11\right) ,\\ M_3(r)=12 \left( 27 r^4+34 r^2+7\right) \sqrt{r^2+1}. \end{array} \end{aligned}$$

Following in the same way we arrive to the fact $W_4(r)$ is decreasing, and $W_4(0)=0$. Therefore, we get $W_4(r)< 0$ for all $r \in (0, 1)$.

By using Theorem 6, we have that there exists a linear combination of these functions with at most three zeros $r_k\in (0,1)$, $k=1,2,3$, and coefficients $a_{lj}$, $b_{lj}$, $c_{lj}$, $d_{lj}$, $e_{lj}$, $f_{lj},\ 0\le l+j\le 1$ such that $f_1(r_k)=0$ and $f'_1(r_k)\ne 0$ for $k=1,2,3$.

In short, applying the averaging theory of first order, there are discontinuous polynomial systems (3) having at most three limit cycles which bifurcate from periodic orbits of the uniform isochronous center (1).

For the proof of the second part of the statement (a) we are going to give concrete examples after the proof. $\square$

Proof of statement (b) of Theorem 1

Using the same arguments as in the proof of the previous statement (a), in this case we transform the system (3) for $m=2$ into the form (4). Thus, using Theorem 3 given in the Appendix 1, the averaging function for this case assumes the form

$$\begin{aligned} f_1(r)=\int _0^{\pi /2} F_1^1(\theta , r)d\theta +\int _{\pi /2}^{\pi } F_{1}^2(\theta , r)d\theta +\int _{\pi }^{2\pi } F_{1}^3(\theta , r) d\theta , \end{aligned}$$

(10)

where

$$\begin{aligned} F_{1}^1(\theta , r)= & {} \,a_{10} r^3 \cos ^2\theta +a_{01} r^3 \sin \theta \cos \theta +a_{10} r \cos ^2\theta +a_{01} r \sin \theta \cos \theta +b_{01} r \sin ^2\theta + \\{} & {} b_{10} r \sin \theta \cos \theta +\frac{\sqrt{2} \left( r^2+1\right) r^2 \cos ^3\theta a_{20}}{\sqrt{r^2 \cos 2 \theta +r^2+2}}+\frac{\sqrt{2} \sin \theta \left( r^2 \sin ^2\theta b_{02}+b_{00}\right) }{\sqrt{r^2 \cos 2 \theta +r^2+2}}+\\{} & {} \frac{\cos \theta \left( 2 r^2 \sin ^2\theta \left( \left( r^2+1\right) a_{02}+b_{11}\right) +\left( r^2+1\right) \left( r^2 \cos 2 \theta +r^2+2\right) a_{00}\right) }{\sqrt{2} \sqrt{r^2 \cos 2 \theta +r^2+2}}+\\{} & {} \frac{\sqrt{2} r^2 \sin \theta \cos ^2 \theta \left( \left( r^2+1\right) a_{11}+b_{00}+b_{20}\right) }{\sqrt{r^2 \cos 2 \theta +r^2+2}},\\ F_{1}^2(\theta , r)= & {} \,c_{10} r^3 \cos ^2\theta +c_{01} r^3 \sin \theta \cos \theta +c_{10} r \cos ^2\theta +c_{01} r \sin \theta \cos \theta +d_{01} r \sin ^2\theta \\{} & {} \quad +d_{10} r \sin \theta \cos \theta +\frac{\sqrt{2} \left( r^2+1\right) r^2 \cos ^3\theta c_{20}}{\sqrt{r^2 \cos 2 \theta +r^2+2}}+\frac{\sqrt{2} \sin \theta \left( r^2 \sin ^2\theta d_{02}+d_{00}\right) }{\sqrt{r^2 \cos 2 \theta +r^2+2}}\\{} & {} \quad +\frac{\cos \theta \left( 2 r^2 \sin ^2\theta \left( \left( r^2+1\right) c_{02}+d_{11}\right) +\left( r^2+1\right) \left( r^2 \cos 2 \theta +r^2+2\right) c_{00}\right) }{\sqrt{2} \sqrt{r^2 \cos 2 \theta +r^2+2}}\\{} & {} \quad +\frac{\sqrt{2} r^2 \sin \theta \cos ^2 \theta \left( \left( r^2+1\right) c_{11}+d_{00}+d_{20}\right) }{\sqrt{r^2 \cos 2 \theta +r^2+2}},\\ F_{1}^3(\theta , r)= & {} \,e_{10} r^3 \cos ^2\theta +e_{01} r^3 \sin \theta \cos \theta +e_{10} r \cos ^2\theta +e_{01} r \sin \theta \cos \theta +f_{01} r \sin ^2\theta \\{} & {} \quad +f_{10} r \sin \theta \cos \theta +\frac{\sqrt{2} \left( r^2+1\right) r^2 \cos ^3\theta e_{20}}{\sqrt{r^2 \cos 2 \theta +r^2+2}}+\frac{\sqrt{2} \sin \theta \left( r^2 \sin ^2\theta f_{02}+f_{00}\right) }{\sqrt{r^2 \cos 2 \theta +r^2+2}}\\{} & {} \quad +\frac{\cos \theta \left( 2 r^2 \sin ^2\theta \left( \left( r^2+1\right) e_{02}+f_{11}\right) +\left( r^2+1\right) \left( r^2 \cos 2 \theta +r^2+2\right) e_{00}\right) }{\sqrt{2} \sqrt{r^2 \cos 2 \theta +r^2+2}}\\{} & {} \quad +\frac{\sqrt{2} r^2 \sin \theta \cos ^2 \theta \left( \left( r^2+1\right) e_{11}+f_{00}+f_{20}\right) }{\sqrt{r^2 \cos 2 \theta +r^2+2}}. \end{aligned}$$

So, integrating (10) by using again Lemma 1 from Appendix 1, the first order averaged function adopts the form

$$\begin{aligned} f_1(r)= & {} \dfrac{1}{8 \pi r}\left( \beta _1 g_1+\beta _2 g_2+\beta _3 g_3+\beta _4 g_4+\beta _5 g_5 +\beta _6 g_6+\beta _7 g_7\right. \nonumber \\{} & {} \left. +\beta _8 g_8+\beta _9 g_9+\beta _{10} g_{10}\right) , \end{aligned}$$

(11)

with

$$\begin{aligned} \beta _1= & {} \,2 a_{00}-2 a_{02}+2 a_{20}-2 b_{11}-2 c_{00}+2 c_{02}-2 c_{20}+2 d_{11},\nonumber \\ \beta _2= & {} \,2 a_{01}+\pi a_{10}+\pi b_{01}+2 b_{10}-2 c_{01}+\pi c_{10}+\pi d_{01}-2 d_{10}+2 \pi e_{10}+2 \pi f_{01},\nonumber \\ \beta _3= & {} \,2 a_{00}-2 a_{02}+2 a_{20}-2 c_{00}+2 c_{02}-2 c_{20},\nonumber \\ \beta _4= & {} \,2 a_{01}+\pi a_{10}-2 c_{01}+\pi c_{10}+2 \pi e_{10},\nonumber \\ \beta _5= & {} \,2 a_{11}+2 c_{11}-4 e_{11},\nonumber \\ \beta _6= & {} \,2 a_{11}+2 b_{00}-2 b_{02}+2 b_{20}+2 c_{11}+2 d_{00}\nonumber \\{} & {} -2 d_{02}+2 d_{20}-4 e_{11}-4 f_{00}+4 f_{02}-4 f_{20},\nonumber \\ \beta _7= & {} \,2 a_{00}+2 a_{02}+2 a_{20}-2 c_{00}-2 c_{02}-2 c_{20},\nonumber \\ \beta _8= & {} \,2 a_{00}+2 a_{02}-2 a_{20}+2 b_{11}-2 c_{00}-2 c_{02}+2 c_{20}-2 d_{11},\nonumber \\ \beta _9= & {} \,-2 a_{11}+2 b_{00}+2 b_{02}-2 b_{20}-2 c_{11}+2 d_{00}\nonumber \\{} & {} +2 d_{02}-2 d_{20}+4 e_{11}-4 f_{00}-4 f_{02}+4 f_{20},\nonumber \\ \beta _{10}= & {} \,-2 a_{11}+4 b_{02}-2 c_{11}+4 d_{02}+4 e_{11}-8 f_{02}, \end{aligned}$$

(12)

and

$$\begin{aligned} \begin{array}{lll} g_1=r,&{} g_2=r^2, &{} g_3=r^3,\\ g_4=r^4, &{} g_5=r^3 \sqrt{r^2+1}, &{} g_6=r \sqrt{r^2+1},\\ g_7=r^2\left( r^2+1\right) \arctan (r), &{} g_8=\left( r^2+1\right) \arctan (r), &{} g_9=\sinh ^{-1}(r), \\ g_{10}=r^2 \sinh ^{-1}(r).&{} &{} \\ \end{array} \end{aligned}$$

We define the ordered set of functions $\{g_1, g_2, g_3, g_4, g_5, g_6, g_7, g_8, g_9, g_{10}\}$, which is an ECT-system on the interval (0, 1). In fact, computing the Wronskians, we obtain

$$\begin{aligned} W_1(g_1)= & {} r,\\ W_2(g_1, g_2)= & {} r^2,\\ W_3(g_1, g_2, g_3)= & {} 2 r^3,\\ W_4(g_1,\ldots , g_4)= & {} 2 r^4,\\ W_5(g_1,\ldots , g_5)= & {} -\frac{36 r^5 \left( r^2-4\right) }{\left( r^2+1\right) ^{7/2}},\\ W_6(g_1,\ldots , g_6)= & {} \frac{3240 r^7}{\left( r^2+1\right) ^7},\\ W_7(g_1,\ldots , g_7)= & {} -\frac{51840 r^{10}}{\left( r^2+1\right) ^{12}},\\ W_8(g_1,\ldots , g_8)= & {} -\frac{1244160 r^3 \left( P_1(r)+P_2(r) \tan ^{-1}(r)\right) }{\left( r^2+1\right) ^{17}},\\ W_9(g_1,\ldots , g_9)= & {} -\frac{238878720 r^6 \left( Q_1(r) \sinh ^{-1}(r)+ Q_2(r)+Q_3(r) \tan ^{-1}(r)\right) }{\left( r^2+1\right) ^{23}},\\ W_{10}(g_1,\ldots , g_{10})- & {} \frac{687970713600 r^{10} \left( R_1(r) \tan ^{-1}(r)+R_2(r) \sinh ^{-1}(r)+R_3(r)\right) }{\left( r^2+1\right) ^{28}}, \end{aligned}$$

where

$$\begin{aligned}{} & {} P_1(r)=r \left( 32 r^6+343 r^4+630 r^2+315\right) ,\ P_2(r)=-105 \left( r^2+1\right) ^2 \left( r^2+3\right) ,\\{} & {} Q_1(r)=420 \left( r^2+1\right) ^2,\ Q_2(r)=r\sqrt{r^2+1} \left( 24 r^6+483 r^4+770 r^2+315\right) ,\\{} & {} Q_3(r)=-105 \sqrt{r^2+1}\left( r^2+1\right) ^2 \left( r^2+7\right) ,\ R_1(r)=-21 \left( r^2+1\right) ^2 \left( r^2+11\right) , \\{} & {} R_2(r)=21 \left( 10 r^2+11\right) \sqrt{r^2+1},\ R_3(r)=r^3 \left( 4 r^4+119 r^2+119\right) . \end{aligned}$$

Clearly, we note that all $W_j\ne 0$ for $j=1,\ldots ,7$ and $r\in (0,1)$. For the functions $W_8$, $W_9$ and $W_{10}$ we use the same arguments as in the proof of statement (a), that is, we will verify that these functions are monotonic. We are going to verify that the function $W_8$ is monotonic. The other cases are identical and for this reason their proofs will be omitted. In order to prove that the sign of $W_8$ for $0<r<1$ is definite, it is enough to study the function $\tilde{W}_8(r)=P_1(r)+P_2(r) \tan ^{-1}(r)$ for $r\in (0, 1)$. For this purpose, we compute the fifth derivative of $\tilde{W}_8(r)$ to obtain

$$\begin{aligned} \text {sgn}\big (\tilde{W}_8^{(5)}(r)\big )=\text {sgn}\left( \frac{r^2}{r^2+1}\right) . \end{aligned}$$

As $\tilde{W}_8^{(4)}(0)=0$, it follows that the fourth derivative of $W_8$ is monotone increasing. Thus $W_8$ is monotone. Proceeding in a similar way, we obtain that $W_8<0$ for all $r\in (0, 1)$.

Applying Theorem 6 we have that there exists a linear combination of the functions $g_j$, $j=1,\ldots ,10$ with at most nine zeros. Thus, there exist $r_k$, $k=1,\ldots ,9$ in (0, 1) and coefficients $a_ {ij}$, $b_ {ij}$, $c_ {ij}$, $d_ {ij}$, $e_ {ij}$, $f_ {ij}$ in $\mathbb {R}$ such that $f_1 (r_k) =0$ and $f'_1(r_k)\ne 0$.

In summary, applying the averaging method of first order, we found that there exist discontinuous polynomial systems (3) having at most nine limit cycles which bifurcate from periodic orbits of the uniform isochronous center of system (1).

For the proof of the second part of statement (b) we are going to give a concrete example to obtain the maximum number of limit cycles after the proof. $\square$

Proof of statement (c) of Theorem 1

The proof of this statement follows the steps of the previous items. So, for $m=3$ in the equation (4) and using Theorem 3 given in the Appendix 1, the averaging function is

$$\begin{aligned} f_1(r)=\int _0^{\pi /2} F_1^1(\theta , r)d\theta +\int _{\pi /2}^{\pi } F_{1}^2(\theta , r)d\theta +\int _{\pi }^{2\pi } F_{1}^3(\theta , r) d\theta , \end{aligned}$$

(13)

where the expressions $F_{1}^1$, $F_{1}^2$ and $F_{1}^3$ which are given in the Appendix 1. Calculating the integrals in (13) using Lemma 1, we obtain

$$\begin{aligned} f_1(r)= & {} \frac{1}{8 \pi r \sqrt{r^2+1}}(k_0 g_0 + k_1 g_1+ k_2 g_2+ k_3 g_3\nonumber \\{} & {} + k_4 g_4+ k_5 g_5+ k_6 g_6+ k_7 g_7+ k_8 g_8 \nonumber \\{} & {} + k_9 g_9+k_{10} g_{10}+ k_{11} g_{11}+ k_{12} g_{12}+ k_{13} g_{13}+ k_{14} g_{14}+ k_{15} g_{15}+ k_{16} g_{16}\nonumber \\{} & {} + k_{17} g_{17}+ k_{18} g_{18}+k_{19} g_{19}+ k_{20} g_{20}+ k_{21} g_{21}+ k_{22} g_{22}+ k_{23} g_{23}+ k_{24} g_{24}),\nonumber \\ \end{aligned}$$

(14)

where

$$\begin{aligned} \alpha _0= & {} -2 \pi \left( a_{12}-a_{30}-b_{03}+b_{21}+c_{12}-c_{30}\right. \\{} & {} \left. -d_{03}+d_{21}+2 e_{12}-2 e_{30}-2 f_{03}+2 f_{21}\right) ,\\ \alpha _1= & {} 2 \left( a_{11}+b_{00}-b_{02}+b_{20}+c_{11}+d_{00}\right. \\{} & {} \left. -d_{02}+d_{20}+2 e_{11}+2 f_{00}-2 f_{02}+2 f_{20}\right) ,\\ \alpha _2= & {} -2 \pi \left( 2 a_{12}-a_{30}-2 b_{03}+b_{21}+2 c_{12}-c_{30}\right. \\ {}{} & {} \left. -2 d_{03}+d_{21}+4 e_{12}-2 e_{30}-4 f_{03}+2 f_{21}\right) ,\\ \alpha _3= & {} 2 \left( 2 a_{11}+b_{00}-b_{02}+b_{20}+2 c_{11}+d_{00}-d_{02}+d_{20}+4 e_{11}+2 f_{00}-2 f_{02}+2 f_{20}\right) ,\\ \alpha _4= & {} -2 \pi \left( a_{12}-b_{03}+c_{12}-d_{03}+2 e_{12}-2 f_{03}\right) ,\\ \alpha _5= & {} 2 \left( a_{11}+c_{11}+2 e_{11}\right) ,\quad \alpha _6=2 a_{00},\quad \alpha _7=a_{11},\quad \alpha _8=-4 \left( a_{11}+b_{00}-b_{02}+b_{20}\right) ,\\ \alpha _9= & {} -2 \left( 3 a_{11}+b_{00}-b_{02}+b_{20}\right) ,\quad \alpha _{10}=-2 c_{00},\quad \alpha _{11}=2 \left( a_{03}-c_{03}\right) ,\\ \alpha _{12}= & {} 2 \left( a_{02}+a_{20}-c_{02}-c_{20}\right) ,\quad \alpha _{13}=2 \left( a_{00}-a_{02}+a_{20}-c_{00}+c_{02}-c_{20}\right) ,\\ \alpha _{14}= & {} 2 \left( 2 a_{02}+b_{11}-2 c_{02}-d_{11}\right) ,\quad \alpha _{15}=2 \left( a_{02}-a_{20}+b_{11}-c_{02}+c_{20}-d_{11}\right) ,\\ \alpha _{16}= & {} 2 \left( a_{00}-a_{02}+a_{20}-b_{11}-c_{00}+c_{02}-c_{20}+d_{11}\right) ,\\ \alpha _{17}= & {} 2 \left( 2 a_{03}-a_{21}+b_{12}-2 c_{03}+c_{21}-d_{12}\right) ,\\ \alpha _{18}= & {} 2 \left( a_{03}-a_{21}+b_{12}-b_{30}-c_{03}+c_{21}-d_{12}+d_{30}\right) ,\quad \alpha _{19}=-4 e_{11},\\ \alpha _{20}= & {} 2 a_{01}-2 a_{03}+\pi a_{10}+\pi a_{12}+2 a_{21}+\pi a_{30}-2 c_{01}+2 c_{03}+\pi c_{10}+\pi c_{12}-2 c_{21}\\{} & {} \quad +\pi c_{30}+2 \pi e_{10}+2 \pi e_{12}+2 \pi e_{30},\\ \alpha _{21}= & {} a_{11}+b_{00}+3 b_{02}+b_{20}-2 c_{11}+4 d_{02}-4 f_{00}-4 f_{02}-4 f_{20},\\ \alpha _{22}= & {} 2 \left( 2 b_{00}-c_{11}+d_{00}+d_{02}-d_{20}-6 f_{00}+2 f_{02}-2 f_{20}\right) ,\\ \alpha _{23}= & {} 2 \pi \left( a_{12}-a_{30}-b_{03}+b_{21}+c_{12}-c_{30}-d_{03}+d_{21}+2 e_{12}-2 e_{30}-2 f_{03}+2 f_{21}\right) ,\\ \alpha _{24}= & {} 2 a_{01}-2 a_{03}+\pi a_{10}+3 \pi a_{12}+2 a_{21}-\pi a_{30}+\pi b_{01}-3 \pi b_{03}+2 b_{10}-2 b_{12}\\{} & {} \quad +\pi b_{21}+2 b_{30}-2 c_{01}+2 c_{03}+\pi c_{10}+3 \pi c_{12}-2 c_{21}-\pi c_{30}+\pi d_{01}-3 \pi d_{03}\\{} & {} \quad -2 d_{10}+2 d_{12}+\pi d_{21}-2 d_{30}+2 \pi e_{10}+6 \pi e_{12}-2 \pi e_{30}+2 \pi f_{01}-6 \pi f_{03}+2 \pi f_{21}, \end{aligned}$$

$$\begin{aligned} \begin{aligned} g_0=&\,1,\quad g_1=r,\quad g_2=r^2,\quad g_3=r^3,\quad g_4=r^4,\\&\quad g_5=r^5,\quad g_6=\left( r^2+1\right) ^{5/2} \tan ^{-1}(r),\\ g_7=&\,r^4 \sqrt{r^2+1} \left( \sinh ^{-1}(r)-2 \tanh ^{-1}\left( \frac{\sqrt{r^2+1}-1}{r}\right) \right) ,\\ g_8=&\frac{1}{2} \sqrt{r^2+1} \sinh ^{-1}(r),\quad g_9=\frac{1}{2} r^2 \sqrt{r^2+1} \sinh ^{-1}(r),\\ g_{10}=&\left( r^2+1\right) ^{5/2} \tan ^{-1}(r),\quad g_{11}=r^4 \sqrt{r^2+1} \ln \left( r^2+1\right) ,\\ g_{12}=&\,r^4 \sqrt{r^2+1} \tan ^{-1}(r),\quad g_{13}=r^3 \sqrt{r^2+1},\\ g_{14}=&\,r^2 \sqrt{r^2+1} \tan ^{-1}(r),\quad g_{15}=\sqrt{r^2+1} \tan ^{-1}(r),\quad g_{16}=r \sqrt{r^2+1},\\ g_{17}=&\,r^2 \sqrt{r^2+1} \ln \left( r^2+1\right) ,\quad g_{18}=\sqrt{r^2+1} \ln \left( r^2+1\right) ,\\ g_{19}=&\left( r^2+1\right) ^{5/2} \sinh ^{-1}(r),\quad g_{20}=r^4 \sqrt{r^2+1},\quad g_{21}=r^2 \sqrt{r^2+1} \sinh ^{-1}(r),\\ g_{22}=&\sqrt{r^2+1} \sinh ^{-1}(r),\quad g_{23}=\sqrt{r^2+1},\quad g_{24}=r^2 \sqrt{r^2+1}. \end{aligned} \end{aligned}$$

(15)

Using the following relations among the coefficients $\alpha _0 + \alpha _4 - \alpha _2=0$, $\alpha _1 + \alpha _5 - \alpha _3=0$, $\alpha _0 + \alpha _{23}=0$, $\alpha _{14}-\alpha _{12}-\alpha _{15}=0$ and applying trigonometric identities, we verify that $g_{15} + 2 g_{14} + g_{12} = g_6$, $g_6 = g_{10}$, $g_{21} = 2 g_{9}$ and $g_{22} = 2 g_{8}$ for $0<r<1$. Thus, the function (14) can be written as

$$\begin{aligned} \begin{aligned} f_1(r)=&\frac{1}{8 \pi r \sqrt{r^2+1}}\left( A_0 G_0+ A_1 G_1+A_2 G_2+A_3 G_3+A_4 G_4+A_5 G_5+A_6 G_6\right. \\&+A_7 G_7+A_8 G_8+A_9 G_9+A_{10} G_{10}+A_{11} G_{11}+A_{12} G_{12}+A_{13} G_{13}\\&\left. +A_{14} G_{14}+A_{15} G_{15}\right) , \end{aligned} \end{aligned}$$

(16)

where

$$\begin{aligned} \begin{aligned}&A_0=\alpha _5,\quad A_1=\alpha _1,\quad A_2=\alpha _4,\quad A_3=\alpha _0,\quad A_4=\alpha _{16},\quad A_5=\alpha _{24},\\&A_6=\alpha _{13},\quad A_7=\alpha _{20},\quad A_8=\alpha _{18},\quad A_9=\alpha _{17},\quad A_{10}=\alpha _{11},\\&A_{11}=\alpha _{15}+\alpha _{6}+\alpha _{10},\quad A_{12}=\alpha _{12}+\alpha _6+\alpha _{10},\quad A_{13}=\alpha _8+2 \alpha _{22},\\&A_{14}=\alpha _9+ 2 \alpha _{21},\quad A_{15}=\alpha _{19}, \end{aligned} \end{aligned}$$

(17)

and

$$\begin{aligned} \begin{aligned}&G_0 =g_3+g_5,\quad G_1 =g_1+g_3,\quad G_2 = g_2+g_4,\quad G_3 =g_0+g_2-g_{23},\\&G_4 = g_{16},\quad G_{5} = g_{24},\quad G_6 = g_{13},\quad G_7 = g_{20},\quad G_8 = g_{12}+g_{14},\quad G_9 =g_{14}+g_{15},\\&G_{10} = g_{11},\quad G_{11} = g_{17},\quad G_{12} = g_{18},\quad G_{13} = \frac{1}{2}g_{22},\quad G_{14} =\frac{1}{2} g_{21},\quad G_{15} = g_{19}.\\ \end{aligned}\nonumber \\ \end{aligned}$$

(18)

For the 16 functions $G_i$ for $i=0,\ldots ,15$ given in (18), we define the ordered set of functions $\{G_4, G_5, G_6, G_7,\ldots , G_0, G_1\}$, which is an ECT-system on the subinterval (0.72, 1) of (0, 1). In fact, using the mathematic software, we calculate the Wronskians,

$$\begin{aligned} W_1(G_4)= & {} r,\\ W_2(G_4, G_5)= & {} r^2,\\ W_3(G_4, G_5, G_6)= & {} 2r^3,\\ W_4(G_4,\ldots , G_7)= & {} 12r^4,\\ W_5(G_4,\ldots , G_0)= & {} -\frac{36 \left( \sqrt{r^2+1}-1\right) ^3 W_{51}}{\left( r^2+1\right) ^{7/2}},\\ W_6(G_4,\ldots , G_1)= & {} \frac{540 \ W_{61}}{\left( r^2+1\right) ^{15/2}},\\ W_7(G_4,\ldots , G_1)= & {} -\frac{48600 \ W_{71}}{\left( r^2+1\right) ^{21/2}},\\ W_8(G_4,\ldots , G_1)= & {} \frac{30618000 \ W_{81}}{\left( r^2+1\right) ^{14}},\\ W_9(G_4,\ldots , G_1)= & {} \frac{1102248000\ r^2\ W_{91} }{\left( r^2+1\right) ^{22}},\\ W_{10}(G_4,\ldots , G_1)= & {} -\frac{132269760000\ r \ W_{10}^{(1)}}{\left( r^2+1\right) ^{30}},\\ W_{11}(G_4,\ldots , G_1)= & {} \frac{7.61873\ldots \times 10^{13}\ r^3 \ \left( W_{11}^{(1)} + W_{11}^{(2)} \right) }{\left( r^2+1\right) ^{38}},\\ W_{12}(G_4,\ldots , G_1)= & {} -\frac{3.83984\ldots \times 10^{17}\ r \ \left( W_{12}^{(1)}+W_{12}^{(2)}\right) }{\left( r^2+1\right) ^{91/2}},\\ W_{13}(G_4,\ldots , G_1)= & {} \frac{1.39340\ldots \times 10^{23} \ \left( W_{13}^{(1)}+W_{13}^{(2)}+W_{13}^{(3)} \right) }{\left( r^2+1\right) ^{52}},\\ W_{14}(G_4,\ldots , G_1)= & {} \frac{1.26409\ldots \times 10^{28}\ \left( W_{14}^{(1)}+W_{14}^{(2)}+W_{14}^{(3)}+W_{14}^{(4)}\right) }{\left( r^2+1\right) ^{121/2}},\\ W_{15}(G_4,\ldots , G_1)= & {} -\frac{6.30732\ldots \times 10^{34}\ \left( W_{15}^{(1)}+W_{15}^{(2)}+W_{15}^{(3)}+W_{15}^{(4)}\right) }{\left( r^2+1\right) ^{69}},\\ W_{16}(G_4,\ldots , G_1)= & {} -\frac{6.60891\ldots \times 10^{42}\ \left( W_{16}^{(1)}+W_{16}^{(2)}+W_{16}^{(3)}+W_{16}^{(4)}\right) }{\left( r^2+1\right) ^{157/2}}. \end{aligned}$$

where the expressions of the Wronskians are presented in the Appendix 1. Note that $W_j\ne 0$ for $j=1,2,3,4$ and $r\in (0,1)$. Taking into account the complexity of $W_j$ for $j=5,\ldots ,16$, we make the change variable $s=\sqrt{r^2+1}\in (1,\sqrt{2})$. Thus, for $W_{j1}$, $j=5,\ldots ,9$ and $W_{10}^{(1)}$, we obtain

$$\begin{aligned} \begin{aligned} W_{51}&=8 s^4+4 s^3-12 s^2-15 s-5,\\ W_{61}&=(s-1)^3 \left( 16 s^5-24 s^3+9 s+3\right) ,\\ W_{71}&=(s-1)^4 \left( 8 s^3+4 s^2-4 s-1\right) ,\\ W_{81}&=(s-1)^4,\\ W_{91}&=(s-1)^4 \left( 280 s^{10}-160 s^9-32 s^7+35 s^6-36 s^5+35 s^4-32 s^3-160 s+280\right) ,\\ W_{10}^{(1)}&=(s-1)^6 \left( 2240 s^{18}+1152 s^{17}-1152 s^{16}+128 s^{15}+384 s^{14}+384 s^{13}+19984 s^{12}\right. \\&+117984 s^{11}-66291 s^{10}-343666 s^9-66291 s^8+117984 s^7+19984 s^6+384 s^5\\&\left. +384 s^4+128 s^3-1152 s^2+1152 s+2240\right) . \end{aligned} \end{aligned}$$

Since these expressions are polynomials according to Sturm’s Theorem, they do not vanish for $s\in (1, \sqrt{2})$. However, for $W_j$, where $j=11,\ldots ,16$, we have

$$\begin{aligned} W_{11}^{(1)}= & {} \,-8960 s^{28}+65536 s^{27}-241920 s^{26}+753664 s^{25}+362880 s^{24}-1253376 s^{23}\\{} & {} -5456640 s^{22}+21827584 s^{21}-28885500 s^{20}+1129984 s^{19}+38481940 s^{18}\\{} & {} -25074432 s^{17}+89115705 s^{16}-222342720 s^{15}+222342720 s^{13}\\{} & {} -89115705 s^{12}+25074432 s^{11}-38481940 s^{10}-1129984 s^9+28885500 s^8\\{} & {} -21827584 s^7+5456640 s^6+1253376 s^5-362880 s^4-753664 s^3+241920 s^2\\{} & {} -65536 s+8960,\\ W_{11}^{(2)}= & {} \,-210 s^4 \left( 4480 s^{20}-1792 s^{18}+792 s^{16}+792 s^{14}+313167 s^{12}-877428 s^{10}\right. \\{} & {} \left. +313167 s^8+792 s^6+792 s^4-1792 s^2+4480\right) \ln (s),\\ W_{12}^{(1)}= & {} \,-s \left( s^2-1\right) \left( 65536 s^{27}-564480 s^{26}+2064384 s^{25}-4300800 s^{24}+7593984 s^{23}\right. \\{} & {} +14474880 s^{22}-65286144 s^{21}+63020160 s^{20}+20160000 s^{19}-262298260 s^{18}\\{} & {} +615087360 s^{17}-448769160 s^{16}-467987520 s^{15}+824895225 s^{14}\\{} & {} +162493056 s^{13}+272267205 s^{12}-1895959296 s^{11}-414022140 s^{10}\\{} & {} +2941091840 s^9-448702380 s^8-1437843456 s^7+448260120 s^6\\{} & {} \left. +137281536 s^5-89863200 s^4+41779200 s^3-8568000 s^2+179200\right) ,\\ W_{12}^{(2)}= & {} \,630 s^7 \left( 18816 s^{18}-79872 s^{16}+113080 s^{14}-67528 s^{12}-171921 s^{10}+1671166 s^8\right. \\{} & {} \left. -4512997 s^6+5343246 s^4-2769200 s^2+476000\right) \ln (s),\\ W_{13}^{(1)}= & {} \,-1179648 s^{27}+752640 s^{26}+7143424 s^{25}-3906560 s^{24}-15974400 s^{23}\\{} & {} +9941120 s^{22}-66232320 s^{21}+33741120 s^{20}+1017390080 s^{19}-624390200 s^{18}\\{} & {} -4255838720 s^{17}+2550766540 s^{16}+8824187008 s^{15}-4993175635 s^{14}\\{} & {} -10220039808 s^{13}+5499143720 s^{12}+6553920512 s^{11}-3430324275 s^{10}\\{} & {} -1937993728 s^9+854882770 s^8+392407680 s^6+94617600 s^5-408267720 s^4\\{} & {} +134332800 s^2-15904000,\\ W_{13}^{(2)}= & {} \,105 \sqrt{s^2-1} \left( 7168 s^{26}-40448 s^{24}+69760 s^{22}+425920 s^{20}-5808440 s^{18}\right. \\{} & {} +22854348 s^{16}-42668703 s^{14}+41412525 s^{12}-20293350 s^{10}+2871044 s^8\\{} & {} \left. +3559424 s^6-3808768 s^4+1637120 s^2-217600\right) \tan ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{13}^{(3)}= & {} \,420 \left( 12672 s^{20}-47872 s^{18}+70168 s^{16}-49992 s^{14}+3583 s^{12}-80212 s^{10}\right. \\{} & {} \left. +642223 s^8-1318192 s^6+1150032 s^4-436480 s^2+54400\right) \ln (s),\\ W_{14}^{(1)}= & {} \,-58982400 s^{25}+39997440 s^{24}+1043660800 s^{23}-684113920 s^{22}\\{} & {} -6228131840 s^{21}+3832319680 s^{20}+19173769216 s^{19}-11127060000 s^{18}\\{} & {} -34227699712 s^{17}+20263629960 s^{16}+34069839360 s^{15}-26550401500 s^{14}\\{} & {} -10378307968 s^{13}+27882409135 s^{12}-18292871296 s^{11}-24129810145 s^{10}\\{} & {} +27479485440 s^9+16116801470 s^8-17532416000 s^7-7510995520 s^6\\{} & {} +5740134400 s^5+2179277800 s^4-788480000 s^3-327958400 s^2+15904000,\\ W_{14}^{(2)}= & {} \,-4620 s^3 \sqrt{s^2-1} \left( 1024 s^{20}-4608 s^{18}+8064 s^{16}+2880 s^{14}+70520 s^{12}\right. \\{} & {} -740076 s^{10}+2121647 s^8-2861640 s^6+2028960 s^4-739200 s^2\\{} & {} \left. +112000\right) \sinh ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{14}^{(3)}= & {} \,105 \sqrt{s^2-1} \left( 358400 s^{24}-6131200 s^{22}+34995840 s^{20}-99496320 s^{18}\right. \\{} & {} +157124352 s^{16}-141607620 s^{14}+76145289 s^{12}-41509226 s^{10}+45003420 s^8\\{} & {} \left. -40685568 s^6+19810560 s^4-4258560 s^2+217600\right) \tan ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{14}^{(4)}= & {} \,420 \left( 11264 s^{24}-56320 s^{22}+112640 s^{20}-112640 s^{18}+93720 s^{16}+243160 s^{14}\right. \\{} & {} -3100715 s^{12}+9907200 s^{10}-15175511 s^8+12518032 s^6-5478160 s^4\\{} & {} \left. +1091840 s^2-54400\right) \ln (s),\\ W_{15}^{(1)}= & {} \,2\sqrt{s^2-1}\left( s^2-1\right) \left( 1590400 \left( s^2-1\right) ^9+13440 (2880 s+281) \left( s^2-1\right) ^8\right. \\{} & {} -140 (133120 s+157113) \left( s^2-1\right) ^7-560 (18304 s+167083) \left( s^2-1\right) ^6\\{} & {} +9 (2123264 s-13008513) \left( s^2-1\right) ^5+2 (7683104 s-22202691) \left( s^2-1\right) ^4\\{} & {} +(6892288 s+2123562) \left( s^2-1\right) ^3+48 (45032 s+3661) \left( s^2-1\right) ^2\\{} & {} \left. -7 (16640 s+46179) \left( s^2-1\right) -14 (2080 s+5131)\right) ,\\ W_{15}^{(2)}= & {} \,-21 s \left( 217600 s^{20}+1647360 s^{18}-29310720 s^{16}+137373440 s^{14}\right. \\{} & {} -330141828 s^{12}+476816010 s^{10}-441037635 s^8+266614200 s^6\\{} & {} \left. -103236000 s^4+23408000 s^2-2352000\right) \sinh ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{15}^{(3)}= & {} \,-21 \left( 2352000 s^{20}-23408000 s^{18}+103236000 s^{16}-266614200 s^{14}\right. \\{} & {} +441037635 s^{12}-476816010 s^{10}+330141828 s^8-137373440 s^6\\{} & {} \left. +29310720 s^4-1647360 s^2-217600\right) \tan ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{15}^{(4)}= & {} \,84 \sqrt{s^2-1} \left( 54400 s^{20}+439040 s^{18}-7101360 s^{16}+30850960 s^{14}\right. \\{} & {} -67881457 s^{12}+87276684 s^{10}-67881457 s^8+30850960 s^6-7101360 s^4\\{} & {} \left. +439040 s^2+54400\right) \ln (s),\\ W_{16}^{(1)}= & {} \sqrt{s^2-1}\left( \left( s^2-1\right) ^8-6599880 \left( s^2-1\right) ^7+210 (239616 s-86321) \left( s^2-1\right) ^6\right. \\{} & {} -21 (212992 s+152179) \left( s^2-1\right) ^5-3 (7987200 s-853873) \left( s^2-1\right) ^4\\{} & {} -26 (139264 s+161315) \left( s^2-1\right) ^3+(793172-372736 s) \left( s^2-1\right) ^2\\{} & {} \left. -36075\left( s^2-1\right) -7605\right) ,\\ W_{16}^{(2)}= & {} \,-3 s \left( 362880 s^{16}-6135360 s^{14}+39797520 s^{12}-132292776 s^{10}+247448841 s^8\right. \\{} & {} \left. -268529240 s^6+166200160 s^4-54096000 s^2+7235200\right) \sinh ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{16}^{(3)}= & {} \,-1248 s^2 \left( 25725 s^{12}-169050 s^{10}+458220 s^8-663040 s^6+542976 s^4\right. \\{} & {} \left. -238336 s^2+43520\right) \tan ^{-1}\left( \sqrt{s^2-1}\right) ,\\ W_{16}^{(4)}= & {} \,384 s^2 \left( s^2-1\right) \sqrt{s^2-1} \left( 2835 s^{12}-43680 s^{10}+244335 s^8-650832 s^6\right. \\{} & {} \left. +867984 s^4-562432 s^2+141440\right) \ln (s). \end{aligned}$$

Now, using similar arguments as in the proof of statement (a), we will verify that the functions are monotonic. To show that $W_{11}$ is monotonic, we calculate the twenty-fifth derivative and we obtain

$$\begin{aligned} W_{11}^{(25)}= & {} \,-\frac{1.46..\times 10^{18}}{s^{21}}\left( 311830289971200 s^{24}-244373125201920 s^{23}\right. \\{} & {} +\, 66820776422400 s^{22}-8006526894080 s^{21}+399782423040 s^{20}\\{} & {} -\, 579394816 s^{18}+6651216 s^{16}+525096 s^{14}+37997596 s^{12}-39922974 s^{10}\\{} & {} \left. +\, 10334511 s^8+36036 s^6+96096 s^4-1188096 s^2+37623040\right) . \end{aligned}$$

As $W_{11}^{(24)}(1)<0$ it follows that the twenty-fourth derivative of $W_{11}$ is monotone decreasing. Therefore $W_{11}$ is monotone. Thus, in a similar way we can obtain that $W_{11}<0$ for all $s\in (1, \sqrt{2})$. For the remaining Wronskians, we proceed in the same way, proving that they are monotonous and for this reason we will omit the proofs. Applying Theorem 6 we have that there exists a linear combination of the functions $G_i$ for $i=1,\ldots ,16$ with at most fifteen zeros. Then, there exist $r_k$, $k=1,\ldots ,15$ in (0.72, 1) and coefficients $a_{ij}$, $b_{ij}$, $c_{ij}$, $d_{ij}$, $e_{ij}$ and $f_{ij}$ in $\mathbb {R}$ such that $f_1(r_k)=0$ and $f_1'(r_k)\ne 0$.

In short, by the averaging method of first order, there exist discontinuous polynomial systems (3) having at most 15 limit cycles which bifurcate periodic orbits of the uniform isochronous center of system (1). This concludes the proof of Theorem 1. $\square$

2.1 Examples

In this subsection, we complete the proof of Theorem 1. Initially we consider the statement (a), that is, we give conditions on the parameters in order to obtain 3, 2, 1 or 0 limit cycles.

First, we construct an example with three limit cycles. Since our objective is to show an example where the function $f_1$ in (9) has three non-degenerate zeros, we will fix the three different roots in the interval (0, 1) and then, by virtue of Theorem 6, we will proceed to find the appropriate coefficients. Without loss of generality, we assume that the zeros are $r_k=k/5$, $k=1,2,3$, so by (9) the following equality must be satisfied

$$\begin{aligned} \alpha _1 G_1(r_k)+ \alpha _2 G_2(r_k)+ \alpha _3 G_3(r_k) + \alpha _4 G_4(r_k)=0,\quad k=1,2,3. \end{aligned}$$

(19)

Solving the previous linear systems, we find

$$\begin{aligned} \alpha _1=16.0547,\ \alpha _2=-24.0775,\ \alpha _3=-9.57552,\ \alpha _4=10. \end{aligned}$$

In this case, the averaged function $f_1(r)$ in (9) takes the form

$$\begin{aligned} f_1(r)=&\frac{0.397887 }{r}\left[ -0.957552 \ln \left( \sqrt{r^2+1}+r\right) +\left( r^4+2. r^2+1\right) \tan ^{-1}(r)\right. \nonumber \\&\left. +r \left( -2.40775 r^3+r^2-0.957552 \sqrt{r^2+1}-0.802285 r+1\right) \right] . \end{aligned}$$

(20)

For the graph of $f_1$ in (20) see Fig. 1.

Fig. 1

The graph of the averaged function $f_1(r)$ in (20)

Now, the objective is to complement the information by exhibiting one explicit example possessing exactly three limit cycles and showing them numerically. In order to describe the three vector fields $Z^i$, $i=1,2,3$ using the values from (8), we obtain the next relation on the coefficients

$$\begin{aligned} \begin{array}{ll} c_{00}=a_{00}-5,&{} e_{10}=-\dfrac{a_{01}}{\pi }-\dfrac{1}{2} a_{10}+\dfrac{c_{01}}{\pi }-\dfrac{1}{2} c_{10}-3.83206,\\ \\ f_{00}=\dfrac{1}{2} b_{00}+\dfrac{1}{2} d_{00}+2.39388,&{} f_{01}=-\dfrac{1}{2} b_{01}-\dfrac{b_{10}}{\pi }-\dfrac{1}{2} d_{01}+\dfrac{d_{10}}{\pi }+2.55518. \end{array} \end{aligned}$$

The rest of the coefficients are arbitrary, and for our purpose, we take $a_{00}=2$, $a_{01}=-1$, $a_{10}=-3$, $c_{01}=4$, $c_{10}=\frac{2}{3}$, $b_{00}=5$, $b_{10}=-1$, $d_{00}= \frac{1}{2}$, $b_{01}=-3$, $d_{01}=0$, $d_{10}=-2$, $e_{00}=1$, $e_{01}=2$, $f_{10}=-1$. Thus, the systems acquire the following form

$$\begin{aligned} Z^1(x,y)={\left\{ \begin{array}{ll} \dot{x}= -y+x^2 y +\epsilon (-3 x-y+2),\\ \dot{y}= x+xy^2+\epsilon (-x-3 y+5),\\ \end{array}\right. } \end{aligned}$$

(21)

$$\begin{aligned} Z^2(x,y)={\left\{ \begin{array}{ll} \dot{x}= -y+x^2 y +\epsilon \left( \frac{2 x}{3}+4 y-3\right) ,\\ \dot{y}= x+xy^2+\epsilon \left( \frac{1}{2}-2 x\right) ,\\ \end{array}\right. } \end{aligned}$$

(22)

and

$$\begin{aligned} Z^3(x,y)={\left\{ \begin{array}{ll} \dot{x}= -y+x^2 y + \epsilon (-1.07384 x+2 y+1),\\ \dot{y}= x+xy^2+ \epsilon (- x+3.73687 y+5.14388).\\ \end{array}\right. } \end{aligned}$$

(23)

We know that for $|\epsilon |\ne 0$ sufficiently small, according to Theorem 1 item (a), the systems (21), (22) and (23) have exactly three limit cycles surrounding the origin by the averaging method of first order. In Fig. 2 we exhibit numerically these three limit cycles.

Fig. 2

The three limit cycles for (21), (22) and (23) for $\epsilon =10^{-4}$

Now, we provide an example where the function $f_1$ in (9) has two zeros. We can choose without loss of generality that $\alpha _4=0$ in (19) and the zeros $r_1=5/10$ and $r_2=7/10$ which satisfy the equation (19). Then solving the system of equations in (19) for $r_1$ and $r_2$, we get

$$\begin{aligned} \alpha =-19.6255,\ \alpha _2=9.04291,\ \alpha _3=2. \end{aligned}$$

Thus, from (8) we have the following relations for the coefficients

$$\begin{aligned} c_{00}=&\,a_{00},&e_{10}=&\,-\frac{a_{01}}{\pi }-\frac{1}{2} a_{10}+\frac{c_{01}}{\pi }-\frac{1}{2} c_{10}+1.43922,\\ f_{00}=&\frac{1}{2} b_{00}+\frac{1}{2} d_{00}-\frac{1}{2},&f_{01}=&\,-\frac{1}{2} b_{01}-\frac{b_{10}}{\pi }-\frac{1}{2} d_{01}+\frac{d_{10}}{\pi }-3.12349. \end{aligned}$$

For this case, the function $f_1(r)$ is of the form

$$\begin{aligned} f_1(r)=\frac{9.04291 \left( r^2+1\right) r^2-19.6255 r^2+2 \left( r \sqrt{r^2+1}+\ln \left( \sqrt{r^2+1}+r\right) \right) }{8 \pi r}.\nonumber \\ \end{aligned}$$

(24)

The graph of the function $f_1$ in (24) is shown in Fig. 3.

Fig. 3

The graph of the averaged function $f_1(r)$ in (24)

Similarly to the previous example, we show an example where the function $f_1$ has a single zero. Then, proceeding in a similar way, we can take $\alpha _i=0$ for $i=3,4$ in (19) and $r_1=5/10$, such that from (8) we get $\alpha _1=-1.25$ and $\alpha _2=1$. In addition, we have the following relations on the parameters

$$\begin{aligned} c_{00}=&\,a_{00},&e_{10}=&\,-\frac{a_{01}}{\pi }-\frac{1}{2} a_{10}+\frac{c_{01}}{\pi }-\frac{1}{2} c_{10}+\frac{1}{2 \pi },\\ f_{00}=&\,\frac{1}{2} b_{00}+\frac{1}{2} d_{00},&f_{01}=&\,-\frac{1}{2} b_{01}-\frac{b_{10}}{\pi }-\frac{1}{2} d_{01}+\frac{d_{10}}{\pi }-0.198944. \end{aligned}$$

So, the averaged function $f_1$ becomes

$$\begin{aligned} f_1(r)=0.0397887 r \left( r^2-0.25\right) . \end{aligned}$$

(25)

See Fig. 4 for the graphical representation of the function $f_1$ in (25).

Fig. 4

The graph of the averaged function $f_1(r)$ in (25)

Finally, we give an example where the function $f_1$ has no zeros. For this, it is enough to consider $\alpha _i=0$ for $i=2,3,4$ in (19), such that the following equalities are satisfied

$$\begin{aligned} c_{00}= a_{00},\ e_{10}=-\frac{a_{01}}{\pi }-\frac{1}{2} a_{10}+\frac{c_{01}}{\pi }-\frac{1}{2} c_{10}, \ f_{00}=\frac{1}{2} b_{00}+\frac{1}{2} d_{00}. \end{aligned}$$

Thus, the function (9) assumes the form $f_1(r)=(\alpha _1 r)/8 \pi$. Clearly $f_1(r)$ has no solution in (0, 1), and therefore, there are no limit cycles bifurcating from (1).

Now we are going to consider item (b). More precisely, we provide conditions on the parameters $a_ {ij}$, $b_ {ij}$, $c_ {ij}$, $d_ {ij}$, $e_ {ij}$, $f_ {ij}$ in order to obtain the maximum number of limit cycles given in item (b) of Theorem 2.

Proceeding in the same way as the examples given for the second part of statement (b) of Theorem 1, we will assume that the zeros of the function $f_1$ in (11) are of the form $r_k=k/11$ with $k=1,\ldots ,9$. Then, by (11) we obtain

$$\begin{aligned} \beta _1=&\,1172.65,&\beta _2=&\,3.45878\times 10^{-7},&\beta _3=&\,506.833,&\beta _4=&\,0.000016853,\\ \beta _5=&\,-822.627,&\beta _6=&\,-1215.06,&\beta _7=&\,524.198,&\beta _8=&\,367.436,\\ \beta _9=&\,-325.023,&\beta _{10}=&\,100.{} & {} {} & {} \end{aligned}$$

So, from (12) we have the following relations among the coefficients

$$\begin{aligned}{} & {} c_{00}=a_{00}-385.022, \ c_{01}=a_{01}+\frac{1}{2} \pi a_{10}+\frac{1}{2} \pi c_{10}+\pi e_{10}-8.42652\times 10^{-6},\nonumber \\{} & {} c_{20}=a_{20}+127.264,\ c_{02}=a_{02}-4.34111,\nonumber \\{} & {} d_{10}=\frac{1}{2} \pi b_{01}+b_{10}+\frac{1}{2} \pi d_{01}+\pi f_{01}+8.25358\times 10^{-6},\ d_{11}= b_{11}+332.909,\nonumber \\{} & {} e_{11}=\frac{1}{2} a_{11}+\frac{1}{2} c_{11}+205.657,\ f_{00}=\frac{1}{2} b_{00}+\frac{1}{2} d_{00}+192.511,\nonumber \\{} & {} f_{20}=\frac{1}{2} b_{20}+\frac{1}{2} d_{20}-4.07322,\ f_{02}=\frac{1}{2} b_{02}+\frac{1}{2} d_{02}+90.3283. \end{aligned}$$

(26)

From the above, the function $f_1$ in (11) becomes

$$\begin{aligned} \begin{aligned} f_1(r)=&\,r \left( r^2 \left( 506.833\, -822.627 \sqrt{r^2+1}\right) +0.000016853 r^3-1215.06 \sqrt{r^2+1}\right. \\&\left. +3.45878 \times 10^{-7} r+1172.65\right) +100 \left( r^2-3.25023\right) \sinh ^{-1}(r)\\&+\left( 524.198 r^4+891.634 r^2+367.436\right) \tan ^{-1}(r). \end{aligned} \end{aligned}$$

(27)

Fig. 5 shows the graph of the function $f_1$ in (27).

Fig. 5

The graph of the averaged function $f_1(r)$ in (27)

2.2 Proof of Corollary 1

Proof of statement (a) of Corollary 1

It is observed that in (9) all the polynomial perturbations are homogeneous, that is, $a_{00}=0$, $b_{00}=0$, $c_{00}=0$, $d_{00}=0$, $e_{00}=0$ and $f_{00}=0$ in the averaging function (9), we get

$$\begin{aligned} \begin{aligned} f_1(r)=&\frac{r}{8\pi }\left( r^2 \left( 2 a_{01}+\pi a_{10}-2 c_{01}+\pi c_{10}+2 \pi e_{10}\right) +2 a_{01}+\pi a_{10}+\pi b_{01}\right. \\&+\left. 2 b_{10}-2 c_{01}+\pi c_{10}+\pi d_{01}-2 d_{10}+2 \pi e_{10}+2 \pi f_{01}\right) . \end{aligned} \end{aligned}$$

(28)

Note that the above equation has a root $r\in (0,1)$ such that $f_1(r)=0$. Therefore, there exist discontinuous polynomial systems (3) with homogeneous polynomial perturbations for $m=1$ with at most one limit cycle which bifurcate from the periodic orbits of the uniform isochronous center of system (1) applying the averaging theory of first order. $\square$

Proof of statement (b) of Corollary 1

Similarly to item (a), assuming that all the polynomial perturbations are homogeneous, that is, $a_{00}=b_{00}=c_{00}=d_{00}=e_{00}=f_{00}=0$ in (11), we have the following equalities, $\beta _1+\beta _5=0$ and $\beta _7+\beta _9=0$. Then, by a convenient regrouping, the function $f_1$ in (11) can be rewritten as

$$\begin{aligned} f_1(r)=\dfrac{1}{8 \pi r}\left( \gamma _1 G_1+\gamma _2 G_2+\gamma _3 G_3+\gamma _4 G_4+\gamma _5 G_5+\gamma _6 G_6+\gamma _7 G_7+\gamma _8 G_8\right) ,\nonumber \\ \end{aligned}$$

(29)

where $G_1=g_2$, $G_2=g_3$, $G_3=g_4$, $G_4=g_5$, $G_5=g_7$, $G_6=g_{10}$, $G_7=g_8-g_1$, $G_8=g_6-g_9$ and

$$\begin{aligned} \gamma _1=&\,2 a_{01}+\pi a_{10}+\pi b_{01}+2 b_{10}-2 c_{01}+\pi c_{10}+\pi d_{01}-2 d_{10}+2 \pi e_{10}+2 \pi f_{01}, \\ \gamma _2=&\,-2 a_{02}+2 a_{20}+2 c_{02}-2 c_{20},\\ \gamma _3=&\,2 a_{01}+\pi a_{10}-2 c_{01}+\pi c_{10}+2 \pi e_{10},\\ \gamma _4=&\,2 a_{11}+2 c_{11}-4 e_{11},\\ \gamma _5=&\,2 a_{02}+2 a_{20}-2 c_{02}-2 c_{20},\\ \gamma _6=&\,-2 a_{11}+4 b_{02}-2 c_{11}+4 d_{02}+4 e_{11}-8 f_{02},\\ \gamma _7=&\,2 a_{02}-2 a_{20}+2 b_{11}-2 c_{02}+2 c_{20}-2 d_{11},\\ \gamma _8=&\,2 a_{11}-2 b_{02}+2 b_{20}+2 c_{11}-2 d_{02}+2 d_{20}-4 e_{11}+4 f_{02}-4 f_{20}. \end{aligned}$$

The Wronskians of the functions $G_j$, $j=1,\ldots ,8$ in the variable r are

$$\begin{aligned} \begin{aligned} W_1(G_1)&=r^2,\\ W_2(G_1, G_2)&=r^4,\\ W_3(G_1, G_2, G_3)&=2 r^6,\\ W_4(G_1,\ldots , G_4)&=\frac{6 r^8}{\left( r^2+1\right) ^{5/2}},\\ W_5(G_1,\ldots , G_5)&=\frac{24 r^{11}}{\left( r^2+1\right) ^{11/2}},\\ W_6(G_1,\ldots , G_6)&=\frac{96 r^{15}}{\left( r^2+1\right) ^9},\\ W_7(G_1,\ldots , G_7)&=\frac{2304 r^9 \left( S_1(r)\tan ^{-1}(r)+S_2(r)\right) }{\left( r^2+1\right) ^{13}},\\ W_8(G_1,\ldots , G_8)&=-\frac{884736 r^{13} \left( T_1(r) \tan ^{-1}(r)+T_2(r) \sinh ^{-1}(r)+T_3(r)\right) }{\left( r^2+1\right) ^{35/2}}, \end{aligned} \end{aligned}$$

with

$$\begin{aligned} \begin{array}{ll} S_1(r)=15 (r^2+1) (4 r^4+21 r^2+21),&{} S_2(r)=-r (16 r^6+228 r^4+525 r^2+315),\\ T_1(r)=-15 (r^2-3)(r^2+1),&{} T_2(r)=15 (4 r^2+3) \sqrt{r^2+1},\\ T_3(r)=2 r (r^4-45 r^2-45).&{} \end{array} \end{aligned}$$

Using the same arguments as in the proof of Theorem 1, it is verified that the functions $W_j\ne 0$ for $j=1,\ldots ,8$ in (0, 1). Thus, by Theorem 6 there is a linear combination of the functions $G_j$ with at most seven zeros. Therefore, there exist $r_k \in (0, 1)$ and the coefficients $a_ {ij}$, $b_ {ij}$, $c_ {ij}$, $d_ {ij}$, $e_ {ij}$, $f_ {ij}$ in $\mathbb {R}$, $i,j\in \{0,1,2\}$ such that $f_1 (r_k) =0$ and $f'_1(r_k)\ne 0$ for $k=1,\ldots ,7$.

In short, applying the averaging theory of first order for discontinuous piecewise differential systems, there exist discontinuous polynomial differential systems for $m=2$ with at most seven limit cycles which bifurcate from the periodic orbits of the uniform isochronous center of system (1). $\square$

Proof of statement (c) of Corollary 1

Analogous to the previous cases, we assume that all perturbation polynomials are homogeneous in (16). Moreover, we obtain the equalities $\alpha _{11}+\alpha _{12}=0$ and $\alpha _{5}+2\alpha _{2}+2\alpha _5=0$. Thus, the function in (16) is written as

$$\begin{aligned} f_1(r)=&\frac{1}{8 \pi r \sqrt{r^2+1}}\left( \rho _0 \bar{G}_0+ \rho _1 \bar{G}_1 + \rho _2 \bar{G}_2 +\rho _3 \bar{G}_3+\rho _4 \bar{G}_4+\rho _5 \bar{G}_5+\rho _6 \bar{G}_6 \right. \nonumber \\&\left. +\rho _7 \bar{G}_7+\rho _{8} \bar{G}_{8}+\rho _{9} \bar{G}_{9}+\rho _{10} \bar{G}_{10}+\rho _{11} \bar{G}_{11}+\rho _{12} \bar{G}_{12}+\rho _{13} \bar{G}_{13}\right) , \end{aligned}$$

(30)

where

$$\begin{aligned} \begin{aligned}&\bar{G}_0=G_0,\quad \bar{G}_1=G_2,\quad \bar{G}_2=G_5,\quad \bar{G}_3=G_6,\quad \bar{G}_4=G_7,\quad \bar{G}_5=G_3,\\&\bar{G}_6=G_1-4 G_{13},\quad \bar{G}_7=2G_{14},\quad \bar{G}_8=G_{10},\quad \bar{G}_{9}=G_8,\quad \bar{G}_{10}=G_9-G_4,\\&\bar{G}_{11}=G_{11},\quad \bar{G}_{12}=G_{12},\quad \bar{G}_{13}=G_{15}-4G_{13}. \end{aligned} \end{aligned}$$

and $\rho _0=A_4$, $\rho _1=A_3$, $\rho _2=A_{18}$, $\rho _3=A_9$, $\rho _4=A_{16}$, $\rho _5=A_1$, $\rho _6=A_2$, $\rho _7=A_6/2$, $\rho _8=A_7$, $\rho _9=A_8$, $\rho _{10}=A_{11}$, $\rho _{11}=A_{13}$, $\rho _{12}=A_{14}$, $\rho _{13}=A_{15}$.

We obtain the following Taylor expansions in the variable r around $r=0$ of the functions $\bar{G}_i$ for $i=0,\ldots ,13$

$$\begin{aligned} \bar{G}_0= & {} r^3 + r^5+\mathcal {O}(r^{16}),\\ \bar{G}_1= & {} r^2 + r^4+\mathcal {O}(r^{16}),\\ \bar{G}_2= & {} \frac{2 r^3}{3}+\frac{r^5}{5}-\frac{13 r^7}{140}+\frac{139 r^9}{2520}-\frac{2749 r^{11}}{73920}+\frac{52069 r^{13}}{1921920}-\frac{95923 r^{15}}{4612608}+\mathcal {O}(r^{16}),\\ \bar{G}_3= & {} r^3+\frac{r^5}{2}-\frac{r^7}{8}+\frac{r^9}{16}-\frac{5 r^{11}}{128}+\frac{7 r^{13}}{256}-\frac{21 r^{15}}{1024}+\mathcal {O}(r^{16}),\\ \bar{G}_4= & {} r^4+\frac{r^6}{2}-\frac{r^8}{8}+\frac{r^{10}}{16}-\frac{5 r^{12}}{128}+\frac{7 r^{14}}{256}+\mathcal {O}(r^{16}),\\ \bar{G}_5= & {} \frac{r^2}{2}+\frac{r^4}{8}-\frac{r^6}{16}+\frac{5 r^8}{128}-\frac{7 r^{10}}{256}+\frac{21 r^{12}}{1024}-\frac{33 r^{14}}{2048}+\mathcal {O}(r^{16}),\\ \bar{G}_6= & {} \frac{2 r^3}{3}+\frac{2 r^5}{15}-\frac{8 r^7}{105}+\frac{16 r^9}{315}-\frac{128 r^{11}}{3465}+\frac{256 r^{13}}{9009}-\frac{1024 r^{15}}{45045}+\mathcal {O}(r^{16}),\\ \bar{G}_7= & {} \frac{r^3}{2}+\frac{r^5}{6}-\frac{r^7}{15}+\frac{4 r^9}{105}-\frac{8 r^{11}}{315}+\frac{64 r^{13}}{3465}-\frac{128 r^{15}}{9009}+\mathcal {O}(r^{16}),\\ \bar{G}_8= & {} r^6-\frac{r^{10}}{24}+\frac{r^{12}}{24}-\frac{71 r^{14}}{1920}+\mathcal {O}(r^{16}),\\ \bar{G}_9= & {} r^3+\frac{7 r^5}{6}+\frac{3 r^7}{40}-\frac{17 r^9}{560}+\frac{649 r^{11}}{40320}-\frac{2911 r^{13}}{295680}+\frac{101237 r^{15}}{15375360}+\mathcal {O}(r^{16}),\\ \bar{G}_{10}= & {} \frac{2 r^3}{3}+\frac{r^5}{5}-\frac{13 r^7}{140}+\frac{139 r^9}{2520}-\frac{2749 r^{11}}{73920}+\frac{52069 r^{13}}{1921920}-\frac{95923 r^{15}}{4612608}+\mathcal {O}(r^{16}),\\ \bar{G}_{11}= & {} r^4-\frac{r^8}{24}+\frac{r^{10}}{24}-\frac{71 r^{12}}{1920}+\frac{31 r^{14}}{960}+\mathcal {O}(r^{16}),\\ \bar{G}_{12}= & {} r^2-\frac{r^6}{24}+\frac{r^8}{24}-\frac{71 r^{10}}{1920}+\frac{31 r^{12}}{960}-\frac{3043 r^{14}}{107520}+\mathcal {O}(r^{16}),\\ \bar{G}_{13}= & {} 2 r^3+\frac{5 r^5}{3}+\frac{r^7}{15}+\frac{2 r^9}{105}-\frac{8 r^{11}}{315}+\frac{16 r^{13}}{693}-\frac{128 r^{15}}{6435}+\mathcal {O}(r^{16}). \end{aligned}$$

Then we construct the $14\times 14$ matrix of the variables $r^l$, $l=1,\ldots ,14$ and we obtain that the rank of such matrix is 13. So, we have that 13 are linearly independent. By Proposition 5, there exists a linear combination of these functions with at least 12 zeros. Moreover, the coefficients of these functions are linearly independent of the variables $a_{ij}, b_{ij}, c_{ij}, d_{ij}, e_{ij}, f_{ij}$ for $0\le i+j\le 3$ because the maximum rank of the Jacobian matrix is 13. Thus, there exist $r_k\in (0,1)$ for $k=1,\ldots ,12$ such that $f_1(r_k)=0$ and $f_1'(r_k)\ne 0$.

In summary, by applying the averaging theory of the first order, there exist discontinuous polynomial systems (3) that have at least 12 limit cycles which bifurcate from periodic orbits of the uniform isochronous center of the system (1). $\square$

Remark 1

A particular case of statement (b) of Corollary 1 is when $a_{00}=b_{00}=c_{00}=d_{00}=e_{00}=f_{00}=a_{10}=b_{10}=c_{10}=d_{10}=e_{10}=f_{10}=0$, that is, we consider only quadratic perturbations for the averaging function (11). In this way, we obtained the following relations among the coefficients $\beta _i$ of $f_1(r)$ in (11),

$$\begin{aligned} \beta _2=\beta _4=0,\ \beta _1+\beta _5=0,\ \beta _7+\beta _9=0. \end{aligned}$$

Then the function (11) becomes

$$\begin{aligned} \begin{aligned} f_1(r)=&\frac{1}{8 \pi r}\left( \gamma _1 G_1 +\gamma _2 G_2+ \gamma _3 G_3 +\gamma _4 G_4 +\gamma _5 G_5+\gamma _6 G_6\right) , \end{aligned} \end{aligned}$$

(31)

where $G_1=g_3$, $G_2=g_5$, $G_3=g_7$, $G_4=g_{10}$, $G_5=g_8-g_1$, $G_6=g_6-g_9$ and

$$\begin{aligned} \gamma _1=&\,- 2 a_{02}+2 a_{20}+2 c_{02}-2 c_{20},\\ \gamma _2=&\,2 a_{11}+2 c_{11}-4 e_{11},\\ \gamma _3=&\,2 a_{02}+2 a_{20}-2 c_{02}-2 c_{20},\\ \gamma _4=&\,-2 a_{11}+4 b_{02}-2 c_{11}+4 d_{02}+4 e_{11}-8 f_{02},\\ \gamma _5=&\,2 a_{02}-2 a_{20}+2 b_{11}-2 c_{02}+2 c_{20}-2 d_{11},\\ \gamma _6=&\,2 a_{11}-2 b_{02}+2 b_{20}+2 c_{11}-2 d_{02}+2 d_{20}-4 e_{11}+4 f_{02}-4 f_{20}. \end{aligned}$$

Using the same arguments as in the proof of Theorem 1, the six functions $G_j$ with $j=1,\ldots ,6$ given in (31) form an ECT-system on (0, 1). Thus all the Wronskians $W_j\ne 0$ with $j=1,\ldots ,6$. Then, by Theorem 6 there exists a linear combination of the functions $G_j$ with at most five zeros. Therefore, there exist $r_k \in (0, 1)$ with $i=1,\ldots ,5$ and coefficients $a_ {ij}$, $b_ {ij}$, $c_ {ij}$, $d_ {ij}$, $e_ {ij}$, $f_ {ij}$ in $\mathbb {R}$, $i,j\in \{0,1,2\}$ such that $f_1 (r_k) =0$ and $f'_1(r_k)\ne 0$.

In summary, applying the averaging theory of first order for discontinuous piecewise differential systems with quadratic perturbations, we found that there exist discontinuous piecewise polynomial systems (3) having at most five limit cycles which bifurcate from periodic orbits of the uniform isochronous center of system (1).

On the other hand, for the case of statement (c) of Corollary 1 when we only consider cubic perturbations for the averaging function (16), we have

$$\begin{aligned} A_2=A_4=A_5=A_6=A_8=A_9=A_{11}=A_{12}=A_{15}=0. \end{aligned}$$

Thus, the function (16) is written as

$$\begin{aligned}&f_1(r)=\frac{1}{8 \pi r \sqrt{r^2+1}}\nonumber \\&\quad \left( \tilde{A}_1 G_1+ \tilde{A}_1 G_1+\tilde{A}_3 G_3+\tilde{A}_7 G_7+\tilde{A}_{10} G_{10}+\tilde{A}_{13} G_{13}+\tilde{A}_{14} G_{14}\right) , \end{aligned}$$

(32)

where

$$\begin{aligned}{} & {} G_1=g_0+g_2-g_{23},\ G_2=g_2+g_4,\ G_3=g_{20},\ G_4=g_{24},\\{} & {} G_5=g_{18},\ G_6=g_{17},\ G_7=g_{11}, \end{aligned}$$

and $\tilde{A}_1=A_3$, $\tilde{A}_2=A_2$, $\tilde{A}_3=A_7$, $\tilde{A}_4=A_5$, $\tilde{A}_5=A_{12}$, $\tilde{A}_6=A_{11}$, $\tilde{A}_7=A_{10}$.

So, the seven functions $G_j$, $j=1,\ldots ,7$ given in (15) form an ETC-system on (0, 1). Then, the Wronskians $W_i\ne 0$, $i=1,\ldots ,7$ are nonvanishing in (0, 1). Therefore, by Theorem 1 there exists a linear combination of the functions $G_i$ with at most six zeros. Thus, there exist $r_k$ in (0, 1), $k=1,\ldots ,6$ and coefficients $a_ {ij}$, $b_ {ij}$, $c_ {ij}$, $d_ {ij}$, $e_ {ij}$, $f_ {ij}$ in $\mathbb {R}$, $i,j\in \{0,1,2,3\}$ such that $f_1 (r_k) =0$ and $f'_1(r_k)\ne 0$.

In short, we conclude that there are planar discontinuous cubic polynomial differential systems (3) having at most six limit cycles bifurcating from the periodic orbits of the periodic annulus of the uniform isochronous center (1).

3 Proof of Theorem 2

To prove the following items, we are assuming that the piecewise differential systems (3) for $i=1,2,3$ must be continuous, that is, systems with $i=1$ and $i=2$ must coincide on $\{x=0, y\ge 0\}$, systems with $i=2$ and $i=3$ must coincide on $\{x\le 0, y=0\}$, and systems with $i=1$ and $i=3$ must coincide on $\{ y=0\}$.

Proof of statement (a) of Theorem 2

Imposing the above conditions, we obtain that

$$\begin{aligned} c_{00}=a_{00},\ c_{10}=a_{10},\ d_{00}=b_{00},\ d_{10}=b_{10}. \end{aligned}$$

Then by applying the previous conditions in the averaged function (9) of item (a) of Theorem 1, we get

$$\begin{aligned} f_1(r)=\frac{1}{4} r \left( 2 \left( r^2+1\right) a_{10}+b_{01}+f_{01}\right) . \end{aligned}$$

Clearly, $f_1$ has one solution $r=\dfrac{1}{\sqrt{2}}\sqrt{\dfrac{-(2 a_{10}+b_{01}+f_{01})}{ a_{10}}}$ whenever $(2 a_{10}+b_{01}+f_{01})a_{10}>0$. Thus, there are continuous piecewise polynomial differential systems (3) with at most one limit cycle that can bifurcate the periodic solutions surrounding the uniform isochronous center (1). $\square$

Proof of statement (b) of Theorem 2

Proceeding analogously as in statement (a) for the case $m=2$ in (3), we have the following conditions

$$\begin{aligned} c_{00}=e_{00}=a_{00},\ c_{20}=e_{20}=a_{20},\ d_{00}=f_{00}=b_{00},\ d_{20}=f_{20}=b_{20}, \\ c_{10}=e_{10}=a_{10},\ d_{10}=f_{10}=b_{10},\ c_{01}=a_{01},\ c_{02}=a_{02},\ d_{01}=b_{01},\ d_{02}=b_{02}. \end{aligned}$$

Thus, the averaged function (11) after regrouping terms becomes

$$\begin{aligned} f_1(r)=\frac{1}{4\pi r}\left( \gamma _1 G_1+\gamma _2 G_2+\gamma _3 G_3+\gamma _4 G_4+\gamma _5 G_5\right) , \end{aligned}$$

(33)

where

$$\begin{aligned} G_1=r^2,\ G_2=r^2 (r^2+1),\ G_3=\sinh ^{-1}(r)-r \sqrt{r^2+1}, \\ G_4=(r^2+1)\tan ^{-1}(r)-r,\ G_5=(r^2+1)\left( \sinh ^{-1}(r)-r \sqrt{r^2+1}\right) , \end{aligned}$$

and $\gamma _1=\pi b_{01}+f_{01}$, $\gamma _2=2 \pi a_{10}$, $\gamma _3=2( b_{02}-f_{02})$, $\gamma _4=-(a_{11}+c_{11}-2 e_{11})$, $\gamma _5=b_{11}-d_{11}$.

Then, the set functions $\{G_j\}$, $j=1,\ldots ,5$ form an extended Chebyshev system on (0, 1) because the Wronskians of these functions are

$$\begin{aligned} \begin{aligned} W_1(G_1)&=r^2,\\ W_2(G_1, G_2)&=2r^5,\\ W_3(G_1, G_2, G_3)&=4 r^3 \left( 4 \sinh ^{-1}(r)-\frac{r \left( 5 r^2+4\right) }{\left( r^2+1\right) ^{3/2}}\right) ,\\ W_4(G_1, G_2, G_3, G_4)&=16 r^5 \left( \frac{r \left( 7 r^2+10\right) }{\left( r^2+1\right) ^3}-\frac{2 \left( 2 r^2+5\right) \sinh ^{-1}(r)}{\left( r^2+1\right) ^{5/2}}\right) ,\\ W_5(G_1, G_2, G_3, G_4, G_5)&=\frac{32 r^4 \left( N_1(r) \tan ^{-1}(r)+N_2(r)+N_3(r) \sinh ^{-1}(r)\right) }{\left( r^2+1\right) ^6},\\ \end{aligned} \end{aligned}$$

(34)

where

$$\begin{aligned} N_1(r)=-4(r^2+1) \left( 8 r^4+4 r^2+5\right) ,\ N_2(r)=r(33 r^6+52 r^4+62 r^2+40), \\ N_3(r)=-2 \sqrt{r^2+1} \left( 6 r^6+3 r^4+13 r^2+10\right) . \end{aligned}$$

Observe that $W_1\ne 0$ and $W_2\ne 0$ for all $r\in (0, 1)$. Then using the same idea that in the proof of Theorem 1 for $W_3$, $W_4$ and $W_5$, it is proved that these functions are monotones on (0, 1). Then, by Theorem 6, there exists a linear combination of them with at most four zeros. Therefore, there exist $r_k \in (0, 1)$ with $i=1,\ldots ,4$ and coefficients $a_ {ij}$, $b_ {ij}$, $c_ {ij}$, $d_ {ij}$, $e_ {ij}$, $f_ {ij}$ in $\mathbb {R}$, $i,j\in \{0,1,2\}$ such that $f_1 (r_k) =0$ and $f'_1(r_k)\ne 0$.

Thus, applying the averaged method of first order the function $f_1$ given in (33) have at most four solutions, that is, four limit cycles which bifurcate from periodic orbits of the system (1)

Proof of statement (c) of Theorem 2

Proceeding in an analogous way as previous statements, for the case $m=3$ in (3), we obtain the following conditions

$$\begin{aligned}{} & {} c_{00}= e_{00}=a_{00},\ d_{00}=f_{00}=b_{00},\ c_{01}=a_{01},\ c_{02}=a_{02},\ c_{03}=a_{03}, \\{} & {} c_{10}=e_{10}=a_{10},\ c_{20}=e_{20}=a_{20},\ c_{30}=e_{30}=a_{30},\\{} & {} d_{01}=b_{01},\ d_{02}=b_{02},\ d_{03}=b_{03}, \\{} & {} d_{10}=f_{10}=b_{10}, \ d_{20}=f_{20}=b_{20},\ d_{30}=f_{30}=b_{30}. \end{aligned}$$

Then, the function (16) with the previous conditions and regrouping terms becomes

$$\begin{aligned} f_1(r)=\frac{1}{8 \pi r}\left( \eta _1 G_1+\eta _2 G_2+\eta _3 G_3+\eta _4 G_4+\eta _5 G_5+\eta _6 G_6+\eta _7 G_7\right) , \end{aligned}$$

(35)

where

$$\begin{aligned} \begin{aligned}&G_1=r^2,\quad G_2=r^4,\quad G_3=-r+(1+r^2)\arctan (r),\\&G_4=(1+r^2)\ln (1+r^2),\quad G_5=\left( r^2+1\right) ^{3/2}-1,\quad G_6=\sqrt{r^2+1}-1,\\&G_7=-2\left( r \left( r^2+1\right) ^{3/2}-\left( r^2+1\right) \sinh ^{-1}(r)\right) ,\\&G_8=\left( r^2+2\right) \sinh ^{-1}(r)-r \sqrt{r^2+1} \left( 3 r^2+2\right) , \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} \eta _1=&\,4 \pi a_{10}+3 \pi a_{12}+2 a_{21}-4 \pi a_{30}+2 \pi b_{01}-6 \pi b_{03}-2 b_{12}+\pi b_{21}+3 \pi c_{12}-2 c_{21}\\&+2 d_{12}+\pi d_{21}+6 \pi e_{12}+2 \pi f_{01}-6 \pi f_{03}+2 \pi f_{21},\\ \eta _2=&\,4 \pi a_{10}+\pi a_{12}+2 a_{21}+4 \pi a_{30}+\pi c_{12}-2 c_{21}+2 \pi e_{12},\\ \eta _3=&\,2 \left( b_{11}-d_{11}\right) ,\ \eta _4=-2 \left( a_{21}-b_{12}-c_{21}+d_{12}\right) ,\\ \eta _5=&\,-2 \pi \left( a_{12}-2 b_{03}+c_{12}+2 e_{12}-2 f_{03}\right) ,\ \eta _6=-2 \pi \left( -4 a_{30}+b_{21}+d_{21}+2 f_{21}\right) ,\\ \eta _7=&\,-a_{11}+6 b_{02}-c_{11}+2 e_{11}-6 f_{02},\ \eta _8=-4 \left( b_{02}-f_{02}\right) . \end{aligned} \end{aligned}$$

We have the following Taylor expansions in the variable r around $r=0$ of the functions $G_i$ for $i=1,\ldots ,8$.

$$\begin{aligned} G_1= & {} \,r^2+ \mathcal {O}(r^{10}),\nonumber \\ G_2= & {} \,r^4+ \mathcal {O}(r^{10}),\nonumber \\ G_3= & {} \frac{2 r^3}{3}-\frac{2 r^5}{15}+\frac{2 r^7}{35}-\frac{2 r^9}{63}+ \mathcal {O}(r^{10}),\nonumber \\ G_4= & {} \,r^2+\frac{r^4}{2}-\frac{r^6}{6}+\frac{r^8}{12}+ \mathcal {O}(r^{10}),\nonumber \\ G_5= & {} \frac{3 r^2}{2}+\frac{3 r^4}{8}-\frac{r^6}{16}+\frac{3 r^8}{128}+ \mathcal {O}(r^{10}),\nonumber \\ G_6= & {} \frac{r^2}{2}-\frac{r^4}{8}+\frac{r^6}{16}-\frac{5 r^8}{128}+ \mathcal {O}(r^{10}),\nonumber \\ G_7= & {} -\frac{4 r^3}{3}-\frac{14 r^5}{15}+\frac{13 r^7}{70}-\frac{19 r^9}{252}+ \mathcal {O}(r^{10}),\nonumber \\ G_8= & {} -\frac{10 r^3}{3}-\frac{19 r^5}{15}+\frac{33 r^7}{140}-\frac{47 r^9}{504}+ \mathcal {O}(r^{10}). \end{aligned}$$

(36)

With (36) we construct the $8 \times 8$ matrix whose inputs are the coefficients of the variable $r^l$ for $l=1,\ldots ,8$, and calculating the rank we obtain 7. By Proposition 5 since there are 7 linearly independent functions among the 8 previous functions, then there exists a linear combination of them with at least 6 zeros. In addition, the coefficients of these functions are linearly independent, because their Jacobian matrix in the variables $a_{ij}, b_{ij}, c_{ij}, d_{ij}, e_{ij}, f_{ij}$ for $0\le i+j\le 3$ has maximum rank, which is 6. Thus, there exists $r_k\in (0,1)$ such that $f_1(r_k)=0$ and $f_1'(r_k)\ne 0$ for $k=1,\ldots ,6$.

In summary, applying the averaged method of first order the function $f_1$ given in (35) have at least six solutions. Consequently, there are planar continuous cubic polynomial differential systems (3) having six limit cycles which bifurcate from periodic orbits of the system (1). $\square$

Appendix

In this section, we give some main results of first order averaging theory to study the discontinuous piecewise differential systems development in Llibre, Novaes and Teixeira in [21].

Theorem 3

(The first order averaging theorem for DPDS). Consider the following discontinuous piecewise differential system

$$\begin{aligned} x'(t)=\epsilon F_1(t,x)+\epsilon ^2 R(t,x,\epsilon ), \end{aligned}$$

(37)

with

$$\begin{aligned} \begin{aligned} F_1(t,x)&=\displaystyle \sum _{j=1}^M \chi _{\bar{S}_j}(t,x)F_1^j (t,x),\\ R(t,x,\epsilon )&=\displaystyle \sum _{j=1}^M \chi _{\bar{S}_j}(t,x)R_1^j (t,x),\\ \end{aligned} \end{aligned}$$

(38)

where $F_1^j:\mathbb {S}^1\mapsto D\rightarrow \mathbb {R}^d$, $R^j:\mathbb {S}^1\times D\times (-\epsilon _0, \epsilon _0)\rightarrow \mathbb {R}^d$ for $j=1,\ldots ,M$ are continuous functions, T-periodic in the variable t and D an open subset of $\mathbb {R}^d$.

We define the averaging function $f_1:D\rightarrow \mathbb {R}^d$ as

$$\begin{aligned} f_1(z)=\frac{1}{T}\int _0^T F_1(t,z)dt. \end{aligned}$$

(39)

Moreover, we assume the following hypotheses:

(HC) There exists $C\subset D$ an open bounded subset such that for each $z\in \bar{C}$ the curve $\{ (t,z): t\in \mathbb {S}^1\}$ reaches transversely the set $\Sigma$ and only at generic points of discontinuity.

(Ha1) For $j=1,\ldots ,M$ the continuous functions $F_1^j$ and $R^j$ are T-periodic with respect to variable t and locally Lipschitz with respect to x. In addition the boundaries of $S_j$, for $j=1,\ldots ,M$ are piecewise $\mathcal {C}^k$-embedded hypersurfaces, $k\ge 1$.

(Ha2) For $a^*\in C$ with $f_1(a^*)=0$, there exists a neighborhood $U\subset C$ of $a^*$ such that $f_1(z)\ne 0$ for all $z\in \bar{U}\setminus \{a^*\}$ and the Brouwer degree of $f_1$ at 0 is $d_B(f_1,U,0)\ne 0$.

Then for $|\epsilon |\ne 0$ sufficiently small, there exists a T-periodic solution $x(t,\epsilon )$ of system (37) such that $x(0,\epsilon )\rightarrow a^*$ as $\epsilon \rightarrow 0$.

The next result provides a method to write the system (3) into the normal form (37).

Theorem 4

Consider the unperturbed system

$$\begin{aligned} \dot{x}=P(x,y)+\epsilon p(x,y),\ \dot{y}=Q(x,y)+\epsilon q(x,y) \end{aligned}$$

(40)

with p, q continuous functions, and its first integral $\mathcal {H}$. Assume that for all (x, y) in the period annulus formed by the oval $\{\Gamma _h \}$, we have $xQ(x, y)-yP(x, y)\ne 0$. Moreover, for all $R\in (\sqrt{h_1}, \sqrt{h_2})$ and all $\theta \in [0,2\pi )$, let $\rho :(\sqrt{h_1}, \sqrt{h_2})\times [0,2\pi )\rightarrow [0,\infty )$ be a continuous functions such that

$$\begin{aligned} \mathcal {H}(\rho (R, \theta )\cos \theta , \rho (R, \theta )\sin \theta )=R^2. \end{aligned}$$

Then the differential equation which describes the dependence between the square root of the energy $R=\sqrt{h}$ and the angle $\theta$ for (40) is

$$\begin{aligned} \dfrac{dR}{d\theta }=\epsilon \dfrac{\mu (x^2+y^2)(Qp-Pq)}{2R(Qx-Py)}+\mathcal {O}(\epsilon ^2), \end{aligned}$$

(41)

where $x=\rho (x, y)\cos \theta$, $y=\rho (x, y)\sin \theta$ and $\mu =\mu (x, y)$ is an integrating factor corresponding to the first integral $\mathcal {H}$ of the system (40)$_{\epsilon =0}$.

We also need the next results to determine the number of zeros of the averaging function (39).

Proposition 5

Let I be a interval of $\mathbb {R}$ and let $f_0, f_1,\ldots ,f_n: I\Longrightarrow \mathbb {R}$ by analytic functions linearly independent, that is, if $\sum _{i=0}^n \ \alpha _i f_i(s)=0$ then $\alpha _0=\ldots =\alpha _n=0$. Assume that one of the functions $f_i$ does not change sign in I. Then there exist $s_i,\ldots ,s_n \in I$ and $\lambda _0,\ldots ,\lambda _n \in \mathbb {R}$ such that every $j\in \{1,\ldots ,n\}$ we have $f(s_j)=\sum _{i=0}^n \ \lambda _i f_i(s_j)=0$ and $f'(s_j)\ne 0$.

Let $\{f_0, f_1,\ldots ,f_n \}$ be a set of analytic functions on an open interval $I\subset \mathbb {R}$. These functions are an extended complete Chebyshev system on I, if and only if, for each $k\in \{0,\ldots ,n\}$ and $s\in I$ the Wronskian

$$\begin{aligned} W(f_0,\ldots ,f_k)(s)=\begin{vmatrix} f_0(s)&f_1(s)&\cdots&f_k(s) \\ f'_0(s)&f'_1(s)&\cdots&f'_k(s) \\ \vdots&\vdots&\ddots&\vdots \\ f_0^k(s)&f_1^k(s)&\cdots&f_k^k(s) \\ \end{vmatrix}\ne 0, \end{aligned}$$

Moreover, for an extended complete Chebyshev system in $I\subset \mathbb {R}$ we have the following well-known result. For a proof see for instance [19].

Theorem 6

Assume that the functions $f_0, f_1,\ldots , f_n$ form an extended complete Chebyshev system on I. Then the maximum number of zeros of the function

$$\begin{aligned} a_0 f_0(x) + a_1 f_1(x) +\ldots + a_n f_n(x)=0, \end{aligned}$$

(42)

on $I\subset \mathbb {R}$ is n. Furthermore, if we can choose the coefficients $a_0, a_1,\ldots ,a_n$ arbitrarily, there are functions of the form in (42) having exactly n isolated zeros in I.

In the following lemma, we give some integrals that appear in the averaging function $f_1$ of Theorem 1 and Theorem 2.

Lemma 1

The following integrals hold:

$$\begin{aligned} I_1=&\displaystyle \int \cos \theta \sqrt{a+b \cos 2 \theta }d\theta \\&=\frac{1}{4} \left( 2 \sin \theta \sqrt{a+b \cos 2 \theta }+\frac{\sqrt{2} (a+b)}{\sqrt{b}} \tan ^{-1}\left( \frac{\sqrt{2b} \sin \theta }{\sqrt{a+b \cos 2 \theta }}\right) \right) ,\\ I_2=&\displaystyle \int \sin \theta \sqrt{a+b \cos 2 \theta }d\theta \\&=\frac{1}{4} \left( \frac{\sqrt{2} (b-a)}{\sqrt{b}} \ln \left( \sqrt{a+b \cos 2 \theta }+\sqrt{2b} \cos \theta \right) -2 \cos \theta \sqrt{a+b \cos 2 \theta }\right) ,\\ I_3=&\displaystyle \int \frac{\sin \theta }{\sqrt{a+b \cos 2 \theta }}d\theta =-\frac{\ln \left( \sqrt{a+b \cos 2 \theta }+\sqrt{2b} \cos \theta \right) }{\sqrt{2b}},\\ I_4=&\displaystyle \int \frac{\cos \theta }{\sqrt{a+b \cos 2 \theta }}d\theta =\frac{1}{\sqrt{2b}}\tan ^{-1}\left( \frac{\sqrt{2b} \sin \theta }{\sqrt{a+b \cos 2 \theta }}\right) ,\\ I_5=&\displaystyle \int \frac{\sin 3\theta }{\sqrt{a+b \cos 2 \theta }}d\theta =\frac{a \ln \left( \sqrt{a+b \cos 2 \theta }+\sqrt{2b} \cos \theta \right) }{\sqrt{2} \ b^{3/2}}-\frac{\cos \theta \sqrt{a+b \cos 2 \theta }}{b},\\ I_6=&\displaystyle \int \frac{\cos 3\theta }{\sqrt{a+b \cos 2 \theta }}d\theta =\frac{\sin \theta \sqrt{a+b \cos 2 \theta }}{b}-\frac{a}{\sqrt{2}\ b^{3/2}} \tan ^{-1}\left( \frac{\sqrt{2b} \sin \theta }{\sqrt{a+b \cos 2 \theta }}\right) . \end{aligned}$$

We show the functions $F_1^j$ for $j=1,2,3$ of (13) in the proof of statement (c) of Theorem 1.

$$\begin{aligned} F_1^1(\theta , r)= & {} \frac{1}{4 \left( \cos (2 \theta ) r^2+r^2+2\right) }\left( 2 \sqrt{2} \left( r^2+1\right) \cos (\theta ) a_{00} \left( \cos (2 \theta ) r^2+r^2+2\right) ^{3/2}\right. \\{} & {} \quad +2 r \left( r^2+1\right) \sin (2 \theta ) a_{01} \left( \cos (2 \theta ) r^2+r^2+2\right) \\{} & {} +\sqrt{2} r^4 \cos (\theta ) a_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (\theta ) a_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^4 \cos (3 \theta ) a_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad -\sqrt{2} r^2 \cos (3 \theta ) a_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \sin (\theta ) a_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (\theta ) a_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \sin (3 \theta ) a_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (3 \theta ) a_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +3 \sqrt{2} r^4 \cos (\theta ) a_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +3 \sqrt{2} r^2 \cos (\theta ) a_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \cos (3 \theta ) a_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (3 \theta ) a_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (\theta ) b_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +4 \sqrt{2} \sin (\theta ) b_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (3 \theta ) b_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +3 \sqrt{2} r^2 \sin (\theta ) b_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^2 \sin (3 \theta ) b_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (\theta ) b_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^2 \cos (3 \theta ) b_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (\theta ) b_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (3 \theta ) b_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +2 r^5 \sin (2 \theta ) a_{03}+2 r^3 \sin (2 \theta ) a_{03}-r^5\\{} & {} \sin (4 \theta ) a_{03}-r^3 \sin (4 \theta ) a_{03}+3 r^5 a_{10}\\{} & {} \quad +7 r^3 a_{10}+4 r a_{10}+4 r^5 \cos (2 \theta ) a_{10}+8 r^3\\{} & {} \cos (2 \theta ) a_{10}+4 r \cos (2 \theta ) a_{10}+r^3 b_{01}\\{} & {} \quad +r^5 \cos (4 \theta ) a_{10}+r^3 \cos (4 \theta ) a_{10}+r^5 a_{12}\\{} & {} +r^3 a_{12}-r^5 \cos (4 \theta ) a_{12}-r^3 \cos (4 \theta ) a_{12}\\{} & {} \quad +2 r^5 \sin (2 \theta ) a_{21}+2 r^3 \sin (2 \theta ) a_{21}+r^5\\{} & {} \sin (4 \theta ) a_{21}+r^3 \sin (4 \theta ) a_{21}+3 r^5 a_{30}\\{} & {} \quad +3 r^3 a_{30}+4 r^5 \cos (2 \theta ) a_{30}+4 r^3 \cos (2 \theta ) a_{30}\\{} & {} +r^5 \cos (4 \theta ) a_{30}+r^3 \cos (4 \theta ) a_{30}\\{} & {} \quad +4 r b_{01}-4 r \cos (2 \theta ) b_{01}-r^3 \cos (4 \theta ) b_{01}\\{} & {} +3 r^3 b_{03}-4 r^3 \cos (2 \theta ) b_{\{0,3\}}\\{} & {} \quad +r^3 \cos (4 \theta ) b_{03}+2 r^3 \sin (2 \theta ) b_{10}\\{} & {} +4 r \sin (2 \theta ) b_{10}+r^3 \sin (4 \theta ) b_{10}+2 r^3 \sin (2 \theta ) b_{12}\\{} & {} \quad -r^3 \sin (4 \theta ) b_{12}+r^3 b_{21}-r^3 \cos (4 \theta ) b_{21}\\{} & {} \left. +2 r^3 \sin (2 \theta ) b_{30}+r^3 \sin (4 \theta ) b_{30}\right) ,\\ F_1^2(\theta , r)= & {} \frac{1}{4 \left( \cos (2 \theta ) r^2+r^2+2\right) }\left( 2 \sqrt{2} \left( r^2+1\right) \right. \\{} & {} \cos (\theta ) c_{00} \left( \cos (2 \theta ) r^2+r^2+2\right) ^{3/2}\\{} & {} \quad +2 r \left( r^2+1\right) \sin (2 \theta ) c_{01} \left( \cos (2 \theta ) r^2+r^2+2\right) \\{} & {} +\sqrt{2} r^4 \cos (\theta ) c_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (\theta ) c_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^4 \cos (3 \theta ) c_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad -\sqrt{2} r^2 \cos (3 \theta ) c_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \sin (\theta ) c_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (\theta ) c_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \sin (3 \theta ) c_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (3 \theta ) c_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +3 \sqrt{2} r^4 \cos (\theta ) c_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +3 \sqrt{2} r^2 \cos (\theta ) c_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \cos (3 \theta ) c_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (3 \theta ) c_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (\theta ) d_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +4 \sqrt{2} \sin (\theta ) d_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (3 \theta ) d_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +3 \sqrt{2} r^2 \sin (\theta ) d_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^2 \sin (3 \theta ) d_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (\theta ) d_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^2 \cos (3 \theta ) d_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (\theta ) d_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (3 \theta ) d_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +2 r^5 \sin (2 \theta ) c_{03}+2 r^3 \sin (2 \theta ) c_{03}-r^5\\{} & {} \sin (4 \theta ) c_{03}-r^3 \sin (4 \theta ) c_{03}+3 r^5 c_{10}\\{} & {} \quad +7 r^3 c_{10}+4 r c_{10}+4 r^5 \cos (2 \theta ) c_{10}+8 r^3\\{} & {} \cos (2 \theta ) c_{10}+4 r \cos (2 \theta ) c_{10}\\{} & {} \quad +r^5 \cos (4 \theta ) c_{10}+r^3 \cos (4 \theta ) c_{10}+r^5 c_{12}\\{} & {} +r^3 c_{12}-r^5 \cos (4 \theta ) c_{12}-r^3 \cos (4 \theta ) c_{12}\\{} & {} \quad +2 r^5 \sin (2 \theta ) c_{21}+2 r^3 \sin (2 \theta ) c_{21}+r^5\\{} & {} \sin (4 \theta ) c_{21}+r^3 \sin (4 \theta ) c_{21}+3 r^5 c_{30}+3 r^3 c_{30}\\{} & {} \quad +4 r^5 \cos (2 \theta ) c_{30}+4 r^3 \cos (2 \theta ) c_{30}+r^5\\{} & {} \cos (4 \theta ) c_{30}+r^3 \cos (4 \theta ) c_{30}+r^3 d_{01}+4 r d_{01}\\{} & {} \quad -4 r \cos (2 \theta ) d_{01}-r^3 \cos (4 \theta ) d_{01}+3 r^3 d_{03}\\{} & {} -4 r^3 \cos (2 \theta ) d_{03}+r^3 \cos (4 \theta ) d_{03}\\{} & {} \quad +2 r^3 \sin (2 \theta ) d_{10}+4 r \sin (2 \theta ) d_{10}+r^3\\{} & {} \sin (4 \theta ) d_{10}+2 r^3 \sin (2 \theta ) d_{12}-r^3 \sin (4 \theta ) d_{12}\\{} & {} \quad \left. +r^3 d_{21}-r^3 \cos (4 \theta ) d_{21}+2 r^3 \sin (2 \theta ) d_{30}+r^3 \sin (4 \theta ) d_{30}\right) ,\\ F_1^3(\theta , r)= & {} \frac{1}{4 \left( \cos (2 \theta ) r^2+r^2+2\right) }\left( 2 \sqrt{2} \left( r^2+1\right) \right. \\{} & {} \cos (\theta ) e_{00} \left( \cos (2 \theta ) r^2+r^2+2\right) ^{3/2}\\{} & {} \quad +2 r \left( r^2+1\right) \sin (2 \theta ) e_{01} \left( \cos (2 \theta ) r^2+r^2+2\right) \\{} & {} +\sqrt{2} r^4 \cos (\theta ) e_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (\theta ) e_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^4 \cos (3 \theta ) e_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad -\sqrt{2} r^2 \cos (3 \theta ) e_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \sin (\theta ) e_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (\theta ) e_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \sin (3 \theta ) e_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (3 \theta ) e_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +3 \sqrt{2} r^4 \cos (\theta ) e_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +3 \sqrt{2} r^2 \cos (\theta ) e_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^4 \cos (3 \theta ) e_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (3 \theta ) e_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (\theta ) f_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +4 \sqrt{2} \sin (\theta ) f_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (3 \theta ) f_{00} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +3 \sqrt{2} r^2 \sin (\theta ) f_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^2 \sin (3 \theta ) f_{02} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \cos (\theta ) f_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} -\sqrt{2} r^2 \cos (3 \theta ) f_{11} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +\sqrt{2} r^2 \sin (\theta ) f_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} +\sqrt{2} r^2 \sin (3 \theta ) f_{20} \sqrt{\cos (2 \theta ) r^2+r^2+2}\\{} & {} \quad +2 r^5 \sin (2 \theta ) e_{03}+2 r^3 \sin (2 \theta ) e_{03}-r^5\\{} & {} \sin (4 \theta ) e_{03}-r^3 \sin (4 \theta ) e_{03}+3 r^5 e_{10}\\{} & {} \quad +7 r^3 e_{10}+4 r e_{10}+4 r^5 \cos (2 \theta ) e_{10}+8 r^3\\{} & {} \cos (2 \theta ) e_{10}+4 r \cos (2 \theta ) e_{10}\\{} & {} \quad +r^5 \cos (4 \theta ) e_{10}+r^3 \cos (4 \theta ) e_{10}+r^5 e_{12}\\{} & {} +r^3 e_{12}-r^5 \cos (4 \theta ) e_{12}\\{} & {} \quad -r^3 \cos (4 \theta ) e_{12}+2 r^5 \sin (2 \theta ) e_{21}+2 r^3\\{} & {} \sin (2 \theta ) e_{21}+r^5 \sin (4 \theta ) e_{21}\\{} & {} \quad +r^3 \sin (4 \theta ) e_{21}+3 r^5 e_{30}+3 r^3 e_{30}+4 r^5\\{} & {} \cos (2 \theta ) e_{30}+4 r^3 \cos (2 \theta ) e_{30}\\{} & {} \quad +r^5 \cos (4 \theta ) e_{30}+r^3 \cos (4 \theta ) e_{30}+r^3 f_{01}+4 r f_{01}-4 r \cos (2 \theta ) f_{01}\\{} & {} \quad -r^3 \cos (4 \theta ) f_{01}+3 r^3 f_{03}-4 r^3 \cos (2 \theta ) f_{03}\\{} & {} +r^3 \cos (4 \theta ) f_{03}+2 r^3 \sin (2 \theta ) f_{10}\\{} & {} \quad +4 r \sin (2 \theta ) f_{10}+r^3 \sin (4 \theta ) f_{10}+2 r^3\\{} & {} \sin (2 \theta ) f_{12}-r^3 \sin (4 \theta ) f_{12}+r^3 f_{21}\\{} & {} \left. -r^3 \cos (4 \theta ) f_{21}+2 r^3 \sin (2 \theta ) f_{30}+r^3 \sin (4 \theta ) f_{30}\right) . \end{aligned}$$

Expressions of the Wronskians in the proof of statement (c) of Theorem 1.

$$\begin{aligned} W_{51}= & {} \,8 r^4+4 \left( r^2+1\right) ^{3/2}-15 \sqrt{r^2+1}+4 r^2-9,\\ W_{61}= & {} \,16 \left( \sqrt{r^2+1}-3\right) r^8+8 \left( 11 \sqrt{r^2+1}-17\right) r^6+15 \left( 7 \sqrt{r^2+1}-8\right) r^4\\{} & {} +4 \left( 7 \sqrt{r^2+1}-6\right) r^2-8 \sqrt{r^2+1}+8,\\ W_{71}= & {} \left( 52 \sqrt{r^2+1}-77\right) r^4+4 \left( 2 \sqrt{r^2+1}-7\right) r^6+\left( 52 \sqrt{r^2+1}-56\right) r^2\\{} & {} +8 \left( \sqrt{r^2+1}-1\right) ,\\ W_{81}= & {} \,r^4-4 \left( \sqrt{r^2+1}-2\right) r^2-8 \sqrt{r^2+1}+8,\\ W_{91}= & {} \,280 r^{14}-40 \left( 32 \sqrt{r^2+1}-107\right) r^{12}+\left( 20883-9792 \sqrt{r^2+1}\right) r^{10}\\{} & {} +\left( 50532-30288 \sqrt{r^2+1}\right) r^8-63 \left( 784 \sqrt{r^2+1}-1105\right) r^6\\{} & {} +\left( 57246-45696 \sqrt{r^2+1}\right) r^4-4200 \left( 6 \sqrt{r^2+1}-7\right) r^2\\{} & {} -8400 \left( \sqrt{r^2+1}-1\right) ,\\ W_{10}^{(1)}= & {} \,2240 r^{24}-235200 \left( 11 \sqrt{r^2+1}-12\right) r^2-470400 \left( \sqrt{r^2+1}-1\right) \\{} & {} -192 \left( 64 \sqrt{r^2+1}-273\right) r^{22}-256 \left( 608 \sqrt{r^2+1}-1647\right) r^{20}\\{} & {} +\left( 1825168-853760 \sqrt{r^2+1}\right) r^{18}+\left( 4514637-2701056 \sqrt{r^2+1}\right) r^{16}\\{} & {} -308 \left( 13208 \sqrt{r^2+1}-14361\right) r^{14}+322 \left( 2432 \sqrt{r^2+1}-15593\right) r^{12}\\{} & {} +252 \left( 44576 \sqrt{r^2+1}-69109\right) r^{10}+63 \left( 215808 \sqrt{r^2+1}-251389\right) r^8\\{} & {} +2352 \left( 1662 \sqrt{r^2+1}-1055\right) r^6-7056 \left( 488 \sqrt{r^2+1}-663\right) r^4,\\ W_{11}^{(1)}= & {} \,r^2 \left( -8960 r^{26}-2207205 \left( 384 \sqrt{r^2+1}-341\right) r^2-339570 \left( 416 \sqrt{r^2+1}\right. \right. \\{} & {} \left. -341\right) +256 \left( 256 \sqrt{r^2+1}-1435\right) r^{24}+896 \left( 1792 \sqrt{r^2+1}-4015\right) r^{22}\\{} & {} +8960 \left( 1440 \sqrt{r^2+1}-2593\right) r^{20}+476 \left( 160768 \sqrt{r^2+1}-300665\right) r^{18}\\{} & {} +28 \left( 12969088 \sqrt{r^2+1}-23627335\right) r^{16}+21 \left( 57997056 \sqrt{r^2+1}\right. \\{} & {} \left. -91589155\right) r^{14}+168 \left( 15076184 \sqrt{r^2+1}-19804525\right) r^{12}\\{} & {} +2205 \left( 1302656 \sqrt{r^2+1}-1385513\right) r^{10}+1470 \left( 701280 \sqrt{r^2+1}-391819\right) r^8\\{} & {} \left. -8085 \left( 166400 \sqrt{r^2+1}-212443\right) r^6-32340 \left( 56368 \sqrt{r^2+1}-56203\right) r^4\right) ,\\ W_{11}^{(2)}= & {} \,-105 \left( r^2+1\right) ^2 \left( 4480 r^{20}+43008 r^{18}+186264 r^{16}+480216 r^{14}+1131159 r^{12}\right. \\{} & {} +1965726 r^{10}+1421700 r^8-798336 r^6-2018709 r^4-1212750 r^2\\{} & {} \left. -242550\right) \ln \left( r^2+1\right) ,\\ W_{12}^{(1)}= & {} \,r^3 \left( -65536 r^{28}+72765 \left( 7159 \sqrt{r^2+1}-8320\right) r^2+145530 \left( 371 \sqrt{r^2+1}-416\right) \right. \\{} & {} +1792 \left( 315 \sqrt{r^2+1}-1664\right) r^{26}+256 \left( 45465 \sqrt{r^2+1}-157792\right) r^{24}\\{} & {} +640 \left( 126819 \sqrt{r^2+1}-329248\right) r^{22}+2560 \left( 87129 \sqrt{r^2+1}-179387\right) r^{20}\\{} & {} +4 \left( 46439365 \sqrt{r^2+1}-125965888\right) r^{18}+84 \left( 5244915 \sqrt{r^2+1}\right. \\{} & {} \left. -21612784\right) r^{16}+105 \left( 40416639 \sqrt{r^2+1}-87793792\right) r^{14}\\{} & {} +84 \left( 161583515 \sqrt{r^2+1}-269437024\right) r^{12}+105 \left( 201928737 \sqrt{r^2+1}\right. \\{} & {} \left. -285638272\right) r^{10}+210 \left( 88325949 \sqrt{r^2+1}-110700928\right) r^8\\{} & {} \left. +1155 \left( 8217371 \sqrt{r^2+1}-9486464\right) r^6+166320 \left( 17285 \sqrt{r^2+1}-19448\right) r^4\right) ,\\ W_{12}^{(2)}= & {} \,315 r \left( r^2+1\right) ^{7/2} \left( 18816 r^{18}+89472 r^{16}+151480 r^{14}+68160 r^{12}-304425 r^{10}\right. \\{} & {} \left. +536217 r^8+167409 r^6-85239 r^4+120120 r^2+20790\right) \ln \left( r^2+1\right) ,\\ W_{13}^{(1)}= & {} \,r^2 \left( -1155 \left( 3328 \sqrt{r^2+1}-30197\right) r^2-4620 \left( 416 \sqrt{r^2+1}-401\right) \right. \\{} & {} -3072 \left( 384 \sqrt{r^2+1}-245\right) r^{24}-20480 \left( 400 \sqrt{r^2+1}-287\right) r^{22}\\{} & {} -128 \left( 173952 \sqrt{r^2+1}-170065\right) r^{20}-448 \left( 240768 \sqrt{r^2+1}-224365\right) r^{18}\\{} & {} +40 \left( 5114496 \sqrt{r^2+1}-1538075\right) r^{16}+4 \left( 325557376 \sqrt{r^2+1}-218802395\right) r^{14}\\{} & {} +\left( 1817405568 \sqrt{r^2+1}-1538051795\right) r^{12}+7 \left( 161977088 \sqrt{r^2+1}\right. \\{} & {} \left. -160013995\right) r^{10}+154 \left( 3080896 \sqrt{r^2+1}-988165\right) r^8+462 \left( 372736 \sqrt{r^2+1}\right. \\{} & {} \left. \left. +606835\right) r^6+1155 \left( 14976 \sqrt{r^2+1}+146843\right) r^4\right) ,\\ W_{13}^{(2)}= & {} \,105 r^3 \left( 7168 r^{24}+52736 r^{22}+143488 r^{20}+573760 r^{18}-1485880 r^{16}-9541356 r^{14}\right. \\{} & {} -14541087 r^{12}-8656956 r^{10}-3129555 r^8-5304156 r^6-5337189 r^4\\{} & {} \left. -1981980 r^2-297297\right) \tan ^{-1}(r),\\ W_{13}^{(2)}= & {} \,210 \left( 12672 r^{20}+78848 r^{18}+209560 r^{16}+308600 r^{14}+258215 r^{12}-17666 r^{10}\right. \\{} & {} \left. +86196 r^8+199320 r^6+62139 r^4+5610 r^2+330\right) \ln \left( r^2+1\right) ,\\ W_{14}^{(1)}= & {} \,39997440 r^{24}-204144640 r^{22}-1053102400 r^{20}-1630692000 r^{18}-505588440 r^{16}\\{} & {} +783218660 r^{14}+422333835 r^{12}-278168275 r^{10}-287355530 r^8-68521530 r^6\\{} & {} +2134055 r^4+640640 \sqrt{r^2+1} r^2+822745 r^2-58982400 \sqrt{r^2+1} r^{24}\\{} & {} +335872000 \sqrt{r^2+1} r^{22}+1359298560 \sqrt{r^2+1} r^{20}+1317666816 \sqrt{r^2+1} r^{18}\\{} & {} +1078034432 \sqrt{r^2+1} r^{16}+822115840 \sqrt{r^2+1} r^{14}+95455360 \sqrt{r^2+1} r^{12}\\{} & {} +15649920 \sqrt{r^2+1} r^{10}+44918016 \sqrt{r^2+1} r^8+32544512 \sqrt{r^2+1} r^6\\{} & {} +5765760 \sqrt{r^2+1} r^4,\\ W_{14}^{(2)}= & {} \,-4620 r \sqrt{1 + r^2}\big (-429 - 3861 r^2 - 6006 r^4 - 79794 r^6 - 298441 r^8 \\{} & {} -348673 r^{10} + 16988 r^{12} + 168824 r^{14} + 37056 r^{16} + 18304 r^{18 }+ 6656 r^{20} \\{} & {} +1024 r^{22} \big ) \sinh ^{-1}(r),\\ W_{14}^{(3)}= & {} \,105 r \left( 358400 r^{24}-1830400 r^{22}-8792960 r^{20}-7905920 r^{18}+2230272 r^{16}\right. \\{} & {} -6422724 r^{14}-25643475 r^{12}-25780040 r^{10}-11223355 r^8-1151436 r^6\\{} & {} \left. -33033 r^4-264264 r^2-33033\right) \tan ^{-1}(r),\\ W_{14}^{(4)}= & {} \,210 \left( 11264 r^{24}+78848 r^{22}+236544 r^{20}+394240 r^{18}+431640 r^{16}+790168 r^{14}\right. \\{} & {} -193699 r^{12}-1248522 r^{10}-627396 r^8-127072 r^6-25751 r^4\\{} & {} \left. -550 r^2+110\right) \ln \left( r^2+1\right) . \end{aligned}$$