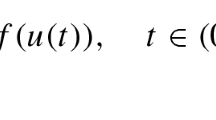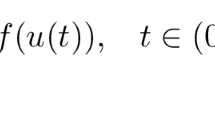Existence of positive solutions for one dimensional Minkowski curvature problem with singularity

Abstract

In this paper, we consider the existence of positive solutions for one dimensional Minkowski curvature problem with either singular weight function or singular nonlinear term. By virtue of fixed point arguments and perturbation technique, we establish the new existence results of positive solutions under different assumptions on the nonlinear term. Moreover, some examples are also given as applications.

1 Introduction

This paper mainly deals with the existence of solutions for one dimensional Minkowski curvature problem of the form

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= \lambda h(t)f(t,u(t)),\quad t\in (0,1),\\ u(0)=u(1)=0, \end{array}\right. } \end{aligned}$$

(1.1)

where $\phi (s)=\frac{s}{\sqrt{1-s^{2}}}$, $s\in (-1,1)$, $\lambda >0$ is a parameter, $h \not \equiv 0$ on any subinterval in (0, 1), and $h\in {\mathcal {H}}=\left\{ h\in C((0,1),[0,\infty )) \ | \ \int _{0}^{1}t(1-t)h(t)\textrm{d}t<\infty \right\}$. It is worth noting that h may be singular at $t=0$ or $t=1$.

Minkowski curvature problem like (1.1) usually plays an important part in differential geometry and physics. For example, it is closely related to the theory of classic relativity (see [5, 12, 26] and the references therein). In the past decades, lots of researchers have devoted to the study of existence and multiplicity of solutions for various nonlinear Minkowski curvature problems and obtained fruitful results (see [6, 10, 17, 28, 29] for one-dimensional case and [7, 8, 11, 19, 20, 22] for higher-dimensional case).

For instance, when $h(t)\equiv 1$ and $f:[0,1]\times {\mathbb {R}}\rightarrow {\mathbb {R}}$ satisfies the $L^1$-Carathéodory conditions, Coelho et al. [10] applied variational and topological methods to prove the existence and multiplicity of positive solutions for (1.1). When $h\in {\mathcal {H}}$, f(t, u) is only dependent on u, $f:[0,\alpha )\rightarrow [0,\infty )$ is continuous with $\alpha >\frac{1}{2}$, and $f_0=\lim _{u\rightarrow 0^{+}}\frac{f(u)}{u}=0$, Yang et al. [29] used Krasnoselskii’s fixed point theorem to show the existence of at least one positive solution for (1.1). Recently, by imposing other assumptions on f, Lee et al. [17] continued to derive the existence of at least two positive solutions for (1.1). While, Lee et al. [17] also discussed the existence of at least two nodal solutions for the case $0<f_0<\infty$. Their proofs rely on bifurcation theories. In a word, the conclusions in [17, 29] are related to existence of one or two solutions for (1.1). However, as far as we know, the study about the existence of three solutions for (1.1) has not been announced yet. In recent years, the topic on existence of three solutions for differential equations has also become one of the most interesting topics (see [4, 19, 22, 30] and the references therein). Inspired by the above observations, our first interest of this paper is to establish the existence of at least three solutions for (1.1). This is the first paper applying the fixed point index arguments to study the existence of three solutions for (1.1). Specially, the exact existence intervals of solutions are also derived (see Theorems 1.1 and 1.3 for details).

Besides weight function h may possess singularity at $t=0$ or $t=1$, our second interest of this paper is to discuss the case that the nonlinear term f(t, u) also has strongly singularity at $u=0$. One example can be given as

$$\begin{aligned} f(t,u)=t^{\alpha }\left( \frac{1}{u^{\beta }}+u^{\gamma }\right) , \end{aligned}$$

where $\alpha ,\beta ,\gamma$ are positive constants. In 1979, Taliaferro [25] studied the existence of solution for a singular boundary value problem of the form

$$\begin{aligned}&{\left\{ \begin{array}{ll} -u''(t)= h(t)\frac{1}{u^{\alpha }(t)},\quad t\in (0,1),\\ u(0)=u(1)=0, \end{array}\right. } \end{aligned}$$

(1.2)

where $\alpha >0$ and $h\in {\mathcal {H}}$. Since then, many researchers have been interested in the singular boundary value problems of various differential equations (see [1,2,3, 15, 23] and the references therein). However, to the best of our knowledge, there have been fewer work about the existence of solutions for the one-dimensional Minkowski curvature problem like (1.1) with singularity which may appear in both the weight function and the nonlinear term. Different from the continuous condition imposed on f(u) at $u=0$ in [17, 29], we aim to study the existence of positive solutions for (1.1) with singular nonlinear term. Due to the appearance of strong singularity of f(t, u) at $u=0$, the results in [17, 29] are not suitable for (1.1) any longer. To overcome the difficulty caused by the strong singularity, we combine perturbation technique with fixed point arguments to establish a new existence result of positive solution for (1.1) (see Theorem 1.4 for details).

More precisely, our main results can be presented as follows. For the sake of narrative convenience, we give the following notations.

$$\begin{aligned} F_0= \limsup _{u\rightarrow 0^{+}}\max _{t\in [0,1]}\frac{f(t,u)}{u},\ \ \ \ \ \ F_{\frac{1}{2}}=\limsup _{u\rightarrow \frac{1}{2}^{-}}\max _{t\in [0,1]}\frac{f(t,u)}{u}. \end{aligned}$$

As $h\in {\mathcal {H}}$, we are not sure whether any solution of (1.1) is of $C^1[0,1]$ or not. Note that $0\le F_0<\infty$ can ensure any solution u of (1.1) is of $C^1[0,1]$ and $\max _{t\in [0,1]}|u(t)|<\frac{1}{2}$. The proof can be easily done by making minor modification of Theorem 2.1 in [28]. Moreover, by Fubini’s theorem, we see that if $h\in {\mathcal {H}}$, then $\int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s+\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s<\infty$.

Theorem 1.1

Assume that $f\in C([0,1]\times [0,\frac{1}{2}), [0,\infty ))$, $0\le F_0<\infty$, $0\le F_{\frac{1}{2}}<1$ and there exist two constants $0<d<a<\frac{1}{32}$ satisfying

$(C_1)$ $f(t,u)\le d$, for all $(t,u)\in [0,1]\times [0,d]$;

$(C_2)$ $f(t,u)\ge \beta \phi (32a)$ for all $(t,u)\in [\frac{1}{4},\frac{3}{4}]\times [a,4a]$, where $\beta$ is a positive constant such that $\lambda _{*}<\lambda ^{*}$,

$$\begin{aligned} \lambda _{*}= & {} \frac{1}{\beta \min \left\{ \int _{\frac{1}{4}}^{\frac{1}{2}}h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^{\frac{3}{4}}h(\tau )\textrm{d}\tau \right\} },\\ \lambda ^{*}= & {} \frac{1}{\max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} }. \end{aligned}$$

Then, for any $\lambda \in (\lambda _{*},\lambda ^{*})$, problem (1.1) must have at least one nonnegative solution $u_1$ and two positive solutions $u_2,u_3$ satisfying $\Vert u_1\Vert <d$, $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_2(t)>a$, $\Vert u_3\Vert >d$ and $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_3(t)<a$.

Remark 1.2

Since h may be singular at $t=0$ and/or $t=1$, we understand u as a solution of (1.1) if $u\in C[0,1]\cap C^1(0,1)$, $|u'(t)|<1$ for $t\in (0,1)$ with $\phi (u')$ absolutely continuous which satisfies (1.1). Particularly, if $u(t)\ge 0$ for all $t\in [0,1]$, then u is called a nonnegative solution. While, if $u(t)> 0$ for all $t\in (0,1)$, then u is called a positive solution of (1.1).

Moreover, condition $0\le F_0<\infty$ implies that $u_1$ may be a trivial solution in Theorem 1.1. Thus, we continue to establish Theorem 1.3 that can guarantee the existence of three positive solutions for (1.1).

Theorem 1.3

Assume that $f\in C([0,1]\times [0,\frac{1}{2}), [0,\infty ))$, $0\le F_0<\infty$, $0\le F_{\frac{1}{2}}<1$ and there exist three constants $0<e<d<a<\frac{1}{32}$ satisfying

$(C_1)$ $f(t,u)\le d$, for all $(t,u)\in [0,1]\times [0,d]$;

$(C_3)$ $f(t,u)\ge \beta _1\phi (8e)$ for all $(t,u)\in [\frac{1}{4},\frac{3}{4}]\times [\frac{e}{4},e]$, $f(t,u)\ge \beta _2\phi (32a)$ for all $(t,u)\in [\frac{1}{4},\frac{3}{4}]\times [a,4a]$, where $\beta _1,\beta _2$ are two positive constants such that $\lambda _{*}<\lambda ^{*}$,

$$\begin{aligned} \lambda _{*}= & {} \frac{1}{\min \{\beta _1,\beta _2\} \min \left\{ \int _{\frac{1}{4}}^{\frac{1}{2}}h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^{\frac{3}{4}}h(\tau )\textrm{d}\tau \right\} }, \\ \lambda ^{*}= & {} \frac{1}{\max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} }. \end{aligned}$$

Then, for any $\lambda \in (\lambda _{*},\lambda ^{*})$, problem (1.1) must have at least three positive solutions $u_1,u_2, u_3$ satisfying $e<\Vert u_1\Vert <d$, $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_2(t)>a$, $\Vert u_3\Vert >d$ and $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_3(t)<a$.

Theorem 1.4

Assume that $f\in C([0,1]\times (0,\infty ), (0,\infty ))$ and satisfies

$(C_4)$ $f(t,u)\le f_1(u)+f_2(u)$ for all $(t,u)\in [0,1]\times (0,\infty )$, where $f_1:(0,\infty )\rightarrow (0,\infty )$ is continuous and nonincreasing, $f_2:[0,\infty )\rightarrow [0,\infty )$ is continuous, and $\frac{f_2}{f_1}$ is nondecreasing on $(0,\infty )$;

$(C_5)$ for each constant $\iota >0$, there exists a function $\psi _\iota \in C([0,1],[0,\infty ))$ satisfying $\psi _\iota (t)>0$ for $t\in (0,1)$ and $f(t,u)\ge \psi _\iota (t)$ for $(t,u)\in [0,1]\times (0,\iota ]$;

$(C_6)$ there exists a constant $r>0$ such that ${\bar{\lambda }}\in (0,\infty )$,

$$\begin{aligned} {\bar{\lambda }}= \frac{\int _0^{r}\frac{\textrm{d}y}{f_1(y)}}{\left[ 1+\frac{f_2(r)}{f_1(r)}\right] \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} }. \end{aligned}$$

Then, for any $\lambda \in (0,{\bar{\lambda }})$, problem (1.1) must have at least one positive solution u satisfying $0<\Vert u\Vert <r$.

The rest of this paper is organized as follows. In Sect. 2, we introduce some necessary preliminaries. In Sect. 3, we present the detailed proofs of Theorems 1.1 and 1.3, and give one corresponding example. Finally, the proof of Theorem 1.4 and corresponding example are given in Sect. 4.

2 Preliminaries

Before proving our main results, let us first present necessary preliminaries. Let K be a cone of the Banach space $(E,\Vert \cdot \Vert )$, $\alpha$ be a continuous functional. Then, for positive constants r, b, d, we denote

$$\begin{aligned} K_r= & {} \{u\in K \ |\ \Vert u\Vert<r\},\\ \partial K_r= & {} \{u\in K \ |\ \Vert u\Vert =r\},\\ K(\alpha ,b,d)= & {} \{u\in K \ | \ b\le \alpha (u),\ \Vert u\Vert \le d\}, \\ \mathring{K}(\alpha ,b,d)= & {} \{u\in K \ |\ b< \alpha (u),\ \Vert u\Vert \le d\}. \end{aligned}$$

Lemma 2.1

(Guo–Krasnoselskii [13, 16]) Let E be a Banach space and let K be a cone in E. Assume that $T:\overline{K_r} \rightarrow K$ is completely continuous such that $Tu\ne u$ for $u\in \partial K_r$.

• (i) If $\Vert Tu\Vert \ge \Vert u\Vert$ for $u\in \partial K_r$, then $i(T, K_r,K)=0.$

• (ii) If $\Vert Tu\Vert \le \Vert u\Vert$ for $u\in \partial K_r$, then $i(T, K_r,K)=1.$

Definition 2.2

([18]) A continuous functional $\alpha :K \rightarrow [0,+\infty )$ is called a concave positive functional on a cone K if $\alpha$ satisfies

$$\begin{aligned} \alpha (\kappa x+(1-\kappa )y)\ge \kappa \alpha (x)+(1-\kappa )\alpha (y),\ \mathrm{for \ all} \ x,y\in K, 0\le \kappa \le 1. \end{aligned}$$

Lemma 2.3

(Leggett–Williams [18]) Let K be a cone in a real Banach space E and $\alpha$ be a concave positive functional on K such that $\alpha (u)\le \Vert u\Vert$ for all $u\in \overline{K_c}$. Suppose $T: \overline{K_c} \rightarrow \overline{K_c}$ is completely continuous and there exist numbers a, b and d, with $0<d<a<b\le c$, satisfying the following conditions:

• (i) $\{u\in K(\alpha ,a,b):\alpha (u)>a\}\ne \emptyset$ and $\alpha (Tu)>a$ if $u\in K(\alpha ,a,b)$;

• (ii) $\Vert Tu\Vert <d$ if $u\in \overline{K_d}$;

• (iii) $\alpha (Tu)>a$ for all $u\in K(\alpha ,a,c)$ with $\Vert Tu\Vert >b$.

Then

$$\begin{aligned}{} & {} i(T,K_d,\overline{K_c})=1,\\{} & {} i(T,\mathring{K}(\alpha ,a,c),\overline{K_c})=1,\\{} & {} i(T,\overline{K_c}\backslash (\overline{K_d}\cup K(\alpha ,a,c)), \overline{K_c})=-1. \end{aligned}$$

Furthermore, T has at least three fixed points $u_1$, $u_2$, $u_3$ in $\overline{K_c}$ such that $\Vert u_1\Vert <d$, $a<\alpha (u_2)$, $d<\Vert u_3\Vert$ with $\alpha (u_3)<a$.

Remark 2.4

From the definition of $\phi$, we have $\phi ^{-1}(s)=\frac{s}{\sqrt{1+s^2}}$ for $s\in {\mathbb {R}}$ and $\phi ^{-1}(s)\le s$ for $s\in [0,\infty )$.

Lemma 2.5

([14]) Assume that $u\in C_0[0,1]\cap C^{1}(0,1)$ satisfies $(\phi (u'(t)))'\le 0$ in (0, 1). Then we have (i) u is concave on [0, 1]; (ii) $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u(t)\ge \frac{1}{4}\Vert u\Vert .$ Here $\Vert u\Vert$ denotes the supremum norm of u.

From now on, we always take $E=C[0,1]$ as Banach space with norm $\Vert u\Vert =\max _{t\in [0,1]}|u(t)|$ and take a cone K defined by

$$\begin{aligned} K=\left\{ u\in E \;| \ u(t) \ \mathrm{is \ nonnegative \ and \ concave \ on } \ [0,1]\right\} . \end{aligned}$$

For the case $f\in C([0,1]\times [0,\frac{1}{2}), [0,\infty ))$, let us consider the Nemytskii operator $N_f: E\rightarrow E$ defined by $N_f(u)(t)=f(t,u(t))$ for $t\in [0,1]$. Applying the similar process of establishing the solution operator in [9], we can define a nonlinear operator $T_{\lambda }$ as follows

$$\begin{aligned} T_\lambda (u)(t) = \left\{ \begin{array}{ll} \int _0^t \phi ^{-1}\left( a(\lambda h N_f(u))+ \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s, &{} t\in [0,\frac{1}{2}],\\ \int _t^1 \phi ^{-1}\left( -a(\lambda h N_f(u)) + \int _{\frac{1}{2}}^s \lambda h(\tau )f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s, &{} t\in [\frac{1}{2}, 1], \end{array} \right. \end{aligned}$$

where $a(\lambda h N_f(u))\in {\mathbb {R}}$ uniquely satisfies

$$\begin{aligned}{} & {} \int _0^{\frac{1}{2}}\phi ^{-1}\left( a(\lambda h N_f(u))+\int _{s}^{\frac{1}{2}}\lambda h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau \right) \textrm{d}s\\{} & {} \quad =\int _{\frac{1}{2}}^{1}\phi ^{-1}\left( -a(\lambda h N_f(u))+\int _{\frac{1}{2}}^{s}\lambda h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

By the definition of $T_\lambda$, we can easily show that $T_\lambda (K)\subset K$ and $T_\lambda$ is completely continuous. One can refer to Lemma 3 in [9] for details. Additionally, u is a solution of (1.1) if and only if u is a fixed point of $T_\lambda$ on K.

Remark 2.6

Once $\lambda$, h and f are fixed, we can regard $a(\lambda hN_f(u))$ as a function of u. For simplicity, we denote $a(\lambda hN_f(u))$ by $a_u$ in the following parts. In particular, by using the similar arguments about the proofs of Lemma 3.1 and Lemma 3.2 in [24], we can easily prove that $a_u:K\rightarrow {\mathbb {R}}$ is continuous and sends any bounded set in K into bounded set in ${\mathbb {R}}$.

For the case $f\in C([0,1]\times (0,\infty ), (0,\infty ))$, we need consider the following auxiliary boundary value problem

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= \lambda h(t)F(t,u(t)),\quad t\in (0,1),\\ u(0)=u(1)=A, \end{array}\right. } \end{aligned}$$

(2.1)

where $\phi (s)=\frac{s}{\sqrt{1-s^{2}}}$, $s\in (-1,1)$, $\lambda >0$, $h\in {\mathcal {H}}$, $h \not \equiv 0$ on any subinterval in (0, 1), $F\in C([0,1]\times {\mathbb {R}},[0,\infty ))$ and A is a fixed nonnegative constant.

Lemma 2.7

Assume that u is a solution of (2.1). Then u satisfies some properties as follows:

• (i) u(t) is concave and $u(t)\ge A$ on [0, 1];

• (ii) there exists a constant $t^*\in (0,1)$ satisfying $u'(t^*)=0$, $u(t^*)=\Vert u\Vert$, and $u'(t)\ge 0$ on $t\in (0,t^*]$, $u'(t)\le 0$ on $t\in (t^*,1)$;

• (iii) $u(t)\ge t(1-t)\Vert u\Vert$ on [0, 1].

Proof

The proof of this lemma can be similar to the proof of Lemma 2.3 in Wang [27]. Here we omit it. $\square$

Finally, we introduce a general existence principle for the special case $\lambda =1$ of problem (2.1)

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= h(t)F(t,u(t)),\quad t\in (0,1),\\ u(0)=u(1)=A, \end{array}\right. } \end{aligned}$$

(2.2)

which will play an important role in the proof of Theorem 1.4.

Lemma 2.8

Assume that there exists a constant $C>A$, C is independent of $\nu$, and $\Vert u\Vert =\max _{t\in [0,1]}|u(t)|\ne C$ for any solution $u\in C[0,1]\cap C^1(0,1)$ to the following problem

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= \nu h(t)F(t,u(t)),\quad t\in (0,1),\ \nu \in (0,1),\\ u(0)=u(1)=A. \end{array}\right. } \end{aligned}$$

(2.3)

Then (2.2) has at least one solution $u\in C[0,1]\cap C^1(0,1)$ and $\Vert u\Vert \le C$.

Proof

The proof of this lemma can be completed by applying the homotopy invariance of degree. One can refer to the proof of Lemma 2.3 in [21] for details. $\square$

3 Case 1: $\mathrm{f\in C([0,1]\times [0,\frac{1}{2}), [0,\infty ))}$

In this section, we present the detailed proofs of Theorems 1.1 and 1.3, and give one corresponding example.

Proof of Theorem 1.1

It is obvious that $(\lambda _{*},\lambda ^{*})$ is not empty because of condition on $\beta$. There will be three steps to complete the proof of this theorem.

Step 1: We show that $T_{\lambda }(\overline{K_c})\subset \overline{K_c}$ for some positive constant c and $\Vert T_{\lambda }(u)\Vert <d$ for $u\in \overline{K_d}$. Since $0\le F_{\frac{1}{2}}<1$, there must exist two constants $\rho , \delta$ such that $0<\rho <1$, $0<\delta <\frac{1}{2}$ and

$$\begin{aligned} f(t,u)\le \rho u,\ \textrm{for} \ (t,u)\in [0,1]\times \left( \frac{1}{2}-\delta ,\frac{1}{2}\right) . \end{aligned}$$

Then, we can obtain

$$\begin{aligned} f(t,u)\le \rho u+\eta ,\ \textrm{for} \ (t,u)\in [0,1]\times [0,\frac{1}{2}), \end{aligned}$$

(3.1)

where $\eta =\max _{(t,u)\in [0,1]\times [0, \frac{1}{2}-\delta ]} f(t,u)$. Take $c>\max \{\frac{\eta }{1-\rho },4a\}$ and let $u\in \overline{K_c}$. We can easily check that $T_\lambda (u)\in K$ and there exists at least one point $\sigma \in (0,1)$ satisfying $T_\lambda (u)(\sigma )=\max _{t\in [0,1]}T_\lambda (u)(t)$ and $T_\lambda (u)'(\sigma )=0$. If $\sigma \in (0,\frac{1}{2}]$, then we can easily derive $a_u=-\int _{\sigma }^{\frac{1}{2}}\lambda h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau$. By Remark 2.4, we have

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert= & {} \int _{0}^{\sigma }\phi ^{-1}\left( a_u+ \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\= & {} \int _{0}^{\sigma }\phi ^{-1}\left( -\int _{\sigma }^{\frac{1}{2}}\lambda h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau + \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\= & {} \int _{0}^{\sigma }\phi ^{-1}\left( \int _s^{\sigma }\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\\le & {} \int _{0}^{\frac{1}{2}}\phi ^{-1}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\\le & {} \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

(3.2)

Similarly, if $\sigma \in (\frac{1}{2},1)$, then we have

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert= & {} \int _{\sigma }^{1}\phi ^{-1}\left( -a_u+ \int _{\frac{1}{2}}^s\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\= & {} \int _{\sigma }^1\phi ^{-1}\left( -\int _{\frac{1}{2}}^\sigma \lambda h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau + \int _{\frac{1}{2}}^s\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\= & {} \int _{\sigma }^1\phi ^{-1}\left( \int _{\sigma }^s\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\\le & {} \int _{\frac{1}{2}}^1\phi ^{-1}\left( \int _{\frac{1}{2}}^s\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\nonumber \\\le & {} \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

(3.3)

Combining (3.2)(3.3) with (3.1), we get

$$\begin{aligned}&\Vert T_{\lambda }(u)\Vert \\&\quad \le \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \le \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) (\rho u(\tau )+\eta ) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s\lambda h(\tau ) (\rho u(\tau )+\eta ) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \le \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) (\rho c+\eta ) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s\lambda h(\tau ) (\rho c+\eta ) \textrm{d}\tau \right) \textrm{d}s\right\} .\\ \end{aligned}$$

From the choice of c and the range of $\lambda$, we see that $\rho c+\eta < c$ and

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert \le \lambda c \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} <c. \end{aligned}$$

Thus, we can obtain $T_{\lambda }(\overline{K_c})\subset \overline{K_c}$. Applying the similar process with the aid of condition $(C_1)$, we can show that for $u\in \overline{K_d}$

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert \le \lambda d \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} <d, \end{aligned}$$

which means that condition (ii) of Lemma 2.3 is satisfied.

Step 2: We show that condition (i) of Lemma 2.3 is also satisfied. For this, we need define

$$\begin{aligned} \alpha (u)=\min _{t\in [\frac{1}{4},\frac{3}{4}]}u(t), \ \textrm{on} \ K. \end{aligned}$$

Clearly, $\alpha$ is a nonnegative continuous concave functional. Taking $b=4a$ and $u(t)\equiv \frac{a+b}{4}=\frac{5a}{4}$ for $t\in [0,1]$, we see $a<u(t)\equiv \frac{5a}{4}<4a=b$. Hence, $\{u\in K(\alpha ,a,b):\alpha (u)>a\}\ne \emptyset$.

Next, let $u\in K(\alpha ,a,b)$, then $\alpha (u)=\min _{t\in [\frac{1}{4},\frac{3}{4}]}u(t)\ge a$ and $\Vert u\Vert \le b=4a$. From condition $(C_2)$, we get

$$\begin{aligned} f(t,u(t))\ge \beta \phi (32a),\ \textrm{for} \ t\in \left[ \frac{1}{4},\frac{3}{4}\right] . \end{aligned}$$

(3.4)

Considering two cases $a_u\ge 0$, $a_u<0$ and using (3.4), we can derive that

$$\begin{aligned}&2\Vert T_{\lambda }(u)\Vert \ge 2T_{\lambda }(u) \left( \frac{1}{2}\right) \\&\quad = \int _0^{\frac{1}{2}} \phi ^{-1}\left( a_u+ \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\\&\qquad +\int _{\frac{1}{2}}^1 \phi ^{-1}\left( -a_u + \int _{\frac{1}{2}}^s \lambda h(\tau )f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s \\&\quad \ge \min \left\{ \int _0^{\frac{1}{2}} \phi ^{-1}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^s \lambda h(\tau )f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \ge \min \left\{ \int _0^{\frac{1}{4}} \phi ^{-1}\left( \int _s^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{3}{4}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^s \lambda h(\tau )f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \ge \min \left\{ \int _0^{\frac{1}{4}} \phi ^{-1}\left( \int _\frac{1}{4}^{\frac{1}{2}}\lambda h(\tau ) f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{3}{4}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^\frac{3}{4} \lambda h(\tau )f(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \ge \min \left\{ \int _0^{\frac{1}{4}} \phi ^{-1}\left( \int _\frac{1}{4}^{\frac{1}{2}}\lambda h(\tau )\beta \phi (32a) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{3}{4}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^\frac{3}{4} \lambda h(\tau )\beta \phi (32a) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \ge \frac{1}{4} \phi ^{-1}\left( \lambda \beta \phi (32a) \min \left\{ \int _\frac{1}{4}^{\frac{1}{2}} h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^\frac{3}{4} h(\tau )\textrm{d}\tau \right\} \right) . \end{aligned}$$

i.e.

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert \ge \frac{1}{8} \phi ^{-1}\left( \lambda \beta \phi (32a) \min \left\{ \int _\frac{1}{4}^{\frac{1}{2}} h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^\frac{3}{4} h(\tau )\textrm{d}\tau \right\} \right) . \end{aligned}$$

Then, for any $\lambda \in (\lambda _{*},\lambda ^{*})$, we have

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert >\frac{1}{8}\phi ^{-1}(\phi (32a))=4a. \end{aligned}$$

Since $T_{\lambda }(u)\in K$ for $u\in K(\alpha ,a,b)$, we see

$$\begin{aligned} \alpha (T_{\lambda }(u))=\min _{t\in [\frac{1}{4},\frac{3}{4}]}T_{\lambda }(u)(t) \ge \frac{1}{4}\Vert T_{\lambda }(u)\Vert . \end{aligned}$$

Hence,

$$\begin{aligned} \alpha (T_{\lambda }(u))\ge \frac{1}{4}\Vert T_{\lambda }(u)\Vert > \frac{1}{4}\cdot 4a=a,\ \textrm{for} \ u\in K(\alpha ,a,b). \end{aligned}$$

Step 3: For all $u\in K(\alpha ,a,c)$ with $\Vert T_{\lambda }(u)\Vert >b$, we get

$$\begin{aligned} \alpha (T_{\lambda }(u))=\min _{t\in [\frac{1}{4},\frac{3}{4}]}T_{\lambda }(u)(t) \ge \frac{1}{4}\Vert T_{\lambda }(u)\Vert >\frac{b}{4}=a, \end{aligned}$$

which means that condition (iii) of Lemma 2.3 holds.

Above all, from Lemma 2.3, we see that for any $\lambda \in (\lambda _{*},\lambda ^{*})$, $T_\lambda$ must have at least three fixed points $u_1$, $u_2$, $u_3$ in $\overline{K_c}$ such that $\Vert u_1\Vert <d$, $\alpha (u_2)>a$, $\Vert u_3\Vert >d$ with $\alpha (u_3)<a$. The proof of Theorem 1.1 can be completed. $\square$

Proof of Theorem 1.3

Obviously, the interval $(\lambda _{*},\lambda ^{*})$ is not empty because of condition on $\beta _1,\beta _2$. Combining the similar arguments in the proof of Theorem 1.1 with the aids of conditions $(C_1)(C_3)$, we can check that the conditions (i) (ii) and (iii) of Lemma 2.3 all hold. Hence, there must exist positive constant c such that

$$\begin{aligned}{} & {} i(T_\lambda ,K_d,\overline{K_c})=1, \end{aligned}$$

(3.5)

$$\begin{aligned}{} & {} i(T_\lambda ,\mathring{K}(\alpha ,a,c),\overline{K_c})=1, \end{aligned}$$

(3.6)

$$\begin{aligned}{} & {} i(T_\lambda ,\overline{K_c}\backslash (\overline{K_d}\cup K(\alpha ,a,c)), \overline{K_c})=-1. \end{aligned}$$

(3.7)

Meanwhile, let $u\in K$ with $\Vert u\Vert =e$. By Lemma 2.5, for $t\in [\frac{1}{4},\frac{3}{4}]$, we have

$$\begin{aligned} e\ge u(t)\ge \frac{1}{4}\Vert u\Vert =\frac{e}{4},f(t,u(t))\ge \beta _1\phi (8e). \end{aligned}$$

(3.8)

Let $u\in \partial K_e$. Combining the arguments in the second step of the proof of Theorem 1.1 with the aid of (3.8), we can obtain that for any $\lambda \in (\lambda _{*},\lambda ^{*})$

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert\ge & {} \frac{1}{8} \phi ^{-1}\left( \lambda \beta _1\phi (8e) \min \left\{ \int _\frac{1}{4}^{\frac{1}{2}} h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^\frac{3}{4} h(\tau )\textrm{d}\tau \right\} \right) \\\\ge & {} \frac{1}{8} \phi ^{-1}\left( \lambda \min \{\beta _1,\beta _2\}\phi (8e) \min \left\{ \int _\frac{1}{4}^{\frac{1}{2}} h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^\frac{3}{4} h(\tau )\textrm{d}\tau \right\} \right) \\\> & {} \frac{1}{8} \phi ^{-1}(\phi (8e))=e. \end{aligned}$$

i.e.

$$\begin{aligned} \Vert T_{\lambda }(u)\Vert >\Vert u\Vert ,\ \textrm{for} \ u\in \partial K_{e}. \end{aligned}$$

(3.9)

By Lemma 2.1 and (3.9), we have

$$\begin{aligned} i(T_\lambda ,K_e,\overline{K_c})=0. \end{aligned}$$

(3.10)

From (3.5)(3.10) and the additivity of the fixed point index, we deduce

$$\begin{aligned} i(T_\lambda ,K_d\backslash \overline{K_e},\overline{K_c})=1. \end{aligned}$$

(3.11)

Hence, from (3.6)(3.7) and (3.11), we get that for any $\lambda \in (\lambda _{*},\lambda ^{*})$, $T_\lambda$ must have at least three fixed points $u_1$, $u_2$, $u_3$ in $\overline{K_c}$ such that $e<\Vert u_1\Vert <d$, $a<\alpha (u_2)$, $d<\Vert u_3\Vert$ with $\alpha (u_3)<a$. The proof of Theorem 1.3 is done. $\square$

Example 1

Consider a Minkowski curvature problem of the form

$$\begin{aligned} \left\{ \begin{array}{ll} -\phi (u')'=\lambda t^{-\frac{3}{2}}f(u),\quad t\in (0,1),\\ u(0)=u(1)=0, \end{array} \right. \end{aligned}$$

(3.12)

where

$$\begin{aligned} f(u) = \left\{ \begin{array}{ll} u, \quad 0\le u< \frac{1}{100},\\ ~\\ \frac{239800}{3}u^{2}-\frac{2395}{3}u,\quad \frac{1}{100}\le u<\frac{1}{40},\\ ~\\ 1200 u,\quad \frac{1}{40}\le u<\frac{1}{10},\\ ~\\ \frac{17995}{6}u(\frac{1}{2}-u)+\frac{1}{3}u,\quad \frac{1}{10}\le u<\frac{1}{2}. \end{array} \right. \end{aligned}$$

(3.13)

It is easy to check that $h(t)=t^{-\frac{3}{2}}\in {\mathcal {H}}$, $h \not \equiv 0$ on any subinterval in (0, 1) and $f\in C([0,\frac{1}{2}), [0,\infty ))$.

$$\begin{aligned} F_0= & {} \limsup _{u\rightarrow 0^+}\frac{u}{u}=1,\\ F_{\frac{1}{2}}= & {} \limsup _{u\rightarrow \frac{1}{2}^{-}}\frac{\frac{17995}{6}u(\frac{1}{2}-u)+\frac{1}{3}u}{u}=\frac{1}{3}<1. \end{aligned}$$

Here, we can take $d=\frac{1}{100}$ such that

$$\begin{aligned} f(u)=u\le \frac{1}{100},\ \textrm{for} \ u\in [0,\frac{1}{100}]. \end{aligned}$$

Condition $(C_1)$ of Theorem 1.1 is satisfied. Meanwhile, we can take $a=\frac{1}{40}$ and $\beta =20$ satisfying $f(u)=1200u\ge 30>\frac{80}{3}= \beta \phi (32a)$ for all $\frac{1}{40}\le u\le \frac{1}{10}$ and

$$\begin{aligned} \frac{1}{\beta \min \left\{ \int _{\frac{1}{4}}^{\frac{1}{2}}h(\tau )\textrm{d}\tau ,\int _{\frac{1}{2}}^{\frac{3}{4}}h(\tau )\textrm{d}\tau \right\} } <\frac{1}{\max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} }. \end{aligned}$$

Condition $(C_2)$ of Theorem 1.1 is also satisfied. Here, we have

$$\begin{aligned} \lambda _{*}= & {} \frac{1}{\beta \min \left\{ \int _{\frac{1}{4}}^{\frac{1}{2}}h(\tau )\textrm{d}\tau , \int _{\frac{1}{2}}^{\frac{3}{4}}h(\tau )\textrm{d}\tau \right\} }\doteq 0.096,\\ \lambda ^{*}= & {} \frac{1}{\max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} }\doteq 0.707. \end{aligned}$$

From Theorem 1.1, for any $\lambda \in (0.096,0.707)$, (3.12) must have at least one nonnegative solution $u_1$ and two positive solutions $u_2,u_3$ satisfying $\Vert u_1\Vert <\frac{1}{100}$, $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_2(t)>\frac{1}{40}$, $\Vert u_3\Vert >\frac{1}{100}$ and $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_3(t)<\frac{1}{40}$.

Moreover, replacing $f(u)=u$ for $0\le u<\frac{1}{100}$ in (3.13) with

$$\begin{aligned} f(u) = \left\{ \begin{array}{ll} 7.996\times 10^{11} u^2+u, \quad 0\le u< \frac{1}{4}\times 10^{-8},\\ ~\\ \frac{1}{10}u^{\frac{1}{2}},\quad \frac{1}{4}\times 10^{-8}\le u<\frac{1}{100}, \end{array} \right. \end{aligned}$$

we can take $e= 10^{-8}$, $d=\frac{1}{100}$, $a=\frac{1}{40}$, $\beta _1=30$ and $\beta _2=20$. Then conditions of Theorem 1.3 are all satisfied. Thus, for any $\lambda \in (0.096,0.707)$, (3.12) must have at least three positive solutions $u_1,u_2,u_3$ satisfying $10^{-8}<\Vert u_1\Vert <\frac{1}{100}$, $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_2(t)>\frac{1}{40}$, $\Vert u_3\Vert >\frac{1}{100}$ and $\min _{t\in [\frac{1}{4},\frac{3}{4}]}u_3(t)<\frac{1}{40}$.

4 Case 2: ${f\in C([0,1]\times (0,\infty ), (0,\infty ))}$

In this section, let us firstly consider a special case $\lambda =1$ of problem (1.1)

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= h(t)f(t,u(t)),\quad t\in (0,1),\\ u(0)=u(1)=0, \end{array}\right. } \end{aligned}$$

(4.1)

and establish an auxiliary existence result of positive solution for (4.1). Then Theorem 1.4 can be easily deduced as a consequence of the auxiliary result. As an application, one corresponding example will also be presented.

Theorem 4.1

Assume that $f\in C([0,1]\times (0,\infty ), (0,\infty ))$ and satisfies

$(C_4)$ $f(t,u)\le f_1(u)+f_2(u)$ for all $(t,u)\in [0,1]\times (0,\infty )$, where $f_1:(0,\infty )\rightarrow (0,\infty )$ is continuous and nonincreasing, $f_2:[0,\infty )\rightarrow [0,\infty )$ is continuous, and $\frac{f_2}{f_1}$ is nondecreasing on $(0,\infty )$;

$(C_5)$ for each constant $\iota >0$, there exists a function $\psi _\iota \in C([0,1],[0,\infty ))$ satisfying $\psi _\iota (t)>0$ for $t\in (0,1)$ and $f(t,u)\ge \psi _\iota (t)$ for $(t,u)\in [0,1]\times (0,\iota ]$;

$(C_7)$ there exists a constant $r>0$ such that

$$\begin{aligned} \frac{\int _0^{r}\frac{\textrm{d}y}{f_1(y)}}{1+\frac{f_2(r)}{f_1(r)}}>\max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} . \end{aligned}$$

Then problem (4.1) has at least one positive solution u with $0<\Vert u\Vert <r$.

Proof

From $(C_7)$, we can choose $\epsilon \in (0,r)$ satisfying

$$\begin{aligned} \frac{\int _{\epsilon }^{r}\frac{\textrm{d}y}{f_1(y)}}{1+\frac{f_2(r)}{f_1(r)}}>\max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} . \end{aligned}$$

(4.2)

Let $n_0\in \{1,2,\cdots \}$ be chosen so that $\frac{1}{n_0}<\epsilon$ and let $N_0=\{n_0,n_0+1,\cdots \}$. In the following parts, we will divide the proof of this theorem into three steps.

Step 1: Show that the following boundary value problem

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= h(t)f(t,u(t)),\quad t\in (0,1),\\ u(0)=u(1)=\frac{1}{n},\quad n\in N_0, \end{array}\right. } \end{aligned}$$

(4.3)

has at least one positive solution $u_n$ for each $n\in N_0$, and $\frac{1}{n}\le u_n(t)<r$ for $t\in [0,1]$. For this, let us consider the modified problem of the form

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= h(t)f^*(t,u(t)),\quad t\in (0,1),\\ u(0)=u(1)=\frac{1}{n},\quad n\in N_0, \end{array}\right. } \end{aligned}$$

(4.4)

where

$$\begin{aligned} f^*(t,u) = \left\{ \begin{array}{ll} f(t,u), &{}\quad u\ge \frac{1}{n},\\ f(t,\frac{1}{n}), &{}\quad u\le \frac{1}{n}, \end{array} \right. \end{aligned}$$

and apply Lemma 2.8 to prove the existence of positive solution of (4.4) for each $n\in N_0$. Thus, we need consider the family of problems

$$\begin{aligned}&{\left\{ \begin{array}{ll} -\left( \phi (u'(t))\right) '= \nu h(t)f^*(t,u(t)),\quad t\in (0,1),\ \ \nu \in (0,1),\\ u(0)=u(1)=\frac{1}{n},\quad n\in N_0. \end{array}\right. } \end{aligned}$$

(4.5)

Let u be a solution of (4.5). By Lemma 2.7, we see that $u''(t)\le 0$ on (0, 1), $u(t)\ge \frac{1}{n}$ for $t\in [0,1]$, there exists one point $\sigma _n\in (0,1)$ such that $u'(\sigma _n)=0$, $\Vert u\Vert =u(\sigma _n)$ and $u'(t)\ge 0$ on $(0,\sigma _n]$, $u'(t)\le 0$ on $(\sigma _n,1)$.

If $\sigma _n\in (0,\frac{1}{2}]$, then we integrate on both sides of the first equation in (4.5) on $[s,\sigma _n]$ for $s\in (0,\sigma _n)$. And from $(C_4)$, we get

$$\begin{aligned} \phi (u'(s))= & {} \int _s^{\sigma _n}\nu h(\tau )f^*(\tau ,u(\tau ))\textrm{d}\tau \\= & {} \int _s^{\sigma _n}\nu h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau \\\le & {} \int _s^{\sigma _n} h(\tau )\left[ f_1(u(\tau ))+f_2(u(\tau ))\right] \textrm{d}\tau \\= & {} \int _s^{\sigma _n} h(\tau )f_1(u(\tau ))\left[ 1+\frac{f_2(u(\tau ))}{f_1(u(\tau ))}\right] \textrm{d}\tau \\\le & {} f_1(u(s))\left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _s^{\sigma _n} h(\tau )\textrm{d}\tau . \end{aligned}$$

Taking $\phi ^{-1}$ on both sides of the above inequality and applying Remark 2.4, we have

$$\begin{aligned} u'(s)\le & {} \phi ^{-1}\left( f_1(u(s))\left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _s^{\sigma _n} h(\tau )\textrm{d}\tau \right) \\\le & {} f_1(u(s))\left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _s^{\sigma _n} h(\tau )\textrm{d}\tau . \end{aligned}$$

i.e.

$$\begin{aligned} \frac{u'(s)}{f_1(u(s))}\le \left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _s^{\sigma _n} h(\tau )\textrm{d}\tau . \end{aligned}$$

(4.6)

Integrating on both sides of the above inequality from 0 to $\sigma _n$, we obtain

$$\begin{aligned} \int _{\frac{1}{n}}^{u(\sigma _n)}\frac{\textrm{d}y}{f_1(y)}\le & {} \left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _0^{\sigma _n}\left( \int _s^{\sigma _n} h(\tau )\textrm{d}\tau \right) \textrm{d}s\\\le & {} \left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _0^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau )\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

It follows from the choice of n that

$$\begin{aligned} \int _{\epsilon }^{u(\sigma _n)}\frac{\textrm{d}y}{f_1(y)}\le \left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _0^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau )\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

(4.7)

Similarly, if $\sigma _n\in (\frac{1}{2},1)$, we can derive

$$\begin{aligned} \int _{\epsilon }^{u(\sigma _n)}\frac{\textrm{d}y}{f_1(y)}\le \left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau )\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

(4.8)

Hence, from (4.7) and (4.8), we have

$$\begin{aligned}&\int _{\epsilon }^{u(\sigma _n)}\frac{\textrm{d}y}{f_1(y)}\nonumber \\&\quad \le \left[ 1+\frac{f_2(u(\sigma _n))}{f_1(u(\sigma _n))}\right] \max \left\{ \int _0^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau )\textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau )\textrm{d}\tau \right) \textrm{d}s\right\} .\nonumber \\ \nonumber \\ \end{aligned}$$

(4.9)

Combining (4.2) with (4.9), we see that $\Vert u\Vert =u(\sigma _n)\ne r$. By Lemma 2.8, we derive that (4.4) has at least one positive solution $u_n$ such that $\frac{1}{n}\le u_n(t)< r$ for $t\in [0,1]$. It means that (4.3) has at least one positive solution $u_n$ such that

$$\begin{aligned} \frac{1}{n}\le u_n(t)< r,\quad \textrm{for} \ t\in [0,1]. \end{aligned}$$

(4.10)

Step 2: Show that there exists a constant $k>0$ such that

$$\begin{aligned} u_n(t)\ge t(1-t)k,\quad \textrm{for} \ t\in [0,1], \forall \ n\in N_0. \end{aligned}$$

(4.11)

In fact, by Lemma 2.7, we see that $u_n\in K$ and $u_n(t)\ge t(1-t)\Vert u_n\Vert$ for $t\in [0,1]$ and each $n\in N_0$. Fix $n\in N_0$, let us define

$$\begin{aligned} T_1(u)(t) = \left\{ \begin{array}{ll} \frac{1}{n}\!+\!\int _0^t \phi ^{-1}\!\left( a(h N_{f^{*}}(u))\!+\! \int _s^{\frac{1}{2}} h(\tau ) f^*(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s, &{}\, t\in [0,\frac{1}{2}],\\ \frac{1}{n}\!+\!\int _t^1 \phi ^{-1}\!\left( -a(h N_{f^{*}}(u))\!+\! \int _{\frac{1}{2}}^s h(\tau )f^*(\tau ,u(\tau )) \textrm{d}\tau \right) \textrm{d}s, &{}\, t\in [\frac{1}{2}, 1], \end{array} \right. \end{aligned}$$

where $a(h N_{f^{*}}(u))\in {\mathbb {R}}$ uniquely satisfies

$$\begin{aligned}{} & {} \int _0^{\frac{1}{2}}\phi ^{-1}\left( a(h N_{f^{*}}(u))+\int _{s}^{\frac{1}{2}} h(\tau )f^*(\tau ,u(\tau ))\textrm{d}\tau \right) \textrm{d}s\\{} & {} \quad =\int _{\frac{1}{2}}^{1}\phi ^{-1}\left( -a(h N_{f^{*}}(u))+\int _{\frac{1}{2}}^{s} h(\tau )f^*(\tau ,u(\tau ))\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

Applying the similar analysis about the solution operator of problem (1.1), we can easily check that $T_1:K\rightarrow K$ is completely continuous, and $u_n$ is a solution of problem (4.4) can be equivalently rewritten as $u_n=T_1(u_n)$ on K. By using Lemma 2.7, condition $(C_5)$ and the arguments in the second step of the proof of Theorem 1.1, we can deduce

$$\begin{aligned}&2\Vert u_n\Vert =2\Vert T_1(u_n)\Vert \ge 2T_1(u_n)\left( \frac{1}{2}\right) \\&\quad = \frac{1}{n}+\int _0^{\frac{1}{2}} \phi ^{-1}\left( a(h N_{f^{*}}(u_n))+ \int _s^{\frac{1}{2}} h(\tau ) f^*(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s\\&\qquad +\frac{1}{n}+\int _{\frac{1}{2}}^1 \phi ^{-1}\left( -a(h N_{f^{*}}(u_n)) + \int _{\frac{1}{2}}^s h(\tau )f^*(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s \\&\quad \ge \min \left\{ \int _0^{\frac{1}{2}} \phi ^{-1}\left( \int _s^{\frac{1}{2}} h(\tau ) f^*(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^s h(\tau )f^*(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad = \min \left\{ \int _0^{\frac{1}{2}} \phi ^{-1}\left( \int _s^{\frac{1}{2}} h(\tau ) f(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{1}{2}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^s h(\tau )f(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \ge \min \left\{ \int _0^{\frac{1}{4}} \phi ^{-1}\left( \int _\frac{1}{4}^{\frac{1}{2}} h(\tau ) f(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s,\int _{\frac{3}{4}}^1 \phi ^{-1}\left( \int _{\frac{1}{2}}^\frac{3}{4} h(\tau )f(\tau ,u_n(\tau )) \textrm{d}\tau \right) \textrm{d}s\right\} \\&\quad \ge \frac{1}{4}\min \left\{ \phi ^{-1}\left( \int _\frac{1}{4}^{\frac{1}{2}} h(\tau )\psi _r(\tau ) \textrm{d}\tau \right) , \phi ^{-1}\left( \int _{\frac{1}{2}}^\frac{3}{4} h(\tau )\psi _r(\tau ) \textrm{d}\tau \right) \right\} . \end{aligned}$$

i.e.

$$\begin{aligned} u_n(t)\ge t(1-t)\Vert u_n\Vert \ge t(1-t)k,\quad \textrm{for} \ t\in [0,1],\quad \forall \ n\in N_0, \end{aligned}$$

where

$$\begin{aligned} k=\frac{1}{8}\min \left\{ \phi ^{-1}\left( \int _\frac{1}{4}^{\frac{1}{2}} h(\tau )\psi _r(\tau ) \textrm{d}\tau \right) , \phi ^{-1}\left( \int _{\frac{1}{2}}^\frac{3}{4} h(\tau )\psi _r(\tau ) \textrm{d}\tau \right) \right\} . \end{aligned}$$

Step 3: Show that $\{u_n\}_{n\in N_0}$ is uniformly bounded and equicontinuous on [0, 1]. It follows from (4.10) that $\{u_n\}_{n\in N_0}$ is uniformly bounded clearly. Then we only need to show its equicontinuity. Exactly, we firstly prove that there exist two constants $c_1,c_2$ such that

$$\begin{aligned} 0<c_1<\inf \{\sigma _n:n\in N_0\}\le \sup \{\sigma _n:n\in N_0\}<c_2<1. \end{aligned}$$

For this, combining the similar deduction process of (4.6) with (4.10), we can easily get

$$\begin{aligned} \frac{u_n'(s)}{f_1(u_n(s))}\le \left[ 1+\frac{f_2(r)}{f_1(r)}\right] \int _s^{\sigma _n} h(\tau )\textrm{d}\tau , \end{aligned}$$

(4.12)

and

$$\begin{aligned} -\frac{u_n'(s)}{f_1(u_n(s))}\le \left[ 1+\frac{f_2(r)}{f_1(r)}\right] \int _{\sigma _n}^s h(\tau )\textrm{d}\tau . \end{aligned}$$

(4.13)

We can apply the contradiction method to prove $\inf \{\sigma _n:n\in N_0\}>c_1>0$. Suppose it is not true, then there must exist a subsequence $N^*$ of $N_0$ satisfying $\sigma _n \rightarrow 0$ as $n\rightarrow \infty$. Integrating on both sides of (4.12) from 0 to $\sigma _n$, we have

$$\begin{aligned} \int _0^{u_n(\sigma _n)}\frac{\textrm{d}y}{f_1(y)}\le \left[ 1+\frac{f_2(r)}{f_1(r)}\right] \int _0^{\sigma _n} \tau h(\tau )\textrm{d}\tau + \int _0^{\frac{1}{n}}\frac{\textrm{d}y}{f_1(y)}. \end{aligned}$$

Since $\frac{1}{n}\rightarrow 0$ and $\sigma _n \rightarrow 0$ as $n\rightarrow \infty$ in $N^*$, we get $u_n(\sigma _n)\rightarrow 0$ as $n\rightarrow \infty$ in $N^*$. That is to say, $u_n\rightarrow 0$ in C[0, 1] as $n\rightarrow \infty$ in $N^*$, which contradicts with (4.11). Similarly, we can also show that $\sup \{\sigma _n:n\in N_0\}<c_2<1$. Thus, from (4.12) and (4.13), we have

$$\begin{aligned} \frac{|u_n'(s)|}{f_1(u_n(s))}\le \left[ 1+\frac{f_2(r)}{f_1(r)}\right] \int _{\min \{s,c_1\}}^{\max \{s,c_2\}} h(\tau )\textrm{d}\tau ,\quad \textrm{for} \ s\in (0,1). \end{aligned}$$

(4.14)

It follows from $h\in {\mathcal {H}}$ that $\int _{\min \{s,c_1\}}^{\max \{s,c_2\}} h(\tau )\textrm{d}\tau \in L^1[0,1]$. Let us define a function $J:[0,\infty )\rightarrow [0,\infty )$ given by

$$\begin{aligned} J(x)=\int _0^x\frac{\textrm{d}y}{f_1(y)}. \end{aligned}$$

(4.15)

It is obvious to see that J is continuous and increasing on $[0,\infty )$. From (4.14) and (4.15), we can also easily check that $\{J(u_n)\}_{n\in N_0}$ is uniformly bounded and equicontinuous on [0, 1]. Then, the equicontinuity of $\{u_n\}_{n\in N_0}$ can be guaranteed by the fact that $J^{-1}$ is uniformly continuous on [0, J(r)] and

$$\begin{aligned} |u_n(t_1)-u_n(t_2)|=|J^{-1}(J(u_n(t_1)))-J^{-1}(J(u_n(t_2)))|,\quad \textrm{for} \ t_1,t_2\in [0,1]. \end{aligned}$$

Finally, from the Arzela–Ascoli theorem, there must exist a subsequence $N_*$ of $N_0$ and a continuous function u such that $u_n$ converging uniformly to u on [0, 1] as $n\rightarrow \infty$ in $N_*,$ $u(0)=u(1)=0$, and $u(t)\ge t(1-t)k$ for $t\in [0,1]$. Specially, $u(t)>0$ for $t\in (0,1)$. Since $u_n$ is the positive solution of (4.3) for each $n\in N_*$, then for $t\in (0,1)$, we can easily deduce that $u_n$ satisfies

$$\begin{aligned} u_n(t)=u_n \left( \frac{1}{2}\right) +\int _{\frac{1}{2}}^{t}\phi ^{-1}\left( \phi \left( u'_n\left( \frac{1}{2}\right) \right) - \int _{\frac{1}{2}}^{s}h(\tau )f(\tau ,u_n(\tau ))\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

By (4.10) and (4.11), we see that the sequence $\{u'_n(\frac{1}{2})\}_{n\in N_*}$ is bounded. Hence $\{u'_n(\frac{1}{2})\}_{n\in N_*}$ must have a convergent subsequence which converges to $\zeta \in {\mathbb {R}}$. For simplicity, we also denote this subsequence as $\{u'_n(\frac{1}{2})\}_{n\in N_*}$. For the fixed $t\in (0,1)$, we see that f is uniformly continuous on any compact subset of $[\min \{t,\frac{1}{2}\}, \max \{t,\frac{1}{2}\}]\times (0,r]$. Taking $n\rightarrow \infty$ in $N_*$, we have

$$\begin{aligned} u(t)=u\left( \frac{1}{2}\right) +\int _{\frac{1}{2}}^{t}\phi ^{-1}\left( \phi (\zeta )- \int _{\frac{1}{2}}^{s}h(\tau )f(\tau ,u(\tau ))\textrm{d}\tau \right) \textrm{d}s. \end{aligned}$$

Let us apply this argument for each $t\in (0,1)$. Thus, we get $-\left( \phi (u'(t))\right) '= h(t)f(t,u(t))$ for $t\in (0,1)$. i.e. u is a positive solution of (4.1). Moreover, from the similar arguments of the first step, we can easily see that $\Vert u\Vert <r$. $\square$

Proof of Theorem 1.4

By the choice of $\lambda$ and $(C_4)(C_5)(C_6)$, we see that conditions of Theorem 4.1 all hold. Thus, from Theorem 4.1, we can easily deduce that (1.1) has at least one positive solution u for $\lambda \in (0,{\bar{\lambda }})$ and $0<\Vert u\Vert <r$. $\square$

Example 2

Consider a Minkowski curvature problem of the form

$$\begin{aligned} \left\{ \begin{array}{ll} -\phi (u')'=\lambda t^{-\frac{3}{2}}(u^{-\frac{1}{2}}+u^{3}),\quad t\in (0,1),\\ u(0)=u(1)=0. \end{array} \right. \end{aligned}$$

(4.16)

Obviously, we see $h(t)=t^{-\frac{3}{2}}\in {\mathcal {H}}$, $h \not \equiv 0$ on any subinterval in (0, 1), $f\in C((0,\infty ), (0,\infty ))$. Take $f_1(u)=u^{-\frac{1}{2}}$, $f_2(u)=u^{3}$, $\psi _\iota (t)=f_1(\iota )$ for $t\in [0,1]$. It is easy to check that conditions $(C_4)(C_5)$ of Theorem 1.4 are both valid. Meanwhile, by choosing $r=1$ and applying some simple calculations, we have

$$\begin{aligned} {\bar{\lambda }}= \frac{\int _0^{1}\frac{\textrm{d}y}{f_1(y)}}{\left[ 1+\frac{f_2(1)}{f_1(1)}\right] \max \left\{ \int _{0}^{\frac{1}{2}}\left( \int _s^{\frac{1}{2}} h(\tau ) \textrm{d}\tau \right) \textrm{d}s, \int _{\frac{1}{2}}^1\left( \int _{\frac{1}{2}}^s h(\tau ) \textrm{d}\tau \right) \textrm{d}s\right\} }\doteq 0.235. \end{aligned}$$

By Theorem 1.4, we deduce that for any $\lambda \in (0,0.235)$, (4.16) must have at least one positive solution u satisfying $0<\Vert u\Vert <1$.