The method developed based on mathematical simulation for determining the relaxation parameters of polymer textile materials allows carrying out a qualitative assessment of their relaxation and recovery properties [1].

To assess the relaxation and recovery properties of polymer textile materials, it is proposed to use several developed criteria that can be combined into one integrated criterion due to the fact that all relaxation parameters of these materials are subject to the probabilistic Cauchy law, which has the property of additivity, and include the assessment of their qualities [2].

To assess the relaxation and recovery properties of polymer textile materials, the following criteria are proposed for consideration [3]:

  • criterion of the material recovery intensity after operation;

  • criterion of the material recoverability degree after operation;

  • criterion of the possibility of material multiple recovery during operation;

  • time criterion of the recovery of the functional and consumer properties of the material after operation;

  • criterion of material resistance to multiple recovery after operation.

Quantitative assessment of the parameters within the proposed criteria of relaxation and recovery properties of polymer textile materials is proposed to be carried out in dimensionless units, which can be described as follows [4].

1. Criterion of the material recovery intensity after operation [5]. Let us denote by

$${\mathrm{\alpha }}_{1}={b}_{n\upvarepsilon }$$
(1)

a dimensionless variable that characterizes the magnitude of the material recovery intensity after operation, which is numerically equal to the intensity of the relaxation process responsible for the recovery of the functional and consumer properties of the considered material. At that, α1 can take any non-negative values (α1 ≥ 0). The smaller the α1 value, the faster the process of material recovery after operation.

2. Criterion of the material recoverability degree after operation [6]. Let us denote by

$${a}_{2}={E}_{\infty }/({E}_{0}+{E}_{\infty })$$
(2)

a dimensionless variable that characterizes the material recoverability degree after operation. At that, α2 takes non- negative values (α2 ≥ 0). The smaller the α2 value, the higher the material recoverability degree after operation. The higher the value of the parameter α2, the less the material has the ability to recover.

3. Criterion of the possibility of material multiple recovery during operation. Let us denote by

$${\mathrm{\alpha }}_{3}=100\%/{\upvarepsilon }_{\mathrm{p}}-1$$
(3)

a dimensionless variable that characterizes the possibility of material multiple recovery during operation, where εp is the value of the rupturing deformation in percent. At that, α3 can take any non-negative values (α3 ≥ 0). The smaller the α3 value, the higher the possibility of material multiple recovery. The value α3 = 0 corresponds to a material with the property of full multiple recovery.

4. Time criterion for the recovery of the functional and consumer properties of the material after operation [8]. Let us denote by

$${\mathrm{\alpha }}_{3}={\overline{\uptau } }_{\upvarepsilon }/{t}_{1}$$
(4)

a dimensionless variable that characterizes the time ability of the material to recover its functional and consumer properties after operation, where t1 is some value of the base time, for example t1 = 60 s; \({\overline{\uptau } }_{\upvarepsilon }\) is the average relaxation time, determined by the formula

$${\overline{\uptau } }_{\upvarepsilon }=\frac{1}{{\upvarepsilon }_{2}-{\upvarepsilon }_{1}}\cdot \underset{{\upvarepsilon }_{1}}{\overset{{\upvarepsilon }_{2}}{\int }}{\uptau }_{\upvarepsilon }\cdot d\upvarepsilon $$
(5)

where ε1, ε2 are the smallest and largest values from the interval of the studied deformations.

At that, α4 can take any non-negative values (α4 ≥ 0). The smaller the α4 value, the faster the material recovers after operation.

5. Criterion of material resistance to multiple recovery after operation. Let us denote by

$${\mathrm{\alpha }}_{5}={E}_{\infty }/\left({E}_{0}-{E}_{\infty }\right)$$
(6)

a dimensionless variable that characterizes the material resistance to multiple recovery after operation. At that, α5 can take any non-negative values (α5 ≥ 0). The smaller the α5 value, the greater the material resistance to multiple recovery after operation. The value α5 = 0 corresponds to the condition of complete material resistance to multiple recovery after operation.

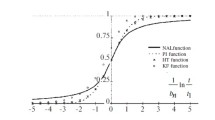
Since the main relaxation characteristics E0, E∞, bnε and τε were determined using mathematical simulation of relaxation processes of polymer textile materials based on the Cauchy probability distribution, the integral distribution function of which is the NAL function, as noted above, all variables α1, α2, α3, α4, α5 also obey the Cauchy probability distribution [7].

The Cauchy probability distribution is quite close to the normal distribution, differing from it in some properties, for example, the slower convergence of the integral function to its asymptotic values. This property of the Cauchy distribution allows more reliable processing of statistical samples with a significant dispersion degree, which is typical for the sample sets of relaxation and recovery characteristics of polymer textile materials.

In view of the above, it should be noted that the probabilistic relaxation characteristic εp is also subject to the Cauchy law.

As integral functions of probability distributions in the study of relaxation, deformation and other properties of textile and light industry materials, in addition to the normal distribution with the “probability integral” function and the Cauchy distribution with the NAL function, the “hyperbolic tangent” function and the “Kohlrausch function”, the graphs of which are geometrically similar and fairly close to each other, are sometimes used [8].

6. Integrated criterion of relaxation and recovery properties. Since all the introduced probabilistic characteristics α1, α2, α3, α4, α5 are distributed according to the Cauchy law, it is advisable to consider a new relaxation-recovery parameter

$${A}_{\mathrm{p}}={\mathrm{\alpha }}_{1}+{\mathrm{\alpha }}_{2}+{\mathrm{\alpha }}_{3}+{\mathrm{\alpha }}_{4}+{\mathrm{\alpha }}_{5},$$
(7)

also distributed according to the Cauchy law.

Based on the properties of the α1, α2, α3, α4, α5 terms, the parameter Ap can take any non-negative values (Ap ≥ 0), and the relaxation and recovery properties of polymer textile materials will be the better, the smaller the value of the relaxation-recovery parameter Ap.

It is clear that in the ideal case, the polymer textile materials with Ap = 0 will have the functional relaxation and recovery characteristics the best in terms of the materials quality. However, it cannot be achieved in practice [9].

Thus, the criterion for optimizing the relaxation and recovery properties of polymer textile materials can be formulated as follows:

$$\frac{\sum_{k=1}^{N}{A}_{pk}}{N}=\frac{\sum_{k=1}^{N}\left({\mathrm{\alpha }}_{1k}+{\mathrm{\alpha }}_{2k}+{\mathrm{\alpha }}_{3k}+{\mathrm{\alpha }}_{4k}+{\mathrm{\alpha }}_{5k}\right)}{N}={\overline{A} }_{\mathrm{p}}\to \mathrm{min},$$
(8)

where N is the number of studied material samples (sample size), \({\overline{\mathrm{A}} }_{\mathrm{p}}\) is the average value of the relaxation-recovery parameter.

As already noted, the developed criterion for optimizing the relaxation and recovery properties of polymer textile materials is integrated and includes optimization of the relaxation and recovery properties of these materials according to five partial criteria [10].

The method for conducting a qualitative assessment of the relaxation and recovery properties of polymer textile materials by the parameters of the mathematical model of the relaxation of these materials has been considered.

The study was financed within the framework of the state task of the Ministry of Science and Higher Education of the Russian Federation, Project No. FSEZ-2023-0003 and within the framework of the grant of the President of the Russian Federation for state support of leading scientific schools of the Russian Federation No. NSh-5349.2022. 4.